Interaction among Multiple Electric Vehicle Chargers: Measurements on Harmonics and Power Quality Issues
Abstract
:1. Introduction
2. Overview of the Effects of Aggregating Several EV Charging Points
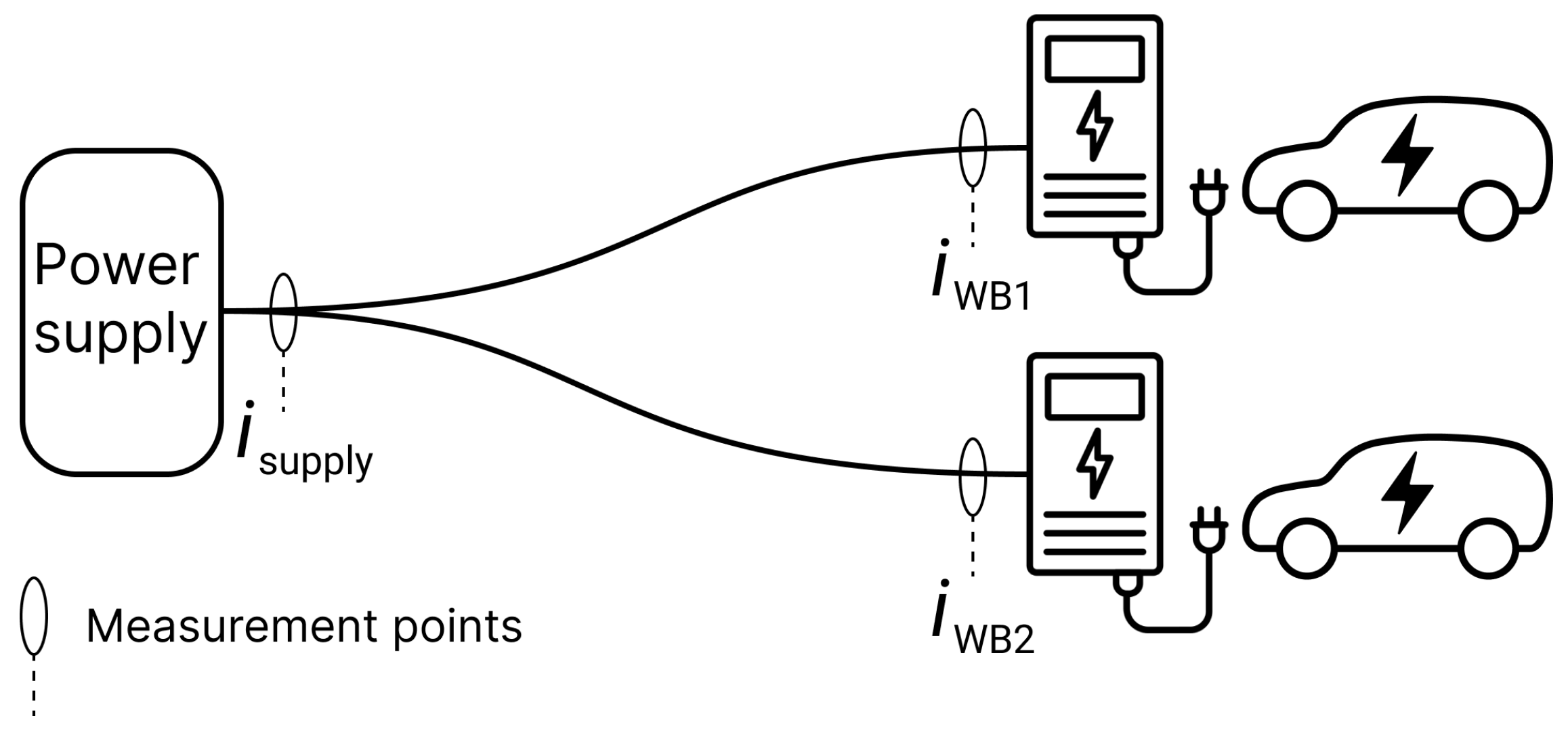
3. Laboratory Set-Up
- Power amplifier: A three-phase linear power amplifier with a nominal power of 7 A per phase has been used. This technology has been chosen because of its performance. In fact, the linear amplifier has a maximum distortion on the generated voltages equal to at the maximum output power [25]. Moreover, the introduced short time delay enables the use of a simple interface topology and guarantees high stability.
- Chargers and electric vehicles: The cars used are two Nissan Leaf, with battery capacities of 62 h and 40 h, respectively, and equipped with the DC CHAdeMO plug. The model of charger (which cannot be disclosed for confidentiality reasons) is a bidirectional WB, with rated power 11 in G2V and 10 in V2G operation.
- Data acquisition: The HBM GEN7tA is a transient recorder and data acquisition system. It has been used to visualize, monitor, record, and post-process the electrical quantities involved in the tests. Three identical Hioki 9018-50 clamp probes have been employed for capturing the currents during the tests. The clamps have a range from 10 to 500 AC, for a total of six ranges, with the amplitude accuracy equal to rdg f.s. (45 to ) and the phase accuracy equal to for frequencies from 40 to 3 .
4. Test Description
4.1. Procedure
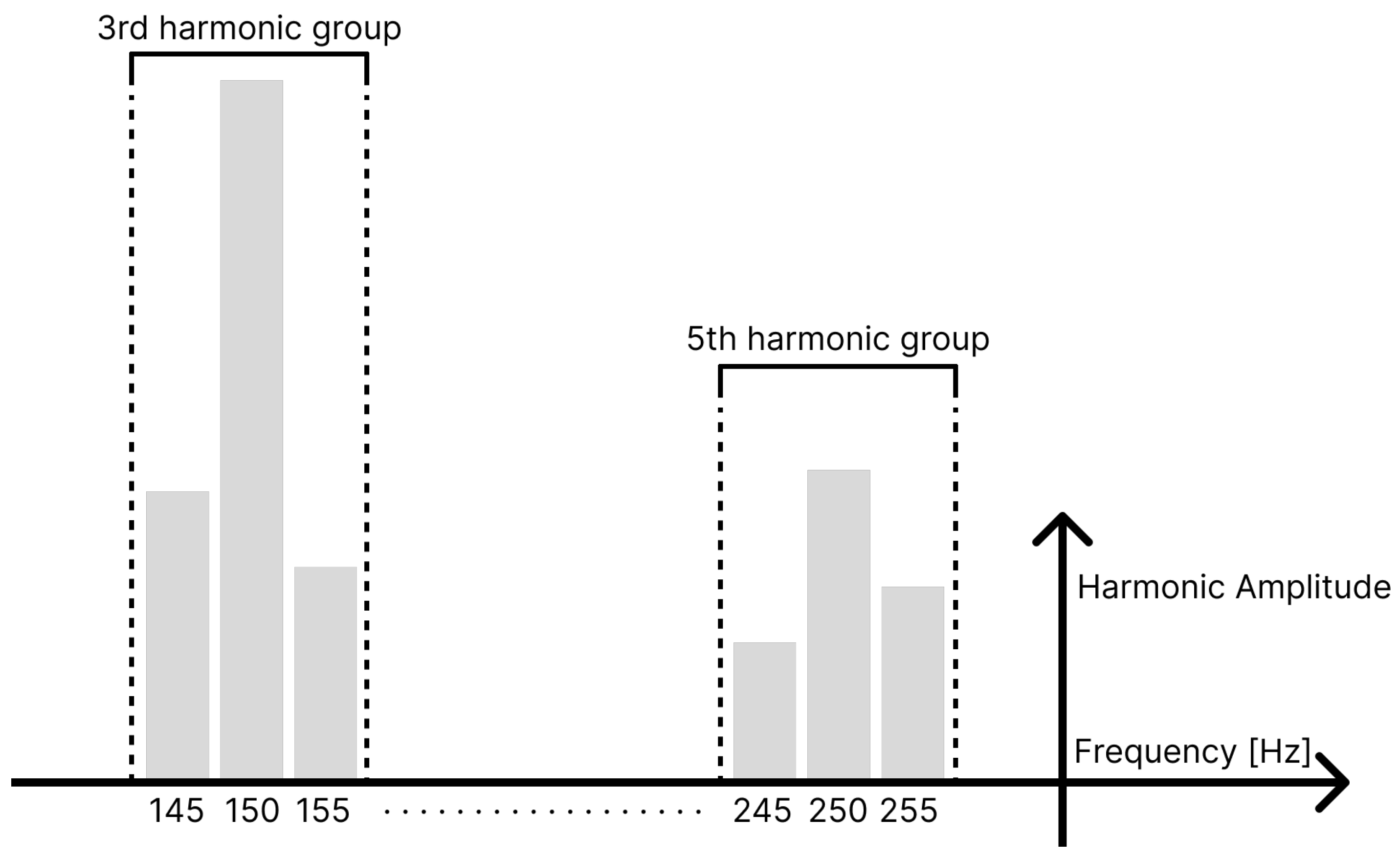
- = Bins width (5 Hz in this case);
- h = Harmonic order;
- = Group value at the order h.
4.2. THD Evaluation
- Individually, by comparing their amplitude to the fundamental frequency;
- Globally, using the THD.
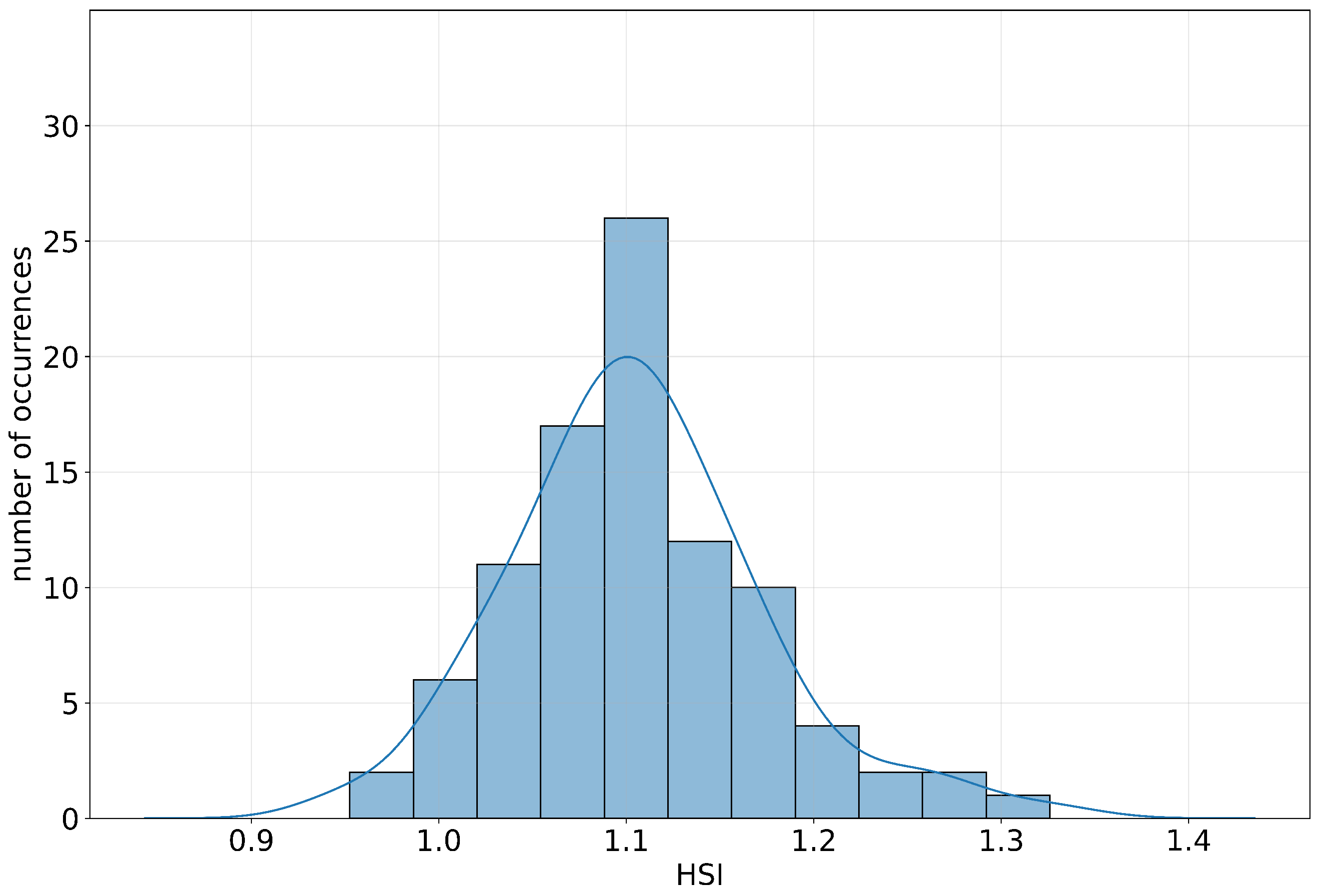
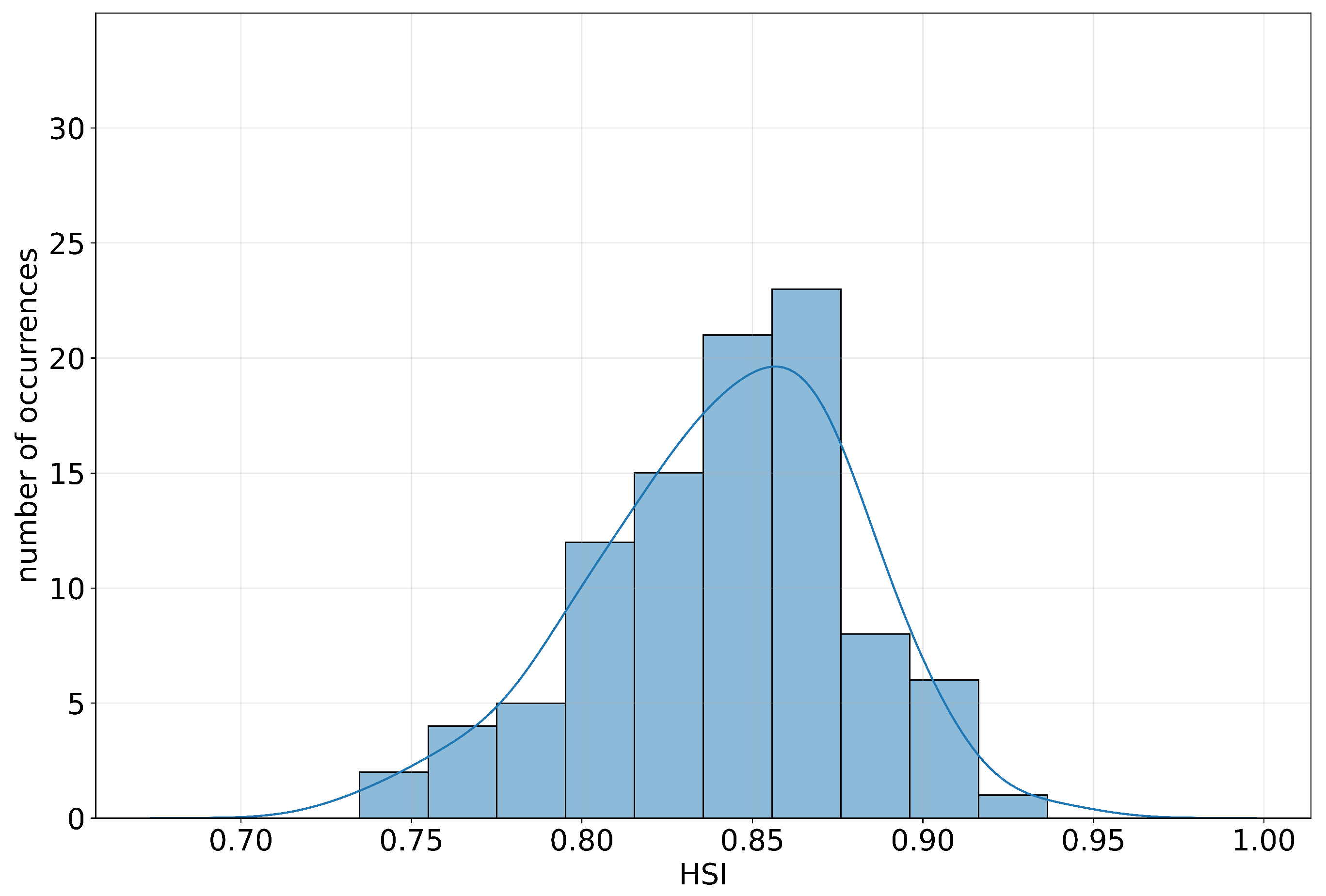
4.3. Harmonic Sum Evaluation
- HSI ≥ 1: The harmonics summed;
- HSI < 1: The harmonics canceled.
4.4. Data Cleaning
- x is the measured sample (taken as column);
- is the mean value of the measured sample;
- S is the variance–covariance matrix of the transposed measured sample;
- T represents the transpose operator.
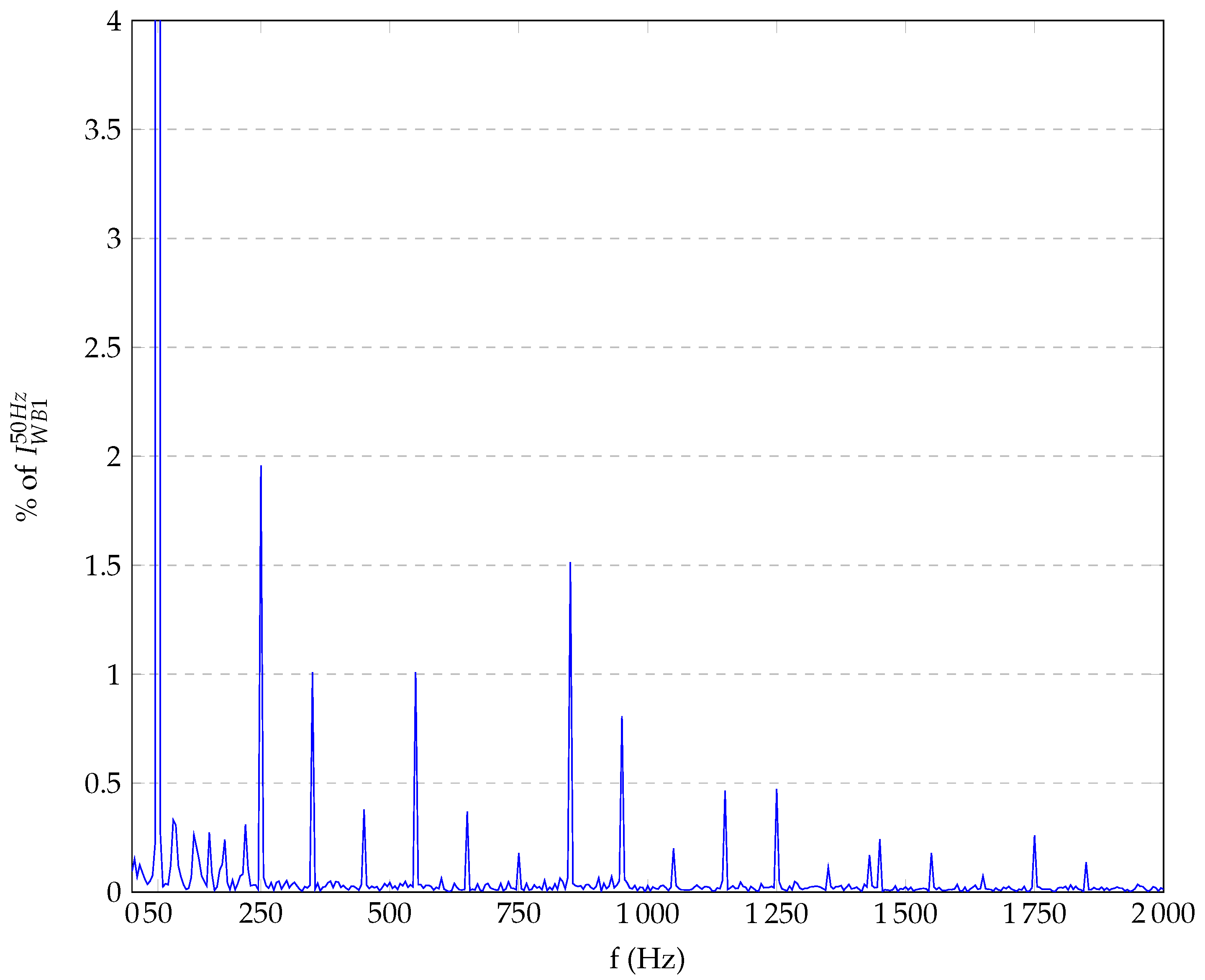
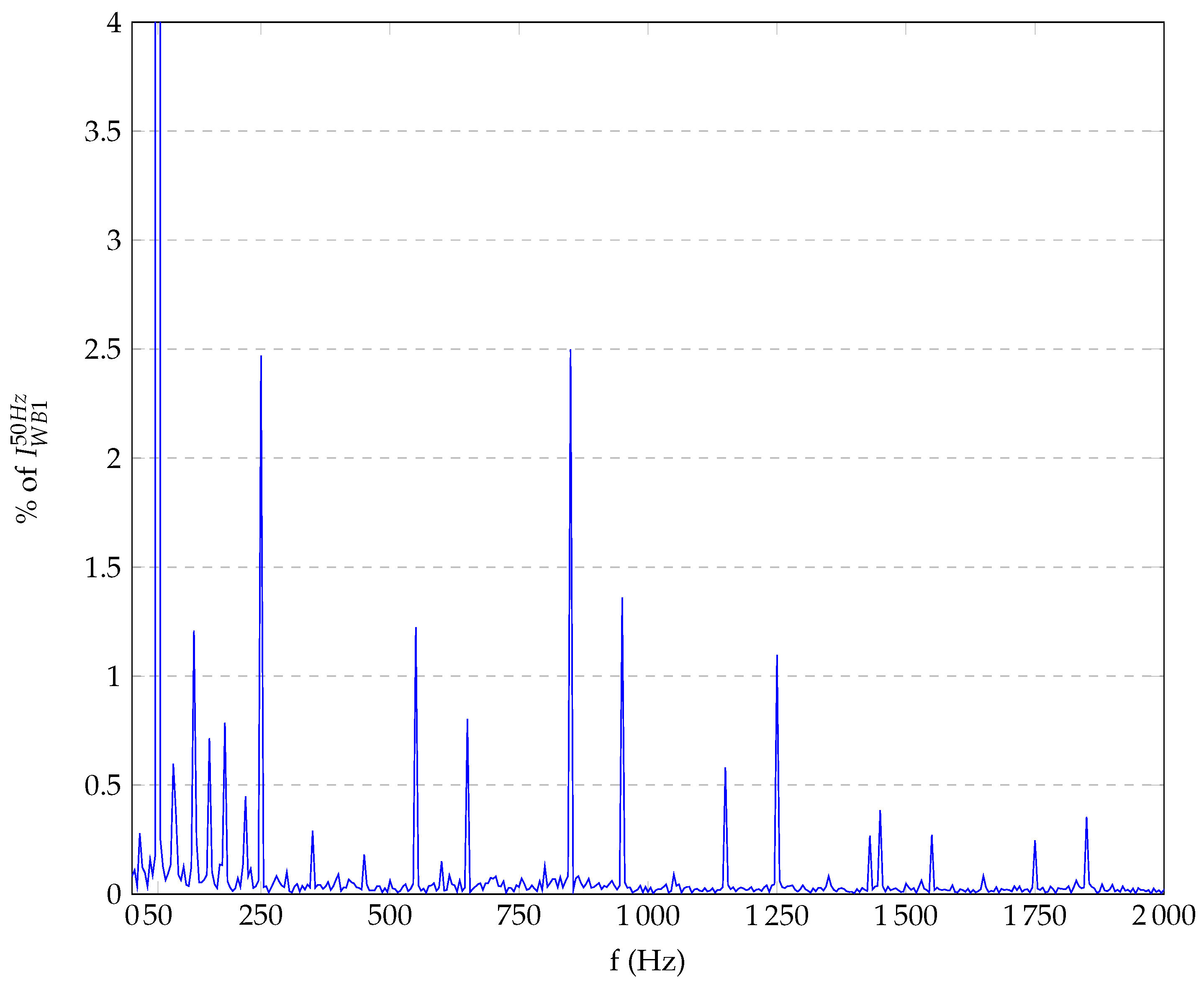
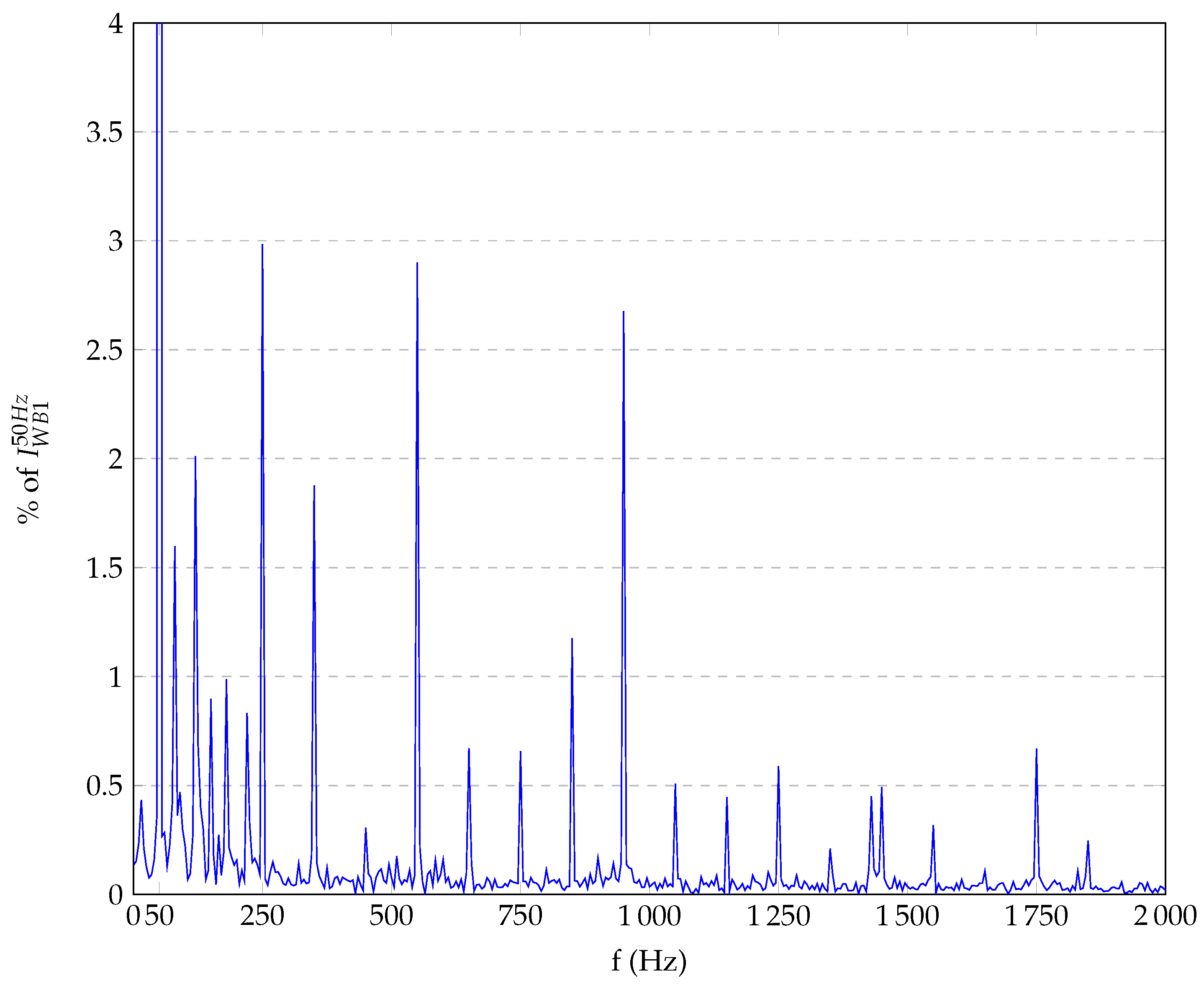
5. Test Results
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| BEV | Battery Electric Vehicle |
| DUT | Device Under Test |
| G2V | Grid-to-Vehicle |
| EV | Electric Vehicle |
| EVCS | Electric Vehicle Charging Station |
| FFT | Fast Fourier Transformation |
| HSI | Harmonic Summation Index |
| PHEV | Plug-in Hybrid Electric Vehicle |
| PHIL | Power Hardware-in-the-Loop |
| RES | Renewable Energy Source |
| RMS | Root Mean Square |
| THD | Total Harmonic Distortion |
| V2X | Vehicle-to-Everything |
| V2B | Vehicle-to-Building |
| V2G | Vehicle-to-Grid |
| WB | WallBox |
Appendix A


References
- EU. European Green Deal. Available online: https://commission.europa.eu/strategy-and-policy/priorities-2019-2024/european-green-deal_en (accessed on 28 July 2019).
- IEA. Global EV Outlook 2023–Analysis; IEA: Paris, France, 2023. [Google Scholar]
- IEA. Technology and Innovation Pathways for Zero-Carbon-Ready Buildings by 2030–Analysis; IEA: Paris, France, 2022. [Google Scholar]
- Savari, G.F.; Sathik, M.J.; Raman, L.A.; El-Shahat, A.; Hasanien, H.M.; Almakhles, D.; Abdel Aleem, S.H.E.; Omar, A.I. Assessment of charging technologies, infrastructure and charging station recommendation schemes of electric vehicles: A review. Ain Shams Eng. J. 2023, 14, 101938. [Google Scholar] [CrossRef]
- Barreto, R.; Faria, P.; Vale, Z. Electric Mobility: An Overview of the Main Aspects Related to the Smart Grid. Electronics 2022, 11, 1311. [Google Scholar] [CrossRef]
- IEEE Std 519-2014 (Revision of IEEE Std 519-1992); IEEE Recommended Practice and Requirements for Harmonic Control in Electric Power Systems. IEEE: New York, NY, USA, 2014; pp. 1–29. [CrossRef]
- IEC 61000-4-30; Standard IEC 61000-4-30 Electromagnetic Compatibility (EMC). Testing and Measurement Techniques. Power Quality Measurement Methods. International Electrotechnical Commission: Geneva, Switzerland, 2015.
- Nour, M.; Chaves-Avila, J.P.; Magdy, G.; Sanchez-Miralles, A. Review of Positive and Negative Impacts of Electric Vehicles Charging on Electric Power Systems. Energies 2020, 13, 4675. [Google Scholar] [CrossRef]
- IEC. International Electrotechnical Vocabulary; International Electrotechnical Committe (IEC): Geneva, Switzerland, 2016. [Google Scholar]
- EN 50160; Standard EN 50160 Voltage Characteristics of Electricity Supplied by Public Electricity Networks. CENELEC: Brussels, Belgium, 2022.
- TR 61000-1-1; IEC TR 61000-1-1 Electromagnetic Compatibility (EMC)-Part 1-1: General-Application and Interpretation of Fundamental Definitions and Terms. International Electrotechnical Commission: Geneva, Switzerland, 2023.
- Dougherty, J.; Stebbins, W. Power quality: A utility and industry perspective. In Proceedings of the 1997 IEEE Annual Textile, Fiber and Film Industry Technical Conference, Greenville, SC, USA, 6–8 May 1997; pp. 5–10. [Google Scholar] [CrossRef]
- Pandya, R.; Bhavsar, F. An Overview on Power Quality Issues In Smart Grid. IOSR J. Electr. Electron. Eng. 2018, 13, 1–4. [Google Scholar]
- Awadallah, M.A.; Singh, B.N.; Venkatesh, B. Impact of EV Charger Load on Distribution Network Capacity: A Case Study in Toronto. Can. J. Electr. Comput. Eng. 2016, 39, 268–273. [Google Scholar] [CrossRef]
- Alghsoon, E.; Harb, A.; Hamdan, M. Power quality and stability impacts of Vehicle to grid (V2G) connection. In Proceedings of the 2017 8th International Renewable Energy Congress (IREC), Dead Sea, Jordan, 21–23 March 2017; pp. 1–6. [Google Scholar] [CrossRef]
- Fast Charging Diversity Impact on Total Harmonic Distortion Due to Phase Cancellation Effect: Fast Charger’s Testing Experimental Results; Publications Office of the European Union: Brussels, Belgium, 2017.
- Mazurek, P.; Chudy, A. An Analysis of Electromagnetic Disturbances from an Electric Vehicle Charging Station. Energies 2022, 15, 244. [Google Scholar] [CrossRef]
- Slangen, T.M.H.; van Wijk, T.; Ćuk, V.; Cobben, J.F.G. The Harmonic and Supraharmonic Emission of Battery Electric Vehicles in The Netherlands. In Proceedings of the 2020 International Conference on Smart Energy Systems and Technologies (SEST), Istanbul, Turkey, 7–9 September 2020; pp. 1–6. [Google Scholar] [CrossRef]
- Bollen, M.H.J.; Olofsson, M.; Larsson, A.; Ronnberg, S.K.; Lundmark, M. Standards for supraharmonics (2 to 150 kHz). IEEE Electromagn. Compat. Mag. 2014, 3, 114–119. [Google Scholar] [CrossRef]
- Rönnberg, S.; Bollen, M. Propagation of Supraharmonics in the Low Voltage Grid. Report 2017:461; Energiforsk: Stockholm, Sweden, 2017. [Google Scholar]
- Lucas, A.; Bonavitacola, F.; Kotsakis, E.; Fulli, G. Grid harmonic impact of multiple electric vehicle fast charging. Electr. Power Syst. Res. 2015, 127, 13–21. [Google Scholar] [CrossRef]
- Dharmakeerthi, C.; Mithulananthan, N.; Saha, T. Overview of the impacts of plug-in electric vehicles on the power grid. In Proceedings of the 2011 IEEE PES Innovative Smart Grid Technologies, Perth, WA, Australia, 13–16 November 2011; pp. 1–8. [Google Scholar] [CrossRef]
- Mazza, A.; Pons, E.; Bompard, E.; Benedetto, G.; Tosco, P.; Zampolli, M.; Jaboeuf, R. A Power Hardware-In-the-Loop Laboratory Setup to Study the Operation of Bidirectional Electric Vehicles Charging Stations. In Proceedings of the 2022 International Conference on Smart Energy Systems and Technologies (SEST), Eindhoven, The Netherlands, 5–7 September 2022; pp. 1–6. [Google Scholar] [CrossRef]
- Benedetto, G.; Bompard, E.; Mazza, A.; Pons, E.; Jaboeuf, R.; Tosco, P.; Zampolli, M. Impact of bidirectional EV charging stations on a distribution network: A Power Hardware-In-the-Loop implementation. Sustain. Energy Grids Netw. 2023, 35, 101106. [Google Scholar] [CrossRef]
- 4Q POWER AMPLIFIER PCU-3x7000-AC/DC-400V-54A-4G with Different Options-User Manual; Spherea Puissance Plus: Colomiers, France, 2019.
- IEEE Std 519-2022 (Revision of IEEE Std 519-2014); IEEE Standard for Harmonic Control in Electric Power Systems. IEEE: New York, NY, USA, 2022; pp. 1–31.
- Ćuk, V.; Cobben, J.F.; Kling, W.L.; Ribeiro, P.F. Analysis of harmonic current summation based on field measurements. IET Gener. Transm. Distrib. 2013, 7, 1391–1400. [Google Scholar] [CrossRef]





| Cases | WB1 (kW) | WB2 (kW) |
|---|---|---|
| Case 1 | 4 | 4 |
| 8 | 6 | |
| 10 | 2 | |
| Case 2 | 11 | 11 |
| Cases | WB1 Power | WB2 Power | Power Supply | WB1 | WB2 |
|---|---|---|---|---|---|
| Case 1 | 4 kW | 4 kW | 5.26% | 5.63% | 5.25% |
| 8 kW | 6 kW | 4.05% | 4.30% | 4.57% | |
| 10 kW | 2 kW | 3.79% | 3.52% | 7.30% | |
| Case 2 | 11 kW | 11 kW | 3.01% | 3.05% | 3.10% |
| Cases | WB1 Power | WB2 Power | Power Supply | WB1 | WB2 |
|---|---|---|---|---|---|
| Case 1 | 4 kW | 4 kW | 2.08% | 2.09% | 2.07% |
| 8 kW | 6 kW | 2.31% | 2.32% | 2.30% | |
| 10 kW | 2 kW | 2.22% | 2.26% | 2.22% | |
| Case 2 | 11 kW | 11 kW | 2.08% | 2.11% | 2.07% |
| Test No. | Harmonic Order | ||||
|---|---|---|---|---|---|
| 1 | 2 | … | 39 | 40 | |
| 0 | 0.9976 | 0.6073 | … | 0.7367 | 1.0349 |
| 1 | 0.9973 | 0.7611 | … | 0.8793 | 0.6176 |
| 2 | 0.9975 | 0.4813 | … | 1.0236 | 0.9997 |
| … | … | … | … | … | … |
| 98 | 0.9977 | 0.3999 | … | 0.8607 | 0.7854 |
| 99 | 0.9974 | 0.4660 | … | 1.0150 | 0.3912 |
| 100 | 0.9976 | 0.8801 | … | 0.5548 | 0.9968 |
| Test No. | Harmonic Order | ||||
|---|---|---|---|---|---|
| 1 | 2 | … | 39 | 40 | |
| 0 | 14.5776 | 0.0249 | … | 0.0082 | 0.0027 |
| 1 | 14.3311 | 0.0224 | … | 0.0082 | 0.0048 |
| 2 | 14.4597 | 0.0187 | … | 0.0048 | 0.0042 |
| … | … | … | … | … | … |
| 98 | 14.4420 | 0.0201 | … | 0.0097 | 0.0059 |
| 99 | 14.4745 | 0.0175 | … | 0.0069 | 0.0037 |
| 100 | 14.5909 | 0.0154 | … | 0.0079 | 0.0025 |
| Test No. | Harmonic Order | ||||
|---|---|---|---|---|---|
| 1 | 2 | … | 39 | 40 | |
| 0 | 4.2347 | 0.0123 | … | 0.0047 | 0.0042 |
| 1 | 4.2324 | 0.0192 | … | 0.0062 | 0.0049 |
| 2 | 4.2647 | 0.0204 | … | 0.0030 | 0.0035 |
| … | … | … | … | … | … |
| 98 | 4.2683 | 0.0167 | … | 0.0045 | 0.0041 |
| 99 | 4.2781 | 0.0128 | … | 0.0032 | 0.0046 |
| 100 | 4.2731 | 0.0106 | … | 0.0049 | 0.0045 |
| Test No. | Harmonic Order | ||||
|---|---|---|---|---|---|
| 1 | 2 | … | 39 | 40 | |
| 0 | 18.7676 | 0.0226 | … | 0.0095 | 0.0071 |
| 1 | 18.5142 | 0.0317 | … | 0.0127 | 0.0060 |
| 2 | 18.6780 | 0.0188 | … | 0.0079 | 0.0077 |
| … | … | … | … | … | … |
| 98 | 18.6665 | 0.0147 | … | 0.0122 | 0.0078 |
| 99 | 18.7041 | 0.0141 | … | 0.0102 | 0.0032 |
| 100 | 18.8189 | 0.0229 | … | 0.0071 | 0.0070 |
| Harmonic Order | Index | Values | ||
|---|---|---|---|---|
| P1 = 10 kW P2 = 2 kW | P1 = 11 kW P2 = 11 kW | P1 = 10 kW P2 = 2 kW | P1 = 11 kW P2 = 11 kW | |
| 17 | 0.98 | 1 | 2.23 | 1.8 |
| 5 | 0.9 | 0.97 | 2.03 | 1.76 |
| 11 | 0.91 | 1.01 | 1.29 | 0.82 |
| 19 | 0.81 | 0.99 | 1.15 | 0.86 |
| 3 | 0.84 | 1.11 | 0.67 | 0.42 |
| 25 | 0.96 | 0.96 | 0.64 | 0.53 |
| 13 | 0.63 | 0.96 | 0.48 | 0.34 |
| 7 | 0.41 | 1.03 | 0.46 | 0.64 |
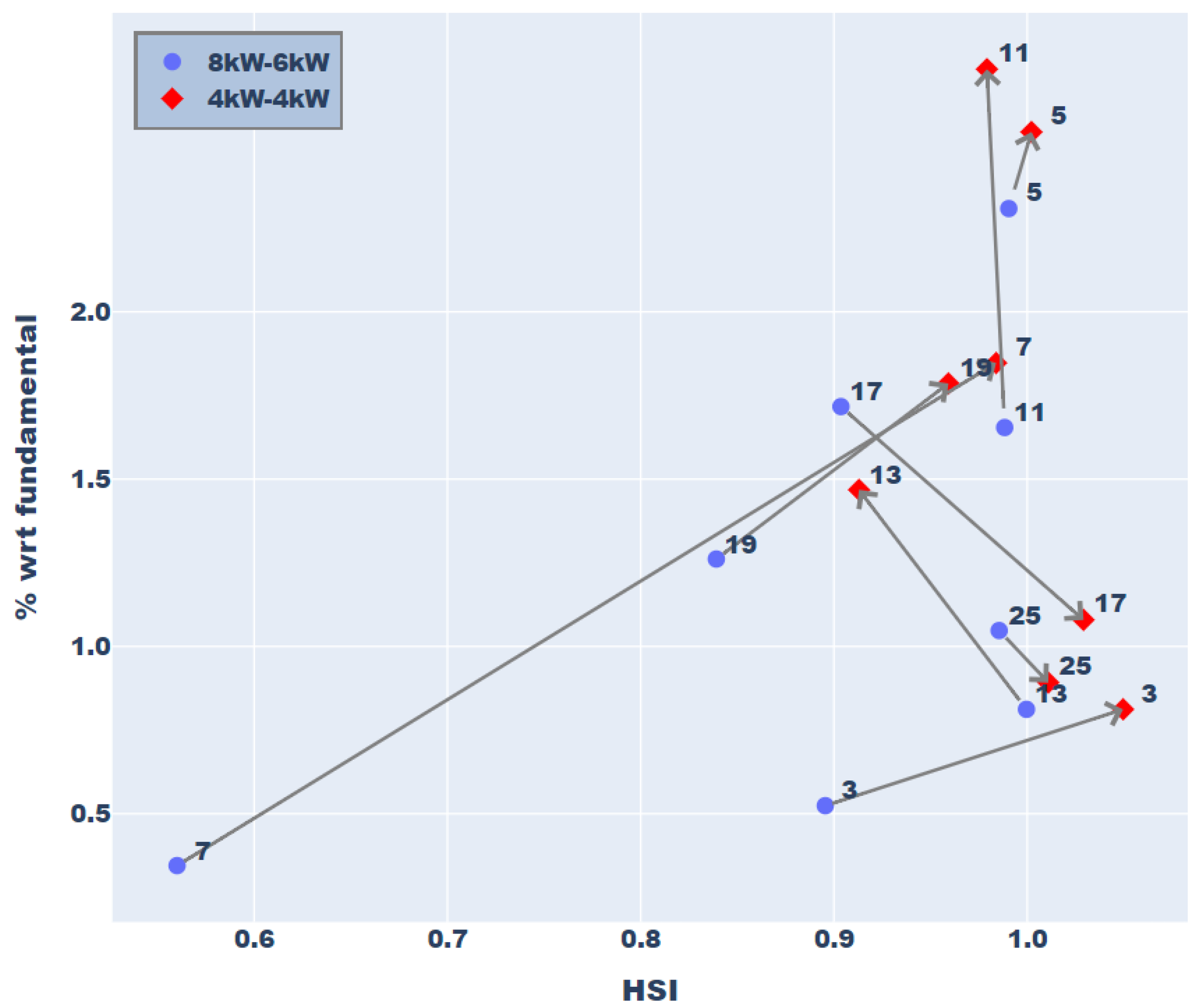
| Harmonic Order | Index | Values | ||
|---|---|---|---|---|
| P1 = 8 kW P2 = 6 kW | P1 = 4 kW P2 = 4 kW | P1 = 8 kW P2 = 6 kW | P1 = 4 kW P2 = 4 kW | |
| 11 | 0.99 | 0.98 | 1.65 | 2.73 |
| 5 | 0.99 | 1 | 2.31 | 2.54 |
| 7 | 0.56 | 0.98 | 0.34 | 1.85 |
| 19 | 0.84 | 0.96 | 1.26 | 1.79 |
| 13 | 1 | 0.91 | 0.81 | 1.47 |
| 17 | 0.9 | 1.03 | 1.72 | 1.08 |
| 25 | 0.99 | 1.01 | 1.05 | 0.89 |
| 3 | 0.9 | 1.05 | 0.52 | 0.81 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mazza, A.; Benedetto, G.; Bompard, E.; Nobile, C.; Pons, E.; Tosco, P.; Zampolli, M.; Jaboeuf, R. Interaction among Multiple Electric Vehicle Chargers: Measurements on Harmonics and Power Quality Issues. Energies 2023, 16, 7051. https://doi.org/10.3390/en16207051
Mazza A, Benedetto G, Bompard E, Nobile C, Pons E, Tosco P, Zampolli M, Jaboeuf R. Interaction among Multiple Electric Vehicle Chargers: Measurements on Harmonics and Power Quality Issues. Energies. 2023; 16(20):7051. https://doi.org/10.3390/en16207051
Chicago/Turabian StyleMazza, Andrea, Giorgio Benedetto, Ettore Bompard, Claudia Nobile, Enrico Pons, Paolo Tosco, Marco Zampolli, and Rémi Jaboeuf. 2023. "Interaction among Multiple Electric Vehicle Chargers: Measurements on Harmonics and Power Quality Issues" Energies 16, no. 20: 7051. https://doi.org/10.3390/en16207051
APA StyleMazza, A., Benedetto, G., Bompard, E., Nobile, C., Pons, E., Tosco, P., Zampolli, M., & Jaboeuf, R. (2023). Interaction among Multiple Electric Vehicle Chargers: Measurements on Harmonics and Power Quality Issues. Energies, 16(20), 7051. https://doi.org/10.3390/en16207051








