Study on Gasoline–Air Mixture Explosion Overpressure Characteristics and Flame Propagation Behaviors in an Annular Cylindrical Confined Space with a Circular Arch
Abstract
:1. Introduction
2. Materials and Methods
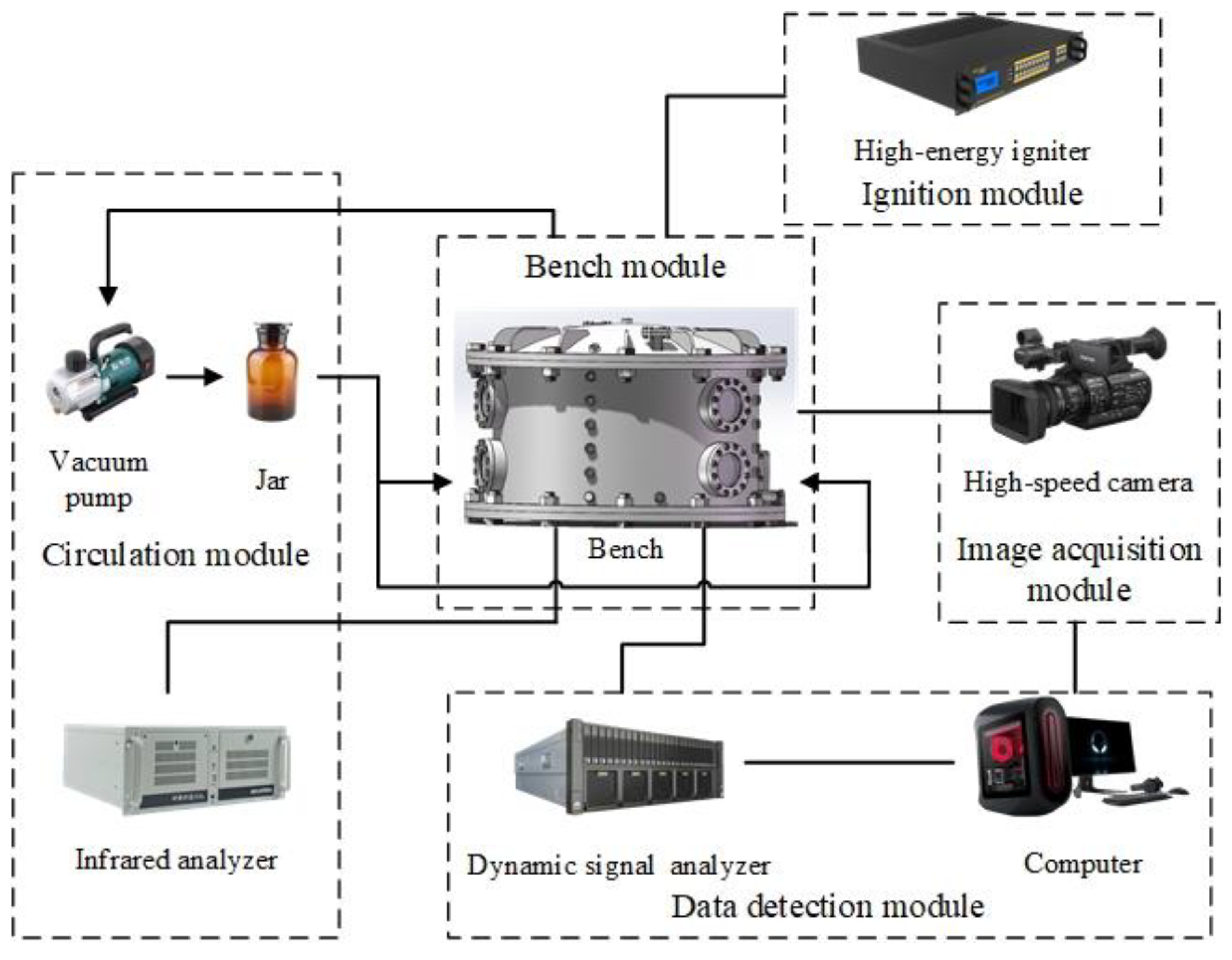
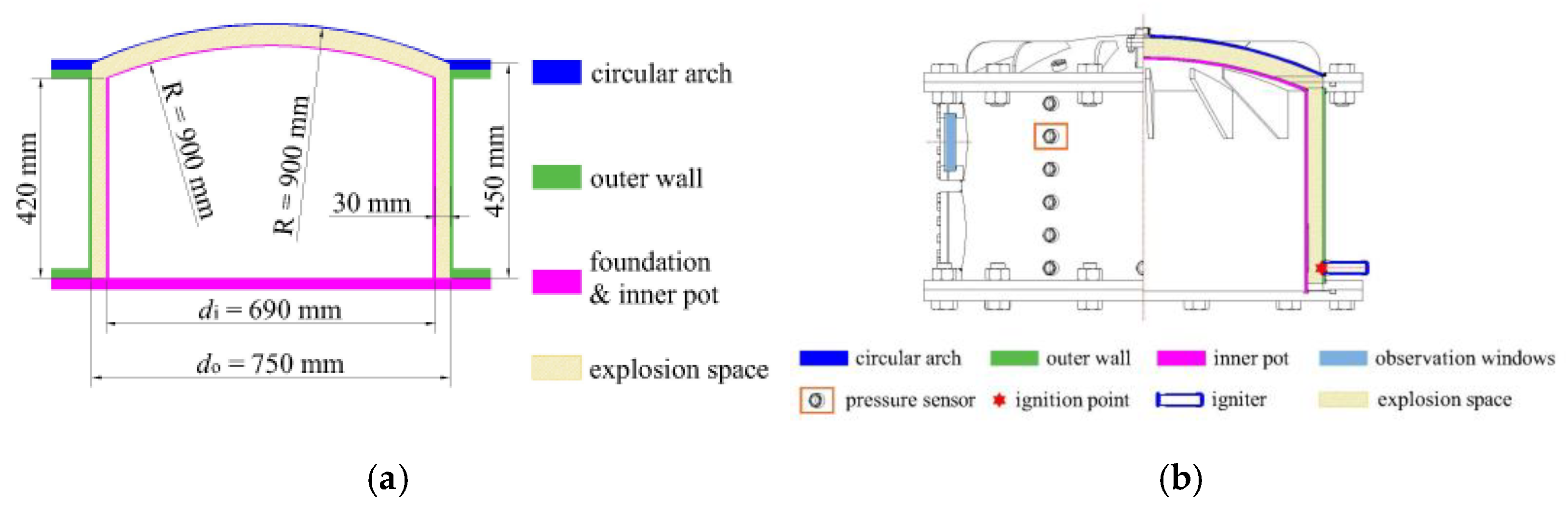
2.1. Experimental System
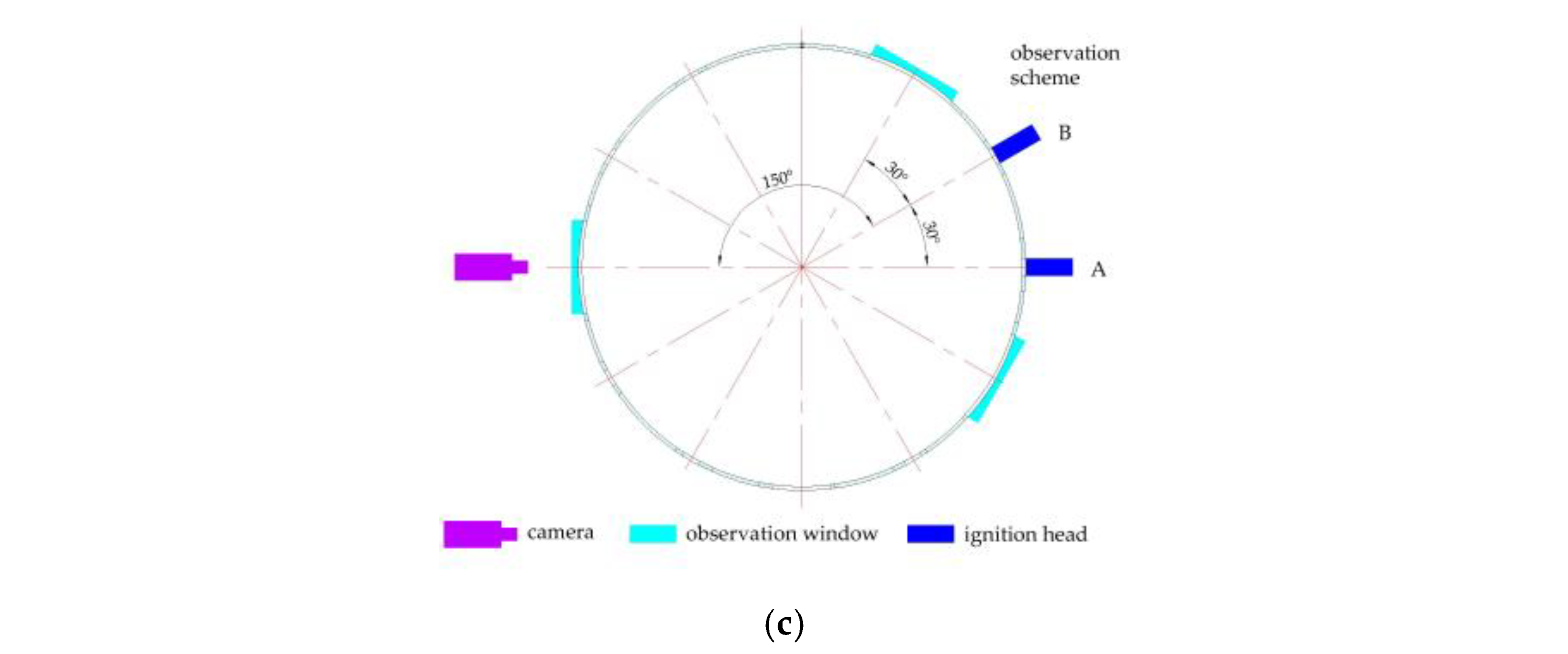
2.2. Experimental Scheme
3. Results and Discussion
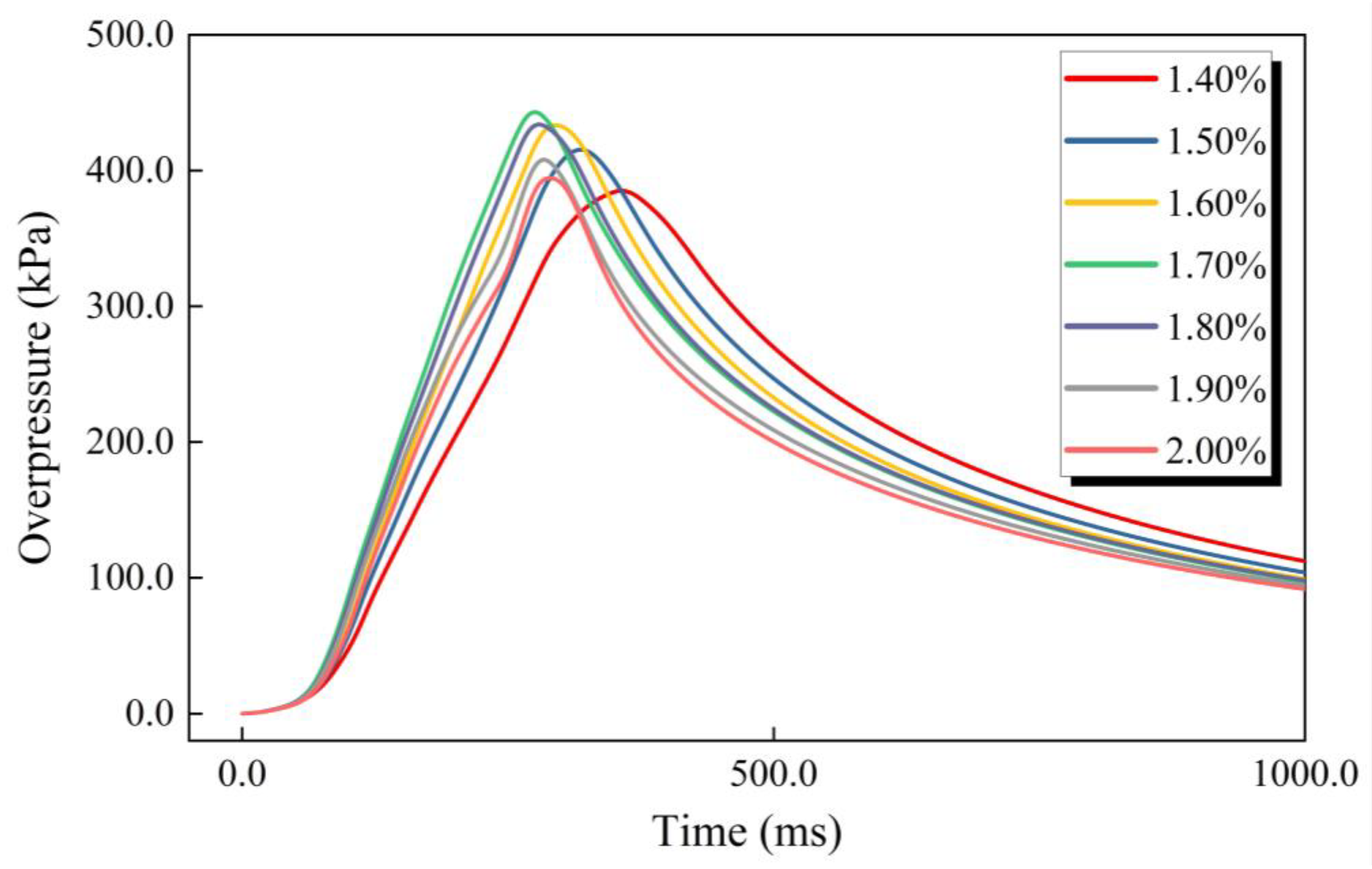
3.1. Explosion Overpressure Development Process
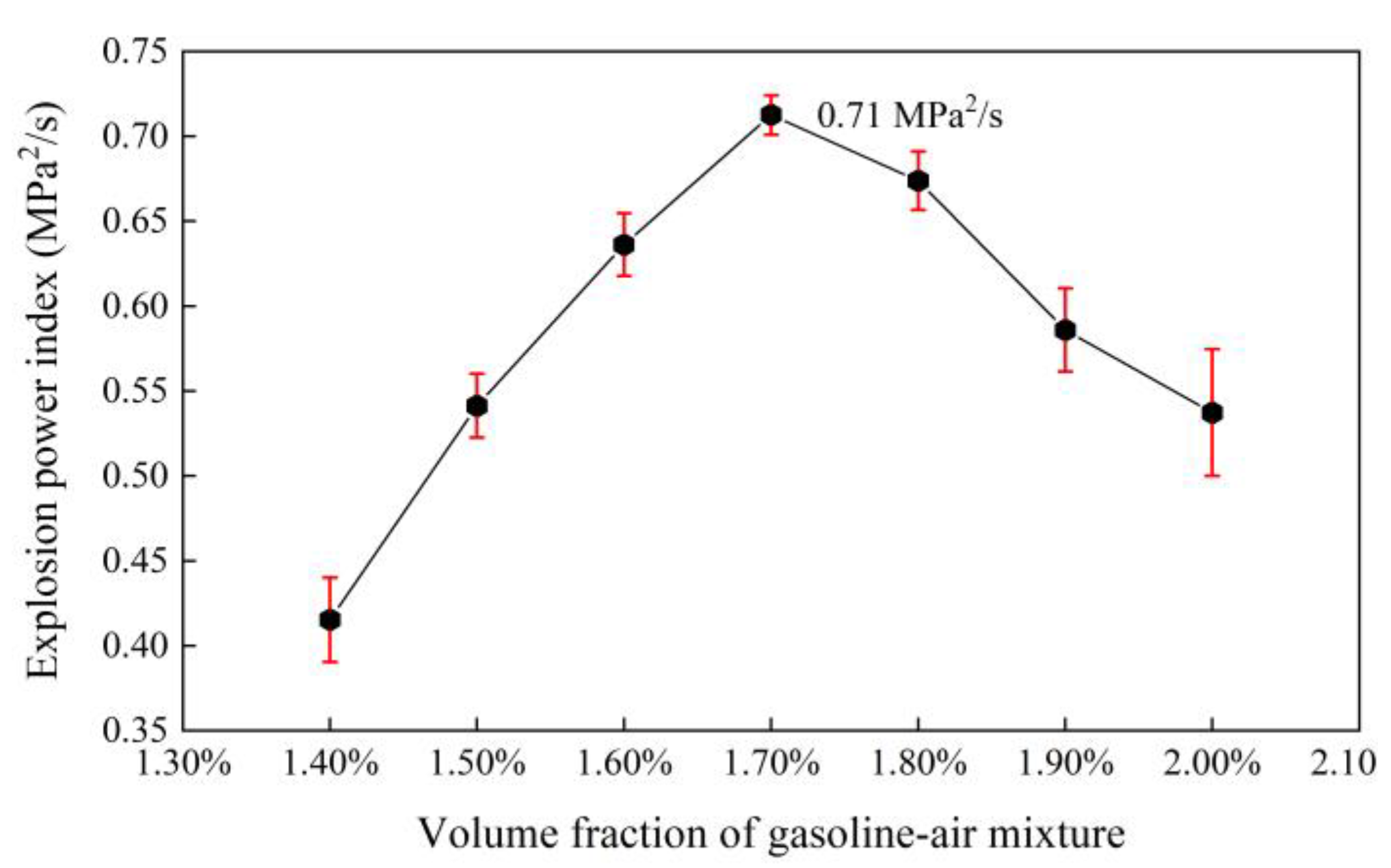
3.2. Explosion Overpressure Variation Characteristics
3.3. Flame Propagation Behaviors
4. Conclusions
- (1)
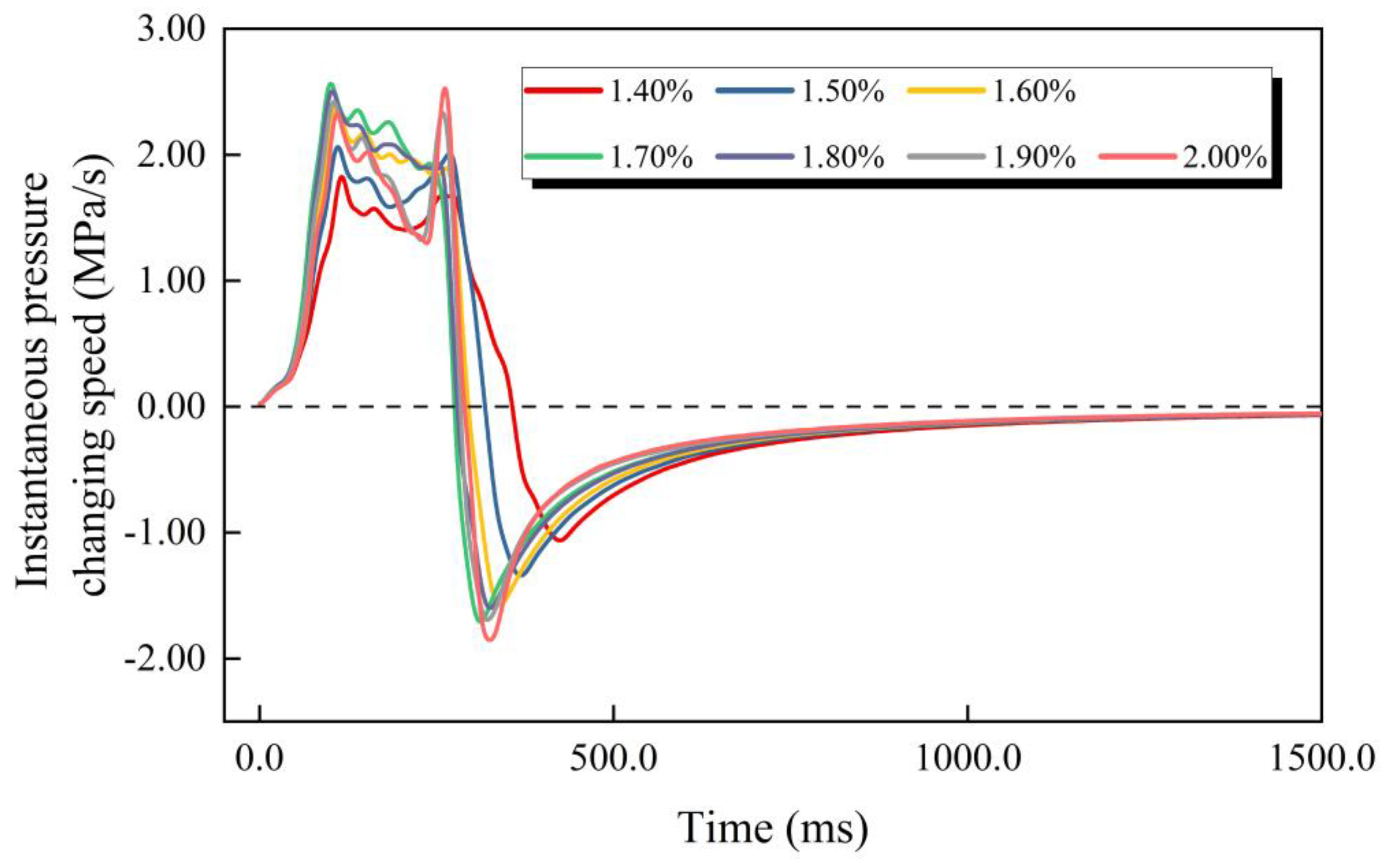
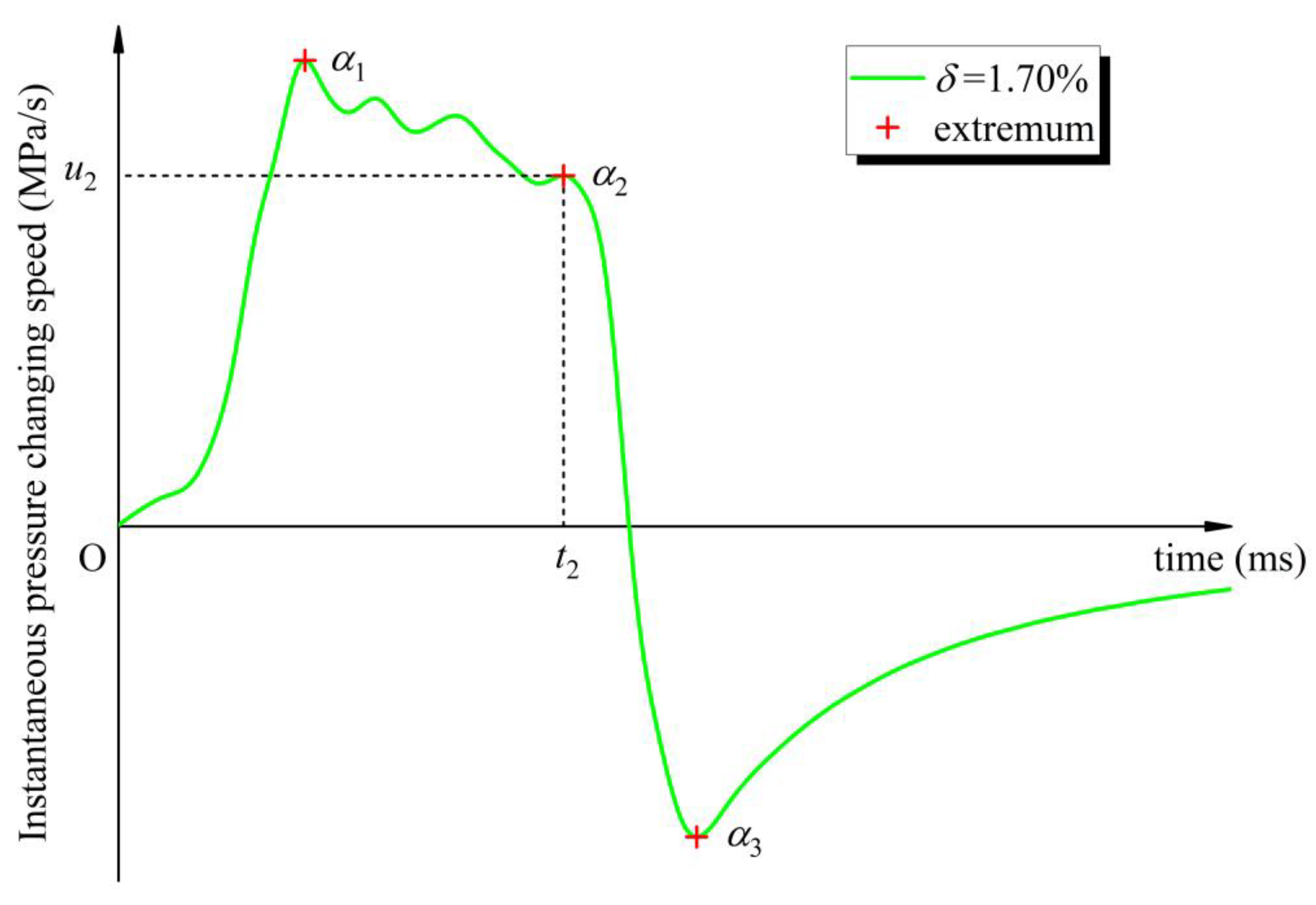
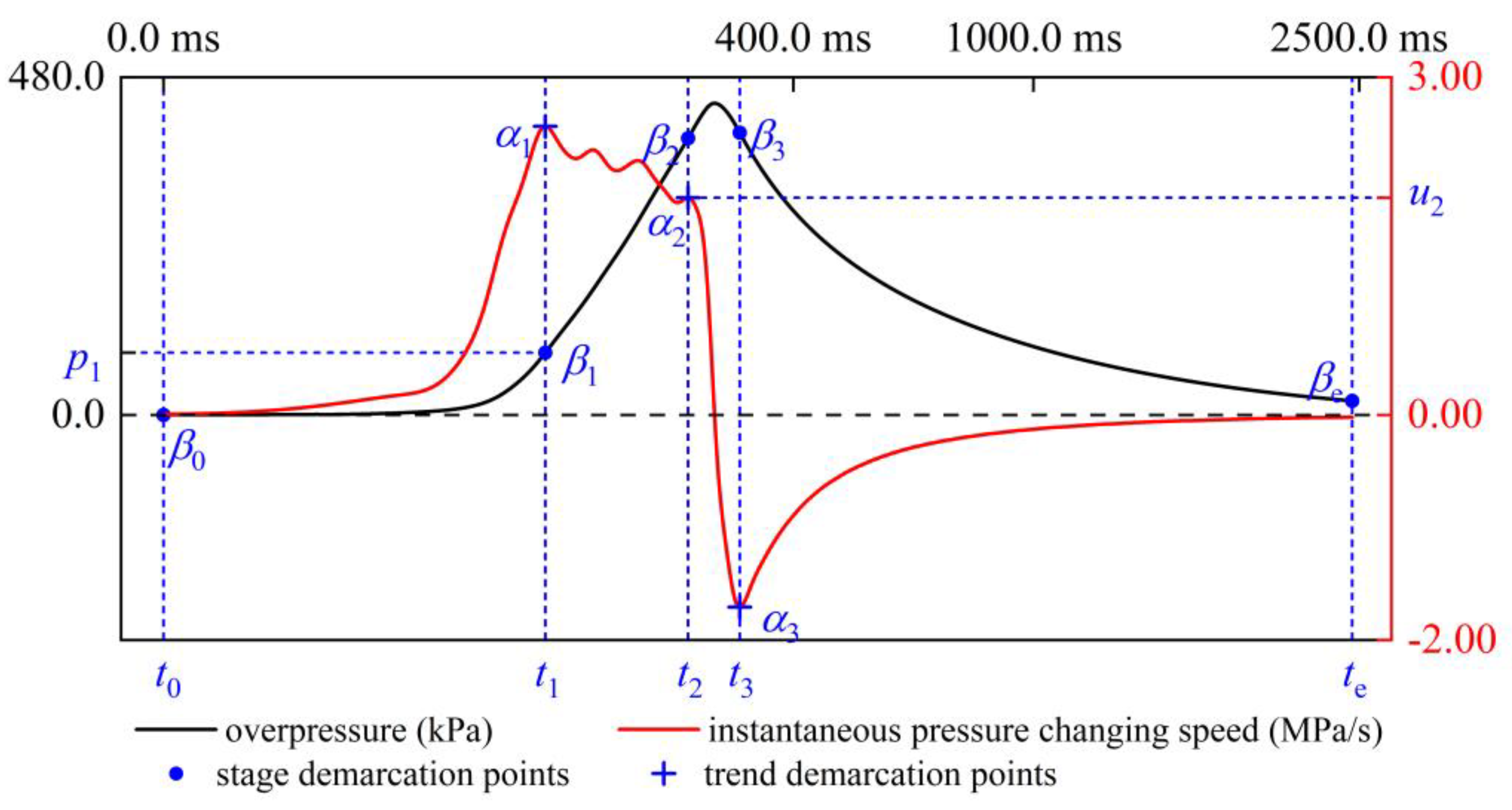
- The overpressure peak (pmax), average pressurization speed (uave), and explosion power index (EPI) first increased and then decreased as the gasoline–air mixture volume fraction (δ) increased during the explosion process in an annular cylindrical confined space with a circular arch, while the time to reach pmax (tmax) first decreased and then increased with δ increasing. The instantaneous pressure changing speed (uins) allowed the exploration of the development process of the explosion overpressure in more detail and more accurately, and showed the characteristics of first increasing, second oscillating, then decreasing, and finally increasing.
- (2)
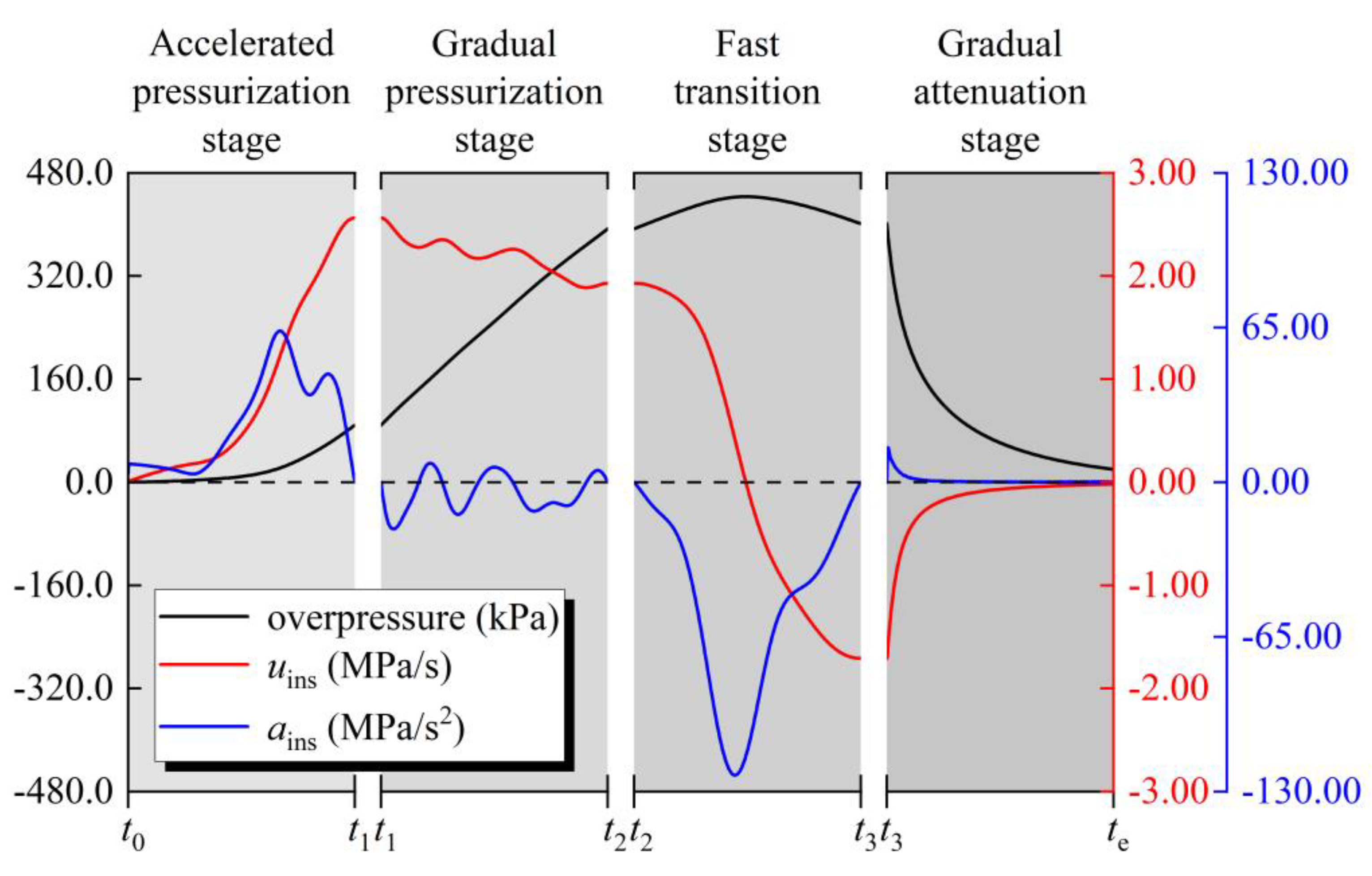
- The explosion process was divided into four stages according to the characteristics he tuins curve: accelerate pressurization, gradual pressurization, fast transition and gradual attenuation stage. The explosion overpressure maintained an accelerated increase in the accelerated pressurization stage but a gradual increase in the next stage, and pmax always appeared in the fast transition stage.
- (3)
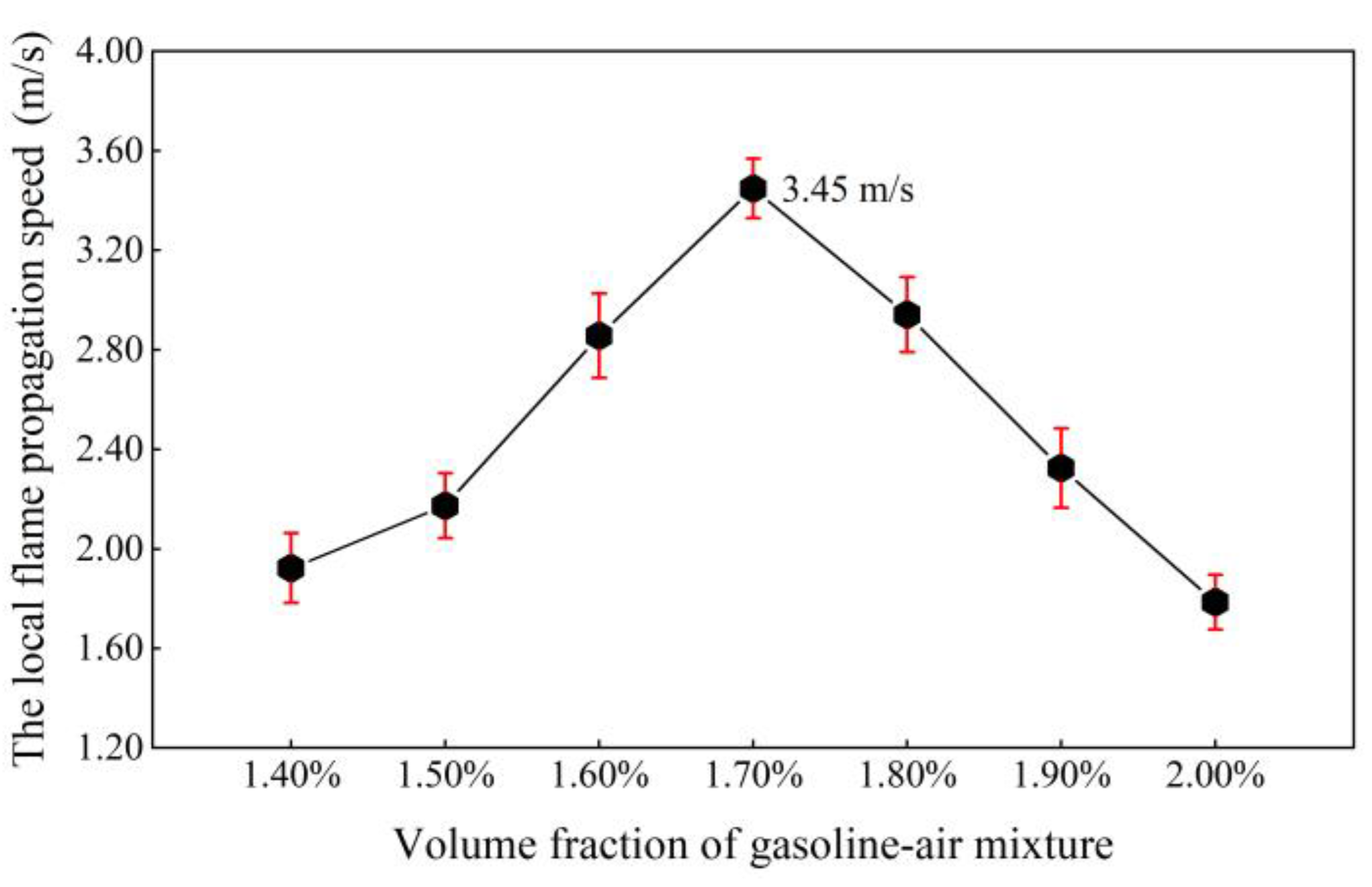
- The explosion flame mainly appeared in blue and yellow. The flame mainly appeared in a cloudlike shape and linear shape before the collision and only in a cloudlike shape during the collision, and extinguished quickly after collision. The local flame propagation speed (uf) first increased and then decreased with δ increasing, and attained the maximal value at δ = 1.70%.
- (4)
- The annular cylindrical confined space with a circular arch had a larger inner surface area in the same volume condition because the circumferential flame propagation distance was halved and the radial direction was narrow, which increased the explosion heat absorption speed, reduced the gasoline–air mixture chemical reaction speed and made the explosion flame propagate slowly. However, in this type of confined space, the structural characteristics of circumferential connection and top communication, which make the explosion flame collide inevitably, promoted positive feedback coupling between the flame and the pressure wave, making pmax and EPI larger and making the gasoline–air mixture explosion more violent and dangerous.
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| δ | Volume fraction of gasoline–air mixture |
| do, di | External diameter, internal diameter of annular cylindrical space |
| λ | Ratio of annular half-perimeter to radial width in a space |
| Φ | Diameter of bench observation window |
| pmax | Overpressure peak |
| tmax | Time to reach pmax |
| uave | Average pressurization speed |
| EPI | Explosion power index |
| uins | Instantaneous pressure changing speed |
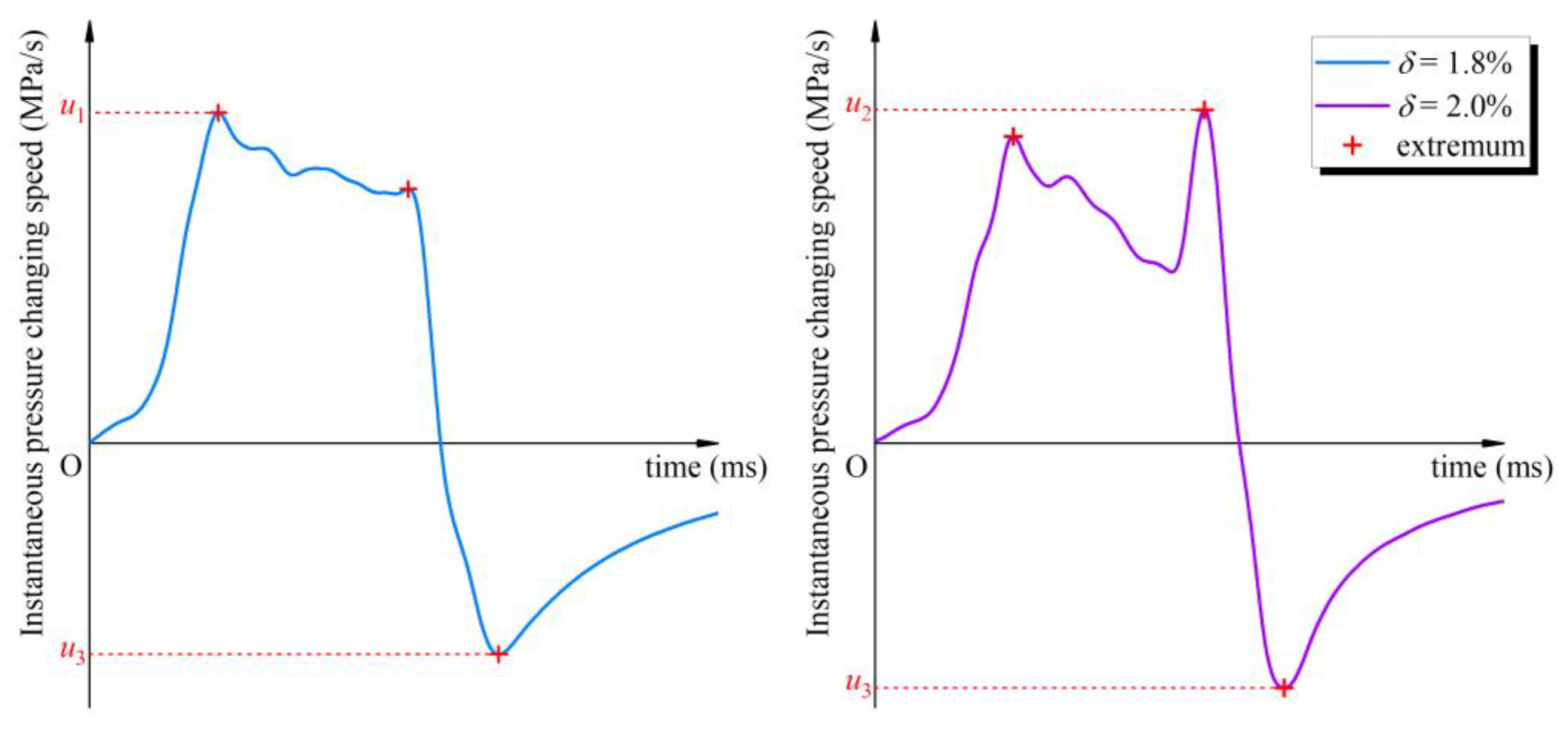
| α1, α2, α3 | Trend demarcation point |
| t1, t2, t3 | The first maximum point, the last maximum point, the last minimum point of uins function |
| u1, u2, u3 | The first maximum, the last maximum, the last minimum of uins function |
| θ | Changing amplitude of u2 compared with u1 |
| ains | Instantaneous pressure changing acceleration |
| β0, β1, β2, β3, βe | Stage demarcation point |
| t0, te | Ignition time, explosion end time |
| p0, p1, p2, p3, pe | Overpressure of each stage demarcation points |
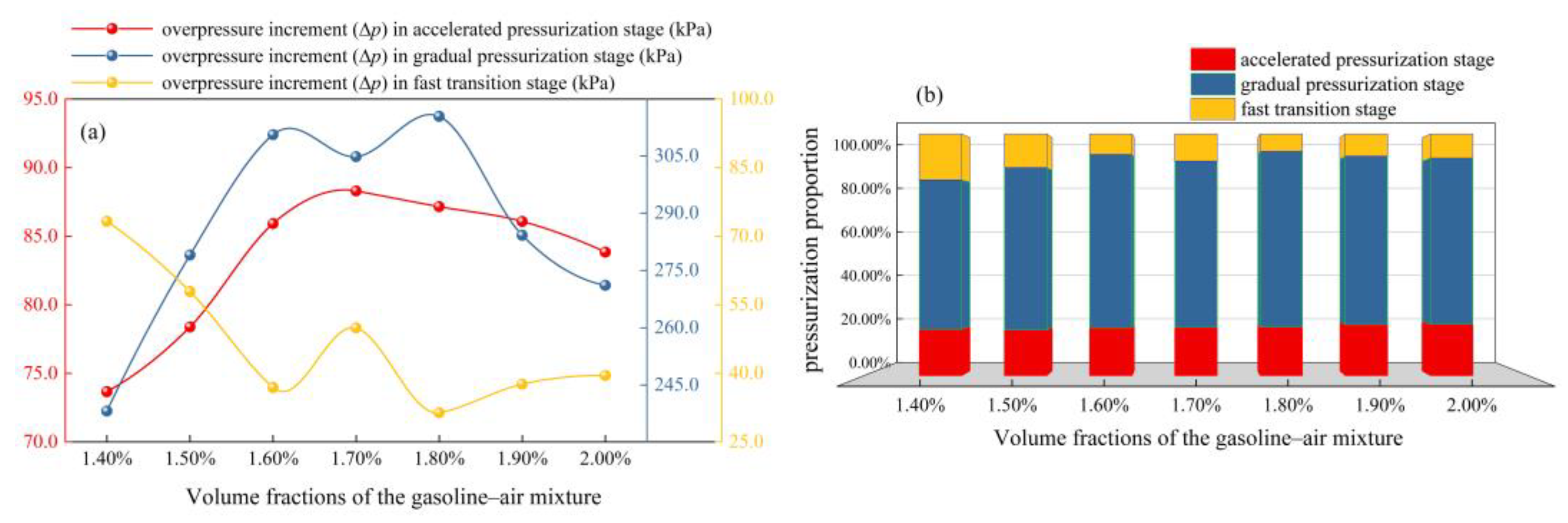
| Δp | Overpressure increment |
| η | Pressurization proportion |
| uf | Local flame propagation speed |
| tf | Duration of flame front from appearance to disappearance |
References
- Li, G.; Zheng, K.; Wang, S.; Chen, W. Comparative study on explosion characteristics of hydrogen and gasoline vapor in a semi-confined pipe based on Large Eddy Simulation. Fuel 2022, 328, 125334. [Google Scholar] [CrossRef]
- Movileanu, C.; Gosa, V.; Razus, D. Explosion of gaseous ethylene-air mixtures in closed cylindrical vessels with central ignition. J. Hazard. Mater. 2012, 235, 108–115. [Google Scholar] [CrossRef]
- Yao, Z.; Deng, H.; Dong, J.; Wen, X.; Zhang, X.; Wang, F.; Chen, G. On explosion characteristics of premixed syngas/air mixtures with different hydrogen volume fractions and ignition positions. Fuel 2020, 288, 119619. [Google Scholar] [CrossRef]
- Askari, O.; Moghaddas, A.; Alholm, A.; Vien, K.; Alhazmi, B.; Metghalchi, H. Laminar burning speed measurement and flame instability study of H2/CO/air mixtures at high temperatures and pressures using a novel multi-shell model. Combust. Flame 2016, 168, 20–31. [Google Scholar] [CrossRef]
- Leo, Y.; Zhang, B. Explosion behavior of methane-air mixtures and Rayleigh-Taylor instability in the explosion process near the flammability limits. Fuel 2022, 324, 124730. [Google Scholar] [CrossRef]
- Liang, W.; Mével, R.; Law, C.K. Role of low-temperature chemistry in detonation of n -heptane/oxygen/diluent mixtures. Combust. Flame 2018, 193, 463–470. [Google Scholar] [CrossRef]
- Jin, K.; Wang, Q.; Duan, Q.; Chen, J.; Sun, J. Effect of ignition position on premixed hydrogen-air flame quenching behaviors under action of metal wire mesh. Fuel 2020, 289, 119750. [Google Scholar] [CrossRef]
- Wang, C.; Guo, J.; Yang, F.; Zhang, G. Effects of ignition position on the ignitability and flame behavior of stratified gasoline vapor. Fuel 2021, 304, 121361. [Google Scholar] [CrossRef]
- Li, G.; Wang, X.; Zhao, X. Experimental study on explosion characteristics of ethanol-gasoline blended fuels. J. Loss. Prevent. Proc. 2020, 64, 104083. [Google Scholar] [CrossRef]
- Peng, H.; Huang, Y.; Deiterding, R.; Luan, Z.; Xing, F.; You, Y. Effects of jet in crossflow on flame acceleration and deflagration to detonation transition in methane–oxygen mixture. Combust. Flame 2018, 198, 69–80. [Google Scholar] [CrossRef]
- Wang, Q.; Jin, S.; Luo, Z.; Dai, A.; Wang, Q.; Li, Z. Flame propagation characteristics of methane explosion under different venting conditions. Fuel 2023, 334, P1. [Google Scholar] [CrossRef]
- Guo, J.; Liu, X.; Wang, C. Experiments on vented hydrogen-air deflagrations: The influence of hydrogen concentration. J. Loss. Prevent. Proc. 2017, 48, 254–259. [Google Scholar] [CrossRef]
- Li, G.; Wang, X.; Xu, H.; Liu, Y.; Zhang, H. Experimental study on explosion characteristics of ethanol gasoline-air mixture and its mitigation using heptafluoropropane. J. Hazard. Mater. 2019, 378, 120711. [Google Scholar] [CrossRef]
- Pan, C.; Li, G.; Wang, X. Effects of top vent locations and gasoline volumes on vented gasoline vapor explosion in closed small-scale vessel. Fuel 2020, 277, 118226. [Google Scholar] [CrossRef]
- Jiang, X.; Yu, B.; Xu, J.; Wang, W.; Jiang, J.; Zhao, Y. Study on concentration distribution of radical groups of gasoline-air explosion in long-narrow confined space based on OH-PLIF. CIESC J. 2020, 71, 5352–5360. [Google Scholar]
- Wang, B.; Du, Y.; Qi, S.; Yuan, G.; Wang, S. Oscillation propagation characteristics of gasoline-air mixture explosion in elongated closed tubes. J. Vib. Shock. 2017, 36, 97–103. [Google Scholar]
- Wei, S.; Du, Y.; Wang, S.; Li, M. Experimental study on deflagration characteristics of gasoline-air mixture in confined space with different shapes. J. Saf. Sci. Technol. 2017, 13, 41–47. [Google Scholar]
- Wang, S.; Du, Y.; Gao, J.; Liang, J.; Zhang, P.; Li, G. An experimental study of internal gasoline-air mixture explosion loading in a semi-underground vault tank. J. Vib. Shock. 2017, 36, 239–244. [Google Scholar]
- Chang, X.; Bai, C.; Zhang, B. The effect of gas jets on the explosion dynamics of hydrogen-air mixtures. Process. Saf. Env. 2022, 162, 384–394. [Google Scholar] [CrossRef]
- Rui, S.; Wang, C.; Guo, S.; Jing, R.; Li, Q. Hydrogen-air explosion with concentration gradients in a cubic enclosure. Process. Saf. Environ. 2021, 151, 141–150. [Google Scholar] [CrossRef]
- Rui, S.; Wang, C.; Luo, X.; Li, Q.; Zhang, H. Experimental study on the effects of ignition location and vent burst pressure on vented hydrogen-air deflagrations in a cubic vessel. Fuel 2020, 278, 118342. [Google Scholar] [CrossRef]
- Shen, X.; He, X.; Sun, J. A comparative study on premixed hydrogen—air and propane—air flame propagations with tulip distortion in a closed duct. Fuel 2015, 161, 248–253. [Google Scholar] [CrossRef]
- Wang, Q.; Luo, X.; Wang, C.; Liu, Y.; Zhou, P.; Li, B. Experimental study on external explosion for vented hydrogen deflagration in a rectangular tube with different vent coefficients. Process. Saf. Environ. 2022, 158, 331–339. [Google Scholar] [CrossRef]
- Sun, S.; Qiu, Y.; Xing, H.; Wang, M. Effects of concentration and initial turbulence on the vented explosion characteristics of methane-air mixtures. Fuel 2020, 267, 117103. [Google Scholar] [CrossRef]
- Ji, H.; Lu, R.; Yang, K.; Jiang, J.; Xing, Z.; Guo, J. Experimental study on methane explosion suppression by heptafluoropropane drived modified ABC powder. Process. Saf. Environ. 2023, 170, 623–635. [Google Scholar] [CrossRef]
- Na Inna, A.M.; Phylaktou, H.N.; Andrews, G.E. Explosion flame acceleration over obstacles: Effects of separation distance for a range of scales. Process. Saf. Environ. 2017, 107, 309–316. [Google Scholar] [CrossRef]
- Saeed, K. Determination of the explosion characteristics of methanol—Air mixture in a constant volume vessel. Fuel 2017, 210, 729–737. [Google Scholar] [CrossRef]
- Wang, K.; Su, M.; Wei, L.; Chen, S.; Kong, X.; Fang, Y. Effect of initial turbulence on explosion behavior of stoichiometric methane-ethylene-air mixtures in confined space. Process. Saf. Environ. 2022, 161, 583–593. [Google Scholar] [CrossRef]
- Cao, Y.; Gao, K.; Li, B.; Xie, L.; Pan, X. Influence of vent size and vent burst pressure on vented ethylene-air explosion: Experimental and numerical study. Process. Saf. Environ. 2023, 170, 297–309. [Google Scholar] [CrossRef]
- Keisuke, N.; Akira, Y.; Makihito, N. Experiments and numerical simulation on the suppression of explosion of propane/air mixture by water mist. Combust. Flame 2021, 223, 192–201. [Google Scholar]
- Yu, M.; Wan, S.; Zheng, K.; Guo, P.; Chu, T.; Yuan, Z. Influence on the methane/air explosion characteristics of the side venting position in a pipeline. Process. Saf. Environ. 2017, 111, 292–299. [Google Scholar] [CrossRef]
- Giurcan, V.; Mitu, M.; Razus, D.; Oancea, D. Pressure and temperature influence on propagation indices of n-butane—Air gaseous mixtures. Process. Saf. Environ. 2017, 111, 94–101. [Google Scholar] [CrossRef]
- Ghiasi, G.; Ahmed, I.; Swaminathan, N. Gasoline flame behavior at elevated temperature and pressure. Fuel 2019, 238, 248–256. [Google Scholar] [CrossRef]
- Mitu, M.; Razus, D.; Giurcan, V.; Oancea, D. Normal burning velocity and propagation speed of ethane-air: Pressure and temperature dependence. Fuel 2015, 147, 27–34. [Google Scholar] [CrossRef]
- Cai, Y.; Jiang, X.; Wang, S.; Liang, J.; Zhang, P. Experimental study on explosion overpressure and flame propagation characteristics of simulated vertical dome oil tank. J. Loss. Prevent. Proc. 2022, 76, 104752. [Google Scholar] [CrossRef]
- Clanet, C.; Searby, G. On the “tulip flame” phenomenon. Combust. Flame 1996, 105, 1. [Google Scholar] [CrossRef]
- Qi, S.; Du, Y.; Zhang, P.; Li, G.; Wang, S.; Li, Y.; Dong, T. Experimental study of gasoline vapor deflagration in a duct with an open end. Combust. Flame 2018, 193, 16–24. [Google Scholar] [CrossRef]
- Li, J.; Jiang, X.; Li, J.; Yu, B.; Zhang, L.; Zhao, Y. Study on influence of length-diameter ratio on explosion characteristics and flame propagation laws of gasoline-air mixture in pipeline. J. Saf. Sci. Technol. 2020, 16, 88–94. [Google Scholar]
- Guo, J.; Li, J.; Tang, Z.; Wang, X.; Zhuang, Y.; Zhang, S.; Duan, Z.; Li, C. Flame propagation in gasoline vapor-air mixtures with concentration gradient in a closed duct. Fuel 2020, 270, 117508. [Google Scholar] [CrossRef]
- Wang, S.; Yan, Z.; Li, X.; Li, G.; Guo, H.; Wu, D. The venting explosion process of premixed fuel vapour and air in a half-open vessel: An analysis of the overpressure dynamic process and flame evolution behaviour. Fuel 2020, 268, 117385. [Google Scholar] [CrossRef]






| δ | 1.40% | 1.50% | 1.60% | 1.70% | 1.80% | 1.90% | 2.00% |
|---|---|---|---|---|---|---|---|
| pmax (kPa) | 385.0713 | 415.2840 | 433.3561 | 442.9971 | 433.8940 | 407.9196 | 394.4552 |
| tmax (ms) | 357.0 | 318.6 | 295.2 | 275.4 | 279.4 | 284.0 | 289.6 |
| uave (MPa/s) | 1.0786 | 1.3035 | 1.4680 | 1.6086 | 1.5530 | 1.4363 | 1.3621 |
| EPI (MPa2/s) | 0.4154 | 0.5413 | 0.6362 | 0.7126 | 0.6738 | 0.5859 | 0.5373 |
| Δ | 1.40% | 1.50% | 1.60% | 1.70% | 1.80% | 1.90% | 2.00% |
|---|---|---|---|---|---|---|---|
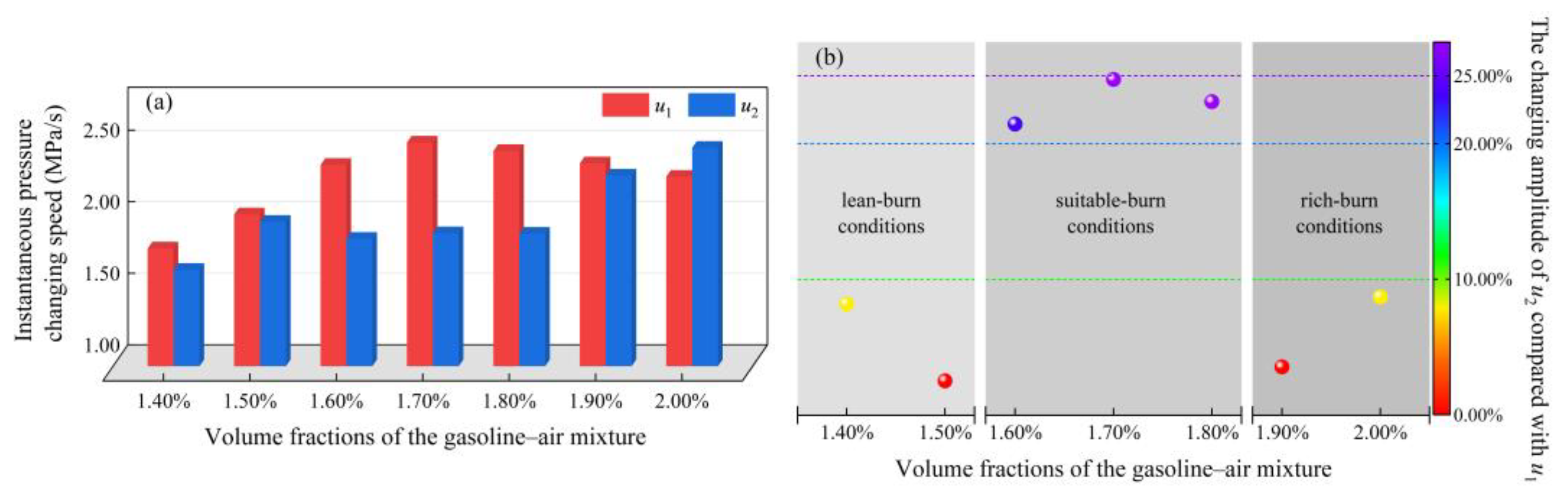
| u1 (Mpa/s) | 1.8230 | 2.0608 | 2.4069 | 2.5630 | 2.5043 | 2.4178 | 2.3236 |
| u2 (Mpa/s) | 1.6737 | 2.0086 | 1.8906 | 1.9289 | 1.9257 | 2.3318 | 2.5260 |
| u3 (Mpa/s) | −1.0624 | −1.3415 | −1.5764 | −1.7081 | −1.5994 | −1.6930 | −1.8534 |
| θ | 8.1920% | 2.5325% | 21.4509% | 24.7399% | 23.1066% | 3.5551% | 8.7096% |
| δ | α1 | α2 | α3 | β0 | β1 | β2 | β3 | βe | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| t1 1 | u1 2 | t2 1 | u2 2 | t3 1 | u3 2 | t0 1 | p0 3 | t1 1 | p1 3 | t2 1 | p2 3 | t3 1 | p3 3 | te 1 | pe 3 | |
| 1.40% | 116.0 | 1.82 | 271.2 | 1.67 | 423.8 | −1.06 | 0.0 | 0.00 | 116.0 | 73.66 | 271.2 | 311.81 | 423.8 | 336.71 | 2585.1 | 20.00 |
| 1.50% | 110.5 | 2.06 | 268.4 | 2.01 | 368.6 | −1.34 | 0.0 | 0.00 | 110.5 | 78.38 | 268.4 | 357.41 | 368.6 | 369.51 | 2484.9 | 20.00 |
| 1.60% | 109.2 | 2.41 | 263.4 | 1.89 | 339.2 | −1.58 | 0.0 | 0.00 | 109.2 | 85.91 | 263.4 | 396.47 | 339.2 | 390.25 | 2420.3 | 20.00 |
| 1.70% | 100.5 | 2.56 | 239.9 | 1.93 | 311.7 | −1.71 | 0.0 | 0.00 | 100.5 | 88.29 | 239.9 | 393.07 | 311.7 | 401.42 | 2457.1 | 20.00 |
| 1.80% | 102.6 | 2.50 | 253.7 | 1.93 | 325.6 | −1.60 | 0.0 | 0.00 | 102.6 | 87.15 | 253.7 | 402.52 | 325.6 | 388.12 | 2426.4 | 20.00 |
| 1.90% | 104.7 | 2.42 | 258.7 | 2.33 | 321.7 | −1.69 | 0.0 | 0.00 | 104.7 | 86.07 | 258.7 | 370.29 | 321.7 | 363.58 | 2581.1 | 20.00 |
| 2.00% | 109.7 | 2.32 | 261.7 | 2.53 | 325.1 | −1.85 | 0.0 | 0.00 | 109.7 | 83.84 | 261.7 | 354.94 | 325.1 | 353.10 | 2577.2 | 20.00 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jiang, X.; Chen, R.; Zhang, P.; Cai, Y.; Zhou, D.; He, D.; Qin, X.; Zhu, S. Study on Gasoline–Air Mixture Explosion Overpressure Characteristics and Flame Propagation Behaviors in an Annular Cylindrical Confined Space with a Circular Arch. Energies 2023, 16, 6944. https://doi.org/10.3390/en16196944
Jiang X, Chen R, Zhang P, Cai Y, Zhou D, He D, Qin X, Zhu S. Study on Gasoline–Air Mixture Explosion Overpressure Characteristics and Flame Propagation Behaviors in an Annular Cylindrical Confined Space with a Circular Arch. Energies. 2023; 16(19):6944. https://doi.org/10.3390/en16196944
Chicago/Turabian StyleJiang, Xinsheng, Ri Chen, Peili Zhang, Yunxiong Cai, Dongliang Zhou, Donghai He, Xizhuo Qin, and Shijie Zhu. 2023. "Study on Gasoline–Air Mixture Explosion Overpressure Characteristics and Flame Propagation Behaviors in an Annular Cylindrical Confined Space with a Circular Arch" Energies 16, no. 19: 6944. https://doi.org/10.3390/en16196944
APA StyleJiang, X., Chen, R., Zhang, P., Cai, Y., Zhou, D., He, D., Qin, X., & Zhu, S. (2023). Study on Gasoline–Air Mixture Explosion Overpressure Characteristics and Flame Propagation Behaviors in an Annular Cylindrical Confined Space with a Circular Arch. Energies, 16(19), 6944. https://doi.org/10.3390/en16196944






