Analytical Model of Critical Ventilation Flow Rate for Accidental Hydrogen Leakage in a Confined Space
Abstract
:1. Introduction
2. Research Methods
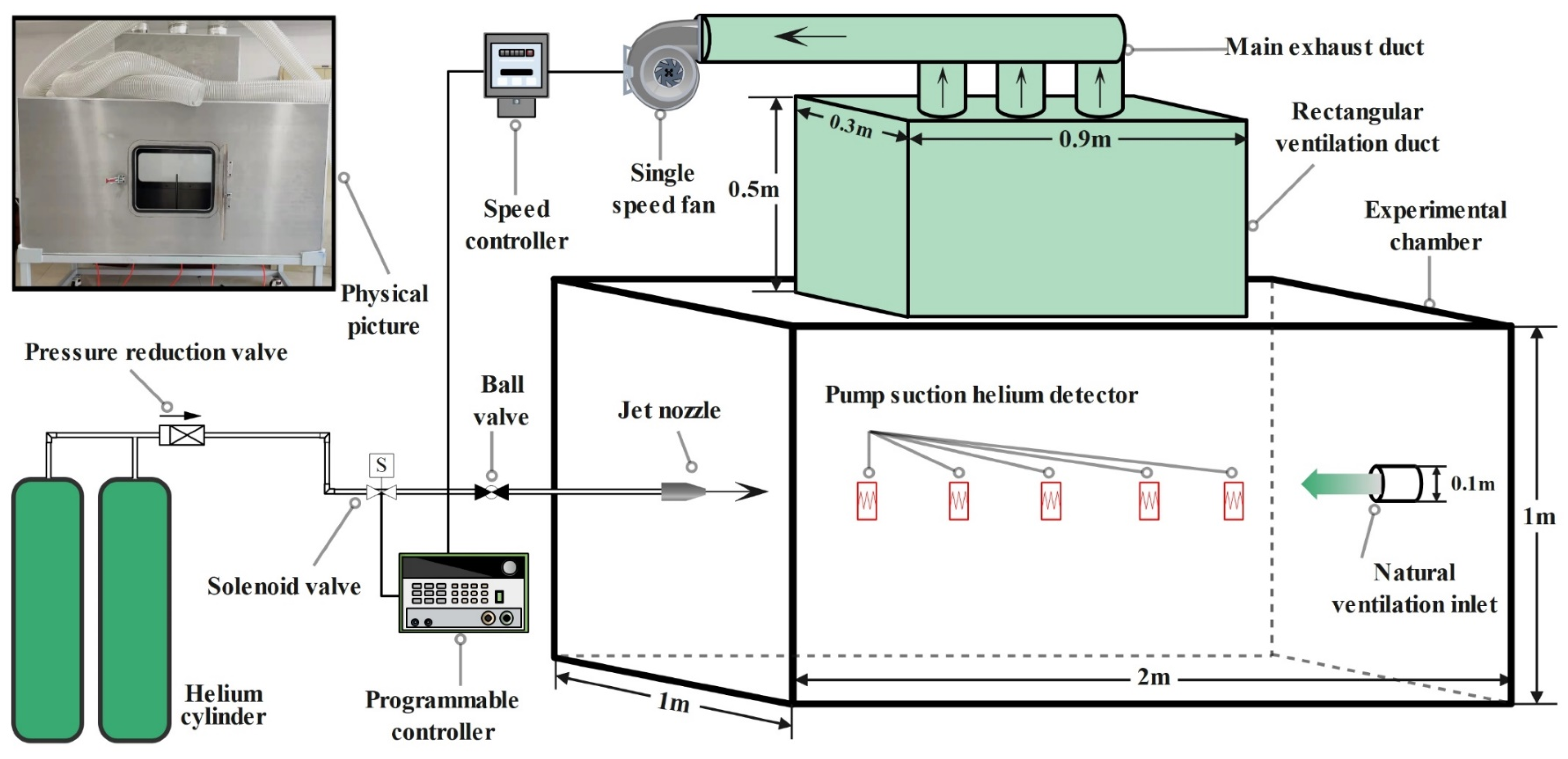
Experimental Equipment and Procedures
3. Results and Discussion
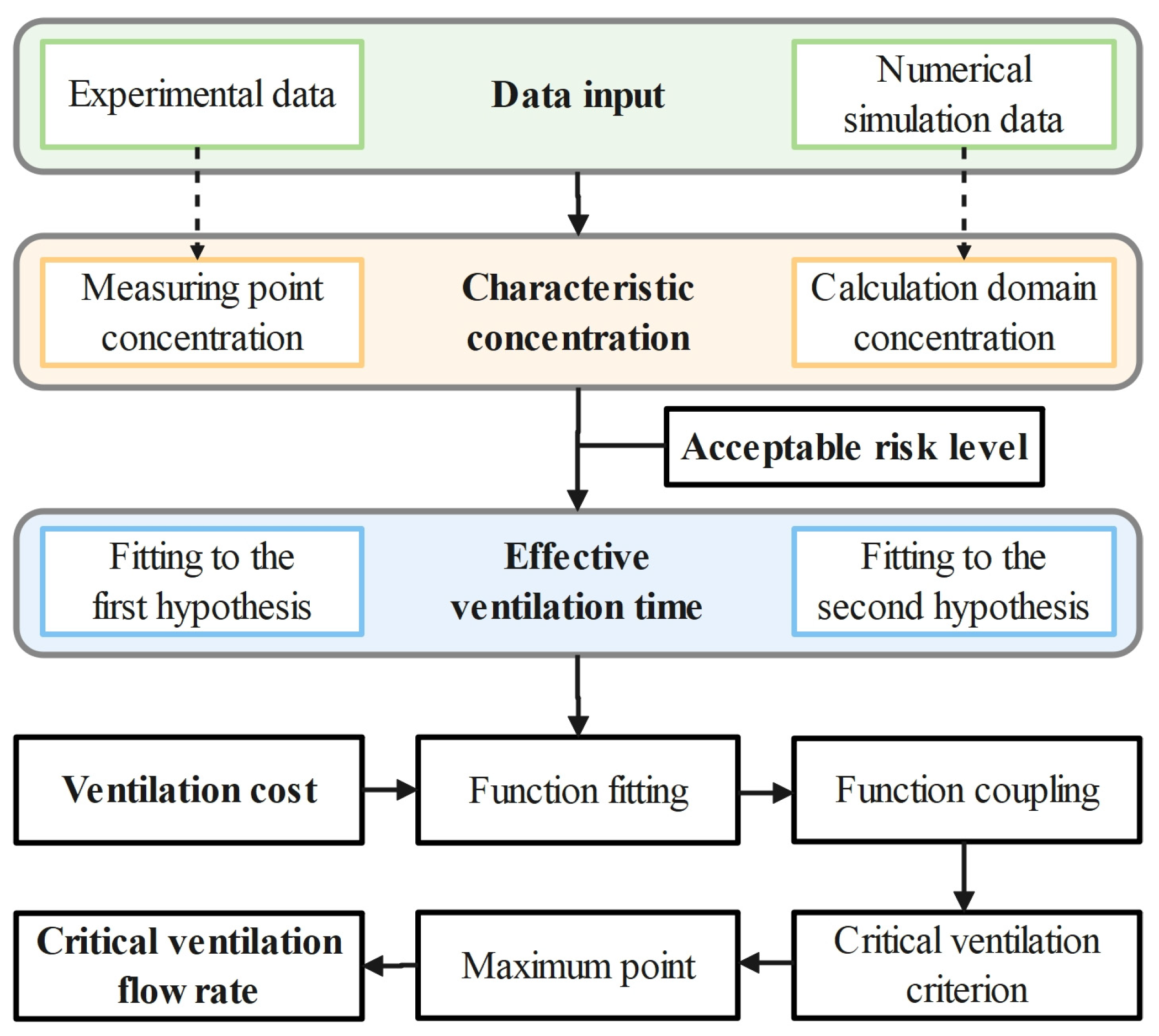
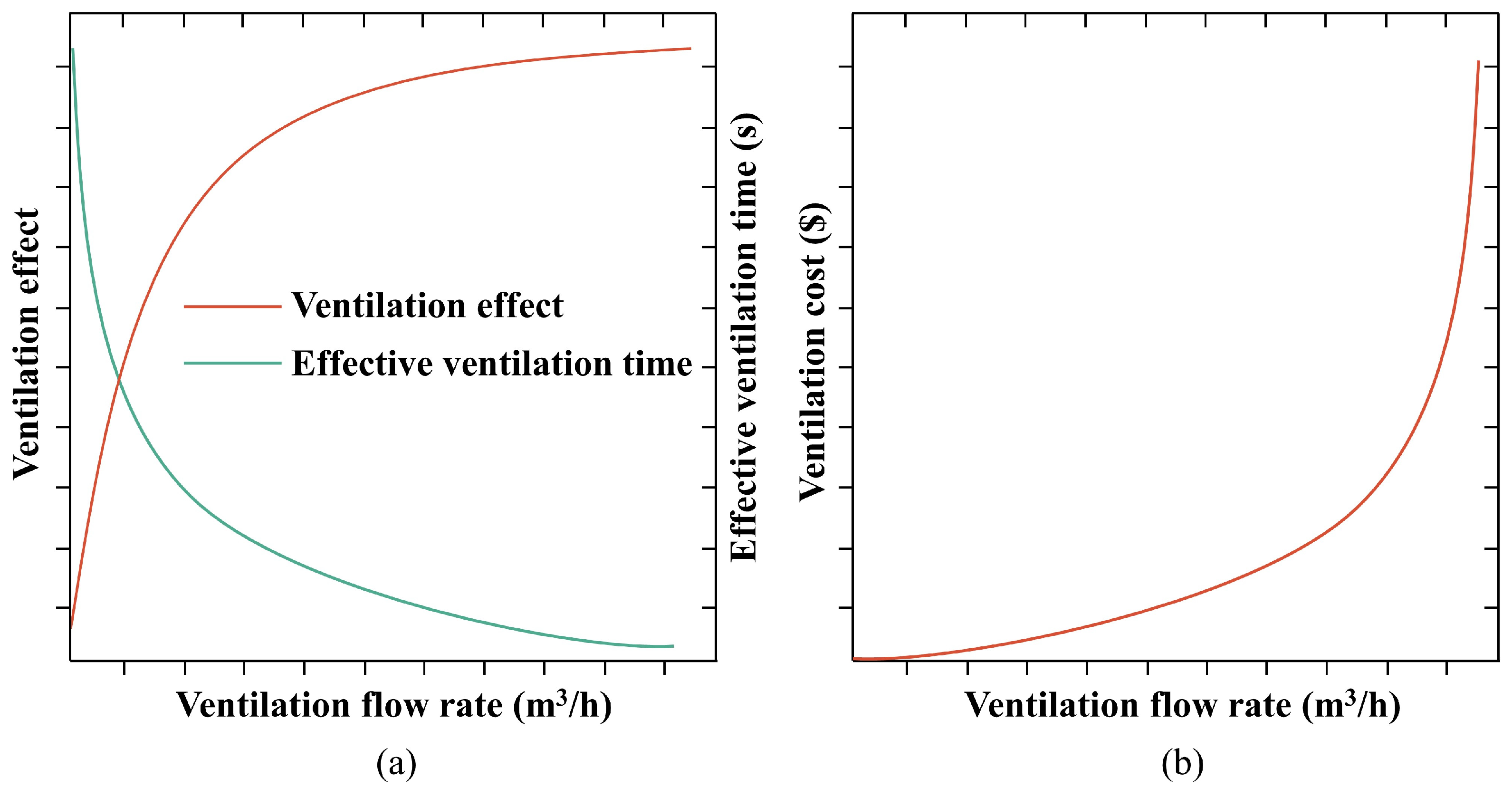
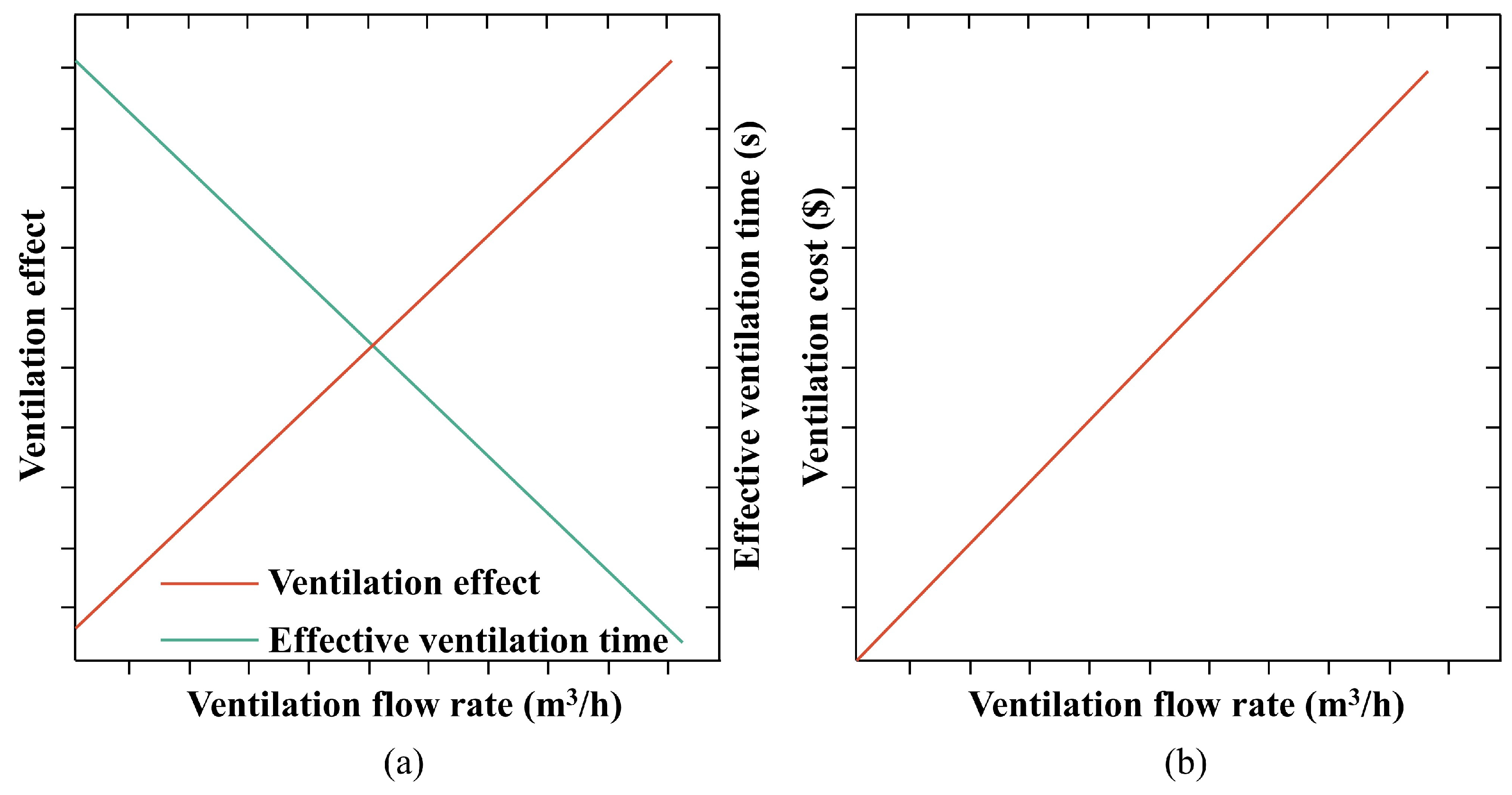
3.1. Analytical Model of the Critical Ventilation Flow Rate
3.2. Model Verification and Case Solution
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| intercept in Equation (4) [-] | maximum ventilation flow rate [m3/h] | ||
| dimensionless ventilation cost [-] | dimensionless ventilation flow rate [-] | ||
| slope in Equation (4) [-] | critical ventilation flow rate [m3/h] | ||
| slope in Equation (5) [-] | coefficient of determination [-] | ||
| critical ventilation criterion [-] | effective ventilation time [s] | ||
| critical ventilation criterion under the first hypothesis [-] | maximum ventilation time [s] | ||
| critical ventilation criterion under the second hypothesis [-] | dimensionless effective ventilation time [-] | ||
| ventilation flow rate [m3/h] | |||
| Greek symbols | |||
| coefficient in Equation (1) [-] | base of the exponential function in Equation (2) [-] | ||
| coefficient in Equation (1) [-] | |||
| Acronyms | |||
| VOL | Volume | ||
References
- Abohamzeh, E.; Salehi, F.; Sheikholeslami, M.; Abbassi, R.; Khan, F. Review of hydrogen safety during storage, transmission, and applications processes. J. Loss Prev. Process Ind. 2021, 72, 104569. [Google Scholar] [CrossRef]
- Klebanoff, L.E.; Pratt, J.W.; LaFleur, C.B. Comparison of the safety-related physical and combustion properties of liquid hydrogen and liquid natural gas in the context of the SF-BREEZE high-speed fuel-cell ferry. Int. J. Hydrog. Energy 2017, 42, 757–774. [Google Scholar] [CrossRef]
- Kozhukhova, A.E.; du Preez, S.P.; Bessarabov, D.G. Catalytic Hydrogen Combustion for Domestic and Safety Applications: A Critical Review of Catalyst Materials and Technologies. Energies. 2021, 14, 4897. [Google Scholar] [CrossRef]
- Usman, M.R. Hydrogen storage methods: Review and current status. Renew. Sustain. Energy Rev. 2022, 167, 112743. [Google Scholar] [CrossRef]
- Cadwallader, L.C.; Herring, J.S. Safety Issues with Hydrogen as a Vehicle Fuel; Idaho National Lab: Idaho Falls, ID, USA, 1999. [Google Scholar]
- Molkov, V. Hydrogen Safety Engineering: The State-of-the-Art and Future Progress; Elsevier: Oxford, UK, 2012. [Google Scholar]
- Nikolaev, A.K.; Romanov, A.V.; Zaripova, N.A.; Fetisov, V.G. Modeling of flow in field pipeline to confirm effectiveness of insertion of splitting couplings in control of rill-washing corrosion. IOP Conf. Ser. Earth Environ. Sci. 2018, 194, 082030. [Google Scholar] [CrossRef]
- Matsuura, K.; Kanayama, H.; Tsukikawa, H.; Inoue, M. Numerical simulation of leaking hydrogen dispersion behavior in a partially open space. Int. J. Hydrog. Energy 2008, 33, 240–247. [Google Scholar] [CrossRef]
- Salva, J.A.; Tapia, E.; Iranzo, A.; Pino, F.J.; Cabrera, J.; Rosa, F. Safety study of a hydrogen leak in a fuel cell vehicle using computational fluid dynamics. Int. J. Hydrog. Energy 2012, 37, 5299–5306. [Google Scholar] [CrossRef]
- Hajji, Y.; Bouteraa, M.; El Cafsi, A.; Belghith, A.; Bournot, P.; Kallel, F. Dispersion and behavior of hydrogen during a leak in a prismatic cavity. Int. J. Hydrog. Energy 2014, 39, 6111–6119. [Google Scholar] [CrossRef]
- Hajji, Y.; Bouteraa, M.; Elcafsi, A.; Belghith, A.; Bournot, P.; Kallel, F. Natural ventilation of hydrogen during a leak in a residential garage. Renew. Sustain. Energy Rev. 2015, 50, 810–818. [Google Scholar] [CrossRef]
- Lee, J.; Cho, S.; Park, C.; Cho, H.; Moon, I. Numerical analysis of hydrogen ventilation in a confined facility with various opening sizes, positions and leak quantities. In Proceedings of the 27th European Symposium on Computer-Aided Process Engineering (ESCAPE), 40A, Barcelona, Spain, 1–5 October 2017; Elsevier Science: Amsterdam, The Netherlands, 2017; pp. 559–564. [Google Scholar]
- Zhang, X.; Wang, Q.; Hou, X.; Li, Y.; Miao, Y.; Li, K.; Zhang, L. Effect of the position and the area of the vent on the hydrogen dispersion in a naturally ventilated cubiod space with one vent on the side wall. Int. J. Hydrog. Energy 2022, 47, 9071–9081. [Google Scholar] [CrossRef]
- Ryu, B.R.; Duong, P.A.; Kim, J.B.; Choi, S.Y.; Shin, J.W.; Jung, J.; Kang, H. The Effect of Ventilation on the Hazards of Hydrogen Release in Enclosed Areas of Hydrogen-Fueled Ship. J. Mar. Sci. Eng. 2023, 11, 1639. [Google Scholar] [CrossRef]
- Brennan, S.; Bengaouer, A.; Carcassi, M.; Cerchiara, G.; Evans, G.; Friedrich, A.; Gentilhomme, O.; Houf, W.; Kotchurko, A.; Kotchourko, N.; et al. Towards Minimising Hazards in Hydrogen and Fuel Cell Stationary Applications: Key Findings of Modelling and Experimental Work in the Hyper Project. In Proceedings of the 21st Hazards Symposium—Process Safety and Environmental Protection, Manchester, UK, 10–12 November 2009; Inst Chemical Engineers. pp. 399–410. [Google Scholar]
- Matsuura, K.; Nakano, M.; Ishimoto, J. Sensing-based risk mitigation control of hydrogen dispersion and accumulation in a partially open space with low-height openings by forced ventilation. Int. J. Hydrog. Energy 2012, 37, 1972–1984. [Google Scholar] [CrossRef]
- Dadashzadeh, M.; Ahmad, A.; Khan, F. Dispersion modelling and analysis of hydrogen fuel gas released in an enclosed area: A CFD-based approach. Fuel 2016, 184, 192–201. [Google Scholar] [CrossRef]
- Lee, J.; Cho, S.; Cho, H.; Cho, S.; Lee, I.; Moon, I.; Kim, J. CFD modeling on natural and forced ventilation during hydrogen leaks in a pressure regulator process of a residential area. Process Saf. Environ. Prot. 2022, 161, 436–446. [Google Scholar] [CrossRef]
- Malakhov, A.A.; Avdeenkov, A.V.; du Toit, M.H.; Bessarabov, D.G. CFD simulation and experimental study of a hydrogen leak in a semi-closed space with the purpose of risk mitigation. Int. J. Hydrog. Energy 2020, 45, 9231–9240. [Google Scholar] [CrossRef]
- Brady, K.; Sung, C.J.; T′Ien, J. Dispersion and catalytic ignition of hydrogen leaks within enclosed spaces. Int. J. Hydrog. Energy 2012, 37, 10405–10415. [Google Scholar] [CrossRef]
- Hajji, Y.; Bouteraa, M.; Elcafsi, A.; Bournot, P. Green hydrogen leaking accidentally from a motor vehicle in confined space: A study on the effectiveness of a ventilation system. Int. J. Energy Res. 2021, 45, 18935–18943. [Google Scholar] [CrossRef]
- Papanikolaou, E.; Venetsanos, A.G.; Cerchiara, G.M.; Carcassi, M.; Markatos, N. CFD simulations on small hydrogen releases inside a ventilated facility and assessment of ventilation efficiency. Int. J. Hydrog. Energy 2011, 36, 2597–2605. [Google Scholar] [CrossRef]
- Matsuura, K.; Nakano, M.; Ishimoto, J. The sensing-based adaptive risk mitigation of leaking hydrogen in a partially open space. Int. J. Hydrog. Energy 2009, 34, 8770–8782. [Google Scholar] [CrossRef]
- Huang, T.; Zhao, M.B.; Ba, Q.X.; Christopher, D.M.; Li, X.F. Modeling of hydrogen dispersion from hydrogen fuel cell vehicles in an underground parking garage. Int. J. Hydrog. Energy 2022, 47, 686–696. [Google Scholar] [CrossRef]
- Prasad, K.; Yang, J. Vertical release of hydrogen in a partially enclosed compartment: Role of wind and buoyancy. Int. J. Hydrog. Energy 2011, 36, 1094–1106. [Google Scholar] [CrossRef]
- Brennan, S.; Bengaouer, A.; Carcassi, M.; Cerchiara, G.; Evans, G.; Friedrich, A.; Gentilhomme, O.; Houf, W.; Kotchourko, A.; Kotchourko, N.; et al. Hydrogen and fuel cell stationary applications: Key findings of modelling and experimental work in the HYPER project. Int. J. Hydrog. Energy 2011, 36, 2711–2720. [Google Scholar] [CrossRef]
- Mylonopoulos, F.; Boulougouris, E.; Trivyza, N.L.; Priftis, A.; Cheliotis, M.; Wang, H.; Shi, G. Hydrogen vs. Batteries: Comparative Safety Assessments for a High-Speed Passenger Ferry. Appl. Sci. 2022, 12, 24. [Google Scholar] [CrossRef]
- Ekoto, I.W.; Houf, W.G.; Evans, G.H.; Merilo, E.G.; Groethe, M.A. Experimental investigation of hydrogen release and ignition from fuel cell powered forklifts in enclosed spaces. Int. J. Hydrog. Energy 2012, 37, 17446–17456. [Google Scholar] [CrossRef]
- Prasad, K.; Pitts, W.; Yang, J.A. Effect of wind and buoyancy on hydrogen release and dispersion in a compartment with vents at multiple levels. Int. J. Hydrog. Energy 2010, 35, 9218–9231. [Google Scholar] [CrossRef]
- Prasad, K. High-pressure release and dispersion of hydrogen in a partially enclosed compartment: Effect of natural and forced ventilation. Int. J. Hydrog. Energy 2014, 39, 6518–6532. [Google Scholar] [CrossRef]
- Matsuura, K. Effects of the geometrical configuration of a ventilation system on leaking hydrogen dispersion and accumulation. Int. J. Hydrog. Energy 2009, 34, 9869–9878. [Google Scholar] [CrossRef]
- Xie, H.; Li, X.F.; Christopher, D.M. Emergency blower ventilation to disperse hydrogen leaking from a hydrogen-fueled vehicle. Int. J. Hydrog. Energy 2015, 40, 8230–8238. [Google Scholar] [CrossRef]
- Gitushi, K.M.; Blaylock, M.L.; Klebanoff, L.E. Hydrogen gas dispersion studies for hydrogen fuel cell vessels II: Fuel cell room releases and the influence of ventilation. Int. J. Hydrog. Energy 2022, 47, 21492–21505. [Google Scholar] [CrossRef]
- Matsuura, K.; Nakano, M.; Ishimoto, J. Acceleration of hydrogen forced ventilation after leakage ceases in a partially open space. Int. J. Hydrog. Energy 2012, 37, 7940–7949. [Google Scholar] [CrossRef]
- Matsuura, K.; Nakano, M.; Ishimoto, J. Dynamic restriction mechanism for the upper limit of exhaust flow rates in the real-time sensing-based forced ventilation control of leaking hydrogen. Int. J. Hydrog. Energy 2015, 40, 4401–4411. [Google Scholar] [CrossRef]
- Matsuura, K.; Nakano, M.; Ishimoto, J. Forced ventilation for sensing-based risk mitigation of leaking hydrogen in a partially open space. Int. J. Hydrog. Energy 2010, 35, 4776–4786. [Google Scholar] [CrossRef]
- Schipachev, A.; Fetisov, V.; Nazyrov, A.; Donghee, L.; Khamrakulov, A. Study of the Pipeline in Emergency Operation and Assessing the Magnitude of the Gas Leak. Energies 2022, 15, 5294. [Google Scholar] [CrossRef]
- Nikolaev, A.K.; Samigullin, G.H.; Samigullina, L.G.; Fetisov, V.G. Non-stationary operation of gas pipeline based on selections of travel. IOP Conf. Ser. Mater. Sci. Eng. 2018, 327, 022074. [Google Scholar] [CrossRef]

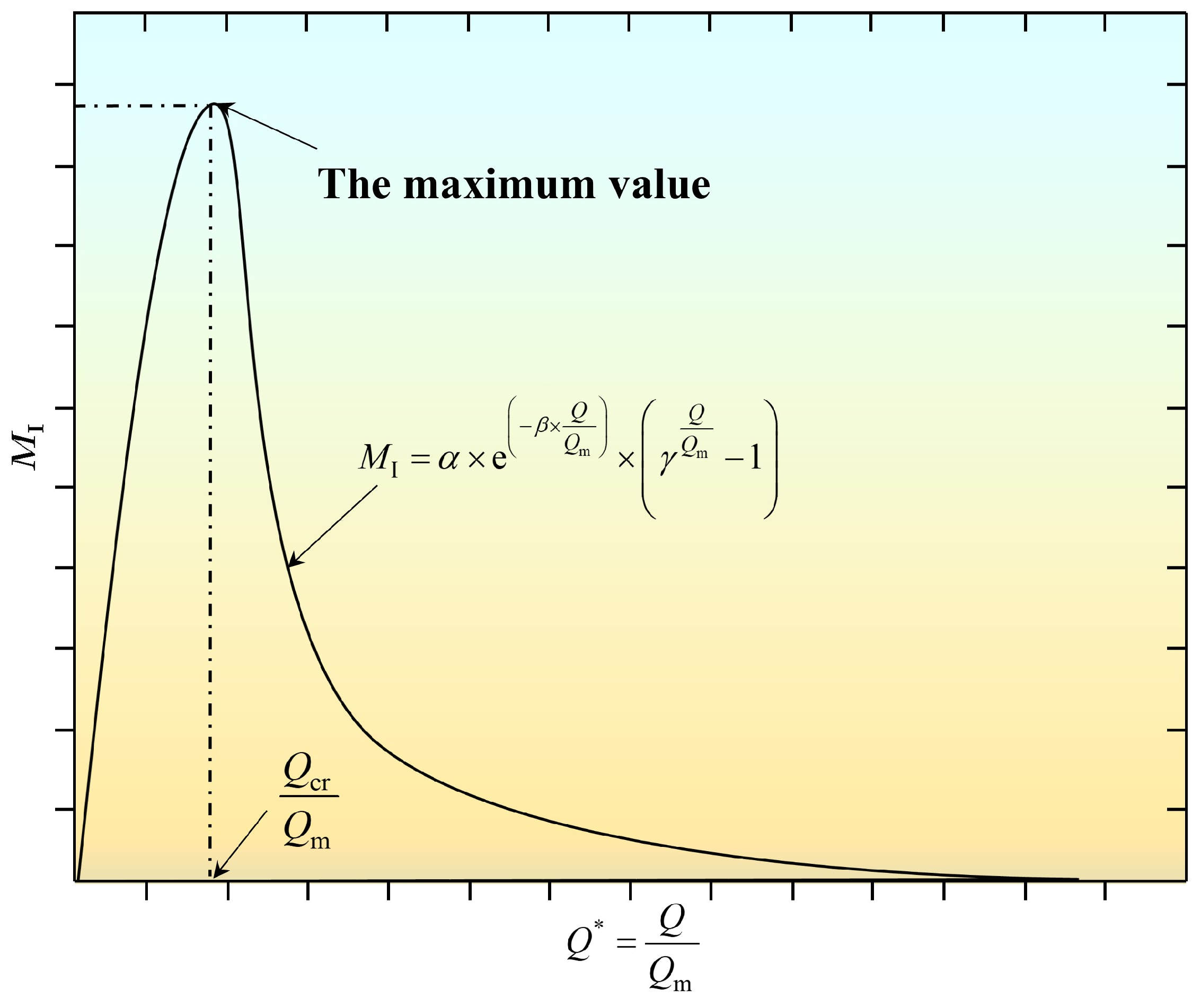
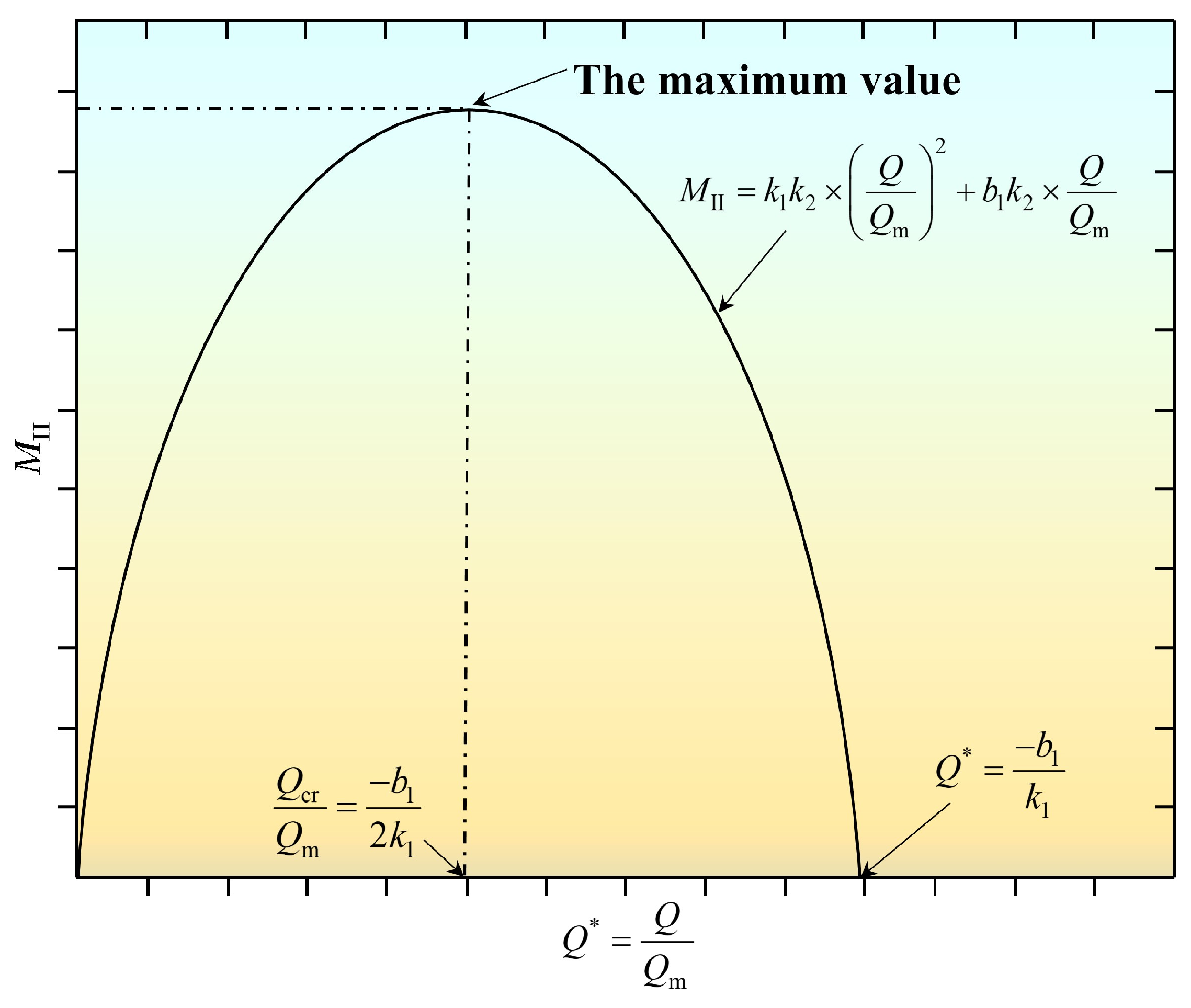
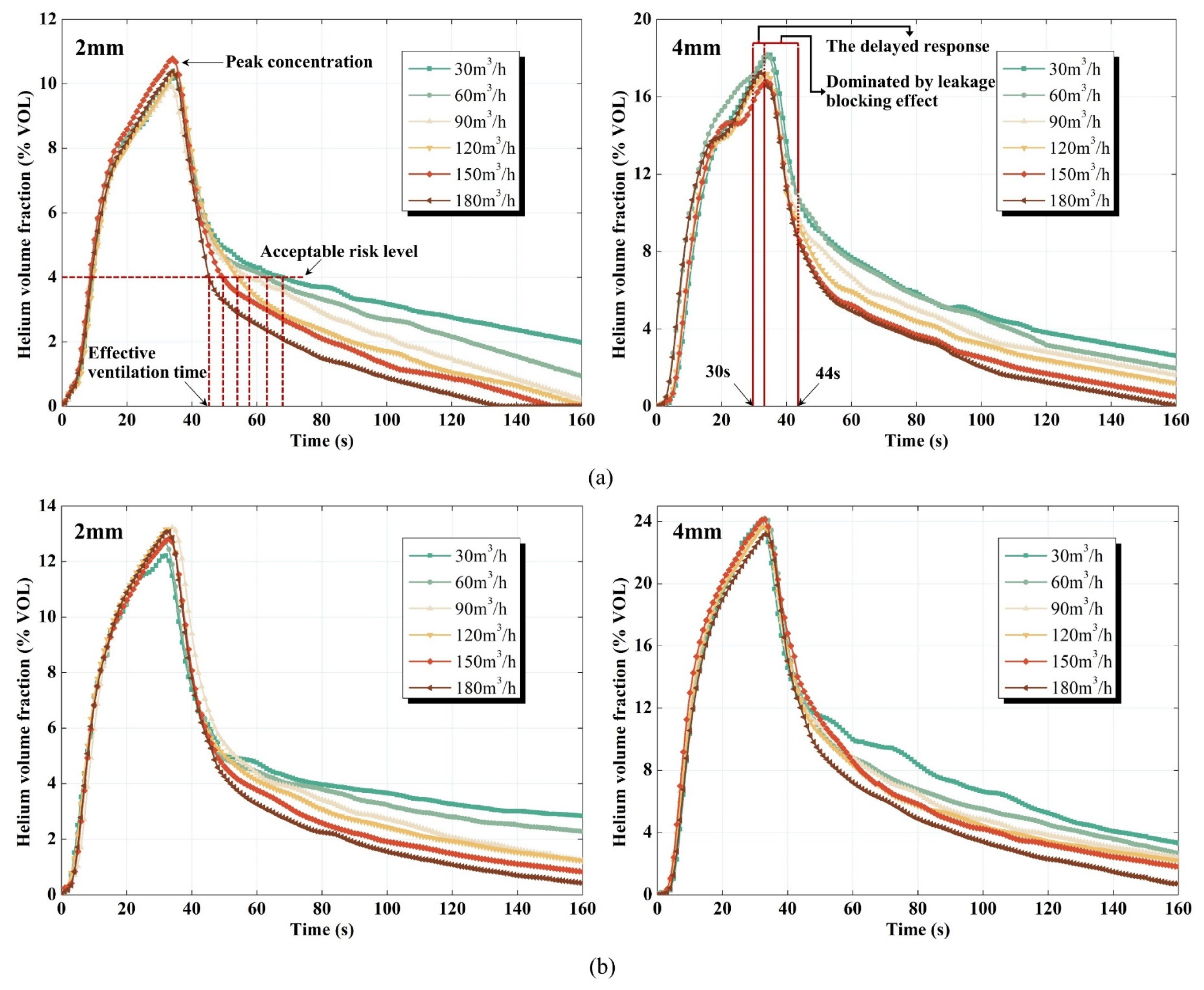
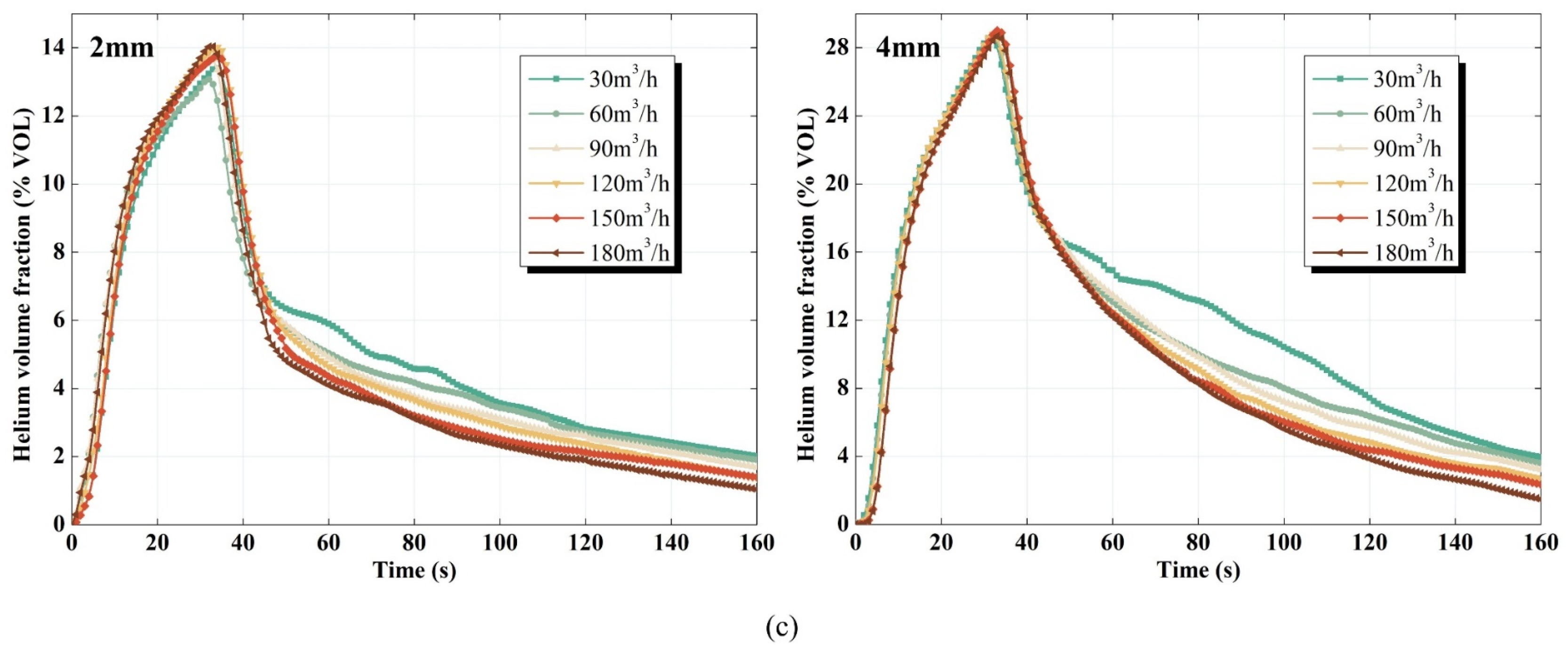
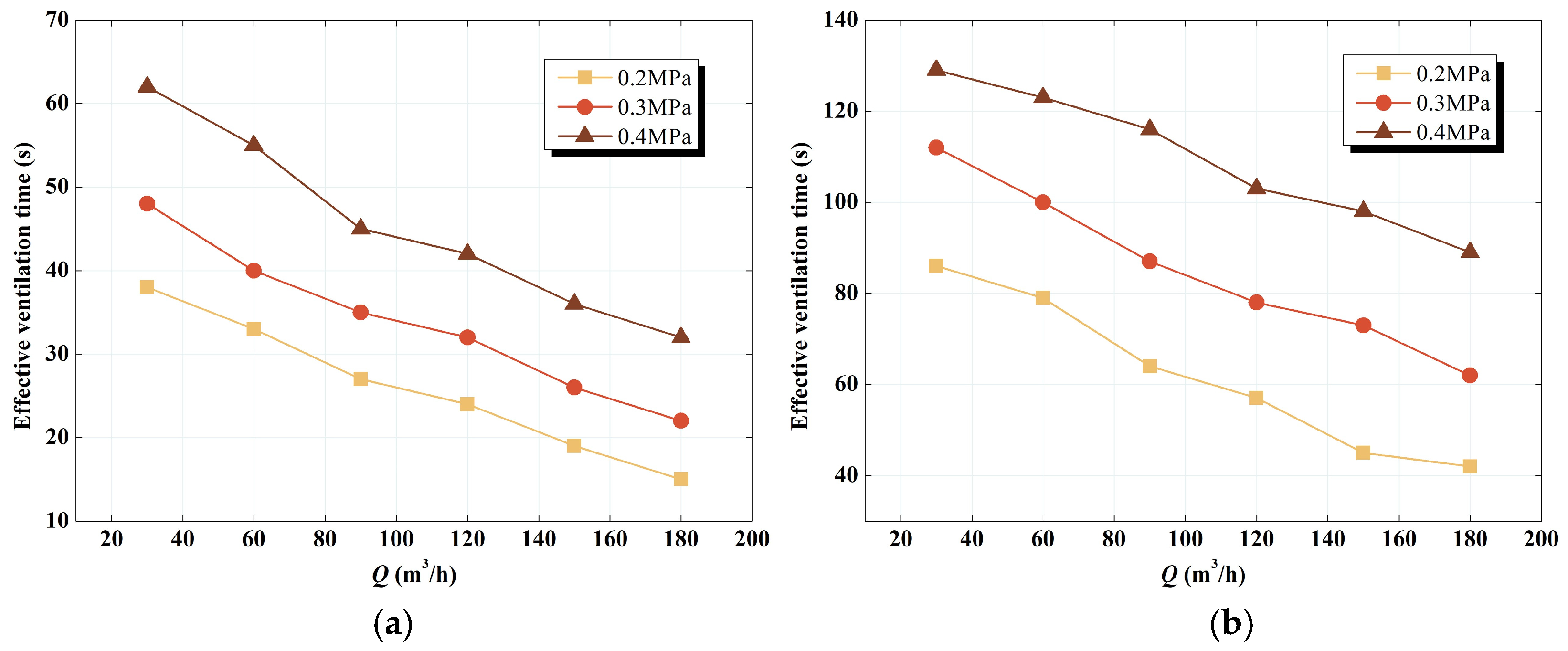
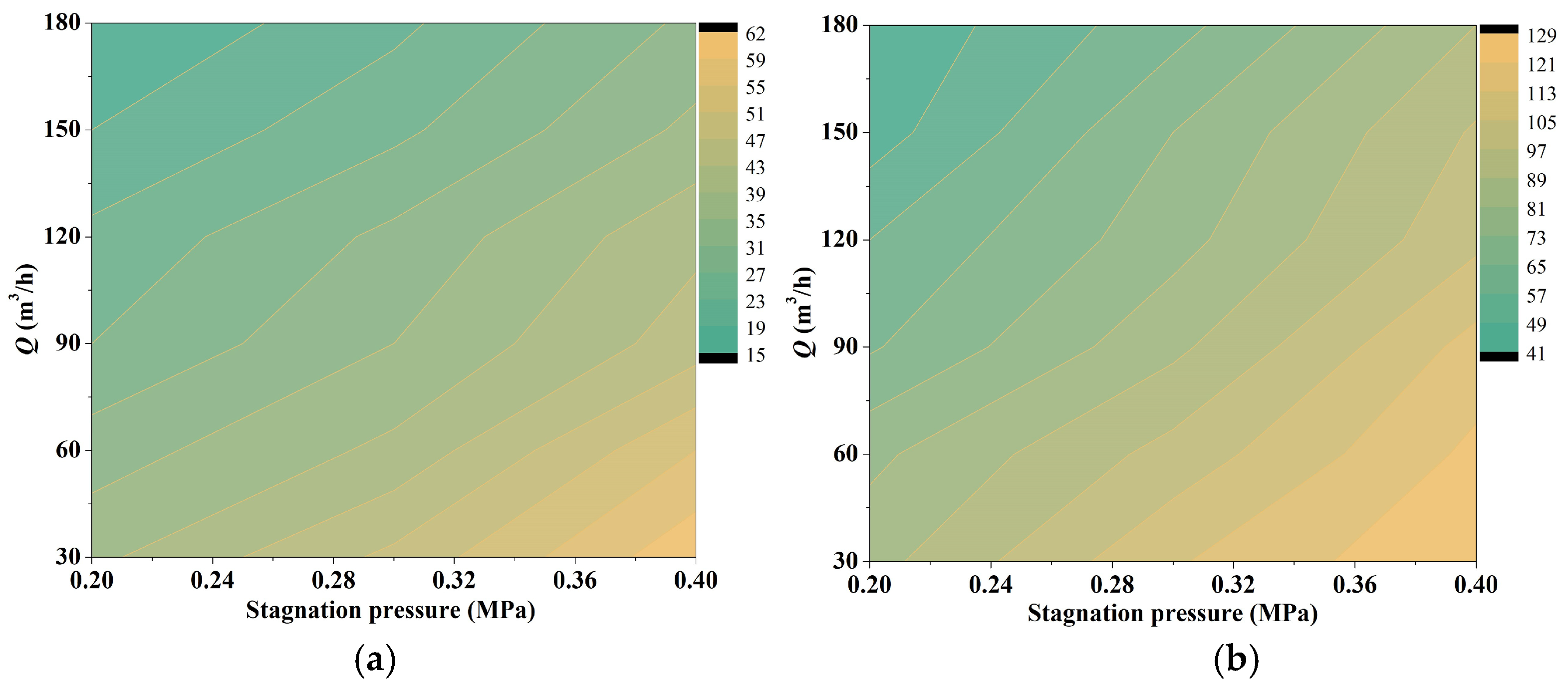

| Ventilation Mode | Author | Research Scenario | Method | Research Topic |
|---|---|---|---|---|
| Natural ventilation | Matsuura K [8] | Partially open hallway | Simulation-CFD-ACE | The effects on the hydrogen concentration distribution of changing vent positions, vent conditions and surrounding atmospheric currents. |
| Salva JA [9] | Hydrogen fuel cell vehicle | Simulation-FLUENT | Risk analysis of hydrogen leakage and diffusion in a vehicle interior by steady state simulation. | |
| Hajji Y [10] | Garage | Simulation-FLUENT | The effects of the roof apex angle and the opening ventilation on hydrogen concentration gradients and stratification in a prismatic residential garage. | |
| Hajji Y [11] | Garage | Simulation-FLUENT | The influence of building geometry and position, the shape and size of openings and ventilation on the formation of combustible hydrogen-air clouds. | |
| Lee J [12] | Partially open space | Simulation-FLUENT & Experimental | Hydrogen release behaviors and the most effective ventilation configuration to reduce hydrogen concentration in a space. | |
| Zhang XL [13] | Partially open space | Simulation-FLUENT | The coupling influence mechanism of the vent position and the vent area on the ventilation effect. | |
| Ryu BR [14] | Enclosed Area | Simulation-FLACS | The impact of ventilation on hydrogen dispersion and concentration within the fuel preparation room. | |
| Forced ventilation and natural ventilation | Brennan S [15] | Hydrogen fuel cells | Simulation-ADREA-HF & Experimental | Ventilation requirements in enclosures containing fuel cells are determined to control hydrogen concentrations in the event of a possible leakage. |
| Matsuura K [16] | Partially open space | Simulation-FDS | A sensing-based risk mitigation control strategy that the volume flow rate of forced ventilation is changed according to the sensing data to achieve the best ventilation effect. | |
| Dadashzadeh M [17] | Garage | Simulation-FDS | Hydrogen diffusion behavior and mitigation measures under different ventilation conditions. | |
| Lee J [18] | Partially open space | Simulation-FLUENT & Experimental | The most effective natural ventilation configuration and the emergency response using nitrogen-forced ventilation. | |
| Malakhov AA [19] | Partially open space | Simulation-STAR-CCM+ & Experimental | The hydrogen distribution and the efficiency of forced ventilation in a partially open space during hydrogen leakage. |
| Nozzle Diameter (mm) | Ventilation Flow Rate (m3/h) | Stagnation Pressure (MPa) | ||
|---|---|---|---|---|
| 2 | 30 | 0.2 | 0.3 | 0.4 |
| 60 | 0.2 | 0.3 | 0.4 | |
| 90 | 0.2 | 0.3 | 0.4 | |
| 120 | 0.2 | 0.3 | 0.4 | |
| 150 | 0.2 | 0.3 | 0.4 | |
| 180 | 0.2 | 0.3 | 0.4 | |
| 4 | 30 | 0.2 | 0.3 | 0.4 |
| 60 | 0.2 | 0.3 | 0.4 | |
| 90 | 0.2 | 0.3 | 0.4 | |
| 120 | 0.2 | 0.3 | 0.4 | |
| 150 | 0.2 | 0.3 | 0.4 | |
| 180 | 0.2 | 0.3 | 0.4 | |
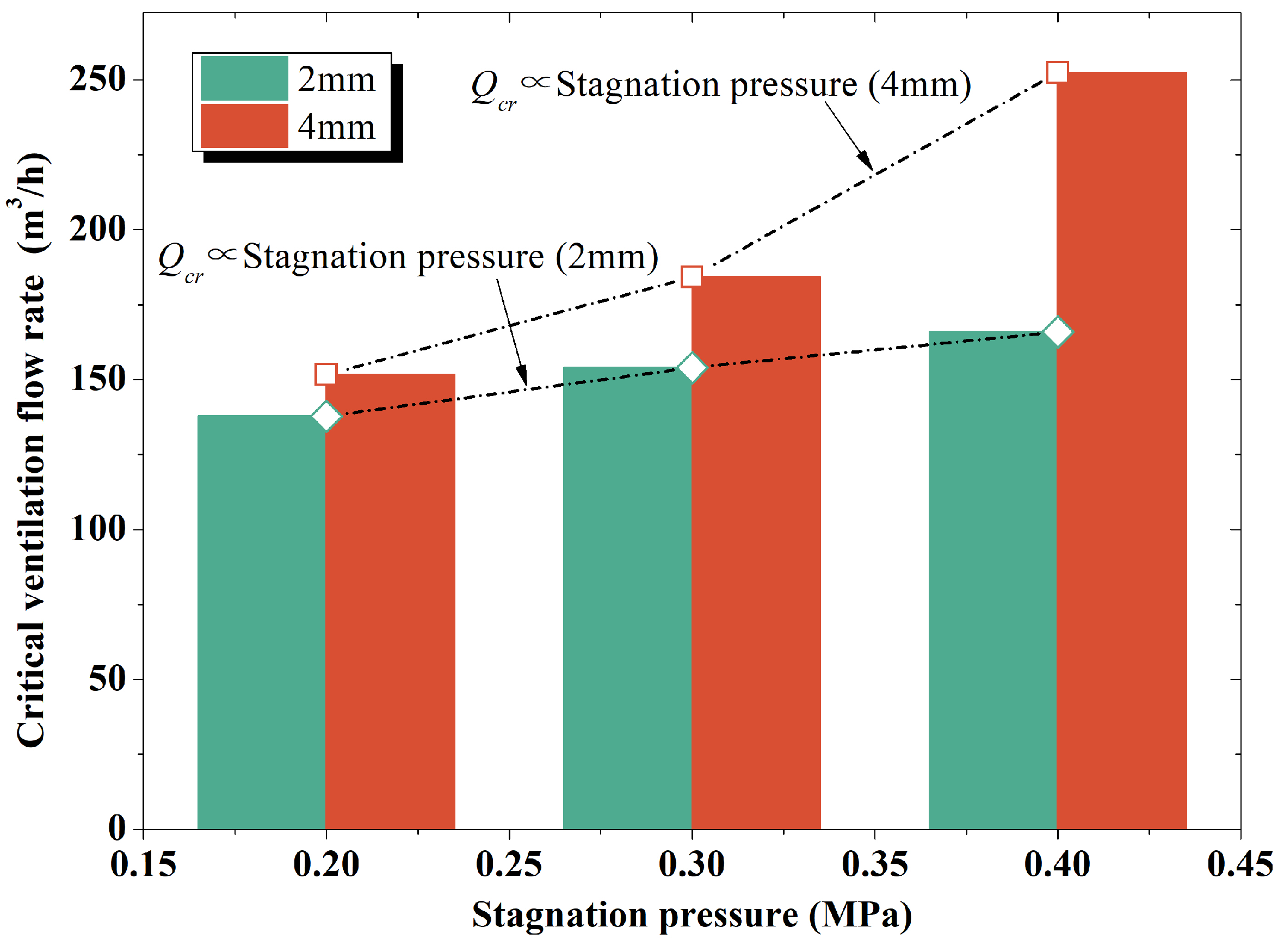
| Nozzle Diameter (mm) | Stagnation Pressure (MPa) | (m3/h) | |||
|---|---|---|---|---|---|
| 2 | 0.2 | −0.21 | 0.32 | 0.99 | 137.8 |
| 2 | 0.3 | −0.23 | 0.39 | 0.98 | 154.0 |
| 2 | 0.4 | −0.28 | 0.51 | 0.97 | 165.9 |
| 4 | 0.2 | −0.43 | 0.73 | 0.98 | 151.7 |
| 4 | 0.3 | −0.45 | 0.92 | 0.98 | 184.2 |
| 4 | 0.4 | −0.38 | 1.07 | 0.99 | 252.4 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sun, X.; Yang, J.; Wang, J.; Chen, X.; Shi, J. Analytical Model of Critical Ventilation Flow Rate for Accidental Hydrogen Leakage in a Confined Space. Energies 2023, 16, 6864. https://doi.org/10.3390/en16196864
Sun X, Yang J, Wang J, Chen X, Shi J. Analytical Model of Critical Ventilation Flow Rate for Accidental Hydrogen Leakage in a Confined Space. Energies. 2023; 16(19):6864. https://doi.org/10.3390/en16196864
Chicago/Turabian StyleSun, Xuxu, Jiale Yang, Jun Wang, Xianfeng Chen, and Jihao Shi. 2023. "Analytical Model of Critical Ventilation Flow Rate for Accidental Hydrogen Leakage in a Confined Space" Energies 16, no. 19: 6864. https://doi.org/10.3390/en16196864
APA StyleSun, X., Yang, J., Wang, J., Chen, X., & Shi, J. (2023). Analytical Model of Critical Ventilation Flow Rate for Accidental Hydrogen Leakage in a Confined Space. Energies, 16(19), 6864. https://doi.org/10.3390/en16196864







