Free Surface Motion of a Liquid Pool with Isothermal Sidewalls as a Benchmark for Marangoni Convection Problems
Abstract
:1. Introduction
2. Problem Setup and Existing Work
2.1. Literature Review
2.2. Definition of the Flow Problem
2.3. Boundary and Initial Conditions
2.4. Governing Equations
2.5. Surface Tension Model
3. Analytical Model
4. CFD
4.1. STAR-CCM+ Setup
4.2. openFOAM Implementation
| Algorithm 1 Calculate Surface Tension Force |
|
5. Results
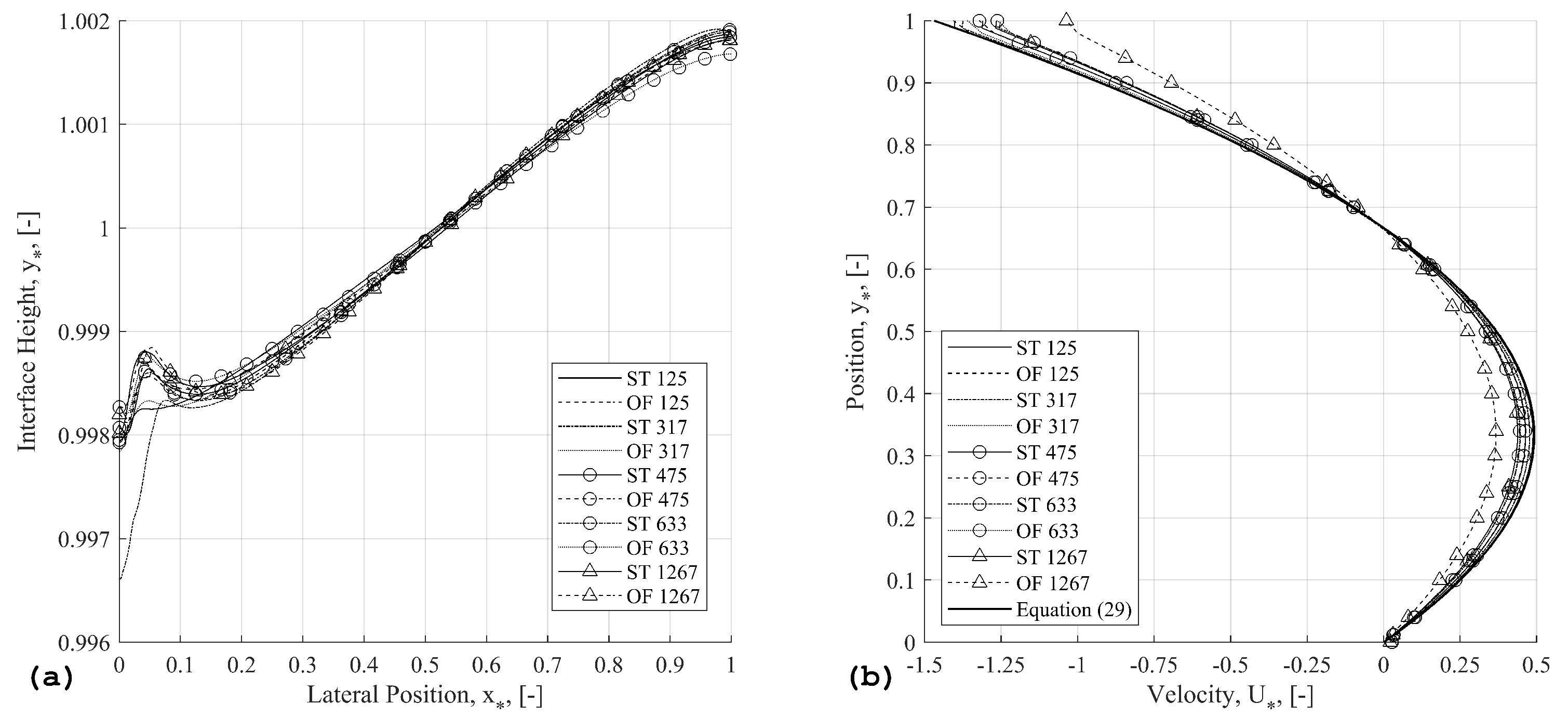
5.1. Effect of Marangoni Number
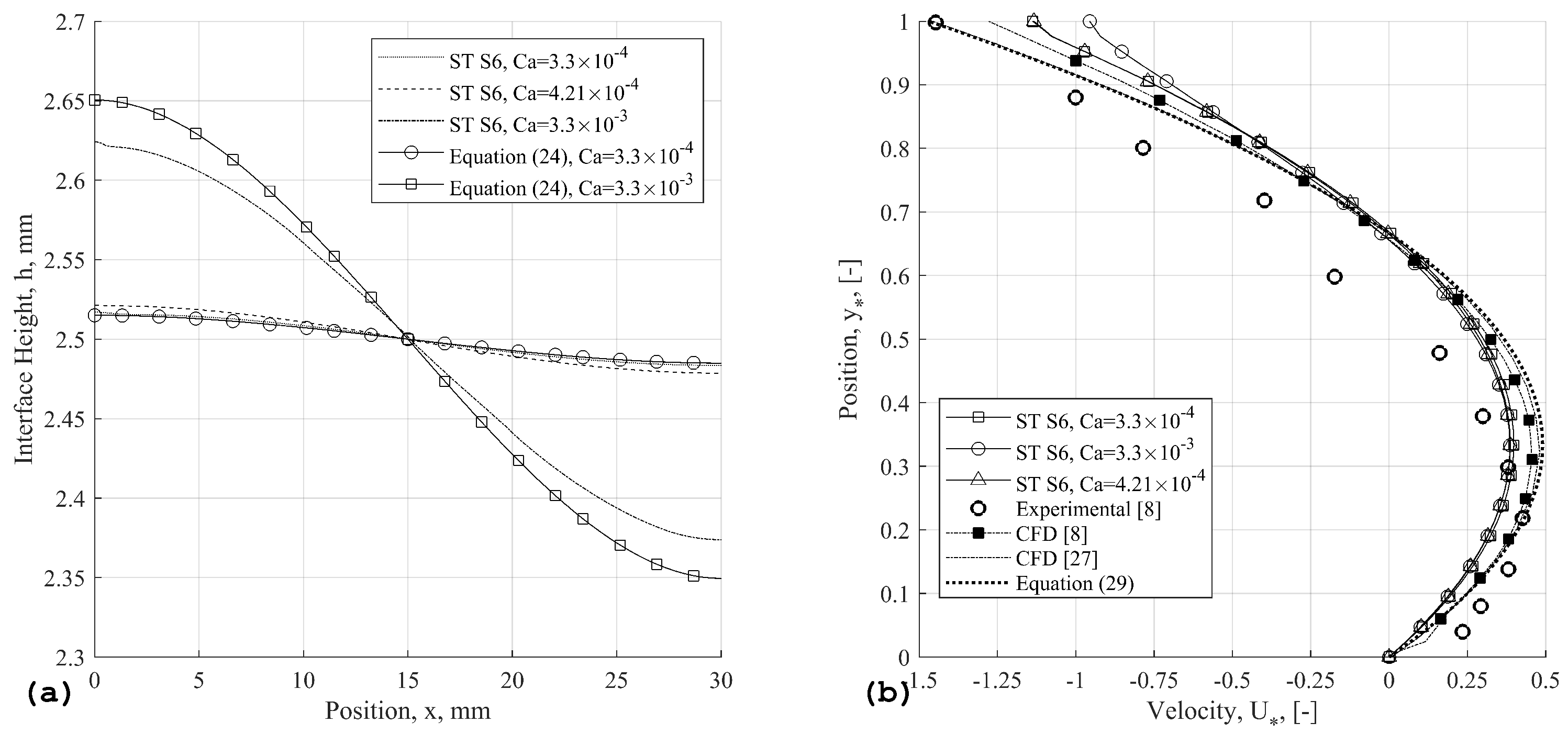
5.2. Effect of Capillary Number
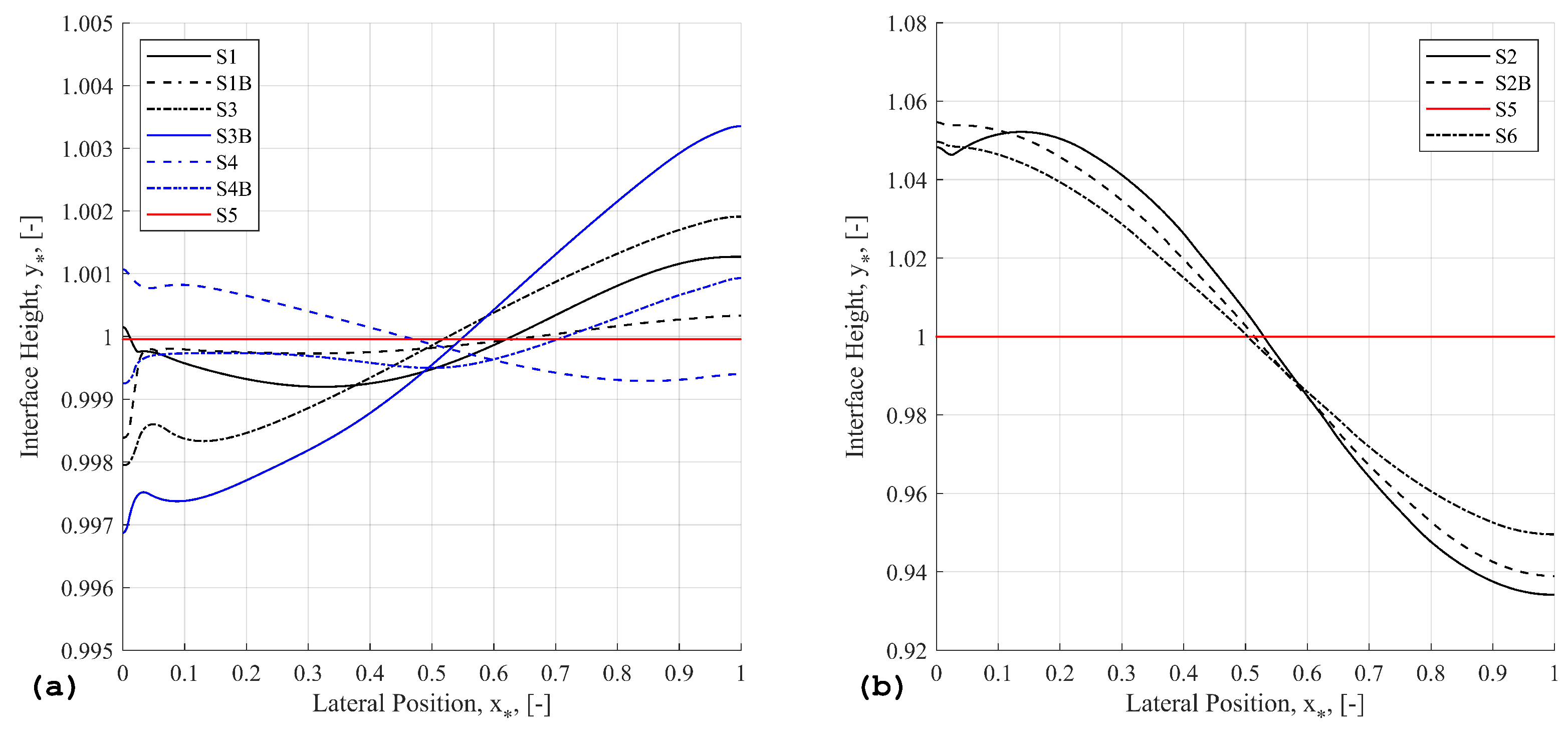
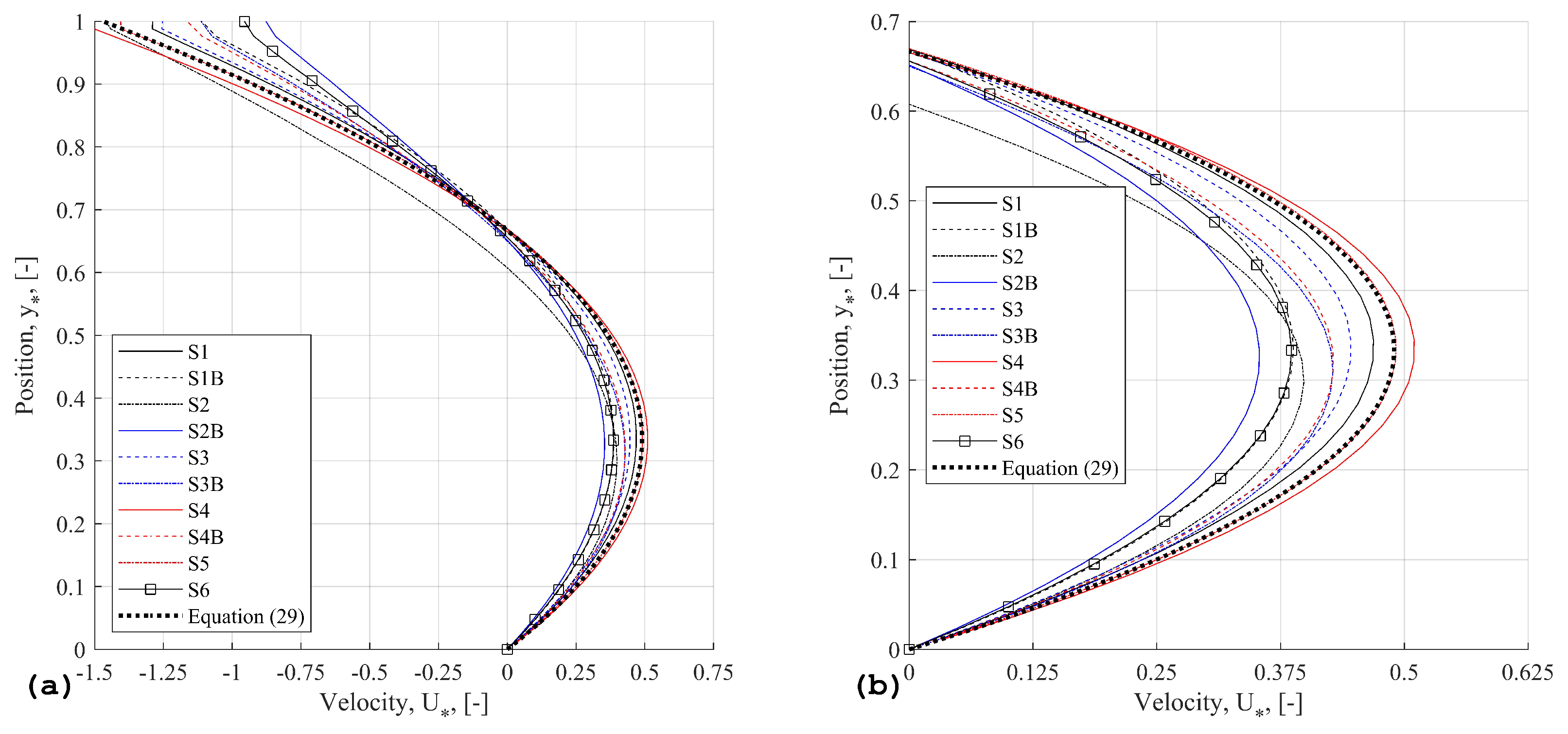
5.3. Effect of Scenario
5.3.1. The Liquid Equation of State
5.3.2. Impact on Velocity Profiles
6. Conclusions
Future Work and Limitations
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Li, C.; Zhao, D.; Wen, J.; Lu, X. Numerical investigation of wafer drying induced by the thermal Marangoni effect. Int. J. Heat Mass Transf. 2019, 132, 689–698. [Google Scholar] [CrossRef]
- Mills, K.C.; Keene, B.J.; Brooks, R.F.; Shirali, A. Marangoni effects in welding. Philos. Trans. R. Soc. London. Ser. Math. Phys. Eng. Sci. 1998, 356, 911–925. [Google Scholar] [CrossRef]
- Schwabe, D.; Scharmann, A. Some evidence for the existence and magnitude of a critical marangoni number for the onset of oscillatory flow in crystal growth melts. J. Cryst. Growth 1979, 46, 125–131. [Google Scholar] [CrossRef]
- Mukherjee, T.; Manvatkar, V.; De, A.; DebRoy, T. Mitigation of thermal distortion during additive manufacturing. Scr. Mater. 2017, 127, 79–83. [Google Scholar] [CrossRef]
- Srimuang, W.; Amatachaya, P. A review of the applications of heat pipe heat exchangers for heat recovery. Renew. Sustain. Energy Rev. 2012, 16, 4303–4315. [Google Scholar] [CrossRef]
- Nguyen, T.T.; Yu, J.; Wayner, P.C.; Plawsky, J.L.; Kundan, A.; Chao, D.F.; Sicker, R.J. Rip currents: A spontaneous heat transfer enhancement mechanism in a wickless heat pipe. Int. J. Heat Mass Transf. 2020, 149, 119–170. [Google Scholar] [CrossRef]
- Sen, A.; Davis, S. Steady thermocapillary flows in two-dimensional slots. J. Fluid Mech. 1982, 121, 163–186. [Google Scholar] [CrossRef]
- Villers, D.; Platten, J. Coupled buoyancy and Marangoni convection in acetone: Experiments and comparison with numerical simulations. J. Fluid Mech. 1992, 234, 487–510. [Google Scholar] [CrossRef]
- Engberg, R.F.; Wegener, M.; Kenig, E.Y. The influence of Marangoni convection on fluid dynamics of oscillating single rising droplets. Chem. Eng. Sci. 2014, 117, 114–124. [Google Scholar] [CrossRef]
- Qin, T.; Tuković, Z.; Grigoriev, R.O. Buoyancy-thermocapillary convection of volatile fluids under their vapors. Int. J. Heat Mass Transf. 2015, 80, 38–49. [Google Scholar] [CrossRef]
- Cao, L. Mesoscopic-scale simulation of pore evolution during laser powder bed fusion process. Comput. Mater. Sci. 2020, 179, 109686. [Google Scholar] [CrossRef]
- Samkhaniani, N.; Stroh, A.; Holzinger, M.; Marschall, H.; Frohnapfel, B.; Wörner, M. Bouncing drop impingement on heated hydrophobic surfaces. Int. J. Heat Mass Transf. 2021, 180, 121777. [Google Scholar] [CrossRef]
- Sabanskis, A.; Virbulis, J. Simulation of the influence of gas flow on melt convection and phase boundaries in FZ silicon single crystal growth. J. Cryst. Growth 2015, 417, 51–57. [Google Scholar] [CrossRef]
- Hadid, H.B.; Roux, B. Thermocapillary convection in long horizontal layers of low-Prandtl-number melts subject to a horizontal temperature gradient. J. Fluid Mech. 1990, 221, 77–103. [Google Scholar] [CrossRef]
- Roux, B. (Ed.) Numerical Simulation of Oscillatory Convection on Low-Pr Fluids; Vieweg+Teubner Verlag: Berlin, Germany, 1990. [Google Scholar] [CrossRef]
- Hadid, H.B.; Roux, B. Buoyancy- and thermocapillary-driven flows in differentially heated cavities for low-Prandtl-number fluids. J. Fluid Mech. 1992, 235, 1. [Google Scholar] [CrossRef]
- Bergman, T.L.; Keller, J.R. Combined Bouyancy, surface tension flow in liquid metals. Numer. Heat Transf. 1988, 13, 49–63. [Google Scholar] [CrossRef]
- Villers, D.; Platten, J. Separation of Marangoni convection from gravitational convection in earth experiments. Physicochem. Hydrodyn. 1987, 8, 173–183. [Google Scholar]
- Sasmal, G.P.; Hochstein, J.I. Marangoni Convection with a Curved and Deforming Free Surface in a Cavity. J. Fluids Eng. 1994, 116, 577–582. [Google Scholar] [CrossRef]
- Kothe, D.; Francois, M.; Sicilian, J. Modeling of thermocapillary forces within a volume tracking algorithm. In Proceedings of the Modeling of Casting, Welding and Advanced Solidification Processes—XI, Opio, France, 28 May–2 June 2006; Volume 2. [Google Scholar]
- Bauer, H.; Eidel, W. Thermo-capillary convection in various infinite rectangular container configurations. Heat Mass Transf. 2003, 40, 123–132. [Google Scholar] [CrossRef]
- Zhou, X.; Huang, H. Numerical Simulation of Steady Thermocapillary Convection in a Two-Layer System Using Level Set Method. Microgravity Sci. Technol. 2010, 22, 223–232. [Google Scholar] [CrossRef]
- Zhou, X.; Huai, X. Numerical Investigation of Thermocapillary Convection in a Liquid Layer with Free Surface. Microgravity Sci. Technol. 2014, 25, 335–341. [Google Scholar] [CrossRef]
- Zhou, X.; Liu, Z.; Huai, X. Evolution of free surface in the formation of thermo-solutocapillary convection within an open cavity. Microgravity Sci. Technol. 2016, 28, 421–430. [Google Scholar] [CrossRef]
- Zhou, X.; Huai, X. Influence of thermal and solutal Marangoni effects on free surface deformation in an open rectangular cavity. J. Therm. Sci. 2016, 26, 255–262. [Google Scholar] [CrossRef]
- Saldi, Z.S. Marangoni Driven Free Surface Flows in Liquid Weld Pools. Ph.D. Thesis, Delft University of Technology, Delft, The Netherlands, 2012. [Google Scholar] [CrossRef]
- Chen, E.; Xu, F. Transient Marangoni convection induced by an isothermal sidewall of a rectangular liquid pool. J. Fluid Mech. 2021, 928, A6. [Google Scholar] [CrossRef]
- Tan, L.H.; Leonardi, E.; Barber, T.J.; Leong, S.S. Experimental and numerical study of Marangoni convection in shallow liquid layers. Int. J. Comput. Fluid Dyn. 2005, 19, 457–473. [Google Scholar] [CrossRef]
- Kamotani, Y.; Ostrach, S.; Pline, A. Analysis of velocity data taken in Surface Tension Driven Convection Experiment in microgravity. Phys. Fluids 1994, 6, 3601–3609. [Google Scholar] [CrossRef]
- Montanero, J.M.; Ferrera, C.; Shevtsova, V.M. Experimental study of the free surface deformation due to thermal convection in liquid bridges. Exp. Fluids 2008, 45, 1087–1101. [Google Scholar] [CrossRef]
- Schwabe, D.; Scharmann, A. Marangoni convection in open boat and crucible. J. Cryst. Growth 1981, 52, 435–449. [Google Scholar] [CrossRef]
- Simcenter STAR-CCM+, version 2206.001; Siemens Digital Industries Software: Plano, TX, USA.
- openFOAM, verson 2106; OpenCFD: Reading, UK.
- Brackbill, J.; Kothe, D.; Zemach, C. A continuum method for modeling surface tension. J. Comput. Phys. 1992, 100, 335–354. [Google Scholar] [CrossRef]
- Simcenter STAR-CCM+ User Guide, version 2206.001; Siemens Digital Industries Software: Plano, TX, USA.
- Scheufler, H.; Roenby, J. TwoPhaseFlow: An OpenFOAM based framework for development of two phase flow solvers. arXiv 2021, arXiv:2103.00870. [Google Scholar]
- Scriven, L. Dynamics of a fluid interface Equation of motion for Newtonian surface fluids. Chem. Eng. Sci. 1960, 12, 98–108. [Google Scholar] [CrossRef]
- Ciccotosto, B. GitHub Repository. cmiFoam with Marangoni. 2022. Available online: https://github.com/brucethemuce/compressibleMultiphaseInterFoam_Marangoni (accessed on 13 July 2022).

| Variable (Unit) | Value | Units |
|---|---|---|
| k | 0.1 | W m−1 K−1 |
| 1 | kg m−3 | |
| 1 | ||
| 299 | ||
| 1/300 | K−1 | |
| 0.01 | N m−2 | |
| 101,325 | ||
| h | 1/12 | |
| L | 1 | |
| g | 0, 9.81 | m s−2 |
| Scenario | Vapor | Liquid | Gravity | Domain |
|---|---|---|---|---|
| S1 | ideal | const. | 0 | closed |
| S2 | ideal | const. | 0 | open |
| S3 | ideal | const. | 1 g | closed |
| S4 | ideal | const. | 1 g | open |
| S5 | const. | const. | - | closed |
| S6 | const. | const. | - | open |
| S1B | ideal | Equation (23) | 0 | closed |
| S3B | ideal | Equation (23) | 1 g | closed |
| S2B | ideal | Equation (23) | 0 | open |
| S4B | ideal | Equation (23) | 1 g | open |
| Scenario | Vapor | Liquid | Gravity | Domain | Max | Orientation | Max |
|---|---|---|---|---|---|---|---|
| S1 | ideal | const. | 0 | closed | 1.001 | + | 0.469 |
| S2 | ideal | const. | 0 | open | 1.052 | − | 0.399 |
| S3 | ideal | const. | 1 g | closed | 1.002 | + | 0.446 |
| S4 | ideal | const. | 1 g | open | 1.001 | − | 0.51 |
| S5 | const. | const. | - | closed | 1 | 0 | 0.491 |
| S6 | const. | const. | - | open | 1.05 | − | 0.386 |
| S1B | ideal | Equation (23) | 0 | closed | 1.000 | + | 0.388 |
| S2B | ideal | Equation (23) | 0 | open | 1.055 | − | 0.353 |
| S3B | ideal | Equation (23) | 1 g | closed | 1.003 | + | 0.428 |
| S4B | ideal | Equation (23) | 1 g | open | 1.001 | + | 0.429 |
| Scenario | Gravity | Domain | Max | S1 Diff | Open/Closed Diff | Diff | Gravity Diff |
|---|---|---|---|---|---|---|---|
| S1 | 0 | closed | 0.469 | 0.0% | |||
| S2 | 0 | open | 0.399 | −14.9% | −14.9% | ||
| S3 | 1 g | closed | 0.446 | −4.9% | −4.9% | ||
| S4 | 1 g | open | 0.51 | 8.7% | 14.3% | 27.8% | |
| S5 | - | closed | 0.491 | 4.7% | |||
| S6 | - | open | 0.386 | −17.7% | −21.4% | ||
| S1B | 0 | closed | 0.388 | −17.3% | −17.3% | ||
| S2B | 0 | open | 0.353 | −24.7% | −9.0% | −11.5% | |
| S3B | 1 g | closed | 0.428 | −8.7% | −4.0% | 10.3% | |
| S4B | 1 g | open | 0.429 | −8.5% | 0.2% | −15.9% | 21.5% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ciccotosto, B.E.; Brooks, C.S. Free Surface Motion of a Liquid Pool with Isothermal Sidewalls as a Benchmark for Marangoni Convection Problems. Energies 2023, 16, 6824. https://doi.org/10.3390/en16196824
Ciccotosto BE, Brooks CS. Free Surface Motion of a Liquid Pool with Isothermal Sidewalls as a Benchmark for Marangoni Convection Problems. Energies. 2023; 16(19):6824. https://doi.org/10.3390/en16196824
Chicago/Turabian StyleCiccotosto, Bruce E., and Caleb S. Brooks. 2023. "Free Surface Motion of a Liquid Pool with Isothermal Sidewalls as a Benchmark for Marangoni Convection Problems" Energies 16, no. 19: 6824. https://doi.org/10.3390/en16196824
APA StyleCiccotosto, B. E., & Brooks, C. S. (2023). Free Surface Motion of a Liquid Pool with Isothermal Sidewalls as a Benchmark for Marangoni Convection Problems. Energies, 16(19), 6824. https://doi.org/10.3390/en16196824








