Effect of Magnetic Shunts on Shell-Type Transformers Characteristics
Abstract
:1. Introduction
1.1. Introduction
1.2. Historical Outline of Calculations of Hlr Transformers with Movable Magnetic Shunts
2. Three-Dimensional Mathematical Modeling of Magnetic Flux Distribution in Radial Dispersion HLR Transformer
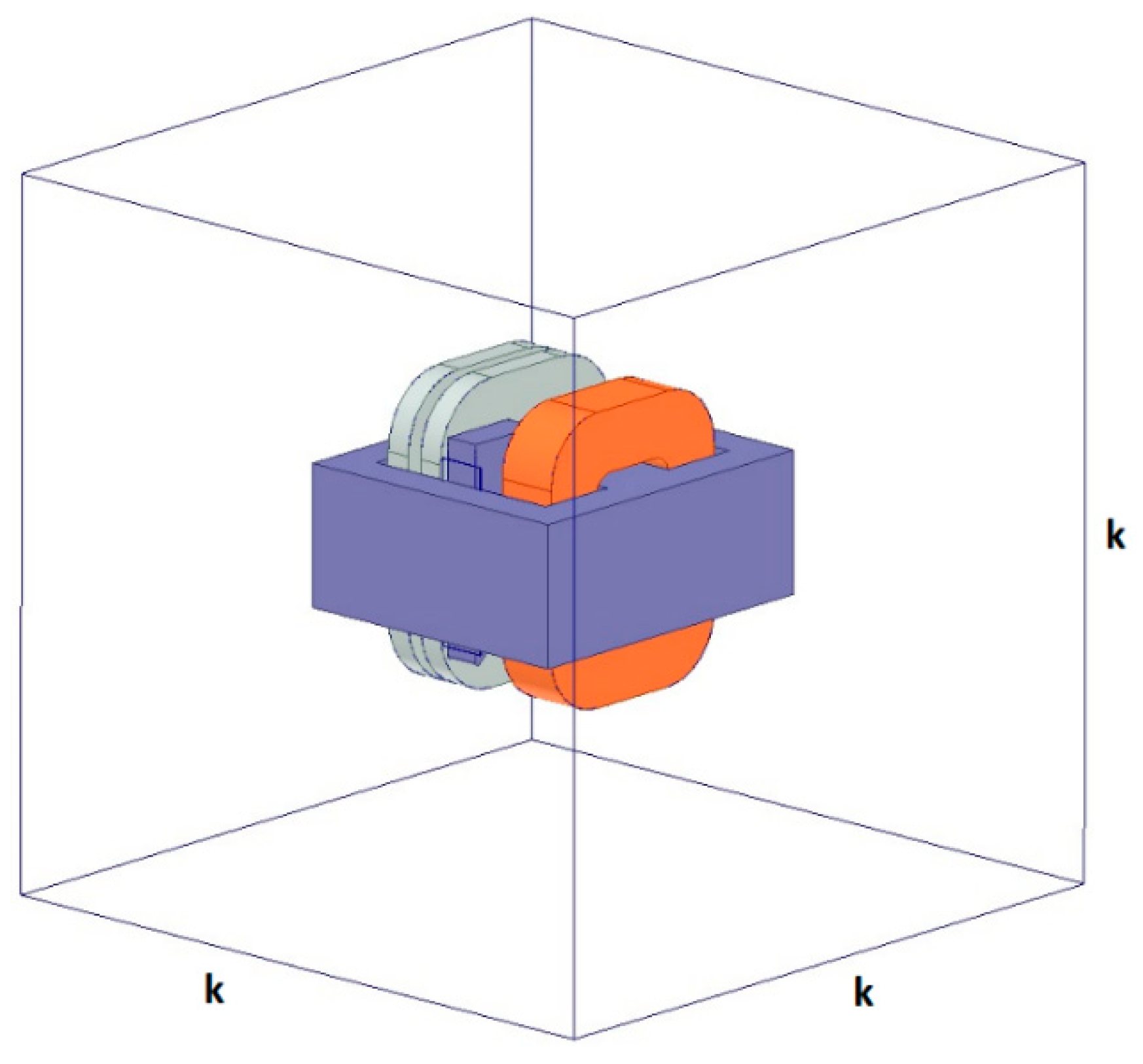
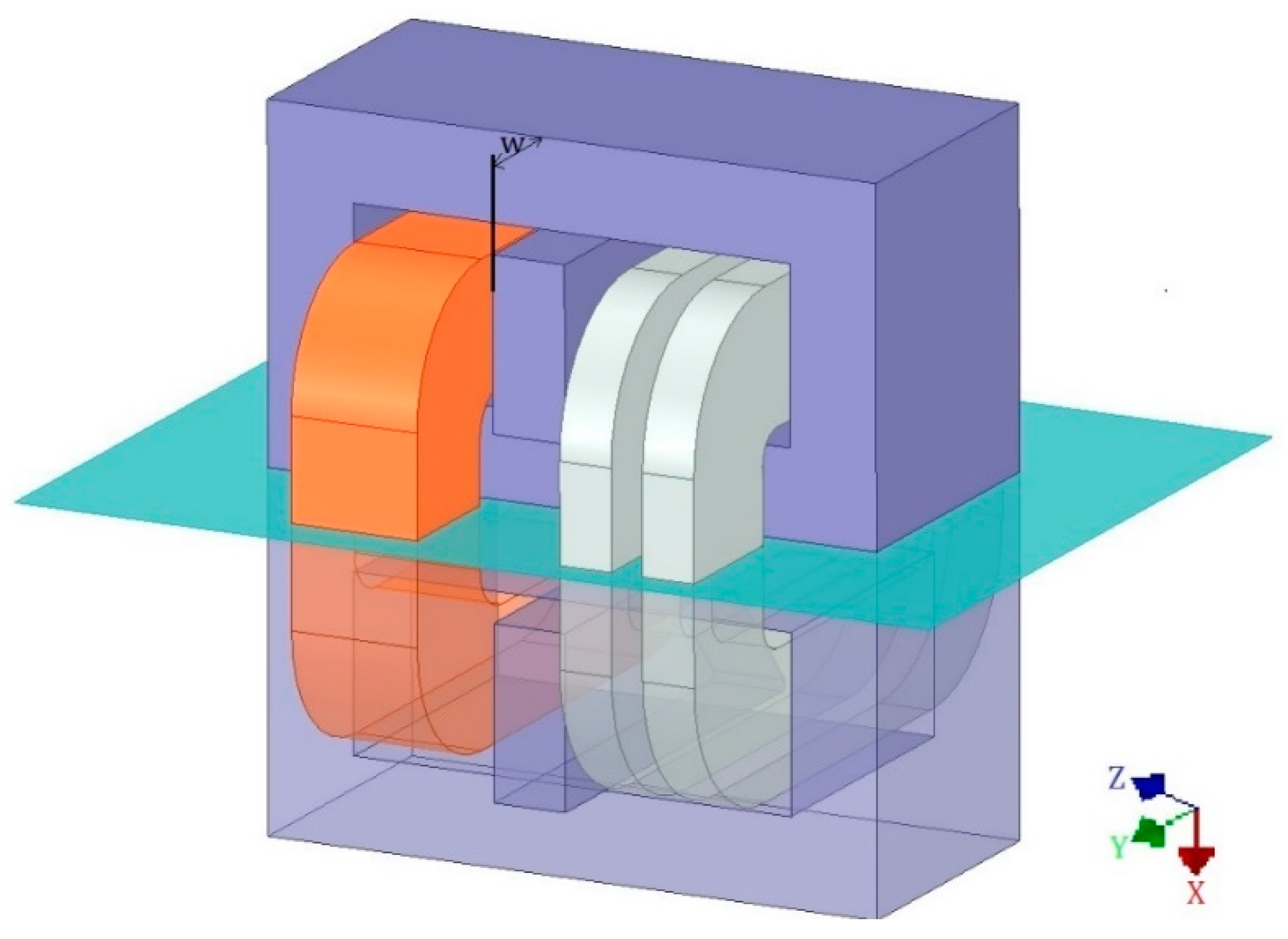
2.1. The Object under Consideration
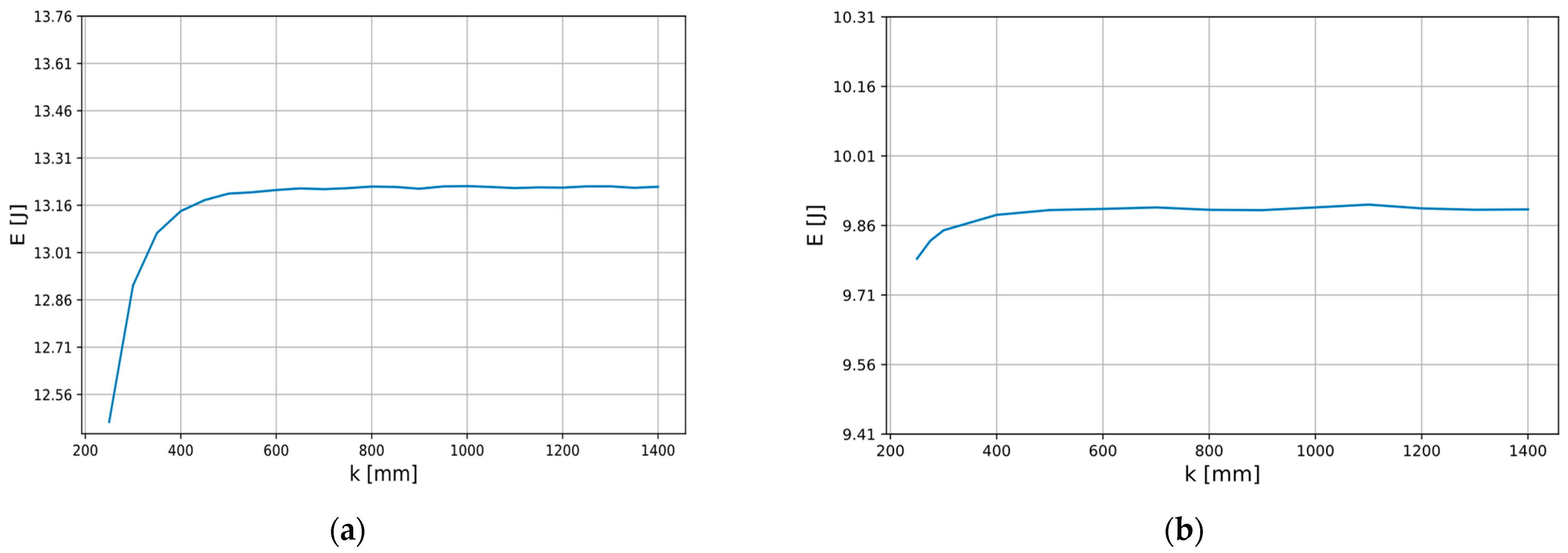
2.2. The Analysed Magnetic Field Area and Its Boundary Conditions
- u(P)—the function sought at points inside the region Ω;
- g(P)—specified function for points P belonging to the S edge of the Ω area.
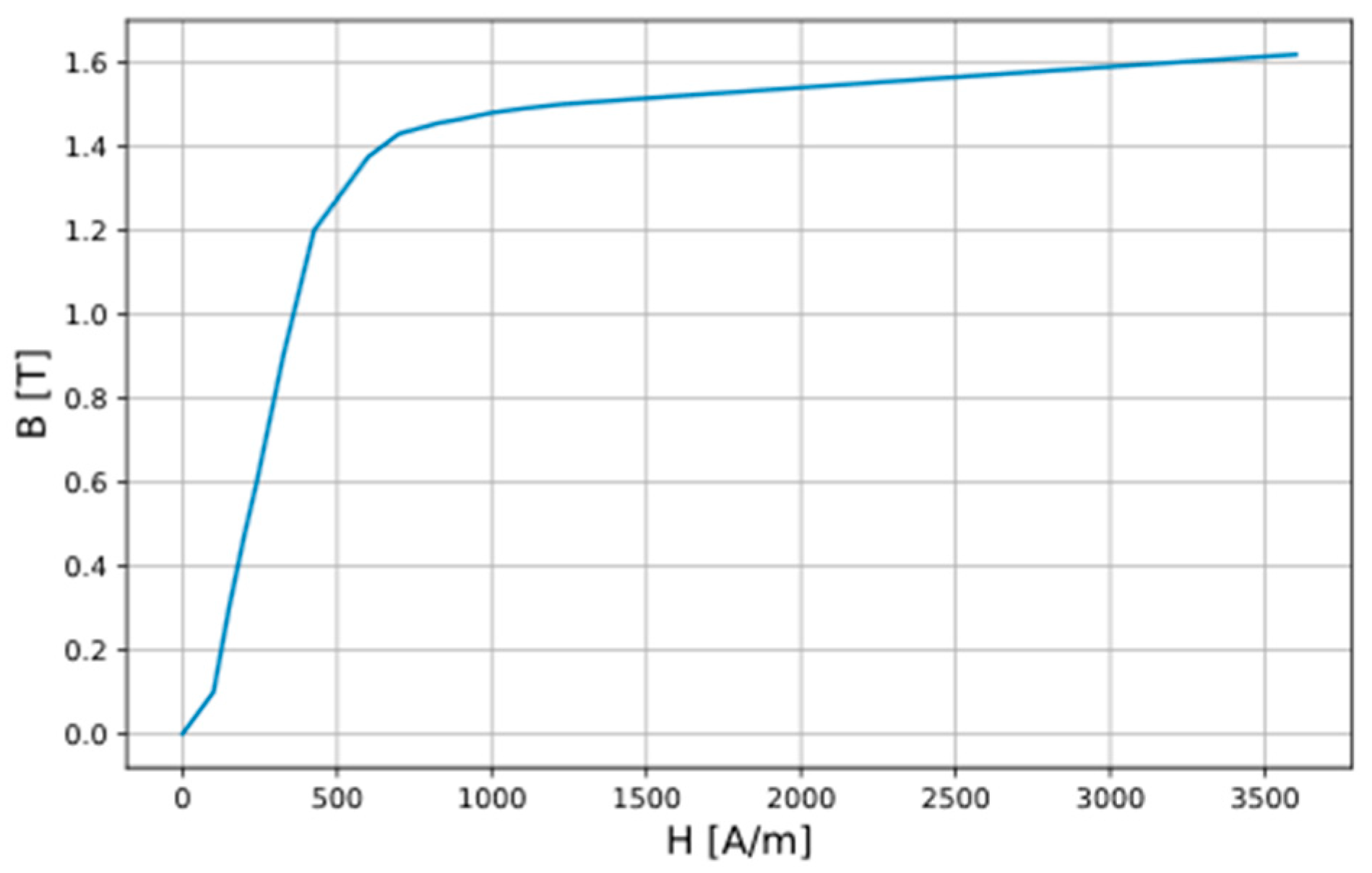
2.3. Partial Differential Equations of the Field and Its Integral Parameters
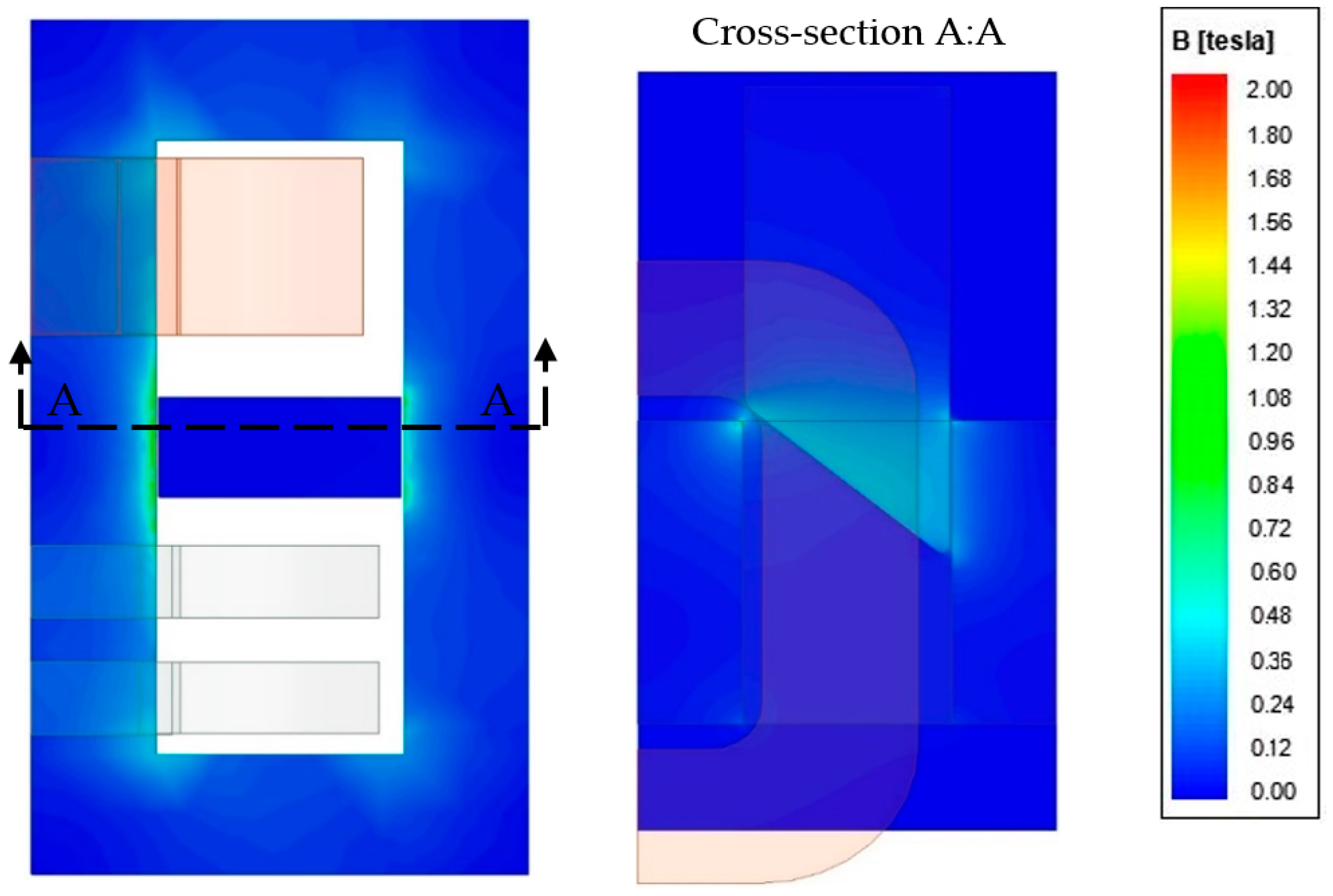
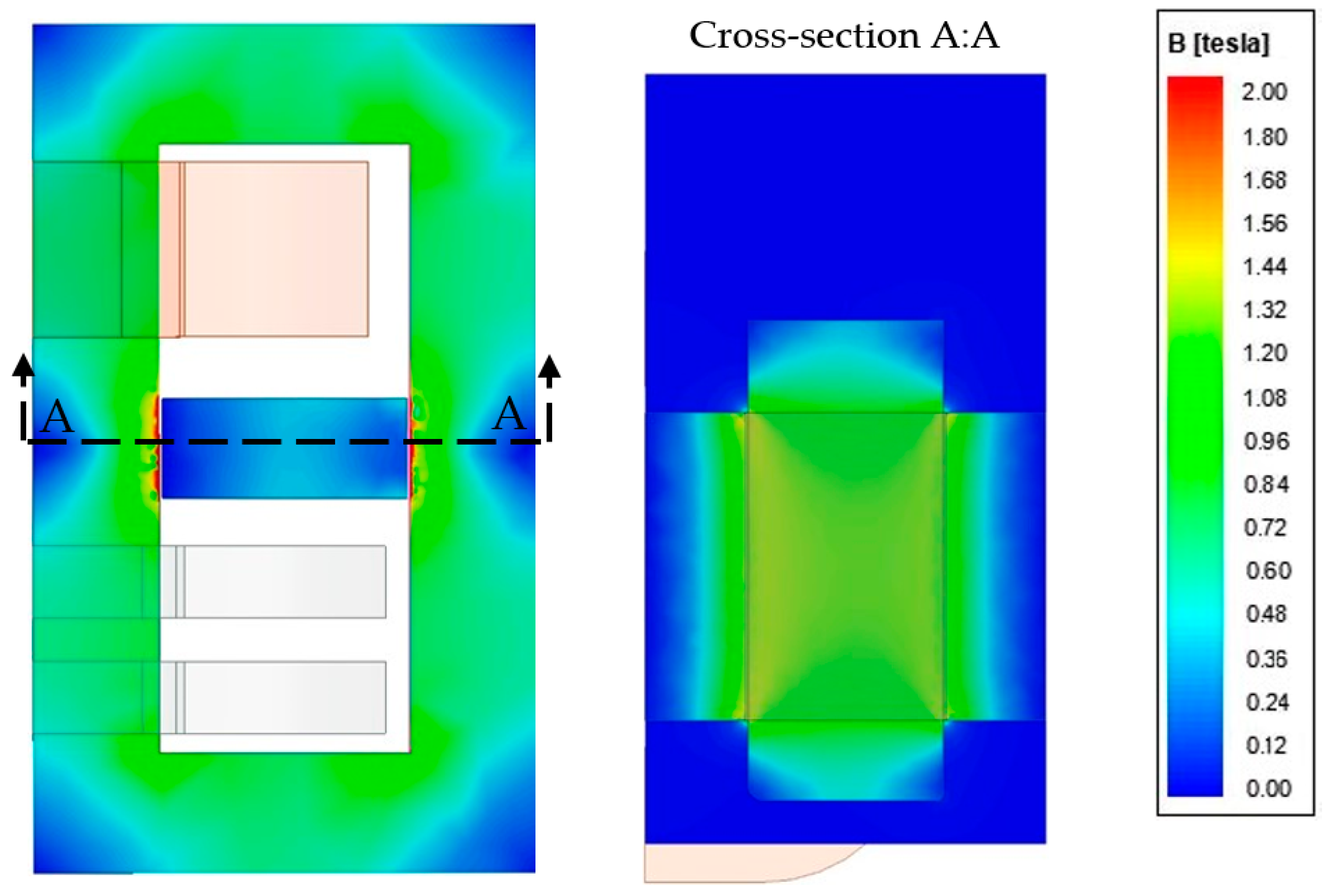
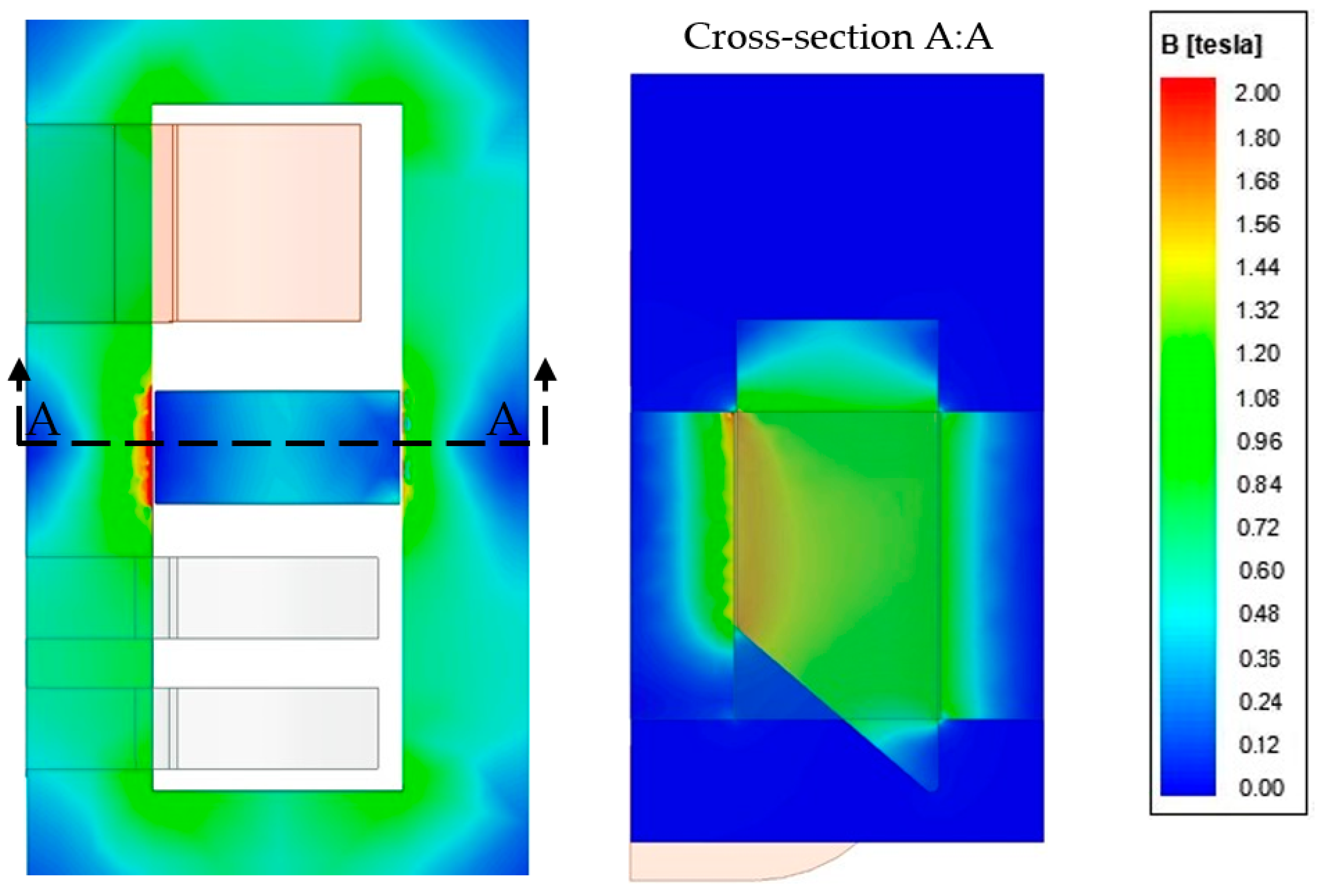
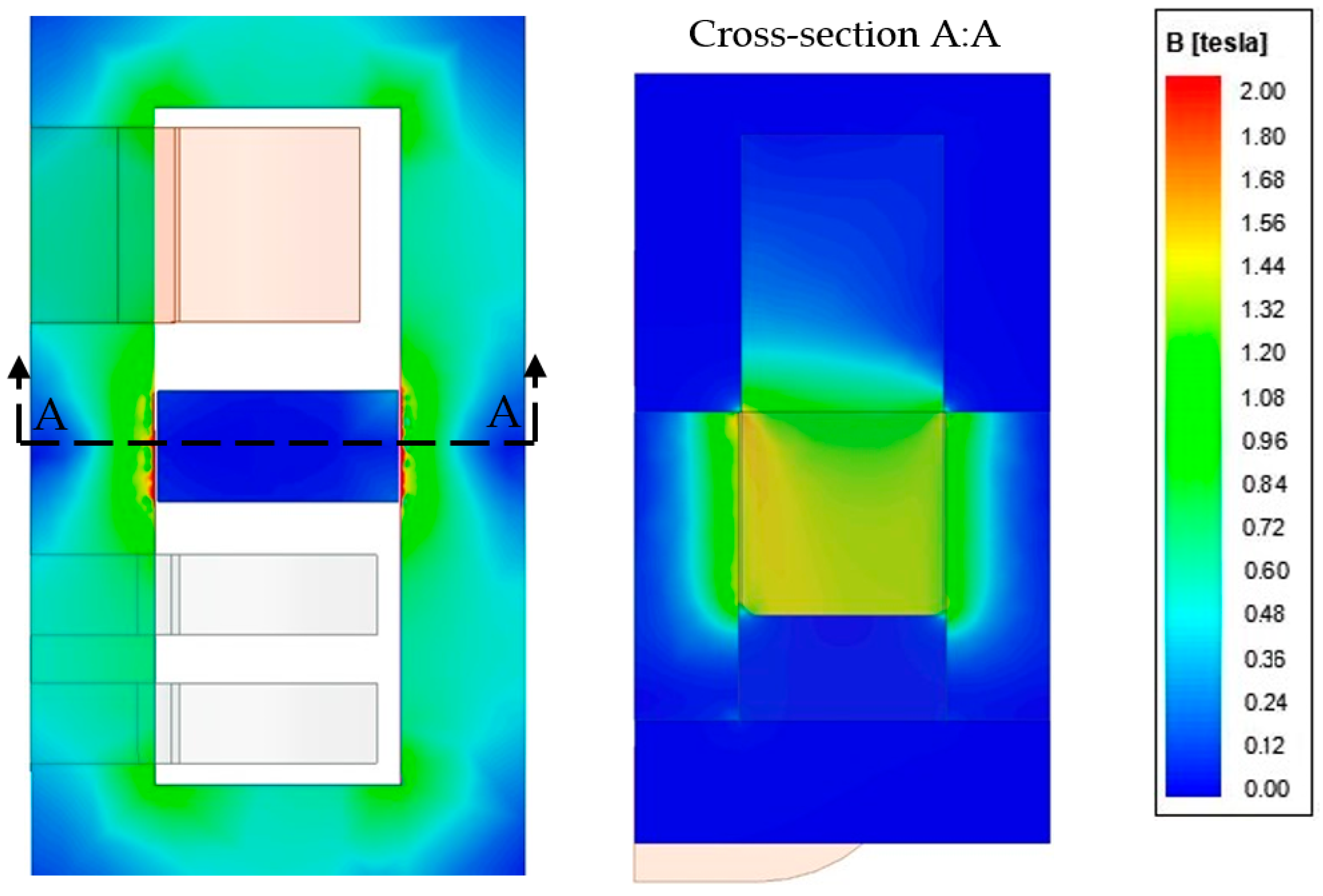
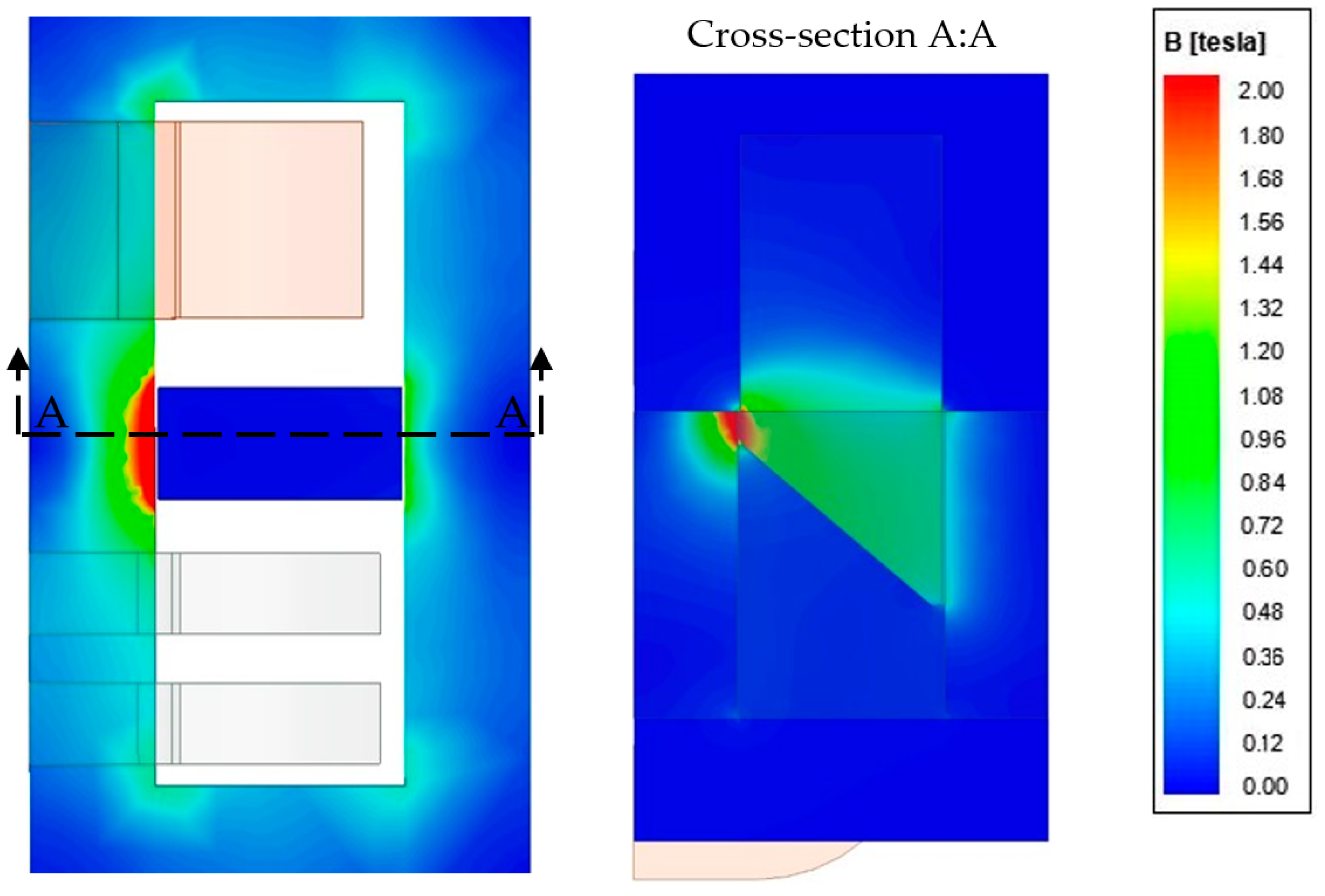
3. Results of Field Analysis for Various Constructions and Partial Protrusion of the Magnetic Shunt
3.1. Magnetic Field in the Transformer with the Same Shunt Construction, but Its Different Protrusion from the Window
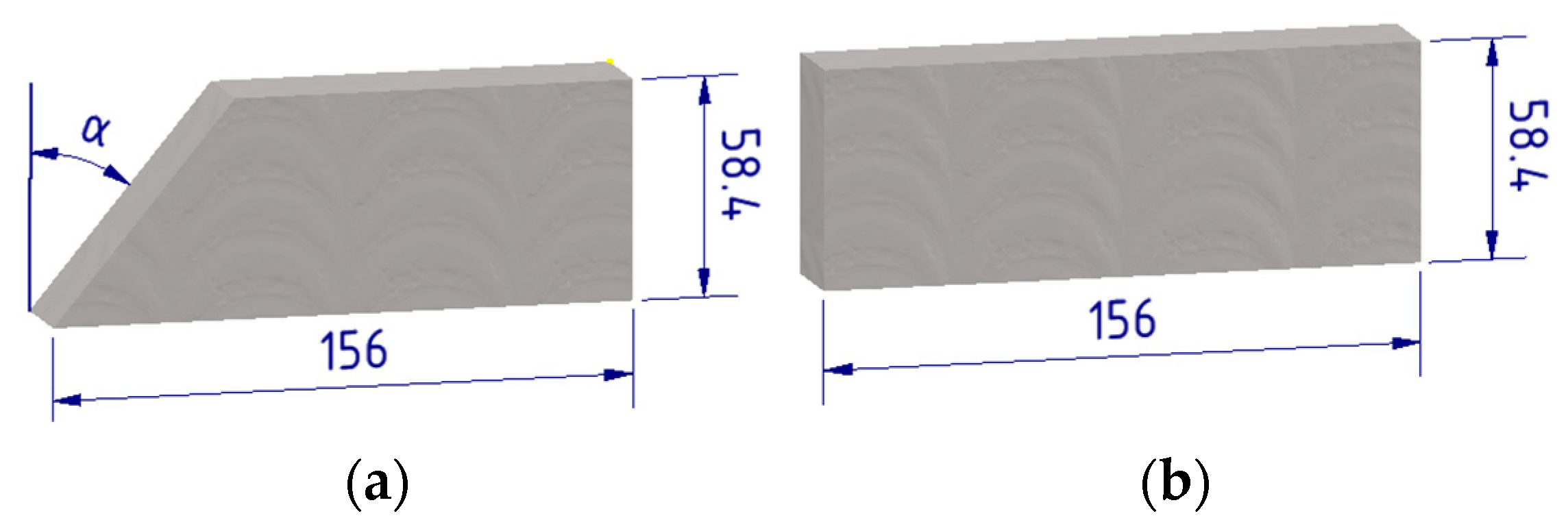
3.2. Field Simulation for Different Chamfering (Beveling) of the Magnetic Shunt
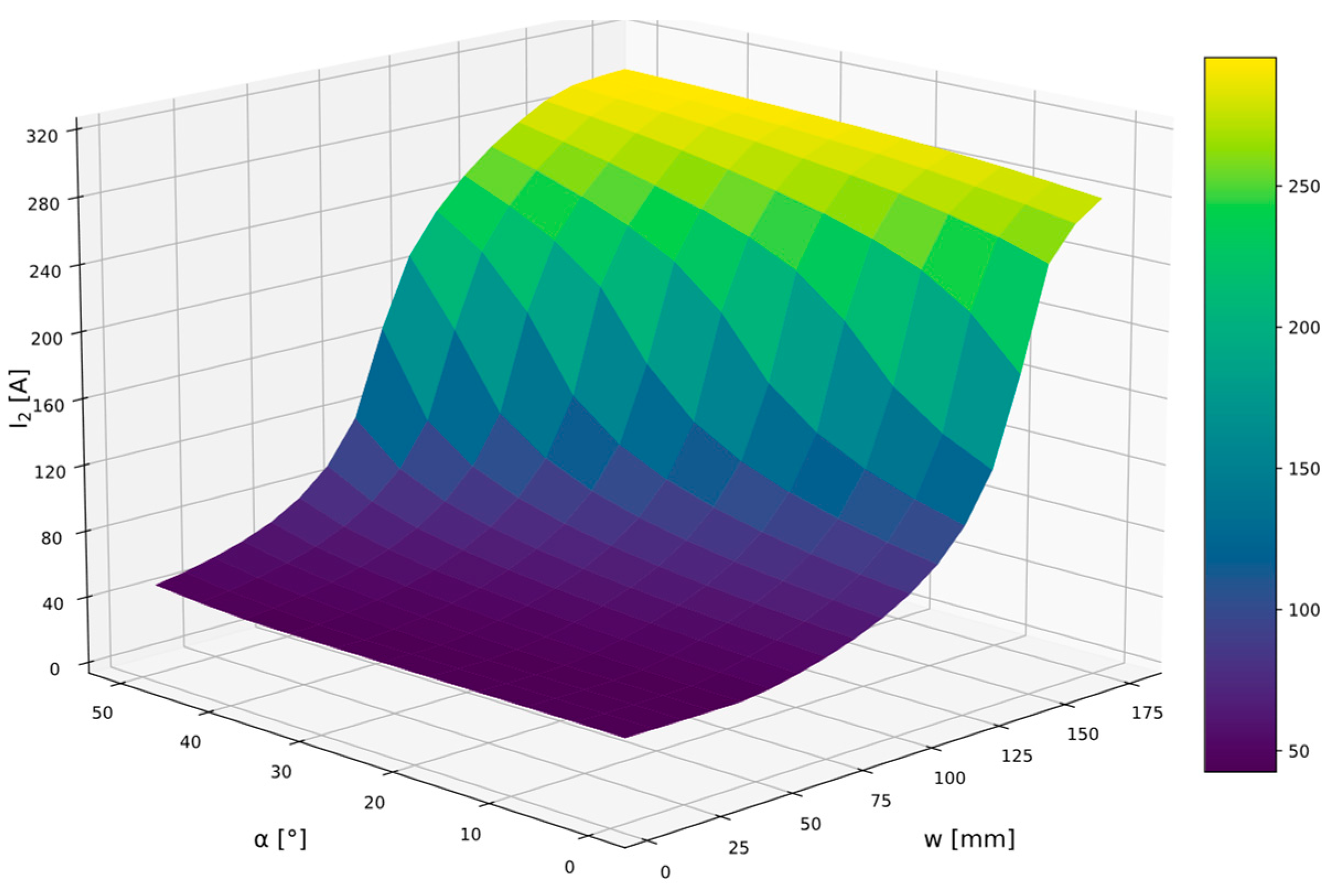
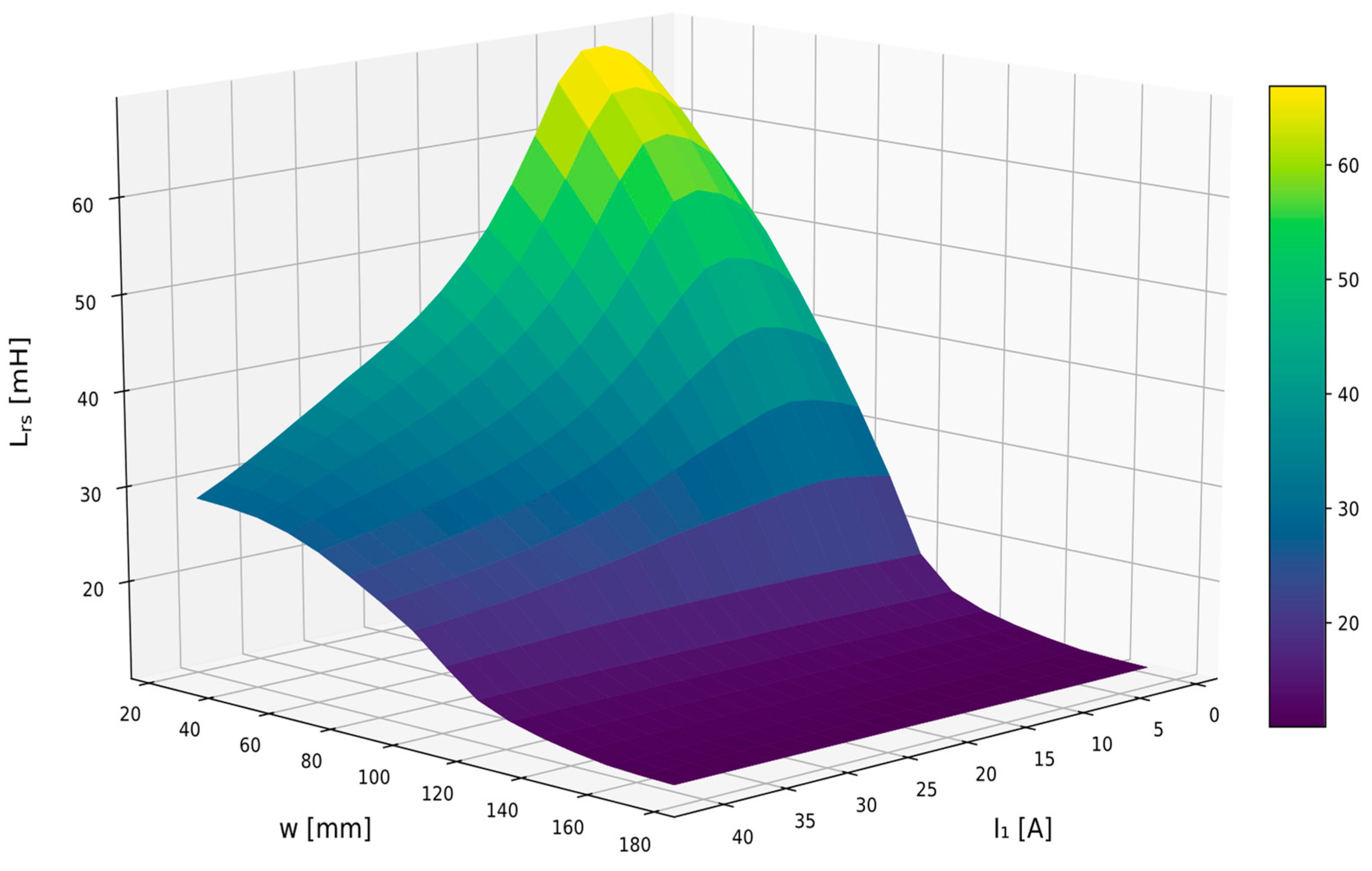
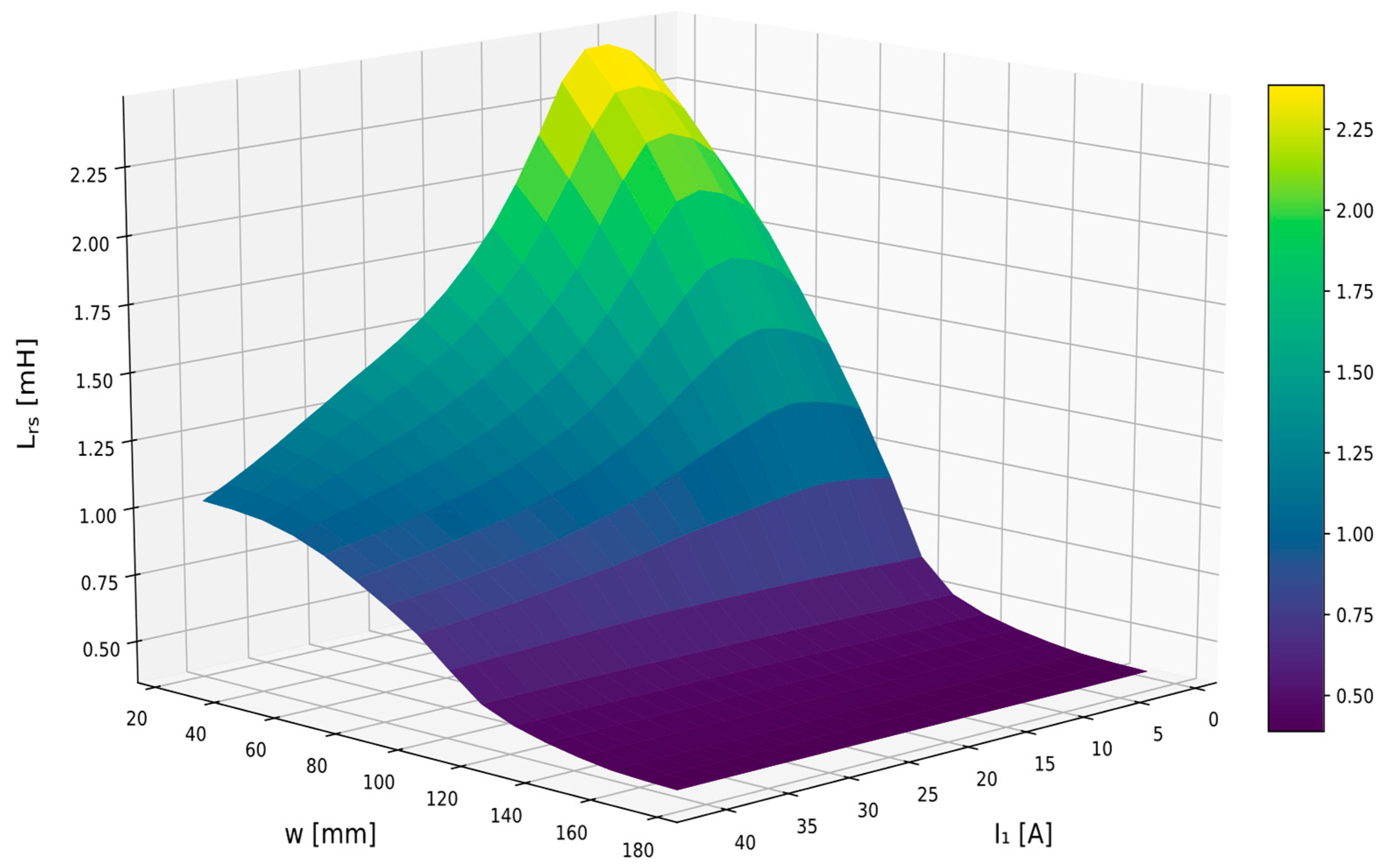
3.3. Short-Circuit Current and Leakage Reactance for Various Positions and Shunt Chamfering (Beveling)
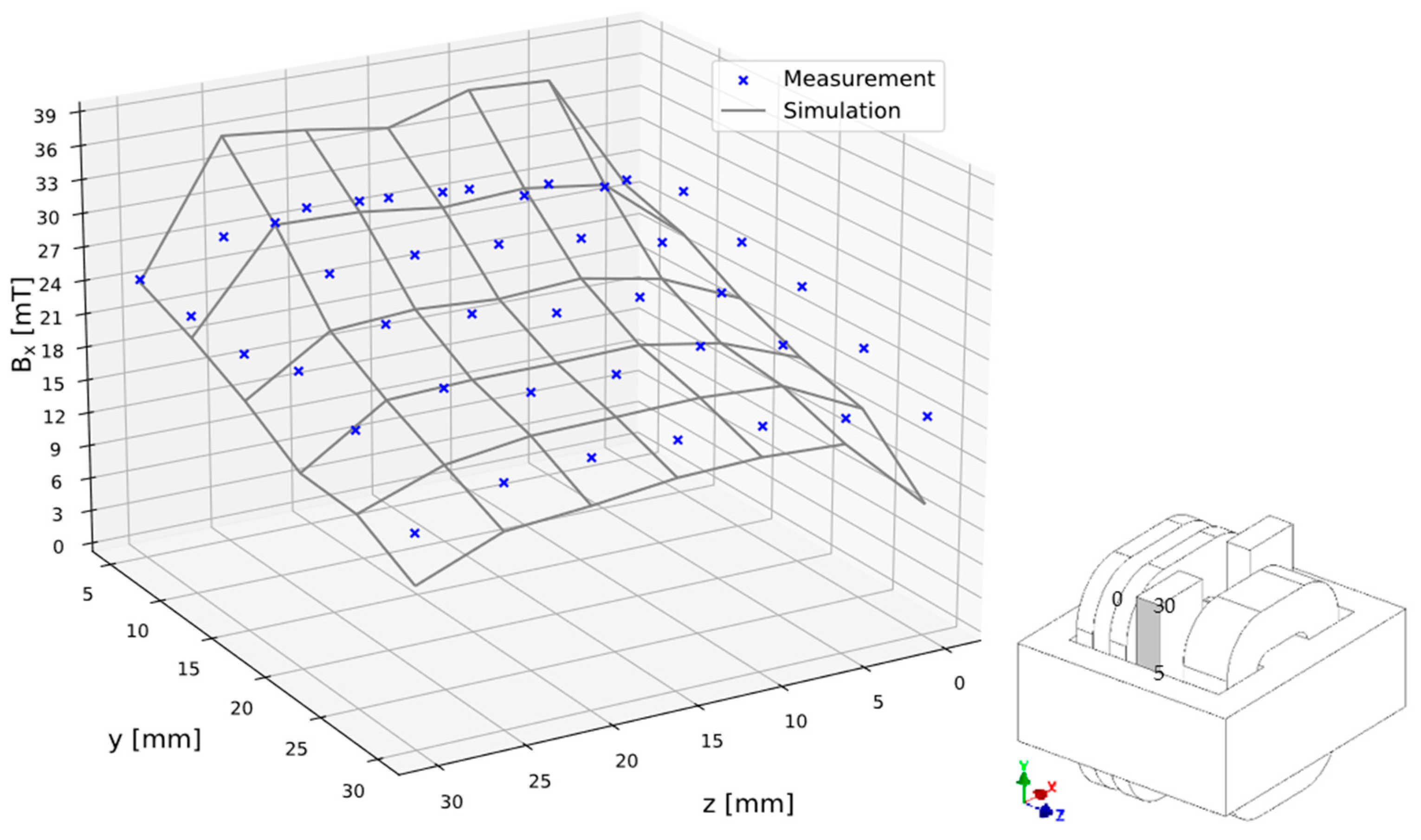
4. Measurement Verification of the Simulations
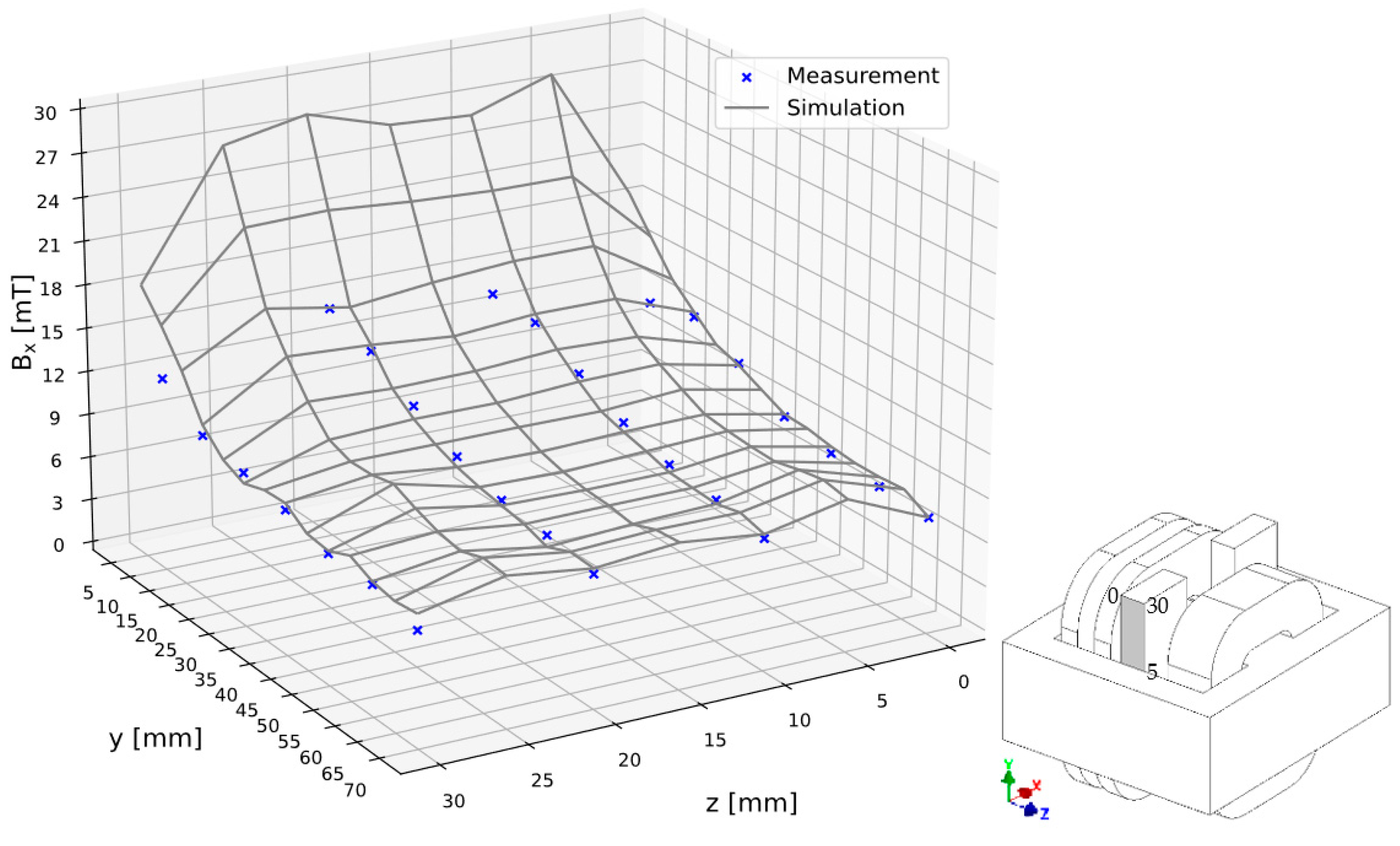
4.1. Comparison of Three-Dimensional Magnetic Flux Density Distribution
4.2. Comparison of the Leakage Reactances
- —calculated leakage reactance;
- —measured leakage reactance.
| Extraction w [mm] | Primary Winding Current Intensity in Amps | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 3 | 5 | 7 | 9 | 11 | 13 | 15 | 17 | 19 | |
| 25 | 7.4% | 2.2% | 1.3% | 5.7% | 11.1% | - | - | - | - | - |
| 35 | 8.6% | 3.5% | 0.5% | 4.9% | 9.7% | - | - | - | - | - |
| 45 | 5.6% | 2.9% | 0.7% | 4.7% | 9.1% | 10.6% | - | - | - | - |
| 55 | 5.6% | 3.1% | 0.7% | 4.5% | 8.3% | 9.5% | - | - | - | - |
| 65 | 3.5% | 3.7% | 0.2% | 3.3% | 6.7% | 8.5% | 9% | 8.7% | - | - |
| 75 | 4.3% | 3.5% | 0.8% | 2.2% | 5.3% | 7.1% | 8.4% | 8.6% | 8.5% | 8.4% |
| 85 | 5.1% | 4.5% | 2% | 0% | 2.8% | 4.7% | 6.6% | 7.8% | 8.1% | 8.4% |
5. Discussion
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Alves, B.S.; Kuo-Peng, P.; Dular, P. Contribution to power transformers leakage reactance calculation using analytical approach. Int. J. Electr. Power Energy Syst. 2019, 105, 470–477. [Google Scholar] [CrossRef]
- Dawood, K.; Cinar, M.A.; Alboyaci, B.; Sonmez, O. A new method for the calculation of leakage reactance in power transformers. J. Electr. Eng. Technol. 2017, 12, 1883–1890. [Google Scholar] [CrossRef]
- Jamali, S.; Nasir, K.; Abbaszadeh, K.; Arand, S.J. The Study of Magnetic Flux Shunts Effects on the Leakage Reactance of Transformers via FEM. Majlesi J. Electr. Eng. 2010, 4, 47–52. [Google Scholar] [CrossRef]
- Hayes, J.G.; O’Donovan, N.; Egan, M.G.; O’Donnell, T. Inductance characterization of high-leakage transformers. In Proceedings of the Conference Proceedings—IEEE Applied Power Electronics Conference and Exposition—APEC, Miami Beach, FL, USA, 9–13 February 2003; Volume 2, pp. 1150–1156. [Google Scholar]
- Grossner, N.R. The Geometry of Regulating Transformers. IEEE Trans. Magn. 1978, 14, 87–94. [Google Scholar] [CrossRef]
- Koteras, D.; Tomczuk, B.; Waindok, A. Implicit iteration calculations using 3D field analyses, to predict the power loss in powder ferromagnets, with their measurement tests. Measurements 2023, 207, 112311. [Google Scholar] [CrossRef]
- Tomczuk, B.; Koteras, D. Magnetic flux distribution in the amorphous modular transformers. J. Magn. Magn. Mater. 2011, 323, 1611–1615. [Google Scholar] [CrossRef]
- O’Connor, P. Test Engineering: A Concise Guide to Cost-Effective Design, Development and Manufacture; John Wiley & Sons: Hoboken, NJ, USA, 2001. [Google Scholar]
- Güemes-Alonso, J.A. A new method for calculating of leakage reactances and iron losses in transformers. In Proceedings of the ICEMS’2001, Fifth International Conference on Electrical Machines and Systems, Shenyang, China, 18–20 August 2001; Volume 1, pp. 178–181. [Google Scholar]
- Kashtiban, A.M.; Hagh, M.T.; Milani, A.R.; Haque, M.T. Finite Element Calculation of Winding Type Effect on Leakage Flux in SinglePhase Shell Type Transformers. In Proceedings of the 5th WSEAS International Conference on Applications of Electrical Engineering, Prague, Czech Republic, 12–14 March 2006; pp. 39–43. [Google Scholar]
- Jahromi, A.N.; Faiz, J.; Mohseni, H. A fast method for calculation of transformers leakage reactance using energy technique. IJE Trans. B Appl. 2003, 16, 41–48. [Google Scholar]
- Mechkov, E.; Tzeneva, R.; Mateev, V.; Yatchev, I. Thermal analysis using 3D FEM model of oil-immersed distribution transformer. In Proceedings of the 19th International Symposium on Electrical Apparatus and Technologies (SIELA), Bourgas, Bulgaria, 29 May–1 June 2016. [Google Scholar]
- Ahmad, A.; Javed, I.; Nazar, W.; Mukhtar, M.A. Short Circuit Stress Analysis Using FEM in Power Transformer on H-V Winding Displaced Vertically & Horizontally. Alex. Eng. J. 2018, 57, 147–157. [Google Scholar]
- Naranpanawe, L.; Saha, T.; Ekanayake, C. Finite element analysis to understand the mechanical defects in power transformer winding clamping structure. In Proceedings of the IEEE Power & Energy Society General Meeting, Chicago, IL, USA, 16–20 July 2017; pp. 1–5. [Google Scholar]
- Gowri, N.V.; Akthar, Z. Finite Element Analysis of Power Transformer. Int. J. Mech. Eng. Technol. (IJMET) 2019, 10, 1384–1391. [Google Scholar]
- Sieradzki, S.; Rygal, R.; Soiński, M. Apparent Core Losses and Core Losses in Five-Limb Amorphous Transformer of 160 kVA. IEEE Trans. Magn. 1998, 3, 1189–1191. [Google Scholar] [CrossRef]
- Mousavi, S.; Shamei, M.; Siadatan, A.; Nabizadeh, F.; Mirimani, S.H. Calculation of Power Transformer Losses by Finite Element Method. In Proceedings of the IEEE Electrical Power and Energy Conference (EPEC), Toronto, ON, Canada, 10–11 October 2018; pp. 1–5. [Google Scholar] [CrossRef]
- Zaitsev, I.; Bereznychenko, V.; Bajaj, M.; Taha, I.B.M.; Belkhier, Y.; Titko, V.; Kamel, S. Calculation of Capacitive-Based Sensors of Rotating Shaft Vibration for Fault Diagnostic Systems of Powerful Generators. Sensors 2022, 22, 1634. [Google Scholar] [CrossRef] [PubMed]
- Tomczuk, B. Calculation of the Magnetic Field and Short-Circuit Reactance of Dissipation Transformers. Ph.D. Thesis, Lodz University of Technology, Lodz, Poland, 1985. [Google Scholar]
- Zakrzewski, K.; Tomczuk, B. Application of the finite difference method for the windings reactance calculations of the stray transformers. Arch. Elektrotechniki PWN 1990, 37, 43–50. (In Polish) [Google Scholar]
- Tomczuk, B. Three-Dimensional Leakage Reactance Calculation and Magnetic Field Analysis for Unbounded Problems. IEEE Trans. Magn. 1992, 28, 1935–1940. [Google Scholar] [CrossRef]
- Tomczuk, B. Analysis of 3-D Magnetic Fields in High Leakage Reactance Transformers. IEEE Trans. Magn. 1994, 30, 2734–2738. [Google Scholar] [CrossRef]
- Kulkarni, S.V.; Khaparde, S.A. Transformer Engineering: Design, Technology, and Diagnostics, 2nd ed.; CRC Press: Boca Raton, FL, USA, 2012. [Google Scholar]
- Ansys Maxwell 3D User’s Guide. Available online: https.www.ansys.com/ (accessed on 13 June 2023).
- Binns, K.J.; Lawrenson, P.J.; Trowbridge, C.W. The Analytical and Numerical Solution of Electric and Magnetic Fields; John Wiley and Sons: New York, NY, USA, 1993. [Google Scholar]
- Sykulski, J.K. Computational Magnetics; Chapman & Hall: London, UK, 1995. [Google Scholar]
- Bandelier, B.; Rioux-Damidau, F. Mixed finite element method for magnetostatics in ℝ3. IEEE Trans. Magn. 1998, 34, 2473–2476. [Google Scholar] [CrossRef]
- Li, Y.; Berthiau, G.; Feliachi, M.; Cheriet, A. 3D finite volume modeling of ENDE using electromagnetic T-formulation. J. Sens. 2012, 2012, 785271. [Google Scholar] [CrossRef]
- Specogna, R.; Suuriniemi, S.; Trevisan, F. Geometric T-Ω approach to solve eddy currents coupled to electric circuits. Int. J. Numer. Methods Eng. 2008, 74, 101–115. [Google Scholar] [CrossRef]
- Codecasa, L.; Dular, P.; Specogna, R.; Trevisan, F. A perturbation method for the T- ω geometric eddy-current formulation. IEEE Trans. Magn. 2010, 46, 3045–3048. [Google Scholar] [CrossRef]
- Farahani, H.F.; Zare, M.; Mohammad, S.; Razi, P.; Khodakarami, A. Finite Element Analysis of Leakage Inductance of 3-Phase Shell-Type and Core Type Transformers. Res. J. Appl. Sci. Eng. Technol. 2012, 4, 1721–1728. [Google Scholar]




| Parameter | Value |
|---|---|
| Rated voltage | 400 [V] |
| Maximum primary winding current | 41 [A] |
| Maximum secondary winding current | 225 [A] |
| Power | 16 [kVA] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tomczuk, B.; Weber, D. Effect of Magnetic Shunts on Shell-Type Transformers Characteristics. Energies 2023, 16, 6814. https://doi.org/10.3390/en16196814
Tomczuk B, Weber D. Effect of Magnetic Shunts on Shell-Type Transformers Characteristics. Energies. 2023; 16(19):6814. https://doi.org/10.3390/en16196814
Chicago/Turabian StyleTomczuk, Bronisław, and Dawid Weber. 2023. "Effect of Magnetic Shunts on Shell-Type Transformers Characteristics" Energies 16, no. 19: 6814. https://doi.org/10.3390/en16196814
APA StyleTomczuk, B., & Weber, D. (2023). Effect of Magnetic Shunts on Shell-Type Transformers Characteristics. Energies, 16(19), 6814. https://doi.org/10.3390/en16196814





