Power Quality Enhancement of Remote Gas Field Generations with Smart Power Converters
Abstract
:1. Introduction
2. Background
3. System Configuration and Control Strategies Overview
4. Power Quality Improvement
5. The Enhancement of Multi-Generator System Dynamics
6. Converter Control Strategy Selection Guidance
7. Discussion
8. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
| Symbol | Description |
|---|---|
| Pgrid*, Qgrid* | Rated grid-side active and reactive power |
| Pgrid, Qgrid | Measured grid-side active and reactive power |
| Vdc*, Vdc | Rated and measured DC link voltage |
| Vpcc*, Vpcc | Rated and measured voltage at point of common coupling |
| Pgen*, Pgen | Rated and measured generator-side active power |
| H | Inertia constant |
| D | Damping constant |
| K | Voltage droop coefficient |
| w*, w | Rated and measured frequency |
| θgrid, θgen | Grid-side and generator-side phase angles |
| V*, V | Rated voltage and generated voltage reference |
| iL2d_grid, iL2q_grid | Measured grid-side output current in dq |
| ucd_grid, ucq_grid | Measured grid-side output voltage in dq |
| iL1d_grid*, iL1q_grid* | Reference of grid-side inductor current in dq |
| iL1d_gen*, iL1q_gen* | Reference of generator-side inductor current in dq |
| iL1d_grid, iL1q_grid | Measured grid-side inductor current in dq |
| iL1d_gen, iL1q_gen | Measured generator-side inductor current in dq |
| Vharmonic | Harmonic voltage |
| Zh | Harmonic impedance |
| Symbol | Description | Value |
|---|---|---|
| Utility Grid | ||
| Vgrid_H | Higher-side line voltage (RMS) | 25 kV |
| Vgrid_L | Lower-side line voltage (RMS) | 480 V |
| fgrid | Grid frequency | 60 Hz |
| Synchronous Generator | ||
| Vgen | Terminal line voltage (RMS) | 480 V |
| Sn | Rated power | 2.5 MVA |
| fn | Rated frequency | 60 Hz |
| Hgen | Inertia constant | 1.4 s |
| Xd, Xd’, Xd’’, Xq, Xq’’ | dq-axis synchronous reactance | 2.4, 0.2, 0.15, 1.77, 0.26 p.u. |
| Tdo’, Tdo’’, Tqo’’ | dq-axis open-circuit time constant | 4.48, 0.07, 0.1 s |
| kGOV | Governor droop coefficient | 16.7 |
| TACT | Actuator time constant | 0.09 s |
| B2B Converter | ||
| Sn | Rated capacity | 3 MVA |
| fn | Nominal frequency | 60 Hz |
| L1, Cf | Filter inductance and capacitance | 0.1 mH, 0.57 mF |
| fsw | Switching frequency | 4 kHz |
| Vdc* | Nominal DC link voltage | 1300 V |
| Cdc | DC link capacitance | 0.095 F |
| Symbol | Description | Value |
|---|---|---|
| Grid-side VSC Controller | ||
| HVSG | Inertia constant | 1.5 s |
| DVSG | Damping constant | 50 |
| KVSG | V-Q coefficient | 0.05 |
| kp,VC, ki,VC | Voltage control loop | 2, 10 |
| kp,CC, ki,CC | Current control loop | 0.8, 10 |
| TLPF | Low-pass filter time constant | 0.002s |
| Generator-side VSC Controller | ||
| kp,pll, ki,pll | Phase-locked loop | 60, 1400 |
| kp,VDC, ki,VDC | DC link voltage control loop | 4, 200 |
| kp,CC, ki,CC | Current control loop | 0.5, 10 |
| Symbol | Description | Value |
|---|---|---|
| Grid-side VSC Controller | ||
| kp,pll, ki,pll | Phase-locked loop | 60, 1400 |
| kp,p, ki,p | Real power controller | 0.4, 40 |
| kp,VAC, ki,VAC | AC voltage controller | 0.2, 20 |
| kp,CC, ki,CC | Current control loop | 0.5, 10 |
| TLPF | Low-pass filter time constant | 0.002 s |
| Generator-side VSC Controller | ||
| kp,pll, ki,pll | Phase-locked loop | 60, 1400 |
| kp,VDC, ki,VDC | DC link voltage control loop | 4, 200 |
| kp,CC, ki,CC | Current control loop | 0.5, 10 |
| Symbol | Description | Value |
|---|---|---|
| Grid-side VSC Controller | ||
| kp,pll, ki,pll | Phase-locked loop | 60, 1400 |
| kp,VDC, ki,VDC | DC link voltage control loop | 4, 200 |
| kp,VAC, ki,VAC | AC voltage controller | 0.4, 20 |
| kp,CC, ki,CC | Current control loop | 0.5, 10 |
| Generator-side VSC Controller | ||
| kp,pll, ki,pll | Phase-locked loop | 60, 1400 |
| kp,p, ki,p | Real power controller | 0.4 40 |
| kp,CC, ki,CC | Current control loop | 0.5, 10 |
| TLPF | Low-pass filter time constant | 0.002s |
Appendix B
References
- Prisyazhniuk, V.A. Alternative Trends in Development of Thermal Power Plants. Appl. Therm. Eng. 2008, 28, 190–194. [Google Scholar] [CrossRef]
- Harfoot, M.B.J.; Tittensor, D.P.; Knight, S.; Arnell, A.P.; Blyth, S.; Brooks, S.; Butchart, S.H.M.; Hutton, J.; Jones, M.I.; Kapos, V.; et al. Present and Future Biodiversity Risks from Fossil Fuel Exploitation. Conserv. Lett. 2018, 11, e12448. [Google Scholar] [CrossRef]
- Ilyushin, Y.V. Development of a Process Control System for the Production of High-Paraffin Oil. Energies 2022, 15, 6462. [Google Scholar] [CrossRef]
- Marinina, O.; Nechitailo, A.; Stroykov, G.; Tsvetkova, A.; Reshneva, E.; Turovskaya, L. Technical and Economic Assessment of Energy Efficiency of Electrification of Hydrocarbon Production Facilities in Underdeveloped Areas. Sustainability 2023, 15, 9614. [Google Scholar] [CrossRef]
- Fekry, H.M.; Eldesouky, A.A.; Kassem, A.M.; Abdelaziz, A.Y. Power Management Strategy Based on Adaptive Neuro Fuzzy Inference System for AC Microgrid. IEEE Access 2020, 8, 192087–192100. [Google Scholar] [CrossRef]
- Statistical Review of World Energy 2022. 2022. Available online: https://www.bp.com/content/dam/bp/business-sites/en/global/corporate/pdfs/energy-economics/statistical-review/bp-stats-review-2022-full-report.pdf (accessed on 1 August 2023).
- Cai, H.; Burnham, A.; Chen, R.; Wang, M. Wells to Wheels: Environmental Implications of Natural Gas as a Transportation Fuel. Energy Policy 2017, 109, 565–578. [Google Scholar] [CrossRef]
- Muttaqi, K.M.; Le, A.D.T.; Negnevitsky, M.; Ledwich, G. A Coordinated Voltage Control Approach for Coordination of OLTC, Voltage Regulator, and DG to Regulate Voltage in a Distribution Feeder. IEEE Trans. Ind. Applicat. 2015, 51, 1239–1248. [Google Scholar] [CrossRef]
- Le, A.D.T.; Muttaqi, K.M.; Negnevitsky, M.; Ledwich, G. Response Coordination of Distributed Generation and Tap Changers for Voltage Support. In Proceedings of the 2007 Australasian Universities Power Engineering Conference, Perth, Australia, 9–12 December 2007; pp. 1–7. [Google Scholar]
- Agalgaonkar, Y.P.; Pal, B.C.; Jabr, R.A. Distribution Voltage Control Considering the Impact of PV Generation on Tap Changers and Autonomous Regulators. IEEE Trans. Power Syst. 2014, 29, 182–192. [Google Scholar] [CrossRef]
- Brady, P.; Dai, C.; Baghzouz, Y. Need to Revise Switched Capacitor Controls on Feeders with Distributed Generation. In Proceedings of the 2003 IEEE PES Transmission and Distribution Conference and Exposition (IEEE Cat. No.03CH37495), Dallas, TX, USA, 7–12 September 2003; pp. 590–594. [Google Scholar]
- Viawan, F.A.; Karlsson, D. Voltage and Reactive Power Control in Systems With Synchronous Machine-Based Distributed Generation. IEEE Trans. Power Deliv. 2008, 23, 1079–1087. [Google Scholar] [CrossRef]
- Hamadi, A.; Rahmani, S.; Al-Haddad, K. A Hybrid Passive Filter Configuration for VAR Control and Harmonic Compensation. IEEE Trans. Ind. Electron. 2010, 57, 2419–2434. [Google Scholar] [CrossRef]
- Beres, R.N.; Wang, X.; Liserre, M.; Blaabjerg, F.; Bak, C.L. A Review of Passive Power Filters for Three-Phase Grid-Connected Voltage-Source Converters. IEEE J. Emerg. Sel. Top. Power Electron. 2016, 4, 54–69. [Google Scholar] [CrossRef]
- Li, Y.R.; Nejabatkhah, F.; Tian, H. Smart Hybrid AC/DC Microgrids: Power Management, Energy Management, and Power Quality Control, 1st ed.; Wiley: Hoboken, NJ, USA, 2022; ISBN 978-1-119-59837-4. [Google Scholar]
- Daratha, N.; Das, B.; Sharma, J. Coordination Between OLTC and SVC for Voltage Regulation in Unbalanced Distribution System Distributed Generation. IEEE Trans. Power Syst. 2014, 29, 289–299. [Google Scholar] [CrossRef]
- Tokiwa, A.; Yamada, H.; Tanaka, T.; Watanabe, M.; Shirai, M.; Teranishi, Y. New Hybrid Static VAR Compensator with Series Active Filter. Energies 2017, 10, 1617. [Google Scholar] [CrossRef]
- Sirjani, R.; Rezaee Jordehi, A. Optimal Placement and Sizing of Distribution Static Compensator (D-STATCOM) in Electric Distribution Networks: A Review. Renew. Sustain. Energy Rev. 2017, 77, 688–694. [Google Scholar] [CrossRef]
- Jiao, W.; Chen, J.; Wu, Q.; Li, C.; Zhou, B.; Huang, S. Distributed Coordinated Voltage Control for Distribution Networks With DG and OLTC Based on MPC and Gradient Projection. IEEE Trans. Power Syst. 2022, 37, 680–690. [Google Scholar] [CrossRef]
- Shyu, K.-K.; Yang, M.-J.; Chen, Y.-M.; Lin, Y.-F. Model Reference Adaptive Control Design for a Shunt Active Power Filter System. In Proceedings of the IECON 2006—32nd Annual Conference on IEEE Industrial Electronics, Paris, France, 6–10 November 2006; pp. 73–78. [Google Scholar]
- Akagi, H.; Fujita, H.; Wada, K. A Shunt Active Filter Based on Voltage Detection for Harmonic Termination of a Radial Power Distribution Line. IEEE Trans. Ind. Applicat. 1999, 35, 638–645. [Google Scholar] [CrossRef]
- Lv, Z.; Luo, A.; Zhou, K.; Jiang, W.; Guo, L.; Shen, Y. Research of Intellective High Quality Inverter-Based Distributed Power Supply System Collocating SVC. In Proceedings of the The 2nd International Symposium on Power Electronics for Distributed Generation Systems, Hefei, China, 16–18 June 2010; pp. 846–851. [Google Scholar]
- Arsoy, A.B.; Liu, Y.; Ribeiro, P.F.; Wang, F. Statcom- SMES. IEEE Ind. Appl. Mag. 2003, 9, 21–28. [Google Scholar] [CrossRef]
- Bhattacharya, A.; Chakraborty, C.; Bhattacharya, S. Shunt Compensation. EEE Ind. Electron. Mag. 2009, 3, 38–49. [Google Scholar] [CrossRef]
- Xue, Z.Y.; Xiahou, K.S.; Li, M.S.; Ji, T.Y.; Wu, Q.H. Diagnosis of Multiple Open-Circuit Switch Faults Based on Long Short-Term Memory Network for DFIG-Based Wind Turbine Systems. IEEE J. Emerg. Sel. Topics Power Electron. 2020, 8, 2600–2610. [Google Scholar] [CrossRef]
- Ni, K.; Hu, Y.; Lagos, D.T.; Chen, G.; Wang, Z.; Li, X. Highly Reliable Back-to-Back Power Converter Without Redundant Bridge Arm for Doubly Fed Induction Generator-Based Wind Turbine. IEEE Trans. Ind. Applicat. 2019, 55, 3024–3036. [Google Scholar] [CrossRef]
- Lu, L.-Y.; Avila, N.F.; Chu, C.-C.; Yeh, T.-W. Model Reference Adaptive Back-Electromotive-Force Estimators for Sensorless Control of Grid-Connected DFIGs. IEEE Trans. Ind. Applicat. 2018, 54, 1701–1711. [Google Scholar] [CrossRef]
- Dinesh, S.; Meenakshi, R.; Suhanya, M.S.; Kumaran, M.S.; Muthu, R. Modeling and Direct Power Control of DFIG for Wind Energy Conversion System with a Back to Back Converter. In Proceedings of the 2014 International Conference on Green Computing Communication and Electrical Engineering (ICGCCEE), Coimbatore, India, 6–8 March 2014; pp. 1–6. [Google Scholar]
- Liu, T.; Wang, X.; Liu, F.; Xin, K.; Liu, Y. Transient Stability Analysis for Grid-Forming Inverters Transitioning from Islanded to Grid-Connected Mode. IEEE Open J. Power Electron. 2022, 3, 419–432. [Google Scholar] [CrossRef]
- Blanco Viejo, C.; Anton, J.C.A.; Robles, A.; Ferrero Martin, F.; Viera, J.C.; Bhosle, S.; Zissis, G. Comparison Between Different Discharge Lamp Models Based on Lamp Dynamic Conductance. IEEE Trans. Ind. Applicat. 2011, 47, 1983–1991. [Google Scholar] [CrossRef]
- Bakshi, B.G.; Roy, B. Development & Simulation of Dynamic Conductance Based High Intensity Discharge Lamp Model Driven by Low Frequency Square Wave Electronic Ballast. In Proceedings of the 2016 IEEE 7th Power India International Conference (PIICON), Bikaner, India, 25–27 November 2016; pp. 1–6. [Google Scholar]
- Wiens, G. FortisAlberta Power Quality Specifications; FortisAlberta: Calgary, AB, Canada, 2021. [Google Scholar]
- Std 1547-2018; IEEE Standard for Interconnection and Interoperability of Distributed Energy Resources with Associated Electric Power Systems Interfaces. IEEE: Piscataway, NJ, USA, 2018.
- Std 421.5-2016; IEEE Recommended Practice for Excitation System Models for Power System Stability Studies. IEEE: Piscataway, NJ, USA, 2016.
- Huang, L.; Xin, H.; Li, Z.; Ju, P.; Yuan, H.; Lan, Z.; Wang, Z. Grid-Synchronization Stability Analysis and Loop Shaping for PLL-Based Power Converters With Different Reactive Power Control. IEEE Trans. Smart Grid 2020, 11, 501–516. [Google Scholar] [CrossRef]
- Gao, X.; Zhou, D.; Anvari-Moghaddam, A.; Blaabjerg, F. Stability Analysis of Grid-Following and Grid-Forming Converters Based on State-Space Model. In Proceedings of the 2022 International Power Electronics Conference (IPEC-Himeji 2022- ECCE Asia), Himeji, Japan, 15–19 May 2022; pp. 422–428. [Google Scholar]
- Morris, J.F.; Ahmed, K.H.; Egea-Alvarez, A. Analysis of Controller Bandwidth Interactions for Vector-Controlled VSC Connected to Very Weak AC Grids. IEEE J. Emerg. Sel. Topics Power Electron. 2021, 9, 7343–7354. [Google Scholar] [CrossRef]
- Liu, F.; Hu, L.; Yuan, G.; Liu, B.; Bian, Y. Control Loop Stability Criterion and Interaction Law Analysis for Grid-Connected Inverter in Weak Grid. IEEE Access 2023, 11, 12829–12842. [Google Scholar] [CrossRef]
- Rosso, R.; Wang, X.; Liserre, M.; Lu, X.; Engelken, S. Grid-Forming Converters: An Overview of Control Approaches and Future Trends. In Proceedings of the 2020 IEEE Energy Conversion Congress and Exposition (ECCE), Detroit, MI, USA, 11–15 October 2020; pp. 4292–4299. [Google Scholar]
- Wang, X.; Taul, M.G.; Wu, H.; Liao, Y.; Blaabjerg, F.; Harnefors, L. Grid-Synchronization Stability of Converter-Based Resources—An Overview. IEEE Open J. Ind. Applicat. 2020, 1, 115–134. [Google Scholar] [CrossRef]
- Li, Y.; Gu, Y.; Green, T.C. Revisiting Grid-Forming and Grid-Following Inverters: A Duality Theory. IEEE Trans. Power Syst. 2022, 37, 4541–4554. [Google Scholar] [CrossRef]
- Rauth, S.S.; Kastha, D.; Bajpai, P. Comparative Analysis of External Configuration Based DFIG-LVRT Enhancement Schemes. In Proceedings of the 2020 21st National Power Systems Conference (NPSC), Gandhinagar, India, 17–19 December 2020; pp. 1–6. [Google Scholar]
- Xiao, X.-Y.; Yang, R.-H.; Zheng, Z.-X.; Wang, Y. Cooperative Rotor-Side SMES and Transient Control for Improving the LVRT Capability of Grid-Connected DFIG-Based Wind Farm. IEEE Trans. Appl. Supercond. 2019, 29, 1–5. [Google Scholar] [CrossRef]
- Wei, Q.; Harley, R.G. Grid Connection Requirements and Solutions for DFIG Wind Turbines. In Proceedings of the 2008 IEEE Energy 2030 Conference, Atlanta, GA, USA, 17–18 November 2008; pp. 1–8. [Google Scholar]
- Yehia, D.M.; Mansour, D.-E.A.; Yuan, W. Fault Ride-Through Enhancement of PMSG Wind Turbines With DC Microgrids Using Resistive-Type SFCL. IEEE Trans. Appl. Supercond. 2018, 28, 1–5. [Google Scholar] [CrossRef]
- Jahanpour-Dehkordi, M.; Vaez-Zadeh, S.; Mohammadi, J. Development of a Combined Control System to Improve the Performance of a PMSG-Based Wind Energy Conversion System Under Normal and Grid Fault Conditions. IEEE Trans. Energy Convers. 2019, 34, 1287–1295. [Google Scholar] [CrossRef]
- Conroy, J.F.; Watson, R. Frequency Response Capability of Full Converter Wind Turbine Generators in Comparison to Conventional Generation. IEEE Trans. Power Syst. 2008, 23, 649–656. [Google Scholar] [CrossRef]
- Xue, T.; Lyu, J.; Wang, H.; Cai, X. A Complete Impedance Model of a PMSG-Based Wind Energy Conversion System and Its Effect On the Stability Analysis of MMC-HVDC Connected Offshore Wind Farms. IEEE Trans. Energy Convers. 2021, 36, 3449–3461. [Google Scholar] [CrossRef]
- Shariatpanah, H.; Fadaeinedjad, R.; Rashidinejad, M. A New Model for PMSG-Based Wind Turbine With Yaw Control. IEEE Trans. Energy Convers. 2013, 28, 929–937. [Google Scholar] [CrossRef]
- Wu, Z.; Gao, D.W.; Zhang, H.; Yan, S.; Wang, X. Coordinated Control Strategy of Battery Energy Storage System and PMSG-WTG to Enhance System Frequency Regulation Capability. IEEE Trans. Sustain. Energy 2017, 8, 1330–1343. [Google Scholar] [CrossRef]
- Kim, C.; Kim, W. Low-Voltage Ride-Through Coordinated Control for PMSG Wind Turbines Using De-Loaded Operation. IEEE Access 2021, 9, 66599–66606. [Google Scholar] [CrossRef]
- Li, Y.; Xu, Z.; Wong, K.P. Advanced Control Strategies of PMSG-Based Wind Turbines for System Inertia Support. IEEE Trans. Power Syst. 2017, 32, 3027–3037. [Google Scholar] [CrossRef]
- Xu, L.; Fan, L.; Miao, Z. DC Impedance-Model-Based Resonance Analysis of a VSC–HVDC System. IEEE Trans. Power Delivery 2015, 30, 1221–1230. [Google Scholar] [CrossRef]
- Tao, S.; Zhao, L.; Liu, Y.; Liao, K. Impedance Network Model of D-PMSG Based Wind Power Generation System Considering Wind Speed Variation for Sub-Synchronous Oscillation Analysis. IEEE Access 2020, 8, 114784–114794. [Google Scholar] [CrossRef]
- Aldin, N.A.N.; Abdellatif, W.S.E.; Elbarbary, Z.M.S.; Omar, A.I.; Mahmoud, M.M. Robust Speed Controller for PMSG Wind System Based on Harris Hawks Optimization via Wind Speed Estimation: A Real Case Study. IEEE Access 2023, 11, 5929–5943. [Google Scholar] [CrossRef]
- Wang, W.; Jiang, L.; Cao, Y.; Li, Y. A Parameter Alternating VSG Controller of VSC-MTDC Systems for Low Frequency Oscillation Damping. IEEE Trans. Power Syst. 2020, 35, 4609–4621. [Google Scholar] [CrossRef]
- Zhu, J.; Wang, X.; Zhao, J.; Yu, L.; Li, S.; Li, Y.; Guerrero, J.M.; Wang, C. Inertia Emulation and Fast Frequency-Droop Control Strategy of a Point-to-Point VSC-HVdc Transmission System for Asynchronous Grid Interconnection. IEEE Trans. Power Electron. 2022, 37, 6530–6543. [Google Scholar] [CrossRef]
- Gubba Ravikumar, K.; Bosley, B.; Clark, T.; Garcia, J. Generation Control System: Using Isochronous Load-Sharing Principles With Gas and Steam Turbine Generators. IEEE Ind. Appl. Mag. 2019, 25, 36–44. [Google Scholar] [CrossRef]
- Li, X.; Zhang, C.; Zhu, L.; Wang, Z.; Guo, L. A PLL-equivalent Model for Low-frequency Stability Analysis of Voltage Source Converter Connected to Weak Grid. IET Energy Syst. Integr. 2023, 5, 180–191. [Google Scholar] [CrossRef]
- Huang, Y.; Yuan, X.; Hu, J.; Zhou, P. Modeling of VSC Connected to Weak Grid for Stability Analysis of DC-Link Voltage Control. IEEE J. Emerg. Sel. Topics Power Electron. 2015, 3, 1193–1204. [Google Scholar] [CrossRef]
- Huang, Y.; Yuan, X.; Hu, J.; Zhou, P.; Wang, D. DC-Bus Voltage Control Stability Affected by AC-Bus Voltage Control in VSCs Connected to Weak AC Grids. IEEE J. Emerg. Sel. Topics Power Electron. 2016, 4, 445–458. [Google Scholar] [CrossRef]
- Hu, J.; Huang, Y.; Wang, D.; Yuan, H.; Yuan, X. Modeling of Grid-Connected DFIG-Based Wind Turbines for DC-Link Voltage Stability Analysis. IEEE Trans. Sustain. Energy 2015, 6, 1325–1336. [Google Scholar] [CrossRef]
- Liu, J.; Miura, Y.; Ise, T. Comparison of Dynamic Characteristics Between Virtual Synchronous Generator and Droop Control in Inverter-Based Distributed Generators. IEEE Trans. Power Electron. 2016, 31, 3600–3611. [Google Scholar] [CrossRef]
- Liu, R.; Ding, L.; Xue, C.; Li, Y. Small-signal Modelling and Analysis of Microgrids with Synchronous and Virtual Synchronous Generators. IET Energy Syst. Integr. 2023, esi2.12099. [Google Scholar] [CrossRef]
- Pogaku, N.; Prodanovic, M.; Green, T.C. Modeling, Analysis and Testing of Autonomous Operation of an Inverter-Based Microgrid. IEEE Trans. Power Electron. 2007, 22, 613–625. [Google Scholar] [CrossRef]


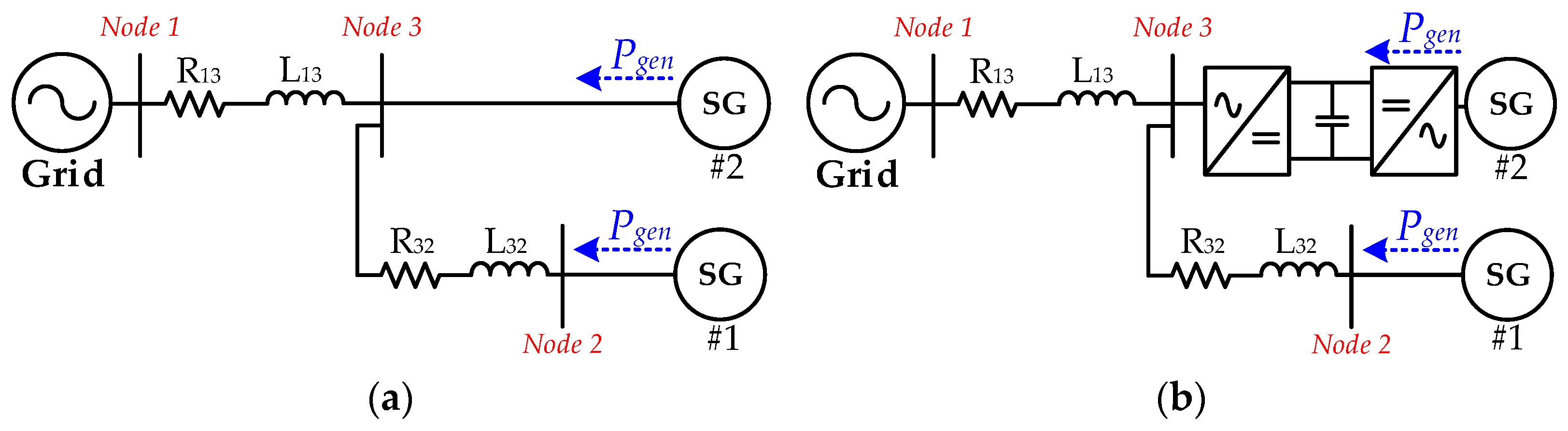
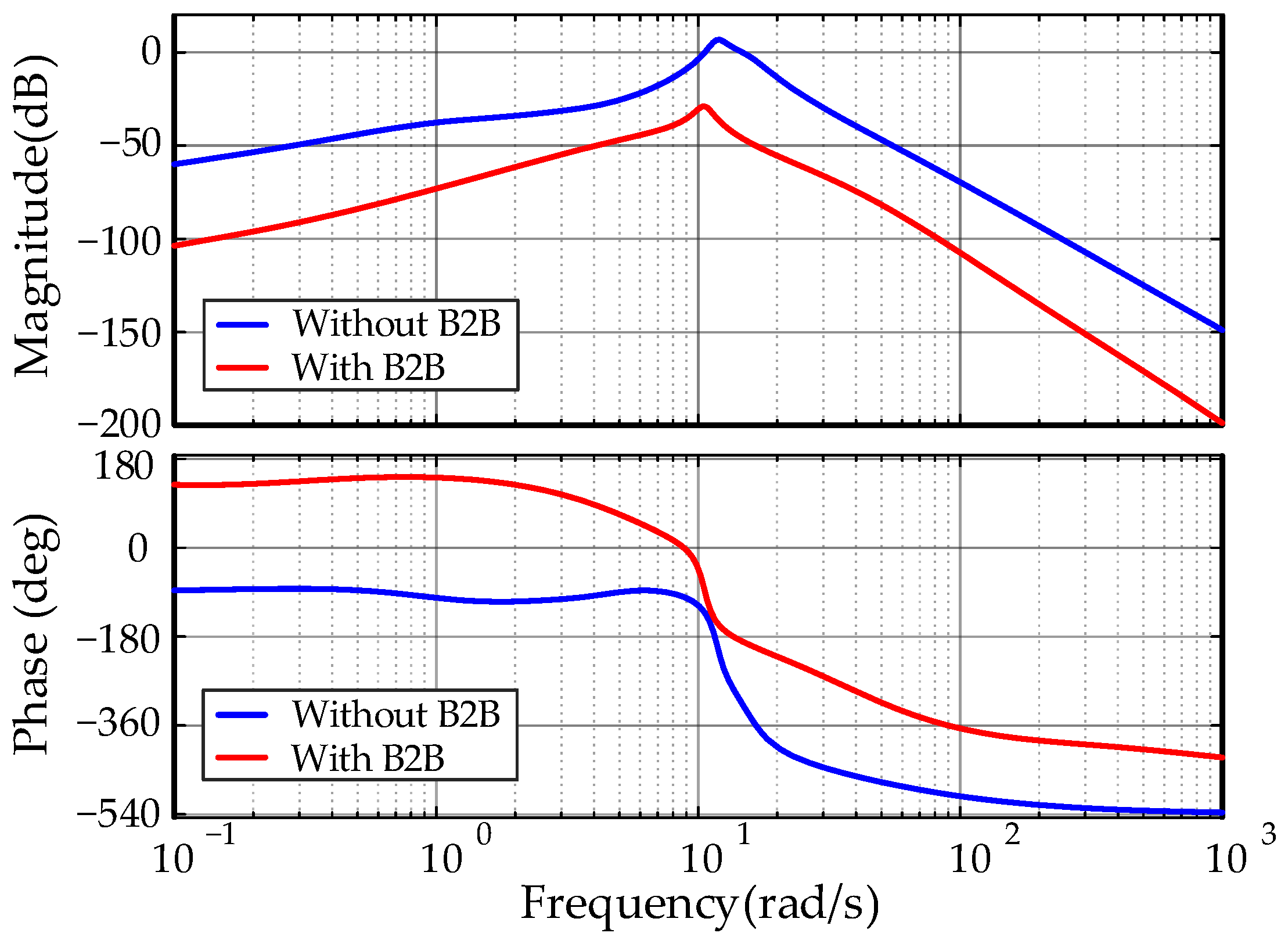


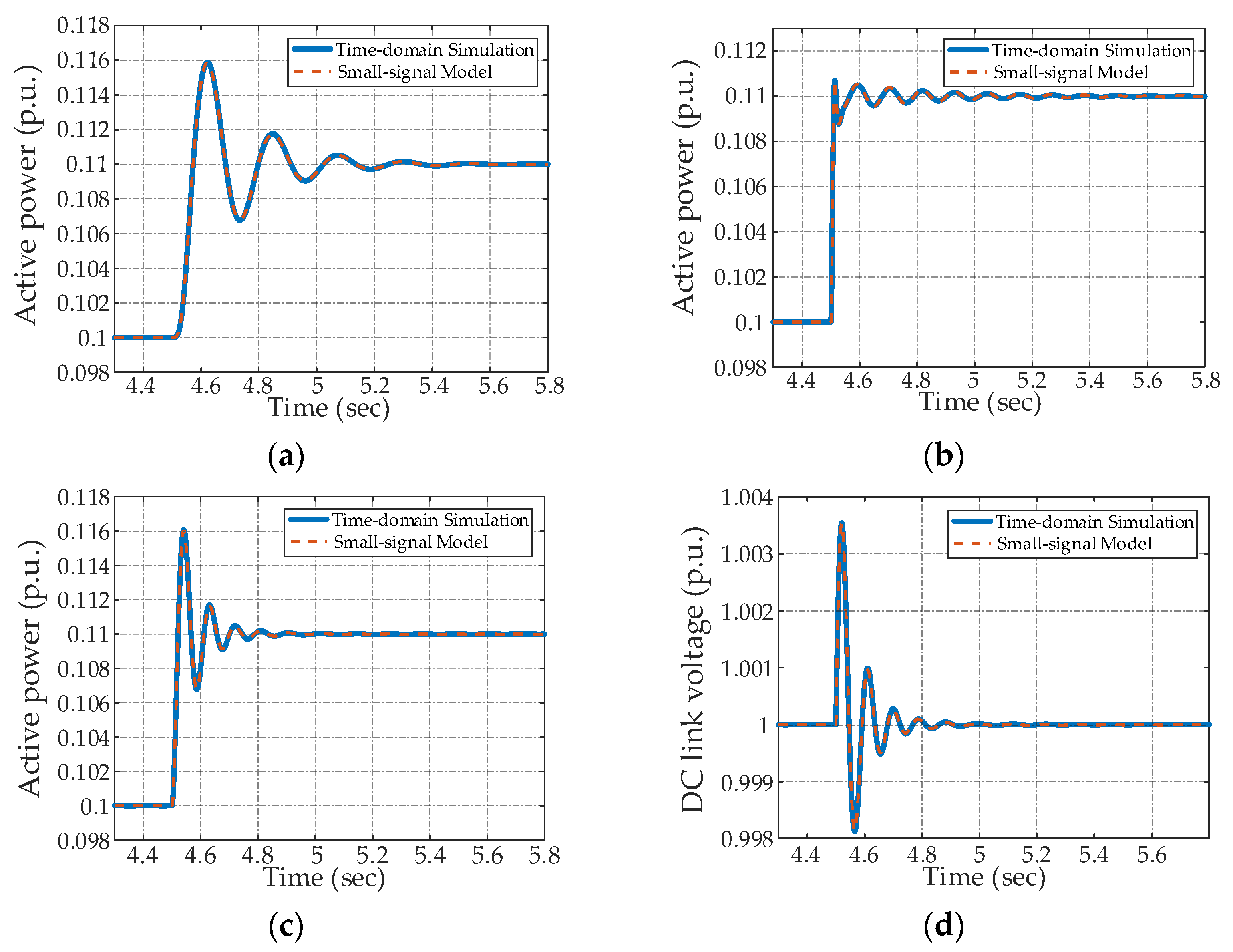
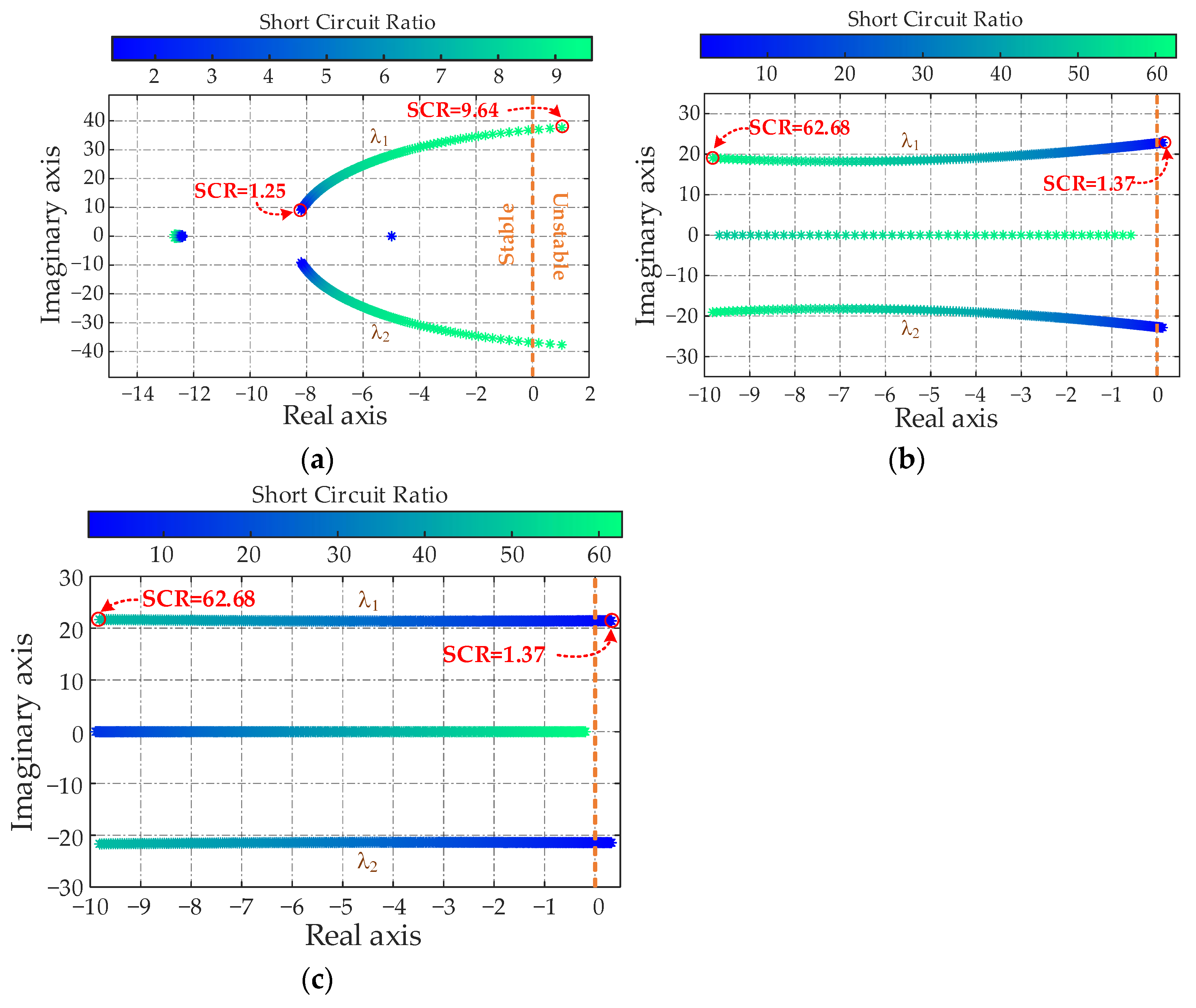
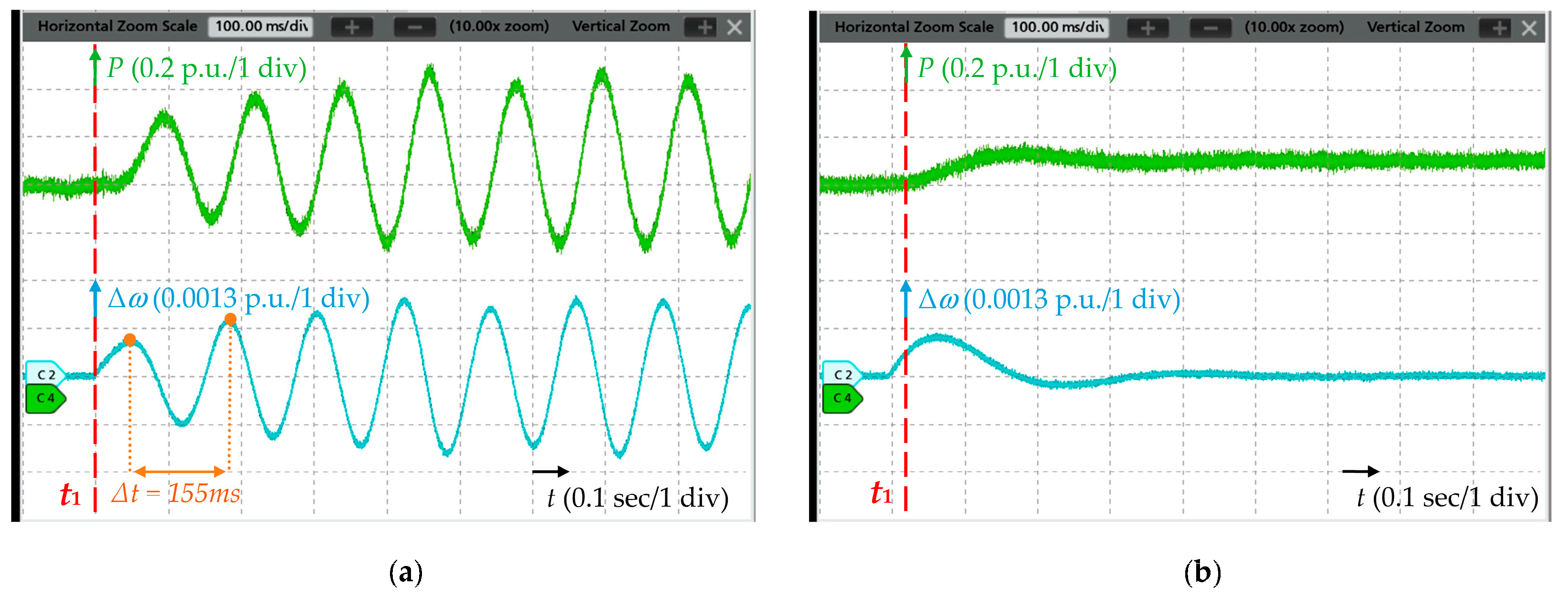
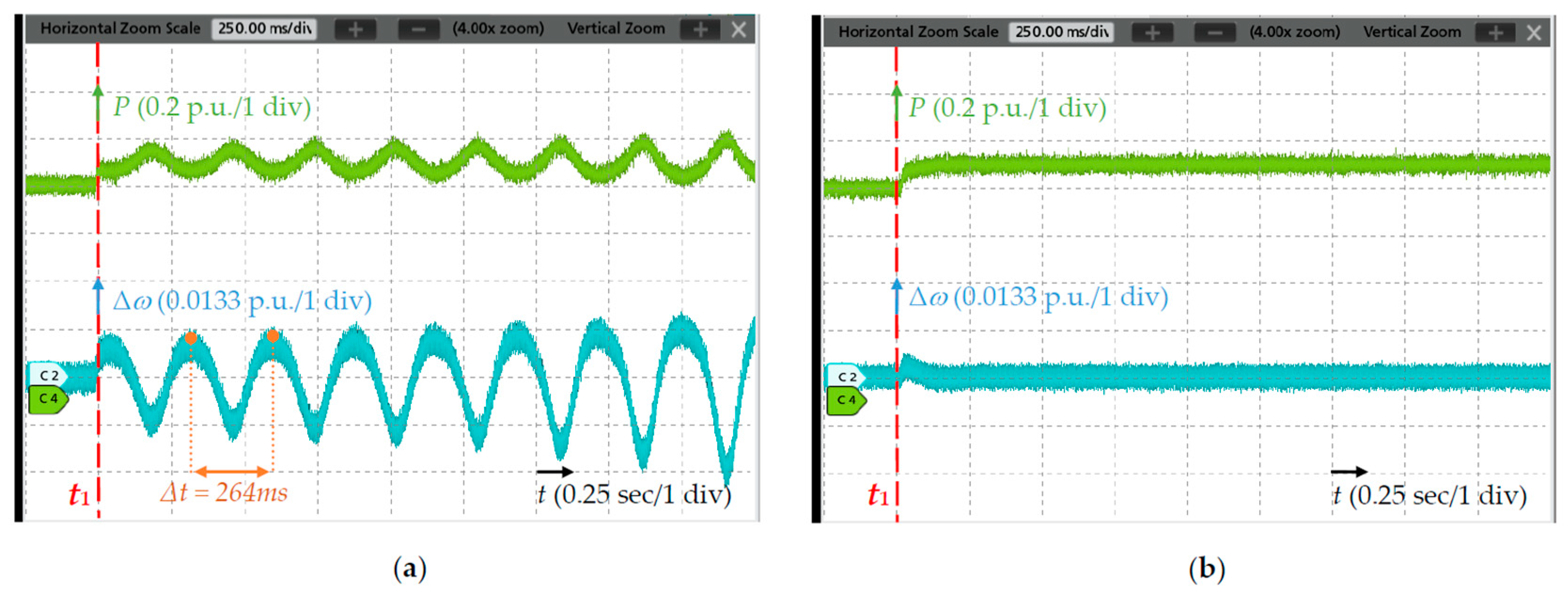
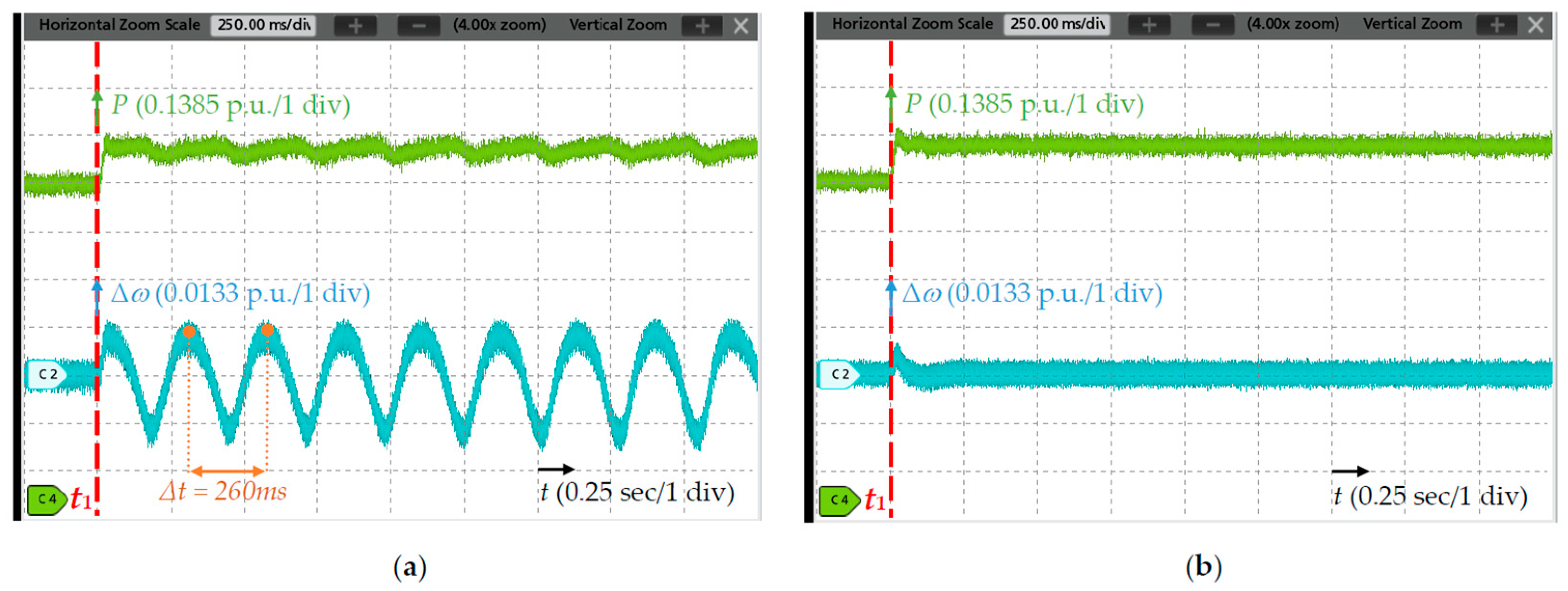
| Node | Linear Load (MVA) | Nonlinear Load (MVA) | Pgen = 1 MVA | Pgen = 2.5 MVA | ||
|---|---|---|---|---|---|---|
| Vmag (p.u.) | THD (%) | Vmag (p.u.) | THD (%) | |||
| 1 | 0.0264 | 0.006 | 1.000 | 0.02 | 1.001 | 0.02 |
| 2 | 0.0180 | 0.004 | 1.002 | 0.06 | 1.005 | 0.05 |
| 3 | 0.0102 | 0.002 | 1.018 | 0.32 | 1.049 | 0.33 |
| 4 | 0.305 | 0.0265 | 1.028 | 0.47 | 1.084 | 0.49 |
| Node | Linear Load (MVA) | Nonlinear Load (MVA) | Vmag (p.u.) | THD (%) |
|---|---|---|---|---|
| 1 | 0.0264 | 0.006 | 1.001 | 0.13 |
| 2 | 0.0180 | 0.004 | 1.003 | 0.54 |
| 3 | 0.0102 | 0.002 | 1.028 | 4.40 |
| 4 | 0.305 | 0.513 | 1.055 | 6.84 |
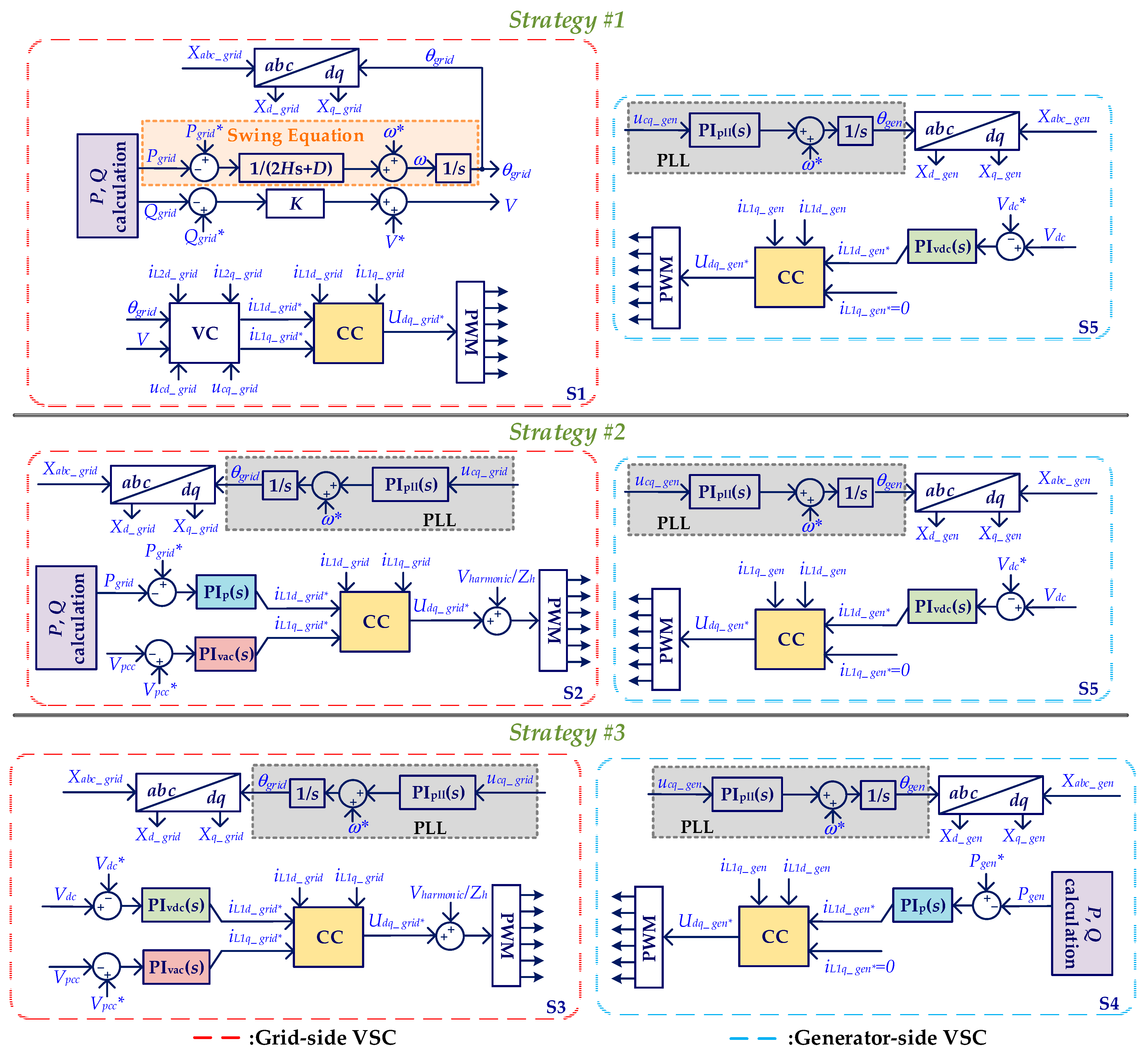
| Generator-Side VSC | Control Objectives | Advantages |
|---|---|---|
| d-axis current reference | DC link voltage | During grid faults, there is no need for additional devices, such as DC choppers or energy storage units, to sustain the DC link voltage [42,43,44,45]. |
| Active power | When connected to certain energy sources with unpredictable operating points, such as wind turbine, the output power can be appropriately controlled [46,47]. | |
| q-axis current reference | Typically set to 0 | Enable the generator-side VSC to operate at unity power factor. |
| Grid-Side VSC | Control Objectives | Advantages | |
|---|---|---|---|
| Grid-following (GFL) | d-axis current reference | DC link voltage | When the generator-side VSC is connected to energy sources with varying operating points influenced by environmental conditions, the grid-side VSC can effectively maintain the DC link voltage [48,49]. |
| Active power | The active power injected to the grid can be accurately regulated [50,51]. | ||
| q-axis current reference | AC voltage magnitude | Grid-side VSC can regulate the output AC voltage [52,53]. | |
| Grid current | The power factor of the output power is controllable [54,55]. | ||
| Grid-forming (GFM) | dq-axis current references | AC voltage | Empowered by GFM control, power generation systems can seamlessly transition between grid-connected and islanded modes while emulating virtual inertia to stabilize frequency [56,57]. |
| Grid-Side VSC | Generator-Side VSC | |
|---|---|---|
| Strategy #1 | AC voltage magnitude and phase angle (GFM)—S1 | DC link voltage (GFL)—S5 |
| Strategy #2 | Active power and AC voltage magnitude (GFL)—S2 | DC link voltage (GFL)—S5 |
| Strategy #3 | DC link voltage and AC voltage magnitude (GFL)—S3 | Active power (GFL)—S4 |
| Without B2B | With B2B | |||
|---|---|---|---|---|
| Strategy #1 | Strategy #2 | Strategy #3 | ||
| Vmag (p.u.) | 1.055 | 1.009 | 1 | 1 |
| THD (%) | 6.84 | 4.45 | 3.33 | 3.48 |
| Strategy #1 | Strategy #2 | Strategy #3 | |
|---|---|---|---|
| SCR ≤ 1.37 | Stable | Unstable | Unstable |
| 1.37 < SCR < 9.64 | Stable | Stable | Stable |
| SCR ≥ 9.64 | Unstable | Stable | Stable |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, W.; Liu, R.; Li, Y. Power Quality Enhancement of Remote Gas Field Generations with Smart Power Converters. Energies 2023, 16, 6684. https://doi.org/10.3390/en16186684
Li W, Liu R, Li Y. Power Quality Enhancement of Remote Gas Field Generations with Smart Power Converters. Energies. 2023; 16(18):6684. https://doi.org/10.3390/en16186684
Chicago/Turabian StyleLi, Wenze, Rui Liu, and Yunwei Li. 2023. "Power Quality Enhancement of Remote Gas Field Generations with Smart Power Converters" Energies 16, no. 18: 6684. https://doi.org/10.3390/en16186684
APA StyleLi, W., Liu, R., & Li, Y. (2023). Power Quality Enhancement of Remote Gas Field Generations with Smart Power Converters. Energies, 16(18), 6684. https://doi.org/10.3390/en16186684




