Reaction Temperature Manipulation as a Process Intensification Approach for CO2 Absorption
Abstract
:1. Introduction
2. Materials and Methods
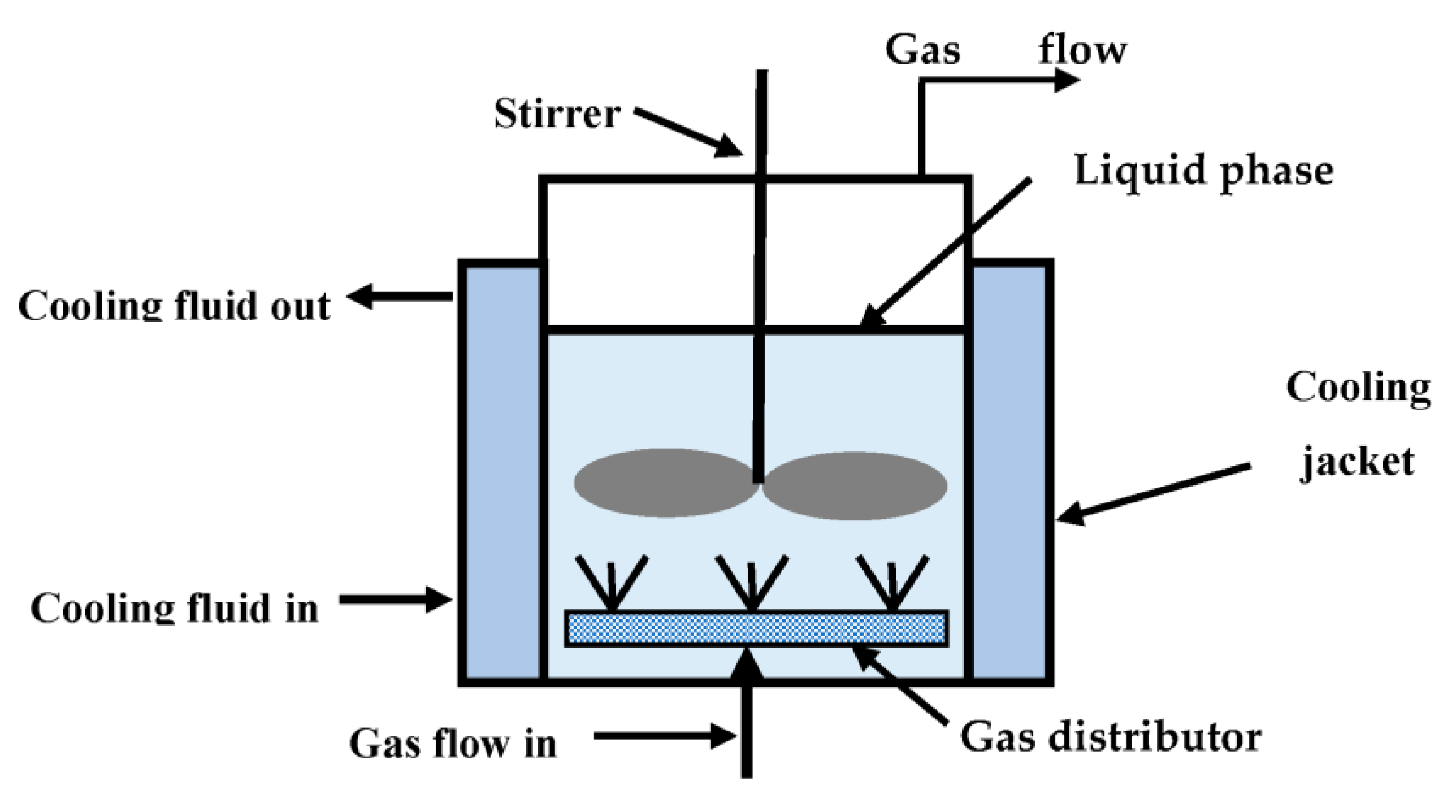
2.1. Chemical Reactor Mass Balance Model
2.2. Chemical Reactor Energy Balance Model
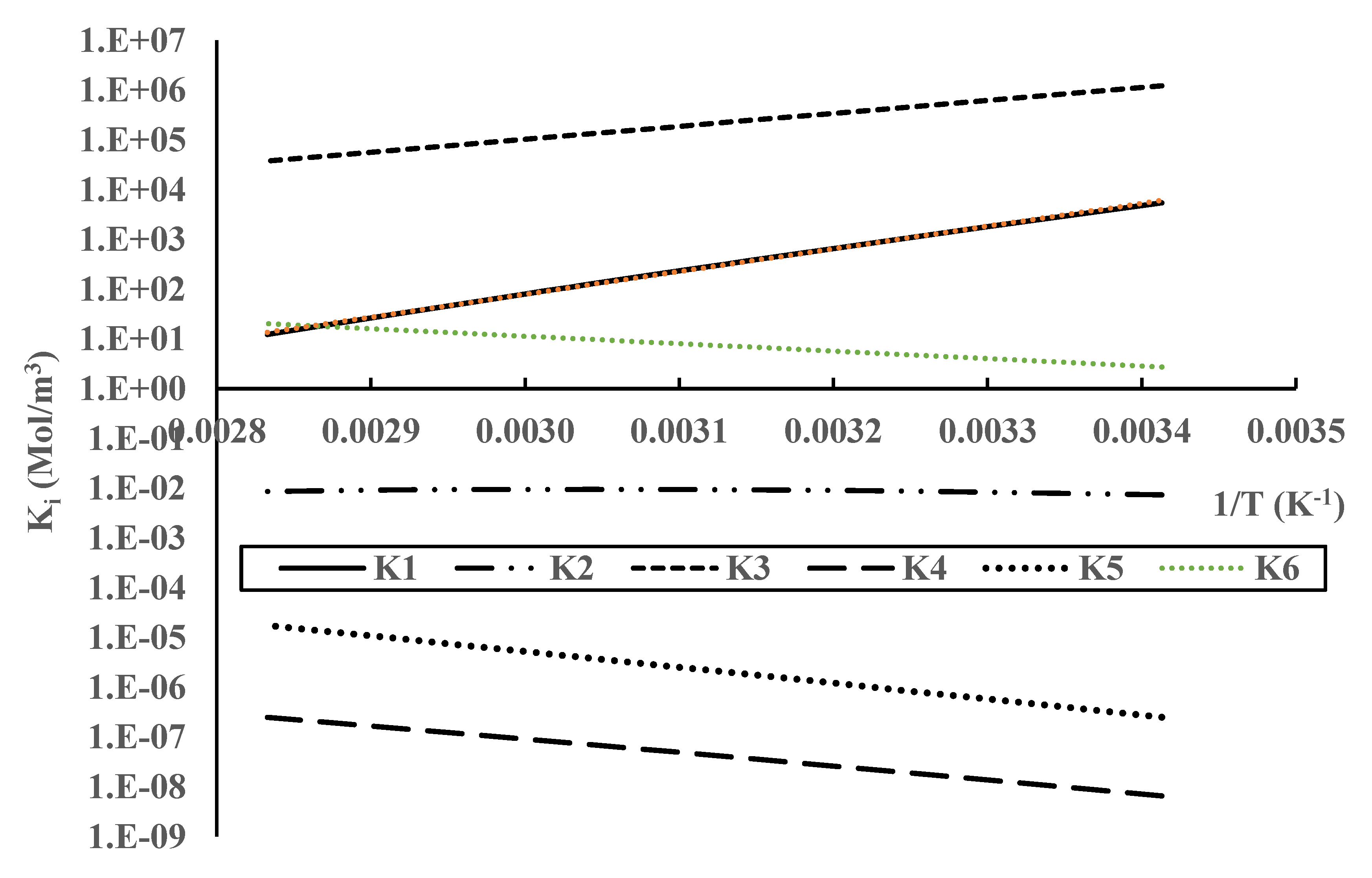
2.3. Parameter Estimation
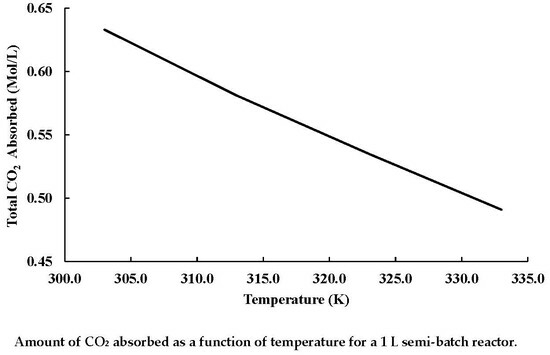
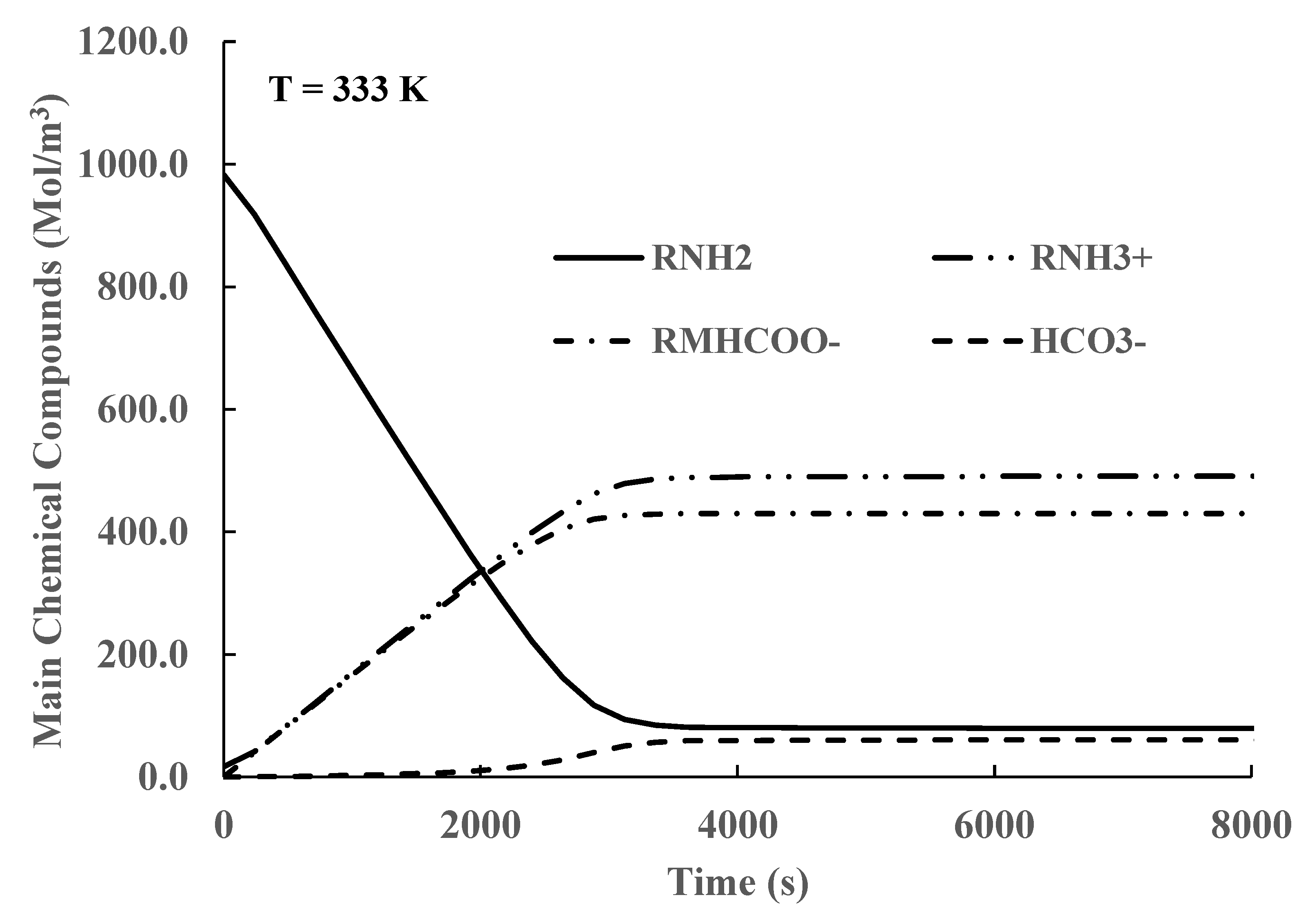
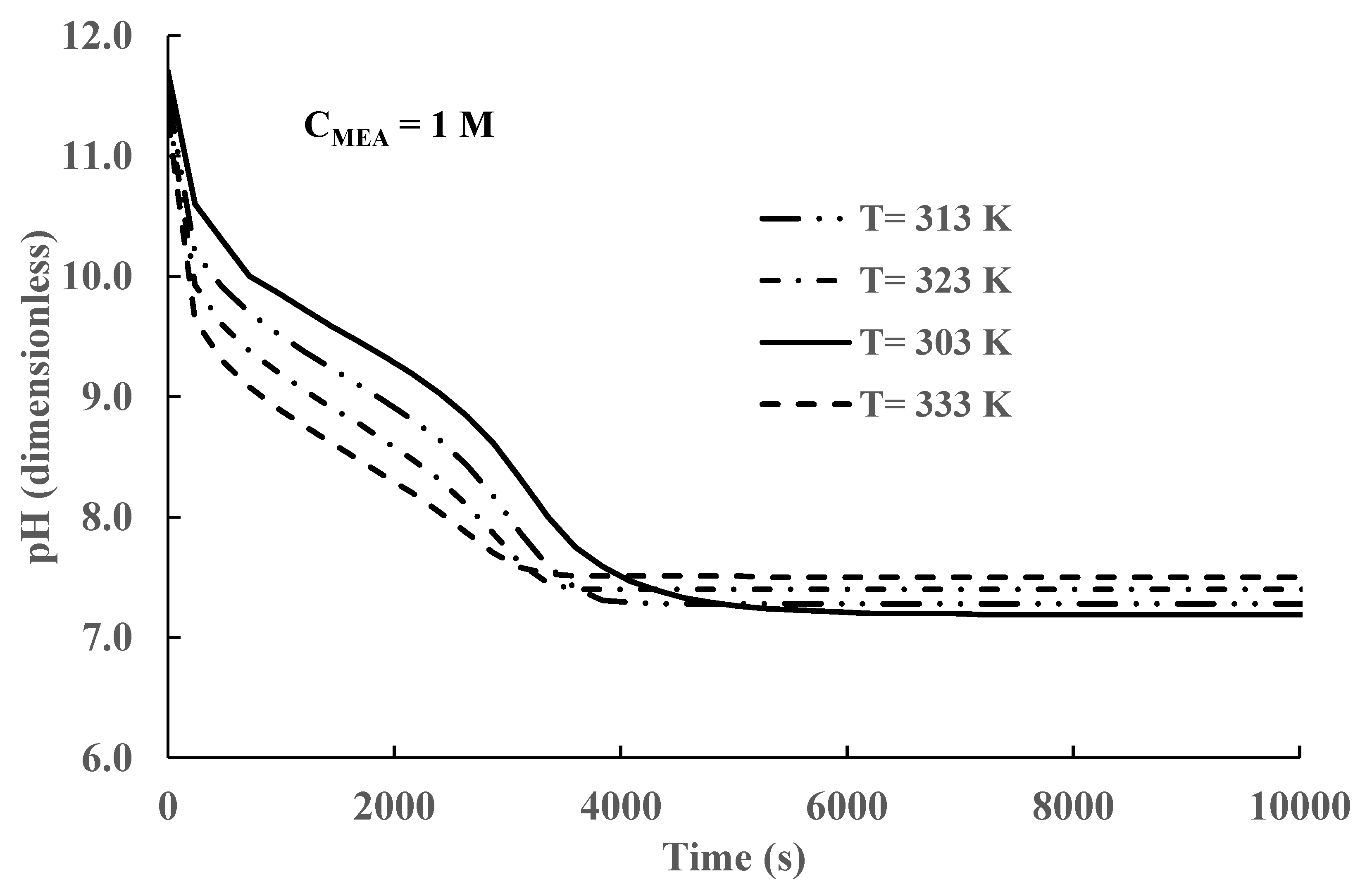
3. Results and Discussion
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Notice
Nomenclature
| aw | Interface area per unit volume (m−1) |
| bi | Blade height (m) |
| Concentration of i-species in k-phase (mol m−3) | |
| Species-i gas phase concentration (mol m−3) | |
| Species-i liquid phase concentration (mol m−3) | |
| Species-i liquid phase equilibrium concentration (mol m−3) | |
| Input gas phase concentrations of i-component (mol m−3) | |
| Output gas phase concentrations of i-species (mol m−3) | |
| Heat capacities of the i-component (J mol−1 K−1) | |
| Heat capacities of the i-component (J mol−1 K−1) | |
| Gas bubble Sauter diameter (m) | |
| Column diameter (m) | |
| DCO2 | CO2 diffusion coefficient (m2 s−1) |
| di | Impeller diameter (m) |
| dT | Reactor diameter (m) |
| Gas phase hold-up (Vg/VT) | |
| E | Enhancement factor (dimensionless) |
| Gas flow number (dimensionless | |
| H | Reactor height (m) |
| Liquid-side CO2 mass transfer coefficient (m s−1) | |
| Forward specific reaction rate constant (m3 mol−1 s−1) | |
| Reverse specific rate constant (m3 mol−1 s−1) | |
| Ki | Equilibrium constant for i-reaction (variable) |
| MEA | Monoethanol amine |
| N | Rotational speed (rps) |
| Molar flow of component-i from the gas into the liquid phase (mol m−3 s−1) | |
| Power consumed by the stirrer with gas phase present (W) | |
| pH | Liquid phase pH (dimensionless) |
| Power consumed by the stirrer without gas phase (W) | |
| Q | Heat of reaction (J mol−1) |
| Qg | Gas flow rate (m3 s−1) |
| R | Alkyl group |
| Mols of species-i generated by chemical reaction (mol m−3 s−1) | |
| Temperature of the gas phase (K) | |
| Temperature of the input gas stream (K) | |
| Temperature of the liquid stream (K) | |
| Global heat transfer coefficient (J m−2 K−1 s−1) | |
| Gas superficial velocity (m s−1) | |
| Liquid superficial velocity (m s−1) | |
| Liquid phase volume (m3) | |
| Total reactor volume (m3) | |
| Greek letters | |
| Heat of vaporization for the i-species (J mol−1) | |
| Heat released by chemical reaction (J mol−1) | |
| Liquid phase density (kg m−3) | |
| Liquid phase surface tension (N m−1) |
Appendix A
| Compound No | Gas | Liquid |
|---|---|---|
| 1 | N2 (g) | MEACO2− |
| 2 | O2 (g) | MEA+ |
| 3 | CO2 (g) | HCO3− |
| 4 | H2O (g) | OH− |
| 5 | MEA (g) | MEA (aq.) |
| 6 | - | CO2 (aq.) |
| 7 | - | H+ |
| 8 | - | CO32− |
References
- IPCC. 2022: Summary for Policymakers. In Climate Change 2022: Mitigation of Climate Change. Contribution of Working Group III to the Sixth Assessment Report of the Intergovernmental Panel on Climate Change; Shukla, P.R., Skea, J., Slade, R., Al Khourdajie, A., van Diemen, R., McCollum, D., Pathak, M., Some, S., Vyas, P., Fradera, R., et al., Eds.; Cambridge University Press: Cambridge, UK; New York, NY, USA, 2022. [Google Scholar] [CrossRef]
- Masson-Delmotte, V.; Zhai, P.; Pörtner, H.-O.; Roberts, D.; Skea, J.; Shukla, P.R.; Pirani, A.; Péan, W.M.-O.C.; Pidcock, R.; Connors, S.; et al. (Eds.) Global Warming of 1.5 °C. An IPCC Special Report on the Impacts of Global Warming of 1.5 °C above Pre-Industrial Levels and Related Global Greenhouse Gas Emission Pathways, in the Context of Strengthening the Global Response to the Threat of Climate Change, Sustainable Development, and Efforts to Eradicate Poverty; IPCC: Geneva, Switzerland, 2018. [Google Scholar]
- Aaron, D.; Tsouris, C. Separation of CO2 from Flue Gas: A Review. Sep. Sci. Technol. 2005, 40, 321–348. [Google Scholar] [CrossRef]
- Greer, T. Modeling and Simulation of Post Combustion CO2 Capturing. Master’s Thesis, Telemark University College, Faculty of Technology, Porsgrunn, Norway, 2008. [Google Scholar]
- Bosch, H.; Versteeg, G.F.; van SwaaiJ, W.P.M. Gas-Liquid Mass Transfer with Parallel Reversible Reactions-III. Absorption of CO2 into Solutions of Blends of Amines. Chem. Eng. Sci. 1989, 44, 2745–2750. [Google Scholar]
- Hagewiesche, D.P.; Ashour, S.S.; Al-Ghawas, H.A.; Sandall, O.C. Absorption of Carbon Dioxide into Aqueous Blends of Monoethanolamine and Methyldiethanolamine. Chem. Eng. Sci. 1995, 50, 1071–1079. [Google Scholar] [CrossRef]
- Sakwattanapong, R.; Aroonwilas, A.; Veawab, A. Reaction Rate of CO2 in Aqueous MEA-AMP Solution: Experiment and Modeling. Energy Procedia 2009, 1, 217–224. [Google Scholar] [CrossRef]
- Gabitto, J.; Tsouris, C. Carbon Dioxide Absorption Modeling for Off-Gas Treatment in the Nuclear Fuel Cycle. Int. J. Chem. Eng. 2018, 2018, 3158147. [Google Scholar] [CrossRef]
- Kasturi, A.S.; Ladshaw, A.; Yiacoumi, S.; Gabitto, J.; Garrabrant, K.; Custelcean, R.; Tsouris, C. CO2 Absorption from Simulated Flue Gas in a Bubble Column. Sep. Sci. Technol. 2019, 54, 2034–2046. [Google Scholar] [CrossRef]
- Versteeg, G.F.; Holst, J.V.; Politiek, P.P.; Niederer, J.P. CO2 Capture from Flue Gas Using Amino Acid Salt Solutions. June 2006. Available online: http://www.co2-cato.nl/doc.php?lid=317 (accessed on 9 April 2018).
- van Holst, J.; Versteeg, G.F.; Brilman, D.W.F.; Hogendoorn, J.A. Kinetic Study of CO2 with Various Amino Acid Salts in Aqueous Solution. Chem. Eng. Sci. 2009, 64, 59–68. [Google Scholar] [CrossRef]
- Vaidya, P.D.; Konduru, P.; Vaidyanathan, M.; Kenig, E.Y. Kinetics of Carbon Dioxide Removal by Aqueous Alkaline Amino Acid salts. Ind. Eng. Chem. Res. 2010, 49, 11067–11072. [Google Scholar] [CrossRef]
- Simons, K.; Brilman, D.W.F.; Mengers, H.; Nijmeijer, K.; Wessling, M. Kinetics of CO2 Absorption in Aqueous Sarcosine Salt Solutions: Influence of Concentration, Temperature, and CO2 Loading. Ind. Eng. Chem. Res. 2010, 49, 9693–9702. [Google Scholar] [CrossRef]
- Shen, S.; Yang, Y.N.; Bian, Y.; Zhao, Y. Kinetics of CO2 Absorption into Aqueous Basic Amino Acid Salt: Potassium Salt of Lysine Solution. Environ. Sci. Technol. 2016, 50, 2054–2063. [Google Scholar] [CrossRef]
- Seipp, C.A.; Williams, N.J.; Kidder, M.K.; Custelcean, R. CO2 Capture from Ambient Air by Crystallization with a Guanidine Sorbent. Angew. Chem. Int. Ed. 2017, 56, 1042–1045. [Google Scholar] [CrossRef] [PubMed]
- Brethomé, F.M.; Williams, N.J.; Seipp, C.A.; Kidder, M.K.; Custelcean, R. Direct Air Capture of CO2 via Aqueous-Phase Absorption and Crystalline-Phase Release using Concentrated Solar Power. Nat. Energy 2018, 3, 553–559. [Google Scholar] [CrossRef]
- Williams, N.J.; Seipp, C.A.; Brethomé, F.M.; Ma, Y.-Z.; Ivanov, A.S.; Bryantsev, V.S.; Kidder, M.K.; Martin, H.J.; Holguin, E.; Garrabrant, K.A.; et al. CO2 Capture via Crystalline Hydrogen-Bonded Bicarbonate Dimers. Chem 2019, 5, 719–730. [Google Scholar] [CrossRef]
- Custelcean, R.; Williams, N.J.; Garrabrant, K.A.; Agullo, P.; Brethomé, F.M.; Martin, H.J.; Kidder, M.K. Direct Air Capture of CO2 with Aqueous Amino Acids and Solid Bis-iminoguanidines (BIGs). Ind. Eng. Chem. Res. 2019, 58, 23338–23346. [Google Scholar] [CrossRef]
- Mahmud, N.; Benamor, A.; Nasser, M.S.; Al-Marri, M.J.; Qiblawey, H.; Tontiwachwuthikul, P. Reaction Kinetics of Carbon Dioxide with Aqueous Solutions of L-Arginine, Glycine & Sarcosine using the Stopped Flow Technique. Int. J. Greenh. Gas Control 2017, 63, 47–58. [Google Scholar]
- Kasturi, A.; Gabitto, J.F.; Custelcean, R.; Tsouris, C. A Process Intensification Approach for CO2 Absorption using Amino Acid Solutions and a Guanidine Compound. Energies 2021, 14, 5821. [Google Scholar] [CrossRef]
- Kim, Y.-H.; Park, L.K.; Yiacoumi, S.; Tsouris, C. Modular Chemical Process Intensification: A Review. Annu. Rev. Chem. Biomol. Eng. 2017, 8, 359–380. [Google Scholar] [CrossRef]
- Le Chatelier, H.L. Sur un énoncé générale des lois des équlibres chimiques. Comptes Rendus Académie Sci. 1884, 99, 786–789. [Google Scholar]
- Hillert, M. Le Chatelier’s Principle—Restated and Illustrated with Phase Diagrams. JPE 1995, 16, 403–410. [Google Scholar] [CrossRef]
- Fogler, H.S. Elements of Chemical Reaction Engineering, 5th ed.; Prentice Hall: Upper Saddle River, NJ, USA, 2016. [Google Scholar]
- Jamal, A.; Meisen, A.; Lim, C.J. Kinetics of Carbon Dioxide Absorption and Desorption in Aqueous Alkanolamine Solutions using a Novel Hemispherical Contactor—I. Experimental Apparatus and Mathematical Modeling. Chem. Eng. Sci. 2006, 61, 6571–6589. [Google Scholar]
- Jamal, A.; Meisen, A.; Lim, C.J. Kinetics of Carbon Dioxide Absorption and Desorption in Aqueous Alkanolamine Solutions using a Novel Hemispherical Contactor—II. Experimental Results and Parameter Estimation. Chem. Eng. Sci. 2006, 61, 6590–6603. [Google Scholar]
- Jang, G.G.; Thompson, J.A.; Sun, X.; Tsouris, C. Process Intensification of CO2 Capture by Low-Aqueous Solvent. Chem. Eng. J. 2021, 426, 131240. [Google Scholar] [CrossRef]
- Tsouris, C.; Jang, G.G.; Thompson, J.A.; Lai, C.; Sun, X. Demonstration and Validation of Additively Manufactured Intensified Device for Enhanced Carbon Capture; Oak Ridge National Lab.: Oak Ridge, TN, USA, 2021. [Google Scholar] [CrossRef]
- Kvamsdal, H.M.; Rochelle, G.T. Effects of the Temperature Bulge in CO2 Absorption from Flue Gas by Aqueous Monoethanolamine. Ind. Eng. Res. 2008, 47, 867–875. [Google Scholar] [CrossRef]
- Kvamsdal, H.M.; Jakobsen, J.P.; Hoff, K.A. Dynamic Modeling and Simulation of a CO2 Absorber Column for Post-Combustion CO2 Capture. Chem. Eng. Process. Process Intensif. 2009, 48, 135–144. [Google Scholar] [CrossRef]
- Bailey, J.E.; Ollis, D.F. Biochemical Engineering Fundamentals, 2nd ed.; McGraw-Hill: New York, NY, USA, 1986. [Google Scholar]
- Zhang, J.; Smith, R. Design and Optimization of Batch and Semi-Batch Reactors. Chem. Eng. Sci. 2004, 59, 459–478. [Google Scholar] [CrossRef]
- Katoh, S.; Horiuchi, J.-I.; Yoshida, F. Biochemical Engineering, 2nd ed.; Wiley & Sons, WILEY-VCH Verlag GmbH & Co. KgaA: Weinheim, Germany, 2015. [Google Scholar]
- Gabitto, J.; Custelcean, R.; Tsouris, C. Simulation of Carbon Dioxide Absorption by Amino Acids in Two-Phase Batch and Bubble Column Reactors. Sep. Sci. Technol. 2019, 54, 2013–2015. [Google Scholar] [CrossRef]
- Morsi, B.I.; Basha, O.M. Mass Transfer in Multiphase Systems. In Mass Transfer-Advancement in Process Modelling; Solecki, M., Ed.; IntechOpen: London, UK, 2017. [Google Scholar] [CrossRef]
- Perry, R.H.; Green, D.W. Chemical Engineers Handbook, 7th ed.; McGraw-Hill: New York, NY, USA, 1999. [Google Scholar]
- Richardson, J.H.; Harker, J.H.; Backhurst, J.R. Chemical Engineering. Vol. 2, Particle Technology and Separation Processes, 5th ed.; Butterworth-Heinemann: Oxford, UK, 2008. [Google Scholar]
- Bouaifi, M.; Hebrard, G.; Bastoul, D.; Roustan, M. A Comparative Study of Gas Hold-Up, Bubble Size, Interfacial Area and Mass Transfer Coefficients in Stirred Gas-Liquid Reactors and Bubble Columns. Chem. Eng. Process. 2001, 40, 97–111. [Google Scholar] [CrossRef]
- Luan, D.; Zhang, S.; Wei, X.; Chen, Y.-M. Study on Mathematical Model to Predict Aerated power Consumption in a Gas-Liquid Stirred Tank. Results Phys. 2017, 7, 4085–4088. [Google Scholar] [CrossRef]
- Lee, J.C.; Meyrick, D.L. Gas–liquid Interfacial Area in Salt Solutions in an Agitated Tank. Trans. Inst. Eng. 1970, 48, T37. [Google Scholar]
- Liu, Y.; Zhang, L.; Watanasiri, S. Representing Vapor-Liquid Equilibrium for an aqueous MEA-CO2 System using the Electrolyte Non-random Two Liquid Model. Ind. Eng. Chem. Res. 1999, 38, 2080–2090. [Google Scholar] [CrossRef]

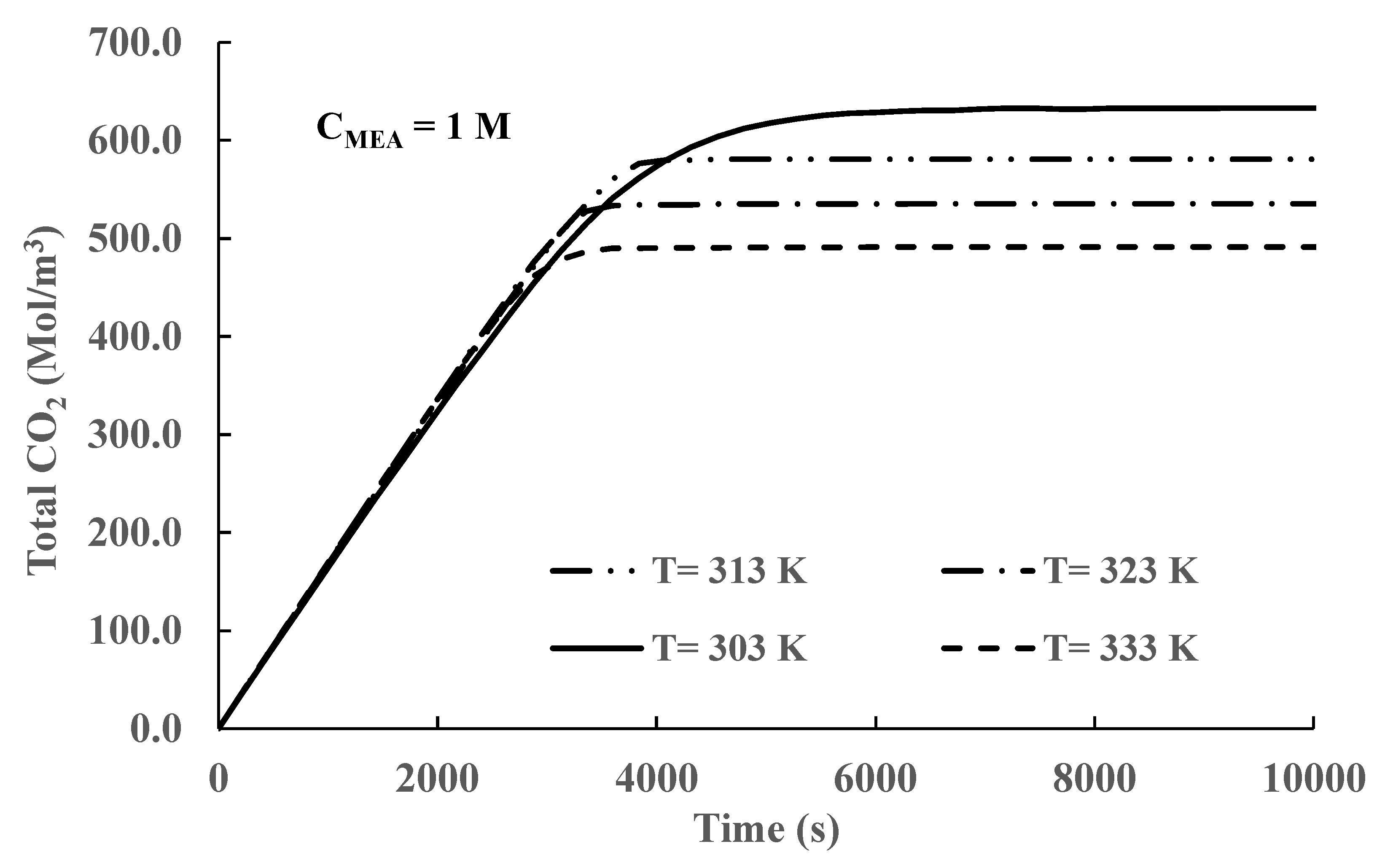
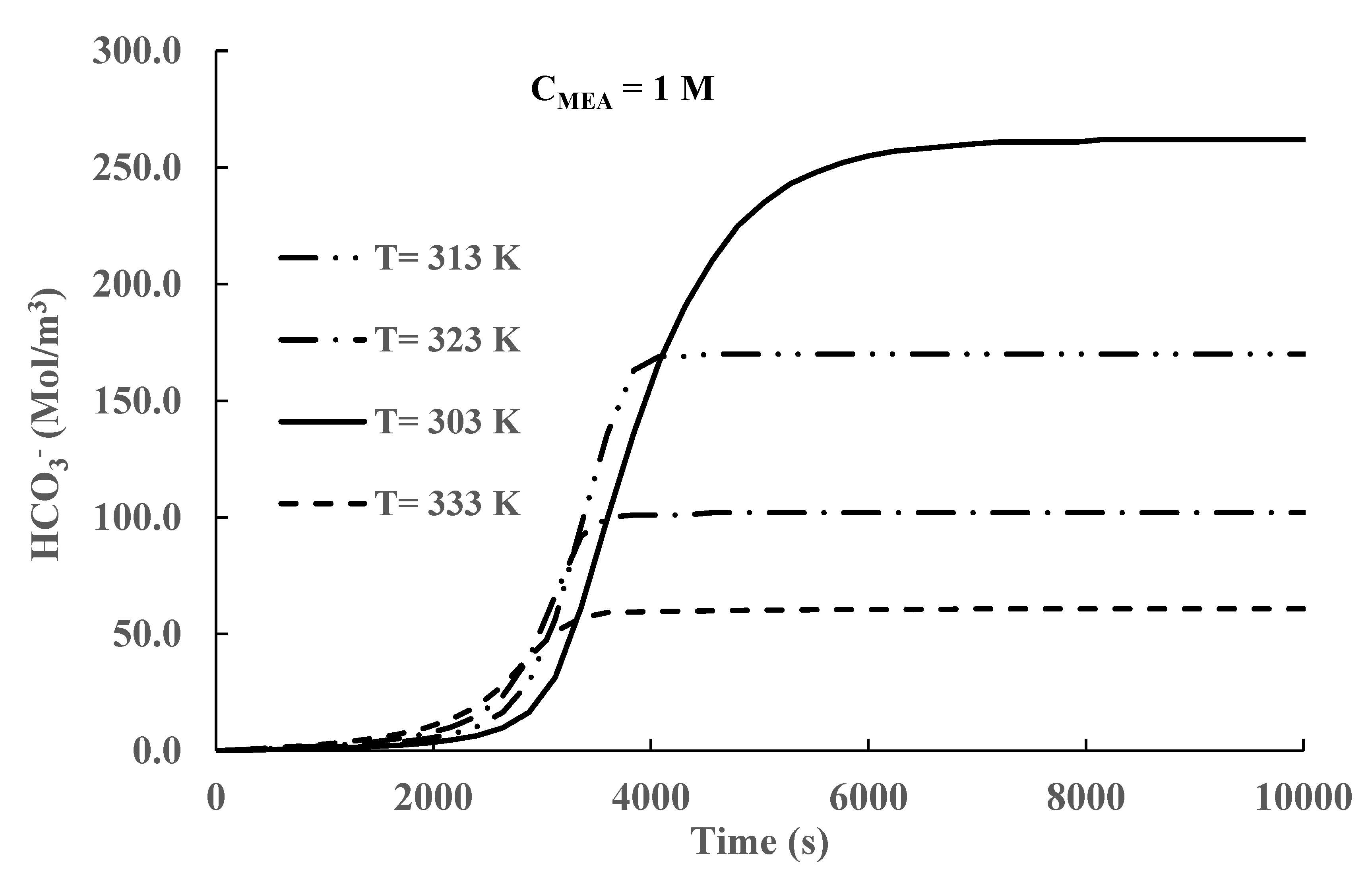
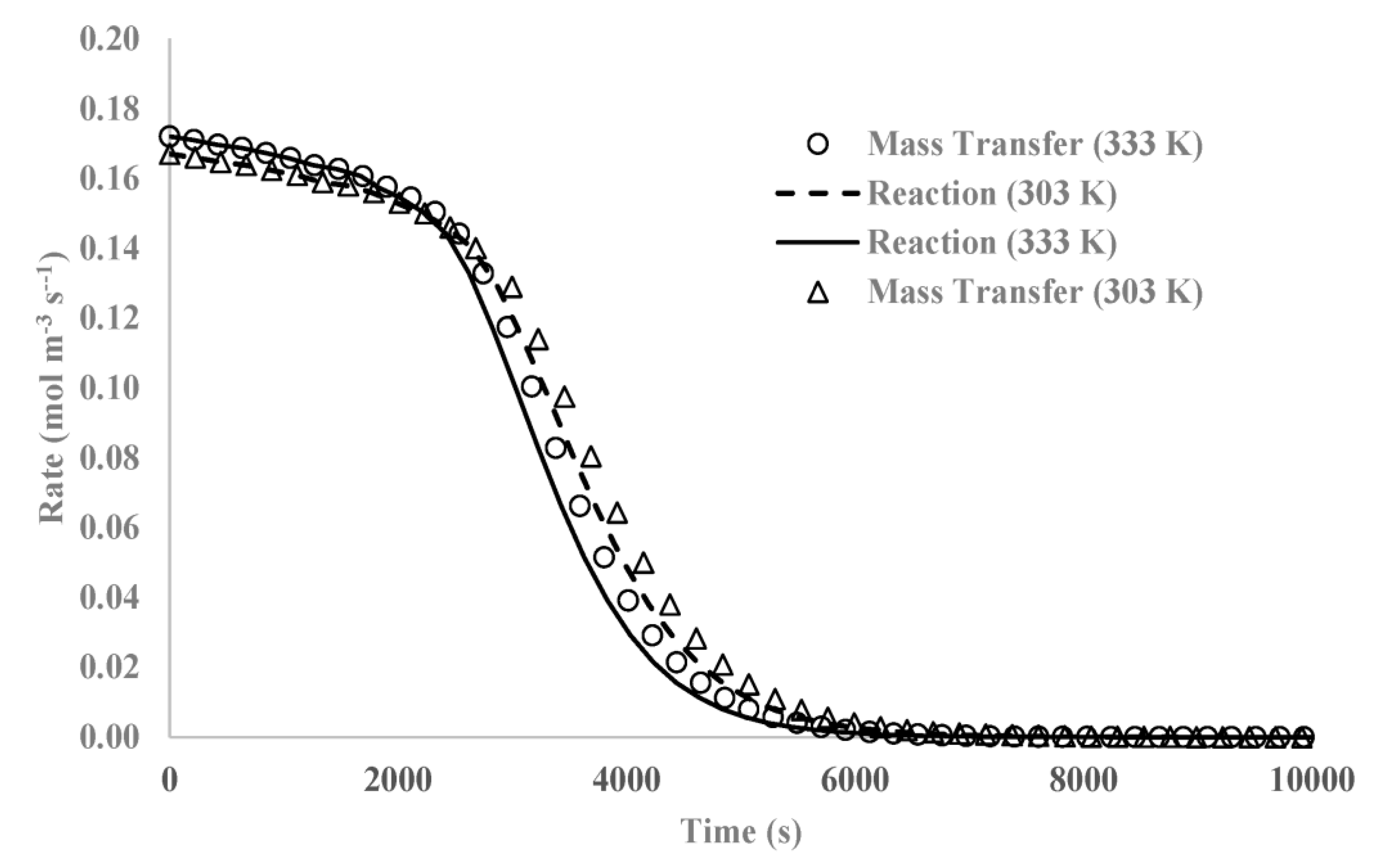
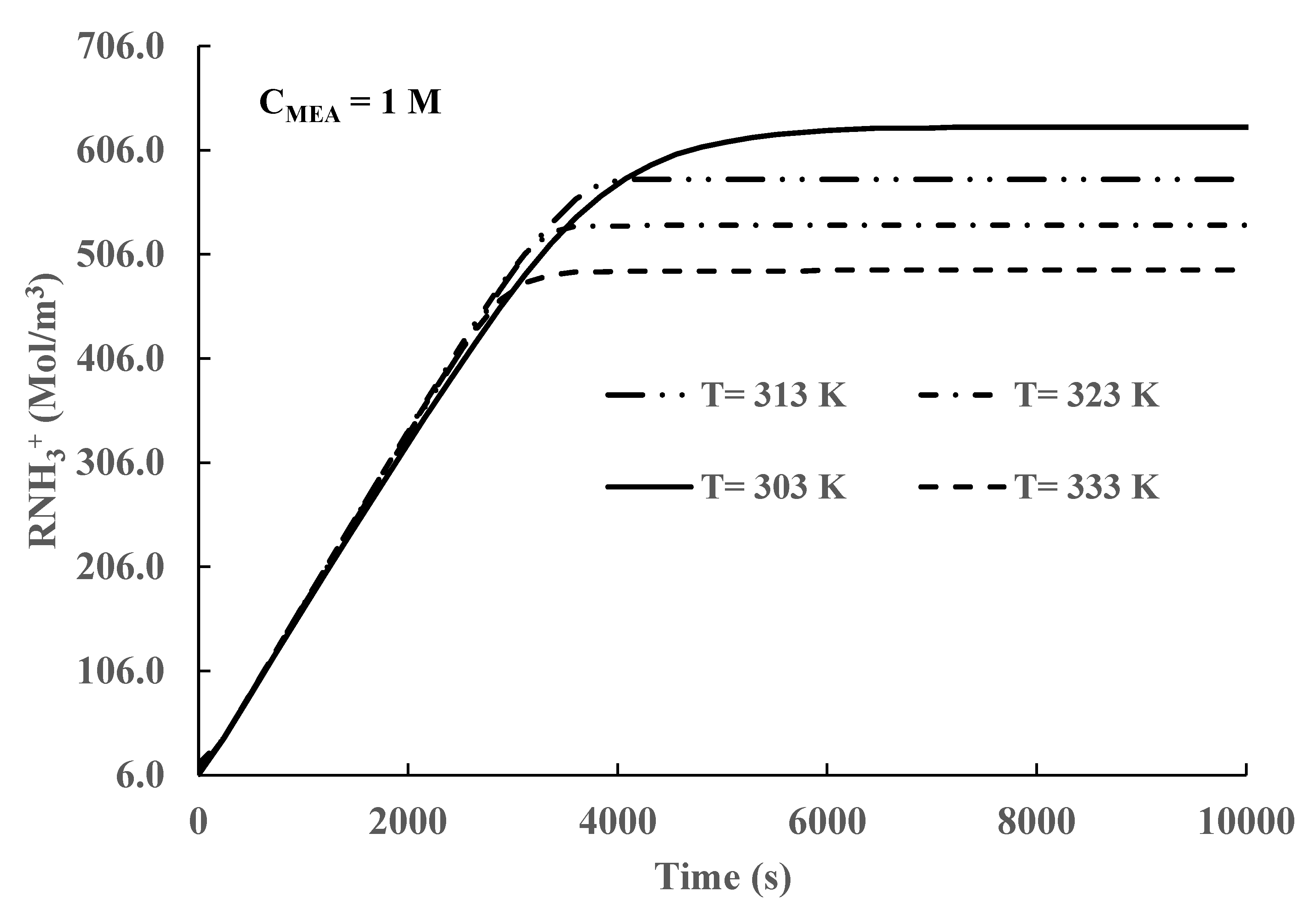



| Parameter | Units | Default Value | Range |
|---|---|---|---|
| m3 | 1.0 × 10−3 | 0.5 × 10−3–2 × 10−3 | |
| Blade Height (bi) | m | 0.02 | 0.01–0.04 |
| Impeller diameter (di) | m | 0.05 | 0.01–0.1 |
| Tank diameter (dT) | m | 0.10 | 0.05–0.20 |
| Gas flow rate (Qg) | m3/s | 3.34 × 10−5 | 1.67 × 10−5–8.34 × 10−5 |
| Input CO2 molar fraction (xCO2) | - | 0.12 | 0.02–0.2 |
| Initial pH | - | 11.7 | 10–14 |
| Rotational speed (N) | 1/s | 6.67 | 1.67–13.34 |
| Initial MEA concentration | mol/m3 | 2000 | 1000–4000 |
| CMEA (mol/m3) | Tl (K) | pH (-) | CO2 Load (-) | Total CCO2 (mol/m3) | Extra CCO2 Absorbed (%) |
|---|---|---|---|---|---|
| 1000 | 303 | 7.19 | 0.633 | 633.0 | 28.7 |
| 1000 | 313 | 7.28 | 0.581 | 581.0 | 18.1 |
| 1000 | 323 | 7.40 | 0.535 | 545.0 | 10.7 |
| 1000 | 333 | 7.50 | 0.492 | 492.0 | 0.0 |
| 2000 | 303 | 7.24 | 0.570 | 1140.0 | 25.0 |
| 2000 | 313 | 7.32 | 0.540 | 1080.0 | 18.4 |
| 2000 | 323 | 7.42 | 0.510 | 1020.0 | 11.8 |
| 2000 | 333 | 7.55 | 0.476 | 912.0 | 0.0 |
| 4000 | 303 | 7.29 | 0.535 | 2140.0 | 23.0 |
| 4000 | 333 | 7.54 | 0.435 | 1740.0 | 0.0 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gabitto, J.F.; Tsouris, C. Reaction Temperature Manipulation as a Process Intensification Approach for CO2 Absorption. Energies 2023, 16, 6522. https://doi.org/10.3390/en16186522
Gabitto JF, Tsouris C. Reaction Temperature Manipulation as a Process Intensification Approach for CO2 Absorption. Energies. 2023; 16(18):6522. https://doi.org/10.3390/en16186522
Chicago/Turabian StyleGabitto, Jorge Federico, and Costas Tsouris. 2023. "Reaction Temperature Manipulation as a Process Intensification Approach for CO2 Absorption" Energies 16, no. 18: 6522. https://doi.org/10.3390/en16186522
APA StyleGabitto, J. F., & Tsouris, C. (2023). Reaction Temperature Manipulation as a Process Intensification Approach for CO2 Absorption. Energies, 16(18), 6522. https://doi.org/10.3390/en16186522