Structural Design and Sealing Performance Analysis of a Nanofluidic Self-Heating Unsealing Rubber Cylinder
Abstract
:1. Introduction
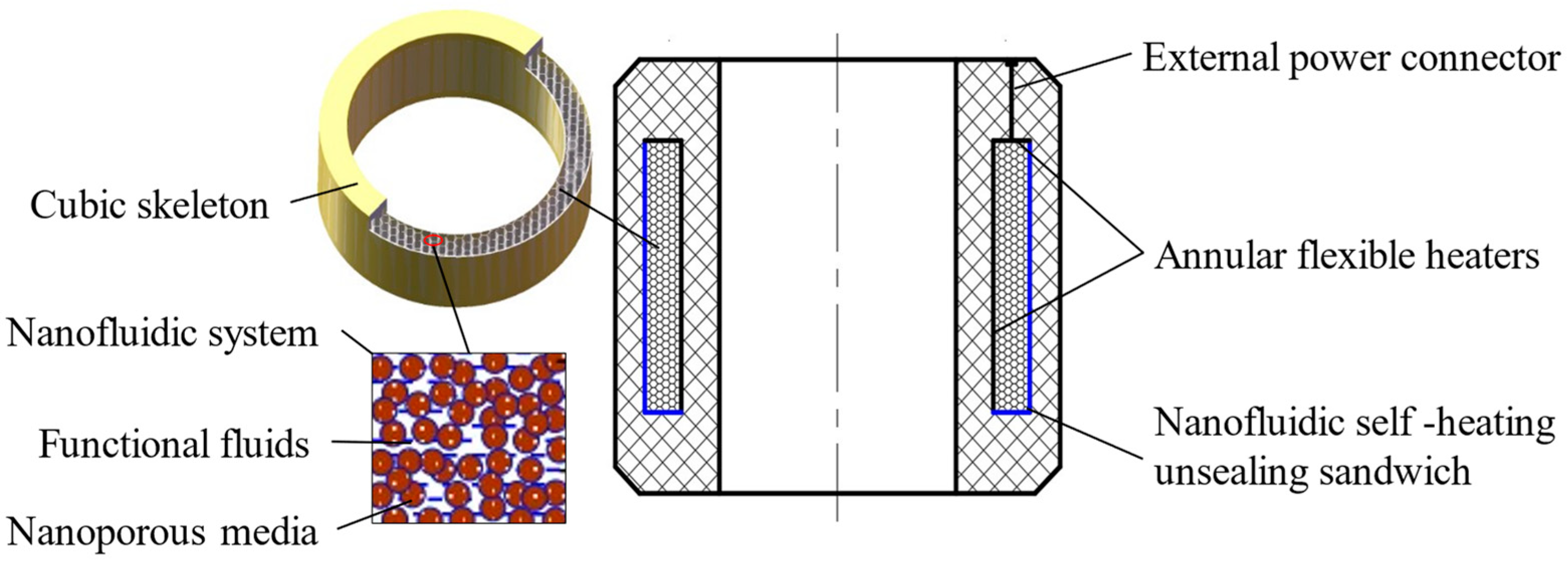
2. Structure Design and Working Principle
3. Size Design Method and Calculation Case Study
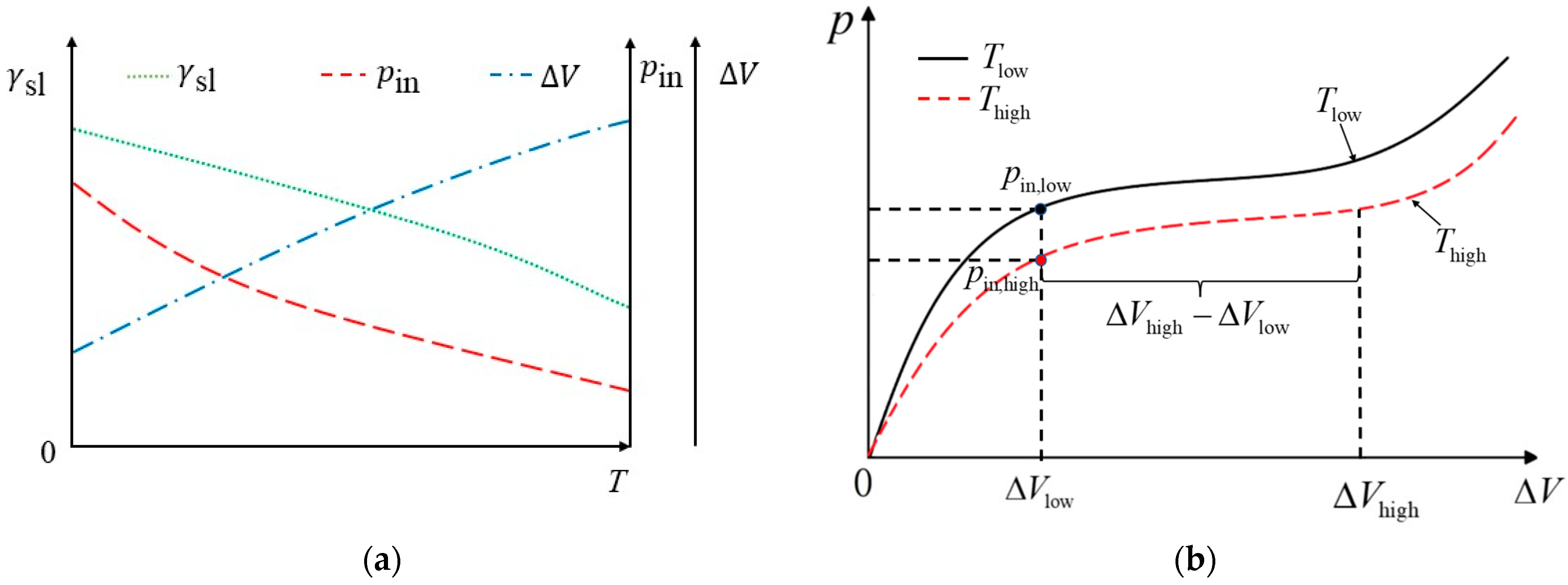
3.1. Design Method of Nanofluidic Self-Heating Unsealing Sandwich Size
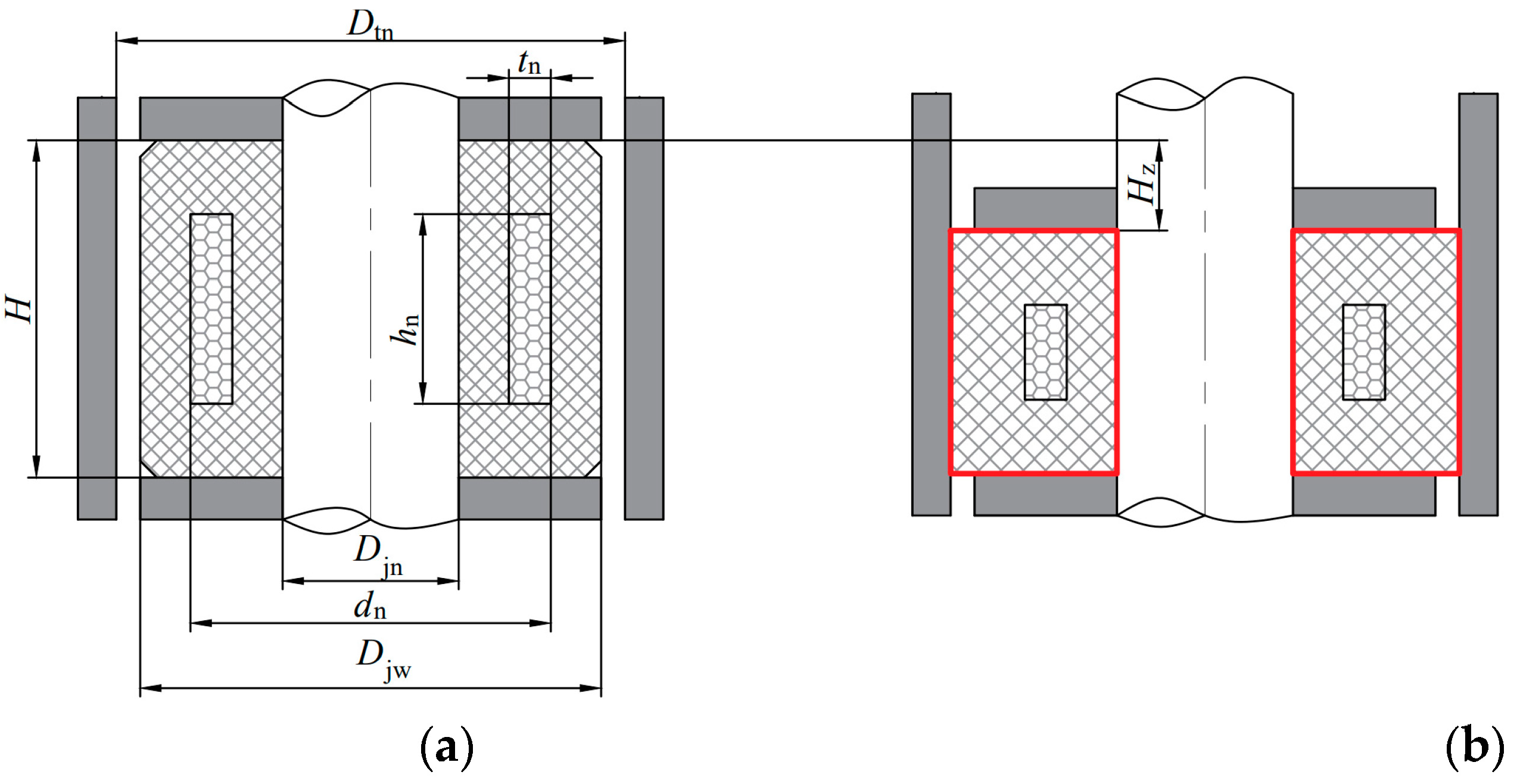
3.2. Case Study of Sandwich Size Calculation
4. Analysis of Sealing Performance
4.1. Finite Element Modeling
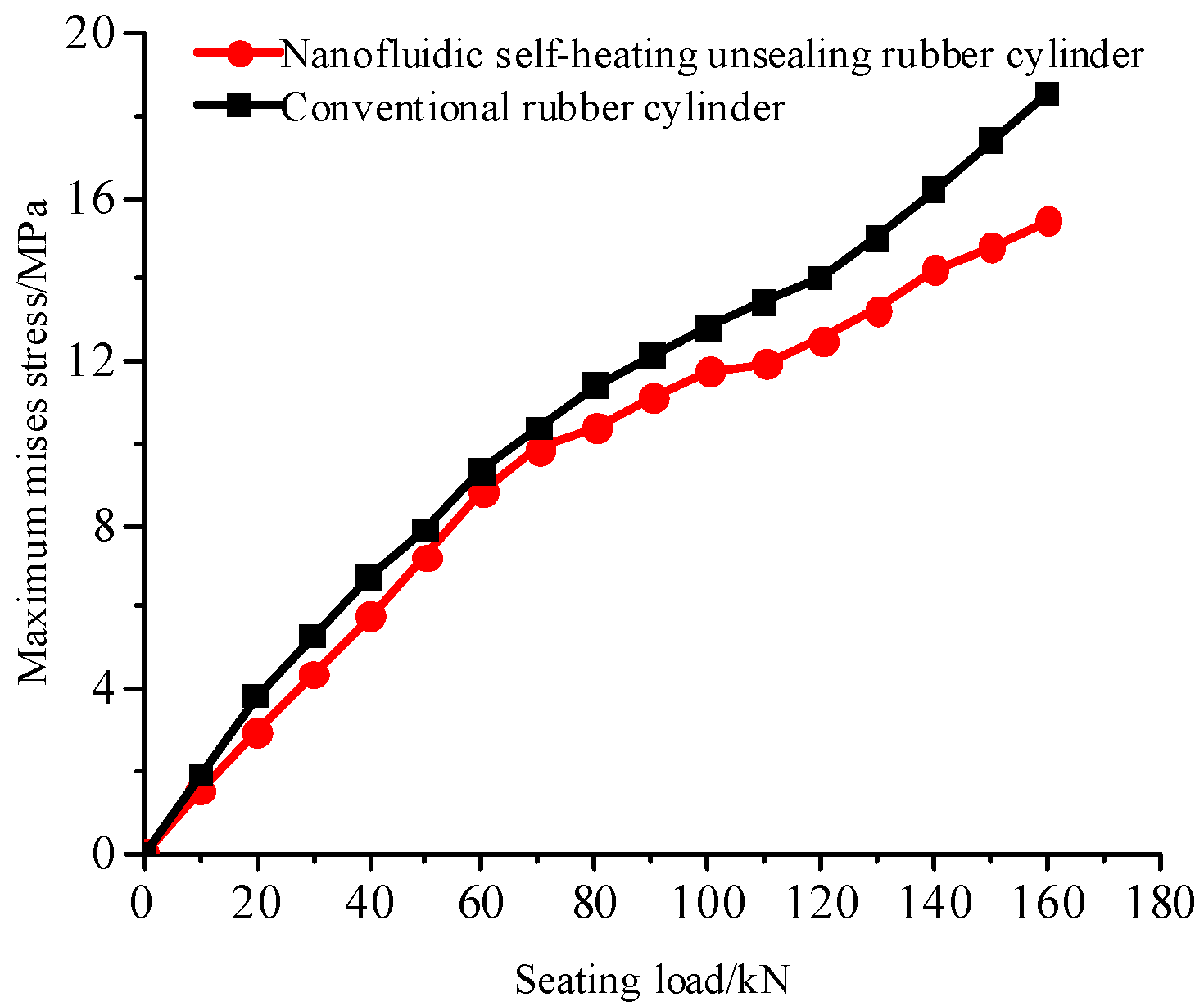
4.2. Analysis of Simulation Results
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Pan, L.; Oldenburg, C.M. Mechanistic modeling of CO2 well leakage in a generic abandoned well through a bridge plug cement-casing gap. Int. J. Greenh. Gas Control 2020, 97, 103025. [Google Scholar] [CrossRef]
- Lei, Q.; Xu, Y.; Cai, B.; Guan, B.; Wang, X.; Bi, G.; Li, H.; Li, S.; Ding, B.; Fu, H.; et al. Progress and prospects of horizontal well fracturing technology for shale oil and gas reservoirs. Pet. Explor. Dev. 2022, 49, 191–199. [Google Scholar] [CrossRef]
- Novy, R.A. Pressure Drops in Horizontal Wells: When Can They Be Ignored? SPE Res. Eng. 1995, 10, 29–35. [Google Scholar] [CrossRef]
- Ratterman, E.E.; Augustine, J.R.; Voll, B.A. New Technology Applications to Increase Oil Recovery by Creating Uniform Flow Profiles in Horizontal Wells: Case Studies and Technology Overview. In Proceedings of the International Petroleum Technology Conference, Doha, Qatar, 21–23 November 2005; p. 10177. [Google Scholar]
- Han, Y.; Liu, Z.; Zhou, H.; Zhang, P.; Guo, M.; Zhan, H.; Ren, Z.; Sun, X. Research of Unthrottled Self-unsetting Packer and Technology String. Mech. Eng. 2019, 339, 70–73. (In Chinese) [Google Scholar]
- Hu, Y.; Yang, K.; Li, J.; Yang, J.; Wang, C.; Miao, Y.; Qiao, R. Research and Application of Multistage Fracturing Self-releasing Packer and Tubing String. Pet. Mach. 2017, 45, 83–86. (In Chinese) [Google Scholar]
- Wang, J.; Jia, H.; You, J. Performance Analysis of Soluble Bridge Plug Materials for Fracturing in Unconventional Oilfields. Math. Probl. Eng. 2022, 2022, 1911173. [Google Scholar] [CrossRef]
- Liu, Y.; Lian, Z.; Chen, J.; Kuang, S.; Mou, Y.; Wang, Y. Design and Experimental Research on Sealing Structure for a Retrievable Packer. Shock. Vib. 2020, 2020, 7695276. [Google Scholar] [CrossRef]
- Feng, Y.; Wu, K. Structure and Performance analysis of gradual Releasing long Tubing packer. Oil Field Equip. 2015, 44, 79–82. [Google Scholar]
- Zhang, P.; Pu, C.; Pu, J.; Wang, Z. Study and Field Trials on Dissolvable Frac Plugs for Slightly Deformed Casing Horizontal Well Volume Fracturing. ACS Omega 2022, 7, 10292–10303. [Google Scholar] [CrossRef]
- Swor, L.; Sonnefeld, A. Self-Removing Frangible Bridge and Fracture Plugs. In Proceedings of the SPE Annual Technical Conference and Exhibition, San Antonio, TX, USA, 24–27 September 2006. [Google Scholar]
- Zachary, W.; Porter, J.; Fripp, M.; Vargus, G. Cost and Value of a Dissolvable Frac Plug. In Proceedings of the SPE/ICoTA Coiled Tubing and Well Intervention Conference and Exhibition, Houston, TX, USA, 21–22 March 2017. [Google Scholar]
- Cheng, K.; Shang, L.; Li, H.; Peng, B.; Li, Z. A novel degradable sealing material for the preparation of dissolvable packer rubber barrel. J. Macromol. Sci. A 2023, 60, 201–216. [Google Scholar] [CrossRef]
- Wang, X.; Zhang, X.; Chen, S. Sealing properties and structure optimization of packer rubber under high pressure and high temperature. Pet. Sci. 2019, 16, 632–644. [Google Scholar]
- Liu, Z.; Li, S.; Zhang, L.; Wang, F.; Wang, P.; Han, L.; Ma, Y.; Zhang, H. Analysis of Sealing Mechanical Properties of Fracturing Packer Under Complex Conditions. J. Fail. Anal. Prev. 2019, 19, 1569–1582. [Google Scholar] [CrossRef]
- Wang, L.; Sheng, X.; Sheng, J. Analysis of the Pressure Expansion of Bridge Plug Tools and Packers by Equivalent Material Method. J. Phys. Conf. Ser. 2019, 1187, 032052. [Google Scholar] [CrossRef]
- Hu, G.; Liu, Y.; Wang, G.; Wu, W.; Xu, Z.; Shen, K. Research on the Influence of Stress Relaxation on the Sealing Performance of the Packer under HTHP. J. Fail. Anal. Prev. 2022, 22, 841–857. [Google Scholar] [CrossRef]
- Ma, W.; Qu, B.; Guan, F. Effect of the friction coefficient for contact pressure of packer rubber. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2014, 228, 2881–2887. [Google Scholar] [CrossRef]
- Zhang, F.; Jiang, X.; Wang, H.; Song, N.; Chen, J.; Duan, J. Mechanical analysis of sealing performance for compression packer. Mech. Ind. 2018, 19, 309–319. [Google Scholar] [CrossRef] [Green Version]
- Dong, L.; Li, K.; Zhu, X.; Li, Z.; Zhang, D.; Pan, Y.; Chen, X. Study on high temperature sealing behavior of packer rubber tube based on thermal aging experiments. Eng. Fail. Anal. 2020, 108, 104321. [Google Scholar] [CrossRef]
- Zheng, X.; Li, B. Study on sealing performance of packer rubber based on stress relaxation experiment. Eng. Fail. Anal. 2021, 121, 105692. [Google Scholar] [CrossRef]
- Feng, G.; Li, S.; Xiao, L.; Song, W. Energy absorption performance of honeycombs with curved cell walls under quasi-static compression. Int. J. Mech. Sci. 2021, 210, 106746. [Google Scholar] [CrossRef]
- Xu, B.; Qiao, Y.; Park, T.; Tak, M.; Zhou, Q.; Chen, X. A conceptual thermal actuation system driven by interface tension of nanofluids. Energy Environ. Sci. 2011, 4, 3632–3639. [Google Scholar] [CrossRef]
- Liu, L.; Zhao, J.; Yin, C.; Culligana, P.J.; Chen, X. Mechanisms of water infiltration into conical hydrophobic nanopores. Phys. Chem. Chem. Phys. 2009, 11, 6520–6524. [Google Scholar] [CrossRef] [Green Version]
- Li, W. Further explanation of the direction of gas-liquid interfacial tension in the derivation of Young’s equation. Chem. Higher Educ. 2016, 33, 84–94. (In Chinese) [Google Scholar]
- Han, A.; Qiao, Y. A volume-memory liquid. Applied Physics Letters. Appl. Phys. Lett. 2007, 91, 173123. [Google Scholar] [CrossRef] [Green Version]
- Zhang, Y.; Li, N.; Lou, R.; Zhang, Y.; Zhou, Q.; Chen, X. Experimental study on thermal effect on infiltration mechanisms of glycerol into ZSM-5 zeolite under cyclic loadings. J. Phys. D Appl. Phys. 2016, 49, 025303. [Google Scholar] [CrossRef]
- Zheng, C.; Zheng, X.; Qin, J.; Liu, P.; Aibaibu, A.; Liu, Y. Nonlinear finite element analysis on the sealing performance of rubber packer for hydraulic fracturing. J. Nat. Gas. Sci. Eng. 2021, 85, 103711. [Google Scholar] [CrossRef]
- Zhang, Y.; Liang, J.; Luo, R.; Min, S.; Dou, Y. Application Characteristics of Zeolite-Based Stuffing for Nanofluidic Packer Rubber. Energies 2022, 15, 7962. [Google Scholar] [CrossRef]
- Liu, Y.; Qian, L.; Zou, J.; Xia, C.; Lian, Z. Study on failure mechanism and sealing performance optimization of compression packer. Eng. Fail. Anal. 2022, 136, 106176. [Google Scholar] [CrossRef]
- Hu, G.; Zhang, P.; Wang, G.; Zhang, M.; Li, M. The influence of rubber material on sealing performance of packing element in compression packer. J. Nat. Gas Sci. Eng. 2017, 38, 120–138. [Google Scholar] [CrossRef]
- Zhang, Y.; Min, S.; Wang, H.; Gao, M.; Dou, Y. Analysis on loading capacity of first-order honeycomb skeleton of nanofluidic packer rubber. Pet. Mach. 2022, 50, 73–78. (In Chinese) [Google Scholar]
- Zhang, Z.; Zhu, X.; Xu, J. Structural parameters optimization of compression packer rubber based on orthogonal test. Nat. Gas Ind. 2019, 39, 80–84. (In Chinese) [Google Scholar]
- Liu, J.; Deng, K.; Liu, S.; Yan, X.; Li, L.; Zou, D.; Lin, Y. Mechanical Behavior and Structure Optimization of Compressed PHP Packer Rubber. J. Mater. Eng. Perform. 2021, 30, 3691–3704. [Google Scholar] [CrossRef]
- Zhang, Y.; Liang, J.; Liu, J.; Xiao, H.; Dou, Y. Energy absorption characteristics of honeycomb structure of nanofluidic packer rubber under coplanar compression. Pet. Mach. 2022, 50, 89–95. (In Chinese) [Google Scholar]
- Jiao, K.; Yun, F.; Yan, Z.; Wang, G.; Jia, P.; Wang, L.; Liu, D.; Hao, X. Optimization and Experimental Study of the Subsea Retractable Connector Rubber Packer Based on Mooney-Rivlin Constitutive Model. J. Mar. Sci. Eng. 2021, 9, 1391. [Google Scholar] [CrossRef]
- Hu, G.; Wang, M.; Wang, G.; He, X. Research on the Influence of Spacer Ring on the Sealing Performance of Packing Element used in Compression Packer. J. Fail. Anal. Prev. 2021, 21, 1605–1621. [Google Scholar] [CrossRef]
- Li, B.; Lu, D.; Zhang, Z. Analysis of mechanical properties of wave packer rubber. J. Appl. Mech. 2018, 35, 828–833. (In Chinese) [Google Scholar]



| Parameters | Numerical Value |
|---|---|
| Specific surface area/m2·g−1 | 3500 |
| Aperture/nm | 2 |
| Pore volume per unit mass/cm3·g−1 | 1.6 |
| Pore quality factor | 1.5 |
| Porosity | 0.5 |
| Parameters | Height/mm | Inner Diameter/mm | Outer Diameter/mm |
|---|---|---|---|
| Casing | 100 | 121.4 | 139.7 |
| Center tube | 100 | 42 | 68 |
| Pressure ring | 10 | 68 | 110 |
| Rubber cylinder | 80 | 68 | 110 |
| Nanofluidic self-heating unsealing sandwich | 45 | 88 | 98 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, Y.; Fan, T.; Zhang, P.; Dou, Y. Structural Design and Sealing Performance Analysis of a Nanofluidic Self-Heating Unsealing Rubber Cylinder. Energies 2023, 16, 4890. https://doi.org/10.3390/en16134890
Zhang Y, Fan T, Zhang P, Dou Y. Structural Design and Sealing Performance Analysis of a Nanofluidic Self-Heating Unsealing Rubber Cylinder. Energies. 2023; 16(13):4890. https://doi.org/10.3390/en16134890
Chicago/Turabian StyleZhang, Yafei, Taihao Fan, Pengbo Zhang, and Yihua Dou. 2023. "Structural Design and Sealing Performance Analysis of a Nanofluidic Self-Heating Unsealing Rubber Cylinder" Energies 16, no. 13: 4890. https://doi.org/10.3390/en16134890
APA StyleZhang, Y., Fan, T., Zhang, P., & Dou, Y. (2023). Structural Design and Sealing Performance Analysis of a Nanofluidic Self-Heating Unsealing Rubber Cylinder. Energies, 16(13), 4890. https://doi.org/10.3390/en16134890









