Optimization and Analysis of an Integrated Liquefaction Process for Hydrogen and Natural Gas Utilizing Mixed Refrigerant Pre-Cooling
Abstract
:1. Introduction
2. Process Description
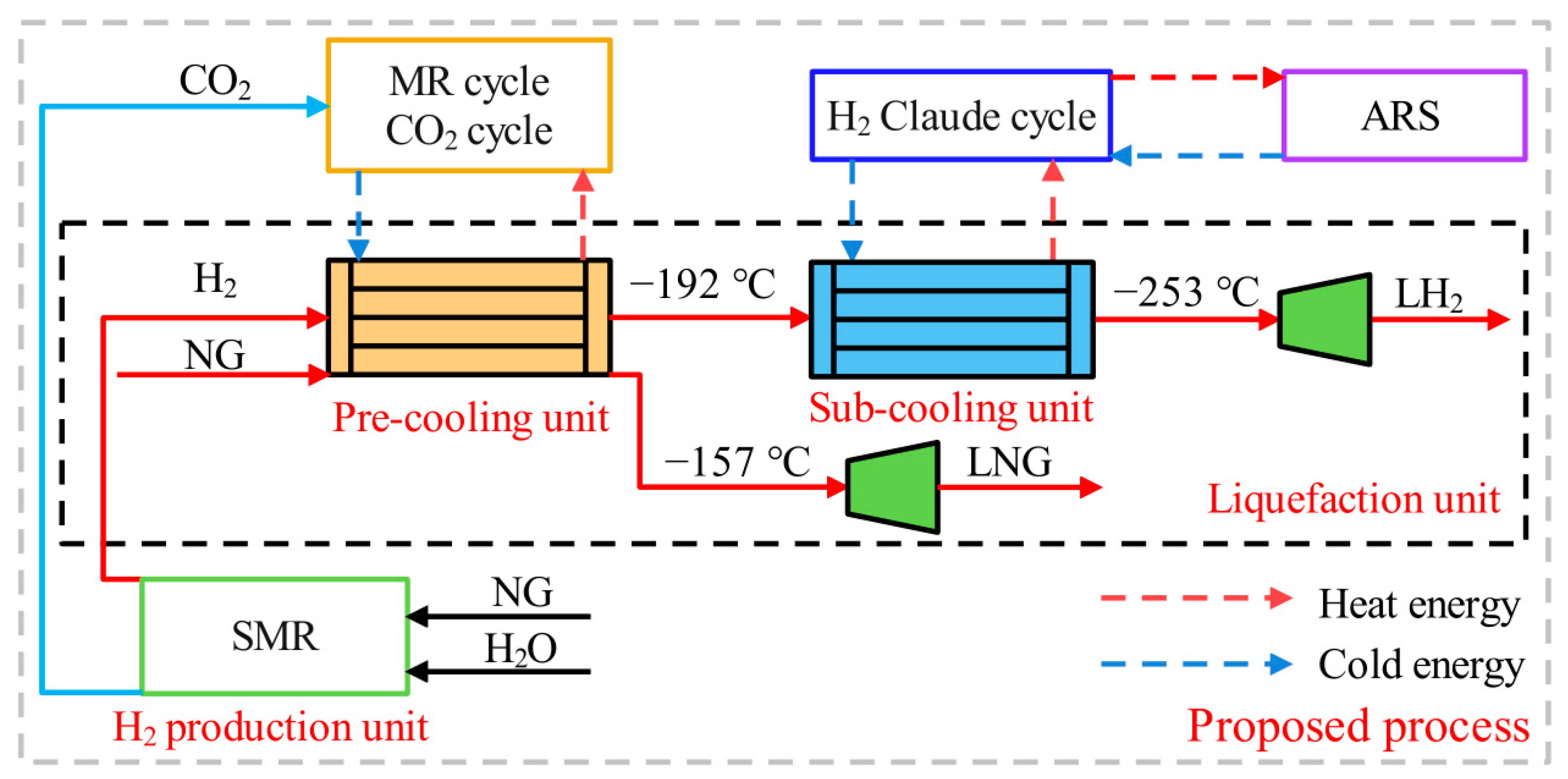
2.1. Overall Configuration
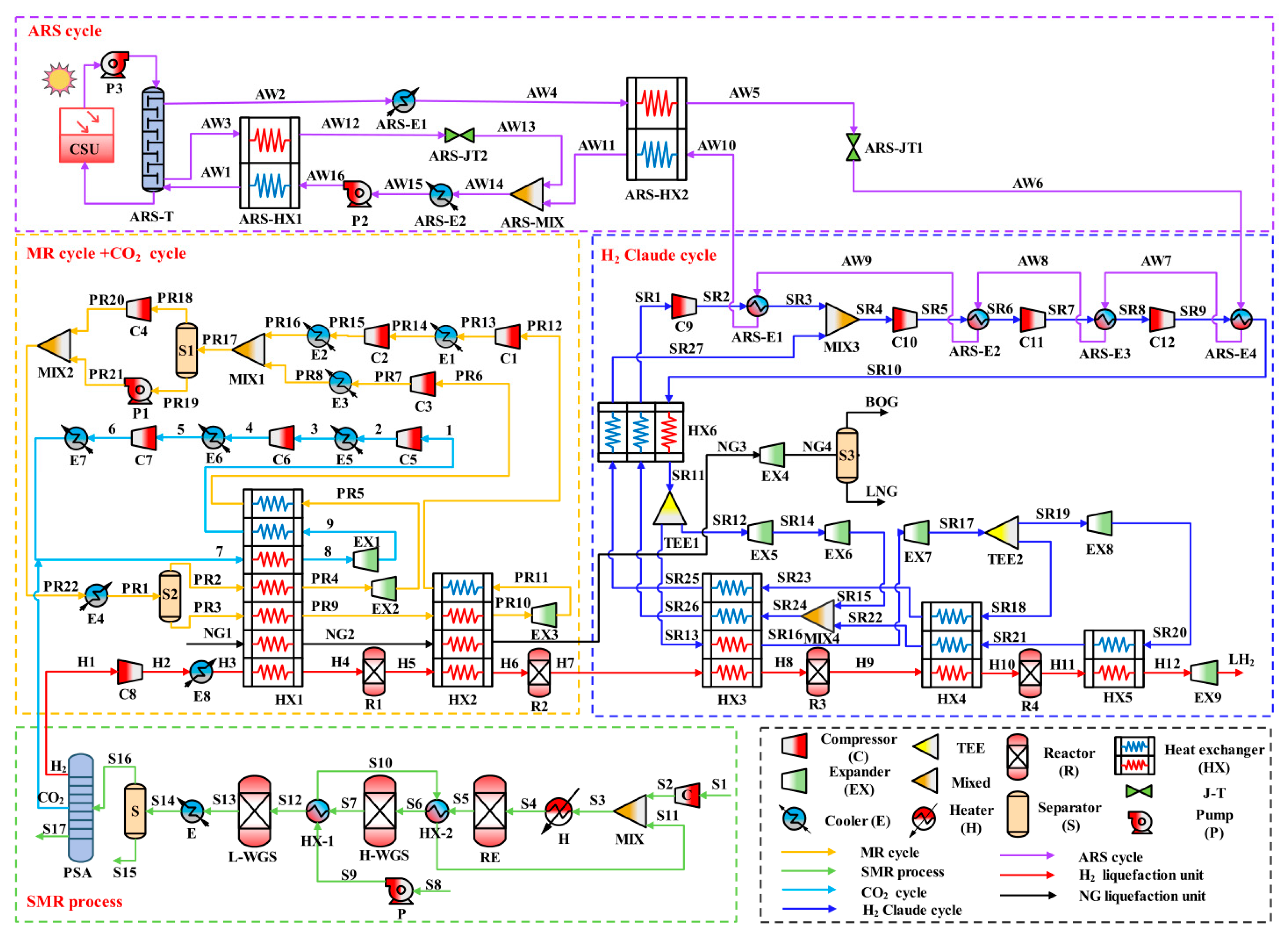
2.2. Proposed Process
3. Process Simulation and Optimization
3.1. Conditions and Assumptions
3.2. Ortho-Para Hydrogen Conversion
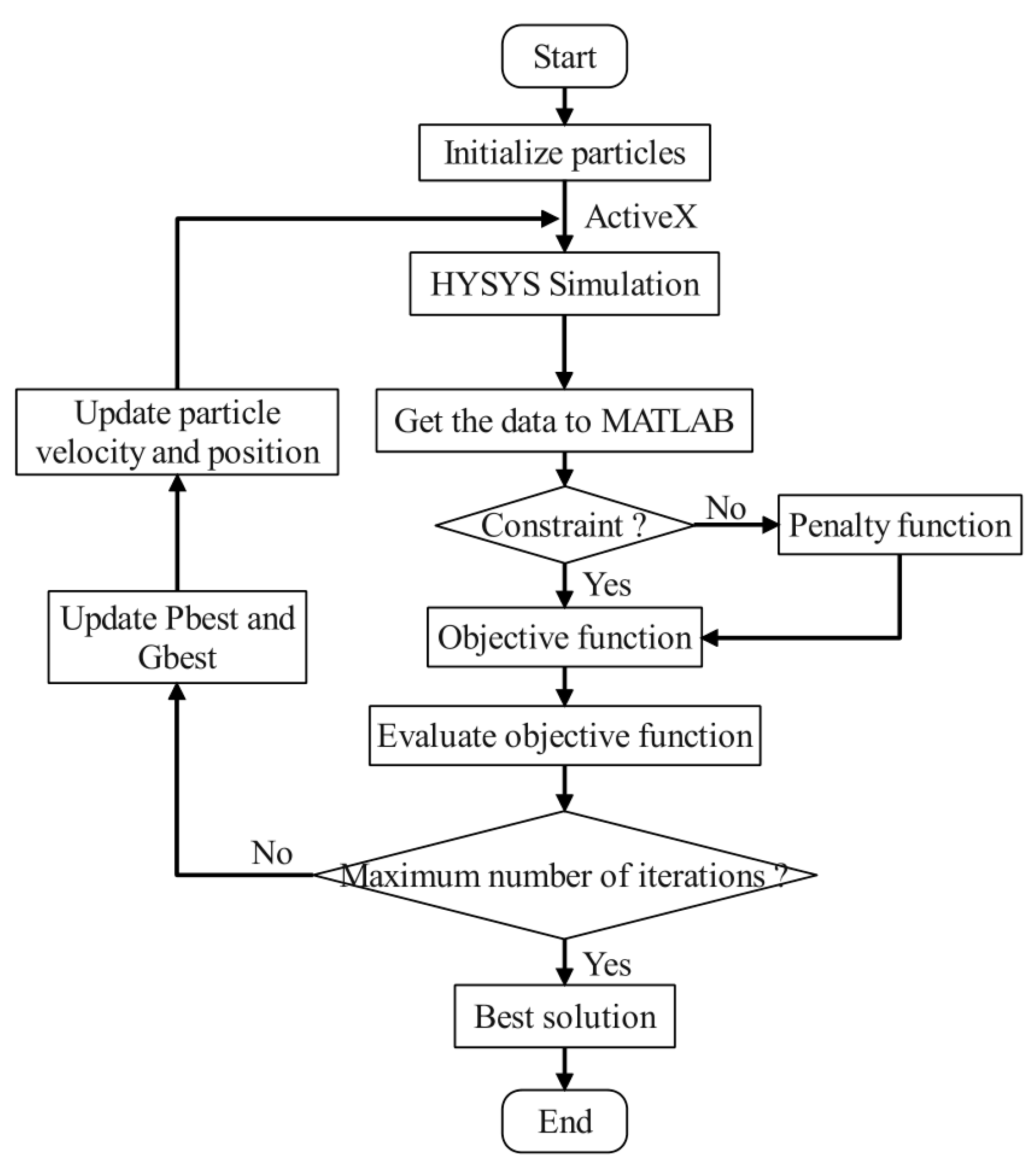
3.3. Process Optimization
4. Results and Discussions
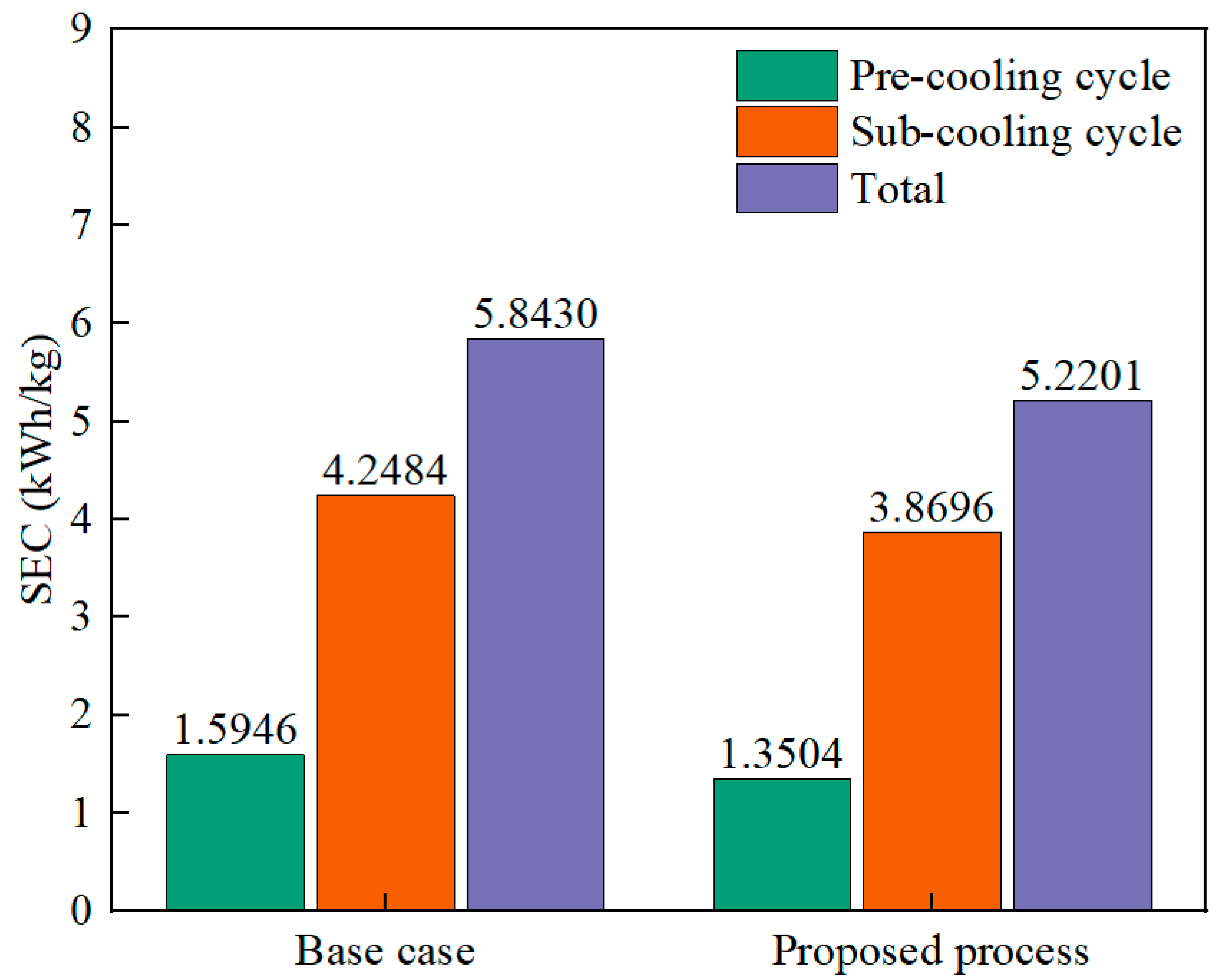
4.1. Energy Analysis
4.2. Composite Curve Analysis
4.3. Exergy Analysis
4.4. Economic Analysis
4.5. Comparison with Other Processes
5. Conclusions and Further Work
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Rusman, N.; Dahari, M. A review on the current progress of metal hydrides material for solid-state hydrogen storage applications. Int. J. Hydrog. Energy 2016, 41, 12108–12126. [Google Scholar] [CrossRef]
- Bairrão, D.; Soares, J.; Almeida, J.; Franco, J.F.; Vale, Z. Green Hydrogen and Energy Transition: Current State and Prospects in Portugal. Energies 2023, 16, 551. [Google Scholar] [CrossRef]
- Sánchez-Bastardo, N.; Schlögl, R.; Ruland, H. Methane pyrolysis for zero-emission hydrogen production: A potential bridge technology from fossil fuels to a renewable and sustainable hydrogen economy. Ind. Eng. Chem. Res. 2021, 60, 11855–11881. [Google Scholar] [CrossRef]
- Aziz, M. Liquid Hydrogen: A Review on Liquefaction, Storage, Transportation, and Safety. Energies 2021, 14, 5917. [Google Scholar] [CrossRef]
- Valenti, G. Hydrogen liquefaction and liquid hydrogen storage. In Compendium of Hydrogen Energy; Elsevier: Amsterdam, The Netherlands, 2016; pp. 27–51. [Google Scholar]
- Zhang, S.; Liu, G. Design and performance analysis of a hydrogen liquefaction process. Clean Technol. Environ. Policy 2021, 24, 51–65. [Google Scholar] [CrossRef]
- Yin, L.; Ju, Y. Review on the design and optimization of hydrogen liquefaction processes. Front. Energy 2020, 14, 530–544. [Google Scholar] [CrossRef]
- Krasae-in, S.; Stang, J.H.; Neksa, P. Development of large-scale hydrogen liquefaction processes from 1898 to 2009. Int. J. Hydrog. Energy 2010, 35, 4524–4533. [Google Scholar] [CrossRef]
- Yilmaz, C. A case study: Exergoeconomic analysis and genetic algorithm optimization of performance of a hydrogen liquefaction cycle assisted by geothermal absorption precooling cycle. Renew Energy 2018, 128, 68–80. [Google Scholar] [CrossRef]
- Aasadnia, M.; Mehrpooya, M. Large-scale liquid hydrogen production methods and approaches: A review. Appl. Energy 2018, 212, 57–83. [Google Scholar] [CrossRef]
- Baker, C.R.; Shaner, R.L. A study of the efficiency of hydrogen liquefaction. Int. J. Hydrog. Energy 1978, 3, 321–334. [Google Scholar] [CrossRef]
- Ohira, K. A Summary of Liquid Hydrogen and Cryogenic Technologies in Japan’s WE-NET Project. In Proceedings of the AIP Conference Proceedings, Anchorage, AK, USA, 22–26 September 2003; American Institute of Physics: Anchorage, AK, USA, 2004; pp. 27–34. [Google Scholar]
- Yin, L.; Ju, Y. Process optimization and analysis of a novel hydrogen liquefaction cycle. Int. J. Refrig. 2020, 110, 219–230. [Google Scholar] [CrossRef]
- Bi, Y.; Yin, L.; He, T.; Ju, Y. Optimization and analysis of a novel hydrogen liquefaction process for circulating hydrogen refrigeration. Int. J. Hydrog. Energy 2022, 47, 348–364. [Google Scholar] [CrossRef]
- Shimko, M.A.; Gardiner, M.R. Innovative Hydrogen Liquefaction Cycle. FY 2008 Annual Progress Report, DOE Hydrogen Program. 2008. Available online: https://www.mendeley.com/catalogue/d58c2233-c427-3524-9a1c-92db5017d0ab/ (accessed on 11 April 2023).
- Yuksel, Y.E.; Ozturk, M.; Dincer, I. Analysis and assessment of a novel hydrogen liquefaction process. Int. J. Hydrog. Energy 2017, 42, 11429–11438. [Google Scholar] [CrossRef]
- Krasae-In, S. Optimal operation of a large-scale liquid hydrogen plant utilizing mixed fluid refrigeration system. Int. J. Hydrog. Energy 2014, 39, 7015–7029. [Google Scholar] [CrossRef]
- Asadnia, M.; Mehrpooya, M. A novel hydrogen liquefaction process configuration with combined mixed refrigerant systems. Int. J. Hydrog. Energy 2017, 42, 15564–15585. [Google Scholar] [CrossRef]
- Sadaghiani, M.S.; Mehrpooya, M.; Ansarinasab, H. Process development and exergy cost sensitivity analysis of a novel hydrogen liquefaction process. Int. J. Hydrog. Energy 2017, 42, 29797–29819. [Google Scholar] [CrossRef]
- Aasadnia, M.; Mehrpooya, M. Conceptual design and analysis of a novel process for hydrogen liquefaction assisted by absorption precooling system. J. Clean. Prod. 2018, 205, 565–588. [Google Scholar] [CrossRef]
- Qyyum, M.A.; Riaz, A.; Naquash, A.; Haider, J.; Qadeer, K.; Nawaz, A.; Lee, H.; Lee, M. 100% saturated liquid hydrogen production: Mixed-refrigerant cascaded process with two-stage ortho-to-para hydrogen conversion. Energy Convers. Manag. 2021, 246, 114659. [Google Scholar] [CrossRef]
- Naquash, A.; Riaz, A.; Lee, H.; Qyyum, M.A.; Lee, S.; Lam, S.S.; Lee, M. Hydrofluoroolefin-based mixed refrigerant for enhanced performance of hydrogen liquefaction process. Int. J. Hydrog. Energy 2022, 47, 41648–41662. [Google Scholar] [CrossRef]
- Lee, H.; Haider, J.; Abdul Qyyum, M.; Choe, C.; Lim, H. An innovative high energy efficiency–based process enhancement of hydrogen liquefaction: Energy, exergy, and economic perspectives. Fuel 2022, 320, 123964. [Google Scholar] [CrossRef]
- Kim, H.; Haider, J.; Qyyum, M.A.; Lim, H. Mixed refrigerant–based simplified hydrogen liquefaction process: Energy, exergy, economic, and environmental analysis. J. Clean. Prod. 2022, 367, 132947. [Google Scholar] [CrossRef]
- Yang, J.-H.; Yoon, Y.; Ryu, M.; An, S.-K.; Shin, J.; Lee, C.-J. Integrated hydrogen liquefaction process with steam methane reforming by using liquefied natural gas cooling system. Appl. Energy 2019, 255, 113840. [Google Scholar] [CrossRef]
- Bian, J.; Yang, J.; Li, Y.; Chen, Z.; Liang, F.; Cao, X. Thermodynamic and economic analysis of a novel hydrogen liquefaction process with LNG precooling and dual-pressure Brayton cycle. Energy Convers. Manag. 2021, 250, 114904. [Google Scholar] [CrossRef]
- Riaz, A.; Qyyum, M.A.; Min, S.; Lee, S.; Lee, M. Performance improvement potential of harnessing LNG regasification for hydrogen liquefaction process: Energy and exergy perspectives. Appl. Energy 2021, 301, 117471. [Google Scholar] [CrossRef]
- Yilmaz, C.; Kaska, O. Performance analysis and optimization of a hydrogen liquefaction system assisted by geothermal absorption precooling refrigeration cycle. Int. J. Hydrog. Energy 2018, 43, 20203–20213. [Google Scholar] [CrossRef]
- Seyam, S.; Dincer, I.; Agelin-Chaab, M. Analysis of a clean hydrogen liquefaction plant integrated with a geothermal system. J. Clean. Prod. 2020, 243, 118562. [Google Scholar] [CrossRef]
- Ghorbani, B.; Mehrpooya, M.; Amidpour, M. A novel integrated structure of hydrogen purification and liquefaction using natural gas steam reforming, organic Rankine cycle and photovoltaic panels. Cryogenics 2021, 119, 103352. [Google Scholar] [CrossRef]
- Karimi, M.; Mehrpooya, M. Proposal and investigation of a novel hybrid hydrogen production and liquefaction process using solid oxide electrolyzer, solar energy, and thermoelectric generator. J. Clean. Prod. 2022, 331, 130001. [Google Scholar] [CrossRef]
- Bi, Y.; Ju, Y. Design and analysis of an efficient hydrogen liquefaction process based on helium reverse Brayton cycle integrating with steam methane reforming and liquefied natural gas cold energy utilization. Energy 2022, 252, 124047. [Google Scholar] [CrossRef]
- Kothari, R.; Buddhi, D.; Sawhney, R. Comparison of environmental and economic aspects of various hydrogen production methods. Renew. Sustain. Energy Rev. 2008, 12, 553–563. [Google Scholar] [CrossRef]
- Geng, J.; Sun, H. A novel integrated hydrogen and natural gas liquefaction process utilizing a modified double mixed refrigerant process pre-cooling system. Appl. Therm. Eng. 2023, 224, 120085. [Google Scholar] [CrossRef]
- Sadaghiani, M.S.; Mehrpooya, M. Introducing and energy analysis of a novel cryogenic hydrogen liquefaction process configuration. Int. J. Hydrog. Energy 2017, 42, 6033–6050. [Google Scholar] [CrossRef]
- Zhang, J.; Meerman, H.; Benders, R.; Faaij, A. Technical and economic optimization of expander-based small-scale natural gas liquefaction processes with absorption precooling cycle. Energy 2020, 191, 116592. [Google Scholar] [CrossRef]
- Sherif, S.A.; Goswami, D.Y.; Stefanakos, E.L.; Steinfeld, A. Handbook of Hydrogen Energy; CRC Press: Boca Raton, FL, USA, 2014; pp. 567–592. [Google Scholar]
- Harkness, R.W.; Deming, W.E. The equilibrium of para and ortho hydrogen. J. American Chemical Society 1932, 54, 2850–2852. [Google Scholar] [CrossRef]
- Faramarzi, S.; Nainiyan, S.M.M.; Mafi, M.; Ghasemiasl, R. A novel hydrogen liquefaction process based on LNG cold energy and mixed refrigerant cycle. Int. J. Refrig. 2021, 131, 263–274. [Google Scholar] [CrossRef]
- Aasadnia, M.; Mehrpooya, M.; Ansarinasab, H. A 3E evaluation on the interaction between environmental impacts and costs in a hydrogen liquefier combined with absorption refrigeration systems. Appl. Therm. Eng. 2019, 159, 113798. [Google Scholar] [CrossRef]
- Cavalcanti, E.J.C. Exergoeconomic and exergoenvironmental analyses of an integrated solar combined cycle system. Renew. Sustain. Energy Rev. 2017, 67, 507–519. [Google Scholar] [CrossRef]
- Geng, J.; Sun, H. Optimization and analysis of a hydrogen liquefaction process: Energy, exergy, economic, and uncertainty quantification analysis. Energy 2023, 262, 125410. [Google Scholar] [CrossRef]
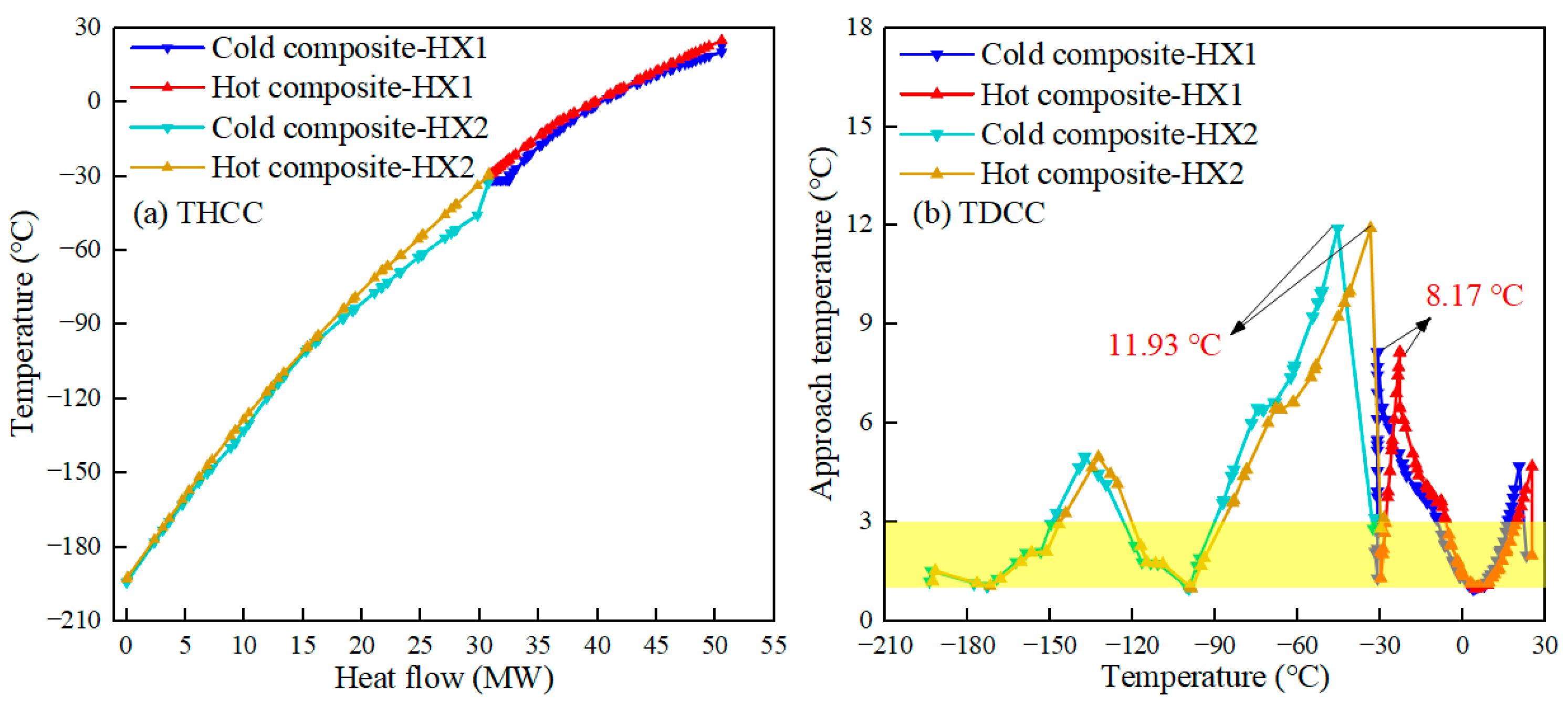

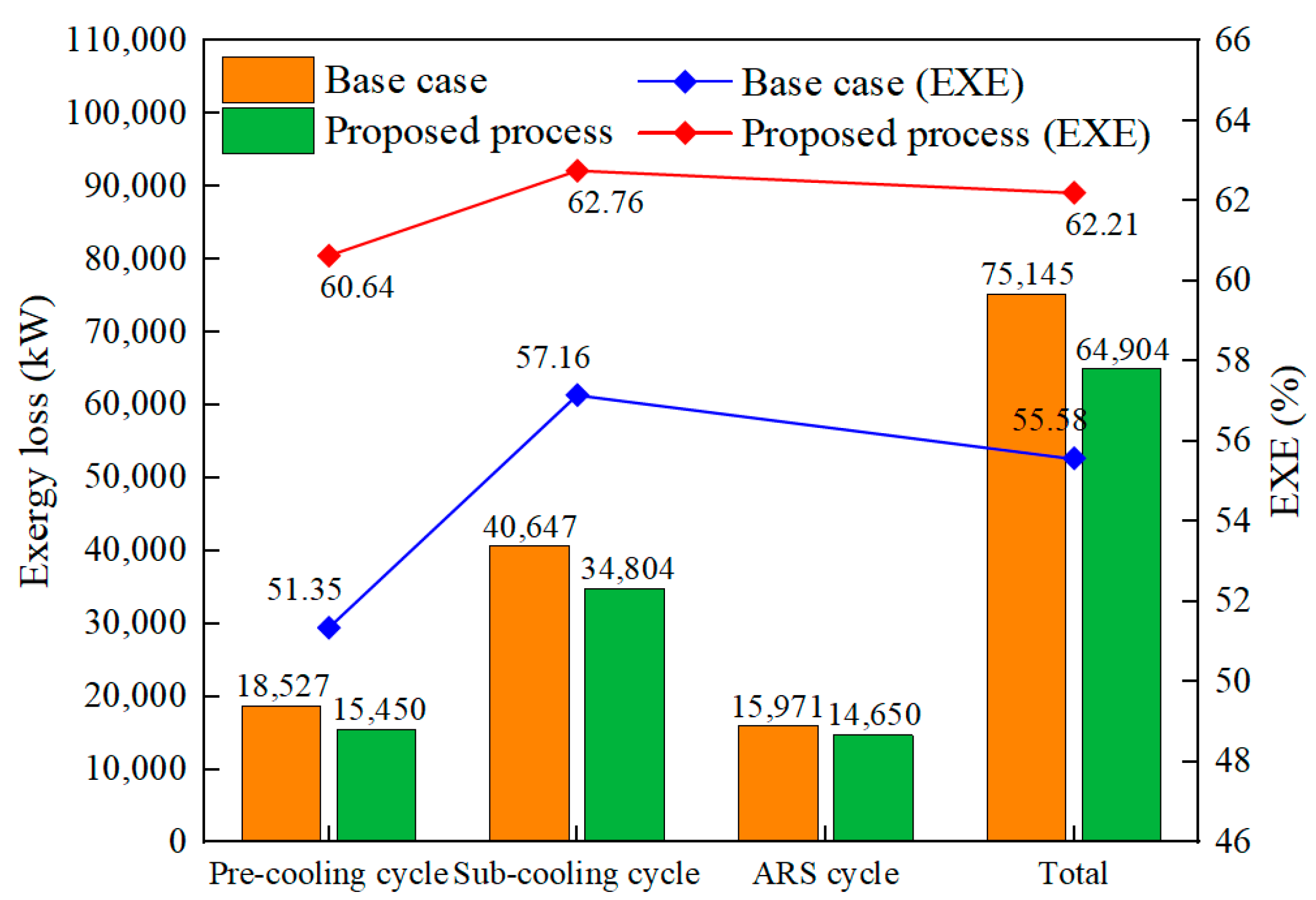
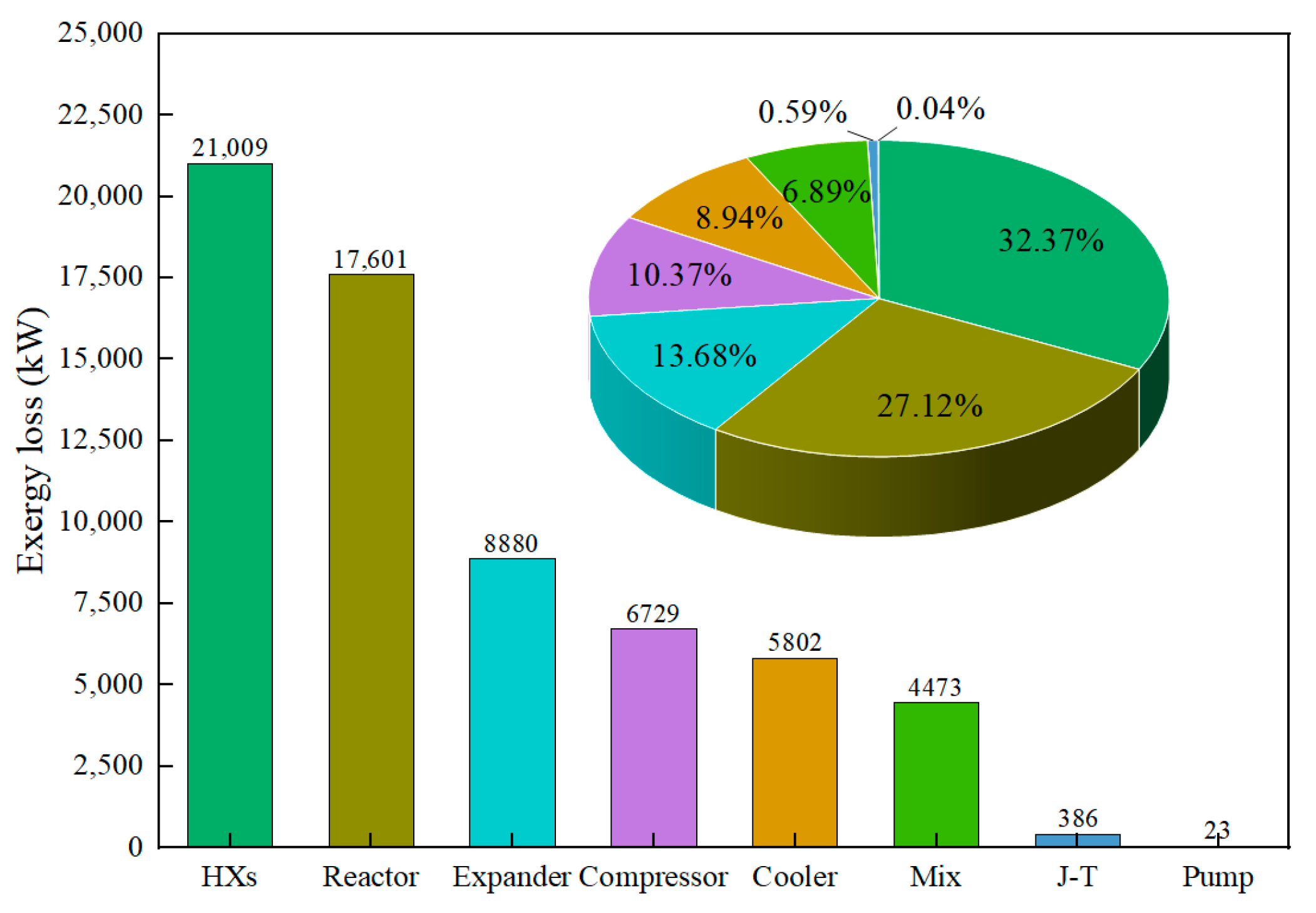

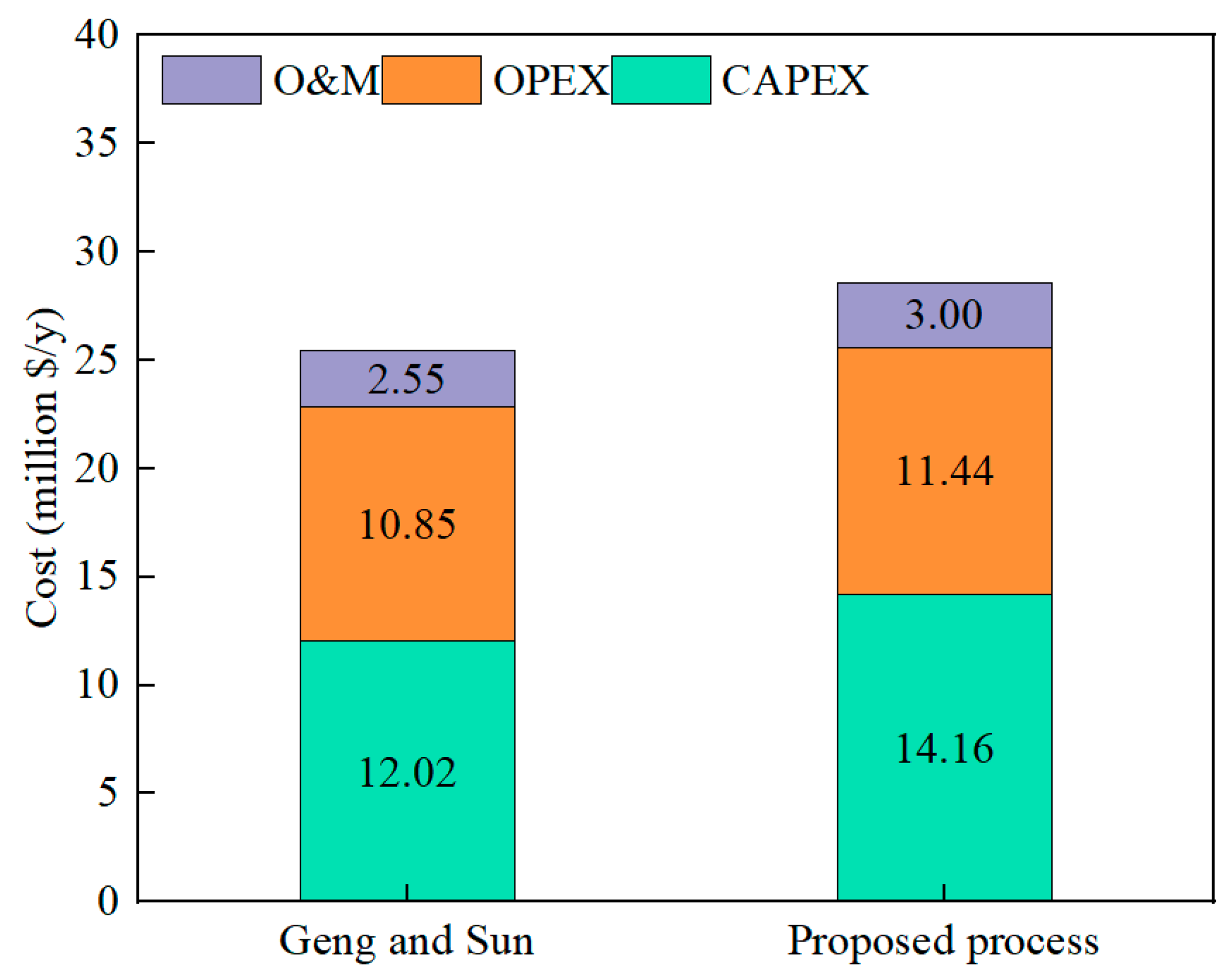
| Processes | Source | SEC (kWh/kg) | EXE (%) |
|---|---|---|---|
| Commercial processes | |||
| LN2 pre-cooling | Praxair [10] | 12.5–15 | 19.3–23.1 |
| Linde Ingolstadt [10] | 13.6 | 21.2 | |
| Linde Leuna [10] | 11.9 | 23.6 | |
| Conceptual processes | |||
| LN2 pre-cooling | Baker and Shaner [11] | 10.85 | 36 |
| Ohira [12] | 8.5 | - | |
| Yin and Ju [13] | 7.1329 | 49.41 | |
| Bi et al. [14] | 7.041 | 54.13 | |
| He pre-cooling | Shimko and Gardiner [15] | 8.73 | 44.55 |
| Yuksel et al. [16] | - | 57.13 | |
| MR pre-cooling | Krasae-In et al. [17] | 5.91 | - |
| Asadnia and Mehrpooya [18] | 7.69 | 39.5 | |
| Sadaghiani et al. [19] | 7.646 | 32 | |
| Aasadnia and Mehrpooya [20] | 6.47 | 45.5 | |
| Qyyum et al. [21] | 6.45 | 47.2 | |
| Naquash et al. [22] | 5.90 | 51.37 | |
| Lee et al. [23] | 4.55 | 67 | |
| Kim et al. [24] | 9.477 | 34 | |
| LNG cold energy pre-cooling | Yang et al. [25] | 11.05 | - |
| Bian et al. [26] | 6.60 | 47 | |
| Riaz et al. [27] | 7.64 | 42.25 | |
| geothermal energy | Yilmaz [9] | 10.06 | 78.3 |
| Yilmaz and Kaska [28] | 11.52 | 69.44 | |
| Seyam et al. [29] | 6.41 | 63.4 | |
| solar energy | Ghorbani et al. [30] | 8.592 | 72.41 |
| Karimi et al. [31] | 5.72 | - | |
| Parameters | Components (mol %) | |||||||
|---|---|---|---|---|---|---|---|---|
| C1 | C2 | C3 | i-C4 | n-C4 | i-C5 | n-C5 | N2 | |
| Value | 87.36 | 6.69 | 3.50 | 0.59 | 0.89 | 0.29 | 0.19 | 0.49 |
| Parameters | Value | Reference |
|---|---|---|
| Adiabatic efficiency of compressor and pump | 90% | [6,19,35] |
| Adiabatic efficiency of the expander | 85% | [6,19,35] |
| Pressure drop of heat exchanger and cooler | 0 | [21] |
| The outlet temperature of the cooler | 25 °C | [34] |
| The molar ratio of ammonia to water in an ammonia solution | 3:1 | [36] |
| Constraint Condition | Value |
|---|---|
| MITA of heat exchanger (°C) [19,21,23,34] | |
| The pressure ratio of the compressor | |
| Vapor fraction of refrigerant at the compressor inlet |
| MITA constraint function: |
| ; Where and . |
| Pressure ratio constraint function: ; ; ; ; ; ; ; ; ; . |
| Vapor fraction constraint functions: ; ; ; ; ; . |
| Parameters | Value |
|---|---|
| Size of populations | 500 |
| Individual and social learning factors | 2 |
| Inertial weight | 0.8 |
| Maximum number of iterations | 500 |
| Optimization Variables | Base Case | Proposed Process | Percentage Change (%) 1 | |
|---|---|---|---|---|
| Pre-cooling cycle: MR cycle | ||||
| Flow rate (kg/h) | 44,243.80 | 37,990.70 | −14.13 | |
| 75,061.31 | 82,048.94 | +9.31 | ||
| 69,013.26 | 73,502.00 | +6.50 | ||
| 88,408.96 | 89,821.49 | +1.60 | ||
| 38,510.84 | 34,561.65 | −10.25 | ||
| 436.50 | 145.85 | −66.59 | ||
| Total flow rate (kg/h) | 315,674.67 | 318,070.63 | +0.76 | |
| Condensation pressure PPR11 (kPa) | 201.69 | 227.71 | +12.91 | |
| Condensation pressure PPR5 (kPa) | 632.18 | 748.90 | +18.46 | |
| Evaporation pressure PPR20 (kPa) | 5150.64 | 4355.28 | −15.44 | |
| Pre-cooling cycle: CO2 cycle | ||||
| Flow rate m1 (kPa) | 30,645.68 | 19,886.94 | −35.11 | |
| Condensation pressure P9 (kPa) | 2501.30 | 1329.67 | −46.84 | |
| Evaporation pressure P6 (kPa) | 6512.89 | 6457.75 | −0.85 | |
| Sub-cooling cycle: H2 Claude cycle | ||||
| Flow rate (kg/h) | mSR12 | 16,800.27 | 16,635.13 | −0.98 |
| mSR18 | 15,023.86 | 12,495.60 | −16.83 | |
| mSR19 | 21,502.93 | 20,652.90 | −3.95 | |
| Total flow rate (kg/h) | 53,327.06 | 49,783.63 | −6.64 | |
| Condensation pressure PSR20 (kPa) | 111.28 | 113.59 | +2.08 | |
| Condensation pressure PSR15/PSR17 (kPa) | 330.24 | 352.29 | +6.68 | |
| Evaporation pressure PSR9 (kPa) | 3512.18 | 3297.08 | −6.12 | |
| Flow rate of LH2 (kg/h) | 12,600 | 12,600 | 0 | |
| Equipment | Exergy Loss/kW |
|---|---|
| Compressor | |
| Pump | |
| Cooler | |
| Expander | |
| Heat exchanger | |
| Reactor | |
| Mixer | |
| J-T valve |
| Parameters | Calculating Equations and Relationships [23,39,40,41] |
|---|---|
| Equipment purchase cost | |
| Compressor | |
| Expander | |
| Pump | |
| Heat exchanger | |
| Cooler | |
| Other equipment | |
| Capital recovery factor (CRF) | |
| Total capital investment (TCI) | |
| Annual CAPEX | |
| OPEX | |
| Compression power cost | compression power (kW) |
| Labor cost | 0.3% of TCI |
| Other cost | 1% of TCI |
| O&M | 2% of TCI |
| Total annualized cost (TAC) | TAC = CAPEX + OPEX + O&M |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yan, F.; Geng, J.; Rong, G.; Sun, H.; Zhang, L.; Li, J. Optimization and Analysis of an Integrated Liquefaction Process for Hydrogen and Natural Gas Utilizing Mixed Refrigerant Pre-Cooling. Energies 2023, 16, 4239. https://doi.org/10.3390/en16104239
Yan F, Geng J, Rong G, Sun H, Zhang L, Li J. Optimization and Analysis of an Integrated Liquefaction Process for Hydrogen and Natural Gas Utilizing Mixed Refrigerant Pre-Cooling. Energies. 2023; 16(10):4239. https://doi.org/10.3390/en16104239
Chicago/Turabian StyleYan, Fengyuan, Jinliang Geng, Guangxin Rong, Heng Sun, Lei Zhang, and Jinxu Li. 2023. "Optimization and Analysis of an Integrated Liquefaction Process for Hydrogen and Natural Gas Utilizing Mixed Refrigerant Pre-Cooling" Energies 16, no. 10: 4239. https://doi.org/10.3390/en16104239
APA StyleYan, F., Geng, J., Rong, G., Sun, H., Zhang, L., & Li, J. (2023). Optimization and Analysis of an Integrated Liquefaction Process for Hydrogen and Natural Gas Utilizing Mixed Refrigerant Pre-Cooling. Energies, 16(10), 4239. https://doi.org/10.3390/en16104239







