Efficient Transfer of the Medium Frequency Magnetic Field Using Anisotropic Metamaterials
Abstract
1. Introduction
2. Materials and Methods
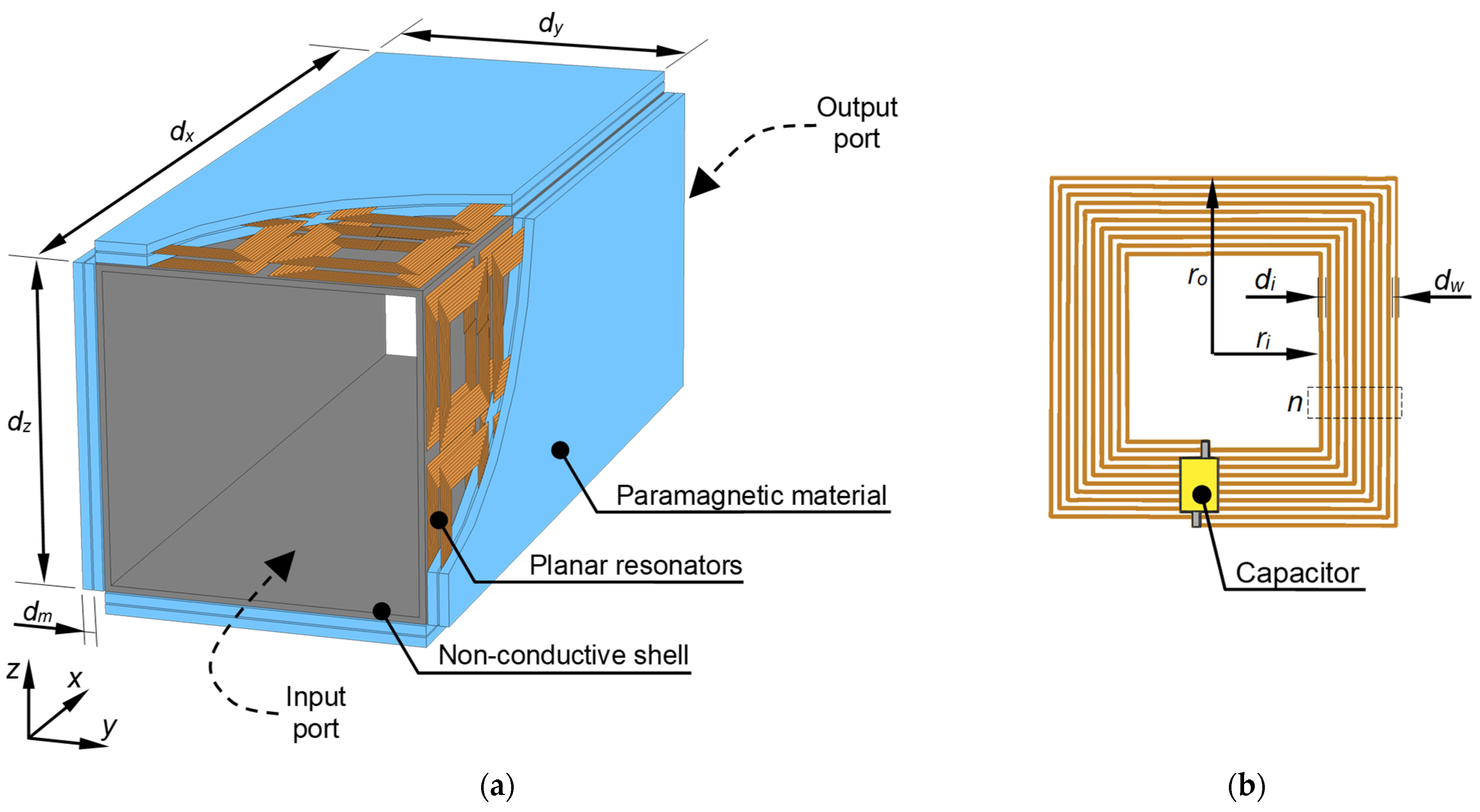
2.1. Structure of the Magnetic Meta-Hose
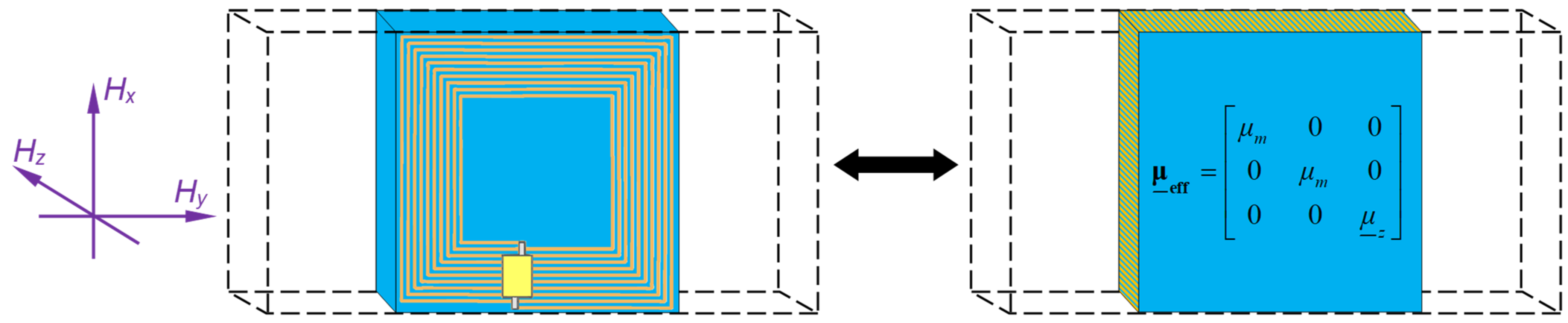
2.2. Metamaterial Properties
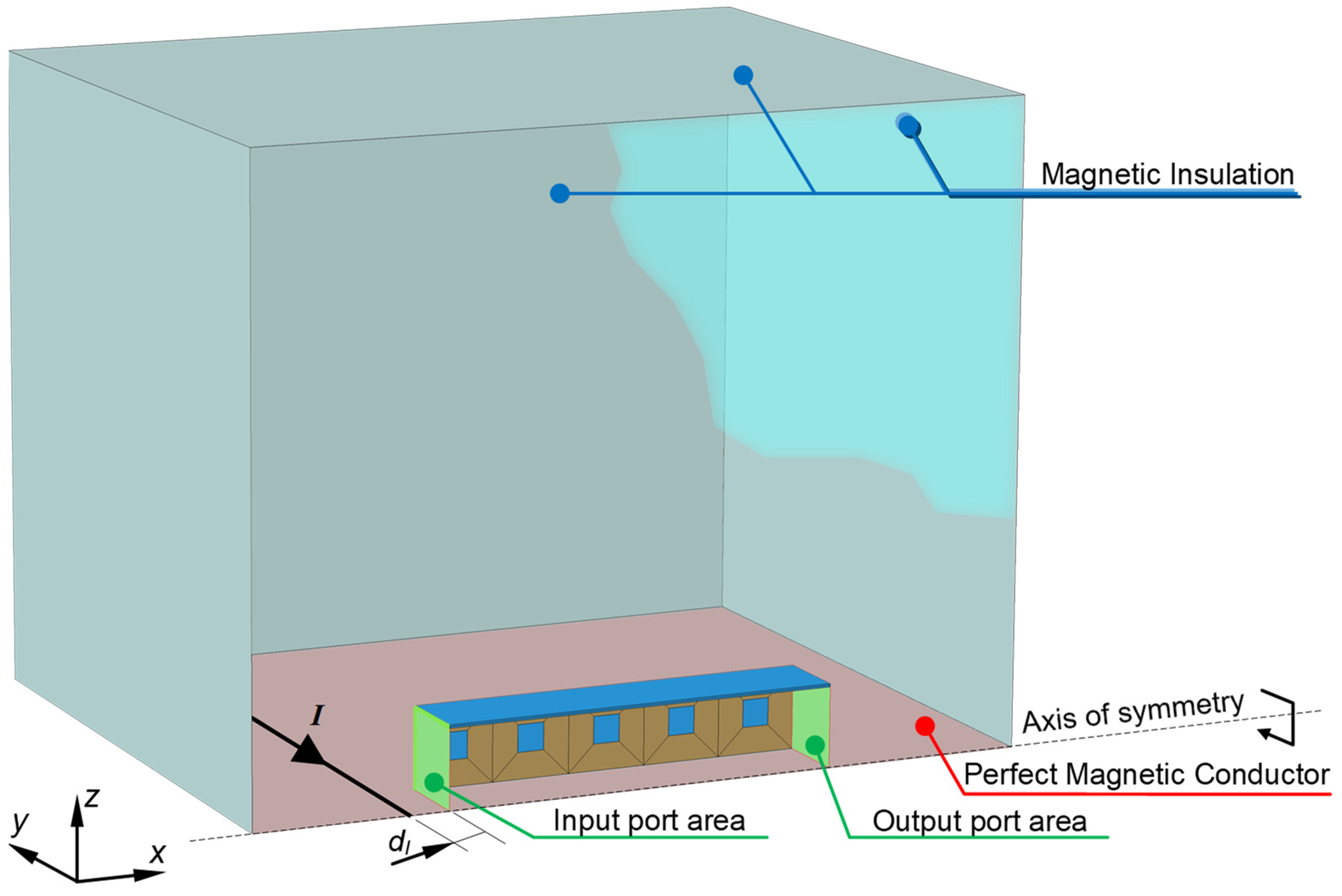
2.3. Simulation Model
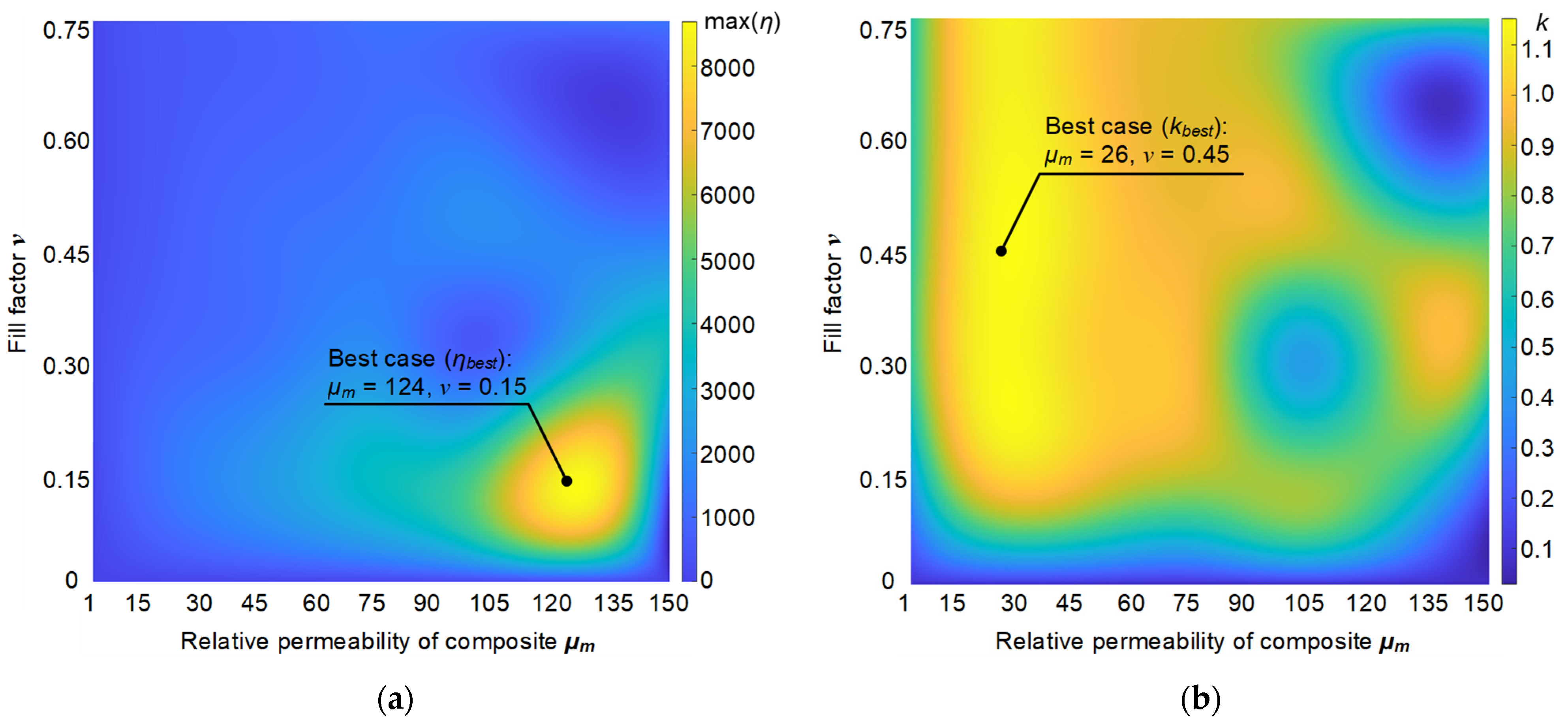
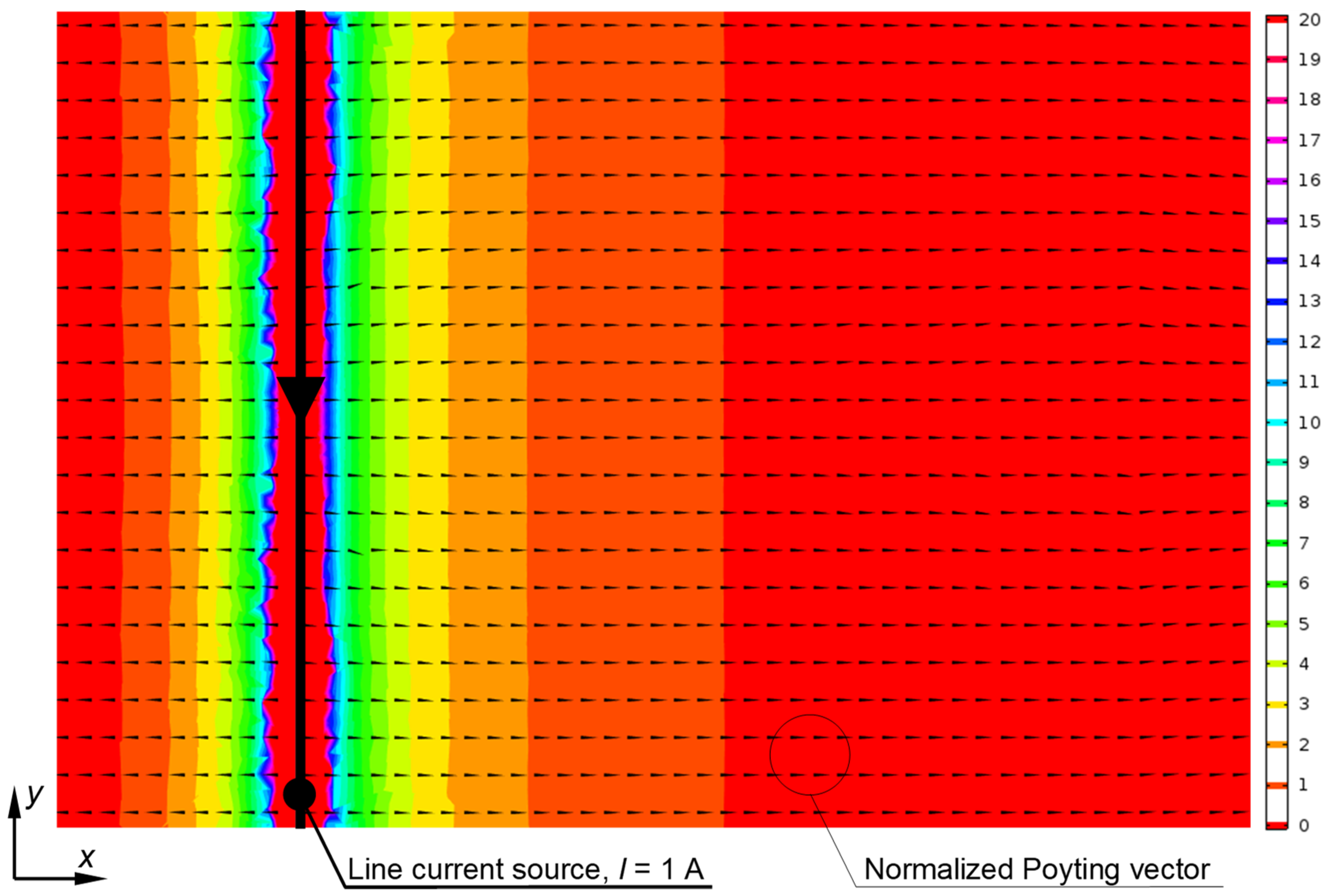
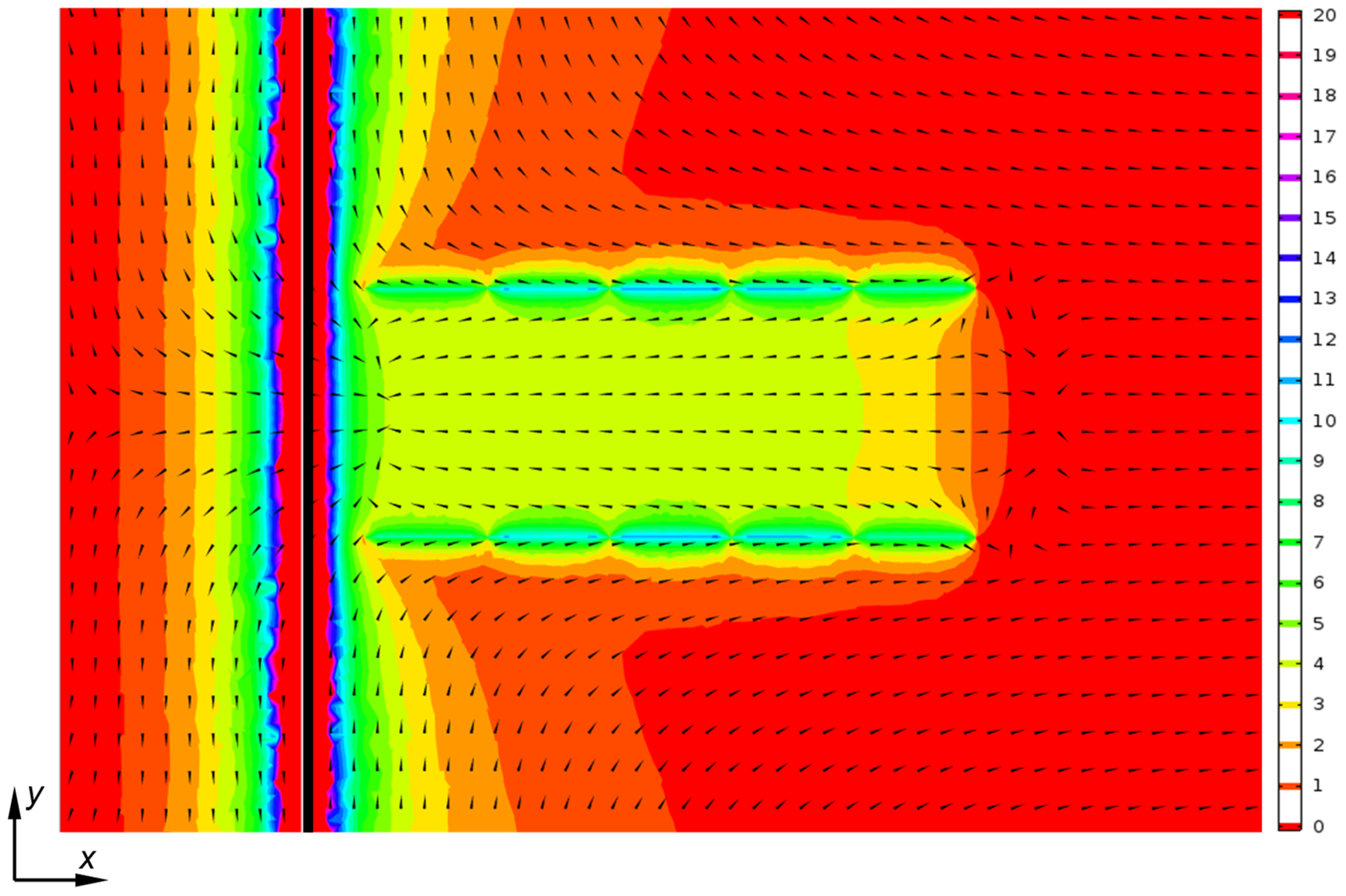
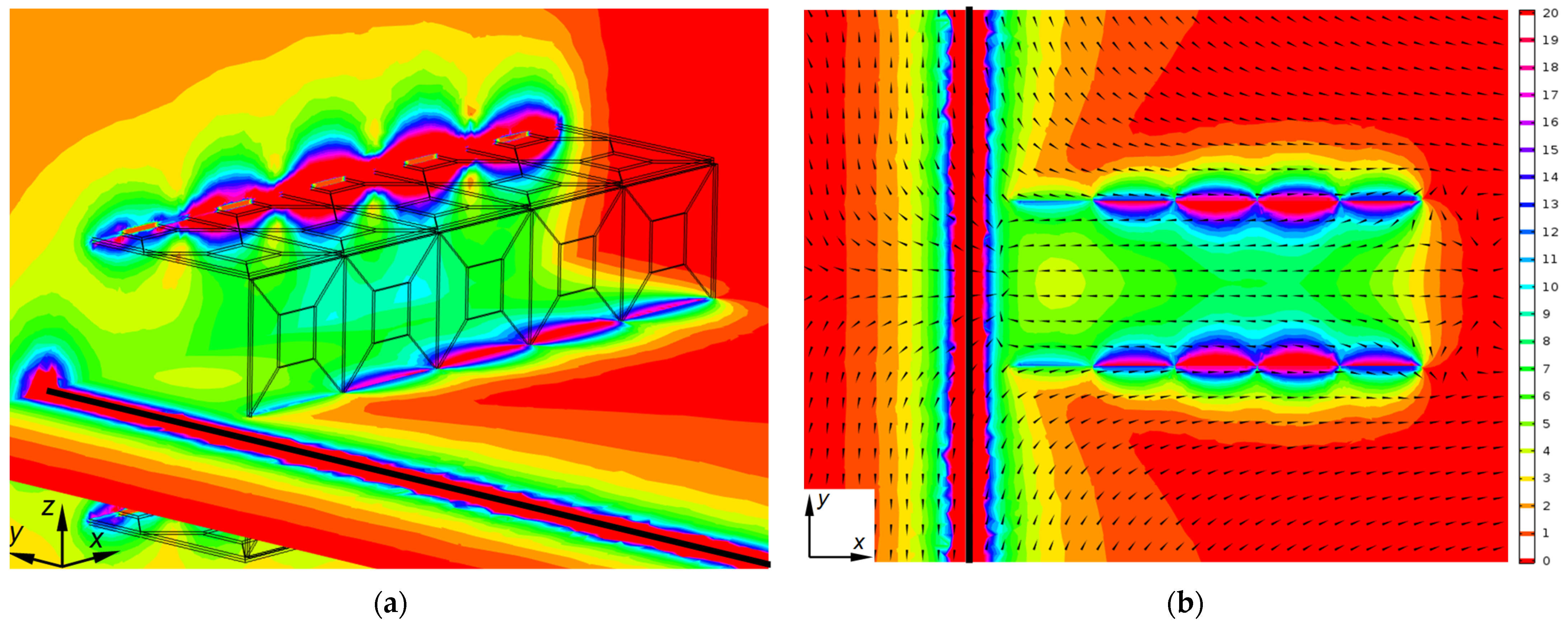
3. Results and Discussion
- (1)
- Start from ν = 0 and µm = 1.
- (2)
- Estimate the inductance (L) using Equations (1)–(4).
- (3)
- Estimate the initial capacitance (C0) using Equation (5).
- (4)
- Run the simulation and optimization algorithm.
- (5)
- Adjust the capacitance during the optimization process to find the maximum η.
- (6)
- Stop and save the magnetic field distribution after identification of max(η).
- (7)
- Increase ν. If ν > 0.9 then increase µm and set ν = 0.
- (8)
- Execute points (2)–(7).
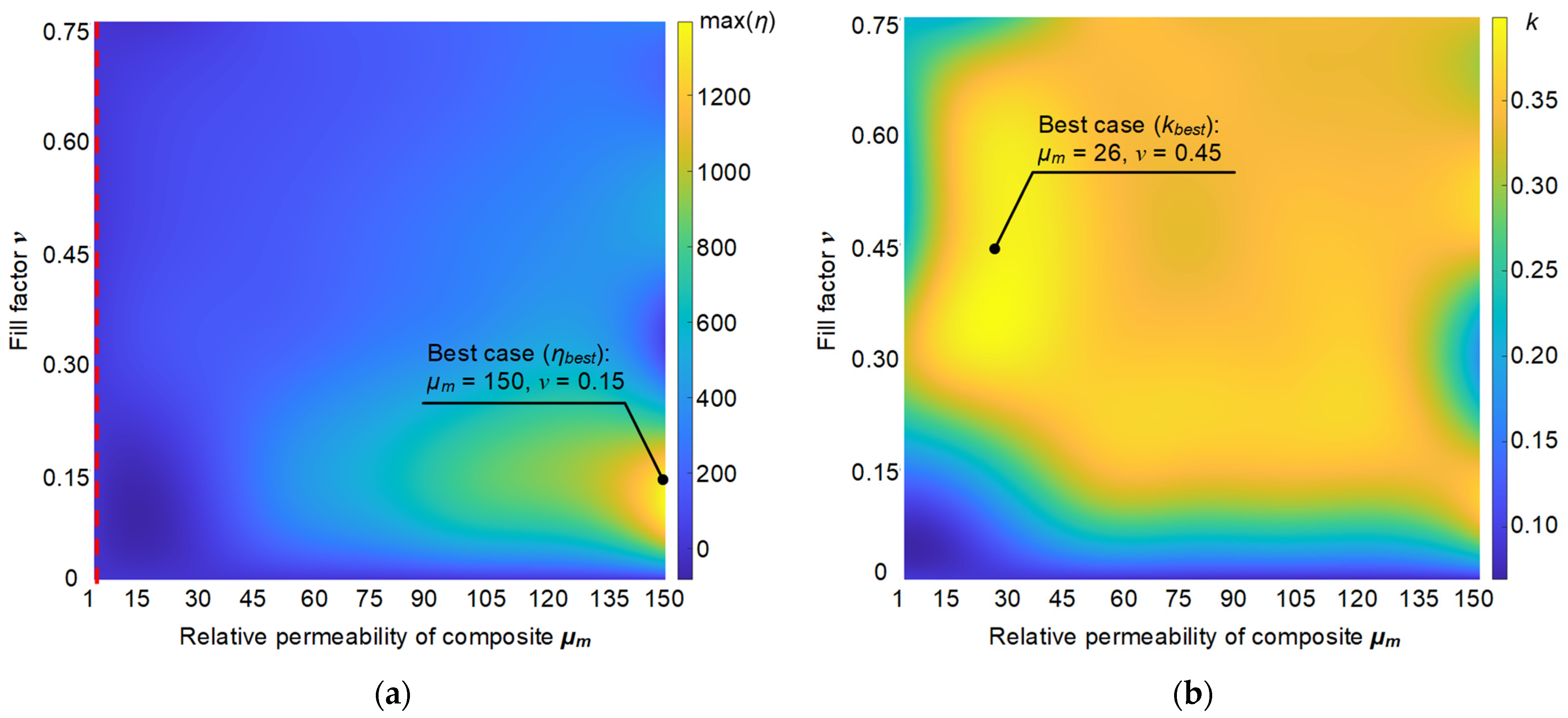
3.1. Optimum Cases for Different Parameters of Metamaterial
3.2. Magnetic Field Distribution
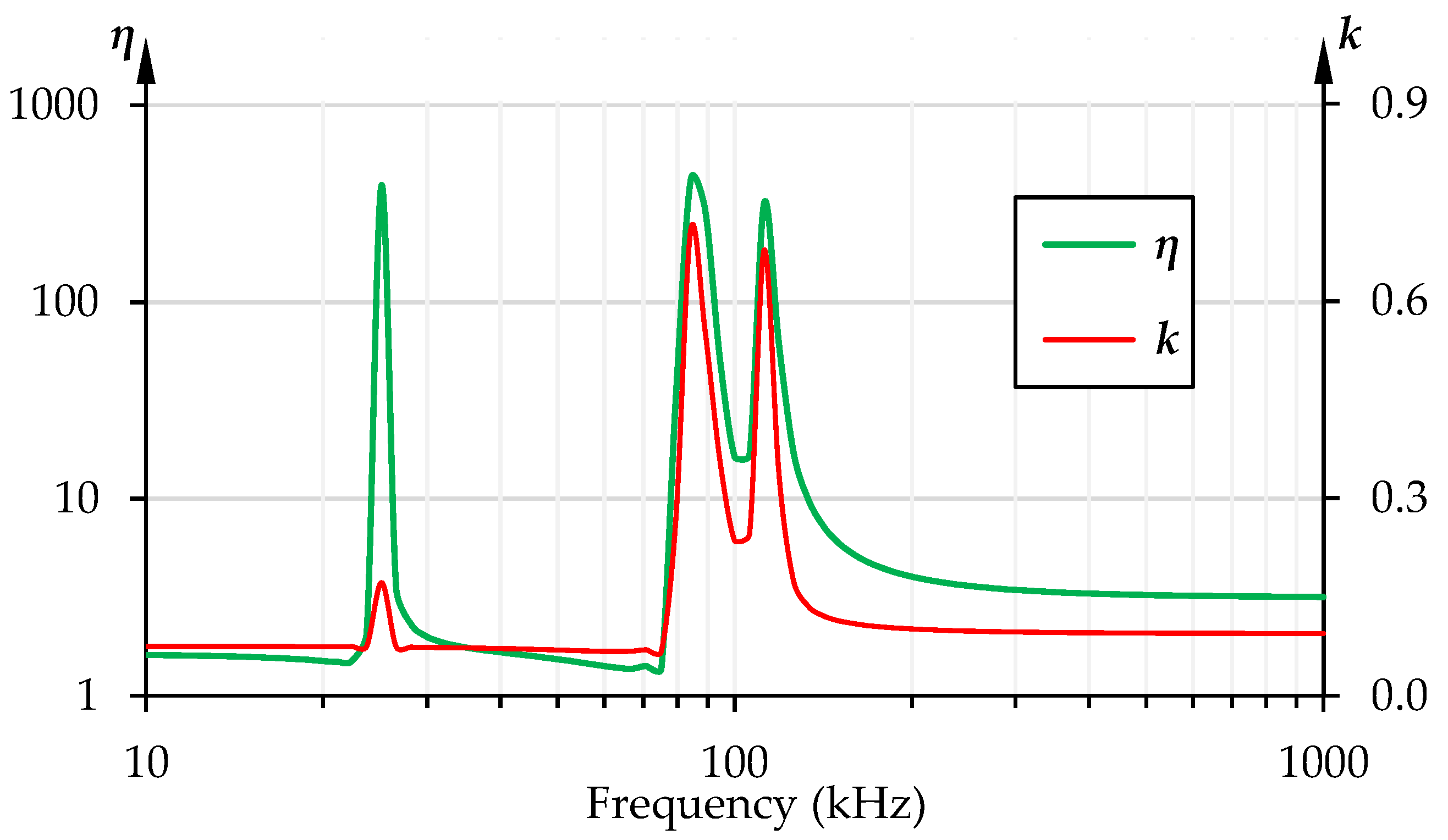
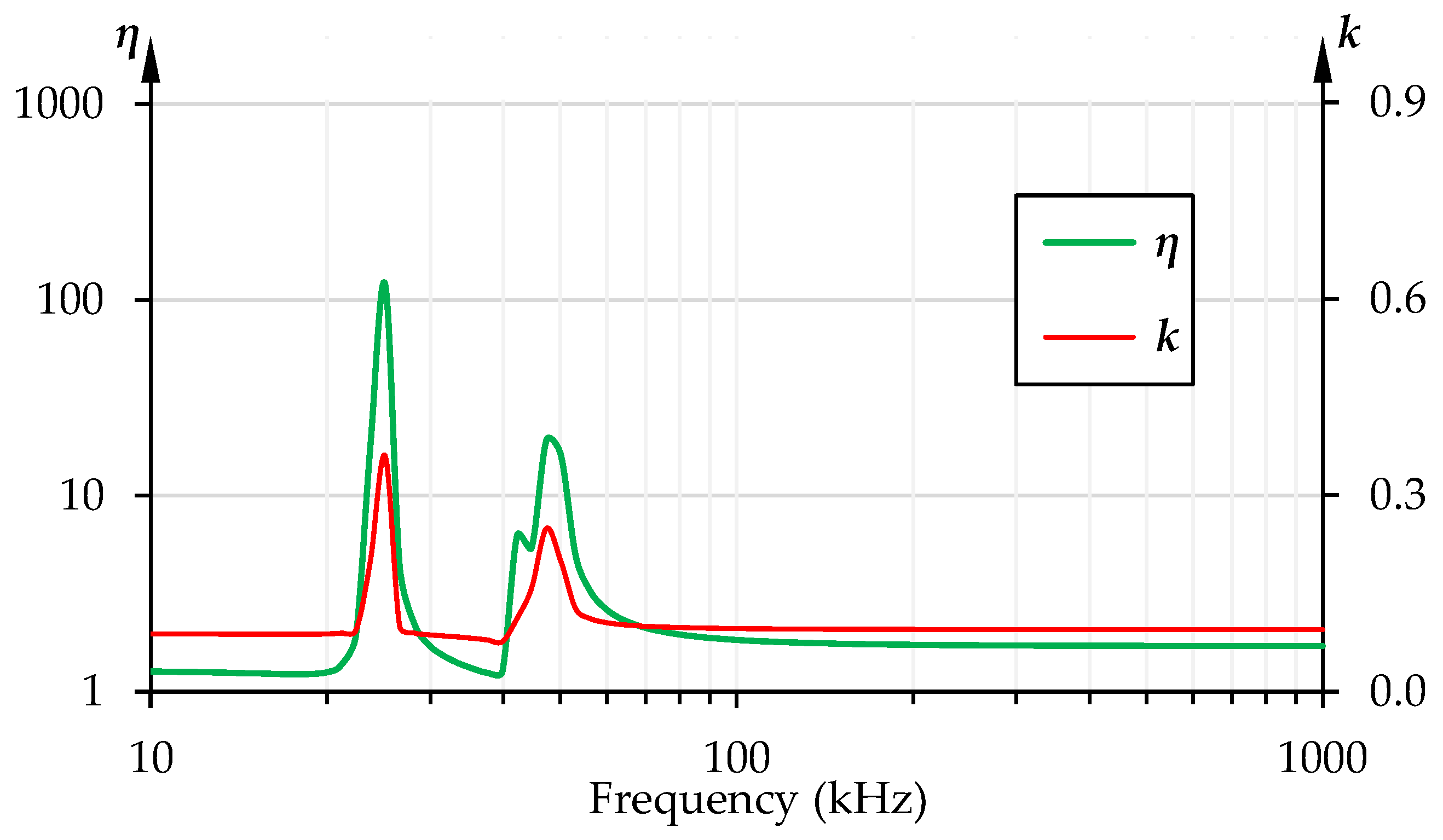
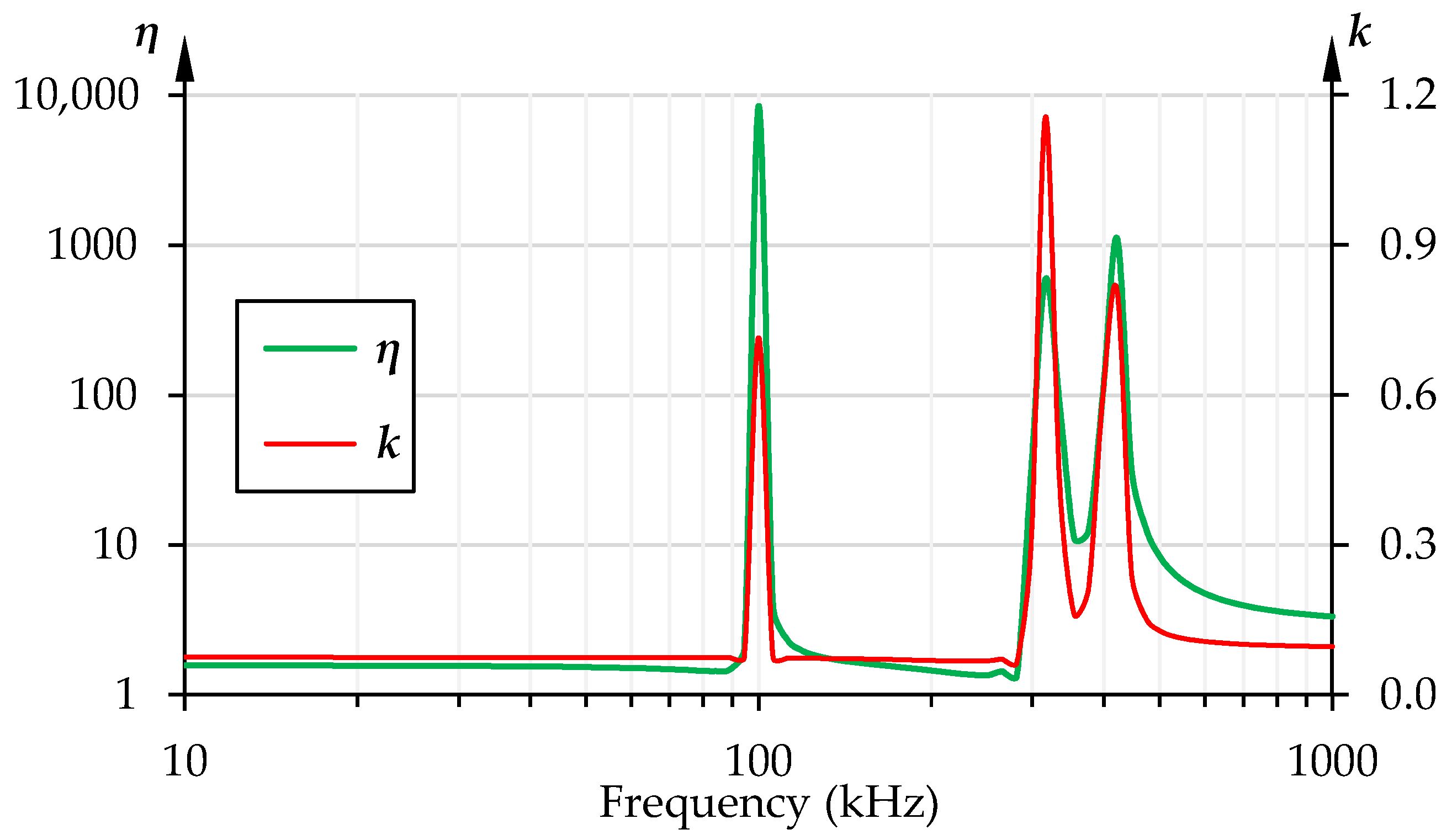
3.3. Frequency Response of MMH
4. Conclusions
Supplementary Materials
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Elahi, E.; Dastgeer, G.; Nazir, G.; Nisar, S.; Bashir, M.; Qureshi, H.A.; Kim, D.-K.; Aziz, J.; Aslam, M.; Hussain, K.; et al. A review on two-dimensional (2D) magnetic materials and their potential applications in spintronics and spin-caloritronic. Comput. Mater. Sci. 2022, 213, 111670. [Google Scholar] [CrossRef]
- Ali, A.; Mitra, A.; Aïssa, B. Metamaterials and Metasurfaces: A Review from the Perspectives of Materials, Mechanisms and Advanced Metadevices. Nanomaterials 2022, 12, 1027. [Google Scholar] [CrossRef] [PubMed]
- Jiang, W.; Ma, Y.; Zhu, J.; Yin, G.; Liu, Y.; Yuan, J.; He, S. Room-temperature broadband quasistatic magnetic cloak. NPG Asia Mater. 2017, 9, e341. [Google Scholar] [CrossRef]
- Zhu, J.; Jiang, W.; Liu, Y.; Yin, G.; Yuan, J.; He, S.; Ma, Y. Three-dimensional magnetic cloak working from dc to 250 kHz. Nat. Commun. 2015, 6, 8931. [Google Scholar] [CrossRef]
- Navau, C.; Prat-Camps, J.; Sanchez, A. Magnetic Energy Harvesting and Concentration at a Distance by Transformation Optics. Phys. Rev. Lett. 2012, 109, 263903. [Google Scholar] [CrossRef]
- Sun, F.; He, S. Static magnetic field concentration and enhancement using magnetic materials with positive permeability. PIER 2014, 142, 579. [Google Scholar] [CrossRef]
- Navau, C.; Mach-Battle, R.; Parra, A.; Prat-Camps, J.; Laut, S.; Del-Valle, N.; Sanchez, A. Enhancing the sensitivity of magnetic sensors by 3D metamaterial shells. Sci. Rep. 2017, 7, 44762. [Google Scholar] [CrossRef]
- Prat-Camps, J.; Navau, C.; Sanchez, A. A Magnetic Wormhole. Sci. Rep. 2015, 5, 12488. [Google Scholar] [CrossRef]
- Mach-Battle, R.; Navau, C.; Sanchez, A. Invisible magnetic sensors. Appl. Phys. Lett. 2018, 112, 162406. [Google Scholar] [CrossRef]
- Steckiewicz, A. High-frequency cylindrical magnetic cloaks with thin layer structure. J. Magn. Magn. Mater. 2021, 534, 168039. [Google Scholar] [CrossRef]
- Bakir, M.; Karaaslan, M.; Dincer, F.; Delihacioglu, K.; Sabah, C. Perfect metamaterial absorber-based energy harvesting and sensor applications in the industrial, scientific, and medical band. Opt. Eng. 2015, 54, 097102. [Google Scholar] [CrossRef]
- Capobianco-Hogan, K.G.; Cervantes, R.; Deshpande, A.; Feege, N.; Krahulik, T.; LaBounty, J.; Sekelsky, R.; Adhyatman, A.; Arrowsmith-Kron, G.; Coe, B.; et al. A magnetic field cloak for charged particle beams. Nucl. Instrum. 2018, 877, 149–156. [Google Scholar] [CrossRef]
- Bhardwaj, A.; Pratap, D.; Semple, M.; Iyer, A.K.; Jayannavar, A.M.; Ramakrishna, S.A. Properties of waveguides filled with anisotropic metamaterials. C. R. Phys. 2020, 21, 677–711. [Google Scholar] [CrossRef]
- Han, T.; Qiu, C.-W. Transformation Laplacian metamaterials: Recent advances in manipulating thermal and dc fields. J. Opt. 2016, 18, 044003. [Google Scholar] [CrossRef]
- Suresh Kumar, N.; Naidu, K.C.B.; Banerjee, P.; Anil Babu, T.; Venkata Shiva Reddy, B. A Review on Metamaterials for Device Applications. Crystals 2021, 11, 518. [Google Scholar] [CrossRef]
- Shan, D.; Wang, H.; Cao, K.; Zhang, J. Wireless power transfer system with enhanced efficiency by using frequency reconfigurable metamaterial. Sci. Rep. 2022, 12, 331. [Google Scholar] [CrossRef]
- Huang, X.; Zhang, C.; Cong, L.; Cai, R.; Yang, F.; Lu, C. Development and prospects of metamaterial in wireless power transfer. IET Power Electron. 2021, 14, 2423–2440. [Google Scholar] [CrossRef]
- Lee, W.; Yoon, Y.-K. Wireless Power Transfer Systems Using Metamaterials: A Review. IEEE Access 2020, 8, 147930–147947. [Google Scholar] [CrossRef]
- Li, W.; Wang, P.; Yao, C.; Zhang, Y.; Tang, H. Experimental investigation of 1D, 2D, and 3D metamaterials for efficiency enhancement in a 6.78 MHz wireless power transfer system. In Proceedings of the IEEE Wireless Power Transfer Conference (WPTC), Aveiro, Portugal, 5–6 May 2016; pp. 1–4. [Google Scholar] [CrossRef]
- Stevens, C.J. Magnetoinductive Waves and Wireless Power Transfer. IEEE Trans. Power Electron. 2015, 30, 6182–6190. [Google Scholar] [CrossRef]
- Alberto, J.; Reggiani, U.; Sandrolini, L.; Albuquerque, H. Accurate Calculation of the Power Transfer and Efficiency in Resonator Arrays for Inductive Power Transfer. PIER B 2019, 83, 61–76. [Google Scholar] [CrossRef]
- Alberto, J.; Reggiani, U.; Sandrolini, L.; Albuquerque, H. Fast Calculation and Analysis of the Equivalent Impedance of a Wireless Power Transfer System Using an Array of Magnetically Coupled Resonators. PIER B 2018, 80, 101–112. [Google Scholar] [CrossRef][Green Version]
- Zhong, W.; Lee, C.K.; Hui, S.Y.R. General Analysis on the Use of Tesla’s Resonators in Domino Forms for Wireless Power Transfer. IEEE Trans. Ind. Electron. 2013, 60, 261–270. [Google Scholar] [CrossRef]
- Wang, B.; Yerazunis, W.; Teo, K.H. Wireless Power Transfer: Metamaterials and Array of Coupled Resonators. Proc. IEEE 2013, 101, 1359–1368. [Google Scholar] [CrossRef]
- Navau, C.; Prat-Camps, J.; Romero-Isart, O.; Cirac, J.I.; Sanchez, A. Long-distance Transfer and Routing of Static Magnetic Fields. Phys. Rev. Lett. 2014, 112, 253901. [Google Scholar] [CrossRef] [PubMed]
- Zhou, P.; Ma, G.; Liu, H.; Li, X.; Zhang, H.; Yang, C.; Ye, C. Transportation of Static Magnetic Fields by a practically realizable Magnetic Hose. IEEE Magn. Lett. 2016, 7, 1300304. [Google Scholar] [CrossRef]
- Sohr, P.; Wei, D.; Wang, Z.; Law, S. Strong Coupling in Semiconductor Hyperbolic Metamaterials. Nano Lett. 2021, 21, 9951–9957. [Google Scholar] [CrossRef]
- Liang, Y.; Koshelev, K.; Zhang, F.; Lin, H.; Lin, S.; Wu, J.; Jia, B.; Kivshar, Y. Bound States in the Continuum in Anisotropic Plasmonic Metasurfaces. Nano Lett. 2020, 20, 6351–6356. [Google Scholar] [CrossRef]
- Moayyed, F.; Dalili Oskouei, H.R.; Mohammadi Shirkolaei, M. High Gain and Wideband Multi-Stack Multilayer Anisotropic Dielectric Antenna. PIER 2021, 99, 103–109. [Google Scholar] [CrossRef]
- Mohammadi Shirkolaei, M.; Ghalibafan, J. Magnetically scannable slotted waveguide antenna based on the ferrite with gain enhancement. Waves Random Complex Media 2021, 1, 1–11. [Google Scholar] [CrossRef]
- Mohammadi Shirkolaei, M.; Aslinezhad, M. Substrate integrated waveguide filter based on the magnetized ferrite with tunable capability. Microw. Opt. Technol. Lett. 2020, 63, 1120–1125. [Google Scholar] [CrossRef]
- Seo, Y.-S.; Hughes, Z.; Hoang, M.; Isom, D.; Nguyen, M.; Rao, S.; Chiao, J.-C. Investigation of wireless power transfer in through-wall applications. In Proceedings of the Asia Pacific Microwave Conference Proceedings, Kaohsiung, Taiwan, 4–7 December 2012; pp. 403–405. [Google Scholar] [CrossRef]
- Wu, K.; Zhao, X.; Bifano, T.G.; Anderson, S.W.; Zhang, X. Auxetics-inspired tunable metamaterials for magnetic resonance imaging. Adv. Mater. 2021, 34, 2109032. [Google Scholar] [CrossRef] [PubMed]
- Hurshkainen, A.; Nikulin, A.; Georget, E.; Larrat, B.; Berrahou, D.; Neves, A.L.; Sabouroux, P.; Enoch, S.; Melchakova, I.; Belov, P.; et al. A novel metamaterial-inspired RF-coil for preclinical dual-nuclei MRI. Sci. Rep. 2018, 8, 9190. [Google Scholar] [CrossRef] [PubMed]
- Markevicius, V.; Navikas, D.; Idzkowski, A.; Valinevicius, A.; Zilys, M.; Janeliauskas, A.; Walendziuk, W.; Andriukaitis, D. An Effective Method of Vehicle Speed Evaluation in Systems Using Anisotropic Magneto-Resistive Sensors. IEEE Intell. Transp. Syst. Mag. 2021, 13, 142–151. [Google Scholar] [CrossRef]
- KEMET. Product Datasheet: Flex Suppressor, Noise Suppression Sheet, Volume 19. Available online: https://content.kemet.com/datasheets/TOK_FS101.pdf (accessed on 22 November 2022).
- Mohan, S.S.; del Mar Hershenson, M.; Boyd, S.P.; Lee, T.H. Simple accurate expressions for planar spiral inductances. IEEE J. Solid-State Circuits 1999, 34, 1419–1424. [Google Scholar] [CrossRef]
- Steckiewicz, A. Homogenization of the vertically stacked medium frequency magnetic metamaterials with multi-turn resonators. Sci. Rep. 2022, 12, 20333. [Google Scholar] [CrossRef]
- Liu, J.; Gong, Z.; Yang, S.; Sun, H.; Zhou, J. Practical Model for Metamaterials in Wireless Power Transfer Systems. Appl. Sci. 2020, 10, 8506. [Google Scholar] [CrossRef]
- Staelin, D.H. Electromagnetics and Applications; Massachusetts Institute of Technology: Cambridge, MA, USA, 2011; pp. 42–45. [Google Scholar]
- Gyselinck, J.; Dular, P. Frequency-domain homogenization of bundles of wires in 2-D magnetodynamic FE calculations. IEEE Trans. Magn. 2005, 41, 1416–1419. [Google Scholar] [CrossRef]
- Meeker, D.C. An improved continuum skin and proximity effect model for hexagonally packed wires. J. Comput. Appl. Math. 2012, 236, 4635–4644. [Google Scholar] [CrossRef]
- Meeker, D.C. Continuum Representation of Wound Coils Via an Equivalent Foil Approach. Finite Element Method Magnetics Website. Available online: http://www.femm.info/examples/prox/notes.pdf (accessed on 20 November 2022).
- COMSOL Inc. AC/DC Module User’s Guide: Chapter 3. COMSOL Multiphysics Official Website. Available online: https://doc.comsol.com/5.4/doc/com.comsol.help.acdc/ACDCModuleUsersGuide.pdf (accessed on 25 November 2022).
- Stankiewicz, J.M.; Choroszucho, A. Efficiency of the Wireless Power Transfer System with Planar Coils in the Periodic and Aperiodic Systems. Energies 2022, 15, 115. [Google Scholar] [CrossRef]
- Zienkiewicz, O.C.; Taylor, R.L.; Zhu, J.Z. The Finite Element Method: Its Basis and Fundamentals, 7th ed.; Elsevier Butterworth-Heinemann: Oxford, UK, 2013; pp. 115–149. [Google Scholar] [CrossRef]



| Length | dx | 50 cm |
| Width | dy | 20 cm |
| Height | dz | 20 cm |
| Permeability of background and coils | μ0 | 4π·10−7 H/m |
| Electrical conductivity of background and paramagnetic composite | σ0 | 0 S/m |
| Electrical conductivity of wires | σw | 5.8 × 107 S/m |
| Wire diameter | dw | 0.2 mm |
| Wire insulation thickness | di | 0.02 mm |
| Composite thickness | dm | 2 mm |
| Coil diameter | 2ro | 10 cm |
| Shape coefficients | c1 | 1.46 |
| c2 | 1.9 | |
| c3 | 0.18 | |
| c4 | 0.13 | |
| Nelder-Mead reflection coeffcient | α | 1 |
| Nelder-Mead expansion coeffcient | γ | 2 |
| Nelder-Mead contraction coeffcient | ρ1 | 0.25 |
| Nelder-Mead shrink coeffcient | ρ2 | 0.25 |
| Optimality tolerance | ε | 0.01 |
| Maximum number of iterations | Max_it | 20 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Steckiewicz, A. Efficient Transfer of the Medium Frequency Magnetic Field Using Anisotropic Metamaterials. Energies 2023, 16, 334. https://doi.org/10.3390/en16010334
Steckiewicz A. Efficient Transfer of the Medium Frequency Magnetic Field Using Anisotropic Metamaterials. Energies. 2023; 16(1):334. https://doi.org/10.3390/en16010334
Chicago/Turabian StyleSteckiewicz, Adam. 2023. "Efficient Transfer of the Medium Frequency Magnetic Field Using Anisotropic Metamaterials" Energies 16, no. 1: 334. https://doi.org/10.3390/en16010334
APA StyleSteckiewicz, A. (2023). Efficient Transfer of the Medium Frequency Magnetic Field Using Anisotropic Metamaterials. Energies, 16(1), 334. https://doi.org/10.3390/en16010334






