Theoretical Investigations on Shadow Band Correction Factors for Diffuse Radiation under Isotropic Conditions without Approximation
Abstract
:1. Introduction
2. Theoretical Study
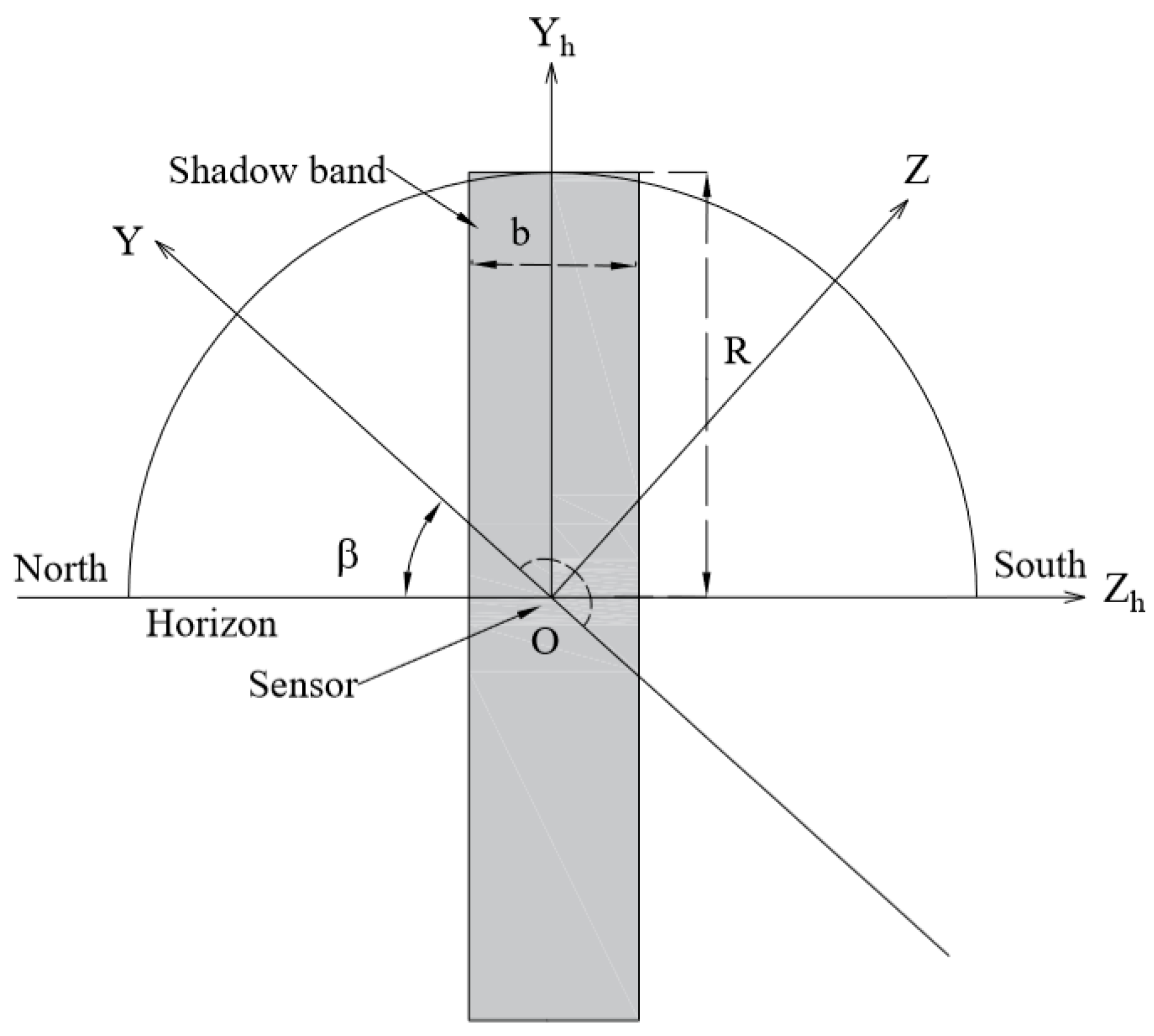
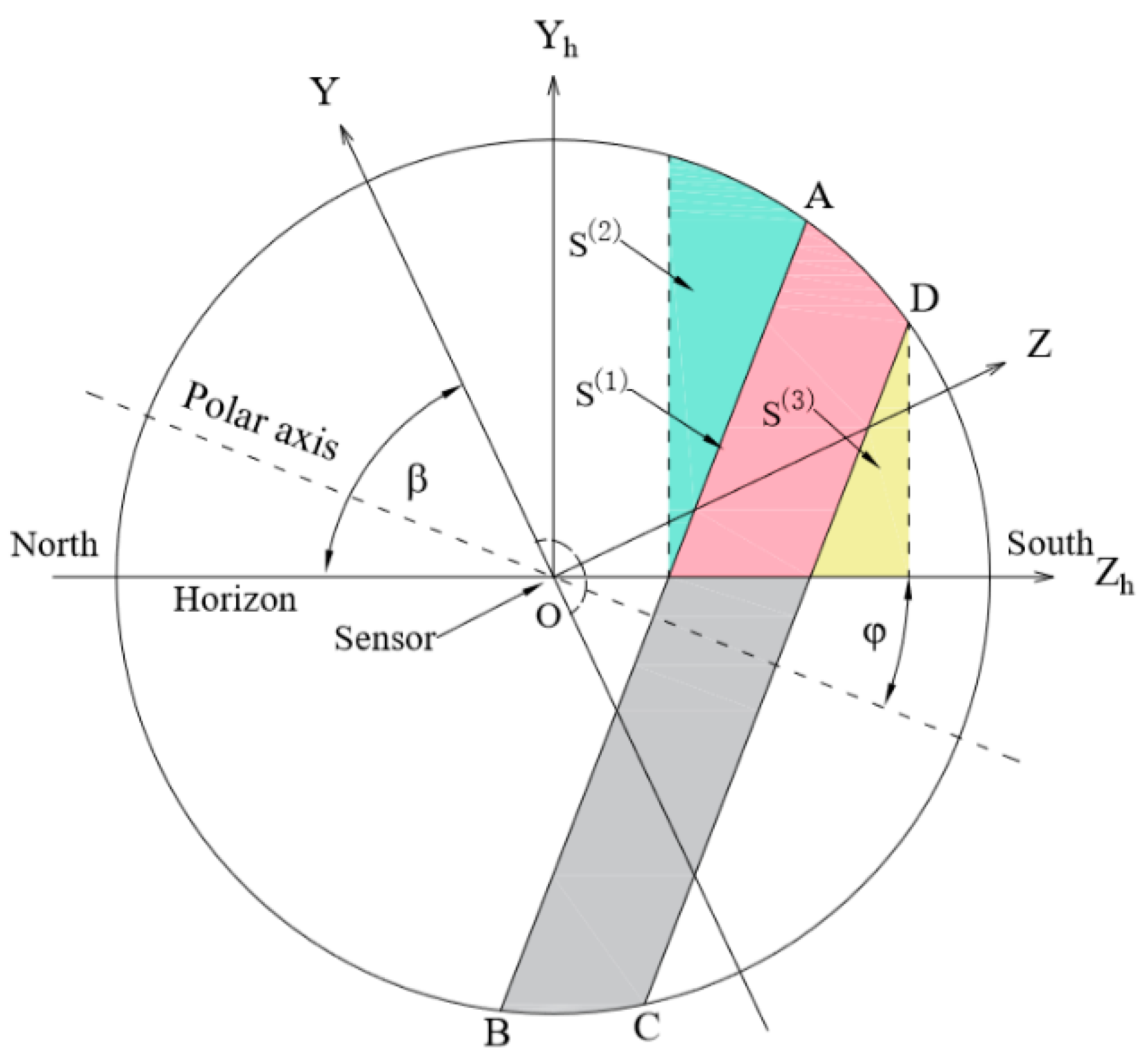
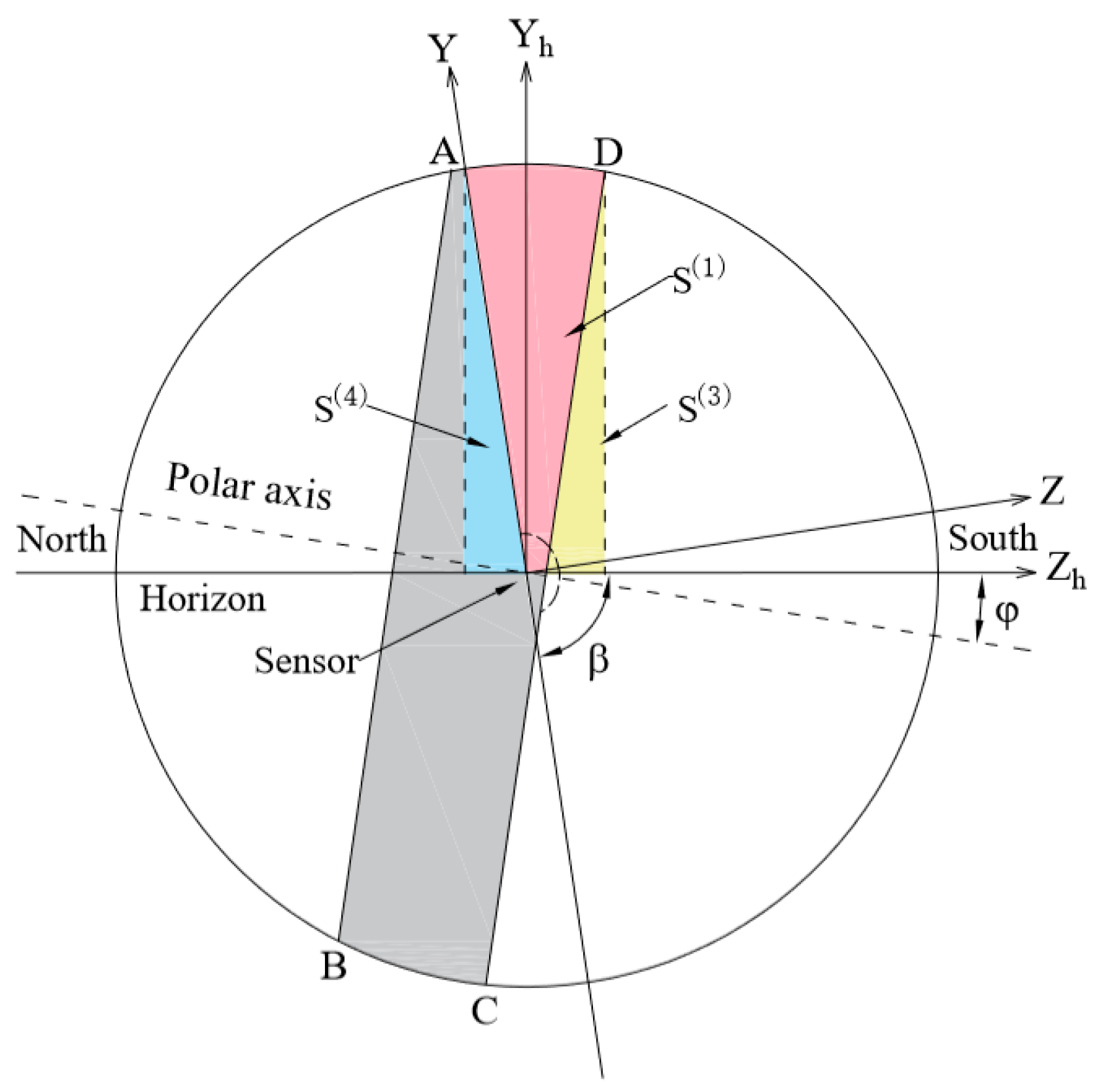
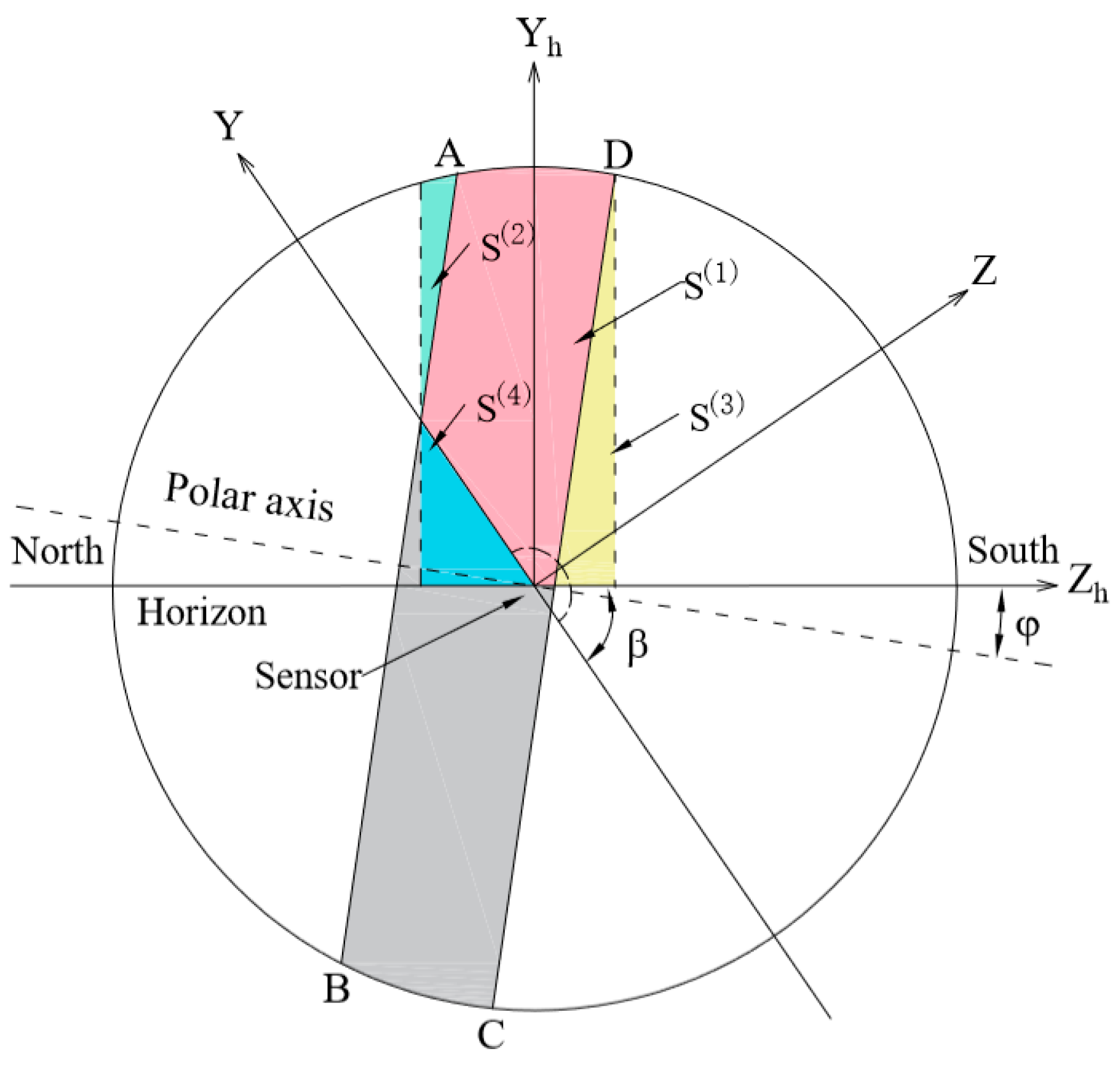
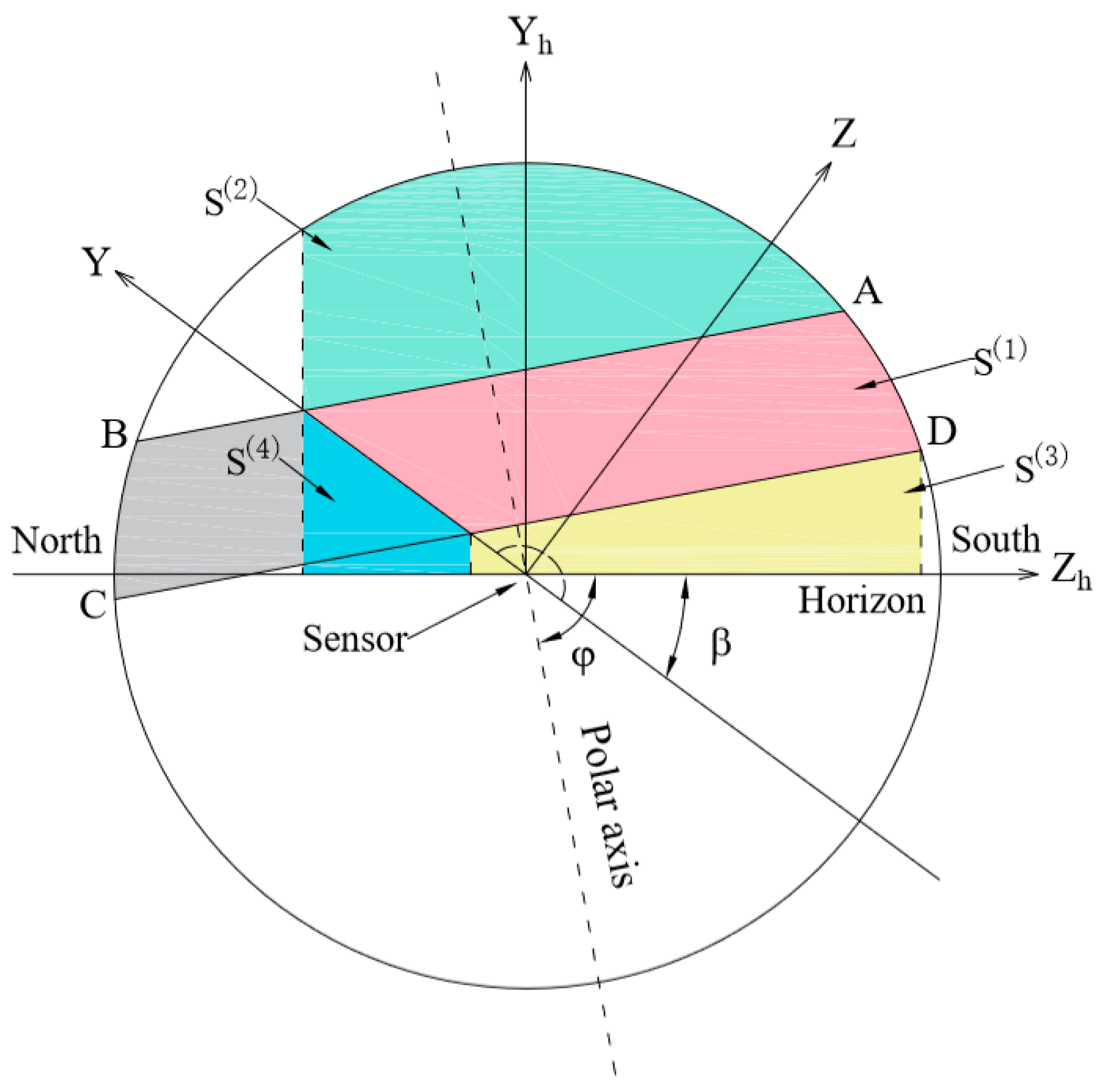
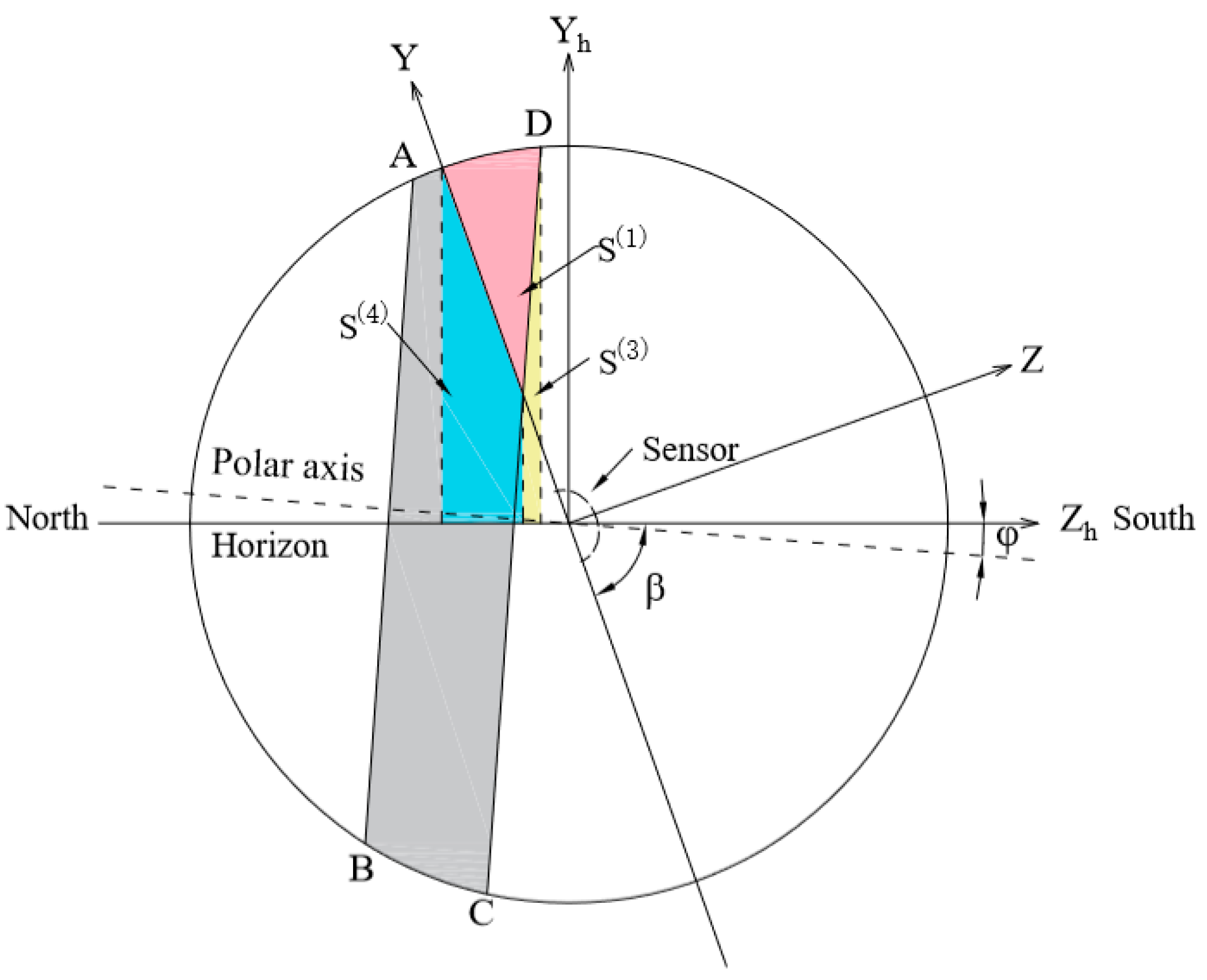
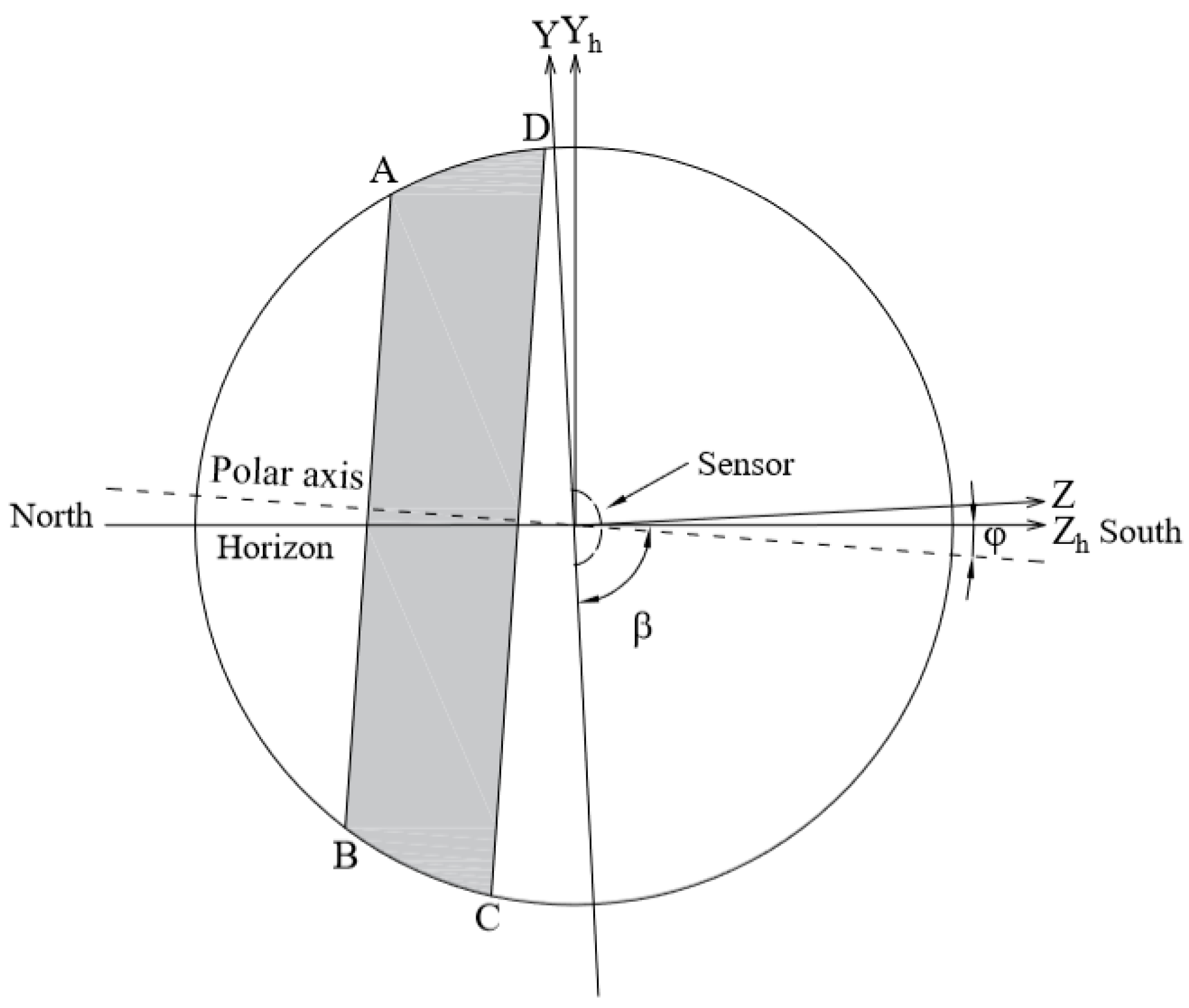
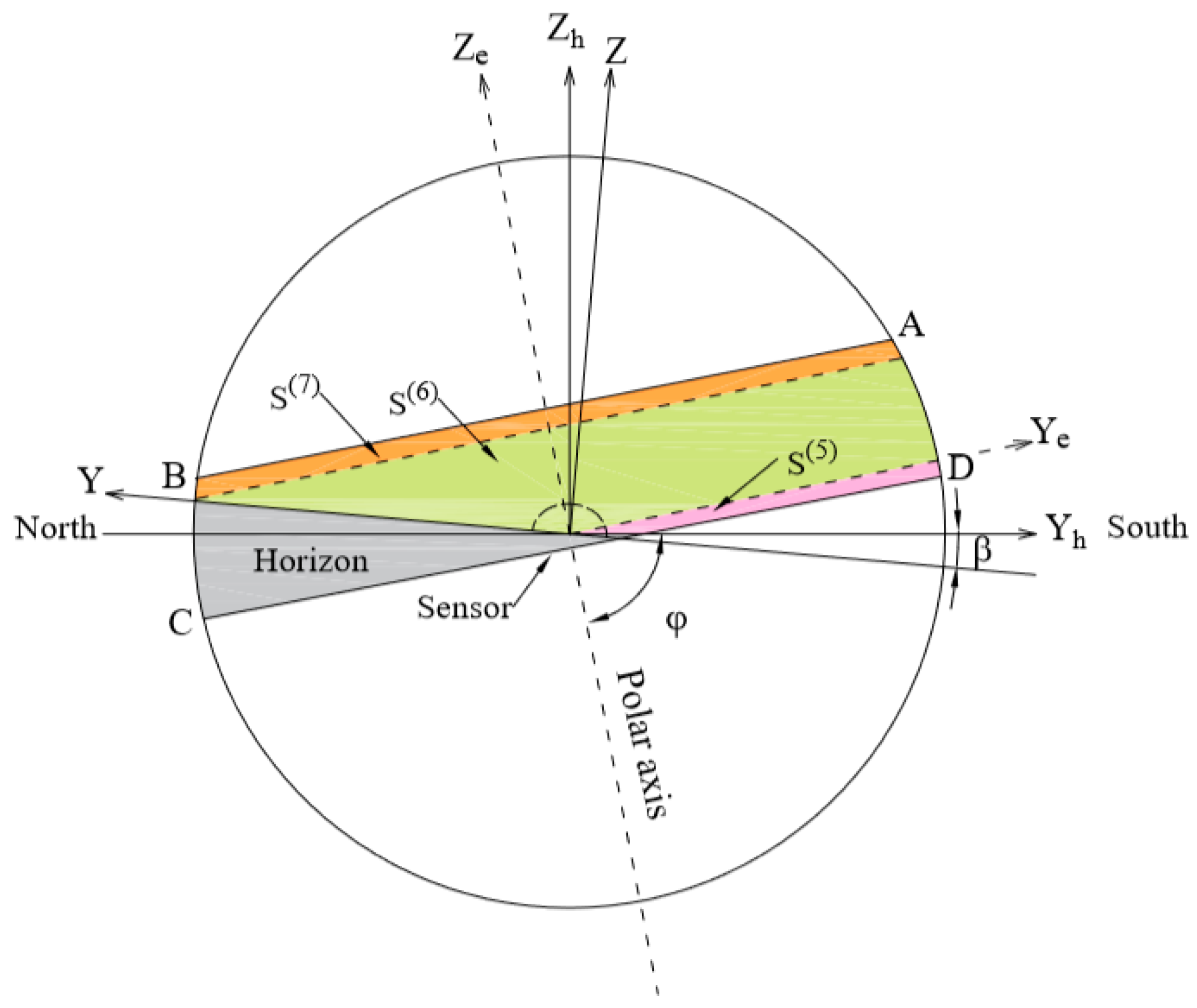
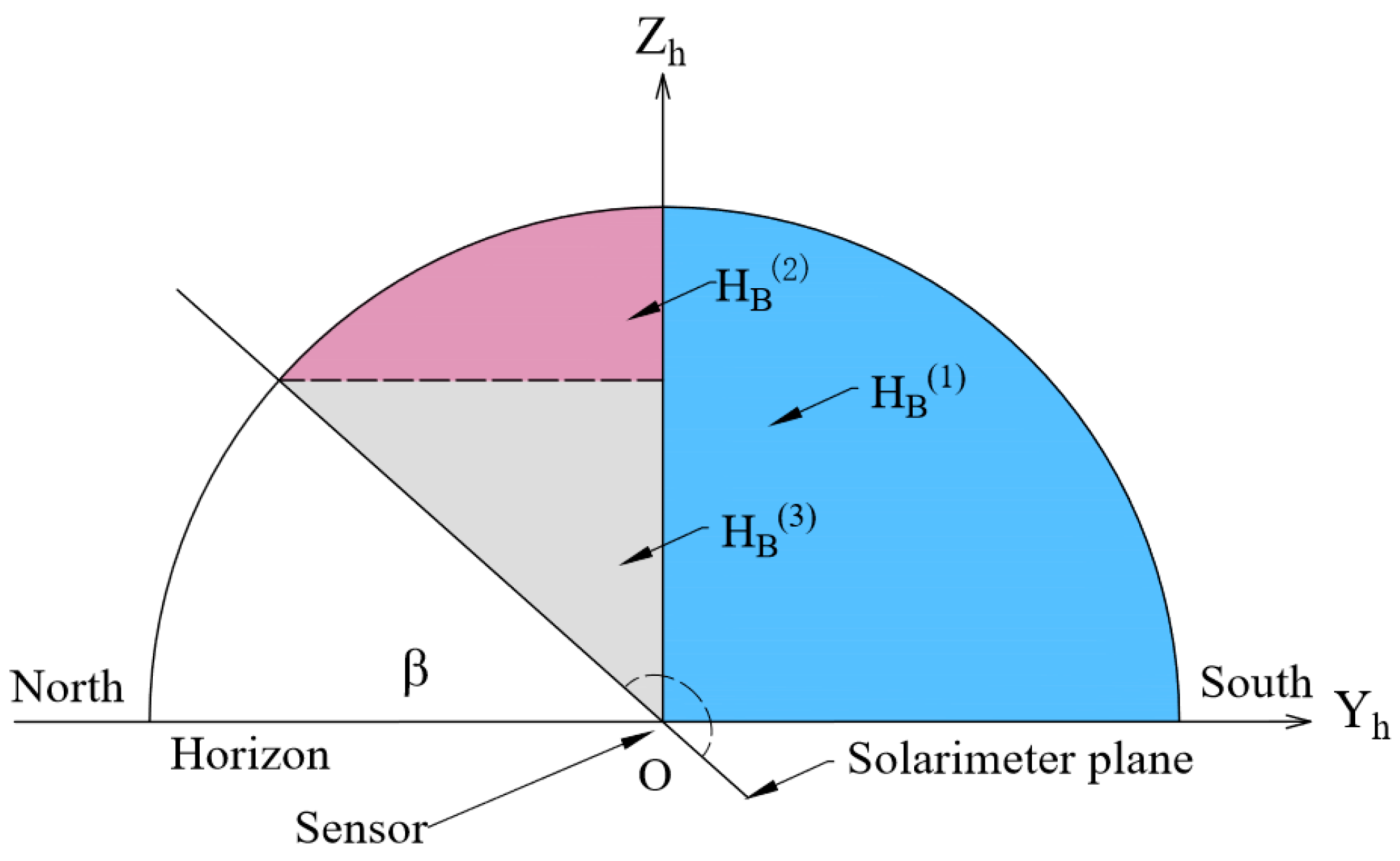
2.1. Discussions in Horizon Coordinate System
2.1.1. Calculation of
2.1.2. Calculation of
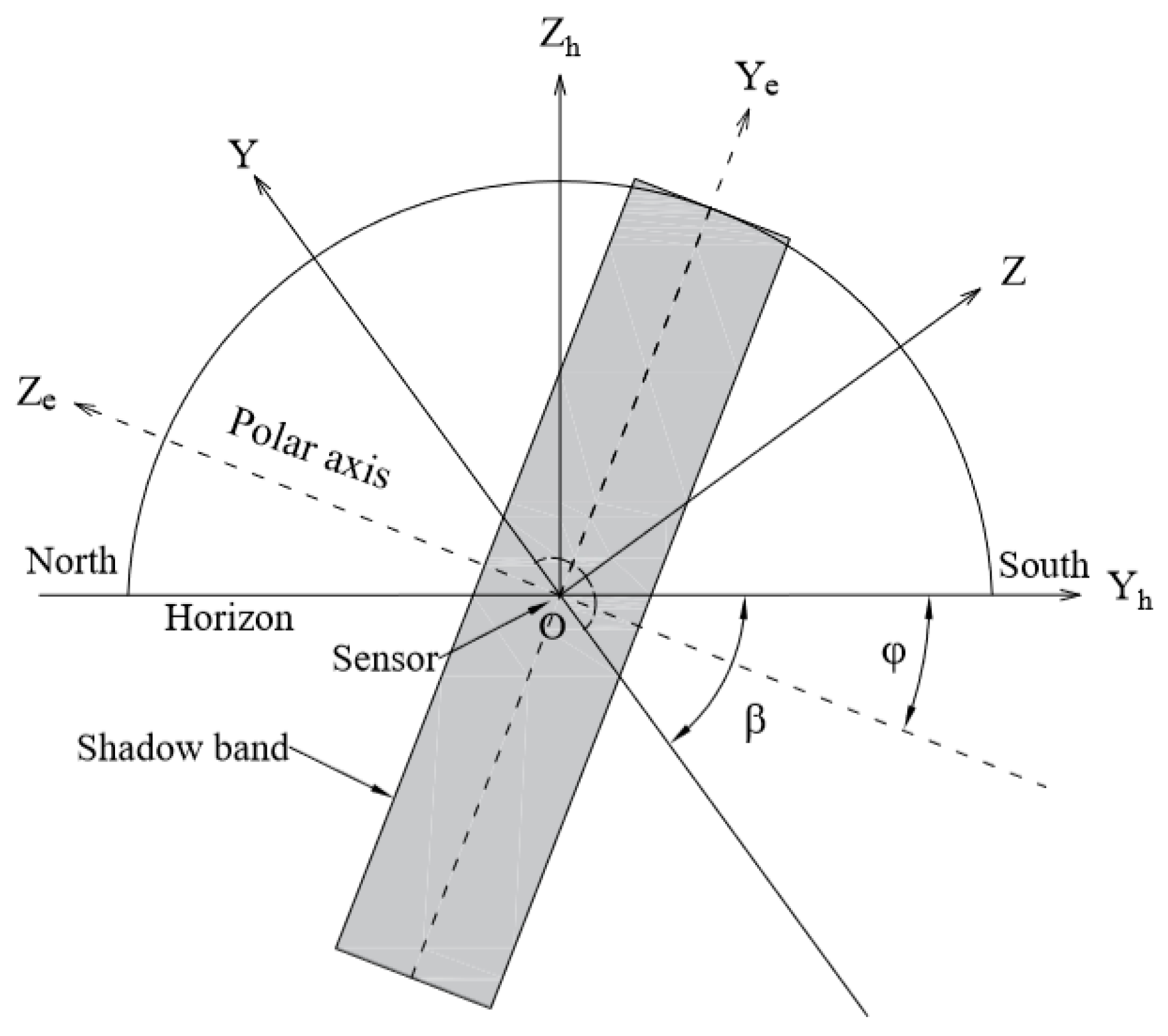
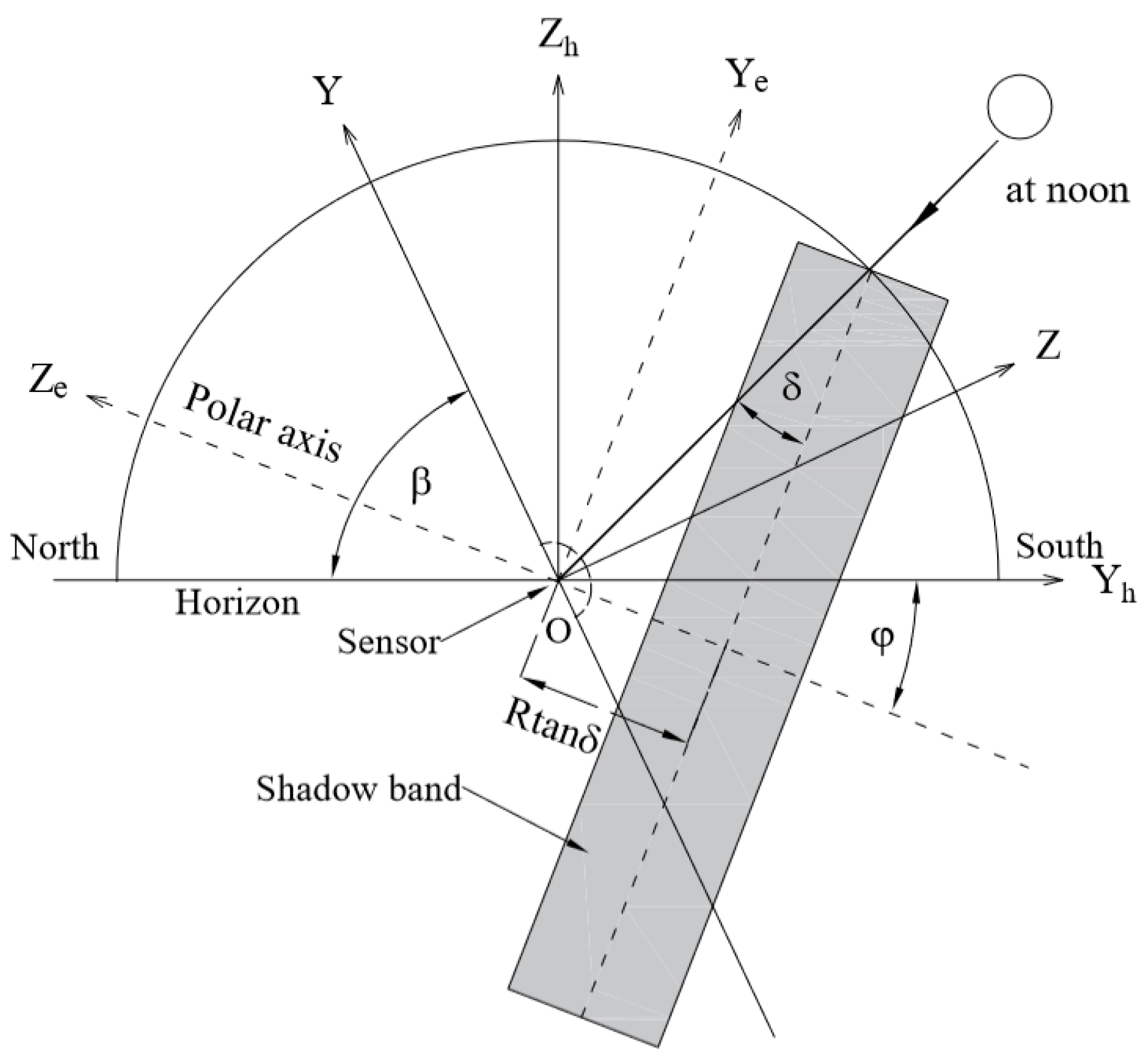
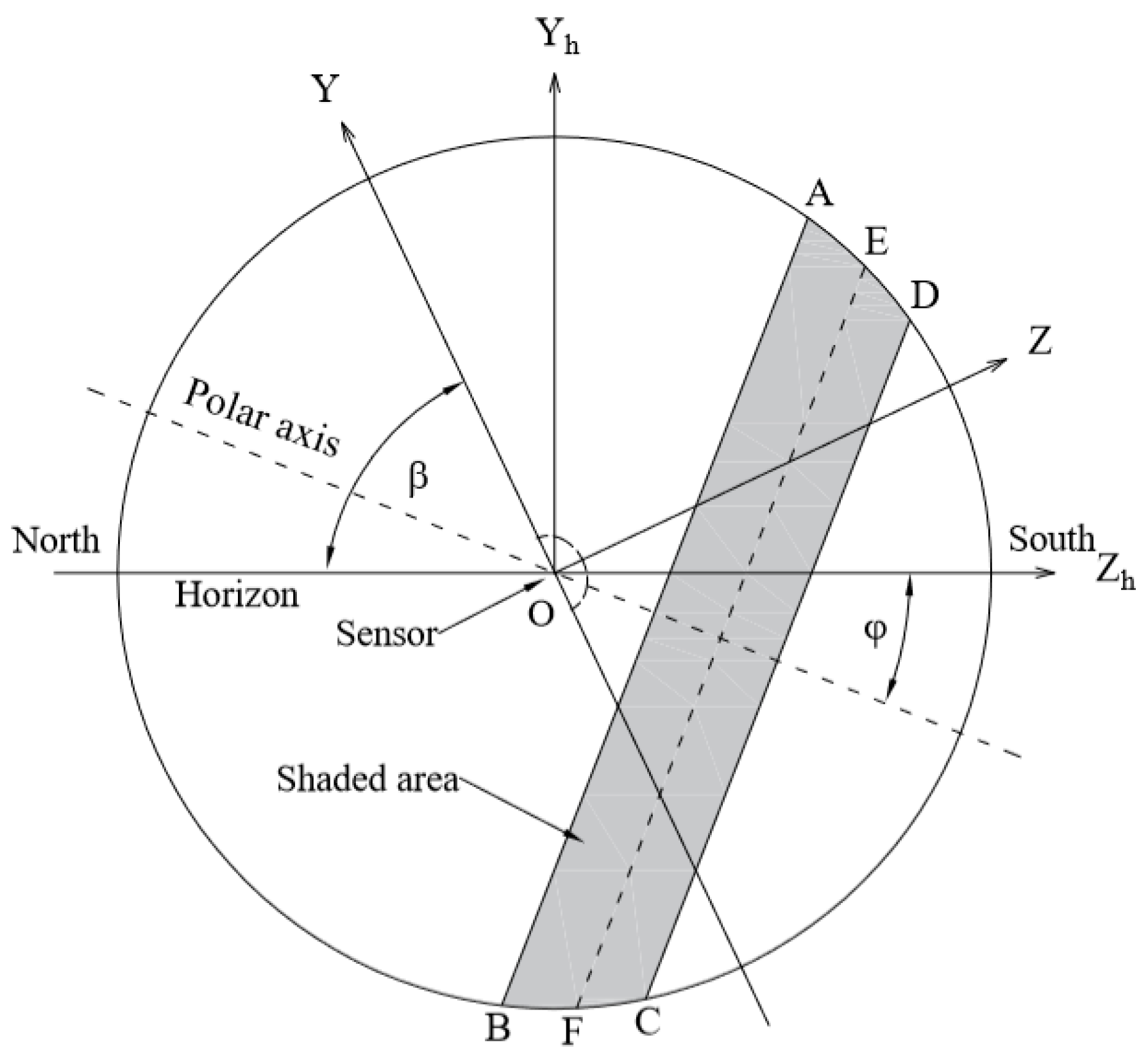
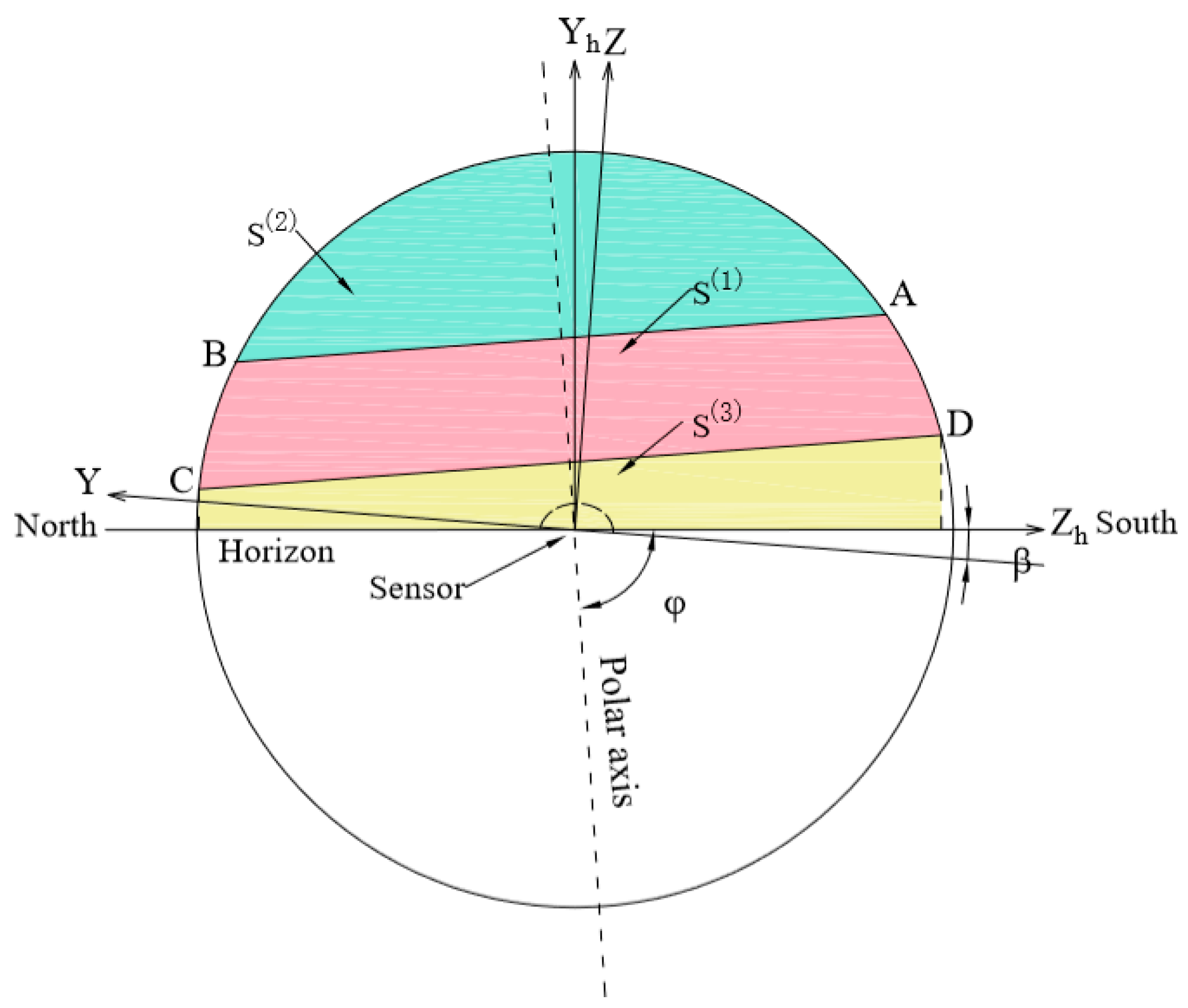
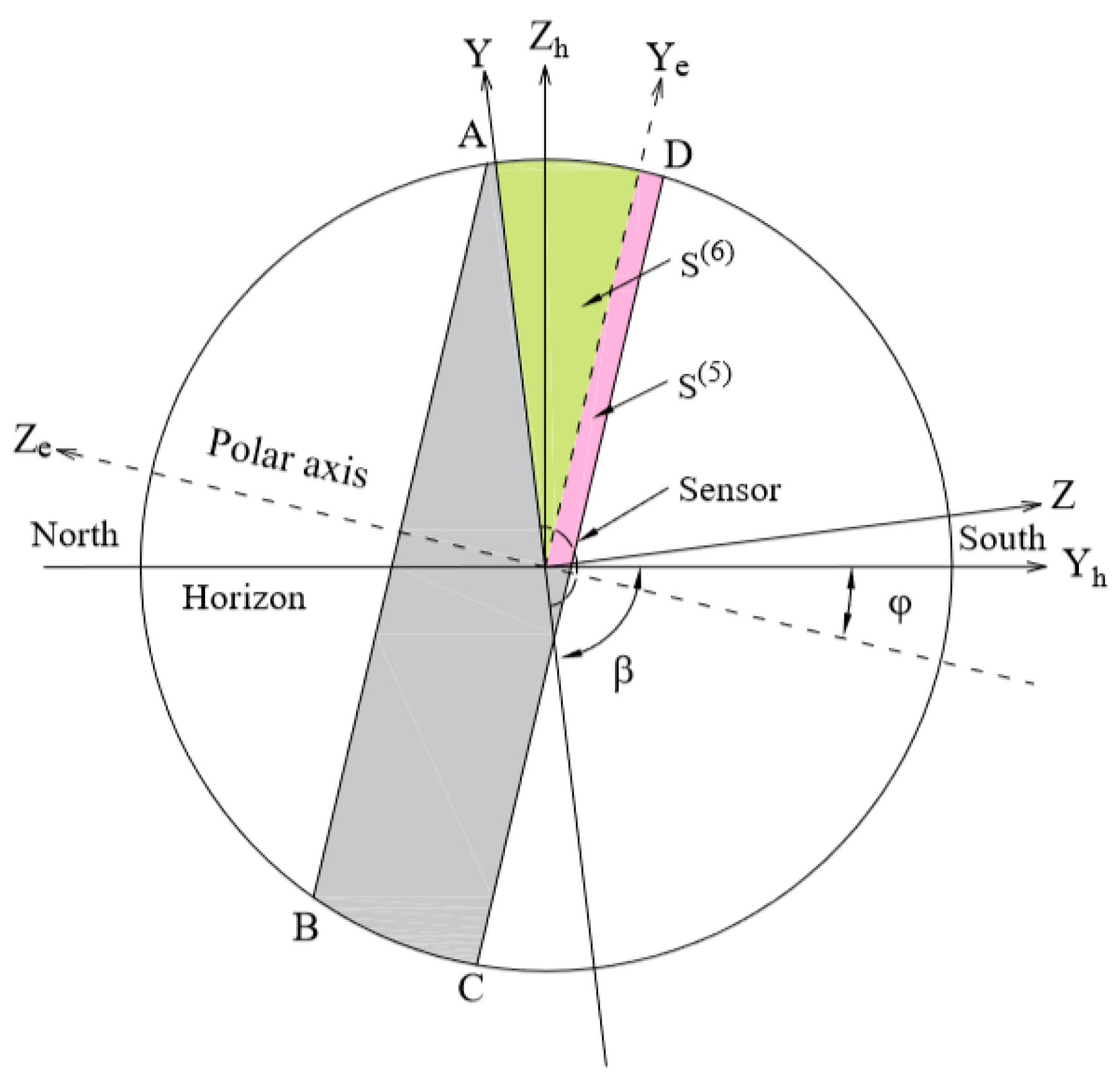
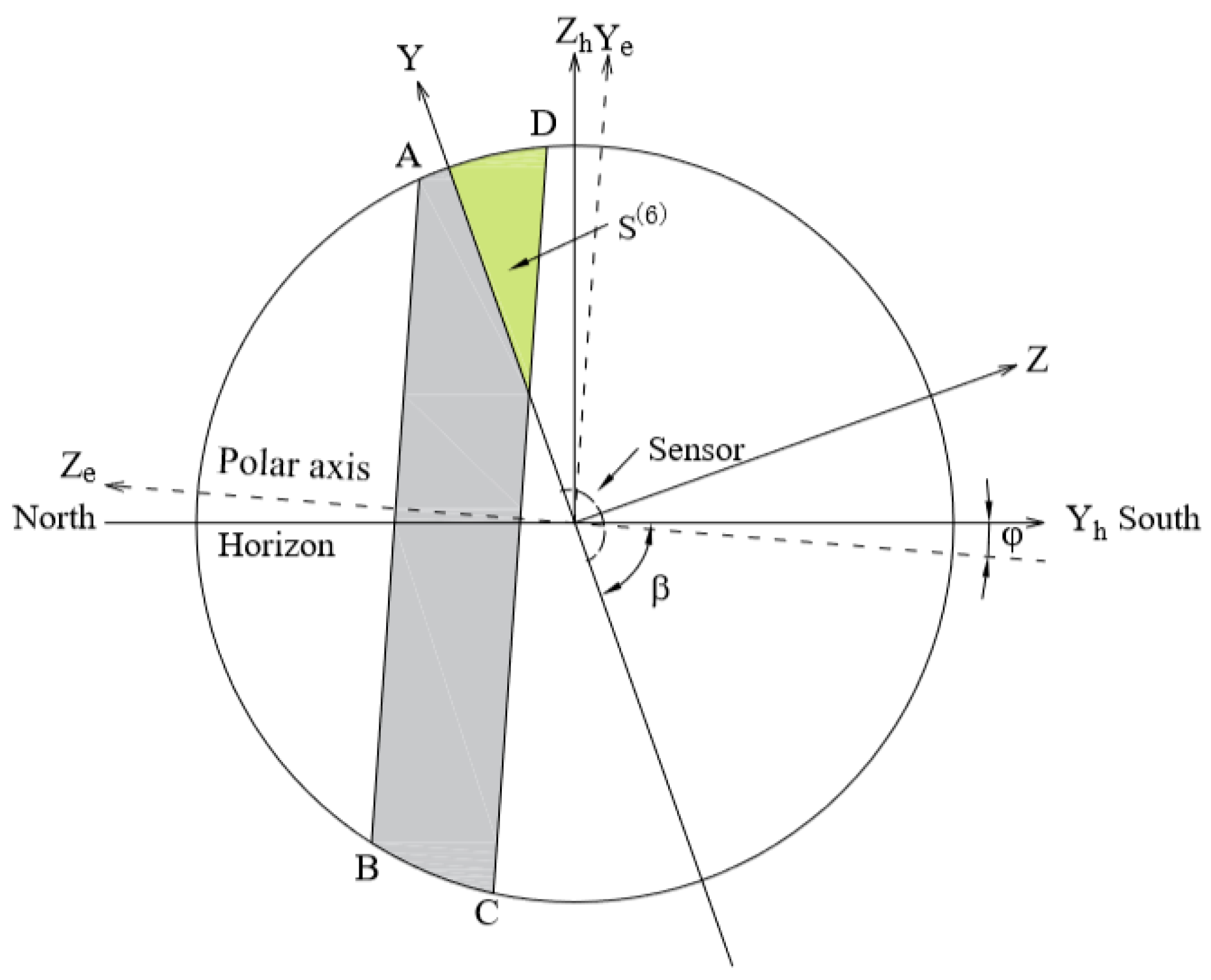
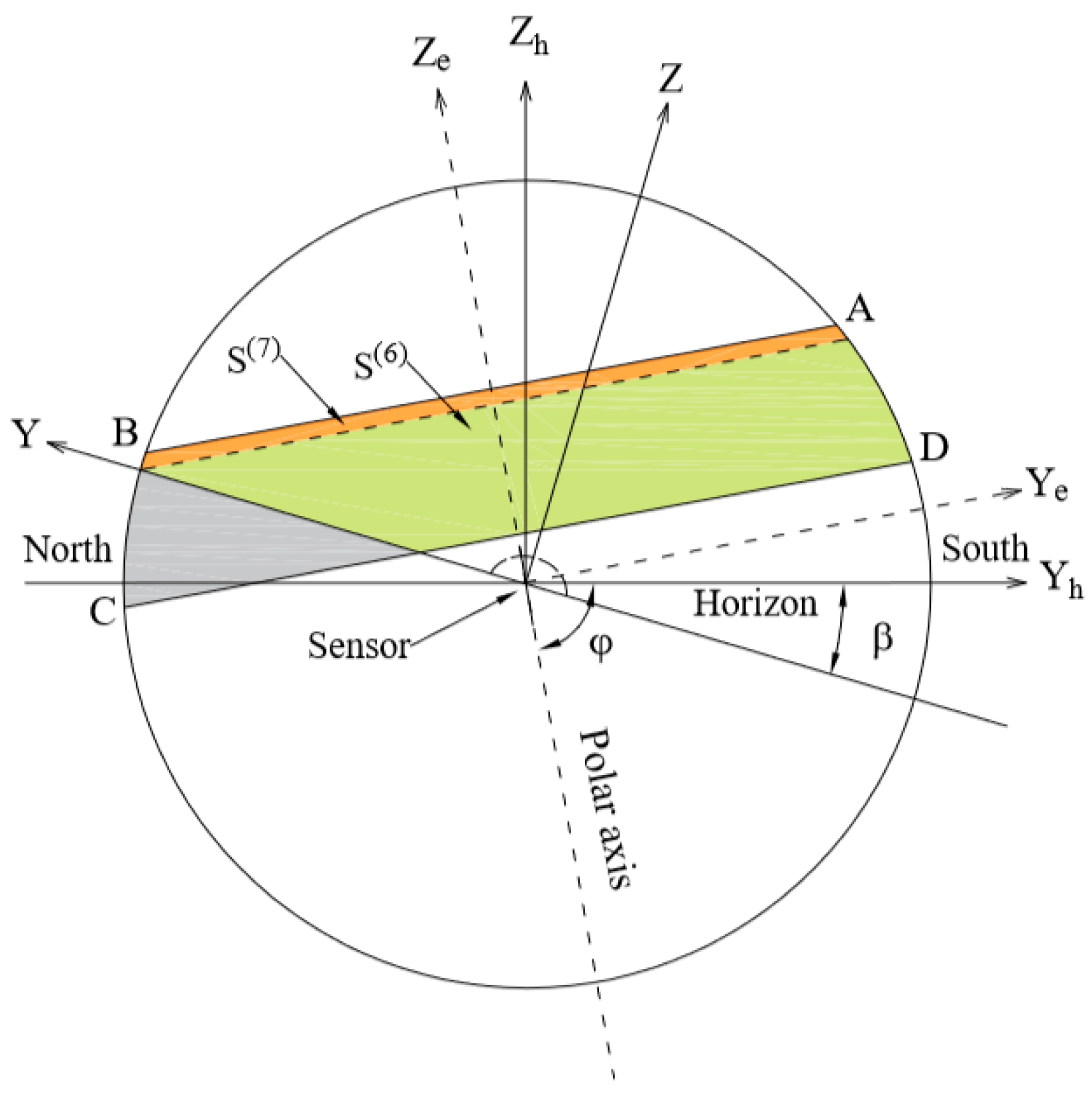
2.2. Discussions in Equatorial Coordinate System
2.2.1. Calculation of
2.2.2. Calculation of
3. Results and Discussions
3.1. Comparisons with the Results Calculated by Drummond’s Expression
3.2. Comparisons with the Experimental Data and the Results Calculated by Bureck’s Expression
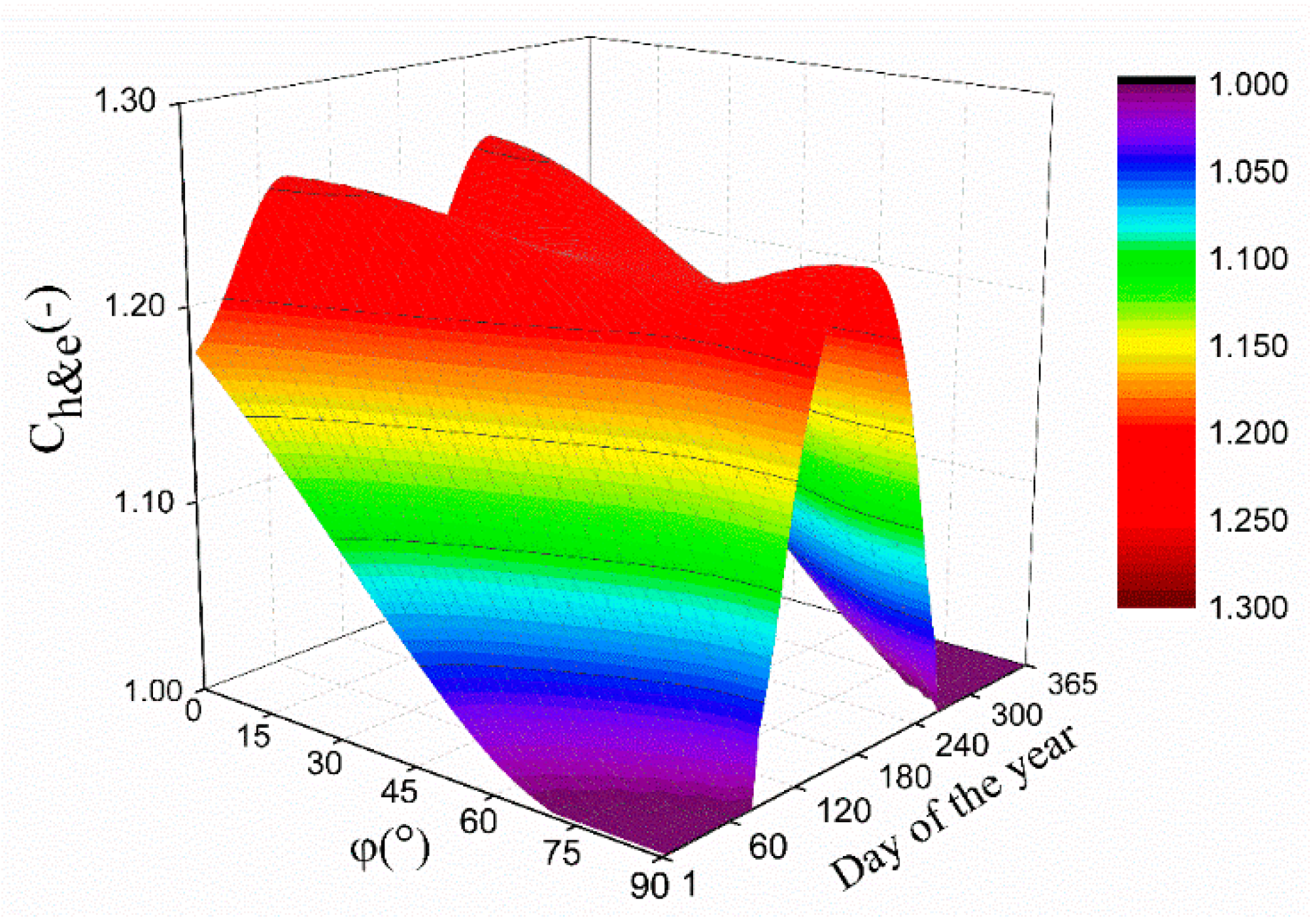
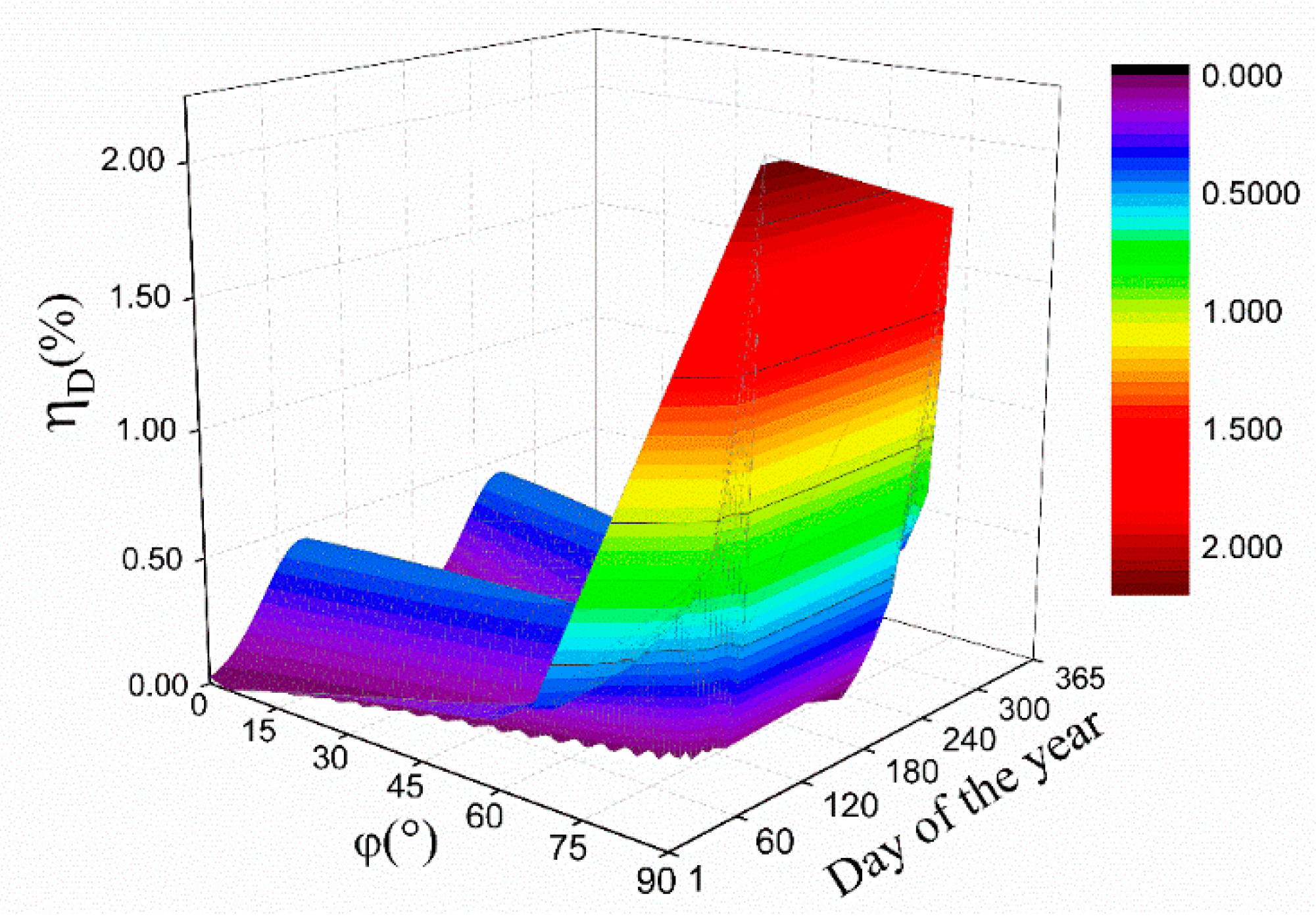
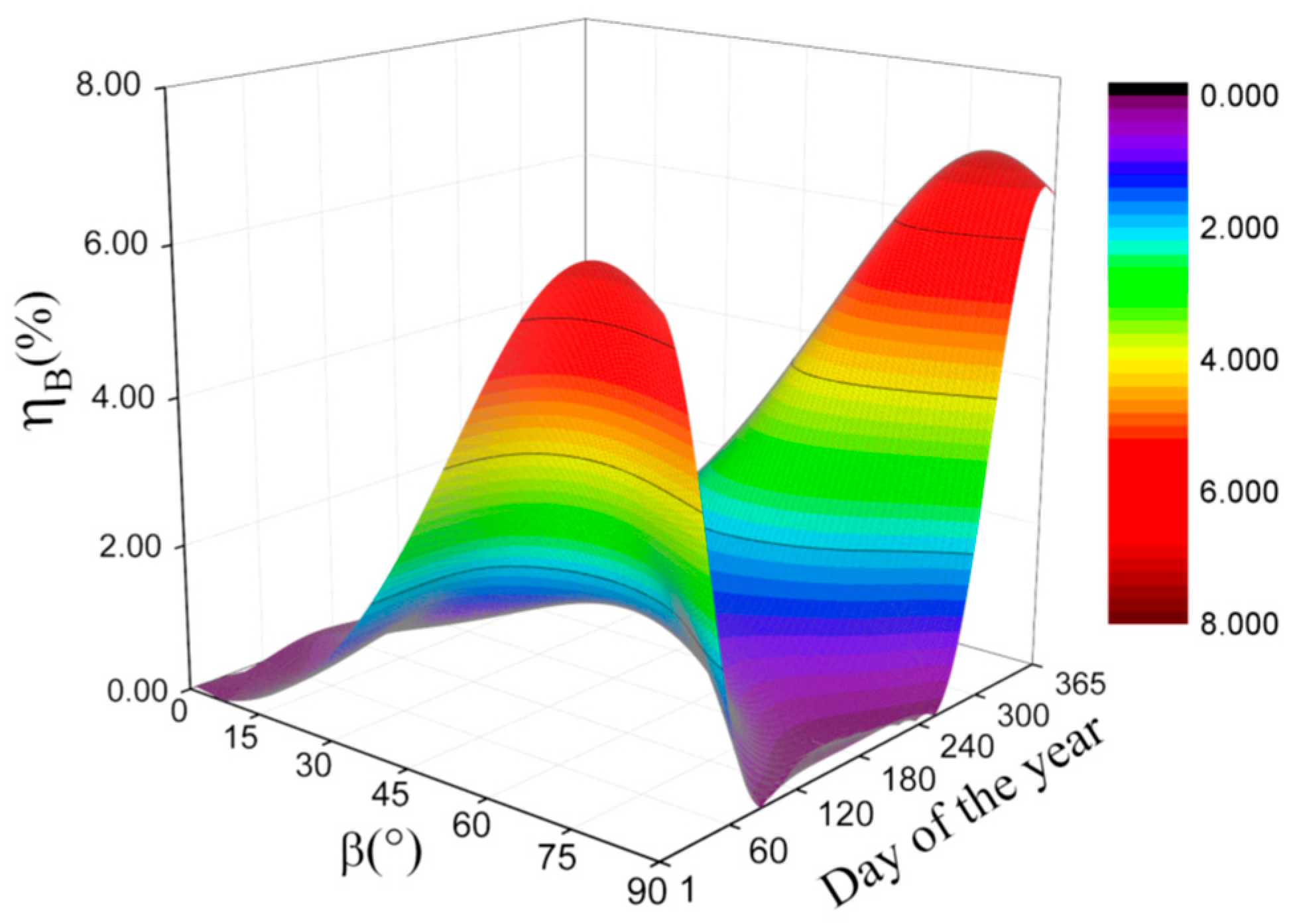
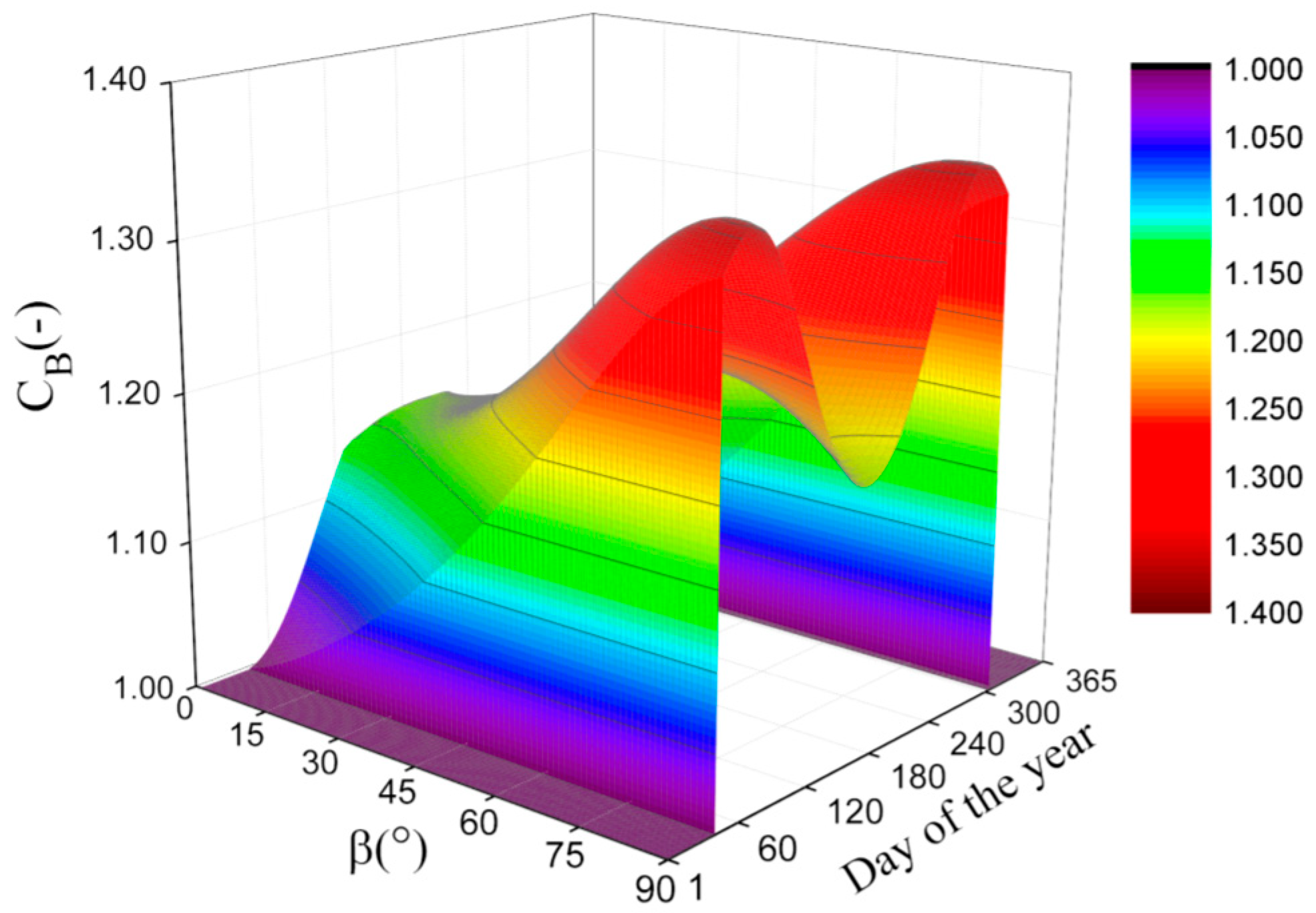
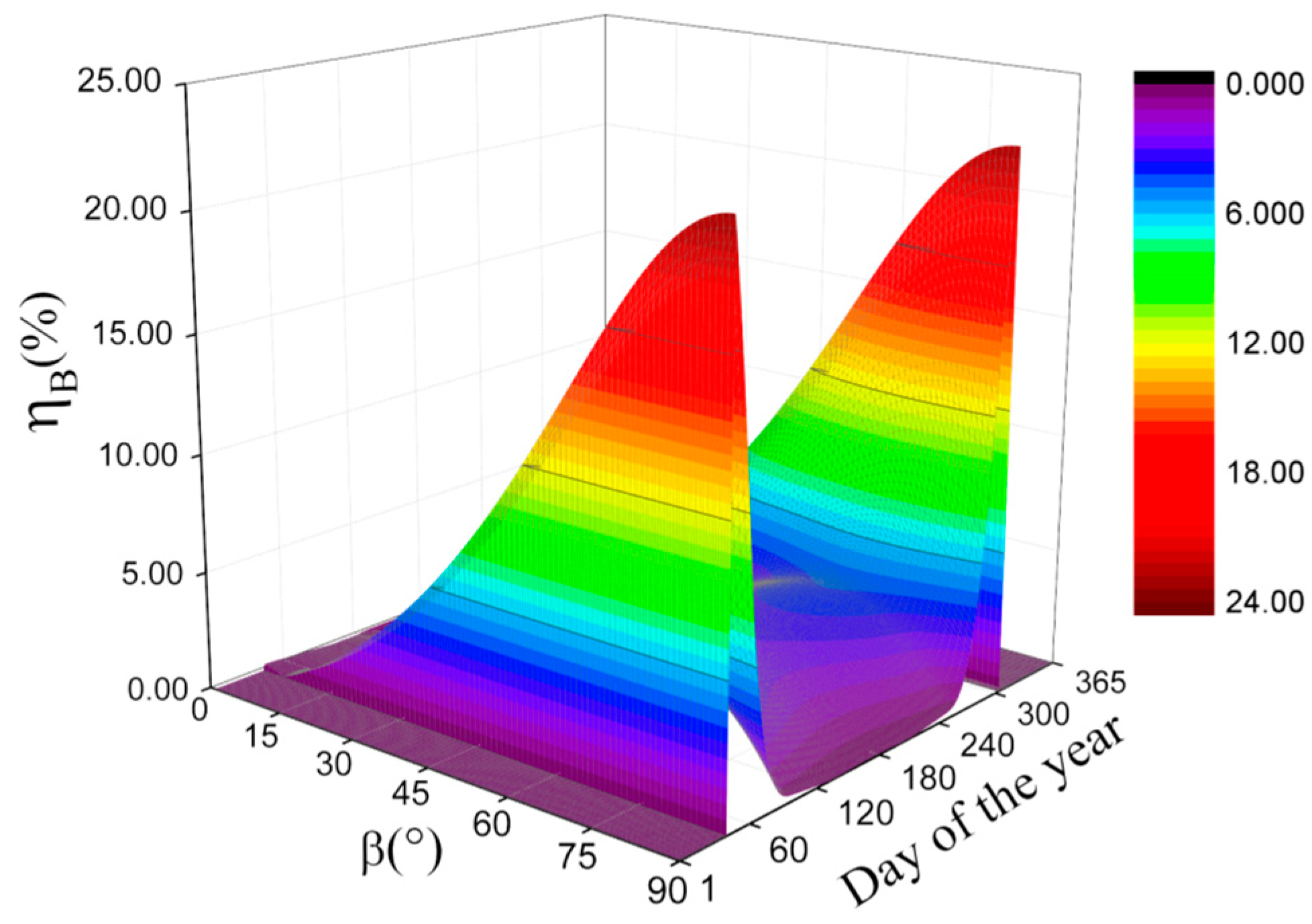
3.3. Discussions on , , and
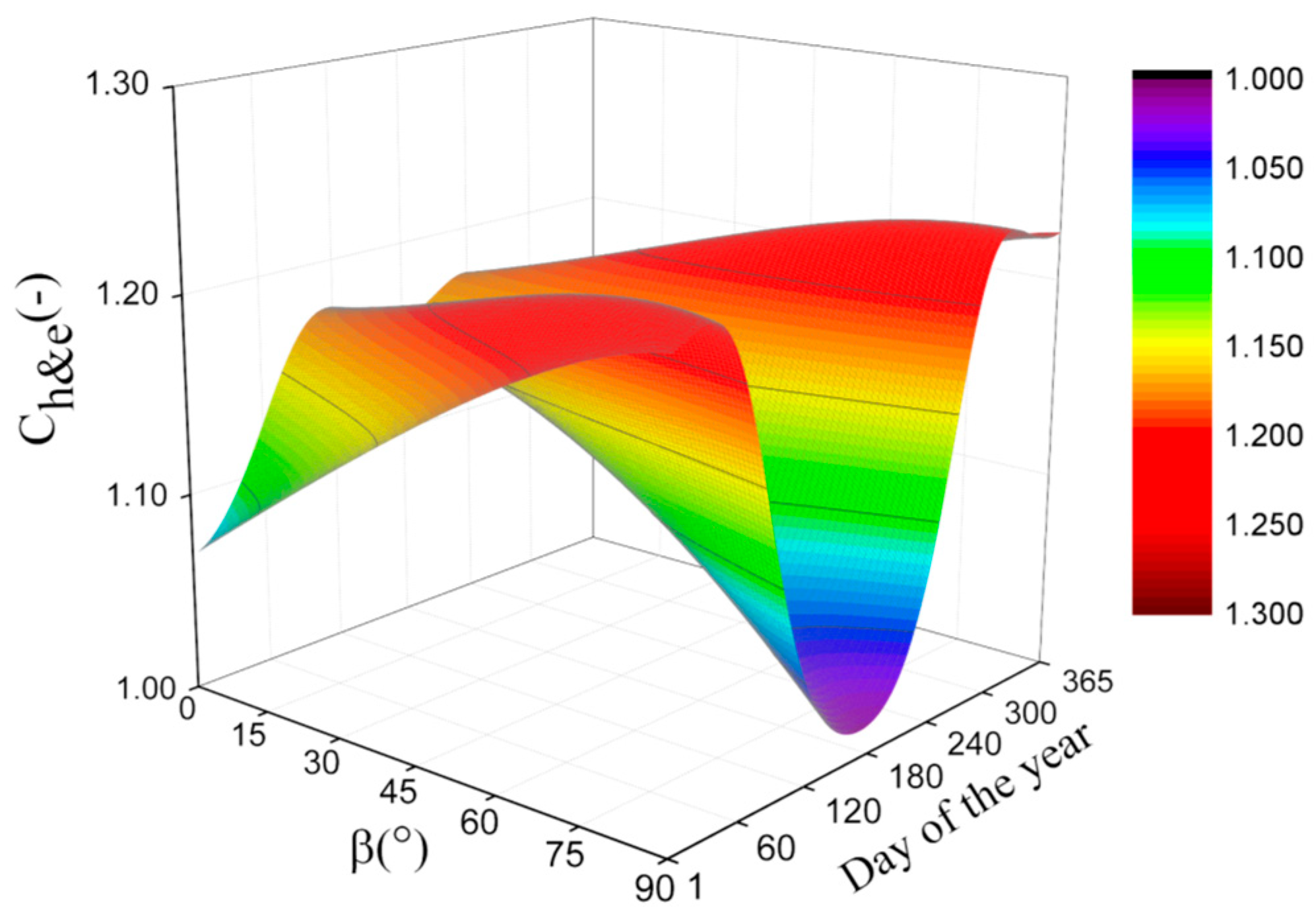
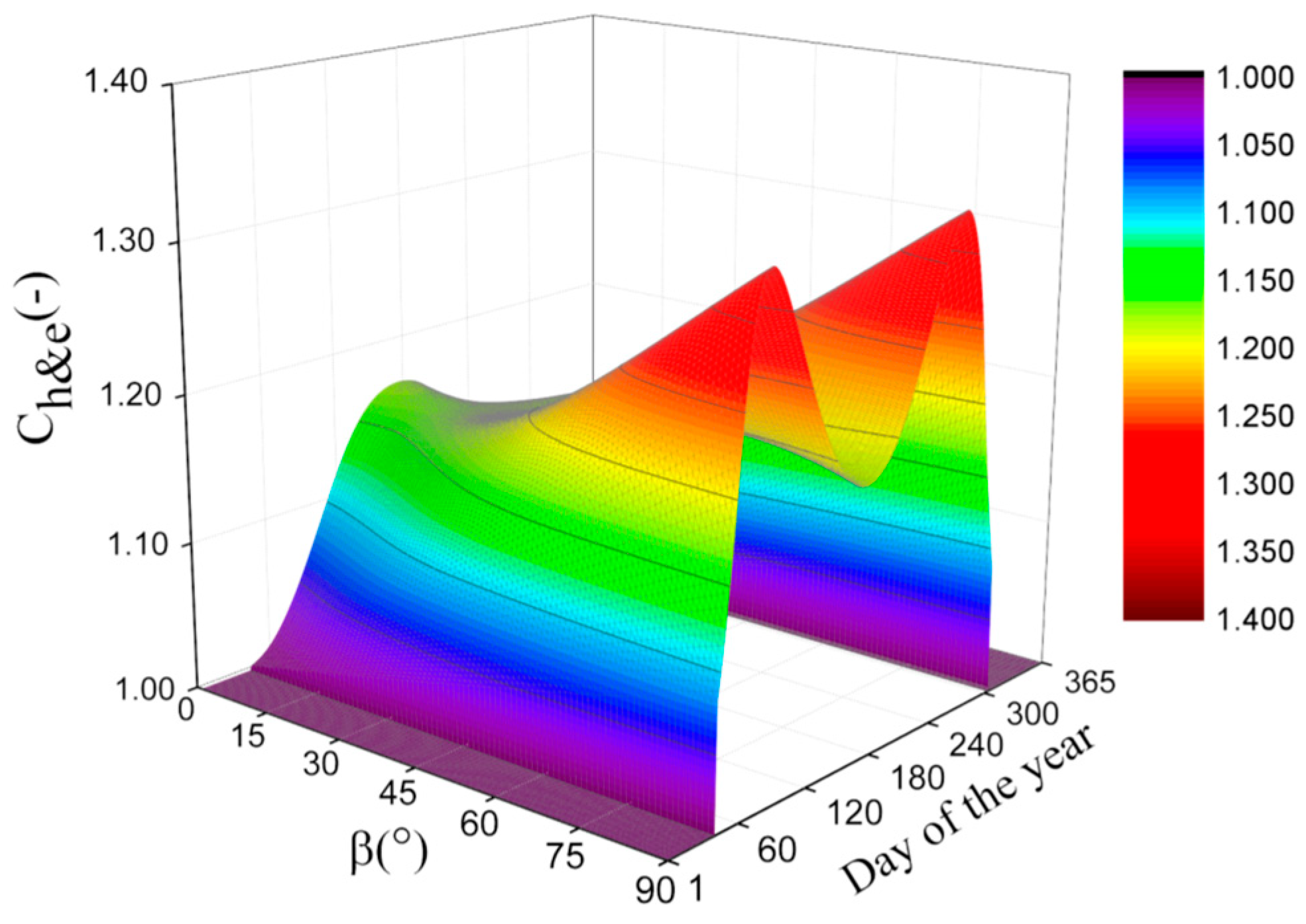
3.4. Discussions on and
4. Conclusions
- and can be calculated by the expressions in any case (), and the results calculated by the different expressions in horizon and equatorial coordinates are equal each other under the same conditions. The correction factors calculated by our expressions are in good agreement with the experimental results from Burek’s paper [7]. Additionally, our expressions are indirectly validated by the experimental results from Drummond’s paper [6] because the maximum relative deviation is less than 2.3%. It is proven that the expressions of and are correct and can give the precise correction factors under isotropic conditions.
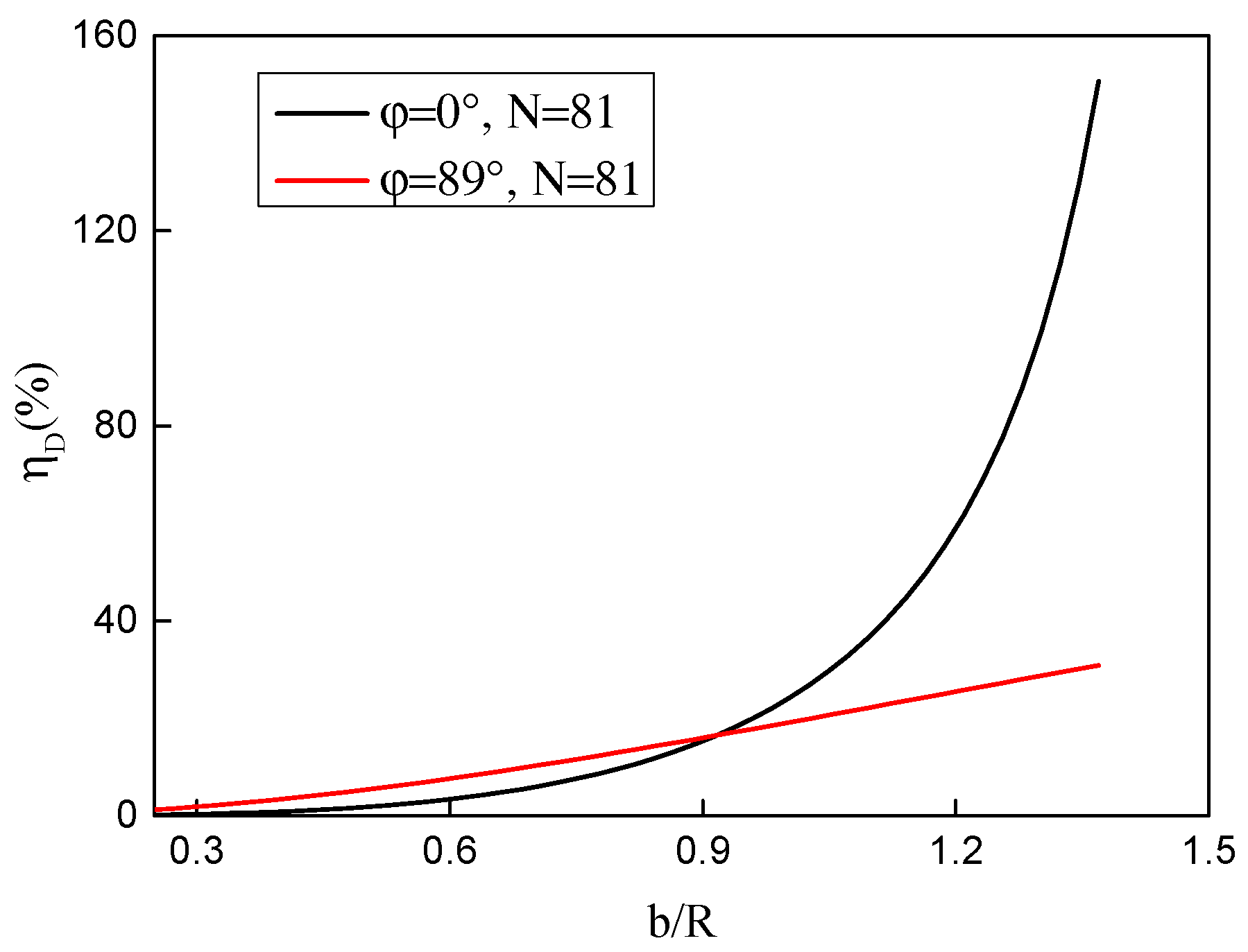
- While and the ratio of width and radius of the shadow band is small under isotropic weather conditions, it is suggested to use the expression of Drummond [6] for the correction factors if it is possible, because the calculation by Drummond’s expression is easier. However, our expressions must be used for the correction factors and when the ratio of the width and radius of the shadow band is large or while the latitude and the declination are satisfied with the condition of , because the expression of Drummond cannot be used to calculate the correction factor under those conditions.
- While under isotropic weather conditions, it is suggested to use our expressions for the correction factors. Especially, while the latitude and the declination are satisfied with the condition of , our expressions must be used for the correction factors because the expression of Burek [7] cannot be used to calculate the correction factor under those conditions. Additionally, while the latitude is close to or in the polar circle, the influences of the assumptions for Burek’s expression in some cases on the calculation results are significant. As an instance, the maximum relative deviation between the results calculated by our expressions and Burek’s expression [7] is more than 22%, while and .
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| b | Width of shadow band (m). which is 0.065 m in this paper. |
| H | Area projected from the sky sphere onto the radiation sensor, modified by ground albedo (m2) |
| r | Radius of a sphere (m) |
| N | Northern hemisphere (-) |
| R | Radius of the shadow band (m) |
| S | Area projected onto the radiation sensor from the sky sphere and shaded by a shadow band (m2) |
| x | Component of Cartesian coordinate system (-) |
| X | Axis of Cartesian coordinate system (-) |
| y | Component of Cartesian coordinate system (-) |
| Y | Axis of Cartesian coordinate system (-) |
| z | Component of Cartesian coordinate system (-) |
| Z | Axis of Cartesian coordinate system (-) |
| α | . It is the angle be between the axis X and the vector projected by the position vector on the plane XOY |
| Slope angle (o) | |
| δ | Decclination (o), positive in northern hemisphere and negative in southern hemisphere |
| Relative deviation (%) | |
| ϕ | Latitude (o), positive in northern hemisphere and negative in southern hemisphere |
| ϕ | . It is the angle between the position vector of one point and the axis Z |
| Sunrise hour angle (radian) | |
| Area integrand (-) | |
| Subscript | |
| B | Calculation by Burek’s expressions |
| D | Calculation by Drummond’s expressions |
| e | Equatorial coordinates |
| g | Projected portion of the hemisphere curved surface area due to ground albedo |
| h | Horizon coordinates |
| i | All points of the curve which intersects the spherical surface and the slope plane |
| l | All points of the left boundary curve of the spherical area shaded by the shadow band above horizontal plane |
| The point both belongs to l (all points of the left boundary curve of the spherical area shaded by the shadow band) and i (all points of the curve which intersects the spherical surface and the slope surface) | |
| max | Maximum of all components |
| min | Minimum of all components |
| r | All points of the right boundary curve of the spherical area shaded by the shadow band above horizontal plane |
| The point both belongs to r (all points of the right boundary curve of the spherical area shaded by the shadow band) and i (all points of the curve which intersects the spherical surface and the slope plane) | |
| s | Projected portion of the hemisphere curved surface area due to sky radiation |
| w | All points of the curve where the horizontal plane intersects with the spherical surface |
| Ground reflectance | |
| 0 | The coordinates before coordinate transformations |
| 1 | Minimum of the α angles of two points on the spherical surface with the same angle ϕ, but Equation (5) and Equation (40) not being included |
| 2 | Maximum of the α angles of two points on the spherical surface with the same angle ϕ, but Equation (5) and Equation (40) not being included |
References
- de Oliveira, A.P.; Machado, A.J.; Escobedo, J.F. A New Shadow-Ring Device for Measuring Diffuse Solar Radiation at the Surface. J. Atmos. Ocean. Technol. 2002, 19, 698–708. [Google Scholar] [CrossRef]
- Robinson, N.; Stoch, L. Sky radiation measurement and corrections. J. Appl. Meteorol. Climatol. 1964, 3, 179–181. [Google Scholar] [CrossRef]
- Brooks, M.J. Performance characteristics of a perforated shadow band under clear sky conditions. Sol. Energy 2010, 84, 2179–2194. [Google Scholar] [CrossRef]
- de Simón-Martín, M.; Alonso-Tristán, C.; González-Peña, D.; Díez-Mediavilla, M. New device for the simultaneous measurement of diffuse solar irradiance on several azimuth and tilting angles. Sol. Energy 2015, 119, 370–382. [Google Scholar] [CrossRef]
- Brooks, M.J.; Roberts, L.W. A data processing algorithm for the perforated shadow band incorporating a ray trace model of pyranometer exposure. In Reliability of Photovoltaic Cells, Modules, Components, and Systems III; SPIE: Bellingham, WA, USA, 2016; Volume 7773, pp. 77730W-1–77730W-9. [Google Scholar]
- Drummond, A.J. On the measurement of sky radiation. Arch. Meteor. Geophys. Bioklim 1956, 7, 413–436. [Google Scholar] [CrossRef]
- Burek, S.A.M.; Norton, B.; Probert, S.D. Analytical and experimental methods for shadow-band correction factors for solarimeters on inclined planes under isotropically diffuse and overcast skies. Sol. Energy 1988, 40, 151–160. [Google Scholar] [CrossRef]
- Rawlins, F.; Readings, C.J. The shade ring correction for measurements of diffuse irradiance under clear skies. Sol. Energy 1986, 37, 407–416. [Google Scholar] [CrossRef]
- Muneer, T.; Zhang, X. A new method for correcting shadow band diffuse irradiance data. ASME 2002, 124, 34–43. [Google Scholar] [CrossRef]
- Lebaron, B.A.; Michalsky, J.J.; Perez, R. A simple procedure for correcting shadow band data for all sky conditions. Sol. Energy 1990, 44, 249–256. [Google Scholar] [CrossRef]
- Kudish, A.I.; Ianetz, A. Analysis of diffuse radiation data for Beer Sheva: Measured (shadow ring) versus calculated (global horizontal beam) values. Sol. Energy 1993, 51, 493–503. [Google Scholar] [CrossRef]
- Batlles, F.J.; Olmo, F.J.; Alados-Arboledas, L. On shadow band correction methods for diffuse irradiance measurements. Sol. Energy 1995, 54, 105–114. [Google Scholar] [CrossRef]
- Vartiainen, E. An anisotropic shadow ring correction method for the horizontal diffuse irradiance measurements. Renew. Energy 1999, 17, 311–317. [Google Scholar] [CrossRef]
- Dal Pai, A.; Escobedo, J.F.; Dal Pai, E.; dos Santos, C.M. Estimation of hourly, daily and monthly mean diffuse radiation based on MEO shadow ring correction. Energy Procedia 2014, 57, 1150–1159. [Google Scholar] [CrossRef]
- Chaiyapinunt, S.; Ruttanasupa, P.; Ariyapoonpong, V.; Duanmeesook, K. A shadow-ring device for measuring diffuse solar radiation on a vertical surface in a tropical zone. Sol. Energy 2016, 136, 629–638. [Google Scholar] [CrossRef]
- Iqbal, M. An Introduction to Solar Radiation, 1st ed.; Academic Press, Inc.: New York, NY, USA, 1983; pp. 307–309. [Google Scholar]






| Day of the Year | |||||
|---|---|---|---|---|---|
| 0 | 81 | 1.2552 | 1.2552 | 1.2604 | 0.45 |
| 350 | 1.172 | 1.172 | 1.1722 | 0.02 | |
| 25 | 81 | 1.2262 | 1.2262 | 1.2308 | 0.38 |
| 350 | 1.1047 | 1.1047 | 1.1034 | 0.12 | |
| 50 | 81 | 1.1514 | 1.1514 | 1.1534 | 0.17 |
| 350 | 1.0341 | 1.0341 | 1.0318 | 0.22 | |
| 89 | 81 | 1.0266 | 1.0266 | 1.0036 | 2.23 |
| 350 | 1.0 | 1.0 | * | * | |
| Date of Experiment | Solarimeter (Degrees) | Measured Correction | Calculated Correction | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| All Day | 1 h | ||||||||||
| 20 August–4 September | 41 | 1.18 | 1.18 | 1.20 | 1.22 | 1.20 | 1.21 | 1.19 | 1.19 | 1.18 | 1.18 |
| 4 September–18 September | 48 | 1.22 | 1.20 | 1.22 | 1.25 | 1.21 | 1.22 | 1.19 | 1.20 | 1.19 | 1.19 |
| 21 September–30 September | 52 | 1.25 | 1.23 | 1.22 | 1.26 | 1.21 | 1.23 | 1.19 | 1.20 | 1.19 | 1.19 |
| 4 October–14 October | 58 | 1.20 | 1.20 | 1.22 | 1.27 | 1.21 | 1.24 | 1.19 | 1.20 | 1.18 | 1.19 |
| Day of the Year | (%) | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| 75 | 60 | 41 | 55.17 | 160.66 | 1076.49 | 941.93 | 1.054 | 1.2056 | 14.39 |
| 303 | 56.99 | 161.11 | 1074.94 | 940.57 | 1.056 | 1.2067 | 14.27 | ||
| 90 | 41 | 61.80 | 201.08 | 807.37 | 807.37 | 1.0829 | 1.3316 | 22.97 | |
| 303 | 63.78 | 201.13 | 806.20 | 806.20 | 1.0859 | 1.3324 | 22.70 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cheng, H.; Zhang, X.; Chen, Z.; Sun, W.; He, K.; Zheng, H.; Tang, Y. Theoretical Investigations on Shadow Band Correction Factors for Diffuse Radiation under Isotropic Conditions without Approximation. Energies 2022, 15, 7174. https://doi.org/10.3390/en15197174
Cheng H, Zhang X, Chen Z, Sun W, He K, Zheng H, Tang Y. Theoretical Investigations on Shadow Band Correction Factors for Diffuse Radiation under Isotropic Conditions without Approximation. Energies. 2022; 15(19):7174. https://doi.org/10.3390/en15197174
Chicago/Turabian StyleCheng, Haiying, Xuan Zhang, Ziqian Chen, Wenhong Sun, Kaiyan He, Hongfei Zheng, and Yanxiu Tang. 2022. "Theoretical Investigations on Shadow Band Correction Factors for Diffuse Radiation under Isotropic Conditions without Approximation" Energies 15, no. 19: 7174. https://doi.org/10.3390/en15197174
APA StyleCheng, H., Zhang, X., Chen, Z., Sun, W., He, K., Zheng, H., & Tang, Y. (2022). Theoretical Investigations on Shadow Band Correction Factors for Diffuse Radiation under Isotropic Conditions without Approximation. Energies, 15(19), 7174. https://doi.org/10.3390/en15197174








