Innovative Optimal Nonstandard Tripping Protection Scheme for Radial and Meshed Microgrid Systems
Abstract
:1. Introduction
1.1. Background
1.2. Literature Review
1.3. Contribution of The Paper
- For improving the performance of the protection system by integrating the DG units and during the occurrence of different fault currents in the MGs, the novel NSTCC is created with consideration to the constraints of the existing model.
- In the OCR scheme, an optimum coordination approach is utilizing NSTCC to reduce the total OT compared to traditional SCs and other NSCs presented in the literature. This paper achieves a significant reduction in total OT without a miscoordination record.
- The proposed new optimal scheme based on NSTCC is developed with a lower number of constraints compared to the optimal coordination in the literature because it uses just one flexible coordination scheme. Therefore, the new proposed approach in this work achieves the optimal solution with minimum computational costs.
- There is no communication required between the OCRs in the proposed optimal coordination strategy in this work, where the measurement of the current is acquired locally. Therefore, the proposed approach provides adequate robustness to the OCR coordination approach, decreasing the demand for communication infrastructure. Moreover, it reduces the computational cost as well as the requirement to gain way in large quantities of the MGs and grid data.
- The sensitivity improvement for the proposed optimal scheme is shown by comparing the results of the NSTCC and the standard curves under different testing and MG operation modes.
- Finally, a comparison analysis has been provided for the proposed optimum coordination approach under various faulty conditions for two DNs types: radial networks (IEEE 9-bus test system and IEC MG benchmark) as well as meshed networks (IEEE 9- and 30-bus test system). This comparison proves the proposed approach’s superiority over others, especially for minimum fault in islanding mode.
1.4. Outline of Paper
2. Problem Statement: OCR Coordination
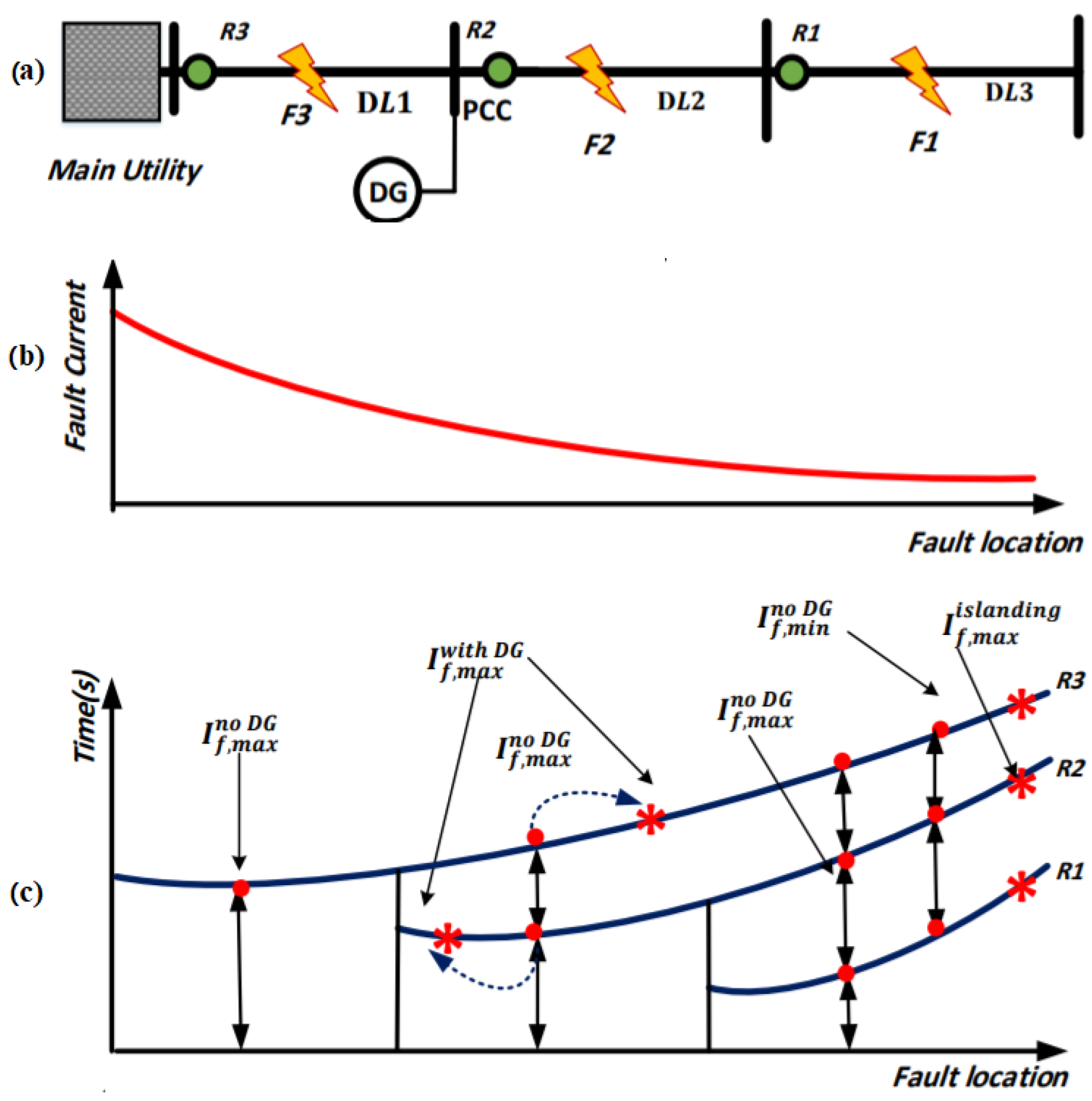
2.1. Problem Description: Illustration-Based Analysis
2.2. The Coordination of Overcurrent Relay
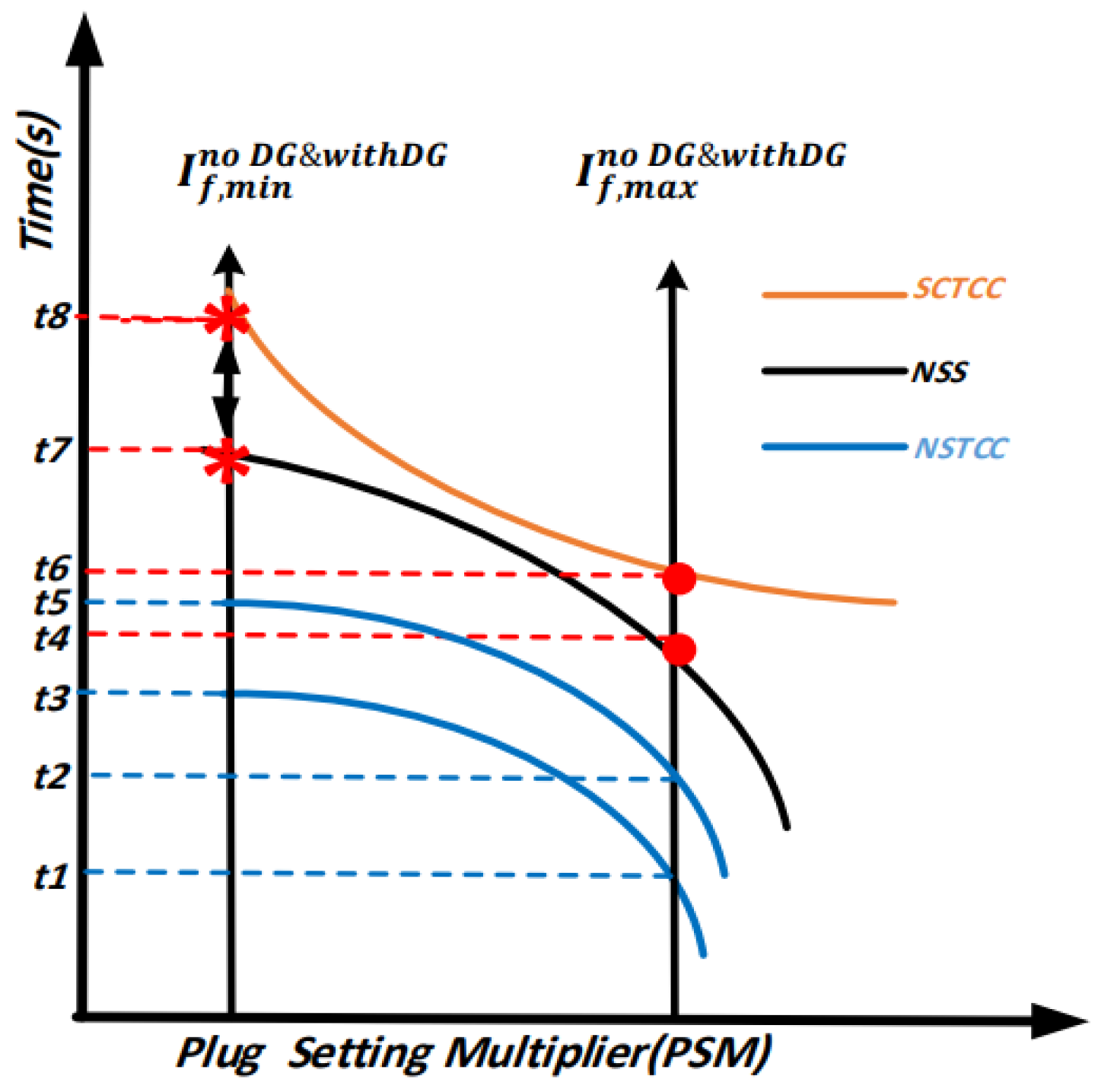
3. The Proposed Methodology: Nonstandard Time Current Characteristics
3.1. Formulating the OCR Coordination Problem
Objective Function
- Coordination Criteria and Selectivity
- Relay Setting, Operating Time Bounds:
- Proposed Setting of Coefficient Bounds:
3.2. Optimization Methods for Solving the OCR Coordination Problem
3.2.1. Genetic Algorithm Optimization (GA)
3.2.2. A Hybrid Algorithm Gravitational Search Algorithm–Sequential Quadratic Programming (GSA–SQP)
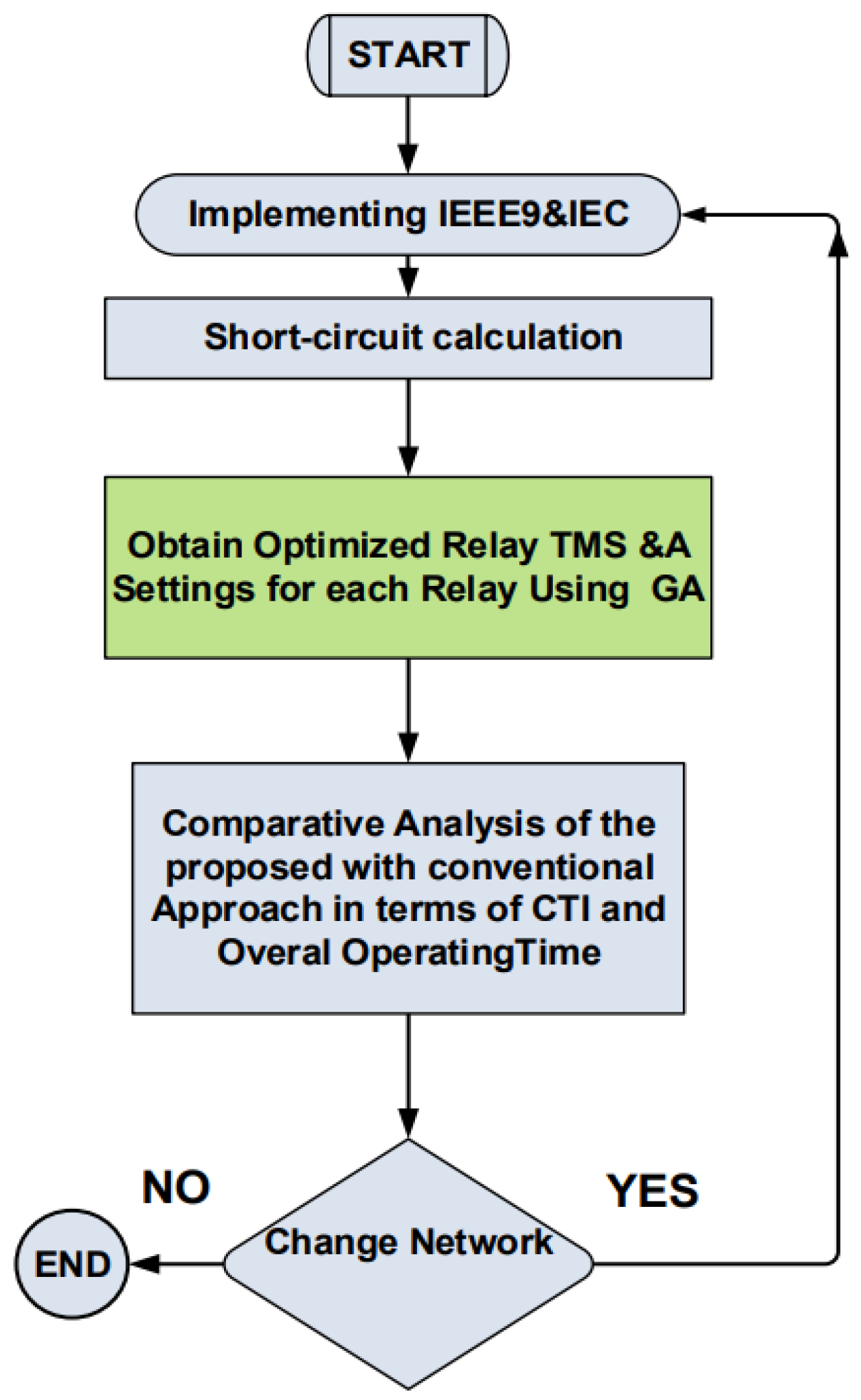
4. Simulation Results and Discussion
- Radial Networks: IEEE 9-bus test system and IEC MG benchmark.
- Meshed Networks: IEEE 9-bus and IEEE 30-bus meshed networks.
4.1. Radial Networks
4.1.1. The Radial 9-Bus Test Systems
- Radial IEEE 9-Bus System without DGs: Mode 1 test results
- Radial IEEE 9-Bus System with DGs: Mode 2 test results
- Radial IEEE 9-Bus System under the islanding condition: Mode 3 test results
- Discussion of the Radial IEEE 9-Bus System results
4.1.2. The Radial IEC MG Test System
- The radial IEC MG Simulation Results in Mode 1
- The radial IEC MG Simulation Results in Mode 2
- The radial IEC MG Simulation Results in Mode 3
- Discussion of the radial IEC MG results
4.2. Meshed Networks
- The objective function for the IEEE 9-bus system is run on the MATLAB software; it includes the short-circuit calculation values for all points that have been illustrated in Figure 13 from point A to L.
- For example, when the fault occurs at the point A: for primary R1, backup R15, and R17, the fault current was 24779 A, 9150 A, and 15632 A, respectively. The OT of primary and backup relays for faults in A have been calculated based on Equation (4). The best value of the A parameter in Equation (4) will be determined by solving the cost function in Equation (6) by using GSA–SQP in the MATLAB platform.
4.2.1. Description of the Meshed 9-Bus Test Systems under Study
4.2.2. Description of the Meshed 30-Bus Test Systems under Study
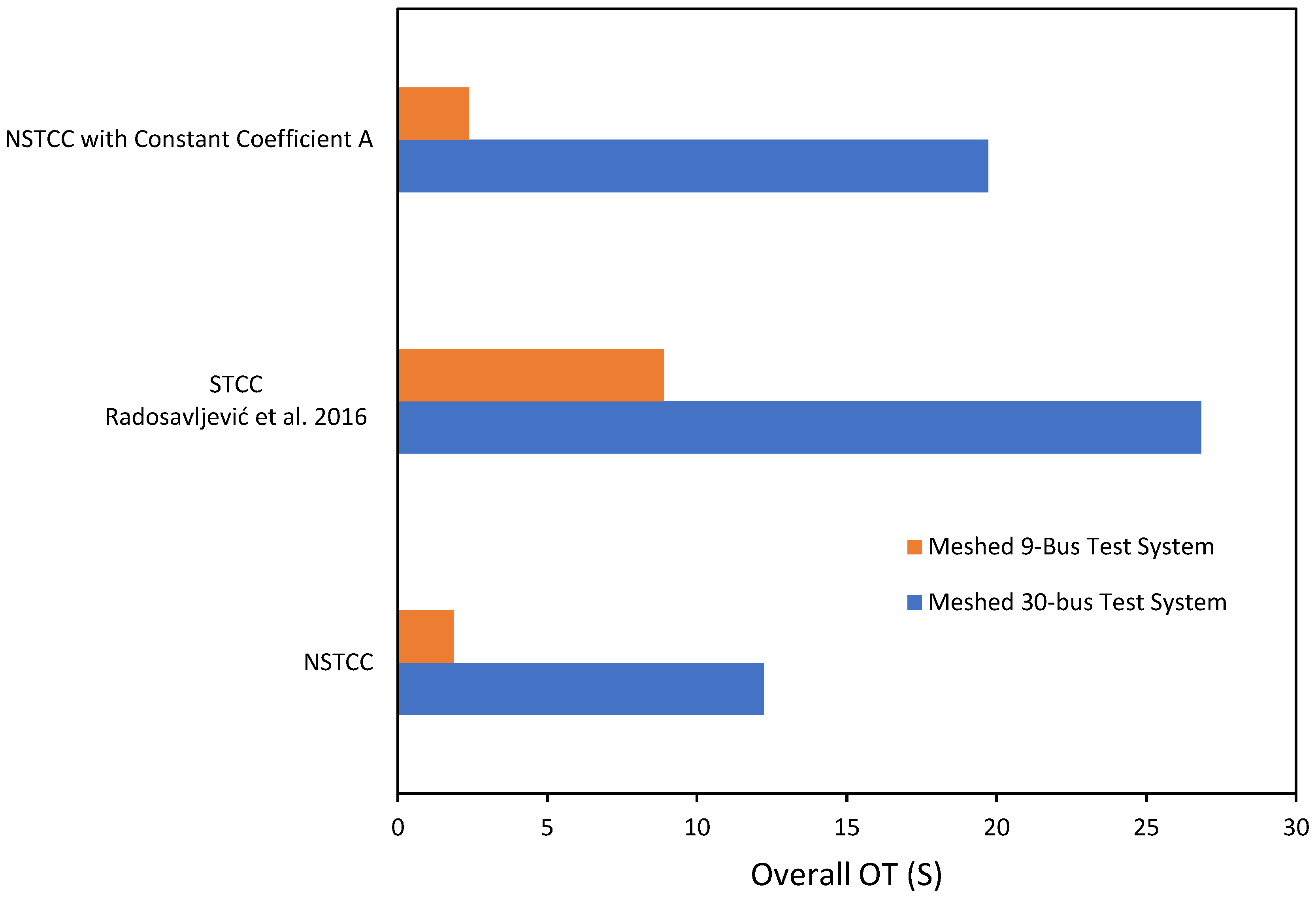
4.2.3. Discussion of the Meshed Network Results
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Alasali, F.; Haben, S.; Holderbaum, W. Energy management systems for a network of electrified cranes with energy storage. Int. J. Electr. Power Energy Syst. 2019, 106, 210–222. [Google Scholar] [CrossRef]
- Gomes, M.; Coelho, P.; Moreira, C. Microgrid protection schemes. In Microgrids Design and Implementation; Springer: Berlin/Heidelberg, Germany, 2019; pp. 311–336. [Google Scholar]
- Abeid, S.; Hu, Y. Overcurrent relays coordination optimisation methods in distribution systems for microgrids: A review. In Proceedings of the 15th International Conference on Developments in Power System Protection (DPSP 2020), Liverpool, UK, 9–12 March 2020. [Google Scholar]
- Jamali, S.; Borhani-Bahabadi, H. Non-communication protection method for meshed and radial distribution networks with synchronous-based DG. Int. J. Electr. Power Energy Syst. 2017, 93, 468–478. [Google Scholar] [CrossRef]
- So, C.; Li, K.; Lai, K.; Fung, K. Application of genetic algorithm for overcurrent relay coordination. In Proceedings of the Sixth International Conference on Developments in Power System Protection (Conf. Publ. No. 434), Nottingham, UK, 25–27 March 1997. [Google Scholar] [CrossRef]
- Zeineldin, H.; El-Saadany, E.; Salama, M. Optimal coordination of overcurrent relays using a modified particle swarm optimization. Electr. Power Syst. Res. 2006, 76, 988–995. [Google Scholar] [CrossRef]
- Bhesdadiya, R.; Trivedi, I.N.; Jangir, P.; Kumar, A. A novel hybrid approach particle swarm optimizer with moth-flame optimizer algorithm. In Advances in Computer and Computational Sciences; Springer: Berlin/Heidelberg, Germany, 2017; pp. 569–577. [Google Scholar]
- Radosavljević, J.; Jevtić, M. Hybrid GSA-SQP algorithm for optimal coordination of directional overcurrent relays. IET Gener. Transm. Distrib. 2016, 10, 1928–1937. [Google Scholar] [CrossRef]
- Purwar, E.; Vishwakarma, D.N.; Singh, S.P. A Novel Constraints Reduction-Based Optimal Relay Coordination Method Considering Variable Operational Status of Distribution System with DGs. IEEE Trans. Smart Grid 2019, 10, 889–898. [Google Scholar] [CrossRef]
- Saberi, N.A.; Sadeh, J.; Rajabi, M.H. A New Index for Constraint Reduction in Relay Coordination Problem Considering Uncertainty. J. Iran. Assoc. Electr. Electron. Eng. 2011, 8, 59–67. [Google Scholar]
- Zeineldin, H.H.; Sharaf, H.M.; Ibrahim, D.K.; El-Zahab, E.E.-D.A. Optimal Protection Coordination for Meshed Distribution Systems With DG Using Dual Setting Directional Over-Current Relays. IEEE Trans. Smart Grid 2016, 7, 1757. [Google Scholar] [CrossRef]
- Beder, H.; Mohandes, B.; El Moursi, M.S.; Badran, E.A.; El Saadawi, M.M. A New Communication-Free Dual Setting Protection Coordination of Microgrid. IEEE Trans. Power Deliv. 2021, 36, 2446–2458. [Google Scholar] [CrossRef]
- Alam, M.N.; Das, B.; Pant, V. An interior point method based protection coordination scheme for directional overcurrent relays in meshed networks. Int. J. Electr. Power Energy Syst. 2016, 81, 153–164. [Google Scholar] [CrossRef]
- Yazdaninejadi, A. Protection coordination of directional overcurrent relays: New time current characteristic and objective function. IET Gener. Transm. Distrib. 2018, 12, 190–199. [Google Scholar] [CrossRef]
- Henville, C. Combined use of definite and inverse time overcurrent elements assists in transmission line ground relay coordination. IEEE Trans. Power Deliv. 1993, 8, 925–932. [Google Scholar] [CrossRef]
- Saleh, K.A.; Zeineldin, H.H.; Al-Hinai, A.; El-Saadany, E.F. Optimal coordination of directional overcurrent relays using a new time–current–voltage characteristic. IEEE Trans. Power Deliv. 2014, 30, 537–544. [Google Scholar] [CrossRef]
- Jamali, S.; Borhani-Bahabadi, H. Recloser time–current–voltage characteristic for fuse saving in distribution networks with DG. IET Gener. Transm. Distrib. 2017, 11, 272–279. [Google Scholar] [CrossRef]
- Kılıçkıran, H.C.; Akdemir, H.; Şengör, I.; Kekezoğlu, B. A non-standard characteristic based protection scheme for distribution networks. Energies 2018, 11, 1241. [Google Scholar] [CrossRef] [Green Version]
- Keil, T.; Jager, J. Advanced coordination method for overcurrent protection relays using nonstandard tripping characteristics. IEEE Trans. Power Deliv. 2007, 23, 52–57. [Google Scholar] [CrossRef]
- Yazdaninejadi, A.; Nazarpour, D.; Golshannavaz, S. Dual-setting directional over-current relays: An optimal coordination in multiple source meshed distribution networks. Int. J. Electr. Power Energy Syst. 2017, 86, 163–176. [Google Scholar] [CrossRef]
- Ojaghi, M.; Ghahremani, R. Piece-wise Linear Characteristic for Coordinating Numerical Overcurrent Relays. IEEE Trans. Power Deliv. 2017, 32, 145–151. [Google Scholar] [CrossRef]
- Azari, M.; Mazlumi, K.; Ojaghi, M. Efficient non-standard tripping characteristic-based coordination method for overcurrent relays in meshed power networks. Electr. Eng. 2022, 1–18. [Google Scholar] [CrossRef]
- Sharaf, H.M.; Zeineldin, H.H.; Ibrahim, D.K.; EL-Zahab, E.E.D.A. A proposed coordination strategy for meshed distribution systems with DG considering user-defined characteristics of directional inverse time overcurrent relays. Int. J. Electr. Power Energy Syst. 2015, 65, 49–58. [Google Scholar] [CrossRef]
- Karegar, H.K. Relay curve selection approach for microgrid optimal protection. Int. J. Renew. Energy Res. (IJRER) 2017, 7, 636–642. [Google Scholar]
- Alasali, F.; El-Naily, N.; Zarour, E.; Saad, S.M. Highly sensitive and fast microgrid protection using optimal coordination scheme and nonstandard tripping characteristics. Int. J. Electr. Power Energy Syst. 2021, 128, 106756. [Google Scholar] [CrossRef]
- Alasali, F.; Zarour, E.; Holderbaum, W.; Nusair, K.N. Highly Fast Innovative Overcurrent Protection Scheme for Microgrid Using Metaheuristic Optimization Algorithms and Nonstandard Tripping Characteristics. IEEE Access 2022, 10, 42208–42231. [Google Scholar] [CrossRef]
- Kida, A.A.; Rivas, A.E.L.; Gallego, L.A. An improved simulated annealing–linear programming hybrid algorithm applied to the optimal coordination of directional overcurrent relays. Electr. Power Syst. Res. 2020, 181, 106197. [Google Scholar] [CrossRef]
- Darabi, A.; Bagheri, M.; Gharehpetian, G.B. Highly sensitive microgrid protection using overcurrent relays with a novel relay characteristic. IET Renew. Power Gener. 2020, 14, 1201–1209. [Google Scholar] [CrossRef]
- Saldarriaga-Zuluaga, S.D.; López-Lezama, J.M.; Muñoz-Galeano, N. Optimal coordination of overcurrent relays in microgrids considering a non-Standard characteristic. Energies 2020, 13, 922. [Google Scholar] [CrossRef] [Green Version]
- Hussain, N.; Nasir, M.; Vasquez, J.C.; Guerrero, J.M. Recent developments and challenges on AC microgrids fault detection and protection systems–a review. Energies 2020, 13, 2149. [Google Scholar] [CrossRef]
- Elmitwally, A.; Kandil, M.S.; Gouda, E.; Amer, A. Mitigation of DGs impact on variable-topology meshed network protection system by optimal fault current limiters considering overcurrent relay coordination. Electr. Power Syst. Res. 2020, 186, 106417. [Google Scholar] [CrossRef]
- Manditereza, P.T.; Bansal, R. Renewable distributed generation: The hidden challenges–A review from the protection perspective. Renew. Sustain. Energy Rev. 2016, 58, 1457–1465. [Google Scholar] [CrossRef]
- Telukunta, V.; Pradhan, J.; Agrawal, A.; Singh, M.; Srivani, S.G. Protection challenges under bulk penetration of renewable energy resources in power systems: A review. CSEE J. Power Energy Syst. 2017, 3, 365–379. [Google Scholar] [CrossRef]
- El-Naily, N.; Saad, S.M.; Mohamed, F.A. Novel approach for optimum coordination of overcurrent relays to enhance microgrid earth fault protection scheme. Sustain. Cities Soc. 2020, 54, 102006. [Google Scholar] [CrossRef]
- Din, Z.; Zhang, J.; Xu, Z.; Zhang, Y.; Zhao, J. Low voltage and high voltage ride-through technologies for doubly fed induction generator system: Comprehensive review and future trends. IET Renew. Power Gener. 2021, 15, 614–630. [Google Scholar] [CrossRef]
- El-Naily, N.; Saad, S.M.; Hussein, T.; Mohamed, F.A. A novel constraint and non-standard characteristics for optimal over-current relays coordination to enhance microgrid protection scheme. IET Gener. Transm. Distrib. 2019, 13, 780–793. [Google Scholar] [CrossRef]
- Chabanloo, R.M.; Maleki, M.G.; Agah, S.M.M.; Habashi, E.M. Comprehensive coordination of radial distribution network protection in the presence of synchronous distributed generation using fault current limiter. Int. J. Electr. Power Energy Syst. 2018, 99, 214–224. [Google Scholar] [CrossRef]
- Aghdam, T.S.; Karegar, H.K.; Zeineldin, H.H. Optimal coordination of double-inverse overcurrent relays for stable operation of DGs. IEEE Trans. Ind. Inform. 2018, 15, 183–192. [Google Scholar] [CrossRef]
- Meskin, M.; Domijan, A.; Grinberg, I. Optimal co-ordination of overcurrent relays in the interconnected power systems using break points. Electr. Power Syst. Res. 2015, 127, 53–63. [Google Scholar] [CrossRef]
- Adelnia, F.; Moravej, Z.; Farzinfar, M. A new formulation for coordination of directional overcurrent relays in interconnected networks. Int. Trans. Electr. Energy Syst. 2015, 25, 120–137. [Google Scholar] [CrossRef]
- Coffele, F.; Booth, C.; Dyśko, A. An adaptive overcurrent protection scheme for distribution networks. IEEE Trans. Power Deliv. 2014, 30, 561–568. [Google Scholar] [CrossRef] [Green Version]
- Benmouyal, G.; Meisinger, M.; Burnworth, J.; Elmore, W.A.; Freirich, K.; Kotos, P.A.; Leblanc, P.R.; Lerley, P.J.; McConnell, J.E.; Mizene, J.; et al. IEEE standard inverse-time characteristic equations for overcurrent relays. IEEE Trans. Power Deliv. 1999, 14, 868–872. [Google Scholar] [CrossRef]
- Darabi, A.; Bagheri, M.; Gharehpetian, G. Dual feasible direction-finding nonlinear programming combined with metaheuristic approaches for exact overcurrent relay coordination. Int. J. Electr. Power Energy Syst. 2020, 114, 105420. [Google Scholar] [CrossRef]
- Protection, O. 7SJ62 SIPROTEC 4 7SJ62 multifunction protection relay. Siemens 2006, 38, 44. [Google Scholar]
- Tirumala Pallerlamudi Srinivas, S.; Swarup, K.S. Optimal Protection Coordination of Nonstandard Overcurrent Relays Using Hybrid QCQP Method. Electr. Power Compon. Syst. 2020, 48, 1327–1338. [Google Scholar] [CrossRef]
- Wadood, A.; Gholami Farkoush, S.; Khurshaid, T.; Kim, C.-H.; Yu, J.; Geem, Z.W.; Rhee, S.-B. An optimized protection coordination scheme for the optimal coordination of overcurrent relays using a nature-inspired root tree algorithm. Appl. Sci. 2018, 8, 1664. [Google Scholar] [CrossRef] [Green Version]
- Alasali, F.; Haben, S.; Holderbaum, W. Stochastic optimal energy management system for RTG cranes network using genetic algorithm and ensemble forecasts. J. Energy Storage 2019, 24, 100759. [Google Scholar] [CrossRef]
- Bedekar, P.P.; Bhide, S.R. Optimum coordination of directional overcurrent relays using the hybrid GA-NLP approach. IEEE Trans. Power Deliv. 2010, 26, 109–119. [Google Scholar] [CrossRef]
- Kennedy, J.; Eberhart, R. Particle swarm optimization. In Proceedings of the ICNN’95-International Conference on Neural Networks, Perth, Australia, 27 November–1 December 1995. [Google Scholar]
- Kar, S. A comprehensive protection scheme for micro-grid using fuzzy rule base approach. Energy Syst. 2017, 8, 449–464. [Google Scholar] [CrossRef]
- Saldarriaga-Zuluaga, S.D.; López-Lezama, J.M.; Muñoz-Galeano, N. Optimal coordination of over-current relays in microgrids considering multiple characteristic curves. Alex. Eng. J. 2021, 60, 2093–2113. [Google Scholar] [CrossRef]
- Saldarriaga-Zuluaga, S.D.; López-Lezama, J.M.; Muñoz-Galeano, N. An approach for optimal coordination of over-current Relays in Microgrids with distributed generation. Electronics 2020, 9, 1740. [Google Scholar] [CrossRef]
- Christie, R. Power Systems Test Case Archive: 30 Bus Power Flow Test Case. 1993. Available online: https://labs.ece.uw.edu/pstca/pf30/pg_tca30bus.htm (accessed on 4 July 2022).





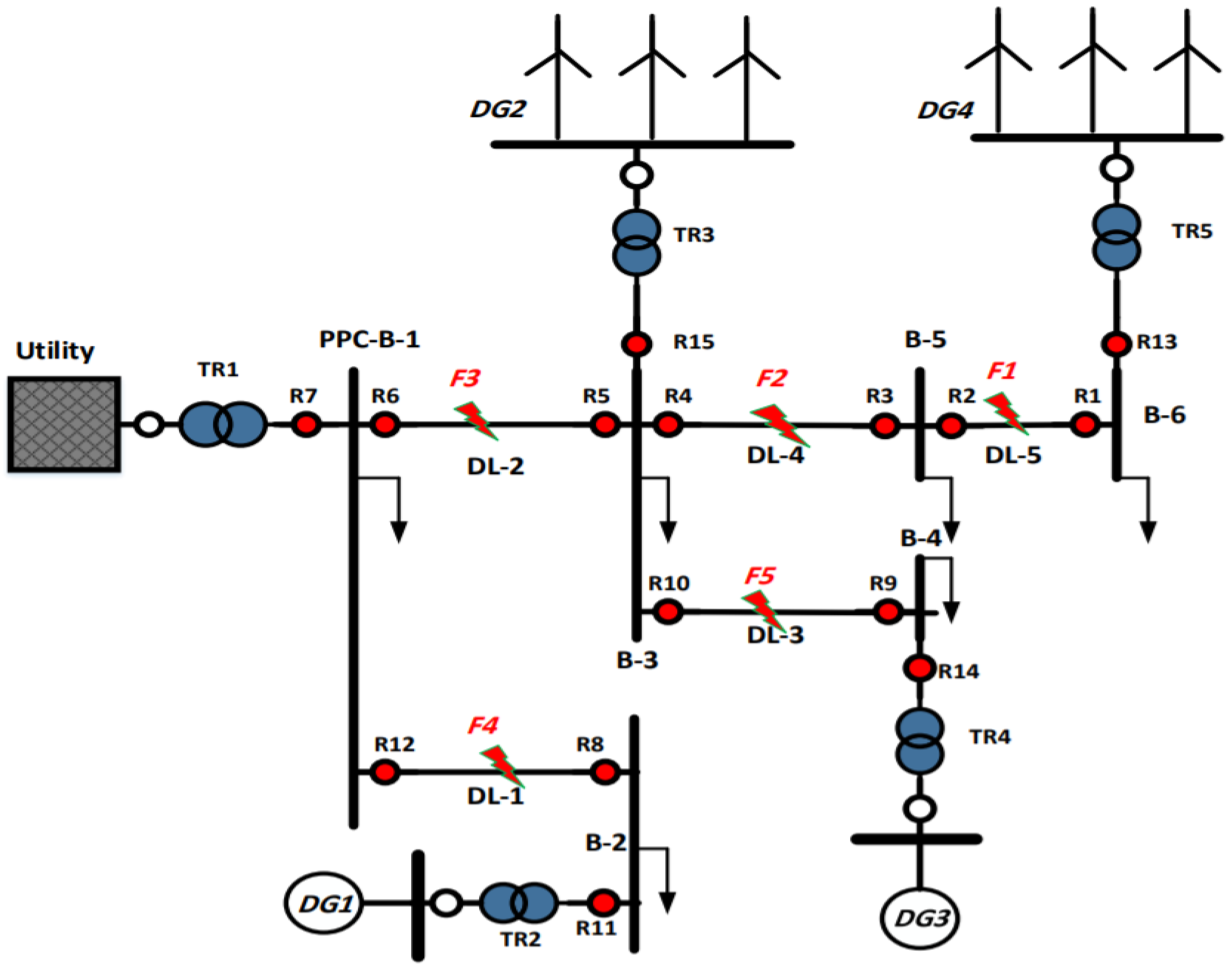



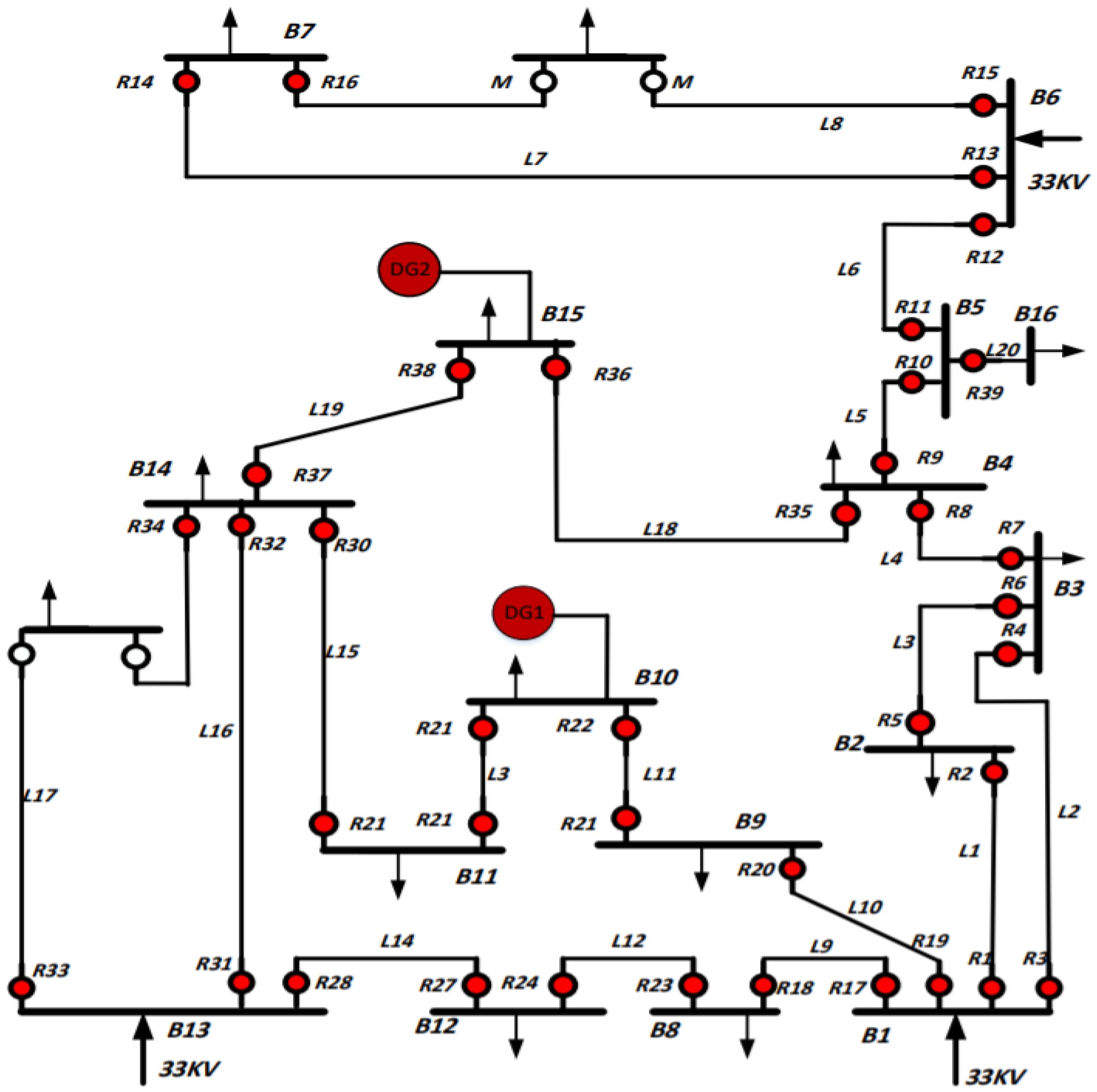
| Ref. | Year | Coefficient Types of Coordination Protection Scheme | Number of Variables | Modes of the Operation | DNs Types | Curve Used | |||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Constants | Variables | One Variable | Two Variables | Three Variables | Without DGs | Grid- Connecting | Islanding | Radial | Meshed | IEC NI Standard | Logarithmic Function | ||
| [15] | 1993 | ✓ | ✗ | ✗ | ✗ | ✗ | ✓ | ✗ | ✗ | ✗ | ✓ | ✓ | ✗ |
| [16] | 2014 | ✓ | ✗ | ✗ | ✗ | ✗ | ✓ | ✓ | ✗ | ✗ | ✓ | ✓ | ✗ |
| [17] | 2017 | ✓ | ✗ | ✗ | ✗ | ✗ | ✓ | ✓ | ✗ | ✓ | ✗ | ✓ | ✗ |
| [18] | 2018 | ✗ | ✓ | ✗ | ✗ | ✓ | ✗ | ✓ | ✗ | ✓ | ✗ | ✓ | ✗ |
| [19] | 2007 | ✓ | ✗ | ✗ | ✓ | ✗ | ✓ | ✗ | ✗ | ✓ | ✗ | ✗ | ✓ |
| [20] | 2017 | ✗ | ✓ | ✗ | ✗ | ✗ | ✗ | ✓ | ✗ | ✓ | ✓ | ✓ | ✗ |
| [21] | 2017 | ✓ | ✗ | ✗ | ✗ | ✗ | - | - | - | ✓ | ✓ | ✓ | ✗ |
| [22] | 2022 | ✗ | ✓ | ✗ | ✓ | ✗ | ✓ | ✗ | ✗ | ✗ | ✓ | ✓ | ✗ |
| [23] | 2015 | ✗ | ✓ | ✗ | ✓ | ✗ | ✓ | ✓ | ✗ | ✗ | ✓ | ✓ | ✗ |
| [24] | 2017 | ✓ | ✗ | ✗ | ✗ | ✗ | ✗ | ✓ | ✓ | ✓ | ✓ | ✓ | ✗ |
| [25] | 2021 | ✓ | ✗ | ✗ | ✗ | ✗ | ✓ | ✓ | ✓ | ✓ | ✗ | ✗ | ✓ |
| [26] | 2022 | ✓ | ✗ | ✗ | ✗ | ✗ | ✓ | ✓ | ✗ | ✓ | ✗ | ✗ | ✓ |
| Proposed approach | ✗ | ✓ | ✓ | ✗ | ✗ | ✓ | ✓ | ✓ | ✓ | ✓ | ✗ | ✓ | |
| Stages | Explanation |
|---|---|
| Stage 1: Initiate the inhabitants | Applying IEEE9 and IEC MG models and calculating the short circuits. Early possible profiles of the OCR operation time (1000 profiles). |
| Stage 2: Population assessment | For each solution, the prime assessment can be obtained via solving the optimum OF, Equation (5) under bounds from Equations (6) to (9). |
| Stage 3: Selecting | The two most excellent OCR OT profiles will be selected as a parent. |
| Stage 4: Crossing | For the following generation, a child profile will be launched, which will keep the popular genes while the rest of them will be chosen from the parent’s profile at random. The child profile must be a solution within the bounds of possibility. |
| Stage 5: Mutating | In each iteration, a single gene is mutated to update the child profile randomly. In this study, the setting of mutation is 0.1 |
| Step 6: The model output and optimal solution | Reaching the maximum number of iterations (200 iterations) by repeating the stages that have been mentioned above, starting with stage 2. |
| Relays | STCC [25] TMS | NSS [25] TMS | NSTCC | |
|---|---|---|---|---|
| TMS | A | |||
| R1 | 0.010 | 0.010 | 0.010 | 5.003 |
| R2 | 0.139 | 0.171 | 0.300 | 5.000 |
| R3 | 0.239 | 0.264 | 0.395 | 5.002 |
| R4 | 0.322 | 0.345 | 0.486 | 5.000 |
| R5 | 0.010 | 0.010 | 0.010 | 5.001 |
| R6 | 0.139 | 0.171 | 0.300 | 5.000 |
| R7 | 0.235 | 0.264 | 0.396 | 5.000 |
| R8 | 0.319 | 0.345 | 0.479 | 5.017 |
| R9 | 0.367 | 0.384 | 0.501 | 5.000 |
| R10, R11, R12 | ||||
| Overall OT(s) | 9.352 | 8.848 | 8.224 | |
| Relays | STCC [25] TMS | NSS [25] TMS | NSTCC | |
|---|---|---|---|---|
| TMS | A | |||
| R1 | 0.010 | 0.010 | 0.010 | 5.001 |
| R2 | 0.139 | 0.171 | 0.276 | 5.084 |
| R3 | 0.237 | 0.264 | 0.397 | 5.001 |
| R4 | 0.321 | 0.345 | 0.437 | 5.001 |
| R5 | 0.010 | 0.0100 | 0.010 | 5.001 |
| R6 | 0.139 | 0.166 | 0.277 | 5.022 |
| R7 | 0.062 | 0.161 | 0.177 | 5.004 |
| R8 | 0.237 | 0.258 | 0.373 | 5.017 |
| R9 | 0.010 | 0.010 | 0.010 | 5.003 |
| R10 | 0.010 | 0.010 | 0.010 | 5.002 |
| R11 | 0.118 | 0.132 | 0.191 | 5.001 |
| R12 | 0.367 | 0.384 | 0.466 | 5.001 |
| Overall OT(s) | 11.282 | 10.353 | 9.327 | |
| Relays | STCC [25] TMS | NSS [25] TMS | NSTCC | |
|---|---|---|---|---|
| TMS | A | |||
| R1 | ||||
| R2 | ||||
| R3 | ||||
| R4 | ||||
| R5 | 0.010 | 0.010 | 0.010 | 2.000 |
| R6 | 0.039 | 0.077 | 0.291 | 2.050 |
| R7 | 0.137 | 0.129 | 0.438 | 2.049 |
| R8 | ||||
| R9 | ||||
| R10 | ||||
| R11 | ||||
| R12 | ||||
| Overall, OT(s) | 1.373 | 1.34 | 1.187 | |
| [36] | [29] | [51] | [52] | NSTCC | ||
|---|---|---|---|---|---|---|
| Relay | TMS | TMS | TMS | TMS | Coefficient A | TMS Proposed |
| R1 | ||||||
| R2 | 0.128 | 0.0500 | 0.05 | 0.0500 | 4.800 | 0.0501 |
| R3 | ||||||
| R4 | 0.259 | 0.1787 | 4.58 | 0.2306 | 4.800 | 0.3977 |
| R5 | ||||||
| R6 | 0.402 | 0.3223 | 2.16 | 0.7715 | 4.886 | 0.9223 |
| R7 | 0.290 | 0.2060 | 0.05 | 0.055 | 4.826 | 0.1117 |
| R8 | ||||||
| R9 | ||||||
| R10 | 0.128 | 0.0500 | 0.05 | 0.0500 | 4.801 | 0.0500 |
| R11 | ||||||
| R12 | 0.010 | 0.0500 | 0.05 | 0.0500 | 4.800 | 0.0504 |
| R13, R14, R15 | ||||||
| OT(s) | 6.64 | 4.99 | 4.4 | 4.19 | - | 2.42 |
| [36] | [29] | [51] | [52] | NSTCC | ||
|---|---|---|---|---|---|---|
| Relay | TMS | TMS | TMS | TMS | Coefficient A | TMS |
| R1 | 0.174 | 0.137 | 0.217 | 0.3893 | 4.801 | 0.188 |
| R2 | 0.139 | 0.050 | 0.050 | 0.050 | 4.800 | 0.251 |
| R3 | 0.087 | 0.050 | 0.050 | 0.050 | 4.801 | 0.050 |
| R4 | 0.278 | 0.189 | 0.235 | 0.421 | 4.801 | 0.066 |
| R5 | 0.172 | 0.115 | 0.197 | 0.394 | 4.801 | 0.050 |
| R6 | 0.401 | 0.320 | 1.550 | 0.819 | 4.853 | 3.000 |
| R7 | 0.284 | 0.244 | 0.050 | 0.273 | 4.820 | 0.103 |
| R8 | 0.223 | 0.191 | 0.567 | 0.606 | 4.801 | 0.050 |
| R9 | 0.069 | 0.050 | 0.050 | 0.050 | 4.808 | 0.050 |
| R10 | 0.141 | 0.050 | 0.050 | 0.050 | 4.801 | 0.050 |
| R11 | 0.244 | 0.218 | 0.470 | 0.452 | 4.802 | 0.144 |
| R12 | 0.150 | 0.050 | 0.050 | 0.050 | 4.840 | 0.050 |
| R13 | 0.194 | 0.167 | 0.325 | 0.746 | 4.800 | 0.245 |
| R14 | 0.116 | 0.100 | 0.104 | 0.172 | 4.801 | 0.145 |
| R15 | 0.170 | 0.136 | 0.280 | 0.297 | 4.801 | 0.115 |
| OT(s) | 17.48 | 13.66 | 11.6 | 12.48 | - | 4.69 |
| [36] | [29] | [51] | [52] | NSTCC | ||
|---|---|---|---|---|---|---|
| Relay | TMS | TMS | TMS | TMS | Coefficient A | TMS |
| R1 | 0.173 | 0.137 | 0.548 | 0.389 | 6.000 | 0.154 |
| R2 | 0.105 | 0.050 | 0.050 | 0.050 | 6.000 | 0.050 |
| R3 | 0.086 | 0.050 | 0.050 | 0.050 | 6.000 | 0.050 |
| R4 | 0.211 | 0.157 | 0.938 | 0.529 | 6.000 | 0.365 |
| R5 | 0.209 | 0.155 | 0.862 | 0.862 | 6.000 | 3.000 |
| R6 | 0.181 | 0.151 | 0.685 | 0.243 | 6.000 | 3.000 |
| R7 | ||||||
| R8 | 0.248 | 0.218 | 0.519 | 0.499 | 6.000 | 0.376 |
| R9 | 0.069 | 0.050 | 0.050 | 0.050 | 6.000 | 0.050 |
| R10 | 0.112 | 0.050 | 0.050 | 0.050 | 6.000 | 0.050 |
| R11 | 0.265 | 0.240 | 0.490 | 0.431 | 6.000 | 0.415 |
| R12 | 0.105 | 0.050 | 0.050 | 0.050 | 6.000 | 0.050 |
| R13 | 0.193 | 0.167 | 0.778 | 0.100 | 6.000 | 0.200 |
| R14 | 0.116 | 0.099 | 0.372 | 0.104 | 6.000 | 0.117 |
| R15 | 0.177 | 0.148 | 0.670 | 0.630 | 6.000 | 1.827 |
| OT(s) | 15.56 | 12.63 | 9.99 | 8.96 | - | 4.055 |
| Backup Relay | Primary Relay | CTI (s) | Backup Relay | Primary Relay | CTI (s) |
|---|---|---|---|---|---|
| 15 | 1 | 0.200 | 11 | 13 | 0.200 |
| 17 | 1 | 0.218 | 21 | 13 | 0.200 |
| 4 | 2 | 0.200 | 16 | 14 | 0.200 |
| 1 | 3 | 0.200 | 19 | 14 | 0.200 |
| 6 | 4 | 0.200 | 13 | 15 | 0.200 |
| 3 | 5 | 0.200 | 19 | 15 | 0.201 |
| 8 | 6 | 0.200 | 2 | 16 | 0.200 |
| 23 | 6 | 0.203 | 17 | 16 | 0.200 |
| 5 | 7 | 0.200 | 2 | 18 | 0.309 |
| 23 | 7 | 0.200 | 15 | 18 | 0.317 |
| 10 | 8 | 0.200 | 13 | 20 | 0.322 |
| 7 | 9 | 0.200 | 16 | 20 | 0.322 |
| 12 | 10 | 0.200 | 11 | 22 | 0.309 |
| 9 | 11 | 0.200 | 14 | 22 | 0.320 |
| 14 | 12 | 0.203 | 5 | 24 | 0.318 |
| 21 | 12 | 0.235 | 8 | 24 | 0.315 |
| Relay | STCC [8] | NSTCC with Constant Coefficient A | NSTCC | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| TMS | Ip | TMS | A | Ip | TMS | A | Ip | ||||
| R1 | 0.276 | 2.500 | 0.629 | 0.680 | 5.800 | 0.500 | 0.201 | 0.645 | 6.250 | 0.500 | 0.029 |
| R2 | 0.091 | 2.500 | 0.332 | 0.107 | 5.800 | 0.500 | 0.157 | 0.102 | 6.250 | 0.500 | 0.155 |
| R3 | 0.197 | 2.500 | 0.524 | 0.264 | 5.800 | 0.500 | 0.146 | 0.267 | 6.250 | 0.500 | 0.161 |
| R4 | 0147 | 2.500 | 0.408 | 0.243 | 5.800 | 0.500 | 0.171 | 0.234 | 6.250 | 0.500 | 0.176 |
| R5 | 0.139 | 2.500 | 0.470 | 0.110 | 5.800 | 0.500 | 0.143 | 0.118 | 6.250 | 0.500 | 0.159 |
| R6 | 0.219 | 2.500 | 0.509 | 0.528 | 5.800 | 0.500 | 0.042 | 0.500 | 6.250 | 0.050 | 0.646 |
| R7 | 0.221 | 2.500 | 0.515 | 0.553 | 5.800 | 0.500 | 0.044 | 0.521 | 6.250 | 0.050 | 0.674 |
| R8 | 0.138 | 2.500 | 0.467 | 0.109 | 5.800 | 0.500 | 0.141 | 0.117 | 6.250 | 0.050 | 0.157 |
| R9 | 0.148 | 2.500 | 0.402 | 0.244 | 5.800 | 0.500 | 0.157 | 0.234 | 6.250 | 0.500 | 0.163 |
| R10 | 0.312 | 3.525 | 0.912 | 0.263 | 5.800 | 0.500 | 0.143 | 0.265 | 6.250 | 0.500 | 0.158 |
| R11 | 0.148 | 2.500 | 0.402 | 0.107 | 5.800 | 0.500 | 0.157 | 0.102 | 6.250 | 0.500 | 0.155 |
| R12 | 0.197 | 2.500 | 0.766 | 0.630 | 5.800 | 0.500 | 0.186 | 0.602 | 6.250 | 0.500 | 0.027 |
| R13 | 0.092 | 2.500 | 0.334 | 0.175 | 5.800 | 0.500 | 0.101 | 0.145 | 6.250 | 0.500 | 0.091 |
| R14 | 0.272 | 2.500 | 0.619 | 0.288 | 5.800 | 0.500 | 0.118 | 0.166 | 6.250 | 0.500 | 0.076 |
| R15 | 0.190 | 2.500 | 0.508 | 0.299 | 5.800 | 0.500 | 0.123 | 0.165 | 6.250 | 0.500 | 0.075 |
| R16 | 0.189 | 2.500 | 0.508 | 0.172 | 5.800 | 0.500 | 0.100 | 0.146 | 6.301 | 0.500 | 0.092 |
| R17 | 0.500 | 0.542 | 0.287 | 5.800 | 0.890 | 0.000 | 0.179 | 6.250 | 0.813 | 0 | |
| R18 | 0.072 | 1.979 | 0.232 | 0.034 | 5.800 | 1.553 | 0.103 | 0.010 | 6.364 | 0.500 | 0.015 |
| R19 | 0.315 | 1.693 | 0.302 | 5.800 | 0.537 | 0.000 | 0.130 | 6.250 | 1.035 | 0 | |
| R20 | 0.064 | 2.201 | 0.205 | 0.017 | 5.800 | 0.785 | 0.033 | 0.010 | 6.250 | 0.500 | 0.014 |
| R21 | 0.482 | 0.597 | 0.188 | 5.800 | 1.445 | 0.000 | 0.116 | 6.250 | 1.632 | 0 | |
| R22 | 0.092 | 1.902 | 0.229 | 0.049 | 5.800 | 0.668 | 0.093 | 0.010 | 6.250 | 0.500 | 0.015 |
| R23 | 0.424 | 0.682 | 0.214 | 5.800 | 0.871 | 0.000 | 0.222 | 6.354 | 0.817 | 0 | |
| R24 | 0.101 | 0.964 | 0.271 | 0.010 | 5.800 | 0.500 | 0.019 | 0.010 | 6.250 | 0.500 | 0.019 |
| OT(s) | 8.892 | 2.377 | 1.870 | ||||||||
| Backup Relay | Primary Relay | CTI (s) | Backup Relay | Primary Relay | CTI (s) | Backup Relay | Primary Relay | CTI (s) |
|---|---|---|---|---|---|---|---|---|
| 1 | 3 | 0.300 | 15 | 19 | 0.300 | 25 | 24 | 0.300 |
| 2 | 4 | 0.514 | 15 | 36 | 0.463 | 28 | 1 | 0.301 |
| 2 | 22 | 0.300 | 16 | 19 | 0.310 | 28 | 2 | 0.413 |
| 3 | 4 | 0.300 | 16 | 34 | 0.301 | 28 | 10 | 0.300 |
| 3 | 21 | 0.436 | 16 | 36 | 0.488 | 29 | 1 | 0.319 |
| 4 | 5 | 0.300 | 17 | 19 | 0.301 | 29 | 2 | 0.431 |
| 4 | 18 | 0.305 | 17 | 34 | 0.305 | 29 | 9 | 0.301 |
| 5 | 6 | 0.300 | 17 | 35 | 0.349 | 30 | 29 | 0.300 |
| 6 | 7 | 0.331 | 18 | 38 | 0.300 | 31 | 28 | 0.300 |
| 6 | 8 | 0.300 | 19 | 37 | 0.301 | 32 | 30 | 0.300 |
| 7 | 27 | 0.302 | 20 | 2 | 0.443 | 33 | 31 | 0.300 |
| 8 | 26 | 0.300 | 20 | 9 | 0.300 | 34 | 32 | 0.300 |
| 9 | 12 | 0.300 | 20 | 10 | 0.317 | 35 | 17 | 0.606 |
| 10 | 11 | 0.300 | 21 | 1 | 0.327 | 35 | 33 | 0.317 |
| 11 | 13 | 0.300 | 21 | 9 | 0.301 | 36 | 16 | 0.507 |
| 12 | 14 | 0.300 | 21 | 10 | 0.317 | 36 | 33 | 0.302 |
| 13 | 15 | 0.300 | 22 | 20 | 0.300 | 37 | 5 | 0.453 |
| 14 | 16 | 0.301 | 23 | 21 | 0.647 | 37 | 23 | 0.300 |
| 14 | 17 | 0.378 | 23 | 22 | 0.300 | 38 | 34 | 0.302 |
| 15 | 19 | 0.300 | 24 | 18 | 0.458 | 38 | 35 | 0.342 |
| 15 | 35 | 0.332 | 24 | 23 | 0.300 | 38 | 36 | 0.474 |
| Relay | STCC [8] | NSTCC with Constant Coefficient A | NSTCC | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| TMS | Ip | TMS | A | Ip | TMS | A | Ip | ||||
| R1 | 0.304 | 3.816 | 0.901 | 0.647 | 5.800 | 1.595 | 0.458 | 0.550 | 4.301 | 2.294 | 0.274 |
| R2 | 0.346 | 1.500 | 0.713 | 0.3223 | 5.800 | 2.207 | 0.352 | 0.331 | 4.185 | 2.594 | 0.164 |
| R3 | 0.276 | 2.756 | 0.946 | 0.395 | 5.800 | 1.895 | 0.707 | 0.323 | 4.199 | 2.501 | 0.441 |
| R4 | 0.258 | 2.693 | 0.766 | 0.424 | 5.800 | 1.669 | 0.525 | 0.477 | 4.089 | 1.892 | 0.237 |
| R5 | 0.263 | 1.659 | 0.661 | 0.317 | 5.800 | 1.690 | 0.436 | 0.2932 | 4.212 | 2.438 | 0.317 |
| R6 | 0.160 | 1.981 | 0.607 | 0.1801 | 5.800 | 1.831 | 0.443 | 0.1756 | 4.123 | 2.028 | 0.301 |
| R7 | 0.173 | 1.824 | 0.430 | 0.203 | 5.800 | 1.529 | 0.215 | 0.2582 | 4.024 | 1.620 | 0.041 |
| R8 | 0.157 | 1.587 | 0.372 | 0.190 | 5.800 | 1.500 | 0.199 | 0.227 | 4.121 | 1.689 | 0.074 |
| R9 | 0.313 | 3.353 | 0.901 | 0.622 | 5.800 | 1.656 | 0.523 | 0.535 | 4.131 | 2.537 | 0.293 |
| R10 | 0.312 | 3.525 | 0.912 | 0.713 | 5.800 | 1.500 | 0.487 | 0.746 | 4.188 | 2.174 | 0.278 |
| R11 | 0.280 | 2.784 | 1.053 | 0.910 | 5.800 | 2.214 | 0.910 | 0.410 | 4.388 | 2.538 | 0.736 |
| R12 | 0.217 | 3.594 | 0.765 | 0.440 | 5.800 | 1.500 | 0.525 | 0.392 | 4.099 | 1.946 | 0.252 |
| R13 | 0.249 | 2.618 | 0.872 | 0.431 | 5.800 | 1.525 | 0.712 | 0.3734 | 4.141 | 2.523 | 0.538 |
| R14 | 0.216 | 2.140 | 0.772 | 0.285 | 5.800 | 1.500 | 0.550 | 0.188 | 4.181 | 1.706 | 0.243 |
| R15 | 0.233 | 1.606 | 0.757 | 0.298 | 5.800 | 1.500 | 0.612 | 0.283 | 4.116 | 1.836 | 0.408 |
| R16 | 0.268 | 3.071 | 0.793 | 0.507 | 5.800 | 1.500 | 0.463 | 0.5937 | 4.089 | 1.660 | 0.083 |
| R17 | 0.147 | 1.629 | 0.318 | 0.246 | 5.800 | 1.620 | 0.187 | 0.207 | 4.058 | 1.924 | 0.010 |
| R18 | 0.241 | 2.493 | 0.735 | 0.439 | 5.800 | 1.500 | 0.562 | 0.416 | 4.119 | 1.946 | 0.312 |
| R19 | 0.241 | 2.839 | 0.728 | 0.447 | 5.800 | 1.627 | 0.535 | 0.468 | 4.116 | 2.142 | 0.320 |
| R20 | 0.144 | 2.398 | 0.499 | 0.254 | 5.800 | 1.500 | 0.430 | 0.217 | 4.143 | 1.943 | 0.256 |
| R21 | 0.1604 | 1.500 | 0.377 | 0.201 | 5.800 | 1.855 | 0.277 | 0.179 | 4.194 | 1.874 | 0.106 |
| R22 | 0.1702 | 4.008 | 0.694 | 0.358 | 5.800 | 1.945 | 0.624 | 0.363 | 4.144 | 2.518 | 0.449 |
| R23 | 0.210 | 2.568 | 0.731 | 0.401 | 5.800 | 1.500 | 0.646 | 0.405 | 4.092 | 2.096 | 0.468 |
| R24 | 0.202 | 2.236 | 0.772 | 0.302 | 5.800 | 2.158 | 0.764 | 0.346 | 4.084 | 2.168 | 0.596 |
| R25 | 0.252 | 2.663 | 0.365 | 5.800 | 2.885 | 0.000 | 0.329 | 4.599 | 3.105 | 0.000 | |
| R26 | 0.111 | 1.510 | 0.625 | 0.100 | 5.800 | 1.500 | 0.334 | 0.116 | 4.089 | 1.623 | 0.293 |
| R27 | 0.122 | 1.636 | 0.557 | 0.100 | 5.800 | 1.500 | 0.285 | 0.107 | 4.021 | 1.597 | 0.210 |
| R28 | 0.193 | 2.549 | 0.963 | 0.259 | 5.800 | 1.808 | 0.690 | 0.235 | 4.174 | 2.157 | 0.572 |
| R29 | 0.164 | 2.673 | 0.731 | 0.2801 | 5.800 | 1.500 | 0.597 | 0.269 | 4.064 | 1.742 | 0.375 |
| R30 | 0.184 | 3.672 | 0.901 | 0.378 | 5.800 | 1.797 | 0.808 | 0.366 | 4.101 | 2.371 | 0.589 |
| R31 | 0.219 | 3.069 | 0.903 | 0.401 | 5.800 | 1.559 | 0.734 | 0.390 | 4.119 | 2.159 | 0.541 |
| R32 | 0.209 | 3.217 | 0.953 | 0.473 | 5.800 | 1.500 | 0.910 | 0.487 | 4.159 | 1.906 | 0.685 |
| R33 | 0.286 | 3.177 | 0.843 | 0.582 | 5.800 | 1.500 | 0.582 | 0.604 | 4.092 | 2.209 | 0.285 |
| R34 | 0.252 | 3.661 | 0.834 | 0.629 | 5.800 | 1.500 | 0.631 | 0.712 | 4.098 | 1.962 | 0.329 |
| R35 | 0.229 | 2.453 | 0.729 | 0.301 | 5.800 | 1.718 | 0.301 | 0.312 | 4.072 | 2.089 | 0.302 |
| R36 | 0.100 | 1.500 | 0.222 | 0.140 | 5.800 | 4.168 | 0.315 | 0.123 | 4.636 | 2.725 | 0.161 |
| R37 | 0.214 | 2.355 | 0.703 | 0.385 | 5.800 | 1.500 | 0.606 | 0.408 | 4.084 | 1.930 | 0.408 |
| R38 | 0.194 | 2.888 | 0.790 | 0.352 | 5.800 | 1.644 | 0.690 | 0.217 | 4.108 | 2.046 | 0.418 |
| OT(s) | 26.826 | 19.727 | 12.231 | ||||||||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abeid, S.; Hu, Y.; Alasali, F.; El-Naily, N. Innovative Optimal Nonstandard Tripping Protection Scheme for Radial and Meshed Microgrid Systems. Energies 2022, 15, 4980. https://doi.org/10.3390/en15144980
Abeid S, Hu Y, Alasali F, El-Naily N. Innovative Optimal Nonstandard Tripping Protection Scheme for Radial and Meshed Microgrid Systems. Energies. 2022; 15(14):4980. https://doi.org/10.3390/en15144980
Chicago/Turabian StyleAbeid, Salima, Yanting Hu, Feras Alasali, and Naser El-Naily. 2022. "Innovative Optimal Nonstandard Tripping Protection Scheme for Radial and Meshed Microgrid Systems" Energies 15, no. 14: 4980. https://doi.org/10.3390/en15144980
APA StyleAbeid, S., Hu, Y., Alasali, F., & El-Naily, N. (2022). Innovative Optimal Nonstandard Tripping Protection Scheme for Radial and Meshed Microgrid Systems. Energies, 15(14), 4980. https://doi.org/10.3390/en15144980







