Stratified Flow of Micropolar Nanofluid over Riga Plate: Numerical Analysis
Abstract
:1. Introduction
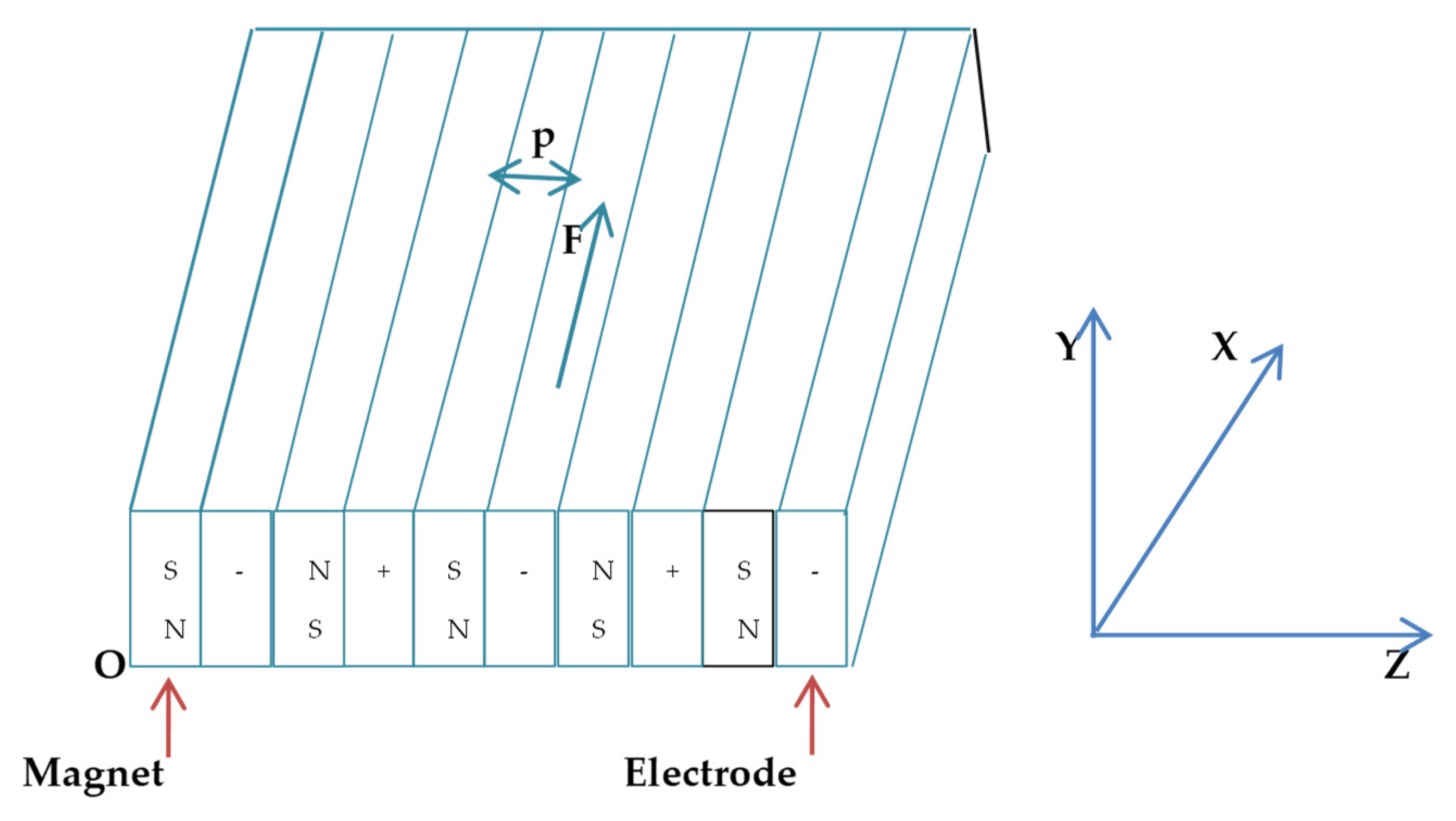
2. Materials and Methods
3. Results
4. Conclusions
- The thermal stratification factor decreases the energy exchange rate while the concentration stratification factor increases the mass exchange rate.
- Energy and mass transport rates are directly related to the local and modified Grashof numbers.
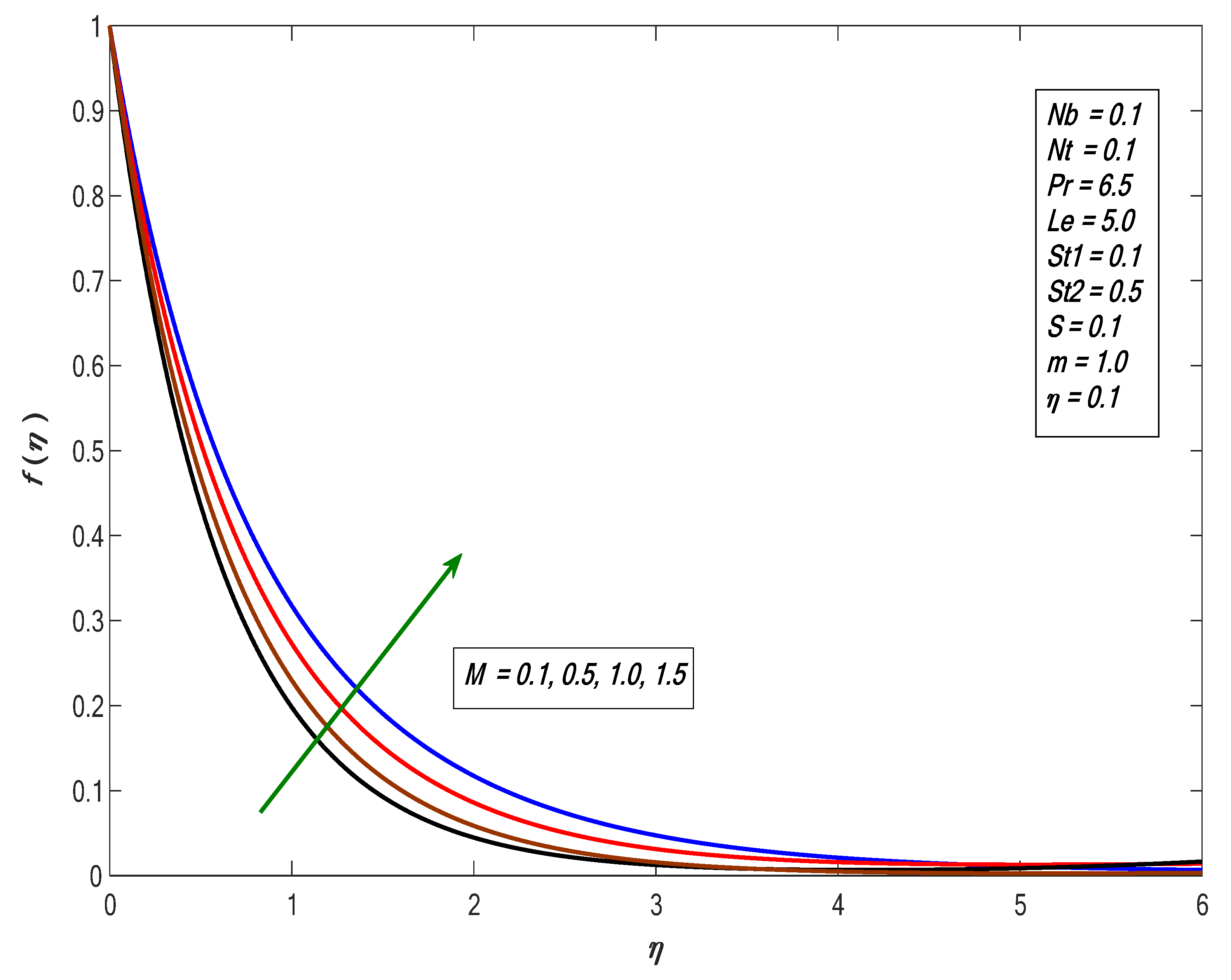
- The dimensionless factor () shows and inverse relationship with the energy and mass exchange rate.
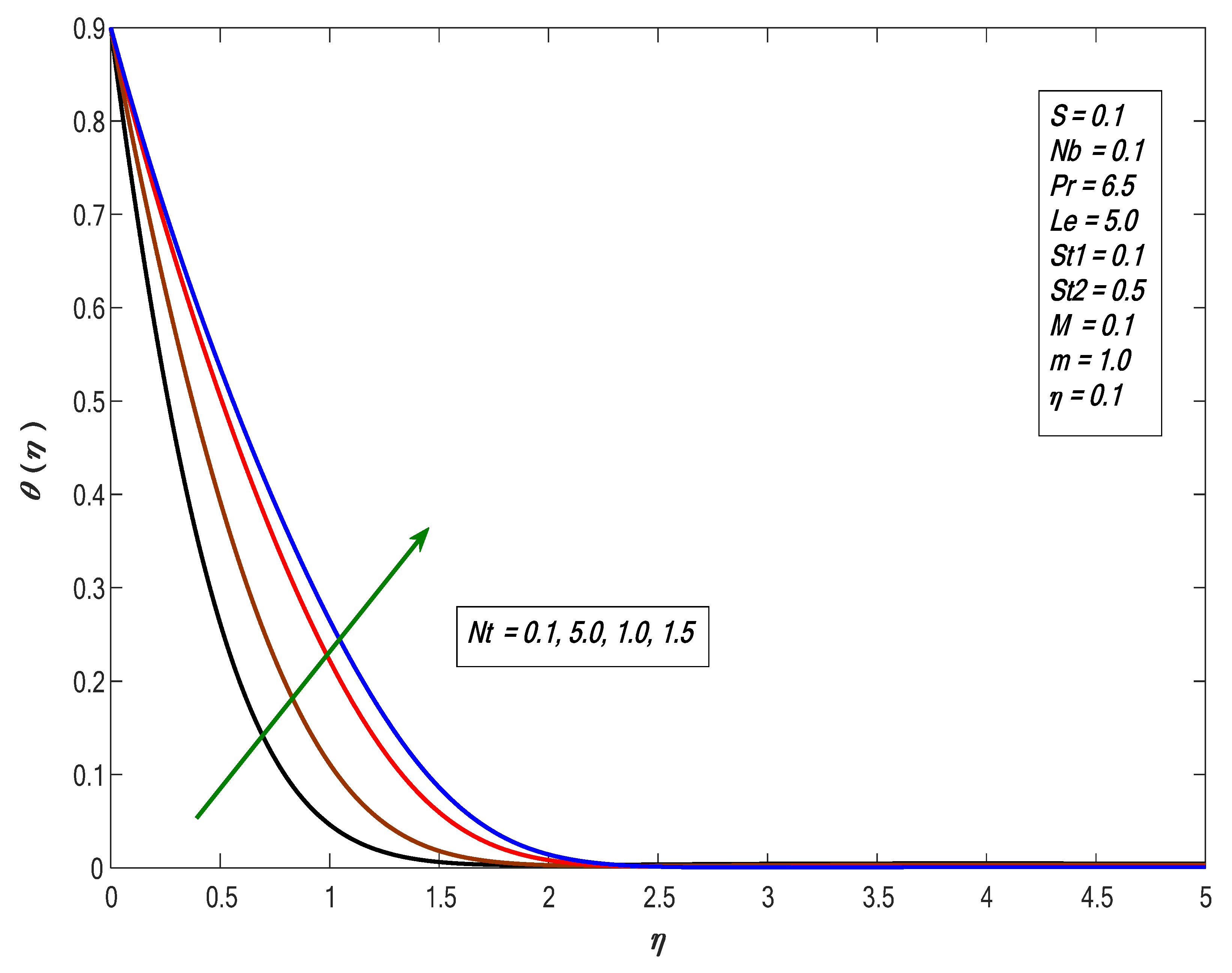
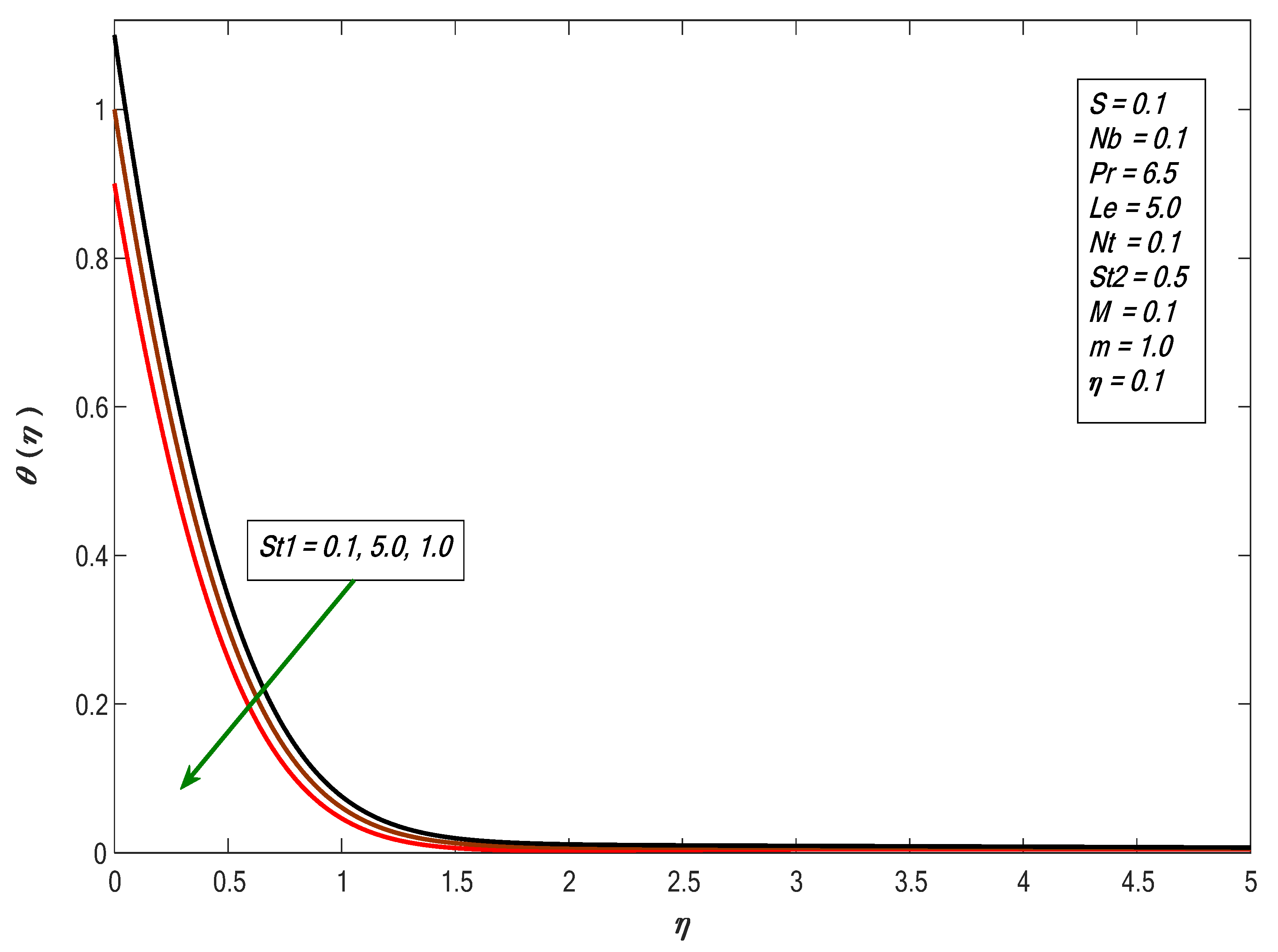
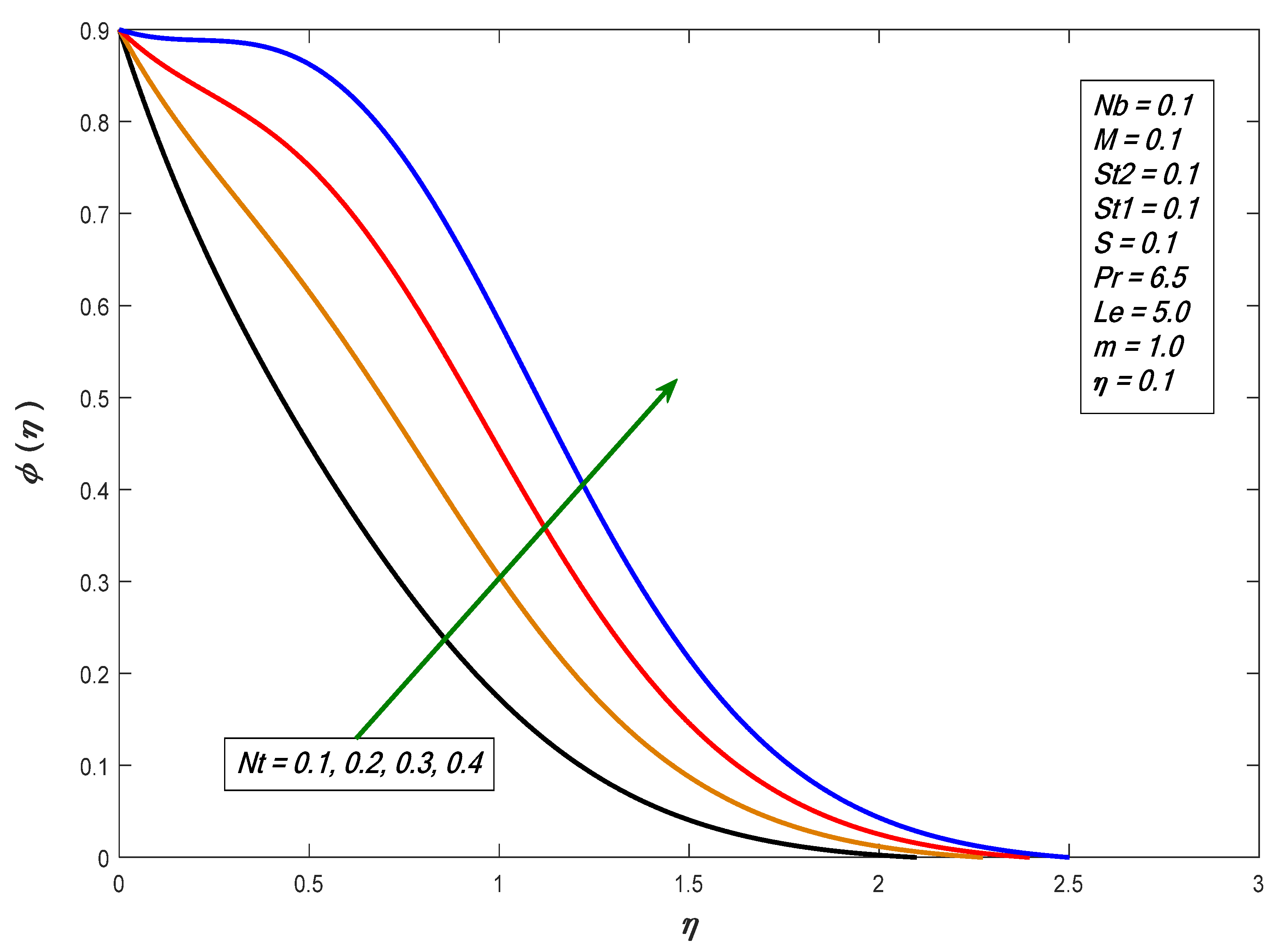
- The temperature profile increases while the concentration profile decreases with increasing .
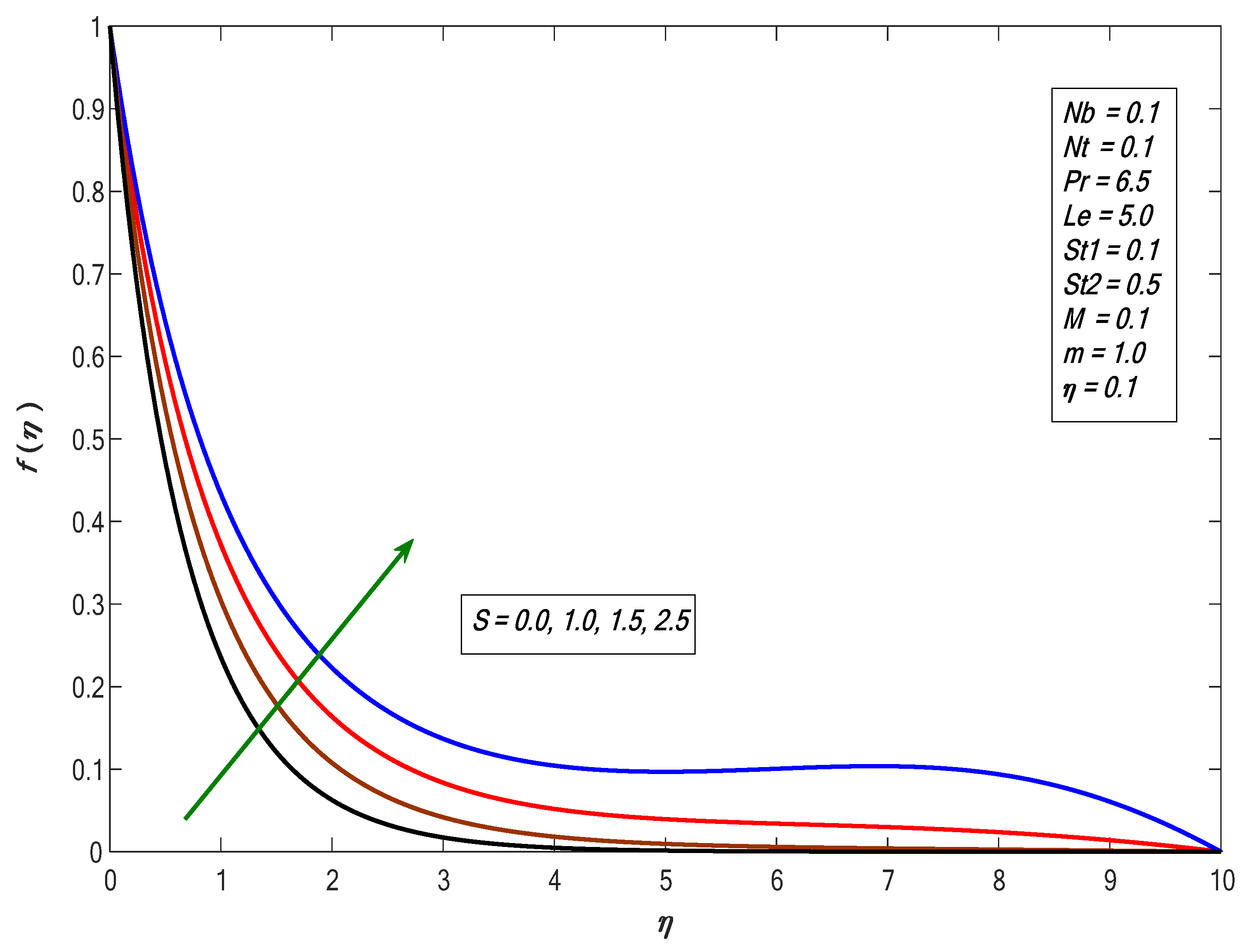
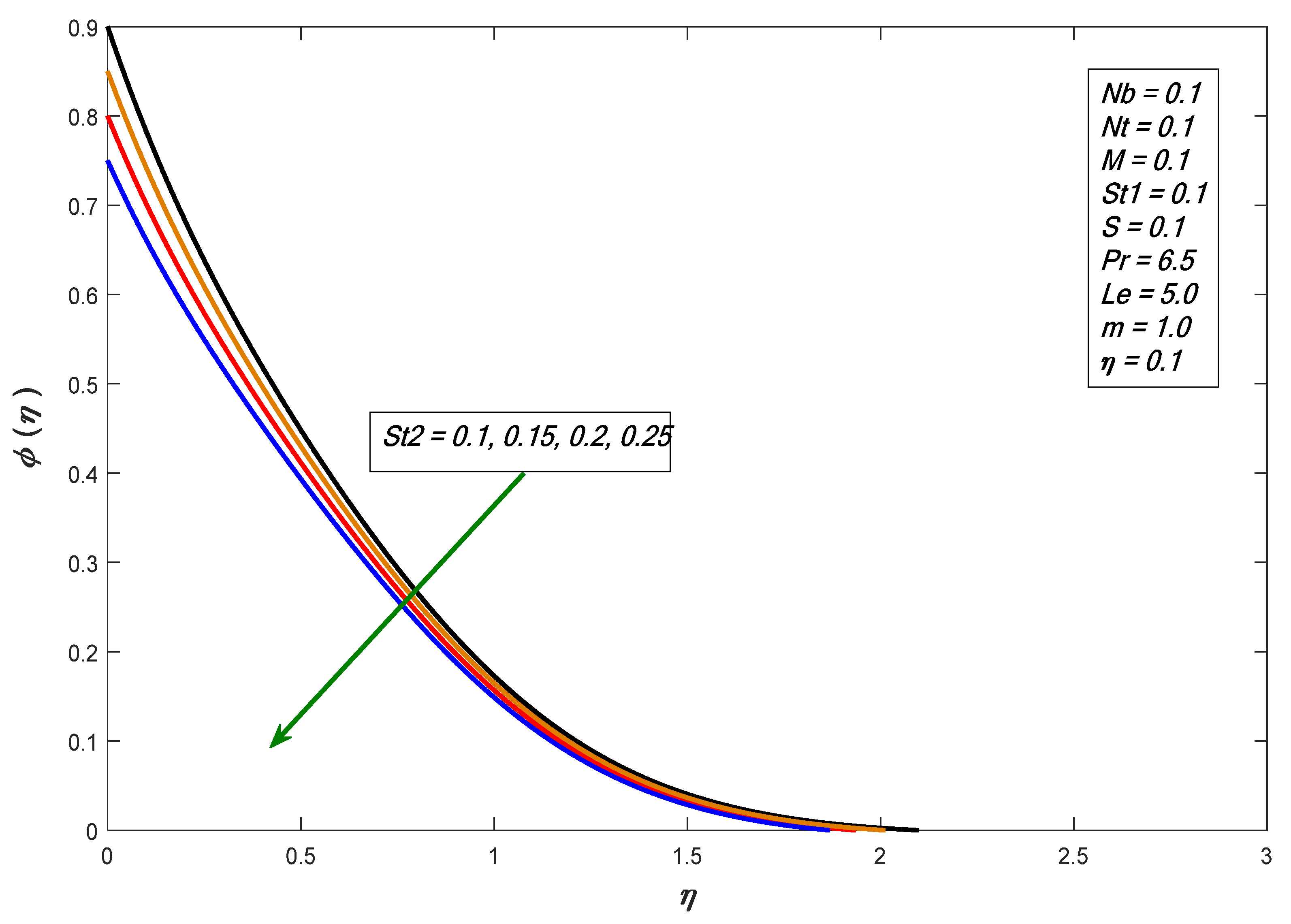
- As increases, both temperature and concentration increase.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| fluid concentration | |
| Skin friction | |
| Specific heat at constant pressure | |
| Surface volume fraction | |
| Ambient nanoparticle volume fraction | |
| Brownian diffusion coefficient | |
| Thermophoretic diffusion coefficient | |
| Similarity function for velocity | |
| Gravitational acceleration | |
| Micro inertia per unit mass | |
| Material parameter | |
| Thermal conductivity | |
| Vertex viscosity | |
| Reference length | |
| Lewis number | |
| Modified Hartmann number | |
| Nondimensional angular velocity | |
| Brownian motion parameter | |
| Thermophoretic parameter | |
| Nusselt number | |
| Prandtl number | |
| Reynolds number | |
| Sherwood number | |
| Thermal stratification | |
| Concentration stratification | |
| Fluid temperature | |
| Wall temperature | |
| Velocity in the direction | |
| Wall Velocity | |
| Ambient velocity | |
| Velocity in the direction | |
| Cartesian coordinate | |
| Thermal diffusivity | |
| Concentration expansion coefficient | |
| Spin gradient viscosity | |
| Modified Grashof number | |
| Local Grashof number | |
| Similarity independent variable | |
| Wall velocity | |
| Dynamic viscosity | |
| Electric conductivity | |
| Dimensionless temperature | |
| Ambient condition | |
| Condition at the wall | |
| Differentiation with respect to |
References
- Sohn, H.; Yang, J.Y.; Lee, H.S.; Park, B.J. Sensing solutions for assessing and monitoring of nuclear power plants (NPPs). In Sensor Technologies for Civil Infrastructures; Woodhead Publishing: Sawston, UK, 2014; pp. 605–637. [Google Scholar]
- Khashi’Ie, N.S.; Arifin, N.M.; Hafidzuddin, E.H.; Wahi, N. Dual Stratified Nanofluid Flow Past a Permeable Shrinking/Stretching Sheet Using a Non-Fourier Energy Model. Appl. Sci. 2019, 9, 2124. [Google Scholar] [CrossRef] [Green Version]
- Yasin, M.H.M.; Arifin, N.M.; Nazar, R.; Ismail, F.; Pop, I. Mixed Convection Boundary Layer Flow Embedded in a Thermally Stratified Porous Medium Saturated by a Nanofluid. Adv. Mech. Eng. 2013, 2013, 121943. [Google Scholar] [CrossRef] [Green Version]
- Hussain, T.; Hussain, S.; Hayat, T. Impact of double stratification and magnetic field in mixed convective radiative flow of Maxwell nanofluid. J. Mol. Liq. 2016, 220, 870–878. [Google Scholar] [CrossRef]
- Besthapu, P.; Haq, R.U.; Bandari, S.; Al-Mdallal, Q.M. Mixed convection flow of thermally stratified MHD nanofluid over an exponentially stretching surface with viscous dissipation effect. J. Taiwan Inst. Chem. Eng. 2017, 71, 307–314. [Google Scholar] [CrossRef]
- Waqas, M.; Khan, M.I.; Hayat, T.; Alsaedi, A. Stratified flow of an Oldroyd-B nanoliquid with heat generation. Results Phys. 2017, 7, 2489–2496. [Google Scholar] [CrossRef]
- Daniel, Y.S.; Aziz, Z.A.; Ismail, Z.; Bahar, A. Unsteady EMHD dual stratified flow of nanofluid with slips impacts. Alex. Eng. J. 2020, 59, 177–189. [Google Scholar] [CrossRef]
- Eswaramoorthi, S.; Alessa, N.; Sangeethavaanee, M.; Namgyel, N. Numerical and Analytical Investigation for Darcy-Forchheimer Flow of a Williamson Fluid over a Riga Plate with Double Stratification and Cattaneo-Christov Dual Flux. Adv. Math. Phys. 2021, 2021, 1867824. [Google Scholar] [CrossRef]
- Mutuku, W.N.; Makinde, O.D. Double stratification effects on heat and mass transfer in unsteady MHD nanofluid flow over a flat surface. Asia Pac. J. Comput. Eng. 2017, 4, 2. [Google Scholar] [CrossRef] [Green Version]
- Aaiza, G.; Khan, I.; Shafie, S. Energy Transfer in Mixed Convection MHD Flow of Nanofluid Containing Different Shapes of Nanoparticles in a Channel Filled with Saturated Porous Medium. Nanoscale Res. Lett. 2015, 10, 1–14. [Google Scholar] [CrossRef] [Green Version]
- Hayat, T.; Ullah, I.; Alsaedi, A.; Waqas, M. Double stratfied flow of nanofluid subject to temperature based thermal conductivity and heat source. Therm. Sci. 2020, 24, 1157–1171. [Google Scholar] [CrossRef]
- Choi, S.U.; Eastman, J.A. Enhancing Thermal Conductivity of Fluids with Nanoparticles; (No. ANL/MSD/CP-84938; CONF-951135-29); Argonne National Lab.: Lemont, IL, USA, 1995. [Google Scholar]
- Kuznetsov, A.; Nield, D. Natural convective boundary-layer flow of a nanofluid past a vertical plate. Int. J. Therm. Sci. 2010, 49, 243–247. [Google Scholar] [CrossRef]
- Sheikholeslami, M.; Sheykholeslami, F.B.; Khoshhal, S.; Mola-Abasia, H.; Ganji, D.D.; Rokni, H.B. Effect of magnetic field on Cu–water nanofluid heat transfer using GMDH-type neural network. Neural Comput. Appl. 2014, 25, 171–178. [Google Scholar] [CrossRef]
- Nield, D.; Kuznetsov, A. Forced convection in a parallel-plate channel occupied by a nanofluid or a porous medium saturated by a nanofluid. Int. J. Heat Mass Transf. 2014, 70, 430–433. [Google Scholar] [CrossRef]
- Wang, R.-J.; Du, J.; Zhu, Z. Effects of wall slip and nanoparticles’ thermophoresis on the convective heat transfer enhancement of nanofluid in a microchannel. J. Therm. Sci. Technol. 2016, 11, JTST00017. [Google Scholar] [CrossRef] [Green Version]
- Rafique, K.; Alotaibi, H.; Nofal, T.A.; Anwar, M.I.; Misiran, M.; Khan, I. Numerical Solutions of Micropolar Nanofluid over an Inclined Surface Using Keller Box Analysis. J. Math. 2020, 2020, 6617652. [Google Scholar] [CrossRef]
- Rafique, K.; Anwar, M.I.; Misiran, M.; Asjad, M.I. Energy and mass transport of micropolar nanofluid flow over an inclined surface with Keller-Box simulation. Heat Transf. 2020, 49, 4592–4611. [Google Scholar] [CrossRef]
- Rafique, K.; Anwar, M.I.; Misiran, M.; Khan, I.; Seikh, A.H.; Sherif, E.-S.M.; Nisar, K.S. Numerical Analysis with Keller-Box Scheme for Stagnation Point Effect on Flow of Micropolar Nanofluid over an Inclined Surface. Symmetry 2019, 11, 1379. [Google Scholar] [CrossRef] [Green Version]
- Koriko, O.K.; Shah, N.A.; Saleem, S.; Chung, J.D.; Omowaye, A.J.; Oreyeni, T. Exploration of bioconvection flow of MHD thixotropic nanofluid past a vertical surface coexisting with both nanoparticles and gyrotactic microorganisms. Sci. Rep. 2021, 11, 16627. [Google Scholar] [CrossRef] [PubMed]
- Mabood, F.; Shamshuddin, M.D.; Mishra, S.R. Characteristics of thermophoresis and Brownian motion on radiative reactive micropolar fluid flow towards continuously moving flat plate: HAM solution. Math. Comput. Simul. 2022, 191, 187–202. [Google Scholar] [CrossRef]
- Srinivasacharya, D.; Sita Ramana, K. Mixed convection of a nanofluid past an inclined wavy surface in the presence of gyrotactic microorganisms. Heat Transf. 2021, 50, 8579–8597. [Google Scholar] [CrossRef]
- Magyari, E.; Keller, B. Heat and mass transfer in the boundary layers on an exponentially stretching continuous surface. J. Phys. D Appl. Phys. 1999, 32, 577. [Google Scholar] [CrossRef]
- Elbashbeshy, E.M.A. Heat transfer over an exponentially stretching continuous surface with suction. Arch. Mech. 2001, 53, 643–651. [Google Scholar]
- Bidin, B.; Nazar, R. Numerical solution of the boundary layer flow over an exponentially stretching sheet with thermal radiation. Eur. J. Sci. Res. 2009, 33, 710–717. [Google Scholar]
- Hayat, T.; Abbas, T.; Ayub, M.; Farooq, M.; Alsaedi, A. Flow of nanofluid due to convectively heated Riga plate with variable thickness. J. Mol. Liq. 2016, 222, 854–862. [Google Scholar] [CrossRef]
- Shaw, S.; Nayak, M.K.; Makinde, O.D. Transient rotational flow of radiative nanofluids over an impermeable Riga plate with variable properties. In Defect and Diffusion Forum; Trans Tech Publications Ltd.: Freinbach, Switzerland, 2018; Volume 387, pp. 640–652. [Google Scholar]
- Nadeem, S.; Malik, M.Y.; Abbas, N. Heat transfer of three-dimensional micropolar fluid on a Riga plate. Can. J. Phys. 2020, 98, 32–38. [Google Scholar] [CrossRef]
- Eid, M.R. Thermal Characteristics of 3D Nanofluid Flow over a Convectively Heated Riga Surface in a Darcy–Forchheimer Porous Material with Linear Thermal Radiation: An Optimal Analysis. Arab. J. Sci. Eng. 2020, 45, 9803–9814. [Google Scholar] [CrossRef]
- Abbas, N.; Nadeem, S.; Saleem, S.; Issakhov, A. Transportation of modified nanofluid flow with time dependent viscosity over a Riga plate: Exponentially stretching. Ain Shams Eng. J. 2021, 12, 3967–3973. [Google Scholar] [CrossRef]
- Alotaibi, H.; Rafique, K. Numerical Analysis of Micro-Rotation Effect on Nanofluid Flow for Vertical Riga Plate. Crystals 2021, 11, 1315. [Google Scholar] [CrossRef]
- Ibrahim, W.; Makinde, O.D. The effect of double stratification on boundary-layer flow and heat transfer of nanofluid over a vertical plate. Comput. Fluids 2013, 86, 433–441. [Google Scholar] [CrossRef]
- Anwar, M.I.; Shafie, S.; Hayat, T.; Shehzad, S.A.; Salleh, M.Z. Numerical study for MHD stagnation-point flow of a micropolar nanofluid towards a stretching sheet. J. Braz. Soc. Mech. Sci. Eng. 2017, 39, 89–100. [Google Scholar] [CrossRef] [Green Version]
- Khan, W.A.; Pop, I. Boundary-layer flow of a nanofluid past a stretching sheet. Int. J. Heat Mass Transf. 2010, 53, 2477–2483. [Google Scholar] [CrossRef]



| Pr | M | Bidin and Nazar [25] | Present Results |
|---|---|---|---|
| 1 | 0 | 0.9548 | 0.9548 |
| 2 | 0 | 1.4714 | 1.4714 |
| 3 | 0 | 1.8691 | 1.8691 |
| Nb | Nt | Pr | Le | M | K | S | m | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 0.1 | 0.1 | 6.5 | 5.0 | 0.1 | 1.0 | 0.1 | 0.1 | 0.5 | 1.0 | 0.1 | 1.0 | 0.1 | 0.9752 | 0.3786 | 0.8619 |
| 0.5 | 0.1 | 6.5 | 5.0 | 0.1 | 1.0 | 0.1 | 0.1 | 0.5 | 1.0 | 0.1 | 1.0 | 0.1 | 0.4772 | 0.6934 | 0.9391 |
| 0.1 | 0.5 | 6.5 | 5.0 | 0.1 | 1.0 | 0.1 | 0.1 | 0.5 | 1.0 | 0.1 | 1.0 | 0.1 | 0.4795 | 0.9095 | 0.6288 |
| 0.1 | 0.1 | 10.0 | 5.0 | 0.1 | 1.0 | 0.1 | 0.1 | 0.5 | 1.0 | 0.1 | 1.0 | 0.1 | 1.0108 | 0.3957 | 0.8560 |
| 0.1 | 0.1 | 6.5 | 10.0 | 0.1 | 1.0 | 0.1 | 0.1 | 0.5 | 1.0 | 0.1 | 1.0 | 0.1 | 0.9090 | 0.7549 | 0.9376 |
| 0.1 | 0.1 | 6.5 | 5.0 | 0.5 | 1.0 | 0.1 | 0.1 | 0.5 | 1.0 | 0.1 | 1.0 | 0.1 | 1.0068 | 0.4239 | 0.3894 |
| 0.1 | 0.1 | 6.5 | 5.0 | 0.1 | 3.0 | 0.1 | 0.1 | 0.5 | 1.0 | 0.1 | 1.0 | 0.1 | 0.9896 | 0.3971 | 1.2545 |
| 0.1 | 0.1 | 6.5 | 5.0 | 0.1 | 1.0 | 0.5 | 0.1 | 0.5 | 1.0 | 0.1 | 1.0 | 0.1 | 0.2550 | 0.4297 | 0.6916 |
| 0.1 | 0.1 | 6.5 | 5.0 | 0.1 | 1.0 | 0.1 | 0.5 | 0.5 | 1.0 | 0.1 | 1.0 | 0.1 | 0.5881 | 0.4757 | 0.9169 |
| 0.1 | 0.1 | 6.5 | 5.0 | 0.1 | 1.0 | 0.1 | 0.1 | 0.7 | 1.0 | 0.1 | 1.0 | 0.1 | 1.0369 | 0.0518 | 0.9238 |
| 0.1 | 0.1 | 6.5 | 5.0 | 0.1 | 1.0 | 1.0 | 0.1 | 0.5 | 2.0 | 0.1 | 1.0 | 0.1 | 0.9742 | 0.3771 | 0.8749 |
| 0.1 | 0.1 | 6.5 | 5.0 | 0.1 | 1.0 | 1.0 | 0.1 | 0.5 | 1.0 | 0.5 | 1.0 | 0.1 | 0.9804 | 0.3823 | 0.7440 |
| 0.1 | 0.1 | 6.5 | 5.0 | 0.1 | 1.0 | 1.0 | 0.1 | 0.5 | 1.0 | 0.1 | 1.5 | 0.1 | 0.9871 | 0.3884 | 0.6191 |
| 0.1 | 0.1 | 6.5 | 5.0 | 0.1 | 1.0 | 1.0 | 0.1 | 0.5 | 1.0 | 0.1 | 1.0 | 0.5 | 0.9771 | 0.3770 | 0.7896 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rafique, K.; Alotaibi, H.; Ibrar, N.; Khan, I. Stratified Flow of Micropolar Nanofluid over Riga Plate: Numerical Analysis. Energies 2022, 15, 316. https://doi.org/10.3390/en15010316
Rafique K, Alotaibi H, Ibrar N, Khan I. Stratified Flow of Micropolar Nanofluid over Riga Plate: Numerical Analysis. Energies. 2022; 15(1):316. https://doi.org/10.3390/en15010316
Chicago/Turabian StyleRafique, Khuram, Hammad Alotaibi, Nida Ibrar, and Ilyas Khan. 2022. "Stratified Flow of Micropolar Nanofluid over Riga Plate: Numerical Analysis" Energies 15, no. 1: 316. https://doi.org/10.3390/en15010316
APA StyleRafique, K., Alotaibi, H., Ibrar, N., & Khan, I. (2022). Stratified Flow of Micropolar Nanofluid over Riga Plate: Numerical Analysis. Energies, 15(1), 316. https://doi.org/10.3390/en15010316






