Review of Impedance-Based Analysis Methods Applied to Grid-Forming Inverters in Inverter-Dominated Grids
Abstract
:1. Introduction
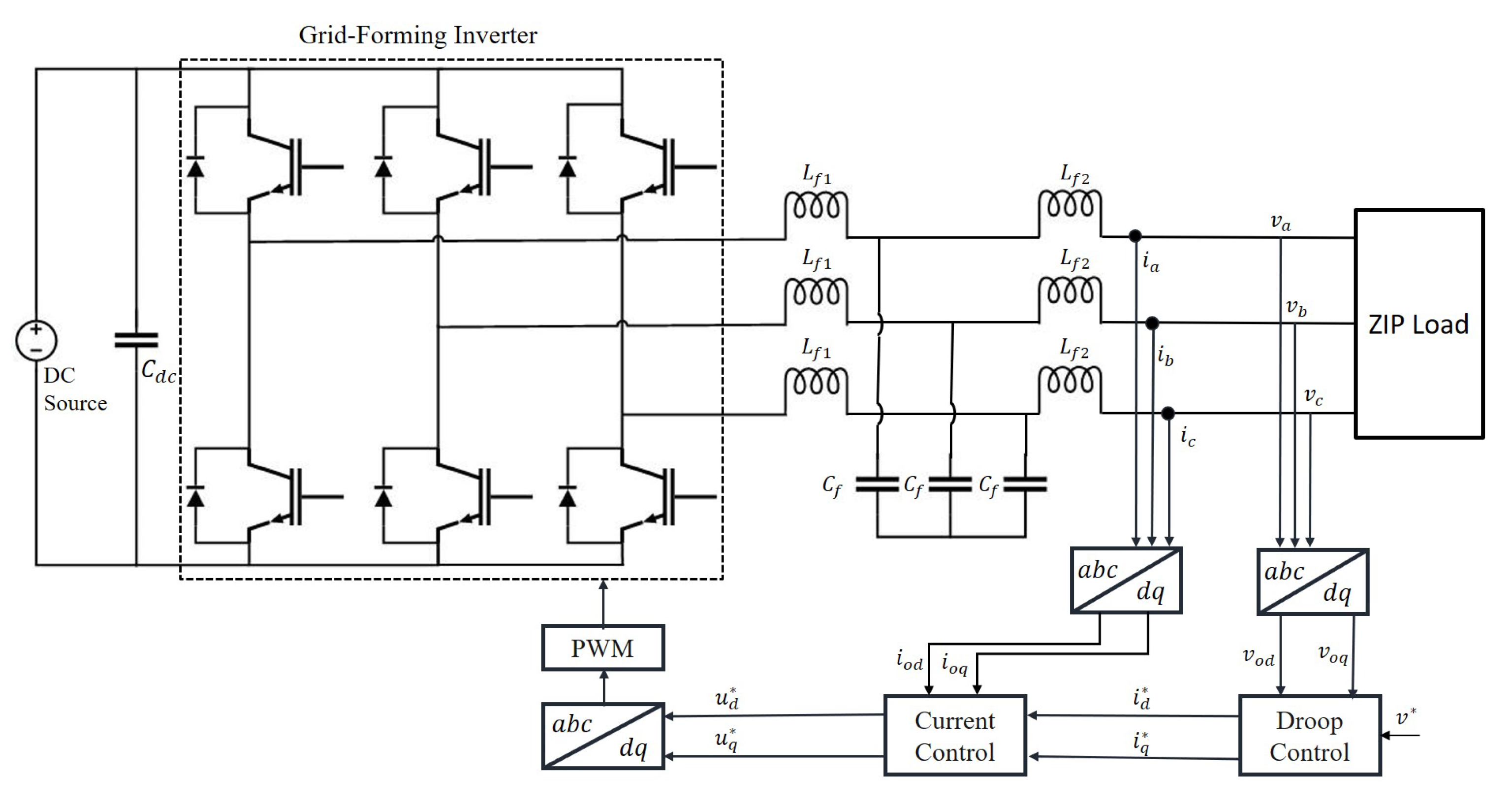
2. Grid-Forming Control
- They control voltage source inverters as dispatchable voltage sources with independent control of voltage and frequency. The output current is then determined by the system loading conditions and the inverter current limits. Their ability to function as an “infinite bus” is limited by the size and strength of the DC source.
- Since they do not rely on a stiff grid for synchronism, grid-forming inverters impose the grid voltage frequency and phase angle reference through their own controls and are capable of blackstart operation without a dedicated phase-locked loop (PLL).
- Grid-forming inverters are voltage-controlled and have a smaller output impedance compared to grid-following inverters, which makes them suitable for weak AC grids.
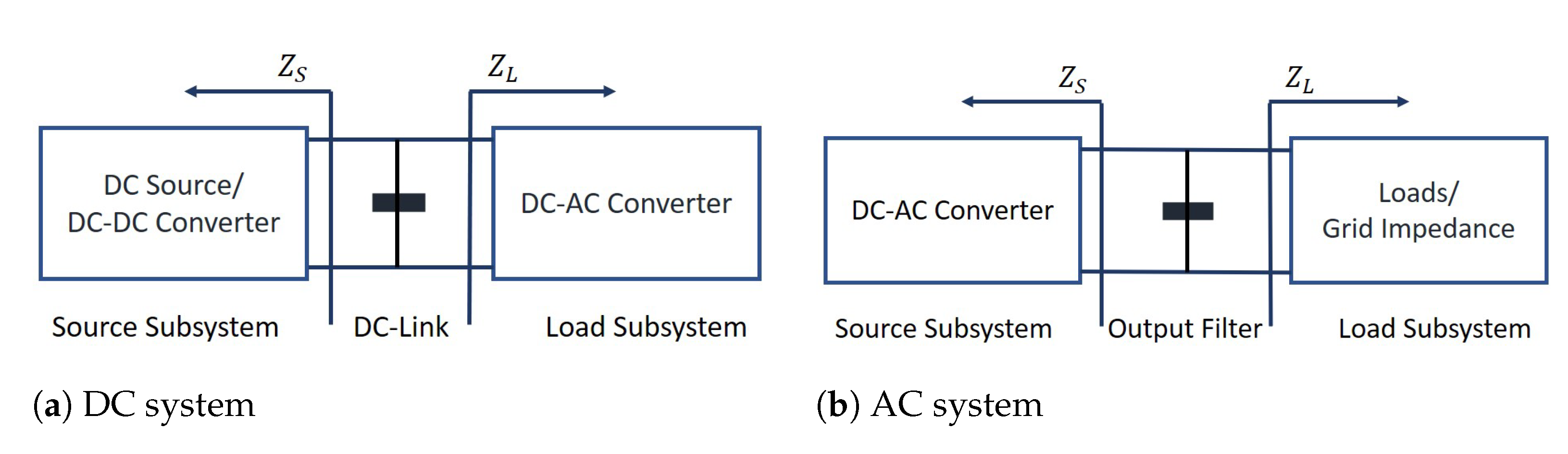
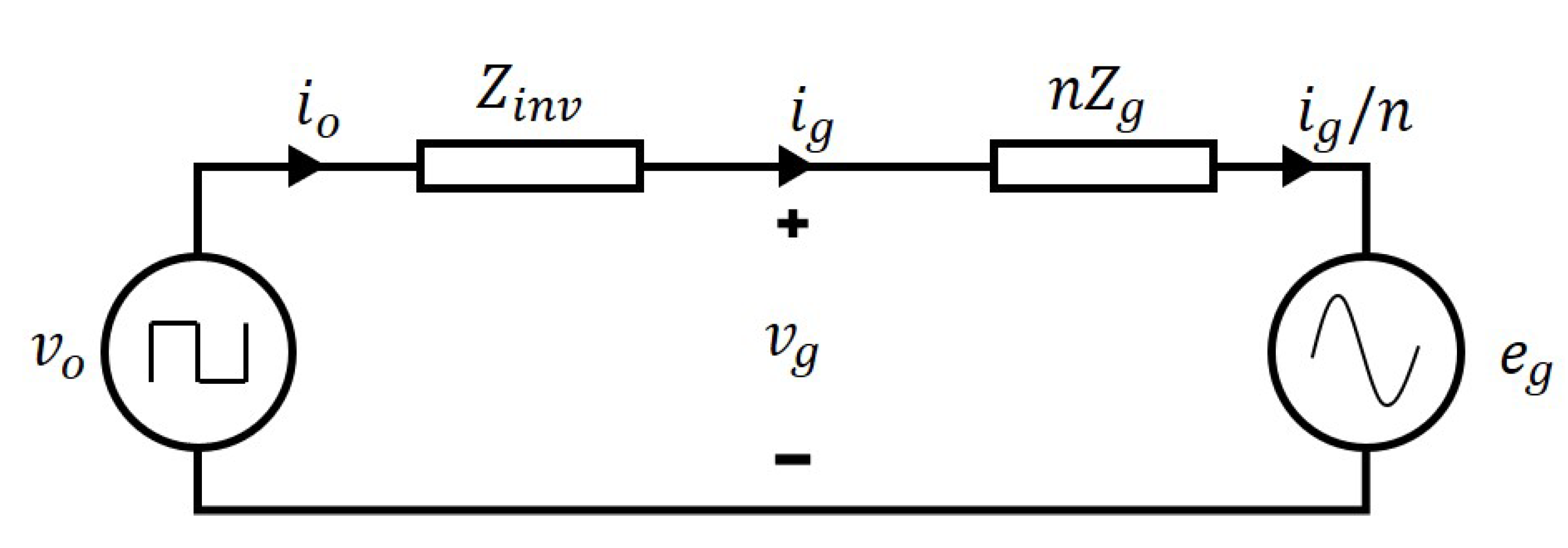
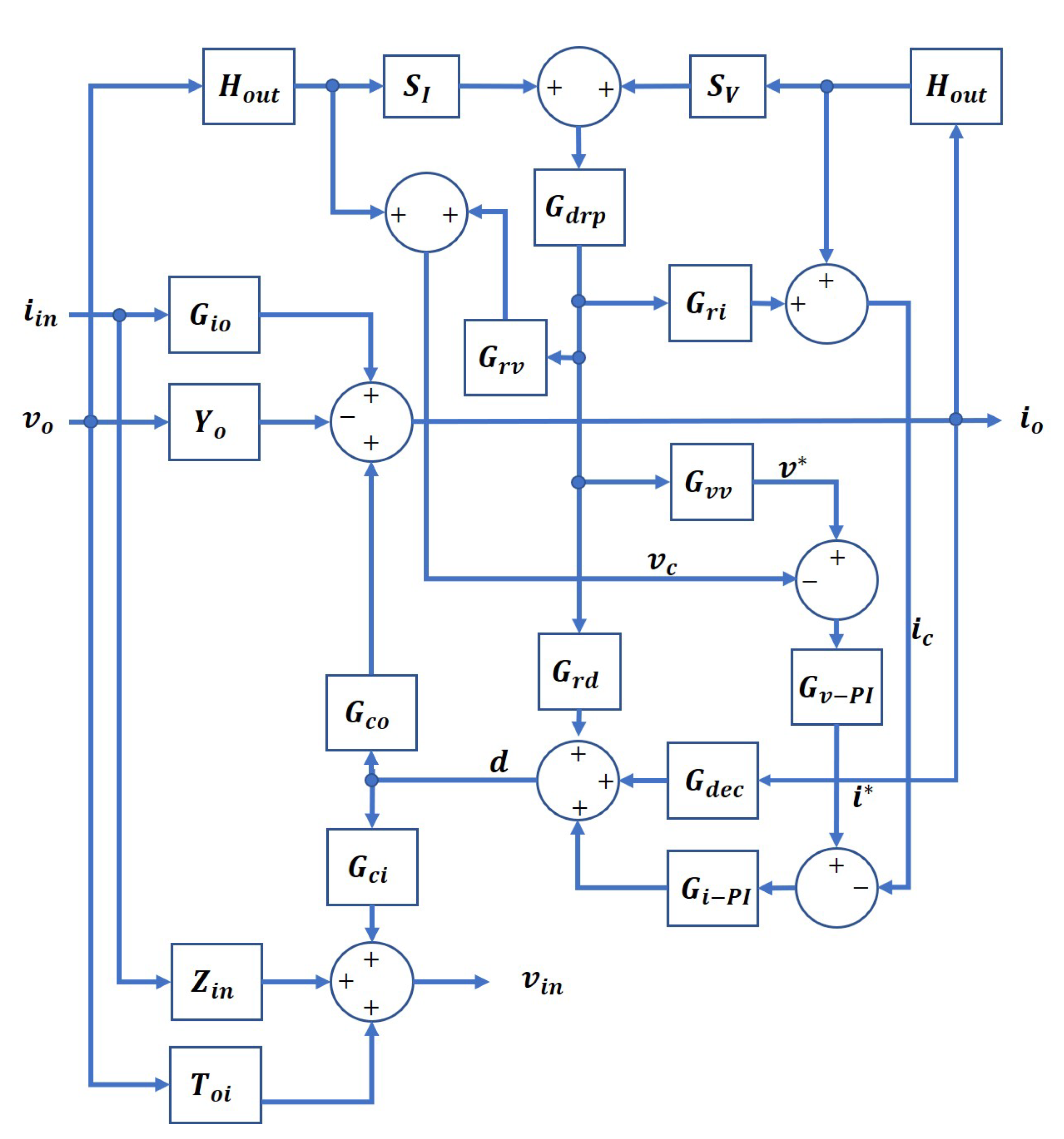
3. Impedance-Based Analysis for Controller Design
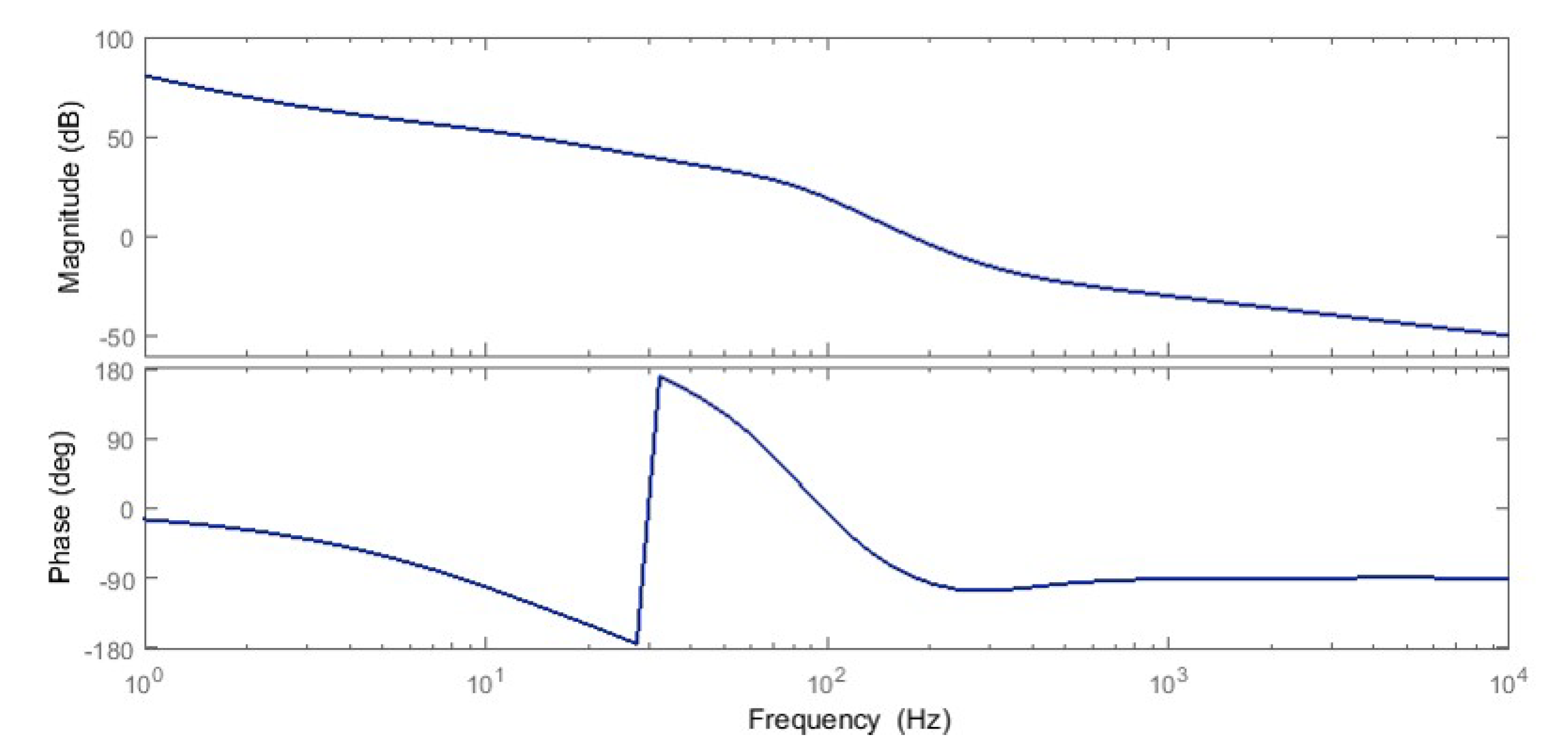
3.1. DC-Side Stability
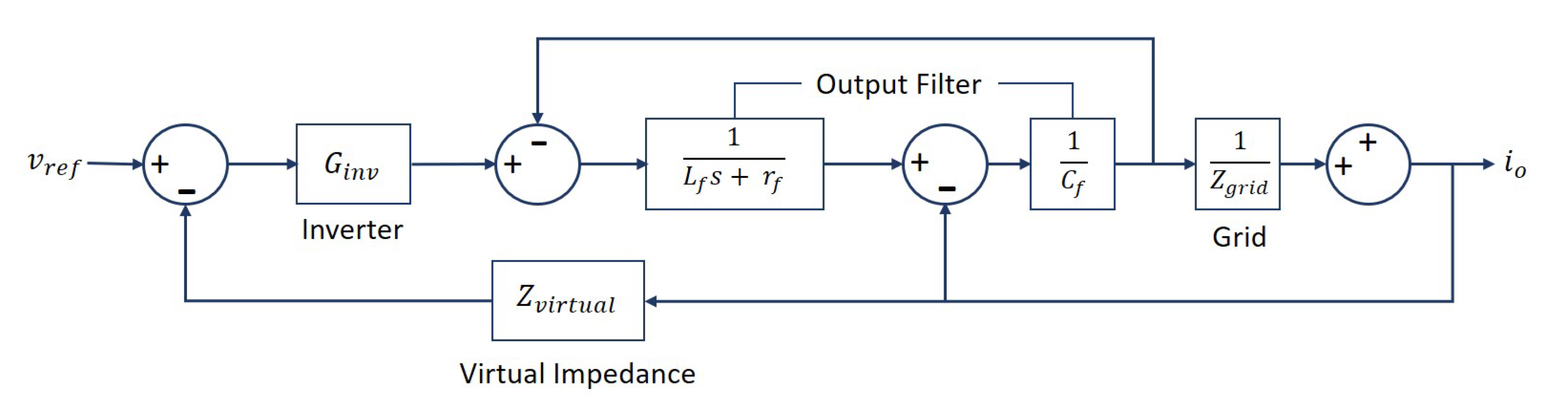
3.2. AC-Side Stability
3.3. Load Disturbance Compensation
3.4. Cross-Coupling in Different Domains
3.5. Improved Power Sharing
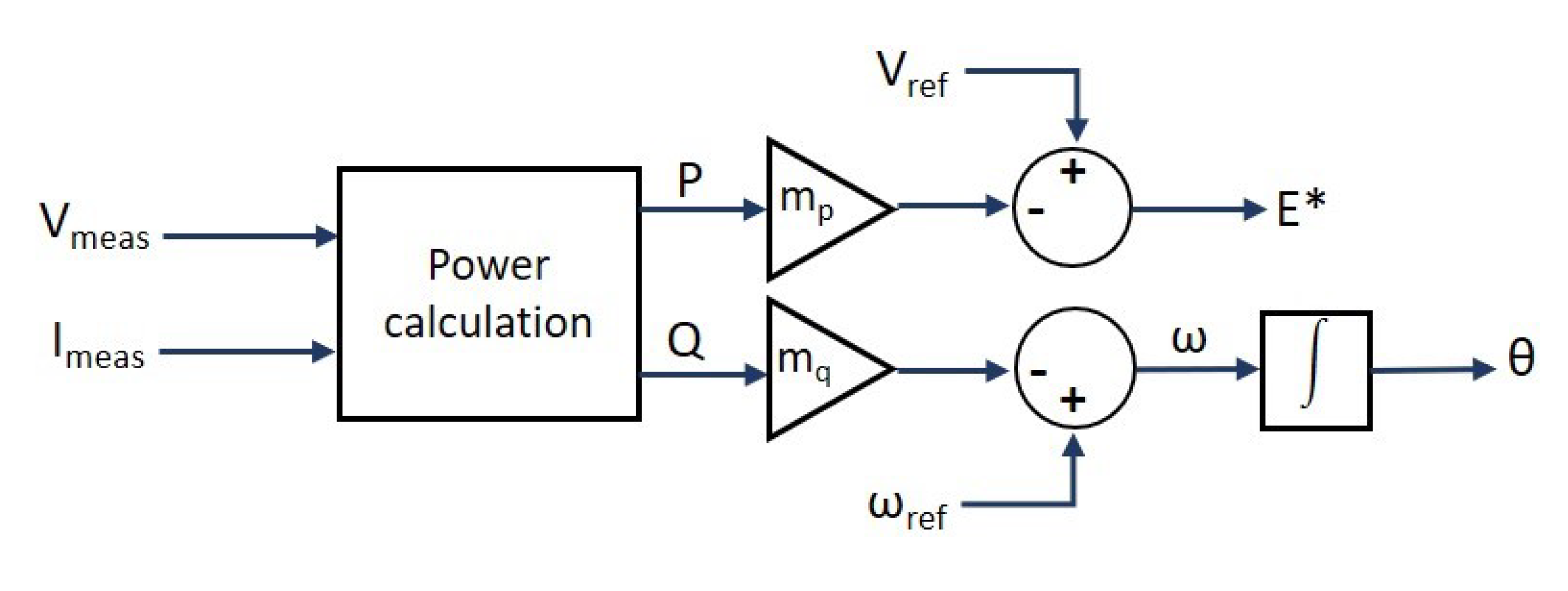
3.6. Synchronization Stability
3.7. Harmonic Stability
4. Application of Impedance Analysis to Droop Control
5. Conclusions
- 1.
- The input impedance should not behave as a negative resistance and remain in the passive region.
- 2.
- The output impedance should also be passive to prevent resonant oscillations in the AC output.
- 3.
- The output impedance should be small enough to not only reduce circulating currents and improve power sharing accuracy but to also reduce the effect of grid-side/load disturbances.
- 4.
- The impact of synchronization control on inverter impedances should be included for a comprehensive analysis.
- 5.
- Couplings among paralleled inverters which create resonant interactions should be compensated.
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Milano, F.; Dörfler, F.; Hug, G.; Hill, D.J.; Verbič, G. Foundations and Challenges of Low-Inertia Systems (Invited Paper). In Proceedings of the 2018 Power Systems Computation Conference (PSCC), Dublin, Ireland, 11–15 June 2018; pp. 1–25. [Google Scholar]
- Tielens, P.; Van Hertem, D. The relevance of inertia in power systems. Renew. Sustain. Energy Rev. 2016, 55, 999–1009. [Google Scholar] [CrossRef]
- Migrate Project: Deliverable 3.1. Description of System Needs and Test Cases. 2016. Available online: https://www.h2020-migrate.eu/_Resources/Persistent/85d17e132de9f44be3a67452723dfb585729cc5d/MIGRATE_D3-1_System%20needs%20and%20test%20cases_v01.pdf (accessed on 7 May 2021).
- Suntio, T.; Messo, T.; Berg, M.; Alenius, H.; Reinikka, T.; Luhtala, R.; Zenger, K. Impedance-Based Interactions in Grid-Tied Three-Phase Inverters in Renewable Energy Applications. Energies 2019, 12, 464. [Google Scholar] [CrossRef] [Green Version]
- Bevrani, H.; Ise, T.; Miura, Y. Virtual Synchronous Generators: A Survey and New Perspectives. Int. J. Electr. Power Energy Syst. 2014, 54, 244–254. [Google Scholar] [CrossRef]
- Ashabani, M. Synchronous converter and synchronous-VSC- state of art of universal control strategies for smart grid integration. In Proceedings of the 2014 Smart Grid Conference (SGC), Tehran, Iran, 9–10 December 2014; pp. 1–8. [Google Scholar]
- Johnson, B.B.; Sinha, M.; Ainsworth, N.G.; Dörfler, F.; Dhople, S.V. Synthesizing Virtual Oscillators to Control Islanded Inverters. IEEE Trans. Power Electron. 2016, 31, 6002–6015. [Google Scholar] [CrossRef]
- Arghir, C.; Jouini, T.; Dörfler, F. Grid-forming control for power converters based on matching of synchronous machines. Automatica 2018, 95, 273–282. [Google Scholar] [CrossRef] [Green Version]
- Luhtala, R.; Messo, T.; Roinila, T.; Alenius, H.; de Jong, E.; Burstein, A.; Fabian, A. Identification of Three-Phase Grid Impedance in the Presence of Parallel Converters. Energies 2019, 12, 2674. [Google Scholar] [CrossRef] [Green Version]
- Li, Y.; Gu, Y.; Zhu, Y.; Junyent Ferre, A.; Xiang, X.; Green, T.C. Impedance Circuit Model of Grid-Forming Inverter: Visualizing Control Algorithms as Circuit Elements. IEEE Trans. Power Electron. 2020. [Google Scholar] [CrossRef]
- Ye, Q.; Mo, R.; Li, H. Multiple Resonances Mitigation of Paralleled Inverters in a Solid-State Transformer (SST) Enabled AC Microgrid. IEEE Trans. Smart Grid 2018, 9, 4744–4754. [Google Scholar] [CrossRef]
- Liu, J.; Miura, Y.; Ise, T. Cost-Function-Based Microgrid Decentralized Control of Unbalance and Harmonics for Simultaneous Bus Voltage Compensation and Current Sharing. IEEE Trans. Power Electron. 2019, 34, 7397–7410. [Google Scholar] [CrossRef]
- Burman, K.; Kandt, A.; Lisell, L.; Booth, S.; Walker, A.; Roberts, J.; Falcey, J. Targeting Net Zero Energy at Marine Corps Base Kaneohe Bay, Hawaii: Assessment and Recommendations; National Renewable Energy Lab. (NREL): Golden, CO, USA, 2011. [Google Scholar]
- Dragičević, T.; Lu, X.; Vasquez, J.C.; Guerrero, J.M. DC Microgrids—Part I: A Review of Control Strategies and Stabilization Techniques. IEEE Trans. Power Electron. 2016, 31, 4876–4891. [Google Scholar]
- Cezar, G.; Rajagopal, R.; Zhang, B. Stability of interconnected DC converters. In Proceedings of the 54th IEEE Conference on Decision and Control (CDC), Osaka, Japan, 15–18 December 2015; pp. 9–14. [Google Scholar]
- Huang, Y.; Yuan, X.; Hu, J.; Zhou, P. Modeling of VSC Connected to Weak Grid for Stability Analysis of DC-Link Voltage Control. IEEE J. Emerg. Sel. Top. Power Electron. 2015, 3, 1193–1204. [Google Scholar] [CrossRef]
- Puukko, J.; Messo, T.; Nousiainen, L.; Huusari, J.; Suntio, T. Negative output impedance in three-phase grid-connected renewable energy source inverters based on reduced-order model. In Proceedings of the IET Conference on Renewable Power Generation (RPG 2011), Edinburgh, UK, 6–8 September 2011; pp. 1–6. [Google Scholar]
- Song, Y.; Hill, D.J.; Liu, T. Impact of DG Connection Topology on the Stability of Inverter-Based Microgrids. IEEE Trans. Power Syst. 2019, 34, 3970–3972. [Google Scholar] [CrossRef]
- Wen, B.; Boroyevich, D.; Mattavelli, P.; Burgos, R.; Shen, Z. Modeling the output impedance negative incremental resistance behavior of grid-tied inverters. In Proceedings of the 2014 IEEE Applied Power Electronics Conference and Exposition—APEC 2014, Fort Worth, TX, USA, 16–20 March 2014; pp. 1799–1806. [Google Scholar]
- Messo, T.; Jokipii, J.; Mäkinen, A.; Suntio, T. Modeling the grid synchronization induced negative-resistor-like behavior in the output impedance of a three-phase photovoltaic inverter. In Proceedings of the 2013 4th IEEE International Symposium on Power Electronics for Distributed Generation Systems (PEDG), Rogers, AR, USA, 8–11 July 2013; pp. 1–7. [Google Scholar]
- Gong, H.; Yang, D.; Wang, X. Identification of the DQ Impedance Model for Three-Phase Power Converter Considering the Coupling Effect of the Grid Impedance. In Proceedings of the 2019 IEEE Applied Power Electronics Conference and Exposition (APEC), Anaheim, CA, USA, 17–21 March 2019; pp. 120–126. [Google Scholar]
- Ray, I.; Tolbert, L.M. The Case Against Phase-Locked Loops in Weak AC Grids. In Proceedings of the 2019 IEEE Electrical Power and Energy Conference (EPEC), Montreal, QC, Canada, 16–18 October 2019; pp. 1–5. [Google Scholar]
- Perez, M.; Ortega, R.; Espinoza, J.R. Passivity-based PI control of switched power converters. IEEE Trans. Control Syst. Technol. 2004, 12, 881–890. [Google Scholar] [CrossRef]
- Azmi, S.A.; Ahmed, K.H.; Finney, S.J.; Williams, B.W. Comparative analysis between voltage and current source inverters in grid-connected application. In Proceedings of the IET Conference on Renewable Power Generation (RPG 2011), Edinburgh, UK, 6–8 September 2011; pp. 1–6. [Google Scholar]
- Bayhan, S.; Abu-Rub, H.; Leon, J.I.; Vazquez, S.; Franquelo, L.G. Power electronic converters and control techniques in AC microgrids. In Proceedings of the IECON 2017—43rd Annual Conference of the IEEE Industrial Electronics Society, Beijing, China, 29 October–1 November 2017; pp. 6179–6186. [Google Scholar]
- NERC. Inverter-Based Resource Performance Guideline; NERC: Atlanta, GA, USA, 2018. [Google Scholar]
- D’silva, S.; Shadmand, M.; Bayhan, S.; Abu-Rub, H. Towards Grid of Microgrids: Seamless Transition between Grid-Connected and Islanded Modes of Operation. IEEE Open J. Ind. Electron. Soc. 2020, 1, 66–81. [Google Scholar] [CrossRef]
- Ansari, S.; Chandel, A.; Tariq, M. A Comprehensive Review on Power Converters Control and Control Strategies of AC/DC Microgrid. IEEE Access 2021, 9, 17998–18015. [Google Scholar] [CrossRef]
- Unruh, P.; Nuschke, M.; Strauß, P.; Welck, F. Overview on Grid-Forming Inverter Control Methods. Energies 2020, 13, 2589. [Google Scholar] [CrossRef]
- Tamrakar, U.; Shrestha, D.; Maharjan, M.; Bhattarai, B.; Hansen, T.; Tonkoski, R. Virtual Inertia: Current Trends and Future Directions. Appl. Sci. 2017, 7, 654. [Google Scholar] [CrossRef]
- Yan, X.; Cui, Y.; Cui, S. Control Method of Parallel Inverters with Self-Synchronizing Characteristics in Distributed Microgrid. Energies 2019, 12, 3871. [Google Scholar] [CrossRef] [Green Version]
- Hossain, M.; Pota, H.; Issa, W.; Hossain, M. Overview of AC Microgrid Controls with Inverter-Interfaced Generations. Energies 2017, 10, 1300. [Google Scholar] [CrossRef]
- Shuai, Z.; Mo, S.; Wang, J.; Shen, Z.J.; Tian, W.; Feng, Y. Droop control method for load share and voltage regulation in high-voltage microgrids. J. Mod. Power Syst. Clean Energy 2016, 4, 76–86. [Google Scholar] [CrossRef] [Green Version]
- Zhang, Z.; Chen, W.; Zhang, Z. A New Seamless Transfer Control Strategy of the Microgrid. Sci. World J. 2014. [Google Scholar] [CrossRef] [PubMed]
- Ashabani, M.; Freijedo, F.D.; Golestan, S.; Guerrero, J.M. Inducverters: PLL-Less Converters with Auto-Synchronization and Emulated Inertia Capability. IEEE Trans. Smart Grid 2016, 7, 1660–1674. [Google Scholar] [CrossRef]
- Tayyebi, A.; Dörfler, F.; Kupzog, F.; Miletic, Z.; Hribernik, W. Grid-Forming Converters—Inevitability, Control Strategies and Challenges in Future Grid Applications. In Proceedings of the Workshop Microgrids Local Energy Communities (CIRED 2018), Ljubljana, Slovenia, 7–8 June 2018. [Google Scholar]
- Monica, P.; Kowsalya, M. Control strategies of parallel operated inverters in renewable energy application: A review. Renew. Sustain. Energy Rev. 2016, 65, 885–901. [Google Scholar] [CrossRef]
- Engler, A. Applicability of droops in low voltage grids. Int. J. Distrib. Energy Resour. 2005, 1, 3–15. [Google Scholar]
- Amin, M.; Molinas, M. Small-Signal Stability Assessment of Power Electronics Based Power Systems: A Discussion of Impedance- and Eigenvalue-Based Methods. IEEE Trans. Ind. Appl. 2017, 53, 5014–5030. [Google Scholar] [CrossRef]
- Oue, K.; Sano, S.; Kato, T.; Inoue, K. Stability Analysis of Grid-Forming Inverter in DQ Frequency Domain. In Proceedings of the 2019 20th Workshop on Control and Modeling for Power Electronics (COMPEL), Toronto, ON, Canada, 16–19 June 2019; pp. 1–8. [Google Scholar]
- Liu, H.; Shah, S.; Sun, J. An impedance-based approach to HVDC system stability analysis and control development. In Proceedings of the 2014 International Power Electronics Conference (IPEC-Hiroshima 2014—ECCE ASIA), Hiroshima, Japan, 18–21 May 2014; pp. 967–974. [Google Scholar]
- Cho, Y.; Hur, K.; Kang, Y.; Muljadi, E. Impedance-Based Stability Analysis in Grid Interconnection Impact Study Owing to the Increased Adoption of Converter-Interfaced Generators. Energies 2017, 10, 1355. [Google Scholar] [CrossRef]
- Riccobono, A.; Santi, E. Comprehensive Review of Stability Criteria for DC Power Distribution Systems. IEEE Trans. Ind. Appl. 2014, 50, 3525–3535. [Google Scholar] [CrossRef]
- Jusoh, A.B. The instability effect of constant power loads. In Proceedings of the PECon 2004, National Power and Energy Conference, Kuala Lumpur, Malaysia, 29–30 November 2004; pp. 175–179. [Google Scholar]
- Liu, J.; Zhang, W.; Rizzoni, G. Robust Stability Analysis of DC Microgrids with Constant Power Loads. IEEE Trans. Power Syst. 2018, 33, 851–860. [Google Scholar] [CrossRef]
- Ashourloo, M.; Khorsandi, A.; Mokhtari, H. Stabilization of DC microgrids with constant-power loads by an active damping method. In Proceedings of the 4th Annual International Power Electronics, Drive Systems and Technologies Conference, Tehran, Iran, 13–14 February 2013; pp. 471–475. [Google Scholar]
- AL-Nussairi, M.K.; Bayindir, R.; Padmanaban, S.; Mihet-Popa, L.; Siano, P. Constant Power Loads (CPL) with Microgrids: Problem Definition, Stability Analysis and Compensation Techniques. Energies 2017, 10, 1656. [Google Scholar] [CrossRef]
- Adam, G.P.; Ahmed, K.H.; Finney, S.J.; Williams, B.W. Generalized modeling of DC grid for stability studies. In Proceedings of the 4th International Conference on Power Engineering, Energy and Electrical Drives, Istanbul, Turkey, 13–17 May 2013; pp. 1168–1174. [Google Scholar]
- Amirkhan, S.; Radmehr, M.; Rezanejad, M.; Khormali, S. An improved passivity-based control strategy for providing an accurate coordination in a AC/DC hybrid microgrid. J. Frankl. Inst. 2019, 356, 6875–6898. [Google Scholar] [CrossRef]
- Sanchez, S.; Molinas, M.; Degano, M.; Zanchetta, P. Stability evaluation of a DC micro-grid and future interconnection to an AC system. Renew. Energy 2014, 62, 649–656. [Google Scholar] [CrossRef]
- Viinamäki, J.; Kuperman, A.; Suntio, T. Grid-Forming-Mode Operation of Boost-Power-Stage Converter in PV-Generator-Interfacing Applications. Energies 2017, 10, 1033. [Google Scholar] [CrossRef] [Green Version]
- Sokal, N.O. System oscillations from negative input resistance at power input port of switching-mode regulator, amplifier, DC/DC converter, or DC/DC inverter. In Proceedings of the 1973 IEEE Power Electronics Specialists Conference, Pasadena, CA, USA, 11–13 June 1973; pp. 138–140. [Google Scholar]
- Sekizaki, S.; Yorino, N.; Sasaki, Y.; Matsuo, K.; Nakamura, Y.; Zoka, Y.; Shimizu, T.; Nishizaki, I. Proposal of a single-phase synchronous inverter with noninterference performance for power system stability enhancement and emergent microgrid operation. Electr. Eng. Jpn. 2019, 207, 3–13. [Google Scholar] [CrossRef]
- Qorai, T.; Gruson, F.; Colas, F.; Guillaud, X.; Debry, M.S.; Prevost, T. Tuning of Cascaded Controllers for Robust Grid-Forming Voltage Source Converter. In Proceedings of the 2018 Power Systems Computation Conference (PSCC), Dublin, Ireland, 11–15 June 2018; p. 8. [Google Scholar]
- Messo, T.; Aapro, A.; Suntio, T.; Roinila, T. Design of grid-voltage feedforward to increase impedance of grid-connected three-phase inverters with LCL-filter. In Proceedings of the 2016 IEEE 8th International Power Electronics and Motion Control Conference (IPEMC-ECCE Asia), Hefei, China, 22–26 May 2016; pp. 2675–2682. [Google Scholar]
- Hans, F.; Schumacher, W.; Chou, S.; Wang, X. Passivation of Current-Controlled Grid-Connected VSCs Using Passivity Indices. IEEE Trans. Ind. Electron. 2019, 66, 8971–8980. [Google Scholar] [CrossRef]
- Zhu, F.; Xia, M.; Antsaklis, P.J. Passivity analysis and passivation of feedback systems using passivity indices. In Proceedings of the 2014 American Control Conference, Portland, OR, USA, 4–6 June 2014; pp. 1833–1838. [Google Scholar]
- Liu, Q.; Caldognetto, T.; Buso, S. Review and Comparison of Grid-Tied Inverter Controllers in Microgrids. IEEE Trans. Power Electron. 2020, 35, 7624–7639. [Google Scholar] [CrossRef]
- Kato, T.; Inoue, K.; Nakajima, Y. Stabilization of grid-connected inverter system with feed-forward control. In Proceedings of the 2017 IEEE Energy Convers. Congr. Expo. (ECCE), Cincinnati, OH, USA, 1–5 October 2017; pp. 3375–3382. [Google Scholar]
- Akhavan, A.; Mohammadi, H.R.; Vasquez, J.C.; Guerrero, J.M. Passivity-Based Design of Plug-and-Play Current-Controlled Grid-Connected Inverters. IEEE Trans. Power Electron. 2020, 35, 2135–2150. [Google Scholar] [CrossRef]
- Wang, J.; Song, Y.; Monti, A. A study of feedforward control on stability of grid-parallel inverter with various grid impedance. In Proceedings of the 2014 IEEE 5th International Symposium on Power Electronics for Distributed Generation Systems (PEDG), Galway, Ireland, 24–27 June 2014; pp. 1–8. [Google Scholar]
- Liu, H.; Liu, H.; Liu, S.; Peng, H. Investigation of load current feed-forward control strategy for wind power grid connected inverter through VSC-HVDC. In Proceedings of the AIP Conference, Hangzhou, China, 17–18 October 2018; p. 1971. [Google Scholar]
- Yu, H.; Awal, M.A.; Tu, H.; Du, Y.; Lukic, S.; Husain, I. Passivity-Oriented Discrete-Time Voltage Controller Design for Grid-Forming Inverters. In Proceedings of the 2019 IEEE Energy Conversion Congress and Exposition (ECCE), Baltimore, MD, USA, 29 September–3 October 2019; pp. 469–475. [Google Scholar]
- Rygg, A.; Molinas, M.; Zhang, C.; Cai, X. Coupled and decoupled impedance models compared in power electronics systems. arXiv 2016, arXiv:1610.04988. [Google Scholar]
- Rygg, A.; Molinas, M.; Zhang, C.; Cai, X. A Modified Sequence-Domain Impedance Definition and Its Equivalence to the dq-Domain Impedance Definition for the Stability Analysis of AC Power Electronic Systems. IEEE J. Emerg. Sel. Top. Power Electron. 2016, 4, 1383–1396. [Google Scholar] [CrossRef] [Green Version]
- Lu, D.; Wang, X.; Blaabjerg, F. Impedance-Based Analysis of DC-Link Voltage Dynamics in Voltage-Source Converters. IEEE Trans. Power Electron. 2019, 34, 3973–3985. [Google Scholar] [CrossRef] [Green Version]
- Kazem Bakhshizadeh, M.; Wang, X.; Blaabjerg, F.; Hjerrild, J.; Kocewiak, L.; Bak, C.L.; Hesselbæk, B. Couplings in Phase Domain Impedance Modeling of Grid-Connected Converters. IEEE Trans. Power Electron. 2016, 31, 6792–6796. [Google Scholar]
- Messo, T.; Aapro, A.; Suntio, T. Generalized multivariable small-signal model of three-phase grid-connected inverter in DQ-domain. In Proceedings of the 2015 IEEE 16th Workshop on Control and Modeling for Power Electronics (COMPEL), Vancouver, BC, Canada, 12–15 July 2015; pp. 1–8. [Google Scholar]
- Wen, B.; Boroyevich, D.; Mattavelli, P.; Shen, Z.; Burgos, R. Experimental verification of the Generalized Nyquist stability criterion for balanced three-phase ac systems in the presence of constant power loads. In Proceedings of the 2012 IEEE Energy Conversion Congress and Exposition (ECCE), Raleigh, NC, USA, 15–20 September 2012; pp. 3926–3933. [Google Scholar]
- Choi, Y.O.; Kim, J. Output Impedance Control Method of Inverter-Based Distributed Generators for Autonomous Microgrid. Energies 2017, 10, 904. [Google Scholar] [CrossRef] [Green Version]
- Asrari, A.; Mustafa, M.; Ansari, M.; Khazaei, J. Impedance Analysis of Virtual Synchronous Generator-Based Vector Controlled Converters for Weak AC Grid Integration. IEEE Trans. Sustain. Energy 2019, 10, 1481–1490. [Google Scholar] [CrossRef]
- Chen, Y.; Guerrero, J.M.; Shuai, Z.; Chen, Z.; Zhou, L.; Luo, A. Fast Reactive Power Sharing, Circulating Current and Resonance Suppression for Parallel Inverters Using Resistive-Capacitive Output Impedance. IEEE Trans. Power Electron. 2016, 31, 5524–5537. [Google Scholar] [CrossRef] [Green Version]
- Yao, W.; Chen, M.; Matas, J.; Guerrero, J.M.; Qian, Z. Design and Analysis of the Droop Control Method for Parallel Inverters Considering the Impact of the Complex Impedance on the Power Sharing. IEEE Trans. Ind. Electron. 2011, 58, 576–588. [Google Scholar] [CrossRef]
- Shen, Z.; Jaksic, M.; Zhou, B.; Mattavelli, P.; Boroyevich, D.; Verhulst, J.; Belkhayat, M. Analysis of Phase Locked Loop (PLL) influence on DQ impedance measurement in three-phase AC systems. In Proceedings of the 2013 Twenty-Eighth Annual IEEE Applied Power Electronics Conference and Exposition (APEC), Long Beach, CA, USA, 17–21 March 2013; pp. 939–945. [Google Scholar]
- Guo, Y. Impedance Analysis of Three-phase LCL-Type Grid- Connected Inverters with Adaptive PLL. In Proceedings of the 2019 3rd International Conference on Electronic Information Technology and Computer Engineering (EITCE), Xiamen, China, 18–20 October 2019; pp. 21–27. [Google Scholar]
- Yi, H.; Wang, X.; Blaabjerg, F.; Zhuo, F. Impedance Analysis of SOGI-FLL-Based Grid Synchronization. IEEE Trans. Power Electron. 2017, 32, 7409–7413. [Google Scholar] [CrossRef]
- Cavazzana, F.; Khodamoradi, A.; Abedini, H.; Mattavelli, P. Analysis of an Impedance Modeling Approach for Droop-Controlled Inverters in System DQ Frame. In Proceedings of the 2019 IEEE Energy Conversion Congress and Exposition (ECCE), Baltimore, MD, USA, 29 September–3 October 2019; pp. 5576–5583. [Google Scholar]
- Wen, B.; Boroyevich, D.; Mattavelli, P.; Burgos, R.; Shen, Z. Impedance-based analysis of grid-synchronization stability for three-phase paralleled converters. In Proceedings of the 2014 IEEE Applied Power Electronics Conference and Exposition—APEC 2014, Fort Worth, TX, USA, 16–20 March 2014; pp. 1233–1239. [Google Scholar]
- Chen, Z.; Luo, A.; Kuang, H.; Zhou, L.; Chen, Y.; Huang, Y. Harmonic resonance characteristics of large-scale distributed power plant in wideband frequency domain. Electr. Power Syst. Res. 2017, 143, 53–65. [Google Scholar] [CrossRef]
- Teodorescu, R.; Liserre, M.; Rodríguez, P. Grid Filter Design. In Grid Converters for Photovoltaic and Wind Power Systems; John Wiley & Sons, Ltd.: Hoboken, NJ, USA, 2010; pp. 289–312. [Google Scholar]
- Céspedes, M.; Sun, J. Impedance shaping of three-phase grid-parallel voltage-source converters. In Proceedings of the 2012 Twenty-Seventh Annual IEEE Applied Power Electronics Conference and Exposition (APEC), Orlando, FL, USA, 5–9 February 2012; pp. 754–760. [Google Scholar] [CrossRef]
- Yang, D.; Ruan, X.; Wu, H. Impedance Shaping of the Grid-Connected Inverter with LCL Filter to Improve Its Adaptability to the Weak Grid Condition. IEEE Trans. Power Electron. 2014, 29, 5795–5805. [Google Scholar] [CrossRef]
- Lakshmanan, S.A.; Jain, A.; Rajpourhit, B.S. A novel current controlled technique with feed forward DC voltage regulator for grid connected solar PV system. In Proceedings of the 2014 Eighteenth National Power Systems Conference (NPSC), Guwahati, India, 18–20 December 2014; pp. 1–6. [Google Scholar]
- Zhong, Q.; Yu, Z. Can the output impedance of an inverter be designed capacitive? In Proceedings of the IECON 2011—37th Annual Conference of the IEEE Industrial Electronics Society, Melbourne, Australia, 7–10 November 2011; pp. 1220–1225. [Google Scholar]
- Agorreta, J.L.; Borrega, M.; López, J.; Marroyo, L. Modeling and Control of N -Paralleled Grid-Connected Inverters With LCL Filter Coupled Due to Grid Impedance in PV Plants. IEEE Trans. Power Electron. 2011, 26, 770–785. [Google Scholar] [CrossRef]
- Lu, M.; Wang, X.; Loh, P.C.; Blaabjerg, F. Resonance Interaction of Multiparallel Grid-Connected Inverters With LCL Filter. IEEE Trans. Power Electron. 2017, 32, 894–899. [Google Scholar] [CrossRef]
- Enslin, J.H.R.; Hulshorst, W.T.J.; Atmadji, A.M.S.; Heskes, P.J.M.; Kotsopoulos, A.; Cobben, J.F.G.; Van der Sluijs, P. Harmonic interaction between large numbers of photovoltaic inverters and the distribution network. In Proceedings of the 2003 IEEE Bologna Power Tech Conference Proceedings, Bologna, Italy, 23–26 June 2003; Volume 3, p. 6. [Google Scholar]
- Akhavan, A.; Mohammadi, H.R.; Vasquez, J.C.; Guerrero, J.M. Coupling effect analysis and control for grid-connected multi-microgrid clusters. IET Power Electron. 2020, 13, 1059–1070. [Google Scholar] [CrossRef]
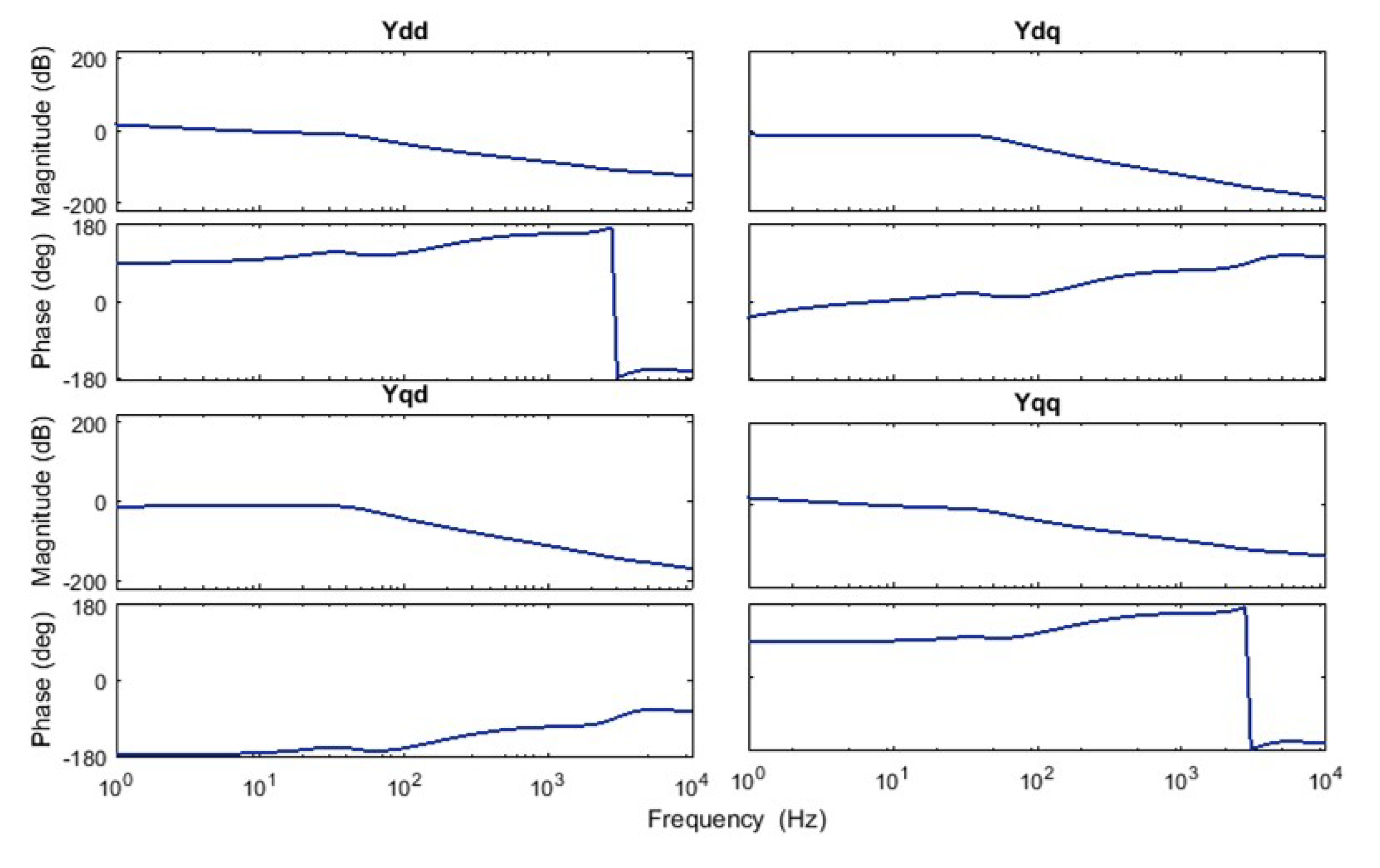
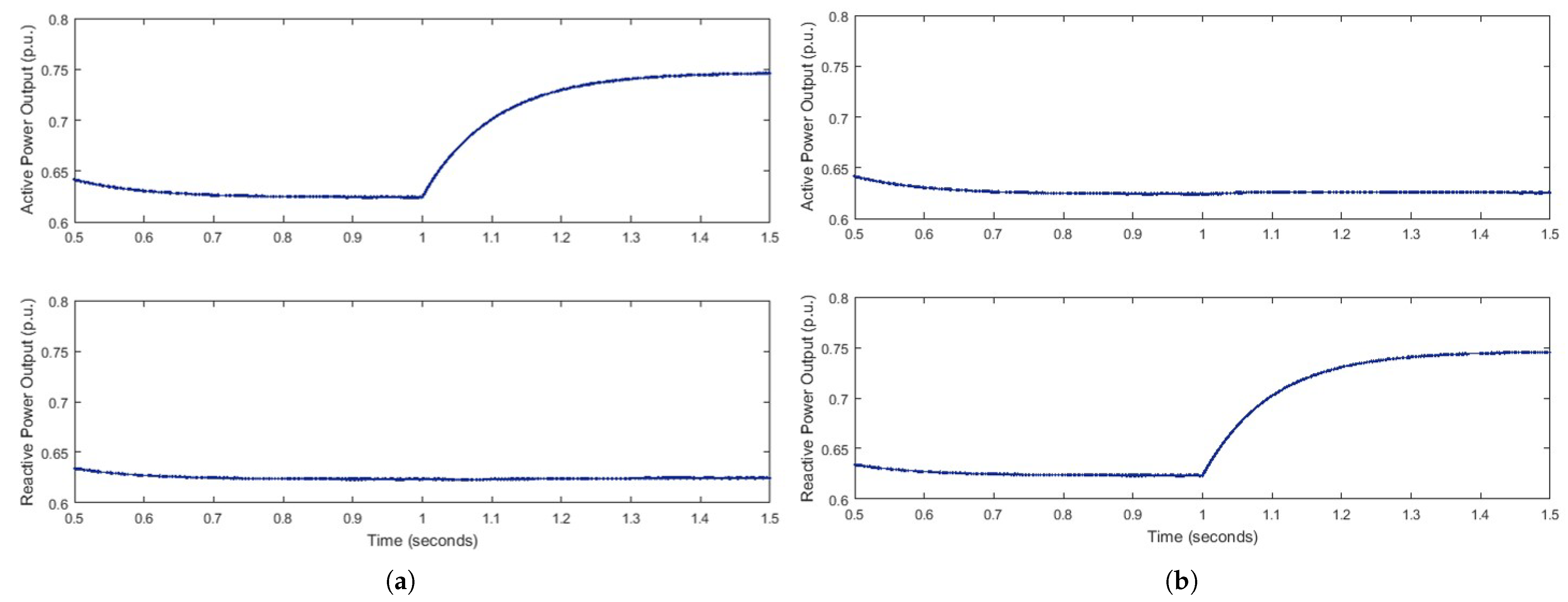
- Ray, I.; Tolbert, L.M. Small-Signal Impedance Analysis of the Impact of Grid-Forming Controllerson their DC and AC Dynamics. In Proceedings of the 2021 IEEE International Symposium on Power Electronics for Distributed Generation Systems (PEDG 2021), Chicago, IL, USA, 28 June–1 July 2021; pp. 1–8. [Google Scholar]
- Zhong, Q.; Boroyevich, D. A droop controller is intrinsically a phase-locked loop. In Proceedings of the IECON 2013—39th Annual Conference of the IEEE Industrial Electronics Society, Vienna, Austria, 10–13 November 2013; pp. 5916–5921. [Google Scholar]



| Control Method | Features |
|---|---|
| Droop Control [33] |
|
| Virtual Synchronous Generator [5] |
|
| Synchronverter [6] |
|
| Direct V-f regulation [34] |
|
| Virtual Oscillator Control [7] |
|
| Matching control [8] |
|
| Inducverter [35] |
|
| Parameter | Value |
|---|---|
| Nominal AC voltage: | 294 V (peak) |
| Nominal AC current: , | 26.7 A, 0 A |
| Nominal DC voltage: | 400 V |
| Nominal DC current: | 15 A |
| DC-link capacitor: C | 5 mF |
| L-filter inductor: | 0.575 mH |
| L-filter resistor: | 0.2 |
| Nominal frequency: | 60 Hz |
| Switching frequency: | 10 kHz |
| Current controller gains: , | 0.105, 35 |
| Voltage controller gains: , | 0.008, 40 |
| Droop coefficients: , | 0.001, 0.001 |
| Sampling period: | 100 s |
| Filter frequency: | 1500 Hz |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ray, I. Review of Impedance-Based Analysis Methods Applied to Grid-Forming Inverters in Inverter-Dominated Grids. Energies 2021, 14, 2686. https://doi.org/10.3390/en14092686
Ray I. Review of Impedance-Based Analysis Methods Applied to Grid-Forming Inverters in Inverter-Dominated Grids. Energies. 2021; 14(9):2686. https://doi.org/10.3390/en14092686
Chicago/Turabian StyleRay, Ishita. 2021. "Review of Impedance-Based Analysis Methods Applied to Grid-Forming Inverters in Inverter-Dominated Grids" Energies 14, no. 9: 2686. https://doi.org/10.3390/en14092686
APA StyleRay, I. (2021). Review of Impedance-Based Analysis Methods Applied to Grid-Forming Inverters in Inverter-Dominated Grids. Energies, 14(9), 2686. https://doi.org/10.3390/en14092686






