Axial-Flux Permanent-Magnet Generator Design for Hybrid Electric Propulsion Drone Applications
Abstract
:1. Introduction
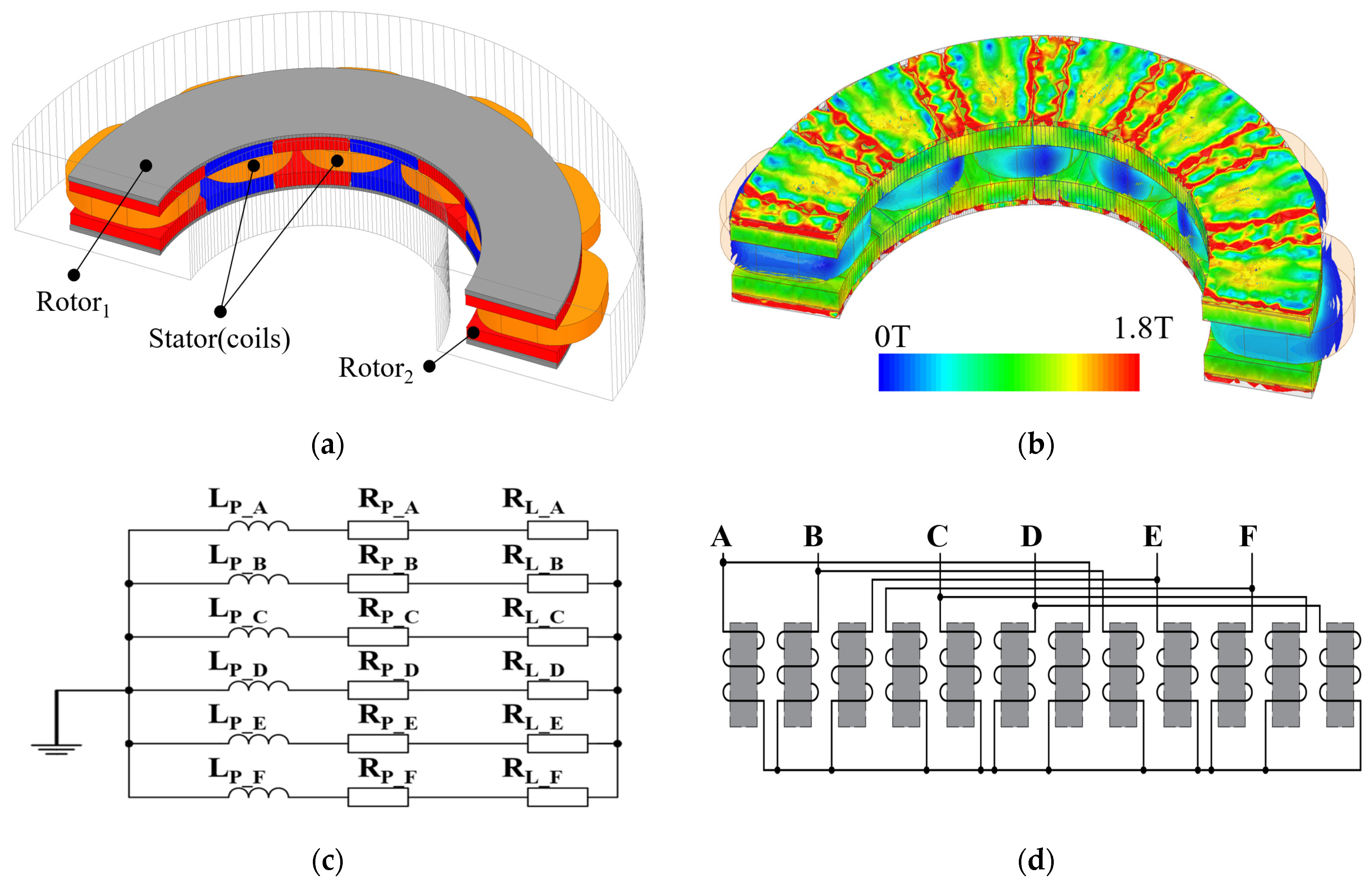
2. AFPM Generator Design
2.1. Design Specifications
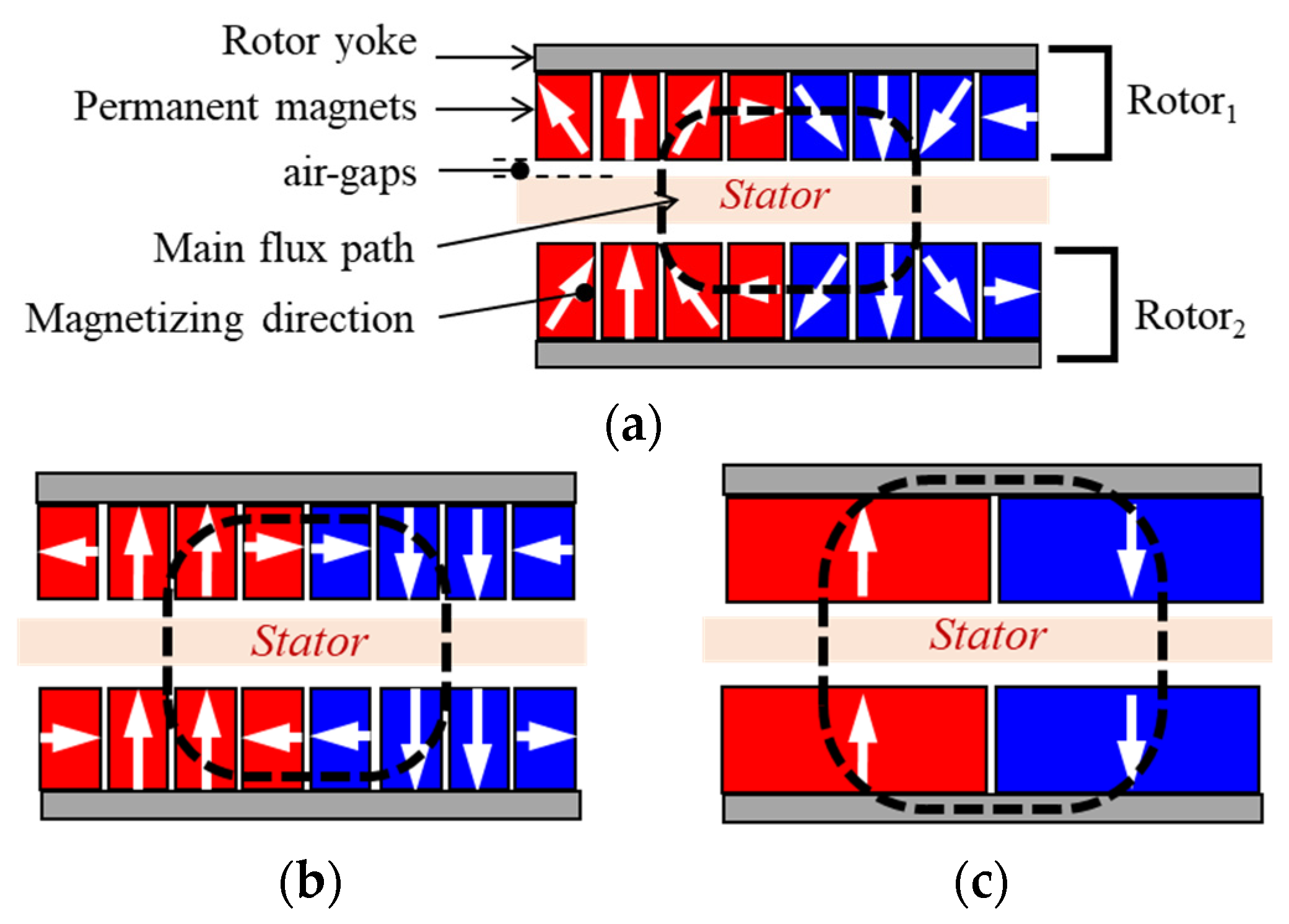
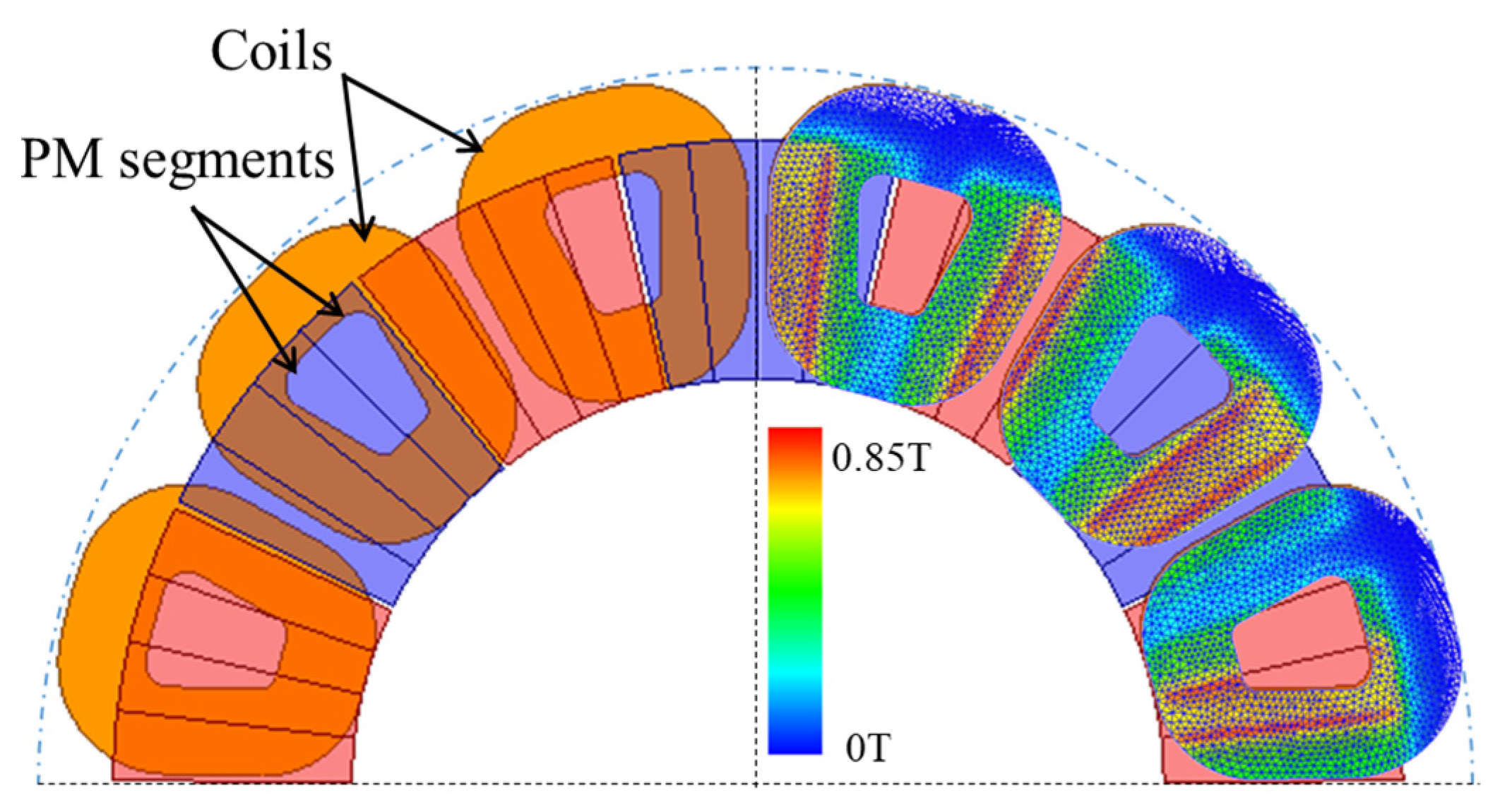
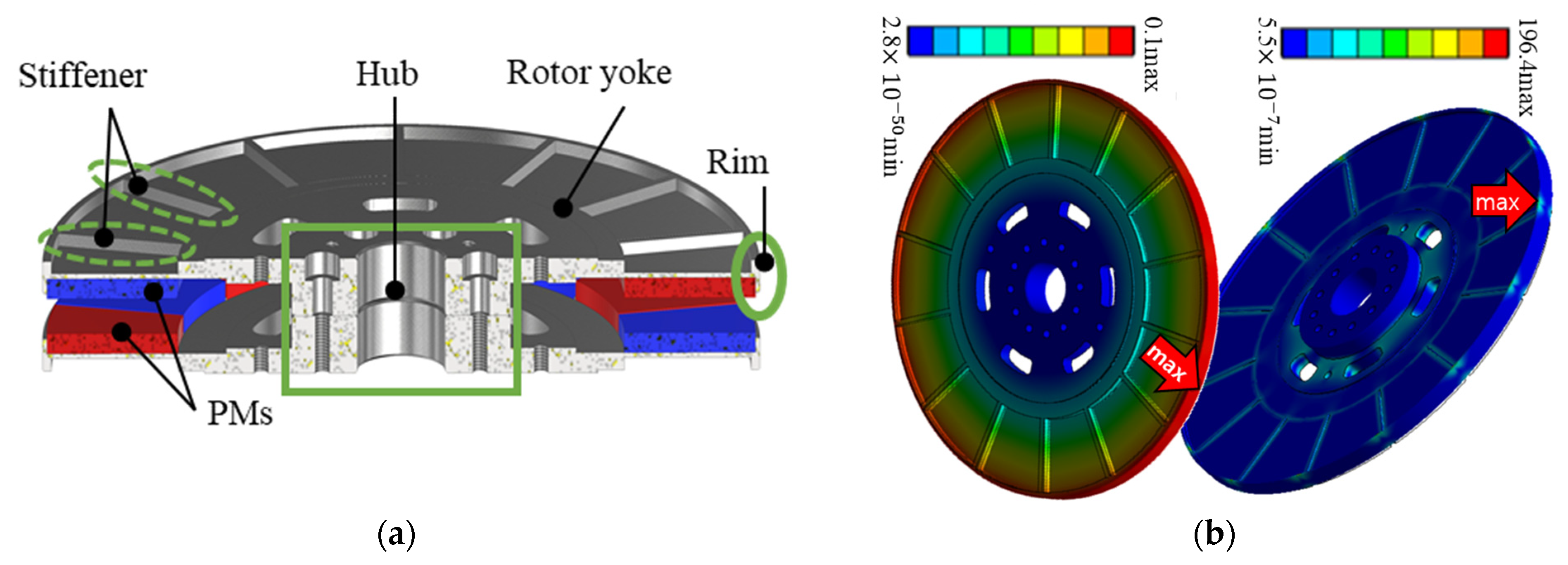
2.2. Rotor Design of the AFPM Generator
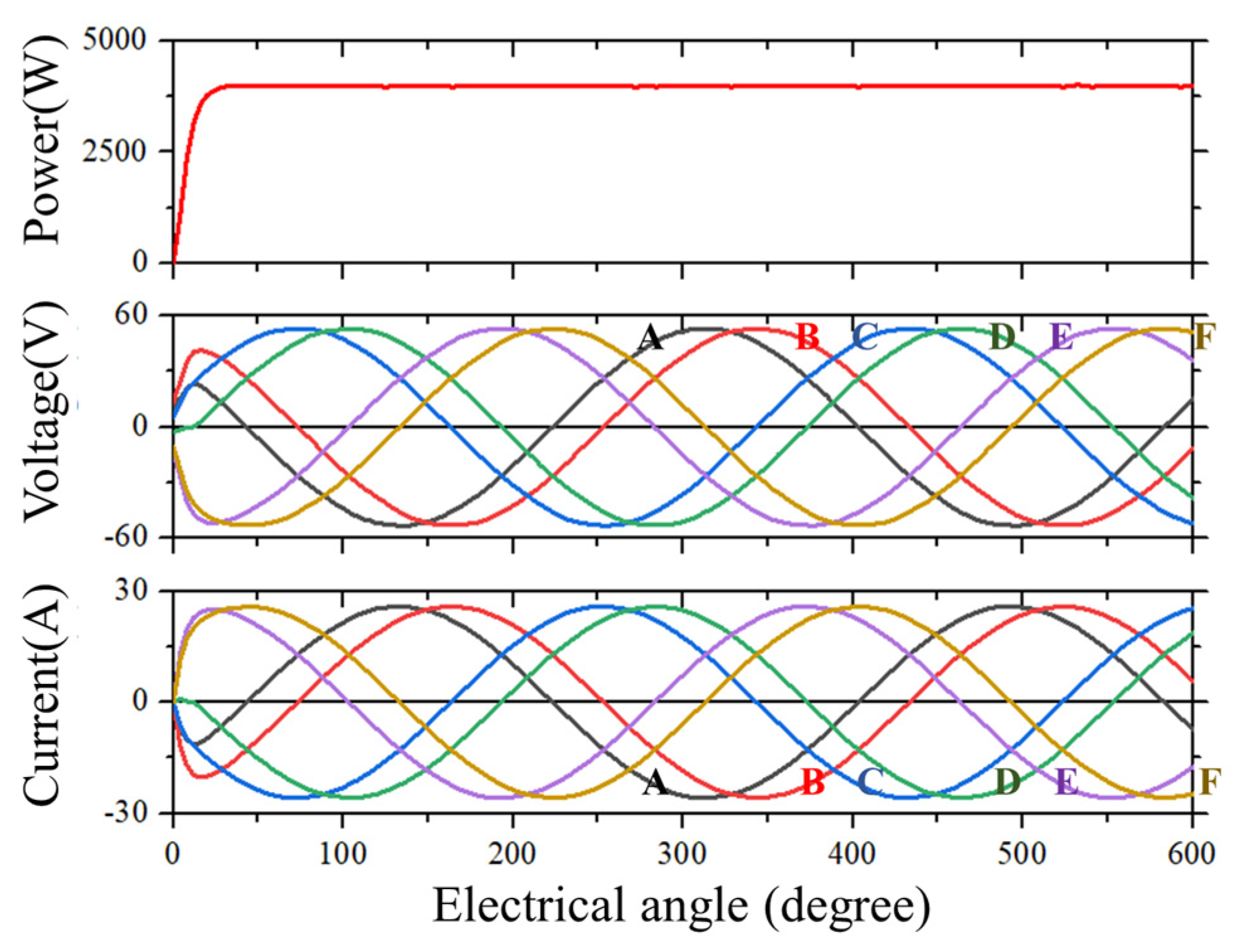
2.3. Stator Design of the AFPM Generator
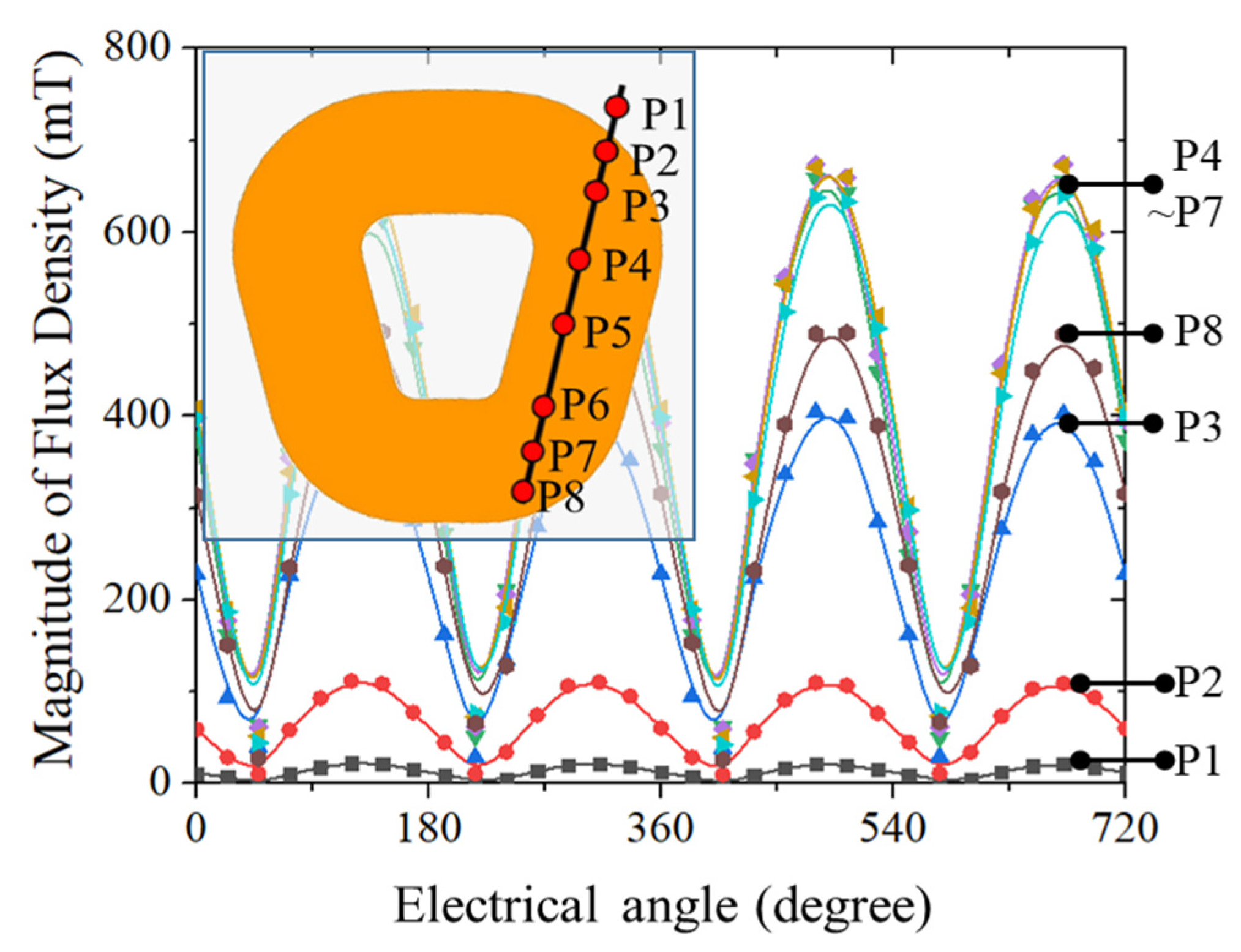
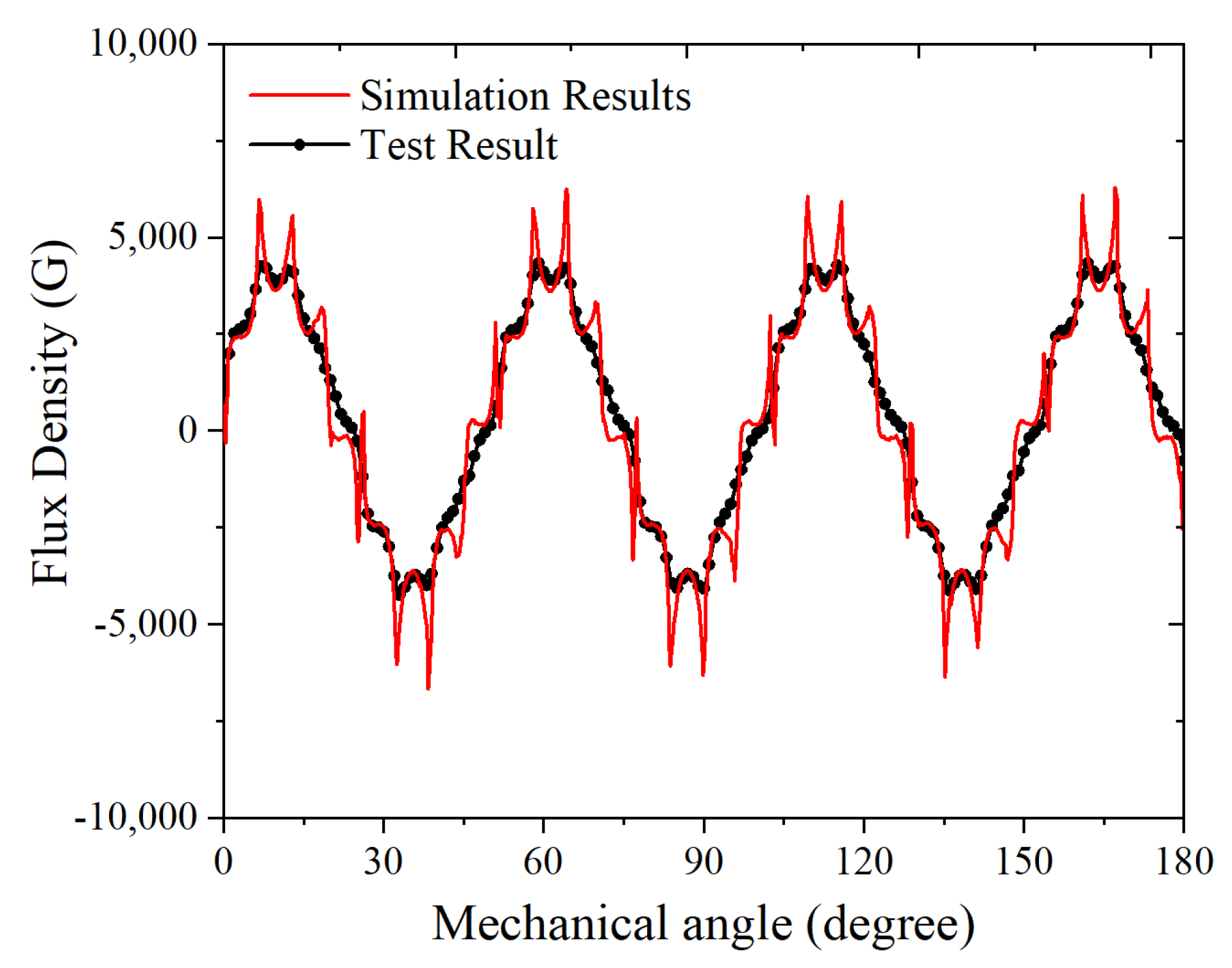
- The change of magnetic flux density over time is sine, and the harmonic component is negligible;
- Since the change in magnetic flux density from point P4 to point P7, which is the straight side of the coil, is similar, it can be represented by one value. The magnetic flux density variation between the layers of the coil is negligible;
- The magnetization directions of the rotor permanent magnets facing each other are opposite to each other as shown in Figure 3a, and the axial length of the coil is short. Therefore, it can be seen that the magnetic flux passing between points P4 to P7 of the coil has only the axial component.
3. Mechanical and Thermal Analysis
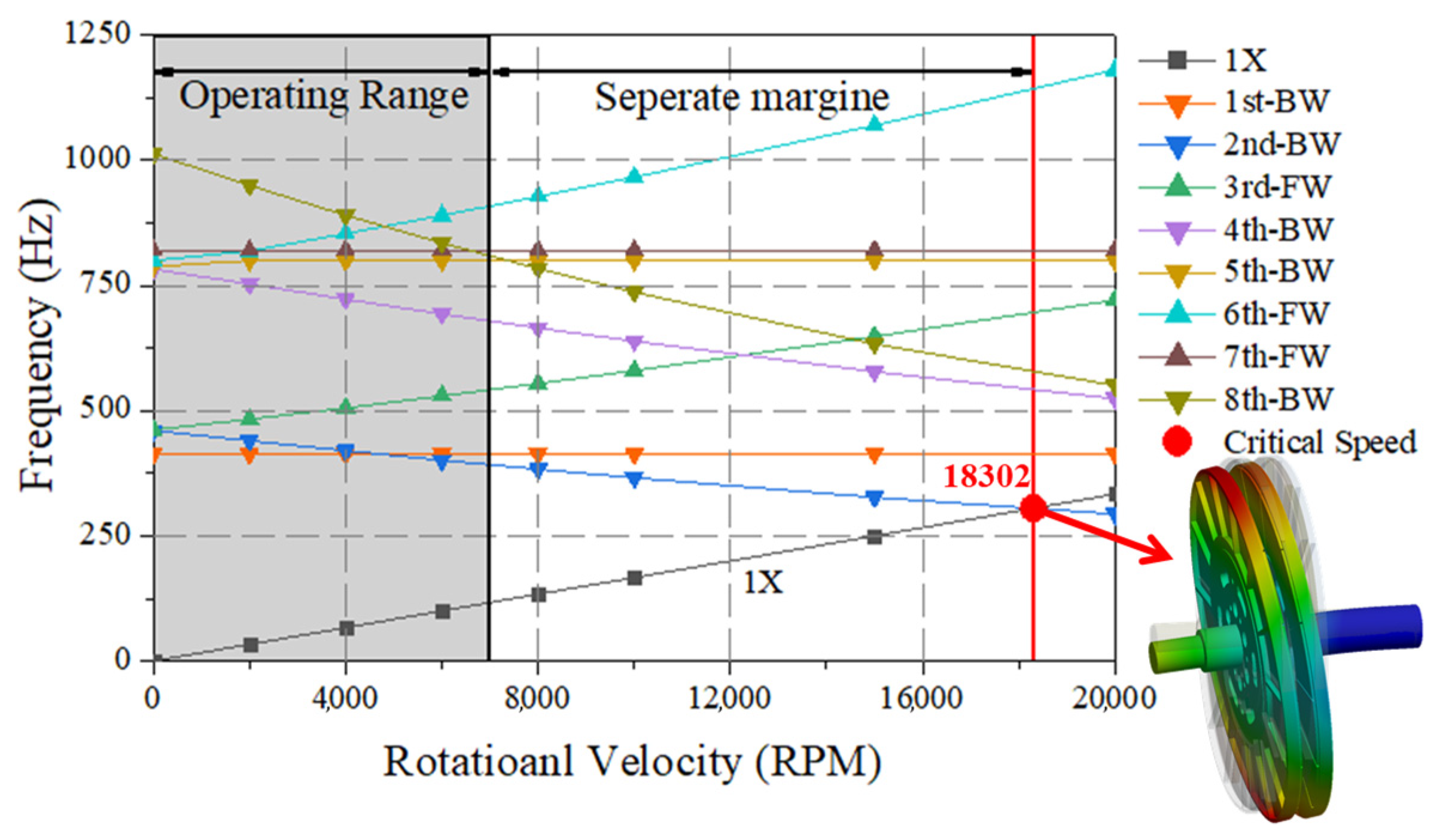
3.1. Mechanical Analysis
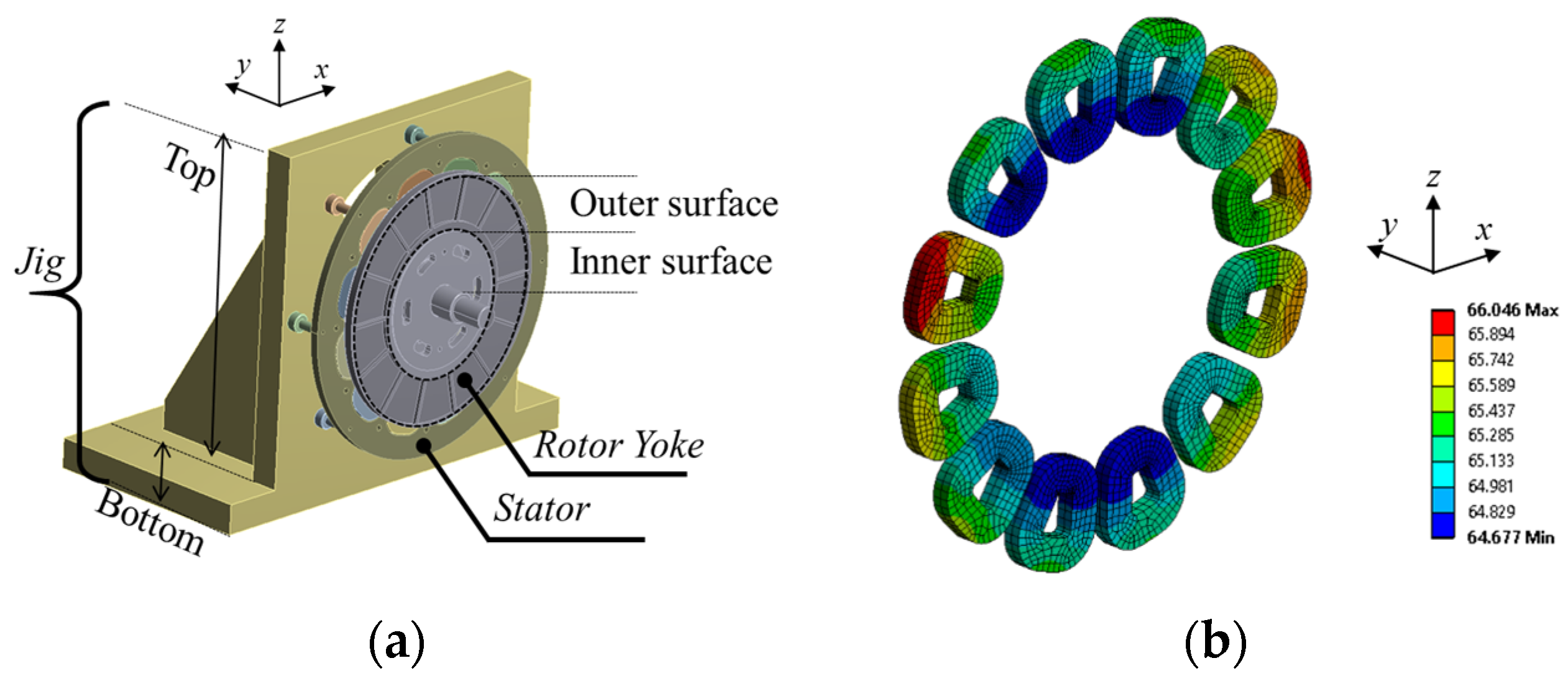
3.2. Thermal Analysis
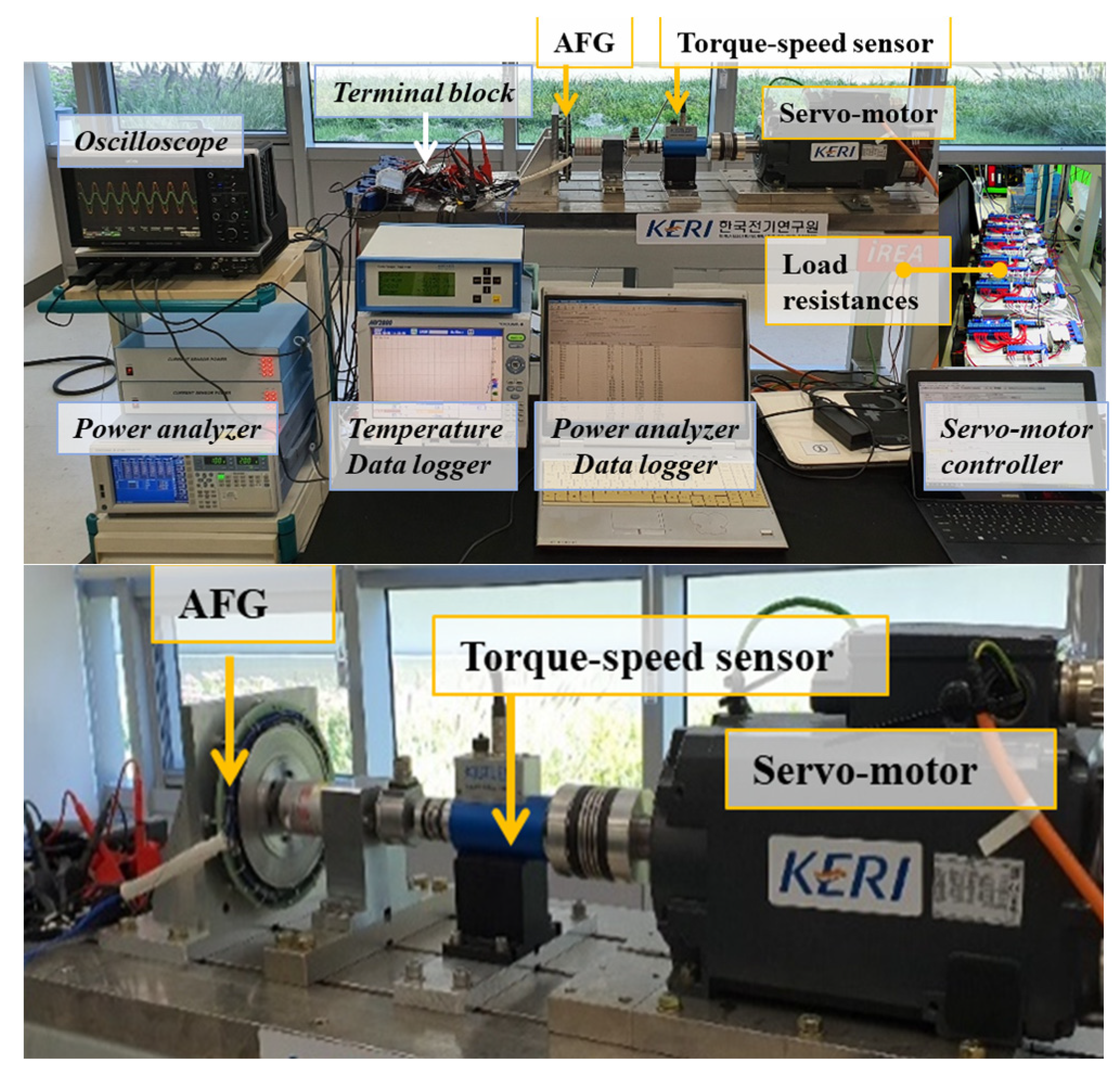
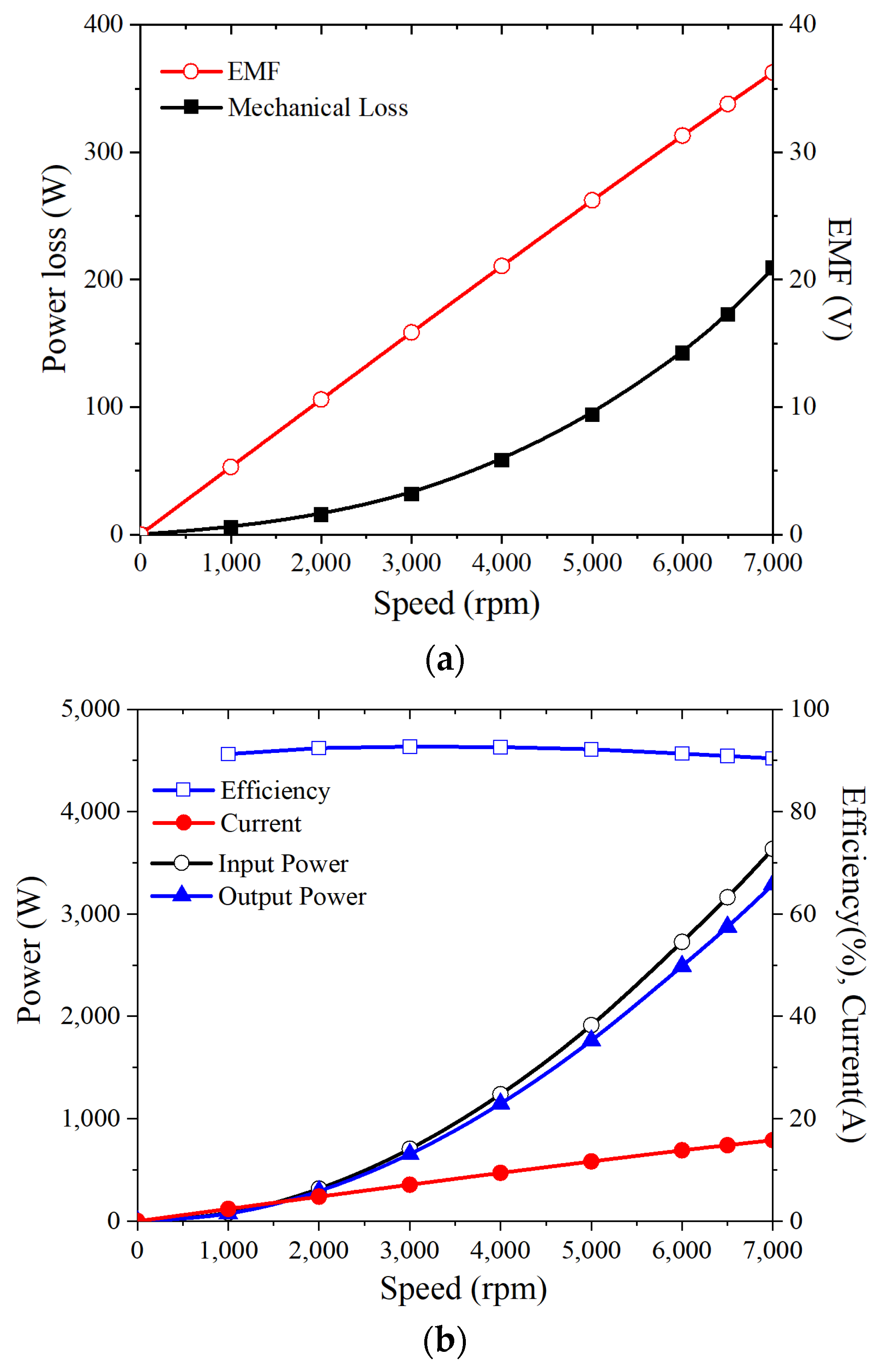
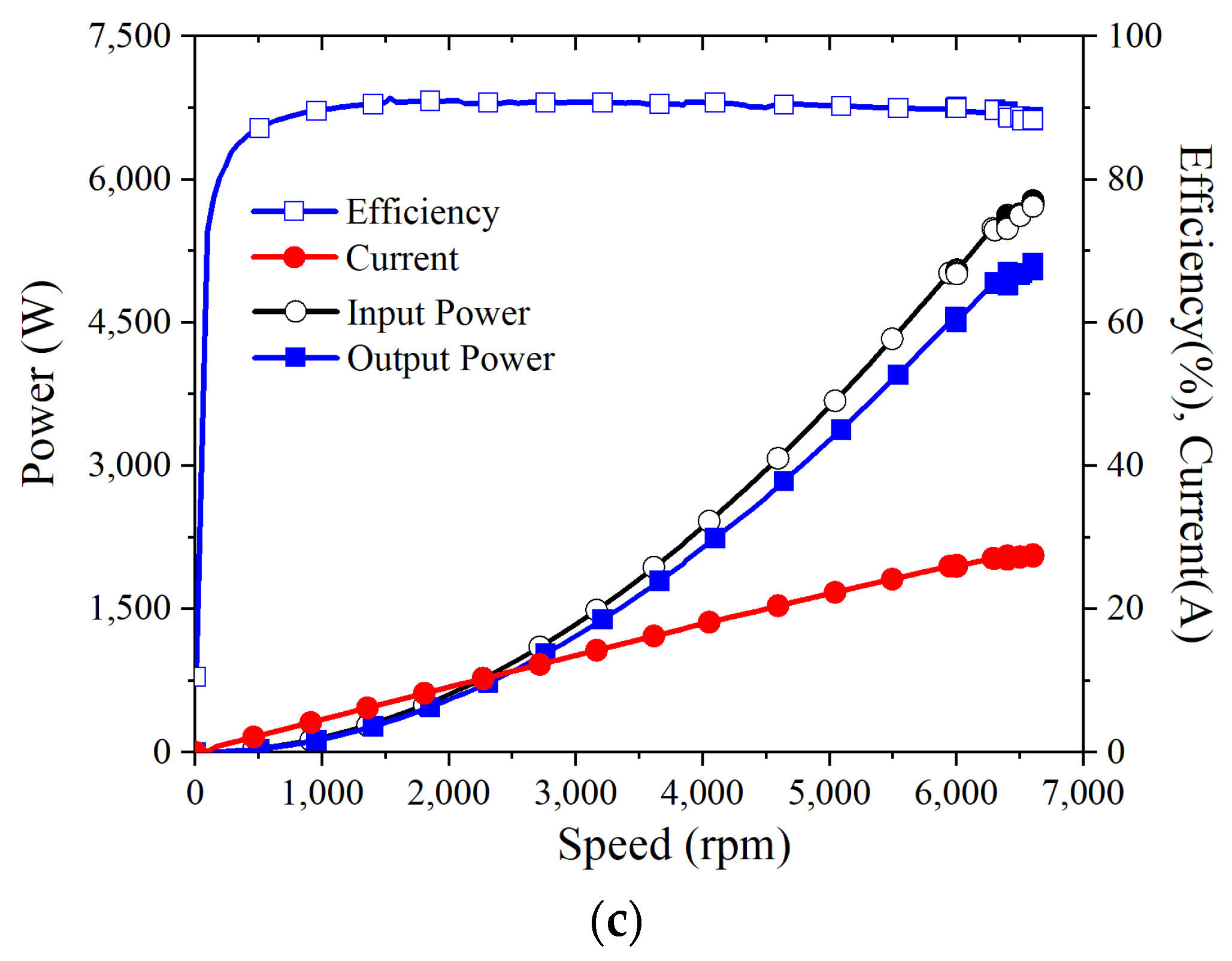
4. Experimental Validation and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Lee, J.Y.; Koo, D.H.; Moon, S.R.; Han, C.K. Design of an Axial Flux Permanent Magnet Generator for a Portable Hand Crank Generating System. IEEE Trans. Magn. 2012, 48, 2977–2980. [Google Scholar] [CrossRef]
- Di Gerlando, A.; Foglia, G.M.; Iacchetti, M.F.; Perini, R. Axial Flux PM Machines with Concentrated Armature Windings: Design Analysis and Test Validation of Wind Energy Generators. IEEE Trans. Ind. Electron. 2010, 58, 3795–3805. [Google Scholar] [CrossRef]
- Arkadan, A.A.; Hijazi, T.M.; Masri, B. Design Evaluation of Conventional and Toothless Stator Wind Power Axial-Flux PM Generator. IEEE Trans. Magn. 2017, 53, 1–4. [Google Scholar] [CrossRef]
- Lee, J.Y.; Park, B.G.; Koo, D.H. Analysis of Mechanical Fixation Made of Aluminum Alloy in an Axial Flux Permanent Magnet Machine. J. Magn. 2014, 19, 309–313. [Google Scholar] [CrossRef]
- Vun, S.T.; McCulloch, M.D.; Leong, C.Y. The development of an electromagnetic analytical design tool for mega-watt-scale YASA generators. In Proceedings of the IET Conference on Renewable Power Generation (RPG 2011), Edinburgh, UK, 6–8 September 2011; pp. 1–6. [Google Scholar]
- Woolmer, T.J.; McCulloch, M.D. Analysis of the Yokeless And Segmented Armature Machine. In Proceedings of the 2007 IEEE Internation-al Electric Machines & Drives Conference, Antalya, Turkey, 3–5 May 2007; pp. 704–708. [Google Scholar]
- Aydin, M.; Huang, S.; Lipo, T.A. Axial Flux Permanent Magnet Disc Machine: A Review. In Proceedings of the 2004 Conference Record of SPEEDAM; 2004; pp. 61–71. Available online: https://www.researchgate.net/publication/228449891_Axial_flux_permanent_magnet_disc_machines_A_review (accessed on 3 December 2021).
- Park, Y.; Kim, H.; Jang, H.; Ham, S.-H.; Lee, J.; Jung, D.-H. Efficiency Improvement of Permanent Magnet BLDC With Halbach Magnet Array for Drone. IEEE Trans. Appl. Supercond. 2020, 30, 1–5. [Google Scholar] [CrossRef]
- Wiltuschnig, I.P.; Eckert, P.R.; Dorrell, D.; Filho, A.F.F. A Study of the Influence of Quasi-Halbach Arrays on a Torus Machine. IEEE Trans. Magn. 2016, 52, 1–4. [Google Scholar] [CrossRef]
- Hu, Y.; Zhu, Z.; Liu, K. Current Control for Dual Three-Phase Permanent Magnet Synchronous Motors Accounting for Current Unbalance and Harmonics. IEEE J. Emerg. Sel. Top. Power Electron. 2014, 2, 272–284. [Google Scholar]
- Barcaro, M.; Bianchi, N.; Magnussen, F. Analysis and Tests of a Dual Three-Phase 12-Slot 10-Pole Permanent-Magnet Motor. IEEE Trans. Ind. Appl. 2010, 46, 2355–2362. [Google Scholar] [CrossRef]
- Wang, R.; Kamper, M.J. Calculaton of Eddy Current Loss in Axial Field Permanent-magnet Machine with Coreless Stator. IEEE Trans. Energy Conv. 2004, 19, 532–538. [Google Scholar] [CrossRef]
- Mahmoudi, A.; Rahim, N.A.; Hew, W.P. Axial-flux permanent-magnet machine modeling, design, simulation and anal-ysis. Sci. Res. Essays 2011, 6, 2525–2549. [Google Scholar]
- Parviainen, A. Design of Axial-Flux Permanent-Magnet Low-Speed Machines and Performance Comparison between Radi-al-Flux and Axial-Flux Machines. Ph.D. Thesis, Lappeenranta University, Lappeenranta, Finland, April 2005. [Google Scholar]
- Hikmawan, M.; Kasim, M.; Irasari, P.; Widiyanto, P. Analysis of magnet shape influence on rotor deflection on axial flux permanent magnet generator. In Proceedings of the 2017 International Conference on Sustainable Energy Engineering and Application (ICSEEA), Jakarta, Indonesia, 23–24 October 2017; pp. 91–97. [Google Scholar]
- Zhang, B.; Seidler, T.; Dierken, R.; Doppelbauer, M. Development of a Yokeless and Segmented Armature Axial Flux Machine. IEEE Trans. Ind. Electron. 2015, 63, 2062–2071. [Google Scholar] [CrossRef]
- Luu, P.T.; Lee, J.-Y.; Lee, J.-H.; Park, J.-W. Electromagnetic and Thermal Analysis of Permanent-Magnet Synchronous Motors for Cooperative Robot Applications. IEEE Trans. Magn. 2020, 56, 1–4. [Google Scholar] [CrossRef]
- Luu, P.T.; Lee, J.Y.; Lee, J.H.; Park, J.W. Electromagnetic and Thermal Analysis of a Permanent Magnet Motor Consid-ering the Effect of Articulated Robot Link. Energies 2020, 13, 3239. [Google Scholar] [CrossRef]
- Lee, J.Y.; Luu, P.T. Electric Motor Design of an Integrated Motor Propulsor for Unmanned Vehicles: The Effect of Wa-terproofing Can. Energies 2020, 13, 2227. [Google Scholar] [CrossRef]
- Lee, J.; Kim, J.; Moon, S.; Chang, J.; Chung, S.; Kang, D.; Hong, J. Dynamic Characteristic Analysis Considering Core Losses in Transverse Flux Linear Machine With Solid Cores. IEEE Trans. Magn. 2009, 45, 1776–1779. [Google Scholar]
- Jang, S.; Cho, H.; Choi, S. Design and Analysis of a High-Speed Brushless DC Motor for Centrifugal Compressor. IEEE Trans. Magn. 2007, 43, 2573–2575. [Google Scholar] [CrossRef]
- Roshen, W.A. A Practical, Accurate and Very General Core Loss Model for Nonsinusoidal Waveforms. IEEE Trans. Power Electron. 2007, 22, 30–40. [Google Scholar] [CrossRef]
- Huang, Y.; Zhu, J.; Guo, Y. Thermal Analysis of High-Speed SMC Motor Based on Thermal Network and 3-D FEA With Rotational Core Loss Included. IEEE Trans. Magn. 2009, 45, 4680–4683. [Google Scholar] [CrossRef]
- Qi, J.; Hua, W.; Zhang, H. Thermal Analysis of Modular-Spoke-Type Permanent-Magnet Machines Based on Thermal Network and FEA Method. IEEE Trans. Magn. 2019, 55, 1–5. [Google Scholar] [CrossRef]
- Srinivas, K.N.; Arumugam, R. Analysis and characterization of switched reluctance motors: Part II. Flow, thermal, and vibration analyses. IEEE Trans. Magn. 2005, 41, 1321–1332. [Google Scholar] [CrossRef]
- Huang, Y.; Zhu, J.; Guo, Y.; Lin, Z.; Hu, Q. Design and Analysis of a High-Speed Claw Pole Motor With Soft Magnetic Composite Core. IEEE Trans. Magn. 2007, 43, 2492–2494. [Google Scholar] [CrossRef] [Green Version]


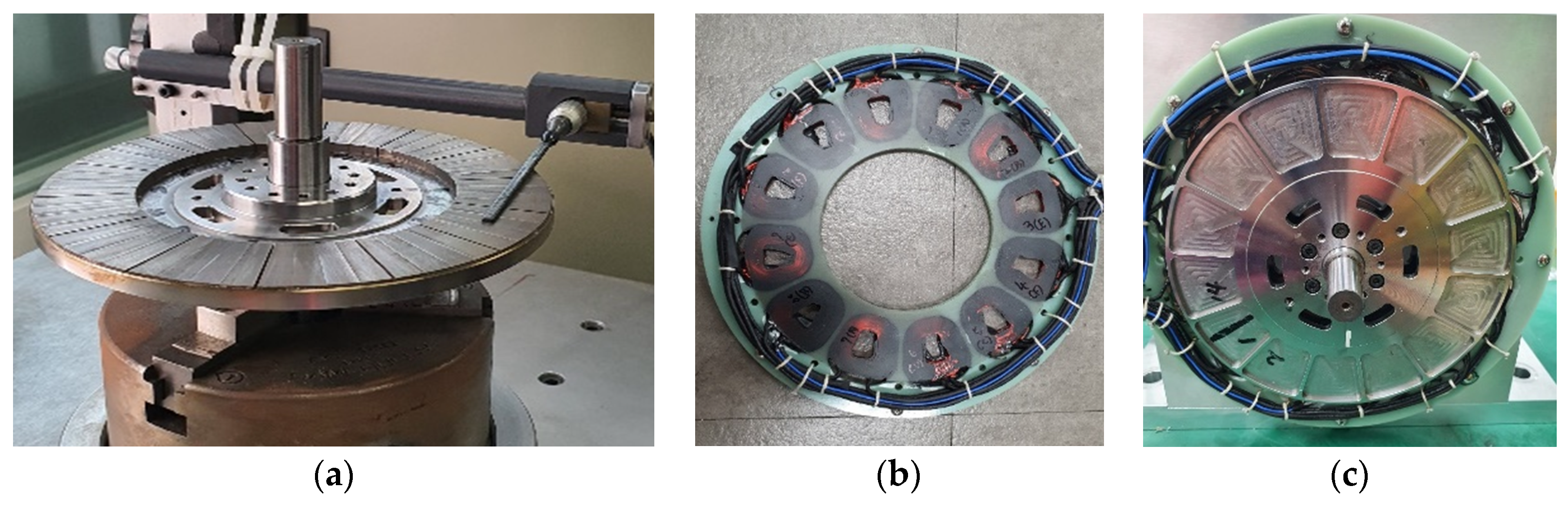

| Parameter | Value | Unit |
|---|---|---|
| Max speed | ≤7000 | rpm |
| Output power | ≥3000 | W |
| No-load voltage constant | ≤11 | mV/rpm |
| Efficiency | ≥93 | % |
| Power density | ≤2.3 | W/kg |
| Cooling condition | Natural cooling |
| Parameter | Value | Unit |
|---|---|---|
| No. of phases | 6 | |
| No. of poles/slots | 14 poles/12 slots | |
| Outer diameter of rotor | 162 | mm |
| Air-gap length (one-side) | 0.5 | mm |
| Total axial thickness | 17 | mm |
| PM material | NdFeB(Br = 1.3T) |
| Parameter | Type-I | Type-II | NA |
|---|---|---|---|
| Rated output power (W) | 3982.4 (139.6%) | 3583.9 (125.6%) | 2853.6 (100%) |
| Phase voltage (V) | 37.4 (138.5%) | 33.7 (124.8%) | 27 (100%) |
| Core loss (W) | 1.5 | 1.3 | 3.7 |
| Copper loss (W) | 112.0 | 89.8 | 57.8 |
| Efficiency (%) | 97.2 | 97.5 | 97.9 |
| Weight (kg) | 1.34 | 1.34 | 1.34 |
| Power density (W/kg) | 2.97 (139.6%) | 2.67 (125.6%) | 2.13 (100%) |
| Rated speed (rpm) | 6500 | 6500 | 6500 |
| Phase current (A) | 18.1 | 16.2 | 13 |
| Current density (A/mm2) | 15.9 | 14.3 | 11.5 |
| No. of parallel circuits | 2 | 2 | 2 |
| No. of turns per coil | 42 | 42 | 42 |
| No. of strands of wire | 70 | 70 | 70 |
| Conductor diameter (mm) | 0.1 | 0.1 | 0.1 |
| Rotor avg. flux density (T) | 1.3 | 1.2 | 1.4 |
| Rotor max. flux density (T) | 2.3 | 2.3 | 2.8 |
| Parameter | Coil-I | Coil-II | Coil-III |
|---|---|---|---|
| Rotating speed (rpm) | 5000 | 5000 | 5000 |
| No. of parallel circuits | 2 | 1 | 1 |
| No. of turns per coil | 72 | 36 | 36 |
| No. of serial turns per phase | 72 | 72 | 36 |
| No. of strands | 1 | 1 | 70 |
| Conductor diameter (mm) | 0.85 | 1.2 | 0.1 |
| Slot fill factor (%) | 62 | 62 | 31 |
| Phase resistance (Ohm) | 0.084 | 0.081 | 0.04 |
| Calculated eddy-current loss power of coils (W) | 110.6 (100%) | 219.7 (199%) | 0.74 (0.7%) |
| Measured loss power of each prototype (W) | 122 (100%) | 242 (198%) | 39 (32%) |
| Max temperature (°C) | 72.6 | 103.7 | 31.3 |
| Parameter | Value |
|---|---|
| Output power (W) | 3000 |
| Speed (rpm) | 7000 |
| Current (A) | 15.5 |
| Copper loss (W) | 96.6 |
| Core loss (W) | 1.5 |
| Mechanical loss (W) | 200 |
| Room temperature (°C) | 28 |
| Convection coefficient on rotor yoke (inner/outer) (W/m2K) | 100/200 |
| Convection coefficient on jig (W/m2K) | 10/Infinite |
| Parameter | Value |
|---|---|
| Coil temperature range (°C) | 65–66 |
| Rotor yoke temperature range (°C) | 39–51 |
| PM temperature range (°C) | 42–47 |
| Bearing temperature range (°C) | 56–67 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lee, J.-Y.; Lee, J.-H.; Nguyen, T.K. Axial-Flux Permanent-Magnet Generator Design for Hybrid Electric Propulsion Drone Applications. Energies 2021, 14, 8509. https://doi.org/10.3390/en14248509
Lee J-Y, Lee J-H, Nguyen TK. Axial-Flux Permanent-Magnet Generator Design for Hybrid Electric Propulsion Drone Applications. Energies. 2021; 14(24):8509. https://doi.org/10.3390/en14248509
Chicago/Turabian StyleLee, Ji-Young, Ji-Heon Lee, and Tung Khanh Nguyen. 2021. "Axial-Flux Permanent-Magnet Generator Design for Hybrid Electric Propulsion Drone Applications" Energies 14, no. 24: 8509. https://doi.org/10.3390/en14248509
APA StyleLee, J.-Y., Lee, J.-H., & Nguyen, T. K. (2021). Axial-Flux Permanent-Magnet Generator Design for Hybrid Electric Propulsion Drone Applications. Energies, 14(24), 8509. https://doi.org/10.3390/en14248509







