A Method for Determining the Impact of Ambient Temperature on an Electrical Cable during a Fire
Abstract
1. Introduction
2. Research Assumptions
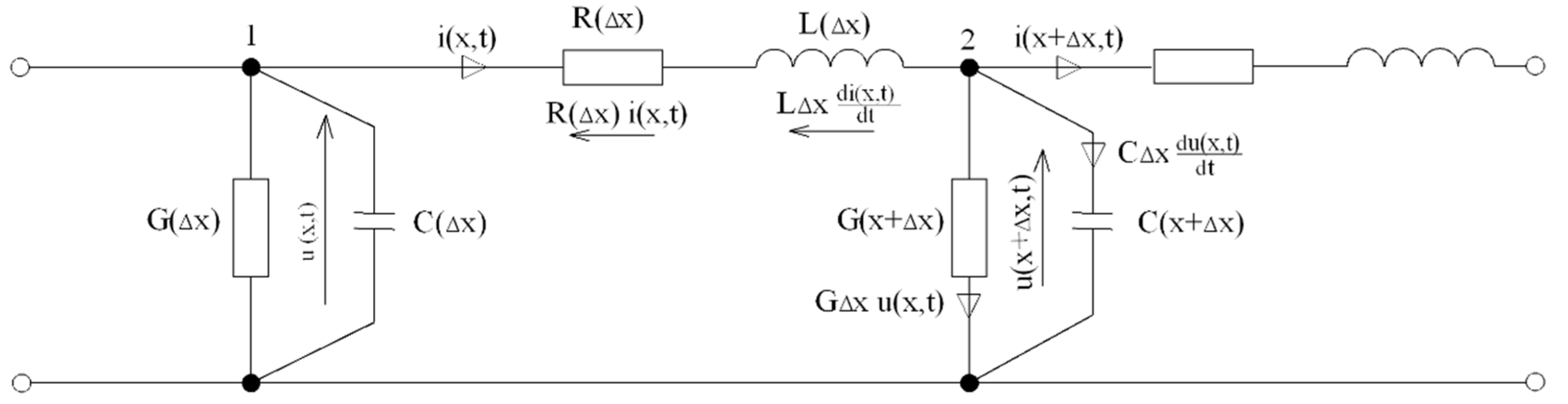
- The use of distributed parameters in the model makes it possible to determine the temperature of the electric wire in each of its geometrical points;
- Thermo-electric analogies enable the thermal state of an electric cable to be described using the parameters of electric circuits known in electrical engineering;
- The development of a heat flow model in an electric cable forced by external environmental conditions will enable the cable temperature to be estimated, which has a significant impact on the line structure and the operating parameters of electric cables;
- The length l and the cross-section s of the cable are known;
- The electric cable is made of conductive copper, e.g., CU-ETP CW004A;
- The cable is homogeneous in material along its entire length (there are no connectors, bridges, etc.);
- The thermal conductivity coefficient k is the material constant of the conductor and does not depend on the heating time t, the temperature of the conductor T, and the geometric dimensions, including the length l;
- The cable cross-section s is in the shape of a circle and is constant along its entire length;
- Inside the conductor, the thermal properties in all directions are the same (no crystal lattice dislocation);
- The internal structure of the conductor does not contain any inclusions or impurities with other elements (no material defects);
- The heating process is slow (thermal conditions of the fire), e.g., the temperature rise time is 120 min;
- The temperature inside the heated area is the same throughout the area;
- A single electric wire is considered;
- During heating, the cable does not conduct electricity, and the power supply will be switched on during an existing fire;
- At the time of measurement, the cable is assumed to be in the prescribed thermal conditions.
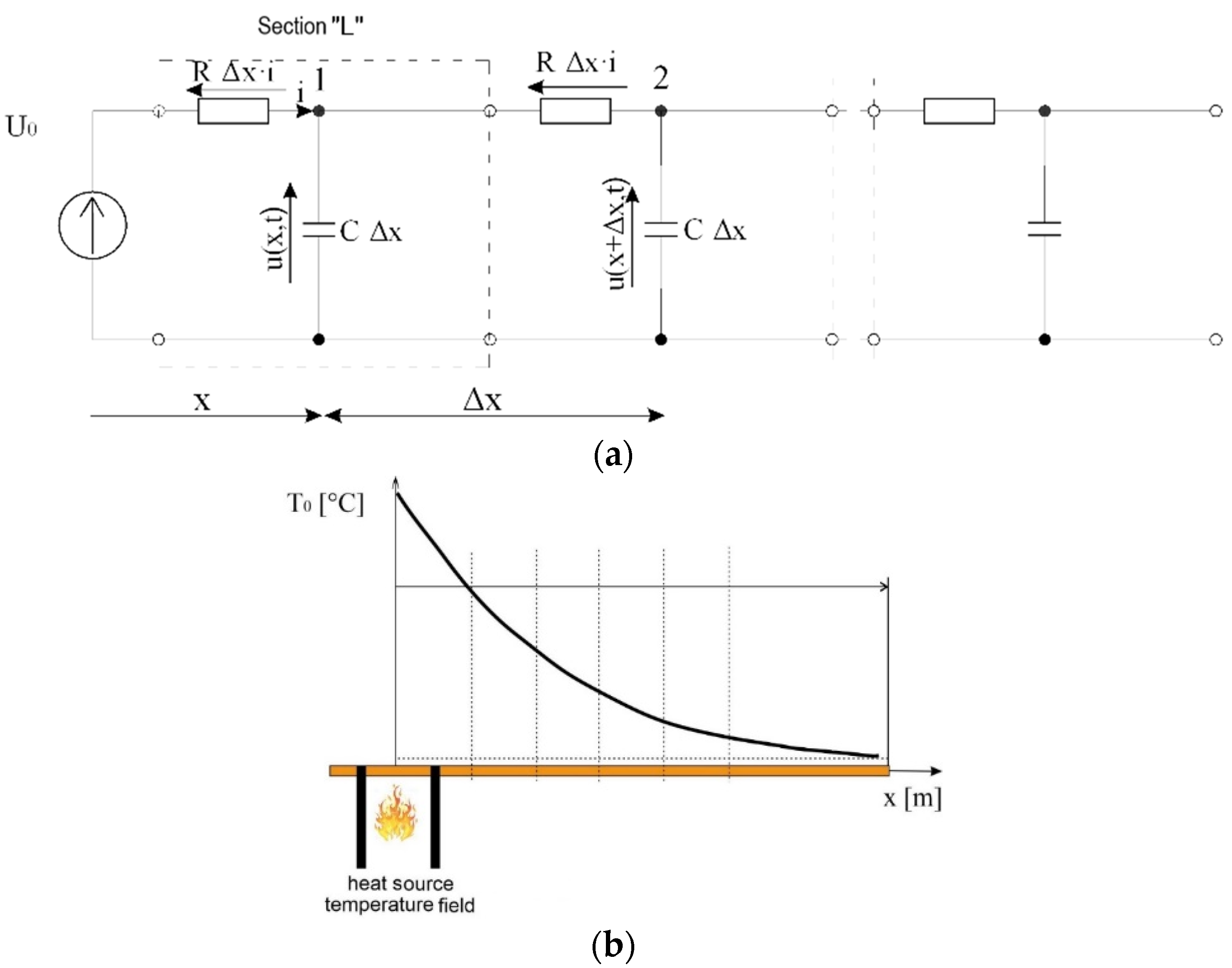
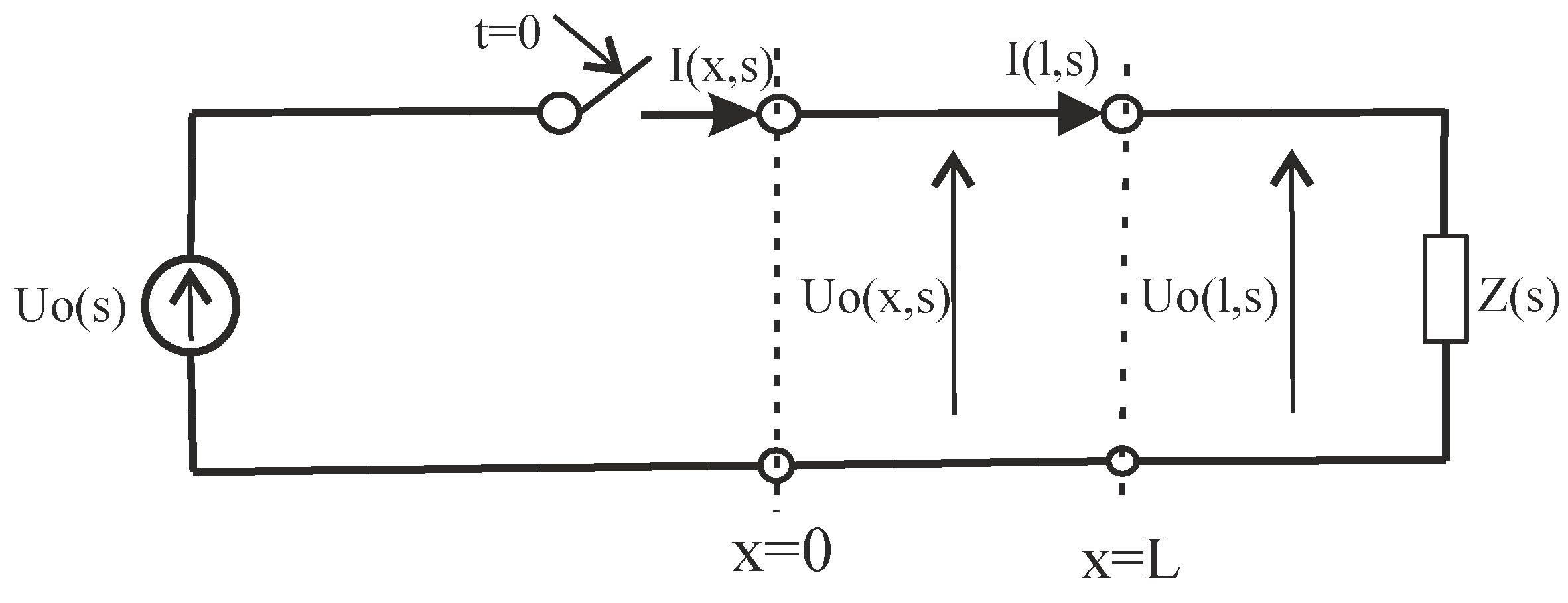
3. Modelling the Heating Up of Electrical Cables
- (a)
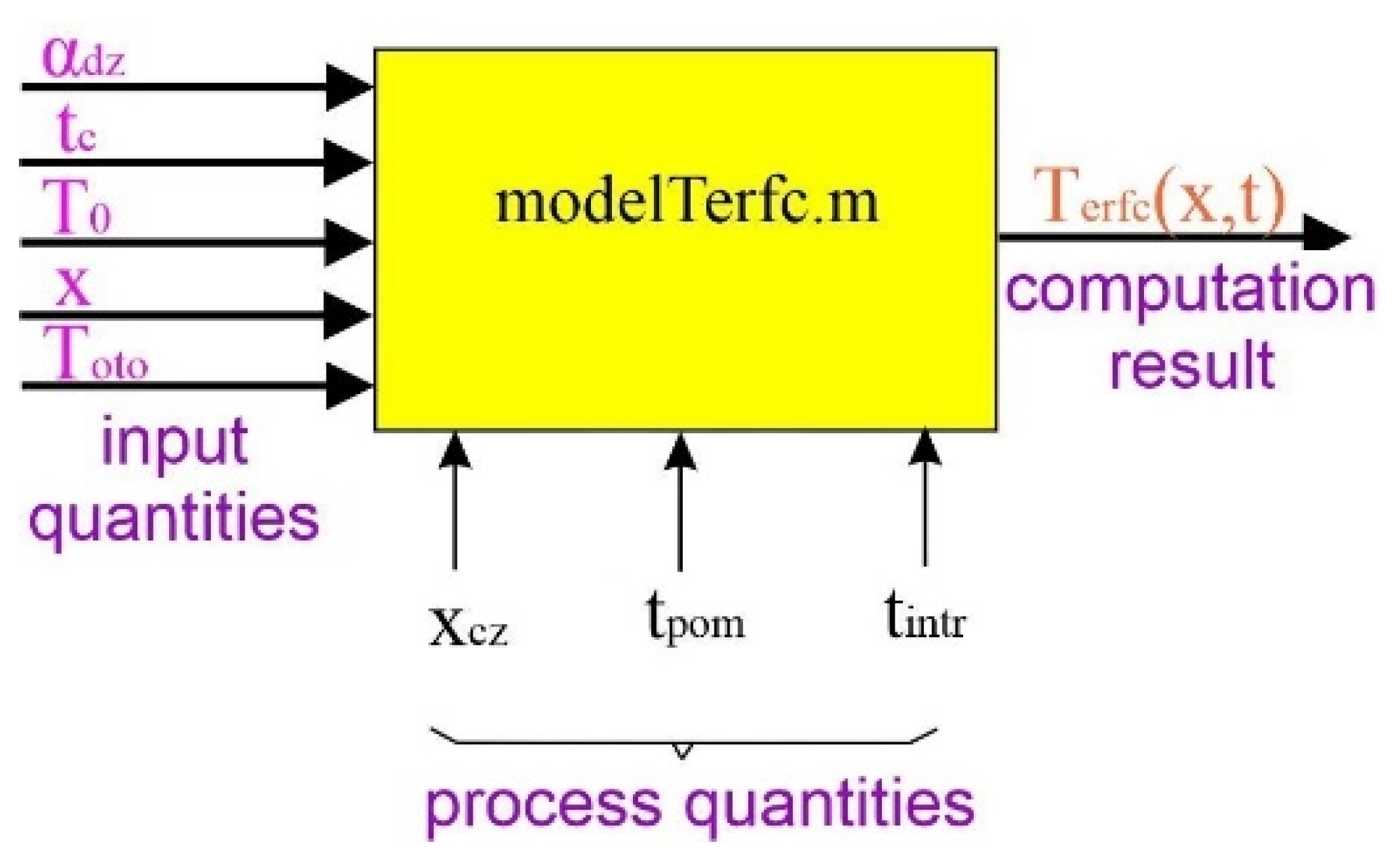
- Equivalent cable heat diffusion coefficient αdz;
- (b)
- Impact time of an external temperature source tc;
- (c)
- Distance x from the heat source, calculated along the cable longitudinal axis;
- (d)
- Cable ambient temperature Toto;
- (e)
- Impact temperature of an external temperature field T0.
- (a)
- Distance between measuring sensors xcz;
- (b)
- Total experiment time tpom;
- (c)
- Time step interval value tintr.
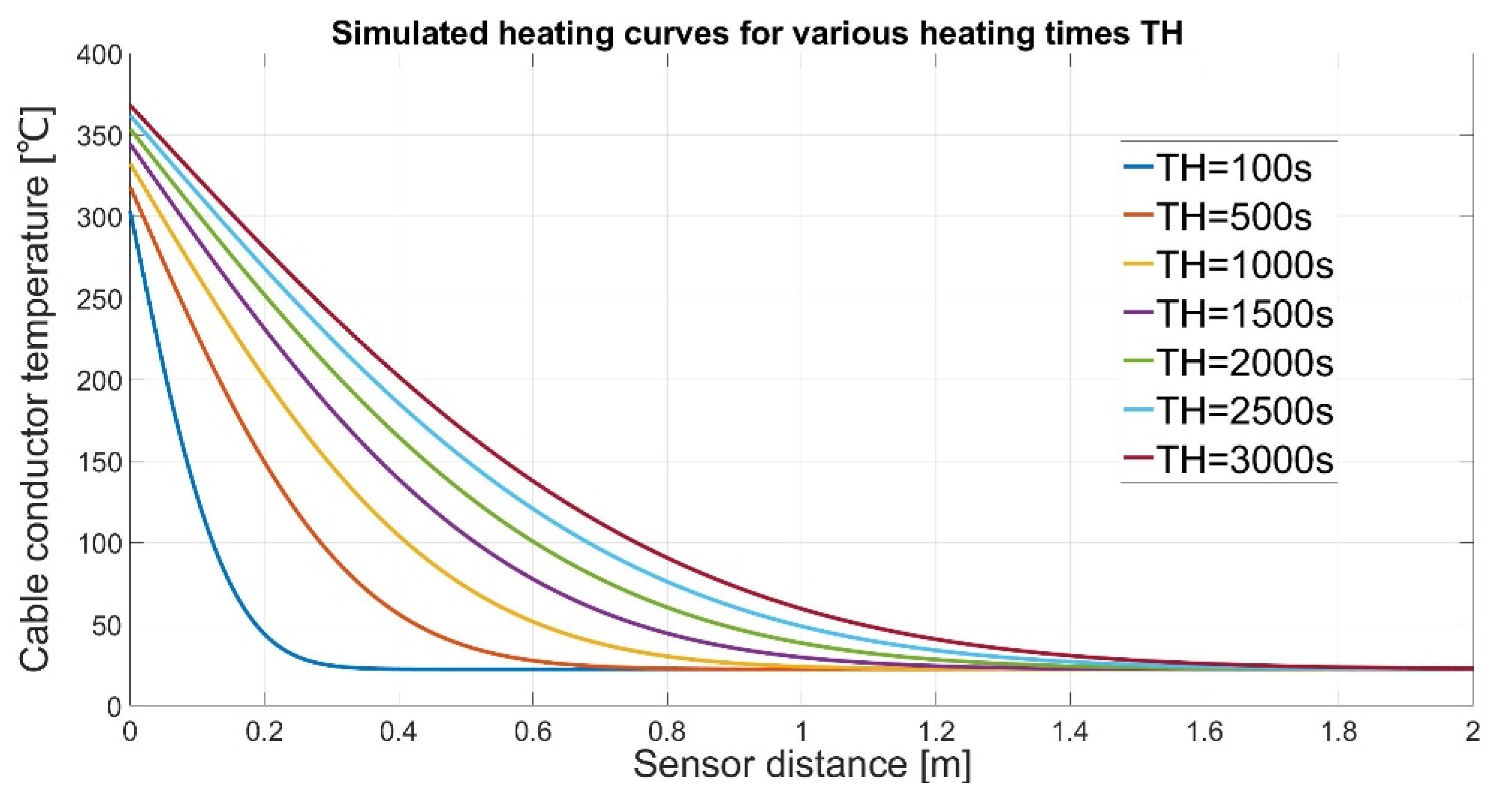
4. Determining Heating Temperature Curves
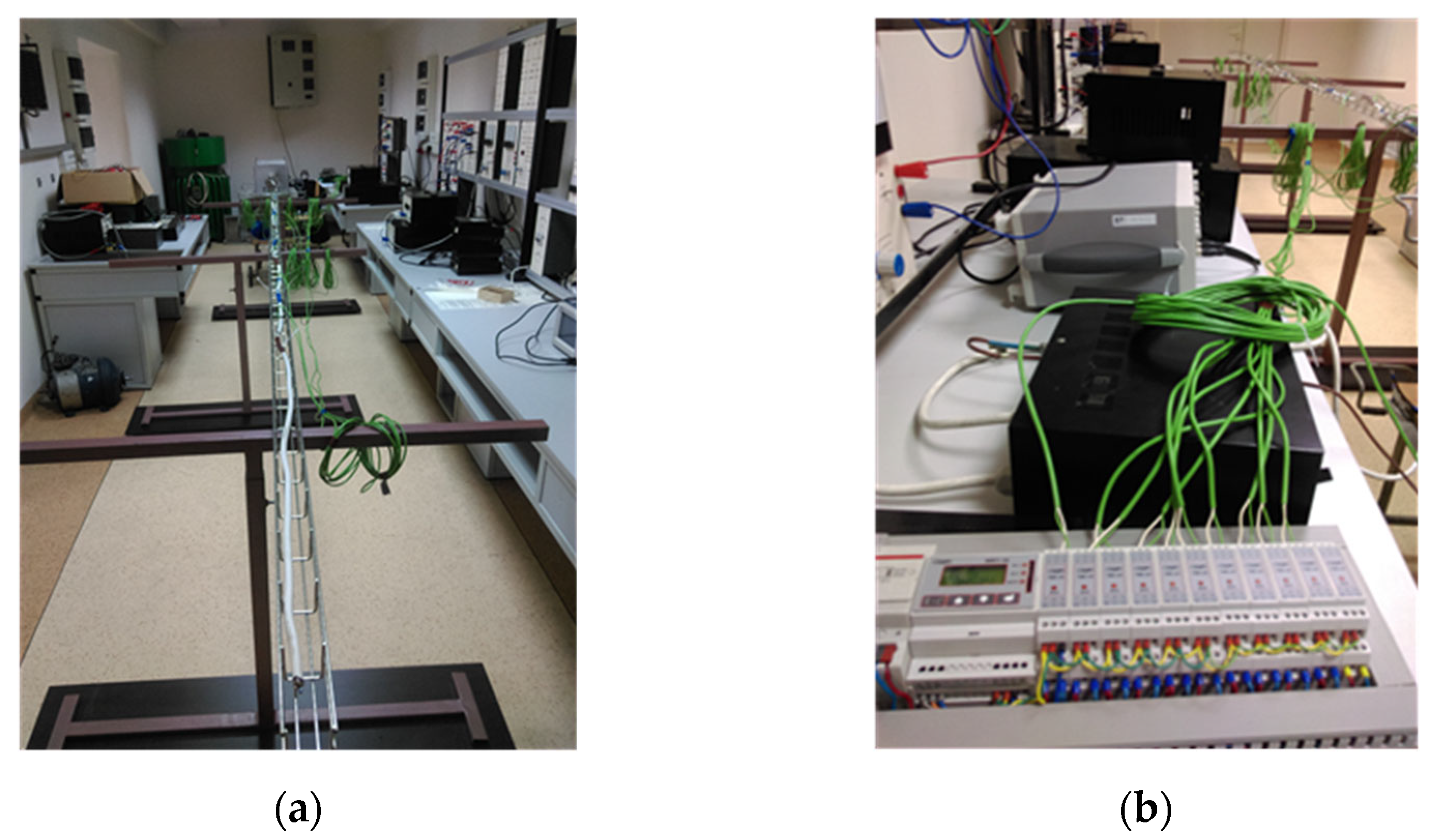
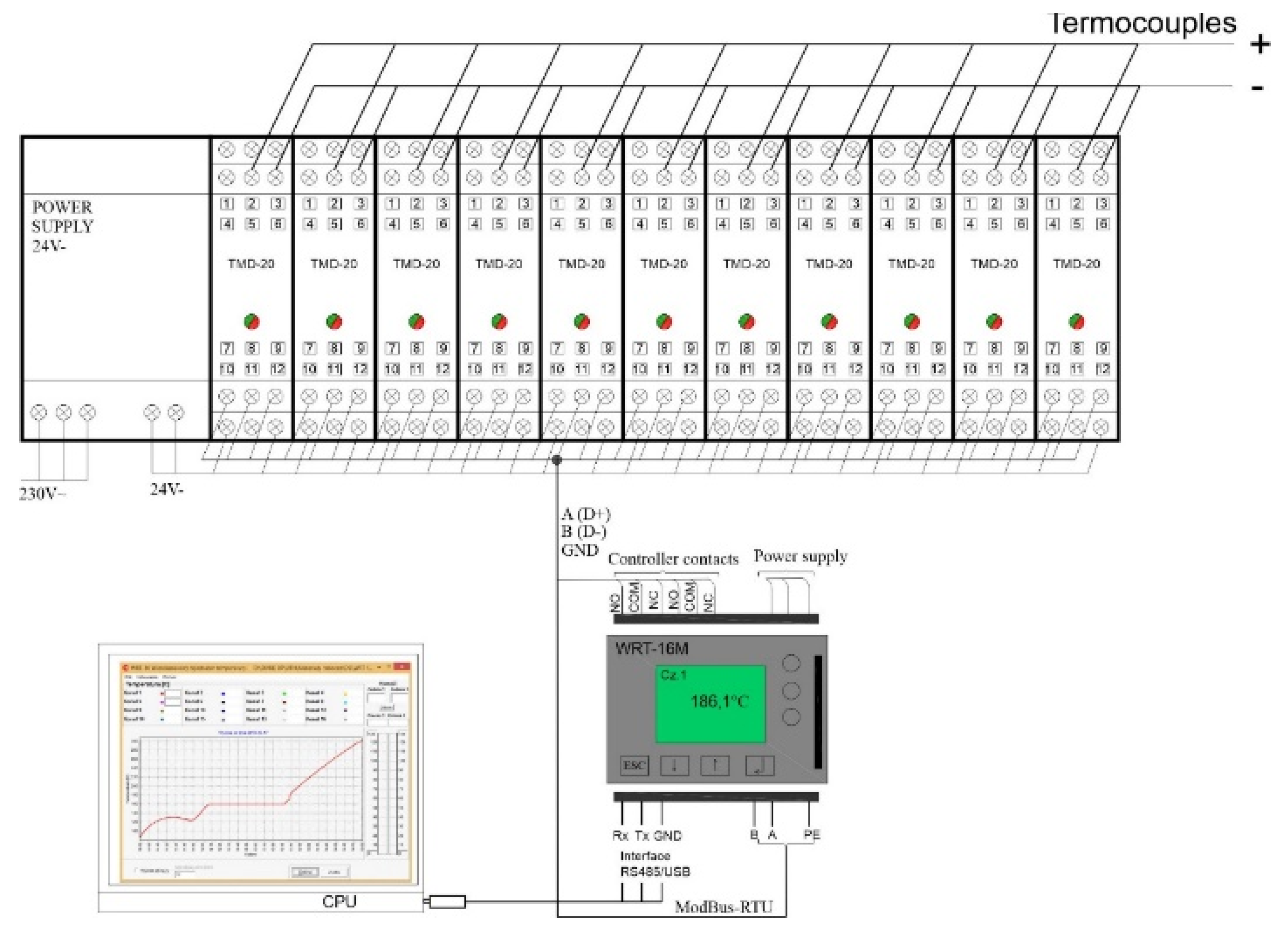
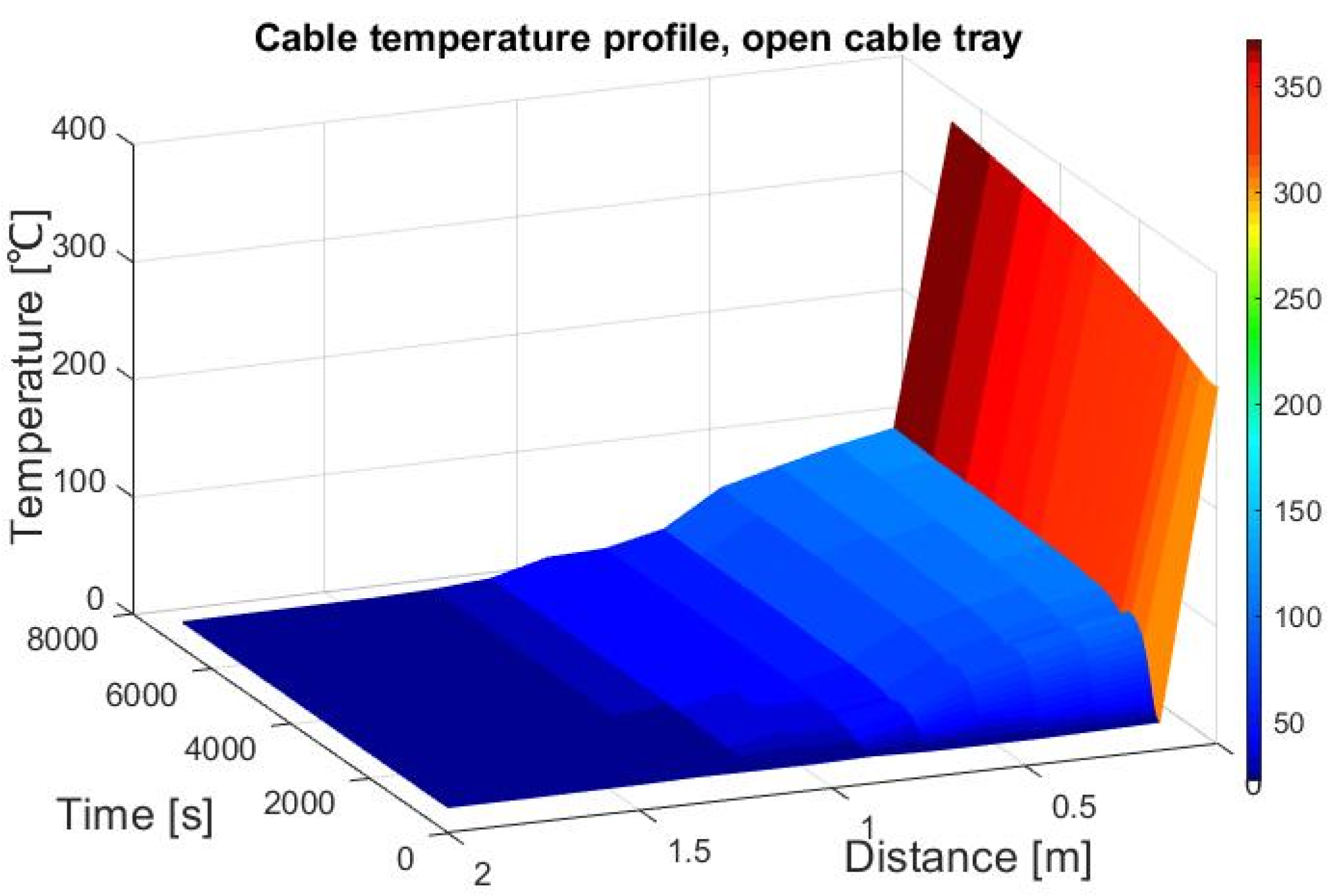
5. Test Bench
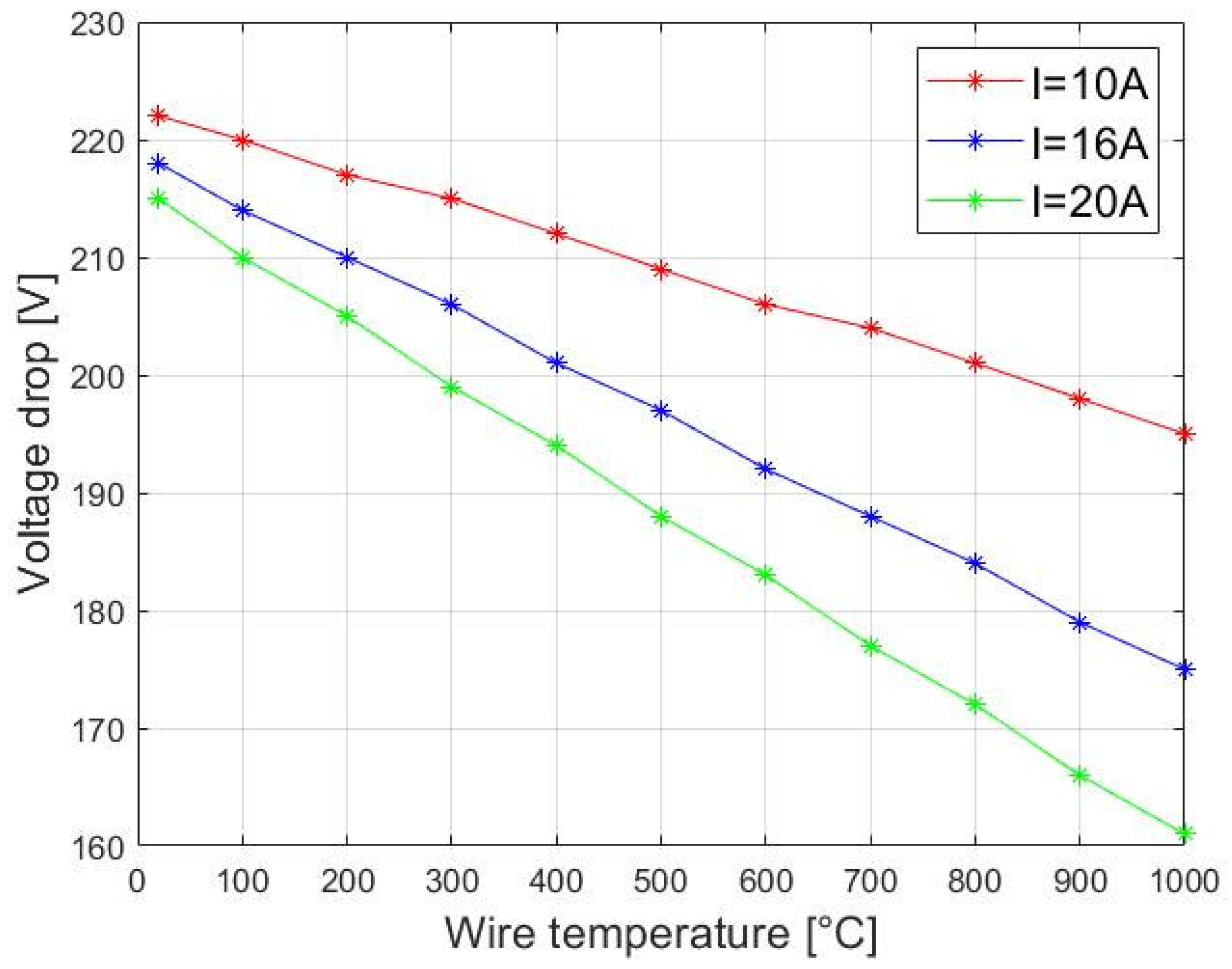
6. Research Experiment Result Analysis
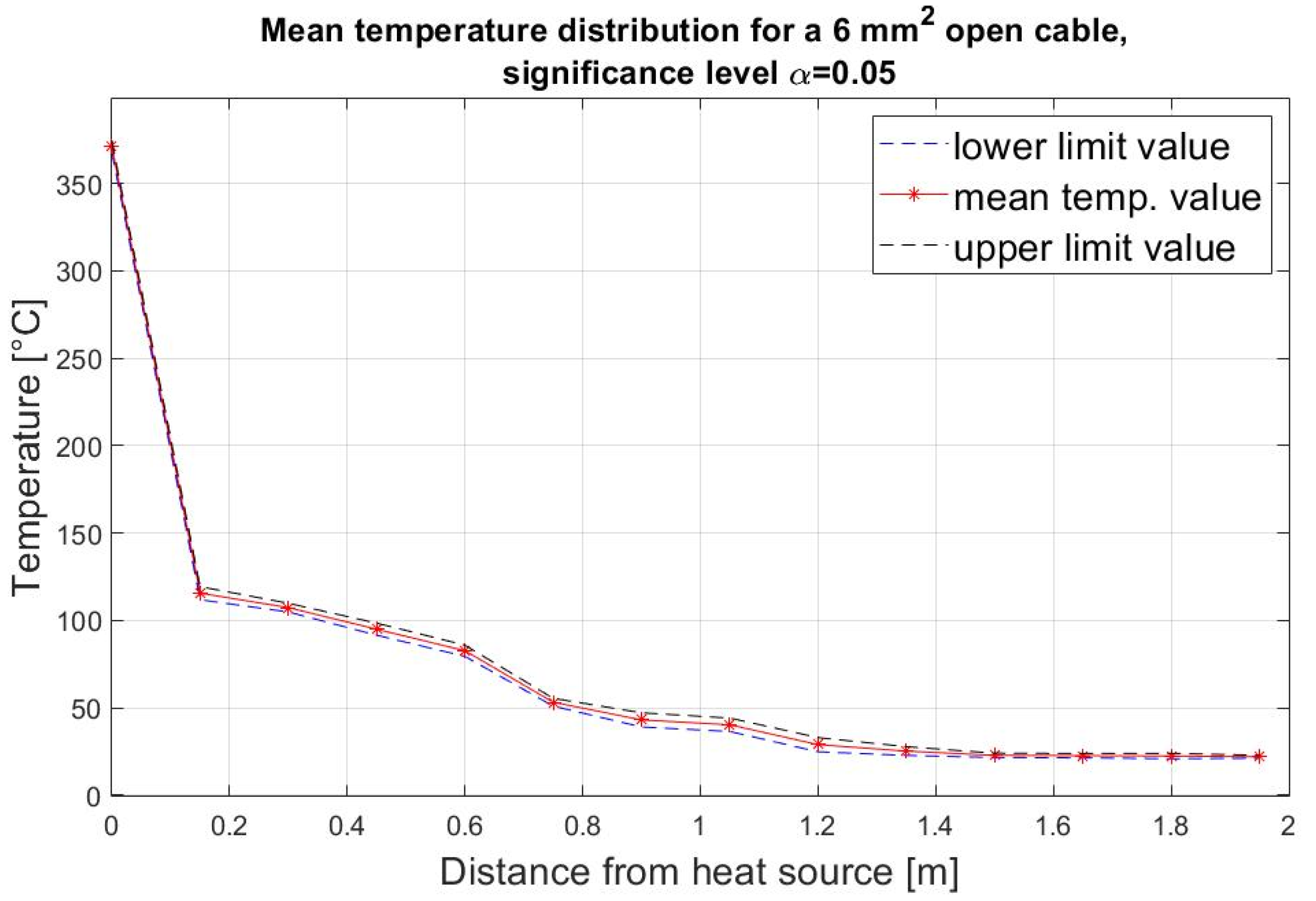
7. Simulation Model Result Analysis
8. Conclusions
- (a)
- The method based on applying the theory distributed parameters to solve a partial differential voltage equation of a transmission line resulted in a solution that can be used as a theoretical model of temperature distribution in electrical cables;
- (b)
- The knowledge of temperature values and distribution within the hot and intermediate zones of the cable enabled the calculation of the increase in the resistance of the cables exposed to a temperature field;
- (c)
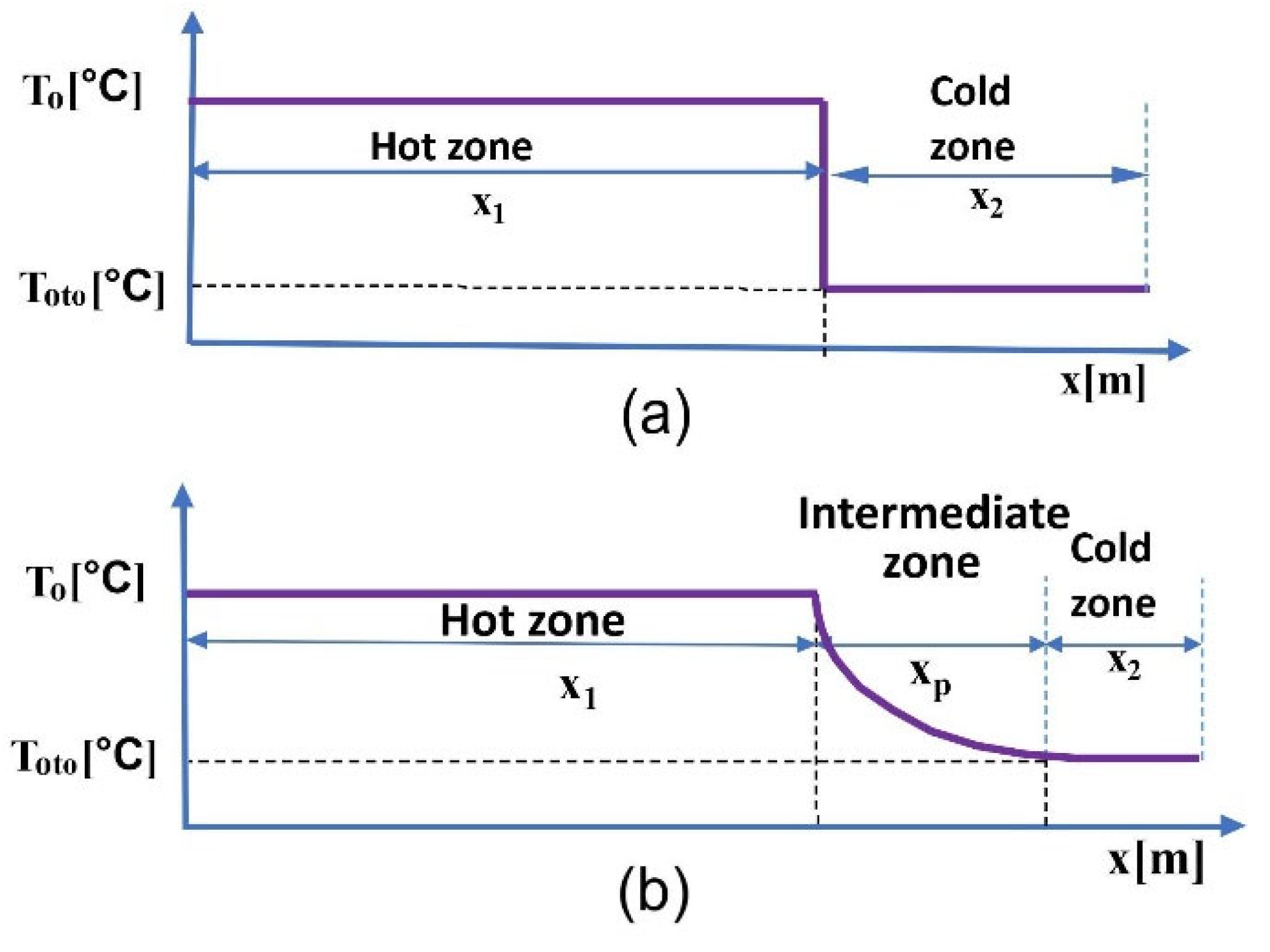
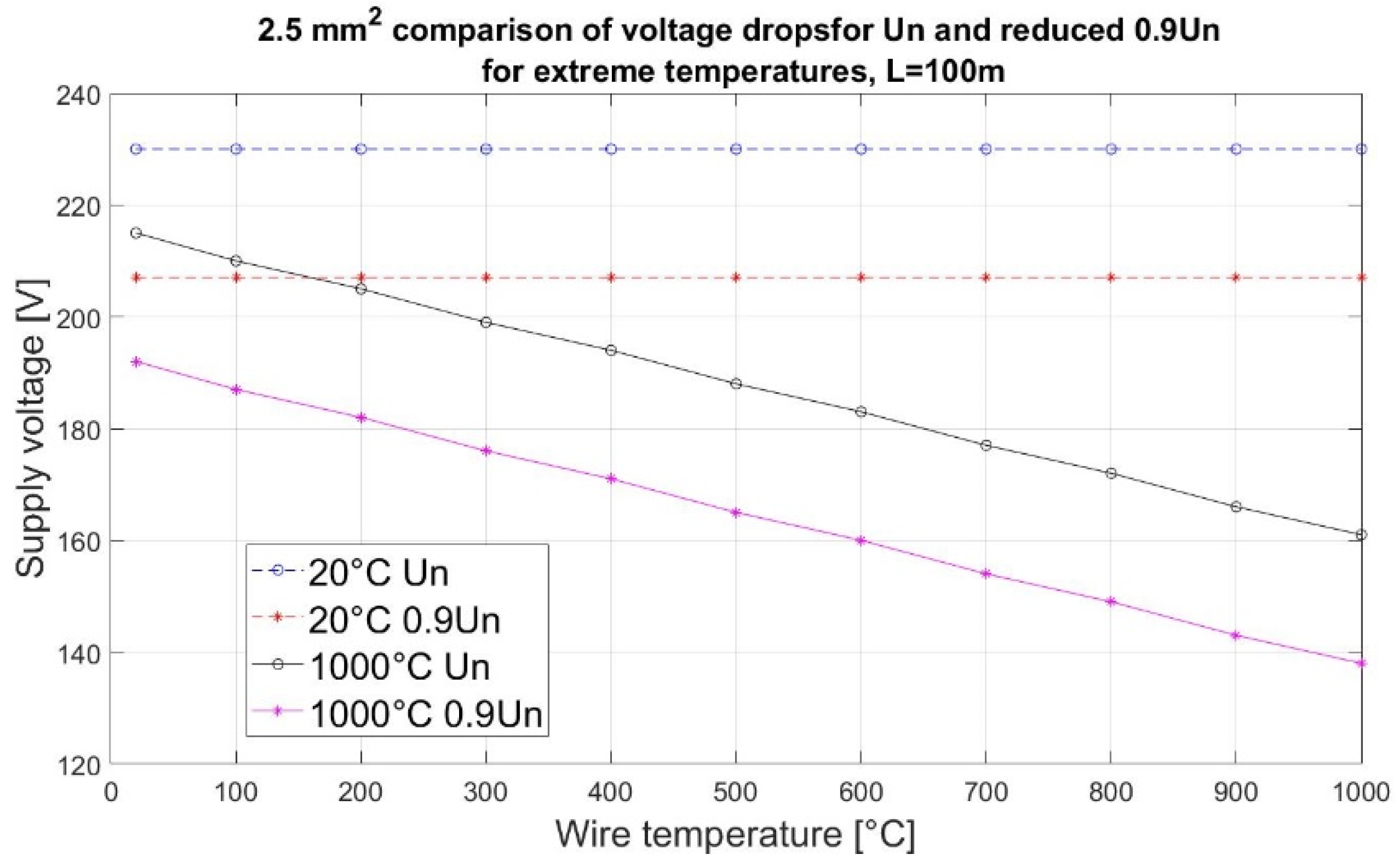
- Failure to take the intermediate zone into account leads to an incorrect determination of the actual voltage drop in an electrical cable;
- (d)
- Determining the thermal parameters of a cable and the time parameters of the heating process enabled the determination of the increase in the resistance of the cable caused by an external temperature excitation;
- (e)
- The value of the excitation temperature and heating time is decisive in terms of determining the impact of temperature on the resistance of evaluated cables.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Acronyms | |
| HFFR | Halogen-free flame-retardant |
| FEM | Finite element method |
| Symbols | |
| kR | Wire resistance increase coefficient |
| t | Time |
| x | Distance |
| ∆x | Elementary line segment |
| k | Thermal conductivity coefficient |
| T | Temperature |
| l | Wire length |
| R | Resistance |
| C | Capacitance |
| L | Inductance |
| U0 | Voltage of the voltage source |
| u | Instantaneous voltage value |
| U | Instantaneous voltage value subjected to Laplace transformation |
| i | Instantaneous current value |
| I | Instantaneous current value subjected to Laplace transformation |
| i(x, t) | Line current at a distance x from the source |
| u(x, t) | Line voltage at a distance x from the source |
| R∆x | Resistance of the elementary line segment |
| G∆x | Conductance of the elementary line segment |
| L∆x | Voltage loss due to the magnetic field of the long line |
| C∆x | Capacitance of elementary distance between the line cables |
| du(x, t)/dt | Change in line voltage depending on the distance x from the source |
| idu(x, t)/dt | Change in line current depending on the distance x from the source |
| u(x + ∆x, t) | Voltage at any point in the line |
| i(x + ∆x, t) | Current at any point in the line |
| Laplace transforms | |
| {du/dx} | Voltage Laplace transforms of the partial derivative over time |
| {di/dx} | Current Laplace transforms of the partial derivative over time |
| U(x, s) | Voltage Laplace transforms for the long line |
| I(x, s) | Current Laplace transforms for the long line |
| s | Laplace transform operator |
| f(t) | Function |
| F’(t) | Derivative of function f(t) |
| γ | Wave transfer constant of the long line |
| Right-hand limit of the f(t) function at point t = 0 | |
| n | Number of the sequential derivative |
| m | Coefficient resulting from the Laplace transformation |
| A1 | First integration constant |
| A2 | Second integration constant |
| Inverse Laplace transform | |
| erfc(x, t) | Completed error function |
| Terfc(x, t) | Temperature value calculated by the model equation |
| αdz | Equivalent cable heat diffusion coefficient |
| Toto | Cable ambient temperature |
| T0 | Impact temperature of an external temperature field |
| tc | Time exposure to an external heat source |
| xcz | Distance between measuring sensors |
| tpom | Total experiment time |
| tintr | Time step interval value |
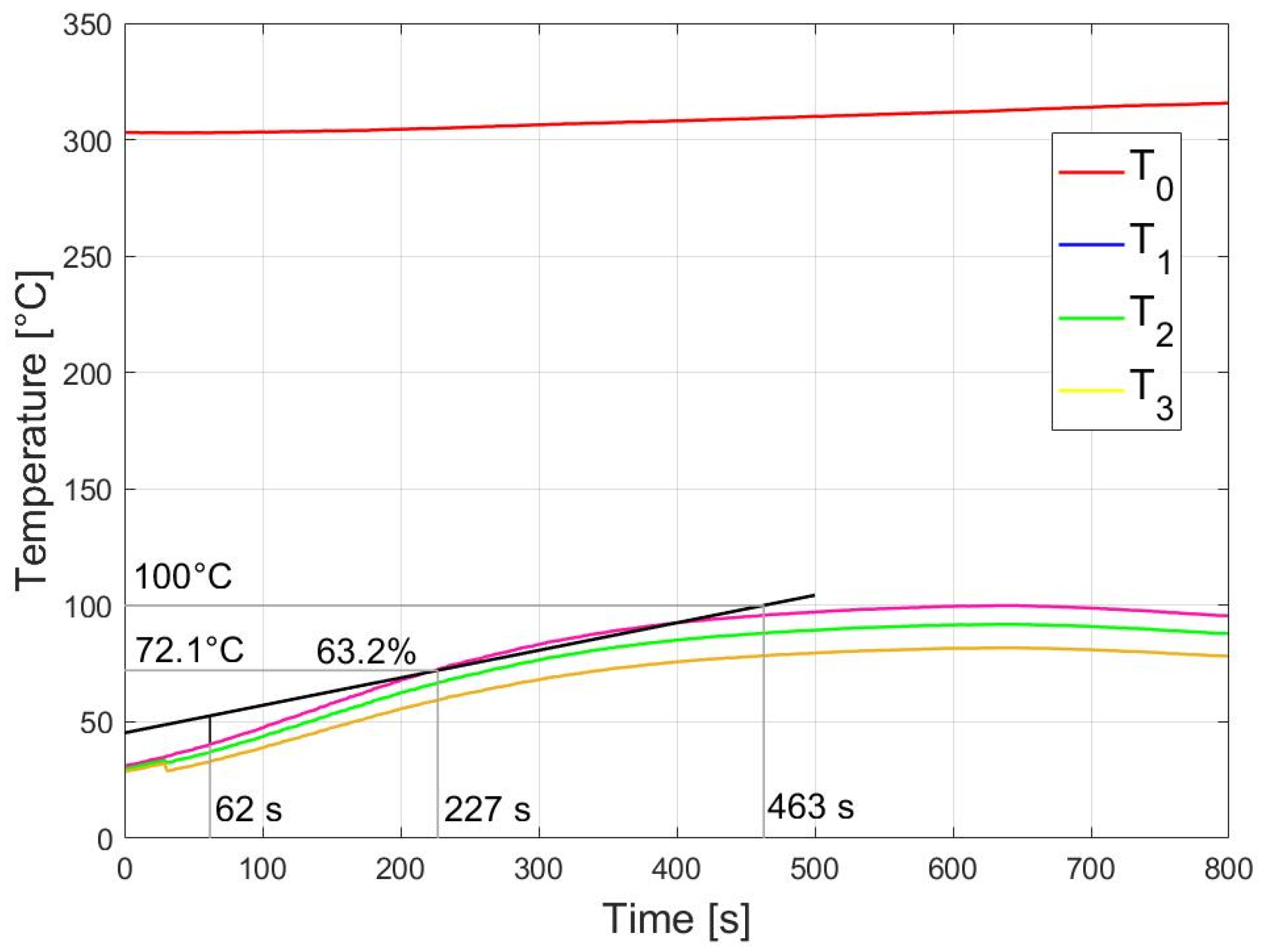
| τ | Time constant |
| τc | Response signal time constant |
| T1 | Temperature measured at a place 0.2 m away from the heat source |
| T2 | Temperature measured at a place 0.4 m away from the heat source |
| T3 | Temperature measured at a place 0.6 m away from the heat source |
| α | significance level value of measurement error |
| TH | Heating time |
| x1 | Length of the hot zone |
| x2 | Length of the cold zone and |
| xp | Length of the intermediate zone. |
| Cm | Heat capacity value |
| RT | Resistance of copper installation cables |
| Un | Supply voltage |
| Und | Reduced permissible voltage |
References
- Enescu, D.; Colella, P.; Russo, A. Thermal Assessment of Power Cables and Impacts on Cable Current Rating: An Overview. Energies 2020, 13, 5319. [Google Scholar] [CrossRef]
- Novak, B.; Tamus, Z.Á.; Koller, L. Heating of Cables Due to Fault Currents. In Proceedings of the 2010 IEEE International Symposium on Electrical Insulation, San Diego, CA, USA, 6–9 June 2010. [Google Scholar] [CrossRef]
- Ocłoń, P.; Pobędza, J.; Walczak, P.; Cisek, P.; Vallati, A. Experimental Validation of a Heat Transfer Model in Underground Power Cable Systems. Energies 2020, 13, 1747. [Google Scholar] [CrossRef]
- Perka, B.; Suproniuk, M.; Piwowarski, K. Application of acoustic surface wave to measure bus bar temperature. Prz. Elektrotechniczny 2019, 95, 120–123. [Google Scholar] [CrossRef]
- Szczegielniak, T.; Kusiak, D.; Jabłoński, P. Thermal Analysis of the Medium Voltage Cable. Energies 2021, 14, 4164. [Google Scholar] [CrossRef]
- Ghoneim, S.S.M.; Mahrouse, A.; Nehmdoh, A.S. Transient Thermal Performance of Power Cable Ascertained Using Finite Element Analysis. Processes 2021, 9, 438. [Google Scholar] [CrossRef]
- Jörgens, C.; Clemens, M. Electric Field and Temperature Simulations of High-Voltage Direct Current Cables Considering the Soil Environment. Energies 2021, 14, 4910. [Google Scholar] [CrossRef]
- Plesca, A. Temperature Distribution of HBC Fuses with Asymmetric Electric Current Ratios Through Fuselinks. Energies 2018, 11, 1990. [Google Scholar] [CrossRef]
- DIN. DIN 4102-12. In Behavior of Building Materials and Elements under the Influence of Fire. Maintaining the Functions of Devices during a Fire. Requirements and Tests; DIN: Berlin, Germany, 1998. [Google Scholar]
- Al–Saud, M.S. Assessment of thermal performance of underground current carrying conductors. IET Gener. Transm. Distrib. 2011, 5, 630–639. [Google Scholar] [CrossRef]
- Granieri, P.P. Heat transfer through cable insulation of Nb-Ti superconducting magnets operating in He II. Cryog. J. 2012, 53, 61–71. [Google Scholar] [CrossRef][Green Version]
- Anders, G.J.; Napieralski, A.; Kulesza, Z. Calculation of the internal thermal resistance and ampacity of 3-core screened cables with fillers. IEEE Trans. Power Deliv. 1999, 14, 729–734. [Google Scholar] [CrossRef]
- Bejan, A.; Kraus, A.D. Heat Transfer Handbook; Wiley: New York, NY, USA, 2003. [Google Scholar]
- Hanna, M.A.; Baxter, C.; Baxter, M.; Salama, M.M.A. Thermal modelling of cables in conduits: (Air gap consideration). In Proceedings of the 1995 Canadian Conference on Electrical and Computer Engineering (CCECEYCCGEI ’95), Montreal, QC, Canada, 5–8 September 1995; pp. 578–581. [Google Scholar] [CrossRef]
- Hiraiwa, Y.; Kasubuchi, T. Temperature dependence of thermal conductivity of soil over a wide range of temperature (5–75 °C). Eur. J. Soil Sci. 2000, 51, 211–218. [Google Scholar] [CrossRef]
- Brakelmann, H.; Anders, G.J.; Cherukupalli, S. Mitigation of a Hot Spot Along a Cable Circuit Using a Novel Cooling Solution. IEEE Trans. Power Deliv. 2020, 35, 592–599. [Google Scholar] [CrossRef]
- Chenzao, F.; Wenrong, S.; Lingyu, Z.; Hongle, L.; Zhoufeil, Y.; Yilin, W. Research on the fast calculation model for transient temperature rise of direct buried cable groups. In Proceedings of the 2018 12th International Conference on the Properties and Applications of Dielectric Materials (ICPADM), Xi’an, China, 20–24 May 2018; pp. 646–652. [Google Scholar] [CrossRef]
- Hui, Z.; Quing, C.; Haofei, S. Study on Temperature Field of Cable Tunnel Fire. In Proceedings of the 2019 9th International Conference on Fire Science and Fire Protection Engineering (ICFSFPE), Chengdu, China, 18–20 October 2019. [Google Scholar] [CrossRef]
- Packa, J.; Durman, V.; Sulova, J. Behaviour of Fire Resistant Cable Insulation with Different Flame Barriers During Water Immersion. In Proceedings of the 2019 20th International Scientific Conference on Electric Power Engineering (EPE), Kouty nad Desnou, Czech Republic, 29 July 2019. [Google Scholar] [CrossRef]
- Ren, Y.; Wu, K.; Coker, D.F.; Quirke, N. Thermal transport in model copper-polyethylene interfaces. J. Chem. Phys. 2019, 151, 174708. [Google Scholar] [CrossRef] [PubMed]
- Robertson, A.F.; Gross, D. An Electrical—Analog method for Transient Heat-Flow Analisis. J. Res. Natl. Stand. 1958, 61, 2892. [Google Scholar]
- Sarajcev, I.; Majstrovic, M.; Medic, I. Calculation of losses in electric power cables as the base for cable temperaturę analysis. WIT Trans. Eng. Sci. 2000, 29, 9. [Google Scholar] [CrossRef]
- Olsen, R.; Anders, G.J.; Holboell, J.; Gudmundsdottir, U.S. Modelling of Dynamic Transmission Cable Temperature Considering Soil-Specific Heat, Thermal Resistivity, and Precipitation. IEEE Trans. Power Deliv. 2013, 28, 1909–1917. [Google Scholar] [CrossRef]
- Suproniuk, M.; Pawlowski, M.; Wierzbowski, M.; Majda-Zdancewicz, E.; Pawlowski, M. Comparison of methods applied in photoinduced transient spectroscopy to determining the defect center parameters: The correlation procedure and the signal analysis based on inverse Laplace transformation. Rev. Sci. Instrum. 2018, 89, 044702. [Google Scholar] [CrossRef] [PubMed]
- Goga, V.; Paulech, J.; Wary, M. Cooling of electrical Cu conductor with PVC insulation-analytical, numerical and fluid flow solution. J. Electr. Eng. 2013, 64, 92–99. [Google Scholar] [CrossRef]
- Nakayama, W. Heat-transfer engineering in systems integration: Outlook for closer coupling of thermal and electrical designs of computers. IEEE Trans. Compon. Packag. Manuf. Technol. Part A 1995, 18, 818–826. [Google Scholar] [CrossRef]
- Purushothaman, S.; de León, F.; Terracciano, M. Calculation of cable thermal rating considering non-isothermal earth surface. IET Gener. Transmiss. Distrib. 2014, 8, 1354–1361. [Google Scholar] [CrossRef]
- Wang, Z.; Karniadakis, G.E.; Chalafant, J.; Chryssostomidis, C.; Babaee, H. High-fidelity modeling and optimization of conjugate heat transfer in arrays of heated cables. In Proceedings of the 2017 IEEE Electric Ship Technologies Symposium (ESTS), Arlington, VA, USA, 14–17 August 2017; pp. 557–563. [Google Scholar] [CrossRef]
- Garrido, C.; Antonio, F.O.; Cidras, J. Theoretical model to calculate steady-state and transient ampacity and temperature in buried cables. IEEE Trans. Power Deliv. 2003, 18, 667–678. [Google Scholar] [CrossRef]
- Gang, L.; LEI Ming, R.; Banyi, Z.; Fan, L.; Yinghong, L.Y. Model Research of Real-time Calculation for Single-core Cable. Temperature Considering Axial Heat Transfer. Gaodianya Jishu/High Volt. Eng. 2012, 38, 1877–1883. [Google Scholar] [CrossRef]
- Suproniuk, M.; Pas, J. Analysis of electrical energy consumption in a public utility buildings. Prz. Elektrotechniczny 2019, 95, 97–100. [Google Scholar] [CrossRef]
- Desmet, J.; Putman, D.; Vanalme, G.; Belmans, R.; Vandommelent, D. Thermal analysis of parallel underground energy cables. In Proceedings of the CIRED 2005—18th International Conference and Exhibition on Electricity, Distribution, Turin, Italy, 6–9 June 2005; pp. 1–4. [Google Scholar] [CrossRef]
- Ariyanayagam, A.D.; Mahen Mahendran, M. Energy Based Time Equivalent Approach to Determine the Fire Resistance Ratings of Light Gauge Steel Frame Walls Exposed to Realistic Design Fire Curves. J. Struct. Fire Eng. 2017, 8, 46–72. [Google Scholar]
- Zhao, H.C.; Lyall, J.S.; Nourbakhsh, G. Probabilistic cable rating based on cable thermal environment studying. In Proceedings of the PowerCon International Conference on Power System Technology (Cat. No.00EX409), Perth, Australia, 4–7 December 2000; Volume 2, pp. 1071–1076. [Google Scholar] [CrossRef]

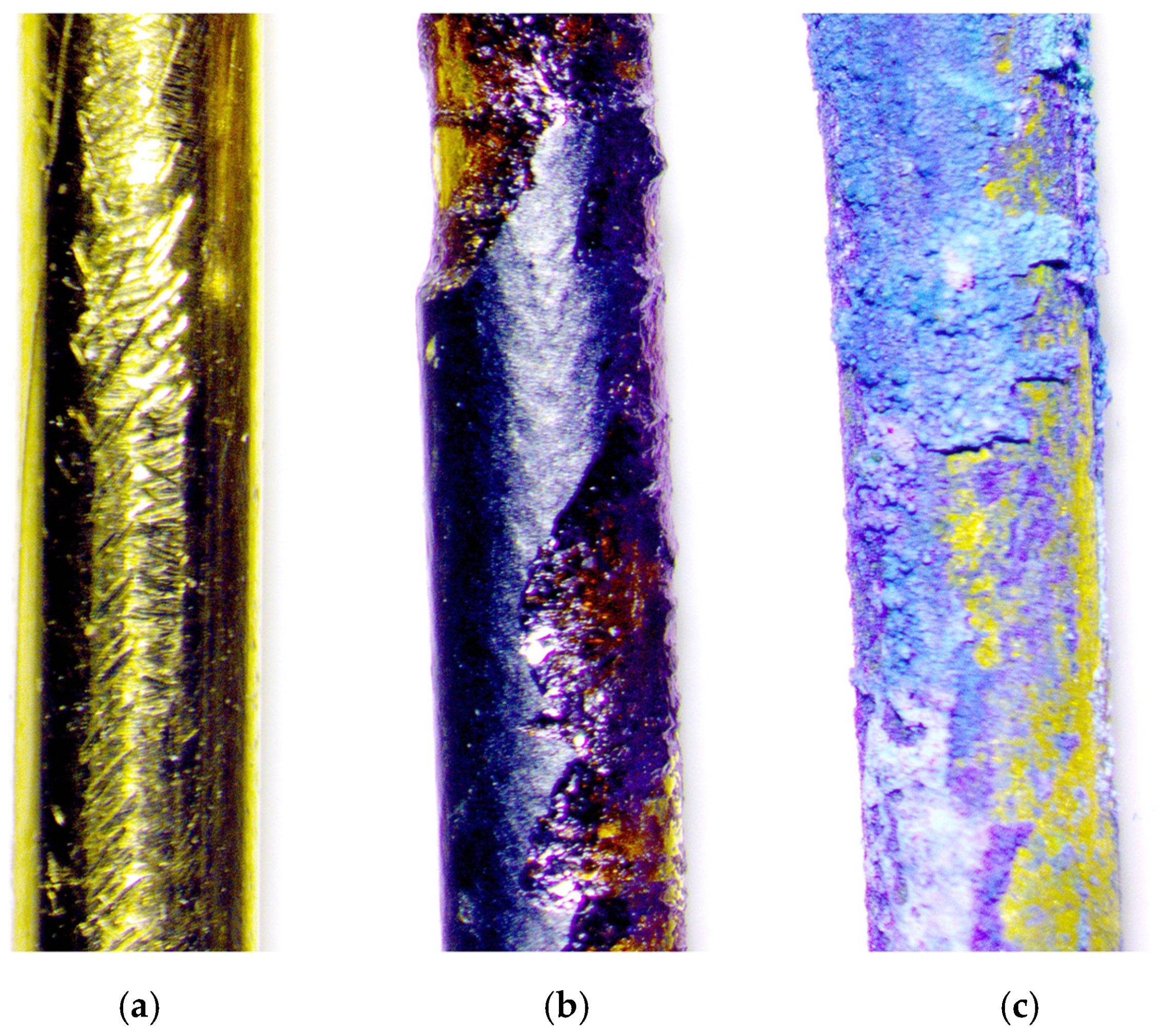

| Material | Thermal Conductivity Coefficient | Specific Heat Capacity |
|---|---|---|
| Copper | 399 | 380 |
| Mica | 0.523 | 1800 |
| Polyethylene | 0.25 | 1000 |
| XLPE | 0.25 | 1000 |
| HFFR insulation | 0.25 | 1000 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Perka, B.; Piwowarski, K. A Method for Determining the Impact of Ambient Temperature on an Electrical Cable during a Fire. Energies 2021, 14, 7260. https://doi.org/10.3390/en14217260
Perka B, Piwowarski K. A Method for Determining the Impact of Ambient Temperature on an Electrical Cable during a Fire. Energies. 2021; 14(21):7260. https://doi.org/10.3390/en14217260
Chicago/Turabian StylePerka, Bogdan, and Karol Piwowarski. 2021. "A Method for Determining the Impact of Ambient Temperature on an Electrical Cable during a Fire" Energies 14, no. 21: 7260. https://doi.org/10.3390/en14217260
APA StylePerka, B., & Piwowarski, K. (2021). A Method for Determining the Impact of Ambient Temperature on an Electrical Cable during a Fire. Energies, 14(21), 7260. https://doi.org/10.3390/en14217260





