Reduction in Human Interaction with Magnetic Resonant Coupling WPT Systems with Grounded Loop
Abstract
1. Introduction
2. Materials and Methods
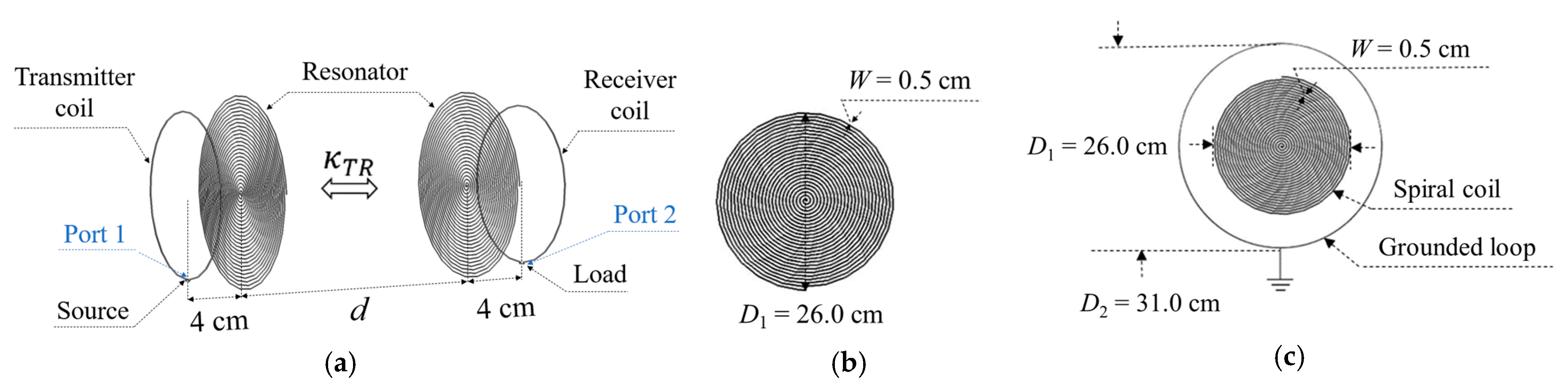
2.1. Geometric Structure of the Proposed MRC-WPT System
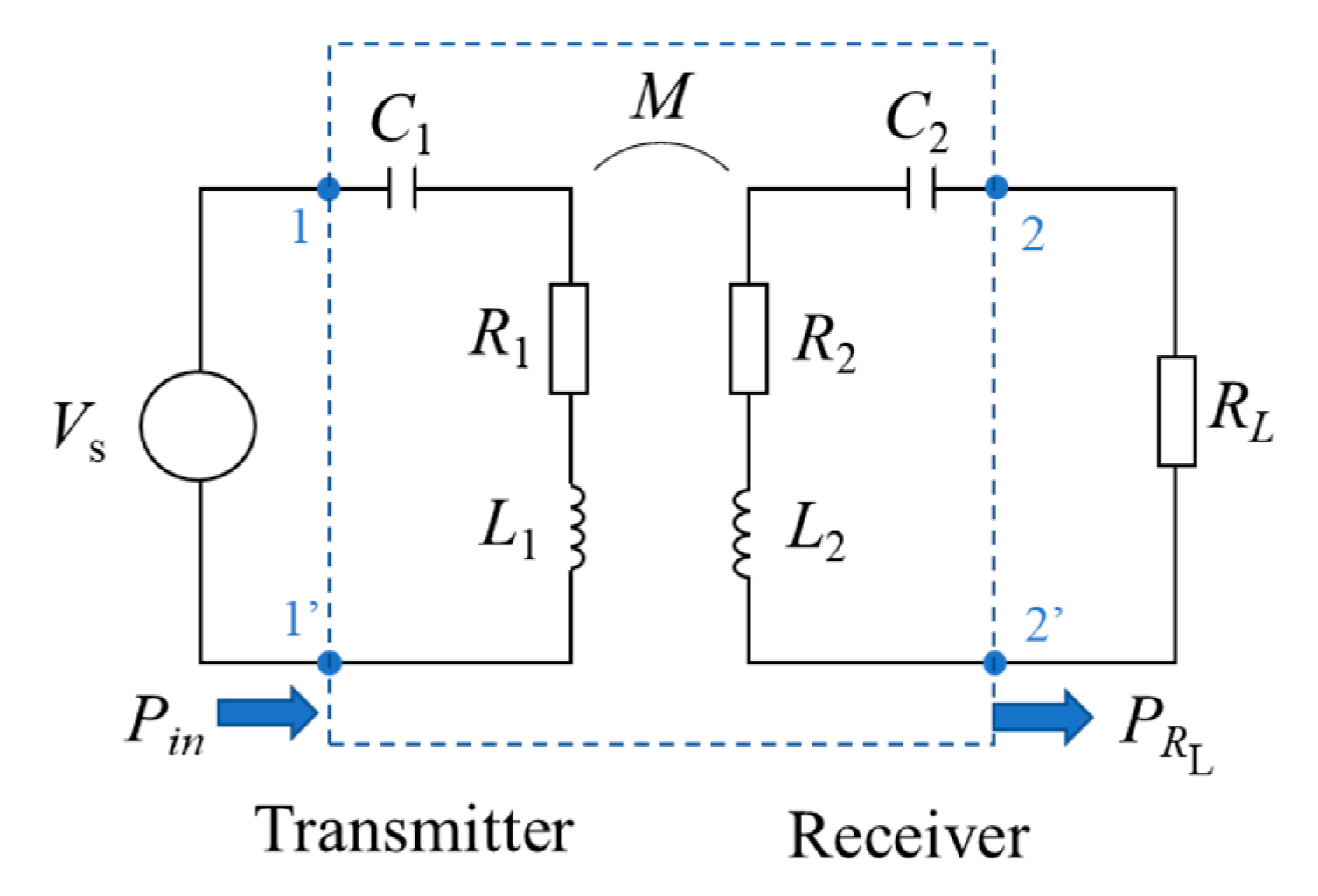
2.2. Transmission Efficiency of the Proposed MRC-WPT System
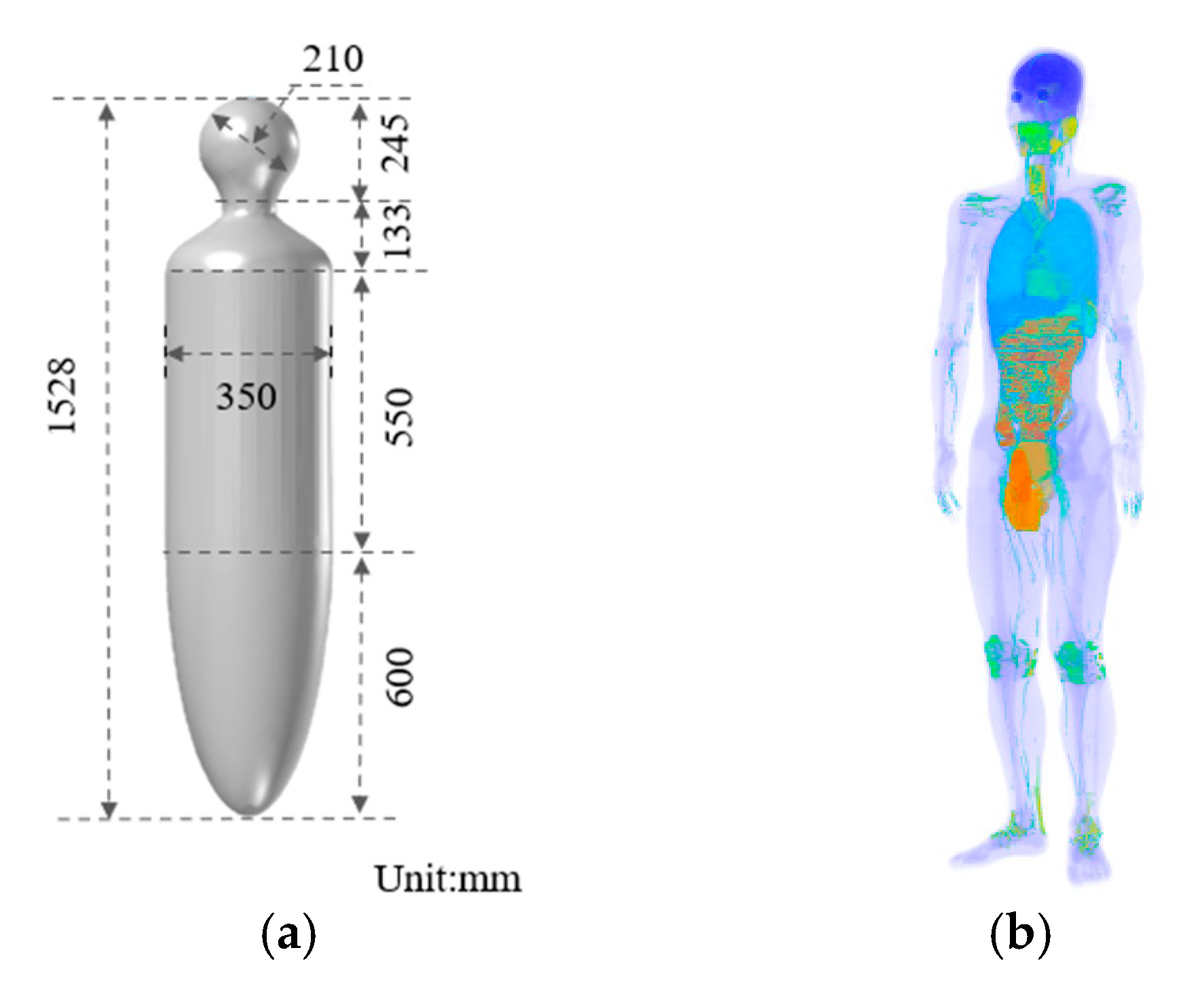
2.3. Human-Body Model
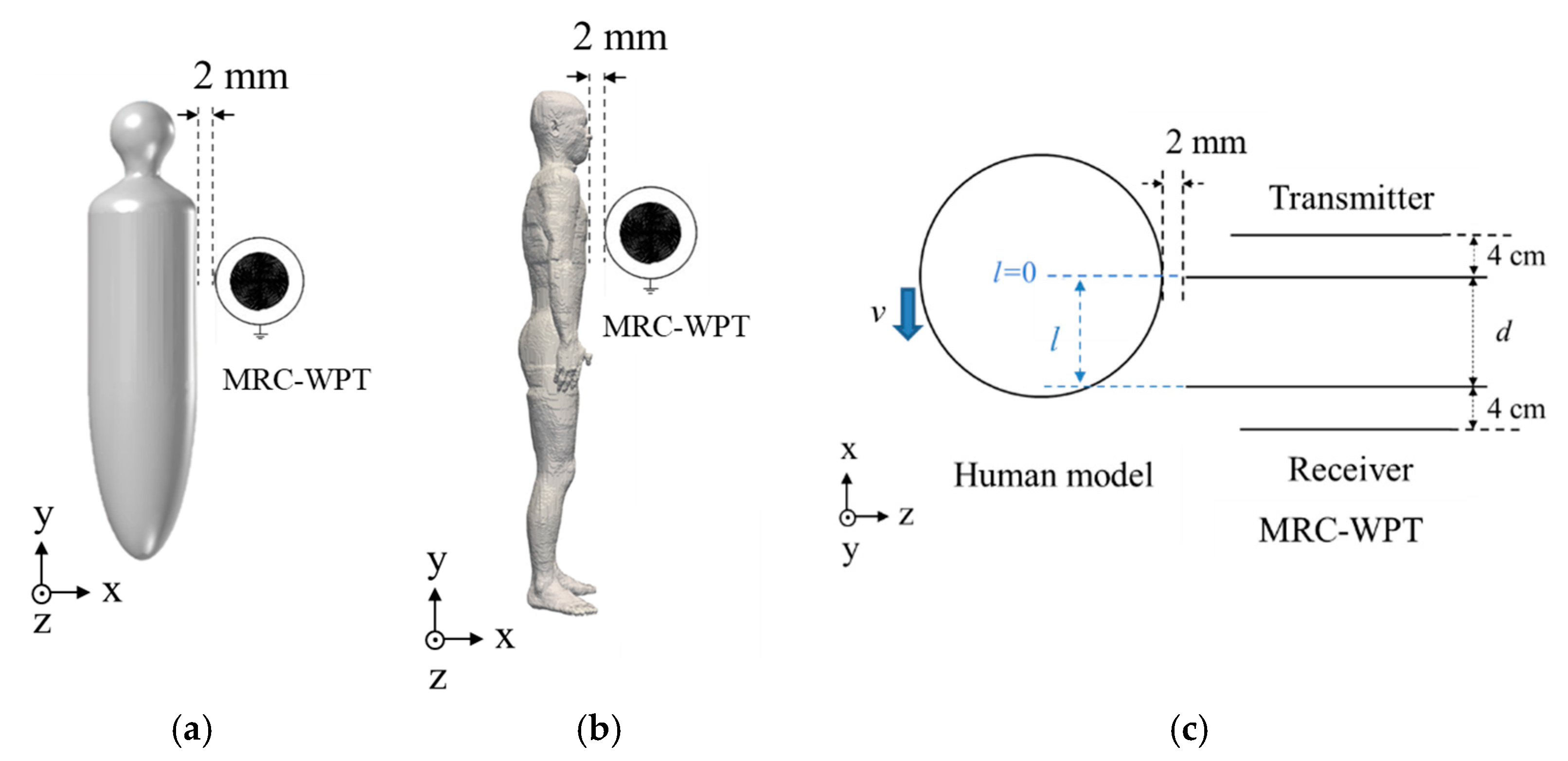
2.4. Exposure Scenarios
2.5. Simulation of Mutual Interaction
2.6. Simulation of Exposure Dose in the TARO Model
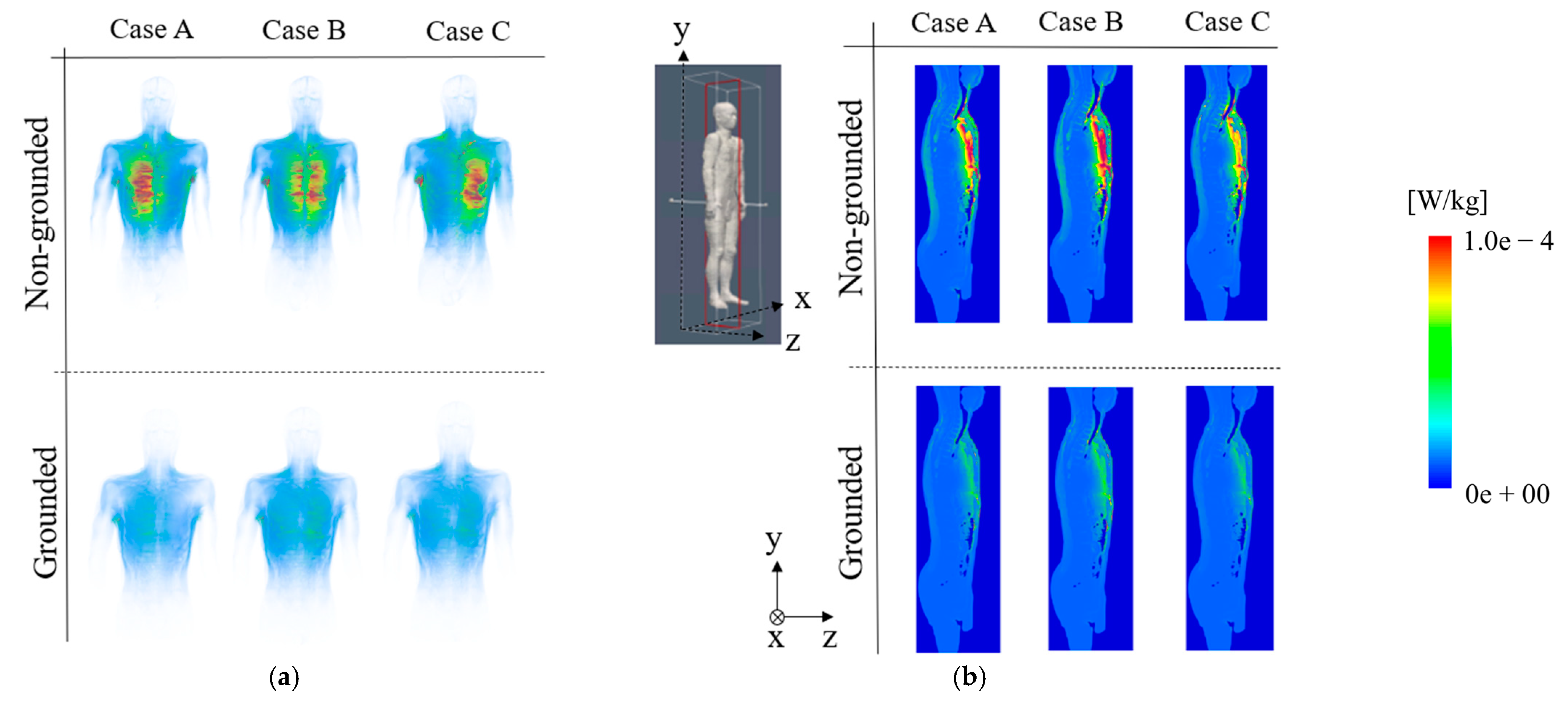
3. Results and Discussion
3.1. Validation of the MRC-WPT System Computation
3.2. Interactions between the Human Body and MRC-WPT System
3.3. Exposure Doses in the TARO Model
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Kurs, A.; Karalis, A.; Moffatt, R.; Joannopoulos, J.D.; Fisher, P.; Soljačić, M. Wireless power transfer via strongly coupled magnetic resonances. Science 2007, 317, 83–86. [Google Scholar] [CrossRef]
- Ho, S.L.; Wang, J.; Fu, W.N.; Sun, M. A Comparative Study Between Novel Witricity and Traditional Inductive Magnetic Coupling in Wireless Charging. IEEE Trans. Magn. 2011, 47, 1522–1525. [Google Scholar] [CrossRef]
- Houran, M.A.; Yang, X.; Chen, W. Magnetically coupled resonance wpt: Review of compensation topologies, resonator structures with misalignment, and emi diagnostics. Electronics 2018, 7, 296. [Google Scholar] [CrossRef]
- Abdelhafeez, M.; Yousef, K.; Abdelraheem, M.; Khaled, E.E.M. Design of 6 GHz High Efficiency Long Range Wireless Power Transfer System Using Offset Reflectors fed by Conical Horns. In Proceedings of the 2019 International Conference on Innovative Trends in Computer Engineering ITCE 2019, Aswan, Egypt, 2–4 February 2019; pp. 365–370. [Google Scholar] [CrossRef]
- Karalis, A.; Joannopoulos, J.D.; Soljačić, M. Efficient wireless non-radiative mid-range energy transfer. Ann. Phys. 2008, 323, 34–48. [Google Scholar] [CrossRef]
- Alashgar, D.; Fujiwara, K.; Nagaoka, N. Fundamental Investigation of Short-Range Inductive Coupling Wireless Power Transmission by Using Series-Series Capacitive Compensation Topology. J. Asian Electr. Veh. 2019, 17, 1811–1822. [Google Scholar] [CrossRef]
- Zhang, Y.; Lu, T.; Zhao, Z.; He, F.; Chen, K.; Yuan, L. Employing Load Coils for Multiple Loads of Resonant Wireless Power Transfer. IEEE Trans. Power Electron. 2015, 30, 6174–6181. [Google Scholar] [CrossRef]
- Pusti, A.; Das, P.K.; Panda, A.K.; Padhi, J.; Kar, D.P. Essential analysis of MRC-WPT system for electric vehicle charging using coupled mode theory. In Proceedings of the 2021 1st Odisha International Conference on Electrical Power Engineering, Communication and Computing Technology (ODICON), Bhubaneswar, India, 8–9 January 2021; pp. 1–5. [Google Scholar] [CrossRef]
- Tan, L.; Elnail, K.E.I.; Ju, M.; Huang, X. Comparative analysis and design of the shielding techniques in WPT systems for charging EVs. Energies 2019, 12, 2115. [Google Scholar] [CrossRef]
- Mohamadi, T. Working frequency in wireless power transfer for implantable biomedical sensors. In Proceedings of the 2011 International Conference on Electrical Engineering and Informatics, Bandung, Indonesia, 17–19 July 2011; pp. 1–5. [Google Scholar] [CrossRef]
- Zakaria, N.A.; Jusoh, M.; Ghazali, N.H.; Yasin, M.N.; Sabapathy, T.; Osman, M.N.; Ahmad, N.; Zakaria, M.Z. A Computational Study on the Magnetic Resonance Coupling Technique for Wireless Power Transfer. MATEC Web Conf. 2017, 140, 01025. [Google Scholar] [CrossRef]
- Campi, T.; Cruciani, S.; Palandrani, F.; de Santis, V.; Hirata, A.; Feliziani, M. Wireless Power Transfer Charging System for AIMDs and Pacemakers. IEEE Trans. Microw. Theory Tech. 2016, 64, 633–642. [Google Scholar] [CrossRef]
- Arai, T.; Hirayama, H. Folded spiral resonator with double-layered structure for near-field wireless power transfer. Energies 2020, 13, 1581. [Google Scholar] [CrossRef]
- Kumazawa, A.; Diao, Y.; Hirata, A.; Hirayama, H. Reduction of Human Interaction with Wireless Power Transfer System Using Shielded Loop Coil. Electronics 2020, 9, 953. [Google Scholar] [CrossRef]
- Laakso, I.; Tsuchida, S.; Hirata, A.; Kamimura, Y. Evaluation of SAR in a human body model due to wireless power transmission in the 10MHz band. Phys. Med. Biol. 2012, 57, 4991–5002. [Google Scholar] [CrossRef]
- Park, S.W. Misaligned effect and exposure assessment for wireless power transfer system using the anatomical whole-body human model. Prog. Electromagn. Res. C 2017, 77, 19–28. [Google Scholar] [CrossRef]
- Škiljo, M.; Blažević, Z.; Poljak, D. Interaction between human and near-field of wireless power transfer system. Prog. Electromagn. Res. C 2016, 67, 1–10. [Google Scholar] [CrossRef][Green Version]
- Hikage, T.; Yamagishi, M.; Shindo, K.; Nojima, T. Active implantable medical device EMI estimation for EV-charging WPT system based on 3D full-wave analysis. In Proceedings of the 2017 Asia-Pacific International Symposium on EMC (APEMC), Seoul, Korea, 20–23 June 2017; pp. 87–89. [Google Scholar] [CrossRef]
- Campi, T.; Cruciani, S.; de Santis, V.; Maradei, F.; Feliziani, M. EMC and EMF safety issues in wireless charging system for an electric vehicle (EV). In Proceedings of the 2017 International Conference of Electrical and Electronic Technologies for Automotive, Torino, Italy, 15–16 June 2017; pp. 23–26. [Google Scholar] [CrossRef]
- Lyu, Y.L.; Meng, F.Y.; Yang, G.H.; Che, B.J.; Wu, Q.; Sun, L.; Erni, D.; Li, J.L.W. A Method of Using Nonidentical Resonant Coils for Frequency Splitting Elimination in Wireless Power Transfer. IEEE Trans. Power Electron. 2015, 30, 6097–6107. [Google Scholar] [CrossRef]
- Hirata, A.; Diao, Y.; Onishi, T.; Sasaki, K.; Ahn, S.; Colombi, D.; de Santis, V.; Laakso, I.; Giaccone, L.; Joseph, W.; et al. Assessment of Human Exposure to Electromagnetic Fields: Review and Future Directions. IEEE Trans. Electromagn. Compat. 2021, 63, 1619–1630. [Google Scholar] [CrossRef]
- Hirata, A.; Ito, F.; Laakso, I. Confirmation of quasi-static approximation in SAR evaluation for a wireless power transfer system. Phys. Med. Biol. 2013, 58, N241–N249. [Google Scholar] [CrossRef] [PubMed]
- ICNIRP. Guidelines for limiting exposure to electromagnetic fields (100 kHz to 300 GHz). Health Phys. 2020, 118, 483–524. [Google Scholar] [CrossRef] [PubMed]
- IEEE. IEEE standard for safety levels with respect to human exposure to electric, magnetic, and electromagnetic fields, 0 Hz to 300 GHz-corrigenda 2. In IEEE Std C95.1-2019/Cor2-2020 (Corrigenda to IEEE Std C95.1-2019); IEEE: New York, NY, USA, 21 October 2020; pp. 1–15. [Google Scholar] [CrossRef]
- Ziegelberger, G.; van Rongen, E.; Croft, R.; Feychting, M.; Green, A.C.; Hirata, A.; d’Inzeo, G.; Marino, C.; Miller, S.; Oftedal, G.; et al. Principles for non-ionizing radiation protection. Health Phys. 2020, 118, 477–482. [Google Scholar] [CrossRef]
- Hoang, L.H.; Scorretti, R.; Burais, N.; Voyer, D. Numerical dosimetry of induced phenomena in the human body by a three-phase power line. IEEE Trans. Magn. 2009, 45, 1666–1669. [Google Scholar] [CrossRef]
- Chatterjee, I.; Gu, Y.G.; Gandhi, O.P. Quantification of Electromagnetic Absorption in Humans from Body-Mounted Communication Transceivers. IEEE Trans. Veh. Technol. 1985, 34, 55–62. [Google Scholar] [CrossRef]
- Nagaoka, T.; Watanabe, S.; Sakurai, K.; Kunieda, E.; Watanabe, S.; Taki, M.; Yamanaka, Y. Development of realistic high-resolution whole-body voxel models of Japanese adult males and females of average height and weight, and application of models to radio-frequency electromagnetic-field dosimetry. Phys. Med. Biol. 2004, 49, 1–15. [Google Scholar] [CrossRef]
- Park, S.W. Investigating human exposure to a practical wireless power transfer system using and the effect about key parameters of dosimetry. PLoS ONE 2020, 15, e0236929. [Google Scholar] [CrossRef]
- Park, S.W. Dosimetry for Resonance-Based Wireless Power Transfer Charging of Electric Vehicles. J. Electromagn. Eng. Sci. 2015, 15, 129–133. [Google Scholar] [CrossRef]
- Arduino, A.; Bottauscio, O.; Chiampi, M.; Giaccone, L.; Liorni, I.; Kuster, N.; Zilberti, L.; Zucca, M. Accuracy Assessment of Numerical Dosimetry for the Evaluation of Human Exposure to Electric Vehicle Inductive Charging Systems. IEEE Trans. Electromagn. Compat. 2020, 62, 1939–1950. [Google Scholar] [CrossRef]
- Miwa, K.; Takenaka, T.; Hirata, A. Electromagnetic Dosimetry and Compliance for Wireless Power Transfer Systems in Vehicles. IEEE Trans. Electromagn. Compat. 2019, 61, 2024–2030. [Google Scholar] [CrossRef]
- Shimamoto, T.; Laakso, I.; Hirata, A. In-situ electric field in human body model in different postures for wireless power transfer system in an electrical vehicle. Phys. Med. Biol. 2015, 60, 163–173. [Google Scholar] [CrossRef]
- Perhirin, S.; Auffret, Y. Influence of fields and SAR evaluation for 13.56 MHz EV resonance-based wireless power charging systems. Microw. Opt. Technol. Lett. 2013, 55, 2562–2568. [Google Scholar] [CrossRef]
- Nadakuduti, J.; Douglas, M.; Lu, L.; Christ, A.; Guckian, P.; Kuster, N. Compliance Testing Methodology for Wireless Power Transfer Systems. IEEE Trans. Power Electron. 2015, 30, 6264–6273. [Google Scholar] [CrossRef]
- Sunohara, T.; Hirata, A.; Laakso, I.; de Santis, V.; Onishi, T. Evaluation of nonuniform field exposures with coupling factors. Phys. Med. Biol. 2015, 60, 8129–8140. [Google Scholar] [CrossRef]
- Wake, K.; Laakso, I.; Hirata, A. Derivation of coupling factors for different wireless power transfer systems: Inter- and intralaboratory comparison. IEEE Trans. Electromagn. Compat. 2016, 59, 1–9. [Google Scholar] [CrossRef]
- Zhang, W.; White, J.C.; Malhan, R.K.; Mi, C.C. Loosely Coupled Transformer Coil Design to Minimize EMF Radiation in Concerned Areas. IEEE Trans. Veh. Technol. 2016, 65, 4779–4789. [Google Scholar] [CrossRef]
- Campi, T.; Cruciani, S.; Maradei, F.; Feliziani, M. Near-Field Reduction in a Wireless Power Transfer System Using LCC Compensation. IEEE Trans. Electromagn. Compat. 2017, 59, 686–694. [Google Scholar] [CrossRef]
- Duan, X.; Norodin, N.S.A.; Sakata, A.; Hotta, M. Fundamental characteristics of some modified shape spiral-resonators used for resonator-coupled type wireless power transfer system. In Proceedings of the 21th IEEE Hiroshima Section Student Symposium (HISS), Okayama, Japan, 9–10 November 2019; pp. 294–297. [Google Scholar]
- Hotta, M.; Norodin, N.S.A.; Zakaria, N.N.M.; Onari, H.; Takegami, T. Influence of lossy objects for resonator-coupled type wireless power transfer system with coplanar dual-spiral resonators. In Proceedings of the Asia-Pacific Microwave Conference APMC, Kyoto, Japan, 6–9 November 2018; pp. 40–42. [Google Scholar] [CrossRef]
- Campi, T.; Cruciani, S.; Maradei, F.; Feliziani, M. Magnetic shielding design of wireless power transfer systems. Annu. Rev. Prog. Appl. Comput. Electromagn. 2015, 2015, 13–14. [Google Scholar]
- Xue, R.; Cheng, K.; Je, M. High-Efficiency Wireless Power Transfer for Biomedical Implants by Optimal. IEEE Trans. Circuits Syst. I Regul. Pap. 2013, 60, 867–874. [Google Scholar] [CrossRef]
- Haus, H.A. Waves and fields in optoelectronics. In CEUR Workshop Proceedings; Prentice Hall: Hoboken, NJ, USA, 1984; p. 897. [Google Scholar]
- Wang, C.S.; Covic, G.A.; Stielau, O.H. Power transfer capability and bifurcation phenomena of loosely coupled inductive power transfer systems. IEEE Trans. Ind. Electron. 2004, 51, 148–157. [Google Scholar] [CrossRef]
- Hirayama, H.; Ozawa, T.; Hiraiwa, Y.; Kikuma, N.; Sakakibara, K. A consideration of electro-magnetic-resonant coupling mode in wireless power transmission. IEICE Electron. Express 2009, 6, 1421–1425. [Google Scholar] [CrossRef]
- IEC62233. Measurement methods for electromagnetic fields of household appliances and similar apparatus with regard to human exposure. Int. Electrotech. Comm. 2008, 3, 33–34. [Google Scholar]
- Gabriel, S.; Lau, R.W.; Gabriel, C. The dielectric properties of biological tissues: III. Parametric models for the dielectric spectrum of tissues. Phys. Med. Biol. 1996, 41, 2271–2293. [Google Scholar] [CrossRef] [PubMed]
- Pico, J.; Bechtold, T.; Hohlfeld, D. Model order reduction using COMSOL Multiphysics® Software—A compact model of a wireless power transfer system. In Proceedings of the 2016 COMSOL Conference, Munich, Germany, 12–14 October 2016; pp. 4–6. [Google Scholar]
- Kaufmann, C.; Günther, M.; Klagges, D.; Knorrenschild, M.; Richwin, M.; Schöps, S.; ter Maten, E.J.W. Efficient frequency-transient co-simulation of coupled heat-electromagnetic problems. J. Math. Ind. 2014, 4, 1–3. [Google Scholar] [CrossRef][Green Version]
- Tierney, B.B.; Grbic, A. Planar Shielded-Loop Resonators. IEEE Trans. Antennas Propag. 2014, 62, 3310–3320. [Google Scholar] [CrossRef]
- Laakso, I.; Hirata, A. Fast multigrid-based computation of the induced electric field for transcranial magnetic stimulation. Phys. Med. Biol. 2012, 57, 7753–7765. [Google Scholar] [CrossRef] [PubMed]
- Laakso, I.; Uusitupa, T.; Ilvonen, S. Comparison of SAR calculation algorithms for the finite-difference time-domain method. Phys. Med. Biol. 2010, 55, N421. [Google Scholar] [CrossRef] [PubMed]
- IEEE. Recommended practice for measurements and computations of radio frequency electromagnetic fields with respect to human exposure to Such Fields, 100 kHz–300 GHz. In IEEE Std C95.3-2002 (Revision of IEEE Std C95.3-1991); IEEE: New York, NY, USA, 2002; p. i-126. [Google Scholar] [CrossRef]
- Norodin, N.S.A.; Nakamura, K.; Hotta, M. Effects of Lossy Mediums for Resonator-Coupled Type Wireless Power Transfer System using Conventional Single- and Dual-Spiral Resonators. IEICE Trans. Electron. 2021, E105-C. [Google Scholar] [CrossRef]
- Awai, I.; Zhang, Y.; Komori, T.; Ishizaki, T. Coupling coefficient of spiral resonators used for wireless power transfer. In Proceedings of the Asia-Pacific Microwave Conference APMC, Yokohama, Japan, 7–9 December 2010; pp. 1328–1331. [Google Scholar]
- Christ, A.; Douglas, M.G.; Roman, J.M.; Cooper, E.B.; Sample, A.P.; Waters, B.H.; Smith, J.R.; Kuster, N. Evaluation of Wireless Resonant Power Transfer Systems With Human Electromagnetic Exposure Limits. IEEE Trans. Electromagn. Compat. 2013, 55, 265–274. [Google Scholar] [CrossRef]
- Rašić, P.; Škiljo, M.; Blažević, Z.; Dorić, V.; Poljak, D. Simulation of human body exposure to near field of high and low RF wireless power transfer systems. Int. J. Eng. Model. 2020, 33, 19–36. [Google Scholar] [CrossRef]

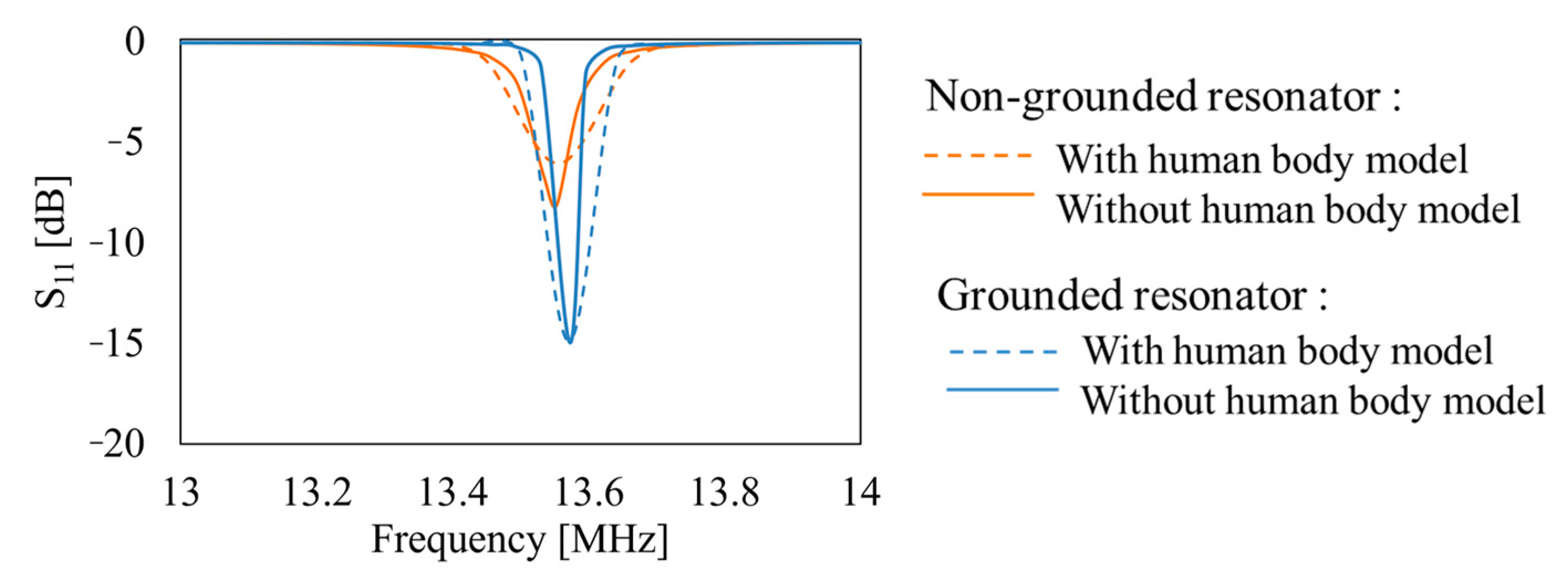
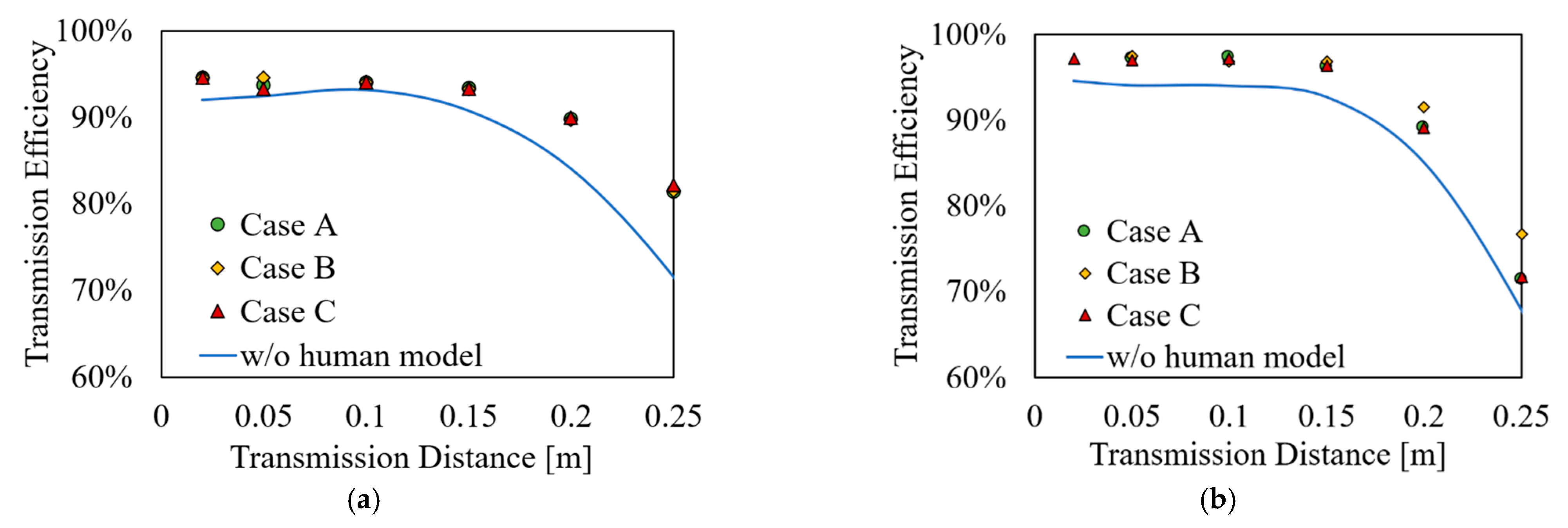
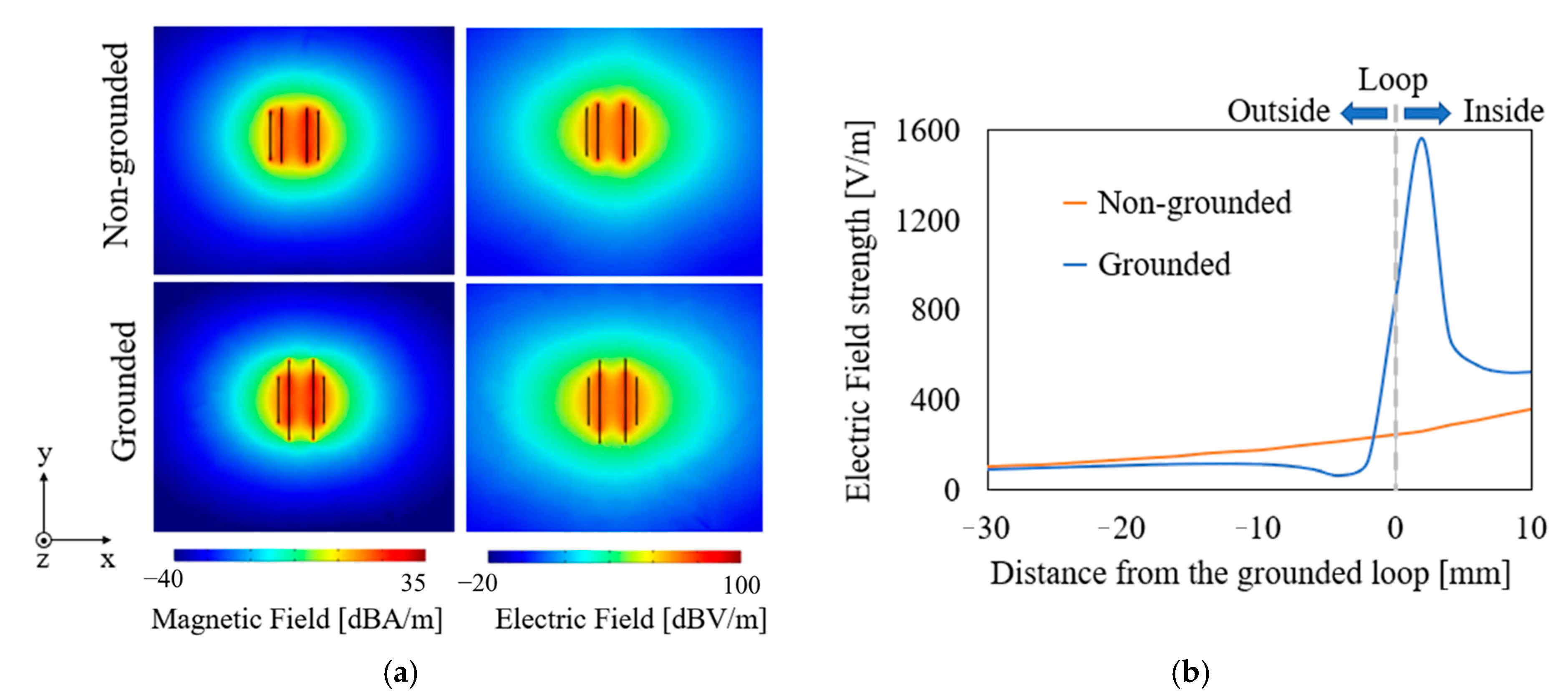
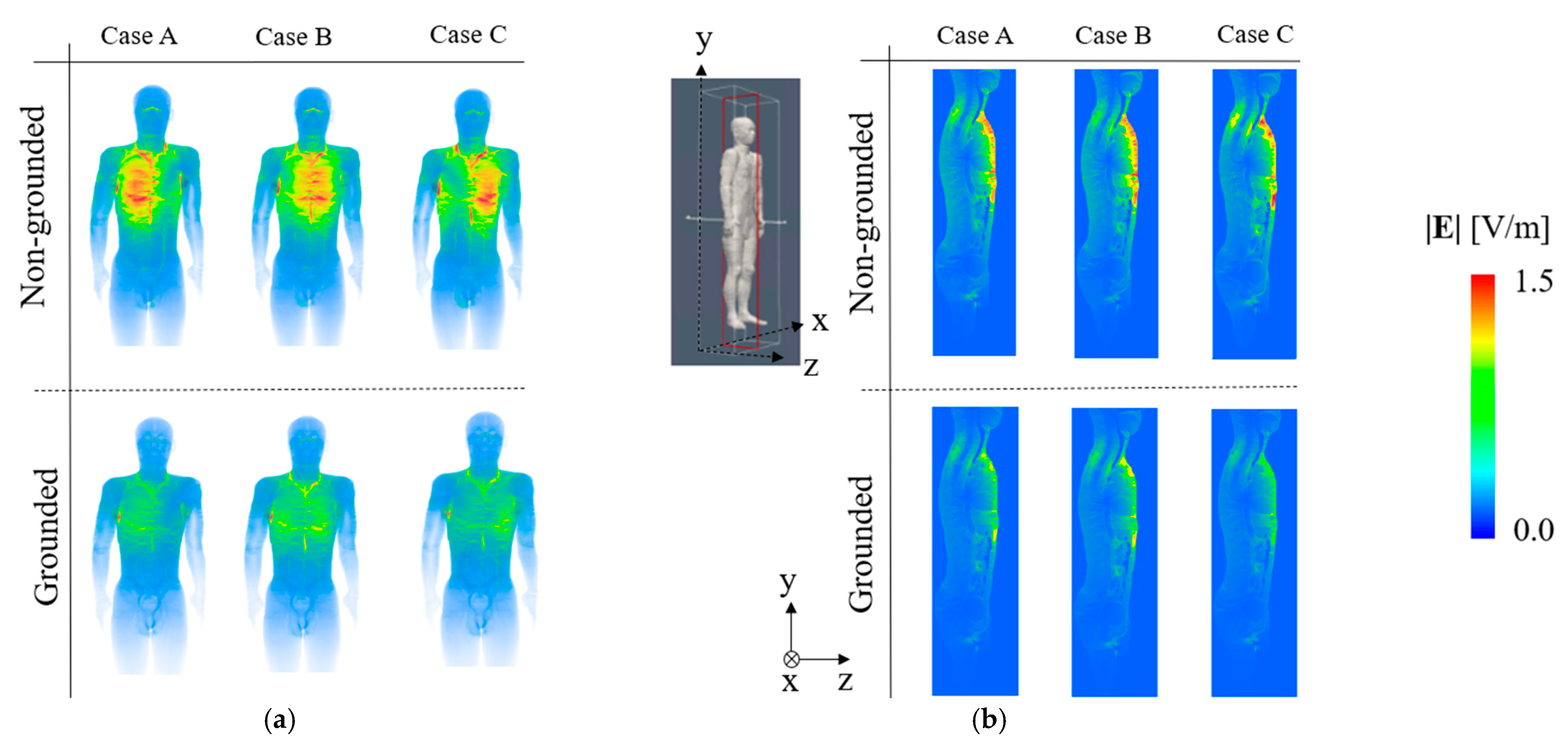
| Resonator Type | Frequency (MHz) | Without Human Body | With Human Body |
|---|---|---|---|
| Non-grounded | 13.55 | −8.16 | −6.06 |
| Grounded | 13.57 | −14.97 | −14.10 |
| Resonator | Quality Factor | Coupling Coefficient | ||
|---|---|---|---|---|
| Without Human Body | With Human Body | Without Human Body | With Human Body | |
| Non-grounded | 215.4 | 222.7 | 0.128 | 0.144 |
| Grounded | 234.2 | 235.3 | 0.146 | 0.198 |
| Model Displacement | Non-Grounded | Grounded | RD |
|---|---|---|---|
| Case A | 3.44 | 1.94 | 43.6% |
| Case B | 3.22 | 2.02 | 37.3% |
| Case C | 2.98 | 1.85 | 37.9% |
| Model Displacement | Non-Grounded | Grounded | RD |
|---|---|---|---|
| Case A | 1.88 × 10−4 | 0.57 × 10−4 | 69.7% |
| Case B | 2.04 × 10−4 | 0.67 × 10−4 | 67.1% |
| Case C | 1.35 × 10−4 | 0.50 × 10−4 | 63.0% |
| Model Displacement | Non-Grounded | Grounded | RD |
|---|---|---|---|
| Case A | 8.34 × 10−6 | 2.87 × 10−6 | 65.6% |
| Case B | 7.94 × 10−6 | 3.48 × 10−6 | 56.1% |
| Case C | 6.12 × 10−6 | 2.39 × 10−6 | 61.0% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Duan, X.; Lan, J.; Diao, Y.; Gomez-Tames, J.; Hirayama, H.; Hotta, M.; Fischer, G.; Hirata, A. Reduction in Human Interaction with Magnetic Resonant Coupling WPT Systems with Grounded Loop. Energies 2021, 14, 7253. https://doi.org/10.3390/en14217253
Duan X, Lan J, Diao Y, Gomez-Tames J, Hirayama H, Hotta M, Fischer G, Hirata A. Reduction in Human Interaction with Magnetic Resonant Coupling WPT Systems with Grounded Loop. Energies. 2021; 14(21):7253. https://doi.org/10.3390/en14217253
Chicago/Turabian StyleDuan, Xianyi, Junqing Lan, Yinliang Diao, Jose Gomez-Tames, Hiroshi Hirayama, Masashi Hotta, George Fischer, and Akimasa Hirata. 2021. "Reduction in Human Interaction with Magnetic Resonant Coupling WPT Systems with Grounded Loop" Energies 14, no. 21: 7253. https://doi.org/10.3390/en14217253
APA StyleDuan, X., Lan, J., Diao, Y., Gomez-Tames, J., Hirayama, H., Hotta, M., Fischer, G., & Hirata, A. (2021). Reduction in Human Interaction with Magnetic Resonant Coupling WPT Systems with Grounded Loop. Energies, 14(21), 7253. https://doi.org/10.3390/en14217253








