The Distribution of the Thermal Field in an Elliptical Electric Conductor Coated with Insulation
Abstract
:1. Introduction
- To determine the stationary distribution of the temperature field in an elliptical conductor coated with insulation;
- To determine the electrical current-carrying capacity (steady-state current rating) of the above-mentioned conductor;
- To develop the analytical–numerical method of analyzing the thermal field in a layered system with different material parameters and Hankel’s condition.
2. Physical and Mathematical Model of the System
- The analysis pertains to a steady state (
- Thermal conduction values for the core λ1 and insulation λ2 are constant (λi = const =>
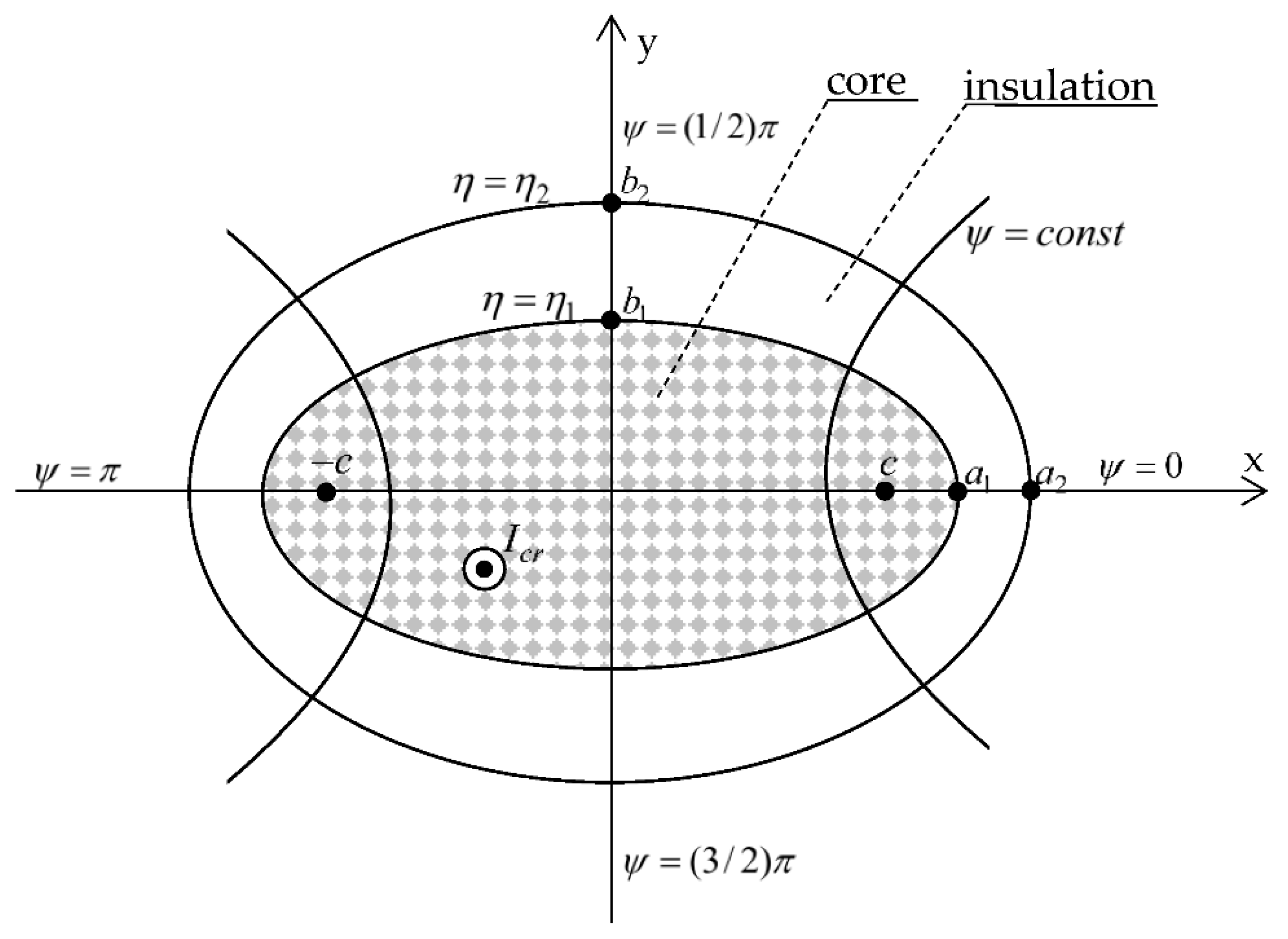
- An elliptic–cylindrical coordinate system is used M(η,ψ,z);
- The length of the conductor is much greater than that of the major axis of the ellipse (l >> 2a2 => νi(M) = νi(η,ψ) − case 2D).
3. Solution of the Boundary Problem
4. Calculation Example
- Numerical computation of the integrals in Equations (22)–(28) and the iterative solution of the system of Equations (20) and (21) with a dense matrix of coefficients: 97%;
- Summation of Equations (12) and (16), conversion of the elliptical coordinates into the Cartesian coordinates, and the visualization of the results: 3%.
5. Numerical Verification of the Solution
6. Final Remarks
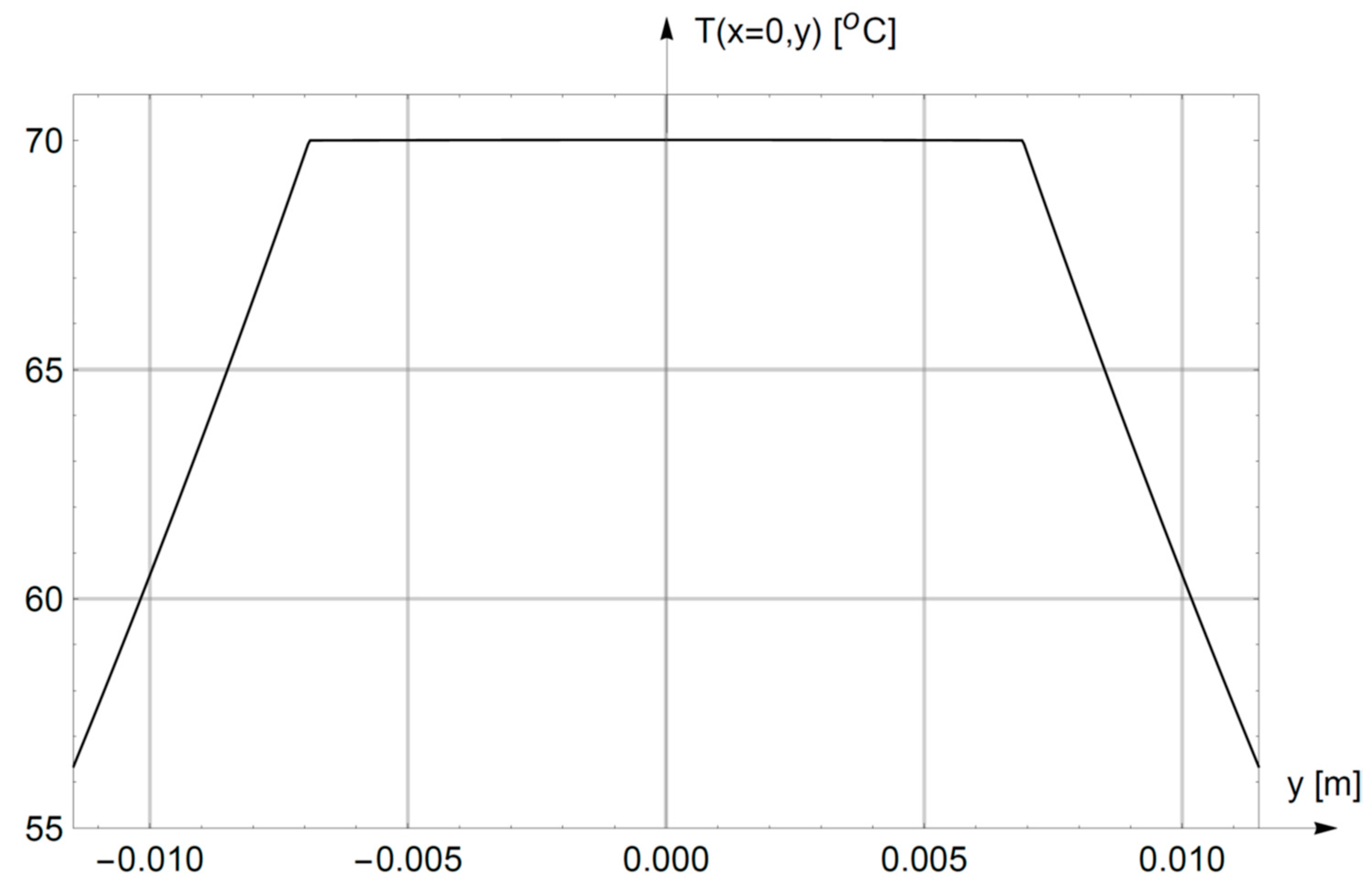
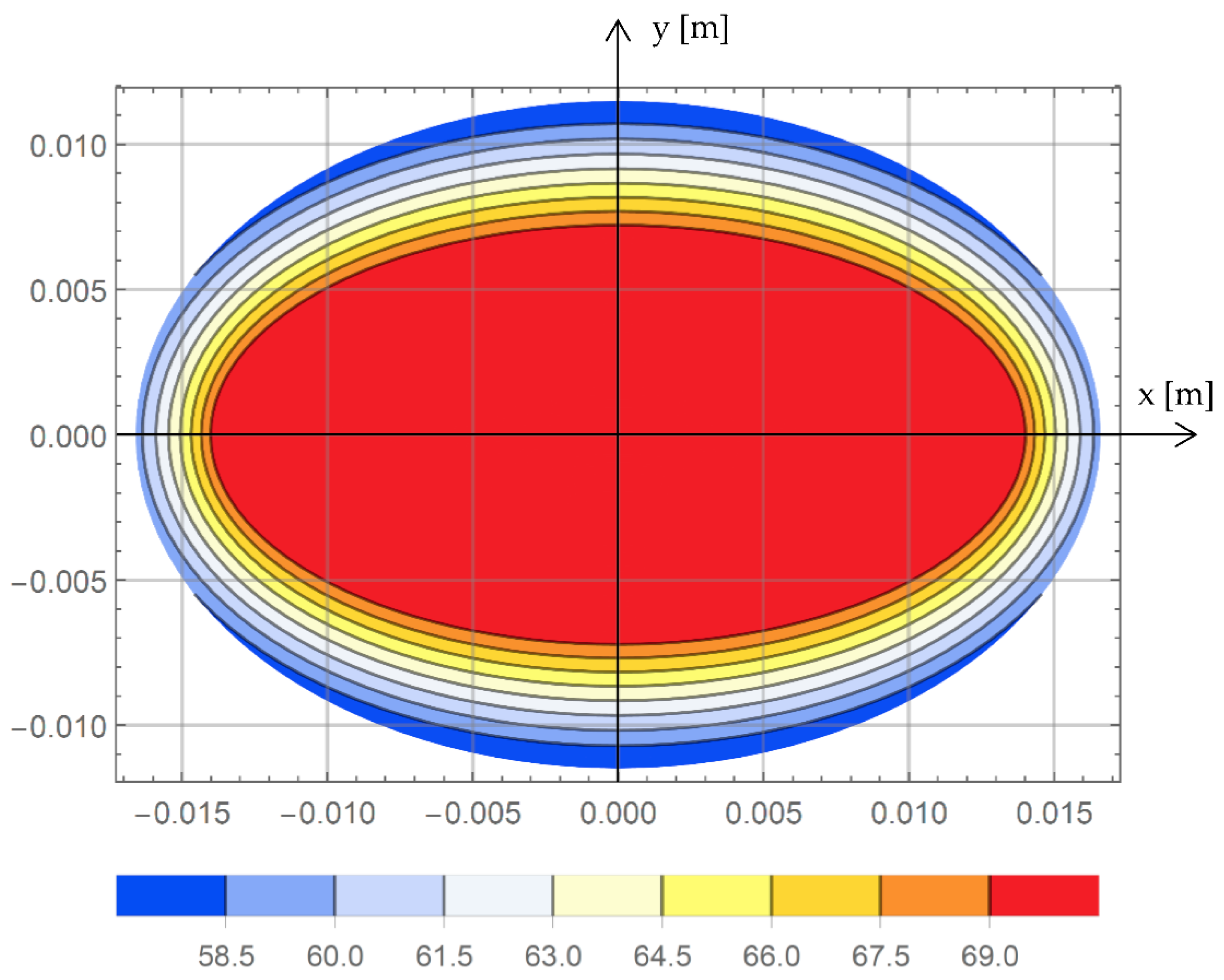
- The thermal field in the core (0 ≤ η ≤ η1) is almost uniform, as can be seen in Figure 2, Figure 3 and Figure 4. The maximum temperature drop in the core is merely T1(η = 0, ψ = π/2) − T1(η = η1, ψ = 0) = 0.034 °C. The physical cause of the above-mentioned phenomenon is the very large equivalent thermal conductivity λ1 of the porous system (aluminum alloy-air) with a packing of (S1/πa1b1) ≈ 0.9;
- Distinct temperature drops occur in PVC insulation (η1 ≤ η ≤ η2), as can be seen in Figure 2, Figure 3 and Figure 4. This temperature drop increases with the thickness of the insulation {(b2 − b1) ≈ 4.57 mm > (a2 − a1) ≈ 2.76 mm} = >{[T2(η = η1, ψ = π/2) − T2(η = η2, ψ = π/2)] = 13.66 °C >> [T2(η = η1, ψ = 0) − T2(η = η2, ψ = 0)] = 10.676 °C}—see Figure 1. The above results from the fact that the thermal resistance of the insulation increases with an increase in its thickness, as well as from Ohm’s thermal law [22]. The temperature drops are nearly linear (Figure 2 and Figure 3);
- The perimeters of the core (η = η1) and the insulation (η = η2) are not isotherms. For η = η1, the temperature changes from T1(η1,ψ = 0) = 69.976 °C to T1(η1,ψ = π/2) = 70 °C, while for η = η2 it changes from T2(η2,ψ = π/2) = 56.34 °C to T2(η2,ψ = 0) = 59.3 °C. The above is due to a change in the distance of points on the perimeters η = η1 and η = η2 from the center of the heat source (η = 0, ψ = π/2). Another cause is the variation in insulation thickness with the coordinate ψ;
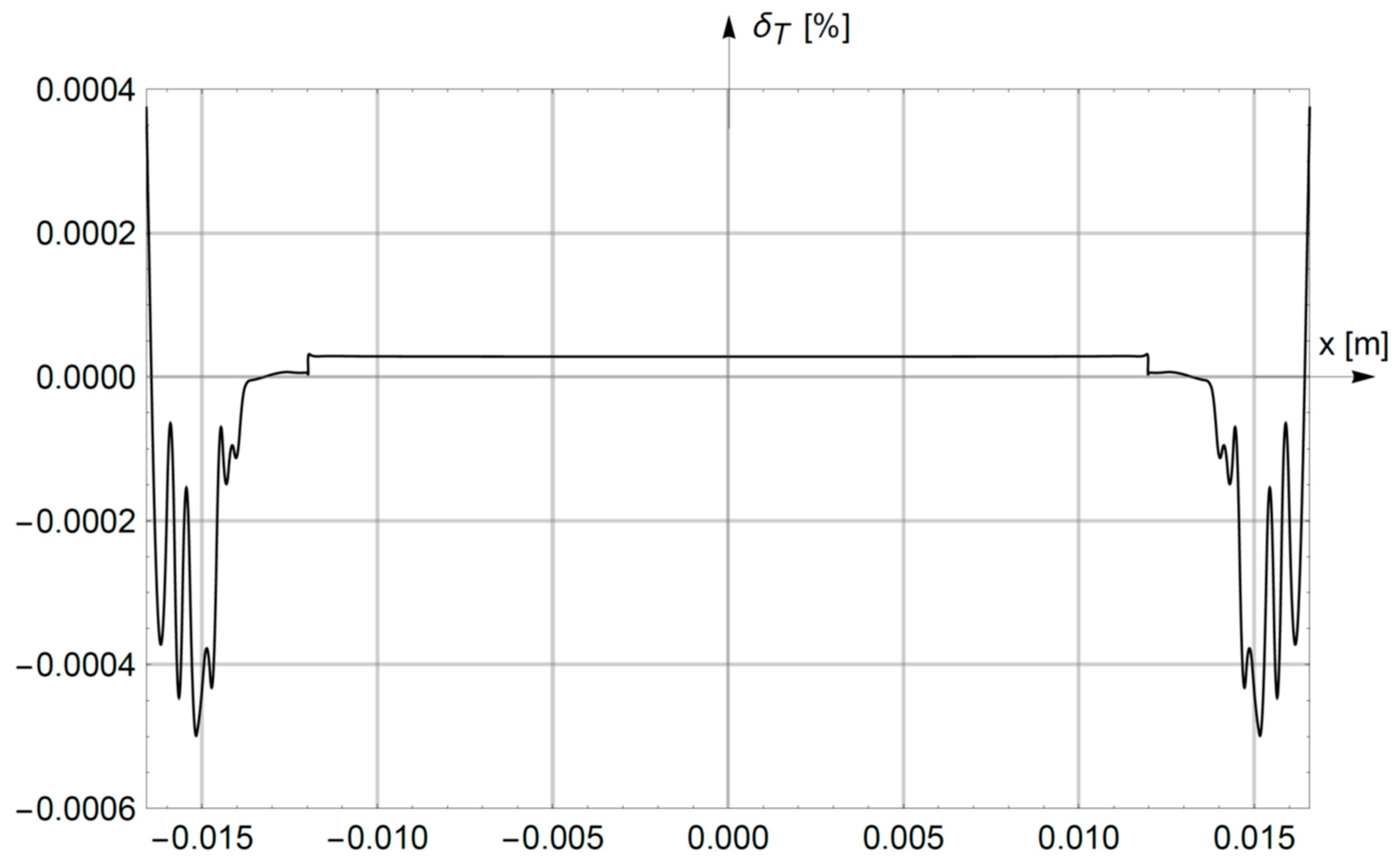
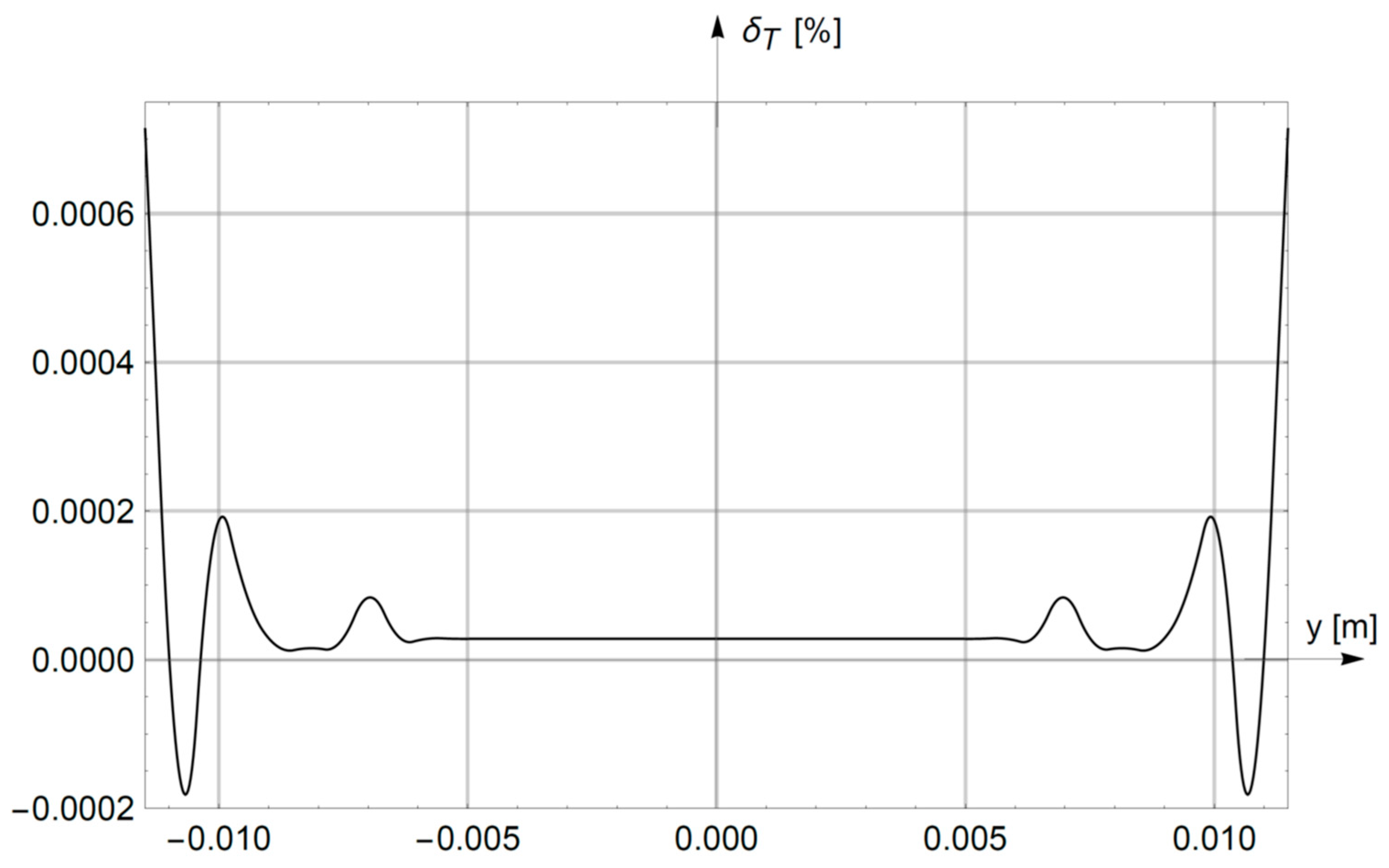
- The relative differences between the temperature distributions in Equation (32) (calculated using the analytical–numerical (AN) method and the finite-element (FE) method) are very small. On the major axis y = 0, the module (32) is smaller than 0.0005% (Figure 5), while on the minor axis x = 0, |δT2| < 0.00075% is true (Figure 6). The smallest differences in Equation (32) are found in the region of uniform field (i.e., in the core). Slightly greater deviations δT2 are observed in the area of temperature drop (i.e., in the insulation); this results from the inequality λ1 >> λ2. Due to the small values of relative differences in Equation (32), the developed AN method leads to practically the same results as the commonly used numerical FE method.
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
List of Symbols
| A0 | Constant in Equation (12) |
| An | Coefficient in Equation (12) |
| a1 | Major semi-axis of the ellipse η = η1 (core surface)—Figure 1 |
| a2 | Major semi-axis of the ellipse η = η2 (insulation surface)—Figure 1 |
| b1 | Minor semi-axis of the ellipse η = η1 (core surface)—Figure 1 |
| b2 | Minor semi-axis of the ellipse η = η2 (insulation surface)—Figure 1 |
| C0, D0, F0, G0 | Constants in Equation (10) |
| c | Abscissa of the focus |
| ci | Specific heat (i = 1 for core, i = 2 for insulation) |
| En, Γn, Kn, Ln | Coefficients in Equation (10) |
| f(n) | Discrete function defined by Equation (17) |
| gi | Efficiency of heat sources (i = 1 for core, i = 2 for insulation) |
| g(n) | Discrete function defined by Equation (18) |
| H0, J0, W0, S0 | Constants in Equation (11) |
| h(m,n) | Integral defined by Equation (28) |
| I1(m,n), I2(m), I3(n), I4 | Integrals defined by Equations (22)–(27) |
| |I| | Root-mean square (RMS) current |
| Icr | Steady-state current rating |
| kn | Skin factor |
| ks | Stranding factor |
| l | Length of the conductor segment |
| N | Number of summed terms of Equations (12) and (16) |
| n | Summation index |
| P | Thermal power |
| P0, R0 | Constants in Equation (13) |
| Pn,Zn,Xn,Yn | Coefficients in Equation (11) |
| Qn, Λn | Coefficients in Equation (13) |
| Heat flux vector | |
| RAC | Alternating-current resistance |
| RDC | Direct-current resistance |
| r(n) | Discrete function defined by Equation (29) |
| S1 | Sum of the cross-sections of the conductive bundles (active cross-section) |
| Ta | Ambient temperature |
| Tmax | Maximum operating temperature |
| V | Volume of the l-length core segment |
| Ti(..,..) | Temperature distributions (i = 1 for core, i = 2 for insulation) |
| α | Total heat transfer coefficient |
| αc | Convective heat transfer coefficient |
| αr | Radiation heat transfer coefficient |
| δTi | Relative differences in temperature increments defined by Equation (32) |
| η1 | Perimeter of the core (η = η1) |
| η2 | Perimeter of the insulation (η = η2) |
| (η,ψ) | Coordinates of an elliptical–cylindrical system |
| λi | Thermal conductivity (i = 1 for core, i = 2 for insulation) |
| μi | Mass density (i = 1 for core, i = 2 for insulations) |
| νi(..,..) | Temperature increase obtained using the AN method (i = 1 for core, i = 2 for insulation) |
| Temperature increase calculated using the FE method (i = 1 for core, i = 2 for insulation) | |
| ρ(Tmax) | Resistivity of the core at the maximum operating temperature |
| Unit vector normal to the surface η = const | |
| Scalar Laplace operator |
References
- Morgan, V.T. The current distribution, resistance and internal inductance of linear power system conductors—A review of explicit equations. IEEE Trans. Power Deliv. 2013, 38, 1252–1262. [Google Scholar] [CrossRef]
- 3M Electrical Product Division. 3MTM Heat Shrinkable Tubing for Bus Bar BBI-A Series 5–35 kV; 3M Inc.: Austin, Texas, USA, 2013. [Google Scholar]
- ABB Catalogue Heat Shrink. Heatshrink Solutions Insulate, Identify and Protect Your Wires and Cables; ABB Inc.: London, UK, 2016. [Google Scholar]
- Eid, E.I.; Abdel-Halim, M.; Easa, A.S. Effect of opposed eccentricity on free convective heat transfer trough elliptical annulus enclosures in blunt and slender orientations. Heat Mass Transf. 2015, 51, 239–250. [Google Scholar] [CrossRef]
- Dragoni, M.; Tallarico, A. Temperature field and heat flow around an elliptical lava tube. J. Volcanol. Geotherm. Res. 2008, 169, 145–153. [Google Scholar] [CrossRef]
- Anders, G.J. Rating of Electric Power Cables: Ampacity Computations for Transmission, Distribution and Industrial Application; McGraw-Hill Professional: New York, NY, USA, 1997. [Google Scholar]
- IEEE Std. 738-2012. IEEE Standard for Calculating the Current-Temperature Relationship of Bare Overhead Conductors; IEEE Standard Association: Piscataway Township, NJ, USA, 2013. [Google Scholar]
- CIGRE Working Group B2.42. Guide for Thermal Rating Calculations of Overhead Lines, Technical Brochure 601; CIGRE: Paris, France, 2014. [Google Scholar]
- Shahmardan, M.M.; Norouzi, M.; Sedaghat, M.H. An exact analytical solution for convective heat transfer in elliptical pipes. AUT J. Mech. Eng. 2017, 1, 131–138. [Google Scholar]
- Maia, C.R.M.; Aperecido, J.B.; Milanez, L. F Heat transfer in laminar flow of non-Newtonian fluids in ducts of elliptical section. Int. J. Therm. Sci. 2006, 45, 1066–1072. [Google Scholar] [CrossRef]
- Erdoğdu, F.; Bulaban, M.O.; Chau, K.V. Modeling of heat conduction in elliptical cross section: I. Development and testing of the model. J. Food Eng. 1998, 38, 223–239. [Google Scholar] [CrossRef]
- Erdoğdu, F.; Bulaban, M.O.; Chau, K.V. Modeling of heat conduction in elliptical cross section: II. Adaptation to thermal processing of shrimp. J. Food Eng. 1998, 38, 241–258. [Google Scholar] [CrossRef]
- Hermany, L.; Lorenzini, G.; Klein, R.J.; dos Santos, E.D.; Isoldi, L.A.; Rocha, L.A.O. Constructal design applied to elliptic tubes in convective heat transfer cross-flow of viscoplastic fluids. Int. J. Heat Mass Transf. 2018, 116, 1054–1063. [Google Scholar] [CrossRef]
- Nag, P.; Molla, M.M.; Hossain, M.A. Non-newtonian effect on natural convection flow over cylinder of elliptic cross section. Appl. Math. Mech. 2020, 41, 361–382. [Google Scholar] [CrossRef]
- Sakr, R.Y.; Berbish, N.S.; Abd-Alziz, A.A.; Hanafi, A.S. Experimental and numerical investigation of natural convection heat transfer in horizontal elliptic annuli. J. Appl. Sci. Res. 2008, 4, 138–155. [Google Scholar] [CrossRef]
- Hoseinzadeh, S.; Sohani, A.; Ashrafi, T.G. An artificial intelligence-based predition way to describe flowing a Newtonian liquid/gas on a permeable flat surface. J. Therm. Anal. Calorim. 2021, 1, 1–7. [Google Scholar]
- Hoseinzadeh, S.; Sohani, A.; Shahverdian, M.H.; Shirkhani, A.; Heyns, S. Acquiring an analytical solution and performing a comparative sensitivity analysis for flowing Maxwell upper-convected fluid on a horizontal surface. Therm. Sci. Eng. Prog. 2021, 23, 100901. [Google Scholar] [CrossRef]
- Nield, D.A.; Bejan, A. Convection in Porous Media; Springer-Verlag: New York, NY, USA, 2013. [Google Scholar]
- Latif, M.J. Heat Conduction; Springer-Verlag: Haidelberg, Germany, 2009. [Google Scholar]
- Hahn, D.W.; Ozisik, M.N. Heat Conduction; John Wiley & Sons: Hoboken, NJ, USA, 2012. [Google Scholar]
- Taler, J. Solving Direct and Inverse Heat Conduction Problems; Springer-Verlag: Berlin, Germay, 2016. [Google Scholar]
- Bergman, T.L.; Lavine, A.S.; Incropera, F.P.; Dewitt, D.P. Fundamentals of Heat and Mass Transfer; John Wiley and Sons: Hoboken, NJ, USA, 2011. [Google Scholar]
- Evans, L.C. Partial Differential Equations; American Mathematical Society: Providence, RI, USA, 2010. [Google Scholar]
- Moon, P.; Spencer, D.E. Field Theory Handbook; Springer-Verlag: Berlin, Germany, 1988. [Google Scholar]
- Abramowitz, A.; Stegun, I.A. Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables; Dover Publications, Inc.: New York, NY, USA, 1972. [Google Scholar]
- Wolfram Research Inc. Mathematica; Wolfram Research Inc.: Champaign, IL, USA, 2020. [Google Scholar]
- Nithiarasu, P.; Lewis, R.W.; Seetharamu, K.N. Fundamentals of the Finite Element Method for Heat and Mass Transfer; John Wiley and Sons: Chichester, UK, 2016. [Google Scholar]
- Brener, S.; Scott, R.L. The Mathematical Theory of Finite Element Method; Springer: Berlin, Germany, 2008. [Google Scholar]
- COMSOL Multiphysics. Documentation for COMSOL; Release 4.3; Comsol Inc.: Stockholm, Sweden, 2013. [Google Scholar]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gołębiowski, J.; Zaręba, M. The Distribution of the Thermal Field in an Elliptical Electric Conductor Coated with Insulation. Energies 2021, 14, 6880. https://doi.org/10.3390/en14216880
Gołębiowski J, Zaręba M. The Distribution of the Thermal Field in an Elliptical Electric Conductor Coated with Insulation. Energies. 2021; 14(21):6880. https://doi.org/10.3390/en14216880
Chicago/Turabian StyleGołębiowski, Jerzy, and Marek Zaręba. 2021. "The Distribution of the Thermal Field in an Elliptical Electric Conductor Coated with Insulation" Energies 14, no. 21: 6880. https://doi.org/10.3390/en14216880
APA StyleGołębiowski, J., & Zaręba, M. (2021). The Distribution of the Thermal Field in an Elliptical Electric Conductor Coated with Insulation. Energies, 14(21), 6880. https://doi.org/10.3390/en14216880




