A DCM Single-Controlled Three-Phase SEPIC-Type Rectifier

Abstract

A discontinuous conduction mode (DCM) three-phase single-ended primary-inductor converter (SEPIC) is presented in this article. The analyzed converter operates as a high-power factor stage in AC–DC conversion systems. As its main features, it presents three controlled switches and a single control signal with simple implementation and low-current harmonic distortion. The converter topology, its design equations, and its operation modes are presented as well as a simulation analysis considering a 3 kW–220 V three-phase input to 400 V DC output converter. The experimental results are included, considering as an application the rectifier stage in low-power wind energy conversion systems (WECS) based on a 1 kW permanent magnet synchronous generator (PMSG) with variable voltage frequencies. From the analysis performed in the paper and the simulation and experimental results revealed, it is concluded that the converter is indicated to be employed in any AC–DC low-power conversion system, such as DC distribution systems, and distributed generation or hybrid systems containing variable-frequency generation.

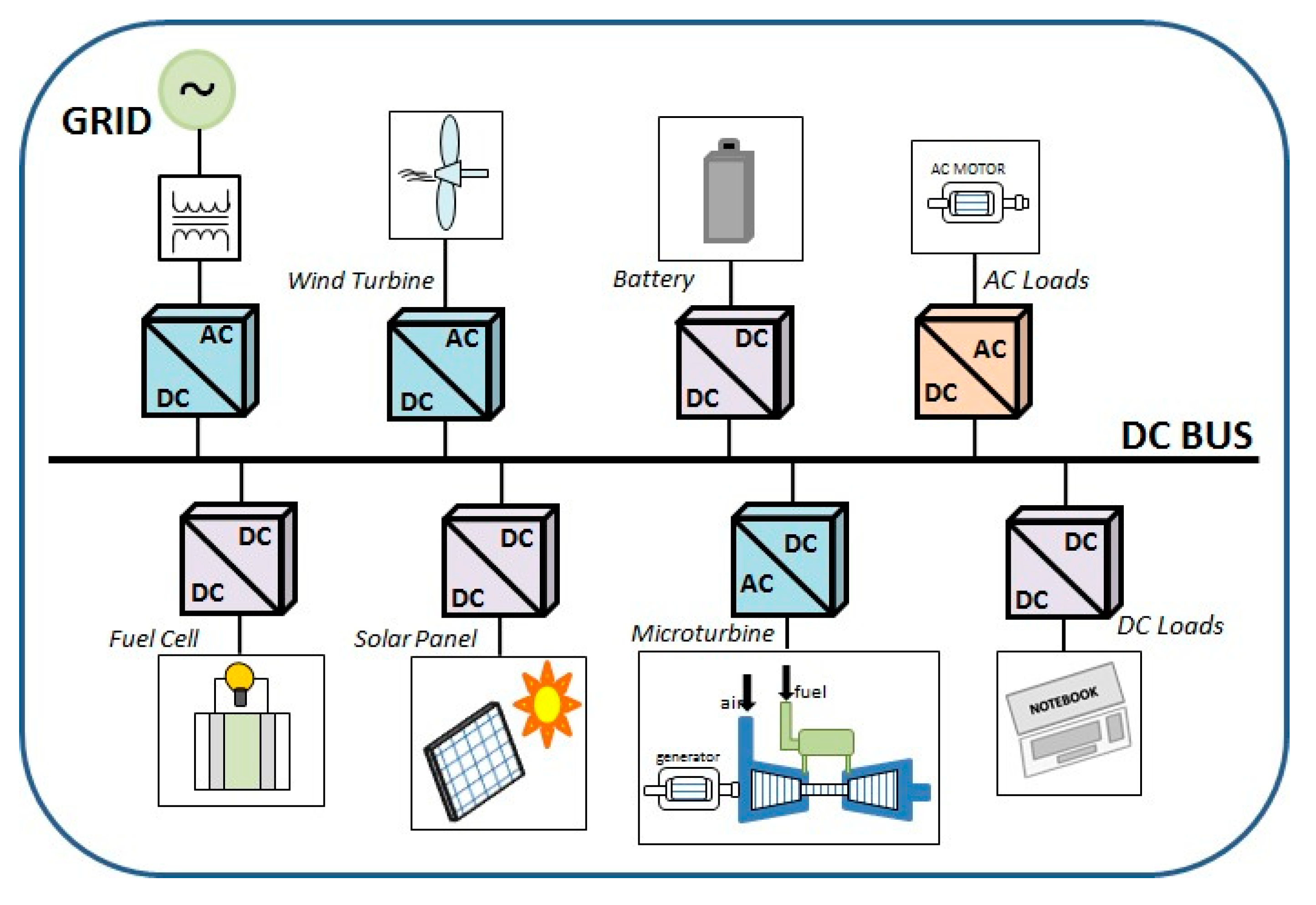
1. Introduction

AC–DC conversion is present in much modern electronic equipment. Widespread distribution systems are AC, and consumer goods are increasingly employing DC sources to feed even AC loads, such as washing machines and air conditioners.

In order to not disturb grid voltage, the current waveform should present restricted harmonic content. The behavior of an AC–DC stage must approach a perfect linear load. High-power-factor rectifiers are AC–DC converters that present low total harmonic distortion (THD).

Even for a DC microgrid, which has received great attention in recent years [1,2,3,4,5,6], AC–DC conversion can perform important tasks. A typical configuration of such an approach is shown in Figure 1. It is noticed that it encompasses some renewable electrical and conventional resources as well as an AC grid connection. Microturbines and wind turbines also require AC–DC conversion.

For a three-phase source, many topologies are presented in the literature. Six controlled switch converters are effective for high power levels, but when it comes to systems with lower power levels, where only unidirectional power flow is required, rectifiers with a reduced number of active elements (single or three controlled switches) operating in the DCM are usually preferred, and the three-phase boost is the most used [7,8,9,10,11,12]. A variety of solutions has recently arisen [13,14,15,16,17,18,19,20,21,22]. In the analysis of the power conversion from the grid to DC bus, the input RMS voltage is almost constant, maintained in the range given by standards. The design of the control loop focuses on the DC bus voltage.

On the other hand, in wind power generation systems, the output power is related to wind speed. Variable-speed PMSG-based microwind turbines at the sub-kW level have been employed for residential and small commercial buildings, where for battery charging, an isolated converter is required for grid interfacing [23].

A simple, cost-effective, and higher-power-density solution applied to low power with uncontrolled devices is presented in the literature, such as [24], where discontinuous conduction mode (DCM) rectification is performed by a bridge rectifier diode. A configuration where a rectifier diode plus a DC–DC boost converter is used in the DCM is presented by the authors of [25,26], and the author of [27] presents a configuration operating in the continuous conduction mode (CCM). This solution, however, presents a typical distorted generator current waveform that leads to a pulsating generator active power. An advantage of this structure reported by the authors of [28] is to control the power provided by the PMSG through a single PWM signal, which negatively does not allow a separated i_d and i_q control and extraction of the maximum power of the generator. Some drawbacks have been reported [29], mainly due to high harmonic current distortions in the generator windings, such as: increasing heating, reduction in machine efficiency, and the production of high-torque ripples due to the use of a diode-bridge rectifier [30].

To alleviate these problems, DCM boost rectifiers have been considered [7,25,31,32,33]. For operation in DCM, an input filter is required for smoothing the high-frequency input current. These solutions still present an important level of low-order harmonics flowing into the generator, resulting in a pulsating power of low frequency, causing power fluctuations, as shown in Figure 2a,b, which show one phase input voltage and current after filtering and electric power drained from the source, respectively. To effectively use available wind energy, the current drawn from the generator should be an image of the generated voltage.

This work presents a high-power-factor DCM rectifier topology able to be used in the grid-connected rectification stage as well as the interface topology of low-power AC generators. The converter employs three controlled switches but only one single gate signal and has as a great advantage the ability to drain a current from the AC source that is an image of the voltage despite the input voltage value, with no current control loop and low-current ripple, absorbing almost constant electric power from the AC source. A differential achievement is that the converter can operate with an output voltage value near the input voltage (peak line value), bringing low-torque oscillations to the machine and almost constant output power.

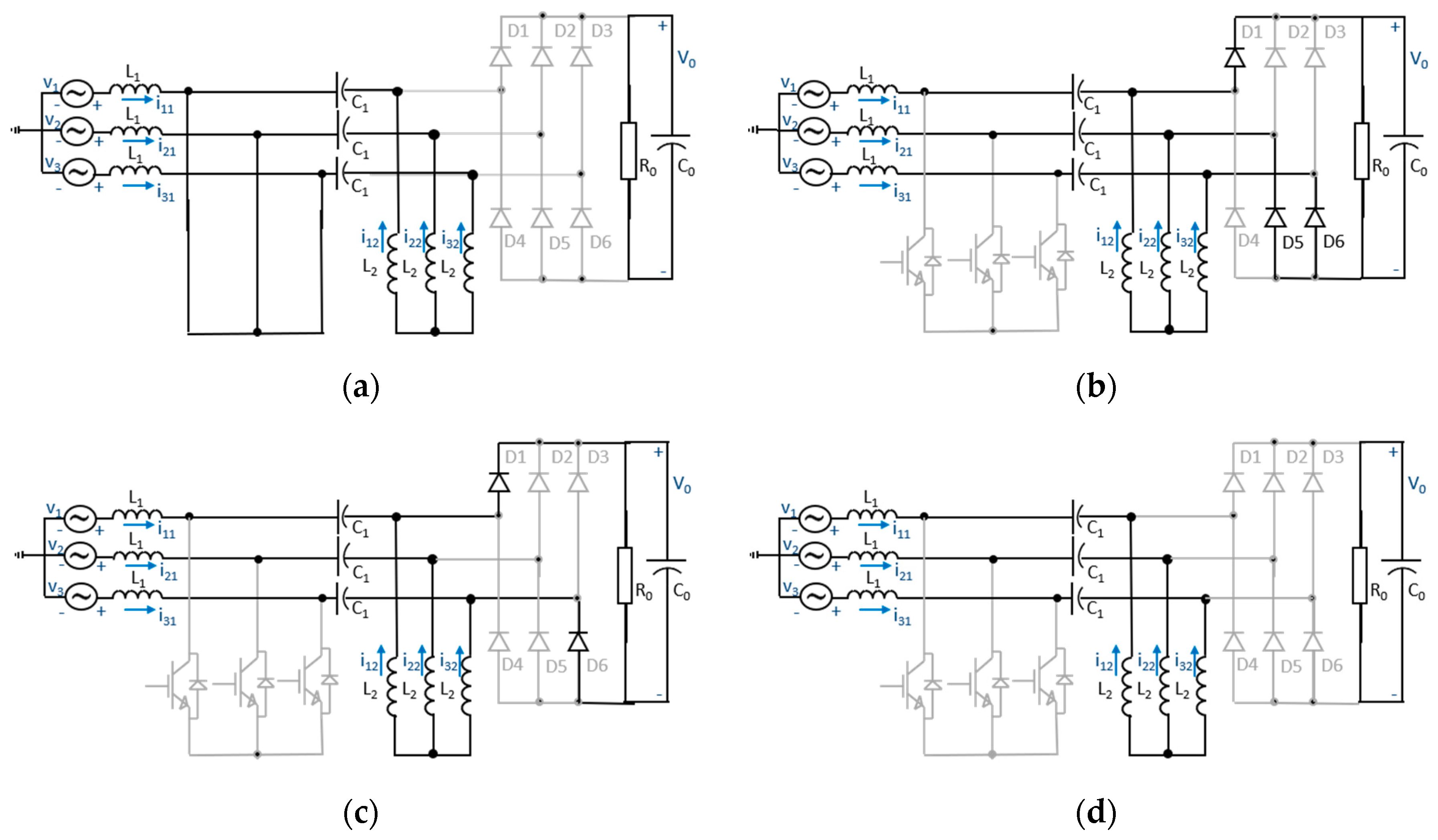
2. Proposed Converter

The proposed converter is shown in Figure 3. The generator inductance is incorporated as its input inductor, represented with the same inductance L₁ for each phase since the converter is a symmetrical three-phase topology. It uses three IGBTs plus reverse diodes (or three MOSFETs). Thus, conduction losses, proportional to the voltage drop in the current path, are reduced. This is an important improvement during this stage, especially at low power.

The topology operates with an output voltage greater than the peak of the line input voltage. The three controlled switches (IGBTs with antiparallel diodes or MOSFETs) are connected to a common point and driven by the same signal. One single gate control is required, reducing its complexity and providing greater robustness to the conversion system. The three-phase SEPIC-type rectifier is indicated for applications where the output voltage is higher but close to the peak voltage in the condition that maximum voltage is generated.

A remarkable feature of this converter is that it provides an input current in phase with the voltage, presenting a high power factor and low harmonic content, as will be detailed further. A notable advantage in this regard is that in order to operate with a high-power factor, there is no need to control the input currents once they naturally remain in phase with the input voltage.

From the point of view of the AC source, the SEPIC-type topology acts as a three-phase loss-free resistor [34]. Compared with commonly used topologies, such as the six controlled switches topology mentioned before, which, in order to operate with a power factor close to the unit value, the converter requires a closed-loop current control, the proposed converter presents an excellent benefit. Another advantage of the proposed converter is the reduction in cost since the number of active elements is smaller (compared with topologies with a similar power level using six controlled switches).

2.1. SEPIC-Type Rectifier Operation Principle

In the following analysis, the AC source is considered, generating three symmetrical voltages: v₁, v₂, and v₃ (positive sequence with v₁ as reference). Input voltages as well as the voltages in series capacitors (three capacitors with the same capacitance C₁ because of the symmetrical three-phase topology) are considered constant in a switching period, and the voltages in these capacitors follow the respective phase voltages over a period. The operation of this converter can be understood by dividing its operation into three stages: connected switches, disconnected switches, and freewheeling operation. Each of these steps is qualitatively described below in the interval from 0° to 30° (v₁ > 0, v₂ < 0, v₃ < 0).

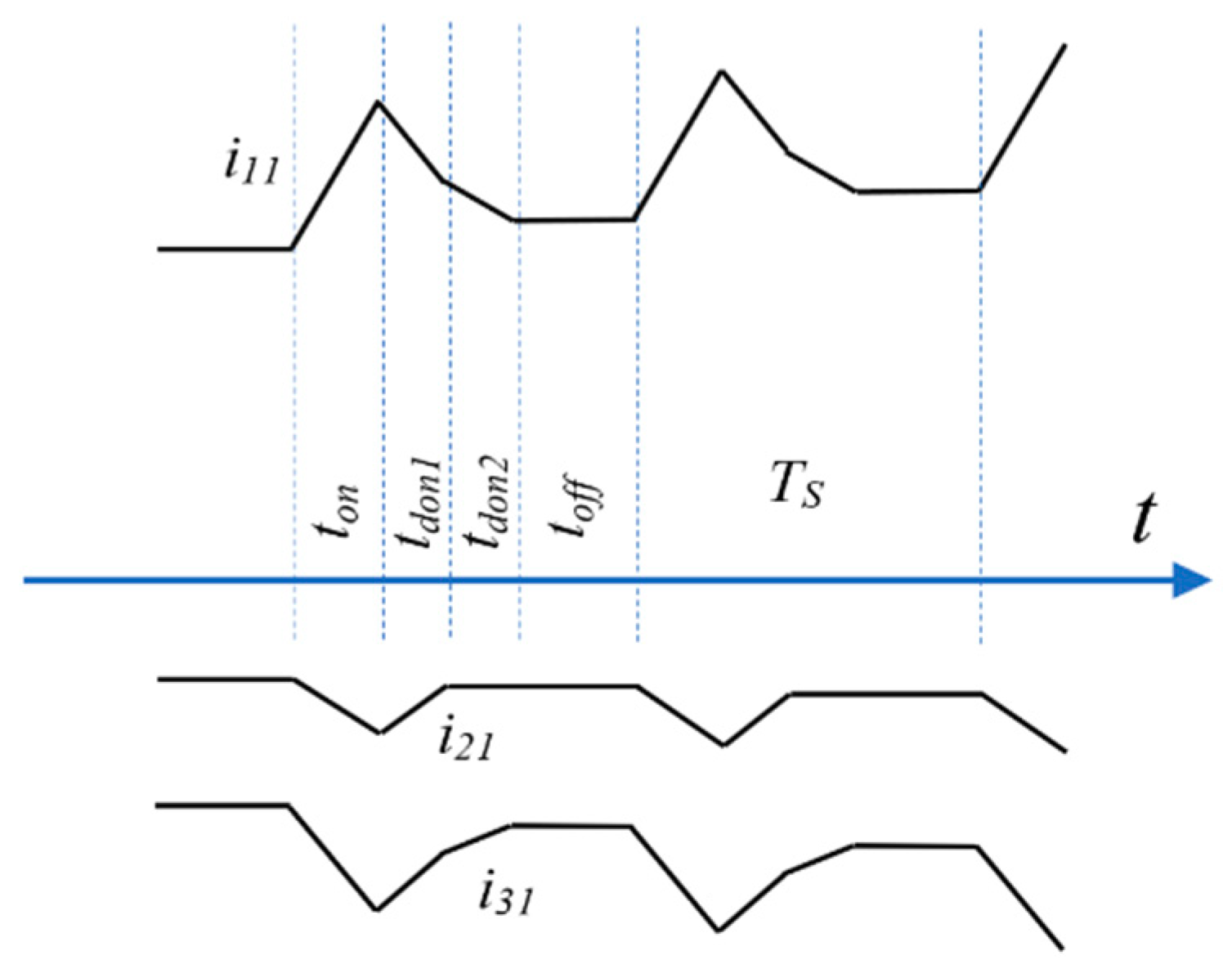
2.1.1. First Stage—Switches Turned on (t_on)

During turned-on time (t_on), the same trigger signal is applied to all three switches. The resulting circuit is shown in Figure 4a. The phase voltages are applied to the inductors since the voltages in the capacitors follow the input voltages. For positive voltages, the currents in the inductors increase in value from the freewheeling values, whereas for negative voltages, the currents in the inductors grow negatively. In this step, as the inductor voltages are the input voltages, diodes are reverse-polarized and do not conduct. From the analysis of this stage, an operating condition is defined: the output voltage ${\mathrm{V}}_{\mathrm{o}}$ must be greater than $\sqrt[3]{{\mathrm{V}}_{\mathrm{e}}}$, where ${\mathrm{V}}_{\mathrm{e}}$ is the peak value of the phase voltage at the input. If this condition, which must be guaranteed in the design of the converter, is not satisfied, some output diodes are directly polarized and a short circuit, involving the output voltage and capacitors C₁, will occur. An output voltage lower than $\sqrt[3]{{\mathrm{V}}_{\mathrm{e}}}$ is possible by using coupled inductors such as L₂ (the same three-phase inductance L₂). This stage lasts for t_on = dT_s, where d is the duty cycle and T_s is the switching period.

2.1.2. Second Stage (t_don1)

When the switches are turned off, the output diodes are directly polarized, and the energy stored in L₁ is transferred to series capacitors C₁ and C₀ (output capacitor). The energy stored in L₂ is transferred to the output (C₀). The equivalent circuit is shown in Figure 4b. Currents decrease linearly (in absolute value) until the smallest current reaches the value of the freewheel.

2.1.3. Third Stage (t_don2)

The remaining inductor currents decrease until all currents reach freewheeling, as shown in Figure 4c.

2.1.4. Fourth Stage—Freewheeling (t_off)

At this stage (Figure 4d), the currents do not pass through any semiconductor. The existence of this stage characterizes the DCM operation, and it ends when the controlled devices are reconnected.

Input currents in a few switching periods and respective time intervals are shown in Figure 5, where i₁₁, i₂₁, and i₃₁ are the currents in the inductors L₁ of Phases 1, 2, and 3, respectively.

2.2. Theoretical Converter Analysis

The equations describing the converter behavior are presented below. In the following equations, as a balanced three-phase converter, input inductors have the same value and are presented as L₁; coupled inductors for each phase are presented as L₂, and series capacitors are presented as C₁. Additionally, i₁₁, i₂₁, and i₃₁ are the currents in the inductors L₁ of Phases 1, 2, and 3, respectively, and i₁₂, i₂₂, and i₃₂ are the currents in the L₂ inductors of Phases 1, 2, and 3, respectively. Freewheeling currents are represented as ${\mathrm{i}}_{1,\mathrm{rl}},{\mathrm{i}}_{2,\mathrm{rl}},{\mathrm{i}}_{3,\mathrm{rl}}$ for each phase. As presented before, the three symmetrical voltages are v₁, v₂, and v₃ and the output voltage is V_o.

It is important to notice that the voltage at the common point of the IGBT connection, although not physically connected to the ground, presents the same potential (usually considered zero). This can be explained by applying Kirchhoff’s current law to that point (currents sum is zero) and due to the fact that the source voltages are symmetrical (their sum is also zero).

The first stage lasts for t_on = dT_s, where d is the duty cycle and T_s is the switching period. Through the application of Kirchhoff’s laws along the equivalent circuits in this stage, for Phase 1, it can be seen that:

$${-\mathrm{v}}_1+{\mathrm{L}}_1\frac{{\mathrm{di}}_{11}}{\mathrm{dt}}=0\hspace{1em}{-\mathrm{v}}_1+{\mathrm{L}}_2\frac{{\mathrm{di}}_{12}}{\mathrm{dt}}=0$$

(1)

$${\mathrm{i}}_{11}=\frac{{\mathrm{v}}_1}{{\mathrm{L}}_1}\mathrm{t}+{\mathrm{i}}_{1,\mathrm{rl}}\hspace{1em}{\mathrm{i}}_{12}=\frac{{\mathrm{v}}_1}{{\mathrm{L}}_2}\mathrm{t}-{\mathrm{i}}_{1,\mathrm{rl}}$$

(2)

Similarly to Phases 2 and 3:

$${\mathrm{i}}_{21}=\frac{{\mathrm{v}}_2}{{\mathrm{L}}_1}\mathrm{t}+{\mathrm{i}}_{2,\mathrm{rl}}\hspace{1em}{\mathrm{i}}_{22}=\frac{{\mathrm{v}}_2}{{\mathrm{L}}_2}\mathrm{t}–{\mathrm{i}}_{2,\mathrm{rl}}$$

(3)

$${\mathrm{i}}_{31}=\frac{{\mathrm{v}}_3}{{\mathrm{L}}_1}\mathrm{t}+{\mathrm{i}}_{3,\mathrm{rl}}\hspace{1em}{\mathrm{i}}_{32}=\frac{{\mathrm{v}}_3}{{\mathrm{L}}_2}\mathrm{t}-{\mathrm{i}}_{3,\mathrm{rl}}$$

(4)

Analysis for the second stage on inductors L₁ is shown from (5) to (10).

$${-\mathrm{v}}_2+{\mathrm{L}}_1\frac{{\mathrm{di}}_{21}}{\mathrm{dt}}+{\mathrm{v}}_2-{\mathrm{v}}_3-{\mathrm{L}}_1\frac{{\mathrm{di}}_{31}}{\mathrm{dt}}+{\mathrm{v}}_3=0$$

(5)

$$\frac{{\mathrm{di}}_{31}}{\mathrm{dt}}=\frac{{\mathrm{di}}_{21}}{\mathrm{dt}}$$

(6)

$${-\mathrm{v}}_1+{\mathrm{L}}_1\frac{{\mathrm{di}}_{11}}{\mathrm{dt}}+{\mathrm{v}}_1+{\mathrm{V}}_{\mathrm{o}}+{\mathrm{v}}_3-{\mathrm{L}}_1\frac{{\mathrm{di}}_{31}}{\mathrm{dt}}-{\mathrm{v}}_3=0$$

(7)

$$\frac{{\mathrm{di}}_{31}}{\mathrm{dt}}–\frac{{\mathrm{di}}_{11}}{\mathrm{dt}}=\frac{{\mathrm{V}}_{\mathrm{o}}}{{\mathrm{L}}_1}$$

(8)

It is a fact that:

$${\mathrm{i}}_{11}+{\mathrm{i}}_{21}+{\mathrm{i}}_{31}=0$$

(9)

It is important to note that this step lasts until the current of the lowest absolute value, in this case i₂₁, reaches the freewheel value. Then:

$${\mathrm{i}}_{21}=\frac{{\mathrm{V}}_{\mathrm{o}}}{{3\mathrm{L}}_1}\mathrm{t}+\frac{{\mathrm{v}}_2{\mathrm{dT}}_{\mathrm{s}}}{{\mathrm{L}}_1}+{\mathrm{i}}_{2,\mathrm{rl}}={\mathrm{i}}_{2,\mathrm{rl}}$$

(10)

The duration of the second stage (t_don1) is shown in (11), where M is the ratio between the output voltage (V_o) and V_e.

$${\mathrm{t}}_{\mathrm{don}1}=\frac{{3\mathrm{dT}}_{\mathrm{s}}\cos (\mathsf{\omega }\mathrm{t}+60)}{\mathrm{M}}$$

(11)

For the third stage, the current i₂₁ is already freewheeling, lasting until the others reach the freewheeling value. Analyzing the L₁ inductors, the following equations can be written.

$${\mathrm{i}}_{21}={\mathrm{i}}_{2,\mathrm{rl}}\Rightarrow \frac{{\mathrm{di}}_{21}}{\mathrm{dt}}=0$$

(12)

$$\frac{{\mathrm{di}}_{11}}{\mathrm{dt}}+\frac{{\mathrm{di}}_{31}}{\mathrm{dt}}=0$$

(13)

Substituting previous equations:

$$\frac{{\mathrm{di}}_{11}}{\mathrm{dt}}=-\frac{{\mathrm{V}}_{\mathrm{o}}}{{2\mathrm{L}}_1}\hspace{1em}{\mathrm{i}}_{11}=-\frac{{\mathrm{V}}_{\mathrm{o}}}{{2\mathrm{L}}_1}\mathrm{t}+(-\frac{{\mathrm{V}}_{\mathrm{o}}}{{3\mathrm{L}}_1}{\mathrm{t}}_{\mathrm{don}1}+\frac{{\mathrm{v}}_1{\mathrm{dT}}_{\mathrm{s}}}{{\mathrm{L}}_1}+{\mathrm{i}}_{1,\mathrm{rl}})$$

(14)

$${\mathrm{i}}_{21}={\mathrm{i}}_{2,\mathrm{rl}}$$

(15)

$$\frac{{\mathrm{di}}_{31}}{\mathrm{dt}}=\frac{{\mathrm{V}}_{\mathrm{o}}}{{2\mathrm{L}}_1}\hspace{1em}{\mathrm{i}}_{31}=\frac{{\mathrm{V}}_{\mathrm{o}}}{{2\mathrm{L}}_1}\mathrm{t}+(\frac{2{\mathrm{V}}_{\mathrm{o}}}{{3\mathrm{L}}_1}{\mathrm{t}}_{\mathrm{don}1}+\frac{{\mathrm{v}}_3{\mathrm{dT}}_{\mathrm{s}}}{{\mathrm{L}}_1}+{\mathrm{i}}_{3,\mathrm{rl}})$$

(16)

To inductors L₂, a similar analysis is performed.

$${\mathrm{i}}_{22}=-{\mathrm{i}}_{2,\mathrm{rl}}\Rightarrow \frac{{\mathrm{di}}_{22}}{\mathrm{dt}}=0$$

(17)

$$\frac{{\mathrm{di}}_{12}}{\mathrm{dt}}+\frac{{\mathrm{di}}_{32}}{\mathrm{dt}}=0$$

(18)

Substituting previous equations:

$$\frac{{\mathrm{di}}_{12}}{\mathrm{dt}}=-\frac{{\mathrm{V}}_{\mathrm{o}}}{{2\mathrm{L}}_2}\hspace{1em}\Rightarrow \hspace{1em}{\mathrm{i}}_{12}=-\frac{{\mathrm{V}}_{\mathrm{o}}}{{2\mathrm{L}}_2}\mathrm{t}+(-\frac{{\mathrm{V}}_{\mathrm{o}}}{{3\mathrm{L}}_2}{\mathrm{t}}_{\mathrm{don}1}+\frac{{\mathrm{v}}_1{\mathrm{dT}}_{\mathrm{s}}}{{\mathrm{L}}_2}{-\mathrm{i}}_{1,\mathrm{rl}})$$

(19)

$${\mathrm{i}}_{22}={-\mathrm{i}}_{2,\mathrm{rl}}$$

(20)

$$\frac{{\mathrm{di}}_{32}}{\mathrm{dt}}=\frac{{\mathrm{V}}_{\mathrm{o}}}{{2\mathrm{L}}_2}\hspace{1em}\Rightarrow \hspace{1em}{\mathrm{i}}_{32}=\frac{{\mathrm{V}}_{\mathrm{o}}}{{2\mathrm{L}}_2}\mathrm{t}+(\frac{2{\mathrm{V}}_{\mathrm{o}}}{{3\mathrm{L}}_2}{\mathrm{t}}_{\mathrm{don}1}+\frac{{\mathrm{v}}_3{\mathrm{dT}}_{\mathrm{s}}}{{\mathrm{L}}_2}{-\mathrm{i}}_{3,\mathrm{rl}})$$

(21)

The third stage lasts (t_don2), which is the time for i₃₁ (along with i₁₁) to become freewheeling.

$${\mathrm{i}}_{31}=\frac{{\mathrm{V}}_{\mathrm{o}}}{{2\mathrm{L}}_1}\mathrm{t}+(\frac{{\mathrm{V}}_{\mathrm{o}}}{{3\mathrm{L}}_1}{\mathrm{t}}_{\mathrm{don}}+\frac{{\mathrm{v}}_3{\mathrm{dT}}_{\mathrm{s}}}{{\mathrm{L}}_1}{+\mathrm{i}}_{3,\mathrm{rl}})={\mathrm{i}}_{3,\mathrm{rl}}$$

(22)

$${\mathrm{t}}_{\mathrm{don}2}=\frac{2\sqrt{3}{\mathrm{dT}}_{\mathrm{s}}}{\mathrm{M}}\sin (\mathsf{\omega }\mathrm{t})$$

(23)

In the last stage, all currents have reached the freewheeling value.

$${\mathrm{i}}_{11}={-\mathrm{i}}_{12}={\mathrm{i}}_{1,\mathrm{rl}}$$

(24)

$${\mathrm{i}}_{21}={-\mathrm{i}}_{22}={\mathrm{i}}_{2,\mathrm{rl}}$$

(25)

$${\mathrm{i}}_{31}={-\mathrm{i}}_{32}={\mathrm{i}}_{3,\mathrm{rl}}$$

(26)

To ensure DCM operation, the switching period must be greater than the sum of the time of the first three intervals. Thus,

$${\mathrm{T}}_{\mathrm{s}}\ge {\mathrm{dT}}_{\mathrm{s}}+\frac{{3\mathrm{dT}}_{\mathrm{s}}\cos (\mathsf{\omega }\mathrm{t}+60)}{\mathrm{M}}+\frac{2\sqrt{3}{\mathrm{dT}}_{\mathrm{s}}}{\mathrm{M}}\sin (\mathsf{\omega }\mathrm{t})$$

(27)

Consequently, the maximum value that the duty cycle can assume is:

$$\mathrm{d}\le \frac{\mathrm{M}}{\mathrm{M}+\sqrt{3}}$$

(28)

The output current (I₀) in the analyzed range is supplied by the converter during times t_don1, and t_don2 and corresponds to the sum of currents i₁₁ + i₁₂. Its mean value (I₀) is provided by (29):

$${\mathrm{I}}_0=\frac{{3\mathrm{d}}^2{\mathrm{T}}_{\mathrm{s}}{\mathrm{V}}_{\mathrm{e}}{}^2}{{4\mathrm{VL}}_{\mathrm{eq}}}$$

(29)

where L_eq is the parallel equivalent of inductors L₁ and L₂ (30):

$${\mathrm{L}}_{\mathrm{eq}}=\frac{{\mathrm{L}}_1{\mathrm{L}}_2}{{\mathrm{L}}_1{+\mathrm{L}}_2}$$

(30)

The averaged output current (29) is a constant value for constant d and T_s. By doing this, the current injected at the output is guaranteed to be constant for all switching periods, meaning that the current at the input does not have low-frequency harmonic components.

From (4), a duty cycle for the proposed SEPIC-type converter can be selected in order to present DCM operation. Using (29), and considering rated power P, the equivalent converter inductance can be derived (31):

$${\mathrm{L}}_{\mathrm{eq}}=\frac{{3\mathrm{d}}^2{\mathrm{T}}_{\mathrm{s}}{\mathrm{V}}_{\mathrm{e}}{}^2}{4\mathrm{P}}$$

(31)

Considering the first stage (t_on), the ripple current is dependent on power (P) and can be written as (32).

$$\mathrm{ripple}(\%)=100\frac{{3\mathrm{dT}}_{\mathrm{s}}{\mathrm{V}}_{\mathrm{e}}{}^2}{{2\mathrm{PL}}_1}$$

(32)

Now, the L₁ value is obtained by (32) and, using (30) and (31), L₂ is derived.

The value of the capacitor of the converter must be calculated considering that the voltage at its terminals remains constant in the switching period; on the other hand, the voltage of the capacitor must follow the input voltage.

Analyzing the first step of operation, it is observed that the voltage in the capacitor varies little if the period of the resonance frequency between C₁ and L₂ is smaller than the t_on interval. This prevents the capacitor from overdischarge. Thus, the first design criterion of the capacitor is given by (33):

$${\mathrm{C}}_1>\frac{{{(\mathrm{dT}}_{\mathrm{s}})}^2}{{4\mathsf{\pi }}^2{\mathrm{L}}_2}$$

(33)

In addition, the smaller the value of C₁, the greater the voltage ripple at its terminals and, consequently, the higher the voltage in the controlled switches and diodes.

On the other hand, the resonance frequency of the equivalent impedance seen by the grid must be significantly higher than the frequency of the grid so that there are no low-frequency oscillations. This requirement is satisfied according to (34):

$${\mathrm{C}}_1<\frac{1}{{\mathsf{\omega }}^2{\mathrm{L}}_{\mathrm{eq}}}$$

(34)

where ω is the grid frequency. Using the presented equations and performing the calculations, the values of the components are known.

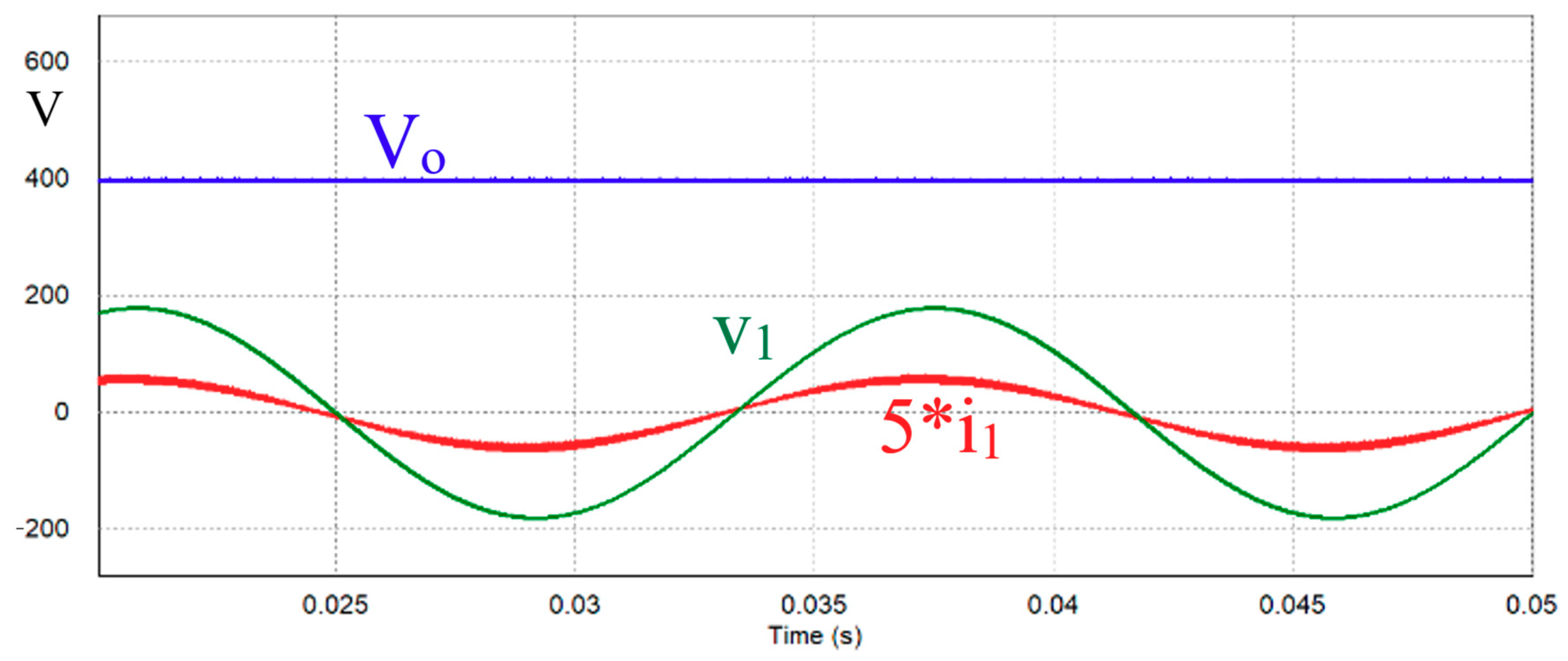
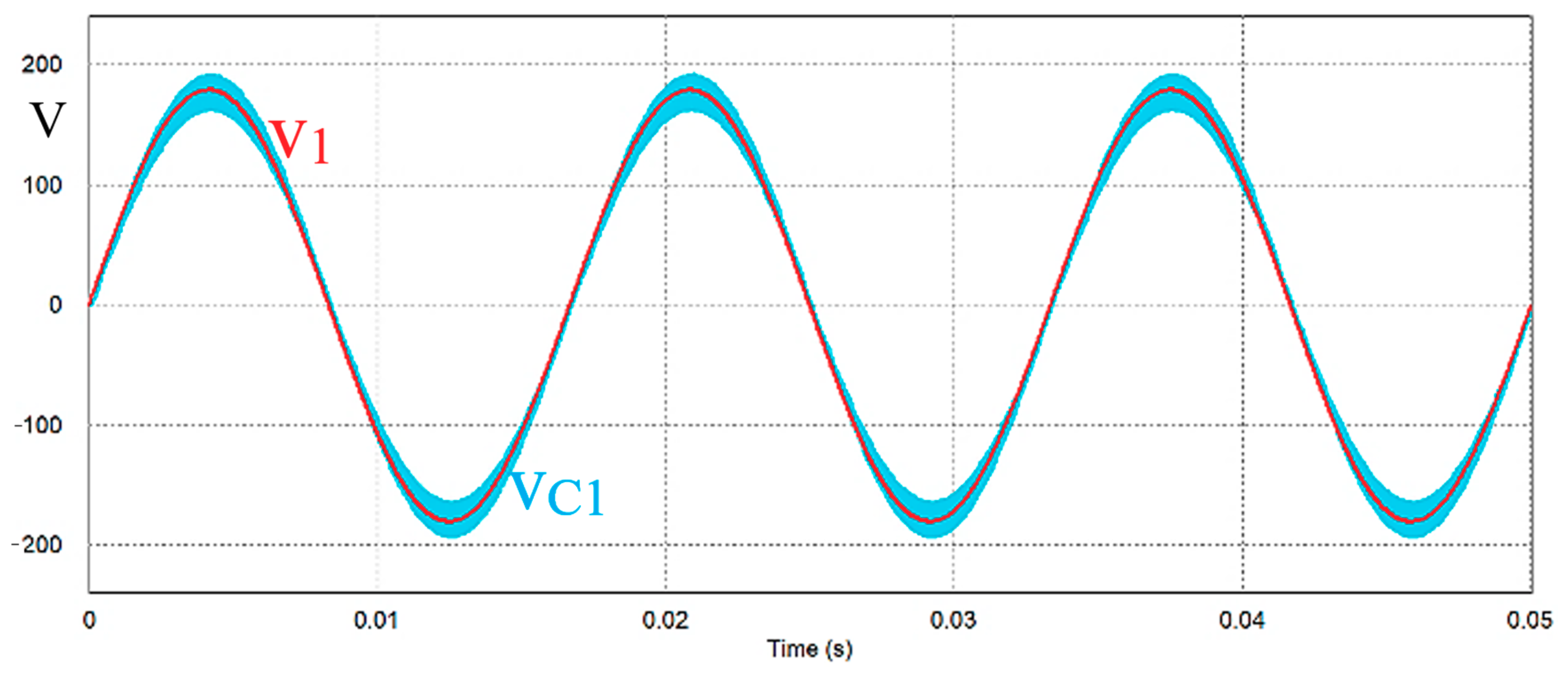
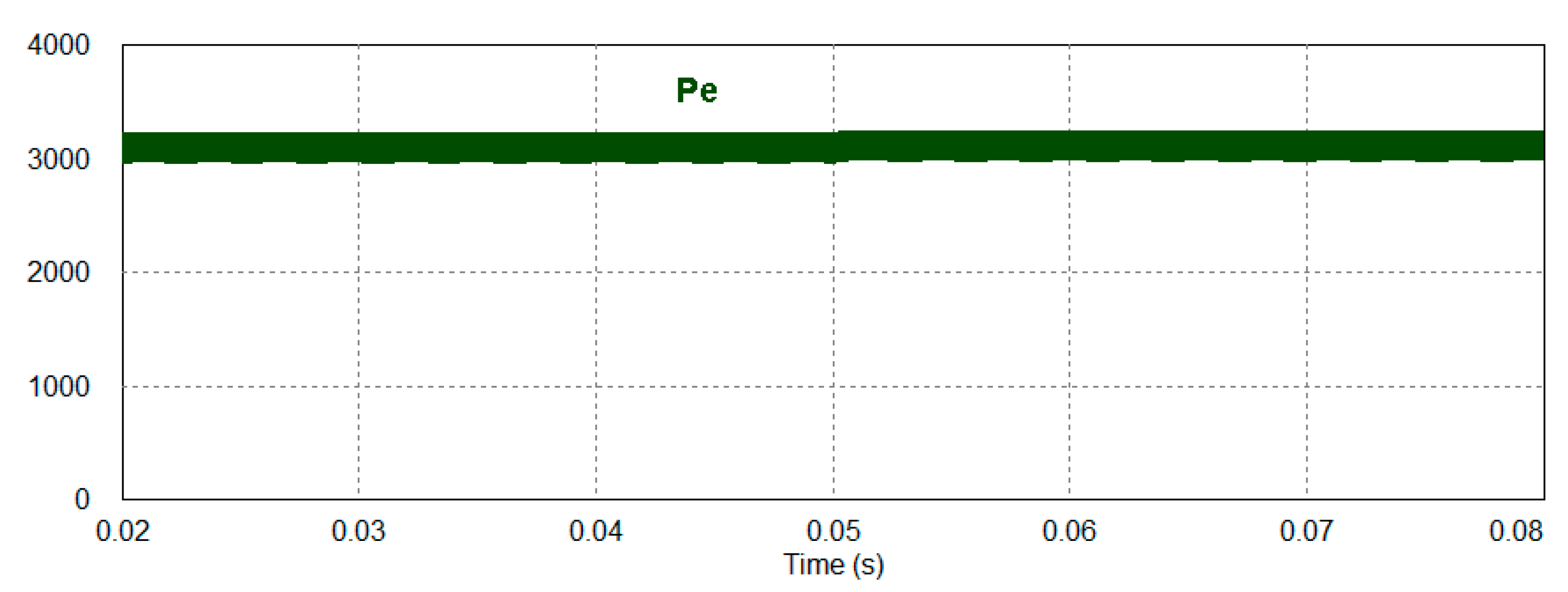
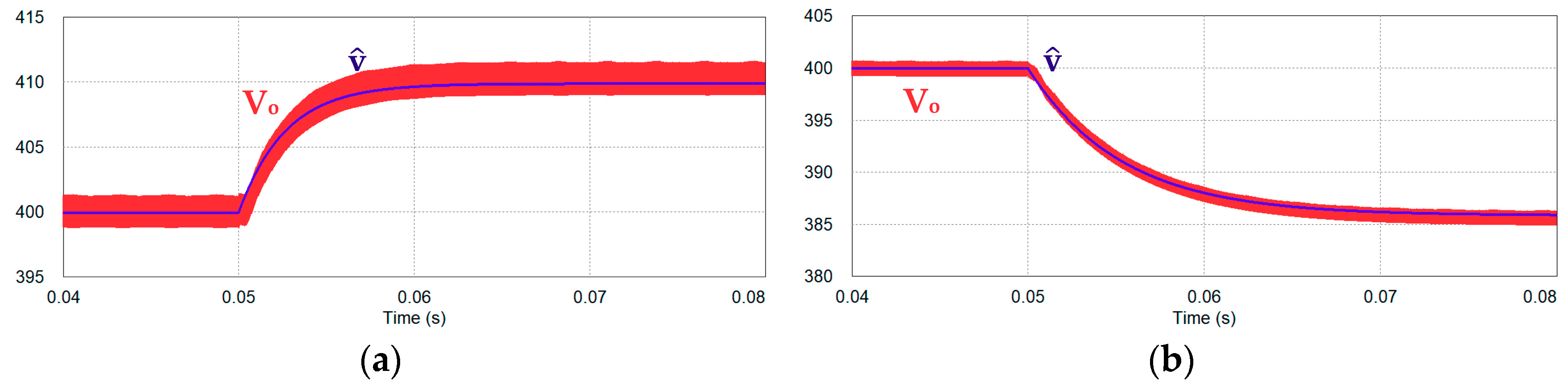
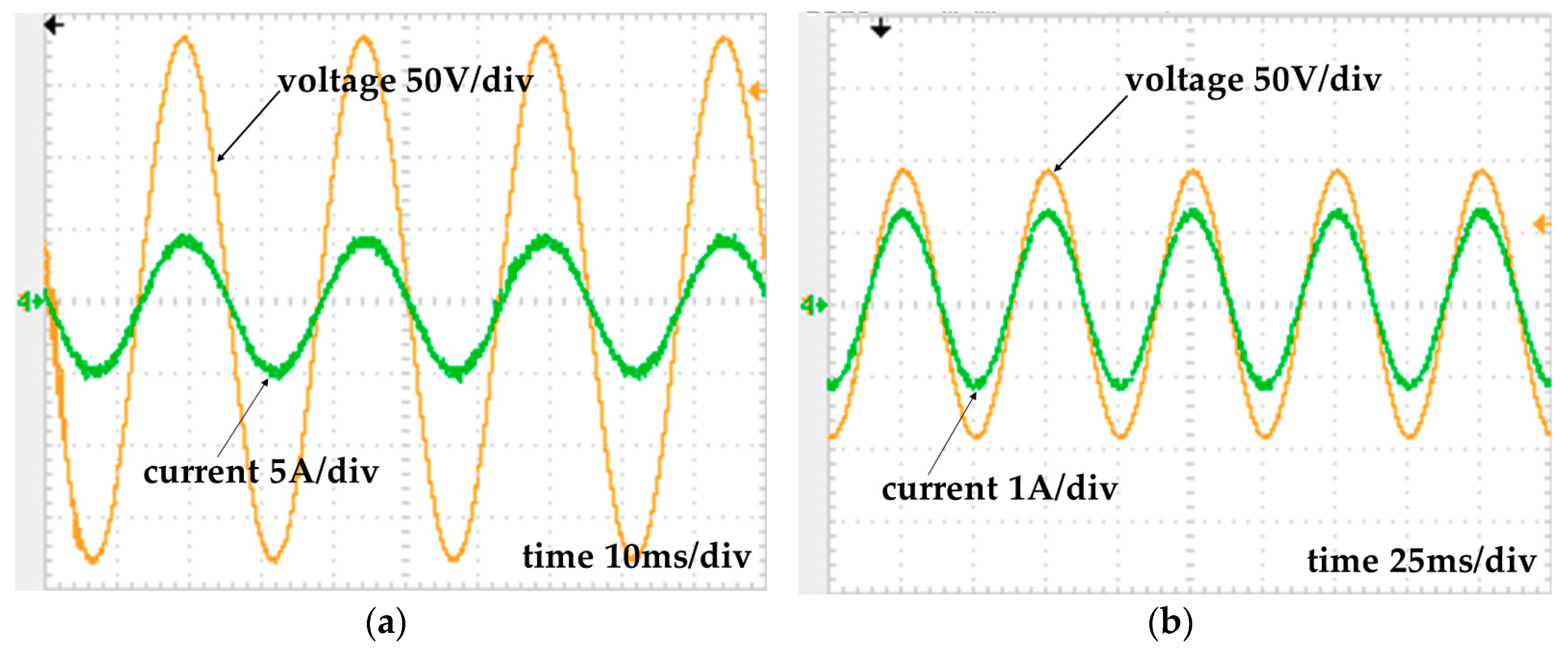
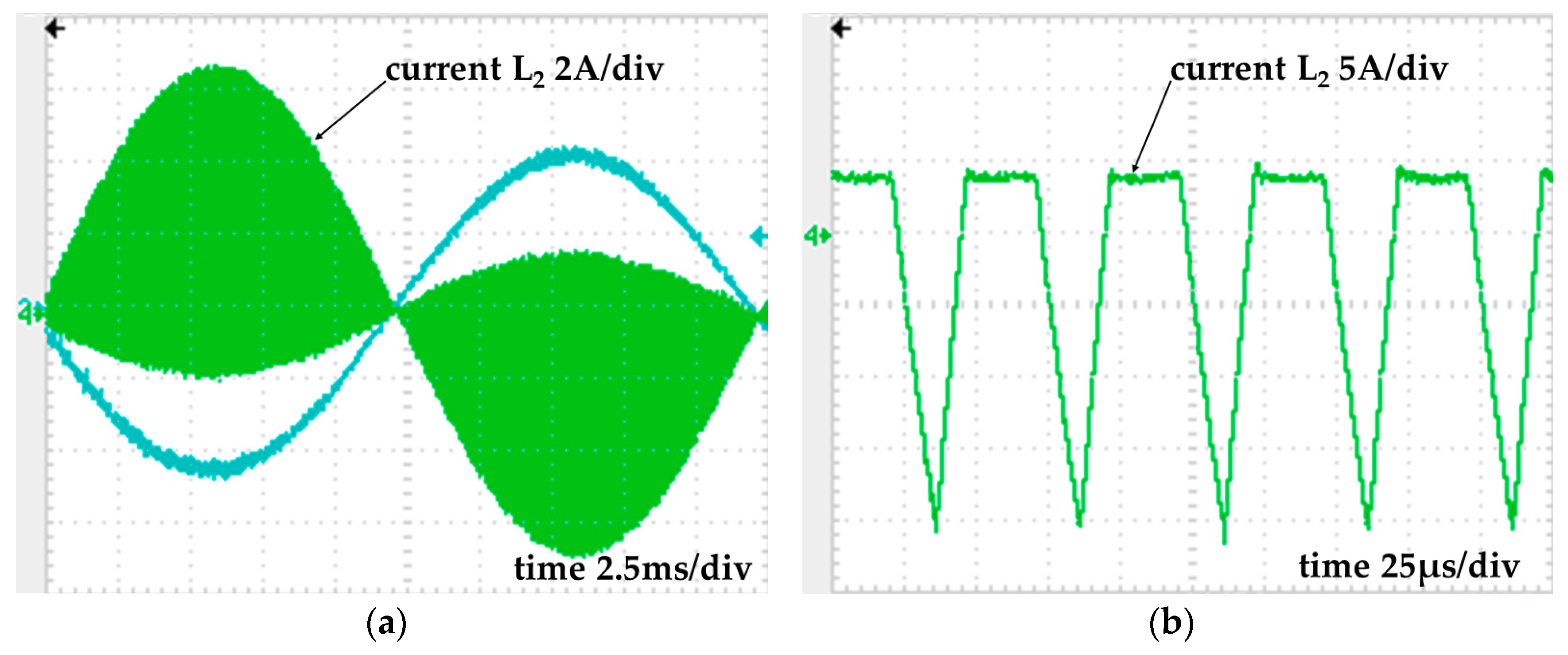
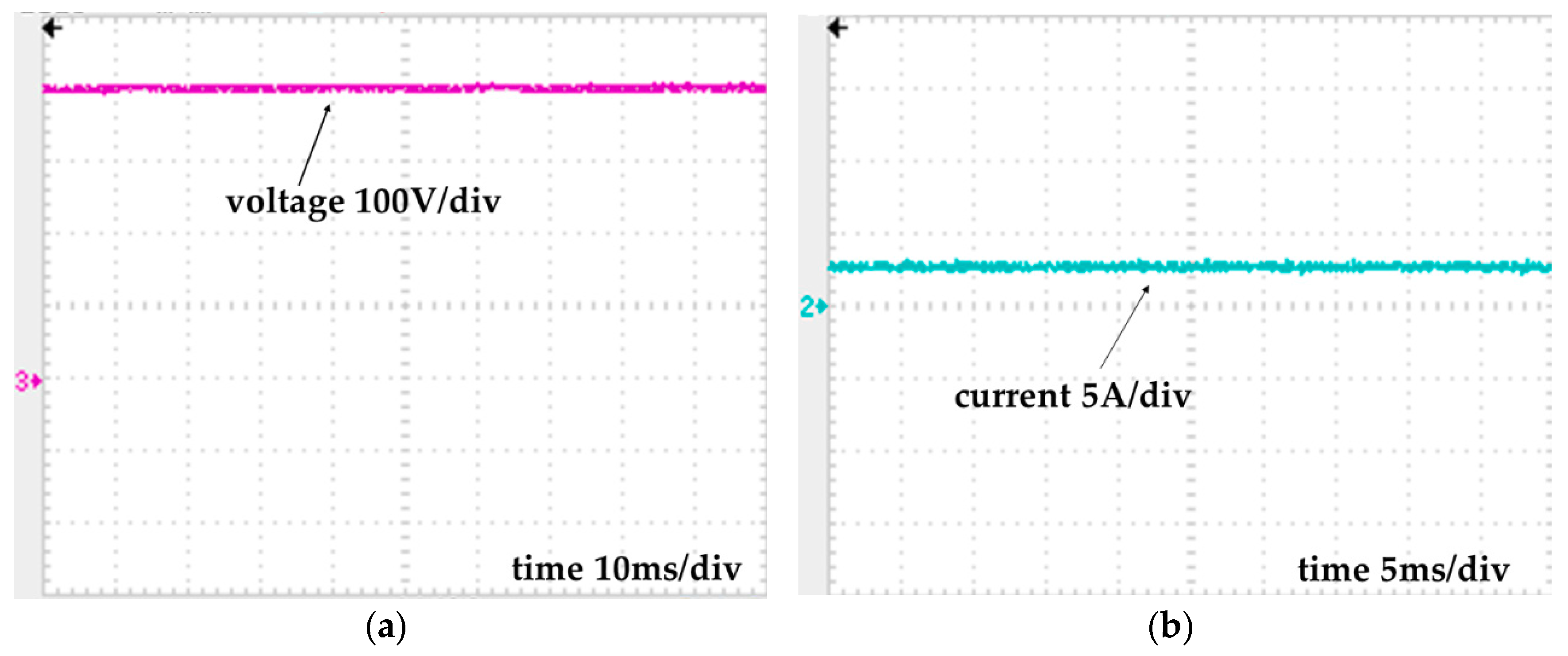
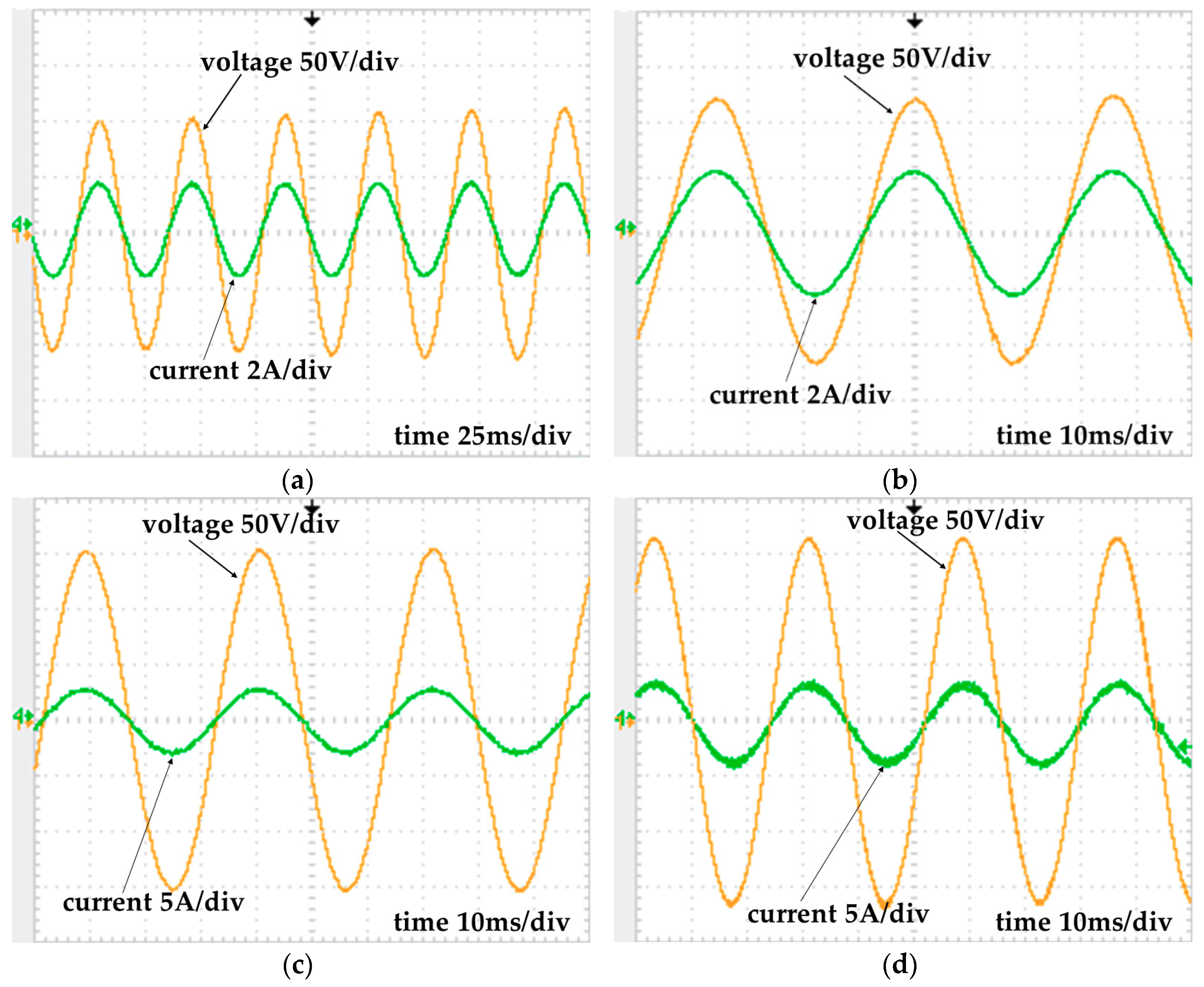
3. Simulation Results

The proposed converter was designed and simulated considering a 3 kW–220 Vac/400 Vdc rectifier. The grid frequency is 60 Hz, whereas the switching frequency is 25 kHz. Using (28), the duty cycle is chosen as 0.4 (35). Through this value applied on (31), L_eq can be calculated (36).

$$\mathrm{d}=0.4\le 0.56$$

(35)

$${\mathrm{L}}_{\mathrm{eq}}=52\mathsf{\mu }\mathrm{H}$$

(36)

Assuming 20% of ripple in (32), inductors are calculated as L₁ = 1.29 mH and L₂ = 54 μH. Considering (33) and (34), 120 nF < C₁ < 0.1 F. After some simulations, C₁ = 10 μF was chosen.

Figure 6 shows the output voltage V_o as well as input voltage (v₁) and current (i₁). The input current waveform is a mirror of the input voltage, except for the switching frequency ripple.

In Figure 7, the phase voltage (v₁) and its respective capacitor voltage (v_C1) are shown, which follow the input phase voltage. This is a characteristic of a SEPIC converter. The electric power absorbed from the source is shown in Figure 8. Opposite to the typical power absorbed from a single-switch three-phase boost rectifier (Figure 2), the absorbed power of this converter is constant without low-order harmonic oscillation.

A transfer function (TF) can be derived by applying small-signal perturbations into the average output current I₀ (29). Cancelation of the steady-state terms results in a first-order TF, as given by (37). A comparison between the simulation result and TF estimation by applying a small step change into the duty cycle is performed. Figure 9a shows, for nominal conditions, the simulated output voltage (red) and the estimated output voltage by the transfer function (blue) when, at t = 0.05 s, the duty cycle is increased by 10%. Figure 9b shows a similar result for half load and a 10% duty cycle decrease. It can be concluded that the TF appropriately represents the dynamic converter behavior.

$$\frac{\widehat{{\mathrm{V}}_{\mathrm{o}}}}{\hat{\mathrm{d}}}=\frac{3\mathrm{D}{\mathrm{T}}_{\mathrm{s}}{{\mathrm{V}}_{\mathrm{e}}}^2}{2{\mathrm{V}}_{\mathrm{o}}{\mathrm{L}}_{\mathrm{eq}}}\times \frac{{\mathrm{R}}_{\mathrm{o}}}{\frac{{\mathrm{R}}_{\mathrm{o}}{\mathrm{C}}_{\mathrm{o}}\mathrm{s}}{2}+1}$$

(37)

4. Experimental Results

The rectifier topology presented was analyzed predicting an application to a low-power wind energy conversion system (WECS) based on a permanent magnet synchronous generator (PMSG) with variable wind speed. The SEPIC-type converter can operate for a wide wind speed range, keeping the DC bus voltage constant despite wind variations and consequent voltage and power variations at the input. For this analysis, the reduction in actual wind speed (v_wind,actual) is considered compared to the nominal value (v_wind,nominal) as n_r, defined in (38),

$${\mathrm{n}}_{\mathrm{r}}=\frac{{\mathrm{v}}_{\mathrm{wind},\mathrm{nominal}}}{{\mathrm{v}}_{\mathrm{wind},\mathrm{actual}}}$$

(38)

For a wind variation in a turbine whose nominal operating value is 12 m/s and a typical cut-in wind speed operation of 3 m/s, the variation between these values is η_r = 4. For this reduction, the generated voltage (which is proportional to the wind, according to Faraday’s law) is reduced by ¼ between the maximum and minimum values. The power, in turn, proportional to the cube of wind speed reduces by 1/64. Considering the output voltage constant, the M ratio increases as wind speed decreases. Considering (28), the duty cycle limit between the conduction–discontinuous operation increases. Thus, the limit of d, given by the nominal condition, is more restrictive than the condition for lower wind speed. Assuming an operation with d below the maximum for nominal condition, the DCM is maintained throughout the complete operating range. The relation between the duty cycle for rated wind speed (d) and any wind speed in the range (d′) can be obtained, as given by (39):

$${\mathrm{d}}^{\prime }=\frac{\mathrm{d}}{\sqrt{{\mathrm{n}}_{\mathrm{r}}}}$$

(39)

A MPPT algorithm is usually employed to track the duty cycle according to wind variations.

The 3ϕ SEPIC-type converter was implemented considering a 1 kW power conversion system. The value used for L₁ is a typical PMSG inductance value for this power, presenting a RMS line voltage of 220 V/40 Hz at 12 m/s nominal wind speed. The converter operates at a switching frequency of f_s = 20 kHz, a DC bus of 400 V, and typical wind speeds (low and high speed) of 6 m/s and 12 m/s, resulting in voltage frequencies of 20 Hz and 40 Hz, respectively. The nominal duty cycle was chosen as 0.28.

Table 1. System specification.

Ve (V)	P (kW)	Vo(V)
220	1	400

shows the system specifications, and

Table 2. Project of a 1 kW 3ϕ SEPIC-type rectifier.

fs (kHz)	d 40 Hz(%)	d 20 Hz(%)	L1 (mH)	L2 (µH)	C1 (µF)	C0 (µF)
20	28	20	12	95	4.7	100

presents the values of the specified components.

In order to emulate the output voltages of a wind generator, the programmable three-phase voltage source Pacific Power Source AMX-360 was used. The nominal power of the source is 6 kVA and allows effective voltages up to 338 V and frequency from 20 Hz to 5 kHz. The assembled workbench is shown in Figure 10.

Table 3. Commercial components used on the workbench.

IGBT	Diode	Capacitor 4.7 µF	L1 (12 mH)	L2 (95 µH)	Capacitor C0 (100 µF)
HGTG11N120CND (FAIRCHILD)	HFA50PA60C (International Rectifier)	940C12P47K-F (Cornell Dubilier)	Made of silicon steel	Built with ferrite core	450 V Eletrolytic (Epcos)

presents the commercial components used.

The results for the possible 12 m/s (40 Hz) and 6 m/s (20 Hz) wind operating points are presented. Figure 11a,b shows the current and voltage at the generator with operation at 40 Hz and 20 Hz, respectively.

One can observe the sine waveform of the current in phase with the voltage in both cases. For 40 Hz, the power factor value for Phase 1 provided in the Pacific Power source display was 0.998. The current THD supplied by the source (considering the odd-order harmonics) was 4.8%. For 20 Hz, the power factor value for Phase 1 provided in the Pacific Power source display was 0.993. The current THD supplied by the source (considering the odd-order harmonics) was 5.7%.

Table 4. SEPIC summary operation: experimental results.

Wind Speed	Power Factor	Current TDH
12 m/s	0.998	5.7%
6 m/s	0.993	4.8%

presents a summary of the converter operation with the data presented previously.

Figure 12a shows the image of current in L₂ inductors for a 1/(40 Hz) period, and Figure 12b shows a zoom of the same current for some switching periods. The discontinuous conduction mode can be observed, characterized here as the time interval the current is constant (freewheeling).

The output voltage (Figure 13a) and output current (Figure 13b) are shown for f = 40 Hz (v_wind = 12 m/s). It can be noticed that the voltage ripple is negligible. For the connected load, the voltage reaches around 400 V, and the current reaches an average value of 2.67 A.

It was possible to check the proper operation of the SEPIC-type rectifier in a wide range of wind speed with a high power factor and low harmonic content, presenting the output voltage according to the designed value. Figure 14 presents results of input voltage and current for equivalent frequencies of f = 24 Hz (v_wind = 7.2 m/s), f = 28 Hz (v_wind = 8.4 m/s), f = 32 Hz (v_wind = 9.6 m/s), and f = 36 Hz (v_wind = 10.8 m/s). An almost perfect sinusoidal input current, following input voltage is obtained only by applying an appropriate duty cycle, corroborating that an unity power factor is achieved.

5. Conclusions

It has been shown that a three-phase SEPIC-type DCM rectifier can be implemented using three active switches controlled by the same gate drive signal. The choice of three switches allows fewer semiconductor devices in the current path during switches turned-on. Equal found in a single-phase SEPIC converter, this one presents an input inductor, an intermedium capacitor, and a second inductor in each phase. With an appropriate element design and DCM operation, as pointed out in the text, the input current will be in phase to the input voltage, resulting in a high-power-factor rectifier.

The simulation (Figure 6) as well experimental results (Figure 11 and Figure 14) validated that the proposed topology naturally presents the input currents in the AC source as an image of the generated voltage, without any control of the current at the converter input. It was possible to achieve a reduced harmonic content, which is a better solution than the equivalent low-power boost topology found in the literature (which provides extremely deformed currents in relation to the voltage). It is relevant to observe that no input filter is required to achieve high-quality input currents in this proposed converter. Again, the SEPIC-type converter is attested to verify that the capacitor voltage is equal to the input voltage along with the line period.

Even though no control loop was performed in the presented results, the output voltage/duty cycle transfer function was presented. It is useful for a control loop to control the output voltage. As the converter operated in the discontinuous conduction mode, the output voltage to duty cycle ratio varied with load variation. The DCM operation ensures the input current waveform without making use of a current loop. The simulation results for small step changes in the duty cycle, shown in Figure 9, validated the developed TF representative of the dynamic converter behavior and adequate as an effective control loop design.

In relation to the generated power, a significant improvement is obtained. Having low THD of the current implies reduced low-frequency oscillations. The output voltage and average output current obtained by the simulation as well as experimentally were continuous, with negligible oscillations, leading to a constant power delivered to the load.

The experimental results were satisfactory for the equivalent wind speed conditions at a nominal value of 12 m/s and for reduced values, keeping a high power factor and reduced harmonic current content, validating the proposal of the SEPIC-type converter to be employed in grid AC–DC conversion as well as PMSG or microturbines for high-power-factor rectification.

It is meaningful that the electric power absorbed from the line or generator is almost constant, only presenting a high-frequency ripple. For a low-power PMSG, it is relevant.

The results obtained have proved the efficacy of the converter for the presented purpose. However, some important points must be considered: the active switches must be able to withstand a maximum voltage equal to the peak line voltage plus the rectified output voltage. This can increase switching losses, especially when the topology is operating as an elevator.

Coupling capacitors, as in a conventional SEPIC converter, are subjected to currents with considerable RMS values. Thus, the use of capacitors that support a high current and have low internal resistance is highly recommended. Although the losses have not been evaluated, the use of semiconductor components (IGBT/MOSFET/diodes) of the Sic or Gan type will certainly contribute to their decrease, making the topology even more competitive.