Exact Symmetric Solutions of MHD Casson Fluid Using Chemically Reactive Flow with Generalized Boundary Conditions

Abstract

Dynamic analysis of magnetic fluids with the combined effect of heat sink and chemical reactions based on their physical properties demonstrates strong shock resistance capabilities, low-frequency response, low energy consumption, and high sensitivity. Therefore, the applied magnetic field always takes diamagnetic, ferromagnetic, and paramagnetic forms. The influence of radiation is considered in the temperature profile. This manuscript investigates an analytic solution of incompressible and magnetic Casson fluid in Darcy’s medium subjected to temperature and concentration dependence within a porous-surfaced plate with generalized boundary conditions. The substantial mathematical technique of the Laplace transform with inversion is invoked in the governing equations of the magnetic Casson fluid. The analytic results are transformed into a special function for the plate with a constant velocity, a plate with linear velocity, a plate with exponential velocity, and a plate with sinusoidal velocity. Graphical illustrations of the investigated analytic solutions at four different times are presented. Our results suggest that the velocity profile decreases by increasing the value of the magnetic field, which reflects the control of resistive force. The Nusselt number remains constant at a fixed Rd and is reduced by raising the Rd value.

1. Introduction

Heat transfer is mainly observed due to variation in the temperature of bodies. This process plays a vital role in mechanization and industries such as climate engineering, device cooling, nuclear power plants, and energy acquisition. The well-known Fourier law for heat transfer [1] and Fick’s law for mass transfer have been widely used in the literature. The fragility of Fourier law is that the initial bugging is immediately perceived by the medium, which is impractical. The classical Fourier law was amended by adding relaxation time to heat flux by Cattaneo [2]. Christov [3] further modified Cattaneo’s law by incorporating a Lie derivative for the heat flux. The Cattaneo–Christov theory has been applied to both Newtonian and non-Newtonian fluids with various physiological effects. The Cattaneo–Christov model was discussed by Straughan for the thermal convection of a viscous fluid [4]. Salahuddin et al. [5] applied the theory given in [3] to Williamson fluid. The flow of Eyring–Powell fluid over an exponentially stretching surface in three dimensions was reported by Hayat and Nadeem [6] following [3]. With the same theory, Maxwell fluid flow with an expanding sheet with changeable thickness was studied by Hayat et al. [7]. Moreover, Hashim and Khan [8] considered Carreau fluid with a slender sheet under the effect of the model in [3]. Oldroyd-B fluid was analyzed employing [3] by Abbasi et al. [9]. Hayat et al. [10] presented a comparative study of viscoelastic fluids through [3]. Unsteady and nonlinear convection of micro- and nanofluids under [3] was recently stated by Upadhya et al. [11].

Non-Newtonian fluids have extensive use in industrial and engineering processes such as the production of paper, polymer processing, geological flows in the earth mantle, ink printing, paint suspensions, and biological flows. Thus, the analysis of such fluids is of substantial research interest and significant importance. Typical characteristics of the flow of non-Newtonian fluids have become a crucial area of research for scientists, mathematicians, engineers, and researchers. Strain rate and stress are a combination of linear and nonlinear relations characterized by Newtonian fluid and non-Newtonian fluids, respectively. With the relationship between strain rate and stress, non-Newtonian fluids, polymer solutions, slurries, and pastes, to mention just a few, are difficult for developing mathematical modeling in terms of differential equations. Due to this reason, non-Newtonian fluids give rise to an abundance of rheological mathematical models of fluids. We classify such fluids models by century: 18th century, from 1867 to 1893 (Barus and Maxwell model), and 19th century, from 1922 to 1995 (Blatter model, Ellis model, Giesekus model, Phan–Thien–Tanner model, Johnson–Tevaarwerk model, Carreau–Yasuda model, Carreau model, Cross model, Rivlin–Ericksen model, Oldroyd-8 constants model, Oldroyd-B model, Rivlin model, generalized Burgers, Eyring, and Williamson fluid model), among others. Among these fluid models, which are the most accurate and treated fluid models in the biofield, is the so-called Casson fluid model (1959). The main significance of this model is to characterize the pseudoplastic properties of yield stress. Common, useful examples of the Casson model are concentrated fruit juices, jelly, tomato sauce, and honey, among others [12,13,14,15,16]. To characterize the rheology of Casson fluid, several authors have adopted different research directions.

Magneto fluid dynamics has become an important topic in recent years. The study of magnetohydrodynamics has assisted real-life applications. For instance, electromagnetic forces can be used to pump liquid metals without the need for any moving parts. The concept of MHD has significant importance in stellar and planetary processes and has also boosted engineering applications, such as direct conversion generators and flow problems of ionized gasses. MHD unsteady flow in a porous channel with convective heat conditions at the surface was explored by Makinde [17], whose study concludes that the presence of a magnetic field strengthens flow control. The boundary layer flow of MHD Maxwell nanofluid was discussed with numerical assistance [18]. Ellahi et al. [19] numerically inquired about the Couette flow of heat transfer in magnetohydrodynamics. Thermal radiation is a ruling factor in the thermodynamic analysis of high-temperature systems such as boilers and solar connectors. Heat and mass relocation analysis with thermal radiation plays a vital role in manufacturing industries, such as the design of flippers, gas motors, and cooling towers; various propulsion devices for aircraft, energy utilization, and food processing; and diverse agricultural, military, and health applications. As a result, a lot of work has been conducted on fluid flow considering radiation in thermal radiation. The effect of thermal radiation has been analyzed for viscoelastic fluids. The Rosseland approximation was applied to characterize the heat flux in a heat equation by Qasim et al. [20]. Ayub et al. [21] discussed the influence of a wall shield on the radiation of a transverse electromagnetic wave. The solution was obtained by the Wiener–Hopf technique.

Maleque [22] described the porous effects of Casson fluid flow with an axial uniform magnetic field in which similarity parameters were applied to reduce nonlinear ordinary differential equations. In order to determine the angular velocity approximately, the numerical shooting method was used. Abd El-Aziz and Afify [23] numerically analyzed the slippage of Casson nanofluid to enhance the warmth transfer of an overextended sheet. Their main emphasis was to validate their obtained results by comparing them with existing literature. Casson fluid over a steady spinning plane based on three-dimensional, thin-film, nanofluid flow was examined by Anwar et al. [24]. They used the homotopy analysis method to calculate the governing equation and Mathematica software (Wolfram Mathematica, New Jersey, NJ, United State) to provide a graphical illustration of the velocity gradient, temperature, and concentration gradient of the Casson model. Although research on the Casson model is ongoing, we include some related studies based on heat transfer [25,26,27,28,29], fractional models of fluids with magnetic field [30,31,32,33,34,35,36,37,38,39,40], and some others [41,42,43,44,45,46,47,48,49,50,51,52,53,54] herein. Motivated by the above discussion, we analyzed an analytic solution of incompressible and magnetic Casson fluid subjected to temperature and concentration dependence within a porous-surfaced plate.

The intention of this manuscript is to develop exact symmetric solutions of MHD Casson fluid with chemically reactive flow with the help of generalized boundary conditions. A substantial mathematical technique of Laplace transforms with inversion is applied to magnetic Casson fluid. In Section 2, dimensionless governing equations are developed. In Section 3, exact solutions of concentration, temperature, and velocity field are developed with the help of the Laplace transformation. In Section 4, analytic solutions are transformed into special functions for a plate with constant velocity, a plate with linear velocity, a plate with exponential velocity, and a plate with sinusoidal velocity. In Section 5, graphical illustrations of the investigated analytic solutions at four different times are presented. Finally, concluding observations are listed in Section 6.

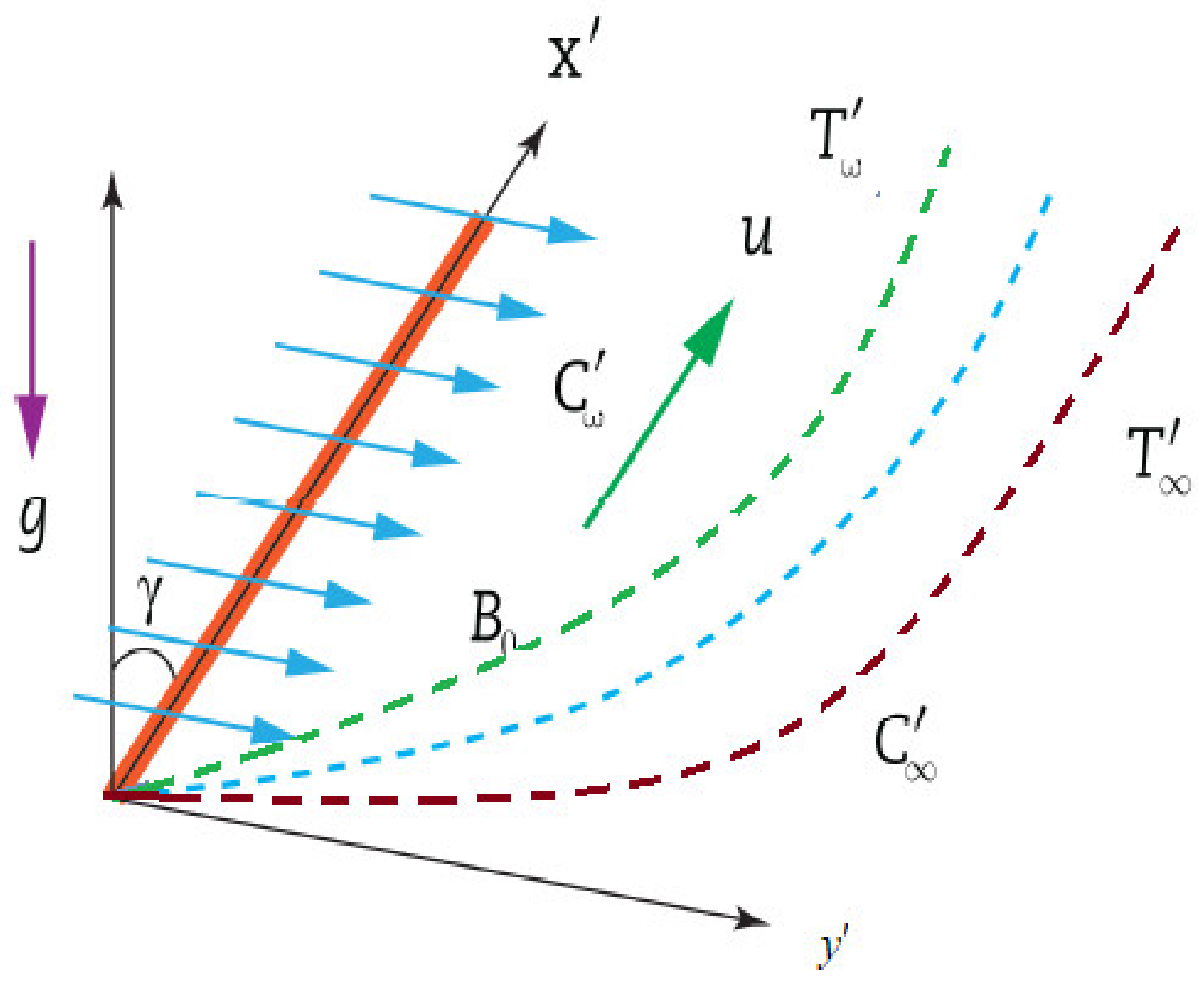
2. Mathematical Formulation

Let us consider incompressible MHD Casson fluid with a permeable surface with inclination angle $\gamma$ and magnetic field $B_o$ normal to the plate. At $t>0,$ the plate started to move with velocity $u^{\prime }\left(y^{\prime },t^{\prime }\right)$, having its concentration and temperature depend upon time as $T_{\infty }^{\prime }+T_w^{\prime }{h}^{\prime }\left(t^{\prime }\right)$, and $C_{\infty }^{\prime }+C_w^{\prime }{g}^{\prime }\left(t^{\prime }\right)$. The physical model describing flow is given in Figure 1. We suppose that the rheological equation for incompressible Casson fluid [12,13] is

$${\tau }_{mn}=\left\{\begin{array}{c}2\left({\mu }_r+\frac{p_x}{\sqrt{2\pi }}\right)e_{mn},\pi >{\pi }_c\\ 2\left({\mu }_r+\frac{p_x}{\sqrt{2{\pi }_c}}\right)e_{mn},\pi <{\pi }_c\end{array}\right..$$

Some necessary assumptions considered to formulate the mathematical model are described as:

(a)

The pressure gradient is absent on the boundary.

(b)

No external electric fields exist because of the neglected polarization effect.

(c)

Due to the consideration of an infinitely long plate, the governing equations only involve time t and axial coordinate y.

(d)

It is assumed that the induced magnetic field is sufficiently weak and has no significant role in the flow process.

Following these assumptions, primary flow, temperature, and concentration equations are derived under Rosseland and Boussinesq’s approximation.

$$\frac{\partial u^{\prime }}{\partial t^{\prime }}=\upsilon \left(1+\frac{1}{\lambda }\right)\frac{{\partial }^2{u}^{\prime }}{\partial y^{\prime 2}}+g\beta \left(T^{\prime }-T_{\infty }^{\prime }\right)cos\gamma +g\beta cos\gamma C^{\prime }-g\beta cos\gamma C_{\infty }^{\prime }-\frac{\sigma B_0^2}{\rho }{u}^{\prime }-\left(\frac{\upsilon }{K_p}+\frac{\upsilon }{K_p}\frac{1}{\lambda }\right)u^{\prime },$$

(1)

$$\rho C_p\left(\frac{\partial T^{\prime }}{\partial t^{\prime }}\right) = K\left(\frac{{\partial }^2{T}^{\prime }}{\partial y^{\prime 2}}\right) - \left(\frac{\partial q_r^{\prime }}{\partial y^{\prime }}\right) + S\left(T^{\prime }-T_{\infty }^{\prime }\right),$$

(2)

$$\left(\frac{\partial C^{\prime }}{\partial t^{\prime }}\right) = D\left(\frac{{\partial }^2{C}^{\prime }}{\partial y^{\prime 2}}\right) + k_c{C}^{\prime }-k_c{C}_{\infty }^{\prime }.$$

(3)

The initial boundary conditions are

$$t^{\prime }\le 0,u^{\prime }\left(y,0\right) = 0,T^{\prime }\left(y,0\right) =T_{\infty },C^{\prime }\left(y,0\right) = C_{\infty },y^{\prime }\ge 0,$$

(4)

$$t^{\prime }>0,u^{\prime }\left(y,t\right) = u_0{f}^{\prime }\left(t^{\prime }\right),T^{\prime }\left(y,t\right) = T_{\infty }^{\prime }+T_w^{\prime }{h}^{\prime }\left(t^{\prime }\right),C^{\prime }\left(y,t\right) = C_{\infty }^{\prime }+C_w^{\prime }{g}^{\prime }\left(t^{\prime }\right),y^{\prime }=0,$$

(5)

$$t^{\prime }>0,u^{\prime }\left(y,t\right)\to 0,T^{\prime }\left(y,t\right)\to T_{\infty }^{\prime },C^{\prime }\left(y,t\right)\to C_{\infty }^{\prime },y^{\prime }\to \infty .$$

(6)

Radiative flux is defined by using the Rosseland approximation [32],

$$q_r=-\frac{4{\sigma }_0\partial T^4}{3k}.$$

(7)

$T^4$ is a linear function and expressed by the Taylor expansion. By neglecting higher powers, we have

$$T^4=T_{\infty }^4+4T{T}_{\infty }^3-4T_{\infty }{T}_{\infty }^4+6T_{\infty }^2{(T-T_{\infty })}^2+\dots \dots ,$$

(8)

Substituting Equations (7) and (8) into Equation (2), the required form of the temperature profile is given as:

$$\rho C_p\frac{\partial T}{\partial t}=K\left(1+\frac{16{\sigma }_0\partial T_{\infty }^3}{3k{k}_1}\right)\frac{{\partial }^{2}T}{\partial y^2}+ST-S{T}_{\infty }.$$

(9)

For the simplification of the governing equations, Equations (1)–(3), we introduce the dimensionless variable among the governing equations of Casson fluid. We define them as described below:

$$y=\frac{u_0}{\upsilon }{y}^{\prime },u=\frac{u^{\prime }}{u_0},t=\frac{u_0^2}{\upsilon }{t}^{\prime },T=\frac{T^{\prime }-T_{\infty }^{\prime }}{T_w^{\prime }},C=\frac{C^{\prime }-C_{\infty }^{\prime }}{C_w^{\prime }},P_r=\frac{\mu C_p}{K},S=\frac{\upsilon }{\rho C_p{u}_0^2}{S}^{\prime },$$

(10)

$$S_c=\frac{\upsilon }{D},M=\frac{\sigma B_0^2\upsilon }{\rho u_0^2},K_c=\frac{\upsilon }{K_c^{\prime }{u}_0^2},K_1=\frac{{\upsilon }^4}{k_p{u}_0^4},k_p=\frac{{\upsilon }^2}{u_0^2}{k}_p^{\prime }.$$

(11)

After invoking the dimensionless quantities in Equations (1)–(3), we simplify the governing equations of Casson fluid as:

$$\frac{\partial u}{\partial t}=\left(1+\frac{1}{\lambda }\right)\frac{{\partial }^{2}u}{\partial y^2}+G_{r}cos\gamma T+G_{c}cos\gamma C-\left(M+\frac{1}{k_1}\left(1+\frac{1}{\lambda }\right)\right)u,$$

(12)

$$\frac{\partial T}{\partial t}=\frac{1}{P_r}\left(1+\frac{4}{3}{R}_d\right)\frac{{\partial }^{2}T}{\partial y^2}-ST,$$

(13)

$$\frac{\partial C}{\partial t}=\frac{1}{S_c}\frac{{\partial }^{2}C}{\partial y^2}-K_{c}C,$$

(14)

with imposed conditions

$$t\le 0,u\left(y,0\right) =0,T\left(y,0\right) = C\left(y,0\right) = 0,y\ge 0,$$

(15)

$$t>0,u\left(y,t\right) =f\left(t\right),T\left(y,t\right) =h\left(t\right),C\left(y,t\right) =g\left(t\right),y=0,$$

(16)

$$t>0,u\left(y,t\right)\to 0,T\left(y,t\right)\to 0,C\left(y,t\right)\to C,y\to \infty .$$

(17)

3. Method of Solution

3.1. Analyticity of Temperature Profile

The analyticity of the temperature distribution can be derived for the coupled equation by utilizing the Laplace transform on (13). Using appropriate conditions (15)–(17), we have

$$q\stackrel{‒}{T}\left(y,q\right)+S\stackrel{‒}{T}\left(y,q\right) =\frac{1}{P_r}\left(1+\frac{4}{3}{R}_d\right)\frac{{\partial }^2\stackrel{‒}{T}\left(y,q\right)}{\partial y^2}.$$

(18)

Equation (18) is a homogenous linear differential equation that can be evaluated by means of elementary approaches with appropriate conditions (15)–(17). The solution for Equation (18) is obtained as

$$\stackrel{‒}{T}\left(y,q\right) =H\left(q\right) \times e^{-y\sqrt{{Pr}_{0}q+{Pr}_{0}S}}$$

(19)

Taking inverse (19) by means of the Laplace transformation, we obtain a suitable result as

$$T\left(y,t\right)={{\displaystyle \int }}_0^t{h}^{\prime }\left(t-s\right) \times \Upsilon \left(y\sqrt{{Pr}_0},q,S,0\right)ds$$

(20)

Nusselt Number

To estimate the heat transfer rate, the Nusselt number is used, which is calculated as:

$$Nu=-{\left.\frac{\partial T\left(y,t\right)}{\partial y}\right|}_{y=0}.$$

3.2. Analyticity of Concentration Profile

The analyticity of the concentration field can be derived for the coupled equation by utilizing the Laplace transform on (14) using appropriate conditions (15)–(17). We arrive at

$$S_c\left(q+K_c\right)\stackrel{‒}{C}\left(y,q\right)=\frac{{\partial }^2\stackrel{‒}{C}\left(y,q\right)}{\partial y^2}.$$

(21)

Equation (21) is a homogenous linear differential equation that can be evaluated by means of elementary approaches with appropriate conditions (15)–(17). The solution of Equation (21) is obtained as

$$\stackrel{‒}{C}\left(y,q\right) = G\left(q\right)e^{-y\sqrt{S_{c}q+S_c{K}_c}}$$

(22)

Taking inverse (22) by means of the Laplace transformation, we obtain a suitable result as

$$C\left(y,t\right)={{\displaystyle \int }}_0^t{g}^{\prime }\left(t-s\right)\times \Upsilon \left(y\sqrt{S_c},q,K_c,0\right)ds,$$

(23)

where the property of special function used on (23) is defined as

$$\Upsilon \left(y,t,x,y,z\right)=L^{-1}\left(\frac{\exp -y\sqrt{z}\sqrt{s+y}}{s-x}\right),$$

(24)

$$\Upsilon \left(y,t,x,y,z\right)=\frac{e^{xt}}{2}\left(e^{-y\sqrt{z}\sqrt{x+y}}erfc\left(\frac{y\sqrt{z}}{2\sqrt{t}}-\sqrt{xt+yt}\right)+e^{y\sqrt{z}\sqrt{x+y}}erfc\left(\frac{y\sqrt{z}}{2\sqrt{t}}+\sqrt{xt+yt}\right)\right).$$

(25)

Sherwood Number

To estimate the mass transfer rate from plate to fluid, the Sherwood number is used, which is calculated as:

$$Sh=-{\left.\frac{\partial C\left(y,t\right)}{\partial y}\right|}_{y=0}.$$

3.3. Analyticity of Velocity Profile

The analyticity of the velocity field can be derived for coupled equations, and by utilizing the Laplace transform on (12) using appropriate conditions (15)–(17), we arrive at

$$\eta q\stackrel{‒}{u}\left(y,q\right) + \eta M_0\stackrel{‒}{u}\left(y,q\right)=\frac{{\partial }^2\stackrel{‒}{u}\left(y,q\right)}{\partial y^2} + \eta G_{r}cos\gamma \stackrel{‒}{T}\left(y,q\right) + \eta G_{c}cos\gamma \stackrel{‒}{C}\left(y,q\right).$$

(26)

The general solution of (26) after the substitution of (19) and (22) is

$$\stackrel{‒}{u}\left(y,q\right) = c_1{e}^{y\sqrt{\eta \left(q+M_0\right)}}+c_2{e}^{-y\sqrt{\eta \left(q+M_0\right)}}-\frac{\eta G_{r}cos\gamma H\left(q\right)e^{-y\sqrt{{Pr}_0(q+S)}}}{a_{1}q+a_2}-\frac{\eta G_{c}cos\gamma G\left(q\right)e^{-y\sqrt{S_{c}q+S_c{K}_c}}}{a_{3}q+a_4}.$$

(27)

Using boundary conditions, the final form of the velocity profile is

$$\begin{gathered} \stackrel{‒}{u}\left(y,q\right) = F\left(q\right)e^{-y\sqrt{\eta \left(q+M_0\right)}}+\frac{\eta G_{r}cos\gamma H\left(q\right)}{a_1\left(q+A_1\right)}\left(e^{y\sqrt{\eta \left(q+M_0\right)}}-e^{-y\sqrt{{Pr}_0(q+S)}}\right) \\ +\frac{\eta G_{c}cos\gamma G\left(q\right)}{a_3\left(q+A_2\right)}\left(e^{y\sqrt{\eta \left(q+M_0\right)}}-e^{-y\sqrt{S_{c}q+S_c{K}_c}}\right). \end{gathered}$$

(28)

Here, we generate some letting parameters to avoid lengthy and cumbersome calculations

$$\begin{gathered} M_0=M+\frac{1}{\eta k_1},{Pr}_0=\frac{P_r}{1+\frac{4}{3}{R}_d},a_1={Pr}_0-\eta ,a_2=S{Pr}_0-\eta M_0, \\ {a}_3=S_c-\eta ,a_2=S_c{K}_c-\eta M_0,A_1=\frac{a_2}{a_1},A_2=\frac{a_4}{a_3},\frac{1}{\eta }=1+\frac{1}{\lambda }. \end{gathered}$$

(29)

Taking inverse (27) by means of the Laplace transformation, we obtain a suitable result as

$$\begin{gathered} u\left(y,t\right) ={{\displaystyle \int }}_0^t{f}^{\prime }\left(t-s\right)\Upsilon \left(y,q,0,M_0,\eta \right)ds+\frac{\eta G_{r}cos\gamma }{a_1}{{\displaystyle \int }}_0^t{h}^{\prime }\left(t-s\right)\left(\Upsilon \left(y,q,-A_1,M_0,\eta \right)-\Upsilon \left(y,q,-A_1,S,{Pr}_0\right)\right)ds \\ +\frac{\eta G_{c}cos\gamma }{a_3}{{\displaystyle \int }}_0^t{g}^{\prime }\left(t-s\right)\left(\Upsilon \left(y,q,-A_2,M_0,\eta \right) - \Upsilon \left(y,q,-A_2,K_c,S_c\right)\right)ds. \end{gathered}$$

(30)

4. Special Cases

It is further noted that some interesting solutions can be recovered from Equation (30), which represents the final solution of velocity with the generalized boundary conditions on temperature, concentration, and velocity. For the sake of new solutions on the basis of generalized boundary conditions, we consider $H\left(q\right) = G\left(q\right) = \frac{1}{q}$ in Equation (28), and we arrive at

$$\begin{array}{cc}\hfill \stackrel{‒}{u}\left(y,q\right) =& F\left(q\right)e^{-y\sqrt{\eta q+\eta M_0}}+\frac{\eta G_{r}cos\gamma }{a_1{A}_1}\left(\frac{e^{-y\sqrt{\eta q+\eta M_0}}}{q}-\frac{e^{-y\sqrt{{Pr}_{0}q+{Pr}_{0}S}}}{q}\right)\\ & -\frac{\eta G_{r}cos\gamma }{a_1{A}_1}\left(\frac{e^{-y\sqrt{\eta q+\eta M_0}}}{q+A_1}-\frac{e^{-y\sqrt{{Pr}_{0}q+{Pr}_{0}S}}}{q+A_1}\right)\hfill \\ & -\frac{\eta G_{r}cos\gamma }{a_1{A}_1}\left(\frac{e^{-y\sqrt{\eta q+\eta M_0}}}{q+A_1}-\frac{e^{-y\sqrt{{Pr}_{0}q+{Pr}_{0}S}}}{q+A_1}\right)\hfill \\ & +\frac{\eta G_{c}cos\gamma }{a_3{A}_2}\left(\frac{e^{-y\sqrt{\eta q+\eta M_0}}}{q}-\frac{e^{-y\sqrt{S_{c}q+S_c{K}_c}}}{q}\right)\hfill \\ & -\frac{\eta G_{c}cos\gamma }{a_3{A}_2}\left(\frac{e^{-y\sqrt{\eta q+\eta M_0}}}{q+A_2}-\frac{e^{-y\sqrt{S_{c}q+S_c{K}_c}}}{q+A_2}\right).\hfill \end{array}$$

(31)

The inverse Laplace of Equation (31) using (24) and (25) is

$$\begin{array}{c}u\left(y,t\right) = {{\displaystyle \int }}_0^t{f}^{\prime }\left(t-s\right) \times \Upsilon \left(y,q,0,M_0,\eta \right)ds\hfill \\ +\frac{\eta G_{r}cos\gamma }{a_1{A}_1}\left(\Upsilon \left(y,q,0,M_0,\eta \right) - \Upsilon \left(y,q,0,S,{Pr}_0\right)\right)\hfill \\ +\frac{\eta G_{r}cos\gamma }{a_1{A}_1}\left(\Upsilon \left(y,q,-A_1,M_0,\eta \right) - \Upsilon \left(y,q,-A_1,S,{Pr}_0\right)\right)\hfill \\ +\frac{\eta G_{c}cos\gamma }{a_3{A}_2}\left(\Upsilon \left(y,q,-A_2,M_0,\eta \right) - \Upsilon \left(y,q,-A_2,K_c,S_c\right)\right)\hfill \\ +\frac{\eta G_{c}cos\gamma }{a_3{A}_2}\left(\Upsilon \left(y,q,0,M_0,\eta \right) - \Upsilon \left(y,q,0,K_c,S_c\right)\right).\hfill \end{array}$$

(32)

The different cases of the velocity field can be considered using Equation (31).

4.1. Motion of Plate with Constant Velocity

The solution for the motion of plate can be achieved by setting $F\left(t\right) = U_{0}H\left(t\right)$. We recover the solution as

$$\begin{array}{c}u\left(y,t\right) = \Upsilon \left(y,q,0,M_0,\eta \right)+\frac{\eta G_{r}cos\gamma }{a_1{A}_1}\left(\Upsilon \left(y,q,0,M_0,\eta \right) - \Upsilon \left(y,q,0,S,{Pr}_0\right)\right)\\ +\frac{\eta G_{r}cos\gamma }{a_1{A}_1}\left(\Upsilon \left(y,q,-A_1,M_0,\eta \right) - \Upsilon \left(y,q,-A_1,S,{Pr}_0\right)\right)\hfill \\ +\frac{\eta G_{c}cos\gamma }{a_3{A}_2}\left(\Upsilon \left(y,q,-A_2,M_0,\eta \right) - \Upsilon \left(y,q,-A_2,K_c,S_c\right)\right)\hfill \\ +\frac{\eta G_{c}cos\gamma }{a_3{A}_2}\left(\Upsilon \left(y,q,0,M_0,\eta \right) - \Upsilon \left(y,q,0,K_c,S_c\right)\right).\hfill \end{array}$$

(33)

4.2. Motion of Plate with Linear Velocity

The solution for the motion of the plate can be achieved by setting $F\left(t\right) = t$. We recover the solution as

$$\begin{array}{c}u\left(y,t\right) = {{\displaystyle \int }}_0^t\Upsilon \left(y,q,0,M_0,\eta \right) + \frac{\eta G_{r}cos\gamma }{a_1{A}_1}\left(\Upsilon \left(y,q,0,M_0,\eta \right) - \Upsilon \left(y,q,0,S,{Pr}_0\right)\right)\\ +\frac{\eta G_{r}cos\gamma }{a_1{A}_1}\left(\Upsilon \left(y,q,-A_1,M_0,\eta \right) - \Upsilon \left(y,q,-A_1,S,{Pr}_0\right)\right)\hfill \\ +\frac{\eta G_{c}cos\gamma }{a_3{A}_2}\left(\Upsilon \left(y,q,-A_2,M_0,\eta \right) - \Upsilon \left(y,q,-A_2,K_c,S_c\right)\right)\hfill \\ +\frac{\eta G_{c}cos\gamma }{a_3{A}_2}\left(\Upsilon \left(y,q,0,M_0,\eta \right) - \Upsilon \left(y,q,0,K_c,S_c\right)\right).\hfill \end{array}$$

(34)

4.3. Motion of Plate with Exponential Acceleration

The solution for the motion of the plate can be achieved by setting $F\left(t\right) = e^{wt}$. We recover the solution as

$$\begin{array}{c}u\left(y,t\right) = \Upsilon \left(y,q,w,M_0,\eta \right)+\frac{\eta G_{r}cos\gamma }{a_1{A}_1}\left(\Upsilon \left(y,q,0,M_0,\eta \right) - \Upsilon \left(y,q,0,S,{Pr}_0\right)\right)\\ +\frac{\eta G_{r}cos\gamma }{a_1{A}_1}\left(\Upsilon \left(y,q,-A_1,M_0,\eta \right) - \Upsilon \left(y,q,-A_1,S,{Pr}_0\right)\right)\hfill \\ +\frac{\eta G_{c}cos\gamma }{a_3{A}_2}\left(\Upsilon \left(y,q,-A_2,M_0,\eta \right) - \Upsilon \left(y,q,-A_2,K_c,S_c\right)\right)\hfill \\ +\frac{\eta G_{c}cos\gamma }{a_3{A}_2}\left(\Upsilon \left(y,q,0,M_0,\eta \right) - \Upsilon \left(y,q,0,K_c,S_c\right)\right).\hfill \end{array}$$

(35)

4.4. Motion of Plate with Sinusoidal Oscillation

The solution for the motion of the plate can be achieved by setting $F\left(t\right) = cos(wt)$. We recover the solution as

$$\begin{array}{c}u\left(y,t\right) = \frac{1}{2}\left(\Upsilon \left(y,q,iw,M_0,\eta \right) = \Upsilon \left(y,q,-iw,M_0,\eta \right)\right)\\ +\frac{\eta G_{r}cos\gamma }{a_1{A}_1}\left(\Upsilon \left(y,q,0,M_0,\eta \right) = \Upsilon \left(y,q,0,S,{Pr}_0\right)\right)\hfill \\ +\frac{\eta G_{r}cos\gamma }{a_1{A}_1}\left(\Upsilon \left(y,q,-A_1,M_0,\eta \right) = \Upsilon \left(y,q,-A_1,S,{Pr}_0\right)\right)\hfill \\ +\frac{\eta G_{c}cos\gamma }{a_3{A}_2}\left(\Upsilon \left(y,s,-A_2,M_0,\eta \right) = \Upsilon \left(y,q,-A_2,K_c,S_c\right)\right)\hfill \\ +\frac{\eta G_{c}cos\gamma }{a_3{A}_2}\left(\Upsilon \left(y,q,0,M_0,\eta \right) = \Upsilon \left(y,q,0,K_c,S_c\right)\right).\hfill \end{array}$$

(36)

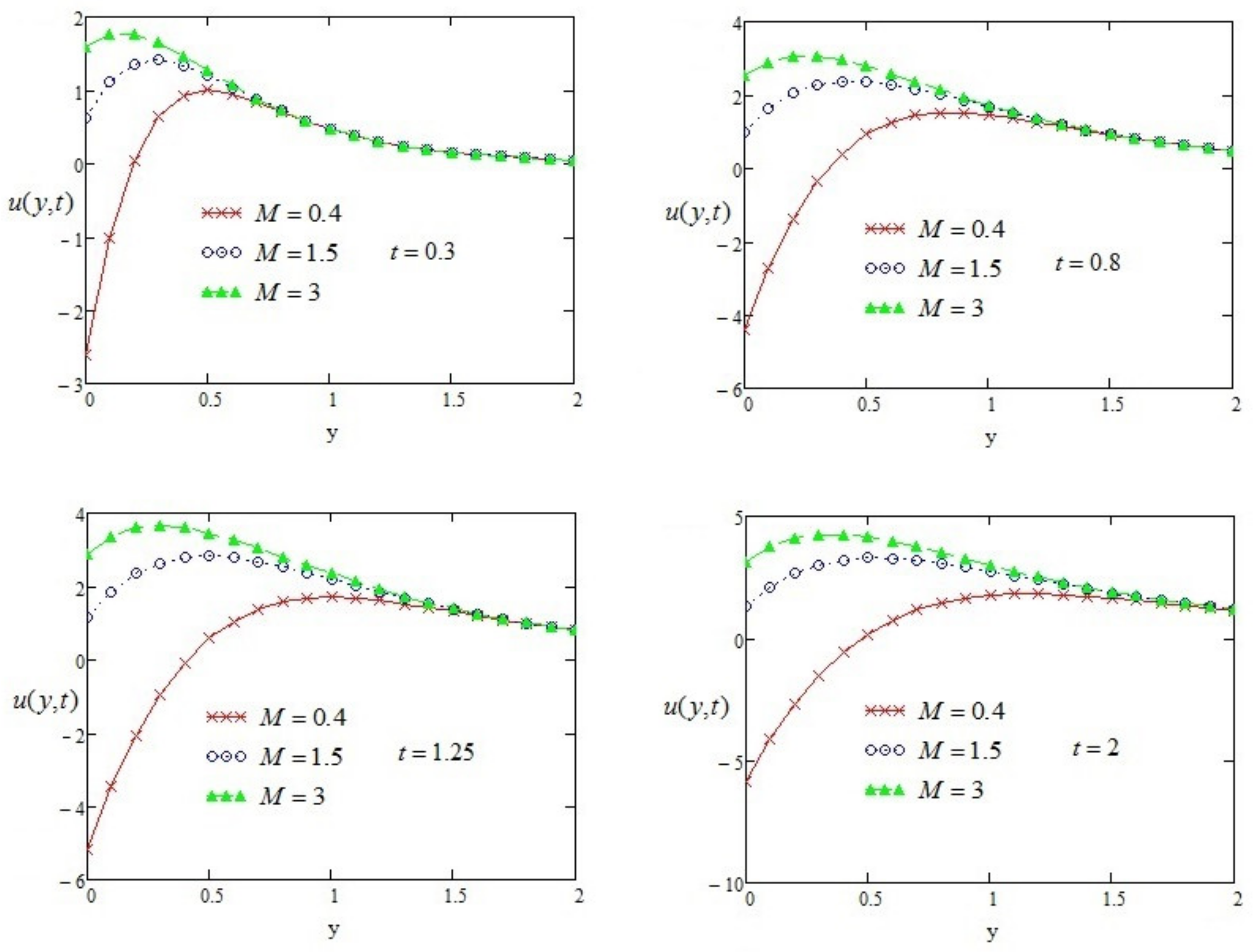
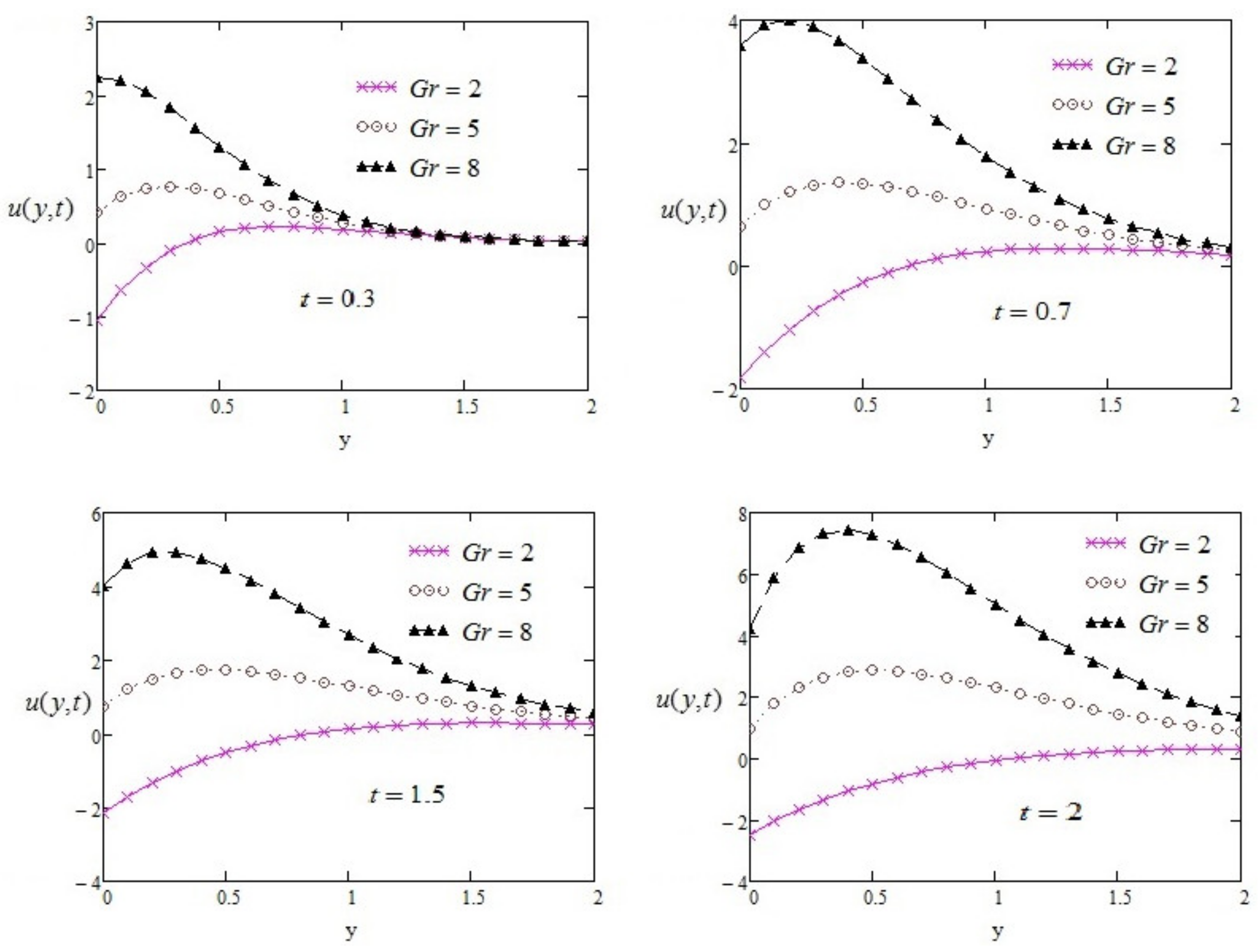
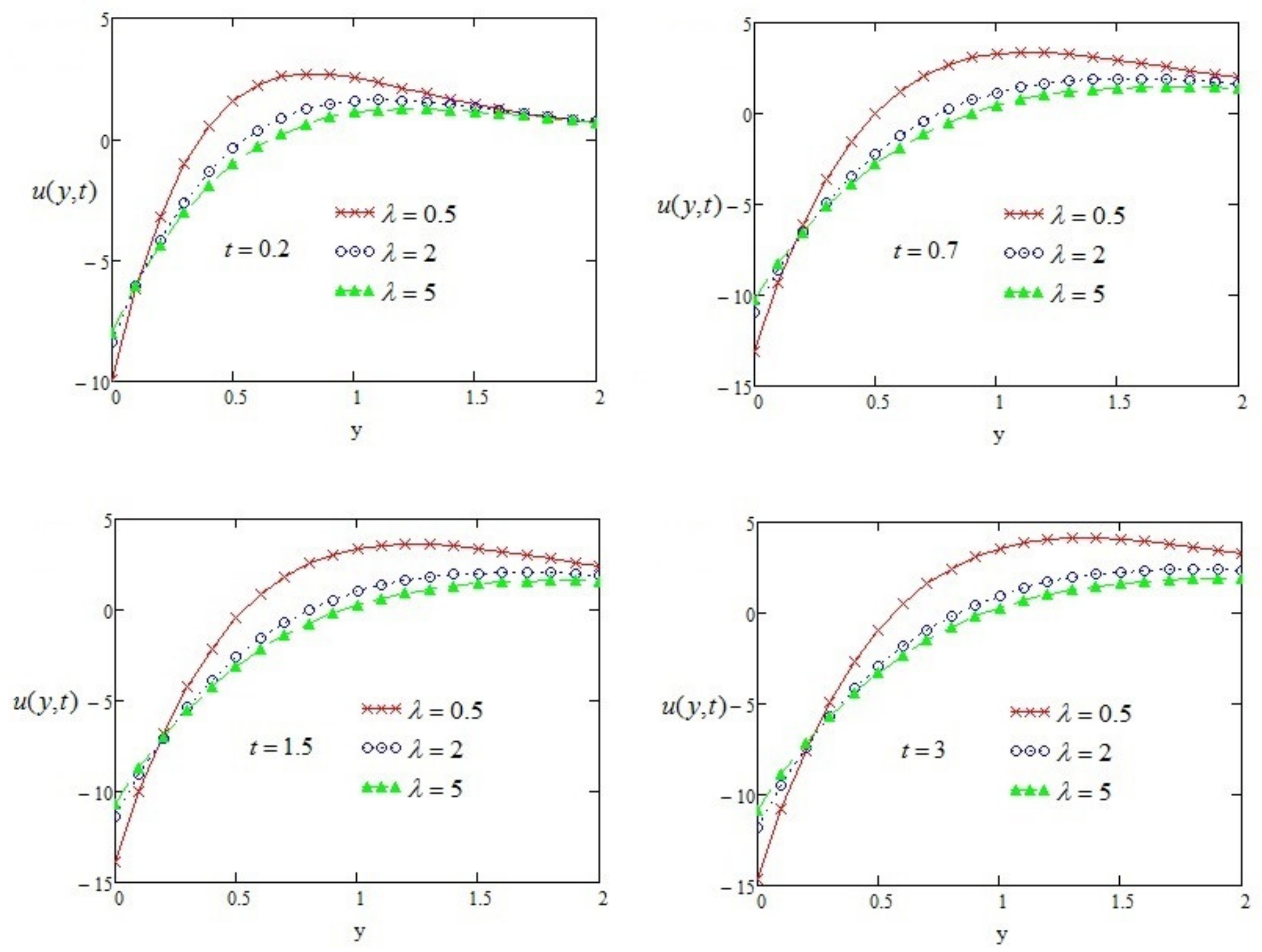
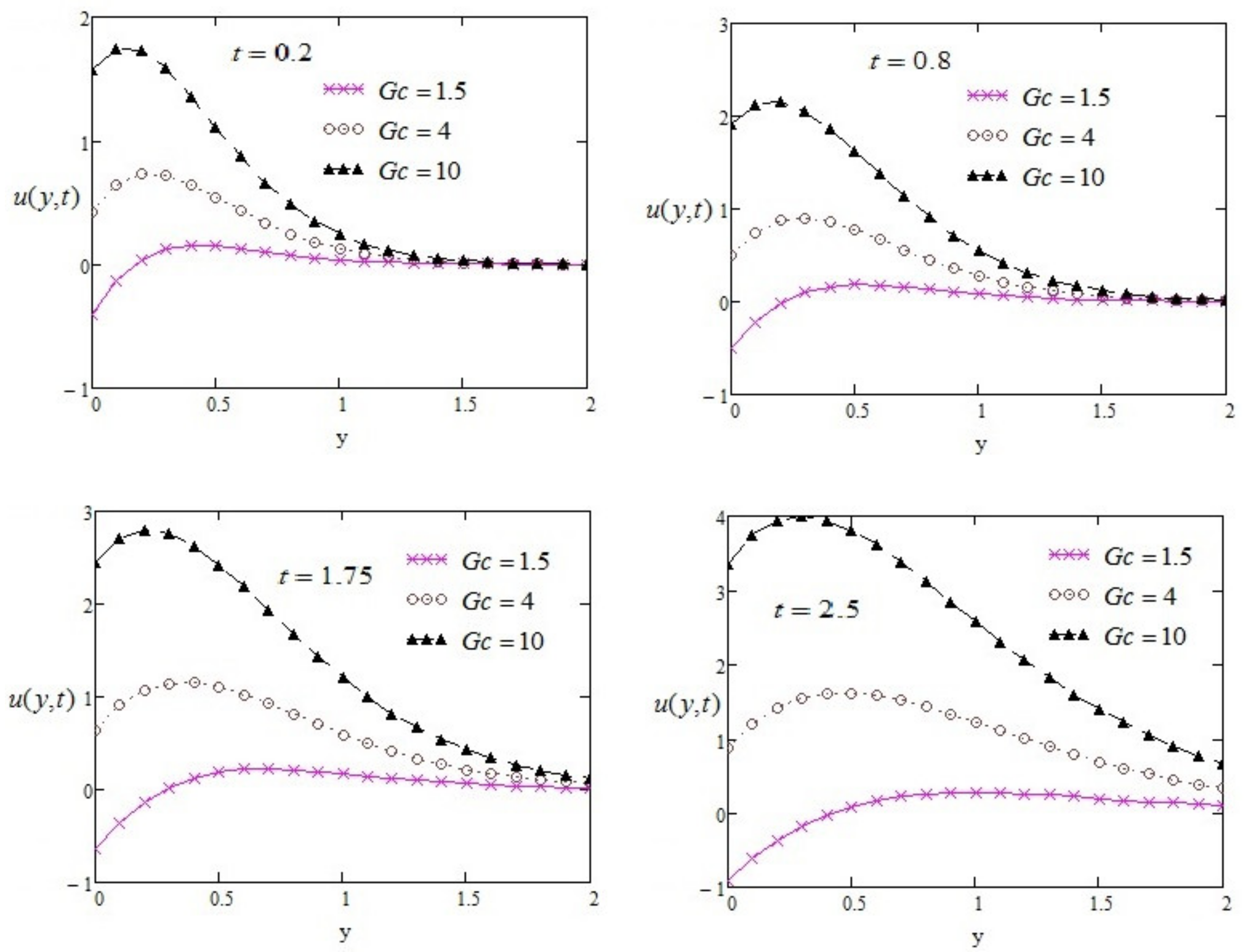
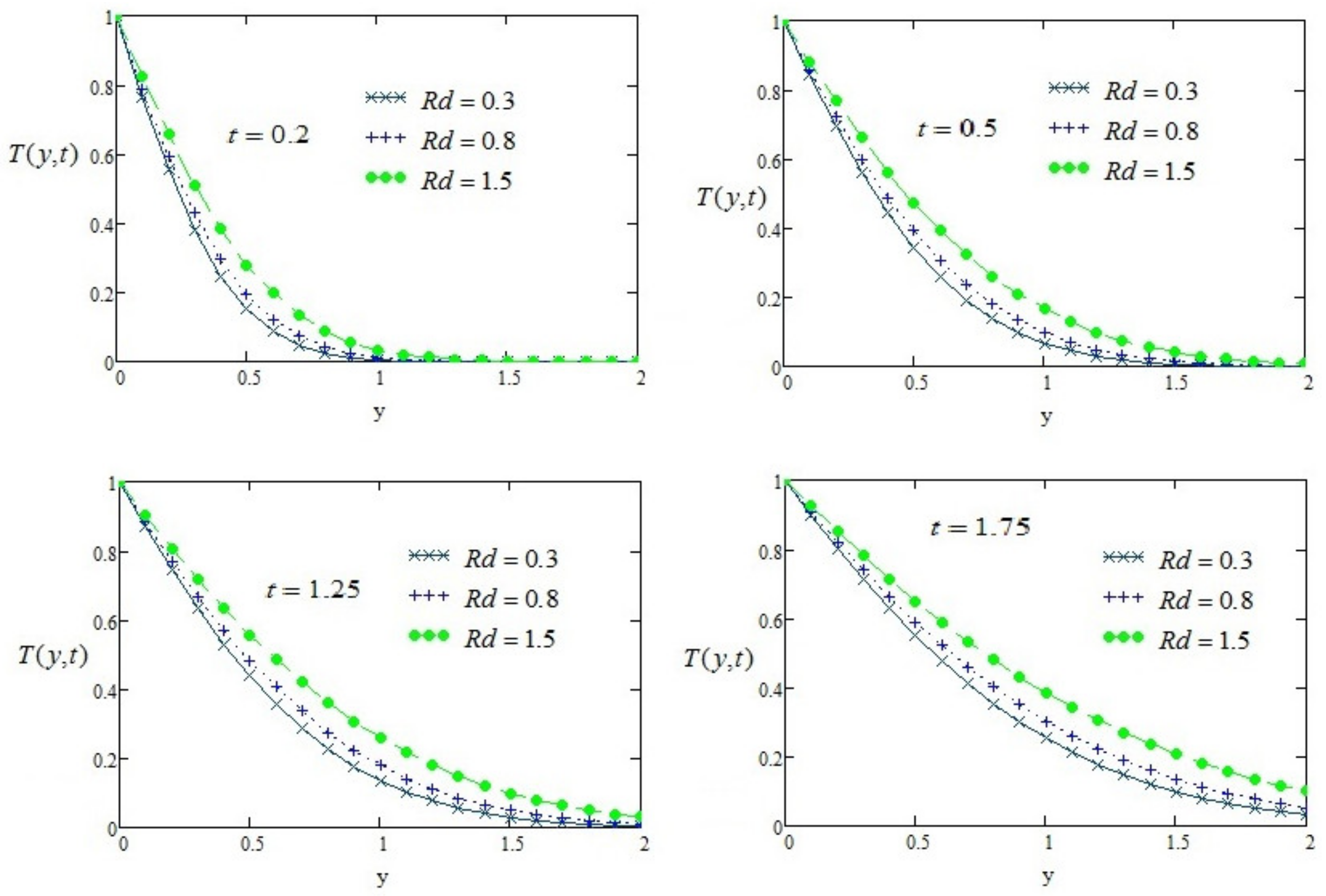
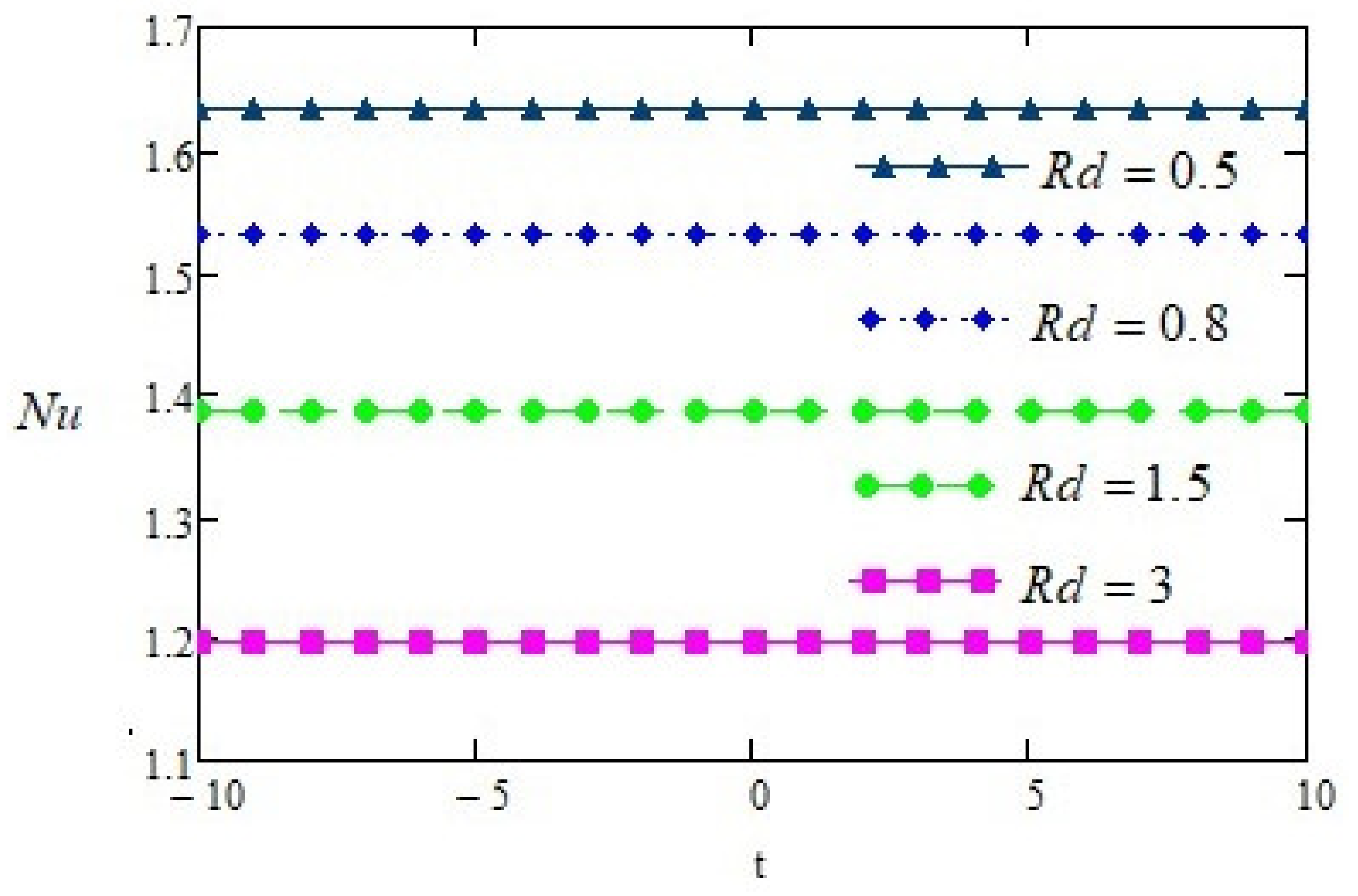
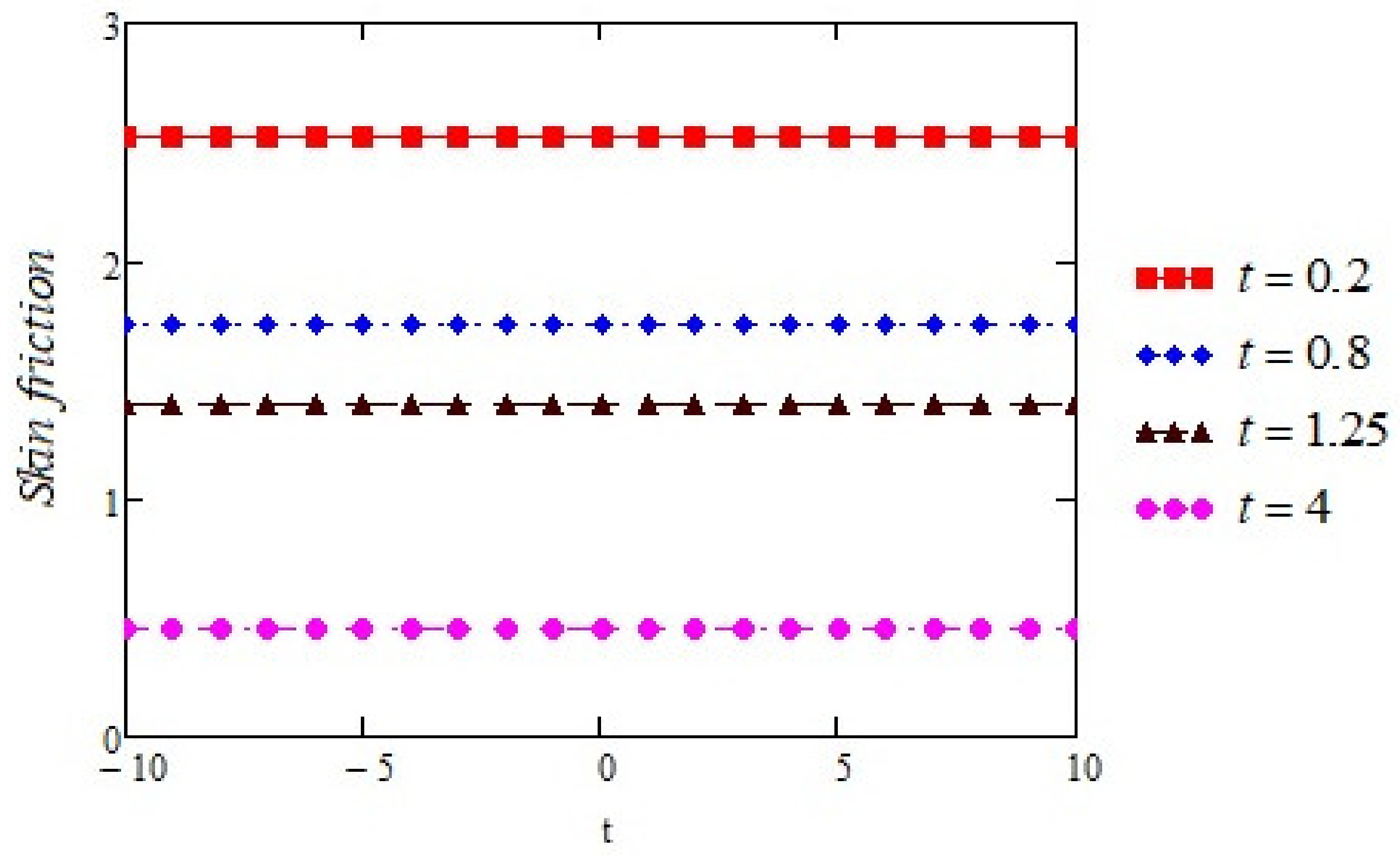
5. Results and Discussion

This section is devoted to the physical interpretation of the heat and mass transfer executed on the motion of free-convection MHD Casson fluid through a limitless plate with porous medium. The impact of thermal radiation, chemical reactions, and magnetic fields are also analyzed via the Laplace transformation to obtain a unique solution. The impact of physical parameters such as $Pr,M,Gc, Gr,K, \lambda$ on the energy velocity profile is discussed with a graphical approach using MATHCAD-15.

Figure 2 investigates the domination of M on the velocity components. With an increase in M, the velocity decreases due to force. It behaves as a drag force. By enhancing the value of M, the Lorentz force also increases. Fluid flow on the boundary layer is slowed down due to this force. It is perceived that the behavior of the fluid profile is effective in the classical model.

Figure 3 analyzes the effect of Casson parameter $\lambda$ on the velocity field. The magnitude of the velocity field enlarges with a small value of $\lambda$. For a large value of $\lambda$, the boundary layer thickness is minimized, which helps to reduce the velocity.

Thermal and isothermal conditions represent the domination of $Gr$, as shown in Figure 4. Physically, $Gr$ shows the relation between thermal and viscous forces. For the variation of time, the behavior of the velocities is unique. The influence of $Gc$ is illustrated in Figure 5. It can be noticed that the resultant velocity increases by enhancing $Gc$. It can also be seen that velocity increases with an increase in time. The behaviors of $Gr$ and $Gc$ on the velocity profile are the same. Figure 6 analyzes the variation of $Rd$ on the velocity with the help of time. The large value of radiation parameter $Rd$ causes an increase in fluid flow. The rate of energy transport of the fluid increases due to an increase in the intensity of the radiation parameter and a decrease in viscosity. Due to such behavior, the fluid moves faster and enhances the fluid velocities. The domination of chemical reaction $Kc$ on the concentration field is discussed in Figure 7. With a large value of $Kc$, the concentration profile decreases. The positive value of $Kc$ is analyzed as destructive, and the negative value behaves as productive. Figure 8 displays the Nusselt number Nu against time, varying the radiation parameter. It is clear that with a fixed value of Rd, Nu remains constant when changing the value of t. By increasing the value of Rd, Nu drops to smaller values, maintaining the same constant trend with increasing t. This shows that the changing radiation parameter values decrease the convective thermal energy flow. Figure 9 shows the effect of skin friction with the variation of time. By increasing the value of time, skin friction drops to a smaller value. This shows that skin friction remains constant with the effect of the variation of time. The existence of the Prandtl number may reflect the control of the thickness of the momentum and the enlargement of thermal conductivity. The value of Prandtl number $Pr$ is fixed. The Prandtl number for water at 17 °C is 7.56 and for air at room temperature is 0.7. The Prandtl number is $Pr=12$ for non-Newtonian fluid.

6. Conclusions

The exact symmetrical and closed-form solution of MHD Casson fluid with chemically reactive flow was analyzed by the Laplace transformation. Generalized boundary conditions along the infinite plate were taken. The graphical approach was used to discuss the influence of the dimensionless parameter on fluid velocity. Key points can be taken from the graphical discussion:

(a)

Velocity can be elevated to enhance the values of the thermal Grashof number (Gr) and the mass Grashof number (Gc).

(b)

Thermal radiation plays a significant role in the development of thermal and momentum boundary layers.

(c)

Velocity can be de-accelerated to enhance the values of the magnetic field (M).

(d)

Temperature can be elevated to enhance the values of the radiation parameter (Rd).

(e)

The concentration field can be reduced by enhancing the value of the chemical reaction parameter Kc.

(f)

The magnitude of the velocity field is enhanced with a small value of $\lambda$.

(g)

The Nusselt number shows the opposite behavior with a higher value of Rd.

(h)

The value of the Prandtl number $Pr$ is 12 for non-Newtonian fluids.