Towards Electrothermal Optimization of a HVDC Cable Joint Based on Field Simulation
Abstract
1. Introduction
- Electrical, thermal, and mechanical stability for all operating conditions including transient overvoltages,
- Minimal Joule losses and thus minimal heat production in the insulation material,
- Lifetime of several decades with an uncritical aging behavior.
2. Electric Field Grading and Cable Joint Model
3. Electrothermal Problem Formulation
4. Simulation Results
4.1. Validation and Steady State Operation
4.2. Optimization of Field Grading Material Layer
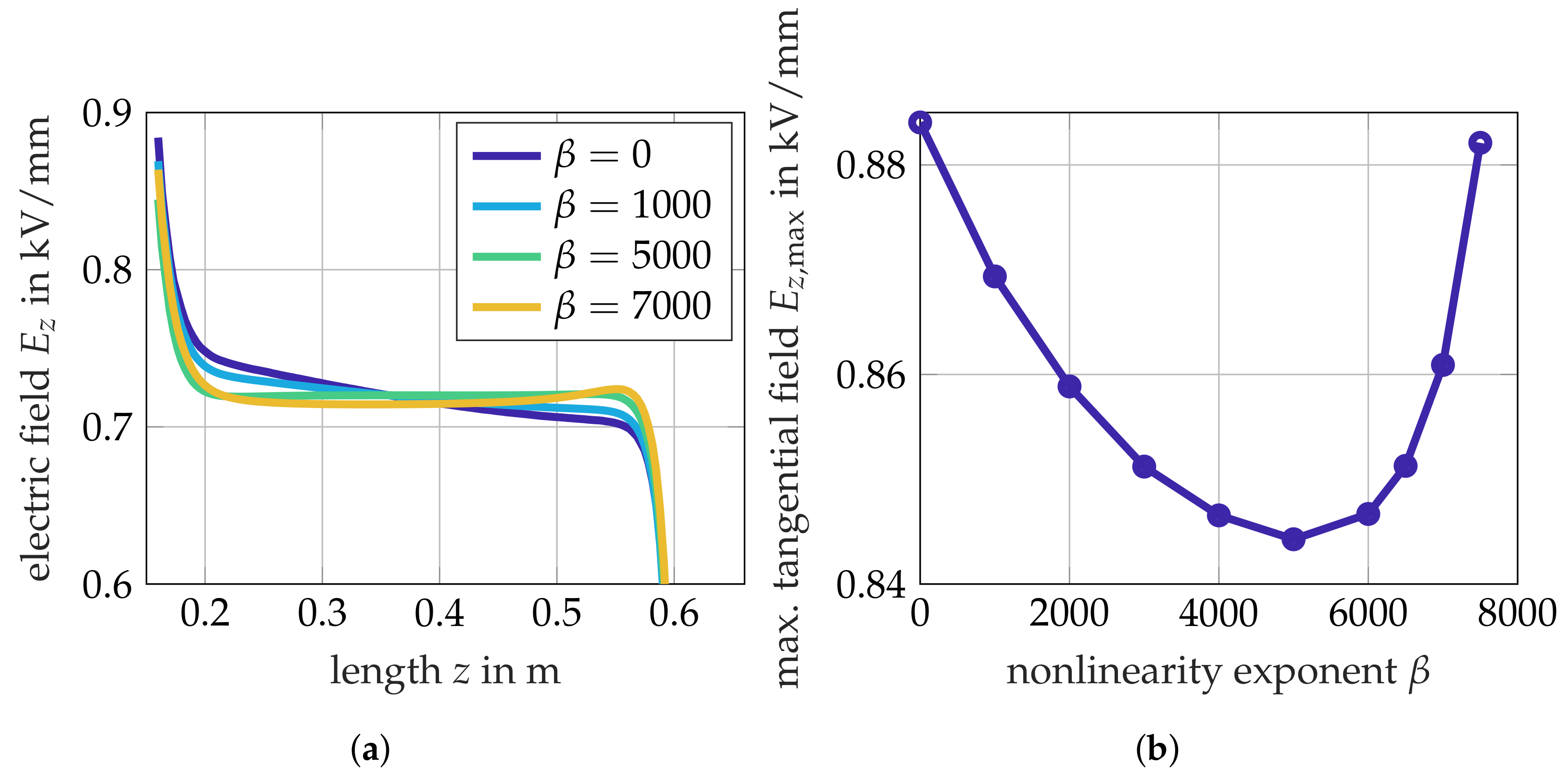
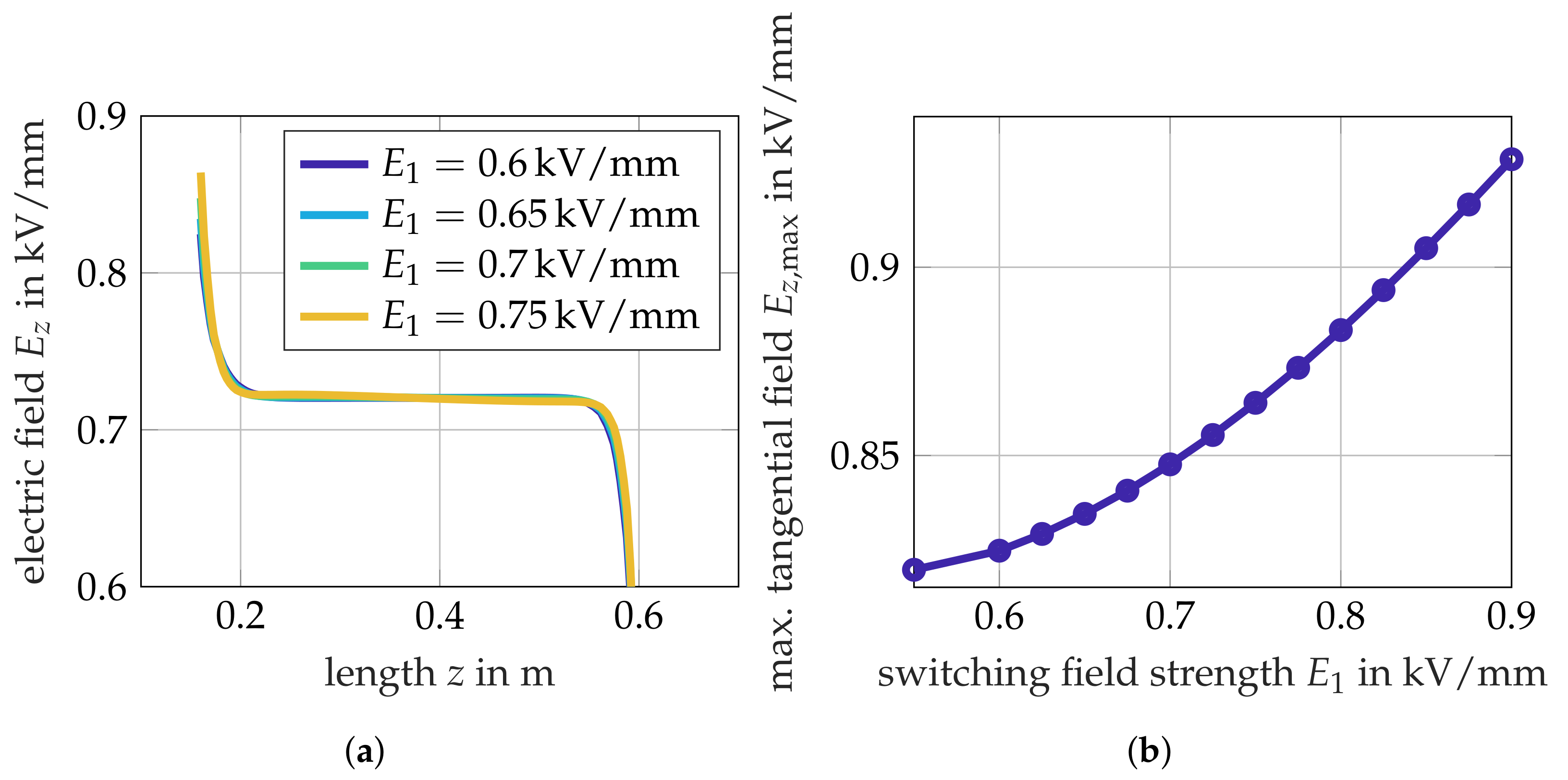
- Maximal tangential electric field strength along the interface between XLPE and FGM (see Figure 1);
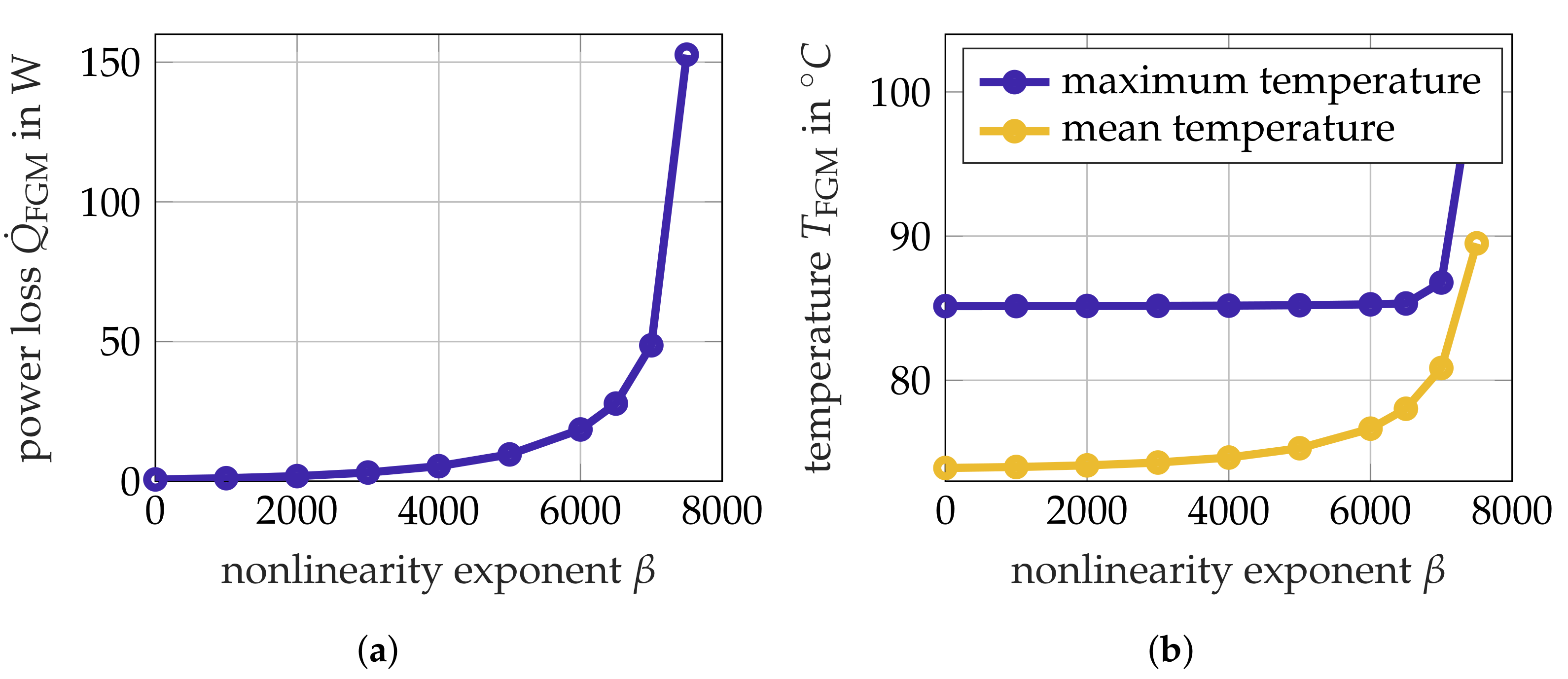
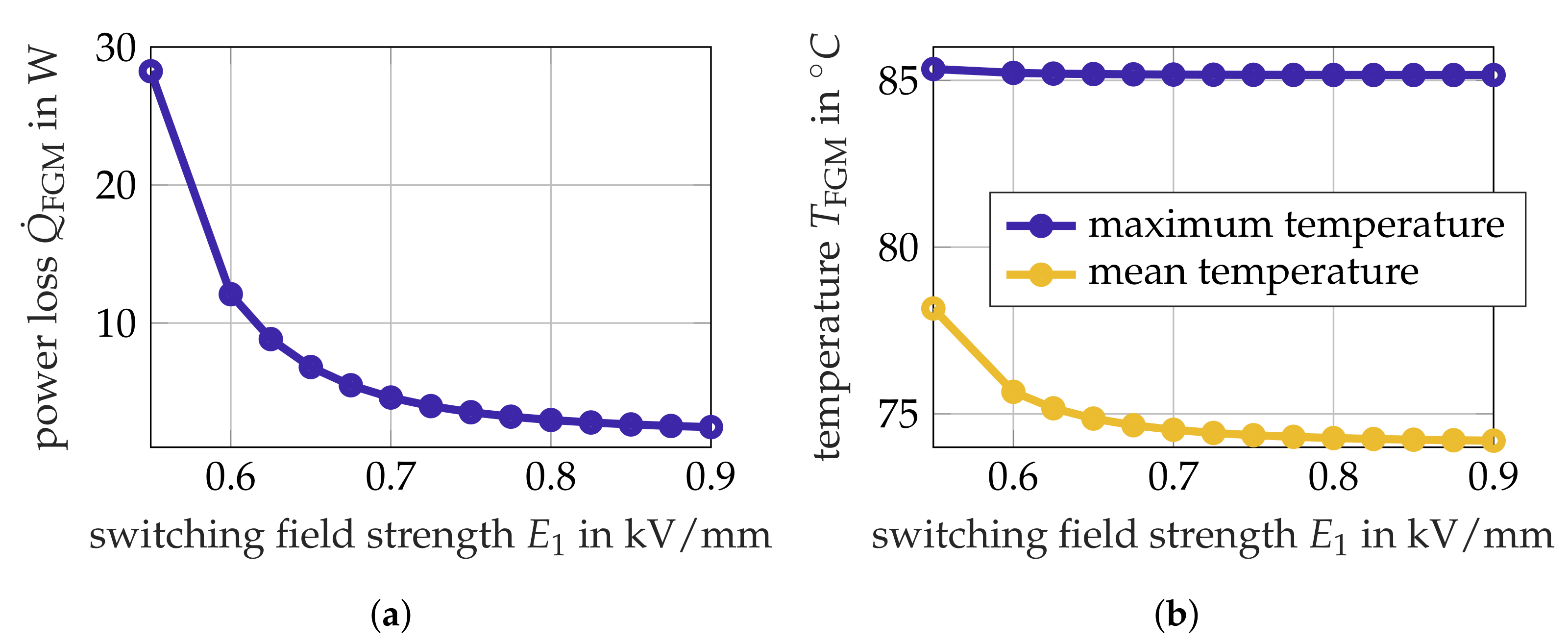
- Electric losses inside the FGM;
- Mean and maximum temperature, , inside the FGM.
4.2.1. Base Conductivity
4.2.2. Temperature Nonlinearity Exponent
4.2.3. Switching Field Strength
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Cigré Working Group D1.56. Field grading in Electrical Insulation Systems; Number TB794; Conseil International des Grands Réseaux Électriques: Paris, France, 2020. [Google Scholar]
- Ghorbani, H.; Jeroense, M.; Olsson, C.O.; Saltzer, M. HVDC Cable Systems—Highlighting Extruded Technology. IEEE Trans. Power Deliv. 2014, 29, 414–421. [Google Scholar] [CrossRef]
- Chen, G.; Hao, M.; Xu, Z.; Vaughan, A.; Cao, J.; Wang, H. Review of high voltage direct current cables. CSEE J. Power Energy Syst. 2015, 1, 9–21. [Google Scholar] [CrossRef]
- 50hertz; Tennet. SuedOstLink: Erstmals Vergabe kunststoffisolierter Erdkabel für 525 Kilovolt Spannung. press release. 2020. [Google Scholar]
- Jörgens, C.; Clemens, M. A Review about the Modeling and Simulation of Electro-Quasistatic Fields in HVDC Cable Systems. Energies 2020, 13, 5189. [Google Scholar] [CrossRef]
- IEC 62067. Power Cables with Extruded Insulation and Their Accessories for Rated Voltages above 150 kV (Um = 170 kV) up to 500 kV (Um = 550 kV)—Test Methods and Requirements. International Standard, VDE Verlag: Berlin, Germany, 2011. Available online: https://webstore.iec.ch/preview/info_iec62067%7Bed2.0.RLV%7Den.pdf (accessed on 13 May 2021).
- IEC 60840. Power Cables with Extruded Insulation and Their Accessories for Rated Voltages above 30 kV (Um = 36 kV) up to 150 kV (Um = 170 kV)—Test Methods and Requirements. International Standard, VDE Verlag: Berlin, Germany, 2020. Available online: https://webstore.iec.ch/publication/63025 (accessed on 13 May 2021).
- IEC 62895. High Voltage Direct Current (HVDC) Power Transmission—Cables with Extruded Insulation and Their Accessories for Rated Voltages up to 320 kV for Land Applications—Test Methods and Requirements. International Standard, VDE Verlag: Berlin, Germany, 2017. Available online: https://webstore.iec.ch/publication/34020 (accessed on 13 May 2021).
- Cigré Working Group B1.32. Recommendations for Testing DC Extruded Cable Systems for Power Transmission at a Rated Voltage up to 500 kV; Number TB496; Conseil International des Grands réseaux Électriques: Paris, France, 2012. [Google Scholar]
- Gießel, M.; Hinrichsen, V.; Göhler, R.; Späck-Leigsnering, Y.; Gjonaj, E.; De Gersem, H. Electro-Thermal Simulations of High Voltage Metal-Oxide Surge Arresters with and without installed Grading Rings with regard to Thermal Stability. In Proceedings of the CIGRÉ Winnipeg 2017 Colloquium, Winnipeg, MB, Canada, 30 September 2017. [Google Scholar]
- Späck-Leigsnering, Y.; Gjonaj, E.; De Gersem, H. Electrothermal Optimization of Field Grading Systems of Station Class Surge Arresters. IEEE J. Multiscale Multiphy. Comput. Tech. 2019, 4, 29–36. [Google Scholar] [CrossRef]
- Clemens, M.; Gjonaj, E.; Pinder, P.; Weiland, T. Numerical simulation of coupled transient thermal and electromagnetic fields with the finite integration method. IEEE Trans. Magn. 2000, 36, 1448–1452. [Google Scholar] [CrossRef]
- Munteanu, I.; Timm, M.; Weiland, T. It’s About Time. IEEE Microw. Mag. 2010, 11, 60–69. [Google Scholar] [CrossRef]
- Späck-Leigsnering, Y.; Gjonaj, E.; De Gersem, H.; Weiland, T.; Gießel, M.; Hinrichsen, V. Electroquasistatic-thermal modeling and simulation of station class surge arresters. IEEE Trans. Magn. 2016, 52, 1–4. [Google Scholar] [CrossRef]
- Boggs, S.; Damon, D.; Hjerrild, J.; Holboll, J.; Henriksen, M. Effect of insulation properties on the field grading of solid dielectric DC cable. IEEE Trans. Power Deliv. 2001, 16, 456–461. [Google Scholar] [CrossRef]
- Qi, X.; Zheng, Z.; Boggs, S. Engineering with nonlinear dielectrics. IEEE Electr. Insul. Mag. 2004, 20, 27–34. [Google Scholar] [CrossRef]
- Bodega, R. Space Charge Accumulation in Polymeric High Voltage DC Cable Systems. Ph.D. Thesis, Delft University of Technology, Delft, The Netherlands, 2006. [Google Scholar]
- Jörgens, C.; Clemens, M. Conductivity-based model for the simulation of homocharges and heterocharges in XLPE high-voltage direct current cable insulation. IET Sci. Meas. Technol. 2019, 13, 975–983. [Google Scholar] [CrossRef]
- Jörgens, C.; Kasolis, F.; Clemens, M. Numerical Simulations of Temperature Stability Limits in High-Voltage Direct Current Cable Insulations. IEEE Trans. Magn. 2019, 55, 1–4. [Google Scholar] [CrossRef]
- Frobin, S.J.; Freye, C.; Niedik, C.F.; Jenau, F.; Haering, D.; Schroeder, G. Thermal Modelling of HVDC Cables under Consideration of Measured Temperature Profiles. In Proceedings of the VDE ETG—Fachtagung Hochspannungstechnik 2018, Berlin, Germany, 12–14 November 2018. [Google Scholar]
- Saltzer, M.; Christen, T.; Sörqvist, T.; Jeroense, M. Electro-thermal simulations of HVDC cable joints. In Proceedings of the ETG Workshop Feldsteuernde Isoliersysteme, Darmstadt, Germany, 22–23 November 2011. [Google Scholar]
- Frobin, S.J.; Niedik, C.F.; Freye, C.; Jenau, F.; Haring, D.; Schroder, G. A Generic Approach for HVDC Cable Accessories Modelling. In Proceedings of the 2018 IEEE 2nd International Conference on Dielectrics (ICD), Budapest, Hungary, 1–5 July 2018. [Google Scholar] [CrossRef]
- Secklehner, M.; Hussain, R.; Hinrichsen, V. Tailoring of new field grading materials for HVDC systems. In Proceedings of the 2017 INSUCON—13th International Electrical Insulation Conference (INSUCON), Birmingham, UK, 16–18 May 2017. [Google Scholar] [CrossRef]
- Hussain, R.; Hinrichsen, V. Simulation of thermal behavior of a 320 kV HVDC cable joint with nonlinear resistive field grading under impulse voltage stress. In Proceedings of the CIGRÉ Winnipeg 2017 Colloquium, Winnipeg, MB, Canada, 30 September 2017. [Google Scholar]
- Jörgens, C.; Clemens, M. Comparison of Two Electro-Quasistatic Field Formulations for the Computation of Electric Field and Space Charges in HVDC Cable Systems. In Proceedings of the 2019 22nd International Conference on the Computation of Electromagnetic Fields (COMPUMAG), Paris, France, 15–19 July 2019. [Google Scholar] [CrossRef]
- Mazzanti, G.; Marzinotto, M. Extruded Cables for High-Voltage Direct-Current Transmission: Advances in Research and Development; Wiley: Hoboken, NJ, USA, 2013. [Google Scholar]
- Küchler, A. High Voltage Engineering: Fundamentals–Technology–Applications, 1st ed.VDI-Buch: Berlin, Germany, 2018. [Google Scholar]
- Farahani, M.; Werle, P.; Hohloch, J.; Hutt, W. Untersuchungen zur Durchschlagfestigkeit von Polyethylen-Silikon-Grenzflächen. In Proceedings of the VDE ETG—Fachtagung Hochspannungstechnik 2018, Berlin, Germany, 12–14 November 2018. [Google Scholar]
- Kaumanns, J. HVDC Cable Systems—State of the Art Technologies and Future Trends. In Proceedings of the International High Voltage Direct Current 2015 Conference, Seoul, Korea, 18–22 October 2015. [Google Scholar]
- Cigré Working Group B1.22. Cable Accessory Workmanship on Extruded High Voltage Cables; Number TB476; Conseil International des Grands Réseaux Électriques: Paris, France, 2011. [Google Scholar]
- Pradhan, M.; Greijer, H.; Eriksson, G.; Unge, M. Functional behaviors of electric field grading composite materials. IEEE Trans. Dielectr. Electr. Insul. 2016, 23, 768–778. [Google Scholar] [CrossRef]
- Qin, S.; Boggs, S. Design considerations for high voltage DC components. IEEE Electr. Insul. Mag. 2012, 28, 36–44. [Google Scholar] [CrossRef]
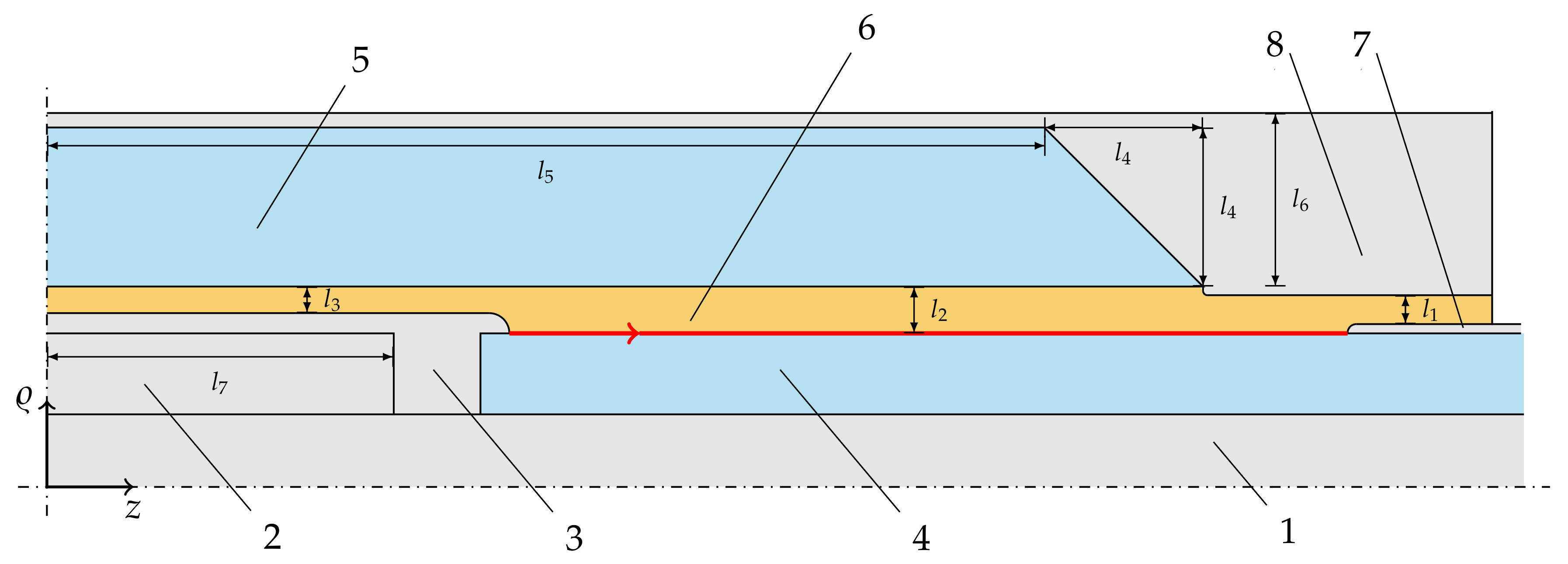

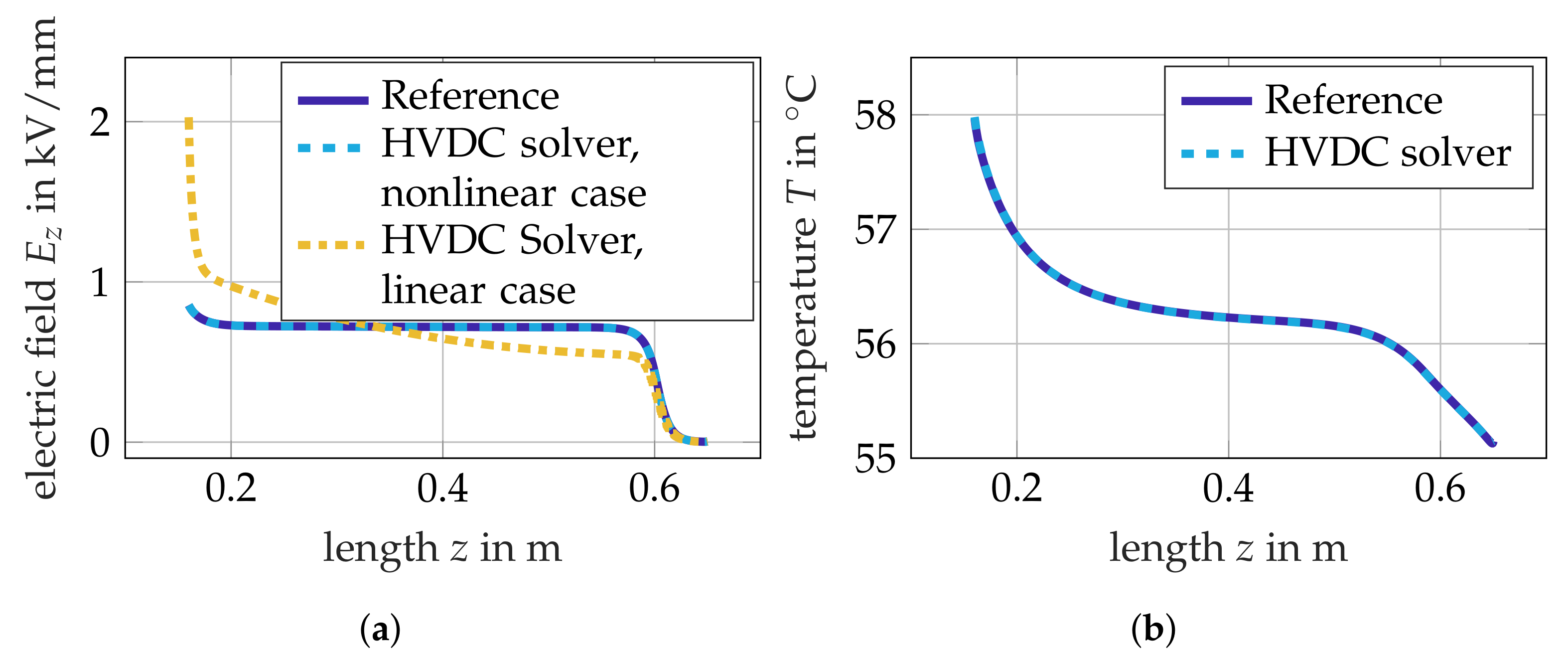

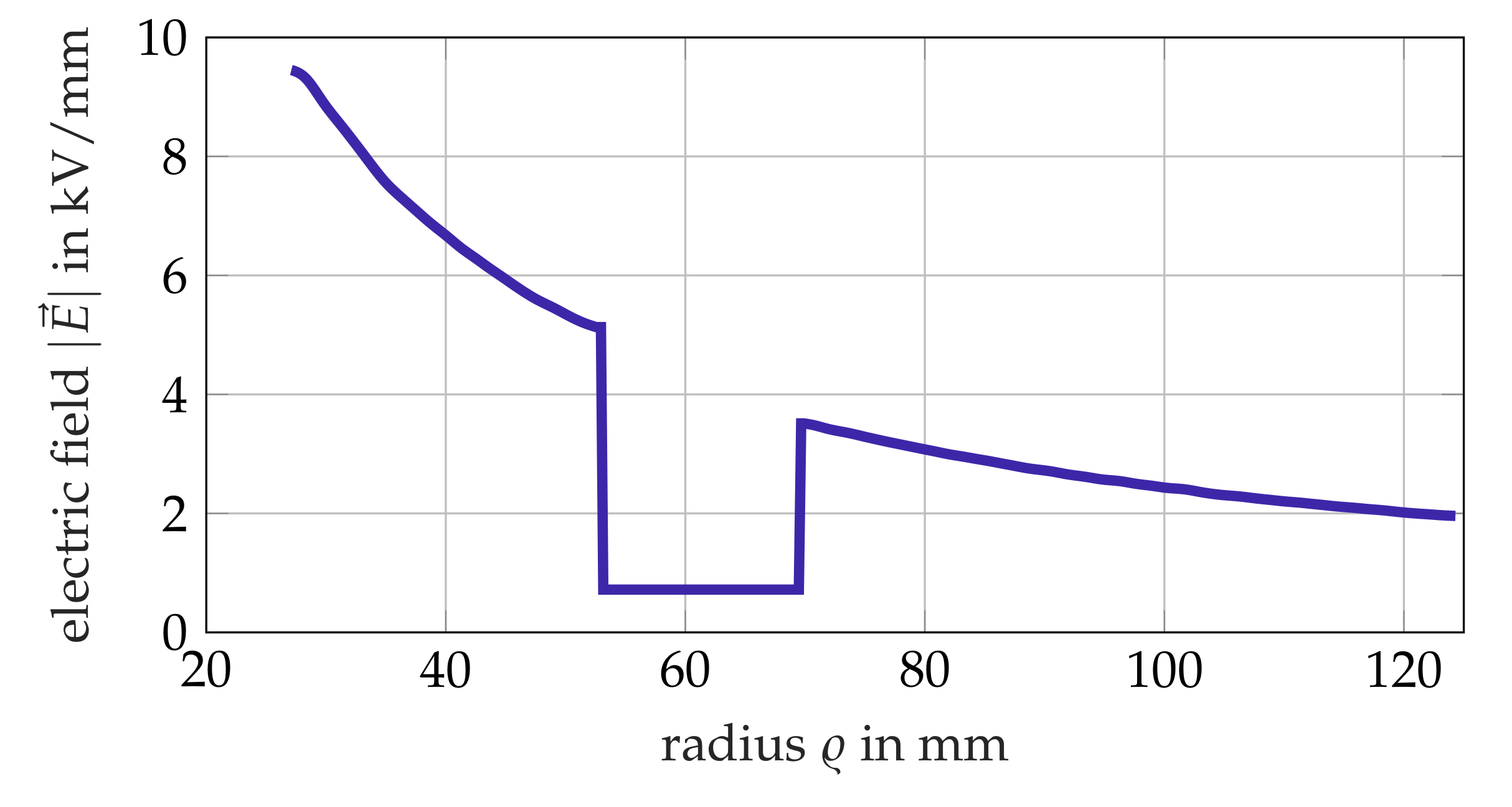

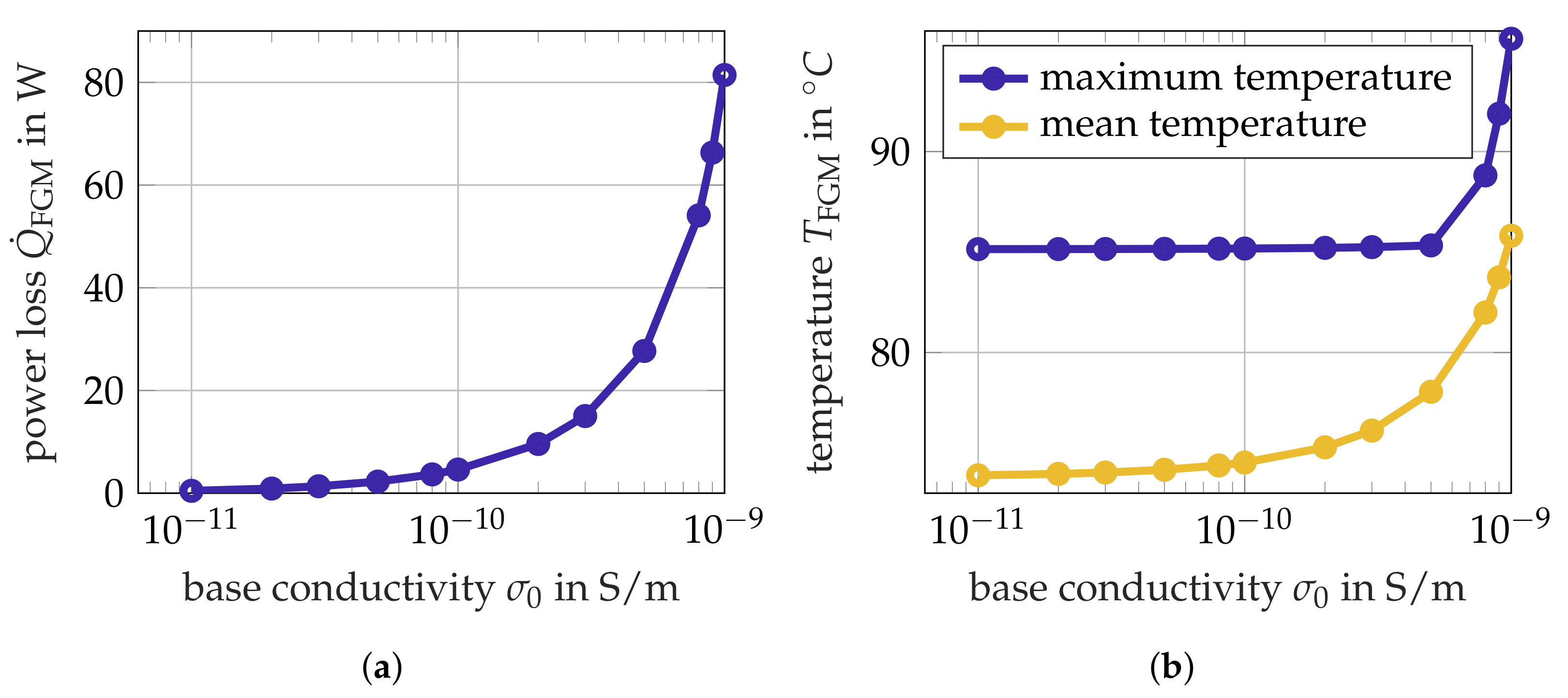
| Material Name | Size in mm | in S/m | in W/(m·K) | |
|---|---|---|---|---|
| copper (1) | length | 2000 | perfect conductor (PEC) | 400 |
| radius | 25.1 | |||
| aluminium (2) | 120 | PEC | 238 | |
| thickness | 28 | |||
| conductive silicone rubber (3) | radius of rounding | 7 | 1 | 0.25 |
| XLPE (4) | length | 1850 | 0.3 | |
| thickness | 26 | |||
| insulating silicone rubber (5) | 55 | 0.22 | ||
| 545 | ||||
| field grading material (6) | 6.9 | see Equation (1) | 0.5 | |
| 16.2 | ||||
| 9.2 | ||||
| outer cable sheath (7) | length | 1350 | 1 | 0.25 |
| thickness | 1.2 | |||
| radius of rounding | 1 | |||
| outer joint sheath (8) | 60 | 1 | 0.25 | |
| radius of rounding | 1.5 | |||
| scenario | 1 | 2 | 3 | 4 |
|---|---|---|---|---|
| conductor temperature in °C | 70 | 70 | 70 | 90 |
| ambient temperature in °C | 0 | 20 | 40 | 20 |
| median of evaluation path in kV/mm | 0.719 | 0.719 | 0.719 | 0.72 |
| maximum of evaluation path in kV/mm | 0.851 | 0.854 | 0.857 | 0.848 |
| power loss within FGM in W | 2.1 | 2.5 | 3 | 4.6 |
| maximum temperature within FGM in °C | 61 | 63 | 64 | 85 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Späck-Leigsnering, Y.; Ruppert, G.; Gjonaj, E.; De Gersem, H.; Koch, M. Towards Electrothermal Optimization of a HVDC Cable Joint Based on Field Simulation. Energies 2021, 14, 2848. https://doi.org/10.3390/en14102848
Späck-Leigsnering Y, Ruppert G, Gjonaj E, De Gersem H, Koch M. Towards Electrothermal Optimization of a HVDC Cable Joint Based on Field Simulation. Energies. 2021; 14(10):2848. https://doi.org/10.3390/en14102848
Chicago/Turabian StyleSpäck-Leigsnering, Yvonne, Greta Ruppert, Erion Gjonaj, Herbert De Gersem, and Myriam Koch. 2021. "Towards Electrothermal Optimization of a HVDC Cable Joint Based on Field Simulation" Energies 14, no. 10: 2848. https://doi.org/10.3390/en14102848
APA StyleSpäck-Leigsnering, Y., Ruppert, G., Gjonaj, E., De Gersem, H., & Koch, M. (2021). Towards Electrothermal Optimization of a HVDC Cable Joint Based on Field Simulation. Energies, 14(10), 2848. https://doi.org/10.3390/en14102848







