Transient Behavior of Vertical Commingled Well in Vertical Non-Uniform Boundary Radii Reservoir
Abstract
1. Introduction
2. Methodology
2.1. Physical Model
- A vertical production well is located at the center of a multilayer reservoir formation with constant initial reservoir pressure (pi) in any layer (j). The formation has a circular-closed boundary with non-uniform boundary radii in the vertical direction, but any layer j has an individual constant thickness (hj) and boundary radius (rej).
- The formation is considered to be isotropic and homogeneous. Any layer has individual physical parameters, including the permeability (kj), porosity (φj) and total compressibility (ctj).
- The formation is fully penetrated by the vertical production well with height (ht) and radius (rw). During the production stage, both the pressure (pj) and rate (qj) of any layer are different. The bottom-hole pressure (pw) and wellbore rate (q) are variable with the production time.
- The porosity of the formation is filled with a single-phase fluid with constant viscosity (μ). Radial flow regime is assumed in any layer (see Figure 1b,c). The isothermal volume factor (B) is descript the volume change of the natural gas from the wellbore to the wellhead.
- The gravity and temperature effects are ignored.
2.2. Mathematical Model
3. Results and Discussion
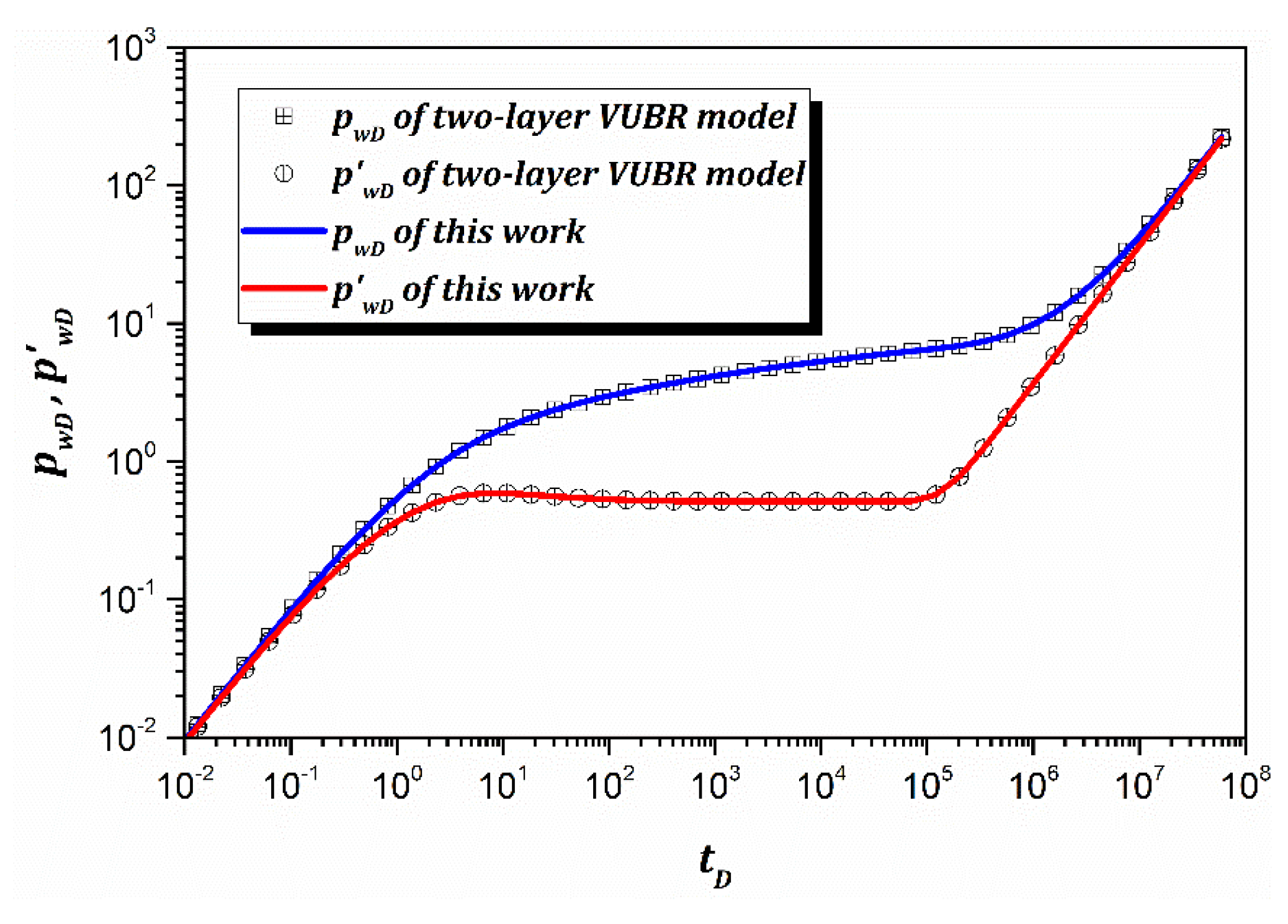
3.1. Solution Validation
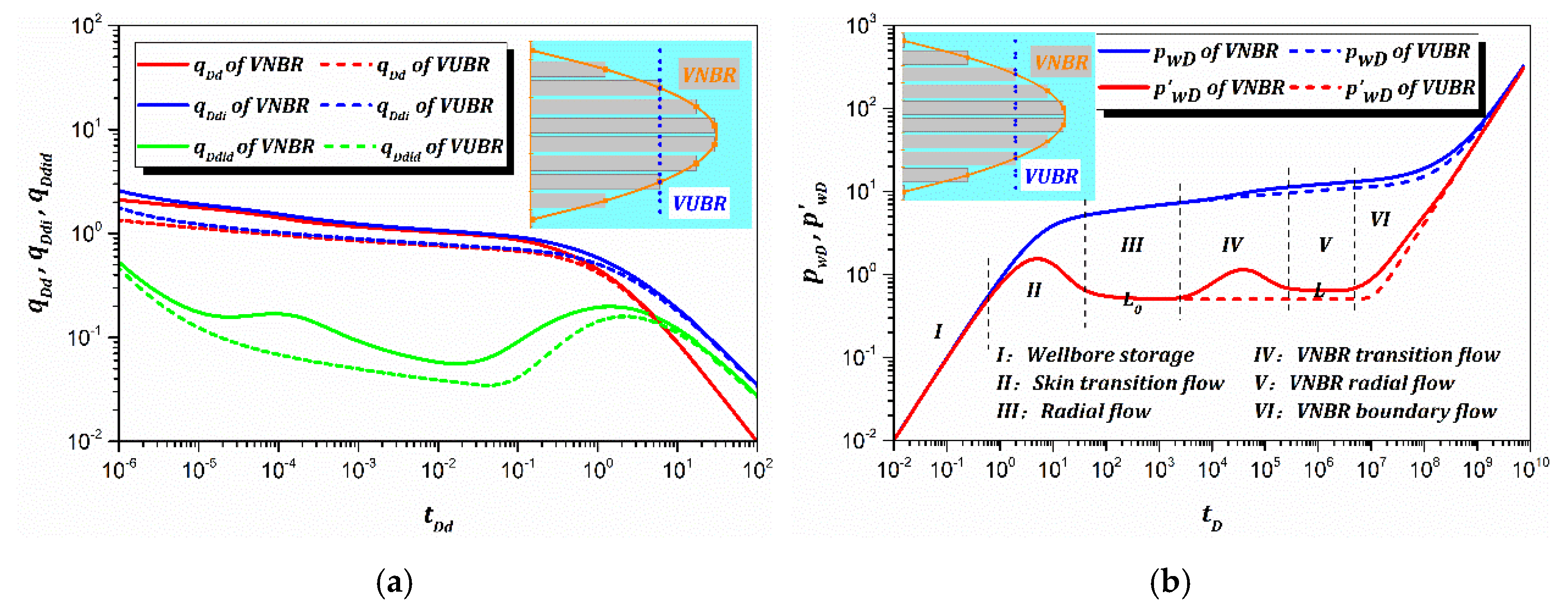
3.2. Combined Type Curve
3.3. Sensitivity Analysis
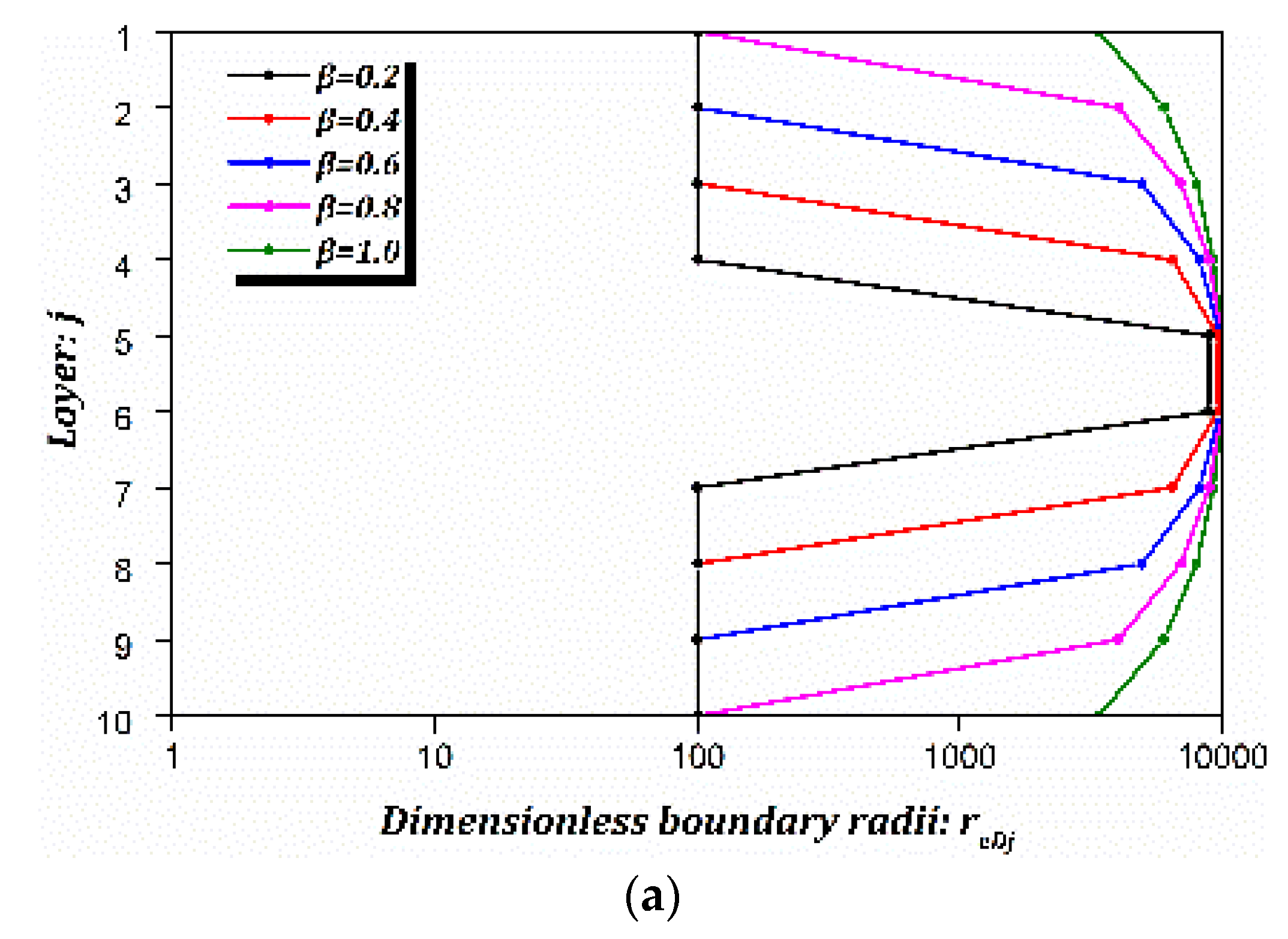
3.3.1. Outer-Convex Thickness
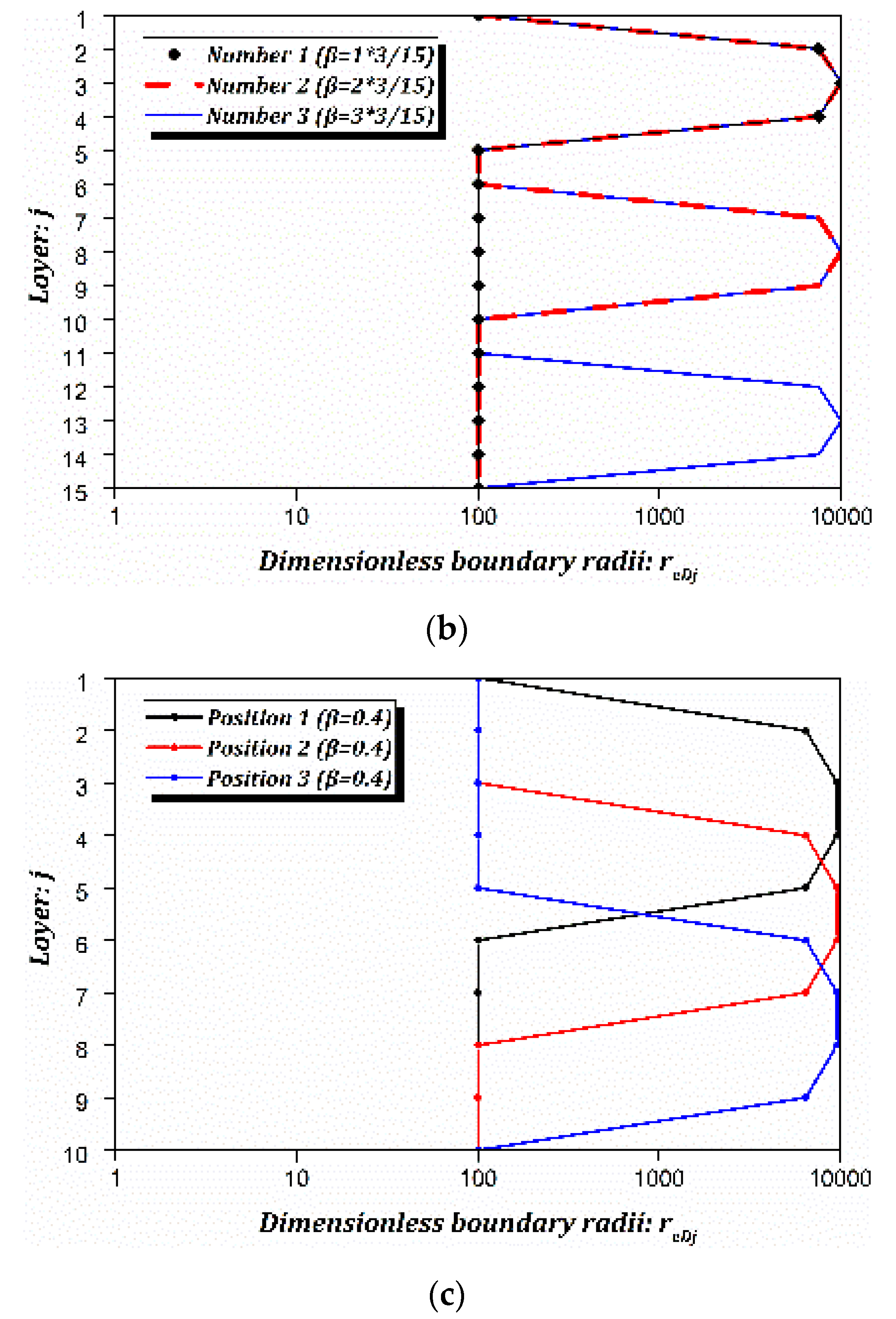
3.3.2. Outer-Convex Number
3.3.3. Outer-Convex Position
4. Conclusions
- (1)
- The production decline behavior of the VNBR reservoir is different from that of the VUBR reservoir, and the normalized dimensionless decline rate integral derivative can magnify the differences in the RTA combined type curves.
- (2)
- A new radial flow regime is identified in the VNBR reservoir that is captured by the PTA combined type curves. This behavior can be easily misinterpreted by a radial composite model as it has two stabilizations of the pressure derivative. Thus, the well testing analyst should be aware of the “equivalent composite zone effect”.
- (3)
- The outer-convex thickness and outer-convex number of the VNBR can be quickly obtained from the second level of pressure derivative in the PTA combined type curve. The equivalent VUBR can be obtained from the boundary radius corresponding to the boundary flow regime based on the “equivalent seepage volume effect”.
- (4)
- The actual exact outer-convex position of the VNBR, especially the VNBR with multiple outer-convexes, can neither be identified by RTA nor PTA combined type curves when the outer-convex total thickness and equivalent distance of the VNBR are unchanged.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| PTA | Pressure transient analysis, abbreviation |
| RTA | Rate transient analysis, abbreviation |
| VNBR | Vertical non-uniform boundary radii, abbreviation |
| VUBR | Vertical uniform boundary radii, abbreviation |
| Δ | The thickness of the inner zone, m |
| Δp | The pressure difference between initial formation and any position, Pa |
| Δpw | The pressure difference between initial formation and wellbore, Pa |
| Aj, Bj | Unknown coefficients of pressure solution equation of layer j, real number |
| B | Isothermal volume factor, m3/m3 |
| C | Wellbore storage factor, m3/Pa |
| CD | Dimensionless wellbore storage factor, real number |
| ctj | Total compressibility of layer j, Pa−1 |
| hj | The thickness of layer j, m |
| ht | The thickness of formation, m |
| Ia, Ka | a-order Bessel function, real number function |
| kj | Permeability of layer j, m2 |
| L | The value of pressure derivative in VNBR radial flow stage, real number |
| L0 | The value of pressure derivative in radial flow, real number |
| Me | Equivalent mobility ratio, real number |
| n | The number of layers, positive integer |
| pi | Initial formation pressure, Pa |
| pj | The pressure of layer j, Pa |
| pjD | Dimensionless pressure of layer j, real number |
| pw | Bottom-hole pressure, Pa |
| p′w | Bottom-hole pressure derivative, Pa |
| pwD | Dimensionless bottom-hole pressure, real number |
| p′wD | Dimensionless bottom-hole pressure derivative, real number |
| q | Rate of production well, m3/s |
| qD | Dimensionless wellbore flow rate, real number |
| qDd | Normalized dimensionless rate, real number |
| qDdi | Normalized dimensionless rate integral, real number |
| qDdid | Normalized dimensionless rate integral derivative, real number |
| qj | Rate of layer j, m3/s |
| qjD | Dimensionless rate of layer j, real number |
| r | Radial distance, m |
| rD | Dimensionless radial distance, real number |
| re_VUBR | Equivalent vertical uniform boundary radii, m |
| reD | Dimensionless boundary radii, real number |
| reD_VUBR | Dimensionless equivalent vertical uniform boundary radii, real number |
| rej | Boundary radius of layer j, m |
| rejD | Dimensionless boundary radius of layer j, real number |
| rw | The radius of the wellbore, m |
| S | Skin factor, real number |
| t | Real production time, s |
| tD | Dimensionless time, real number |
| tDd | Normalized dimensionless time, real number |
| u | Laplace variable, real number |
| χj | Mobility thickness product ratio of layer, fraction |
| β | Outer flow-layer ratio, fraction |
| δ | The thickness of the outer-convex zone, m |
| μ | The viscosity of fluid, Pa· |
| σj | Kernel function of layer j in the Laplace domain, real number function |
| φj | The porosity of layer j, fraction |
| ωj | Storability ratio of layer j, fraction |
Appendix A
| Defined Parameters | Expressions | |
|---|---|---|
| Constant Production Pressure (RTA Model) | Constant Production Rata (PTA Model) | |
| Dimensionless pressure | pD = 2πkaht(pi − p)/(qμB) | pD = (pi − p)/(pi − pw) |
| Dimensionless wellbore flow rate | qD = 1 | qD = qμB/[2πkaht(pi − pw)] |
| Dimensionless bottom-hole pressure | pWd = 2πkaht(pi − pw)/(qμB) | pwD = 1 |
| Dimensionless thickness of layer j | hjD = hj/ht | |
| Average reservoir permeability | ka = ∑(kjhjD) | |
| Average reservoir storability coefficient | (φct)a = ∑[(φct)jhjD] | |
| Dimensionless radial distance | rD = r/rw | |
| Dimensionless time | tD = kat/[μ(φct)arw2] | |
| Dimensionless wellbore storage coefficient | CD = C/[2πht(φct)arw2] | |
| Mobility thickness product ratios in layer j | χj = kjhjD/ka | |
| Storability ratios in layer j | ωj = hjD(φct)j/(φct)a | |
Appendix B
References
- Sarisittitham, S.; Jamiolahmady, M. Decline Curve Analysis for Tight Gas and Gas Condensate Reservoirs. In Proceedings of the International Petroleum Technology Conference, Kuala Lumpur, Malaysia, 10–12 December 2014; IPTC-18188-MS. International Petroleum Technology Conference: Richardson, TX, USA, 2014. [Google Scholar] [CrossRef]
- Zuo, L.; Yu, W.; Wu, K. A fractional decline curve analysis model for shale gas reservoirs. Int. J. Coal Geol. 2016, 163, 140–148. [Google Scholar] [CrossRef]
- Wang, M.; Fan, Z.; Xing, G.; Zhao, W.; Song, H.; Su, P. Rate Decline Analysis for Modeling Volume Fractured Well Production in Naturally Fractured Reservoirs. Energies 2018, 11, 43. [Google Scholar] [CrossRef]
- He, Y.; Cheng, S.; Qin, J.; Wang, Y.; Feng, N.; Hu, L.; Huang, Y.; Fang, R.; Yu, H. A semi-analytical approach to estimate the locations of malfunctioning horizontal wellbore through bottom-hole pressure and its application in Hudson oilfield. In Proceedings of the SPE Middle East Oil & Gas Show and Conference, Manama, UK, 6–9 March 2017. [Google Scholar] [CrossRef]
- Wang, Y.; Ayala, L.F. Explicit Determination of Reserves for Variable-Bottomhole-Pressure Conditions in Gas Rate-Transient Analysis. SPE J. 2019, 25, 369–390. [Google Scholar] [CrossRef]
- Zhang, X.; Wang, X.; Hou, X.; Xu, W. Rate Decline Analysis of Vertically Fractured Wells in Shale Gas Reservoirs. Energies 2017, 10, 1602. [Google Scholar] [CrossRef]
- Satman, A. An Analytical Study of Transient Flow in Stratified Systems with Fluid Banks. Society of Petroleum Engineers. In Proceedings of the SPE Annual Technical Conference and Exhibition, San Antonio, TX, USA, 4–7 October 1981. [Google Scholar] [CrossRef]
- Satman, A.; Oskay, M. Effect of A Tilted Front on Well Test Analysis; Society of Petroleum Engineers: Richardson, TX, USA, 1985. [Google Scholar]
- Tariq, S.; Ramey, H. Drawdown Behavior of a Well with Storage and Skin Effect Communicating with Layers of Different Radii and Other Characteristics. In Proceedings of the SPE Annual Fall Technical Conference and Exhibition, Houston, TX, USA, 1–3 October 1978. [Google Scholar] [CrossRef]
- Anbarci, K.; Grader, A.; Ertekin, T. Determination of Front Locations in a Multilayer Composite Reservoir. In Proceedings of the SPE Annual Technical Conference and Exhibition, San Antonio, TX, USA, 8–11 October 1989. [Google Scholar] [CrossRef]
- Ehlig Economides, C.; Joseph, J. A New Test for Determination of Individual Layer Properties in a Multilayered Reservoir. SPE Form. Eval. 1987, 2, 261–283. [Google Scholar] [CrossRef]
- Fetkovich, M.J.; Bradley, M.D.; Works, A.M.; Thrasher, T.S. Depletion Performance of Layered Reservoirs Without Crossflow. SPE Form. Eval. 1990, 5, 310–318. [Google Scholar] [CrossRef]
- El Banbi, A.H.; Wattenbarger, R.A. Analysis of Commingled Tight Gas Reservoirs. In Proceedings of the SPE Annual Technical Conference and Exhibition, Denver, CO, USA, 6–9 October 1996. [Google Scholar] [CrossRef]
- Villanueva Triana, B.; Civan, F. Rigorous Simulation of Production from Commingled Multilayer. Reservoirs under Various Crossflow and Boundary Conditions. In Proceedings of the SPE Production and Operations Symposium, Oklahoma City, OK, USA, 23–26 March 2013. [Google Scholar] [CrossRef]
- Milad, B. Modeling and Simulation of Production from Commingled Multilayer Zones and Hydraulically Fractured Horizontal Wells in Unconventional Gas Reservoirs. Master’s Thesis, University of Oklahoma, Norman, OK, USA, 2013. [Google Scholar]
- Aly, A.; Chen, H.; Lee, W. Pre-Production Pressure Analysis of Commingled Reservoir with Unequal Initial Pressures. In Proceedings of the Permian Basin Oil and Gas Recovery Conference, Midland, TX, USA, 16–18 March 1994. [Google Scholar] [CrossRef]
- Jordan, C.; Mattar, L. Comparison of Pressure Transient Behavior of Composite and Multi-Layer Reservoirs. In Proceedings of the SPE Canadian International Petroleum Conference, Calgary, AB, Canada, 4–8 June 2000. [Google Scholar] [CrossRef]
- Lolon, E.; Archer, R.A.; Ilk, D.; Blasingame, T.A. New Semi-analytical Solutions for Multilayer Reservoirs. In Proceedings of the CIPC/SPE Gas Technology Symposium 2008 Joint Conference, Calgary, AB, Canada, 16–19 June 2008. [Google Scholar] [CrossRef]
- Larsen, L. Boundary Effects in Pressure-Transient Data from Layered Reservoirs. In Proceedings of the SPE Latin America and Caribbean Mature Fields Symposium, Salvador, Bahia, Brazil, 15–16 March 1989. [Google Scholar] [CrossRef]
- Boussila, A.K.; Tiab, D.; Owayed, J. Pressure Behavior of Well Near a Leaky Boundary in Heterogeneous Reservoirs. In Proceedings of the SPE Production and Operations Symposium, Oklahoma City, OK, USA, 23–26 March 2003. [Google Scholar] [CrossRef]
- Shi, W.; Cheng, S.; Meng, L.; Gao, M.; Zhang, J.; Shi, Z.; Wang, F.; Duan, L. Pressure transient behavior of layered commingled reservoir with vertical inhomogeneous closed boundary. J. Petrol. Sci. Eng. 2020, 189, 106995. [Google Scholar] [CrossRef]
- Gomes, E.; Ambastha, A.K. An Analytical Pressure-Transient Model for Multilayered, Composite Reservoirs with Pseudosteady-State Formation Crossflow; Society of Petroleum Engineers: Richardson, TX, USA, 1993. [Google Scholar] [CrossRef]
- Stehfest, H. Numerical inversion of Laplace transforms. Commun. ACM 1970, 13, 624. [Google Scholar] [CrossRef]
- Blasingame, T.A.; McCray, T.L.; Lee, W.J. Decline curve analysis for variable pressure drop/variable flowrate systems. In Proceedings of the SPE Gas Technology Symposium, Houston, TX, USA, 22–24 January 1991; SPE-21513-MS. Society of Petroleum Engineers: Richardson, TX, USA, 1991. [Google Scholar] [CrossRef]
- Van Everdingen, A.F.; Hurst, W. The Application of the Laplace Transformation to Flow Problems in Reservoirs. J. Pet. Technol. 1949, 1, 305–324. [Google Scholar] [CrossRef]
- Lefkovits, H.C.; Hazebroek, P.; Allen, E.E.; Matthews, C.S. A Study of the Behavior of Bounded Reservoirs Composed of Stratified Layers. Soc. Petrol. Eng. J. 1961, 1, 43–58. [Google Scholar] [CrossRef]


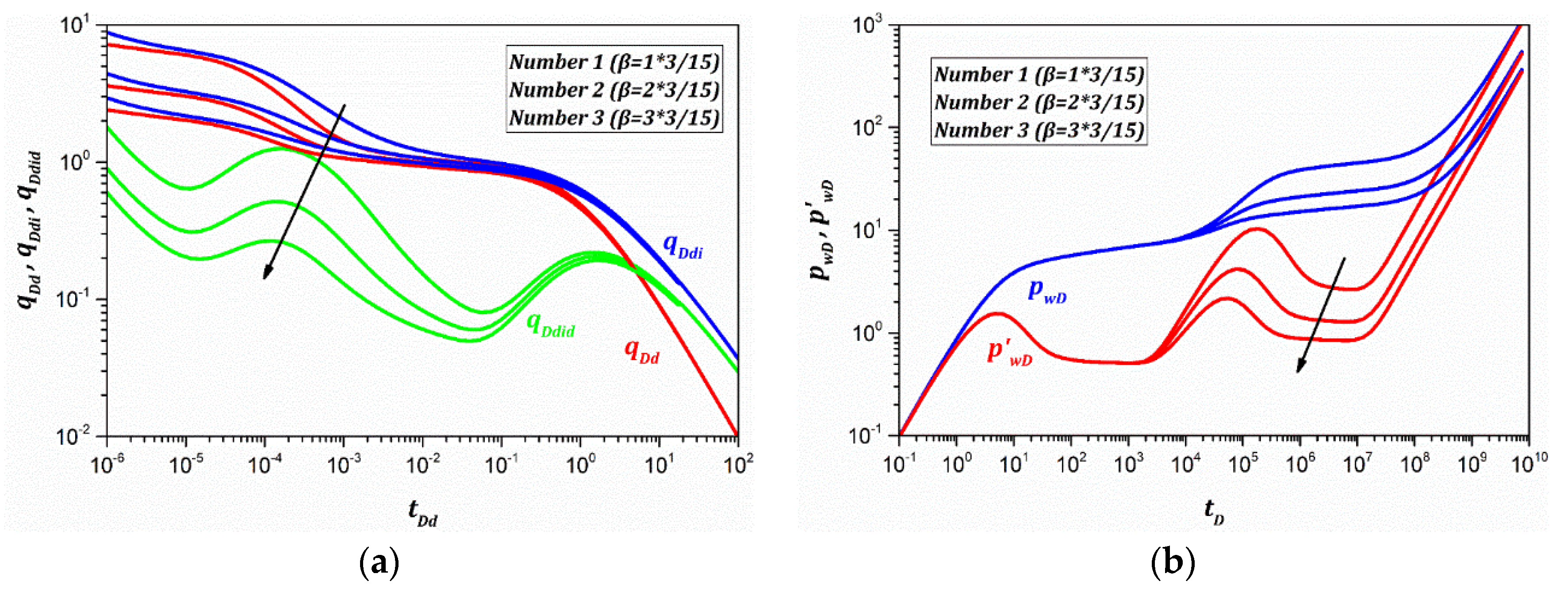
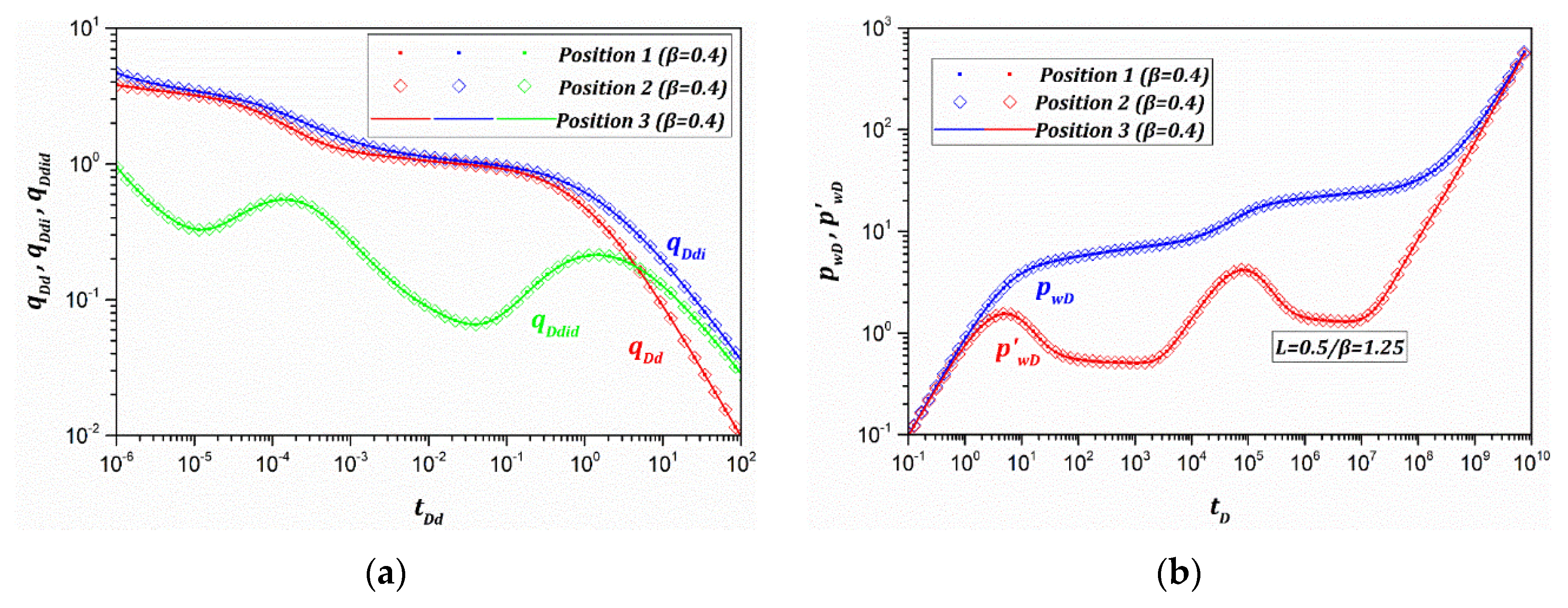
| Parameters | Value | |
|---|---|---|
| VNBR | VUBR | |
| Dimensionless wellbore storage (CD) | 1.0 | 1.0 |
| skin factor (S) | 0.3 | 0.3 |
| Mobility thickness product ratio (χ1) | 1/n | 0.5 |
| Storability ratio (ω1) | 1/n | 0.5 |
| Parameters | Value | |
|---|---|---|
| VNBR | VUBR | |
| Number of layers (n) | 10 | 10 |
| Dimensionless thickness of any layer (hjD) | 1/n | 1/n |
| Dimensionless boundary radii (reD) | (100,10,000) | 7030.0 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sun, B.; Shi, W.; Zhang, R.; Cheng, S.; Zhang, C.; Di, S.; Cui, N. Transient Behavior of Vertical Commingled Well in Vertical Non-Uniform Boundary Radii Reservoir. Energies 2020, 13, 2305. https://doi.org/10.3390/en13092305
Sun B, Shi W, Zhang R, Cheng S, Zhang C, Di S, Cui N. Transient Behavior of Vertical Commingled Well in Vertical Non-Uniform Boundary Radii Reservoir. Energies. 2020; 13(9):2305. https://doi.org/10.3390/en13092305
Chicago/Turabian StyleSun, Bing, Wenyang Shi, Rui Zhang, Shiqing Cheng, Chengwei Zhang, Shiying Di, and Nan Cui. 2020. "Transient Behavior of Vertical Commingled Well in Vertical Non-Uniform Boundary Radii Reservoir" Energies 13, no. 9: 2305. https://doi.org/10.3390/en13092305
APA StyleSun, B., Shi, W., Zhang, R., Cheng, S., Zhang, C., Di, S., & Cui, N. (2020). Transient Behavior of Vertical Commingled Well in Vertical Non-Uniform Boundary Radii Reservoir. Energies, 13(9), 2305. https://doi.org/10.3390/en13092305






