A Parametric Study of Wave Energy Converter Layouts in Real Wave Models
Abstract
:1. Introduction
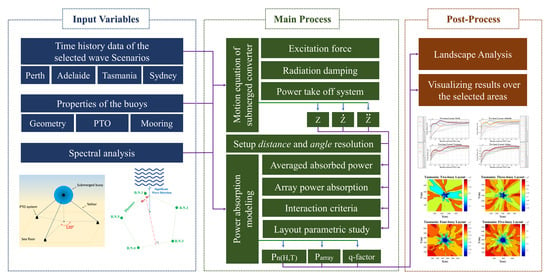
2. Numerical Modelling
2.1. Wave Energy Converter
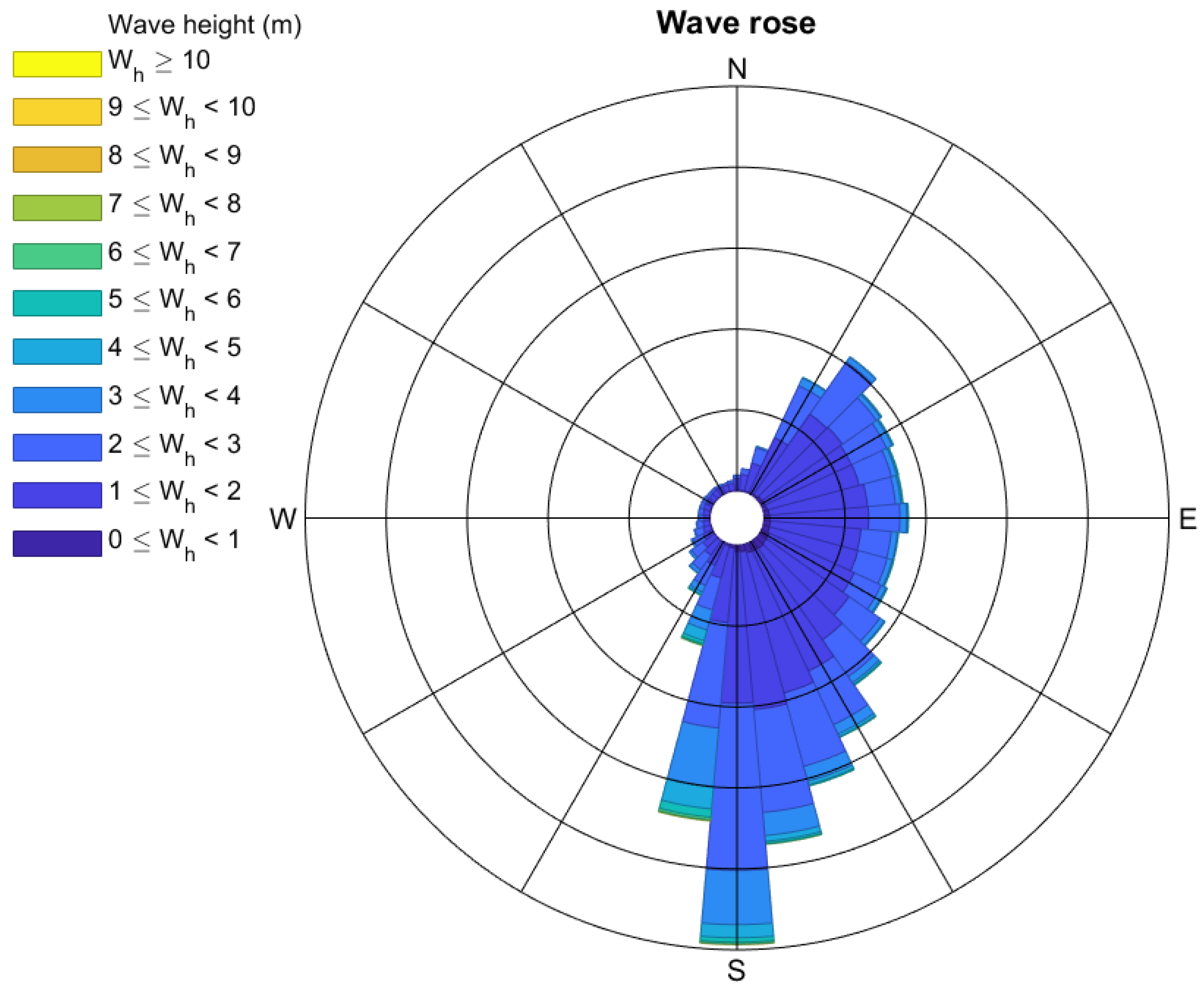
2.2. Wave Resource
2.3. Array Interaction Criteria
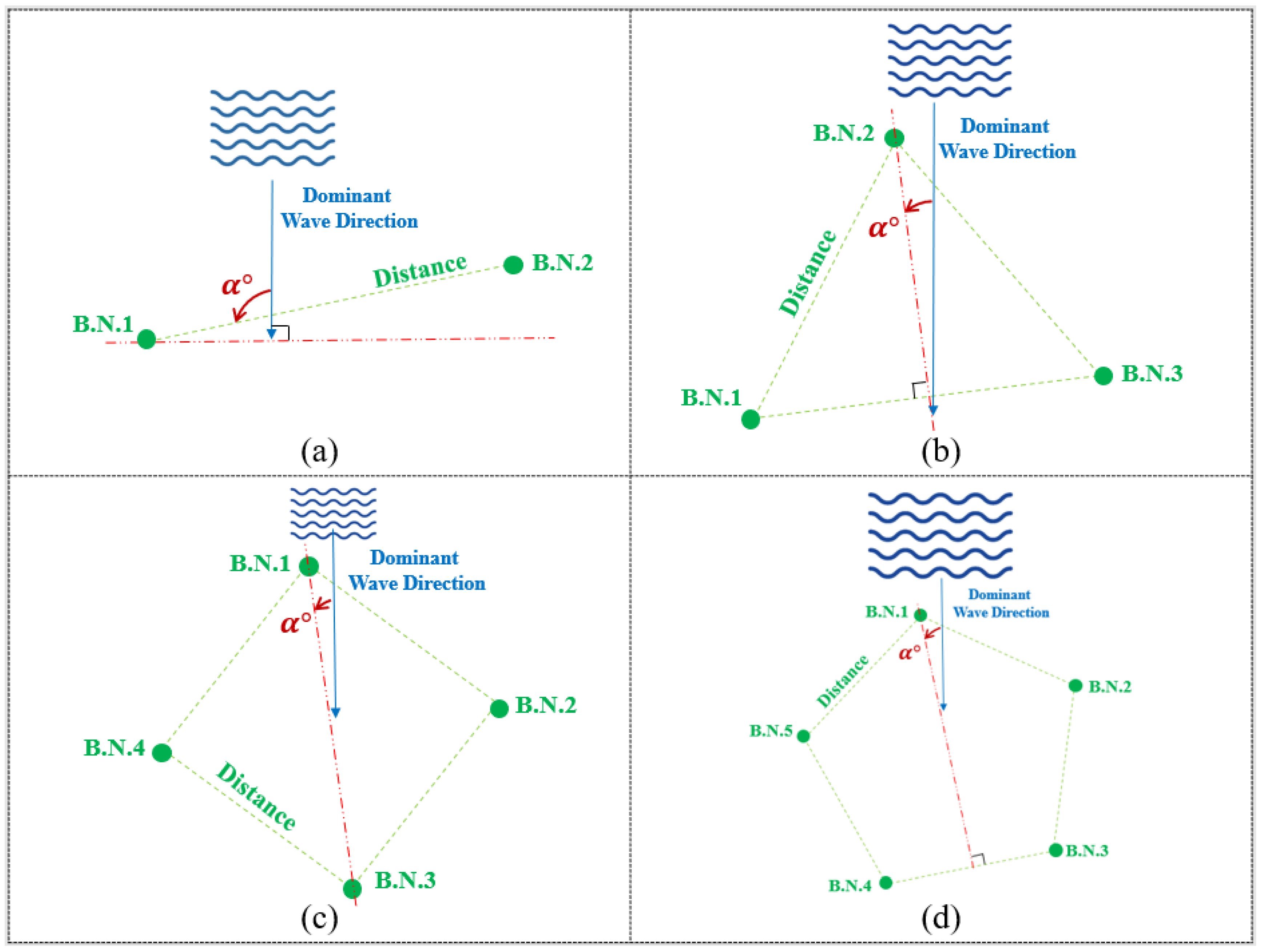
3. Layout Assessment Routine
4. Results and Discussions
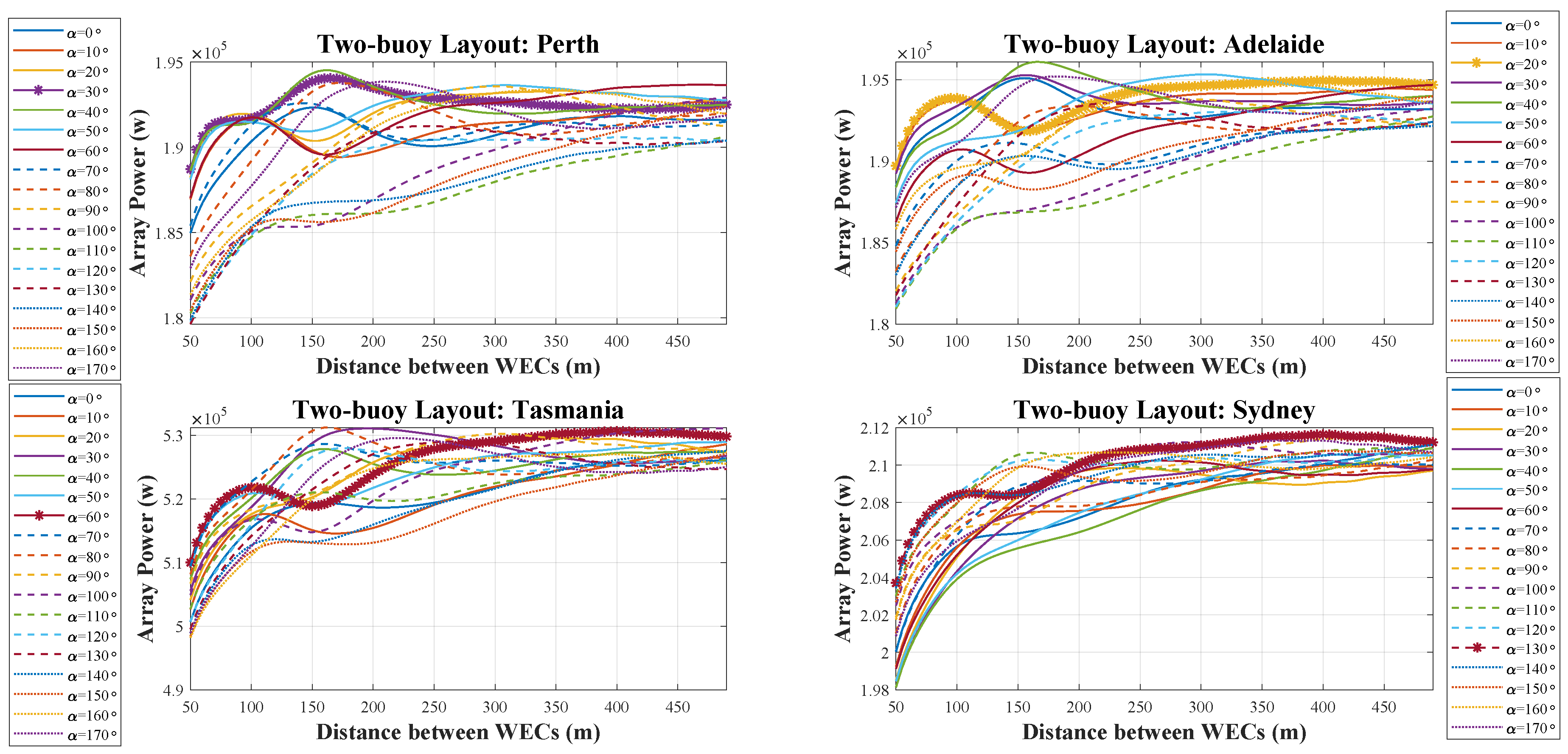
4.1. Sensitivity of Two-Buoy Array Performance to Distance
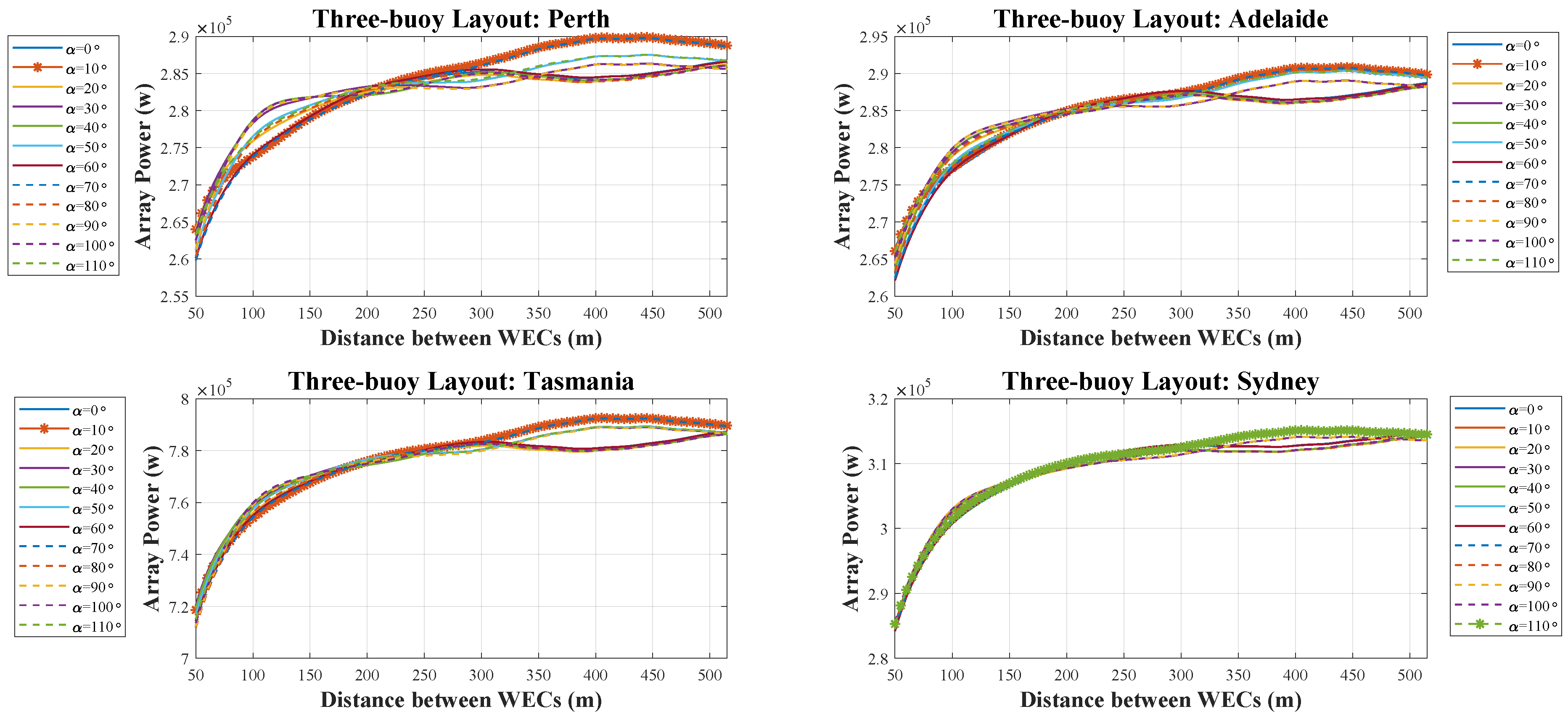
4.2. Sensitivity of Three-Buoy Array Performance to Distance
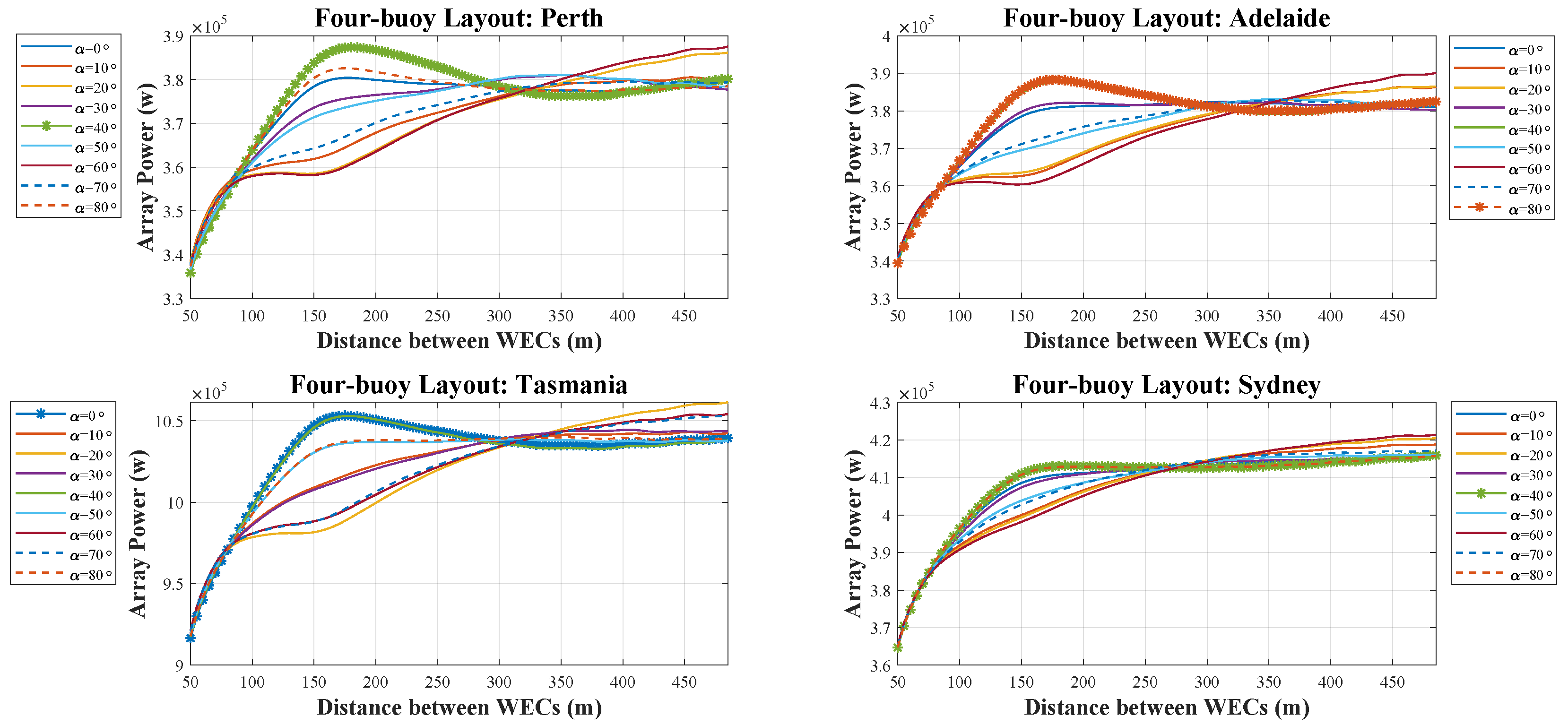
4.3. Sensitivity of Four-Buoy Array Performance to Distance
4.4. Sensitivity of Five-Buoy Array Performance to Distance
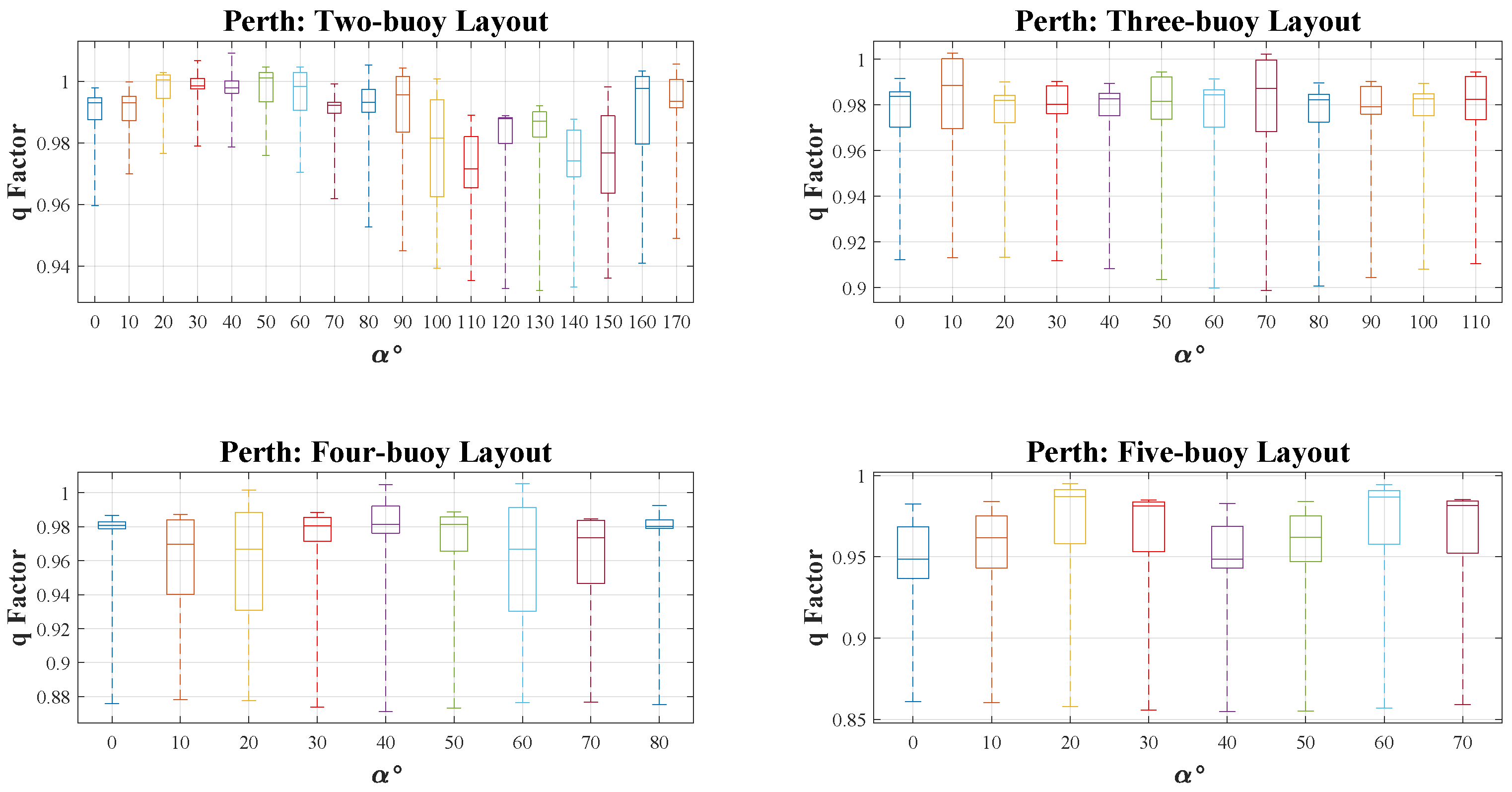
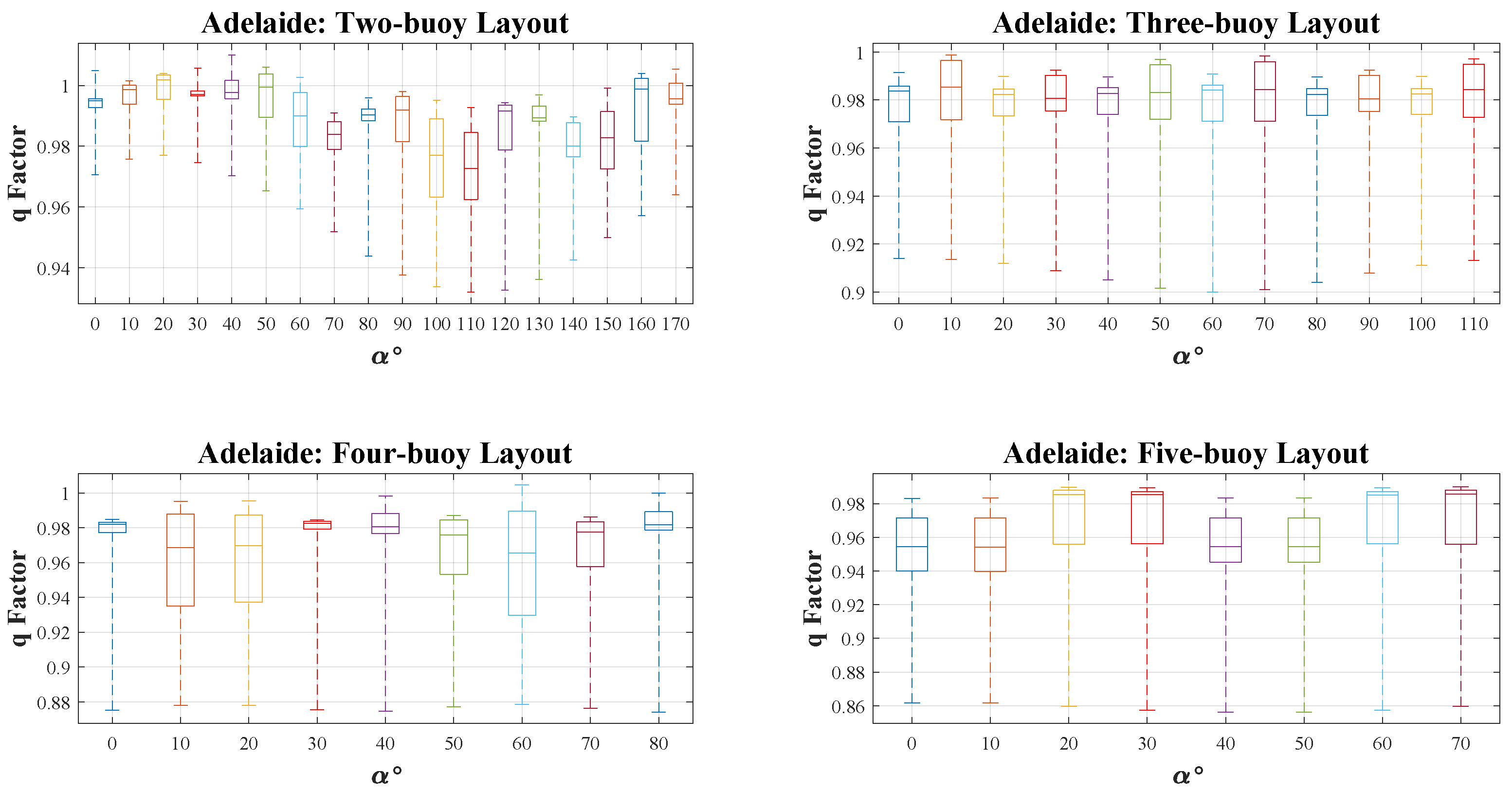
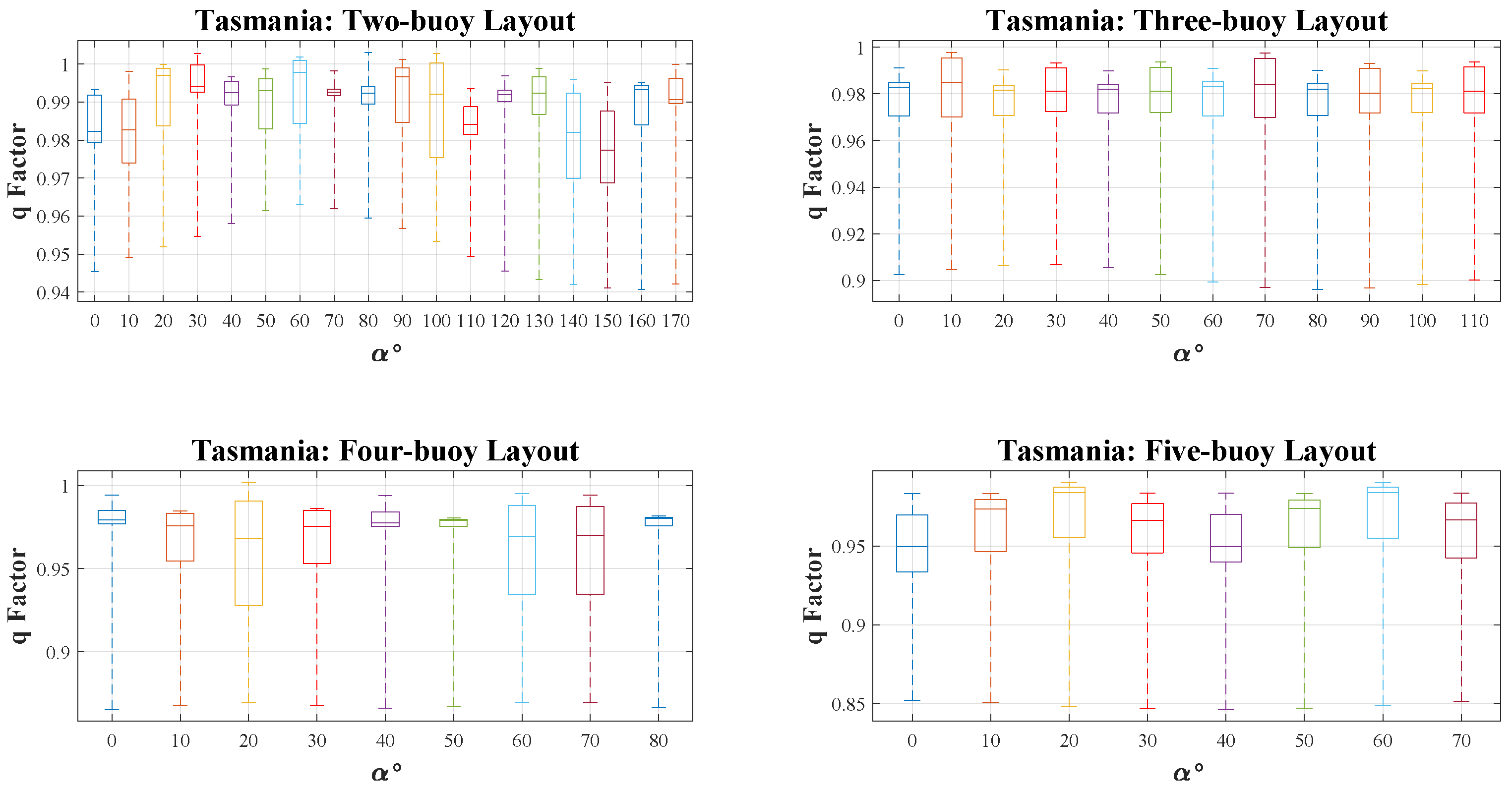
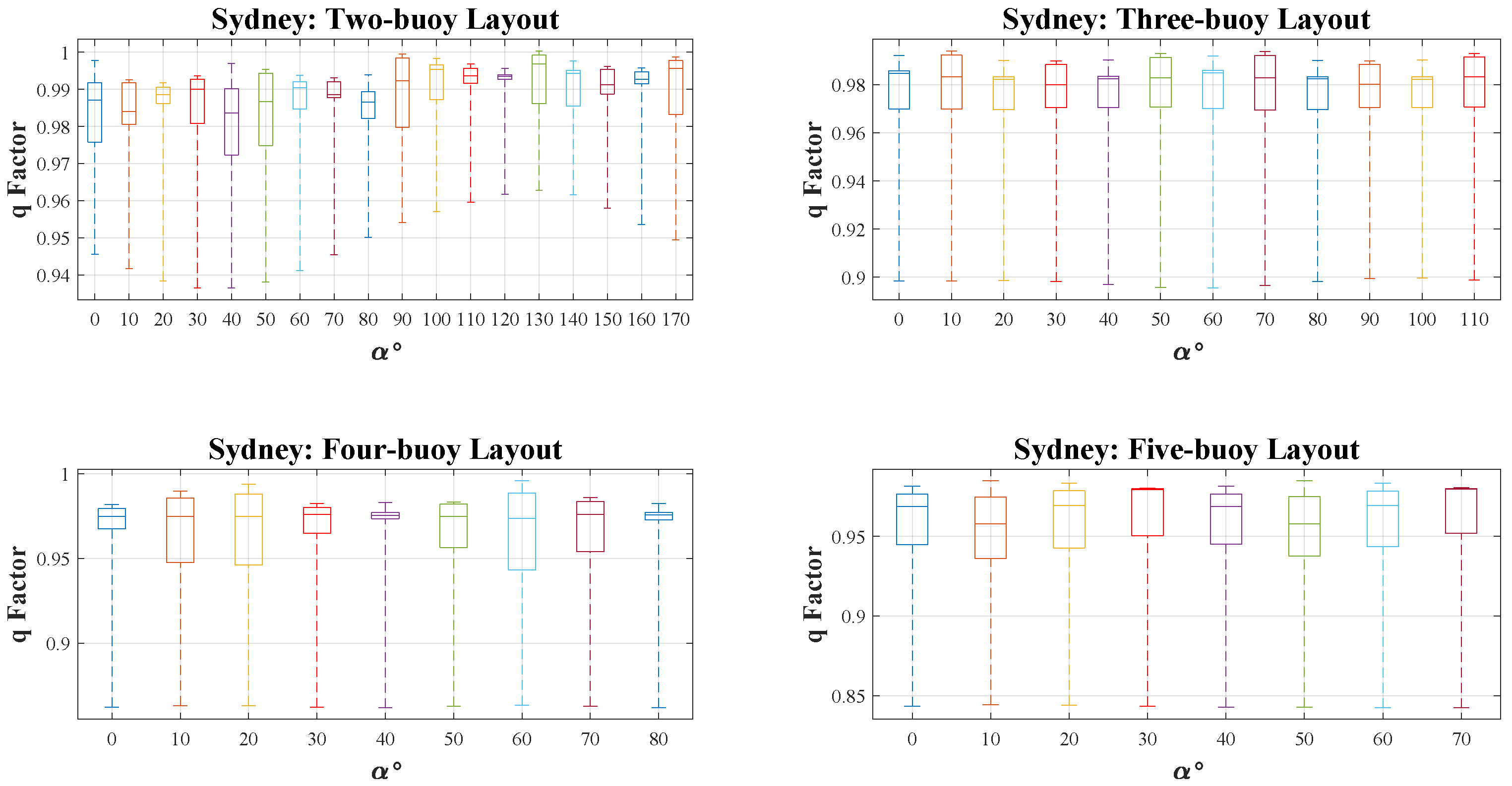
4.5. Sensitivity Analysis of q-Factor to the Relative Angle of Rotation
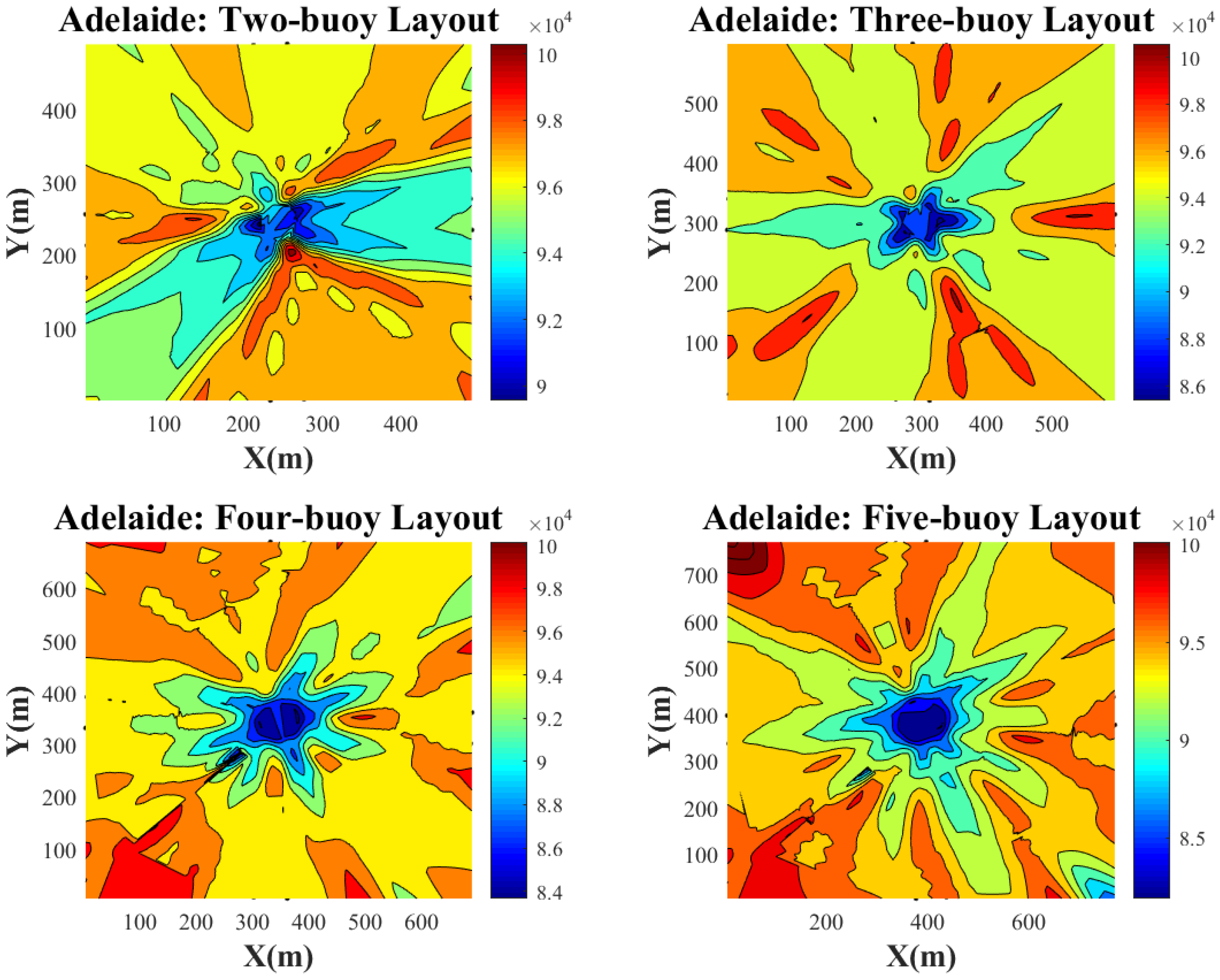
4.6. Landscape Analysis
4.7. Interaction Based Layout Selection
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| WEC | Wave Energy Converter |
| PTO | Power Take-off |
| PSO | Particle Swarm Optimisation |
| GA | Genetic Algorithm |
| EA | Evolutionary Algorithms |
| DE | Differential Evolution |
| GWO | Gray Wolf Optimiser |
| ML | Machine Learning |
References
- Barstow, S.; Mørk, G.; Mollison, D.; Cruz, J. The wave energy resource. In Ocean Wave Energy; Springer: Berlin/Heidelberg, Germany, 2008; pp. 93–132. [Google Scholar]
- (US) EIA; Government Printing Office. International Energy Outlook 2016, with Projections to 2040; Government Printing Office: Washington, DC, USA, 2016.
- Clément, A.; McCullen, P.; Falcão, A.; Fiorentino, A.; Gardner, F.; Hammarlund, K.; Lemonis, G.; Lewis, T.; Nielsen, K.; Petroncini, S.; et al. Wave energy in Europe: Current status and perspectives. Renew. Sustain. Energy Rev. 2002, 6, 405–431. [Google Scholar] [CrossRef]
- Antonio, F.d.O. Wave energy utilization: A review of the technologies. Renew. Sustain. Energy Rev. 2010, 14, 899–918. [Google Scholar]
- Linton, C. Radiation and diffraction of water waves by a submerged sphere in finite depth. Ocean Eng. 1991, 18, 61–74. [Google Scholar] [CrossRef]
- Babarit, A. On the park effect in arrays of oscillating wave energy converters. Renew. Energy 2013, 58, 68–78. [Google Scholar] [CrossRef] [Green Version]
- Babarit, A.; Hals, J.; Muliawan, M.; Kurniawan, A.; Moan, T.; Krokstad, J. Numerical Estimation of Energy Delivery from a Selection of Wave Energy Converters—Final Report; Report; Ecole Centrale de Nantes & Norges Teknisk-Naturvitenskapelige Universitet: Nantes, France, 2011. [Google Scholar]
- Göteman, M.; Engström, J.; Eriksson, M.; Isberg, J.; Leijon, M. Methods of reducing power fluctuations in wave energy parks. J. Renew. Sustain. Energy 2014, 6, 043103. [Google Scholar] [CrossRef] [Green Version]
- Göteman, M.; Engström, J.; Eriksson, M.; Isberg, J. Optimizing wave energy parks with over 1000 interacting point-absorbers using an approximate analytical method. Int. J. Mar. Energy 2015, 10, 113–126. [Google Scholar] [CrossRef] [Green Version]
- Giassi, M.; Castellucci, V.; Göteman, M. Economical layout optimization of wave energy parks clustered in electrical subsystems. Appl. Ocean Res. 2020, 101, 102274. [Google Scholar] [CrossRef]
- Neshat, M.; Sergiienko, N.Y.; Amini, E.; Majidi Nezhad, M.; Astiaso Garcia, D.; Alexander, B.; Wagner, M. A New Bi-Level Optimisation Framework for Optimising a Multi-Mode Wave Energy Converter Design: A Case Study for the Marettimo Island, Mediterranean Sea. Energies 2020, 13, 5498. [Google Scholar] [CrossRef]
- Budal, K. Theory for absorption of wave power by a system of interacting bodies. J. Ship Res. 1977, 21, 248–254. [Google Scholar]
- Evans, D. A theory for wave-power absorption by oscillating bodies. J. Fluid Mech. 1976, 77, 1–25. [Google Scholar] [CrossRef]
- Budal, K. Interacting Point Absorbers with Controlled Motion; Pascal Academic Press: London, UK, 1980. [Google Scholar]
- Thomas, G.; Evans, D. Arrays of three-dimensional wave-energy absorbers. J. Fluid Mech. 1981, 108, 67–88. [Google Scholar] [CrossRef]
- Simon, M. Multiple scattering in arrays of axisymmetric wave-energy devices. Part 1. A matrix method using a plane-wave approximation. J. Fluid Mech. 1982, 120, 1–25. [Google Scholar] [CrossRef]
- Castro, F.A.; Chiang, L.E. Design optimization and experimental validation of a two-body Wave Energy Converter with adjustable Power Take-Off parameters. Energy Sustain. Dev. 2020, 56, 19–32. [Google Scholar] [CrossRef]
- Neshat, M.; Alexander, B.; Wagner, M. A hybrid cooperative co-evolution algorithm framework for optimising power take off and placements of wave energy converters. Inf. Sci. 2020, 534, 218–244. [Google Scholar] [CrossRef]
- Bonovas, M.I.; Anagnostopoulos, I.S. Modelling of operation and optimum design of a wave power take-off system with energy storage. Renew. Energy 2020, 147, 502–514. [Google Scholar] [CrossRef]
- Calvário, M.; Gaspar, J.; Kamarlouei, M.; Hallak, T.; Soares, C.G. Oil-hydraulic power take-off concept for an oscillating wave surge converter. Renew. Energy 2020, 159, 1297–1309. [Google Scholar] [CrossRef]
- Tronchin, L.; Manfren, M.; Nastasi, B. Energy analytics for supporting built environment decarbonisation. Energy Procedia 2019, 157, 1486–1493. [Google Scholar] [CrossRef]
- Mazzoni, S.; Ooi, S.; Nastasi, B.; Romagnoli, A. Energy storage technologies as techno-economic parameters for master-planning and optimal dispatch in smart multi energy systems. Appl. Energy 2019, 254, 113682. [Google Scholar] [CrossRef]
- Nastasi, B. Hydrogen policy, market, and R&D projects. In Solar Hydrogen Production; Elsevier: Amsterdam, The Netherlands, 2019; pp. 31–44. [Google Scholar]
- Bozzi, S.; Giassi, M.; Miquel, A.M.; Antonini, A.; Bizzozero, F.; Gruosso, G.; Archetti, R.; Passoni, G. Wave energy farm design in real wave climates: The Italian offshore. Energy 2017, 122, 378–389. [Google Scholar] [CrossRef] [Green Version]
- De Andrés, A.; Guanche, R.; Meneses, L.; Vidal, C.; Losada, I. Factors that influence array layout on wave energy farms. Ocean Eng. 2014, 82, 32–41. [Google Scholar] [CrossRef]
- Engström, J.; Eriksson, M.; Göteman, M.; Isberg, J.; Leijon, M. Performance of large arrays of point absorbing direct-driven wave energy converters. J. Appl. Phys. 2013, 114, 204502. [Google Scholar] [CrossRef] [Green Version]
- Vicente, P.C.; Falcão, A.d.O.; Justino, P.A. A time domain analysis of arrays of floating point-absorber wave energy converters including the effect of nonlinear mooring forces. In Proceedings of the ICOE’2010—3rd International Conference on Ocean Energy, Bilbao, Spain, 6–8 October 2010. [Google Scholar]
- Yang, S.; Ringsberg, J.; Johnson, E. Analysis of interaction effects between WECs in four types of wave farms. In Proceedings of the 3rd International Conference on Renewable Energies Offshore (RENEW 2018), Lisbon, Portugal, 8–10 October 2018; pp. 647–658. [Google Scholar]
- Sergiienko, N.Y.; Neshat, M.; da Silva, L.S.; Alexander, B.; Wagner, M. Design optimisation of a multi-mode wave energy converter. arXiv 2020, arXiv:2001.08966. [Google Scholar]
- Neshat, M.; Alexander, B.; Sergiienko, N.Y.; Wagner, M. Optimisation of Large Wave Farms Using a Multi-Strategy Evolutionary Framework. In Proceedings of the 2020 Genetic and Evolutionary Computation Conference, GECCO’20, Cancún, Mexico, 14 June 2020; Association for Computing Machinery: New York, NY, USA, 2020; pp. 1150–1158. [Google Scholar] [CrossRef]
- Jusoh, M.; Ibrahim, M.; Daud, M.; Yusop, Z.; Albani, A.; Rahman, S.; Mohad, S. Parameters estimation of hydraulic power take-off system for wave energy conversion system using genetic algorithm. In Proceedings of the International Conference on Sustainable Energy and Green Technology, Bangkok, Thailand, 11–14 December 2019; Volume 463, p. 012129. [Google Scholar]
- Rodríguez, C.A.; Rosa-Santos, P.; Taveira-Pinto, F. Hydrodynamic optimization of the geometry of a sloped-motion wave energy converter. Ocean Eng. 2020, 199, 107046. [Google Scholar] [CrossRef]
- M’zoughi, F.; Bouallègue, S.; Garrido, A.J.; Garrido, I.; Ayadi, M. Water cycle algorithm–based airflow control for oscillating water column—Based wave energy converters. Proc. Inst. Mech. Eng. Part I J. Syst. Control Eng. 2020, 234, 118–133. [Google Scholar] [CrossRef]
- Chen, M. Hydrodynamic Analysis and Optimization of a Hinged-Type Wave Energy Converter-SeaWEED. Ph.D. Thesis, Memorial University of Newfoundland, St. John’s, NL, Canada, 2020. [Google Scholar]
- Liu, Z.; Wang, Y.; Hua, X. Prediction and optimization of oscillating wave surge converter using machine learning techniques. Energy Convers. Manag. 2020, 210, 112677. [Google Scholar] [CrossRef]
- Izquierdo-Pérez, J.; Brentan, B.M.; Izquierdo, J.; Clausen, N.E.; Pegalajar-Jurado, A.; Ebsen, N. Layout Optimization Process to Minimize the Cost of Energy of an Offshore Floating Hybrid Wind–Wave Farm. Processes 2020, 8, 139. [Google Scholar] [CrossRef] [Green Version]
- Esmaeilzadeh, S.; Alam, M.R. Shape optimization of wave energy converters for broadband directional incident waves. Ocean Eng. 2019, 174, 186–200. [Google Scholar] [CrossRef] [Green Version]
- Lyu, J.; Abdelkhalik, O.; Gauchia, L. Optimization of dimensions and layout of an array of wave energy converters. Ocean Eng. 2019, 192, 106543. [Google Scholar] [CrossRef]
- Kelly, M.; Alam, M.R. Shape Optimization of a Submerged Pressure Differential Wave Energy Converter for Load Reductions. In Proceedings of the International Conference on Offshore Mechanics and Arctic Engineering, Glasgow, UK, 9–14 June 2019; American Society of Mechanical Engineers: New York, NY, USA, 2019; Volume 58899, p. V010T09A030. [Google Scholar]
- Neshat, M.; Alexander, B.; Sergiienko, N.; Wagner, M. New insights into position optimisation of wave energy converters using hybrid local search. Swarm Evol. Comput. 2020, 59, 100744. [Google Scholar] [CrossRef]
- Neshat, M.; Abbasnejad, E.; Shi, Q.; Alexander, B.; Wagner, M. Adaptive neuro-surrogate-based optimisation method for wave energy converters placement optimisation. In Proceedings of the International Conference on Neural Information Processing, Sydney, Australia, 12–15 December 2019; Springer: Berlin/Heidelberg, Germany, 2019; pp. 353–366. [Google Scholar]
- Jabrali, A.; Khatyr, R.; Naciri, J.K. Viscous effects and energy recovery optimization for freely floating and bottom fixed wave energy converters. Int. J. Renew. Energy Res. 2019, 9, 290–300. [Google Scholar]
- Wang, L.; Ringwood, J.V. Geometric optimization of a hinge-barge wave energy converter. In Proceedings of the 13th European Wave and Tidal Energy Conference, Naples, Italy, 1–6 September 2019; p. 1389. [Google Scholar]
- Faraggiana, E.; Masters, I.; Chapman, J. Design of an optimization scheme for the WaveSub array. In Advances in Renewable Energies Offshore, Proceedings of the 3rd International Conference on Renewable Energies Offshore (RENEW), Lisbon, Portugal, 8–10 October 2018; Soares, G., Ed.; Taylor & Francis Group: London, UK, 10 October 2019; pp. 633–638. [Google Scholar]
- Neshat, M.; Alexander, B.; Sergiienko, N.Y.; Wagner, M. A Hybrid Evolutionary Algorithm Framework for Optimising Power Take off and Placements of Wave Energy Converters. In Proceedings of the Genetic and Evolutionary Computation Conference, GECCO’19, Prague, Czech Republic, 14 July 2019; Association for Computing Machinery: New York, NY, USA, 2019; pp. 1293–1301. [Google Scholar] [CrossRef] [Green Version]
- Vatchavayi, S.R. Heuristic Optimization of Wave Energy Converter Arrays. Ph.D. Thesis, University of Minnesota, Minneapolis, MN, USA, 2019. [Google Scholar]
- Amini, E. Locating and Evaluating the Oscillating Surge Wave Energy Converter Using Grey Wolf Optimizer Algorithm and WEC-Sim Toolbox. Ph.D. Thesis, University of Tehran, Tehran, Iran, 2019. [Google Scholar]
- Amini, E.; Naeeni, S.T.O.; Ghaderi, P. Investigating Wave Energy Potential in Southern Coasts of the Caspian Sea and Evaluating the Application of Gray Wolf Optimizer Algorithm. arXiv 2019, arXiv:1912.13201. [Google Scholar]
- Sharp, C.; DuPont, B. Wave energy converter array optimization: A genetic algorithm approach and minimum separation distance study. Ocean Eng. 2018, 163, 148–156. [Google Scholar] [CrossRef]
- Neshat, M.; Alexander, B.; Wagner, M.; Xia, Y. A detailed comparison of meta-heuristic methods for optimising wave energy converter placements. In Proceedings of the Genetic and Evolutionary Computation Conference, Kyoto, Japan, 15–19 July 2018; ACM: New York, NY, USA, 2018; pp. 1318–1325. [Google Scholar]
- Fang, H.W.; Feng, Y.Z.; Li, G.P. Optimization of Wave Energy Converter Arrays by an Improved Differential Evolution Algorithm. Energies 2018, 11, 3522. [Google Scholar] [CrossRef] [Green Version]
- Arbonès, D.R.; Sergiienko, N.Y.; Ding, B.; Krause, O.; Igel, C.; Wagner, M. Sparse incomplete lu-decomposition for wave farm designs under realistic conditions. In Proceedings of the International Conference on Parallel Problem Solving from Nature, Coimbra, Portugal, 8–12 September 2018; Springer: Berlin/Heidelberg, Germany, 2018; pp. 512–524. [Google Scholar]
- Abdelkhalik, O.; Darani, S. Optimization of nonlinear wave energy converters. Ocean Eng. 2018, 162, 187–195. [Google Scholar] [CrossRef]
- Giassi, M.; Göteman, M. Layout design of wave energy parks by a genetic algorithm. Ocean Eng. 2018, 154, 252–261. [Google Scholar] [CrossRef]
- Göteman, M.; Engström, J.; Eriksson, M.; Isberg, J. Fast modeling of large wave energy farms using interaction distance cut-off. Energies 2015, 8, 13741–13757. [Google Scholar] [CrossRef] [Green Version]
- López-Ruiz, A.; Bergillos, R.J.; Raffo-Caballero, J.M.; Ortega-Sánchez, M. Towards an optimum design of wave energy converter arrays through an integrated approach of life cycle performance and operational capacity. Appl. Energy 2018, 209, 20–32. [Google Scholar] [CrossRef]
- Borgarino, B.; Babarit, A.; Ferrant, P. Impact of wave interactions effects on energy absorption in large arrays of wave energy converters. Ocean Eng. 2012, 41, 79–88. [Google Scholar] [CrossRef] [Green Version]
- Carnegie Clean Energy Limited (formerly Carnegie Wave Energy). Carnegie CETO 6 Technology. Available online: https://arena.gov.au/projects/carnegie-ceto-6-technology/ (accessed on 15 July 2020).
- Sergiienko, N. Wave Energy Converter (WEC) Array Simulator. 2020. Available online: https://www.mathworks.com/matlabcentral/fileexchange/71840-wave-energy-converter-wec-array-simulator (accessed on 27 July 2020).
- Sergiienko, N.Y.; Cazzolato, B.S.; Ding, B.; Arjomandi, M. An optimal arrangement of mooring lines for the three-tether submerged point-absorbing wave energy converter. Renew. Energy 2016, 93, 27–37. [Google Scholar] [CrossRef] [Green Version]
- Nolte, J.D.; Ertekin, R. Wave power calculations for a wave energy conversion device connected to a drogue. J. Renew. Sustain. Energy 2014, 6, 013117. [Google Scholar] [CrossRef] [Green Version]
- Cummins, W.E. The Impulse Response Function and Ship Motions; Report; DTIC Document: Rockville, MD, USA, 1962.
- Sergiienko, N.; Cazzolato, B.; Ding, B.; Hardy, P.; Arjomandi, M. Performance comparison of the floating and fully submerged quasi-point absorber wave energy converters. Renew. Energy 2017, 108, 425–437. [Google Scholar] [CrossRef] [Green Version]
- Flavià, F.F.; Babarit, A.; Clément, A.H. On the numerical modeling and optimization of a bottom-referenced heave-buoy array of wave energy converters. Int. J. Mar. Energy 2017, 19, 1–15. [Google Scholar] [CrossRef] [Green Version]
- Zwolan, P.; Czaplewski, K. Sea waves models used in maritime simulators. Zesz. Nauk. Morska W Szczecinie 2012, 104, 186–190. [Google Scholar]
- Chen, W.; Gao, F.; Meng, X.; Fu, J. Design of the wave energy converter array to achieve constructive effects. Ocean Eng. 2016, 124, 13–20. [Google Scholar] [CrossRef]
- De Andres, A.; Maillet, J.; Hals Todalshaug, J.; Möller, P.; Bould, D.; Jeffrey, H. Techno-Economic Related Metrics for a Wave Energy Converters Feasibility Assessment. Sustainability 2016, 8, 1109. [Google Scholar] [CrossRef] [Green Version]






| Objective | WECs Type | WECs Number | Method | Year | References |
|---|---|---|---|---|---|
| Design & PTOs | submerged | 2 | Experimental observations | 2020 | [17] |
| Layout & PTOs | fully-submerged | 4, 16 | Cooperative EAs | 2020 | [18] |
| Design & PTOs | fully-submerged | 1 | Hybrid EAs | 2020 | [29] |
| Layout | fully-submerged | 50, 100 | Multi-strategy EAs | 2020 | [30] |
| Design & PTOs | heaving WEC | 1 | Evolutionary and GA | 2020 | [19] |
| PTOs | oscillating wave surge converter | 1 | GA | 2020 | [20,31] |
| Design | sloped-motion WEC | 1 | Heuristic optimization | 2020 | [32] |
| PTOs | oscillating water column-based | 1 | Water cycle algorithm | 2020 | [33] |
| PTOs | hinged-type WECs | 1 | Experimental observations | 2020 | [34] |
| PTOs | oscillating wave surge converter | 1 | GA and ML | 2020 | [35] |
| Layout | submerged | 25 | PSO | 2020 | [36] |
| Design | submerged flat plate | 1 | GA | 2019 | [37] |
| Design & Layout | cylindrical heaving WECs | 3, 5, 7 | GA | 2019 | [38] |
| Design | submerged | 2 | GA | 2019 | [39] |
| Layout | fully-submerged | 4, 16 | Smart heuristic | 2019 | [40] |
| Layout | fully-submerged | 4, 16 | Nuro-adaptive EA | 2019 | [41] |
| PTOs | freely floating | 2 | EAs | 2019 | [42] |
| Design | hinge-barge WEC | 2 | gradient-based method | 2019 | [43] |
| Design | fully-submerged | 1, 2, 3 | GA, PSO | 2019 | [44] |
| Layout & PTOs | fully-submerged | 16 | Hybrid EAs | 2019 | [45] |
| Layout & PTOs | fully-submerged | 4, 9 | Heuristics | 2019 | [46] |
| Feasibility Study & Design | oscillating wave surge converter | 3 | Numerical and GWO | 2019 | [47] |
| Layout | heaving WEC | 1 | GWO | 2019 | [48] |
| Layout | heave-constrained cylinder | 5 | improved GA | 2018 | [49] |
| Layout | fully-submerged | 4, 16 | Local search | 2018 | [50] |
| Layout | oscillating WEC | 3, 5, 8 | improved DE | 2018 | [51] |
| Layout & LCoE | fully-submerged | 4, 9, 36 | Multi-objective EAs | 2018 | [52] |
| PTOs | submerged | 1 | Hidden GA | 2018 | [53] |
| Layout & PTOs | submerged | 4, 7, 9, 14 | hybrid GA | 2018 | [54] |
| Layout | semi-submerged | 1000 | approximate analytical method | 2015 | [9] |
| Layout | submerged | 32 | randomized geometries | 2013 | [26] |
| Layout | floating + partially submerged | 4 | sensitivity analysis | 2014 | [25] |
| Design & Layout | submerged | 4 | sensitivity analysis | 2017 | [24] |
| Design | point-absorbing WECs | 100 | analytical multiple scattering | 2015 | [55] |
| Design & Layout | floating over-topping WECs | 9 | Down-scaling techniques | 2018 | [56] |
| Design & PTOs | heaving WEC | 9, 16, 25 | sensitivity analysis | 2012 | [57] |
| Parameter | Perth | Adelaide | Sydney | Tasmania |
|---|---|---|---|---|
| Two-buoy layout maximum q-factor | 1.0091 | 1.0163 | 1.0003 | 1.003 |
| (degrees) | 40 | 0.00 | 130 | 80 |
| distance(meter) | 160 | 165 | 400 | 160 |
| Three-buoy layout maximum q-factor | 1.0026 | 0.9987 | 0.9939 | 0.9976 |
| (degrees) | 10 | 10 | 10 | 10 |
| distance(meter) | 445 | 445 | 445 | 405 |
| Four-buoy layout maximum q-factor | 1.0053 | 1.0046 | 0.9958 | 1.0019 |
| (degrees) | 60 | 60 | 60 | 20 |
| distance(meter) | 485 | 485 | 485 | 485 |
| Five-buoy layout maximum q-factor | 0.9949 | 0.9899 | 0.9849 | 0.9905 |
| (degrees) | 18 | 63 | 45 | 18 |
| distance(meter) | 250 | 275 | 450 | 250 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Amini, E.; Golbaz, D.; Amini, F.; Majidi Nezhad, M.; Neshat, M.; Astiaso Garcia, D. A Parametric Study of Wave Energy Converter Layouts in Real Wave Models. Energies 2020, 13, 6095. https://doi.org/10.3390/en13226095
Amini E, Golbaz D, Amini F, Majidi Nezhad M, Neshat M, Astiaso Garcia D. A Parametric Study of Wave Energy Converter Layouts in Real Wave Models. Energies. 2020; 13(22):6095. https://doi.org/10.3390/en13226095
Chicago/Turabian StyleAmini, Erfan, Danial Golbaz, Fereidoun Amini, Meysam Majidi Nezhad, Mehdi Neshat, and Davide Astiaso Garcia. 2020. "A Parametric Study of Wave Energy Converter Layouts in Real Wave Models" Energies 13, no. 22: 6095. https://doi.org/10.3390/en13226095
APA StyleAmini, E., Golbaz, D., Amini, F., Majidi Nezhad, M., Neshat, M., & Astiaso Garcia, D. (2020). A Parametric Study of Wave Energy Converter Layouts in Real Wave Models. Energies, 13(22), 6095. https://doi.org/10.3390/en13226095