Voltage Stability Analysis in Medium-Voltage Distribution Networks Using a Second-Order Cone Approximation
Abstract
:1. Introduction
2. Exact Formulation
2.1. Objective Function
2.2. Set of Constraints
3. SOCP Reformulation
4. Test Systems and Simulation Scenarios
4.1. 33-Nodes Test Feeder
4.2. 69-Nodes Test Feeder
4.3. Simulation Scenarios
- ✓
- Case 1 (C1): The original configuration of the AC distribution network; i.e., without penetration of distributed generation or capacitor banks.
- ✓
- Case 2 (C2): The operation of the distribution network considering the location of distributed generators reported in [24] which are operated with unity power factor.
- ✓
- Case 3 (C3): The operation of the distribution network considering the location of fixed-step capacitor banks as recommended in [25].
- ✓
- For the 33-nodes test feeder in the C2 the distributed generators are included at nodes 14, 24, and 30 with power injections of about 770.9 kW, 1096.9 kW and 1065.8 kW, respectively. On the other hand, for capacitor banks in the C3, we consider two banks of 450 kVAr located at nodes 13 and 24, and a bank of 900 kVAr positioned at node 30.
- ✓
- For the 69-nodes test feeder in the C2, the distributed generators are included at nodes 12, 61, and 64 with power injections of about 813.1 kW, 1444.7 kW and 289.6 kW, respectively. For capacitor banks in the C3, we consider two banks of 300 kVAr located at nodes 11 and 18, and a bank of 1200 kVAr positioned at node 61.
5. Computational Validation
5.1. 33-Nodes Test Feeder
5.2. Evaluation of the Simulation Cases
5.3. Effect of Renewables in the Stability Margin
- ✓
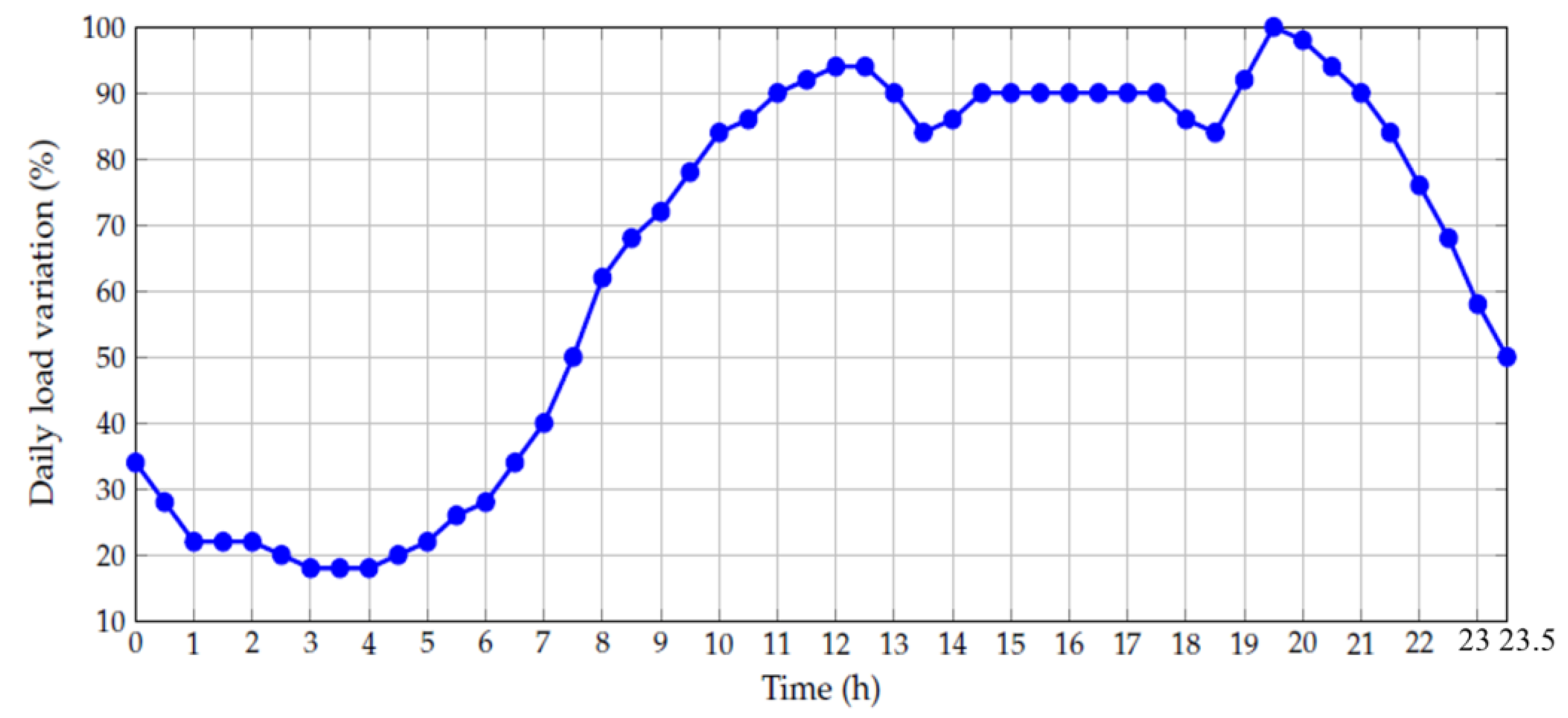
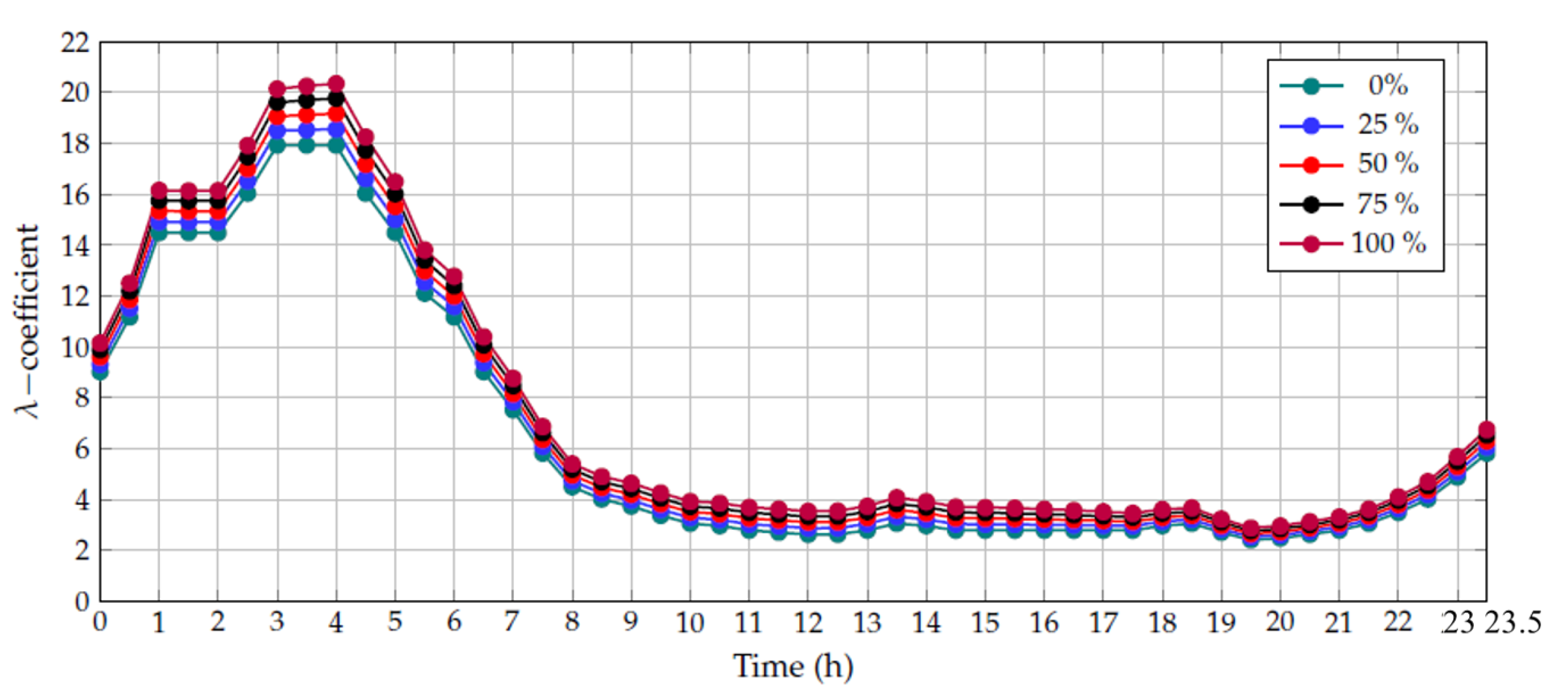
- In the time periods when the demand is low (see the period between 0 h and 8 h in Figure 5), the -coefficient is higher taking values upper than 6 with a maximum between 18 and 22 in the period between 3 and 5 h. This behavior is explained by the fact that the chargeability coefficient is a factor that multiplies the load at each operating condition. This implies that for low demands, this will increase until the point of the voltage-collapse.
- ✓
- For the time periods with higher demand (upper than 10 h in Figure 5) it is possible to see that the -coefficient oscillates between 2 and 4, and we also observe that depending on the level of distributed generation penetration, this increases respect to the base case (0% of renewable generation availability), i.e., the renewable generation has positive effects regarding voltage stability margin since for all the penetration cases the loadability coefficient is enlarged.
- ✓
- Note that the minimum -coefficient is presented at 19.5 h. At this point when the renewable energy penetration is 0%, the -coefficient is 2.407 (see Table 3 for the C1), and when the renewable energy penetration is 100%, this factor is 2.883. Note that this modest increment is because the renewable generation based on photovoltaic is zero at this time and the wind power is also in low values; nevertheless, this increment contributes to the voltage stability margin improvement.
5.4. 69-Nodes Test Feeder
5.5. Additional Results
- ✓
- All the MINLP solvers available in GAMS, different optimal solutions are reached, which oscillate between 3.3010 and 3.3184. In addition, these solvers identify different nodes and sizes of the distributed generators being node 32 the most recurrent location in all the solutions.
- ✓
- The MI-SOCP strategy allows finding the global optimal solution of this problem with a -coefficient of 3.3187. We can ensure that this is indeed the global optimum since an exhaustive search has been implemented to evaluate all the possible combinations. After 2 h of simulations, the combination of nodes 15, 18, and 32 is the best possible scheme to enlarge the voltage stability margin in the 33-nodes test feeder.
- ✓
- Regarding processing times it is worth mentioning that the GAMS solvers and the proposed MI-SOCP have faster performance to obtain optimal solutions since running time of all the simulation cases was lower than 10 s. This is considered in literature a negligible time in planning purposes, since physical installations of these distributed generators can take several weeks or months.
6. Conclusions and Future Works
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Temiz, A.; Almalki, A.M.; Kahraman, Ö.; Alshahrani, S.S.; Sönmez, E.B.; Almutairi, S.S.; Nadar, A.; Smiai, M.S.; Alabduljabbar, A.A. Investigation of MV Distribution Networks with High-Penetration Distributed PVs: Study for an Urban Area. Energy Procedia 2017, 141, 517–524. [Google Scholar] [CrossRef]
- Hernández, J.; Medina, A.; Jurado, F. Impact comparison of PV system integration into rural and urban feeders. Energy Convers. Manag. 2008, 49, 1747–1765. [Google Scholar] [CrossRef]
- Montoya, O.D.; Gil-González, W.; Giral, D.A. On the Matricial Formulation of Iterative Sweep Power Flow for Radial and Meshed Distribution Networks with Guarantee of Convergence. Appl. Sci. 2020, 10, 5802. [Google Scholar] [CrossRef]
- Jiménez, R.; Serebrisky, T.; Mercado, J. Sizing Electricity Losses in Transmission and Distribution Systems in Latin America and the Caribbean; Techreport; Inter-American Development Bank: Washington, DC, USA, 2014. [Google Scholar]
- Prakash, K.; Lallu, A.; Islam, F.; Mamun, K. Review of Power System Distribution Network Architecture. In Proceedings of the 2016 3rd Asia-Pacific World Congress on Computer Science and Engineering (APWC on CSE), Nadi, Fiji, 5–6 December 2016; IEEE: Piscataway, NJ, USA, 2016. [Google Scholar] [CrossRef]
- Zaheb, H.; Danish, M.S.S.; Senjyu, T.; Ahmadi, M.; Nazari, A.M.; Wali, M.; Khosravy, M.; Mandal, P. A Contemporary Novel Classification of Voltage Stability Indices. Appl. Sci. 2020, 10, 1639. [Google Scholar] [CrossRef] [Green Version]
- Ghaffarianfar, M.; Hajizadeh, A. Voltage Stability of Low-Voltage Distribution Grid with High Penetration of Photovoltaic Power Units. Energies 2018, 11, 1960. [Google Scholar] [CrossRef] [Green Version]
- Ranjan, R.; Das, D. Voltage Stability Analysis of Radial Distribution Networks. Electr. Power Compon. Syst. 2003, 31, 501–511. [Google Scholar] [CrossRef]
- Aly, M.M.; Abdel-Akher, M. A continuation power-flow for distribution systems voltage stability analysis. 2012 IEEE International Conference on Power and Energy (PECon), Kota Kinabalu, Malaysia, 2–5 December 2012; IEEE: Piscataway, NJ, USA, 2012. [CrossRef]
- Chen, C.; Wang, J.; Li, Z.; Sun, H.; Wang, Z. PMU uncertainty quantification in voltage stability analysis. IEEE Trans. Power Syst. 2014, 30, 2196–2197. [Google Scholar] [CrossRef]
- Sumit, B.; Chattopadhyay, T.K.; Chanda, C.K. Voltage stability margin of distribution networks for composite loads. In Proceedings of the 2012 IEEE Annual IEEE India Conference (INDICON), Kochi, India, 7–9 December 2012; IEEE: Piscataway, NJ, USA, 2012; pp. 582–587. [Google Scholar] [CrossRef]
- Song, Y.; Hill, D.J.; Liu, T. Static voltage stability analysis of distribution systems based on network-load admittance ratio. IEEE Trans. Power Syst. 2018, 34, 2270–2280. [Google Scholar] [CrossRef]
- Triştiu, I.; Iantoc, A.; Poştovei, D.; Bulac, C.; Arhip, M. Theoretical analysis of voltage instability conditions in distribution networks. In Proceedings of the 2019 IEEE 54th International Universities Power Engineering Conference (UPEC), Bucharest, Romania, 3–6 September 2019; IEEE: Piscataway, NJ, USA, 2019; pp. 1–5. [Google Scholar] [CrossRef]
- Sinder, R.L.; Assis, T.M.; Taranto, G.N. Impact of photovoltaic systems on voltage stability in islanded distribution networks. J. Eng. 2019, 18, 5023–5027. [Google Scholar] [CrossRef]
- Montoya, O.D. Numerical Approximation of the Maximum Power Consumption in DC-MGs With CPLs via an SDP Model. IEEE Trans. Circuits Syst. II 2019, 66, 642–646. [Google Scholar] [CrossRef]
- Amin, W.T.; Montoya, O.D.; Grisales-Noreña, L.F. Determination of the Voltage Stability Index in DC Networks with CPLs: A GAMS Implementation. In Communications in Computer and Information Science; Springer International Publishing: Berlin/Heidelberg, Germany, 2019; pp. 552–564. [Google Scholar] [CrossRef]
- Montoya, O.D.; Gil-Gonzalez, W.; Garrido, V.M. Voltage Stability Margin in DC Grids With CPLs: A Recursive Newton Raphson Approximation. IEEE Trans. Circuits Syst. II 2020, 67, 300–304. [Google Scholar] [CrossRef]
- Chen, Y.; Xiang, J.; Li, Y. SOCP Relaxations of Optimal Power Flow Problem Considering Current Margins in Radial Networks. Energies 2018, 11, 3164. [Google Scholar] [CrossRef] [Green Version]
- Candelo, J.E.; Delgado, G.C. Voltage stability assessment using fast non-dominated sorting algorithm. DYNA 2019, 86, 60–68. [Google Scholar] [CrossRef]
- Adebayo, I.; Sun, Y. New Performance Indices for Voltage Stability Analysis in a Power System. Energies 2017, 10, 2042. [Google Scholar] [CrossRef] [Green Version]
- Lobo, M.S.; Vandenberghe, L.; Boyd, S.; Lebret, H. Applications of second-order cone programming. Linear Algebra Appl. 1998, 284, 193–228. [Google Scholar] [CrossRef] [Green Version]
- Yamashita, M.; Mullin, T.J.; Safarina, S. An efficient second-order cone programming approach for optimal selection in tree breeding. Optim. Lett. 2018, 12, 1683–1697. [Google Scholar] [CrossRef] [Green Version]
- Lavaei, J.; Low, S.H. Zero Duality Gap in Optimal Power Flow Problem. IEEE Trans. Power Syst. 2012, 27, 92–107. [Google Scholar] [CrossRef] [Green Version]
- Montoya, O.D.; Gil-González, W.; Grisales-Noreña, L. An exact MINLP model for optimal location and sizing of DGs in distribution networks: A general algebraic modeling system approach. Ain Shams Eng. J. 2020, 11, 409–418. [Google Scholar] [CrossRef]
- Tamilselvan, V.; Jayabarathi, T.; Raghunathan, T.; Yang, X.S. Optimal capacitor placement in radial distribution systems using flower pollination algorithm. Alex. Eng. J. 2018, 57, 2775–2786. [Google Scholar] [CrossRef]
- Morais, H.; Sousa, T.; Perez, A.; Jóhannsson, H.; Vale, Z. Energy Optimization for Distributed Energy Resources Scheduling with Enhancements in Voltage Stability Margin. Math. Probl. Eng. 2016, 2016, 1–20. [Google Scholar] [CrossRef]
- Onlam, A.; Yodphet, D.; Chatthaworn, R.; Surawanitkun, C.; Siritaratiwat, A.; Khunkitti, P. Power Loss Minimization and Voltage Stability Improvement in Electrical Distribution System via Network Reconfiguration and Distributed Generation Placement Using Novel Adaptive Shuffled Frogs Leaping Algorithm. Energies 2019, 12, 553. [Google Scholar] [CrossRef] [Green Version]
- Montoya, O.D.; Serra, F.M.; Angelo, C.H.D. On the Efficiency in Electrical Networks with AC and DC Operation Technologies: A Comparative Study at the Distribution Stage. Electronics 2020, 9, 1352. [Google Scholar] [CrossRef]
- Montoya, O.D.; Gil-González, W. Dynamic active and reactive power compensation in distribution networks with batteries: A day-ahead economic dispatch approach. Comput. Electr. Eng. 2020, 85, 106710. [Google Scholar] [CrossRef]
- Montoya, O.D.; Gil-González, W.; Grisales-Noreña, L.; Orozco-Henao, C.; Serra, F. Economic Dispatch of BESS and Renewable Generators in DC Microgrids Using Voltage-Dependent Load Models. Energies 2019, 12, 4494. [Google Scholar] [CrossRef] [Green Version]
- Diamond, S.; Boyd, S. CVXPY: A Python-embedded modeling language for convex optimization. J. Mach. Learn. Res. 2016, 17, 2909–2913. [Google Scholar] [CrossRef]
- Quan, R.; Jian, J.B.; Mu, Y.D. Tighter relaxation method for unit commitment based on second-order cone programming and valid inequalities. Int. J. Electr. Power Energy Syst. 2014, 55, 82–90. [Google Scholar] [CrossRef]
- Benson, H.Y.; Sağlam, Ü. Mixed-Integer Second-Order Cone Programming: A Survey. INFORMS 2014, 1, 13–36. [Google Scholar] [CrossRef] [Green Version]




| Node i | Node j | [Ω] | [Ω] | [kW] | [kW] |
|---|---|---|---|---|---|
| 1 | 2 | 0.0922 | 0.0477 | 100 | 60 |
| 2 | 3 | 0.4930 | 0.2511 | 90 | 40 |
| 3 | 4 | 0.3660 | 0.1864 | 120 | 80 |
| 4 | 5 | 0.3811 | 0.1941 | 60 | 30 |
| 5 | 6 | 0.8190 | 0.7070 | 60 | 20 |
| 6 | 7 | 0.1872 | 0.6188 | 200 | 100 |
| 7 | 8 | 1.7114 | 1.2351 | 200 | 100 |
| 8 | 9 | 1.0300 | 0.7400 | 60 | 20 |
| 9 | 10 | 1.0400 | 0.7400 | 60 | 20 |
| 10 | 11 | 0.1966 | 0.0650 | 45 | 30 |
| 11 | 12 | 0.3744 | 0.1238 | 60 | 35 |
| 12 | 13 | 1.4680 | 1.1550 | 60 | 35 |
| 13 | 14 | 0.5416 | 0.7129 | 120 | 80 |
| 14 | 15 | 0.5910 | 0.5260 | 60 | 10 |
| 15 | 16 | 0.7463 | 0.5450 | 60 | 20 |
| 16 | 17 | 1.2890 | 1.7210 | 60 | 20 |
| 17 | 18 | 0.7320 | 0.5740 | 90 | 40 |
| 2 | 19 | 0.1640 | 0.1565 | 90 | 40 |
| 19 | 20 | 1.5042 | 1.3554 | 90 | 40 |
| 20 | 21 | 0.4095 | 0.4784 | 90 | 40 |
| 21 | 22 | 0.7089 | 0.9373 | 90 | 40 |
| 3 | 23 | 0.4512 | 0.3083 | 90 | 50 |
| 23 | 24 | 0.8980 | 0.7091 | 420 | 200 |
| 24 | 25 | 0.8960 | 0.7011 | 420 | 200 |
| 6 | 26 | 0.2030 | 0.1034 | 60 | 25 |
| 26 | 27 | 0.2842 | 0.1447 | 60 | 25 |
| 27 | 28 | 1.0590 | 0.9337 | 60 | 20 |
| 28 | 29 | 0.8042 | 0.7006 | 120 | 70 |
| 29 | 30 | 0.5075 | 0.2585 | 200 | 600 |
| 30 | 31 | 0.9744 | 0.9630 | 150 | 70 |
| 31 | 32 | 0.3105 | 0.3619 | 210 | 100 |
| 32 | 33 | 0.3410 | 0.5302 | 60 | 40 |
| Node i | Node j | [Ω] | [Ω] | [kW] | [kW] | Node i | Node j | [Ω] | [Ω] | [kW] | [kW] |
|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 2 | 0.0005 | 0.0012 | 0 | 0 | 3 | 36 | 0.0044 | 0.0108 | 26 | 18.55 |
| 2 | 3 | 0.0005 | 0.0012 | 0 | 0 | 36 | 37 | 0.0640 | 0.1565 | 26 | 18.55 |
| 3 | 4 | 0.0015 | 0.0036 | 0 | 0 | 37 | 38 | 0.1053 | 0.1230 | 0 | 0 |
| 4 | 5 | 0.0251 | 0.0294 | 0 | 0 | 38 | 39 | 0.0304 | 0.0355 | 24 | 17 |
| 5 | 6 | 0.3660 | 0.1864 | 2.6 | 2.2 | 39 | 40 | 0.0018 | 0.0021 | 24 | 17 |
| 6 | 7 | 0.3811 | 0.1941 | 40.4 | 30 | 40 | 41 | 0.7283 | 0.8509 | 102 | 1 |
| 7 | 8 | 0.0922 | 0.0470 | 75 | 54 | 41 | 42 | 0.3100 | 0.3623 | 0 | 0 |
| 8 | 9 | 0.0493 | 0.0251 | 30 | 22 | 42 | 43 | 0.0410 | 0.0478 | 6 | 4.3 |
| 9 | 10 | 0.8190 | 0.2707 | 28 | 19 | 43 | 44 | 0.0092 | 0.0116 | 0 | 0 |
| 10 | 11 | 0.1872 | 0.0619 | 145 | 104 | 44 | 45 | 0.1089 | 0.1373 | 39.22 | 26.3 |
| 11 | 12 | 0.7114 | 0.2351 | 145 | 104 | 45 | 46 | 0.0009 | 0.0012 | 39.22 | 26.3 |
| 12 | 13 | 1.0300 | 0.3400 | 8 | 5 | 4 | 47 | 0.0034 | 0.0084 | 0 | 0 |
| 13 | 14 | 1.0440 | 0.3450 | 8 | 5 | 47 | 48 | 0.0851 | 0.2083 | 79 | 56.4 |
| 14 | 15 | 1.0580 | 0.3496 | 0 | 0 | 48 | 49 | 0.2898 | 0.7091 | 384.7 | 274.5 |
| 15 | 16 | 0.1966 | 0.0650 | 45 | 30 | 49 | 50 | 0.0822 | 0.2011 | 384.7 | 274.5 |
| 16 | 17 | 0.3744 | 0.1238 | 60 | 35 | 8 | 51 | 0.0928 | 0.0473 | 40.5 | 28.3 |
| 17 | 18 | 0.0047 | 0.0016 | 60 | 35 | 51 | 52 | 0.3319 | 0.1140 | 3.6 | 2.7 |
| 18 | 19 | 0.3276 | 0.1083 | 0 | 0 | 9 | 53 | 0.1740 | 0.0886 | 4.35 | 3.5 |
| 19 | 20 | 0.2106 | 0.0690 | 1 | 0.6 | 53 | 54 | 0.2030 | 0.1034 | 26.4 | 19 |
| 20 | 21 | 0.3416 | 0.1129 | 114 | 81 | 54 | 55 | 0.2842 | 0.1447 | 24 | 17.2 |
| 21 | 22 | 0.0140 | 0.0046 | 5 | 3.5 | 55 | 56 | 0.2813 | 0.1433 | 0 | 0 |
| 22 | 23 | 0.1591 | 0.0526 | 0 | 0 | 56 | 57 | 1.5900 | 0.5337 | 0 | 0 |
| 23 | 24 | 0.3463 | 0.1145 | 28 | 20 | 57 | 58 | 0.7837 | 0.2630 | 0 | 0 |
| 24 | 25 | 0.7488 | 0.2475 | 0 | 0 | 58 | 59 | 0.3042 | 0.1006 | 100 | 72 |
| 25 | 26 | 0.3089 | 0.1021 | 14 | 10 | 59 | 60 | 0.3861 | 0.1172 | 0 | 0 |
| 26 | 27 | 0.1732 | 0.0572 | 14 | 10 | 60 | 61 | 0.5075 | 0.2585 | 1244 | 888 |
| 3 | 28 | 0.0044 | 0.0108 | 26 | 18.6 | 61 | 62 | 0.0974 | 0.0496 | 32 | 23 |
| 28 | 29 | 0.0640 | 0.1565 | 26 | 18.6 | 62 | 63 | 0.1450 | 0.0738 | 0 | 0 |
| 29 | 30 | 0.3978 | 0.1315 | 0 | 0 | 63 | 64 | 0.7105 | 0.3619 | 227 | 162 |
| 30 | 31 | 0.0702 | 0.0232 | 0 | 0 | 64 | 65 | 1.0410 | 0.5302 | 59 | 42 |
| 31 | 32 | 0.3510 | 0.1160 | 0 | 0 | 11 | 66 | 0.2012 | 0.0611 | 18 | 13 |
| 32 | 33 | 0.8390 | 0.2816 | 10 | 10 | 66 | 67 | 0.0047 | 0.0014 | 18 | 13 |
| 33 | 34 | 1.7080 | 0.5646 | 14 | 14 | 12 | 68 | 0.7394 | 0.2444 | 28 | 20 |
| 34 | 35 | 1.4740 | 0.4873 | 4 | 4 | 68 | 69 | 0.0047 | 0.0016 | 28 | 20 |
| Cases | SOCP | GAMS-IPOPT | GAMS-CONOPT4 | GAMS-KNITRO |
|---|---|---|---|---|
| C1 | 2.4069 | 2.4069 | 2.4069 | 2.4069 |
| C2 | 3.0802 | 3.0802 | 3.0802 | 3.0802 |
| C3 | 2.6994 | 2.6994 | 2.6994 | 2.6994 |
| Time (s) | PV1 (p.u) | PV2 (p.u) | WT1 (p.u) | WT2 (p.u) | Time (s) | PV1 (p.u) | PV2 (p.u) | WT1 (p.u) | WT2 (p.u) |
|---|---|---|---|---|---|---|---|---|---|
| 0.0 | 0 | 0 | 0.633118295 | 0.489955551 | 12.0 | 0.924486326 | 0.975683083 | 0.972218577 | 0.942224932 |
| 0.5 | 0 | 0 | 0.629764678 | 0.467954207 | 12.5 | 1 | 1 | 0.980049847 | 0.949956724 |
| 1.0 | 0 | 0 | 0.607259323 | 0.449443905 | 13.0 | 0.982041153 | 0.978264398 | 0.981135531 | 0.963773634 |
| 1.5 | 0 | 0 | 0.609254545 | 0.435019277 | 13.5 | 0.913674689 | 0.790055240 | 0.988644844 | 0.974977461 |
| 2.0 | 0 | 0 | 0.605557422 | 0.437220792 | 14.0 | 0.829407079 | 0.882557147 | 0.991393173 | 0.986750539 |
| 2.5 | 0 | 0 | 0.630055346 | 0.437621534 | 14.5 | 0.691912077 | 0.603658738 | 0.998815517 | 0.995058133 |
| 3.0 | 0 | 0 | 0.684246423 | 0.450949300 | 15.0 | 0.733063295 | 0.606324907 | 1 | 1 |
| 3.5 | 0 | 0 | 0.758357805 | 0.453259348 | 15.5 | 0.598435064 | 0.357393267 | 0.996070963 | 0.998107341 |
| 4.0 | 0 | 0 | 0.783719339 | 0.469610539 | 16.0 | 0.501133849 | 0.328035635 | 0.987258076 | 0.997690423 |
| 4.5 | 0 | 0 | 0.815243582 | 0.480546213 | 16.5 | 0.299821403 | 0.142423488 | 0.976519817 | 0.993076899 |
| 5.0 | 0 | 0 | 0.790557706 | 0.501783479 | 17.0 | 0.177117518 | 0.142023463 | 0.929542167 | 0.982629597 |
| 5.5 | 0 | 0 | 0.738679217 | 0.527600299 | 17.5 | 0.062736095 | 0.072956701 | 0.876413965 | 0.972084487 |
| 6.0 | 0 | 0 | 0.744958950 | 0.586555316 | 18.0 | 0 | 0.019081590 | 0.791155379 | 0.930225756 |
| 6.5 | 0 | 0 | 0.718989730 | 0.652552760 | 18.5 | 0 | 0.008339287 | 0.691292162 | 0.891253999 |
| 7.0 | 0.039123365 | 0.026135642 | 0.769603567 | 0.697699990 | 19.0 | 0.000333920 | 0 | 0.708839248 | 0.781950905 |
| 7.5 | 0.045414292 | 0.051715061 | 0.822376817 | 0.774442755 | 19.5 | 0 | 0 | 0.724074349 | 0.660094138 |
| 8.0 | 0.065587179 | 0.110148398 | 0.826492212 | 0.820205405 | 20.0 | 0 | 0 | 0.712881960 | 0.682715246 |
| 8.5 | 0.132615282 | 0.263094042 | 0.848620129 | 0.871057775 | 20.5 | 0 | 0 | 0.733954043 | 0.686617947 |
| 9.0 | 0.236870796 | 0.431175761 | 0.876523598 | 0.876973635 | 21.0 | 0 | 0 | 0.719897641 | 0.681865563 |
| 9.5 | 0.410356256 | 0.594273035 | 0.904128455 | 0.877065236 | 21.5 | 0 | 0 | 0.705502389 | 0.717315757 |
| 10.0 | 0.455017818 | 0.730402039 | 0.931213527 | 0.897955131 | 22.0 | 0 | 0 | 0.703007456 | 0.718080346 |
| 10.5 | 0.542364455 | 0.830347309 | 0.955557477 | 0.903245007 | 22.5 | 0 | 0 | 0.686551618 | 0.726890145 |
| 11.0 | 0.726440265 | 0.875407050 | 0.965504834 | 0.916903429 | 23.0 | 0 | 0 | 0.687238555 | 0.734452193 |
| 11.5 | 0.885104984 | 0.898815348 | 0.971037333 | 0.924757605 | 23.5 | 0 | 0 | 0.682569771 | 0.739699146 |
| Cases | SOCP | GAMS-IPOPT | GAMS-CONOPT4 | GAMS-KNITRO |
|---|---|---|---|---|
| C1 | 2.2118 | 2.2118 | 2.2118 | 2.2118 |
| C2 | 2.9382 | 2.9382 | 2.9382 | 2.9382 |
| C3 | 2.4779 | 2.4779 | 2.4779 | 2.4779 |
| Solver | Location | Size [pu] | -Coefficient | Proc. Times [s] |
|---|---|---|---|---|
| BONMIN | 3.3091 | 9.4770 | ||
| DICOPT | 3.3074 | 2.6730 | ||
| KNITRO | 3.3184 | 2.6730 | ||
| SBB | 3.3010 | 2.8250 | ||
| MI-SOCP | 3.3187 | 5.1406 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Montoya, O.D.; Gil-González, W.; Arias-Londoño, A.; Rajagopalan, A.; Hernández, J.C. Voltage Stability Analysis in Medium-Voltage Distribution Networks Using a Second-Order Cone Approximation. Energies 2020, 13, 5717. https://doi.org/10.3390/en13215717
Montoya OD, Gil-González W, Arias-Londoño A, Rajagopalan A, Hernández JC. Voltage Stability Analysis in Medium-Voltage Distribution Networks Using a Second-Order Cone Approximation. Energies. 2020; 13(21):5717. https://doi.org/10.3390/en13215717
Chicago/Turabian StyleMontoya, Oscar Danilo, Walter Gil-González, Andrés Arias-Londoño, Arul Rajagopalan, and Jesus C. Hernández. 2020. "Voltage Stability Analysis in Medium-Voltage Distribution Networks Using a Second-Order Cone Approximation" Energies 13, no. 21: 5717. https://doi.org/10.3390/en13215717
APA StyleMontoya, O. D., Gil-González, W., Arias-Londoño, A., Rajagopalan, A., & Hernández, J. C. (2020). Voltage Stability Analysis in Medium-Voltage Distribution Networks Using a Second-Order Cone Approximation. Energies, 13(21), 5717. https://doi.org/10.3390/en13215717








