Optimization Model for the Long-Term Operation of an Interprovincial Hydropower Plant Incorporating Peak Shaving Demands
Abstract
1. Introduction
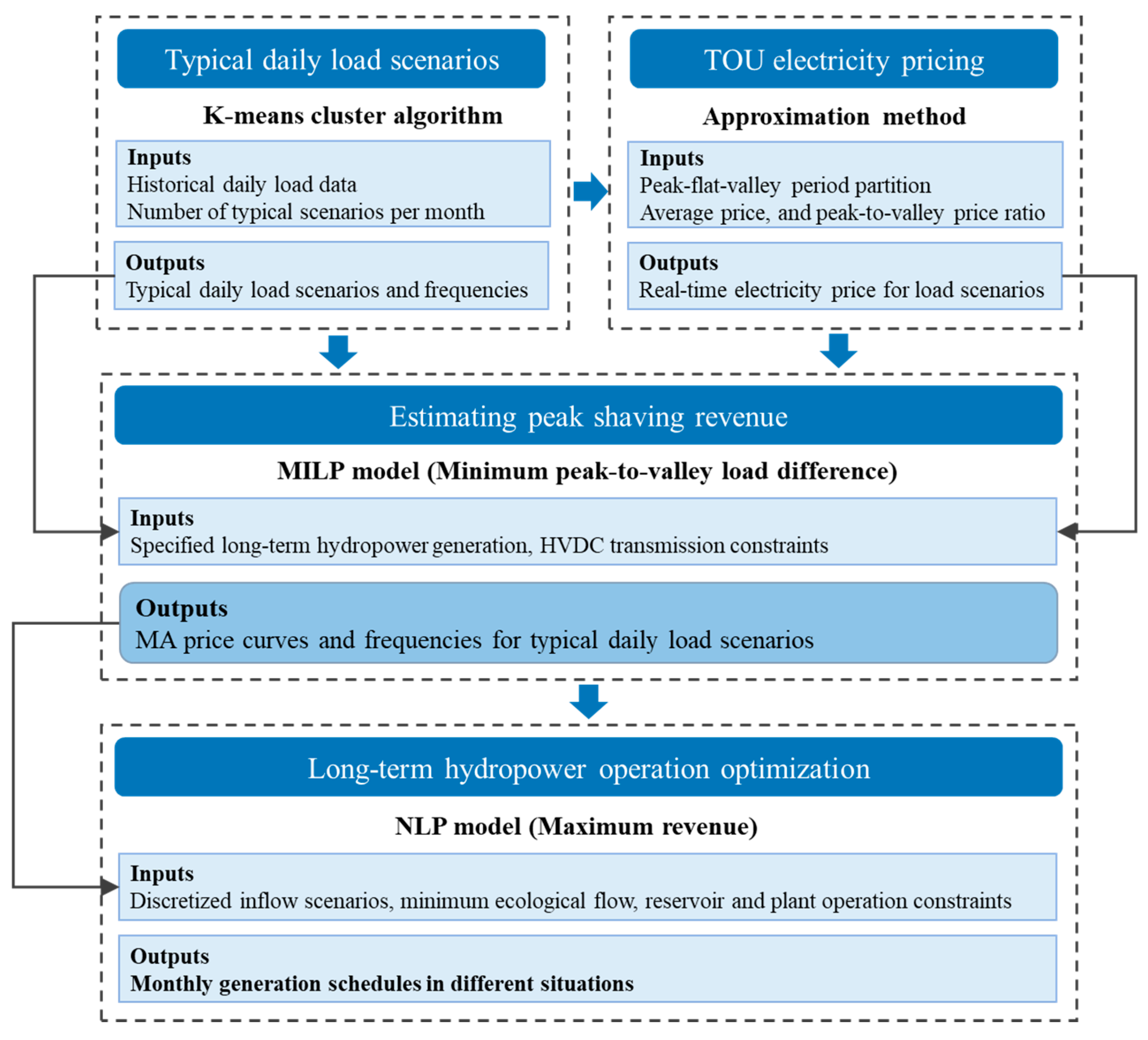
2. Methodology
2.1. TOU Pricing for Typical Daily Load Scenarios
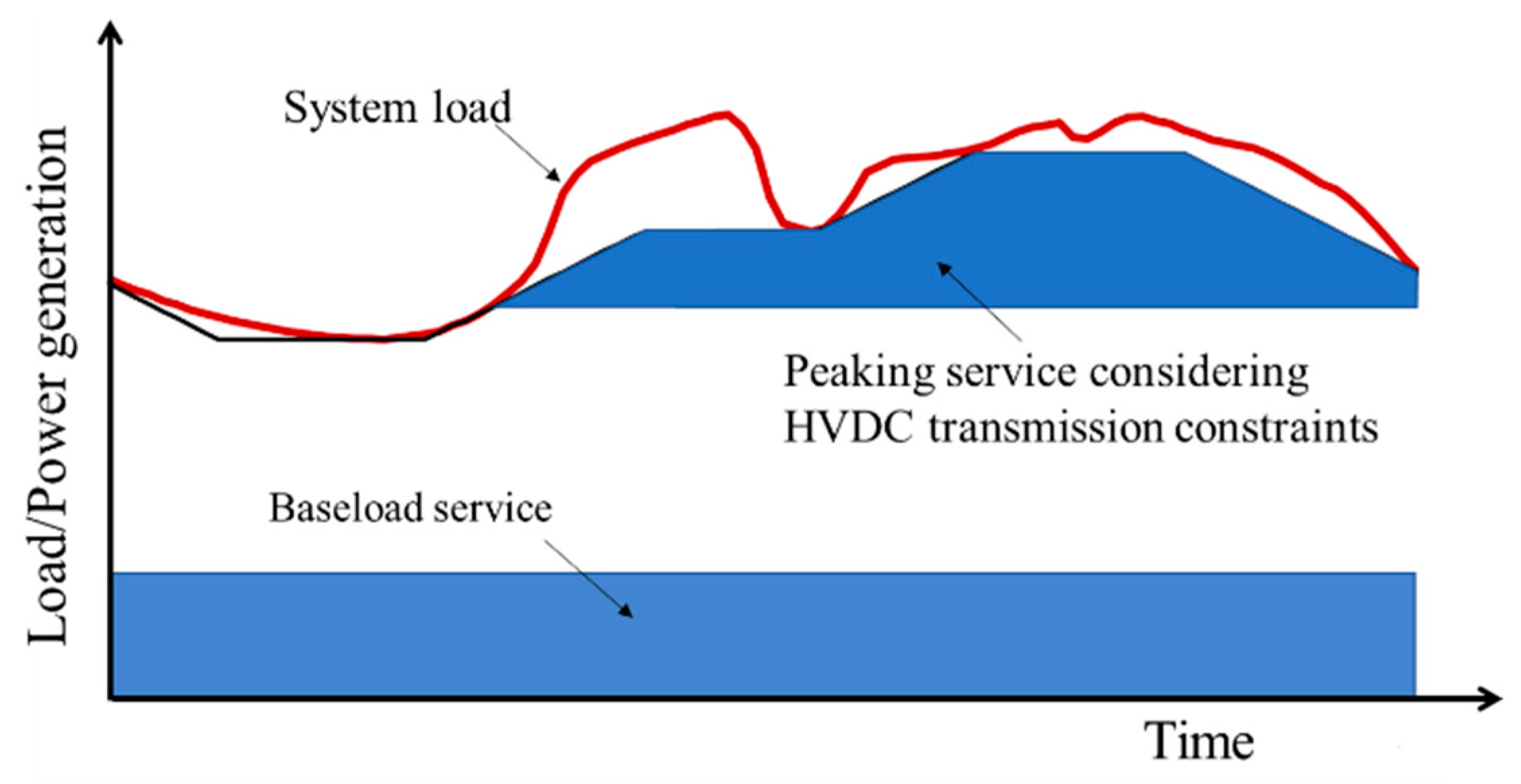
2.2. Estimating Peak Shaving Revenue
2.2.1. Objective Function
2.2.2. Constraints
2.2.3. MILP Model Reformulation
2.3. Modeling the Long-Term Operation of a Hydropower Plant
2.4. Solutions
3. Case Study
3.1. Xiluodu–Zhejiang HVDC Transmission Project
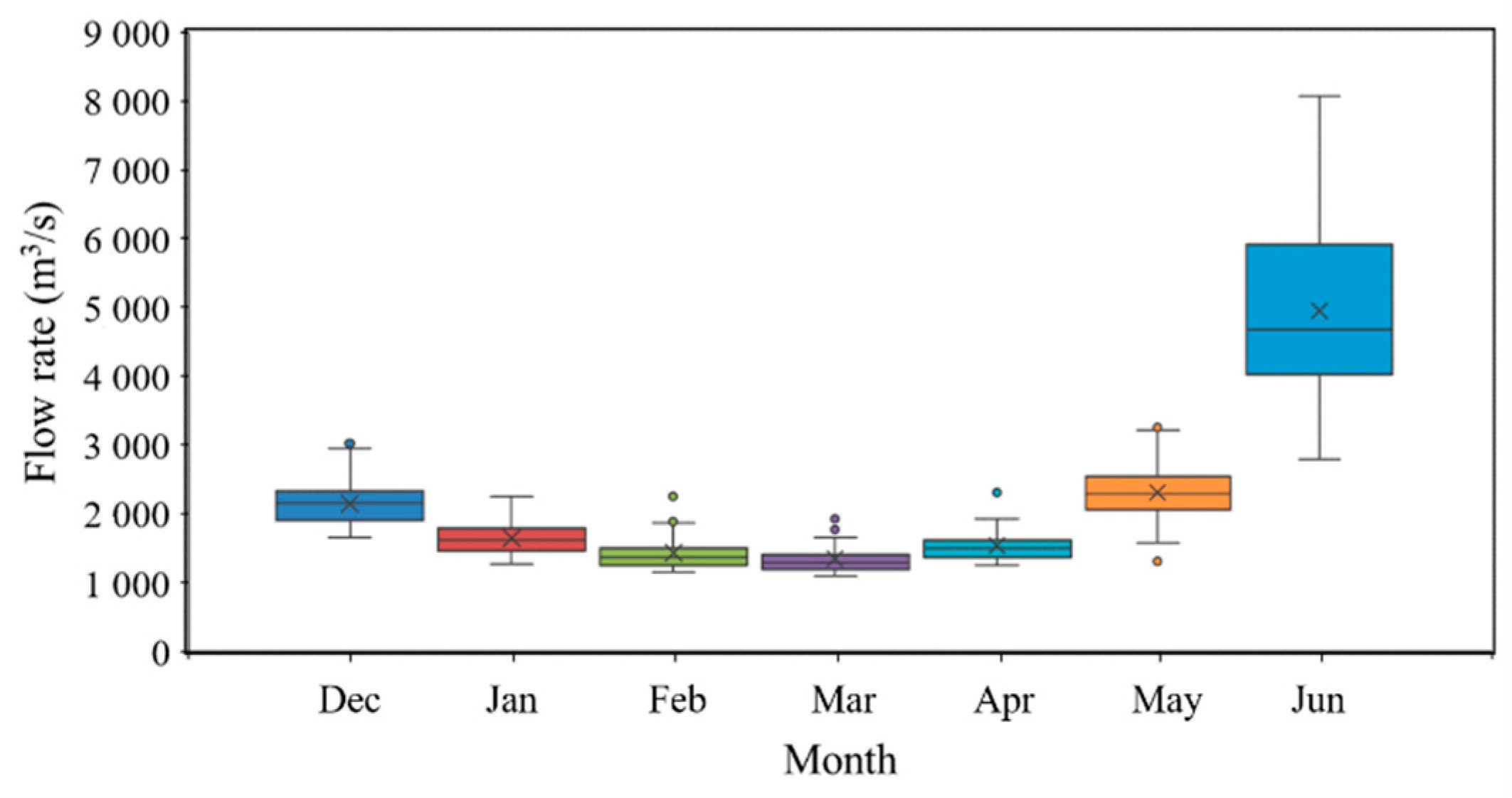
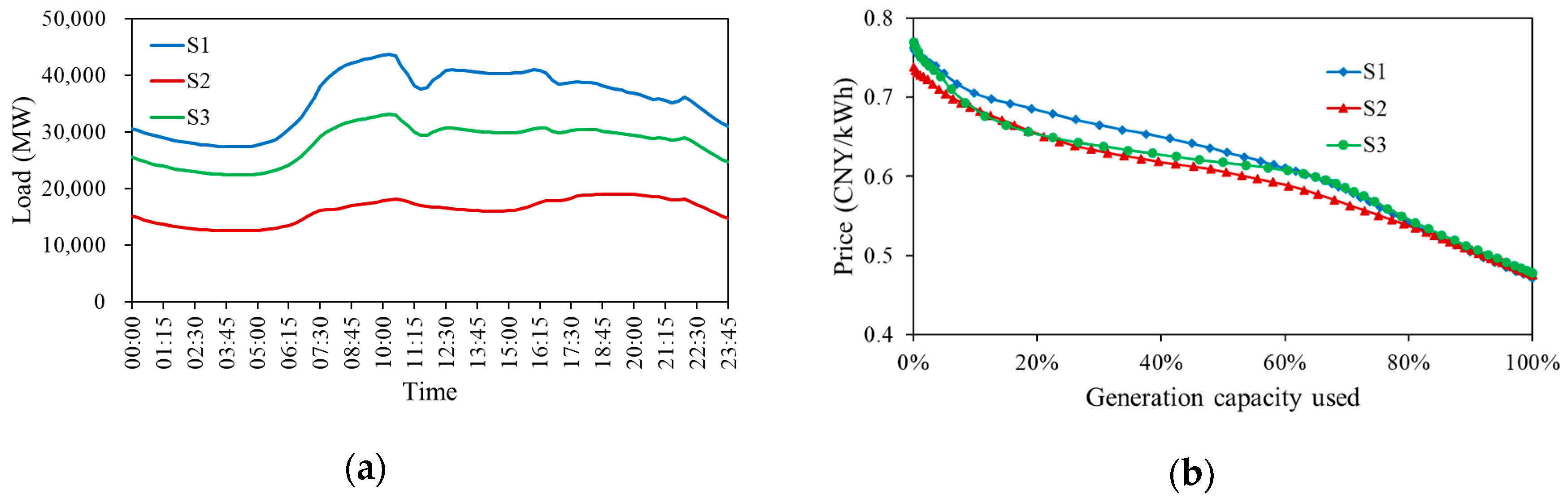
3.2. Input Data and Settings
4. Results and Discussion
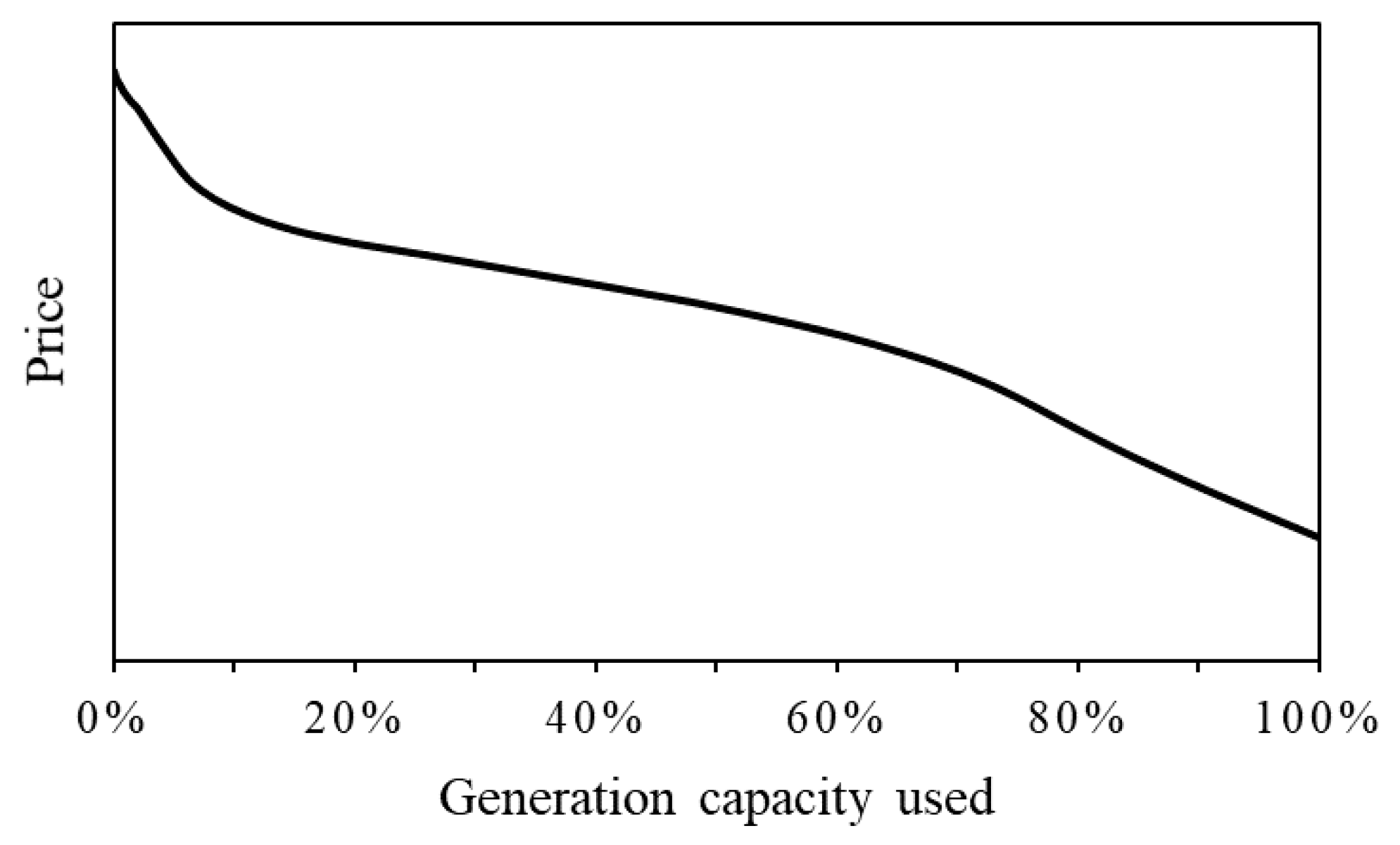
4.1. Relationship between Energy Production and Peak Shaving Revenue
4.2. Effect of HVDC Transmission Stability Constraints
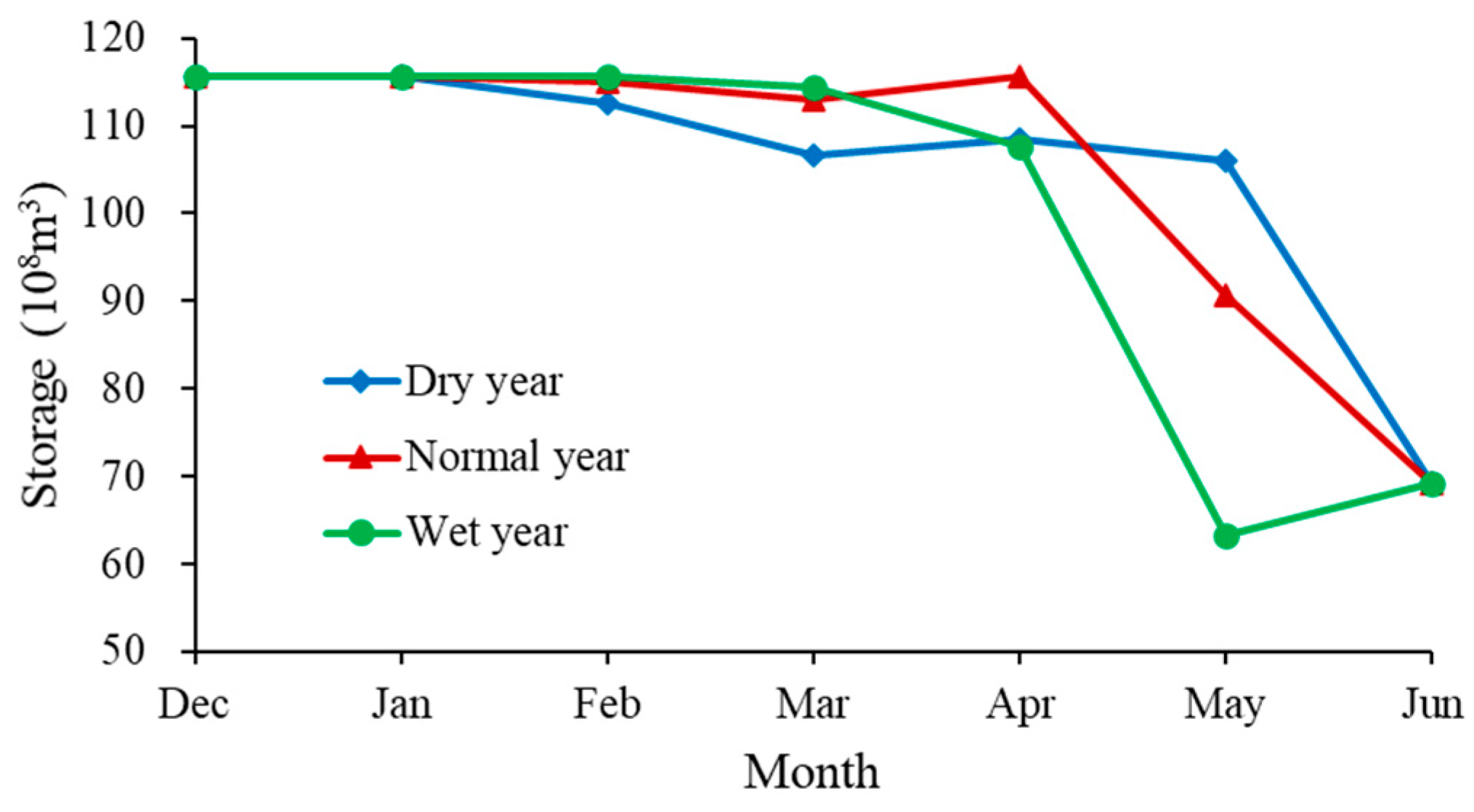
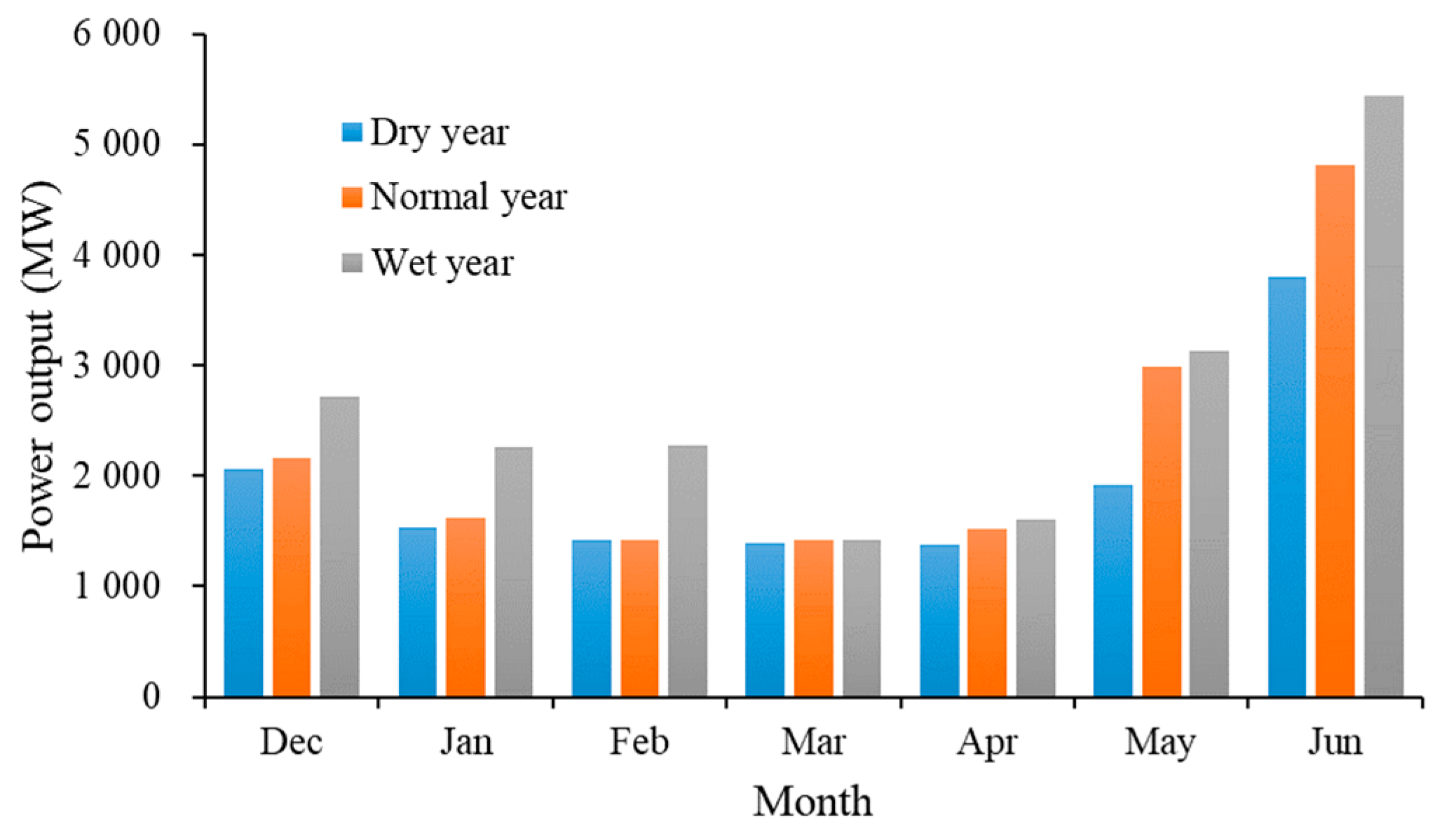
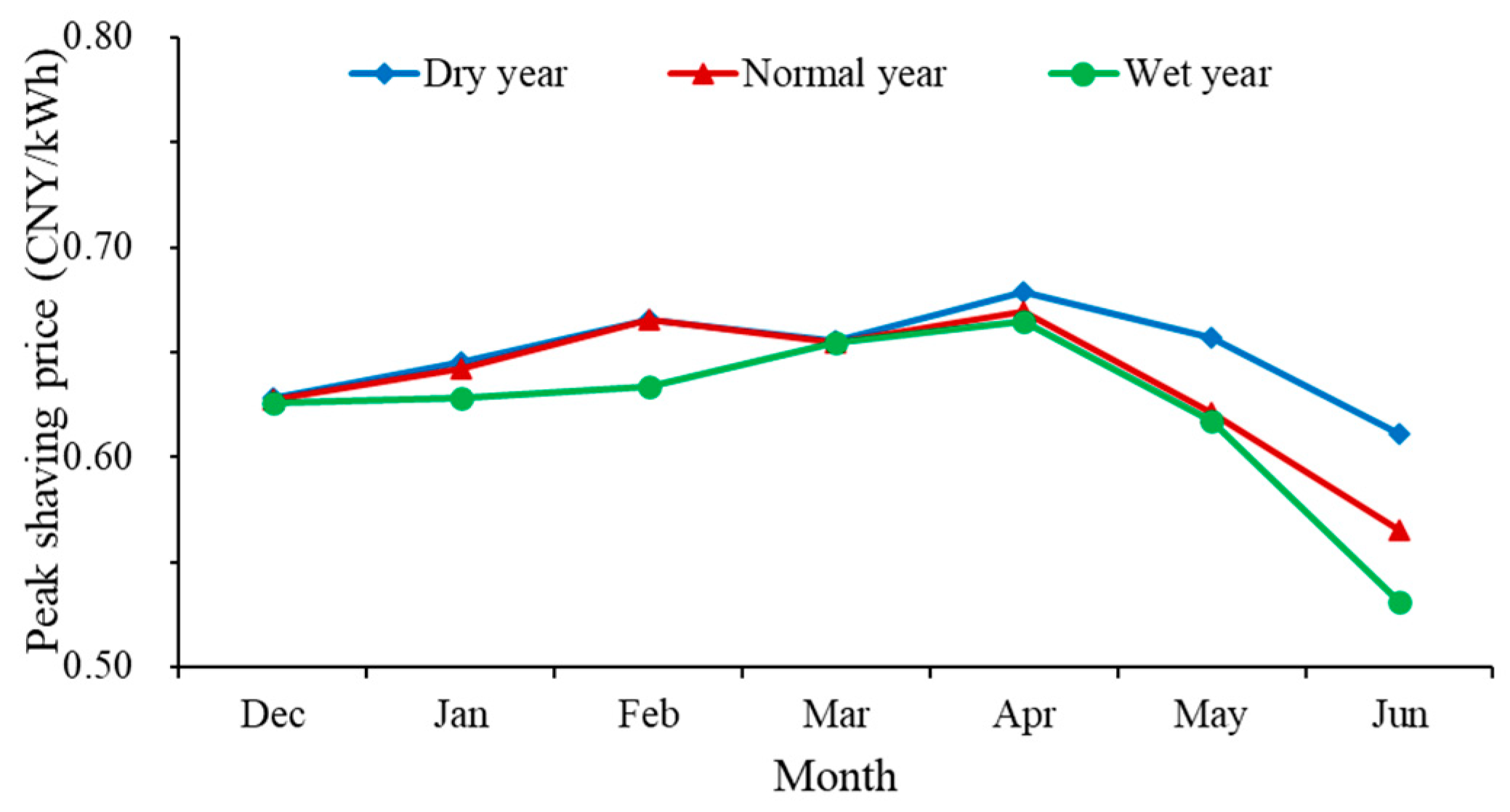
4.3. Operation Schemes in Different Inflow Scenarios
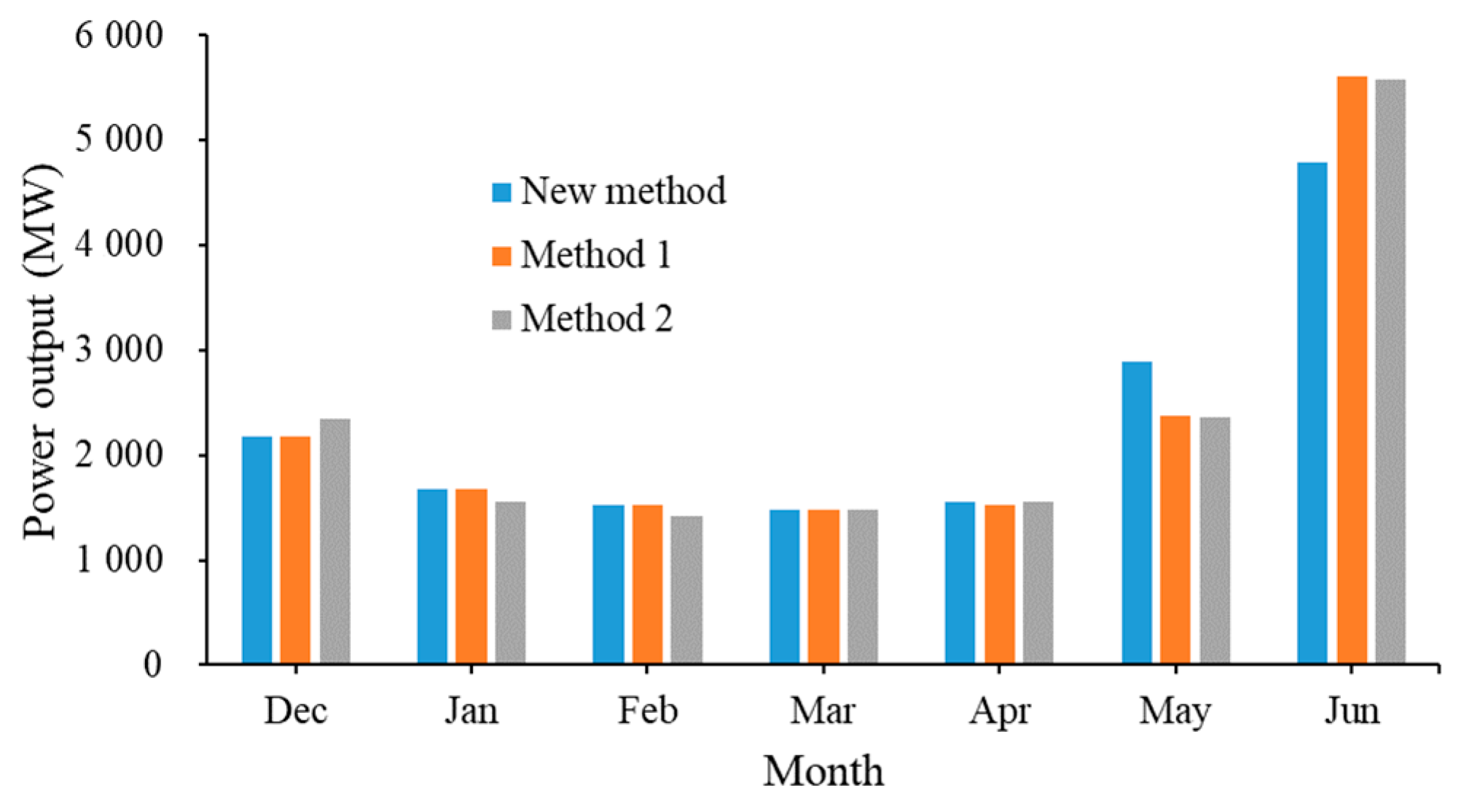
4.4. Performance Comparison
5. Conclusions
- (1)
- The MA price curves, which characterize the relationship between energy production and peak shaving revenue, verified that the marginal revenue of peak shaving decreases with increasing power generation.
- (2)
- HVDC transmission stability constraints significantly limit the peak shaving ability of the XHP, which leads to significant reductions in energy prices and generation revenue.
- (3)
- Compared with the existing optimization methods, the model proposed in this paper can effectively increase long-term cumulative power generation revenue, although we found a small reduction in energy production. Thus, the proposed approach is effective for exploring the revenue potential of interprovincial hydropower transmission to meet peak shaving demands.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- International Hydropower Association (IHA). 2019 Hydropower Status Report; IHA: London, UK, 2019. [Google Scholar]
- Li, Y.; Li, Y.B.; Ji, P.F.; Yang, J. The status quo analysis and policy suggestions on promoting China׳s hydropower development. Renew. Sustain. Energy Rev. 2015, 51, 1071–1079. [Google Scholar] [CrossRef]
- Zeng, M.; Peng, L.L.; Fan, Q.N.; Zhang, Y.J. Trans-regional electricity transmission in China: Status, issues and strategies. Renew. Sustain. Energy Rev. 2016, 66, 572–583. [Google Scholar]
- Shen, J.J.; Zhang, X.F.; Wang, J.; Cao, R.; Wang, S.; Zhang, J. Optimal Operation of Interprovincial Hydropower System Including Xiluodu and Local Plants in Multiple Recipient Regions. Energies 2019, 12, 144. [Google Scholar] [CrossRef]
- Farahmand, H.; Jaehnert, S.; Aigner, T.; Huertas-Hernando, D. Nordic hydropower flexibility and transmission expansion to support integration of North European wind power. Wind Energy 2015, 18, 1075–1103. [Google Scholar] [CrossRef]
- Hennig, T.; Wang, W.L.; Magee, D.; He, D.M. Yunnan’s Fast-Paced Large Hydropower Development: A Powershed-Based Approach to Critically Assessing Generation and Consumption Paradigms. Water 2016, 8, 476. [Google Scholar] [CrossRef]
- Xie, K.G.; Dong, J.Z.; Tai, H.M.; Hu, B.; He, H.L. Optimal planning of HVDC-based bundled wind-thermal generation and transmission system. Energy Convers. Manag. 2016, 115, 71–79. [Google Scholar] [CrossRef]
- Cheng, C.T.; Yan, L.Z.; Mirchi, A.; Madani, K. China’s booming hydropower: System modeling challenges and opportunities. J. Water Resour. Plan. Manag. 2017, 143, 1–5. [Google Scholar] [CrossRef]
- Ding, N.; Duan, J.H.; Xue, S.; Zeng, M.; Shen, J.F. Overall review of peaking power in China: Status quo, barriers and solutions. Renew. Sustain. Energy Rev. 2015, 42, 503–516. [Google Scholar] [CrossRef]
- Gu, Y.J.; Xu, J.; Chen, D.C.; Wang, Z.; Li, Q.Q. Overall review of peak shaving for coal-fired power units in China. Renew. Sustain. Energy Rev. 2016, 54, 723–731. [Google Scholar] [CrossRef]
- Ye, Y.J.; Huang, W.B.; Ma, G.W.; Wang, J.L.; Liu, Y.; Hu, Y.L. Cause analysis and policy options for the surplus hydropower in southwest China based on quantification. J. Renew. Sustain. Energy 2018, 10, 015908. [Google Scholar] [CrossRef]
- Su, C.G.; Cheng, C.T.; Wang, P.L.; Shen, J.J. Optimization Model for the Short-Term Operation of Hydropower Plants Transmitting Power to Multiple Power Grids via HVDC Transmission Lines. IEEE Access 2019, 7, 139236–139248. [Google Scholar] [CrossRef]
- Shen, J.J.; Cheng, C.T.; Wang, S.; Yuan, X.Y.; Sun, L.F.; Zhang, J. Multiobjective optimal operations for an interprovincial hydropower system considering peak-shaving demands. Renew. Sustain. Energy Rev. 2020, 120, 109617. [Google Scholar] [CrossRef]
- Hammons, T.J.; Lescale, V.F.; Uecker, K.; Haeusler, M.; Retzmann, D.; Staschus, K.; Lepy, S. State of the art in ultrahigh-voltage transmission. Proc. IEEE 2012, 100, 360–390. [Google Scholar] [CrossRef]
- Xie, M.F.; Zhou, J.Z.; Li, C.L.; Lu, P. Daily generation scheduling of cascade hydro plants considering peak shaving constraints. J. Water Resour. Plan. Manag. 2015, 142, 04015072. [Google Scholar] [CrossRef]
- Feng, Z.K.; Niu, W.J.; Cheng, C.T.; Zhou, J.Z. Peak shaving operation of hydro-thermal-nuclear plants serving multiple power grids by linear programming. Energy 2017, 135, 210–219. [Google Scholar] [CrossRef]
- Shen, J.J.; Cheng, C.T.; Cheng, X.; Lund, J.R. Coordinated operations of large-scale UHVDC hydropower and conventional hydro energies about regional power grid. Energy 2016, 95, 433–446. [Google Scholar] [CrossRef]
- Sreekanth, J.; Datta, B.; Mohapatra, P.K. Optimal short-term reservoir operation with integrated long-term goals. Water Resour. Manag. 2012, 26, 2833–2850. [Google Scholar] [CrossRef]
- Xu, B.; Zhong, P.A.; Stanko, Z.; Zhao, Y.F.; Yeh, W.W. A multiobjective short-term optimal operation model for a cascade system of reservoirs considering the impact on long-term energy production. Water Resour. Res. 2015, 51, 3353–3369. [Google Scholar] [CrossRef]
- Ming, B.; Liu, P.; Guo, S.L.; Cheng, L.; Zhang, J.W. Hydropower reservoir reoperation to adapt to large-scale photovoltaic power generation. Energy 2019, 179, 268–279. [Google Scholar] [CrossRef]
- English, J.; Niet, T.; Lyseng, B.; Keller, V.; Palmer-Wilson, K.; Robertson, B.; Wild, P.; Rowe, A. Flexibility requirements and electricity system planning: Assessing inter-regional coordination with large penetrations of variable renewable supplies. Renew. Energy 2020, 145, 2770–2782. [Google Scholar] [CrossRef]
- Morillo, J.L.; Zephyr, L.; Perez, J.F.; Anderson, C.L.; Cadena, A. Risk-averse stochastic dual dynamic programming approach for the operation of a hydro-dominated power system in the presence of wind uncertainty. Int. J. Electr. Power Energy Syst. 2020, 115, 105469. [Google Scholar] [CrossRef]
- Madani, K.; Lund, J.R. Modeling California′s high-elevation hydropower systems in energy units. Water Resour. Res. 2009, 45. [Google Scholar] [CrossRef]
- Olivares, M.A.; Lund, J.R. Representing energy price variability in long-and medium-term hydropower optimization. J. Water Resour. Plan. Manag. 2012, 138, 606–613. [Google Scholar] [CrossRef]
- Ak, M.; Kentel, E.; Savasaneril, S. Operating policies for energy generation and revenue management in single-reservoir hydropower systems. Renew. Sustain. Energy Rev. 2017, 78, 1253–1261. [Google Scholar] [CrossRef]
- Moreira, A.; Pozo, D.; Street, A.; Sauma, E. Reliable renewable generation and transmission expansion planning: Co-optimizing system′s resources for meeting renewable targets. IEEE Trans. Power Syst. 2016, 32, 3246–3257. [Google Scholar] [CrossRef]
- Zhang, H.X.; Lu, Z.X.; Hu, W.; Wang, Y.T.; Dong, L.; Zhang, J.T. Coordinated optimal operation of hydro-wind-solar integrated systems. Appl. Energy 2019, 242, 883–896. [Google Scholar] [CrossRef]
- Zhao, L.; Yang, Z.Y.; Lee, W.J. The impact of time-of-use (TOU) rate structure on consumption patterns of the residential customers. IEEE Trans. Ind. Appl. 2017, 53, 5130–5138. [Google Scholar] [CrossRef]
- Yang, H.J.; Wang, L.; Zhang, Y.Y.; Ma, Y.H.; Zhou, M. Reliability evaluation of power system considering time of use electricity pricing. IEEE Trans. Power Syst. 2018, 34, 1991–2002. [Google Scholar] [CrossRef]
- Wu, X.Y.; Li, S.M.; Cheng, C.T.; Miao, S.M.; Ying, Q.L. Simulation-Optimization Model to Derive Operation Rules of Multiple Cascaded Reservoirs for Nash Equilibrium. J. Water Resour. Plan. Manag. 2019, 145, 04019013. [Google Scholar] [CrossRef]
- Castro, P.M.; Grossmann, I.E. Generalized disjunctive programming as a systematic modeling framework to derive scheduling formulations. Ind. Eng. Chem. Res. 2012, 51, 5781–5792. [Google Scholar] [CrossRef]
- Tong, B.; Zhai, Q.Z.; Guan, X.H. An MILP Based Formulation for Short-Term Hydro Generation Scheduling with Analysis of the Linearization Effects on Solution Feasibility. IEEE Trans. Power Syst. 2013, 28, 3588–3599. [Google Scholar] [CrossRef]
- Feng, Z.K.; Niu, W.J.; Wang, W.C.; Zhou, J.Z.; Cheng, C.T. A mixed integer linear programming model for unit commitment of thermal plants with peak shaving operation aspect in regional power grid lack of flexible hydropower energy. Energy 2019, 175, 618–629. [Google Scholar] [CrossRef]
- Xu, B.; Boyce, S.E.; Zhang, Y.; Liu, Q.; Guo, L.; Zhong, P.A. Stochastic Programming with a Joint Chance Constraint Model for Reservoir Refill Operation Considering Flood Risk. J. Water Resour. Plan. Manag. 2017, 143, 04016067. [Google Scholar] [CrossRef]
- Li, X.; Li, T.J.; Wei, J.H.; Wang, G.Q.; Yeh, W.W.G. Hydro unit commitment via mixed integer linear programming: A case study of the Three Gorges Project, China. IEEE Trans. Power Syst. 2014, 29, 1232–1241. [Google Scholar] [CrossRef]
- Gau, C.Y.; Schrage, L.E. Implementation and testing of a branch-and-bound based method for deterministic global optimization: Operations research applications. In Frontiers in Global Optimization; Floudas, C.A., Pardalos, P.M., Eds.; Springer: Boston, MA, USA, 2004; Volume 74, pp. 145–164. [Google Scholar]
- Zhu, F.L.; Zhong, P.A.; Sun, Y.M.; Yeh, W.W.G. Real-time optimal flood control decision making and risk propagation under multiple uncertainties. Water Resour. Res. 2017, 53, 10635–10654. [Google Scholar] [CrossRef]
- Xu, B.; Zhu, F.L.; Zhong, P.A.; Chen, J.; Liu, W.F.; Ma, Y.F.; Guo, L.; Deng, X.L. Identifying long-term effects of using hydropower to complement wind power uncertainty through stochastic programming. Appl. Energy 2019, 253, 113535. [Google Scholar] [CrossRef]
- Wang, J.; Cheng, C.T.; Wu, X.Y.; Shen, J.J.; Cao, R. Optimal Hedging for Hydropower Operation and End-of-Year Carryover Storage Values. J. Water Resour. Plan. Manag. 2019, 145, 04019003. [Google Scholar] [CrossRef]
- Zhou, Y.L.; Guo, S.L.; Liu, P.; Xu, C.Y. Joint operation and dynamic control of flood limiting water levels for mixed cascade reservoir systems. J. Hydrol. 2014, 519, 248–257. [Google Scholar] [CrossRef]
- Mu, J.; Ma, C.; Zhao, J.Q.; Lian, J.J. Optimal operation rules of Three-gorge and Gezhouba cascade hydropower stations in flood season. Energy Conv. Manag. 2015, 96, 159–174. [Google Scholar] [CrossRef]
- Tilmant, A.; Kelman, R. A stochastic approach to analyze trade-offs and risks associated with large-scale water resources systems. Water Resour. Res. 2007, 43. [Google Scholar] [CrossRef]
- Zambon, R.C.; Barros, M.T.L.; Lopes, J.E.G.; Barbosa, P.S.F.; Francato, A.L.; Yeh, W.W.G. Optimization of large-scale hydrothermal system operation. J. Water Resour. Plan. Manag. 2012, 138, 135–143. [Google Scholar] [CrossRef]






| Component | Parameter | Value | Unit |
|---|---|---|---|
| Reservoir of XHP | Installed capacity | 12,600 | MW |
| Normal water level | 600.00 | m | |
| Dead water level | 540.00 | m | |
| Flood-limited water level | 560.00 | m | |
| Active storage | 64.6 | 108 m3 | |
| Minimum ecological flow | 1400 | m3/s | |
| XZ-line | Transmission capacity | 8000 | MW |
| Maximum ramping capacity (ΔPH) | 600 | MW |
| Factor Case () | Power Generation (108 kWh) | Total Revenue (108 CNY) | Average Price (CNY/kWh) | Peak Price (CNY/kWh) | Baseload Price (CNY/kWh) |
|---|---|---|---|---|---|
| No constraints | 114.95 | 63.52 | 0.553 | 0.647 | 0.476 |
| 16 | 115.9 | 61.51 | 0.531 | 0.597 | 0.476 |
| 32 | 116.20 | 60.91 | 0.524 | 0.582 | 0.476 |
| 48 | 116.52 | 60.51 | 0.519 | 0.571 | 0.476 |
| Hydrological Pattern | Power Generation (108 kWh) | Total Revenue (108 CNY) | Average Price (CNY/kWh) | Peak Price (CNY/kWh) |
|---|---|---|---|---|
| Dry | 97.18 | 51.37 | 0.529 | 0.627 |
| Normal | 114.79 | 60.95 | 0.531 | 0.599 |
| Wet | 135.68 | 72.66 | 0.536 | 0.588 |
| Expected value | 115.9 | 61.51 | 0.531 | 0.597 |
| Hydrological Pattern | Models | Power Generation (108 kWh) | Total Revenue (108 CNY) | Average Price (CNY/kWh) |
|---|---|---|---|---|
| Dry | Method 1 | 97.72 | 51.26 | 0.525 |
| Method 2 | 97.57 | 51.15 | 0.524 | |
| Normal | Method 1 | 117.01 | 59.92 | 0.512 |
| Method 2 | 116.74 | 59.74 | 0.512 | |
| Wet | Method 1 | 138.61 | 71.94 | 0.519 |
| Method 2 | 136.05 | 70.39 | 0.517 | |
| Expected value | Method 1 | 117.75 | 60.85 | 0.517 |
| Method 2 | 117.19 | 60.61 | 0.517 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cao, R.; Shen, J.; Cheng, C.; Wang, J. Optimization Model for the Long-Term Operation of an Interprovincial Hydropower Plant Incorporating Peak Shaving Demands. Energies 2020, 13, 4804. https://doi.org/10.3390/en13184804
Cao R, Shen J, Cheng C, Wang J. Optimization Model for the Long-Term Operation of an Interprovincial Hydropower Plant Incorporating Peak Shaving Demands. Energies. 2020; 13(18):4804. https://doi.org/10.3390/en13184804
Chicago/Turabian StyleCao, Rui, Jianjian Shen, Chuntian Cheng, and Jian Wang. 2020. "Optimization Model for the Long-Term Operation of an Interprovincial Hydropower Plant Incorporating Peak Shaving Demands" Energies 13, no. 18: 4804. https://doi.org/10.3390/en13184804
APA StyleCao, R., Shen, J., Cheng, C., & Wang, J. (2020). Optimization Model for the Long-Term Operation of an Interprovincial Hydropower Plant Incorporating Peak Shaving Demands. Energies, 13(18), 4804. https://doi.org/10.3390/en13184804







