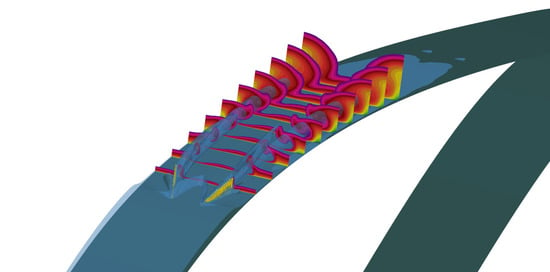
jBAY Modeling of Vane-Type Vortex Generators and Study on Airfoil Aerodynamic Performance
Abstract
1. Introduction
2. Materials and Methods
2.1. jBAY Model
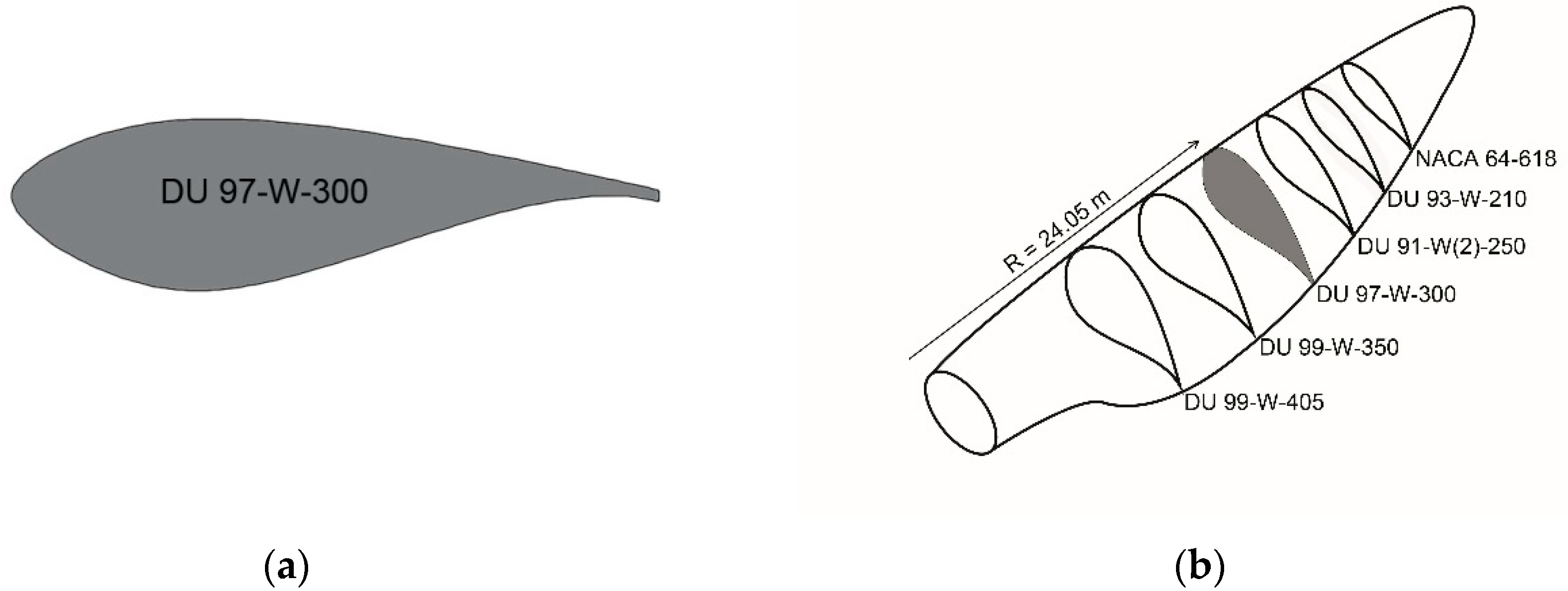
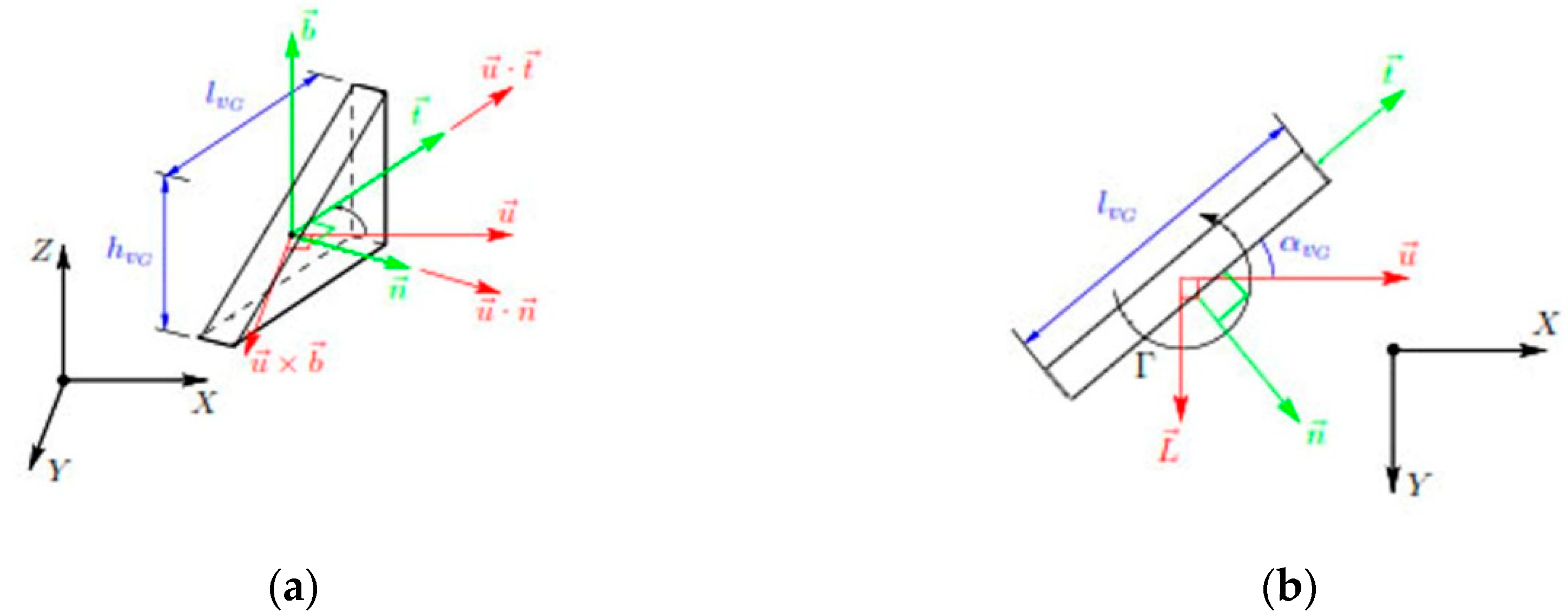
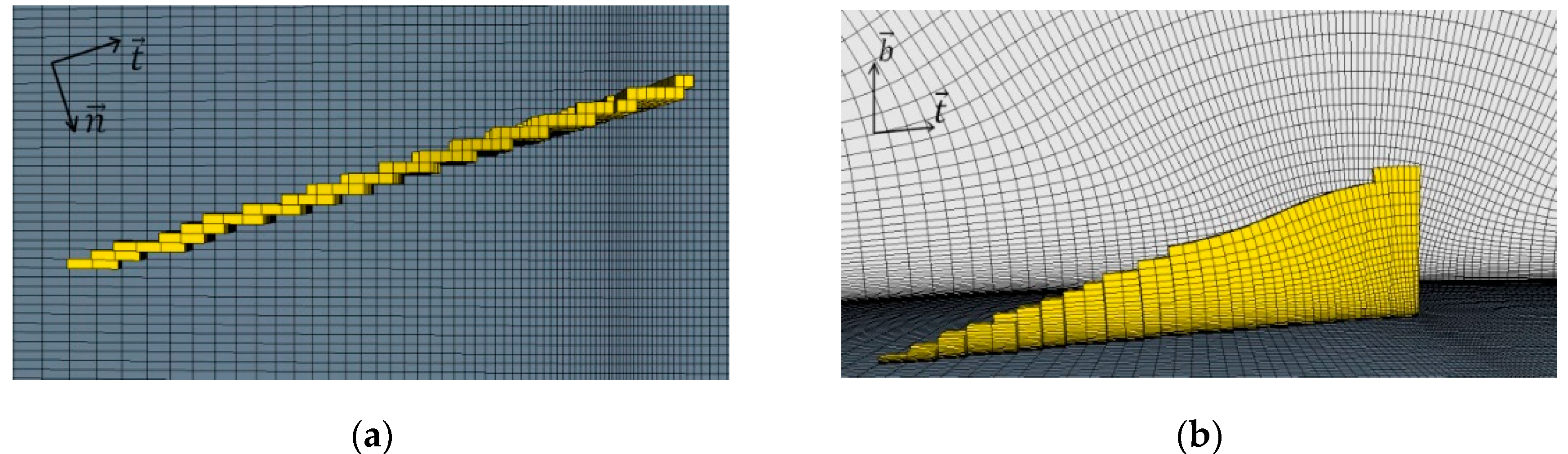
2.2. Computational Domain and VG Setup
2.3. Numerical Models
3. Results
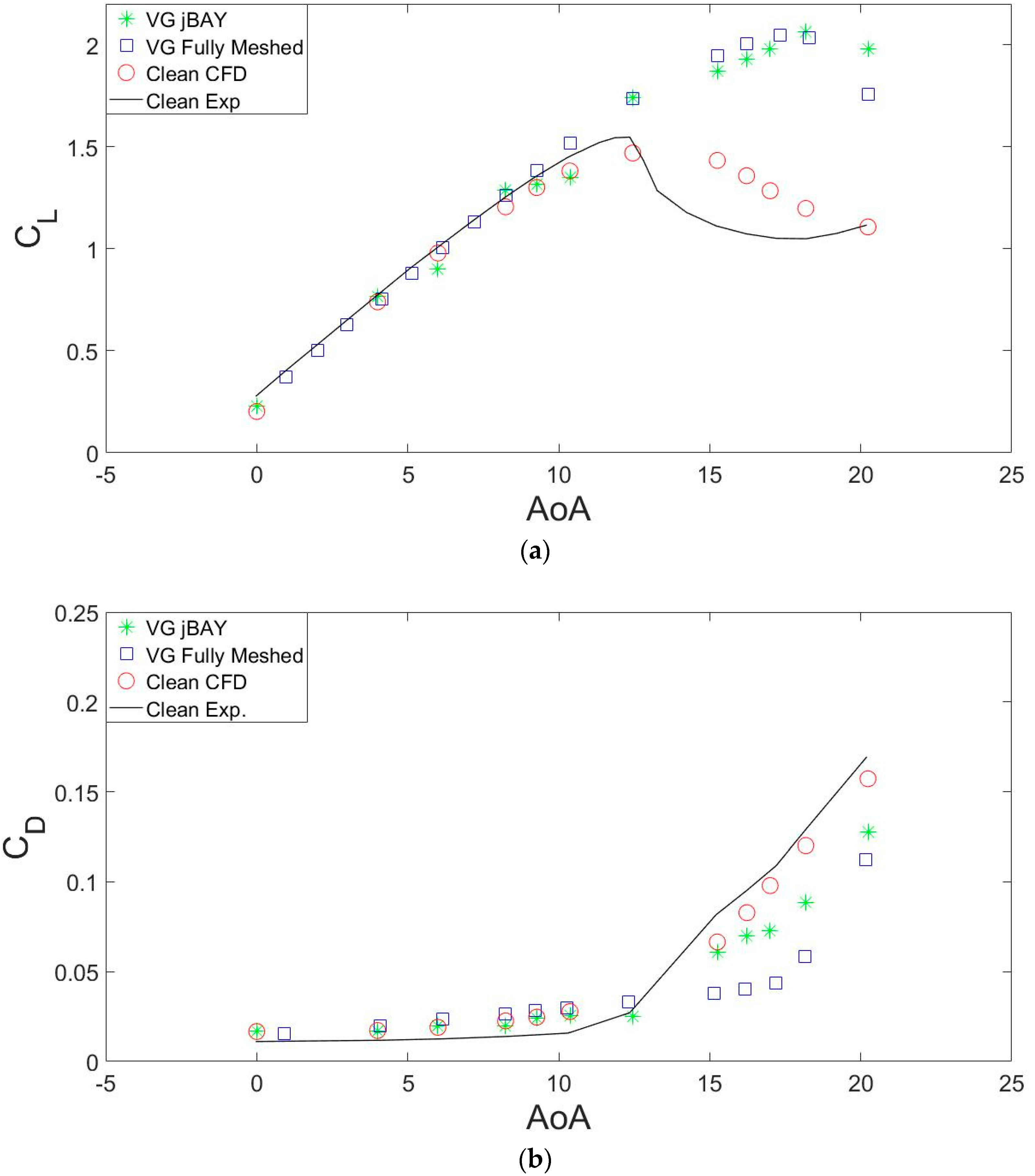
3.1. Lift and Drag Coefficients
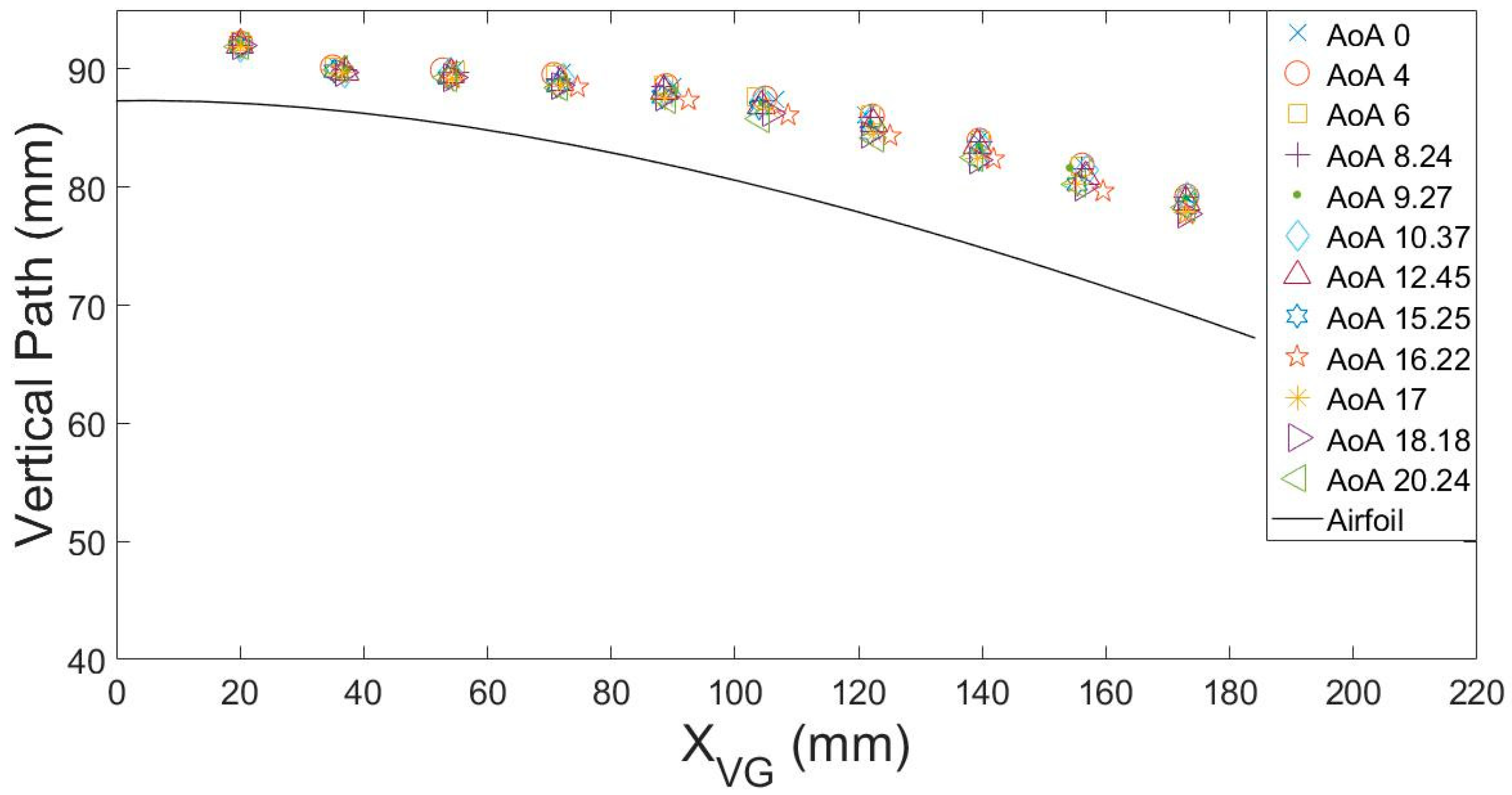
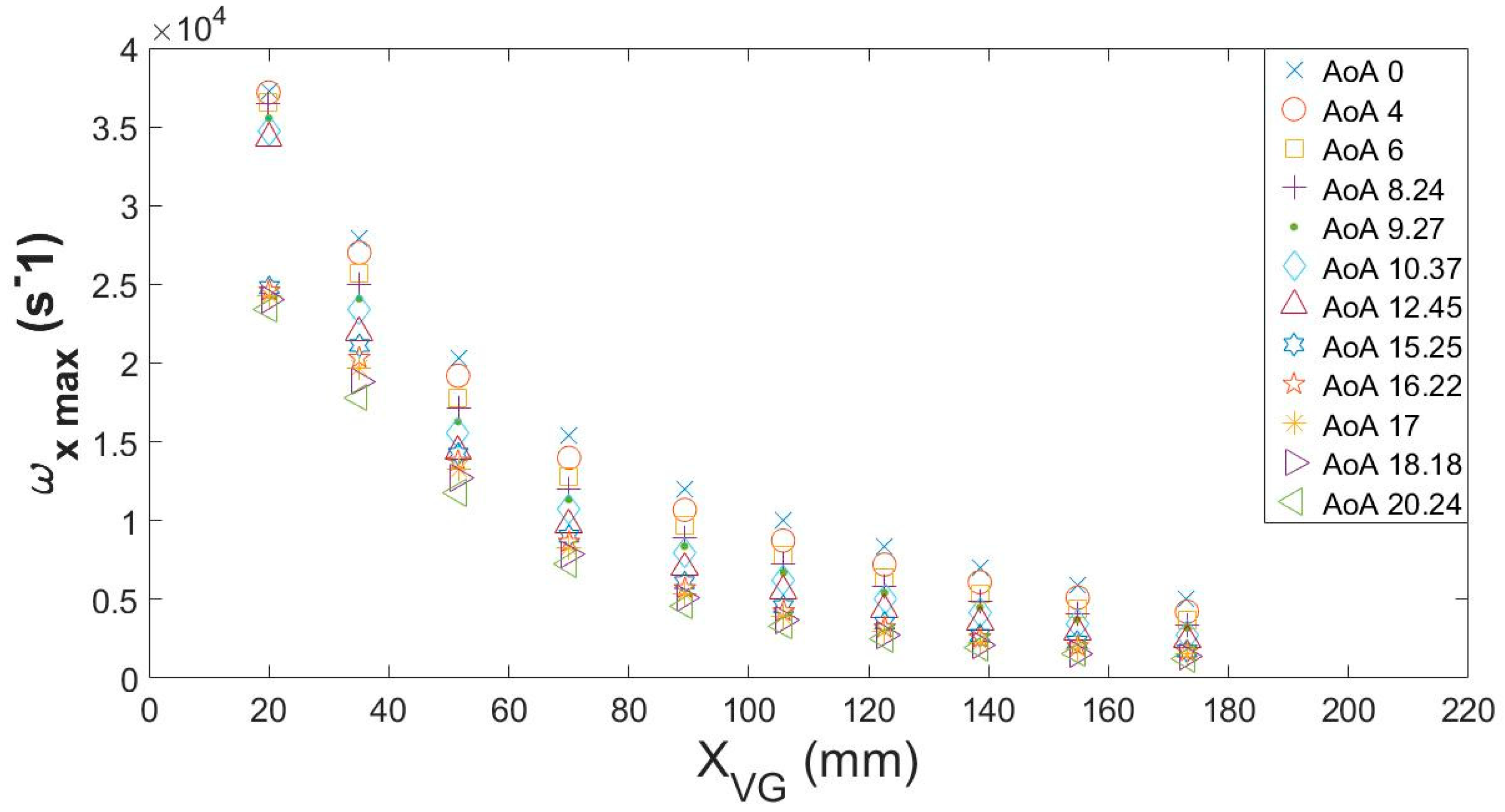
3.2. Vortex Trajectory and Decay
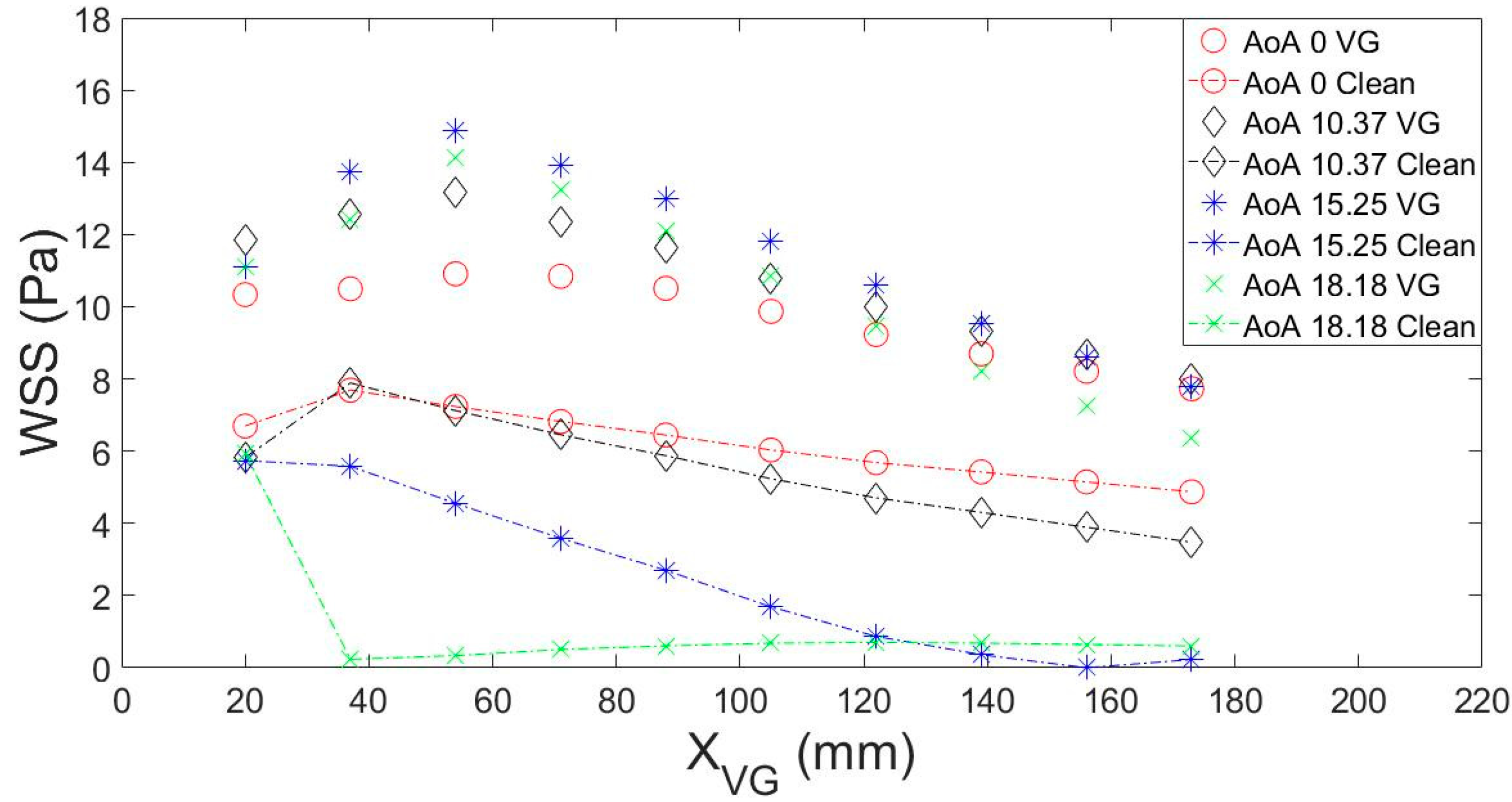
3.3. Wall Shear Stress
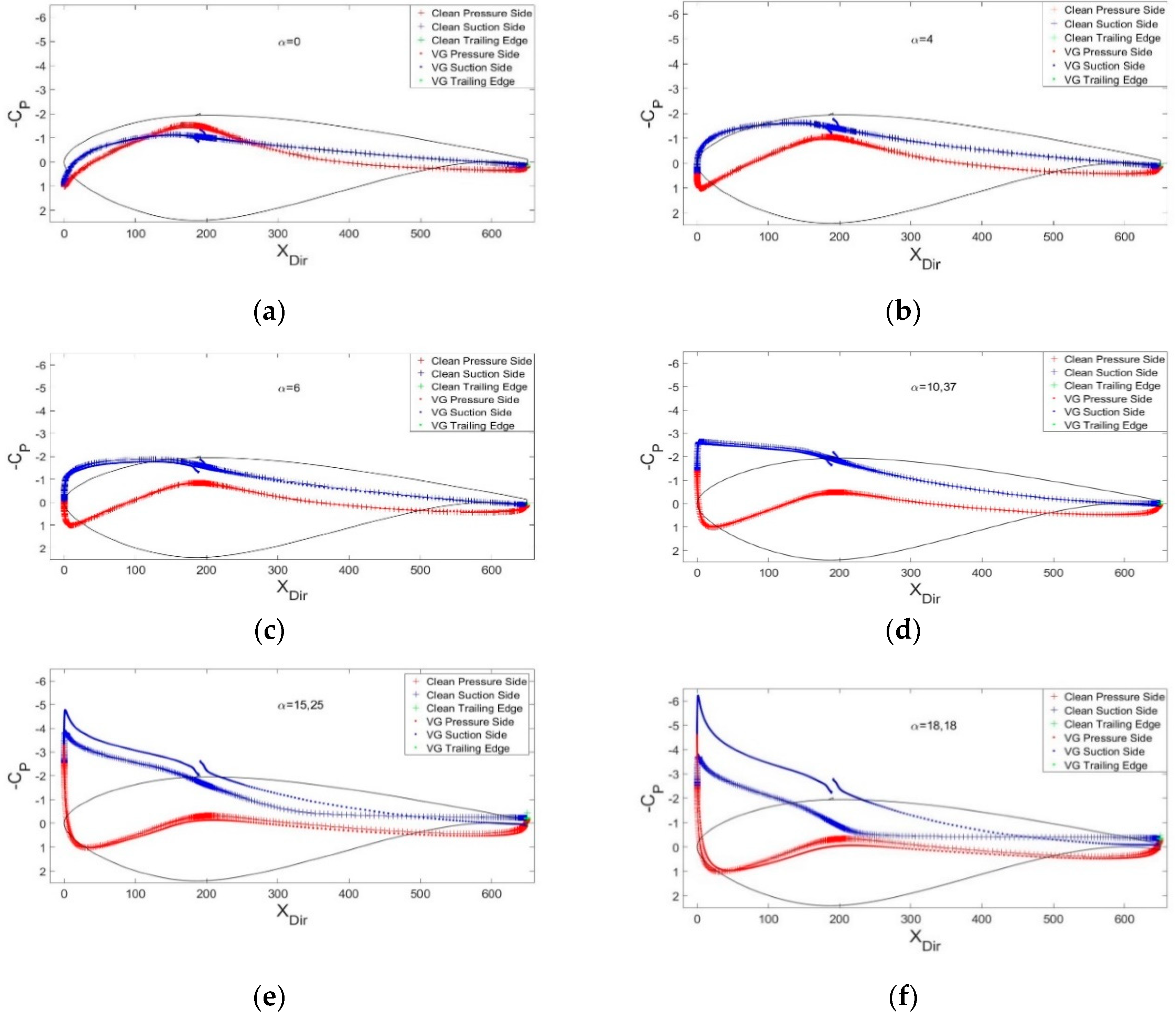
3.4. Pressure Coefficient Distribution
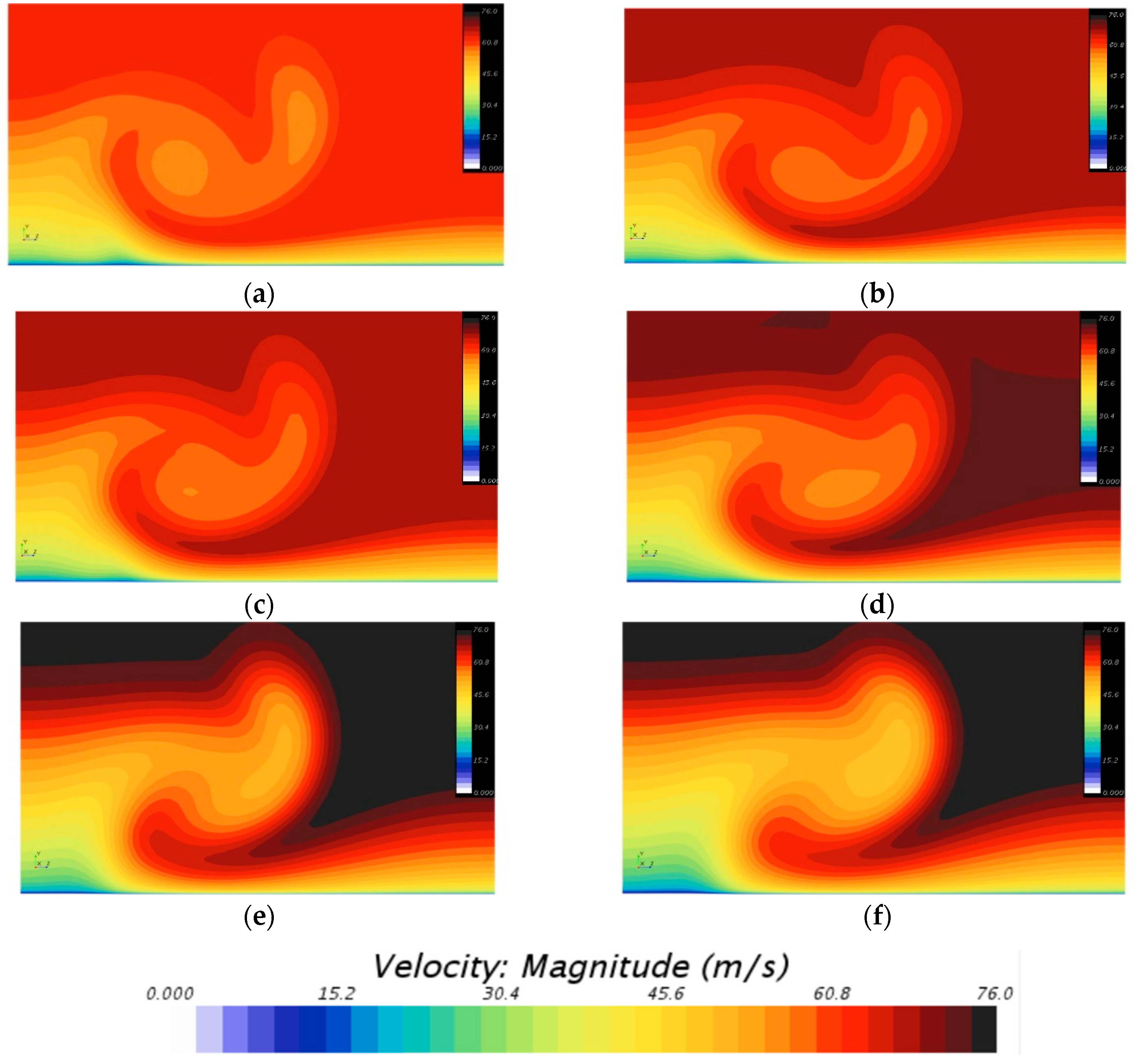
3.5. Vortex Vizualization
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| Definition | Unit | |
| CFD | Computational fluid dynamics | |
| DES | Detached eddy simulation | |
| VG | Vortex generator | |
| RANS | Reynolds-averaged Navier–Stokes | |
| SST | Shear stress transport | |
| β | Vane incident angle | deg |
| Δt | Time step | s |
| Δx | Cell size | m |
| λ | Distance between two devices in co-rotating VG configuration Distance between pairs in counter-rotating VG configuration | mm |
| Local density | kg/m3 | |
| ω x max | Peak vorticity | s−1 |
| AoA | Angle of attack | deg |
| c | Airfoil chord length | m |
| C | Courant number | |
| CD | Drag coefficient | |
| CL | Lift coefficient | |
| CP | Pressure coefficient | |
| Relaxation factor | ||
| h | Height of vortex generator | mm |
| l | Length of vortex generator | mm |
| L | Distance between two devices in trailing edge in counter-rotating configuration | mm |
| Re | Reynolds number | |
| Area of vortex generator | m2 | |
| Free stream velocity | m/s | |
| WSS | Wall shear stress | Pa |
| XVG | VG position from airfoil leading edge | mm |
References
- Aramendia, I.; Fernandez-Gamiz, U.; Antonio Ramos-Hernanz, J.; Sancho, J.; Manuel Lopez-Guede, J.; Zulueta, E. Flow Control Devices for Wind Turbines. Energy Harvest. Energy Effic. Technol. Methods Appl. 2017, 37, 629–655. [Google Scholar] [CrossRef]
- Houghton, T.; Bell, K.; Doquet, M. Offshore transmission for wind: Comparing the economic benefits of different offshore network configurations. Renew. Energy 2016, 94, 268–279. [Google Scholar] [CrossRef]
- Errasti, I.; Fernandez-Gamiz, U.; Martinez-Filgueira, P.; Blanco, J.M. Source term modelling of vane-type vortex generators under adverse pressure gradient in OpenFOAM. Energies 2019, 12, 605. [Google Scholar] [CrossRef]
- Baldacchino, D.; Manolesos, M.; Ferreira, C.; Salcedo, A.G.; Aparicio, M.; Chaviaropoulos, T.; Diakakis, K.; Florentie, L.; García, N.R.; Papadakis, G. Experimental benchmark and code validation for airfoils equipped with passive vortex generators. J. Phys. Conf. Ser. 2016, 753, 022002. [Google Scholar] [CrossRef]
- Fernandez-Gamiz, U.; Zulueta, E.; Boyano, A.; Ramos-Hernanz, J.A.; Manuel Lopez-Guede, J. Microtab Design and Implementation on a 5 MW Wind Turbine. Appl. Sci. 2017, 7, 536. [Google Scholar] [CrossRef]
- Martinez-Filgueira, P.; Fernandez-Gamiz, U.; Zulueta, E.; Errasti, I.; Fernandez-Gauna, B. Parametric study of low-profile vortex generators. Int. J. Hydrogen Energy 2017, 42, 17700–17712. [Google Scholar] [CrossRef]
- Johnson, S.J.; Baker, J.P.; van Dam, C.P.; Berg, D. An overview of active load control techniques for wind turbines with an emphasis on microtabs. Wind Energy 2010, 13, 239–253. [Google Scholar] [CrossRef]
- Van Rooij, R.; Timmer, W. Roughness sensitivity considerations for thick rotor blade airfoils. J. Sol. Energy Eng. 2003, 125, 468–478. [Google Scholar] [CrossRef]
- United Aircraft Corporation, Research Department; Taylor, H. Summary Report on Vortex Generators; United Aircraft Corporation, Research Department: East Hartford, CT, USA, 1950. [Google Scholar]
- Bragg, M.; Gregorek, G. Experimental study of airfoil performance with vortex generators. J. Aircr. 1987, 24, 305–309. [Google Scholar] [CrossRef]
- Schepers, G. Avatar: Advanced aerodynamic tools of large rotors. In Proceedings of the 33rd Wind Energy Symposium, Kissimmee, FL, USA, 5–9 January 2015; p. 0497. [Google Scholar]
- Zahle, F.; Tibaldi, C.; Verelst, D.R.; Bitche, R.; Bak, C. Aero-elastic optimization of a 10 MW wind turbine. In Proceedings of the 33rd Wind Energy Symposium, Kissimmee, FL, USA, 5–9 January 2015; p. 0491. [Google Scholar]
- Fernandez-Gamiz, U.; Errasti, I.; Gutierrez-Amo, R.; Boyano, A.; Barambones, O. Computational modelling of rectangular sub-boundary layer vortex generators. Appl. Sci. 2018, 8, 138. [Google Scholar] [CrossRef]
- Zaman, K.; Reeder, M.; Samimy, M. Control of an Axisymmetrical Jet using Vortex Generators. Phys. Fluids 1994, 6, 778–793. [Google Scholar] [CrossRef]
- Astolfi, D.; Castellani, F.; Terzi, L. Wind Turbine Power Curve Upgrades. Energies 2018, 11, 1300. [Google Scholar] [CrossRef]
- Terzi, L.; Lombardi, A.; Castellani, F.; Astolfi, D. Innovative methods for wind turbine power curve upgrade assessment. In Proceedings of the Windeurope Conference 2018 within the Global Wind Summit, Hamburg, Germany, 25–28 September 2018; Volume 1102. [Google Scholar] [CrossRef]
- Florentie, L.; Hulshoff, S.J.; van Zuijlen, A.H. Adjoint-based optimization of a source-term representation of vortex generators. Comput. Fluids 2018, 162, 139–151. [Google Scholar] [CrossRef]
- Øye, S. The effect of vortex generators on the performance of the ELKRAFT 1000 kw turbine. In Proceedings of the 9th IEA Symposium on Aerodynamics of Wind Turbines, Stockholm, Sweden, 11–12 December 1995. ISSN 0590-8809. [Google Scholar]
- Miller, G. Comparative performance tests on the Mod-2, 2.5-MW wind turbine with and without vortex generators. In Proceedings of the DOE/NASA Workshop on Horizontal Axis Wind Turbine Technology, Cleveland, OH, USA, 1 May 1995. [Google Scholar]
- Schubauer, G.B.; Spangenberg, W.G. Forced mixing in boundary layers. J. Fluid Mech. 1960, 8, 10–32. [Google Scholar] [CrossRef]
- Lin, J. Review of research on low-profile vortex generators to control boundary-layer separation. Prog. Aerospace Sci. 2002, 38, 389–420. [Google Scholar] [CrossRef]
- Gao, L.; Zhang, H.; Liu, Y.; Han, S. Effects of vortex generators on a blunt trailing-edge airfoil for wind turbines. Renew. Energy 2015, 76, 303–311. [Google Scholar] [CrossRef]
- Betterton, J.; Hackett, K.; Ashill, P.; Wilson, M.; Woodcock, I.; Tilman, C.; Langan, K. Laser doppler anemometry investigation on sub boundary layer vortex generators for flow control. In Proceedings of the 10th Symposium on Application of Laser Techniques to Fluid Mechanics, Lisbon, Portugal, 10–13 July 2000; pp. 10–12. [Google Scholar]
- Godard, G.; Stanislas, M. Control of a decelerating boundary layer. Part 1: Optimization of passive vortex generators. Aerospace Sci. Technol. 2006, 10, 181–191. [Google Scholar] [CrossRef]
- Jirasek, A. Vortex-generator model and its application to flow control. J. Aircr. 2005, 42, 1486–1491. [Google Scholar] [CrossRef]
- Bray, T.P. A Parametric Study of Vane and Air-Jet Vortex Generators. Ph.D. Thesis, College of Aeronautics, Cranfield University, Bedford, UK, 1998. [Google Scholar]
- Bur, R.; Coponet, D.; Carpels, Y. Separation control by vortex generator devices in a transonic channel flow. Shock Waves 2009, 19, 521. [Google Scholar] [CrossRef]
- Gad-elHak, M.; Bushnell, D. Separation Control—Review. J. Fluids Eng.-Trans. ASME 1991, 113, 5–30. [Google Scholar] [CrossRef]
- Zhang, L.; Li, X.; Yang, K.; Xue, D. Effects of vortex generators on aerodynamic performance of thick wind turbine airfoils. J. Wind Eng. Ind. Aerodyn 2016, 156, 84–92. [Google Scholar] [CrossRef]
- Hansen, M.O.L.; Charalampous, A.; Foucaut, J.; Cuvier, C.; Velte, C.M. Validation of a Model for Estimating the Strength of a Vortex Created from the Bound Circulation of a Vortex Generator. Energies 2019, 12, 2781. [Google Scholar] [CrossRef]
- Fernandez-Gamiz, U.; Marika Velte, C.; Réthoré, P.; Sørensen, N.N.; Egusquiza, E. Testing of self-similarity and helical symmetry in vortex generator flow simulations. Wind Energy 2016, 19, 1043–1052. [Google Scholar] [CrossRef]
- Bender, E.; Anderson, B.; Yagle, P. Vortex generator modeling for Navier-Stokes codes. In Proceedings of the Third ASME/JSME Joint Fluids Engineering Conference, California, USA, 18–23 July 1999; pp. FEDSM99–FEDSM6919. [Google Scholar]
- Dudek, J.C. Modeling vortex generators in a Navier-Stokes code. AIAA J. 2011, 49, 748–759. [Google Scholar] [CrossRef]
- Jonkman, J.; Butterfield, S.; Musial, W.; Scott, G. Definition of A 5-MW Reference Wind Turbine for Offshore System Development, Technical Report; National Renewable Energy Laboratory (U.S.): Golden, CO, USA, 2009. [CrossRef]
- Siemens STAR CCM+ Version 13.02.011. Available online: http://mdx.plm.automation.siemens.com/ (accessed on 9 April 2020).
- Fernandez, U.; Réthoré, P.-E.; Sørensen, N.N.; Velte, C.M.; Zahle, F.; Egusquiza, E. Comparison of four different models of vortex generators. In Proceedings of the EWEA 2012—European Wind Energy Conference & Exhibition European Wind Energy Association (EWEA), Copenhagen, Denmark, 16–19 March 2012; pp. 1–15. [Google Scholar]
- Menter, F. Zonal two equation kw turbulence models for aerodynamic flows. In Proceedings of the 23rd Fluid Dynamics, Plasmadynamics, and Lasers Conference, Orlando, FL, USA, 6–9 July 1993; p. 2906. [Google Scholar]
- Zhong, W.; Tang, H.; Wang, T.; Zhu, C. Accurate RANS Simulation of Wind Turbine Stall by Turbulence Coefficient Calibration. Appl. Sci. 2018, 8, 1444. [Google Scholar] [CrossRef]
- Meana-Fernandez, A.; Fernandez Oro, J.M.; Arguelles Diaz, K.M.; Velarde-Suarez, S. Turbulence-Model Comparison for Aerodynamic-Performance Prediction of a Typical Vertical-Axis Wind-Turbine Airfoil. Energies 2019, 12, 488. [Google Scholar] [CrossRef]
- Guo, C.; Guo, H.; Hu, J.; Song, K.; Zhang, W.; Wang, W. Large Eddy Simulation of Flow over Wavy Cylinders with Different Twisted Angles at a Subcritical Reynolds Number. J. Mar. Sci. Eng. 2019, 7, 227. [Google Scholar] [CrossRef]
- Sorensen, N.N.; Zahle, F.; Bak, C.; Vronsky, T. Prediction of the Effect of Vortex Generators on Airfoil Performance. In Science of Making Torque from Wind 2014 (Torque 2014); Journal of Physics: Conference Series; IOP Publishing: Copenhagen, Denmark, 2014; Volume 524. [Google Scholar] [CrossRef]
- Fernandez-Gamiz, U.; Zamorano, G.; Zulueta, E. Computational study of the vortex path variation with the VG height. In Science of Making Torque from Wind 2014 (Torque 2014); IOP Publishing: Copenhagen, Denmark, 2014; Volume 524. [Google Scholar] [CrossRef]
- Li, S.; Zhang, L.; Yang, K.; Xu, J.; Li, X. Aerodynamic Performance of Wind Turbine Airfoil DU 91-W2-250 under Dynamic Stall. Appl. Sci. 2018, 8, 1111. [Google Scholar] [CrossRef]
- Raffel, M.; Favier, D.; Berton, E.; Rondot, C.; Nsimba, M.; Geissler, W. Micro-PIV and ELDV wind tunnel investigations of the laminar separation bubble above a helicopter blade tip. Meas. Sci. Technol. 2006, 17, 1652–1658. [Google Scholar] [CrossRef]
- Zhen, T.K.; Zubair, M.; Ahmad, K.A. Experimental and Numerical Investigation of the Effects of Passive Vortex Generators on Aludra UAV Performance. Chin. J. Aeronaut. 2011, 24, 577–583. [Google Scholar] [CrossRef]
- Ibarra-Udaeta, I.; Errasti, I.; Fernandez-Gamiz, U.; Zulueta, E.; Sancho, J. Computational Characterization of a Rectangular Vortex Generator on a Flat Plate for Different Vane Heights and Angles. Appl. Sci. 2019, 9, 995. [Google Scholar] [CrossRef]
- Urkiola, A.; Fernandez-Gamiz, U.; Errasti, I.; Zulueta, E. Computational characterization of the vortex generated by a Vortex Generator on a flat plate for different vane angles. Aerospace Sci. Technol. 2017, 65, 18–25. [Google Scholar] [CrossRef]



| h [mm] | d [mm] | a [mm] | b [mm] | β [°] |
|---|---|---|---|---|
| 5 | 17 | 10 | 20 | 18 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chillon, S.; Uriarte-Uriarte, A.; Aramendia, I.; Martínez-Filgueira, P.; Fernandez-Gamiz, U.; Ibarra-Udaeta, I. jBAY Modeling of Vane-Type Vortex Generators and Study on Airfoil Aerodynamic Performance. Energies 2020, 13, 2423. https://doi.org/10.3390/en13102423
Chillon S, Uriarte-Uriarte A, Aramendia I, Martínez-Filgueira P, Fernandez-Gamiz U, Ibarra-Udaeta I. jBAY Modeling of Vane-Type Vortex Generators and Study on Airfoil Aerodynamic Performance. Energies. 2020; 13(10):2423. https://doi.org/10.3390/en13102423
Chicago/Turabian StyleChillon, Sergio, Antxon Uriarte-Uriarte, Iñigo Aramendia, Pablo Martínez-Filgueira, Unai Fernandez-Gamiz, and Iosu Ibarra-Udaeta. 2020. "jBAY Modeling of Vane-Type Vortex Generators and Study on Airfoil Aerodynamic Performance" Energies 13, no. 10: 2423. https://doi.org/10.3390/en13102423
APA StyleChillon, S., Uriarte-Uriarte, A., Aramendia, I., Martínez-Filgueira, P., Fernandez-Gamiz, U., & Ibarra-Udaeta, I. (2020). jBAY Modeling of Vane-Type Vortex Generators and Study on Airfoil Aerodynamic Performance. Energies, 13(10), 2423. https://doi.org/10.3390/en13102423