Metal Hydride Beds-Phase Change Materials: Dual Mode Thermal Energy Storage for Medium-High Temperature Industrial Waste Heat Recovery
Abstract
1. Introduction
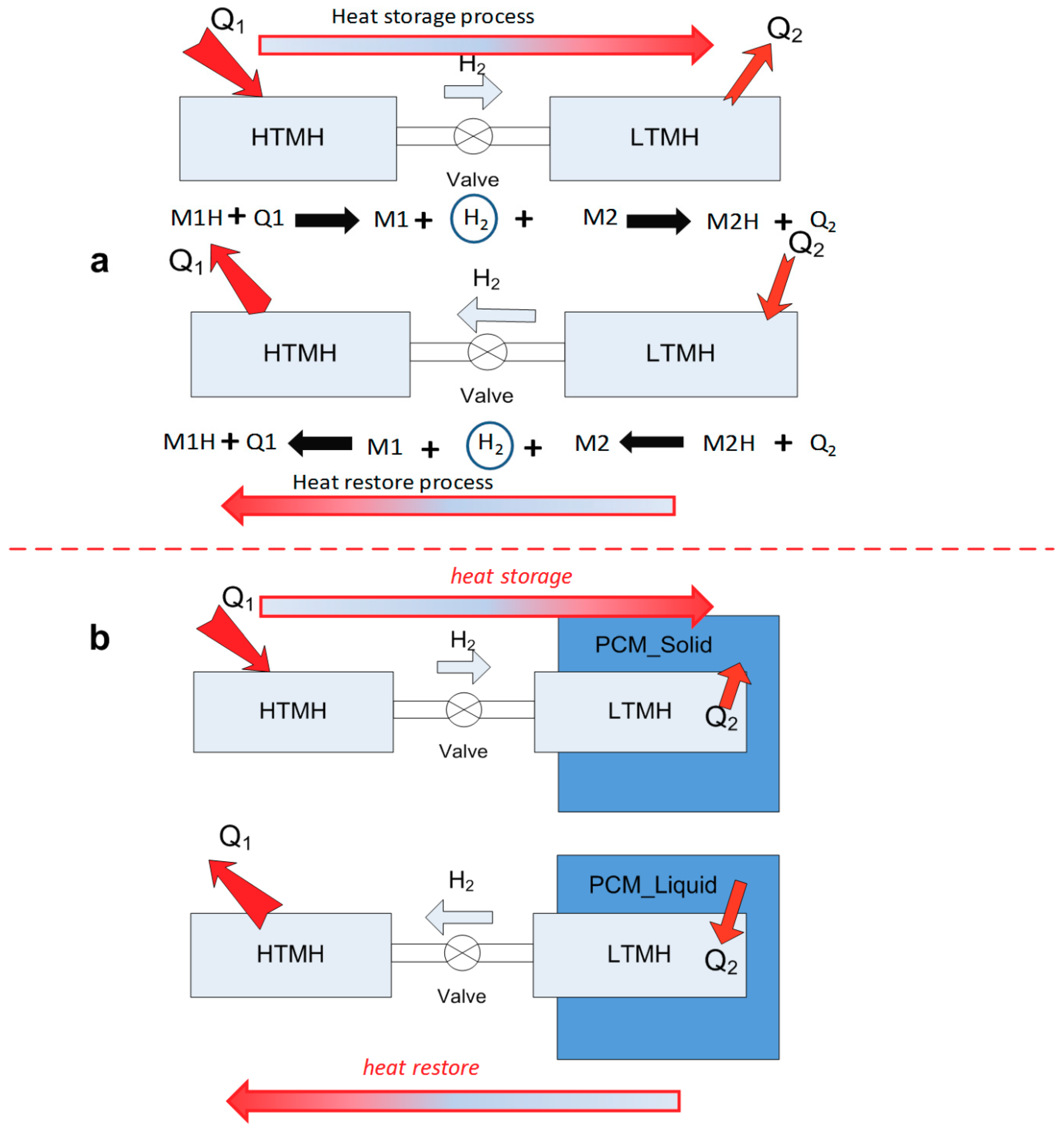
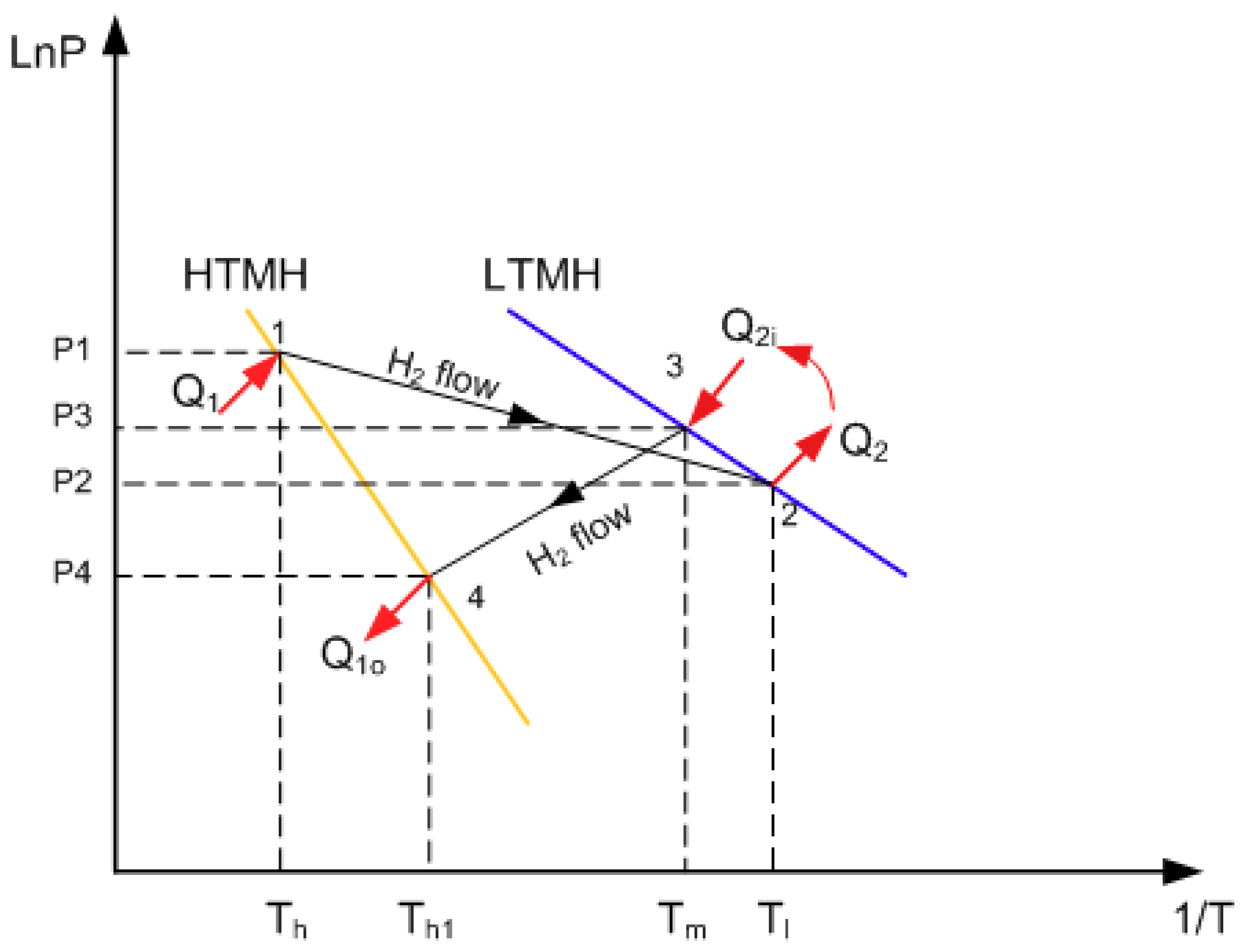
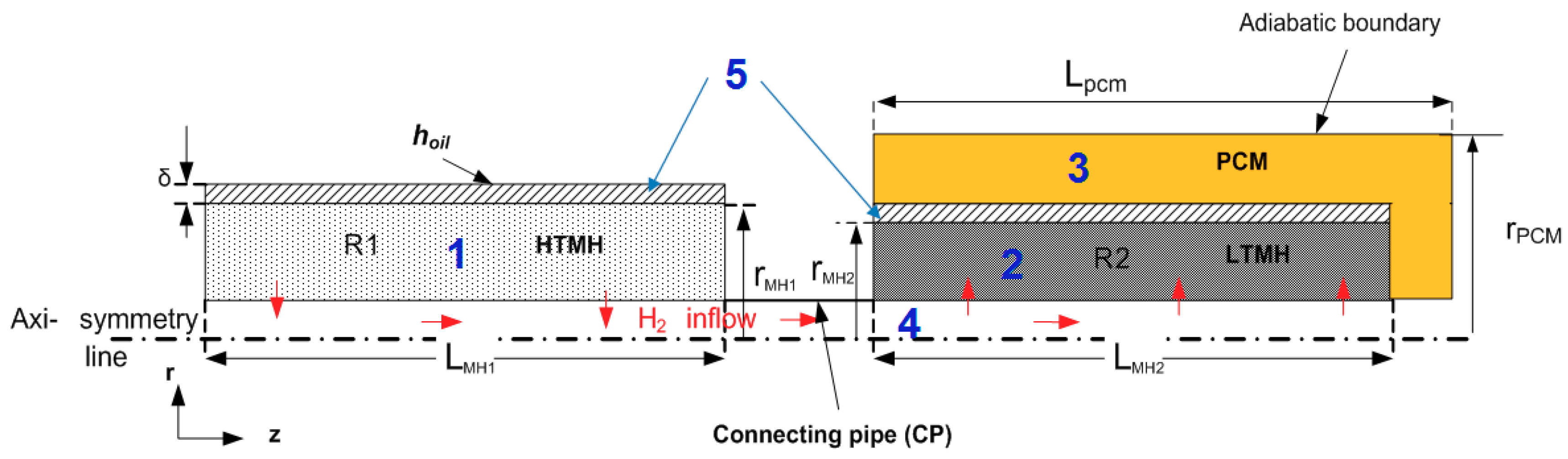
2. Problem Description
3. Mathematical Model
3.1. Governing Equations
3.1.1. Metal Hydride Reactors
- The thermo-physical properties of hydrides are temperature and concentration-independent.
- The thermal equilibrium between the gas and solid is established.
- The thermal loss due to the radiative heat transfer is neglected for the case of Mg hydride materials
- The hydrogen gas pressure inside the reaction bed is constant.
- The equilibrium pressure in the absorption and desorption processes is unchanged i.e., the hysteresis is negligible.
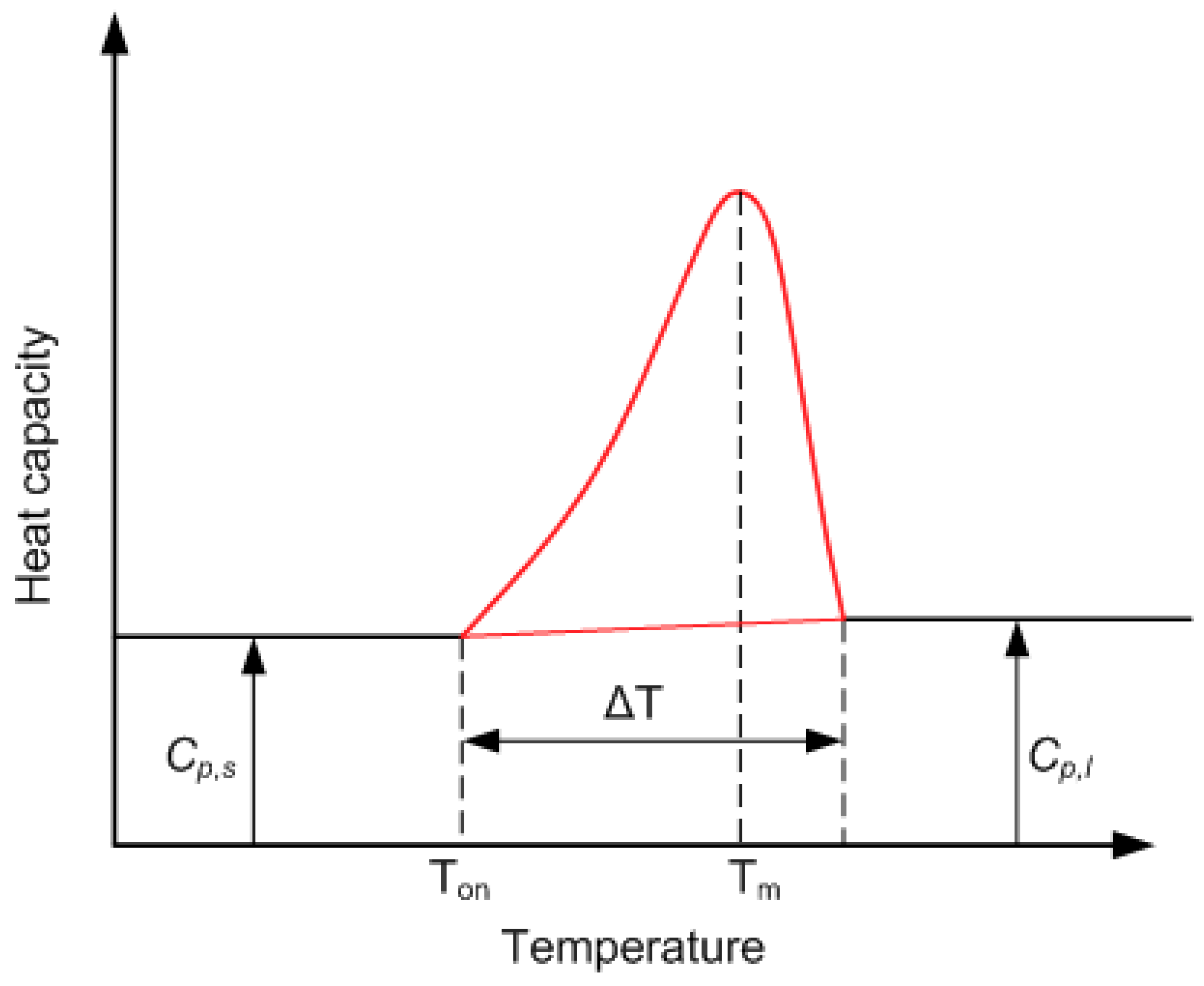
3.1.2. Phase Change Materials
- The thermo-physical properties (density, solid-liquid specific heat, thermal conductivity) of the PCM are assumed constant.
- The latent heat of phase change is temperature-independent.
- The natural convection is disregarded.
- metal hydride-reactor wall (domains 1-5, 2-5),
- reactor wall-heat transfer fluid,
- reactor wall-phase change material (domains 5-3)
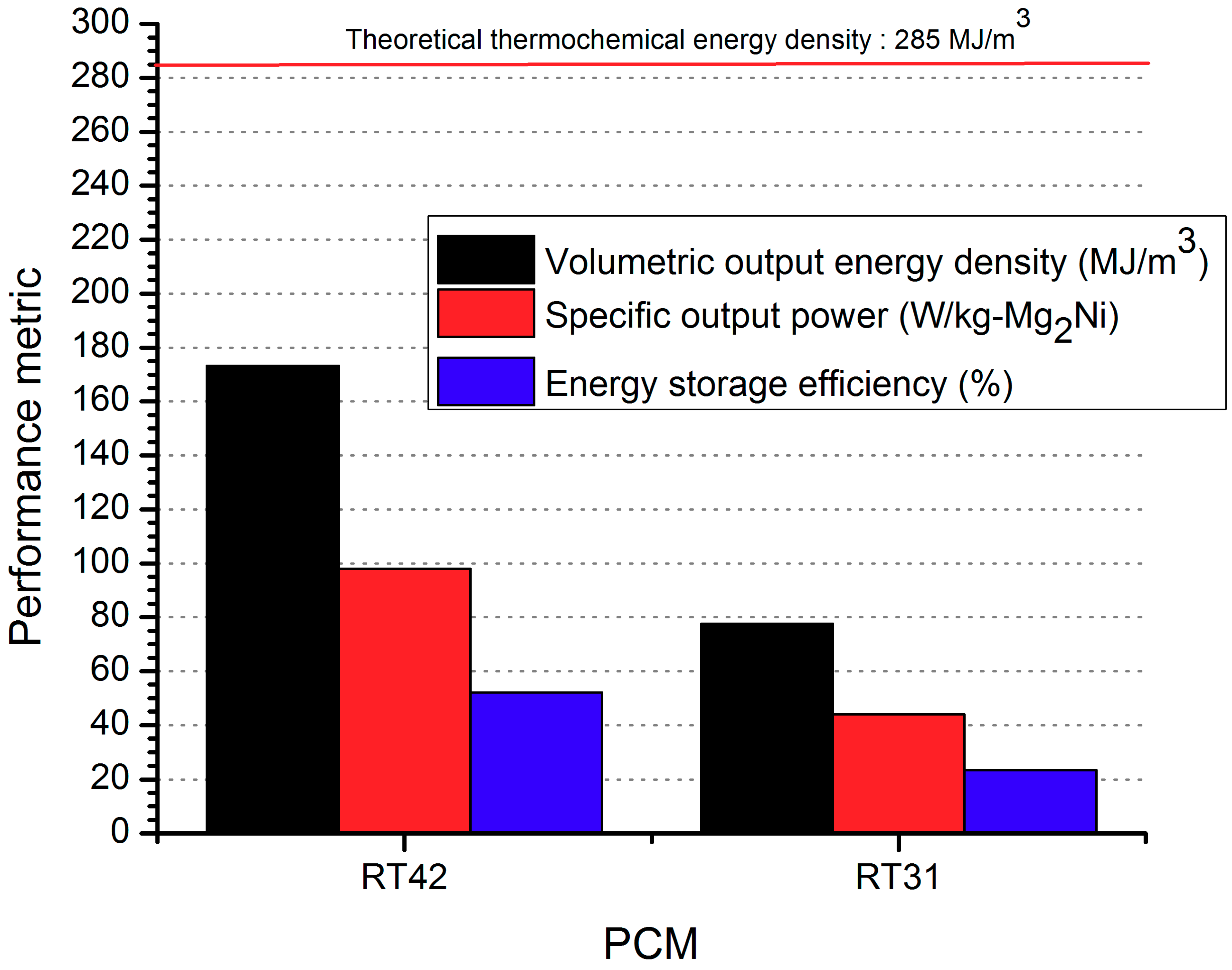
3.2. Performance Indicators of the Heat Storage System
- The volumetric energy storage density:
- The specific power during the heat-discharging step:
- The energy storage efficiency:where Vs is the total volume of the heat storage components , tc/d is the charging/discharging time, and mHTMH is the mass of the high temperature metal hydride.
4. Results and Discussion
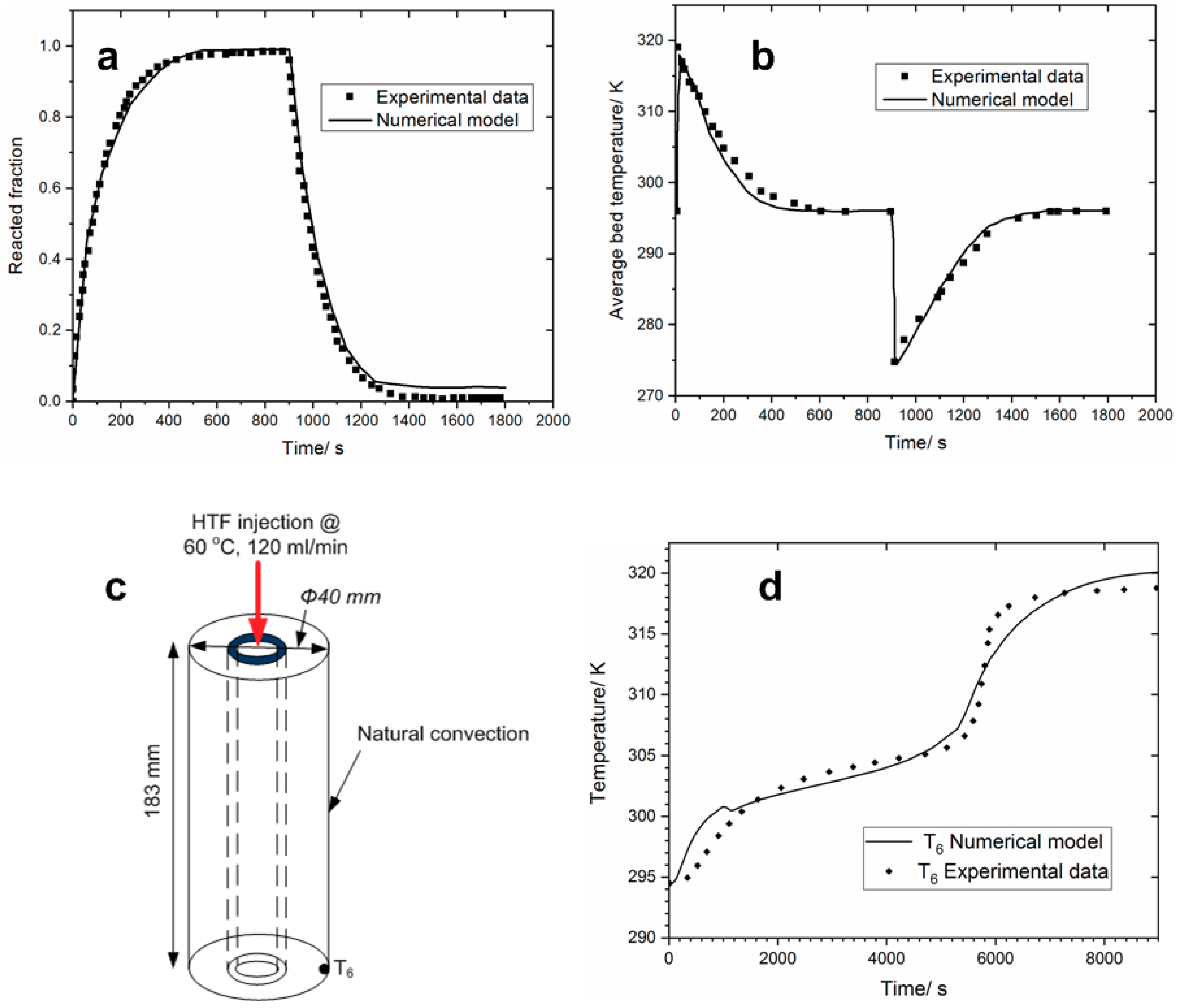
4.1. Model Validation
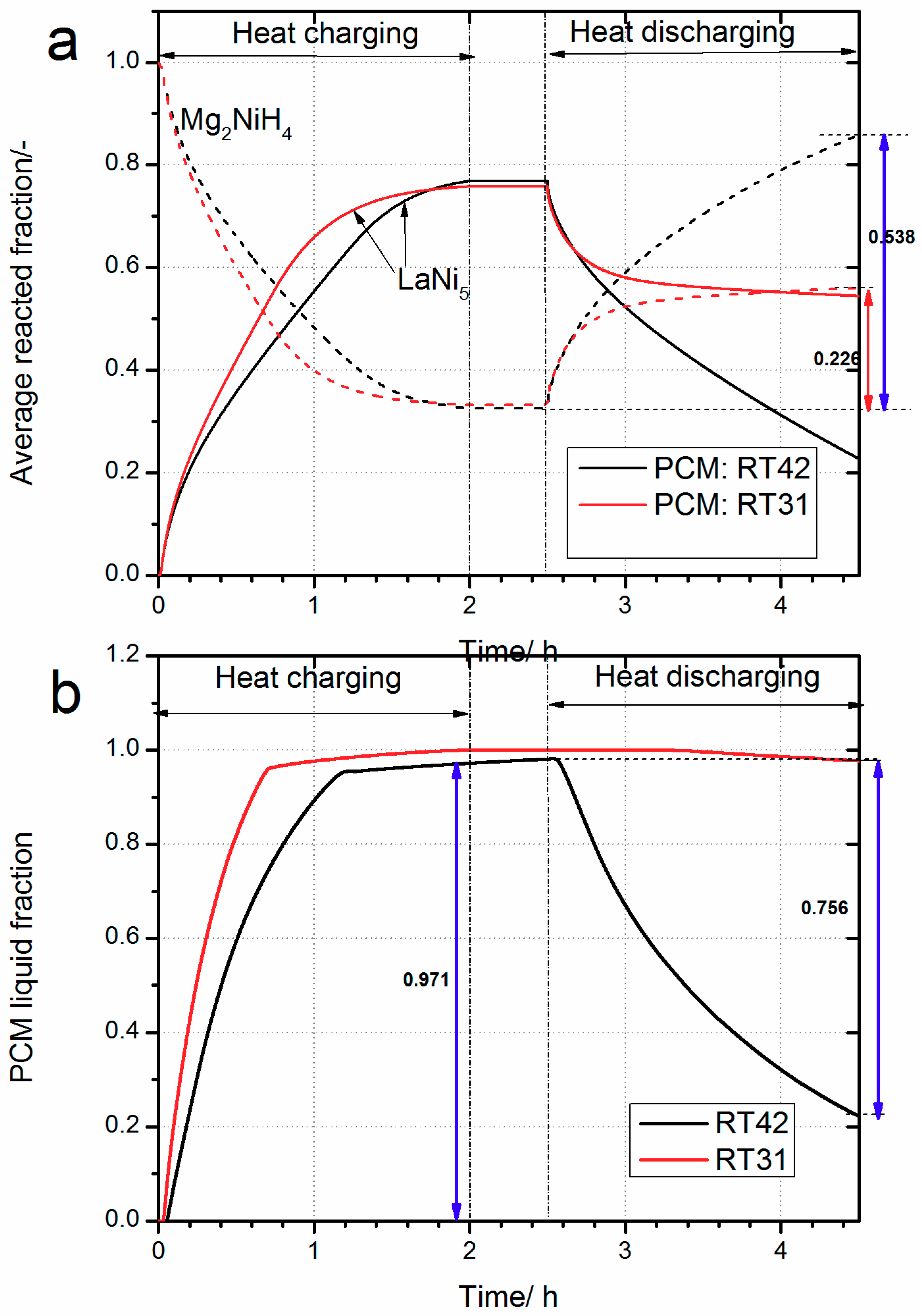
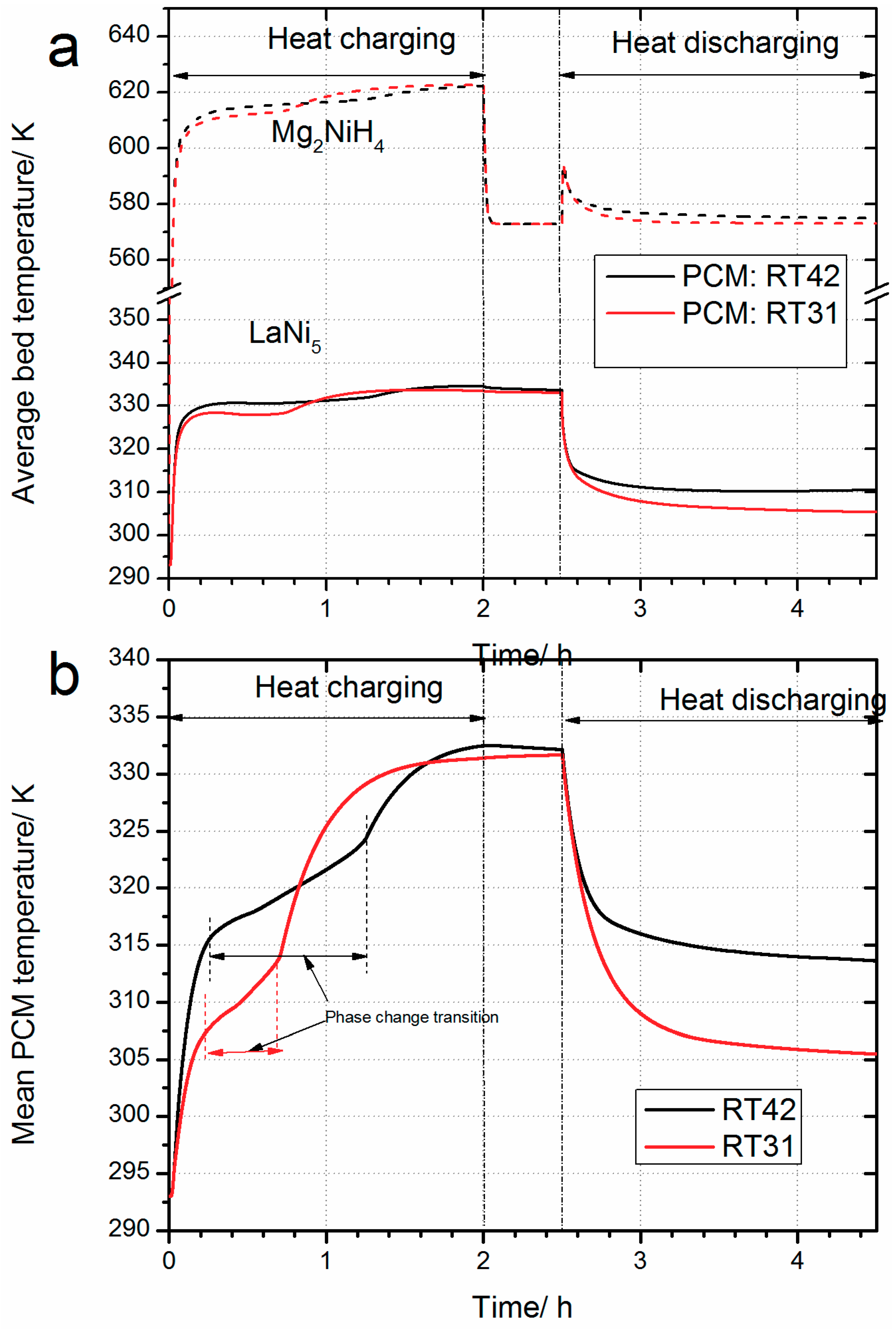
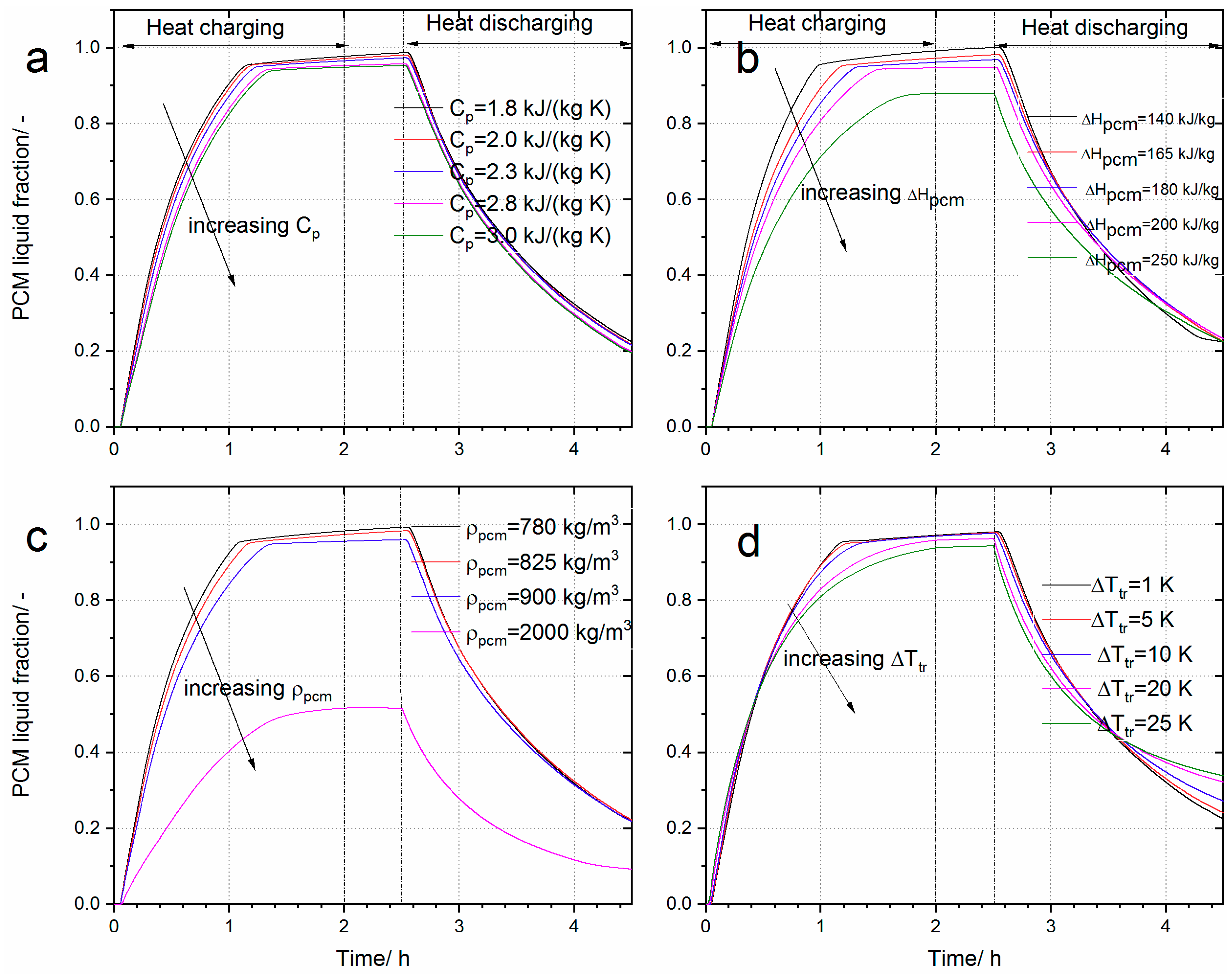
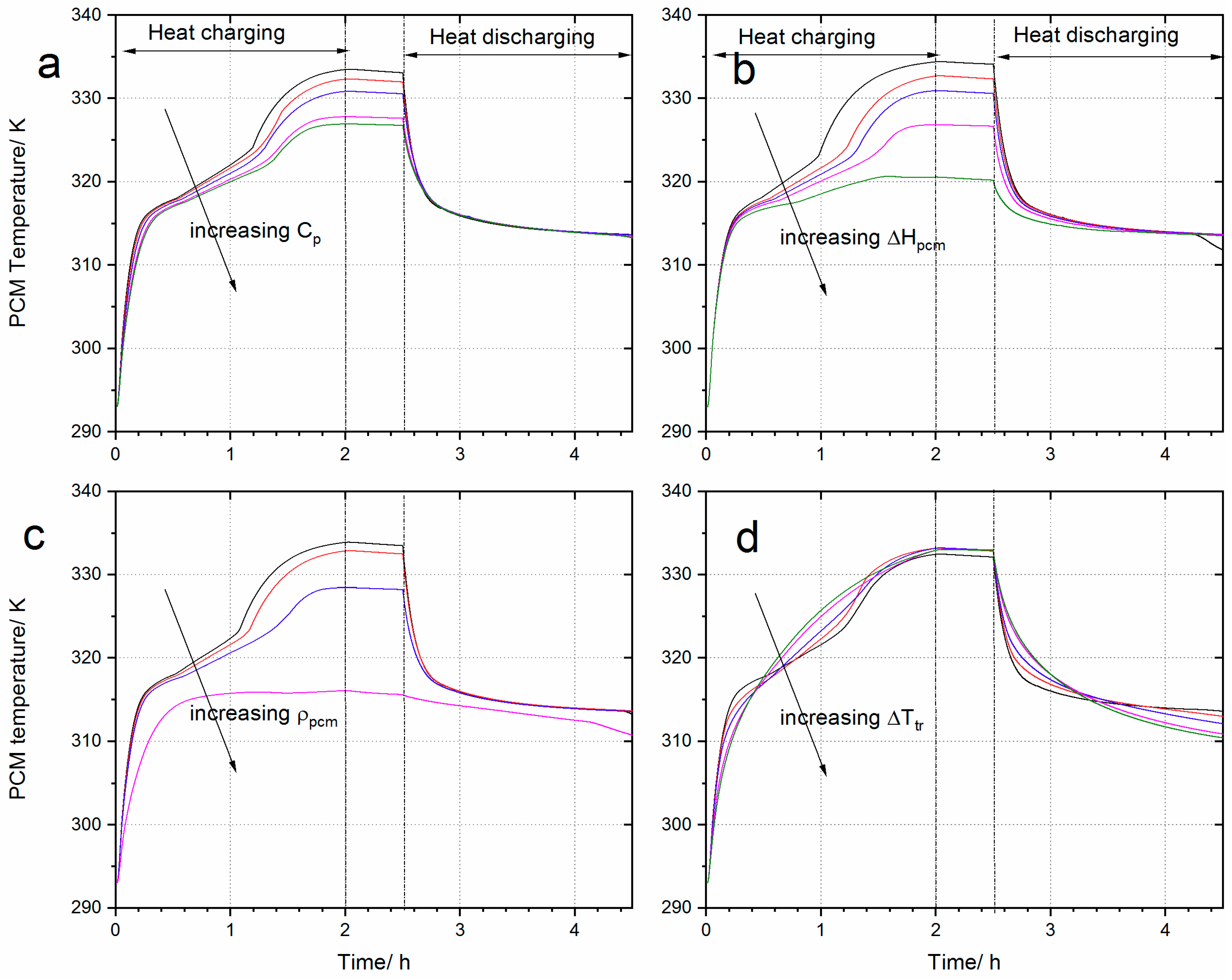
4.2. Effect of PCM Thermo-Physical Properties on the Thermal Energy Storage System
4.2.1. The Effect of the PCM Melting Temperature Tm
4.2.2. The Effect of Cp, ∆Ttr, ∆Hpcm and ρpcm on the Performance of the Heat Storage System
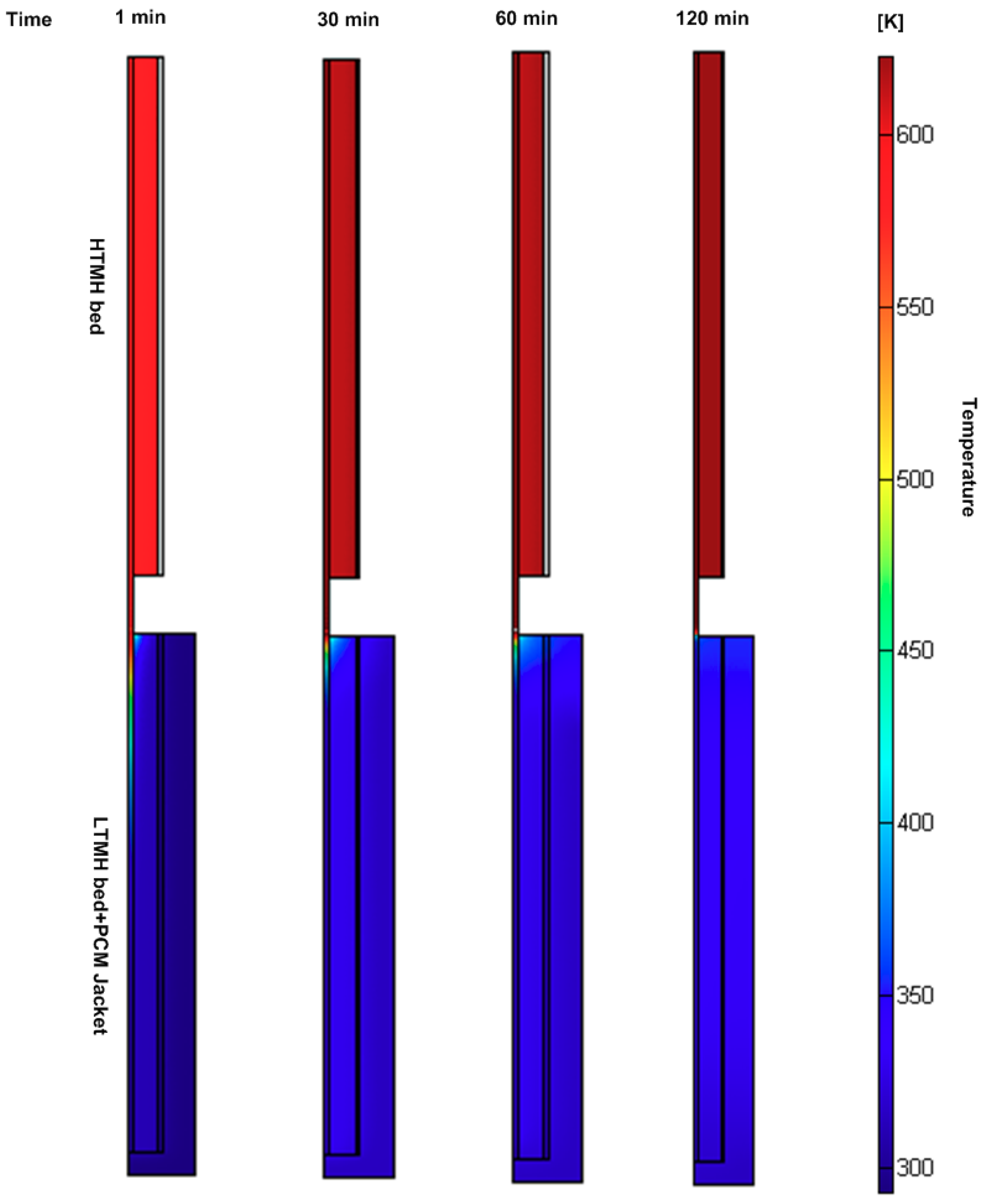
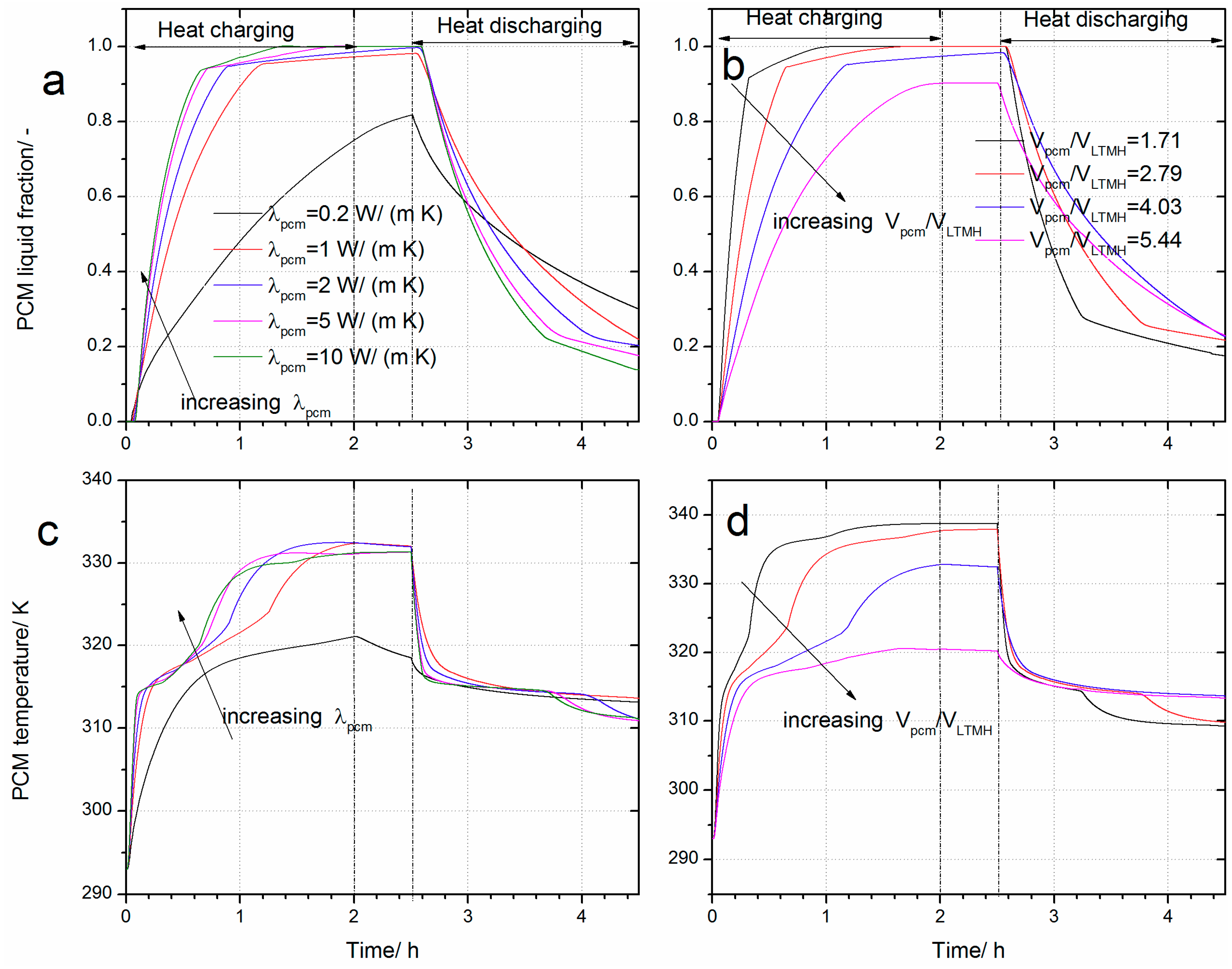
4.3. The Effect of Design Parameters
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| A | Surface area (m2) |
| C | Heat capacity (J·mol−1∙K−1) |
| E | Activation energy (J·mol−1) |
| f | pressure-dependent function, PCM melting fraction |
| g | conversion fraction-dependent function |
| hoil | convective heat transfer coefficient (W·m−2· K−1) |
| HTF | heat transfer fluid |
| HTMH | high temperature metal hydride |
| ΔH | heat of reaction (J·mol−1) |
| k | rate constant (s−1) |
| L | reactor length (m) |
| LTMH | low temperature metal hydride |
| M | molecular weight (g·mol−1) |
| p | pressure (bar) |
| PCM | phase change material |
| Q | heat flux (J) |
| r | radius (m) |
| R | universal gas constant (8.314 J·mol−1·K−1) |
| ΔS | entropy of reaction (J·mol−1·K−1) |
| S | heat transfer surface (m2) |
| t | time (s) |
| ΔT | temperature range of phase transition |
| V | velocity (m·s−1), reactor volume (m3) |
| wt | hydrogen storage capacity (%) |
| Greek letters | |
| α | conversion fraction |
| ε | porosity |
| thermal inertia | |
| material density (kg·m−3) | |
| η | energy storage efficiency |
| λ | thermal conductivity (W·m−1·K−1) |
| μ | dynamic viscosity (Pa·s) |
| Subscripts | |
| a | absorption |
| c | charging |
| d | desorption, discharging |
| eff | effective |
| eq | equilibrium |
| i | inlet |
| f | fluid |
| g | gas |
| l | liquid |
| m | melting |
| MH | metal hydride |
| mix | mixture |
| o | outlet |
| on | onset |
| s | system, solid |
| tr | transition |
| w | wall |
References
- Taymaz, I. An experimental study of energy balance in low heat rejection diesel engine. Energy 2006, 31, 364–371. [Google Scholar] [CrossRef]
- Chan, C.W.; Ling-Chin, J.; Roskilly, A.P. A review of chemical heat pumps, thermodynamic cycles and thermal energy storage technologies for low grade heat utilization. Appl. Therm. Eng. 2013, 50, 1257–1273. [Google Scholar] [CrossRef]
- Kwak, D.H.; Binns, M.; Kim, J.K. Integrated design and optimization of technologies for utilizing low grade heat in process industries. Appl. Energy 2014, 131, 307–322. [Google Scholar] [CrossRef]
- Poudel, B.; Hao, Q.; Ma, Y.; Lan, Y.C.; Minnich, A.; Yu, B.; Yan, X.; Wang, D.; Muto, A.; Vashaee, D.; et al. High-thermoelectric performance of nanostructured bismuth antimony telluride bulk alloys. Science 2008, 320, 634–638. [Google Scholar] [CrossRef] [PubMed]
- Singh, D.V.; Pedersen, E. A review of waste heat recovery technologies for maritime applications. Energy Convers Manag. 2016, 111, 315–328. [Google Scholar] [CrossRef]
- Wang, T.; Luan, W.; Wang, W.; Tu, S.T. Waste heat recovery through plate heat exchanger based thermoelectric generator system. Appl. Energy 2014, 136, 860–865. [Google Scholar] [CrossRef]
- Gou, X.; Xiao, H.; Yang, S. Modeling experimental study and optimization on low temperature waste heat thermoelectric generator system. Appl. Energy 2010, 87, 3131–3136. [Google Scholar] [CrossRef]
- Yamankaradeniz, N.; Bademlioglu, A.H.; Kaynakli, O. Performance assessments of an organic Rankine cycle with internal heat exchanger based on exergetic approach. J. Energy Resour. Technol. 2018, 140, 102001–102008. [Google Scholar] [CrossRef]
- Bademlioglu, A.H.; Canbolat, A.S.; Yamankaradeniz, N.; Kaynakli, O. Investigation of parameters affecting Organic Rankine Cycle efficiency by using Taguchi and ANOVA methods. Appl. Therm. Eng. 2018, 145, 221–228. [Google Scholar] [CrossRef]
- Song, J.; Song, Y.; Gu, C.W. Thermodynamic analysis and performance optimization of an Organic Rankine Cycle (ORC) waste heat recovery system for marine diesel engines. Energy 2015, 82, 976–985. [Google Scholar] [CrossRef]
- Larsen, U.; Pierobon, L.; Haglind, F.; Gabrielii, C. Design and optimisation of organic Rankine cycles for waste heat recovery in marine applications using the principles of natural selection. Energy 2013, 55, 803–812. [Google Scholar] [CrossRef]
- Miró, L.; Gasia, J.; Cabeza, L.F. Thermal energy storage (TES) for industrial waste heat (IWH) recovery: A review. Appl. Energy 2016, 179, 284–301. [Google Scholar] [CrossRef]
- Magro, F.D.; Jimenez-Arreola, M.; Romagnoli, A. Improving energy recovery efficiency by retrofitting a PCM-based technology to an ORC system operating under thermal power fluctuations. Appl. Energy 2017, 208, 972–985. [Google Scholar] [CrossRef]
- Yu, X.; Li, Z.; Lu, Y.; Huang, R.; Roskilly, A.P. Investigation of organic Rankine cycle integrated with double latent thermal energy storage for engine waste heat recovery. Energy 2019, 170, 1098–1112. [Google Scholar] [CrossRef]
- Pandiyarajan, V.; Chinnappandian, M.; Raghavan, V.; Velraj, R. Second law analysis of a diesel engine waste heat recovery with a combined sensible and latent heat storage system. Energy Policy 2011, 39, 6011–6020. [Google Scholar] [CrossRef]
- Ortega-Fernández, I.; Rodríguez-Aseguinolaza, J. Thermal energy storage for waste heat recovery in the steelworks: The case study of the REslag project. Appl. Energy 2019, 237, 708–719. [Google Scholar] [CrossRef]
- Gopal, K.N.; Subbarao, R.; Pandiyarajan, V.; Velraj, R. Thermodynamic analysis of a diesel engine integrated with a PCM based energy storage system. Int. J. Thermodyn. 2010, 13, 15–21. [Google Scholar]
- Wang, W.; Guo, S.; Li, H.; Yan, J.; Zhao, J.; Li, X.; Ding, J. Experimental study on the direct/indirect contact energy storage container in mobilized thermal energy system (M-TES). Appl. Energy 2014, 119, 181–189. [Google Scholar] [CrossRef]
- Guo, S.P.; Zhao, J.; Wang, W.L.; Yan, J.Y.; Jin, G.; Wang, X.T. Techno-economic assessment of mobilized thermal energy storage for distributed users: A case study in China. Appl. Energy 2017, 194, 481–486. [Google Scholar] [CrossRef]
- Li, H.; Wang, W.; Yan, J.; Dahlquist, E. Economic assessment of the mobilized thermal energy storage (M-TES) system for distributed heat supply. Appl. Energy 2013, 104, 178–186. [Google Scholar] [CrossRef]
- Lizana, J.; Chacartegui, R.; Barrios-Padura, A.; Valverde, J.M. Advances in thermal energy storage materials and their applications towards zero energy buildings: A critical review. Appl. Energy 2017, 203, 219–239. [Google Scholar] [CrossRef]
- Cui, H.T. Experimental investigation on the heat charging process by paraffin filled with high porosity copper foam. Appl. Therm. Eng. 2012, 39, 26–28. [Google Scholar] [CrossRef]
- Merlin, K.; Soto, J.; Delaunay, D.; Traonvouez, L. Industrial waste heat recovery using an enhanced conductivity latent heat thermal energy storage. Appl. Energy 2016, 183, 491–503. [Google Scholar] [CrossRef]
- Py, X.; Olives, R.; Mauran, S. Paraffin/porous-graphite-matrix composite as a high and constant power thermal storage material. Int. J. Heat Mass Transf. 2001, 44, 2727–2737. [Google Scholar] [CrossRef]
- Yang, X.; Lu, Z.; Bai, Q.; Zhang, Q.; Jin, L.; Yan, J. Thermal performance of a shell-and-tube latent heat thermal energy storage unit: Role of annular fins. Appl. Energy 2017, 202, 558–570. [Google Scholar] [CrossRef]
- Jouhara, H.; Almahmoud, S.; Chauhan, A.; Delpech, B.; Bianchi, G.; Tassou, S.A.; Llera, R.; Lago, F.; Arribas, J.J. Experimental and theoretical investigation of a flat heat pipe heat exchanger for waste heat recovery in the steel industry. Energy 2017, 141, 1928–1939. [Google Scholar] [CrossRef]
- Ma, H.; Yin, L.; Shen, X.; Lu, W.; Sun, Y.; Zhang, Y.; Deng, N. Experimental study on heat pipe assisted heat exchanger used for industrial waste heat recovery. Appl. Energy 2016, 169, 177–186. [Google Scholar] [CrossRef]
- Li, T.X.; Xu, J.X.; Yan, T.; Wang, R.Z. Development of sorption thermal battery for low-grade waste heat recovery and combined cold and heat energy storage. Energy 2016, 107, 347–359. [Google Scholar] [CrossRef]
- Verde, M.; Harby, K.; de Boer, R.; Corber, J.M. Performance evaluation of a waste-heat driven adsorption system for automotive air-conditioning: Part I: Modeling and experimental validation. Energy 2016, 116, 526–538. [Google Scholar] [CrossRef]
- Gao, P.; Wang, L.W.; Wang, R.Z.; Zhang, X.F.; Li, D.P.; Liang, Z.W.; Cai, A.F. Experimental investigation of a MnCl2/CaCl2-NH3 two-stage solid sorption freezing system for a refrigerated truck. Energy 2016, 103, 16–26. [Google Scholar] [CrossRef]
- Lu, Y.Z.; Wang, R.Z.; Jianzhou, S.; Xu, Y.X.; Wu, J.Y. Practical experiments on an adsorption air conditioner powered by the exhausted heat from a diesel locomotive. Appl. Therm. Eng. 2004, 24, 1051–1059. [Google Scholar] [CrossRef]
- Jiang, L.; Wang, L.W.; Wang, R.Z.; Zhu, F.Q.; Lu, Y.J.; Roskilly, A.P. Experimental investigation on an innovative resorption system for energy storage and upgrade. Energy Convers. Manag. 2017, 138, 651–658. [Google Scholar] [CrossRef]
- Jiang, L.; Roskilly, A.P.; Wang, R.Z.; Wang, L.W.; Lu, Y.J. Analysis on innovative modular sorption and resorption thermal cell for cold and heat cogeneration. Appl. Energy 2017, 204, 767–779. [Google Scholar] [CrossRef]
- Qin, F.; Chen, J.P.; Lu, M.Q.; Chen, Z.J.; Zhou, Y.M.; Yang, K. Development of a metal hydride refrigeration system as an exhaust gas-driven automobile air conditioner. Renew. Energy 2007, 32, 2034–2052. [Google Scholar] [CrossRef]
- Yang, F.S.; Wang, G.X.; Zhang, Z.X.; Rudolph, V. Investigation on the influences of heat transfer enhancement measures in a thermally driven metal hydride heat pump. Int. J. Hydrogen Energy 2010, 35, 9725–9735. [Google Scholar] [CrossRef]
- Klein, H.P.; Groll, M. Development of a two-stage metal hydride system as topping cycle in cascading sorption systems for cold generation. Appl. Therm. Eng. 2002, 22, 631–639. [Google Scholar] [CrossRef]
- Isselhorst, A.; Groll, M. Two-stage metal hydride heat transformer laboratory model. J. Alloy. Comp. 1995, 231, 888–894. [Google Scholar] [CrossRef]
- Bogdanovic, B.; Ritter, A.; Spliethoff, B. A process steam generator based on the high temperature magnesium hydride/magnesium heat storage system. Int. J. Hydrogen Energy 1995, 20, 811–822. [Google Scholar] [CrossRef]
- Reiser, A.; Bogdanovic, B.; Schlichte, K. The application of Mg-based metal hydrides as heat energy storage systems. Int. J. Hydrogen Energy 2000, 25, 425–430. [Google Scholar] [CrossRef]
- Nyallang, S.N.; Lototskyy, M.; Tolj, I. Selection of metal hydrides-based thermal energy storage: Energy storage efficiency and density targets. Int. J. Hydrogen Energy 2018, 43, 22568–22583. [Google Scholar]
- Corgnale, C.; Hardy, B.; Motyka, T.; Zidan, R.; Teprovich, J.; Peters, B. Screening analysis of metal hydride based thermal energy storage systems for concentrating solar power plants. Renew. Sustain. Energy Rev. 2014, 38, 821–833. [Google Scholar] [CrossRef]
- Yang, F.; Zhen Wu, Z.; Liu, S.; Zhang, Y.; Geoff Wang, G.; Zhang, Z.; Wang, Y. Theoretical formulation and performance analysis of a novel hydride heat Pump(HHP) integrated heat recovery system. Energy 2018, 163, 208–220. [Google Scholar] [CrossRef]
- Mellouli, S.; Abhilash, E.; Askri, F.; Nasrallah, S.B. Integration of thermal energy storage unit in a metal hydride hydrogen storage tank. Appl. Therm. Eng. 2016, 102, 1185–1196. [Google Scholar] [CrossRef]
- Darzi, A.A.R.; Afrouzi, H.H.; Moshfegh, A.; Farhadi, M. Absorption and desorption of hydrogen in long metal hydride tank equipped with phase change material jacket. Int. J. Hydrogen Energy 2016, 41, 9595–9610. [Google Scholar] [CrossRef]
- Tong, L.; Xiao, J.; Bénard, P.; Chahine, R. Thermal management of metal hydride hydrogen storage reservoir using phase change materials. Int. J. Hydrogen Energy 2019, 44, 21055–21066. [Google Scholar] [CrossRef]
- Nyamsi, S.N.; Yang, F.; Zhang, Z. An optimization study on the finned tube heat exchanger used in hydride hydrogen storage system- analytical method and numerical simulation. Int. J. Hydrogen Energy 2012, 37, 16078–16092. [Google Scholar] [CrossRef]
- Longeon, M.; Soupart, A.; Fourmigué, J.F.; Bruch, A.; Marty, P. Experimental and numerical study of annular PCM storage in the presence of natural convection. Appl. Energy 2013, 112, 175–184. [Google Scholar] [CrossRef]
- Siyabi, I.A.; Khanna, S.; Mallick, T.; Sundaram, S. An experimental and numerical study on the effect of inclination angle of phase change materials thermal energy storage system. J. Energy Storage 2019, 23, 57–68. [Google Scholar] [CrossRef]
- Chiu, J.N.W.; Martin, V. Submerged finned heat exchanger latent heat storage design and its experimental verification. Appl. Energy 2012, 93, 507–513. [Google Scholar] [CrossRef]
- Miled, A.; Mellouli, S.; Ben Maad, H.; Askri, F. Improvement of the performance of metal hydride pump by using phase change heat exchanger. Int. J. Hydrogen Energy 2017, 42, 26343–26361. [Google Scholar] [CrossRef]
- Laurencelle, F.; Goyette, J. Simulation of heat transfer in metal hydride reactor with aluminum foam. Int. J. Hydrogen Energy 2007, 32, 2957–2964. [Google Scholar] [CrossRef]
- Wang, J.F.; Xie, H.Q.; Xin, Z.; Li, Y.; Chen, L.F. Enhancing thermal conductivity of palmitic acid based phase change materials with carbon nanotubes as fillers. Sol. Energy 2010, 84, 339–344. [Google Scholar] [CrossRef]
- Jun, F.K.; Yuichi, H.; Yoshi, M.; Osamu, M. Improvement of thermal characteristics of latent heat thermal energy storage units using carbon-fiber brushes: Experiments and modeling. Int. J. Heat Mass Transf. 2003, 46, 4513–4525. [Google Scholar]


| Parameter/Unit | Mg2Ni | LaNi5 |
|---|---|---|
| Enthalpy of formation/kJ∙mol−1 | 64.5 | 30.5 |
| Entropy of formation/J∙mol−1∙K−1 | 122.2 | 108 |
| Activation energy, abs-des/kJ∙mol−1 | 52.20/63.46 | 21.17/16.47 |
| Rate constant abs-des/s−1 | 175/5452.2 | 59.18/9.57 |
| Density/kg∙m−3 | 3200 | 8400 |
| Specific heat, M-MH/J∙kg−1∙K−1 | 697 | 419 |
| Hydrogen capacity/wt.% | 3.6 | 1.39 |
| Porosity | 0.5 | 0.5 |
| Permeability/m2 | 1 × 10−12 | 1 × 10−12 |
| Effective thermal conductivity/W∙m−1∙K−1 | 1 | 1 |
| Hydride thickness/m | 0.015 | 0.015 |
| H2 filter radius, r0/m | 0.003 | 0.003 |
| Reactor length LMH/m | 0.45 | 0.45 |
| Parameter/Unit | RT31 | RT42 |
|---|---|---|
| Melting temperature Tm/°C | 31 | 42 |
| Density: solid-liquid/kg∙m−3 | 880–760 | 880–760 |
| Thermal conductivity/W∙m−1∙K−1 | 0.2 | 0.2 |
| Specific heat, Cp/J∙kg−1∙K−1 | 2000 | 2000 |
| Latent heat of fusion, ∆Hpcm/kJ∙kg−1 | 165 | 165 |
| Volumetric energy density/MJ∙m−3 | 145 | 145 |
| PCM jacket radius/m | 0.04 | 0.04 |
| Reactor length Lpcm/m | 0.47 | 0.47 |
| Parameters | - | Performance Indicators | ||
|---|---|---|---|---|
| Thermo-physical properties | Range | Energy density/MJ∙m−3 | Power output/W∙kg−1-Mg2Ni | Energy recovery efficiency/% |
| Cp/J∙kg−1∙K−1 | 1800 | 328.89 | 97.52 | 52.42 |
| 2000 | 334.12 | 97.91 | 51.81 | |
| 2300 | 337.38 | 98.34 | 51.15 | |
| 3000 | 342.81 | 97.76 | 50.41 | |
| Max deviation | 4.23% | 0.8% | −3.83% | |
| ρpcm/kg∙m−3 | 780 | 323.27 | 96.73 | 52.9 |
| 900 | 340.13 | 98.13 | 50.71 | |
| 2000 | 369.26 | 98.84 | 47.32 | |
| Max deviation | 14.22% | 2.18% | −10.54% | |
| ∆Hpcm/kJ∙kg−1 | 140 | 315.84 | 94.89 | 53.11 |
| 180 | 337.41 | 98.05 | 51.66 | |
| 200 | 342.06 | 97.78 | 50.53 | |
| 250 | 349.06 | 97.16 | 49.20 | |
| Max deviation | 10.51% | 3.33% | −7.36% | |
| ∆Ttr/K | 1 | 333.06 | 97.91 | 51.97 |
| 5 | 332.18 | 97.40 | 52.71 | |
| 10 | 331.17 | 96.81 | 51.68 | |
| 20 | 329.35 | 92.71 | 49.76 | |
| Max deviation | −1.11% | −5.31% | −4.25% | |
| Design parameters | Range | - | ||
| Vpcm/VLTMH | 1.71 | 343.92 | 59.33 | 47.36 |
| 2.79 | 345.21 | 83.84 | 53.05 | |
| 4.03 | 331.27 | 98.14 | 52.37 | |
| 5.44 | 285.91 | 91.91 | 46.73 | |
| Max deviation | −16.86% | 54.91% | 13.34% | |
| λpcm/W∙m−1∙K−1 | 0.2 | 267.88 | 51.42 | 33.93 |
| 1 | 333.69 | 98.11 | 51.98 | |
| 2 | 332.54 | 105.30 | 55.98 | |
| 5 | 331.05 | 107.62 | 57.47 | |
| 10 | 332.12 | 109.67 | 58.37 | |
| Max deviation | 23.98% | 113.28% | 72.03% | |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nyallang Nyamsi, S.; Tolj, I.; Lototskyy, M. Metal Hydride Beds-Phase Change Materials: Dual Mode Thermal Energy Storage for Medium-High Temperature Industrial Waste Heat Recovery. Energies 2019, 12, 3949. https://doi.org/10.3390/en12203949
Nyallang Nyamsi S, Tolj I, Lototskyy M. Metal Hydride Beds-Phase Change Materials: Dual Mode Thermal Energy Storage for Medium-High Temperature Industrial Waste Heat Recovery. Energies. 2019; 12(20):3949. https://doi.org/10.3390/en12203949
Chicago/Turabian StyleNyallang Nyamsi, Serge, Ivan Tolj, and Mykhaylo Lototskyy. 2019. "Metal Hydride Beds-Phase Change Materials: Dual Mode Thermal Energy Storage for Medium-High Temperature Industrial Waste Heat Recovery" Energies 12, no. 20: 3949. https://doi.org/10.3390/en12203949
APA StyleNyallang Nyamsi, S., Tolj, I., & Lototskyy, M. (2019). Metal Hydride Beds-Phase Change Materials: Dual Mode Thermal Energy Storage for Medium-High Temperature Industrial Waste Heat Recovery. Energies, 12(20), 3949. https://doi.org/10.3390/en12203949







