Research on Large-Signal Stability of DC Microgrid Based on Droop Control
Abstract
:1. Introduction
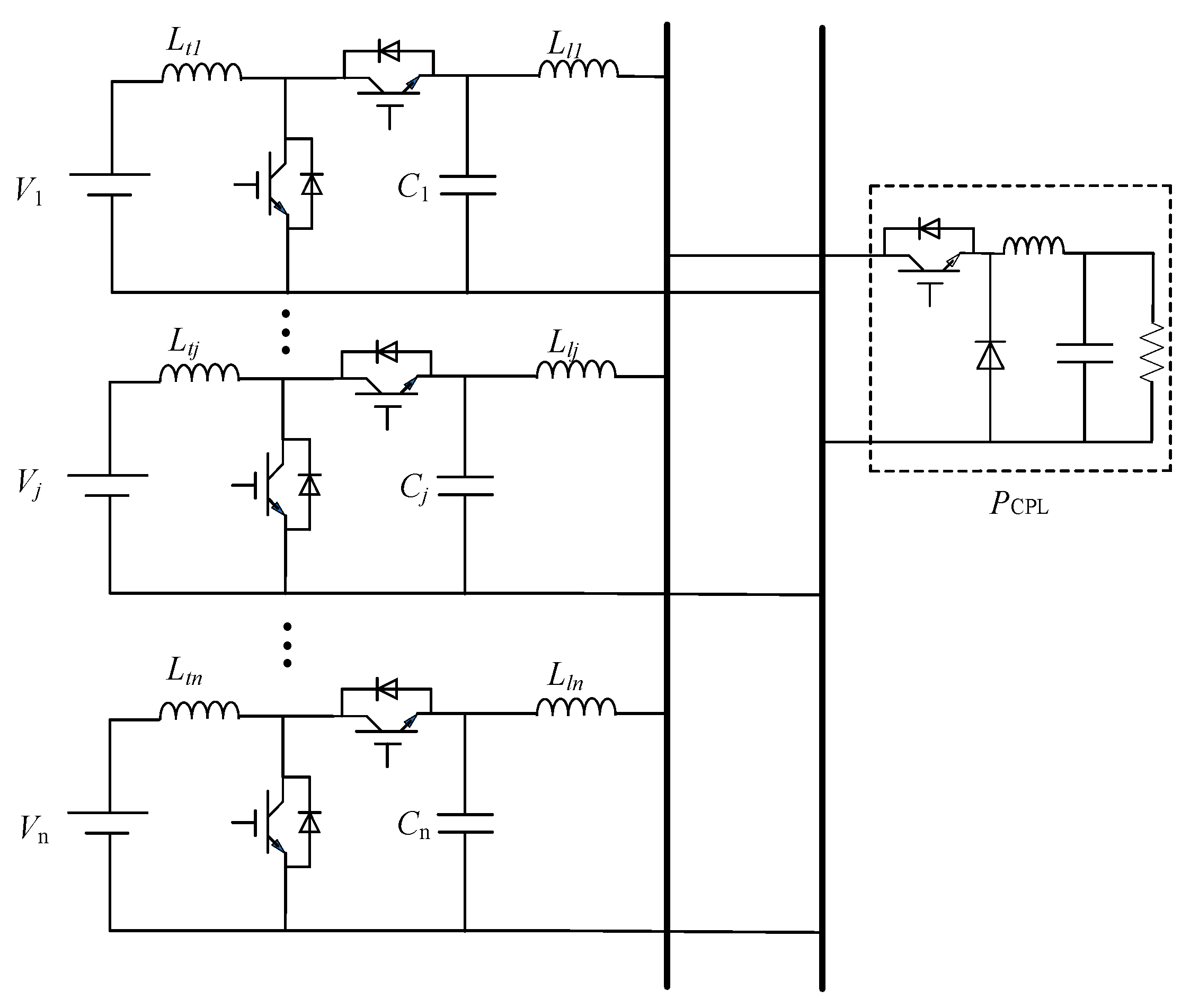
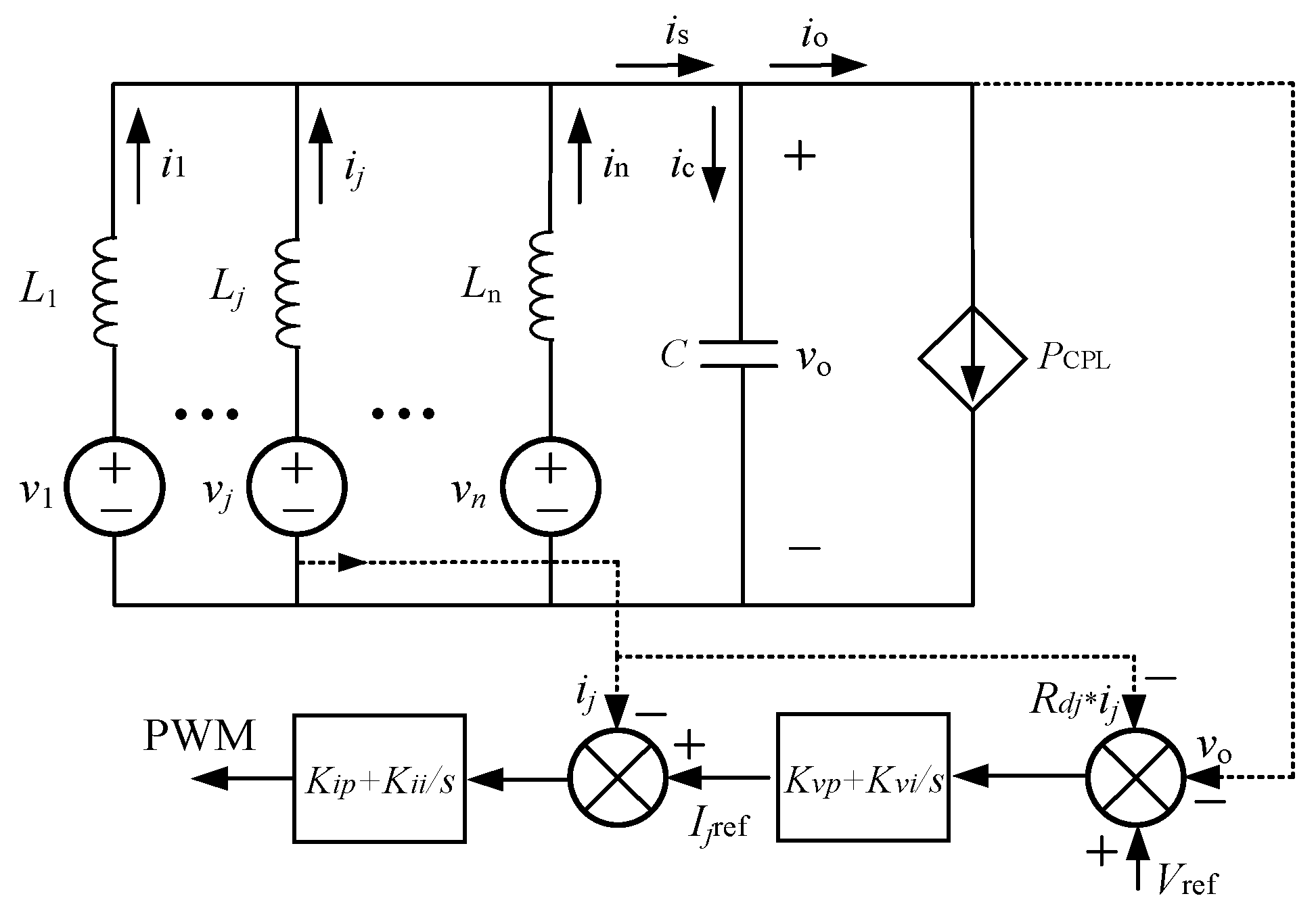
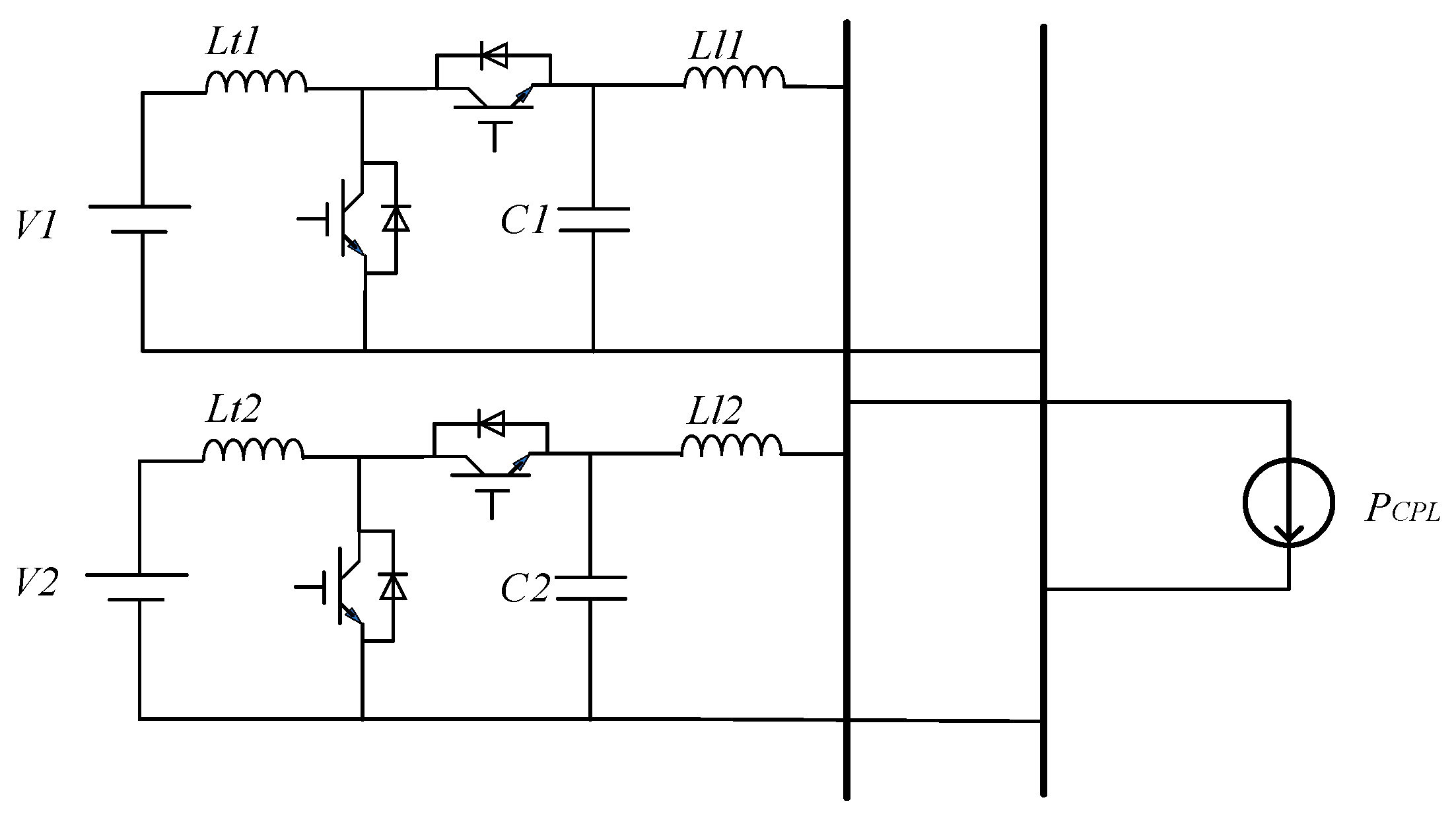
2. DC Microgrid Structure and Large Signal Model
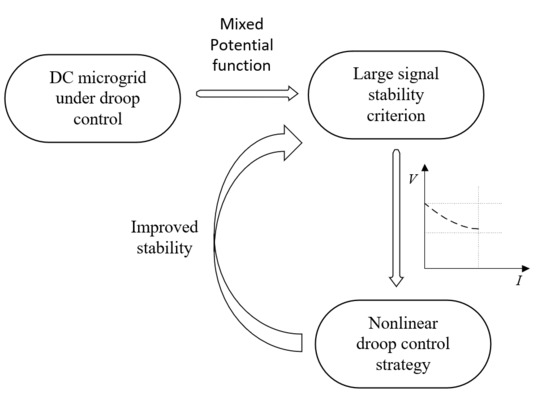
3. Application of Mixed Potential Function Method in the DC Microgrid
3.1. Mixed Potential Function Theory
- Calculate the potential function of all non-energy storage components in the circuit;
- Calculate the energy contained in the capacitive component of the circuit, that is, the product of the capacitor voltage and the capacitor current;
- Add the above two to obtain the mixed potential function of the circuit.
3.2. Large Signal Stability Criterion of the System
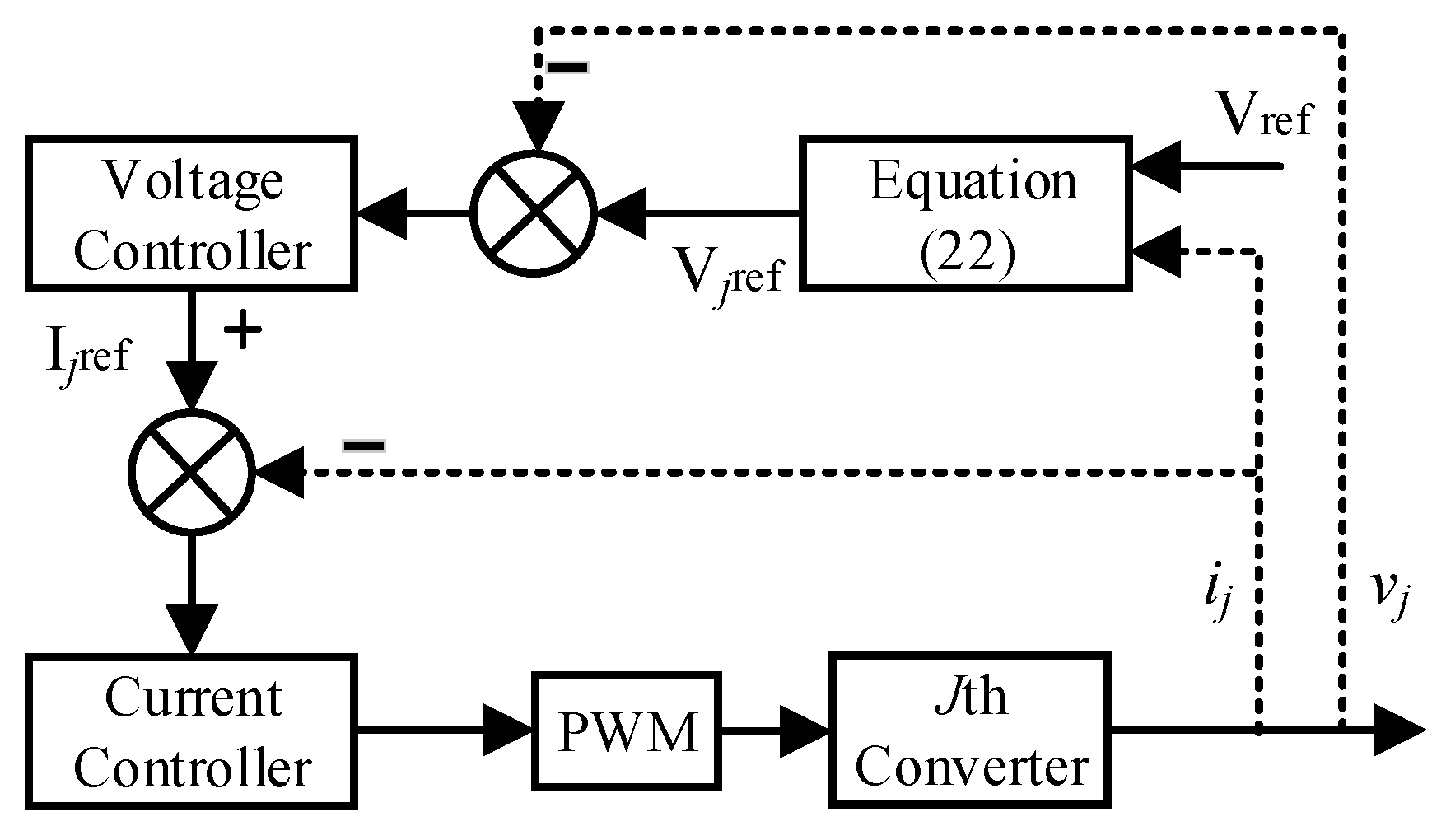
4. Nonlinear Droop Control Strategy
4.1. Design of Nonlinear Droop Curve
- The starting point of the droop curve should be set at the initial voltage point of the unloaded state.
- The abscissa of the end point of the droop curve is the maximum current value allowed to flow through the inverter, and the ordinate is the lowest allowable voltage value of the bus.
4.2. Large Signal Stability Analysis with Nonlinear Droop Control
5. Simulation
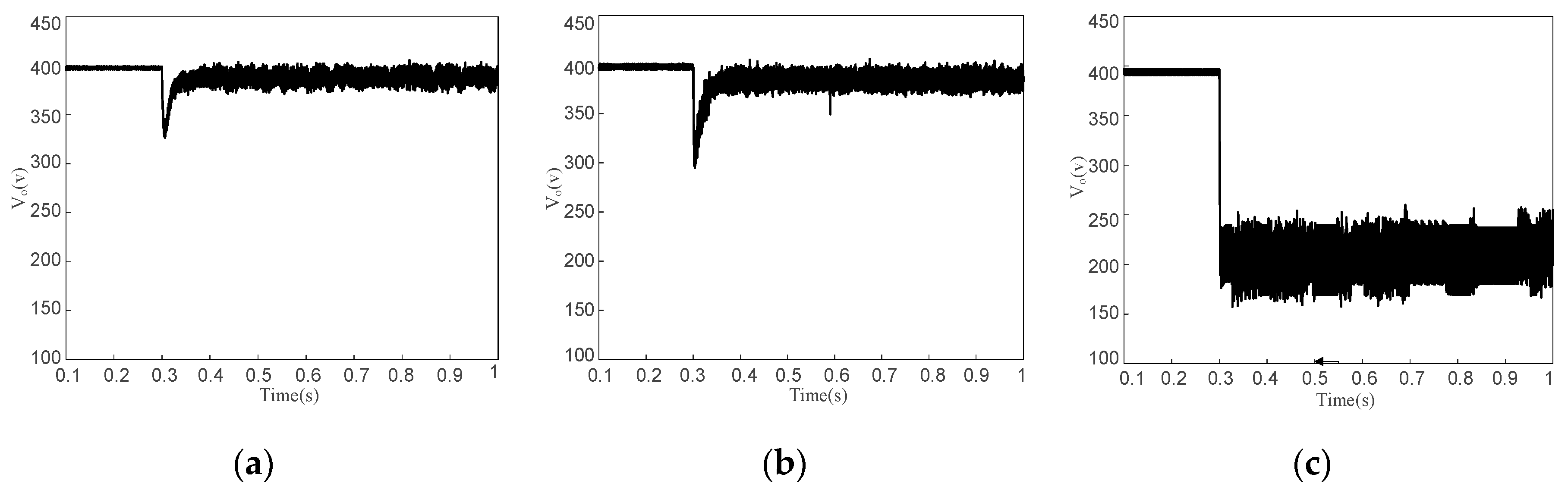
5.1. Verification of Large Signal Stability Criteria with Conventional Droop Control
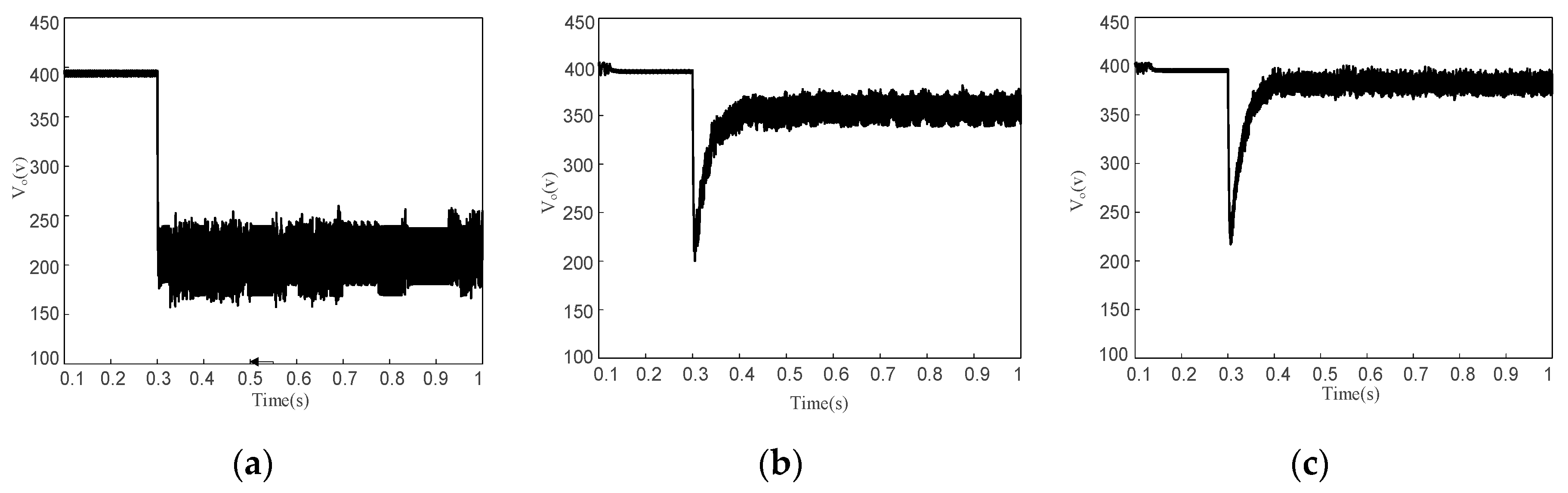
5.2. Validity Verification of Nonlinear Droop Control Strategy
6. Conclusions
Author Contributions
Conflicts of Interest
Appendix A
References
- Lasseter, R.H.; Eto, J.H.; Schenkman, B. CERTS microgrid laboratory test bed. IEEE Trans. Power Deliv. 2001, 26, 325–332. [Google Scholar] [CrossRef]
- Zhang, Y.; Li, Y. Energy management strategy for supercapacitor in autonomous DC microgrid using virtual impedance. Appl. Power Electron. Conf. Expo. 2015, 725–730. [Google Scholar] [CrossRef]
- Rana, M.M.; Xiang, W.; Wang, E. Smart grid state estimation and stabilization. Int. J. Electr. Power Energy Syst. 2018, 102, 152–159. [Google Scholar] [CrossRef]
- Li, W.; Gu, Y.; Wang, Y.; Xiang, X.; He, X. Control Architecture and Hierarchy Division for Renewable Energy DC Microgrids. Autom. Electr. Power Syst. 2015, 39, 156–163. [Google Scholar]
- Li, M.; Huang, W.; Tai, N.; Yu, M. Lyapunov-Based Large Signal Stability Assessment for VSG Controlled Inverter-Interfaced Distributed Generators. Energies 2018, 11, 2273. [Google Scholar] [CrossRef]
- Wang, R.; Wu, Y.; He, G.; Lv, Y.; Du, J.; Li, Y. Impedance Modeling and Stability Analysis for Cascade System of Three-Phase PWM Rectifier and LLC Resonant Converter. Energies 2018, 11, 3050. [Google Scholar] [CrossRef]
- Weinstein, M.I. Lyapunov stability of ground states of nonlinear dispersive evolution equations. Commun. Pure Appl. Math. 1986, 39, 51–67. [Google Scholar] [CrossRef]
- Rivetta, C.H.; Emadi, A.; Williamson, G.A.; Jayabalan, R.; Fahimi, B. Analysis and control of a buck DC-DC converter operating with constant power load in sea and undersea vehicles. Ind. Appl. IEEE Trans. 2006, 42, 559–572. [Google Scholar] [CrossRef]
- Rivetta, C.H.; Emadi, A.; Williamson, G.A. Large-signal analysis of a DC-DC buck power converter operating with constant power load. In Proceedings of the 29th Annual Conference of the IEEE Industrial Electronics Society, 2003, IECON’03, Roanoke, VA, USA, 2–6 November 2003. [Google Scholar] [CrossRef]
- Marx, D.; Magne, P.; Nahid-Mobarakeh, B.; Pierfederici, S.; Davat, B. Large signal stability analysis tools in dc power systems with constant power loads and variable power loads—A review. IEEE Trans. Power Electron. 2012, 27, 1773–1787. [Google Scholar] [CrossRef]
- Magne, P.; Marx, D.; Nahid-Mobarakeh, B.; Pierfederici, S. Large-Signal stabilization of a dc-link supplying a constant power load using a virtual capacitor: impact on the domain of attraction. IEEE Trans. Ind. Appl. 2012, 48, 878–887. [Google Scholar] [CrossRef]
- Liu, X.; Zhou, Y.; Zhang, W. Large signal stability criteria for constant power loads with damped filters. Trans. China Electrotech. Soc. 2011, 26, 154–160. [Google Scholar]
- Liu, X.; Zhou, Y.; Zhang, W.; Ma, S. Stability criteria for constant power loads with multistage filters. IEEE Trans. Veh. Technol. 2011, 60, 2042–2049. [Google Scholar] [CrossRef]
- Tahim, A.P.N.; Pagano, D.J.; Lenz, E.; Stramosk, V. Modeling and stability analysis of islanded dc microgrids under droop control. IEEE Trans. Power Electron. 2015, 30, 4597–4607. [Google Scholar] [CrossRef]
- Su, M.; Liu, Z.; Sun, Y.; Han, H.; Hou, X. Stability analysis and stabilization methods of dc microgrid with multiple parallel-connected dc–dc converters loaded by CPLs. IEEE Trans. Smart Grid 2017, 9, 132–142. [Google Scholar] [CrossRef]
- Li, Z.; Kong, L.; Pei, W.; Ye, H.; Deng, W. Large-Disturbance stability analysis of droop-controlled dc microgrid based on mixed potential function. Power Syst. Technol. 2018, 42, 3725–3734. [Google Scholar]
- Zhao, Z.; Hu, J.; Xue, H.; Huang, R.; Li, X.; Zhang, X. Large signal stability analysis of dc microgrid under droop control with constant power load. Chin. Autom. Congr. (CAC) 2017, 1046–1051. [Google Scholar] [CrossRef]
- Brayton, R.K.; Moser, J.K. A theory of nonlinear networks. Q. Appl. Math. 1964, 22, 1–33. [Google Scholar] [CrossRef]
- Weiss, L.; Mathis, W.; Trajkovic, L. A Generalization of Brayton-Moser’s Mixed Potential Function. IEEE Trans. Circuits Syst. Fundam. Theory Appl. 1998, 45, 423–427. [Google Scholar] [CrossRef]
- Liang, H.; Zheng, C.; Gao, Y.; Li, P. Research on improved droop control strategy for microgrid. Proc CSEE 2017, 37, 4901–4910. [Google Scholar]
- Liang, H.; Dong, Y.; Huang, Y.; Zheng, C.; Li, P. Modeling of multiple master–slave control under island microgrid and stability analysis based on control parameter configuration. Energies 2018, 11, 2223. [Google Scholar] [CrossRef]

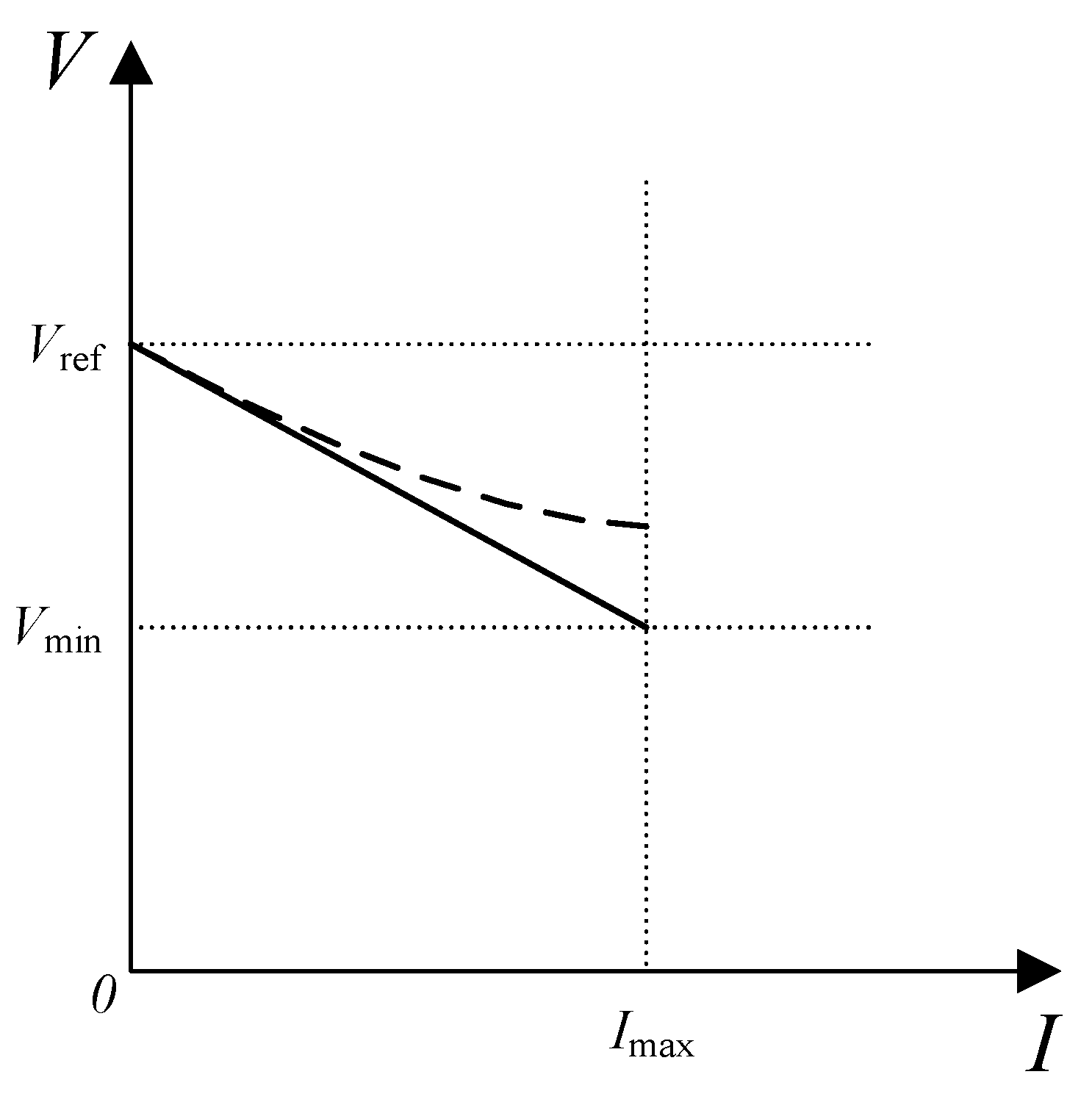

| Parameters | Value |
|---|---|
| Inductance of converter1 (Lt1) | 0.1 mH |
| Inductance of converter2 (Lt2) | 0.15 mH |
| Capacitance of converter1 (C1) | 1000 μF |
| Capacitance of converter2 (C2) | 2000 μF |
| Line inductance of micro-source1 (Ll1) | 70 μH |
| Line inductance of micro-source1 (Ll2) | 40 μH |
| Droop coefficient of micro-source1 (Rd1) | 0.8 |
| Droop coefficient of micro-source1 (Rd2) | 0.6 |
| Proportional coefficient of voltage loop (Kvp) | 0.1 |
| Integral coefficient of voltage loop (Kvi) | 80 |
| Proportional coefficient of current loop (Kip) | 20 |
| Integral coefficient of current loop (Kii) | 100 |
| Switching frequency | 20 kHz |
| Output voltage of load | 200 V |
| Sequence Number | Kvp | Rd1 | Rd2 | Calculation Result |
|---|---|---|---|---|
| 1 | 0.05 | 0.8 | 0.6 | Unstable |
| 2 | 0.15 | 0.8 | 0.6 | Stable |
| 3 | 0.15 | 2.8 | 2.1 | Unstable |
| 4 | 0.15 | 0.4 | 0.3 | Stable |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liang, H.; Huang, Y.; Sun, H.; Liu, Z. Research on Large-Signal Stability of DC Microgrid Based on Droop Control. Energies 2019, 12, 3186. https://doi.org/10.3390/en12163186
Liang H, Huang Y, Sun H, Liu Z. Research on Large-Signal Stability of DC Microgrid Based on Droop Control. Energies. 2019; 12(16):3186. https://doi.org/10.3390/en12163186
Chicago/Turabian StyleLiang, Haifeng, Yuxi Huang, Hao Sun, and Zhiqian Liu. 2019. "Research on Large-Signal Stability of DC Microgrid Based on Droop Control" Energies 12, no. 16: 3186. https://doi.org/10.3390/en12163186
APA StyleLiang, H., Huang, Y., Sun, H., & Liu, Z. (2019). Research on Large-Signal Stability of DC Microgrid Based on Droop Control. Energies, 12(16), 3186. https://doi.org/10.3390/en12163186