A Resonant Coupling Power Transfer System Using Two Driving Coils
Abstract
1. Introduction
2. Theory and Design
2.1. Theoretical Basis
2.2. Proposed Coil Model
3. Results and Discussions
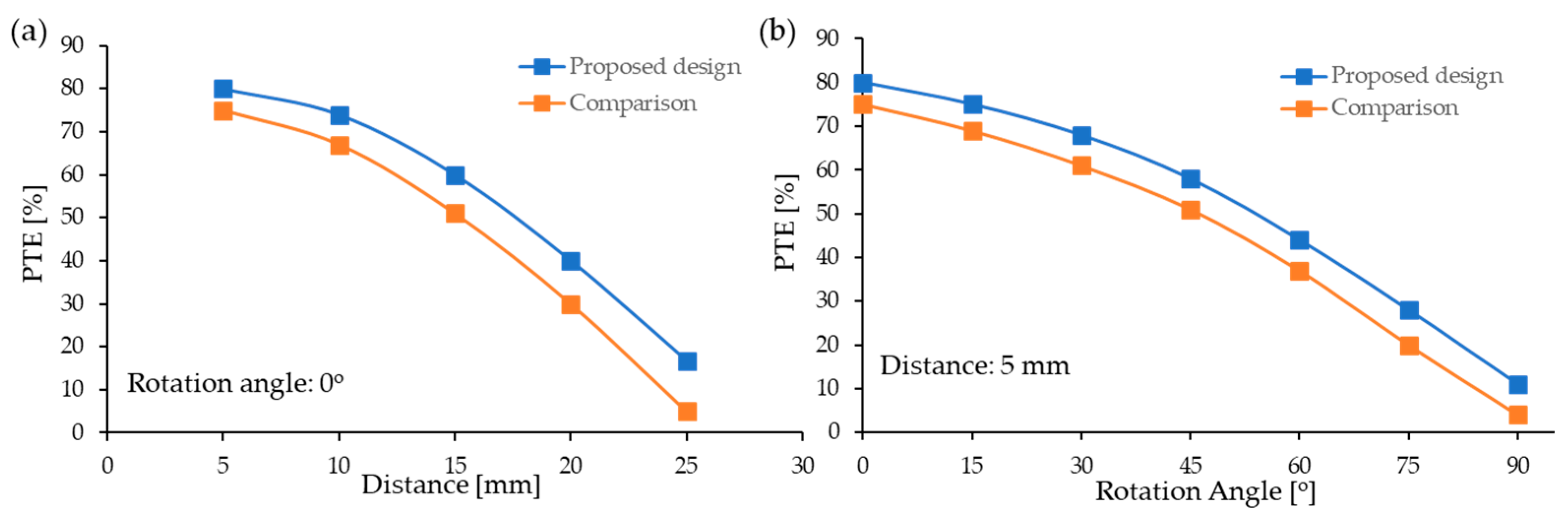
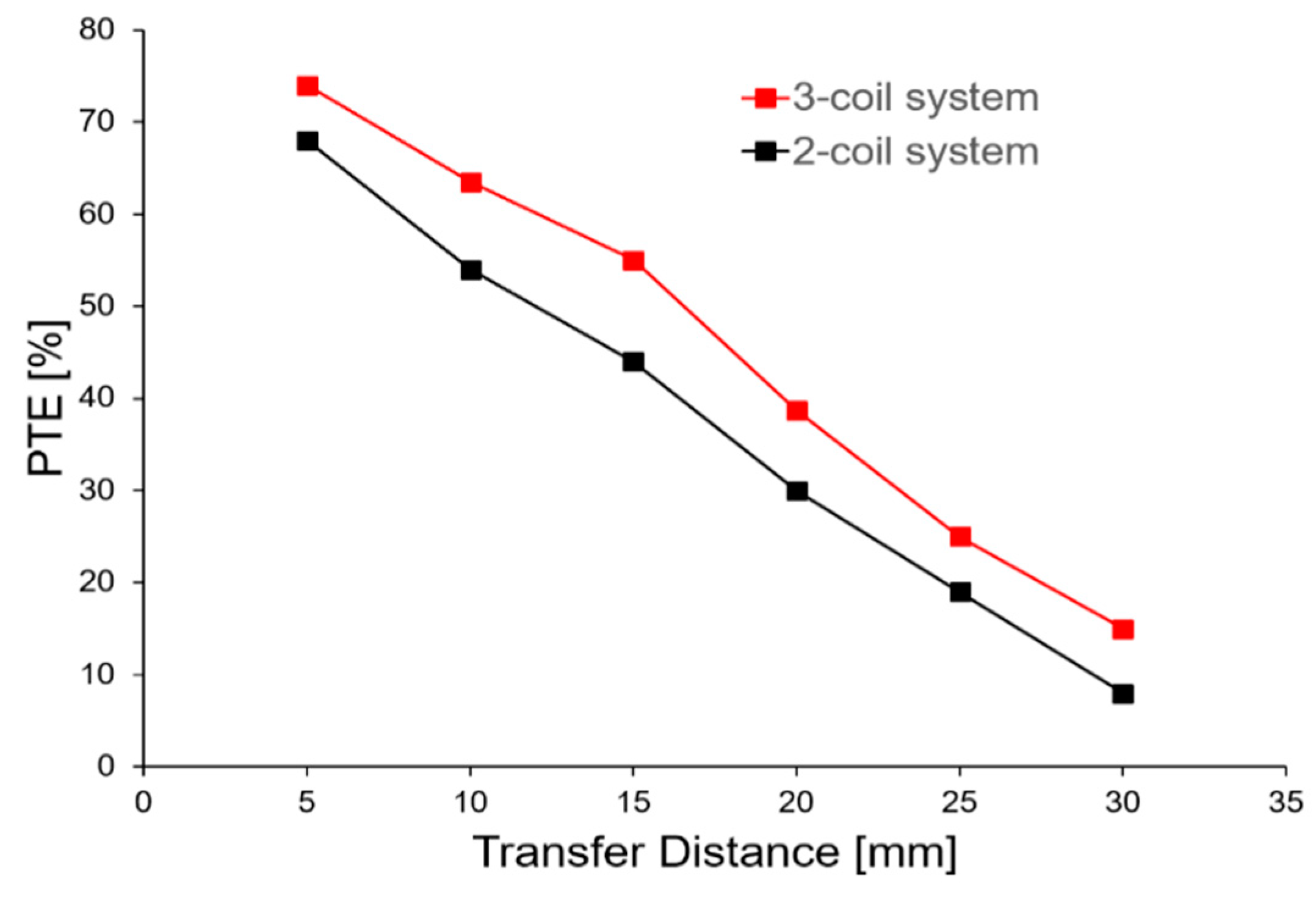
3.1. Power Transfer Efficiency
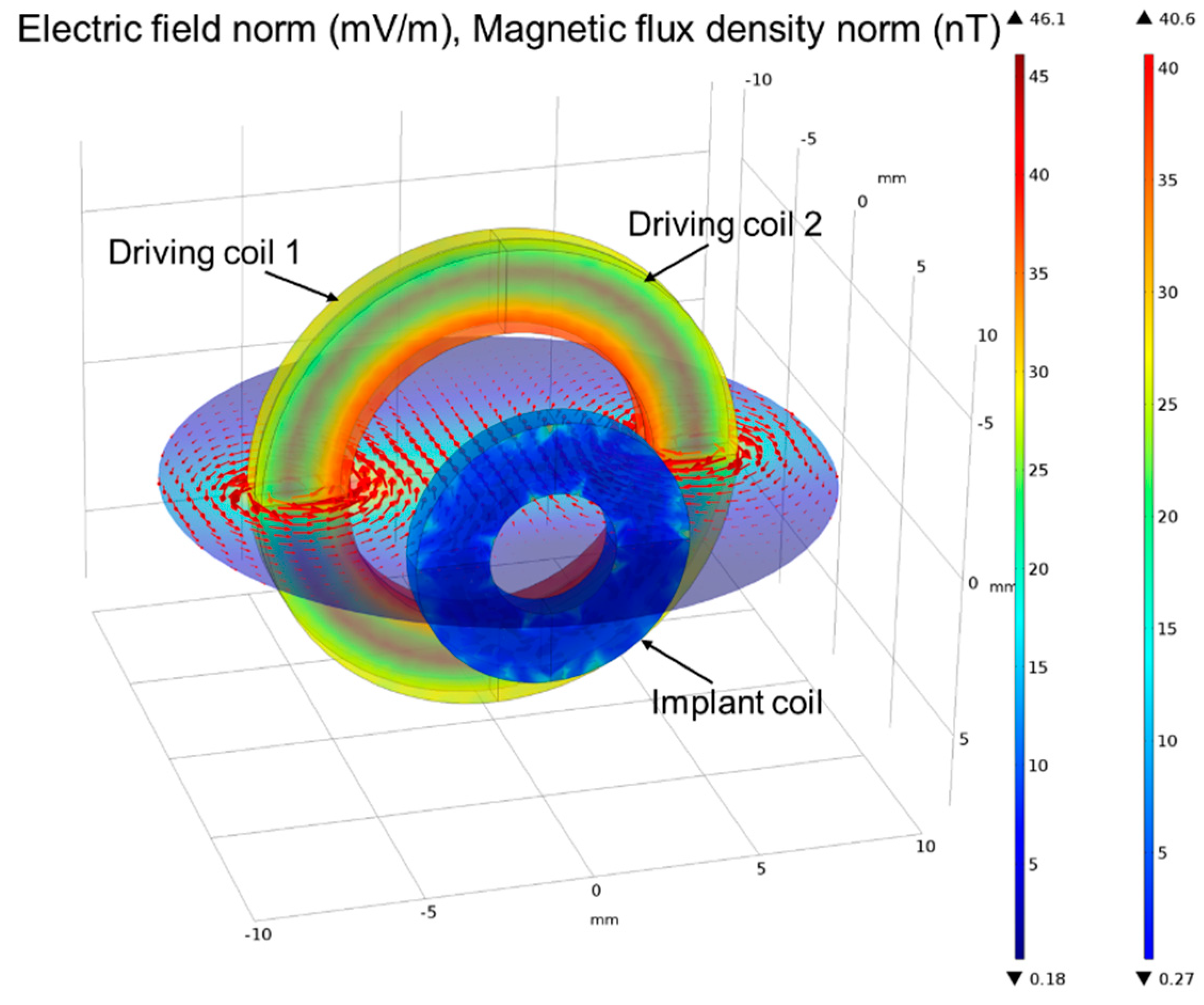
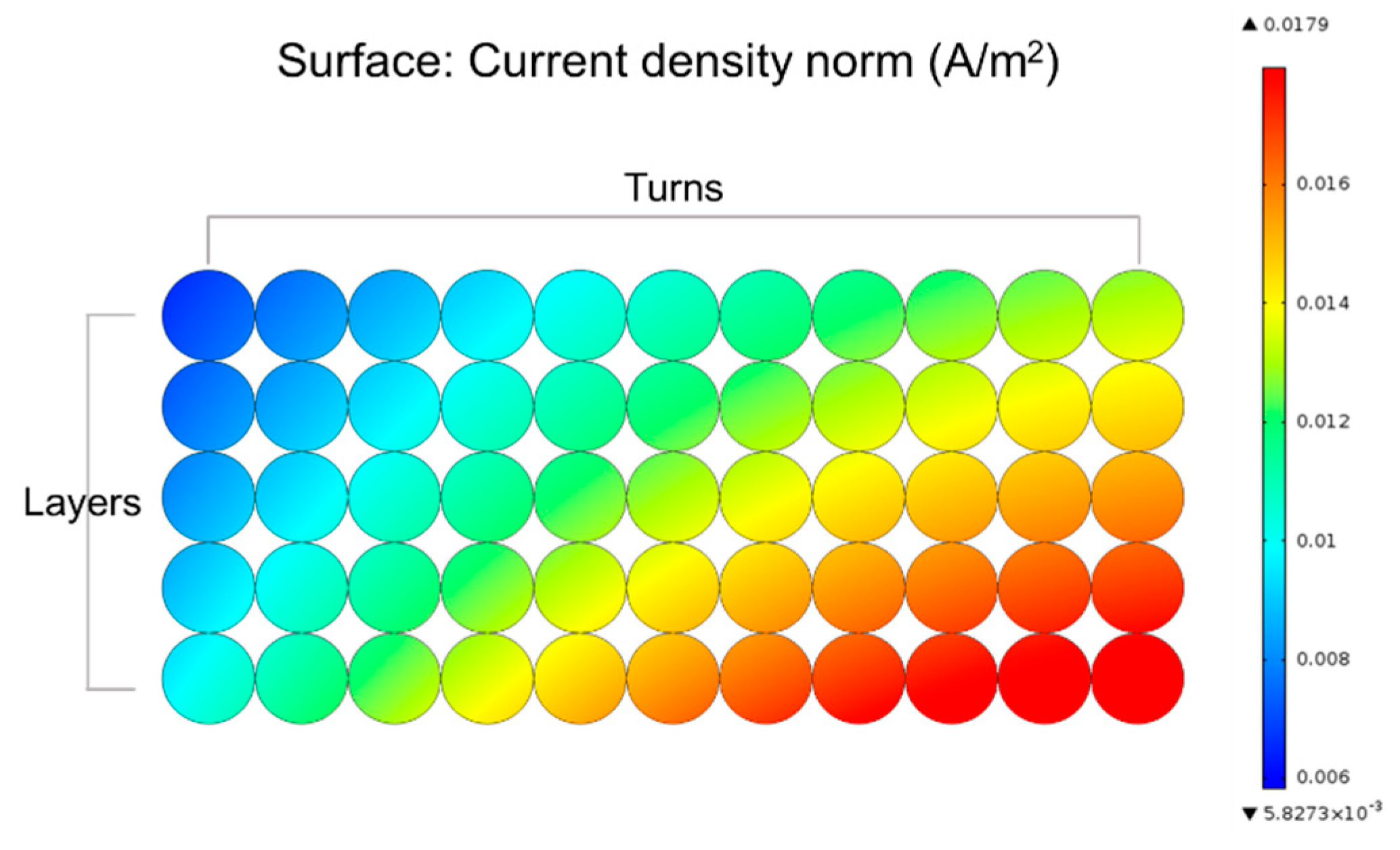
3.2. Simulation Results
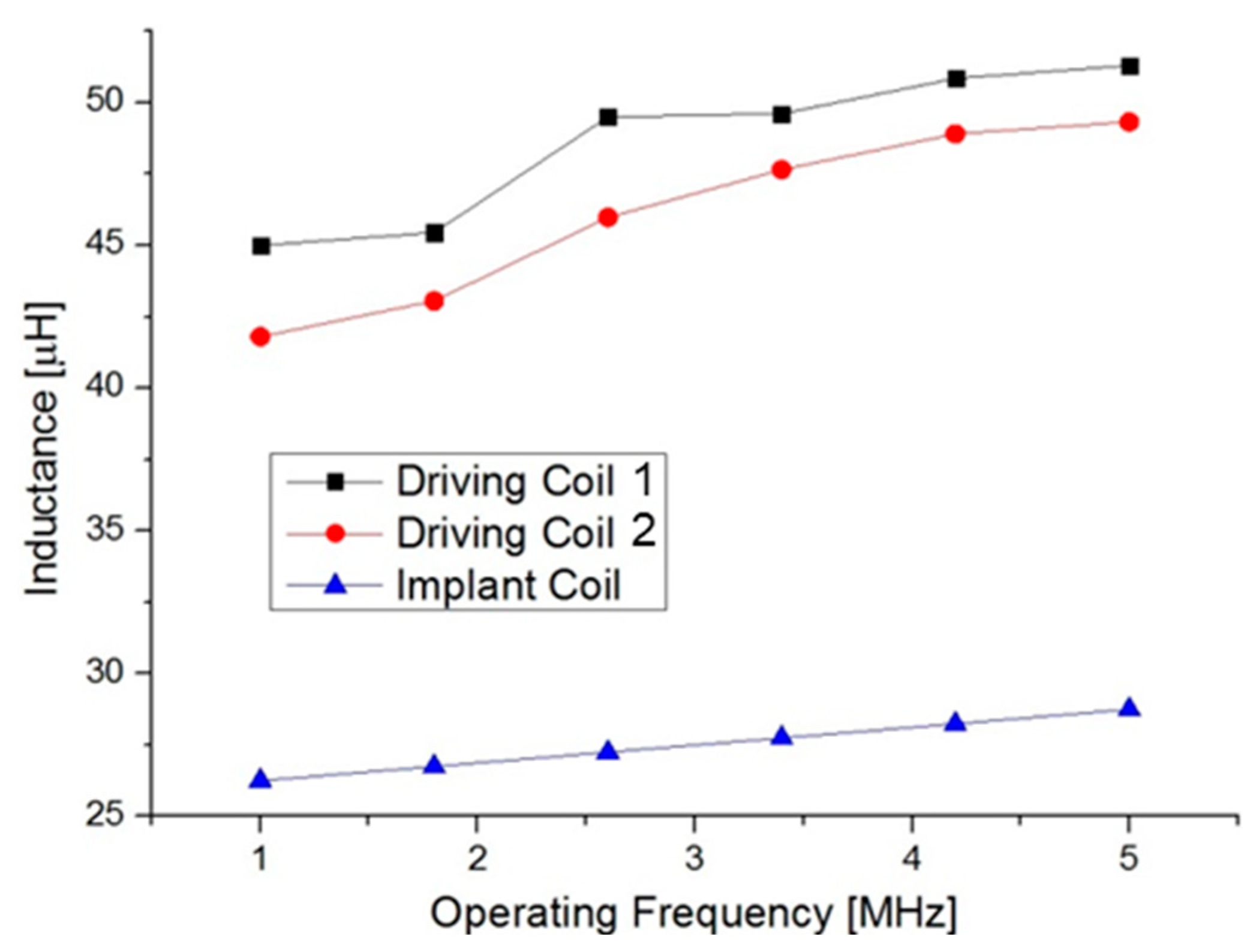
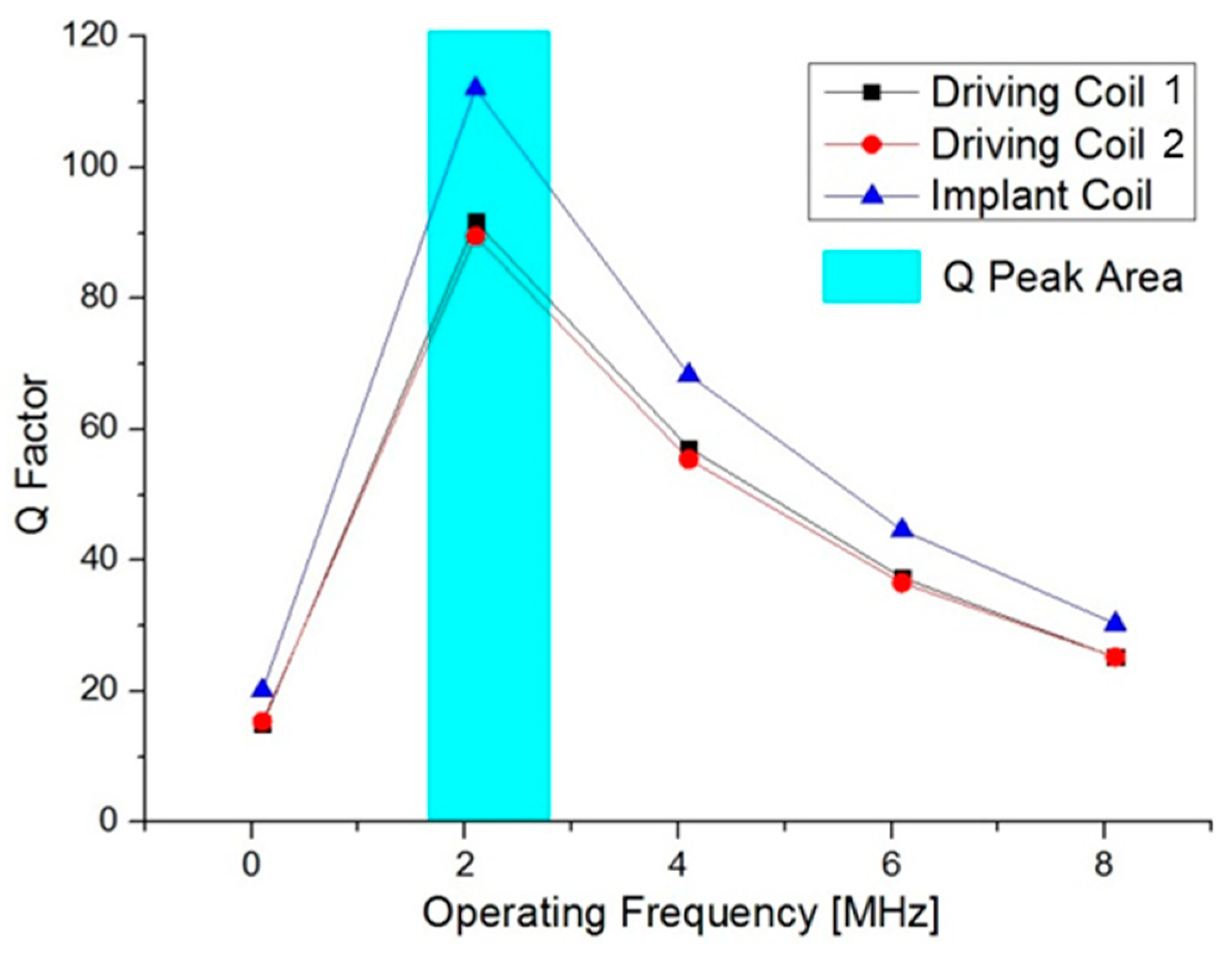
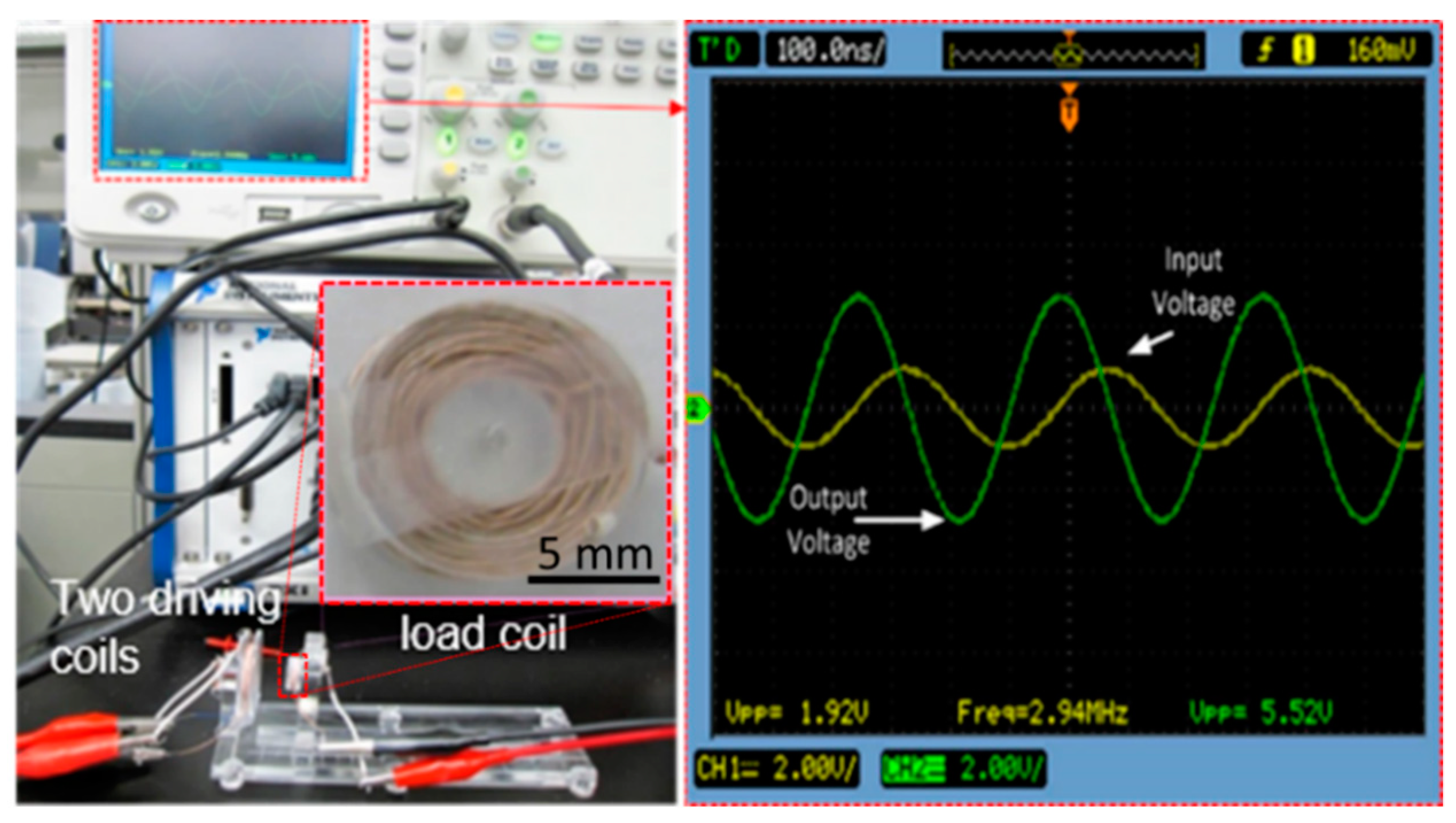
3.3. Experimental Measurements
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Yi, Y.; Zaher, A.; Yassine, O.; Kosel, J.; Foulds, I. A remotely operated drug delivery system with an electrolytic pump and a thermo-responsive valve. Biomicrofluidics 2015, 9, 052608. [Google Scholar] [CrossRef] [PubMed]
- Yang, Z.; Liu, W.; Basham, E. Inductor modeling in wireless links for implantable electronics. IEEE Trans. Magn. 2007, 43, 3851–3860. [Google Scholar] [CrossRef]
- Yi, Y.; Buttner, U.; Foulds, I. A cyclically actuated electrolytic drug delivery device. Lab Chip 2015, 15, 3540–3548. [Google Scholar] [CrossRef] [PubMed]
- Xue, R.F.; Cheng, K.W.; Je, M. High-Efficiency Wireless Power Transfer for Biomedical Implants by Optimal Resonant Load Transformation. IEEE Trans. Circuits Syst. I Regul. Pap. 2013, 60, 867–874. [Google Scholar] [CrossRef]
- Yi, Y.; Chen, J.; Selvaraj, M.; Hsiang, Y.; Takahata, K. Wireless Hyperthermia Stent System for Restenosis Treatment and Testing with Swine Model. IEEE Trans. Biomed. Eng. 2019, (in press).
- Yi, Y.; Huang, R.; Li, C. Flexible substrate-based thermo-responsive valve applied in electromagnetically powered drug delivery system. J. Mater. Sci. 2019, 54, 3392–3402. [Google Scholar] [CrossRef]
- Baker, M.; Sarpeshkar, R. Feedback Analysis and Design of RF Power Links for Low-Power Bionic Systems. IEEE Trans. Biomed. Circuits Syst. 2007, 1, 28–38. [Google Scholar] [CrossRef] [PubMed]
- Han, L.; Li, L. Integrated wireless communications and wireless power transfer: An overview. Phys. Commun. 2017, 25, 555–563. [Google Scholar] [CrossRef]
- Catrysse, M.; Hermans, B.; Puers, R. An inductive power system with integrated bi-directional data-transmission. Sens. Actuators A Phys. 2004, 115, 221–229. [Google Scholar] [CrossRef]
- Yi, Y.; Buttner, U.; Fan, Y.; Foulds, I. Design and optimization of a 3-coil resonance-based wireless power transfer system for biomedical implants. Int. J. Circuit Theory Appl. 2014, 43, 1379–1390. [Google Scholar] [CrossRef]
- Kiani, M.; Jow, U.M.; Ghovanloo, M. Design and Optimization of a 3-Coil Inductive Link for Efficient Wireless Power Transmission. IEEE Trans. Biomed. Circuits Syst. 2011, 5, 579–591. [Google Scholar] [CrossRef] [PubMed]
- RamRakhyani, A.; Mirabbasi, S.; Chiao, M. Design and Optimization of Resonance-Based Efficient Wireless Power Delivery Systems for Biomedical Implants. IEEE Trans. Biomed. Circuits Syst. 2011, 5, 48–63. [Google Scholar] [CrossRef] [PubMed]
- Kurs, A.; Karalis, A.; Moffatt, R.; Joannopoulos, J.; Fisher, P.; Soljacic, M. Wireless Power Transfer via Strongly Coupled Magnetic Resonances. Science 2007, 317, 83–86. [Google Scholar] [CrossRef] [PubMed]
- Yi, Y.; Kosel, J. A remotely operated drug delivery system with dose control. Sens. Actuators A Phys. 2017, 261, 177–183. [Google Scholar] [CrossRef]
- Jow, U.; Ghovanloo, M. Design and Optimization of Printed Spiral Coils for Efficient Transcutaneous Inductive Power Transmission. IEEE Trans. Biomed. Circuits Syst. 2007, 1, 193–202. [Google Scholar] [CrossRef] [PubMed]
- Kim, J.W.; Son, H.C.; Kim, K.H.; Park, Y.J. Efficiency Analysis of Magnetic Resonance Wireless Power Transfer With Intermediate Resonant Coil. IEEE Antennas Wirel. Propag. Lett. 2011, 10, 389–392. [Google Scholar] [CrossRef]
- Ahn, D.; Hong, S. Effect of Coupling Between Multiple Transmitters or Multiple Receivers on Wireless Power Transfer. IEEE Trans. Ind. Electron. 2013, 60, 2602–2613. [Google Scholar] [CrossRef]
- Arakawa, T.; Coguri, S.; Krogmeier, J.V.; Kruger, A.; Love, D.J.; Mudumbai, R.; Swabey, M.A. Optimizing Wireless Power Transfer from Multiple Transmit Coils. IEEE Access 2018, 6, 23828–23838. [Google Scholar] [CrossRef]
- Park, S.; Kim, M. Numerical Exposure Assessment Method for Low Frequency Range and Application to Wireless Power Transfer. PLoS ONE 2016, 11, e0166720. [Google Scholar] [CrossRef] [PubMed]
- Christ, A.; Douglas, M.; Nadakuduti, J.; Kuster, N. Assessing Human Exposure to Electromagnetic Fields from Wireless Power Transmission Systems. Proc. IEEE 2013, 101, 1482–1493. [Google Scholar] [CrossRef]
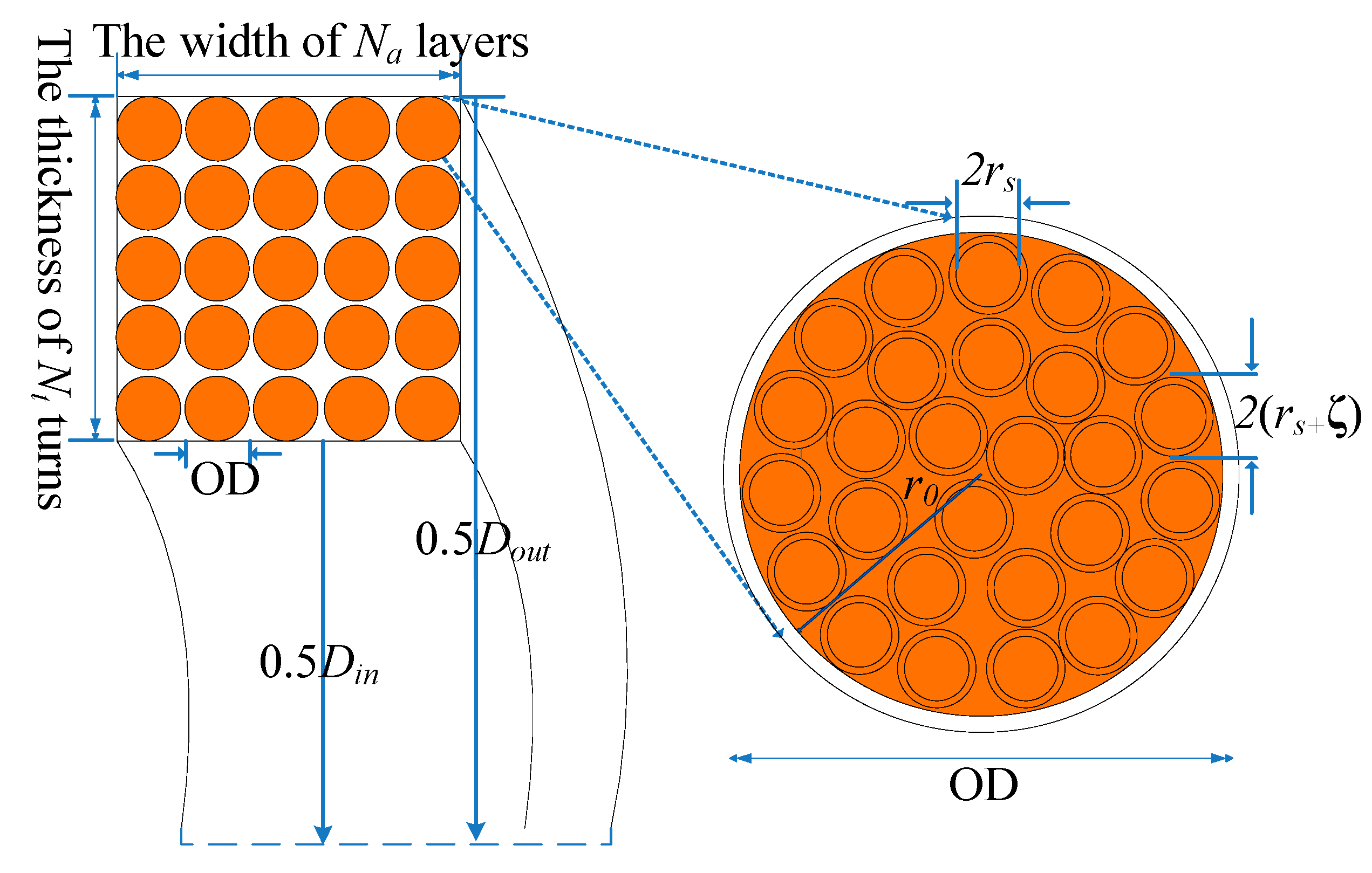

| Radius of wire per strand, rs | 19.9 [µm] |
| Number of strands, Ns | 20 |
| Area efficiency, β | 55% |
| Conductivity, σ | 58 [S/mm2] |
| Isolation Thickness, ζ | 3 [µm] |
| Inner radius, r0 | 110 [µm] |
| Relative permittivity, εr | 3 |
| Coil Num. | Inductance M (2.5 MHz) | Inductance S (2.5 MHz) | Resistance M (2.5 MHz) | Q-factor M (2.5 MHz) | Q-factor S (2.5MHz) |
|---|---|---|---|---|---|
| 1 | 55 uH | 49 uH | 11 Ω | 82 | 90 |
| 2 | 53 uH | 46 uH | 11 Ω | 78 | 87 |
| 3 | 34 uH | 27 uH | 5 Ω | 95 | 112 |
| Type | Coil Num. | Outer Dia. (mm) | Inner Dia. (mm) | Turn/Layers Nt | Layers Na | DC Resistance (Ω) | Capacitance (pF) |
|---|---|---|---|---|---|---|---|
| Driving Coil | 1 | 21 | 12 | 13 | 4 | 2.2 | 110 |
| Driving Coil | 2 | 21 | 12 | 13 | 4 | 2.5 | 110 |
| Load Coil | 3 | 12 | 6 | 11 | 5 | 1.8 | 200 |
| Ref. | Design | Size (cm) | Frequency (MHz) | Distance (λ·10−3) | PTE |
|---|---|---|---|---|---|
| [10] | 2T-1R | Tx: π × 1.52 Rx: π × 1.752 | 6.76 | 0.34 | 17% |
| [11] | 1T-2R | Tx: π × 2.152 Rx: π × 0.52 | 13.56 | 0.45 | 78.6% |
| [17] | 1T-2R | Tx: 35 × 30 Rx: 31.5 × 22.5 | 0.66 | 0.35 | 59.7% |
| This work | 2T-1R | Tx: π × 0.62 Rx: π × 0.32 | 2.9 | 0.05 | 74% |
| This work | 2T-1R | Tx: π × 0.62 Rx: π × 0.32 | 2.9 | 0.2 | 38% |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, C.; Wang, B.; Huang, R.; Yi, Y. A Resonant Coupling Power Transfer System Using Two Driving Coils. Energies 2019, 12, 2914. https://doi.org/10.3390/en12152914
Li C, Wang B, Huang R, Yi Y. A Resonant Coupling Power Transfer System Using Two Driving Coils. Energies. 2019; 12(15):2914. https://doi.org/10.3390/en12152914
Chicago/Turabian StyleLi, Changping, Bo Wang, Ruining Huang, and Ying Yi. 2019. "A Resonant Coupling Power Transfer System Using Two Driving Coils" Energies 12, no. 15: 2914. https://doi.org/10.3390/en12152914
APA StyleLi, C., Wang, B., Huang, R., & Yi, Y. (2019). A Resonant Coupling Power Transfer System Using Two Driving Coils. Energies, 12(15), 2914. https://doi.org/10.3390/en12152914





