Investigation on the Impact of Air Admission in a Prototype Francis Turbine at Low-Load Operation
Abstract
1. Introduction
2. Prototype Site Measurements
2.1. Prototype Unit and Experimental Setup
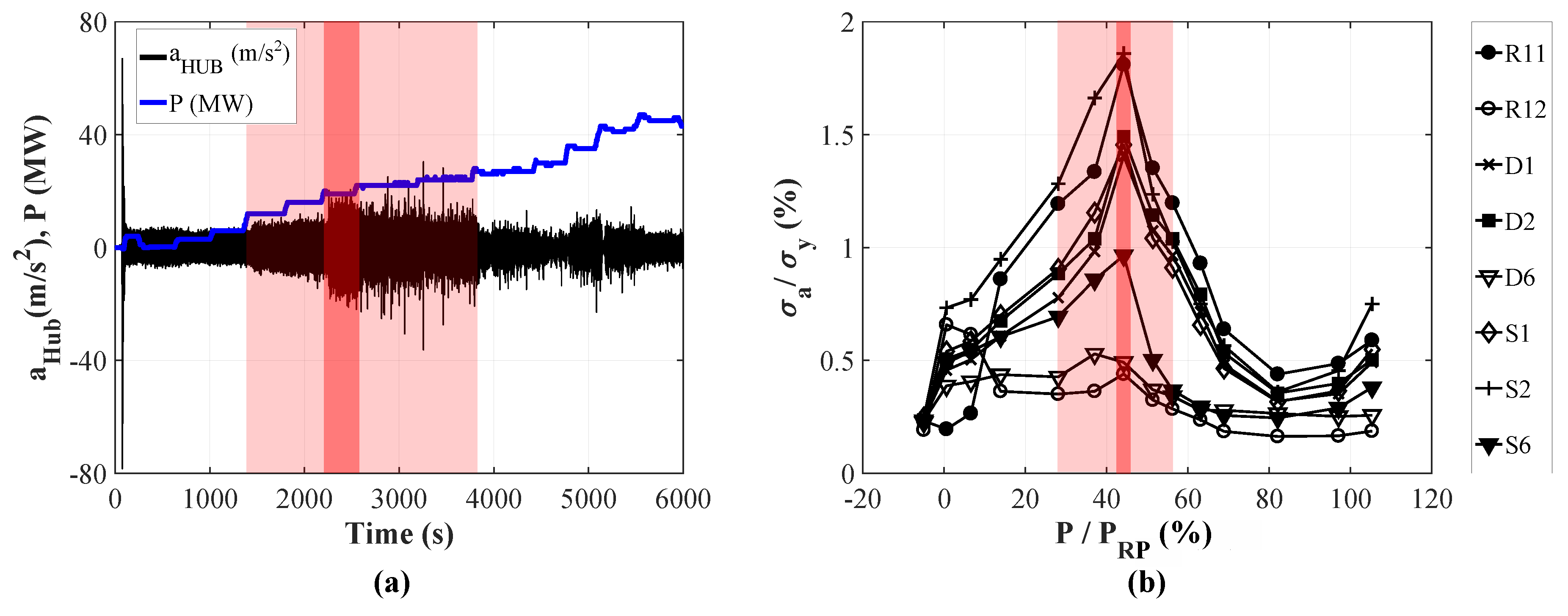
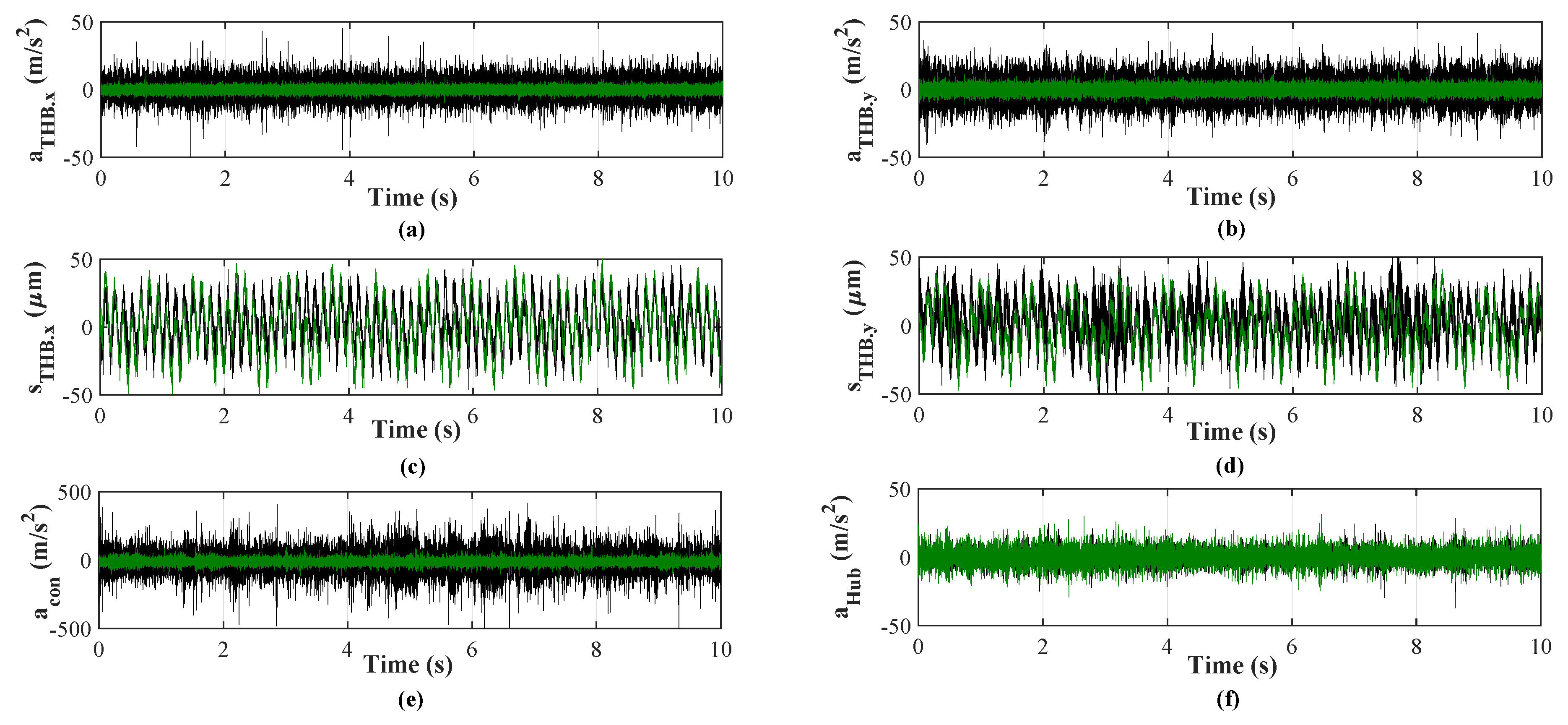
2.2. Measurement Procedure and Plant Behavior
3. Numerical Model
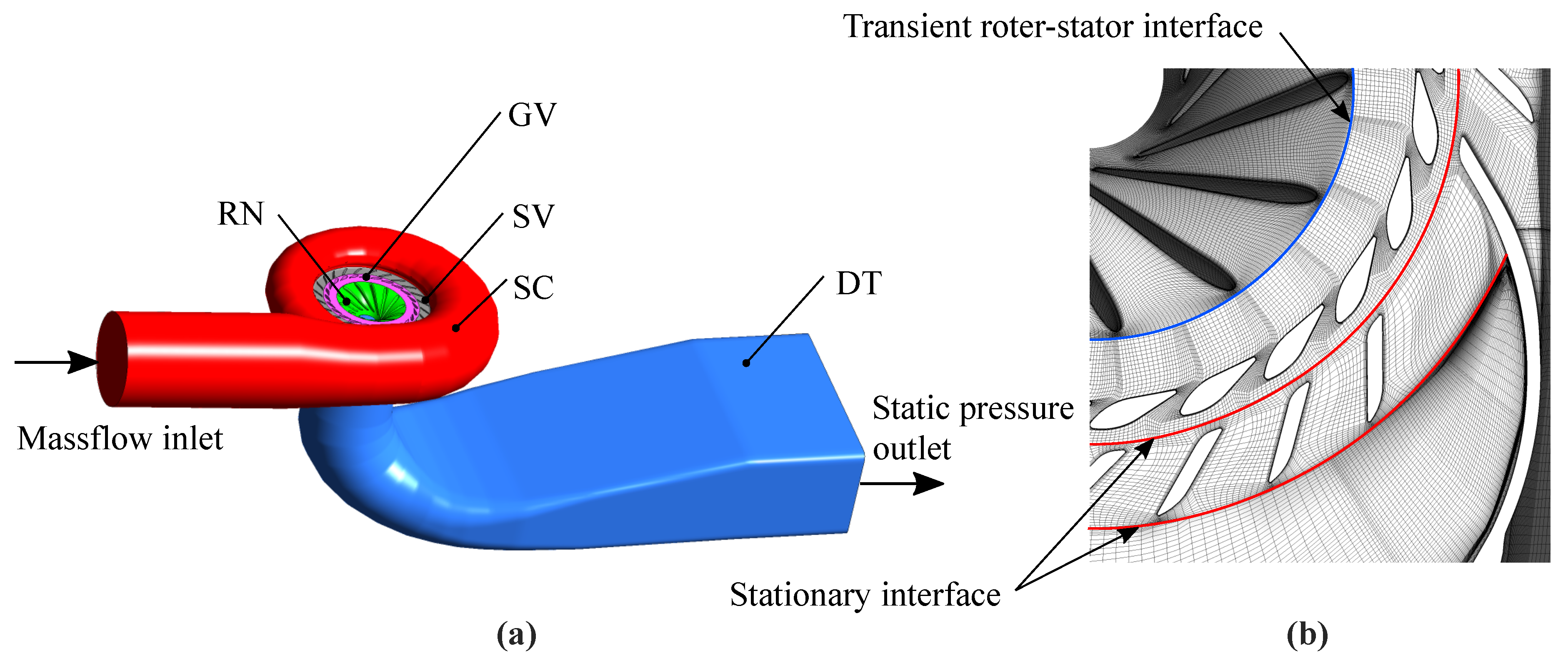
3.1. Geometry and Mesh
3.2. CFD-Setup
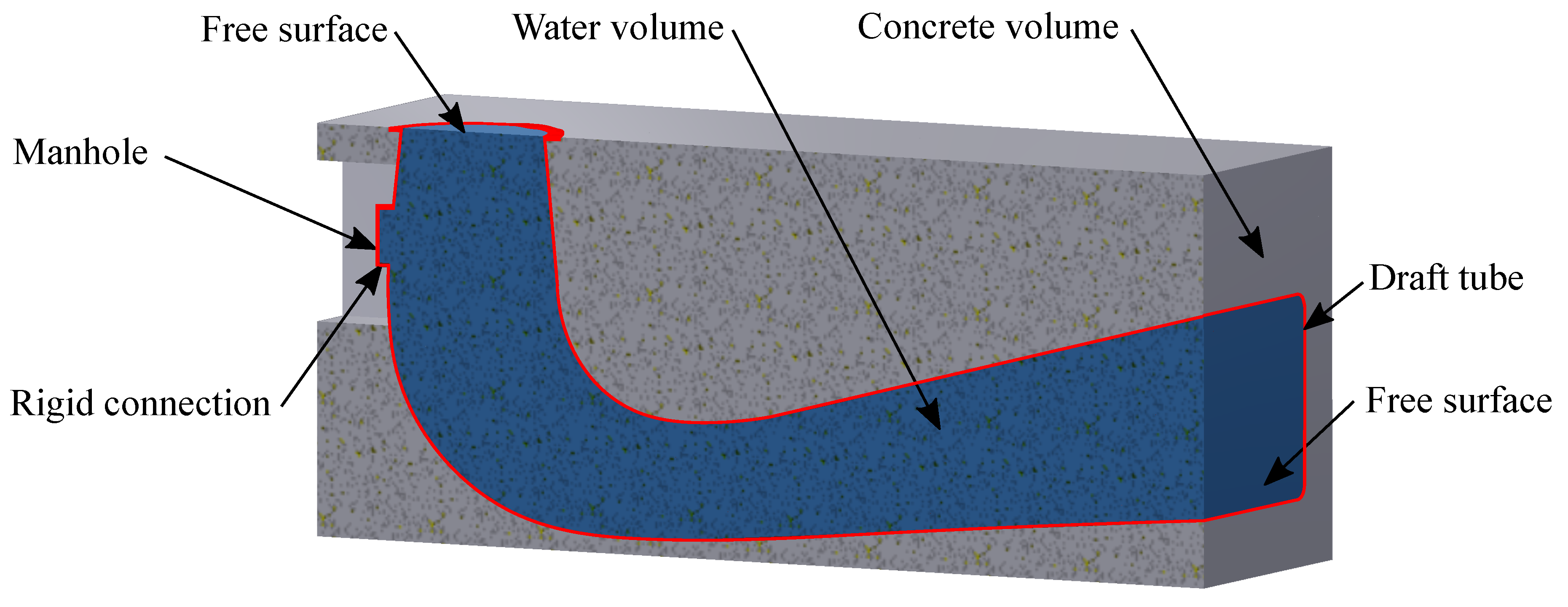
3.3. FEM-Setup
3.3.1. Modal Analysis
3.3.2. Transient FEM Analysis
3.4. Life Expectancy
4. Results and Discussion
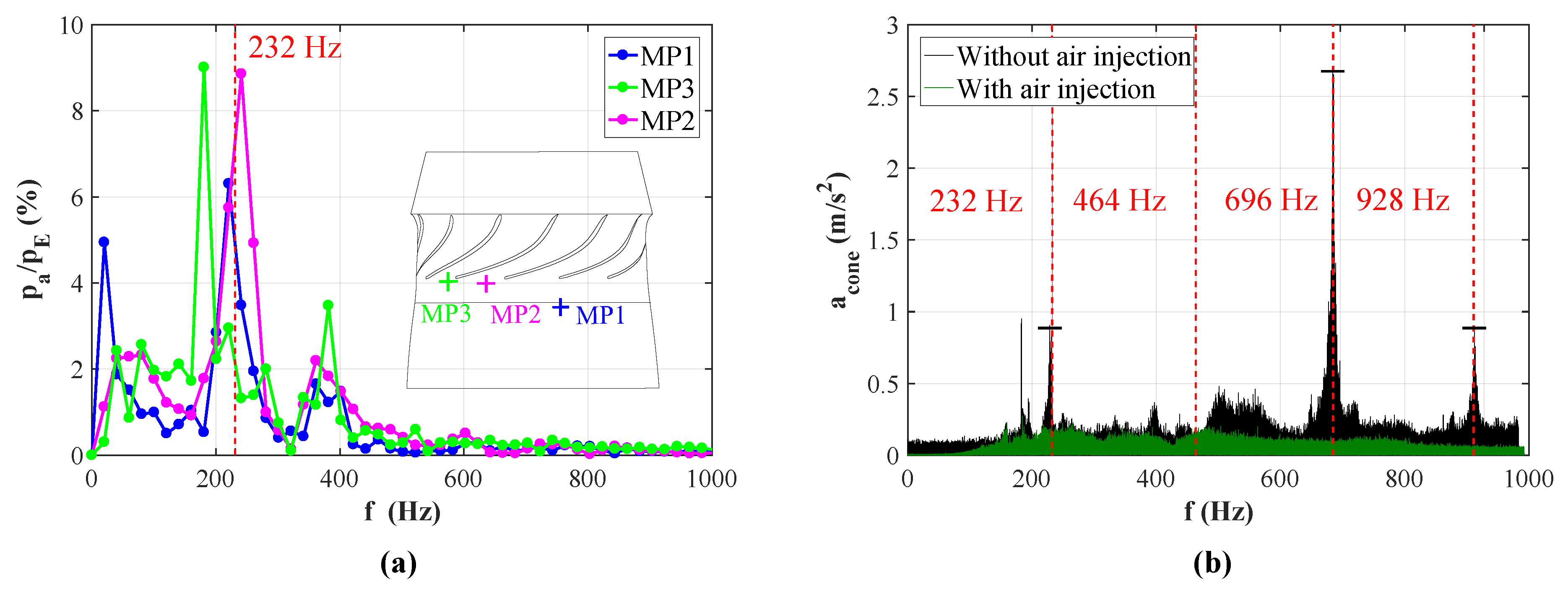
4.1. Cavitation Behavior and Pressure Oscillations
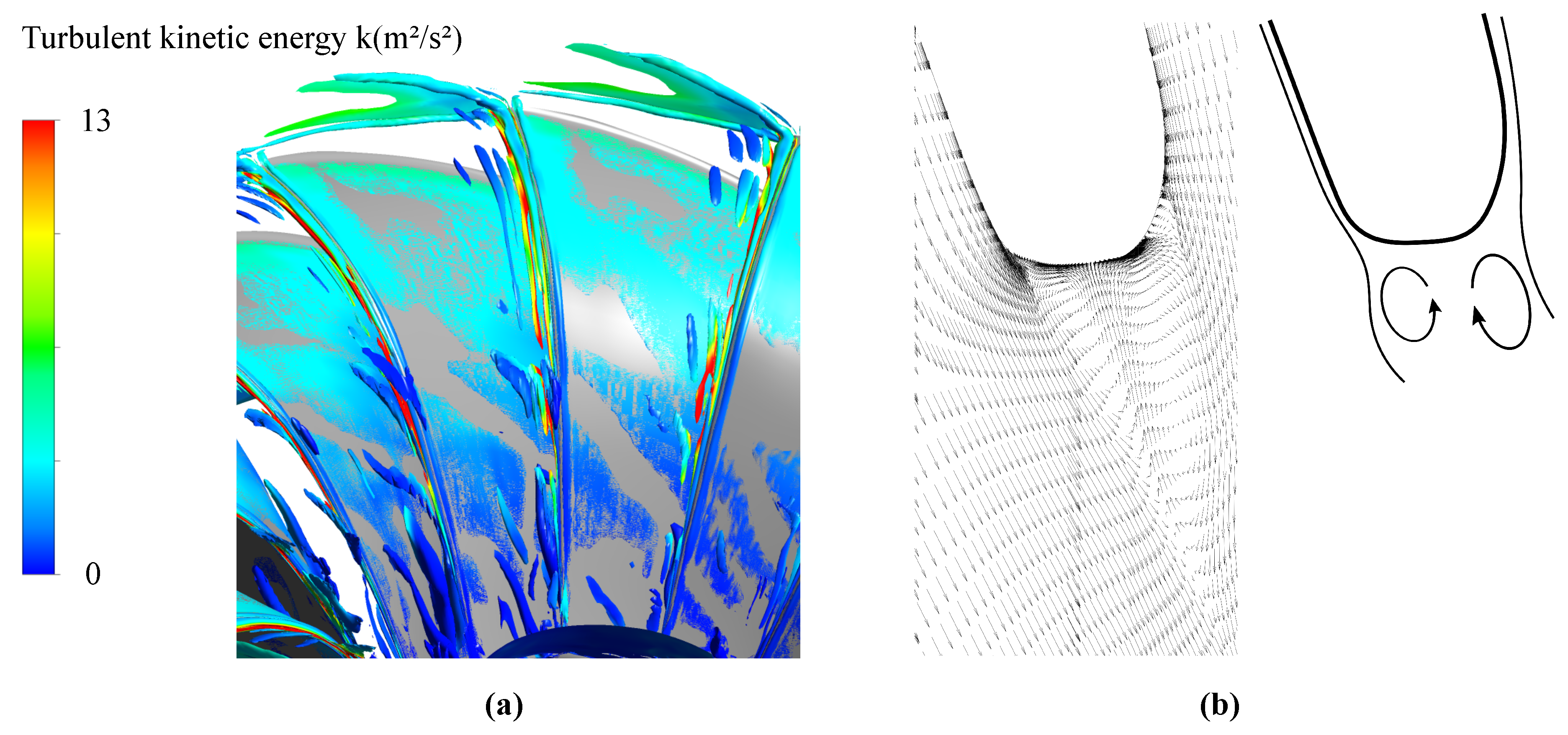
4.2. Trailing Edge Vortex Shedding
4.3. Modal Analysis of the Draft Tube
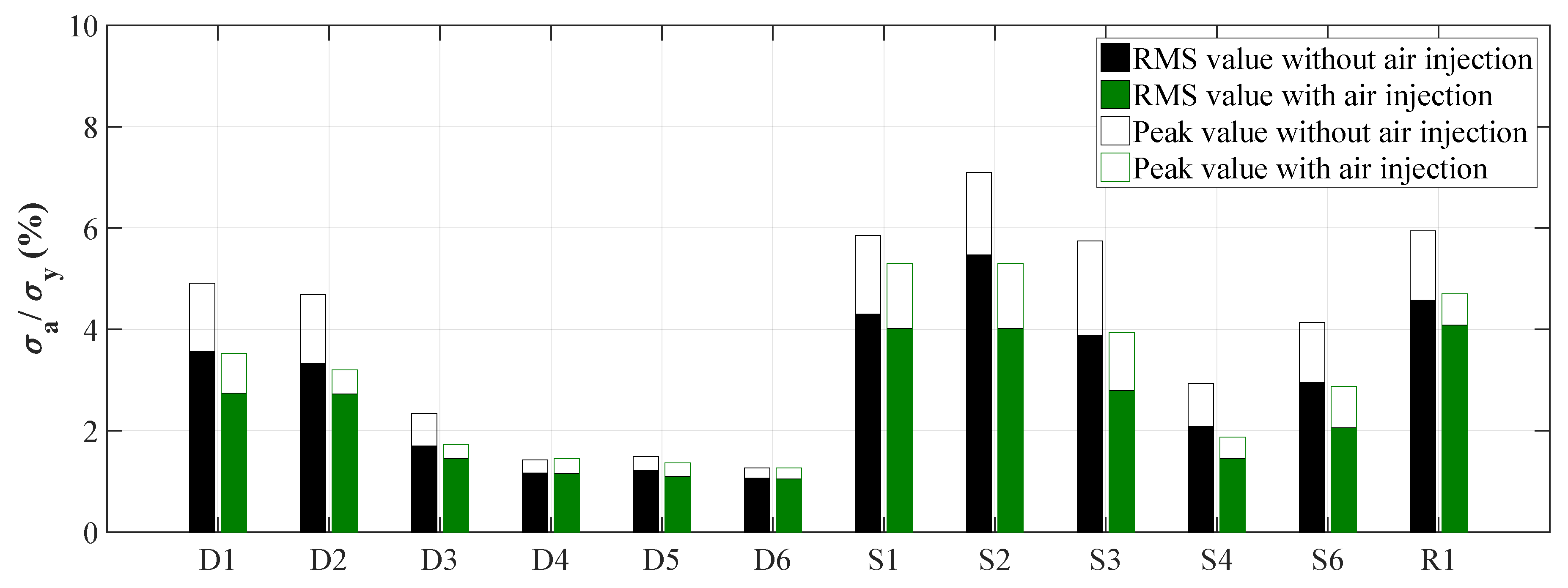
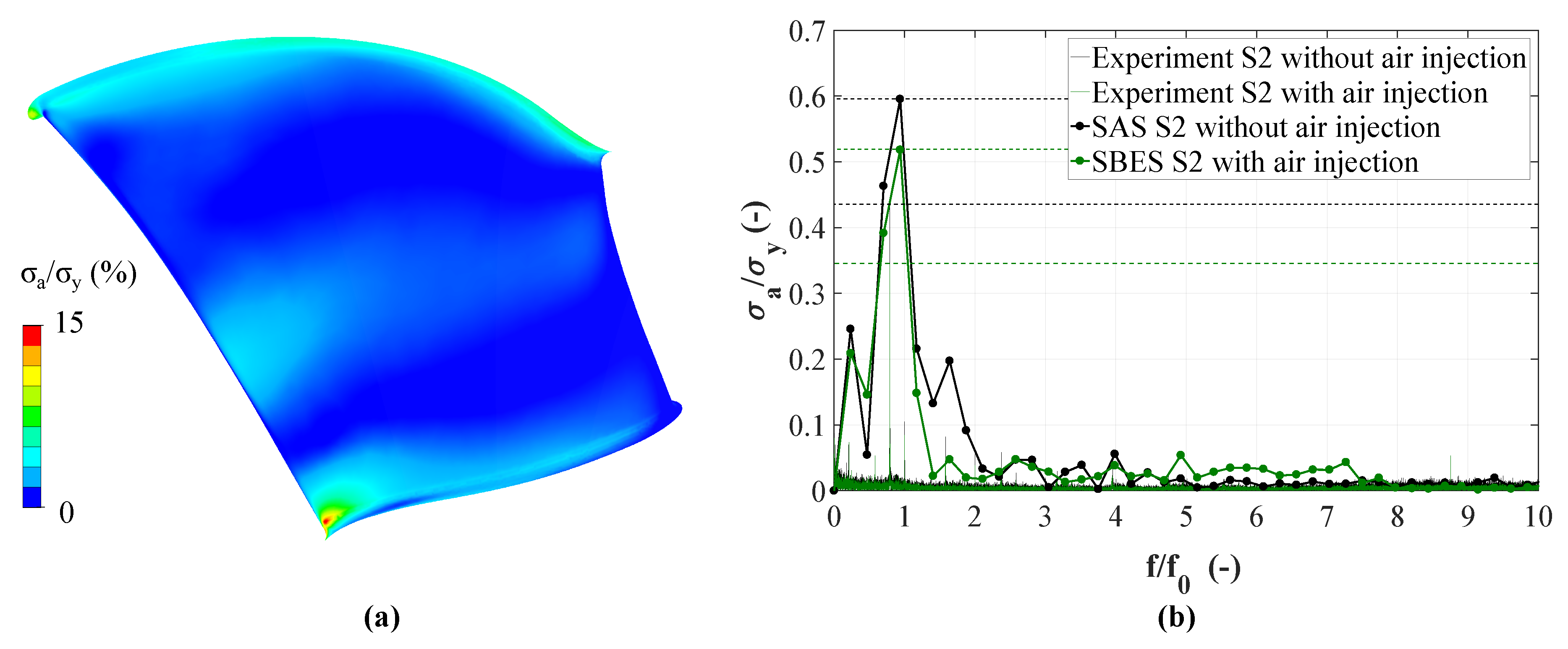
4.4. Fatigue Damage and Dynamical Stress
5. Conclusions
- A huge draft tube vortex, with a frequency corresponding to approximately , is the most concerning fact in terms of high dynamical stress and runner fatigue damage.
- Both turbulence models showed quite similar behavior and a good agreement with the measurement. However, in contrast to single flow analysis the pressure amplitudes of the simulation tend to be higher than the ones obtained by the sensor.
- An improved runner model, targeting separation effects, showed the appearance of trailing edge vortex shedding.
- A simplified modal analysis of the draft tube confirmed that the vibrations of the machine set are related to vortex shedding.
- The air injection not only significantly reduced the vibrations of the machine set and might have a positive effect on cavitation, but also improved runner fatigue life.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| Acronyms | |
| CDS | Central deference scheme |
| CFD | Computational fluid dynamics |
| CFL | Courant number |
| D | Pressure side |
| DES | Detached Eddy Simulation |
| DT | Draft tube |
| GGI | General grid interface |
| FEM | Finite element method |
| FFT | Fast-Fourier-Transformation |
| FSI | Fluid-structure-interaction |
| GV | Guide vanes |
| GVA | Guide vanes with inlets for air injection |
| LES | Large Eddy Simulation |
| MP | Monitor point |
| RANS | Reynolds averaged Navier-Stokes equations |
| R1 | T-rosette |
| RMS | root mean square |
| Acronyms | |
| RN | Runner |
| RNVS | Refined runner domain |
| RP | Rated point |
| S | Suction side |
| SAS | Scale Adaptive Simulation |
| SBES | Stress Blended Eddy Simulation |
| SC | Spiral casing |
| SRS | Scale-Resolving Simulation |
| SST | Shear stress transport |
| SV | Stay vanes |
| URANS | Unsteady RANS |
| VIV | Vortex-induced-vibrations |
| Greek Symbols | |
| Rayleigh-Parameter, () | |
| Rayleigh-Parameter, () | |
| Water density, () | |
| Vapor density, () | |
| Stress amplitude, () | |
| Yield strength, () | |
| Damping ratio, (-) | |
| Frequency limit, () | |
| Frequency limit, () | |
| Latin Symbols | |
| Acceleration measured at the draft tube cone, () | |
| Acceleration measured at the hollow hub, () | |
| Acceleration measured at the turbine bearing, () | |
| Damping marix, () | |
| C | Damage factor, (-) |
| D | Outer diameter of the runner, (m) |
| Empirical factor for condensation, (-) | |
| Empirical factor for vapor, (-) | |
| f | Frequency, () |
| Rotational frequency, () | |
| Vortex shedding frequency, () | |
| g | Gravity constant, () |
| H | Turbine head, (m) |
| Stiffness marix, () | |
| k | Turbulent kinetic energy, () |
| L | Characteristic lateral dimension, (m) |
| Mass marix, () | |
| Interphase mass transfer rate, () | |
| Number of load cycles until the S-N curve is reached, (-) | |
| Speed factor, (-) | |
| Number of load cycles, (-) | |
| P | Power, () |
| Rated power, () | |
| p | Pressure, () |
| Pressure amplitude, () | |
| Draft tube cone pressure amplitude, () | |
| Pressure in the bubble, () | |
| Draft tube cone pressure, () | |
| Draft tube outlet pressure, () | |
| Dynamic pressure (RN outlet), () | |
| Latin Symbols | |
| Bubble radius, (m) | |
| Nucleation site radius, (m) | |
| Nucleation volume fraction, (-) | |
| Bubble volume fraction, (-) | |
| Strouhal number, (-) | |
| Relative displacement measured at the turbine bearing, (m) | |
| Circumferential velocity (RN outlet), () | |
| v | Velocity, () |
References
- Trivedi, C.; Gandhi, B.; Michel, C.J. Effect of transients on Francis turbine runner life: A review. J. Hydraul. Res. 2013, 51, 121–132. [Google Scholar] [CrossRef]
- Yamamoto, K.; Müller, A.; Favrel, A.; Avellan, F. Experimental evidence of inter-blade cavitation vortex development in Francis turbines at deep part load condition. Exp. Fluids 2017, 58, 142. [Google Scholar] [CrossRef]
- Goyal, R.; Gandhi, B.K. Review of hydrodynamics instabilities in Francis turbine during off-design and transient operations. Renew. Energy 2018, 116, 697–709. [Google Scholar] [CrossRef]
- Xiao, R.; Wang, Z.; Luo, Y. Dynamic stresses in a Francis turbine runner based on fluid-structure interaction analysis. Tsinghua Sci. Technol. 2008, 13, 587–592. [Google Scholar] [CrossRef]
- Trudel, A.; Sabourin, M. Metallurgical and Fatigue Assessments of Welds in Cast Welded Hydraulic Turbine Runners. IOP Conf. Ser. Earth Environ. Sci. 2014, 22, 012015. [Google Scholar] [CrossRef]
- Rheingans, W. Power swings in hydroelectric power plants. Trans. ASME 1940, 62, 171–184. [Google Scholar]
- Seidel, U.; Mende, C.; Hübner, B.; Weber, W.; Otto, A. Dynamic Loads in Francis Runners and Their Impact on Fatigue Life. IOP Conf. Ser. Earth Environ. Sci. 2014, 22, 032054. [Google Scholar] [CrossRef]
- Egusquiza, E.; Valero, C.; Huang, X.; Jou, E.; Guardo, A.; Rodriguez, C. Failure investigation of a large pump-turbine runner. Eng. Fail. Anal. 2012, 23, 27–34. [Google Scholar] [CrossRef]
- Frunzăverde, D.; Muntean, S.; Mărginean, G.; Campian, V.; Marşavina, L.; Terzi, R.; Şerban, V. Failure Analysis of a Francis Turbine Runner. IOP Conf. Ser. Earth Environ. Sci. 2010, 12, 012115. [Google Scholar] [CrossRef]
- Thicke, R. Practical solutions for draft tube instability. Water Power Dam Constr. 1981, 32, 31–37. [Google Scholar]
- Qian, Z.; Li, W.; Huai, W.; Wu, Y. The effect of runner cone design on pressure oscillation characteristics in a Francis hydraulic turbine. Proc. Inst. Mech. Eng. Part A J. Power Energy 2012, 226, 137–150. [Google Scholar] [CrossRef]
- Dörfler, P.; Sick, M.; Coutu, A. Flow-Induced Pulsation and Vibration in Hydroelectric Machinery: Engineer’s Guidebook for Planning, Design and Troubleshooting; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2012. [Google Scholar]
- Muntean, S.; Susan-Resiga, R.F.; Câmpian, V.C.; Dumpravă, C.; Cuzmoş, A. In situ unsteady pressure measurements on the draft tube cone of the Francis turbine with air injection over an extended operating range. UPB Sci. Bull. Ser. D 2014, 76, 173–180. [Google Scholar]
- Nakanishi, K.; Ueda, T. Air supply into draft tube of Francis turbine. Fuji Electr. Rev. 1964, 10, 81–91. [Google Scholar]
- March, P. Hydraulic and environmental performance of aerating turbine technologies. In EPRI-DOE Conference on Environmentally-Enhanced Hydropower Turbines: Technical Papers; EPRI: Palo Alto, CA, USA; U.S. Department of Energy: Washington, DC, USA, 2011; pp. 19–21. [Google Scholar]
- Dörfler, P.; Bloch, R.; Mayr, W.; Hasler, O. Vibration tests on a high-head (740 m) Francis turbine: Field test results from Hausling. In Proceedings of the 14th IAHR Symposium on Hydraulic Machinery and Cavitation, Trondheim, Norway, 20–23 June 1988; pp. 241–252. [Google Scholar]
- Von Fellenberg, S.; Häussler, W.; Michler, W. High-Pressure Air Injection on a Low-Head Francis Turbine. IOP Conf. Ser. Earth Environ. Sci. 2014, 22, 012031. [Google Scholar] [CrossRef]
- Zhang, Y.; Zheng, X.; Li, J.; Du, X. Experimental study on the vibrational performance and its physical origins of a prototype reversible pump turbine in the pumped hydro energy storage power station. Renew. Energy 2019, 130, 667–676. [Google Scholar] [CrossRef]
- Chen, T.; Zhang, Y.; Li, S. Instability of large-scale prototype Francis turbines of Three Gorges power station at part load. Proc. Inst. Mech. Eng. Part A J. Power Energy 2016, 230, 619–632. [Google Scholar] [CrossRef]
- Chirkov, D.; Shcherbakov, P.; Cherny, S.; Skorospelov, V.; Turuk, P. Numerical investigation of the air injection effect on the cavitating flow in Francis hydro turbine. Thermophys. Aeromech. 2017, 24, 691–703. [Google Scholar] [CrossRef]
- Unterluggauer, J.; Doujak, E.; Bauer, C. Numerical fatigue analysis of a prototype Francis turbine runner in low-load operation. In Proceedings of the European Turbomachinery Conference, Laussanne, Switzerland, 8–12 April 2019; pp. 1–12. [Google Scholar]
- Eichhorn, M.; Taruffi, A.; Bauer, C. Expected load spectra of prototype Francis turbines in low-load operation using numerical simulations and site measurements. J. Phys. Conf. Ser. 2017, 813, 012052. [Google Scholar] [CrossRef]
- International Electrotechnical Commission. Hydraulic Turbines, Storage Pumps and Pump-Turbines-Model Acceptance Tests: IEC 60193: 1999; International Electrotechnical Commission: Geneva, Switzerland, 1999. [Google Scholar]
- Unterluggauer, J.; Doujak, E.; Bauer, C. Fatigue analysis of a prototype Francis turbine based on strain gauge measurements. In 20th International Seminar on Hydropower Plants; Technische Universität Wien Eigenverlag: Vienna, Austria, 2018. [Google Scholar]
- Papillon, B.; Sabourin, M.; Couston, M.; Deschenes, C. Methods for air admission in hydro turbines. In Proceedings of the 21st IAHR Symposium on Hydraulic Machinery and Systems, Lausanne, Switzerland, 9–12 September 2002; pp. 1–6. [Google Scholar]
- Qian, Z.D.; Yang, J.D.; Huai, W.X. Numerical simulation and analysis of pressure pulsation in Francis hydraulic turbine with air admission. J. Hydrodyn. 2007, 19, 467–472. [Google Scholar] [CrossRef]
- Celik, B.; Ghia, U.; Roache, P.; Freitas, C.; Coleman, H.; Raad, P. Procedure for Estimation and Reporting of Uncertainty Due to Discretization in CFD Applications. J. Fluids Eng. 2008, 130, 78001. [Google Scholar] [CrossRef]
- Menter, F. Zonal two equation k-w turbulence models for aerodynamic flows. In Proceedings of the 23rd Fluid Dynamics, Plasma Dynamics, and Lasers Conference, Orlando, FL, USA, 6–9 July 1993; p. 2906. [Google Scholar]
- Menter, F.; Egorov, Y. The scale-adaptive simulation method for unsteady turbulent flow predictions. Part 1: Theory and model description. Flow Turbul. Combust. 2010, 85, 113–138. [Google Scholar] [CrossRef]
- Menter, F. Stress-Blended Eddy Simulation (SBES)—A New Paradigm in Hybrid RANS-LES Modeling. In Symposium on Hybrid RANS-LES Methods; Springer: Berlin/Heidelberg, Germany, 2016; pp. 27–37. [Google Scholar]
- Menter, F. Stress-Blended Eddy Simulation (SBES)—A New Paradigm in Hybrid RANS-LES Modeling. In Progress in Hybrid RANS-LES Modelling; Hoarau, Y., Peng, S.H., Schwamborn, D., Revell, A., Eds.; Springer International Publishing: Cham, Switzerland, 2018; pp. 27–37. [Google Scholar]
- Plesset, M.S.; Prosperetti, A. Bubble dynamics and cavitation. Annu. Rev. Fluid Mech. 1977, 9, 145–185. [Google Scholar] [CrossRef]
- Bakir, F.; Rey, R.; Gerber, A.; Belamri, T.; Hutchinson, B. Numerical and experimental investigations of the cavitating behavior of an inducer. Int. J. Rotating Mach. 2004, 10, 15–25. [Google Scholar] [CrossRef]
- Mössinger, P.; Conrad, P.; Jung, A. Transient Two-Phase CFD Simulation of Overload Pressure Pulsation in a Prototype Sized Francis Turbine Considering the Waterway Dynamics. IOP Conf. Ser. Earth Environ. Sci. 2014, 22, 032033. [Google Scholar] [CrossRef]
- Nocker, A. Modal Analysis of a Concreted Draft Tube of a Francis Machine. Bachelor’s Thesis, Technical University of Vienna, Vienna, Austria, 2019. [Google Scholar]
- De Boer, A.; van Zuijlen, A.H.; Bijl, H. Comparison of conservative and consistent approaches for the coupling of non-matching meshes. Comput. Methods Appl. Mech. Eng. 2008, 197, 4284–4297. [Google Scholar] [CrossRef]
- Thévenaz, P.; Blu, T.; Unser, M. Interpolation revisited [medical images application]. IEEE Trans. Med. Imaging 2000, 19, 739–758. [Google Scholar] [CrossRef] [PubMed]
- Zhu, W.; Xiao, R.; Yang, W.; Liu, J.; Wang, F. Study on Stress Characteristics of Francis Hydraulic Turbine Runner Based on Two-Way FSI. IOP Conf. Ser. Earth Environ. Sci. 2012, 15, 052016. [Google Scholar] [CrossRef]
- Morissette, J.; Chamberland-Lauzon, J.; Nennemann, B.; Monette, C.; Giroux, A.; Coutu, A.; Nicolle, J. Stress Predictions in a Francis Turbine at No-Load Operating Regime. IOP Conf. Ser. Earth Environ. Sci. 2016, 49, 072016. [Google Scholar] [CrossRef]
- Gülich, J.F. Centrifugal Pumps; Springer: Berlin/Heidelberg, Germany, 2008; Volume 2. [Google Scholar]
- Maly, A.; Eichhorn, M.; Bauer, C. Experimental investigation of transient pressure effects in the side chambers of a reversible pump turbine model. In 19. Internationales Seminar Wasserkraftanlagen//19th International Seminar on Hydropower Plants; Technische Universität Wien, Ed.; Technische Universität Wien Eigenverlag: Vienna, Austria, 2016; pp. 675–684. [Google Scholar]
- Clough, R.W.; Penzien, J. Dynamics of Structures; Computers & Structures, Inc.: Berkeley, CA, USA, 1995. [Google Scholar]
- Chowdhury, I.; Dasgupta, S.P. Computation of Rayleigh damping coefficients for large systems. Electron. J. Geotech. Eng. 2003, 8, 1–11. [Google Scholar]
- Rodriguez, C.; Egusquiza, E.; Escaler, X.; Liang, Q.; Avellan, F. Experimental investigation of added mass effects on a Francis turbine runner in still water. J. Fluids Struct. 2006, 22, 699–712. [Google Scholar] [CrossRef]
- Magnoli, M.V. Numerical Simulation of Pressure Oscillations in Large Francis Turbines at Partial and Full Load Operating Conditions and Their Effects on the Runner Structural Behaviour and Fatigue Life. Ph.D. Thesis, Technische Universität München, Munich, Germany, 2014. [Google Scholar]
- Flores, M.; Urquiza, G.; Rodríguez, J.M. A fatigue analysis of a hydraulic Francis turbine runner. World J. Mech. 2012, 2, 28–34. [Google Scholar] [CrossRef]
- Johannesson, P. Extrapolation of load histories and spectra. Fatigue Fract. Eng. Mater. Struct. 2006, 29, 209–217. [Google Scholar] [CrossRef]
- Gagnon, M.; Tahan, S.; Bocher, P.; Thibault, D. The Role of High Cycle Fatigue (HCF) Onset in Francis Runner Reliability. IOP Conf. Ser. Earth Environ. Sci. 2012, 15, 022005. [Google Scholar] [CrossRef]
- Thibault, D.; Gagnon, M.; Godin, S. The effect of materials properties on the reliability of hydraulic turbine runners. Int. J. Fluid Mach. Syst. 2015, 8, 254–263. [Google Scholar] [CrossRef]
- Jošt, D.; Škerlavaj, A.; Morgut, M.; Nobile, E. Numerical prediction of cavitating vortex rope in a draft tube of a Francis turbine with standard and calibrated cavitation model. J. Phys. Conf. Ser. 2017, 813, 012045. [Google Scholar] [CrossRef]
- Goldwag, E.; Berry, D. Von Karman hydraulic vortexes cause stay vane cracking on propeller turbines at the little long generating station of Ontario hydro. J. Eng. Power 1968, 90, 213–217. [Google Scholar]
- Trivedi, C. Compressible large eddy simulation of a Francis turbine during speed-no-load: Rotor stator interaction and inception of a vortical flow. J. Eng. Gas Turbines Power 2018, 140, 112601. [Google Scholar] [CrossRef]
- Fisher, R.K.; Seidel, U.; Grosse, G.; Gfeller, W.; Klinger, R. A case study in resonant hydroelastic vibration: the causes of runner cracks and the solutions implemented for the Xiaolangdi hydroelectric project. In Proceedings of the XXI IAHR Symposium on Hydraulic Machinery and Systems, Lausanne, Switzerland, 9–12 September 2002. [Google Scholar]
- Koopmann, G. The vortex wakes of vibrating cylinders at low Reynolds numbers. J. Fluid Mech. 1967, 28, 501–512. [Google Scholar] [CrossRef]

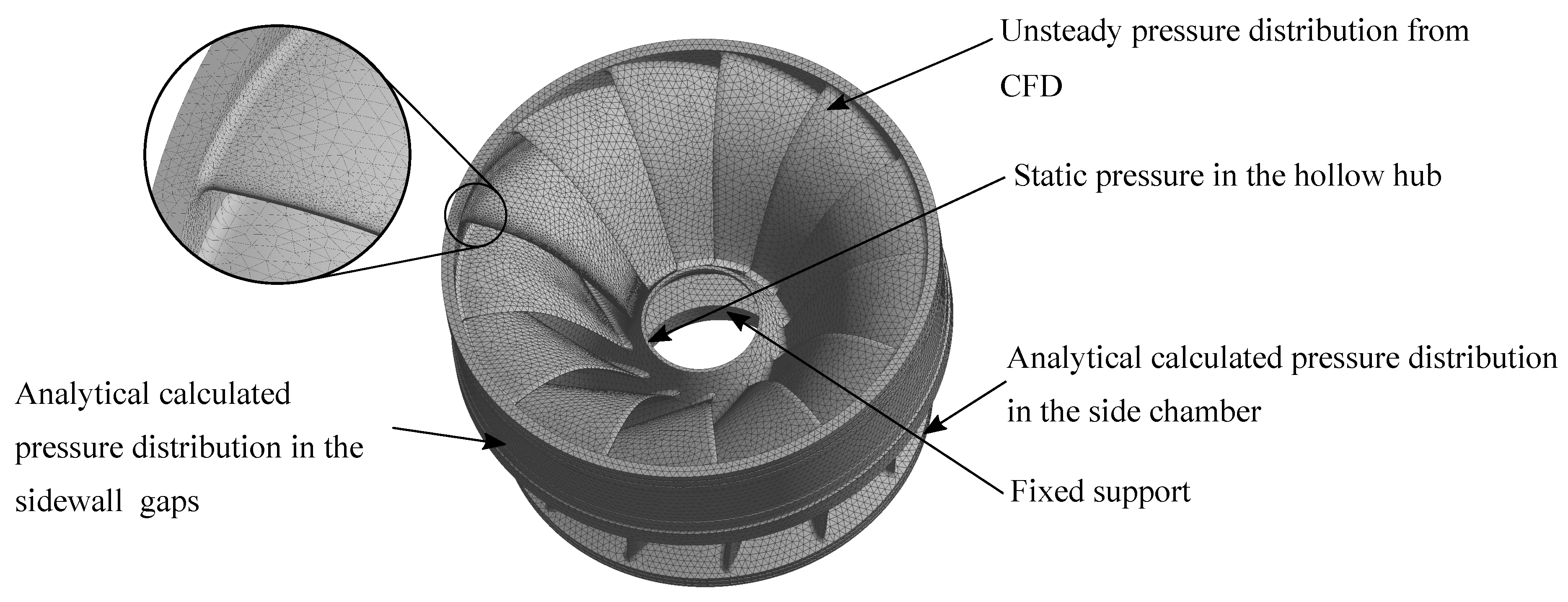
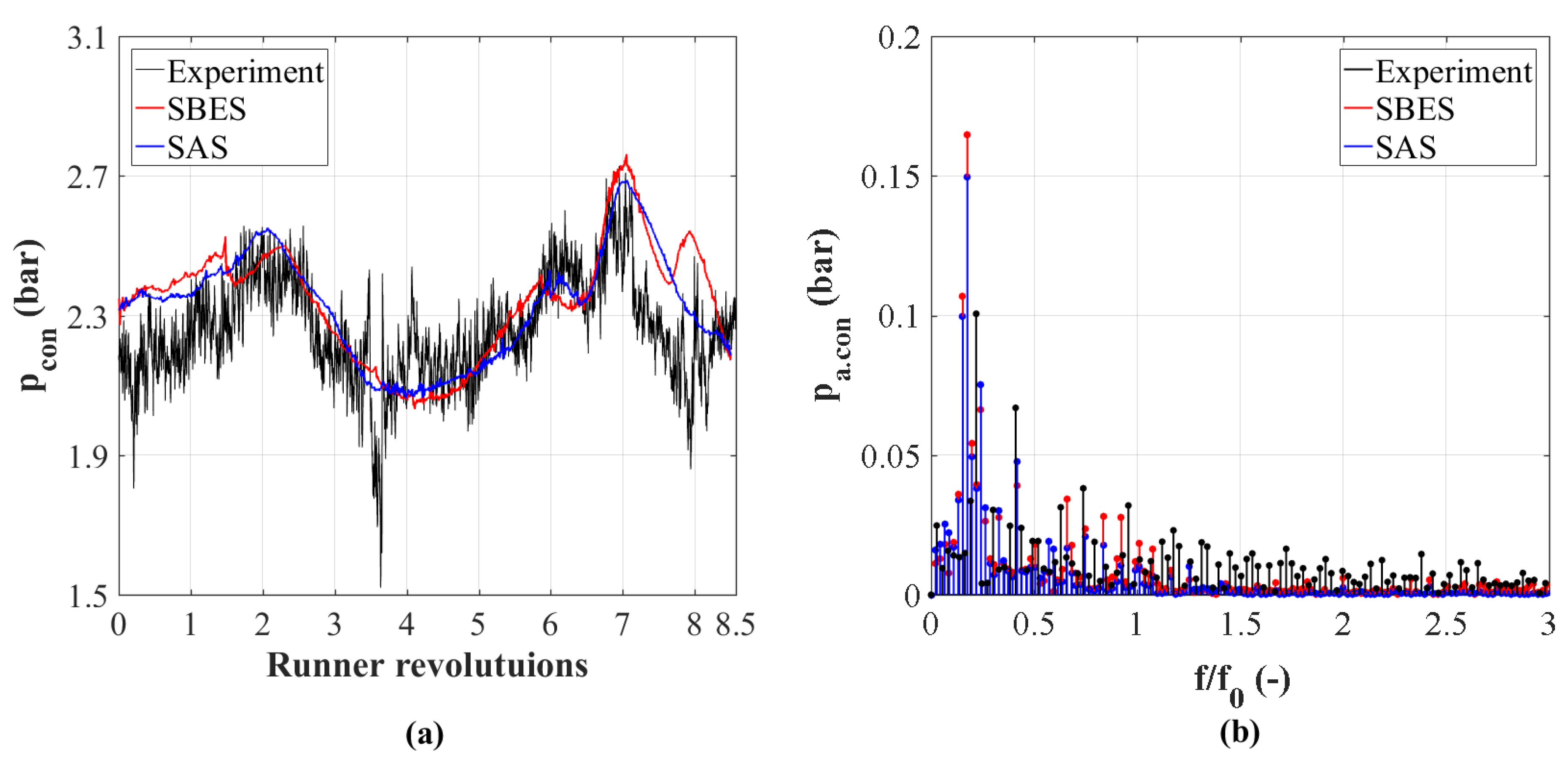
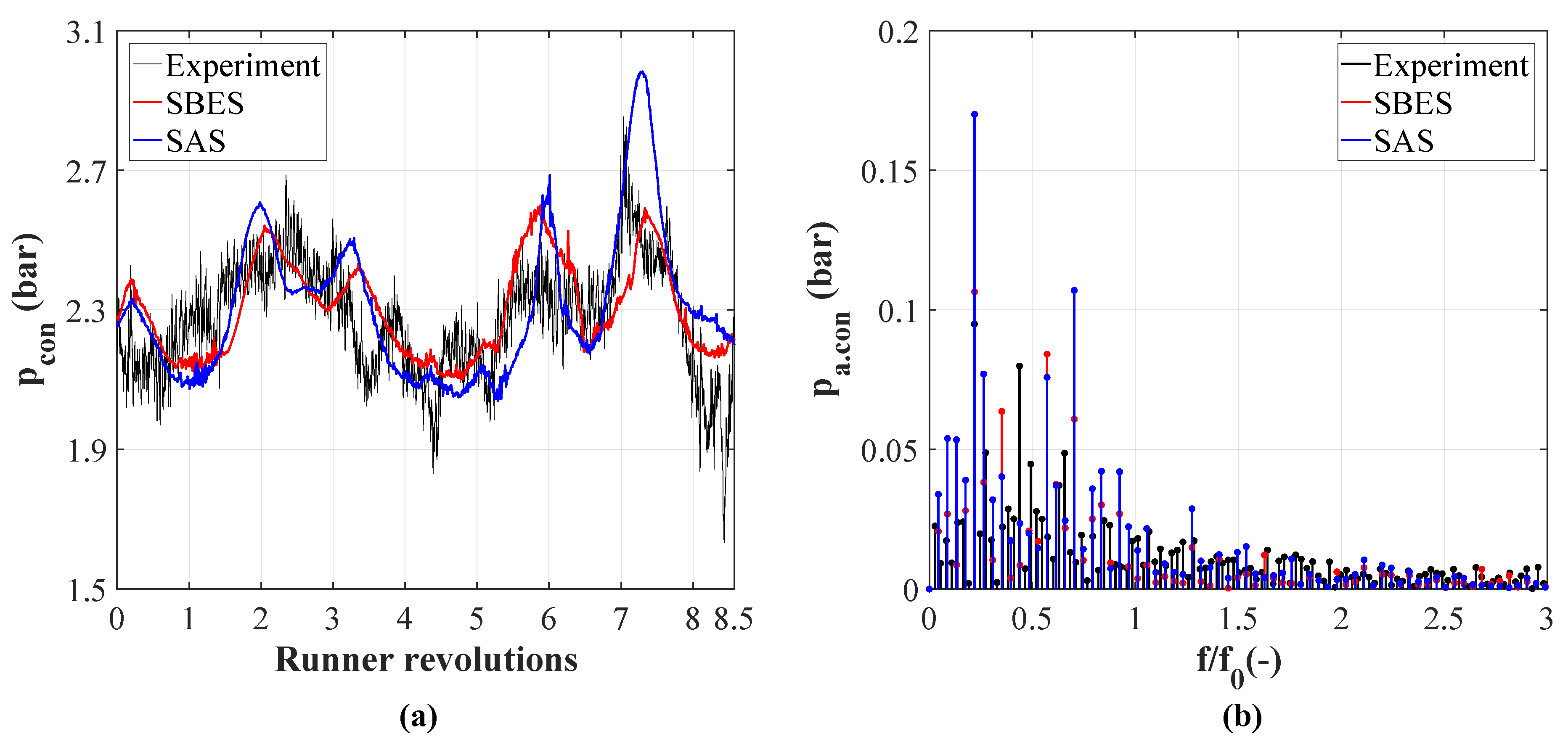
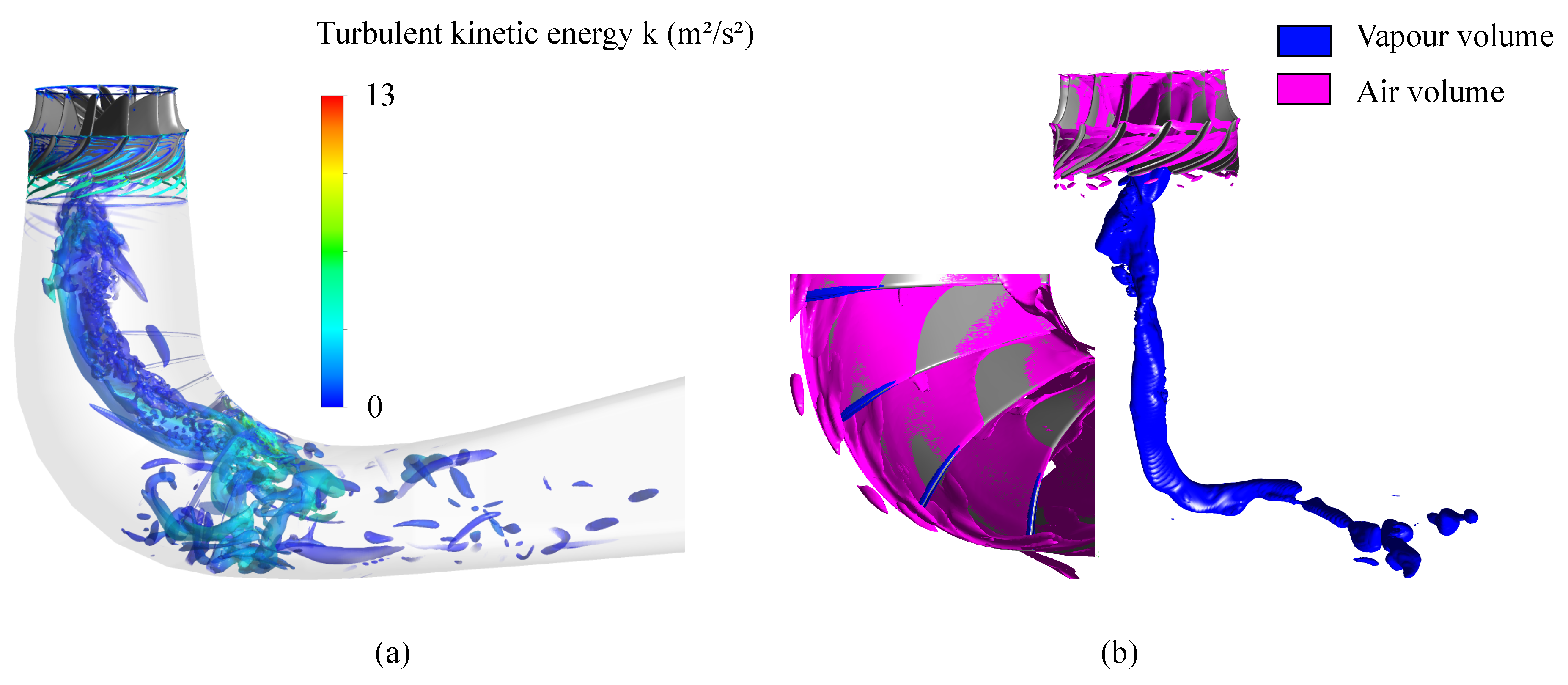


| Domain | SC | SV | GV | RN | DT | GVA | RN VS |
|---|---|---|---|---|---|---|---|
| Number of cells (million) | 20 | ||||||
| Minimum determinant (-) | |||||||
| Minimum angle () | 27 | ||||||
| (-) | 32 |
| Description Parameters | Parameters |
|---|---|
| Damping ratio (-) | |
| Low limit frequency (Hz) | 1 |
| Upper limit frequency (Hz) | |
| Rayleigh coefficient (-) | |
| Rayleigh coefficient (-) |
| Description Parameters | Parameters |
|---|---|
| Material (-) | 13 4 |
| Survival probability (%) | |
| Mean stress (N/mm) | 150 |
| Logarithmic standard deviation of (-) | |
| Stress ratio (-) | 1 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Unterluggauer, J.; Maly, A.; Doujak, E. Investigation on the Impact of Air Admission in a Prototype Francis Turbine at Low-Load Operation. Energies 2019, 12, 2893. https://doi.org/10.3390/en12152893
Unterluggauer J, Maly A, Doujak E. Investigation on the Impact of Air Admission in a Prototype Francis Turbine at Low-Load Operation. Energies. 2019; 12(15):2893. https://doi.org/10.3390/en12152893
Chicago/Turabian StyleUnterluggauer, Julian, Anton Maly, and Eduard Doujak. 2019. "Investigation on the Impact of Air Admission in a Prototype Francis Turbine at Low-Load Operation" Energies 12, no. 15: 2893. https://doi.org/10.3390/en12152893
APA StyleUnterluggauer, J., Maly, A., & Doujak, E. (2019). Investigation on the Impact of Air Admission in a Prototype Francis Turbine at Low-Load Operation. Energies, 12(15), 2893. https://doi.org/10.3390/en12152893





