Challenges in the Electromagnetic Modeling of Road Embedded Wireless Power Transfer
Abstract
:1. Introduction
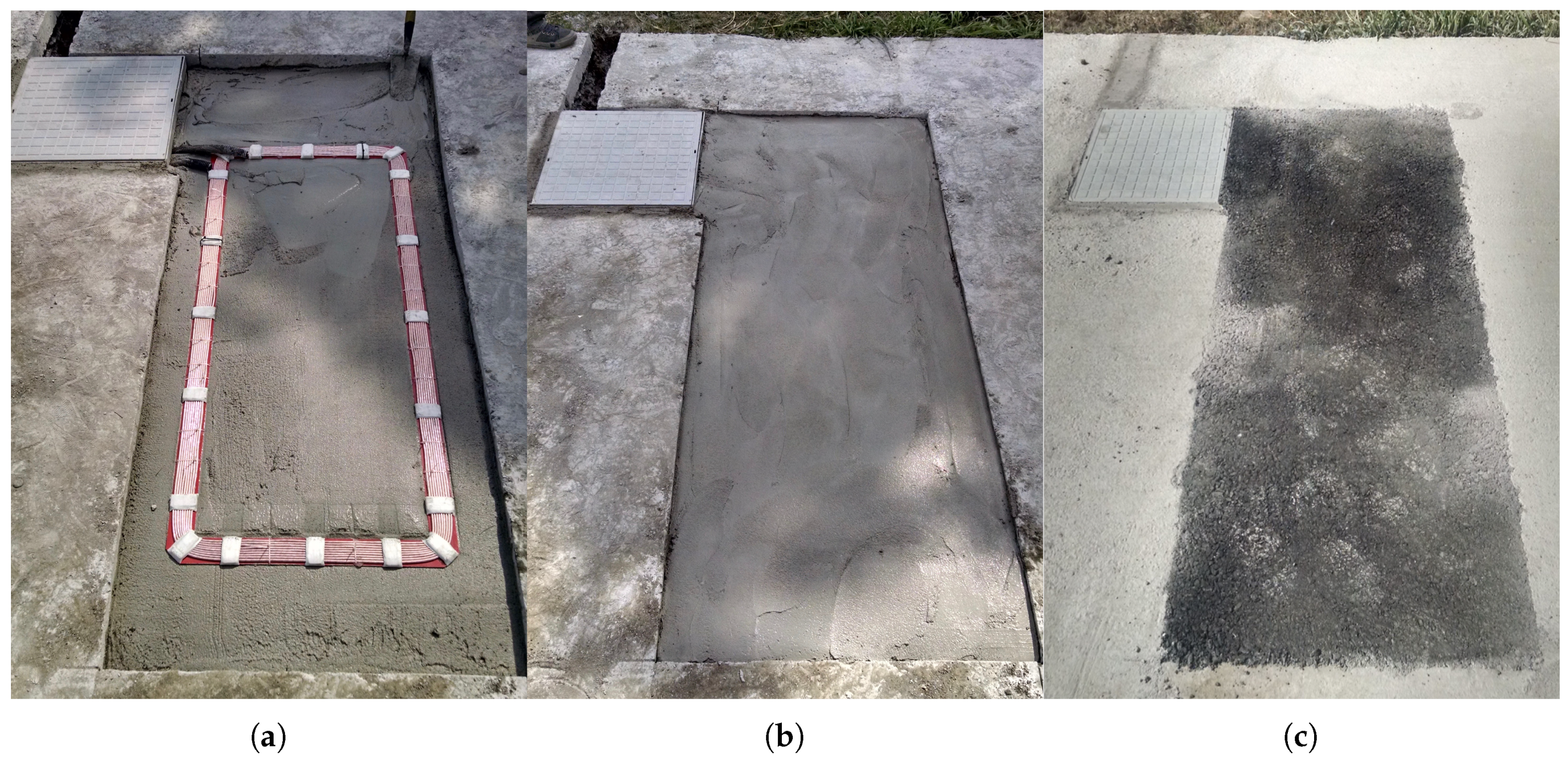
2. The Embedment Experiment
3. Electromagnetic Characterization of Concrete
- electric resistivity ,
- relative electric permittivity ,
- relative magnetic permeability .
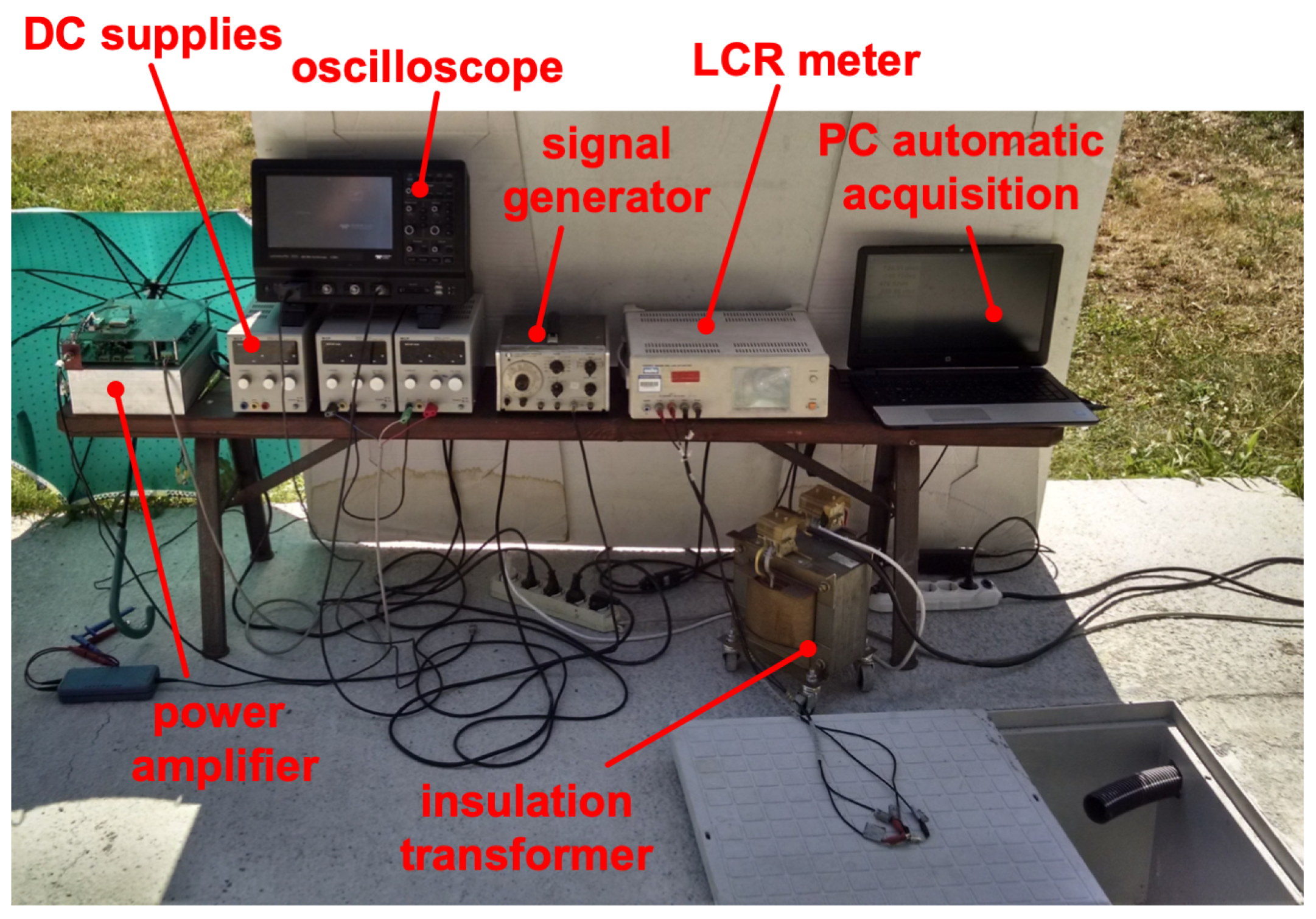
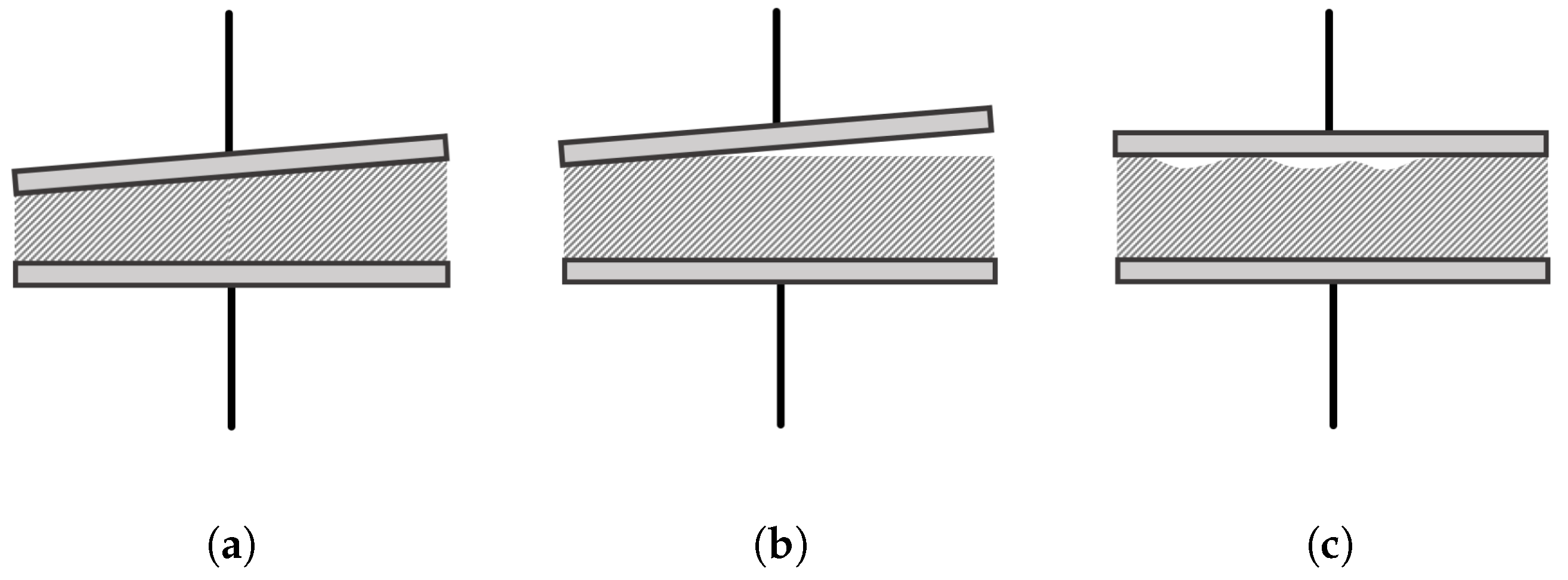
3.1. Methodology and Measurement Setup
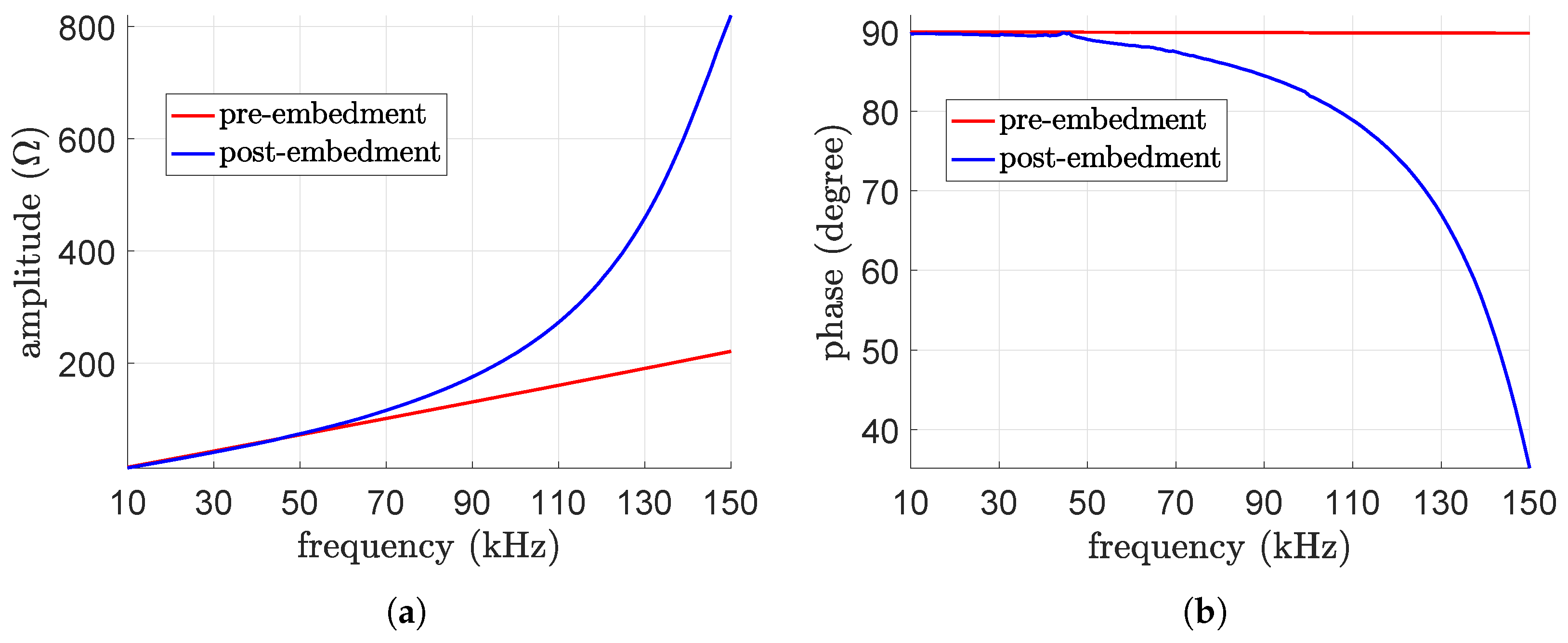
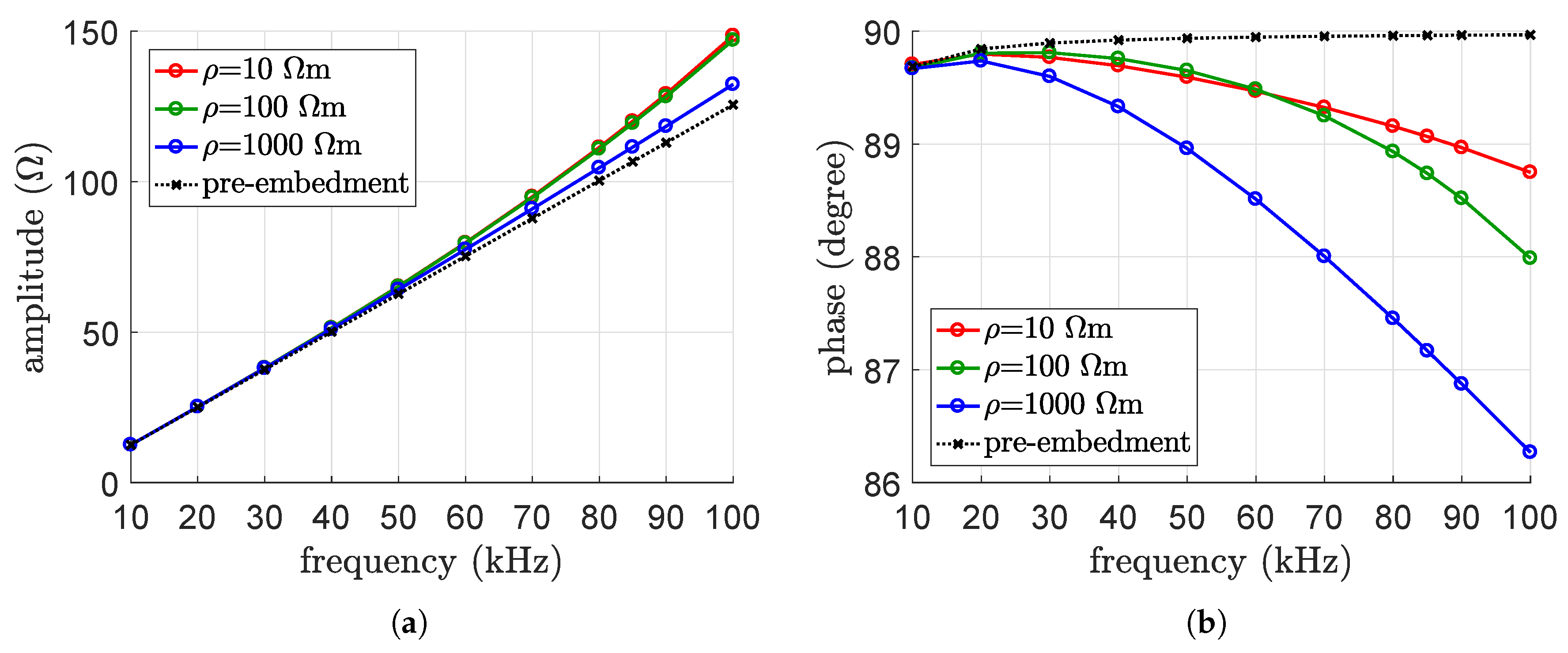
3.2. Measurements
4. Numerical Model and Parametric Analysis
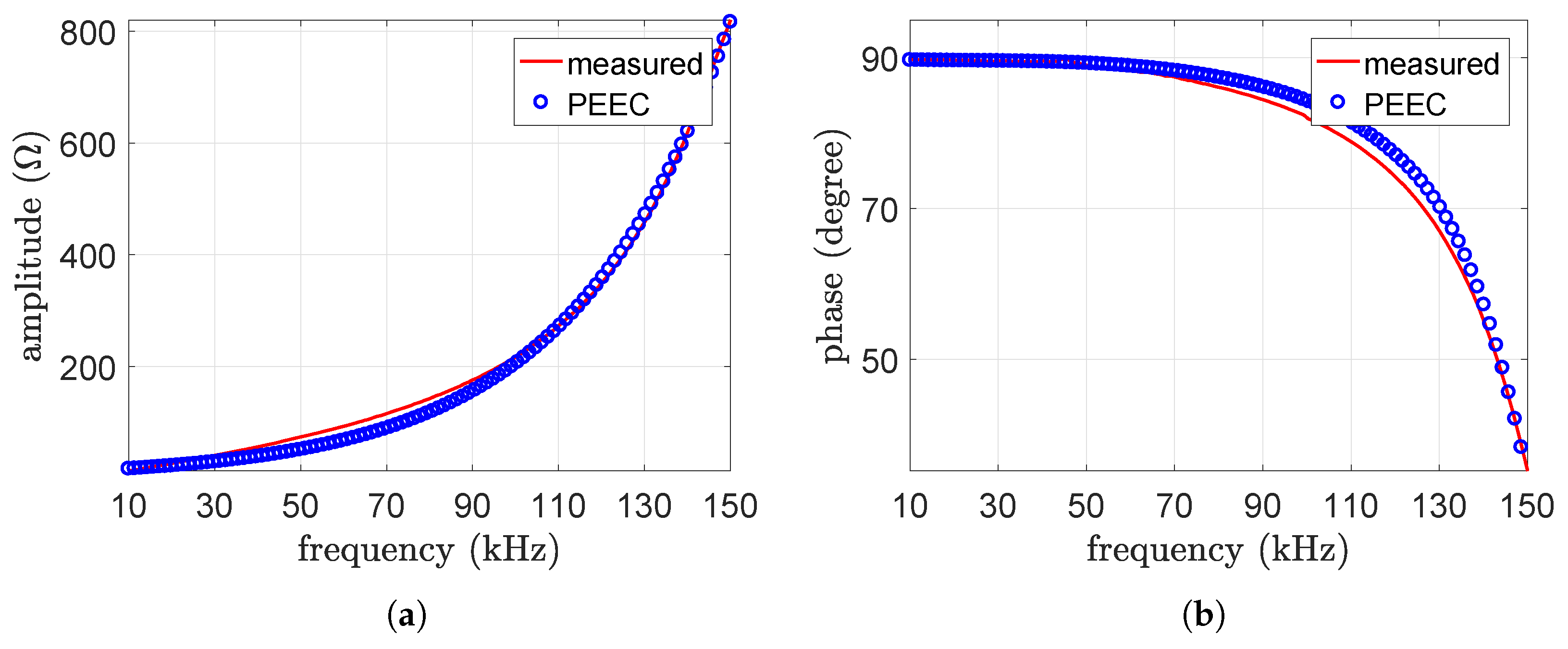
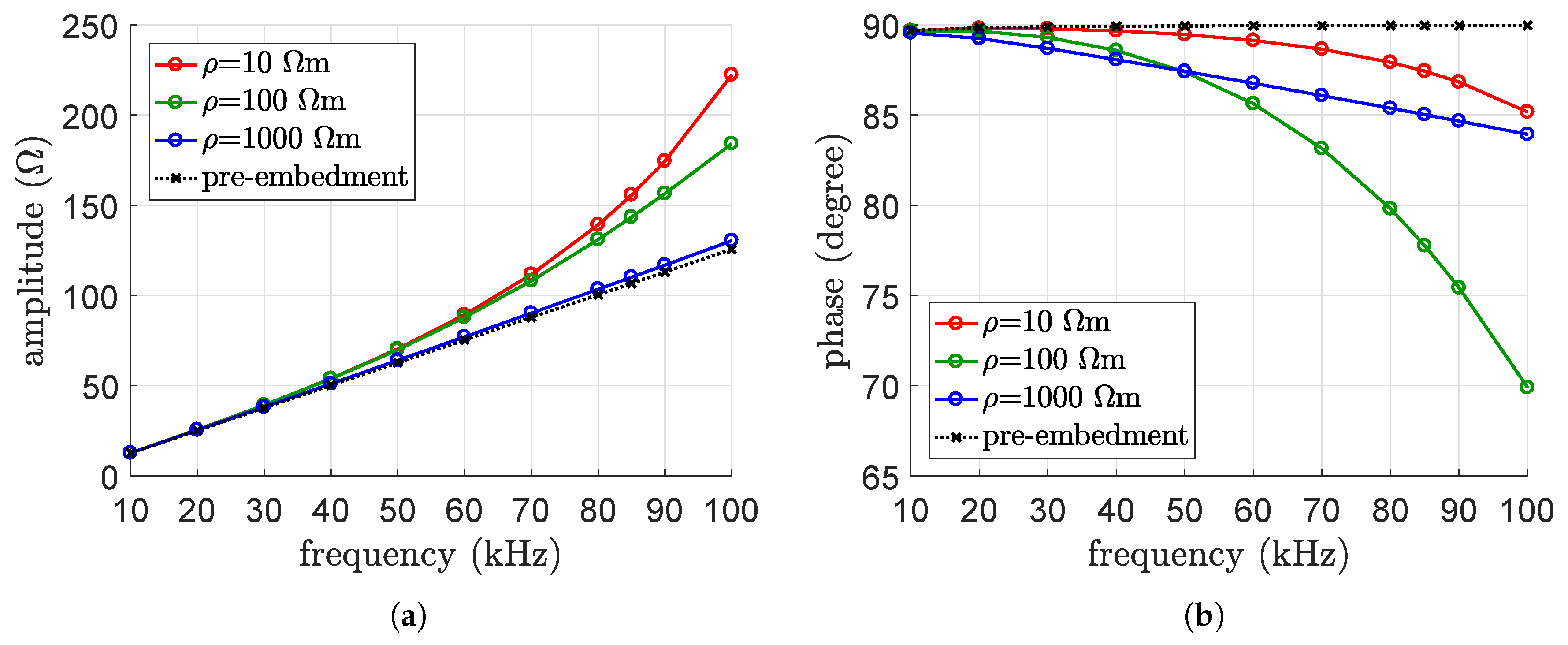
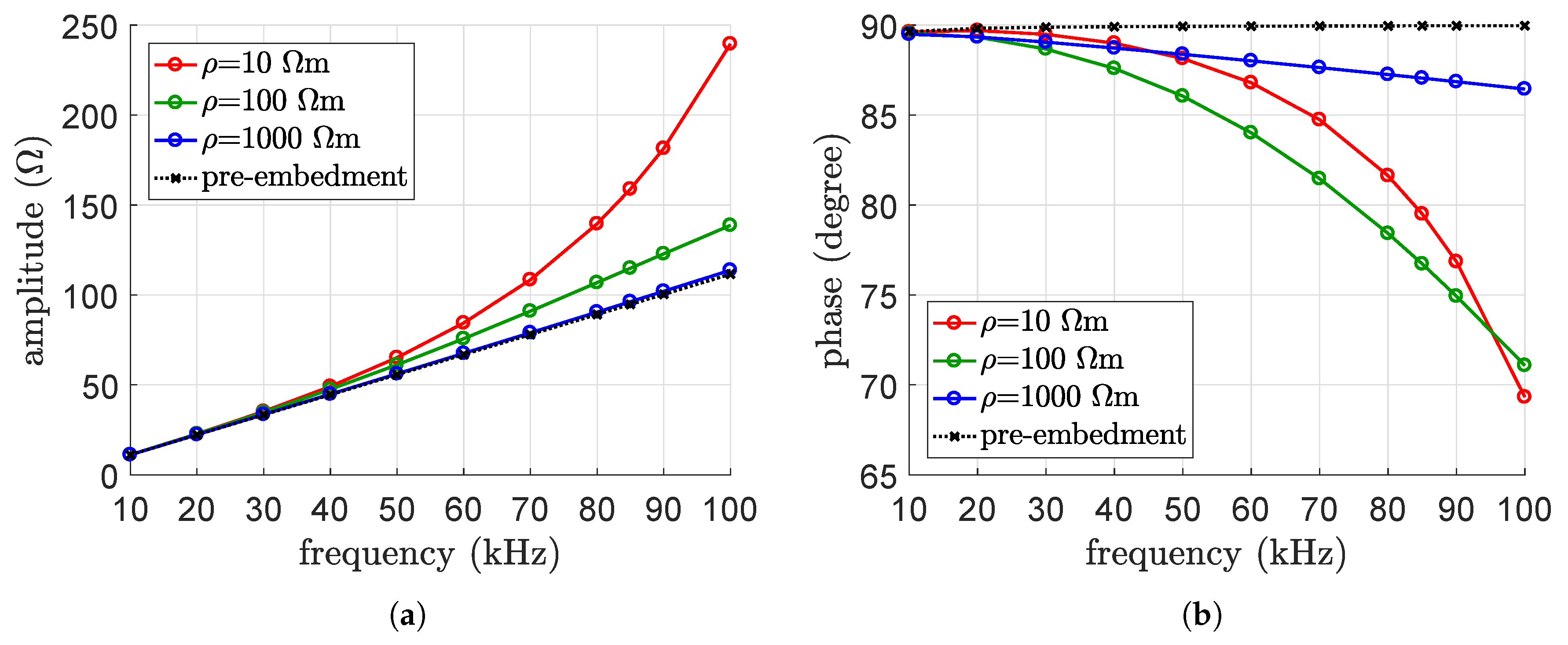
4.1. Model Validation
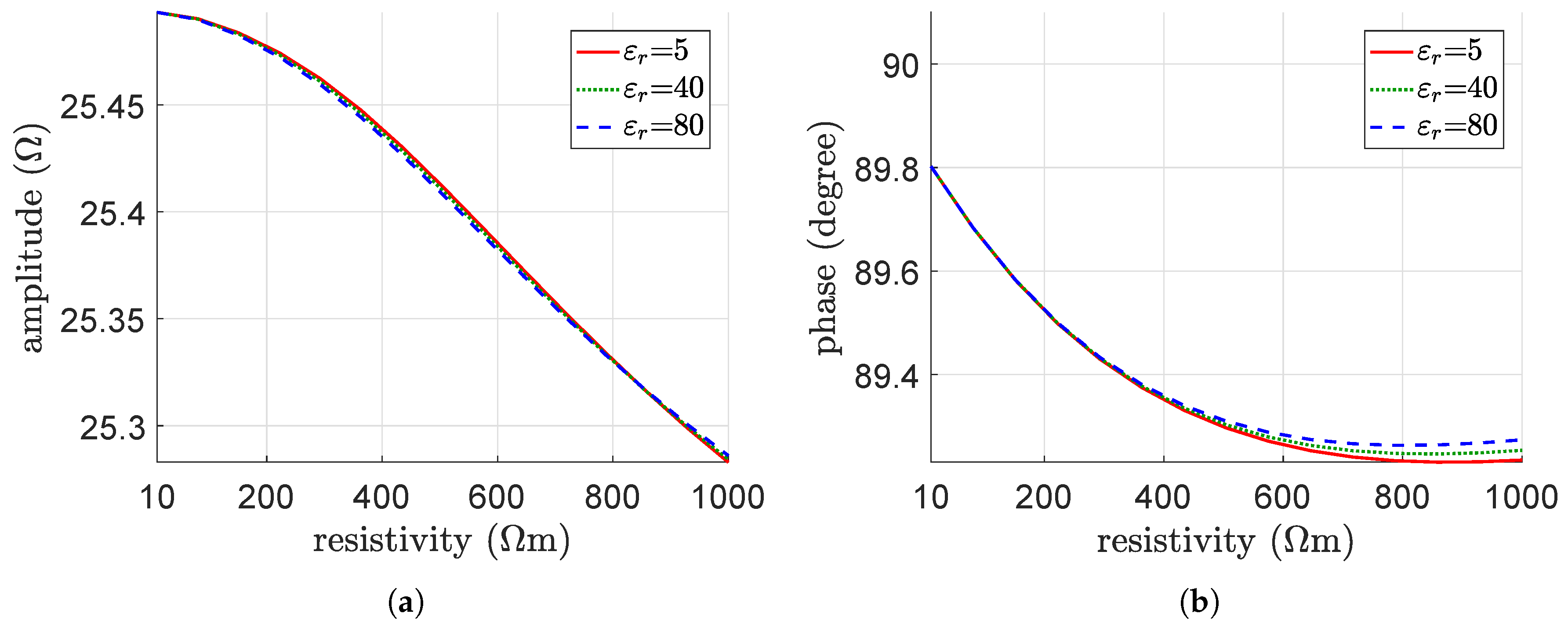
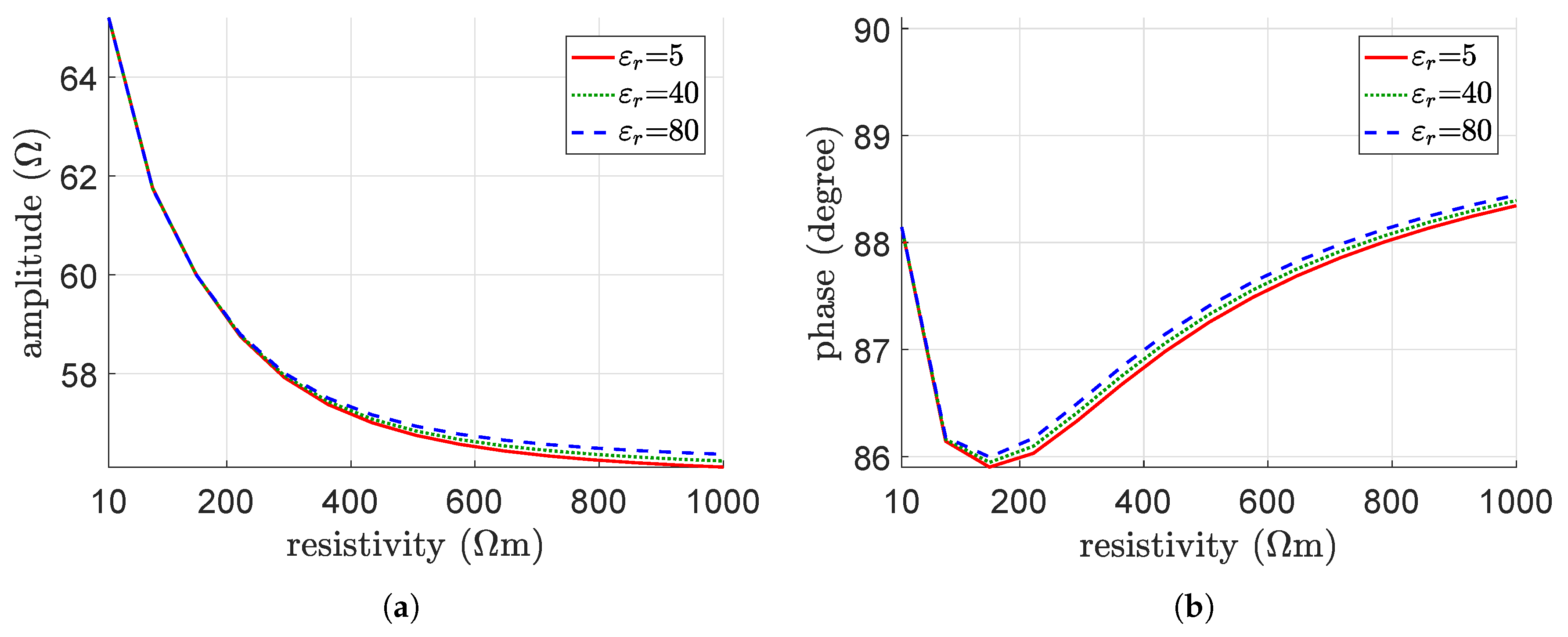
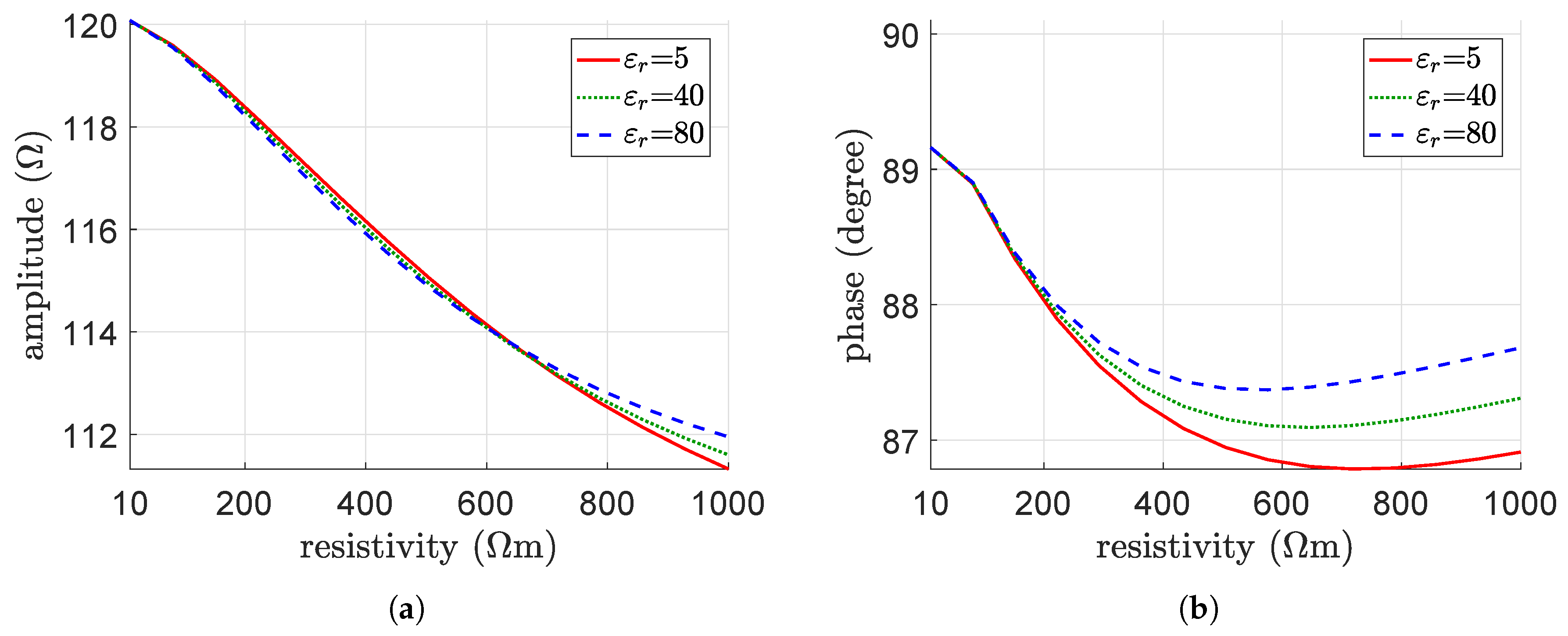
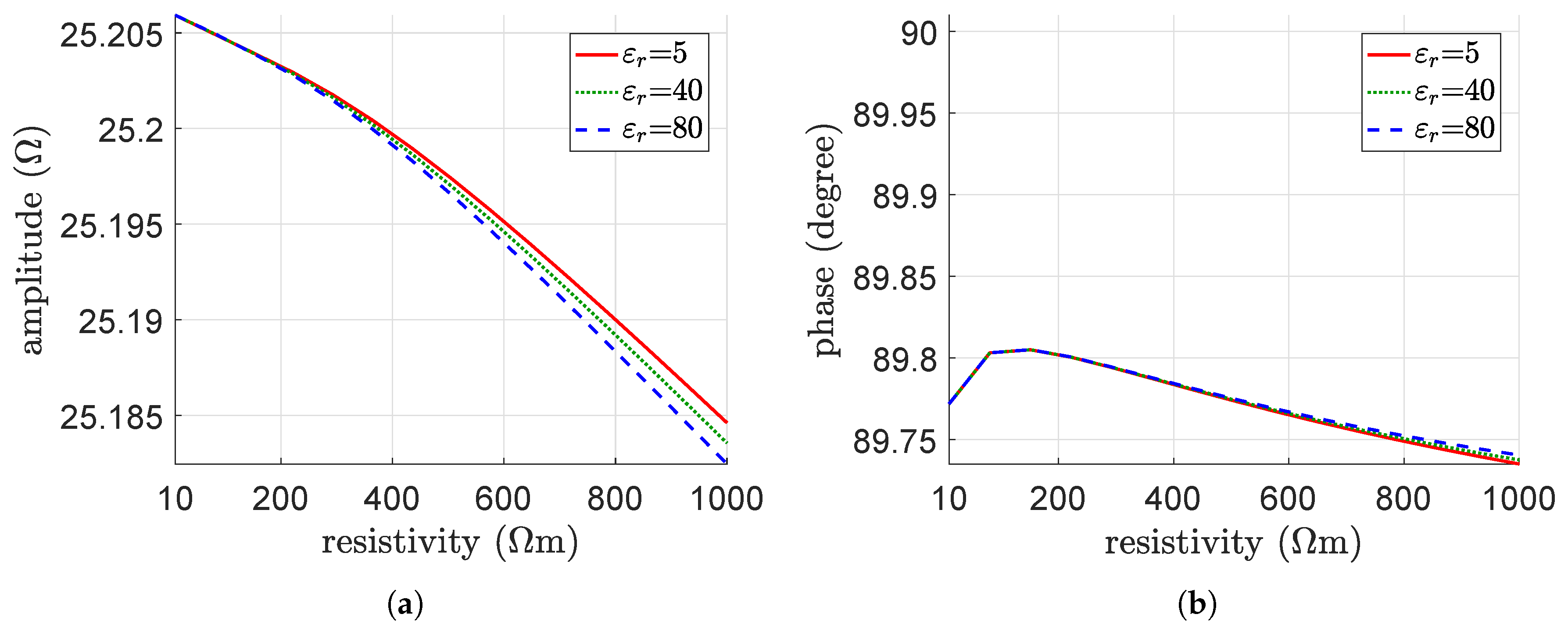
4.2. Parametric Analysis
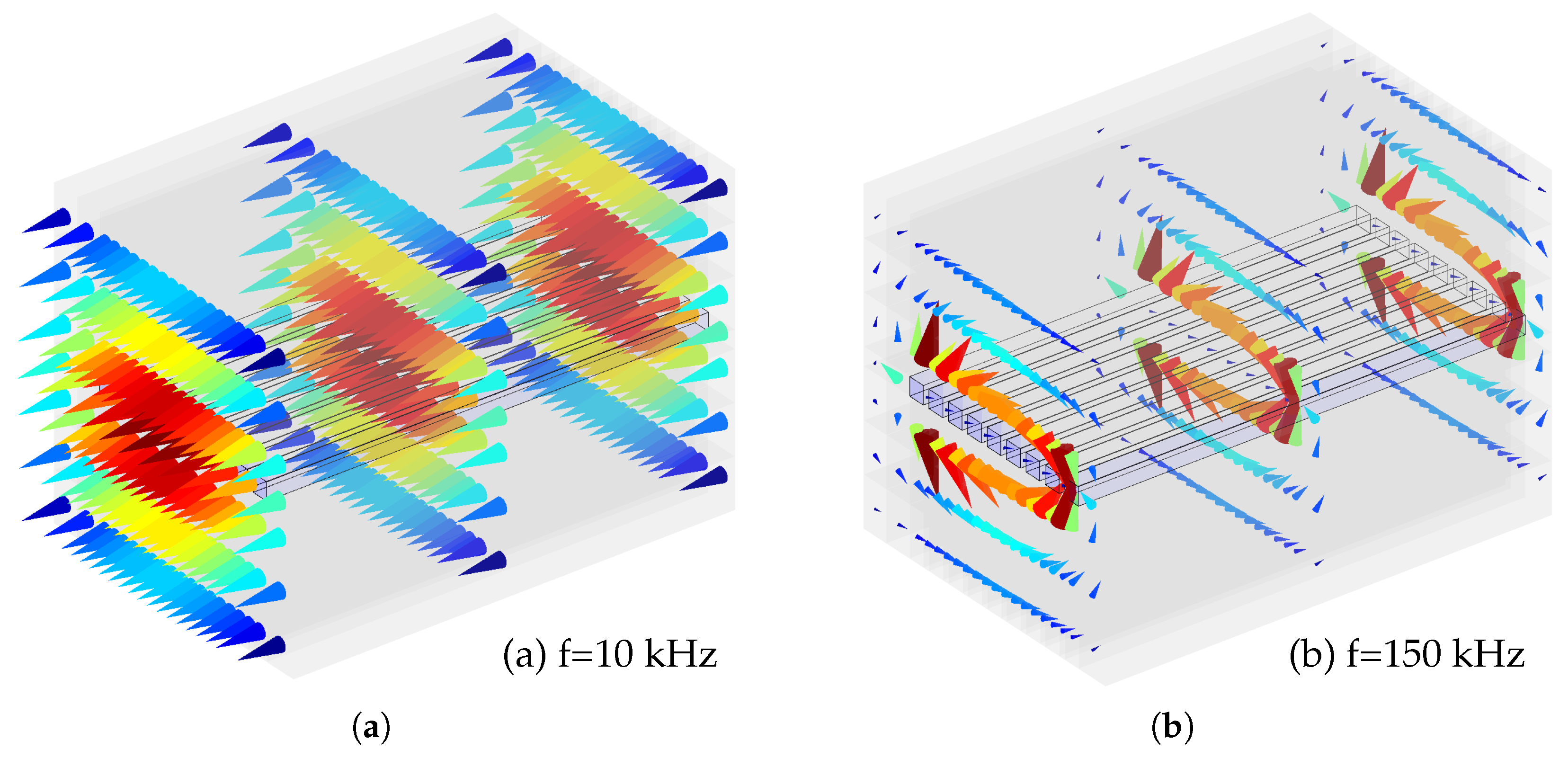
5. Interpretation of the Results
6. Conclusions
- the electromagnetic characterization of the concrete involved in the road embedment (at 28 days after pouring),
- the study of the influence of the turns spacing, the insulator thickness, and the concrete resistivity and relative permittivity on the overall electromagnetic behavior of the embedded coil.
Author Contributions
Funding
Conflicts of Interest
References
- Bernhart, W.; Schlick, T.; Olschewski, I.; Busse, A.; Riederle, S.; Pieper, G. E-mobility Index 2018; Roland Berger GmbH Automotive Competence Center: Munich, Germany; Forschungsgesellschaft Kraftfahrwesen mbH Aachen: Aachen, Germany, 2018. [Google Scholar]
- Bateman, D.; Leal, D.; Reeves, S.; Emre, M.; Stark, L.; Ognissanto, F.; Myers, R.; Lamb, M. Electric Road Systems: A Solution for the Future—TRL ACADEMY REPORT PPR875; TRL: Wokingham, UK, 2018. [Google Scholar]
- Cirimele, V.; Diana, M.; Freschi, F.; Mitolo, M. Inductive Power Transfer for Automotive Applications: State-of-the-Art and Future Trends. IEEE Trans. Ind. Appl. 2018, 54, 4069–4079. [Google Scholar] [CrossRef]
- Choi, S.Y.; Gu, B.W.; Jeong, S.Y.; Rim, C.T. Advances in wireless power transfer systems for roadway-powered electric vehicles. IEEE J. Emerg. Sel. Top. Power Electron. 2015, 3, 18–36. [Google Scholar] [CrossRef]
- Amditis, A.; Karaseitanidis, G.; Damousis, I.; Guglielmi, P.; Cirimele, V. Dynamic wireless charging for more efficient FEVS: The fabric project concept. In Proceedings of the MedPower 2014, Athens, Greece, 2–5 November 2014. [Google Scholar]
- FABRIC Project. Available online: https://fabric-project.eu (accessed on 8 July 2019).
- Choi, S.Y.; Gu, B.W.; Jeong, S.Y.; Rim, C.T. UltraslimS-typepower supply rails for roadway-powered electric vehicles. IEEE Trans. Power Electron. 2015, 30, 6456–6468. [Google Scholar] [CrossRef]
- Villa, J.; Sanz, J.; Peri, J.; Acerete, R. Victoria project: Static and dynamic wireless charging for electric buses. In Proceedings of the Business Intelligence on Emerging Technologies IDTechEX Conference, Berlin, Germany, 27–28 April 2016. [Google Scholar]
- Azad, A.N.; Echols, A.; Kulyukin, V.A.; Zane, R.; Pantic, Z. Analysis, Optimization, and Demonstration of a Vehicular Detection System Intended for Dynamic Wireless Charging Applications. IEEE Trans. Transp. Electr. 2019, 5, 147–161. [Google Scholar] [CrossRef]
- Chen, F.; Kringos, N. Towards new infrastructure materials for on-the-road charging. In Proceedings of the IEEE International Electric Vehicle Conference (IEVC), Florence, Italy, 17–19 December 2014. [Google Scholar]
- International SAE J2954 Standard. Wireless Power Transfer for Light-Duty Plug-In/Electric Vehicles and Alignment Methodology; SAE International: Warrendale, PA, USA, 2017. [Google Scholar]
- Lee, S.H.; Lee, B.S.; Lee, J.H. A new design methodology for a 300-kW, low flux density, large air gap, online wireless power transfer system. IEEE Trans. Ind. Appl. 2016, 52, 4234–4242. [Google Scholar] [CrossRef]
- Nikolaides, A. Highway Engineering: Pavements, Materials and Control of Quality; CRC Press: Boca Raton, FL, USA, 2014. [Google Scholar]
- Sandrolini, L.; Reggiani, U.; Ogunsola, A. Modelling the electrical properties of concrete for shielding effectiveness prediction. J. Phys. D Appl. Phys. 2007, 40, 5366. [Google Scholar] [CrossRef]
- Daniels, D. Ground Penetrating Radar, 2nd ed.; The Institute of Electrical Engineers: London, UK, 2004. [Google Scholar]
- IEEE Guide for Safety in AC Substation Grounding; IEEE Std 80-2000 (Revision of IEEE Std 80-1986); IEEE: New York, NY, USA, 2000.
- Wilson, J.G.; Whittington, H.W. Variations in the electrical properties of concrete with change in frequency. IEE Proc. A-Phys. Sci. Meas. Instrum. Manag. Educ. 1990, 137, 246–254. [Google Scholar] [CrossRef]
- Whittington, H.W.; McCarter, J.; Forde, M.C. The conduction of electricity through concrete. Mag. Concr. Res. 1981, 33, 48–60. [Google Scholar] [CrossRef]
- Reilly, J.P. Applied Bioelectricity: From Electrical Stimulation to Electropathology; Springer Science & Business Media: Berlin, Germany, 2012. [Google Scholar]
- Chen, F.; Taylor, N.; Kringos, N.; Birgisson, B. A study on dielectric response of bitumen in the low-frequency range. Road Mater. Pavement Des. 2015, 16 (Suppl. S1), 153–169. [Google Scholar] [CrossRef]
- Chen, F.; Taylor, N.; Balieu, R.; Kringos, N. Dynamic application of the Inductive Power Transfer (IPT) systems in an electrified road: Dielectric power loss due to pavement materials. Constr. Build. Mater. 2017, 147, 9–16. [Google Scholar] [CrossRef]
- Bishop, C. The relationship between loss, conductivity, and dielectric constant. Adv. Eng. Electromagn. 2001. Available online: http://pleclair.ua.edu/impedance/The%20Relationship%20Between%20Loss.pdf (accessed on 8 July 2019).
- Lorrain, P.; Corson, D.R.; Lorrain, F. Electromagnetic Fields and Waves; Freeman: Dallas, TX, USA, 1988. [Google Scholar]
- Torchio, R.; Alotto, P.; Bettini, P.; Voltolina, D.; Moro, F. A 3D PEEC formulation based on the cell method for full-wave analyses with conductive, dielectric, and magnetic media. IEEE Trans. Magn. 2017, 54, 1–4. [Google Scholar] [CrossRef]
- Freschi, F.; Repetto, M. A general framework for mixed structured/unstructured PEEC modelling. Appl. Comput. Electromagn. Soc. J. 2008, 23, 200–206. [Google Scholar]
- Torchio, R.; Moro, F.; Meunier, G.; Guichon, J.-M.; Chadebec, O. An Extension of Unstructured-PEEC Method to Magnetic Media. IEEE Trans. Magn. 2019, 55, 1–4. [Google Scholar] [CrossRef]
- Kriemann, R. HLIBPro (v.2.7.1). Available online: http://www.hlibpro.com (accessed on 18 May 2019).
- Torchio, R.; Bettini, P.; Alotto, P. PEEC-Based Analysis of Complex Fusion Magnets During Fast Voltage Transients With H-Matrix Compression. IEEE Trans. Magn. 2017, 53, 1–4. [Google Scholar] [CrossRef]
- Voltolina, D.; Bettini., P.; Alotto, P.; Moro, F.; Torchio, F. High-Performance PEEC Analysis of Electromagnetic Scatterers. IEEE Trans. Magn. 2019, 55, 1–4. [Google Scholar] [CrossRef]
- Fabbri, M. Magnetic flux density and vector potential of uniform polyhedral sources. IEEE Trans. Magn. 2018, 44, 32–36. [Google Scholar] [CrossRef]
- Järvenpää, S.; Taskinen, M.; Ylä-Oijala, P. Singularity extraction technique for integral equation methods with higher order basis functions on plane triangles and tetrahedra. Int. J. Numer. Methods Eng. 2003, 58, 1149–1165. [Google Scholar] [CrossRef]
- Arcioni, P.; Bressan, M.; Perregrini, L. On the evaluation of the double surface integrals arising in the application of the boundary integral method to 3D problems. IEEE Trans. Microw. Theory Tech. 1997, 45, 436–439. [Google Scholar] [CrossRef]
- Sievers, D.; Eibert, T.F.; Hansen, V. Correction to “On the Calculation of Potential Integrals for Linear Source Distributions on Triangular Domains”. IEEE Trans. Antennas Propag. 2005, 53, 3113. [Google Scholar] [CrossRef]
- Guglielmi, P.; Cirimele, V.; Perrone, W. Procedimento per l’installazione Diretta su Strada di Induttori per Trasferimento Induttivo di Potenza Elettrica. Italian Patent 102018000006495, 20 June 2018. [Google Scholar]
- Torchio, R.; Di Rienzo, L.; Codecasa, L. Stochastic PEEC Method Based on Polynomial Chaos Expansion. IEEE Trans. Magn. 2019, 53, 1–4. [Google Scholar] [CrossRef]

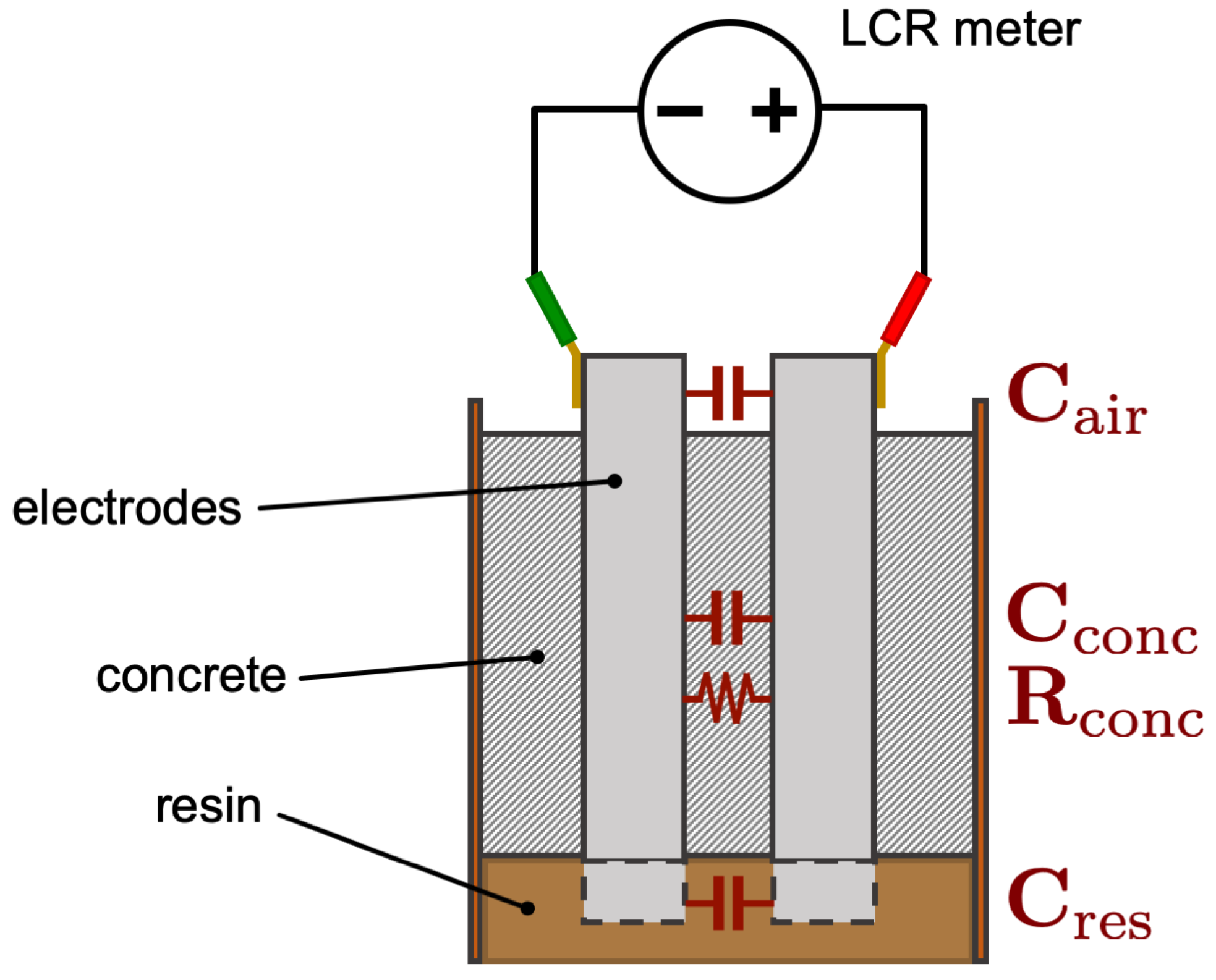
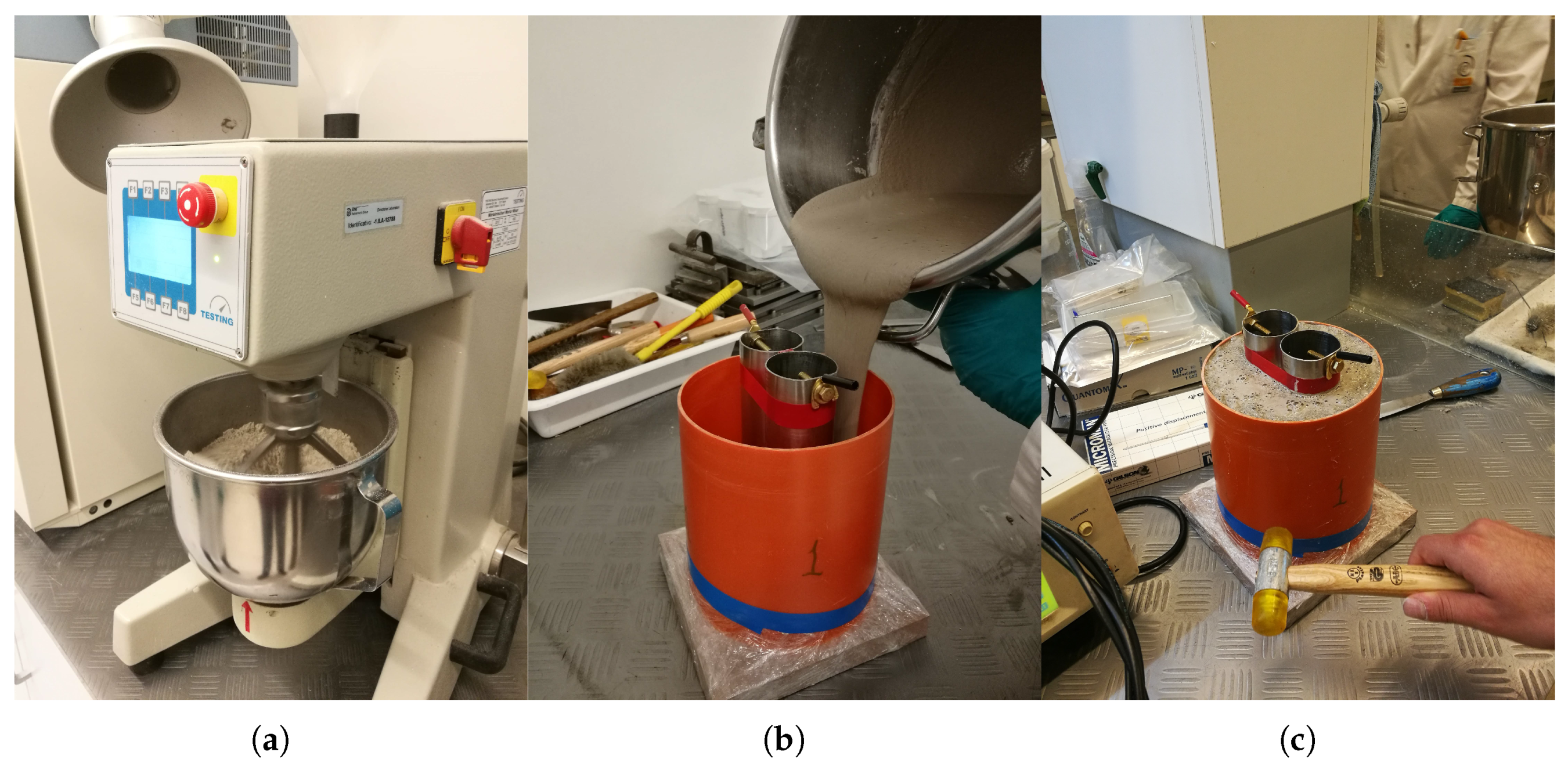
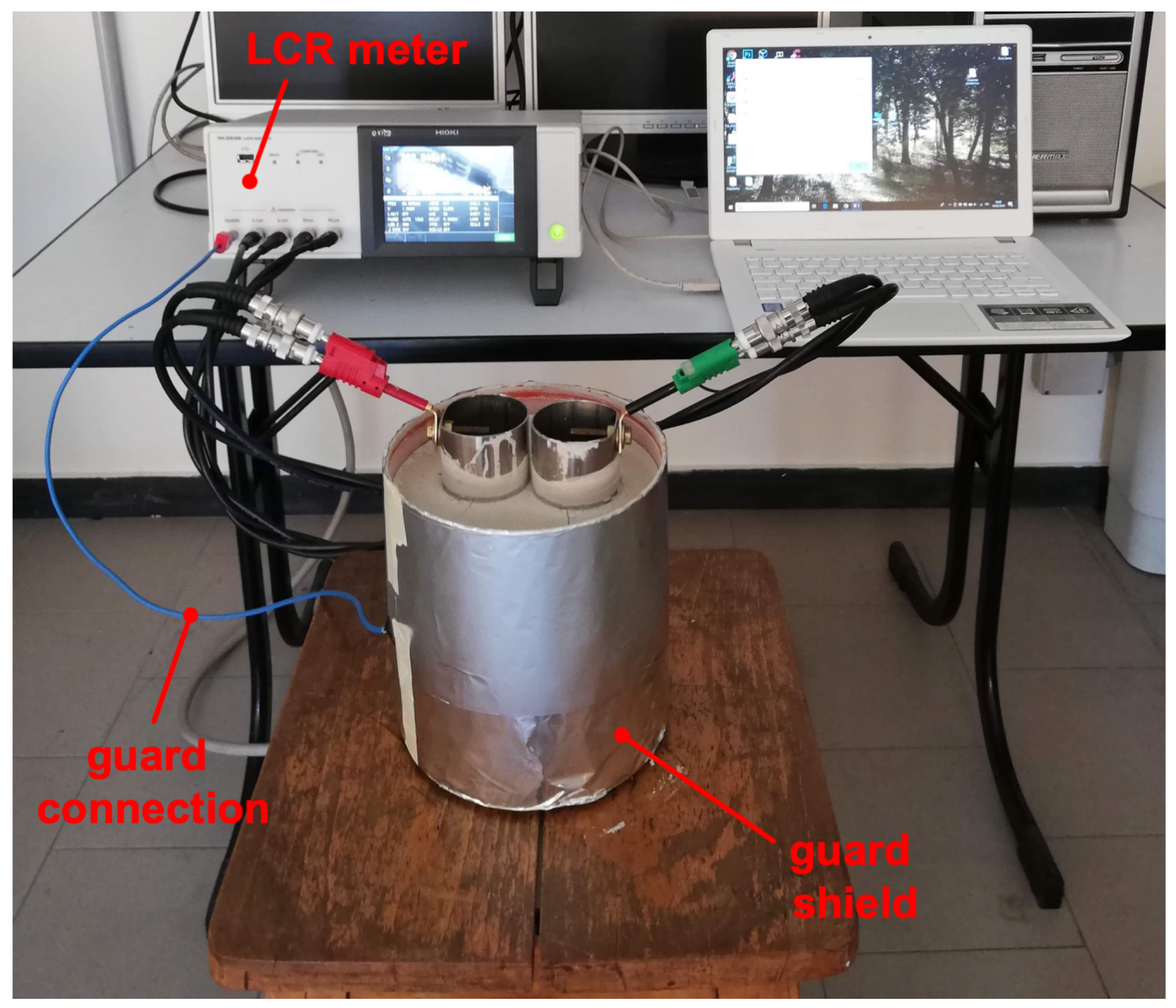
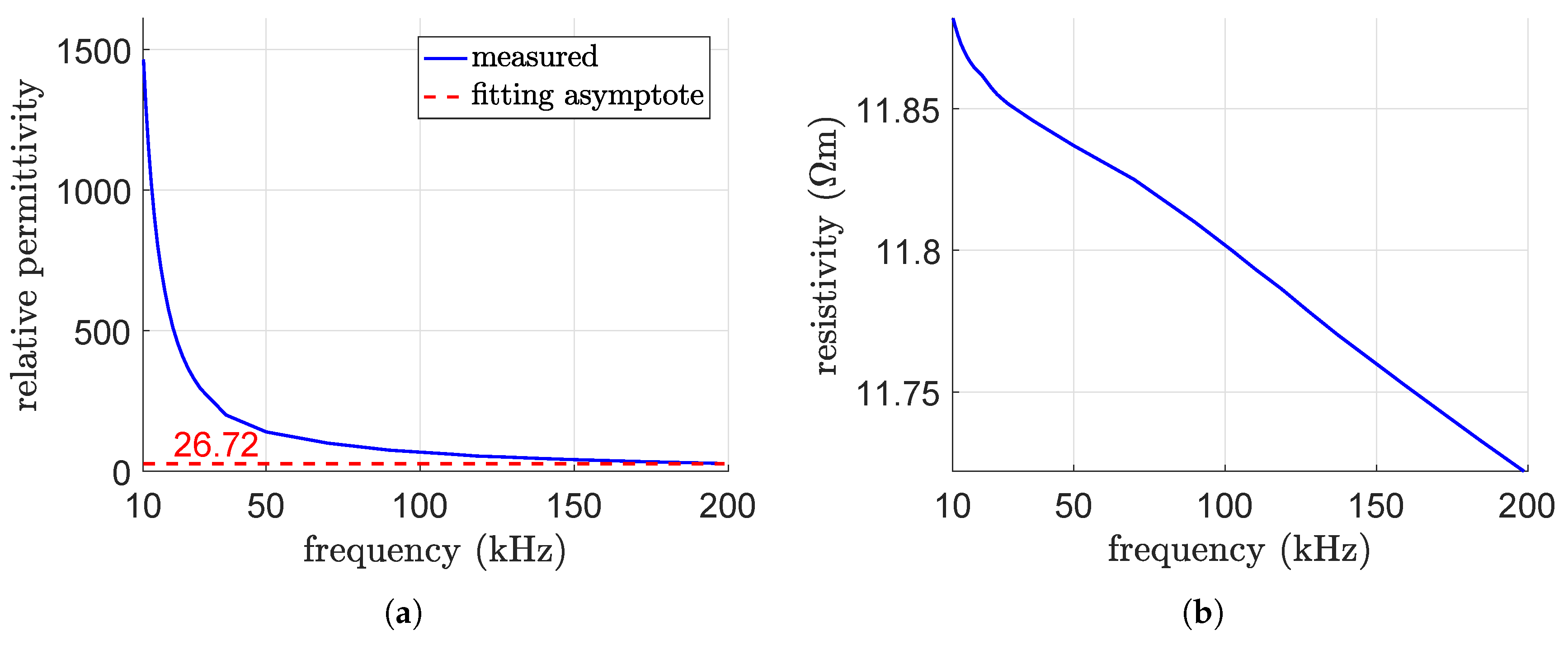






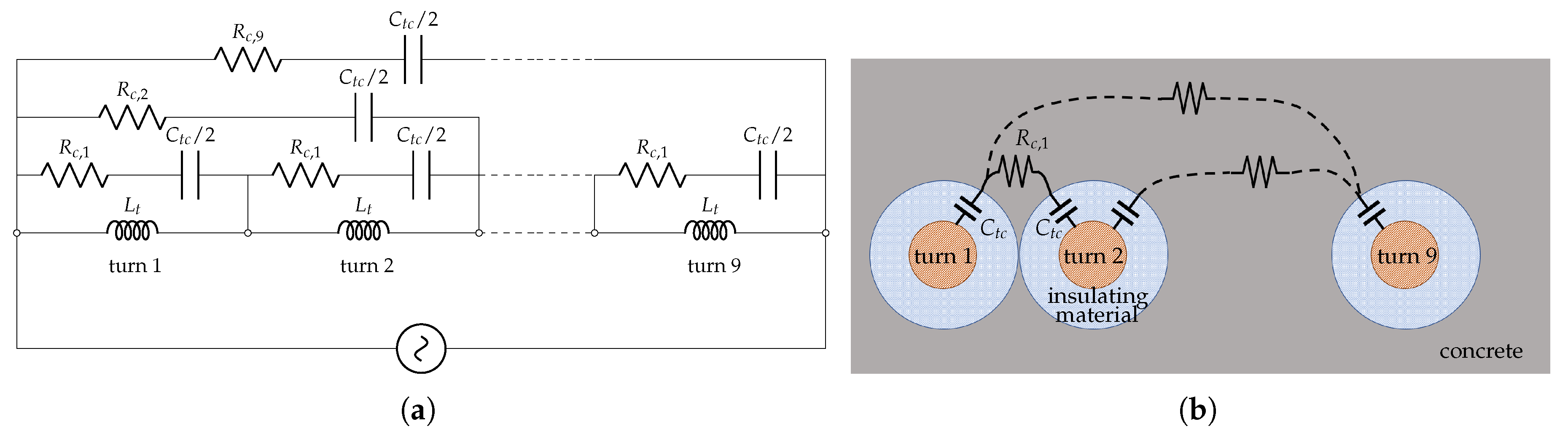
| Layer Length | Capacitance Form Factor | Resistance form Factor | |
|---|---|---|---|
| air | mm | m | - |
| concrete | mm | m | m |
| resin | mm | m | - |
| Frequency (kHz) | ||
|---|---|---|
| 20 | ||
| 85 | ||
| 200 | ||
| asym. value | - |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cirimele, V.; Torchio, R.; Virgillito, A.; Freschi, F.; Alotto, P. Challenges in the Electromagnetic Modeling of Road Embedded Wireless Power Transfer. Energies 2019, 12, 2677. https://doi.org/10.3390/en12142677
Cirimele V, Torchio R, Virgillito A, Freschi F, Alotto P. Challenges in the Electromagnetic Modeling of Road Embedded Wireless Power Transfer. Energies. 2019; 12(14):2677. https://doi.org/10.3390/en12142677
Chicago/Turabian StyleCirimele, Vincenzo, Riccardo Torchio, Antonio Virgillito, Fabio Freschi, and Piergiorgio Alotto. 2019. "Challenges in the Electromagnetic Modeling of Road Embedded Wireless Power Transfer" Energies 12, no. 14: 2677. https://doi.org/10.3390/en12142677
APA StyleCirimele, V., Torchio, R., Virgillito, A., Freschi, F., & Alotto, P. (2019). Challenges in the Electromagnetic Modeling of Road Embedded Wireless Power Transfer. Energies, 12(14), 2677. https://doi.org/10.3390/en12142677







