A Holistic Review on Biomass Gasification Modified Equilibrium Models
Abstract
:1. Introduction
1.1. Existing Reviews
1.2. Motivation and Objective of the Review
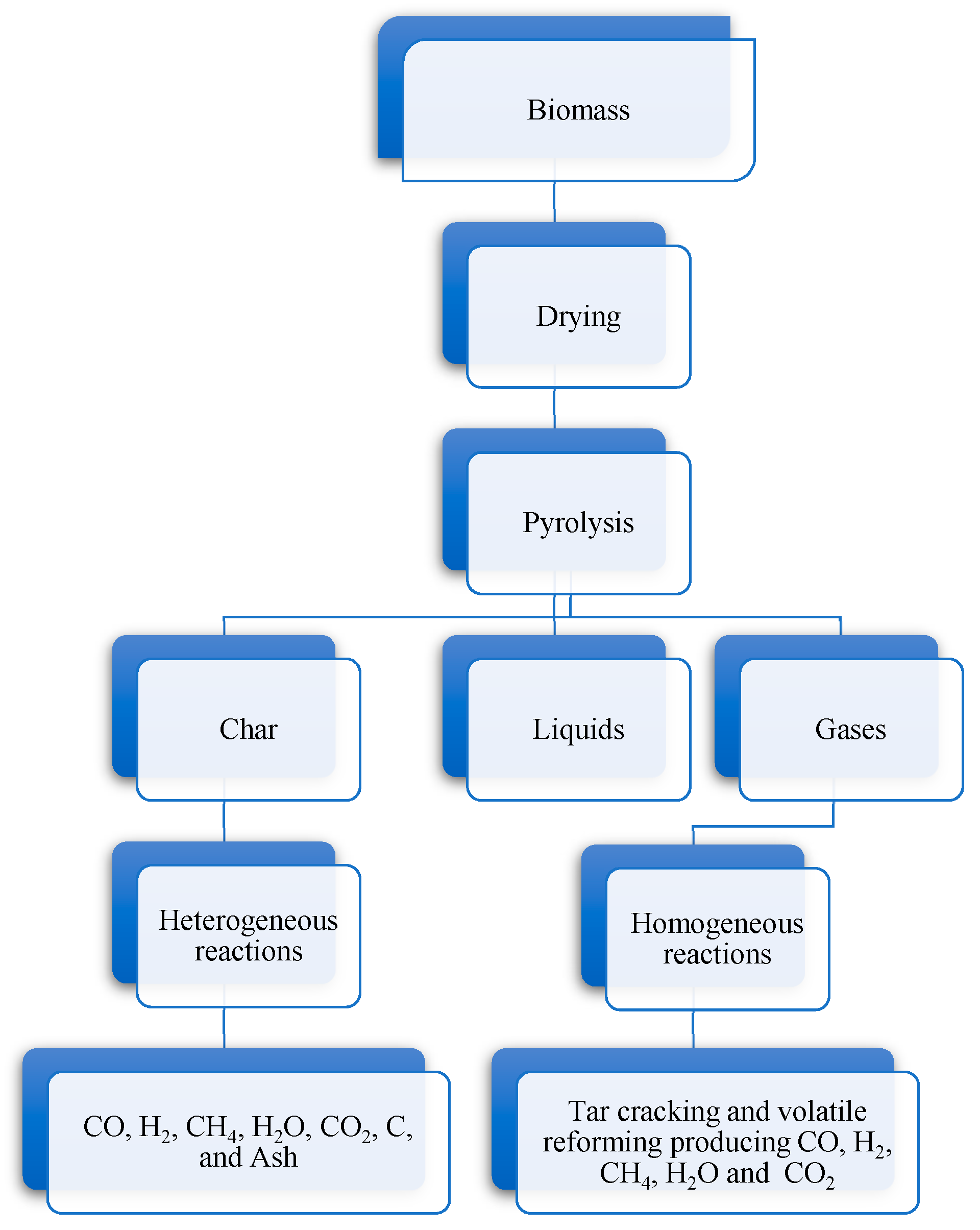
2. Gasification Process
- Drying—where moisture is transformed into steam at temperatures around 100–200 °C. At these temperatures no chemical reaction takes place; the biomass is not decomposed. For a produced gas with high calorific value, the vast majority of gasification systems use biomass with moisture content in-between 10 to 20%;
- Pyrolysis—is the thermal decomposition (devolatilization) of the dry biomass in the absence of oxygen at temperatures in-between 150–700 °C releasing the volatiles components and a residue containing char and ash. The volatiles produced are a mixture comprising mostly carbon monoxide, hydrogen, carbon dioxide, light hydrocarbons, tar (liquid fraction) and water vapor;
- Oxidation—various oxidation chemical reactions take place in a gasification scenario releasing the heat needed for the endothermic reactions. The reaction between the char and oxygen, forming carbon dioxide. The hydrogen in the biomass is oxidized to generate water. The oxygen is present in sub-stoichiometric amounts; partial oxidation of carbon might occur, resulting in the production of carbon monoxide;
- Reduction—various chemical reactions mainly endothermic occur without the presence of oxygen due to its consumption in the oxidation reactions. The main products of the reduction reactions are hydrogen, carbon monoxide and methane.
3. Gasification Equilibrium Models
- Steady state;
- Reactions reach the equilibrium state (infinite residence time);
- Homogeneous mixing with uniform pressure and temperature;
- Kinetic and potential energies are neglected;
- Perfect gas behavior of the gas phase;
- Pyrolysis is considered a single step reaction producing gas, tar and char;
- Gasifying medium is enough to convert all carbon of the biomass;
- The gasifier operates at constant pressure and temperature;
- The reactor is considered adiabatic;
- The produced gas does not contain oxygen;
- Nitrogen is considered as inert;
- Solely major species compose the produced gas (CO, H2, CO2, CH4, N2 and H2O);
- Tar is not modeled or modeled in the gas phase;
- Ashes are not considered in energy balances.
3.1. Stoichiometric Modeling
3.2. Non-Stoichiometric Modeling
4. Modified Equilibrium Models
4.1. Stoichiometric Method
4.2. Non-Stoichiometric Method
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Faaij, A.P.C. Bio-energy in Europe: Changing technology choices. Energy Policy 2006, 34, 322–342. [Google Scholar] [CrossRef]
- Maniatis, K. Progress in biomass gasification: An overview. In Progress in Thermochemical Biomass Conversion; Editor Bridgwater, A.V., Ed.; Blackwell Science: London, UK, 2001; pp. 1–31. ISBN 9780632055333. [Google Scholar]
- Bartocci, P.; Zampilli, M.; Bidini, G.; Fantozzi, F. Hydrogen-rich gas production through steam gasification of charcoal pellet. Appl. Therm. Eng. 2018, 132, 817–823. [Google Scholar] [CrossRef]
- Kirkels, A.; Verbong, G. Biomass Gasification: Still promising? A 30-year global overview. Renew. Sustain. Energy Rev. 2011, 15, 471–481. [Google Scholar] [CrossRef]
- Ahmad, A.A.; Zawawi, N.A.; Kasim, F.H.; Inayat, A.; Khasri, A. Assessing the gasification performance of biomass: A review on biomass gasification process conditions, optimization and economic evaluation. Renew. Sustain. Energy Rev. 2016, 53, 1333–1347. [Google Scholar] [CrossRef]
- Basu, P. Biomass Gasification and Pyrolysis: Practical Design and Theory, 2nd ed.; Elsevier: Oxford, UK, 2013; ISBN 978-970-12-3964885. [Google Scholar]
- Paethanom, A.; Bartocci, P.; D’Alessandro, B.; D’Amico, M.; Testarmata, F.; Moriconi, N.; Slopiecka, K.; Yoshikawa, K.; Fantozzi, F. A low-cost pyrogas cleaning system for power generation: Scaling up from lab to pilot. Appl. Energy 2013, 111, 1080–1088. [Google Scholar] [CrossRef]
- Molino, A.; Larocca, V.; Chianese, S.; Musmarra, D. Biofuels production by biomass gasification: A review. Energies 2018, 11, 811. [Google Scholar] [CrossRef]
- Chang, K.-H.; Lou, K.-R.; Ko, C.-H. Potential of bioenergy production from biomass wastes of rice paddies and forest sectors in Taiwan. J. Clean. Prod. 2019, 206, 460–476. [Google Scholar] [CrossRef]
- Couto, N.; Silva, V.; Monteiro, E.; Brito, P.; Rouboa, A. Modeling of fluidized bed gasification: Assessment of zero-dimensional and CFD approaches. J. Therm. Sci. 2015, 24, 378–385. [Google Scholar] [CrossRef]
- Gómez-Barea, A.; Leckner, B. Modeling of biomass gasification in fluidized bed. Prog. Energy Combust. Sci. 2010, 36, 444–509. [Google Scholar] [CrossRef]
- Puig-Arnavat, M.; Bruno, J.C.; Coronas, A. Review and analysis of biomass gasification models. Renew. Sustain. Energy Rev. 2010, 14, 2841–2851. [Google Scholar] [CrossRef]
- Baruah, D.; Baruah, D.C. Modeling of biomass gasification: A Review. Renew. Sustain. Energy Rev. 2014, 39, 806–815. [Google Scholar] [CrossRef]
- Patra, T.K.; Sheth, P.N. Biomass gasification models for downdraft gasifier: A state-of-the-art review. Renew. Sustain. Energy Rev. 2015, 50, 583–593. [Google Scholar] [CrossRef]
- Fantozzi, F.; Frassoldati, A.; Bartocci, P.; Cinti, G.; Quagliarini, F.; Bidini, G.; Ranzi, E.M. An experimental and kinetic modeling study of glycerol pyrolysis. Appl. Energy 2016, 184, 68–76. [Google Scholar] [CrossRef]
- Mahinpey, N.; Gomez, A. Review of gasification fundamentals and new findings: Reactors, feedstock, and kinetic studies. Chem. Eng. Sci. 2016, 148, 14–31. [Google Scholar] [CrossRef]
- Karmakar, M.K.; Datta, A.B. Generation of hydrogen rich gas through fluidized bed gasification of biomass. Bioresour. Technol. 2011, 102, 1907–1913. [Google Scholar] [CrossRef]
- Altafini, C.R.; Wander, P.R.; Barreto, R.M. Prediction of the working parameters of a wood waste gasifier through an equilibrium model. Energy Convers. Manag. 2003, 44, 2763–2777. [Google Scholar] [CrossRef]
- Sharma, A.K. Equilibrium and kinetic modelling of char reduction reactions in a downdraft biomass gasifier: A comparison. Sol. Energy 2008, 52, 918–928. [Google Scholar] [CrossRef]
- Versteeg, H.K.; Malalasekera, W. An Introduction to Computational Fluid Dynamics, 2nd ed.; Prentice Hall: London, UK, 2007; ISBN 978-970-13-127498-3. [Google Scholar]
- Di Blasi, C. Modeling chemical and physical processes of wood and biomass pyrolysis. Prog. Energy Combust. Sci. 2008, 34, 47–90. [Google Scholar] [CrossRef]
- Loha, C.; Gu, S.; De Wilde, J.; Mahanta, P.; Chatterjee, K. Advanced in mathematical modeling of fluidized bed gasification. Renew. Sustain. Energy Rev. 2014, 40, 688–715. [Google Scholar] [CrossRef]
- Gerber, S.; Behrendt, F.; Oevermann, M. An Eulerian modeling approach of wood gasification in a bubbling fluidized bed reactor using char as bed material. Fuel 2010, 89, 2903–2917. [Google Scholar] [CrossRef]
- Couto, N.; Silva, V.; Monteiro, E.; Brito, P.S.D.; Rouboa, A. Experimental and numerical analysis of coffee husks biomass gasification in a fluidized bed reactor. Energy Procedia 2013, 36, 591–595. [Google Scholar] [CrossRef]
- Couto, N.; Monteiro, E.; Silva, V.; Rouboa, A. Hydrogen-rich gas from gasification of Portuguese municipal solid wastes. Int. J. Hydrogen Energy 2016, 41, 10619–10630. [Google Scholar] [CrossRef]
- Ismail, T.M.; Abd El-Salam, M.; Monteiro, E.; Rouboa, A. Fluid Dynamics Model on Fluidized Bed Gasifier Using Agro-Industrial Biomass as Fuel. Waste Manag. 2018, 73, 476–486. [Google Scholar] [CrossRef] [PubMed]
- Monteiro, E.; Ismail, T.M.; Ramos, A.; Abd El-Salam, M.; Brito, P.; Rouboa, A. Experimental and modeling studies of Portuguese peach stone gasification on an autothermal bubbling fluidized bed pilot plant. Energy 2018, 142, 862–877. [Google Scholar] [CrossRef]
- Sikarwar, V.S.; Zhao, M.; Clough, P.; Yao, J.; Zhong, X.; Memon, M.Z.; Shah, N.; Anthony, E.J.; Fennell, P.S. An overview of advances in biomass gasification. Energy Environ. Sci. 2016, 9, 2939–2977. [Google Scholar] [CrossRef] [Green Version]
- La Villetta, M.; Costa, M.; Massarotti, N. Modelling approaches to biomass gasification: A review with emphasis on the stoichiometric method. Renew. Sustain. Energy Rev. 2017, 74, 71–88. [Google Scholar] [CrossRef]
- Kangas, P.; Hannula, I.; Koukkari, P.; Hupa, M. Modelling super-equilibrium in biomass gasification with the constrained Gibbs energy method. Fuel 2014, 129, 631–653. [Google Scholar] [CrossRef]
- Pajarre, R.; Koukkari, P.; Kangas, P. Constrained and extended free energy minimisation for modelling of processes and materials. Chem. Eng. Sci. 2016, 146, 244–258. [Google Scholar] [CrossRef]
- Vakalis, S.; Patuzzi, F.; Baratieri, M. Thermodynamic modeling of small scale biomass gasifiers: Development and assessment of the “Multi-Box” approach. Bioresour. Technol. 2016, 206, 173–179. [Google Scholar] [CrossRef]
- Biagini, E.; Barontini, F.; Tognotti, L. Development of a bi-equilibrium model for biomass gasification in a downdraft bed reactor. Bioresour. Technol. 2016, 201, 156–165. [Google Scholar] [CrossRef]
- Lim, Y.-I.; Lee, U.-D. Quasi-equilibrium thermodynamic model with empirical equations for air–steam biomass gasification in fluidized-beds. Fuel Process. Technol. 2014, 128, 199–210. [Google Scholar] [CrossRef]
- Higman, C.; Burgt, M. Gasification; Elsevier Science: New York, NY, USA, 2003; ISBN 0-7506-7707-7704. [Google Scholar]
- Couto, N.; Rouboa, A.; Silva, V.; Monteiro, E.; Bouziane, K. Influence of the biomass gasification processes on the final composition of syngas. Energy Procedia 2013, 36, 596–606. [Google Scholar] [CrossRef]
- Molino, A.; Chianese, S.; Musmarra, D. Biomass gasification technology: The state of the art overview. J. Energy Chem. 2016, 25, 10–25. [Google Scholar] [CrossRef]
- Demirbaş, A. Hydrogen Production from Biomass by the Gasification Process. Energy Sources 2002, 24, 59–68. [Google Scholar] [CrossRef]
- Salem, A.M.; Paul, M.C. An integrated kinetic model for downdraft gasifier based on a novel approach that optimises the reduction zone of gasifier. Biomass Bioenergy 2018, 109, 172–181. [Google Scholar] [CrossRef]
- Milhé, M.; Steene, L.; Haube, M.; Commandré, J.-M.; Fassinou, W.-F.; Flamant, G. Autothermal and allothermal pyrolysis in a continuous fixed bed reactor. J. Anal. Appl. Pyrolysis 2013, 103, 102–111. [Google Scholar] [CrossRef]
- Smith, R.W.; Missen, W.R. Chemical Reaction Equilibrium Analysis: Theory and Algorithms; Wiley Interscience: New York, NY, USA, 1983; ISBN 978-0471093473. [Google Scholar]
- Jarungthammachote, S.; Dutta, A. Thermodynamic equilibrium model and second law analysis of a downdraft waste gasifier. Energy 2007, 32, 1660–1669. [Google Scholar] [CrossRef]
- Rodrigues, R.; Secchi, A.R.; Marcilio, N.R.; Godinho, M. Modeling of biomass gasification applied to a combined gasifier-combustor unit: Equilibrium and kinetic approaches. Comput. Aided Chem. Eng. 2009, 27, 657–662. [Google Scholar] [CrossRef]
- Lan, C.; Lyu, Q.; Qie, Y.; Jiang, M.; Liu, X.; Zhang, S. Thermodynamic and kinetic behaviors of coal gasification. Thermochim. Acta 2018, 666, 174–180. [Google Scholar] [CrossRef]
- Puig Arnavat, M. Performance Modelling and Validation of Biomass Gasifiers for Trigeneration Plants. Ph.D. Thesis, Universitat Rovira I Virgili, Tarragona, Spain, 2011. [Google Scholar]
- Zainal, Z.A.; Ali, R.; Lean, C.H.; Seetharamu, K.N. Prediction of performance of a downdraft gasifier using equilibrium modeling for different biomass materials. Energy Convers. Manag. 2001, 42, 1499–1515. [Google Scholar] [CrossRef]
- Souza-Santos, M.L. Solid Fuels Combustion and Gasification, 2nd ed.; CRC Press: Boca Raton, FL, USA, 2010; ISBN 9781420047493. [Google Scholar]
- Talebi, G.; Goethem, M.W.M. Synthesis Gas from Waste Plasma Gasification for Fueling Lime Kiln. Chem. Eng. Trans. 2014, 37, 619–624. [Google Scholar] [CrossRef]
- Materazzi, M.; Lettieri, P.; Mazzei, L.; Taylor, R.; Chapman, C. Thermodynamic modelling and evaluation of a two-stage thermal process for waste gasification. Fuel 2013, 108, 356–369. [Google Scholar] [CrossRef] [Green Version]
- Hernández, J.J.; Ballesteros, R.; Aranda, G. Characterisation of tars from biomass gasification: Effect of the operating conditions. Energy 2013, 50, 333–342. [Google Scholar] [CrossRef]
- Nguyen, H.; Berguerand, N.; Thunman, H. Applicability of a kinetic model for catalytic conversion of tar and light hydrocarbons using process-activated ilmenite. Fuel 2018, 231, 8–17. [Google Scholar] [CrossRef]
- Mountouris, A.; Voutsas, E.; Tassios, D. Solid waste plasma gasification: Equilibrium model development and exergy analysis. Energy Convers. Manag. 2006, 47, 1723–1737. [Google Scholar] [CrossRef]
- Prins, M.J.; Ptasinski, K.J.; Janssen, G. From coal to biomass gasification: Comparison of thermodynamic efficiency. Energy 2007, 32, 1248–1259. [Google Scholar] [CrossRef]
- Gumz, W. Gas Producers and Blast Furnaces: Theory and Methods of Calculation; John Wiley & Sons: New York, NY, USA, 1950. [Google Scholar]
- Melgar, A.; Pérez, J.F.; Laget, H.; Horillo, A. Thermochemical equilibrium modelling of a gasifying process. Energy Convers. Manag. 2007, 48, 59–67. [Google Scholar] [CrossRef]
- Sharma, S.; Sheth, P.N. Air-steam biomass gasification: Experiments, modeling and simulation. Energy Convers. Manag. 2016, 110, 307–318. [Google Scholar] [CrossRef]
- Wang, Y.; Kinoshita, C.M. Kinetic model of biomass gasification. Sol. Energy 1993, 51, 19–25. [Google Scholar] [CrossRef]
- Huang, H.-J.; Ramaswamy, S. Modeling Biomass Gasification Using Thermodynamic Equilibrium Approach. Appl. Biochem. Biotechnol. 2009, 154, 193–204. [Google Scholar] [CrossRef]
- Abuadala, A.; Dincer, I.; Naterer, G.F. Exergy analysis of hydrogen production from biomass gasification. Int. J. Hydrogen. Energy 2010, 35, 4981–4990. [Google Scholar] [CrossRef]
- Fryda, L.; Panopoulos, K.D.; Karl, J.; Kakaras, E. Exergetic analysis of solid oxide fuel cell and biomass gasification integration with heat pipes. Energy 2008, 33, 292–299. [Google Scholar] [CrossRef]
- Sadaka, S.; Ghaly, A.E.; Sabbah, M.A. Two phase biomass air—Steam gasification model for fluidized bed reactors: Part I—Model development. Biomass Bioenergy 2002, 22, 439–462. [Google Scholar] [CrossRef]
- Karamarkovic, R.; Karamarkovic, V. Energy and exergy analysis of biomass gasification at different temperatures. Energy 2010, 35, 537–549. [Google Scholar] [CrossRef]
- Ngo, S.I.; Nguyen, B.; Lim, Y.-I.; Song, B.-H.; Lee, U.-D.; Choi, Y.-T.; Song, J.-H. Performance evaluation for dual circulating fluidized-bed steam gasifier of biomass using quasi-equilibrium three-stage gasification model. Appl. Energy 2011, 88, 5208–5220. [Google Scholar] [CrossRef]
- Puig-Arnavat, M.; Bruno, J.C.; Coronas, A. Modified Thermodynamic Equilibrium Model for Biomass Gasification: A Study of the Influence of Operating Conditions. Energy Fuels 2012, 26, 1385–1394. [Google Scholar] [CrossRef]
- Azzone, E.; Morini, M.; Pinelli, M. Development of an equilibrium model for the simulation of thermochemical gasification and application to agricultural residues. Renew. Energy 2012, 46, 248–254. [Google Scholar] [CrossRef]
- Barman, N.S.; Ghosh, S.; Sudipta, D. Gasification of biomass in a fixed bed downdraft gasifier—A realistic model including tar. Bioresour. Technol. 2012, 107, 505–511. [Google Scholar] [CrossRef]
- Silva, V.; Rouboa, A. Using a two-stage equilibrium model to simulate oxygen air enriched gasification of pine biomass residues. Fuel Process. Technol. 2013, 109, 111–117. [Google Scholar] [CrossRef]
- Mendiburu, A.Z.; Carvalho, J.A.; Coronado, C.J.R. Thermochemical equilibrium modeling of biomass downdraft gasifier: Stoichiometric models. Energy 2014, 66, 189–201. [Google Scholar] [CrossRef]
- Ratnadhariya, J.K.; Channiwala, S.A. Experimental studies on molar distribution of CO/CO2 and CO/H2 along the length of downdraft wood gasifier. Energy Convers. Manag. 2010, 51, 452–458. [Google Scholar] [CrossRef]
- Costa, M.; La Villetta, M.; Massarotti, N. Optimal tuning of a thermo-chemical equilibrium model for downdraft biomass gasifiers. Chem. Eng. Trans. 2015, 43, 439–444. [Google Scholar] [CrossRef]
- Rupesh, S.; Muraleedharan, C.; Arun, P. A comparative study on gaseous fuel generation capability of biomass materials by thermo-chemical gasification using stoichiometric quasi-steady-state model. Int. J. Energy Environ. Eng. 2015, 6, 375–384. [Google Scholar] [CrossRef] [Green Version]
- Gagliano, A.; Nocera, F.; Patania, F.; Brun, O.M.; Castaldo, D.G. A robust numerical model for characterizing the syngas composition in a downdraft gasification process. C. R. Chim. 2016, 19, 441–449. [Google Scholar] [CrossRef]
- Adams, T.N. A simple fuel bed model for predicting particulate emissions from a wood-waste boiler. Combust. Flame 1980, 39, 225–239. [Google Scholar] [CrossRef]
- Aydin, E.S.; Yucel, O.; Sadikoglu, H. Development of a semi-empirical equilibrium model for downdraft gasification systems. Energy 2017, 130, 86–98. [Google Scholar] [CrossRef]
- Desrosiers, R. Thermodynamics of gas-char reactions. In A Survey of Biomass Gasification; Reed, T.B., Ed.; Solar Energy Research Institute: Golden, CO, USA, 1979. [Google Scholar]
- Double, J.M.; Bridgwater, A.V. Sensitivity of theoretical gasifier performance to system parameters. In Energy from Biomass, 3rd E.C. Conference, Venice, Italy, 25–29 March; Coombs, J., Hall, D.O., Eds.; Elsevier: London, UK, 1985; pp. 915–919. [Google Scholar]
- Prins, M.J.; Ptasinski, K.J.; Janssen, F.J.J.G. Thermodynamics of gas-char reactions first and second law analysis. Chem. Eng. Sci. 2003, 58, 1003–1011. [Google Scholar] [CrossRef]
- Wurzenberger, J.C.; Wallner, S.; Raupenstrauch, H.; Khinast, J.G. Thermal conversion of biomass: Comprehensive reactor and particle modeling. AICHE J. 2002, 48, 2398–2411. [Google Scholar] [CrossRef]
- Zhang, L.; Xu, C.; Champagne, P. Overview of recent advances in thermo-chemical conversion of biomass. Energy Convers. Manag. 2010, 51, 969–982. [Google Scholar] [CrossRef]
- Jablonski, W.; Gaston, K.R.; Nimlos, M.R.; Carpenter, D.L.; Feik, C.J.; Phillips, S.D. Pilot scale gasification of corn stover, switchgrass, wheat straw, and wood: 2. Identification of global chemistry using multivariate curve resolution techniques. Ind. Eng. Chem. Res. 2009, 48, 10691–10701. [Google Scholar] [CrossRef]
- Park, H.J.; Park, S.H.; Sohn, J.M.; Park, J.; Jeon, J.-K.; Kim, S.-S.; Park, Y.-K. Steam reforming of biomass gasification tar using benzene as a model compound over various Ni supported metal oxide catalysts. Bioresour. Technol. 2010, 101, 101–103. [Google Scholar] [CrossRef] [PubMed]
- Di Blasi, C. Dynamic behaviour of stratified downdraft gasifiers. Chem. Eng. Sci. 2000, 55, 2931–2944. [Google Scholar] [CrossRef]
- Buragohain, B.; Mahanta, P.; Moholkar, V.S. Performance correlations for biomass gasifiers using semi-equilibrium non-stoichiometric thermodynamic models. Int. J. Energy Res. 2012, 36, 590–618. [Google Scholar] [CrossRef]
- Ghassemi, H.; Shahsavan-Markadeh, R. Effects of various operational parameters on biomass gasification process: A modified equilibrium model. Energy Convers. Manag. 2014, 79, 18–24. [Google Scholar] [CrossRef]
- Baratieri, M.; Pieratti, E.; Nordgreen, T.; Grigiante, M. Biomass Gasification with Dolomite as Catalyst in a Small Fluidized Bed Experimental and Modelling Analysis. Waste Biomass Valorization 2010, 1, 283–291. [Google Scholar] [CrossRef]
- Mendiburu, A.Z.; Carvalho, J.A.; Zanzi, R.; Coronado, C.R.; Silveira, J.L. Thermochemical equilibrium modeling of a biomass downdraft gasifier: Constrained and unconstrained non-stoichiometric models. Energy 2014, 71, 624–637. [Google Scholar] [CrossRef]
- Gambarotta, A.; Morini, M.; Zubani, A. A non-stoichiometric equilibrium model for the simulation of the biomass gasification process. Appl. Energy 2018, 227, 119–127. [Google Scholar] [CrossRef]
- Barba, D.; Prisciandaro, M.; Salladini, A.; Celso, M.G. The Gibbs Free Energy Gradient Method for RDF gasification modeling. Fuel 2011, 90, 1402–1407. [Google Scholar] [CrossRef]
- Yakaboylu, O.; Harinck, J.; Smit, K.G.; Jong, W. Testing the constrained equilibrium method for the modeling of supercritical water gasification of biomass. Fuel Process. Technol. 2015, 138, 74–85. [Google Scholar] [CrossRef]
- Li, X.T.; Grace, J.R.; Lim, C.J.; Watkinson, A.P.; Chen, H.P.; Kim, J.R. Biomass gasification in a circulating fluidized bed. Biomass Bioenergy 2004, 26, 171–193. [Google Scholar] [CrossRef]
- Jarungthammachote, S.; Dutta, A. Equilibrium modeling of gasification: Gibbs free energy minimization approach and its application to spouted bed and spout-fluid bed gasifiers. Energy Convers. Manag. 2008, 49, 1345–1356. [Google Scholar] [CrossRef]
- Matsui, I.; Kunii, D.; Furusawa, T. Study of fluidized bed steam gasification of char by thermogravimetrically obtained kinetics. J. Chem. Eng. Jpn. 1985, 18, 105–113. [Google Scholar] [CrossRef]
- Sreejith, C.C.; Arun, P.; Muraleedharan, C. Thermochemical Analysis of Biomass Gasification by Gibbs free energy minimization model—Part: I (optimization of pressure and temperature). Int. J. Green Energy 2013, 10, 231–256. [Google Scholar] [CrossRef]
- Linjewile, T.M.; Agarwal, P.K. The product CO/CO2 ratio from petroleum coke spheres in fluidized bed combustion. Fuel 1995, 74, 5–11. [Google Scholar] [CrossRef]
- Curran, H.J.; Gaffuri, P.; Pitz, W.J.; Westbrook, C.K. A comprehensive modeling study of iso-octane oxidation. Combust. Flame 2002, 129, 253–280. [Google Scholar] [CrossRef] [Green Version]
- Himmelblau, D.M. Applied Nonlinear Programming; McGraw-Hill Book Company: New York, NY, USA, 1972; ISBN 978-0070289215. [Google Scholar]
- Kalivas, J.H. Adaptation of Simulated Annealing to Chemical Optimization Problems; Elsevier Science B.V.: Amsterdam, The Netherlands, 1995; ISBN 9780080544748. [Google Scholar]


| Authors | Review | Objectives |
|---|---|---|
| Gómez-Barea and Leckner (2010) [11] | Modeling of biomass gasification in fluidized bed | The objective of this article is to review the modeling of fluidized bed gasification of biomass and wastes. The work emphasizes the prediction of the performance of a fluidized bed biomass gasification in terms of gas composition, carbon conversion and gasification efficiency. |
| Puig-Arnavat et al. (2010) [12] | Review and analysis of biomass gasification models | The objective of this article is to review gasification process modelling in order to underline the role of gasification models. This review purposes to compare and analyze various biomass gasification models available in the literature. |
| Baruah and Baruah (2014) [13] | Modeling of biomass gasification: A review | The objective of this article is to review and compare some biomass gasification models available in the literature. A comparative study of the developed models is performed with emphasis on their applicability and limitations. |
| Loha et al. (2014) [22] | Advances in mathematical modeling of fluidized bed gasification | The objective of this article is to review the fluidized bed gasification models. Advantages and disadvantages of the modeling approaches and major results obtained are discussed. |
| Patra and Sheth (2015) [14] | Biomass gasification models for downdraft gasifier: A state-of-the-art review | The objective of this article is to review the current state-of-the- art of modeling of biomass gasification in fixed beds. A review of the gasification process is offered as departing point for the description of the gasification models. |
| Villetta et al. (2017) [29] | Modelling approaches to biomass gasification: A review with emphasis on the stoichiometric method | The objective of this article is to present a general overview of gasification models, highlighting those based on the stoichiometric method. The aim is to discuss the effect of biomass moisture content, equivalence ratio, pressure variations and oxygen enrichment on the quality of the produced gas. |
| Model Designation | Features |
|---|---|
| Thermodynamic equilibrium |
|
| Modified equilibrium models |
|
| Quasi-temperature model |
|
| Constrained free energy method |
|
| Author(s) (Year) | Model’s Designation | Model Features |
|---|---|---|
| Zainal et al. (2001) [46] | Equilibrium model based on equilibrium constants | Global gasification reaction: Equilibrium is calculated by two independent reactions (3) and (9), three partial mass balances for C, H and O and one heat balance. The gasification temperature is fixed. Oxygen content and the produced gas composition are the unknowns. |
| Mountouris et al. (2006) [52] | Equilibrium model based on equilibrium constants | Global gasification reaction: Equilibrium is calculated using three independent reactions (2), (9) and (14), three partial mass balances (C, H and O) and by a heat balance. This model allows soot formation, as a solid carbon by-product (C) and exergy calculations for the process optimization. |
| Prins et al. (2007) [53] | Quasi-equilibrium model | Global biomass formula: CH1.4O0.6 Equilibrium is calculated by three independent reactions (1), (2), and (3), mass balances and heat balance. The model special feature is the use of the carbon boundary temperature (the temperature achieved when the exact quantity of oxygen is supplied and complete gasification is achieved). The quasi-equilibrium temperature approach was first introduced by Gumz [54], through which the equilibria of the reactions defined in the model are evaluated at a temperature lower than the process temperature. It can be concluded that the gasification efficiency is remarkable affected when the gasification reactions (9) and (10) are kinetically limited and do not contribute enough to the carbon conversion. The equilibrium model thus indicates the maximum efficiency that can be achieved. |
| Jarungthammachote and Dutta (2007) [42] | Thermodynamic equilibrium model based on equilibrium constants | Global gasification reaction: Equilibrium is calculated using two independent reactions (3) and (9), three partial mass balances (C, H and O) and a heat balance. The modification done to improve the model’s performance is the multiplication of equilibrium constants by coefficients. 11.28 and 0.91 are the coefficients used for the methanation and water-gas shift reactions, respectively. |
| Melgar et al. (2007) [55] | Thermochemical equilibrium model | Global gasification reaction: Two independent reactions (3) and (9), five partial mass balances (C, H, O, N, and S) and a heat balance calculate equilibrium. The equilibrium constants are calculated from the Gibbs free energy. This model introduces the sulfur in the biomass global formula and the corresponding formation of SO2 on the products side. |
| Sharma (2008) [56] | Equilibrium model of global reduction reactions | Global gasification reaction: Equilibrium is calculated by five partial mass balances (C, H, O, N and S), five reduction reactions (1), (2), (3), (9) and (14), and two approximations:
The equilibrium constants are calculated from the Gibbs free energy. |
| Huang and Ramaswamy (2009) [58] | Thermodynamic equilibrium model and modified model based on experimental compositions | Global gasification reaction: Equilibrium is calculated by three partial mass balances (C, H and O), and three independent reactions (2), (9) and (14). The equilibrium model is modified by adjusting the equilibrium constants of the reactions (9) and (14) based on experimental data. The coefficient factors were determined by fixing the fraction of CO and CH4 in the syngas from average experimental data values. |
| Abuadala et al. (2010) [59] | None | Global gasification reaction: Equilibrium is calculated by four partial mass balances (C, H, O and N), the independent reactions (3), and the following approximations for char and tar: |
| Karamarkovic and Karamarkovic (2010) [62] | Stoichiometric chemical equilibrium model and modified equilibrium models | The carbon boundary point (CBP) divided the model into two parts:
Equilibrium is calculated by four partial mass balances (C, H, O and N), by three independent reactions (1), (2), and (3) for the first part of the model and the reactions (9) and (11) for the second part of the model. Unknowns of the first part of the model are: temperature, amount of gasifying agent, amount and composition of produced gas. Unconverted carbon is defined only for the first part of the model. Unconverted carbon quantity is equal to zero, when gasification occurs at the CBP. Heterogeneous thermodynamic equilibrium of the produced gas and a given amount of unconverted carbon are calculated in the first part of the model. To achieve a better agreement with the experimental data, the reactions (9) and (11) are multiplied by 0.63 and 420, respectively. The artificial temperature differences for these reactions are 164 K and –226 K (referred by Prins et al. [53] as quasi-equilibrium temperatures). |
| Ngo et al. (2011) [63] | Quasi-equilibrium three-stage model | The three main stages in which this model is divided are:
Stage 1: pyrolysis Equilibrium is calculated by three mass balances (C, H, and O) and the following empirical equations derived on the basis of experimental data: Stage 2: char–gas reactions Equilibrium is calculated by three independent reactions (1), (2), and a secondary char-steam reaction C + 2H2O = CO2 + 2H2 The ratio of steam involved in the char–gas equilibrium reactions (β) is estimated from experimental data: Stage 3: gas-phase reactions Only the water–gas shift reaction is considered (Equation ((9)) being the equilibrium constant corrected by a non-equilibrium factor (κ) |
| Puig-Arnavat et al. (2012) [64] | Modified thermodynamic equilibrium model based on equilibrium constants | Biomass chemical formula was defined as CHxOyNz. All products leaving the gasifier were considered in the gas phase (H2, CO, CH4, CO2, H2O and N2). Preheated air and steam were used as gasifying agents. A pure thermodynamic equilibrium model was used following the procedure described by Zainal et al. [46] or Jarungthammachote and Dutta [42] along with the following modifications:
|
| Azzone et al. (2012) [65] | Equilibrium model | Global gasification reaction: Equilibrium is calculated by three partial mass balances (C, H, and O) by two independent reactions ((3) and (9)) and a heat balance. To consider that not all the carbon participates in the equilibrium reactions, a carbon fraction participating factor (α) was introduced representing the carbon that participates in the equilibrium reactions, while the remaining carbon by-passes the reaction zone. The molar amount of carbon that by-passes the chemical equilibrium is equal to (1 – α) = (1 − δ), being the parameter δ a function of ER defined as follows: |
| Barman et al. (2012) [66] | Realistic equilibrium model | Global gasification reaction: Equilibrium is calculated by three partial mass balances (C, H, and O), by three independent reactions (3), (9) and (11) and a heat balance. Tar is modeled with the chemical formula CH1.003O0.33 and its yield is assumed to be 4.5% (mass percentage). The Equilibrium model is modified by adjusting the equilibrium constant of the methane reaction that is multiplied by 3.5. |
| Silva and Rouboa (2013) [67] | Two-stage equilibrium model | Global gasification reaction: Equilibrium is calculated by three partial mass balances (C, H, and O), by five independent reactions (1)–(3), (9) and (11) and a heat balance. The equilibrium model is called two-stage due to the division in two stages:
This model is considered a modified equilibrium model because the methodology proposed by Jarungthammachote and Dutta [42] to correct the equilibrium constants by means of multiplicative factors was applied. |
| Lim and Lee (2014) [34] | Quasi-equilibrium thermodynamic model with empirical equations | Global gasification reaction: is the heat of the reaction at the standard temperature and pressure, i.e., 25 °C and 1 bar. Equilibrium is calculated by three partial mass balances (C, H, and O), by two independent reactions (3) and (9) and a heat balance. The unconverted carbon is obtained by a correlation 1 − fc based on experimental data of steam fluidized bed gasification. The carbon conversion factor fc used was: The model is modified by including multiplicative factors to correct the equilibrium constants of the reactions (3) and (9) obtained based on 43 experimental data sets for fluidized bed gasifiers as a function of the equivalence ratio: |
| Mendiburu et al. (2014) [68] | Equilibrium models and quasi-equilibrium model | Global gasification reaction: Equilibrium is calculated by three partial mass balances (C, H, and O), by five independent reactions (1)–(3), (9) and (11) and a heat balance. In the present work unconverted carbon is not considered. The pure equilibrium model is modified given rise to three models:
|
| Costa et al. (2015) [70] | Thermo-chemical equilibrium model | Global gasification reaction: Tar is modeled as benzene (C6H6) and its yield is supposed to obey the following empirical relation: Tar = 35.98 exp(−0.0029T) as in Abuadala et al. [59]. Equilibrium is calculated by three partial mass balances (C, H, and O), by three independent reactions (1), (3) and (9) and a heat balance. The equilibrium model is modified by multiplying the equilibrium constant of the three independent reactions (1), (3) and (9) by 0.58, 11.9 and 0.02, respectively. An optimization objective function is used to minimize the error between the numerical results of the syngas composition and the experimental measurements. |
| Rupesh et al. (2015) [71] | Stoichiometric quasi-steady-state model | Global gasification reaction: Tar is modeled as benzene (C6H6) and its yield is supposed to obey the following empirical relation: Tar = 35.98 exp(−0.00298T) as in Abuadala et al. [59] α is a carbon conversion factor expressed as a function of equivalence ratio (ER) and temperature (T): as in Lim and Lee [34]. Equilibrium is calculated by four partial mass balances (C, H, O and N) and by two independent reactions (3) and (9). The equilibrium model is modified by multiplying the equilibrium constant of the independent reactions (3) and (9) by coefficients as function of ER, 0.12e3.2ER and 41–50ER, respectively. These correction coefficients are obtained by minimizing the RMS error between the numerical results of the syngas composition and the experimental measurements. |
| Gagliano et al. (2016) [72] | Robust numerical model | Global gasification reaction: Equilibrium is calculated by three partial mass balances (C, H, and O), by two independent reactions (3) and (9) and a heat balance. Tar is modeled as C6H6.2O0.2 as in Adams [73] and the thermochemical properties assumed to be the same as benzene. The tar and char yields are fixed at 4.5% and 10.5% (mass percentage), respectively. |
| Aydin et al. (2017) [74] | semi-empirical equilibrium model | Global gasification reaction: Tar is assumed to be benzene (C6H6) and ϕ is the carbon fraction factor equal to: Equilibrium is calculated by three partial mass balances (C, H, and O), by two independent reactions (3) and (9), one heat balance and an equivalence correlation function of biomass oxygen content given by: The equilibrium constants of the water-gas shift and methanation reactions are multiplied by coefficients obtained by fitting the model with experimental data within the temperature range of 973–1390 K and the ER range of 0.21–0.5 in order to increase the accuracy of the results: |
| Author(s) (Year) | Model Designation | Model Features | |
|---|---|---|---|
| Altafini et al. (2003) [18] | Equilibrium model based on minimization of the Gibbs free energy | The model contains equations of the:
The Gibbs free energy minimization is reached by the Lagrange multipliers method and the non-linear equation system is solved by the Newton–Raphson method. | |
| Li et al. (2004) [90] | Non-stoichiometric equilibrium model based on direct minimization of Gibbs free energy | Equilibrium is calculated by the minimization of Gibbs free energy using the Lagrange multipliers, mass balances, and energy conservation equation. 42 gaseous and two solid species involving C, H, O, N and S are considered. The model is modified to consider non-equilibrium effects of pyrolysis products like carbon and methane. An availability function is applied to each element, leading to a modified element amount vector affecting the gas, and assuming complete conversion for all elements other than carbon and hydrogen: b = (βcnc, βHnH, nO, nN, nS). The fraction of carbon converted into gaseous species is: βC,1 = 0.25 + 0.75e(-ER/0.23). A fraction of the carbon leaves the system without achieving equilibrium and is produced during the pyrolysis stage. That fraction entering the gas phase exists as methane. Experimental mass balance calculations suggest that this fraction can be approximated by: βc,2 = 0.11(1 − ER). The availability of carbon (overall fraction of carbon entering chemical equilibrium) is: β = βc,1 − βc,2. The availability of hydrogen at equilibrium (one mole of methane comprehends four moles of hydrogen atoms) is: βH = 1 − (4βC,2nC/nH). It is assumed that the reaction system is controlled by non-equilibrium factors and is composed by a mainstream in chemical equilibrium and a bypass zone. | |
| Jarungthammachote and Dutta (2008) [91] | Equilibrium modeling of gasification: Gibbs free energy minimization approach | The model considers that the produced gas is composed by six main components (H2, CO, CO2, CH4, N2 and H2O). Equilibrium is calculated by the minimization of the Gibbs free energy using the Lagrange multipliers and an energy balance assuming a heat loss of 1% of the HHV of the feedstock as in Altafini et al. [18]. The model is modified introducing the carbon conversion effect in the model:
| |
| Baratieri et al. (2010) [85] | Thermodynamic equilibrium model | Equilibrium is calculated by the minimization of the Gibbs free energy using the Lagrange multipliers and an energy balance. The non-linear system is solved using the Newton–Raphson method as in Altafini et al. [18]. The equilibrium model was modified to consider the residual char formed and the methane concentration. To calibrate the model, the measured concentration of hydrocarbons (CH4 and C2H4) and the amount of char collected were considered through:
| |
| Barba et al. (2011) [88] | Gibbs free energy gradient method model | Global gasification reaction Equilibrium is calculated by the minimization of Gibbs free energy, four mass balances (C, H, O and N), two equilibrium reactions (9) and (14), and an energy balance. Two steps were used to model the gasification process. In the first step, RDF is decomposed to produce a carbonaceous residue and a primary gas represented by: In the second step, the produced gas composition of the first step is modified by the water shift (9) and steam reforming (14) reactions. In this model an unreacted solid carbonaceous residue is formed and considered insensitive to process operating conditions. Consequently, two parameters (δ and γ) are defined as function of gasifying medium/feedstock ratio and reaction temperature: Steam: Air: Where ; | |
| Buragohain et al. (2012) [83] | Semi-equilibrium non-stoichiometric thermodynamic model | Equilibrium is calculated minimizing the Gibbs free energy using the Lagrange multipliers method and subjecting mass balance to constraints and an energy balance. To modify the model, the extent of carbon conversion was considered. The designation semi-equilibrium comes from the carbon conversion being taken as an independent parameter; four levels of carbon conversion (70, 80, 90, 100%) were considered. FACTSAGE software was used to carried out simulations. | |
| Materazzi et al. (2013) [49] | Thermodynamic equilibrium model | This equilibrium model follows the overall framework of single-stage equilibrium models. The system considers 43 different species. The ashes are inert but considered as part of thermal capacity in the reactor. Heat losses (Qloss) in the respective gasification phase is estimated to be 10% of the HHV of the feedstock supplied in the conversion phase. The thermodynamic equilibrium model of a two-stage process is formulated as follows: The char (solid carbon) is further converted in a steam–oxygen environment according to: The model uses the correlations given by Matsui et al. [92] and Linjewile et al. [94] with splitting factors 1.3 and 1.2 for the temperature range of 700–800 °C. A solid carbonaceous residue constitutes the unreacted solid from the first stage. The equilibrium is calculated, including the preliminary conversion process, by the minimization of the objective function, mass and energy balances for the two sequential stages. The solver applies the generalized reduced gradient method to solve the nonlinear system. | |
| Sreejith et al. (2013) [93] | Gibbs free energy minimization model | Equilibrium for steam gasification is calculated minimizing the Gibbs free energy. The gases are treated as real gases using the Redlich–Kwong equation of state. The mixture properties are computed based on the Amagat’s law. Minimization is performed in each iterative loop till the convergence of the mixture compressibility factor is achieved using the simulating annealing algorithm. This model is proposed in order to arrive at the optimum values for the reactor working parameters of temperature and pressure. Global gasification reaction: CxHyOz + aH2O + heat → bCO + dCO2 + eH2 + fCH4 + gH2O The constraints are elemental balances of C, H, and O as obtained from the global gasification chemical reaction and the non-negativity constraint | |
| Kangas et al. (2014) [30] | Super-equilibrium with the constrained Gibbs energy method | A chemical system with 14 species in the gaseous phase (H2, CO, CH4, C2H2, C2H4, C2H6, C3H8, C6H6, C10H8, NH3, O2, N2, H2O, SO2), water in liquid phase (H2O) and char and ash (C and SiO2) in the solid phases is considered. Equilibrium is calculated by the minimization of the Gibbs free energy via the Lagrange multipliers. The following constraints were considered: | |
| Constraint | Expression (mol/kg dry biomass) | ||
| C in char | 71.664 + 0.012906 × (T/K) | ||
| C in tar | 3.0 | ||
| N in ammonia | 0.042 | ||
| C in hydrocarbons | 17.642 − 0.009545 × (T/K) | ||
| H in hydrocarbons | 50.376 − 0.02732 × (T/K) | ||
| C in unsaturated and aromatic hydrocarbons | 3.9261 − 0.00208 × (T/K) | ||
| CH4 | 7.074 − 0.003 × (T/K) | ||
| C2H2 | 0.06454 − 0.00004 × (T/K) | ||
| C2H4 | 2.987 − 0.002 × (T/K) | ||
| C2H6 | 1.196 − 0.001 × (T/K) | ||
| C3H8 | 0.150921 − 0.000155 × (T/K) | ||
| C6H6 | 0.27 | ||
Six methods for modeling of global or local equilibrium were implemented:
| |||
| Mendiburu et al. (2014) [86] | Thermochemical equilibrium modeling: Constrained and unconstrained non-stoichiometric models | Global gasification reaction Equilibrium is calculated by the minimization of Gibbs free energy using the Lagrange multipliers, three mass balances (C, H and O) and a heat balance. Four models were developed: M1—Pure non-stoichiometric equilibrium model M2—non-stoichiometric constrained equilibrium model - methane content was constrained by the following empirical correlation: where the variables X1, X2 and X3 are the hydrogen to moisture content in the biomass, the normalized equivalence ratio and normalized gasification temperature, respectively. M3—kinetic constraint that determines the apparent gasification rate was considered. M4—implements simultaneously the two aforementioned constraints. | |
| Ghassemi and Markadeh (2014) [84] | Modified equilibrium model based on Gibbs free energy minimization | Global gasification reaction: Equilibrium is calculated by the minimization of Gibbs free energy using the Lagrange multipliers, three elemental mass balances (C, H and O) and a heat balance. The equilibrium model is modified by introducing carbon conversion and tar formation constraints. The carbon conversion expression of Azzone et al. [65] is implemented: Tar is modeled with the chemical formula CH1.003O0.33 and its yield is assumed to be 4.5% (mass percentage) as in Barman et al. [66] | |
| Yakaboylu et al. (2015) [89] | Constrained equilibrium model | The model comprises two parts:
The constraints included are the carbon and hydrogen gasification efficiencies and constrained amounts for specific compounds. A distinctive aspect of the model is the consideration of real gases using the Peng−Robinson equation of state. | |
| Vakalis et al. (2016) [32] | Multi-stage thermodynamic model: Multi-box approach | The multi-box approach is used due to the separation of the reactor into various processes, instead of using a conventional single-stage (also known as black-box) model approach.
| |
| Biagini et al. (2016) [33] | Bi-equilibrium model | The naming bi-equilibrium model finds explanation in the bypass of the oxidation zone of some pyrolysis products usually underestimated in equilibrium models. The model is developed considering the multi-phase nature of the gasification process:
The reactor heat losses were assumed to be 5% of the biomass thermal energy. The ash properties were assumed from SiO2. | |
| Gambarotta et al. (2018) [87] | Non-stoichiometric equilibrium model | Global gasification reaction: Equilibrium is calculated minimizing the Gibbs free energy, five mass balances (C, H, O, N and S) and an energy balance. α is the factor that takes into account the carbon not participating in the gasification. This factor is a function of the air-to-fuel ratio proposed by Azzone et al. [65] and firstly introduced by Li et al. [79]. Tar production is neglected. | |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ferreira, S.; Monteiro, E.; Brito, P.; Vilarinho, C. A Holistic Review on Biomass Gasification Modified Equilibrium Models. Energies 2019, 12, 160. https://doi.org/10.3390/en12010160
Ferreira S, Monteiro E, Brito P, Vilarinho C. A Holistic Review on Biomass Gasification Modified Equilibrium Models. Energies. 2019; 12(1):160. https://doi.org/10.3390/en12010160
Chicago/Turabian StyleFerreira, Sérgio, Eliseu Monteiro, Paulo Brito, and Cândida Vilarinho. 2019. "A Holistic Review on Biomass Gasification Modified Equilibrium Models" Energies 12, no. 1: 160. https://doi.org/10.3390/en12010160
APA StyleFerreira, S., Monteiro, E., Brito, P., & Vilarinho, C. (2019). A Holistic Review on Biomass Gasification Modified Equilibrium Models. Energies, 12(1), 160. https://doi.org/10.3390/en12010160






