Three-Dimensional Temperature Field Calculation and Analysis of an Axial-Radial Flux-Type Permanent Magnet Synchronous Motor
Abstract
1. Introduction
2. Heat Source Determination for Thermal Analysis
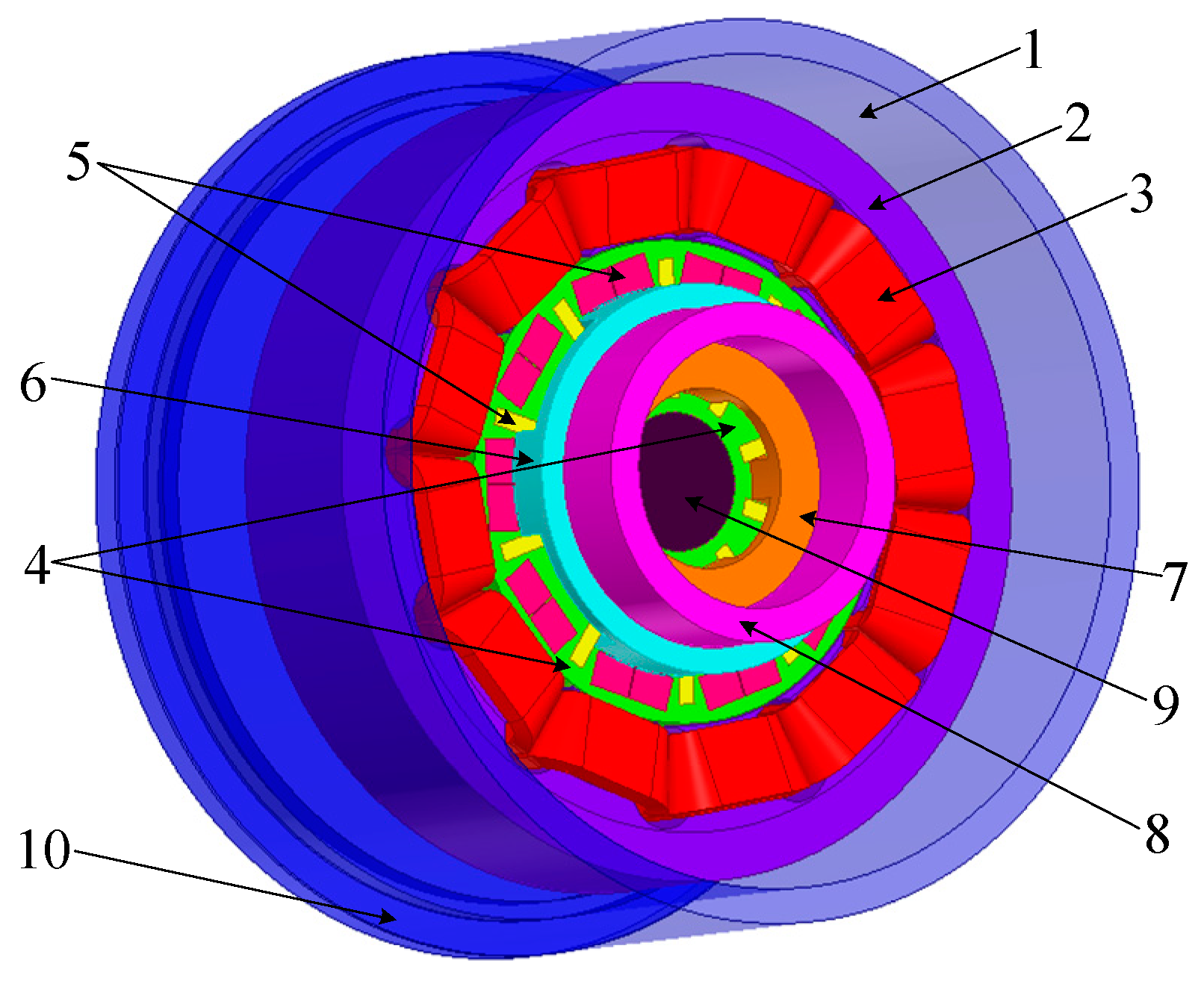
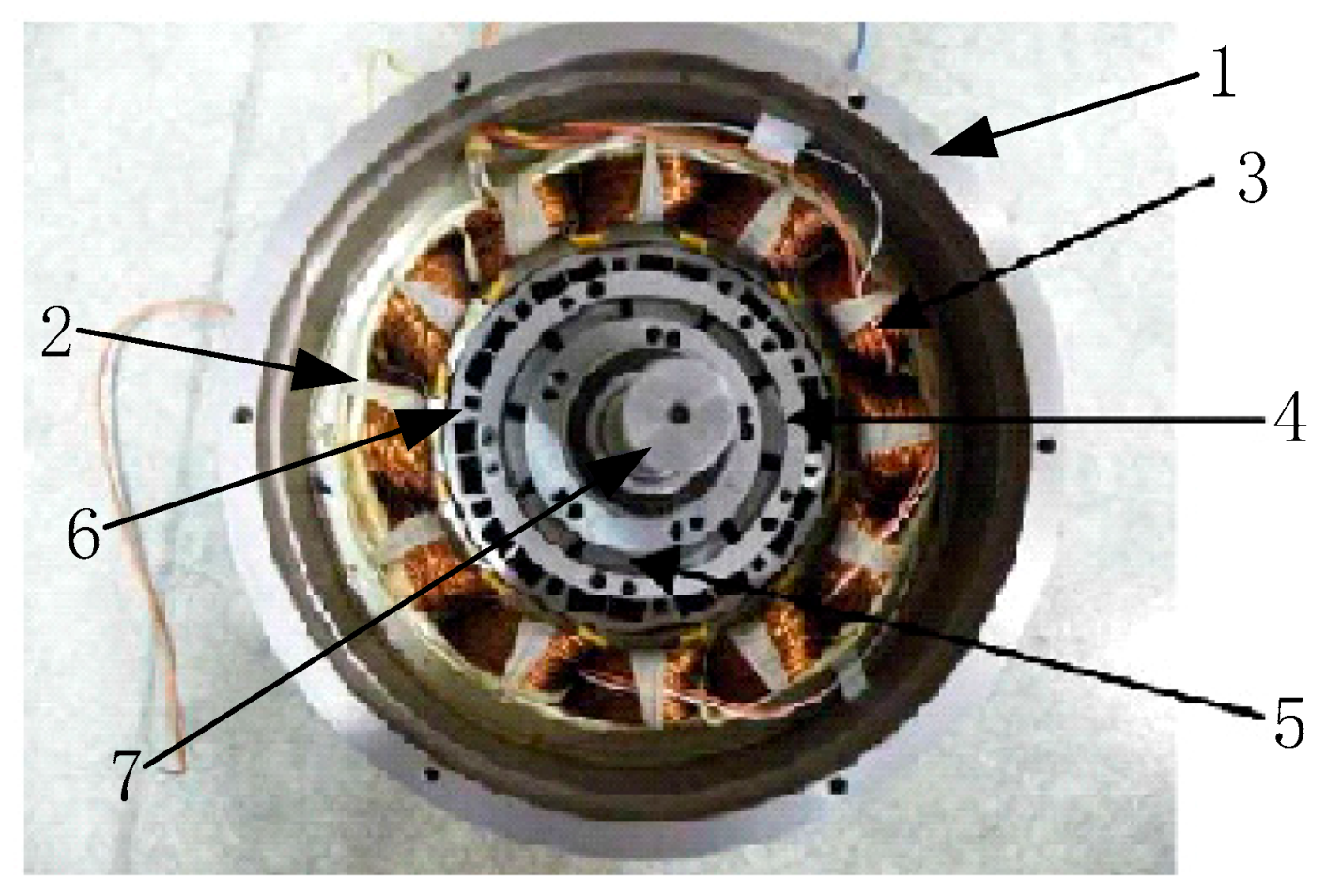
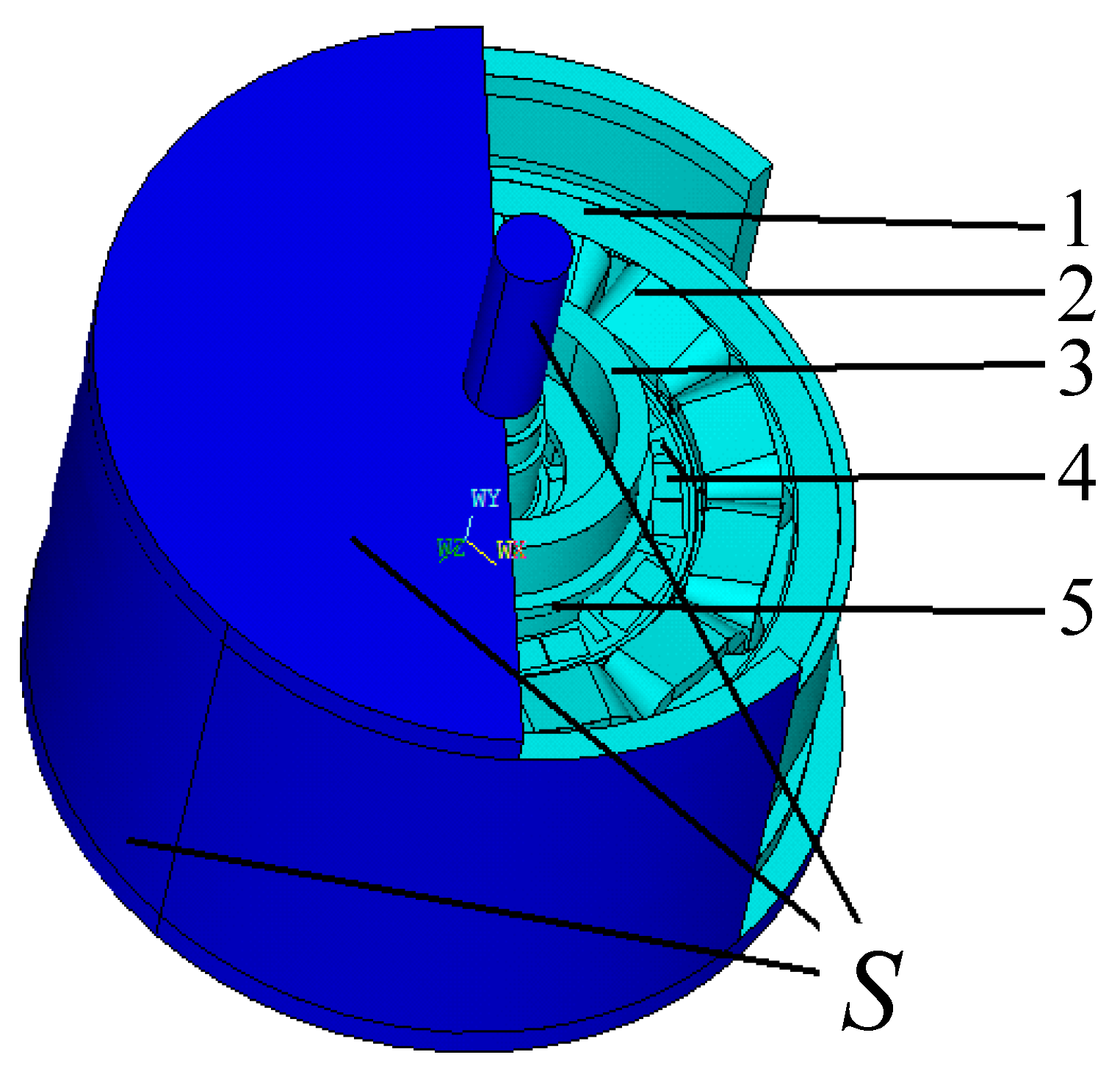
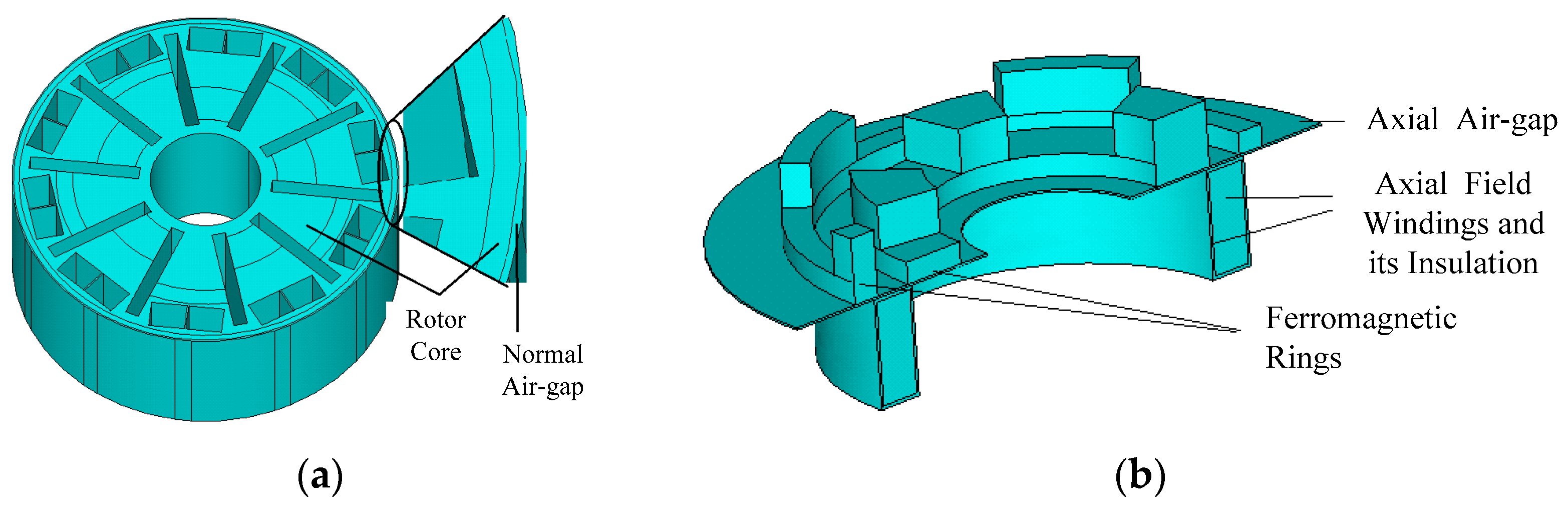
2.1. Physical Structure of ARFTPMSM
2.2. Mathematical Model for Electromagnetic Calculations
- The current harmonics produced by the controller are ignored, so the machine is fed on ac source.
- Because of the low frequency and the multi-strand windings, the displacement current within the armature windings is regardless.
- According to the temperature measurements, the temperature rise of the machine is very small, thus, the influences of the temperature on material conductivity and permeability are ignored.
- Since the end cover and the enclosure are developed by using high magnetic permeability materials, thus, the flux that is leaked into the air can be ignored.
3. Mathematical Model for Thermal Calculation
3.1. Assumptions and Boundary Conditions
- Since the rotor core is developed by using solid iron, the eddy loss is distributed with a certain thickness according to the skin effect. So, when considering the real state of the loss distributions, the eddy current loss of the rotor core is distributed within a certain penetration depth of the rotor core.
- During the loss calculations, the temperature effect on the material properties are ignored, thus, the influence of the temperature on the heat transfer is regardless.
- Due to the low rotating speed and totally enclosed structure, the influence of the flowing air within the machine on the heat transfer is also ignored.
- During the assembly process, the machine is vacuum-dipped to evacuate the air within the connect joints, so it regards that there is no air-gap between the connection joints.
3.2. Thermal Conductivity of Air-Gap
3.2.1. Thermal Conductivity of Normal Air-Gap
3.2.2. Thermal Conductivity of Axial Air-Gap
3.3. Heat Transfer Coefficient of the Enclosure
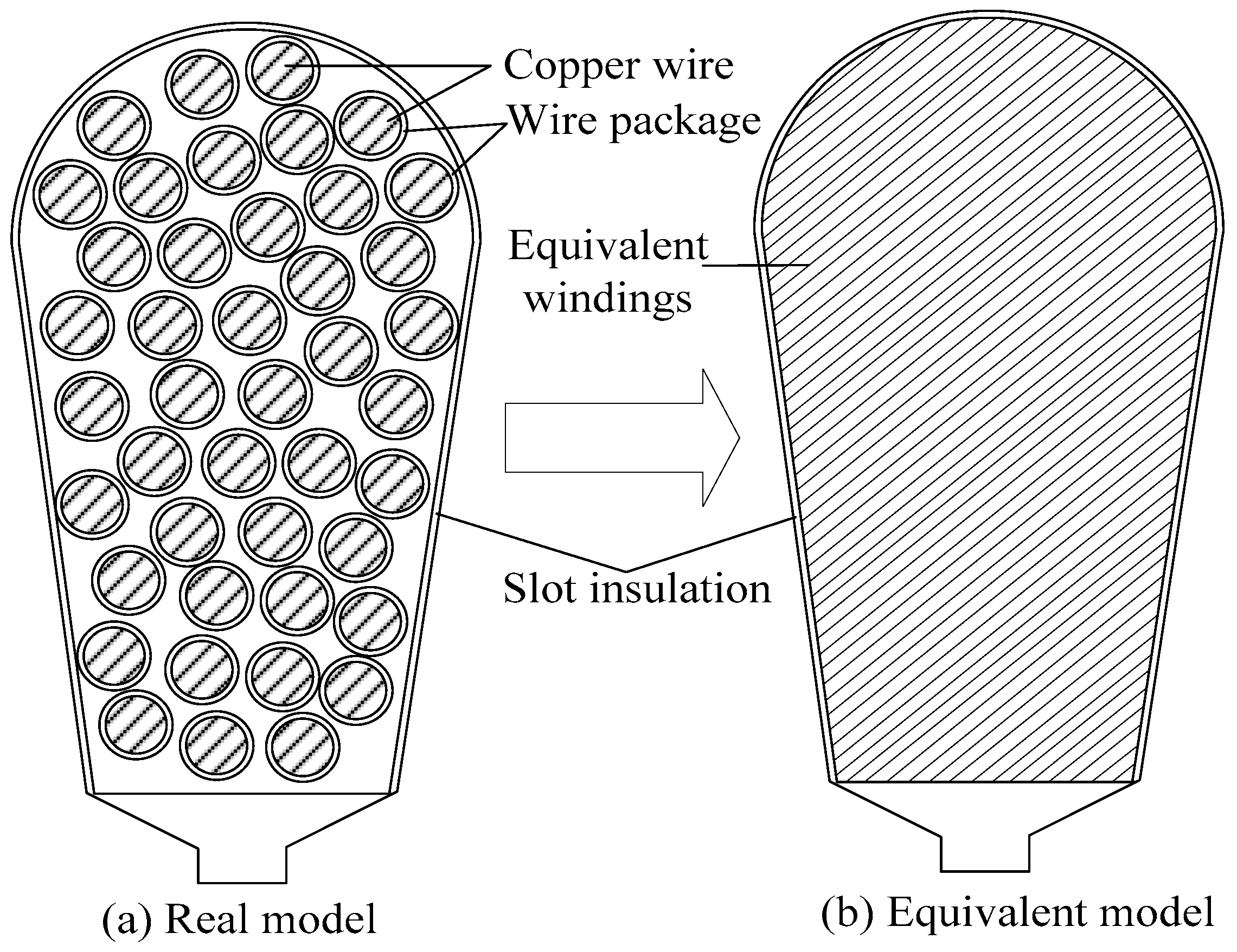
3.4. Thermal Conductivity of Armature Windings
4. Steady-State Temperature Field Calculation for Prototype
4.1. Verification for Mathematical Models
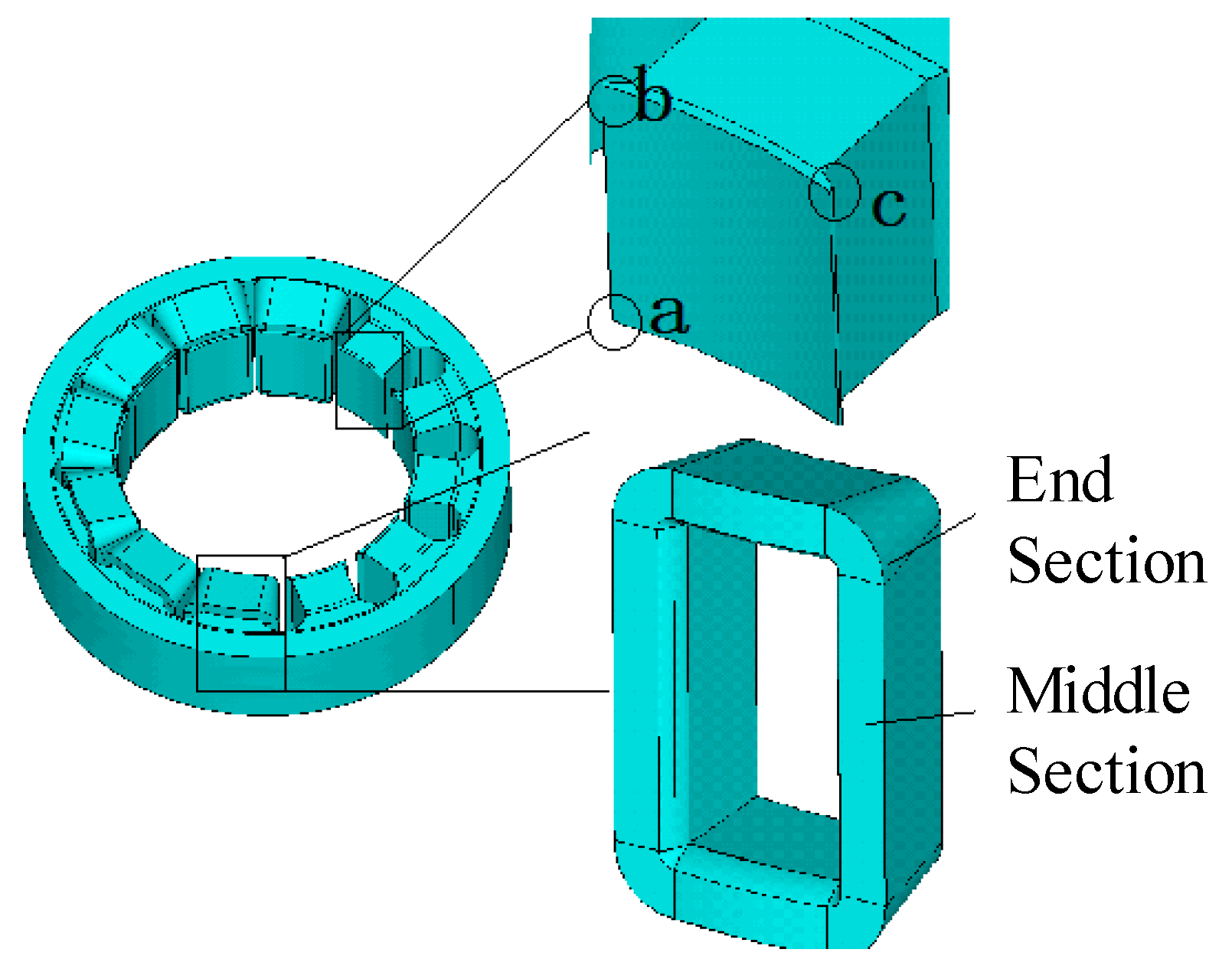
4.2. Temperature Field under Different AMMF
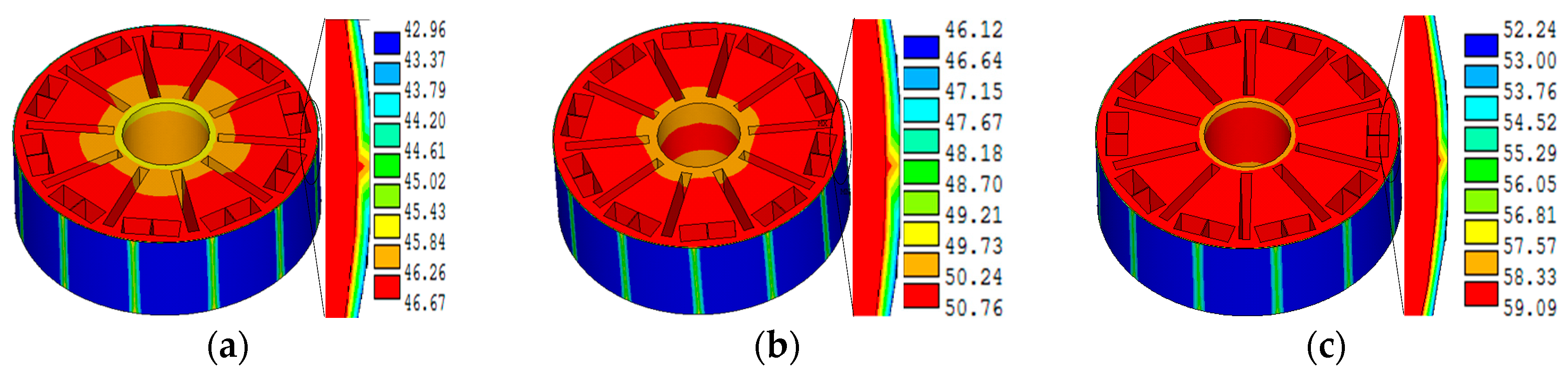
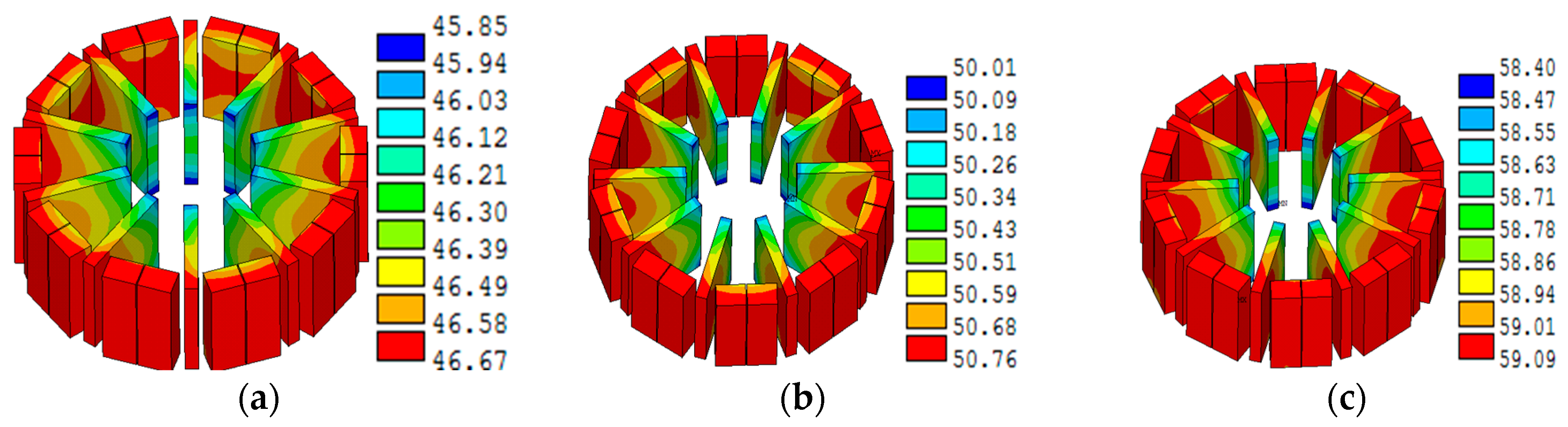
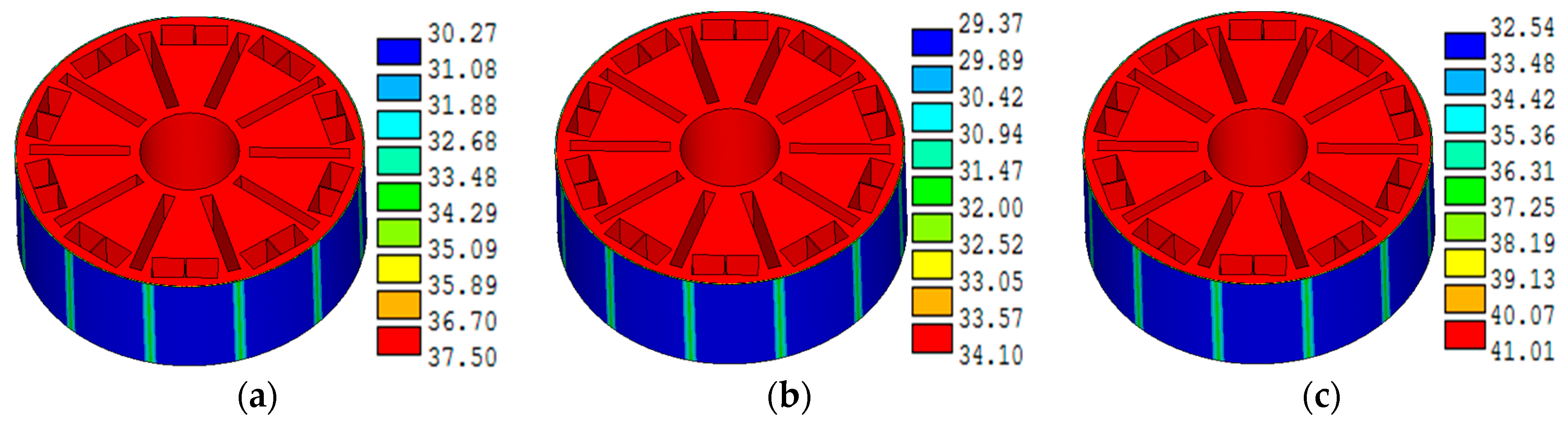
4.2.1. Thermal Analysis for the Rotor Components
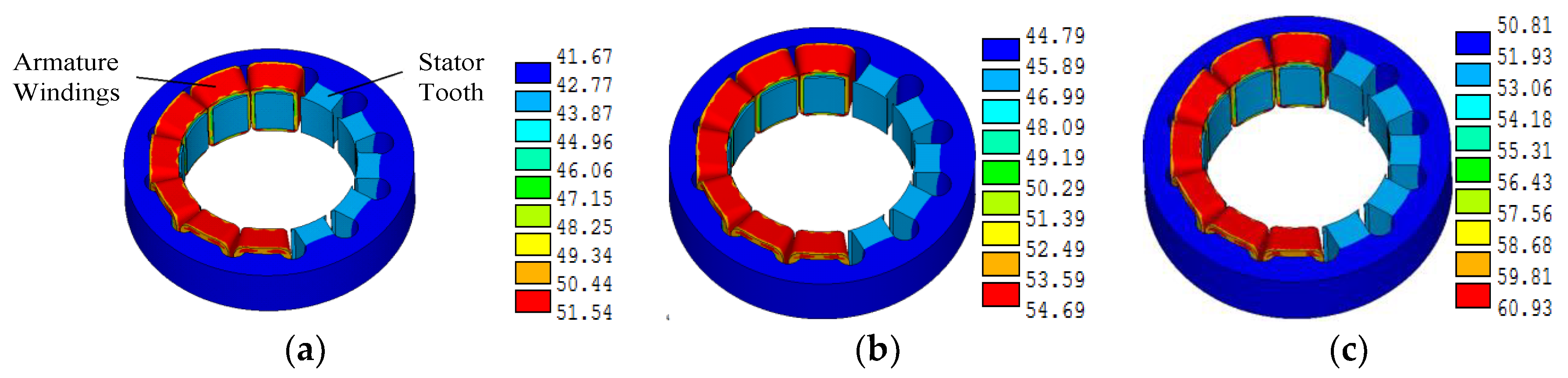
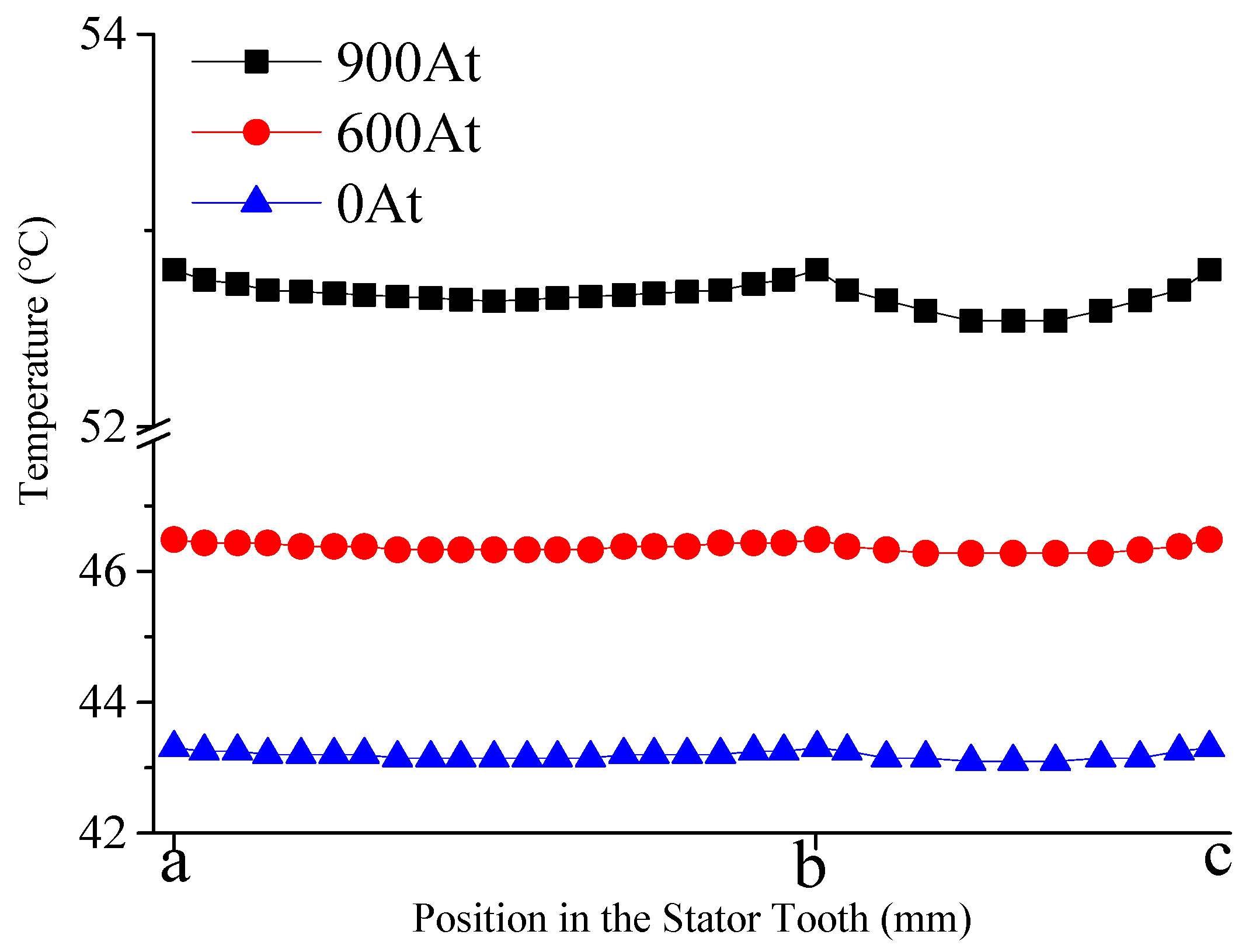
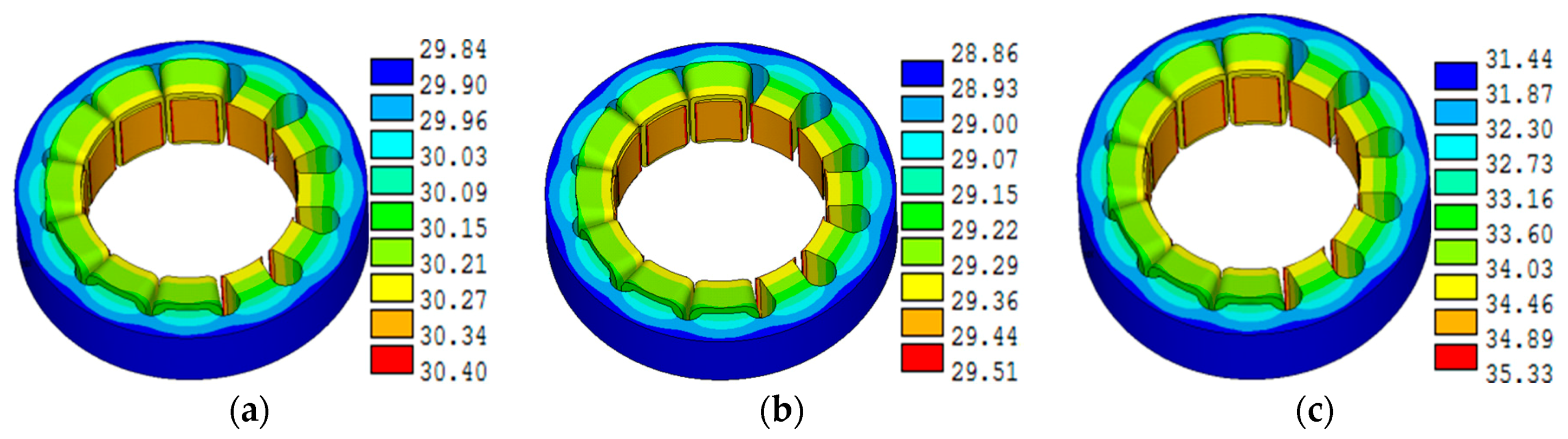
4.2.2. Thermal Analysis for the Stator Components
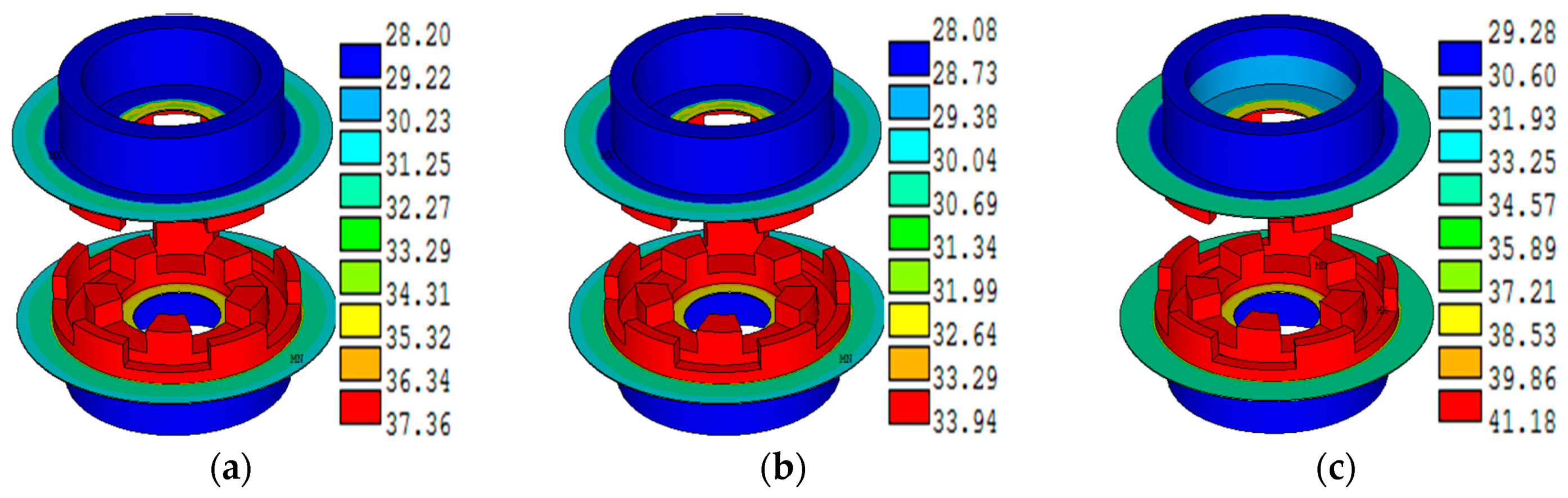
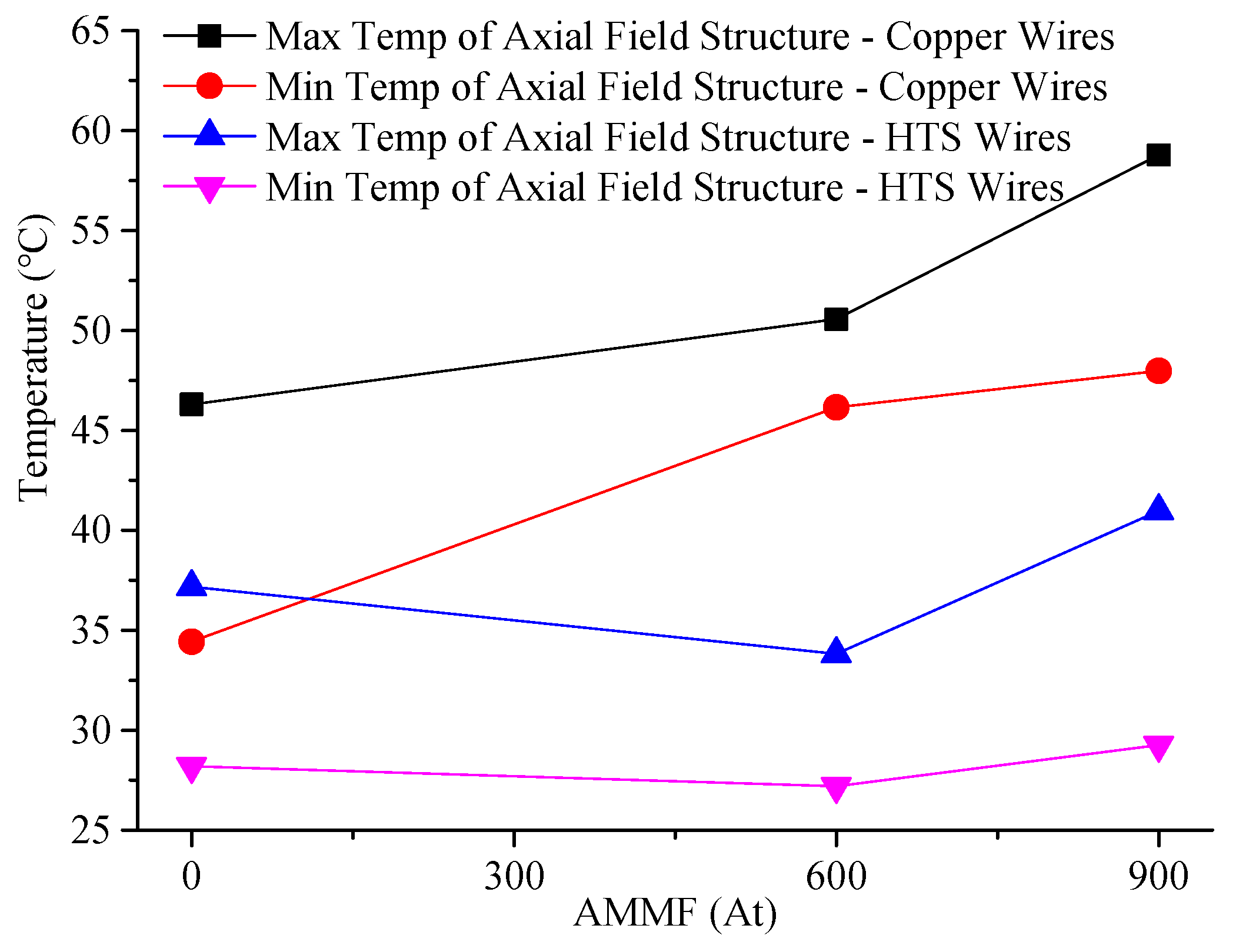
4.2.3. Thermal Analysis for Axial Field Structure
5. Temperature Field for ARFTPMSM with HTS Windings
5.1. Temperature Field for the Rotor Components
5.2. Temperature Field for the Stator
5.3. Temperature Field for the Axial Field Structure
6. Conclusions
- Validated by the experiments carried out on the prototype, the differences between the calculations and the measurements of the end armature temperature and enclosure are 1.05 °C and 0.96 °C, respectively. It indicates that the established models and the presented assumptions are reasonable for the thermal analysis of ARFTPMSM.
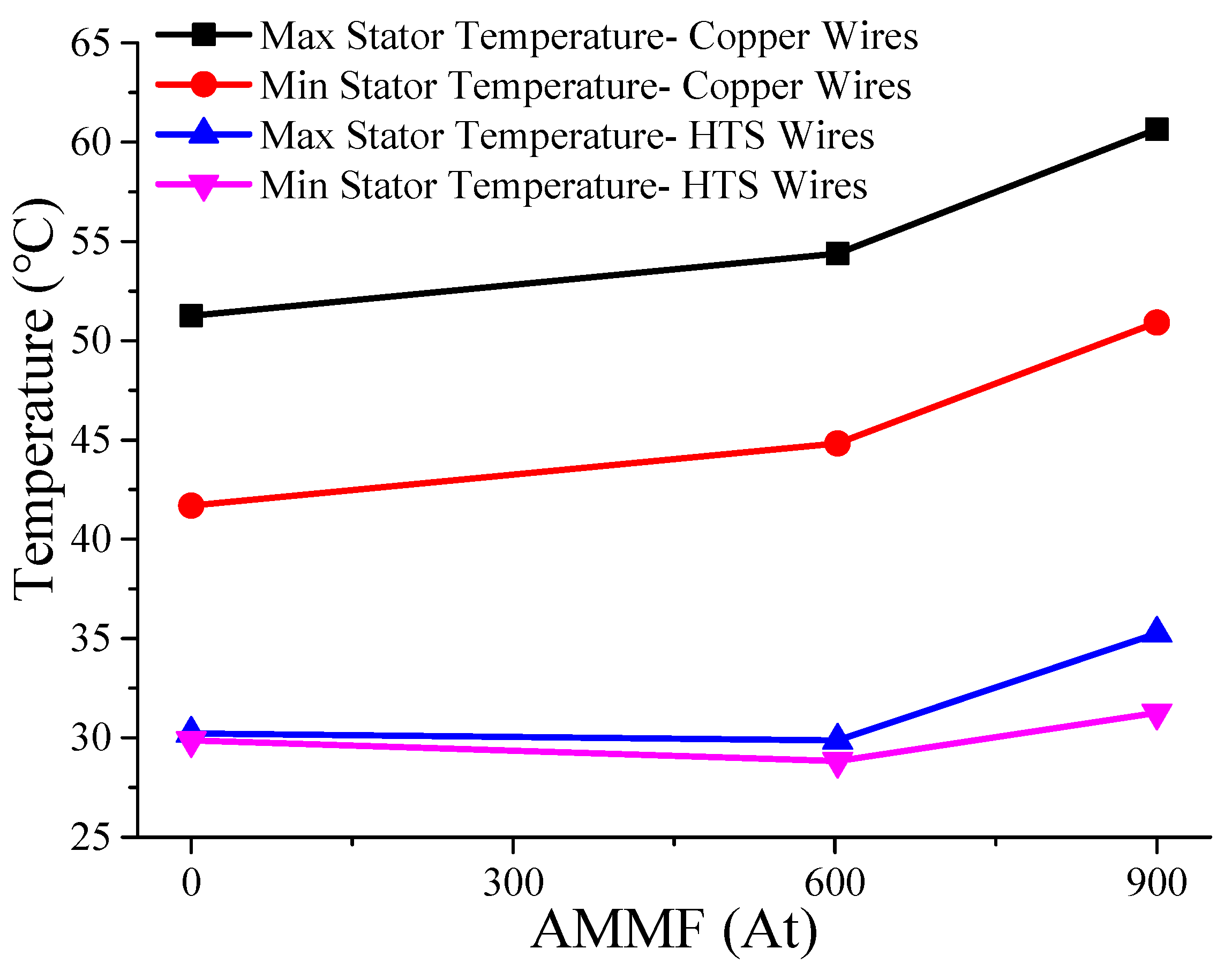
- For machine with copper wires, although AMMF can adjust the normal air gap flux density, but it can also increase the harmonic components of the normal air gap flux density. Thus, the temperature of machine components increases as AMMF increases, e.g., the max temperature of the rotor core of the machine under 600 At and 900 At AMMF increases by about 4.09 °C and 12.42 °C, respectively, while when compared with the machine with 0 At AMMF. While AMMF rises from 0 At to 600 At, the average temperature of armature windings increases about 6.9%; however, while AMMF rises from 600 At to 900 At, the average temperature increases about 15.2%.
- While the machine is developed by HTS wires, the temperature distribution is quite difference with the machine with copper wires. Viewing from the temperature distribution of rotor, the max rotor temperature of machine with copper wires is about 47.96% larger than that of the machine with HTS wires that are under 600 At AMMF.
- For the machine with copper wires, the differences between the max and min stator temperature under 0 At, 600 At, and 900 At are 9.57 °C, 9.57 °C, and 9.74 °C, respectively. The temperature gradient do not change as AMMF increases. However, for a machine with HTS wires, the differences between max and min stator temperature under 0 At, 600 At, and 900 At are 0.35 °C, 1.04 °C, and 4 °C, respectively. The temperature gradient increases as AMMF.
Author Contributions
Funding
Conflicts of Interest
References
- Baik, S.K.; Kwon, Y.K.; Kim, H.M.; Kim, S.H.; Lee, J.D.; Kim, Y.C.; Park, H.J.; Kwon, W.S.; Park, G.S. Electrical Parameter Evaluation of a 1MW HTS Motor Via Analysis and Experiments. Cryogenics 2009, 49, 271–276. [Google Scholar] [CrossRef]
- Kwon, Y.K.; Kim, H.M.; Baik, S.K.; Lee, E.Y.; Lee, J.D.; Kim, Y.C.; Lee, S.H.; Hong, J.P.; Jo, Y.S.; Ryu, K.S. Performance Test of a 1MW Class HTS Synchronous Motor for Industrial Application. Phys. C Supercond. 2008, 468, 2081–2086. [Google Scholar] [CrossRef]
- Baik, S.; Kwon, Y. Electrical Design of a 17 MW Class HTS Motor for Ship Propulsion. J. Supercond. Nov. Magn. 2013, 26, 1283–1287. [Google Scholar] [CrossRef]
- Kovalev, L.K.; Ilushin, K.V.; Penkin, V.T.; Kovalev, K.L.; Koneev, S.M.A.; Modestov, K.A.; Larionoff, S.A.; Gawalek, W.; Oswald, B. Electrical Machines with Bulk HTS Elements. Phys. C Supercond. 2001, 357–360, 860–865. [Google Scholar] [CrossRef]
- Ji-Kwang, L.; Sang-Ho, P.; Yungil, K.; Seyeon, L.; Woo-Seok, K.; Kyeongdal, C.; Song-Yop, H. Electrical Properties Analysis and Test Result of Windings for a Fully Superconducting 10 Hp Homopolar Motor. IEEE Trans. Appl. Supercond. 2012, 22, 5201405. [Google Scholar] [CrossRef]
- Yoshida, K.; Matsumoto, H. Electromagnetic Force Analysis of Hts Bulk in DC-Magnetic Fields Due to Electromagnet. Phys. C Supercond. 2002, 378, 848–852. [Google Scholar] [CrossRef]
- Masson, P.J.; Tixador, P.; Ordonez, J.C.; Morega, A.M.; Luongo, C.A. Electro-Thermal Model for HTS Motor Design. IEEE Trans. Appl. Supercond. 2007, 17, 1529–1532. [Google Scholar] [CrossRef]
- Nakamura, T.; Miyake, H.; Ogama, Y.; Morita, G.; Muta, I.; Hoshino, T. Fabrication and Characteristics of HTS Induction Motor by the Use of Bi-2223/Ag Squirrel-Cage Rotor. IEEE Trans. Appl. Supercond. 2006, 16, 1469–1472. [Google Scholar] [CrossRef][Green Version]
- Jin, J.; Zheng, L.H.; Guo, Y.G.; Zhu, J.G.; Grantham, C.; Sorrell, C.C.; Xu, W. High-Temperature Superconducting Linear Synchronous Motors Integrated with HTS Magnetic Levitation Components. IEEE Trans. Appl. Supercond. 2012, 22, 5202617. [Google Scholar]
- Fang, J.; Sheng, L.; Li, D.; Zhao, J.; Li, S.; Qin, W.; Fan, Y.; Zheng, Q.L.; Zhang, W. Influence Analysis of Structural Parameters and Operating Parameters on Electromagnetic Properties of HTS Linear Induction Motor. Phys. Procedia 2012, 27, 408–411. [Google Scholar] [CrossRef][Green Version]
- Gamble, B.; Snitchler, G.; MacDonald, T. Full Power Test of a 36.5 MW HTS Propulsion Motor. IEEE Trans. Appl. Supercond. 2011, 21, 1083–1088. [Google Scholar] [CrossRef]
- Huaming, W.; Bailey, W.; Goddard, K.; Al-Mosawi, M.; Beduz, C.; Yifeng, Y. Performance Test of a 100 kW HTS Generator Operating at 67 K-77 K. IEEE Trans. Appl. Supercond. 2009, 19, 1652–1655. [Google Scholar] [CrossRef]
- Morita, G.; Nakamura, T.; Muta, I. Theoretical Analysis of a YBCO Squirrel-Cage Type Induction Motor Based on an Equivalent Circuit. Supercond. Sci. Technol. 2006, 19, 473–478. [Google Scholar] [CrossRef]
- Sato, K.; Kumagai, M.; Ito, K.; Watanabe, Y.; Gocho, Y. An Approach to Optimal Thermal Design of Superconducting Generator Rotor. IEEE Trans. Energy Convers. 1986, EC-1, 217–224. [Google Scholar] [CrossRef]
- Kulkarni, D.P.; Rupertus, G.; Chen, E. Experimental Investigation of Contact Resistance for Water Cooled Jacket for Electric Motors and Generators. IEEE Trans. Energy Convers. 2012, 27, 204–210. [Google Scholar] [CrossRef]
- Yen, F.; Li, J.; Zheng, S.J.; Liu, L.; Ma, G.T.; Wang, J.S.; Wang, S.Y.; Liu, W. A Single-Sided Linear Synchronous Motor with a High Temperature Superconducting Coil as the Excitation System. Supercond. Sci. Technol. 2010, 23, 105015. [Google Scholar] [CrossRef]
- Li, W.; Han, J.; Huo, F.; Zhou, X.; Zhang, Y.; Li, Y. Influence of the End Ventilation Structure Change On the Temperature Distribution in the End Region of Large Water-Hydrogen-Hydrogen Cooled Turbogenerator. IEEE Trans. Energy Convers. 2013, 28, 278–288. [Google Scholar]
- Ruan, L.; Gu, G.; Tian, X.; Yuan, J. The Comparison of Cooling Effect between Evaporative Cooling Method and Inner Water Cooling Method for the Large Hydro Generator. In Proceedings of the International Conference on Electrical Machines and Systems, Seoul, Korea, 8–11 October 2007; pp. 989–992. [Google Scholar]
- Li, W.; Qiu, H.; Yi, R.; Zhang, X.; Li, L. Three-Dimensional Electromagnetic Field Calculation and Analysis of Axial-Radial Flux-Type High-Temperature Superconducting Synchronous Motor. IEEE Trans. Appl. Supercond. 2013, 23, 5200607. [Google Scholar] [CrossRef]
- Sander, M.; Grilli, F. FEM-Calculations on the Frequency Dependence of Hysteretic Losses in Coated Conductors. J. Phys. Conf. Ser. 2010, 234, 022030. [Google Scholar] [CrossRef]
- Zhao, Y.; Sorrell, C.C.; Cheng, C.H. Frequency Dependent Behavior of AC Loss in High Temperature Superconductors. Phys. C Supercond. Its Appl. 2001, 357, 614–616. [Google Scholar] [CrossRef]
- Tsukamoto, O.; Li, Z. Ac Loss Characteristics of Bi2223/Ag Sheathed Tape Wires Subjected to Mechanical Strains and Stresses. Supercond. Sci. Technol. 2007, 20, S282–S292. [Google Scholar] [CrossRef]
- Wang, Y.; Zhao, X.; Han, J.; Dai, S.; Xiao, L.; Lin, L. AC Losses and Mechanical Stability in 630 kV a Three-Phase HTS Transformer Windings. Supercond. Sci. Technol. 2007, 20, 463. [Google Scholar] [CrossRef]
- Li, C.; Pei, Y.; Ni, R.; Cheng, S. Analysis of 3D Static Temperature Field of Water Cooling Induction Motor in Mini Electric Vehicle. In Proceedings of the International Conference on Electrical Machines and Systems (ICEMS), Beijing, China, 20–23 August 2011; pp. 1–5. [Google Scholar]
- Li, W.; Song, C.; Li, L.; Cao, J. Electromagnetic analysis of axial-radial flux-type fully superconducting synchronous motor. In Proceedings of the CESS, New York, NY, USA, 30 May–1 June 2011; pp. 96–101. (In Chinese). [Google Scholar]
- Sahin, F. Design and Development of a High-Speed Axial-Flux Permanent-Magnet Machine. Ph.D. Thesis, Technische Universiteit Eindhoven, Eindhoven, The Netherlands, 2001. [Google Scholar]
- Madani, N. Design of a Permanent Magnet Synchronous Generator for a Vertical Axis Wind Turbine. Master’s Thesis, Teknik Och Teknologier, Stockholm, Sweden, 2011. [Google Scholar]
- Zanis, R.; Borisavljevic, A.; Jansen, J.W.; Lomonova, E.A. Iron Loss Investigation of Miniaturized Magnetic Gears Having Solid Cores. In Proceedings of the International Conference on Electrical Machines and Systems, Boston, MA, USA, 17–21 July 2014; pp. 3078–3082. [Google Scholar]
- Li, W.; Cao, J.; Zhang, X. Electrothermal Analysis of Induction Motor with Compound Cage Rotor Used for Phev. IEEE Trans. Ind. Electron. 2010, 57, 660–668. [Google Scholar]
- Xyptras, J.; Hatziathanassiou, V. Thermal Analysis of an Electrical Machine Taking Into Account the Iron Losses and the Deep-Bar Effect. IEEE Trans. Energy Convers. 1999, 14, 996–1003. [Google Scholar] [CrossRef]
- Ball, K.S.; Farouk, B.; Dixit, V.C. An Experimental Study of Heat Transfer in a Vertical Annulus with a Rotating Inner Cylinder. Int. J. Heat Mass Transf. 1989, 32, 1517–1527. [Google Scholar] [CrossRef]
- Hatziathanassiou, V.; Xypteras, J.; Archontoulakis, G. Electrical-Thermal Coupled Calculation of an Asynchronous Machine. Arch. Elektrotech. 1994, 77, 117–122. [Google Scholar] [CrossRef]
- Wei, Y.; Meng, D.; Wen, J. Heat Transfer Inner Electric Machines; China Machine Press: Beijing China, 1998. (In Chinese) [Google Scholar]
- Cao, J.; Li, W.; Cheng, S.; Zhang, X. Temperature Field Calculation and Associated Factor Analysis of Induction Motor with Compound Cage Rotor. In Proceedings of the CSEE, Dallas, TX, USA, 21 April 2008; pp. 96–103. (In Chinese). [Google Scholar]






| Items | Value |
|---|---|
| Rated power/kW | 3.5 |
| Frequency/Hz | 20.83 |
| Synchronous speed/(r/min) | 250 |
| Core length/mm | 50 |
| Stator outer diameter/mm | 230 |
| Stator inner diameter/mm | 140 |
| Slots | 12 |
| Poles | 10 |
| Cooling type | Self-cooling |
| Items | Material | Relative Permeability | Bulk Conductivity |
|---|---|---|---|
| Stator core | DW310-35 | Refer to Figure 4 | 0 |
| Armature windings | Copper wire | 1 | 5.91 × 107 S/m |
| Rotor core | DT-4 | Refer to Figure 4 | 1.03 × 107 S/m |
| Permanent Magnets | N35EH | 1.05 | 6.85 × 105 S/m |
| Outer Ferromagnetic Ring | DT-4 | Refer to Figure 4 | 1.03 × 107 S/m |
| Inner Ferromagnetic Ring | DT-4 | Refer to Figure 4 | 1.03 × 107 S/m |
| Axial Field Windings | Copper bar | 1 | 5.91 × 107 S/m |
| Shaft | Steel 45 | Refer to Figure 4 | 2.0 × 107 S/m |
| Enclosure | Steel 45 | Refer to Figure 4 | 2.0 × 107 S/m |
| End Cover | Steel 45 | Refer to Figure 4 | 2.0 × 107 S/m |
| Items | Value |
|---|---|
| Stator core loss | 1.96 W |
| windings loss | 17.4 W |
| Ferromagnetic rings loss | 0.76 W |
| Rotor loss | 14.8 W |
| Items | Mass Density | Specific Heat Capacity | Thermal Conductivity |
|---|---|---|---|
| Stator core | 7600 kg/m3 | 470 J/(kg K) | 43 W/(m K) in x,y-axis 0.62 W/(m K) in z-axis |
| Armature windings | 8900 kg/m3 | 504 J/(kg K) | 1.7 W/(m K) |
| Slot insulation | 1020 kg/m3 | 1000 J/(kg K) | 0.23 W/(m K) |
| Slot wedge | 900 kg/m3 | 800 J/(kg K) | 0.25 W/(m K) |
| Normal air-gap | 1.2 kg/m3 | 1005 J/(kg K) | 0.045 W/(m K) |
| Permanent magnets | 7600 kg/m3 | 502 J/(kg K) | 12 W/(m K) |
| Rotor core | 7870 kg/m3 | 460J J/(kg K) | 48 W/(m K) |
| Shaft | 7850 kg/m3 | 472 J/(kg K) | 49.8 W/(m K) |
| Enclosure & end cover | 7850 kg/m3 | 472 J/(kg K) | 49.8 W/(m K) |
| Axial field windings | 8900 kg/m3 | 504 J/(kg K) | 398 W/(m K) |
| Axial ferromagnetic rings | 7870 kg/m3 | 460 J/(kg K) | 48 W/(m K) |
| Axial air gap | 1.2 kg/m3 | 1005 J/(kg K) | 0.045 W/(m K) |
| Items | Calculation Results | Measurements | Error (%) |
|---|---|---|---|
| End armature windings/°C | 51.15 | 50.10 | 2.10% |
| Enclosure/°C | 32.04 | 33.00 | −2.91% |
| AMMF/AT | End Armature Windings/°C | Enclosure/°C |
|---|---|---|
| 0 | 51.15 | 32.0 |
| 600 | 54.7 | 36.8 |
| 900 | 60.9 | 52.8 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, D.; Wen, Y.; Li, W.; Feng, B.; Cao, J. Three-Dimensional Temperature Field Calculation and Analysis of an Axial-Radial Flux-Type Permanent Magnet Synchronous Motor. Energies 2018, 11, 1208. https://doi.org/10.3390/en11051208
Li D, Wen Y, Li W, Feng B, Cao J. Three-Dimensional Temperature Field Calculation and Analysis of an Axial-Radial Flux-Type Permanent Magnet Synchronous Motor. Energies. 2018; 11(5):1208. https://doi.org/10.3390/en11051208
Chicago/Turabian StyleLi, Dong, Yinghong Wen, Weili Li, Bo Feng, and Junci Cao. 2018. "Three-Dimensional Temperature Field Calculation and Analysis of an Axial-Radial Flux-Type Permanent Magnet Synchronous Motor" Energies 11, no. 5: 1208. https://doi.org/10.3390/en11051208
APA StyleLi, D., Wen, Y., Li, W., Feng, B., & Cao, J. (2018). Three-Dimensional Temperature Field Calculation and Analysis of an Axial-Radial Flux-Type Permanent Magnet Synchronous Motor. Energies, 11(5), 1208. https://doi.org/10.3390/en11051208





