A Novel Approach for Searching the Upper/Lower Bounds of Uncertainty Parameters in Microgrids
Abstract
1. Introduction
- (1)
- An algorithm is proposed to search for the upper/lower bounds of uncertainty parameters in MGs. In addition, a method is proposed for LFT to configure the uncertainty parameters, which has general applicability to the determination of uncertainty parameters.
- (2)
- The method for configuring DG units is described in detail, and it can readily be extended to an MG with multiple sources. Based on theoretical procedures, we demonstrate how to study the uncertainty parameters in other MG structures.
- (3)
- If this method is applied correctly, it is possible to accurately identify the stability boundaries for uncertainty parameters.
2. Problem Statement
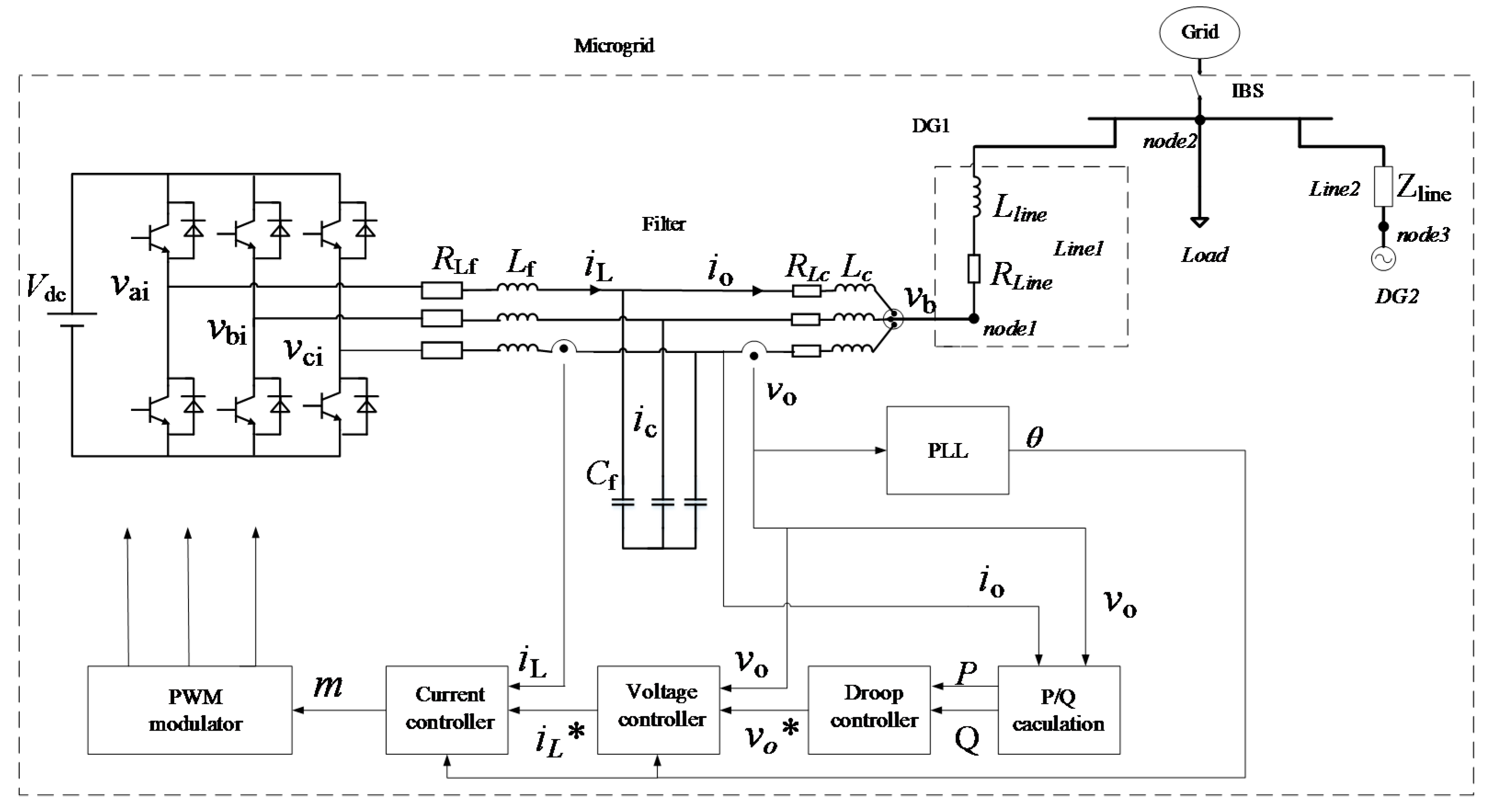
2.1. Description of an MG and Its Controller
2.2. The Impact of Droop Parameters on Microgrid Stability
3. Methodology
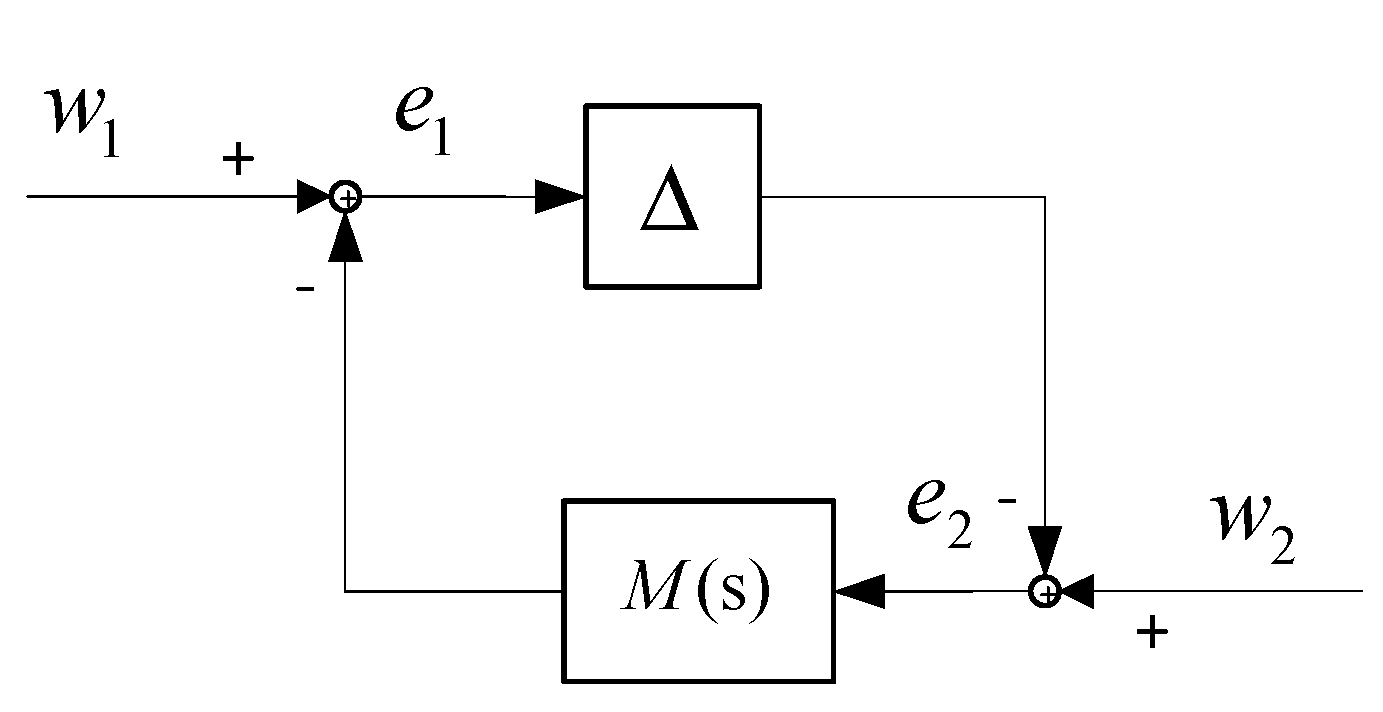
3.1. Analysis of the Stability of a System with Uncertainty Parameters
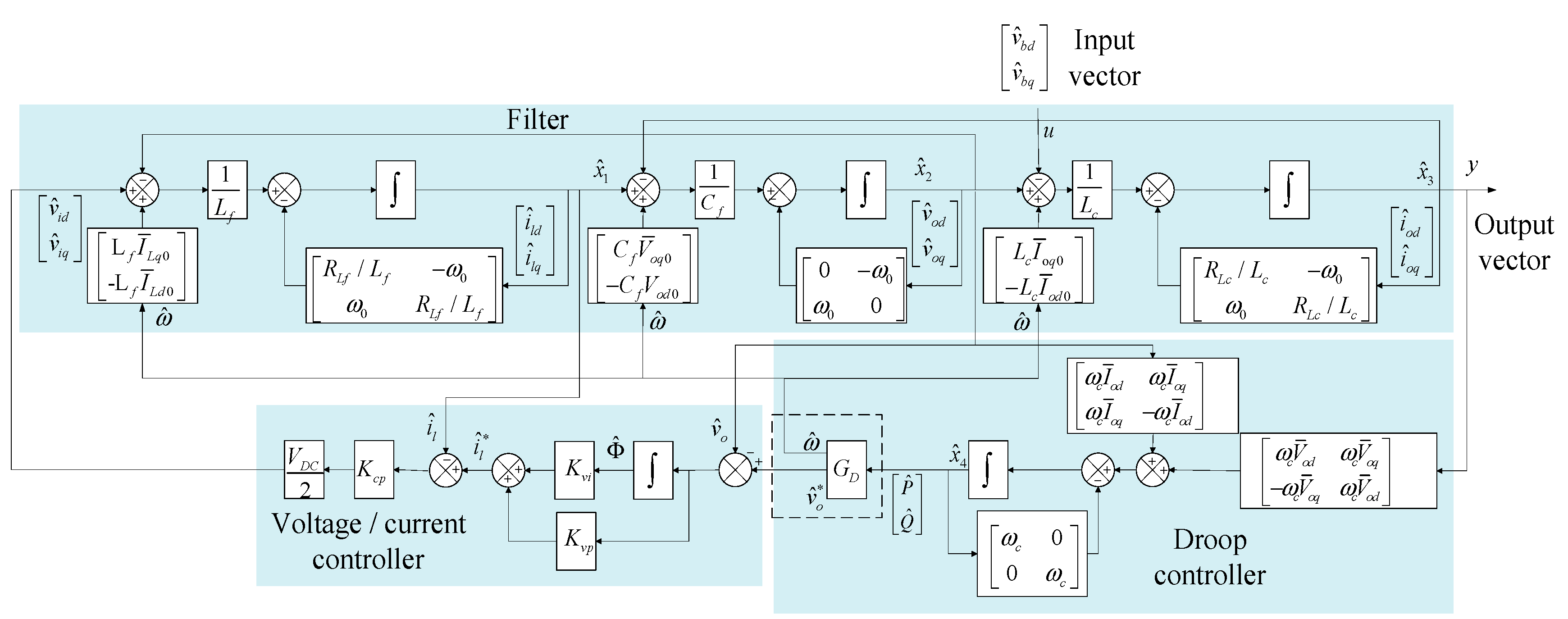
3.2. Proposed Method for Configuring an MG
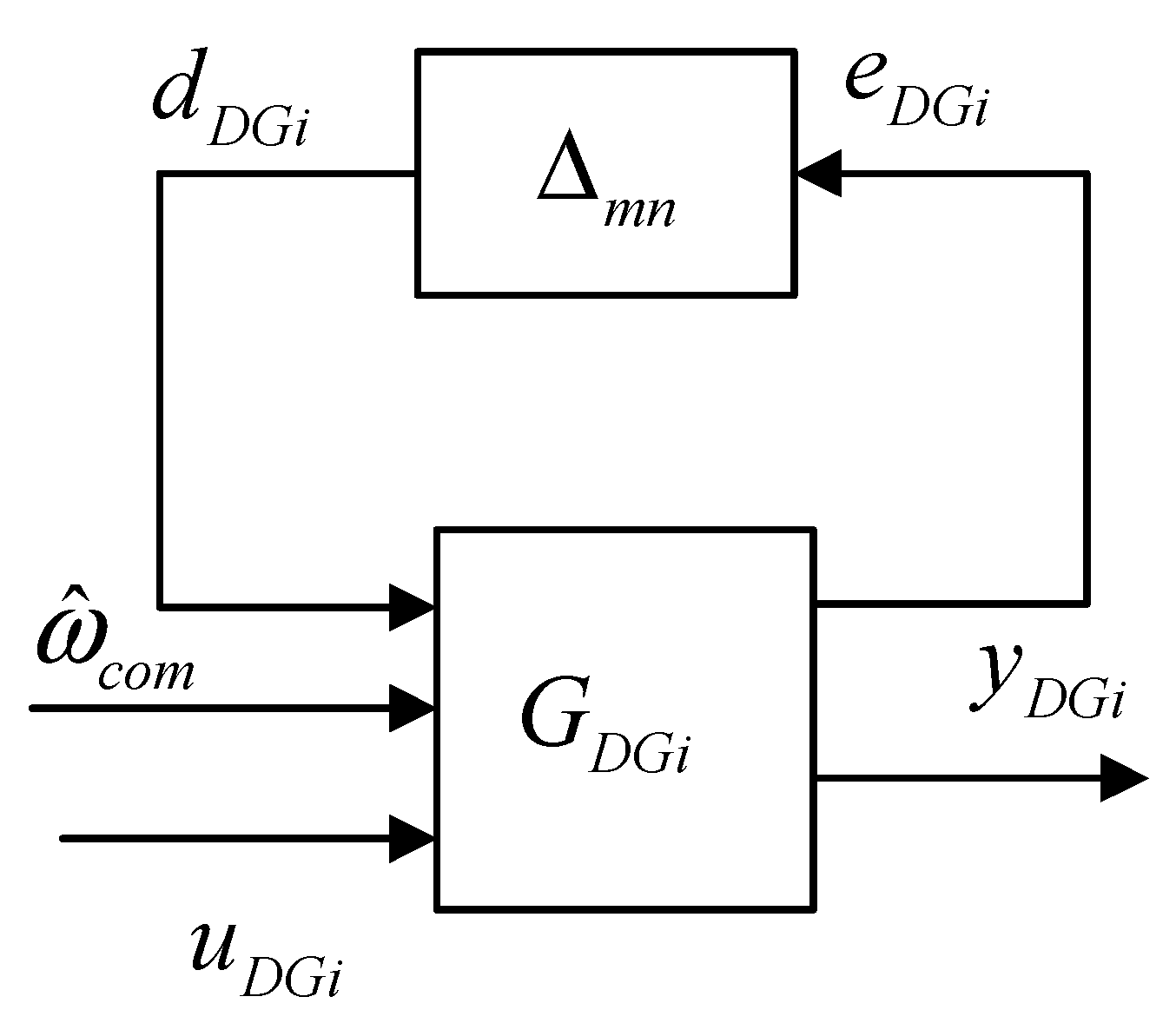
3.2.1. Proposed LFT Configuration for Uncertainty Parameters
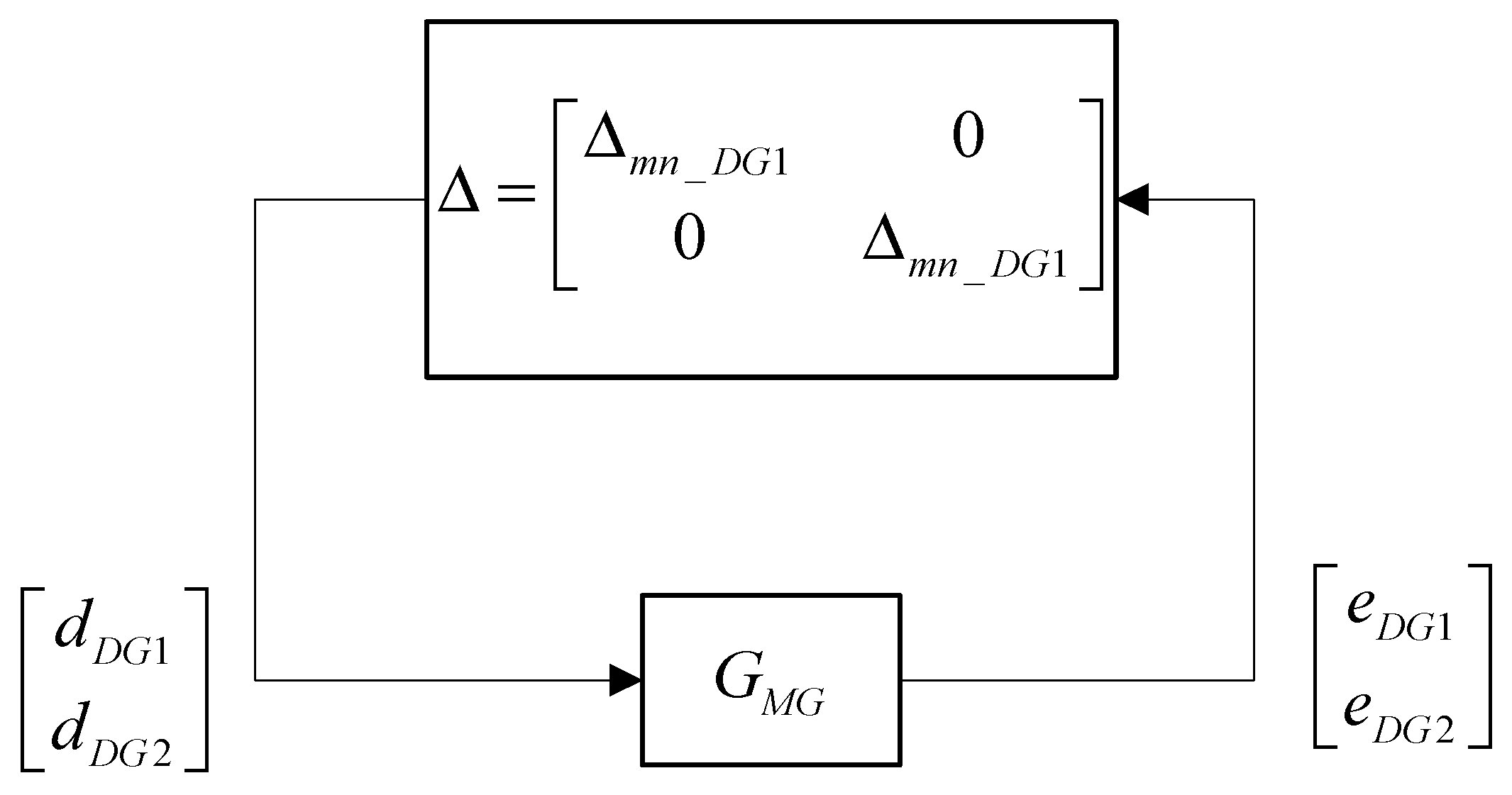
3.2.2. Framework of the MG for Analysis
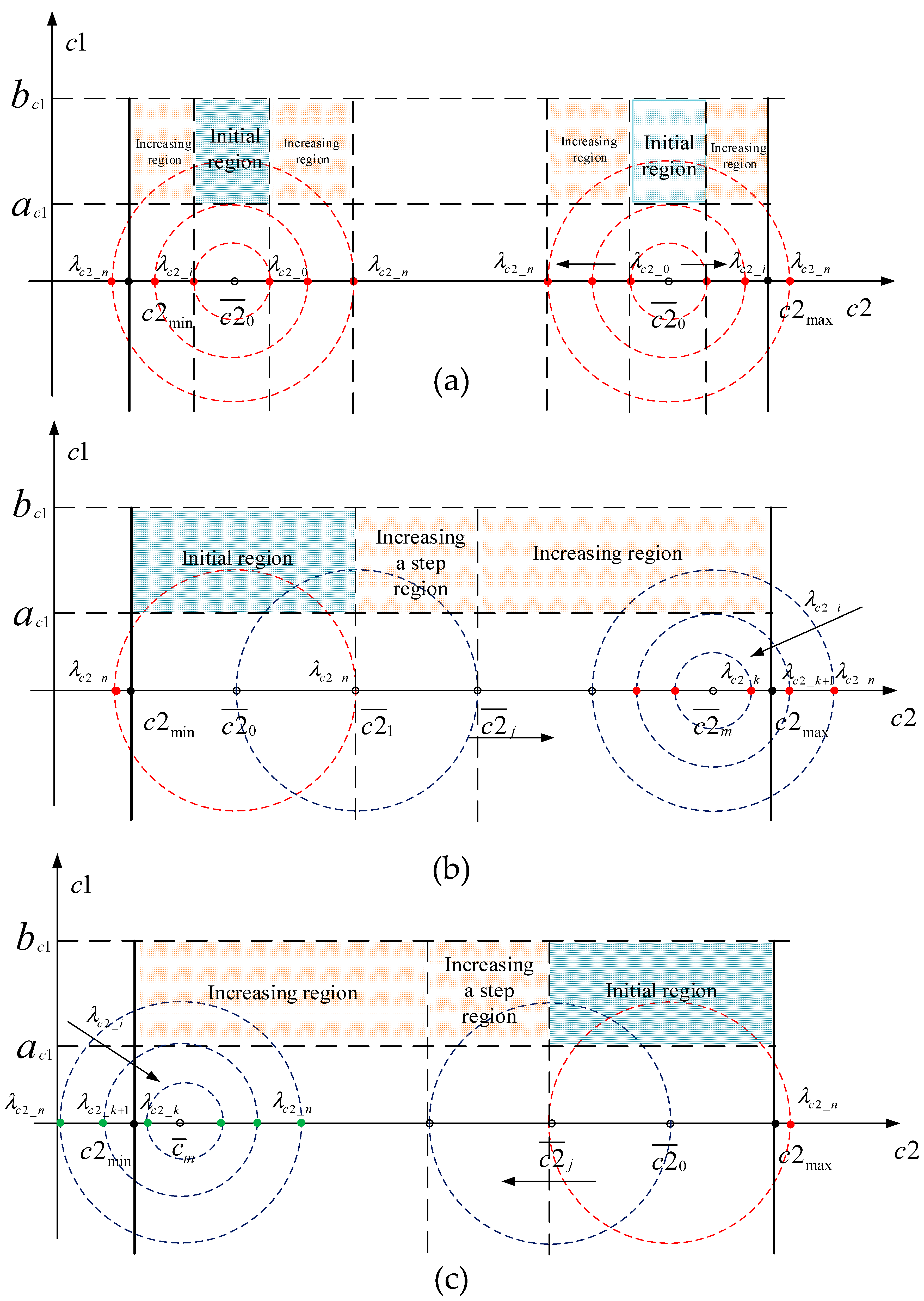
3.3. Stable Upper/Lower Bound Analysis for a Single Uncertainty Parameter
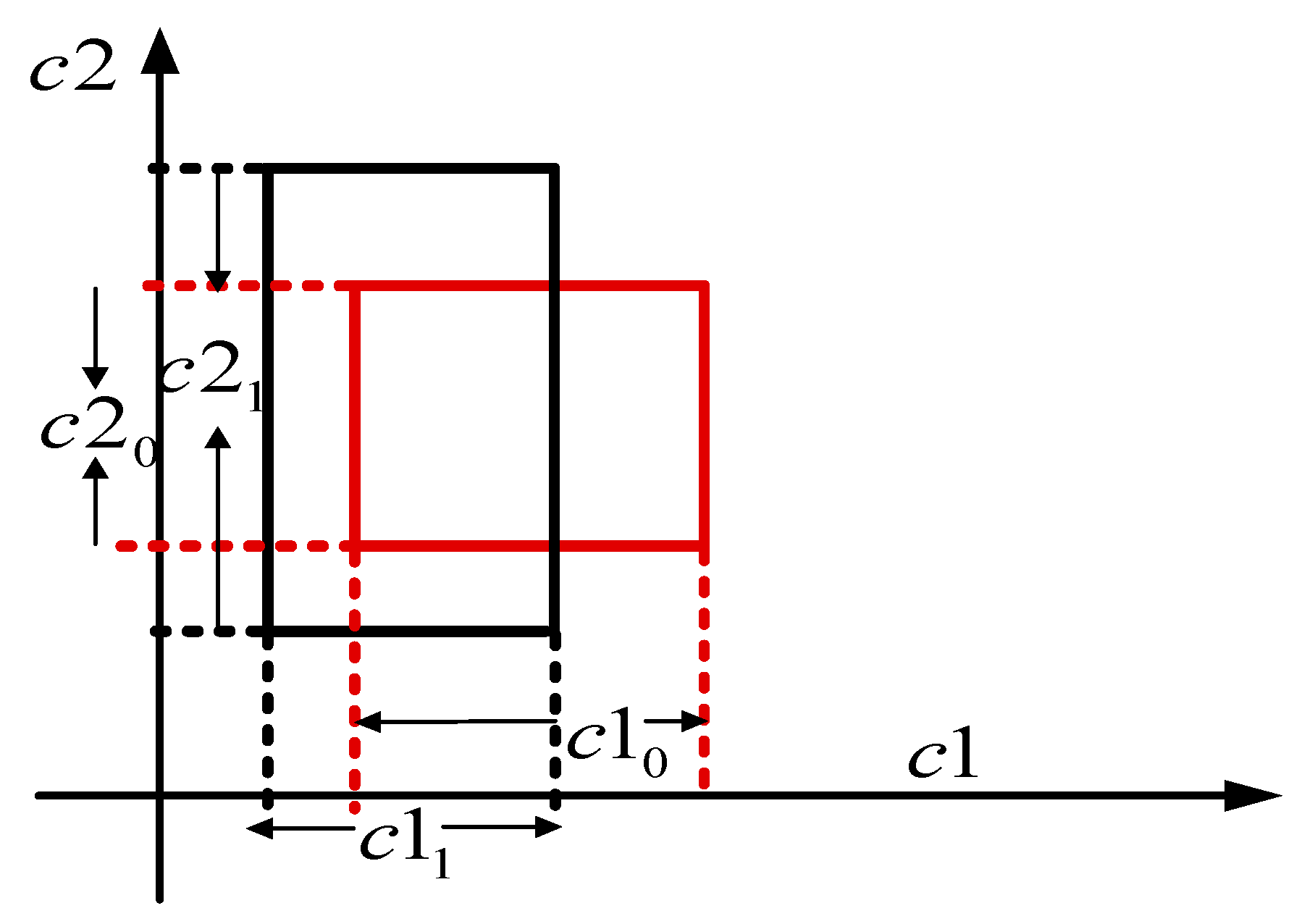
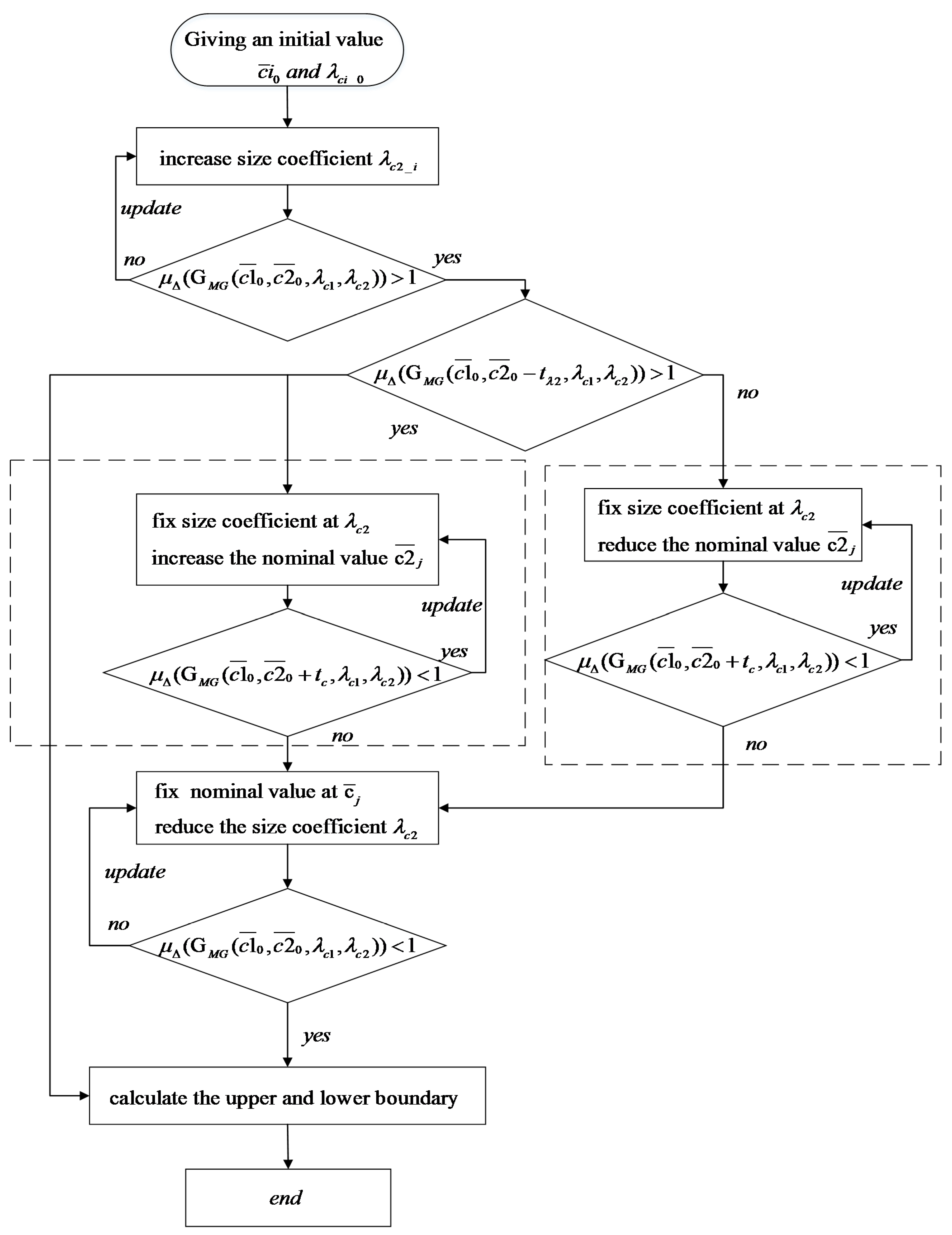
3.4. Analysis of Stability Boundaries for Two Uncertainty Parameters
4. Study Cases
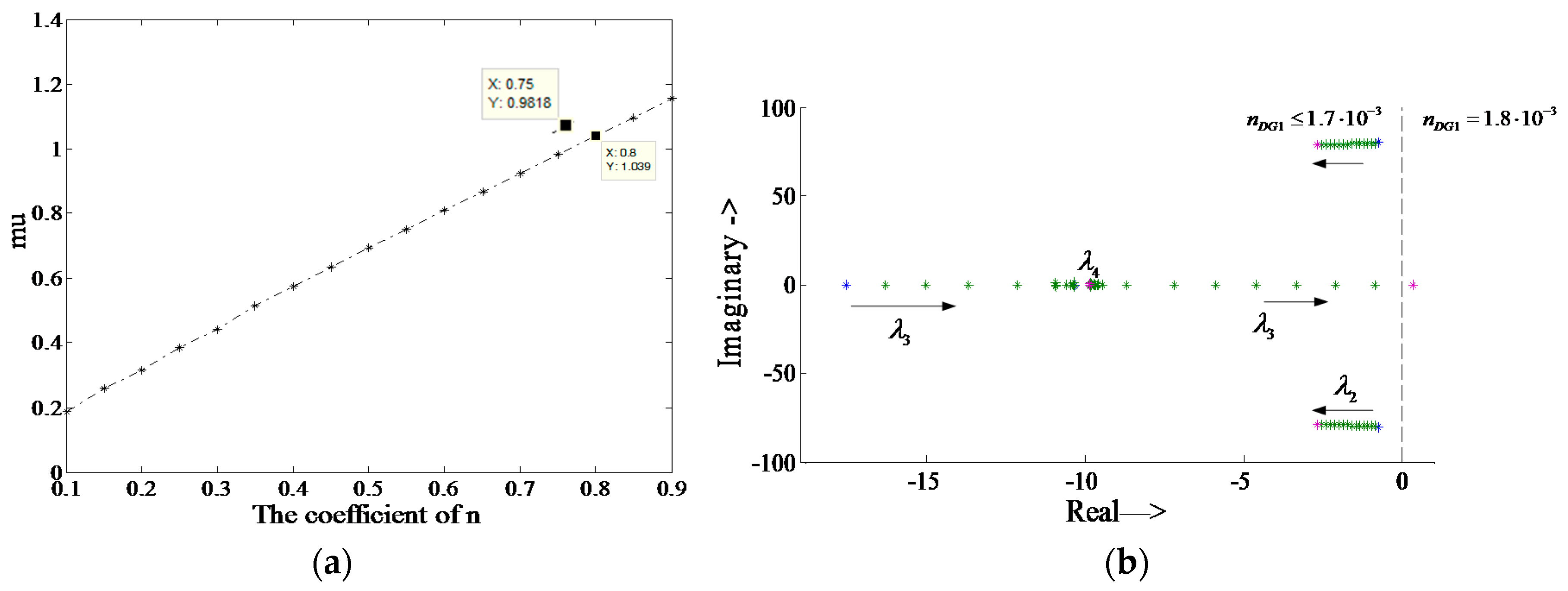
4.1. Case 1: Analysis of the Upper/Lower Stability Bounds for a Single Uncertainty Parameter
4.2. Case 2: Analysis of the Stability Range for Two Uncertainty Parameters
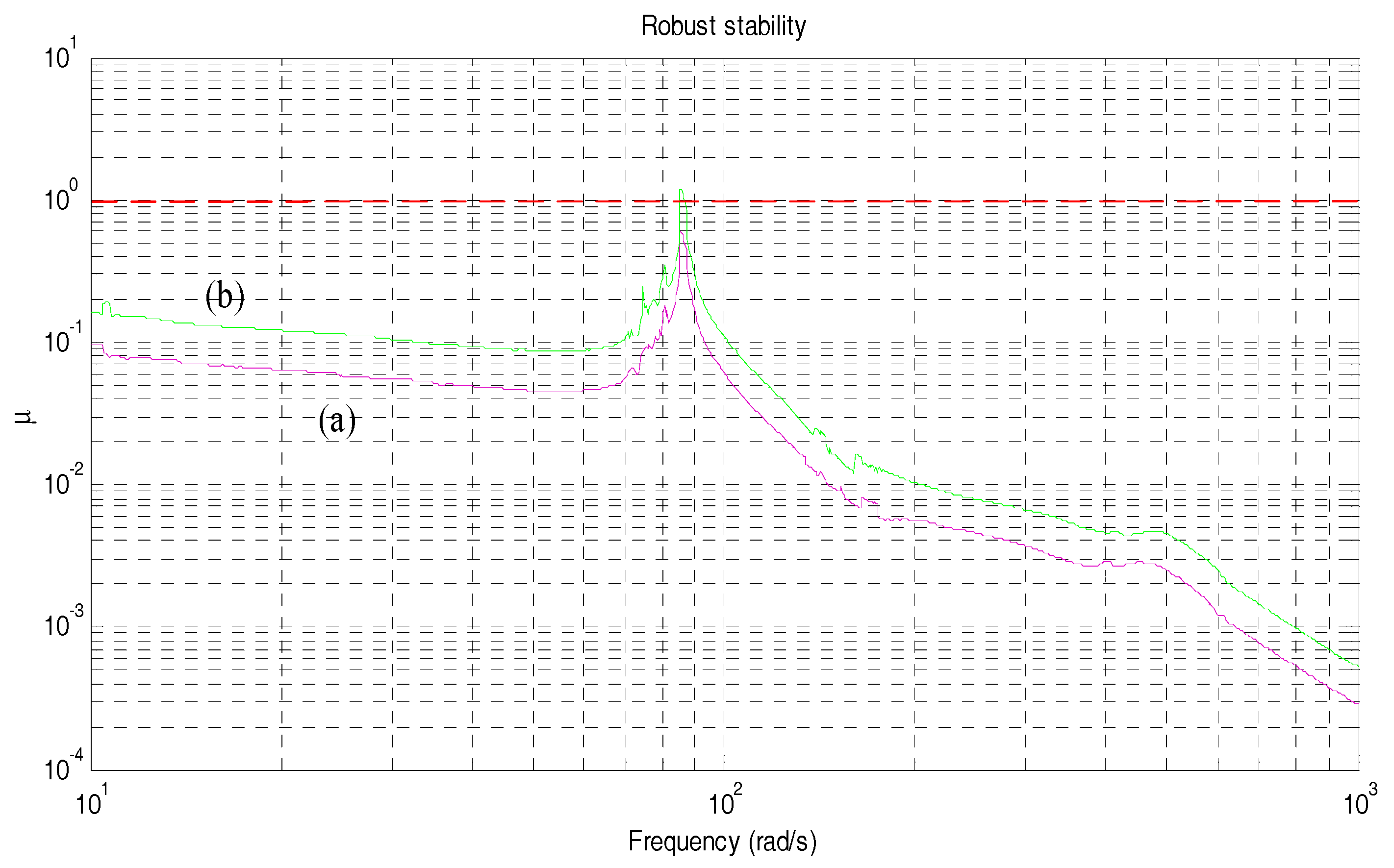
5. Simulation Study
6. Conclusions
Author Contributions
Acknowledgments
Conflicts of Interest
Appendix A
Appendix B
References
- Juan, M.C.; Leopoldo, G.F.; Jan, T.B.; Eduardo, G.; Ramón, C.P.; Prats, M.A.M.; José, I.L.; Narciso, M.A. Power electronic systems for the grid integration of renewable energy sources: A survey. IEEE Trans. Power Electron. 2006, 53, 1002–1016. [Google Scholar]
- Abdel-Rahim, N.M.; Quaicoe, J.E. Analysis and design of a multiple feedback loop control strategy for single-phase voltage-source UPS inverters. IEEE Trans. Power Electron. 1996, PE-11, 532–541. [Google Scholar] [CrossRef]
- Mohamed, Y.; El-Saadany, E. Adaptive decentralized droop controller to preserve power sharing stability of paralleled inverters in distributed generation microgrids. IEEE Trans. Power Electron. 2008, 23, 2806–2816. [Google Scholar] [CrossRef]
- Doyle, J. Analysis of feedback systems with structured uncertainties. IEE Proc. D (Control Theory Appl.) 1982, 129, 242–250. [Google Scholar] [CrossRef]
- Urquizo, J.; Calderón, C.; James, P. Using a local framework combining principal component regression and Monte Carlo simulation for uncertainty and sensitivity analysis of a domestic energy model in sub-city areas. Energies 2017, 10, 1986. [Google Scholar] [CrossRef]
- Zhou, K.; Doyle, J.; Glover, K. Robust and Optimal Control; Prentice Hall: Upper Saddle River, NJ, USA, 1996. [Google Scholar]
- Davari, M.; Mohamed, Y.A.R.I. Robust multi-objective control of VSC-based DC-voltage power port in hybrid AC/DC multi-terminal microgrids. IEEE Trans. Smart Grid 2013, 4, 1597–1612. [Google Scholar] [CrossRef]
- Coelho, E.A.A.; Cortizo, P.; Gracia, P.F.D. Small signal stability for parallel-connected inverters in stand-alone ac supply systems. IEEE Trans. Ind. Appl. 2002, 38, 533–542. [Google Scholar] [CrossRef]
- Pogaku, N.; Prodanovic, M.; Green, T.C.; Kling, W.L.; van der Sluis, L. Modeling analysis and testing of autonomous operation of an inverter-based microgrid. IEEE Trans. Power Electron. 2007, 22, 613–625. [Google Scholar] [CrossRef]
- Yang, L.; Xu, Z.; Østergaard, J.; Dong, Z.Y.; Wong, K.; Ma, X. Oscillatory stability and eigen value sensitivity analysis of a DFIG wind turbine system. IEEE Trans. Energy Convers. 2011, 26, 328–339. [Google Scholar] [CrossRef]
- Katiraei, F.; Iravani, M.R.; Lehn, P.W. Small-signal dynamic model of a microgrid including conventional and electronically interfaced distributed resources. IET Gener. Transm. Distrib. 2007, 1, 369–378. [Google Scholar] [CrossRef]
- Riccobono, A.; Santi, E. Comprehensive review of stability criteria for DC power distribution systems. IEEE Trans. Ind. Appl. 2014, 50, 3525–3535. [Google Scholar] [CrossRef]
- Sun, J. Impedance-based stability criterion for grid-connected inverters. IEEE Trans. Power Electron. 2011, 26, 3075–3078. [Google Scholar] [CrossRef]
- Feng, X.; Liu, J.; Lee, F.C. Impedance specifications for stable DC distributed power systems. IEEE Trans. Power Electron. 2002, 17, 157–168. [Google Scholar] [CrossRef]
- Riccobono, A.; Santi, E. A novel Passivity-Based Stability Criterion (PBSC) for switching converter DC distribution systems. In Proceedings of the 27th IEEE Annual IEEE Applied Power Electronics Conference and Exposition, Orlando, FL, USA, 5–9 February 2012; pp. 2560–2567. [Google Scholar]
- Castellanos, R.; Messina, A.; Sarmiento, H. Robust stability analysis of large power systems using the structured singular value theory. Int. J. Electr. Power Energy Syst. 2005, 27, 389–397. [Google Scholar] [CrossRef]
- Sumsurooah, S.; Odavic, M.; Bozhko, S. A modeling methodology for robust stability analysis of nonlinear electrical power systems under parameter uncertainties. IEEE Trans. Ind. Appl. 2016, 52, 4416–4425. [Google Scholar] [CrossRef]
- Salis, V.; Costabeber, A.; Cox, S.M.; Zanchetta, P.; Formentini, A. Stability boundary analysis in single-phase grid-connected inverters with PLL by TLP theory. IEEE Trans. Power Electron. 2017, 33, 4023–4036. [Google Scholar] [CrossRef]
- Zheng, J.H.; Wang, Y.; Wang, Z.; Zhu, S.; Wang, X.; Xinwei, S. Study on microgrid operation modes switching based on eigenvalue analysis. APAP 2011, 1, 445–450. [Google Scholar]
- Haddadi, A. A μ-based approach to small-signal stability analysis of an interconnected distributed energy resource unit and load. IEEE Trans. Power Deliv. 2015, 30, 1715–1726. [Google Scholar] [CrossRef]
- Guerrero, J.M.; Vicuna, L.G.; Matas, J.; Castilla, M.; Miret, J. A wireless controller to enhance dynamic performance of parallel inverters in distributed generation systems. IEEE Trans. Power. Electron. 2004, 19, 1205–1213. [Google Scholar] [CrossRef]
- Alvarado, F.L. Bifurcations in nonlinear systems: Computational issues. In Proceedings of the International Symposium on Circuits and Systems (ISCAS), New Orleans, LA, USA, 29 April–3 May 1990. [Google Scholar]
- Hill, D. Nonlinear computation and control for small disturbance stability. In Proceedings of the IEEE Summer Meeting, Seattle, WA, USA, 16–20 July 2000. [Google Scholar]
- De Souza, A.C.Z. Tangent vector applied to voltage collapse and loss sensitivity studies. Electr. Power Syst. Res. 1998, 47, 65–70. [Google Scholar] [CrossRef]
- Tiranuchit, A.; Thomas, R. A posturing strategy against voltage instabilities in electric power systems. IEEE Trans. Power Syst. 1988, 3, 87–93. [Google Scholar] [CrossRef]
- Yorino, N.; Li, H.-Q.; Sasaki, H. A predictor/corrector scheme for obtaining q-limit points for power flow studies. IEEE Trans. Power Syst. 2005, 20, 130–137. [Google Scholar] [CrossRef]
- Rommes, J.; Martins, N. Computing large-scale system eigenvalues most sensitive to parameter changes, with applications to power system small-signal stability. IEEE Trans. Power Syst. 2008, 23, 434–442. [Google Scholar] [CrossRef]
- Luo, C.; Ajjarapu, V. Sensitivity-based efficient identification of oscillatory stability margin and damping margin using continuation of invariant subspaces. IEEE Trans. Power Syst. 2011, 26, 1484–1492. [Google Scholar] [CrossRef]
- Yang, D.; Ajjarapu, V. Critical eigenvalues tracing for power system analysis via continuation of invariant subspaces and projected Arnoldi method. IEEE Trans. Power Syst. 2007, 22, 324–332. [Google Scholar] [CrossRef]
- Wang, Y.; Wang, J.; Zeng, W.; Liu, H.; Chai, Y. H Robust control of an LCL-type Grid-connected inverter with large-scale grid impedance perturbation. Energies 2018, 11, 57. [Google Scholar] [CrossRef]
- Li, Y.W.; Vilathgamuwa, D.M.; Loh, P.C. Design analysis and real-time testing of a controller for multibus microgrid system. IEEE Trans. Power Electron. 2004, 19, 1195–1204. [Google Scholar] [CrossRef]
- Doyle, J.C.; Wall, J.C.; Stein, G. Performance and robustness analysis for structured uncertainty. In Proceedings of the 21st IEEE Conference on Decision and Control, Orlando, FL, USA, 8–10 December 1982. [Google Scholar]
- Shah, S.; Sensarma, P.S. Three degree of freedom robust voltage controller for instantaneous current sharing among voltage source inverters in parallel. IEEE Trans. Power Electron. 2010, 25, 3003–3014. [Google Scholar] [CrossRef]

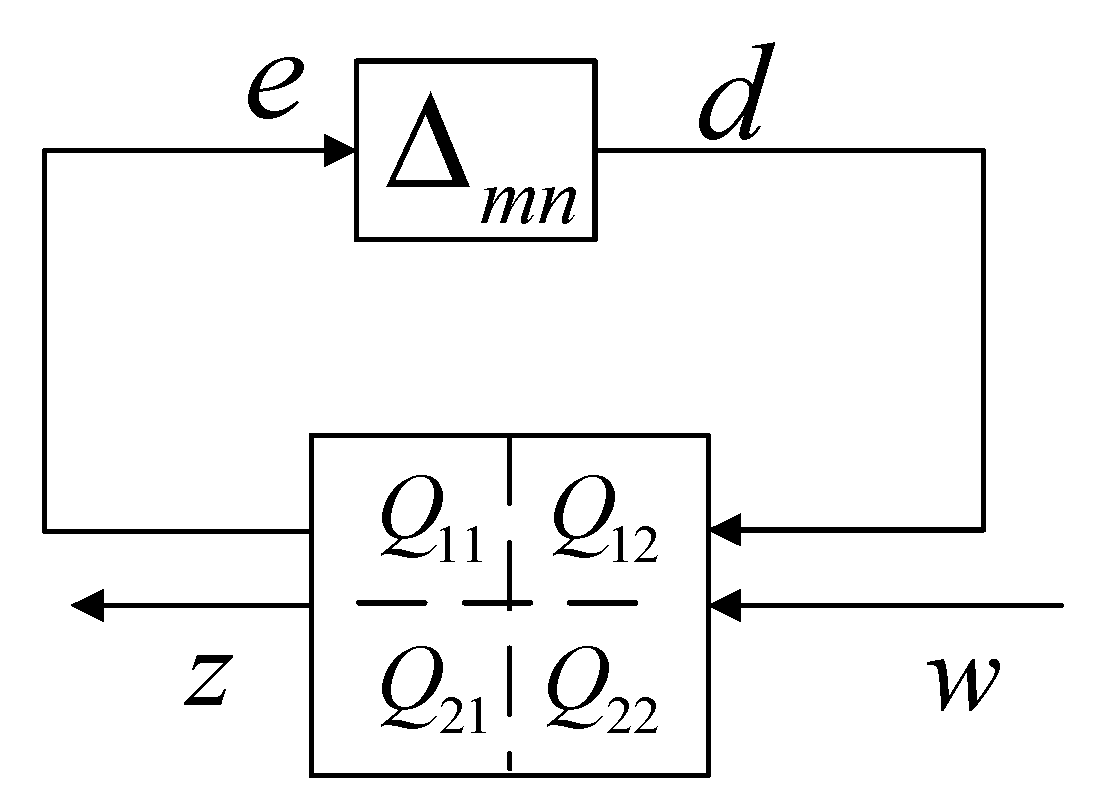



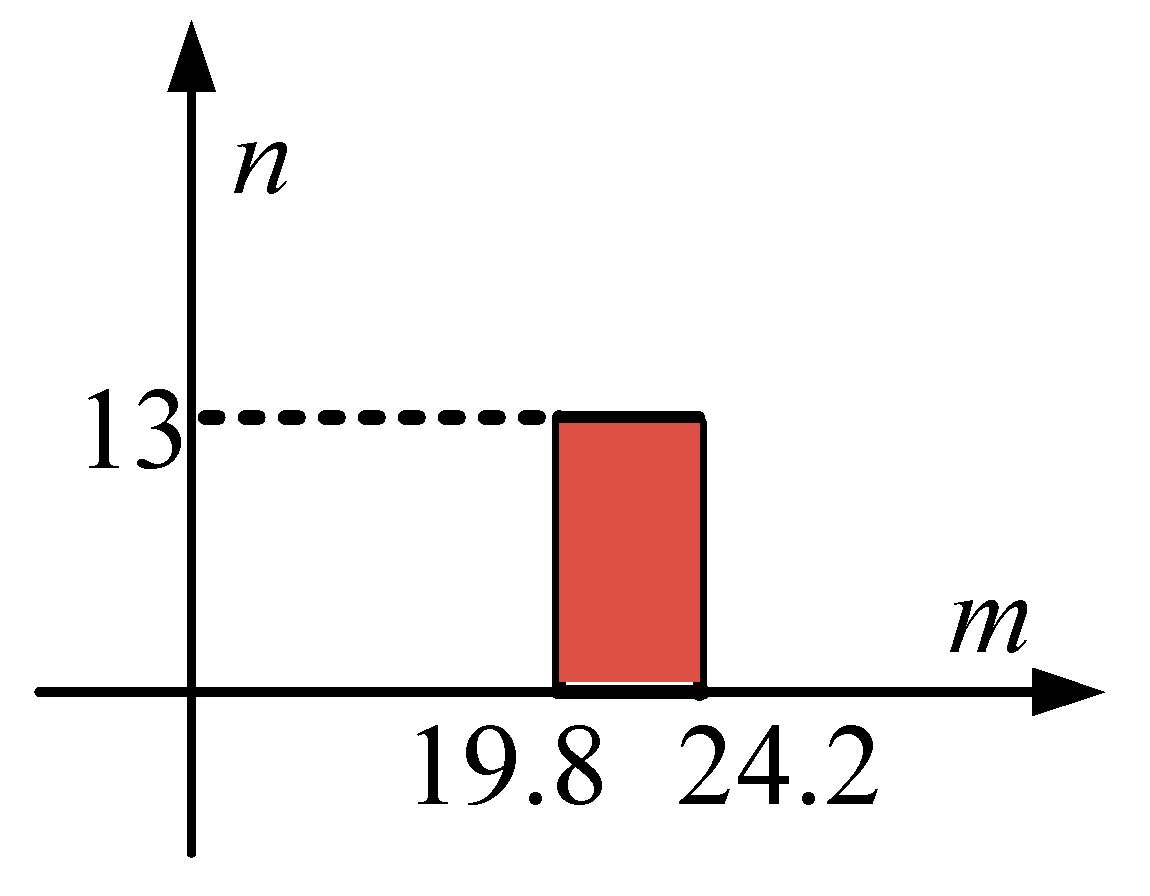
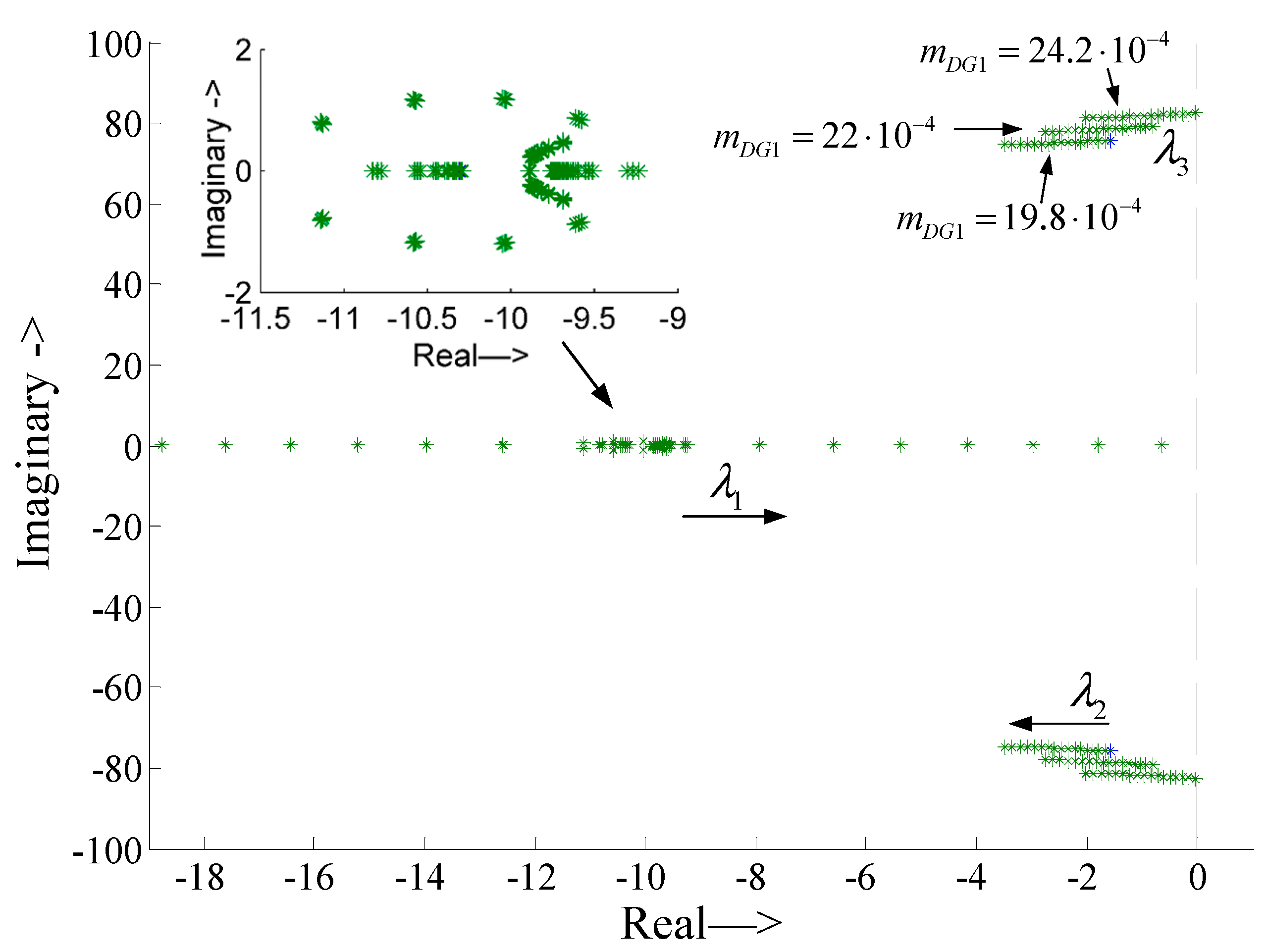

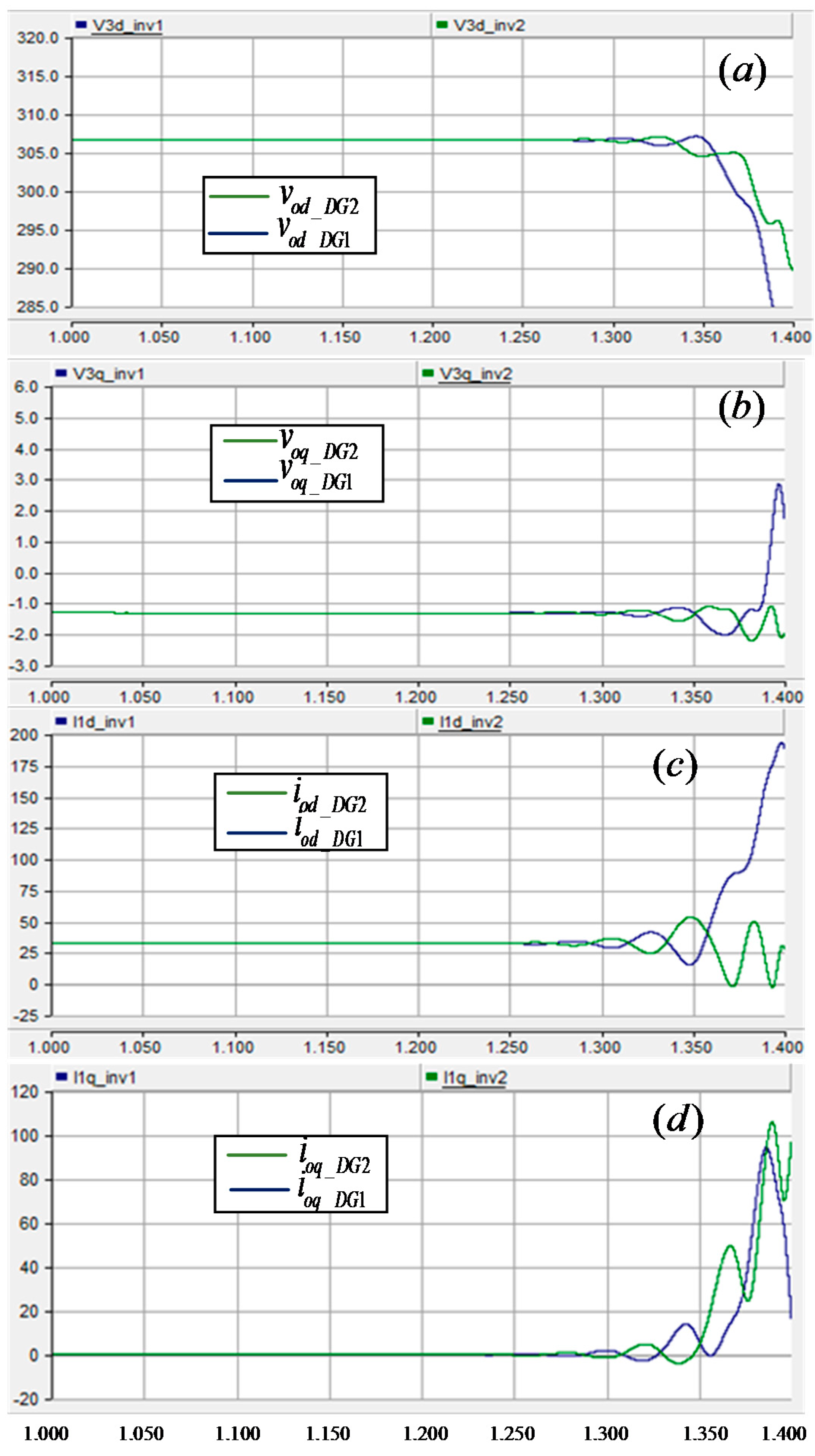
| Parameter | Symbol | Value |
|---|---|---|
| Inverter switching frequency | 5 kHz | |
| Filter inductance | 1.5 mH | |
| Parasitic resistor | 0.001 | |
| Filter capacitance | 102 μF | |
| Filter inductance | 0.53 mH | |
| Parasitic resistor | 0.05 | |
| Rating of output voltage | 311 V | |
| Nominal frequency | 50 Hz | |
| droop | ||
| droop | ||
| Parameters for lines 1 and 2 | 0.1 | |
| 1.12 mH | ||
| Load parameters | 4.279 | |
| 2.94 mH |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ding, X.; Li, K.; Li, Y.; Cai, D.; Luo, Y.; Dong, Y. A Novel Approach for Searching the Upper/Lower Bounds of Uncertainty Parameters in Microgrids. Energies 2018, 11, 1035. https://doi.org/10.3390/en11051035
Ding X, Li K, Li Y, Cai D, Luo Y, Dong Y. A Novel Approach for Searching the Upper/Lower Bounds of Uncertainty Parameters in Microgrids. Energies. 2018; 11(5):1035. https://doi.org/10.3390/en11051035
Chicago/Turabian StyleDing, Xiaojun, Kaicheng Li, Yuanzheng Li, Delong Cai, Yi Luo, and Youli Dong. 2018. "A Novel Approach for Searching the Upper/Lower Bounds of Uncertainty Parameters in Microgrids" Energies 11, no. 5: 1035. https://doi.org/10.3390/en11051035
APA StyleDing, X., Li, K., Li, Y., Cai, D., Luo, Y., & Dong, Y. (2018). A Novel Approach for Searching the Upper/Lower Bounds of Uncertainty Parameters in Microgrids. Energies, 11(5), 1035. https://doi.org/10.3390/en11051035





