An Analysis for the Influences of Fracture Network System on Multi-Stage Fractured Horizontal Well Productivity in Shale Gas Reservoirs
Abstract
:1. Introduction
2. Mathematical Model
2.1. Model Assumption
- (1)
- The model is derived for single-phase isothermal gas flow in shale.
- (2)
- Both natural fractures and hydraulic fractures are main flow paths, and filled with the free gas. Additionally, shale matrix is filled with part of the free gas and almost all of the adsorption gas.
- (3)
- The inter-porosity flux between shale matrix and fracture system is described by the pseudo-steady mechanism.
- (4)
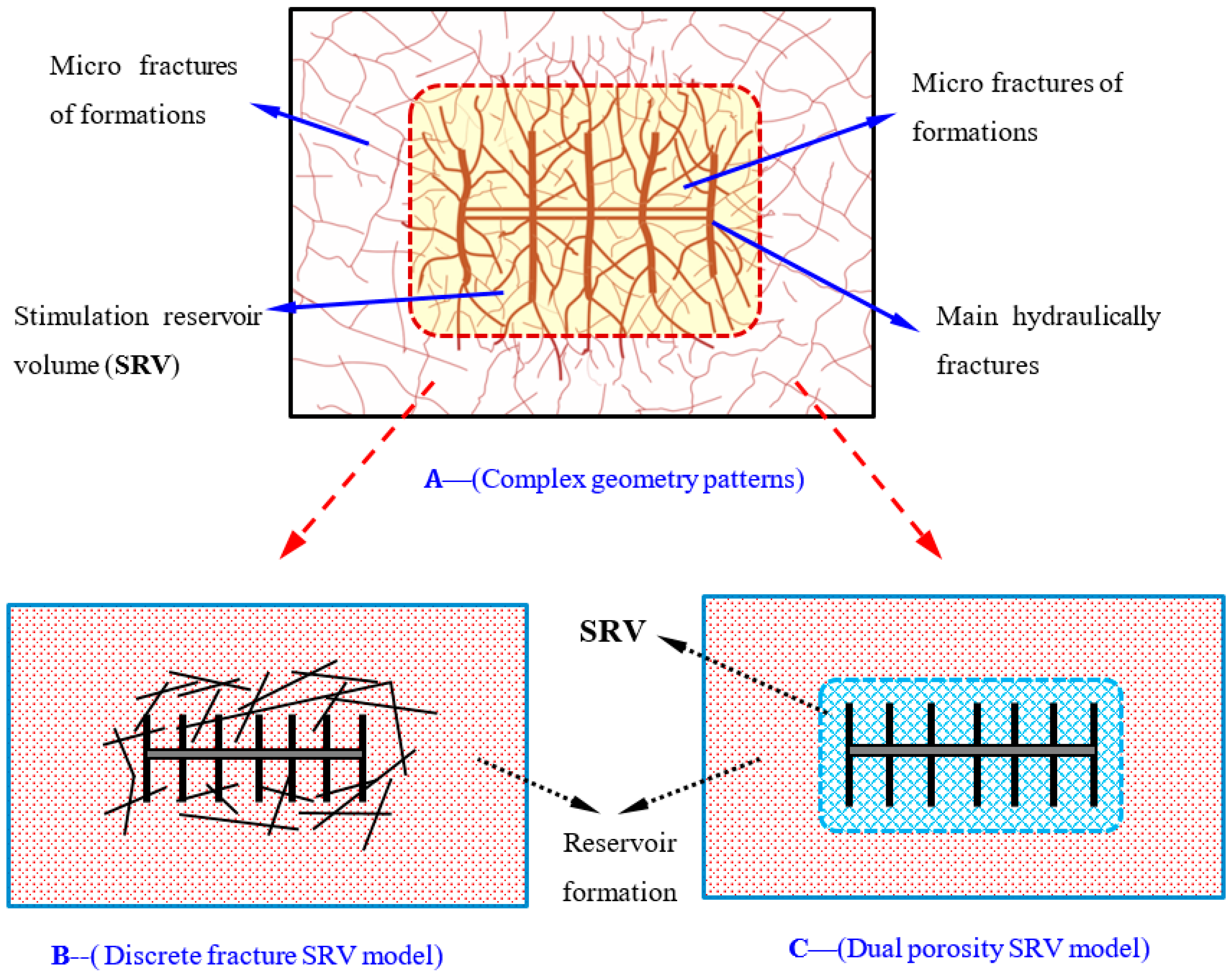
- With the consideration of the stress sensitivity, a dual-porosity composite model and a DFN model are established to reflect the effects of fracture networks.
- (5)
- The main hydraulic fractures are assumed to be finite-conductivity.
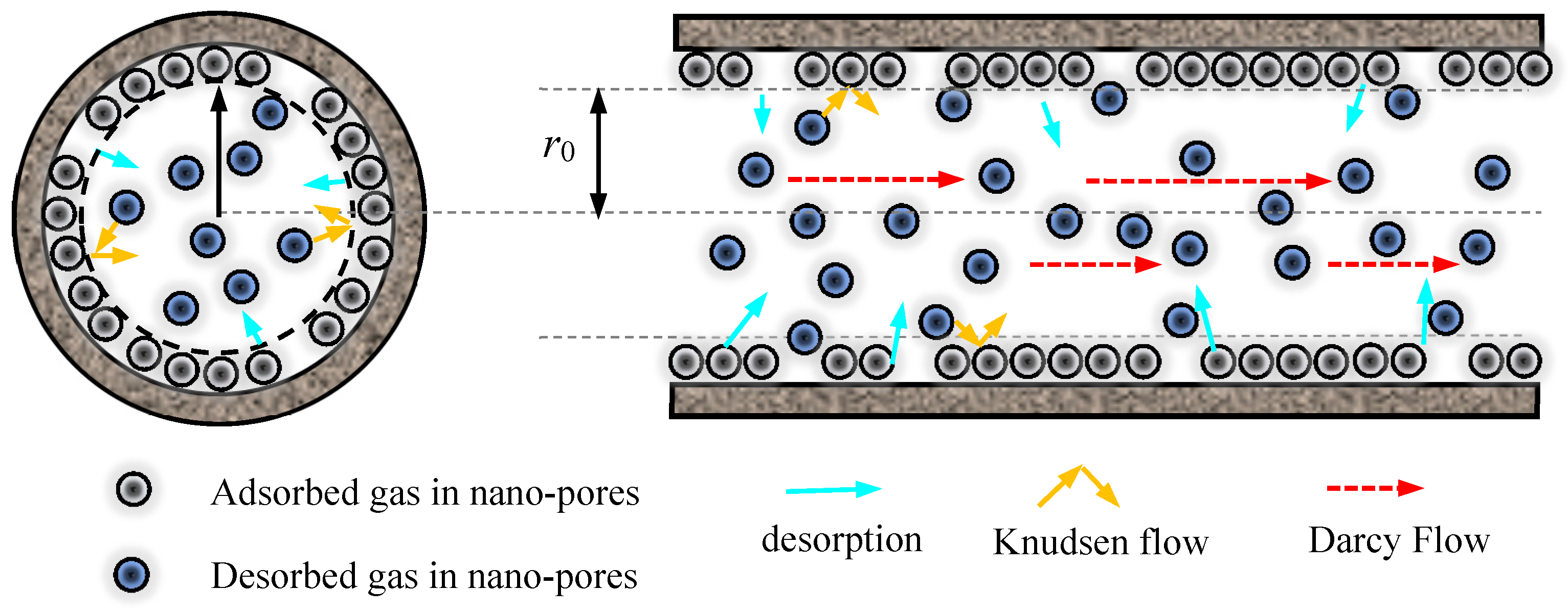
2.2. Adsorption/Desorption
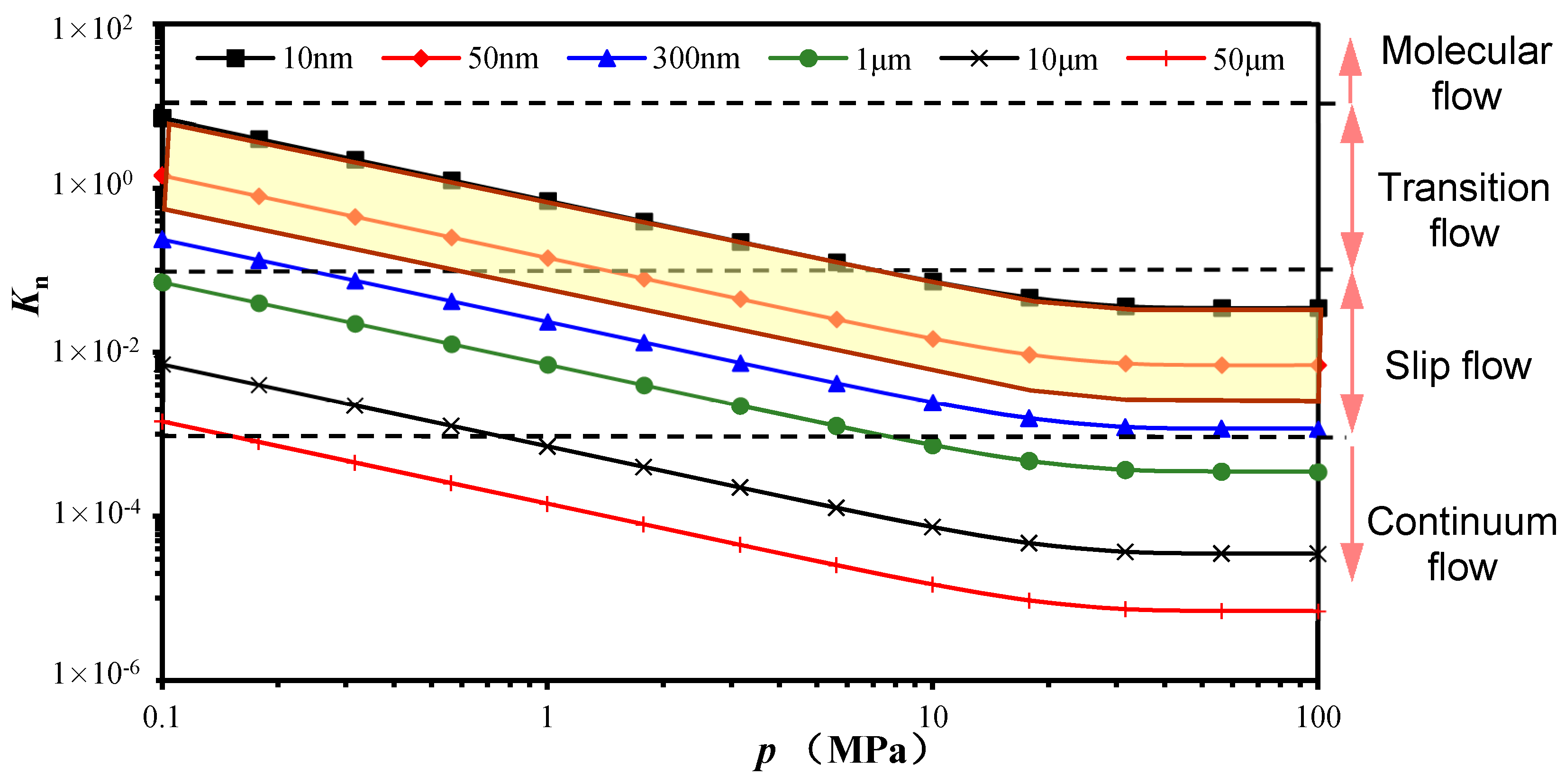
2.3. Flow Regimes and Mechanisms in Micro-Pores
2.3.1. Slip Flow
2.3.2. Knudsen Flow
2.3.3. Total Gas Flux
2.4. Flow-Governing Equations
2.5. Numerical Solution
3. Results and Discussion
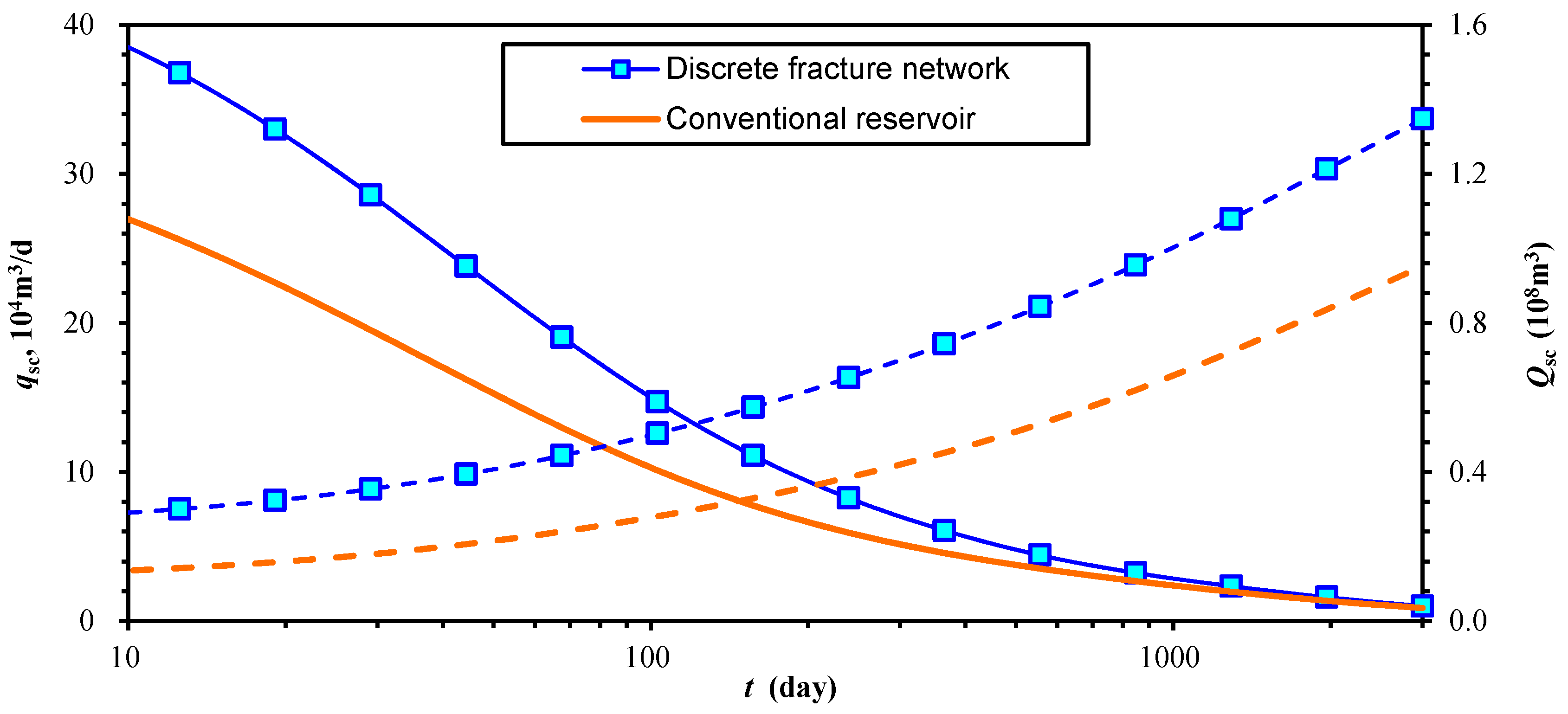
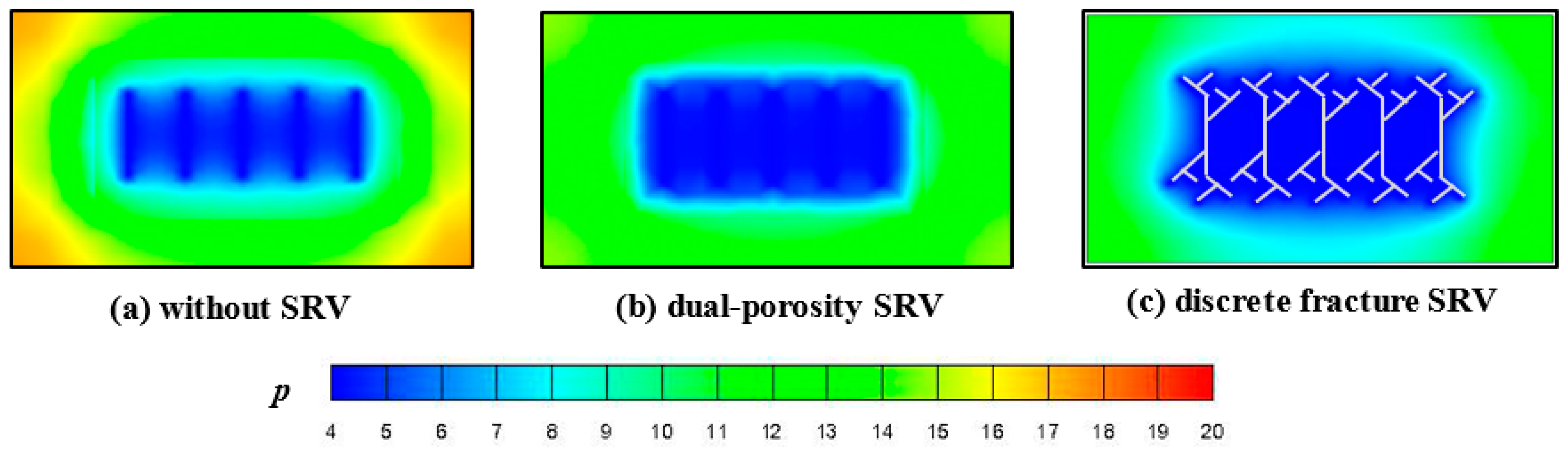
3.1. Effects of Fracture Network on Production
- (1)
- They improve the free gas flow capability in the early period.
- (2)
- They increase the flow interface between the fractured and un-fractured zone.
- (3)
- They stimulate the desorption of the adsorbed gas;
3.2. Sensitivity Analyses
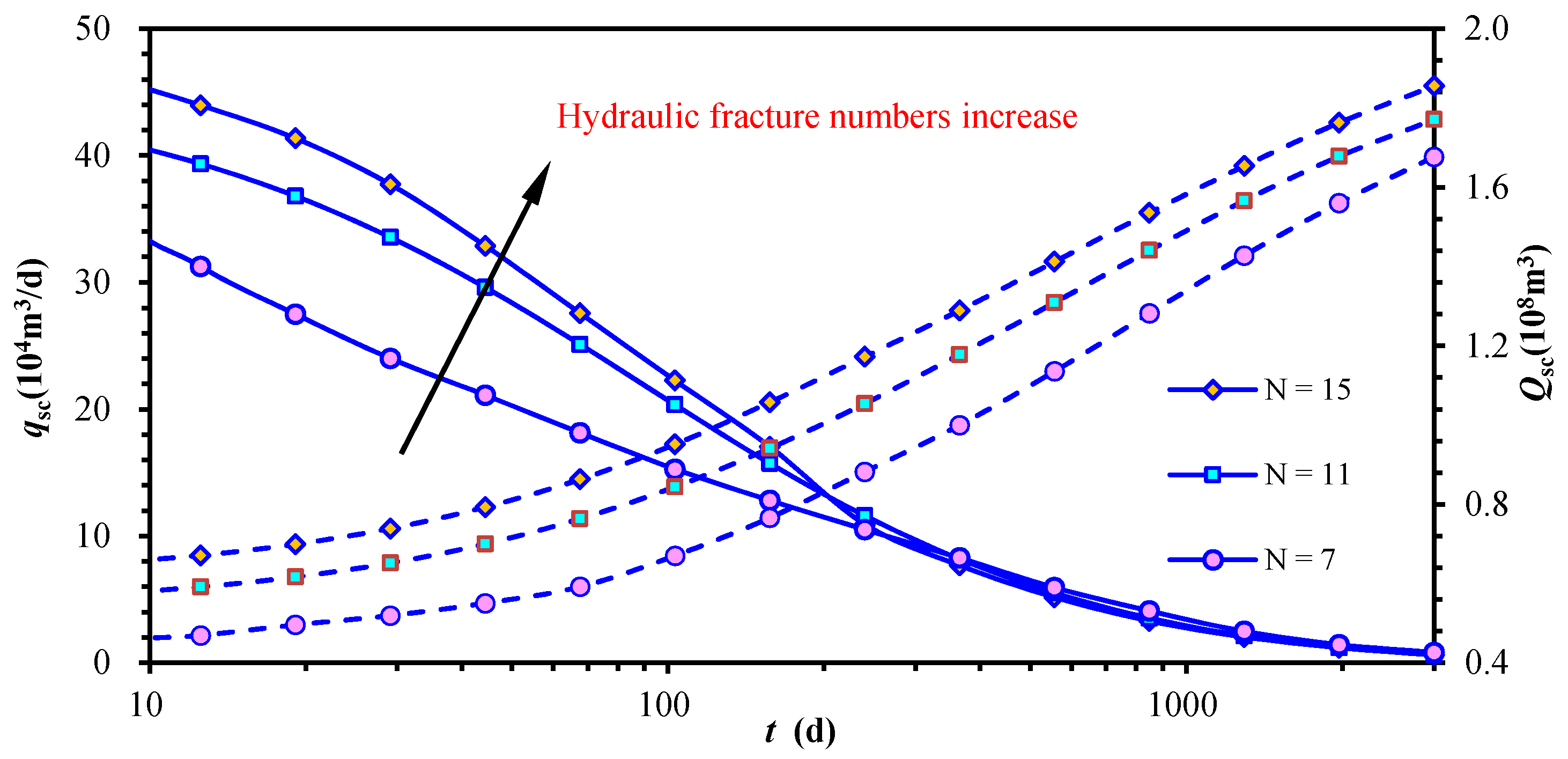
3.2.1. Hydraulic Fracture Numbers (N)
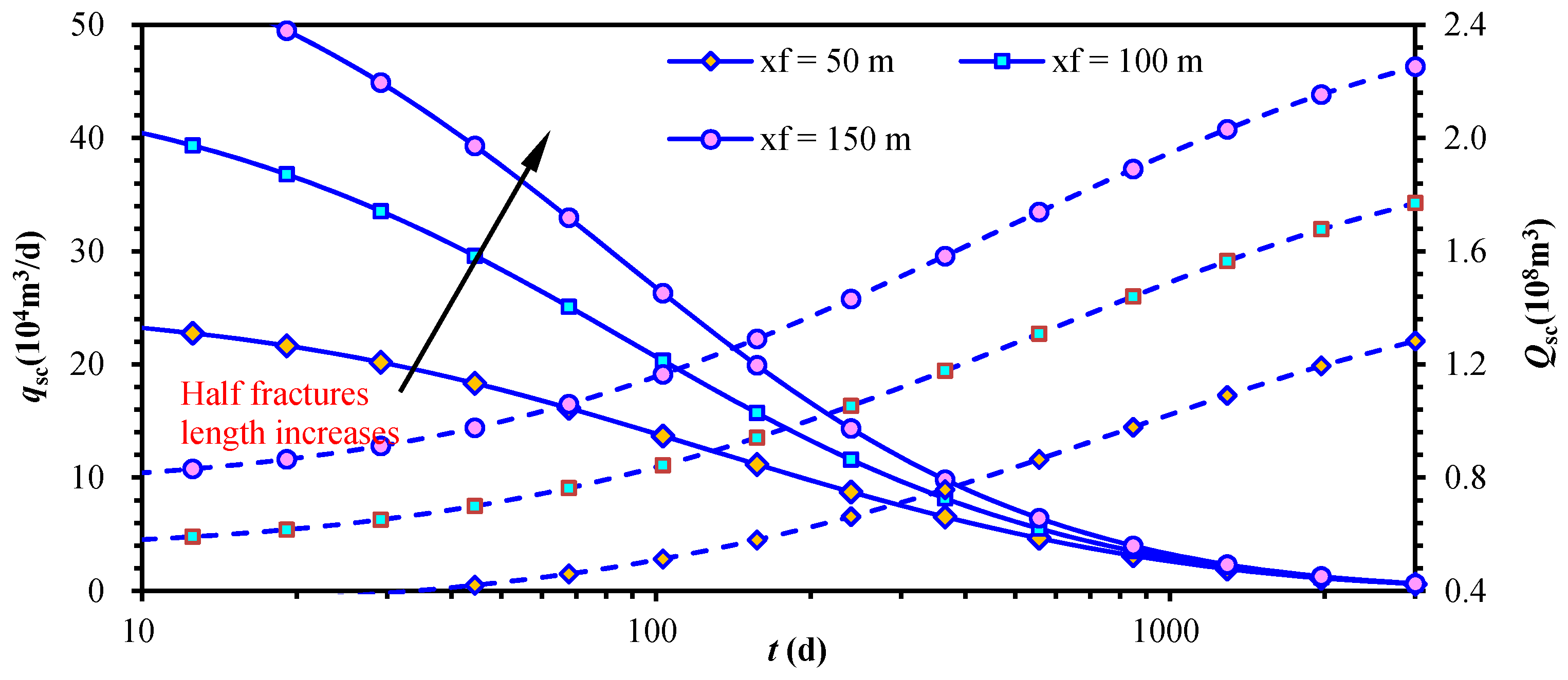
3.2.2. Hydraulic Fracture Length (xF)
3.2.3. Hydraulic Fracture Height (hF)
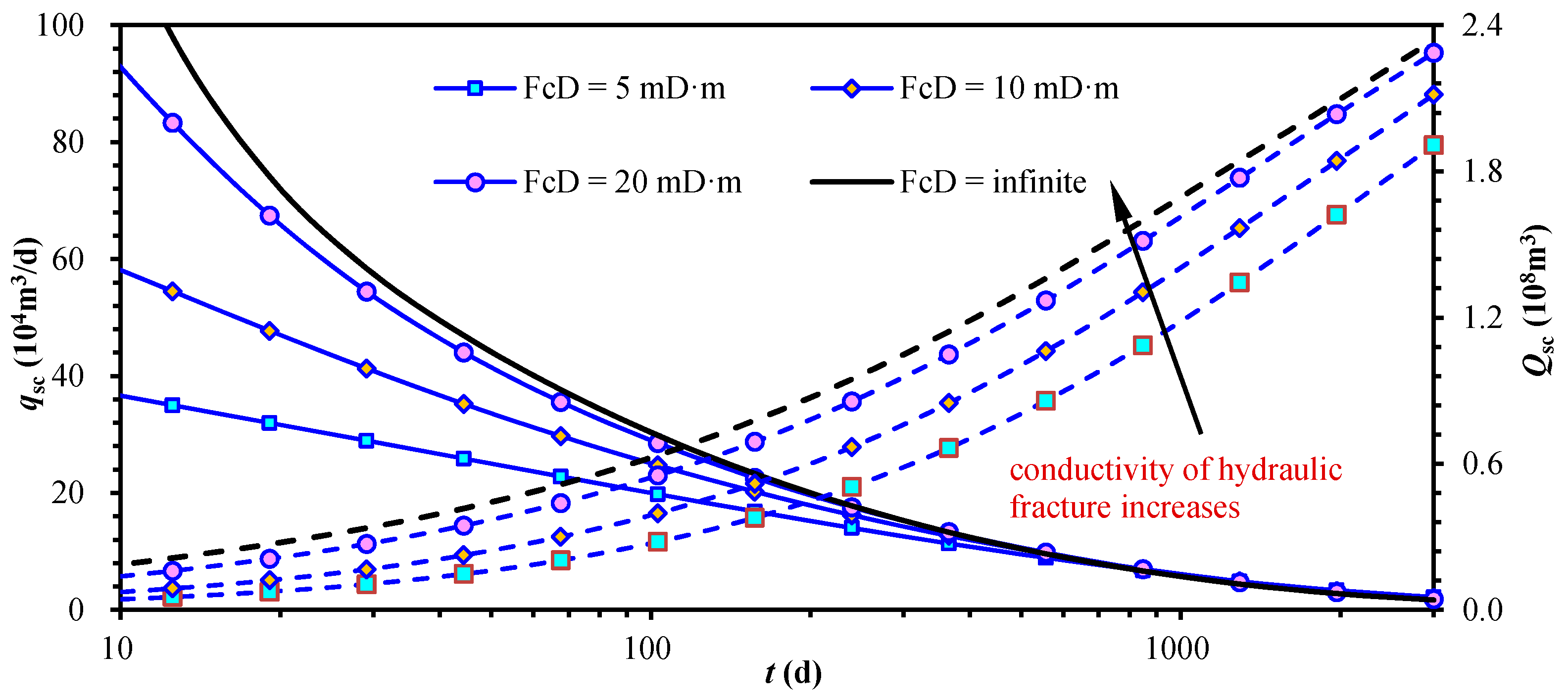
3.2.4. Hydraulic Fracture Conductivity (FcD)
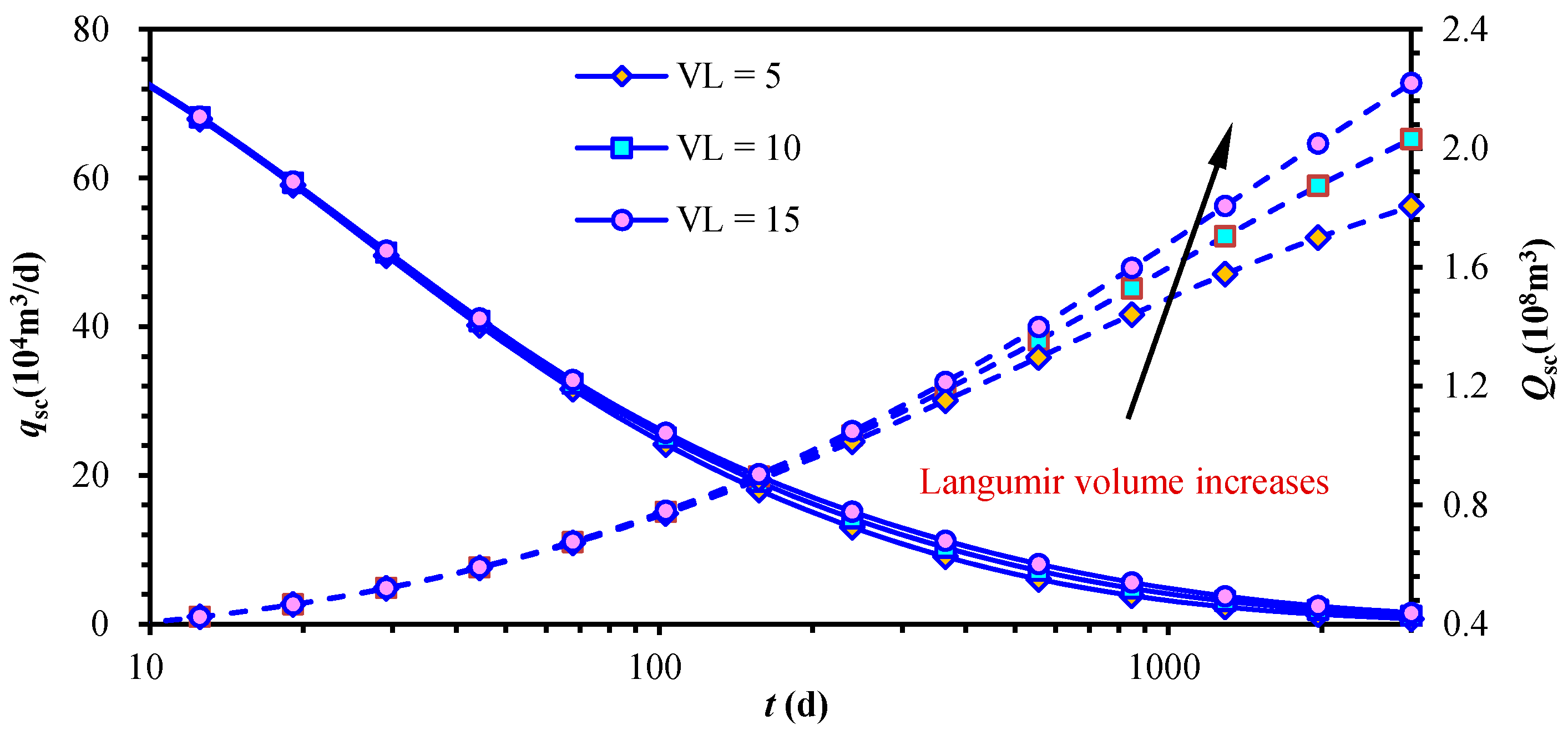
3.2.5. Langmuir Volume (Vm)
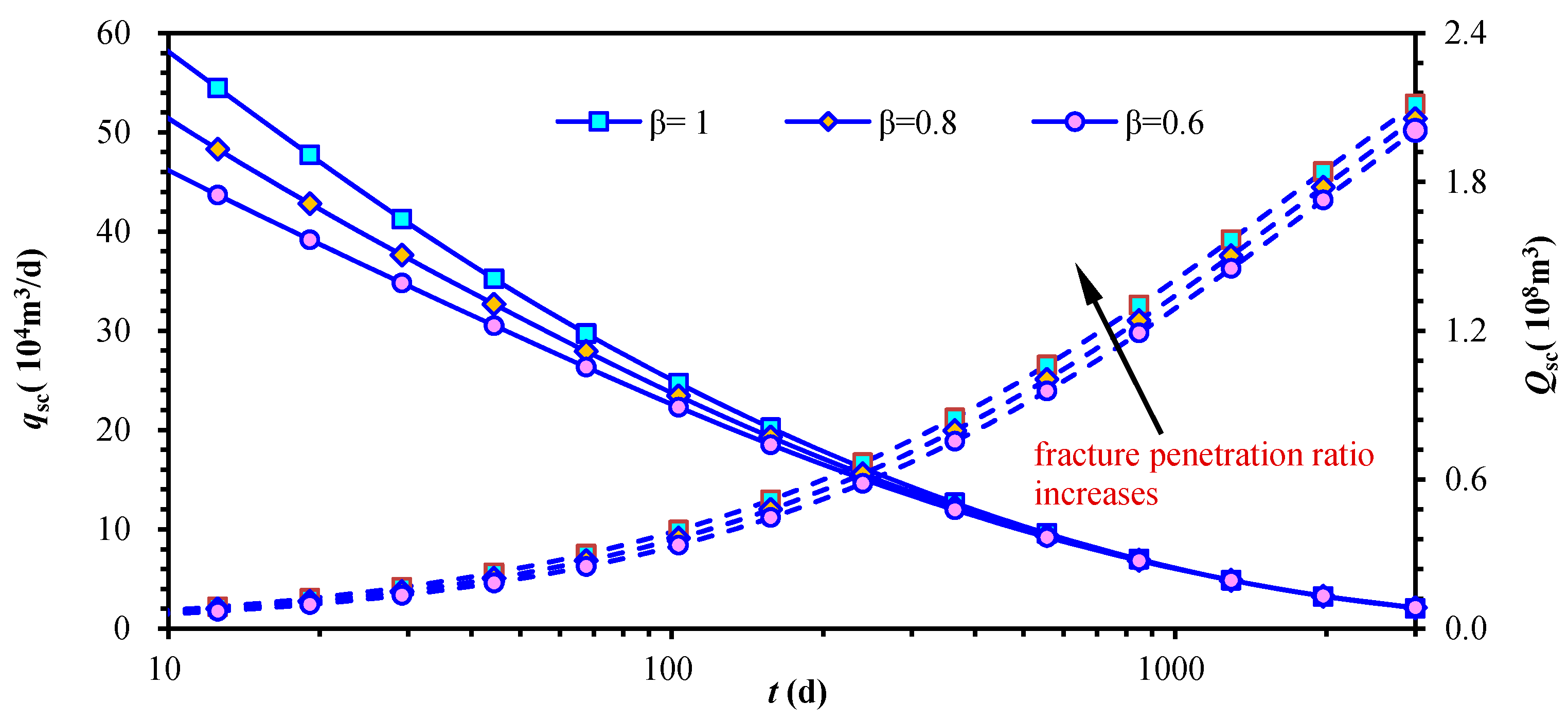
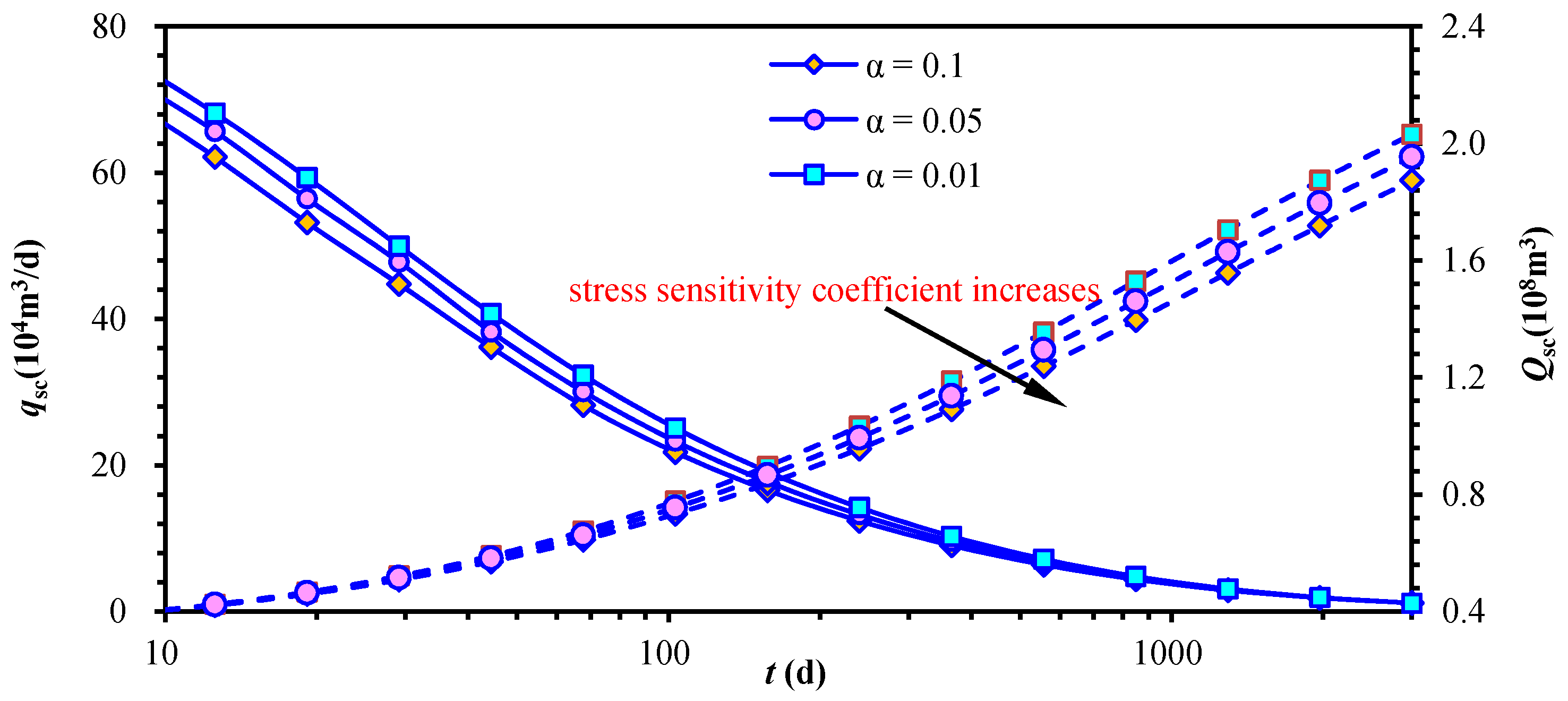
3.2.6. Stress Sensitivity Coefficient (α)
4. Conclusions
- (1)
- The contribution of fracture networks on productivities can be described as:
- (a)
- They improve the free gas flow capability in the early period.
- (b)
- They increase the flow interface between the fractured and un-fractured zone.
- (c)
- They stimulate the desorption of the adsorbed gas.
- (2)
- Once the fracture distribution can be obtained quantitatively, the DFN model may present a more accurate description of the gas flow dynamic and the inter-porosity flux in the early flow period. Nevertheless, limited by uncertainty of the fracture network, the dual-porosity model is still a useful and recommended method for numerical simulation in shale.
- (3)
- Sensitivity analyses reveal that the increases of hydraulic fracture number, length and Langmuir volume represent a higher flow rate, and the marginal effect of fractures number should be noticed in fracturing engineering.
Acknowledgments
Author Contributions
Conflicts of Interest
Nomenclature
| Bg | the ratio of gas volume at reservoir condition and gas volume at surface condition, rm3/sm3 |
| h | formation thickness, m |
| Kn | Knudsen number, dimensionless |
| kB | Boltzmann constant, kB = 1.3806 × 10−23 J/K |
| k | permeability, mD |
| L | characteristic length of shale pore, nm |
| Mg | gas molecular weight, g/mol |
| pL | Langmuir pressure, MPa |
| R | gas constant, 8.314 J/(mol·K) |
| T | temperature, K |
| VL | Langmuir volume, sm3/m3 |
| VE | adsorbed gas volume per unit rock volume at a constant pressure, sm3/m3 |
| Z | the ratio of real gas volume and ideal gas volume, m3/m3 |
| λ | gas molecular mean free path, nm |
| δ | molecule collision distance, nm |
| μ | gas viscosity, mPa·s |
| ϕ | porosity, fraction |
| Subscript | |
| m | matrix |
| f | fracture |
| F | hydraulic fracture |
References
- Wang, K.; Liu, H.; Luo, J.; Wu, K.; Chen, Z. A comprehensive model coupling embedded discrete fractures, multiple interacting continua and geomechanics in shale gas reservoirs with multi-scale fractures. Energy Fuel 2017, 31, 7758–7776. [Google Scholar] [CrossRef]
- Wu, K.; Li, X.; Wang, C.; Yu, W.; Chen, Z. Model for surface diffusion of adsorbed gas in nanopores of shale reservoirs. Ind. Eng. Chem. Res. 2015, 54, 3225–3236. [Google Scholar] [CrossRef]
- Chen, Z.; Huan, G.; Ma, Y. Computational Methods for Multiphase Flows in Porous Media; Society for Industrial and Applied Mathematics: Philadelphia, PA, USA, 2006. [Google Scholar]
- Bustin, R.M.; Bustin, A.M.; Cui, A.; Ross, D.; Pathi, V.M. Impact of shale properties on pore structure and storage characteristics. In Proceedings of the SPE Shale Gas Production Conference, Fort Worth, TX, USA, 16–18 November 2008. [Google Scholar]
- Jenkins, C.D.; Boyer, C.M. Coalbed- and shale-gas reservoirs. J. Pet. Technol. 2008, 60, 92–97. [Google Scholar] [CrossRef]
- Curtis, B.J. Fractured shale-gas systems. AAPG Bull. 2002, 86, 1921–1938. [Google Scholar]
- Wu, Y.S.; Li, J.; Ding, D.; Wang, C.; Di, Y. A generalized framework model for the simulation of gas production in unconventional gas reservoirs. SPE J. 2014, 19, 845–857. [Google Scholar] [CrossRef]
- Hill, D.G.; Nelson, C.R. Gas Productive Fractured Shales: An Overview and Update. Gas TIPS 2000, 6, 4–13. [Google Scholar]
- Mengal, S.A.; Wattenbarger, R.A. Accounting for adsorbed gas in shale gas reservoirs. In Proceedings of the SPE Middle East Oil and Gas Show and Conference, Manama, Bahrain, 25–28 September 2011. [Google Scholar]
- Zhang, Z.Y.; Yang, S.B. On the adsorption and desorption trend of shale gas. J. Exp. Mech. 2012, 27, 492–497. (In Chinese) [Google Scholar]
- Alnoaimi, K.R.; Kovscek, A.R. Experimental and numerical analysis of gas transport in shale including the role of sorption. In Proceedings of the SPE Annual Technical Conference and Exhibition, New Orleans, LA, USA, 30 September–2 October 2013. [Google Scholar]
- Yu, W.; Sepehrnoori, K.; Patzek, T.W. Evaluation of gas adsorption in Marcellus Shale. In Proceedings of the SPE Annual Technical Conference and Exhibition, Amsterdam, The Netherlands, 27–29 October 2014. [Google Scholar]
- Lu, X.C.; Li, F.C.; Watson, A.T. Adsorption studies of natural gas storage in Devonian Shales. SPE Form. Eval. 1995, 10, 109–113. [Google Scholar] [CrossRef]
- Shabro, V.; Torres-Verdin, C.; Sepehrnoori, K. Forecasting gas production in organic shale with the combined numerical simulation of gas diffusion in Kerogen, Langmuir desorption from surfaces, and advection in nanopores. In Proceedings of the 2012 SPE Annual Technical Conference and Exhibition, San Antonio, TX, USA, 8–10 October 2012. [Google Scholar]
- Dong, Z.; Holditch, S.A.; Mcvay, D.A. Resource evaluation for shale gas reservoirs. In Proceedings of the SPE Hydraulic Fracturing Technology Conference, The Woodlands, TX, USA, 6–8 February 2012. [Google Scholar]
- Haghshenas, B.; Clarkson, C.R.; Chen, S. Multi-porosity multi-permeability models for shale gas reservoirs. In Proceedings of the SPE Unconventional Resources Conference Canada, Calgary, AB, Canada, 5–7 November 2013. [Google Scholar]
- Ertekin, T.; King, G.R.; Schwerer, F.C. Dynamic gas slippage: A unique dual-mechanism approach to the flow of gas in tight formations. SPE Form. Eval. 1986, 1, 43–52. [Google Scholar] [CrossRef]
- Roy, S.; Raju, R. Modeling gas flow through micro channels and nanopores. J. Appl. Phys. 2003, 93, 4870–4878. [Google Scholar] [CrossRef]
- Ozkan, E.; Raghavan, R.; Apaydin, O.G. Modeling of fluid transfer from shale matrix to fracture network. In Proceedings of the SPE Annual Technical Conference and Exhibition, Florence, Italy, 20–22 September 2010. [Google Scholar]
- Javadpour, F.; Fisher, D.; Unsworth, M. Nanoscale gas flow in shale gas sediments. J. Gas Can. Pet. Technol. 2007, 46, 55–61. [Google Scholar] [CrossRef]
- Javadpour, F. Nanopores and apparent permeability of gas flow in mudrocks (shales and siltstone). J. Gas Can. Pet. Technol. 2009, 48, 16–21. [Google Scholar] [CrossRef]
- Javadpour, F.; Moghanloo, G.R. Contribution of Methane Molecular Diffusion in Kerogen to Gas-in-Place and Production. In Proceedings of the SPE Western Regional & AAPG Pacific Section Meeting 2013 Joint Technical Conference, Monterey, CA, USA, 19–25 April 2013. [Google Scholar]
- Civan, F. Effective correlation of apparent gas permeability in tight porous media. Trans. Porous Media 2010, 82, 375–384. [Google Scholar] [CrossRef]
- Civan, F.; Rai, C.S.; Sondergeld, C.H. Shale-gas permeability and diffusivity inferred by improved formulation of relevant retention and transport mechanisms. Trans. Porous Media 2011, 86, 925–944. [Google Scholar] [CrossRef]
- Civan, F.; Devegowda, D.; Sigal, R. Critical evaluation and improvement of methods for determination of matrix permeability of shale. In Proceedings of the SPE Annual Technical Conference and Exhibition, New Orleans, LA, USA, 30 September–2 October 2013. [Google Scholar]
- Swami, V.; Settari, A. A pore scale gas flow model for shale gas reservoir. In Proceedings of the Americas Unconventional Resources Conference, Pittsburgh, PA, USA, 5–7 June 2012. [Google Scholar]
- Deng, J.; Zhu, W.Y.; Ma, Q. A new seepage model for shale gas reservoir and productivity analysis of fractured well. Fuel 2014, 124, 232–240. [Google Scholar] [CrossRef]
- Wu, Y.S.; Moridis, G.J.; Bai, B.; Zhang, K. A Multi-Continuum Model for Gas Production in Tight Fractured Reservoirs. In Proceedings of the SPE Hydraulic Fracturing Technology Conference, The Woodlands, TX, USA, 19–21 January 2009. [Google Scholar]
- Bello, R.O.; Watenbargen, R.A. Multi-stage hydraulically fractured horizontal shale gas well rate transient analysis. In Proceedings of the 2010 SPE North Africa Technical Conference and Exhibition-Energy Management in a Challenging Economy, Cairo, Egypt, 14–17 February 2010. [Google Scholar]
- Aboaba, A.; Cheng, Y. Estimation of fracture properties for a horizontal well with multiple hydraulic fractures in gas shale. In Proceedings of the 2010 SPE Eastern Regional Meeting, Morgantown, WV, USA, 13–15 October 2010. [Google Scholar]
- Ozkan, E.; Brown, M.; Raghavan, R.; Kazemi, H. Comparison of fractured-horizontal-well performance in tight sand and shale reservoirs. SPE Reserv. Eval. Eng. 2011, 14, 248–259. [Google Scholar] [CrossRef]
- Brown, M.; Ozkan, E.; Ragahavan, R.; Kazemi, H. Practical solutions for pressure-transient responses of fractured horizontal wells in unconventional shale reservoirs. SPE Reserv. Eval. Eng. 2011, 14, 663–676. [Google Scholar] [CrossRef]
- Stalgorova, E.; Mattar, L. Practical analytical model to simulate production of horizontal wells with branch fractures. In Proceedings of the SPE Canadian Unconventional Resources Conference, Calgray, AB, Canada, 30 October–1 November 2012. [Google Scholar]
- Jiang, J.M.; Rami, M.Y. A multimechanistic multicontinuum model for simulating shale gas reservoir with complex fractured system. Fuel 2015, 161, 333–344. [Google Scholar] [CrossRef]
- Wang, J.; Luo, H.; Liu, H.; Ji, Y.; Cao, F.; Li, Z.; Sepehrnoori, K. Variations of Gas Flow Regimes and Petro-Physical Properties During Gas Production Considering Volume Consumed by Adsorbed Gas and Stress Dependence Effect in Shale Gas Reservoirs. In Proceedings of the 2015 SPE Annual Technical Conference & Exhibition, Houston, TX, USA, 28–30 September 2015. [Google Scholar]
- Wang, F.P.; Reed, R.M. Pore networks and fluid flow in gas shales. In Proceedings of the 2009 SPE Annual Technical Conference & Exhibition, New Orleans, LA, USA, 4–7 October 2009. [Google Scholar]
- Silin, D.; Kneafsey, T. Shale gas: Nanometer-scale observations and well modelling. J. Can. Pet. Technol. 2012, 51, 464–475. [Google Scholar] [CrossRef]
- Zhao, Y.L.; Zhang, L.H.; Zhao, J.Z.; Luo, J.X.; Zhang, B.N. Triple porosity modeling of transient well test and rate decline analysis for multi-fractured horizontal well in shale gas reservoirs. J. Pet. Sci. Eng. 2013, 110, 253–262. [Google Scholar] [CrossRef]
- Wang, H.T. Performance of multiple fractured horizontal wells in shale gas reservoirs with consideration of multiple mechanisms. J. Hydrol. 2014, 510, 299–312. [Google Scholar] [CrossRef]
- Computer Modeling Group Ltd. (CMG). GEM User Guide; Computer Modeling Group Ltd.: Calgary, AB, Canada, 2012. [Google Scholar]
- Karimi-Fard, M.; Firoozabadi, A. Numerical simulation of water injection in 2D fractured media using discrete-fracture network. In Proceedings of the 2001 SPE Annual Technical Conference & Exhibition, New Orleans, LA, USA, 30 September–3 October 2001. [Google Scholar]
- Karimi-Fard, M.; Durlofsky, L.J.; Aziz, K. An Efficient Discrete-Fracture Model Applicable for General-Purpose Reservoir Simulators. In Proceedings of the 2003 SPE Reservoir Simulation Symposium, Houston, TX, USA, 3–5 February 2003. [Google Scholar]
- Moinfar, A.; Varavei, A.; Sepehrnoori, K.; Johns, R.T. Development of a coupled dual continuum and discrete fracture model for the simulation of unconventional reservoirs. In Proceedings of the 2013 SPE Reservoir Simulation Symposium, The Woodlands, TX, USA, 18–20 February 2013. [Google Scholar]

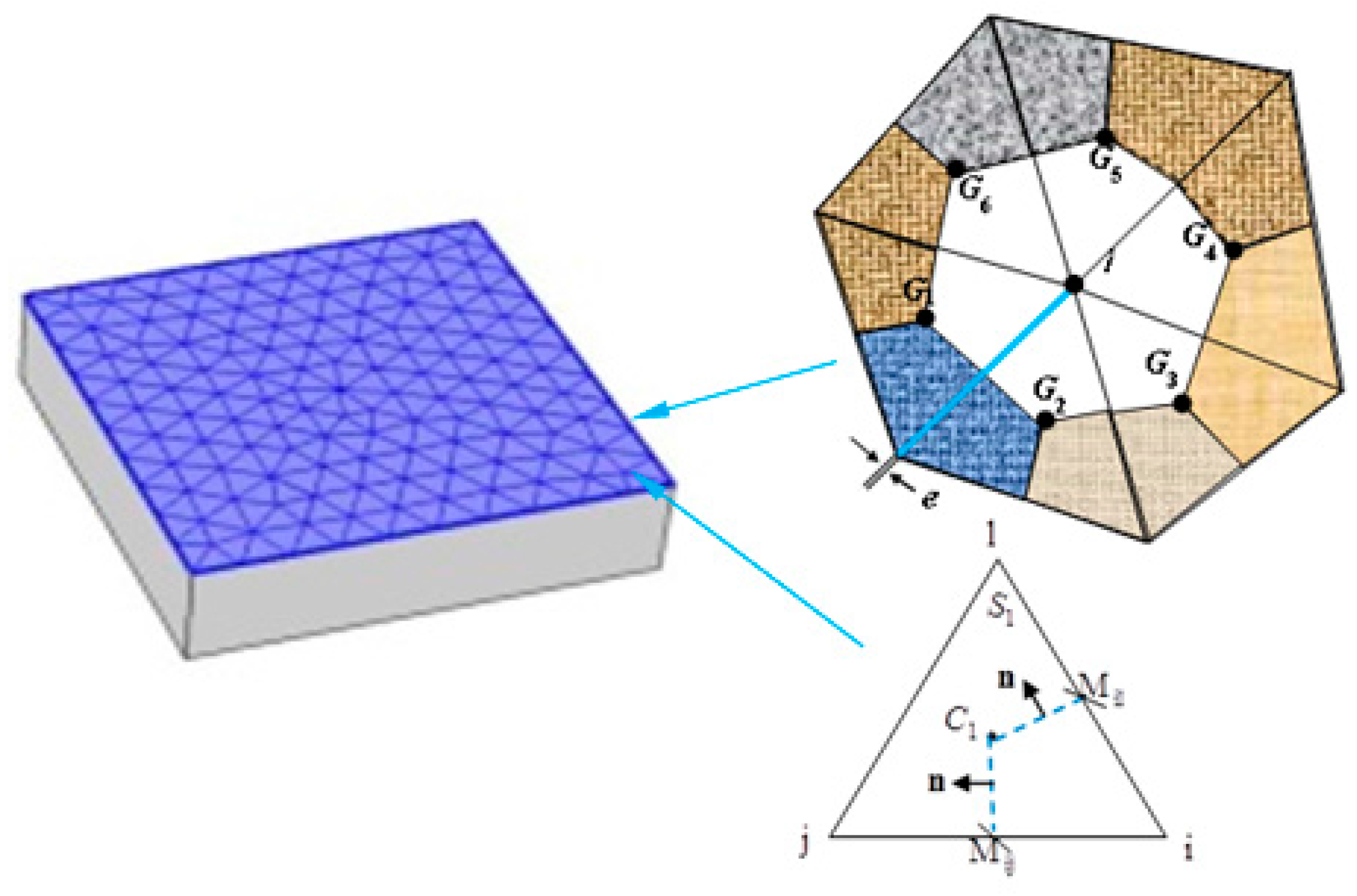
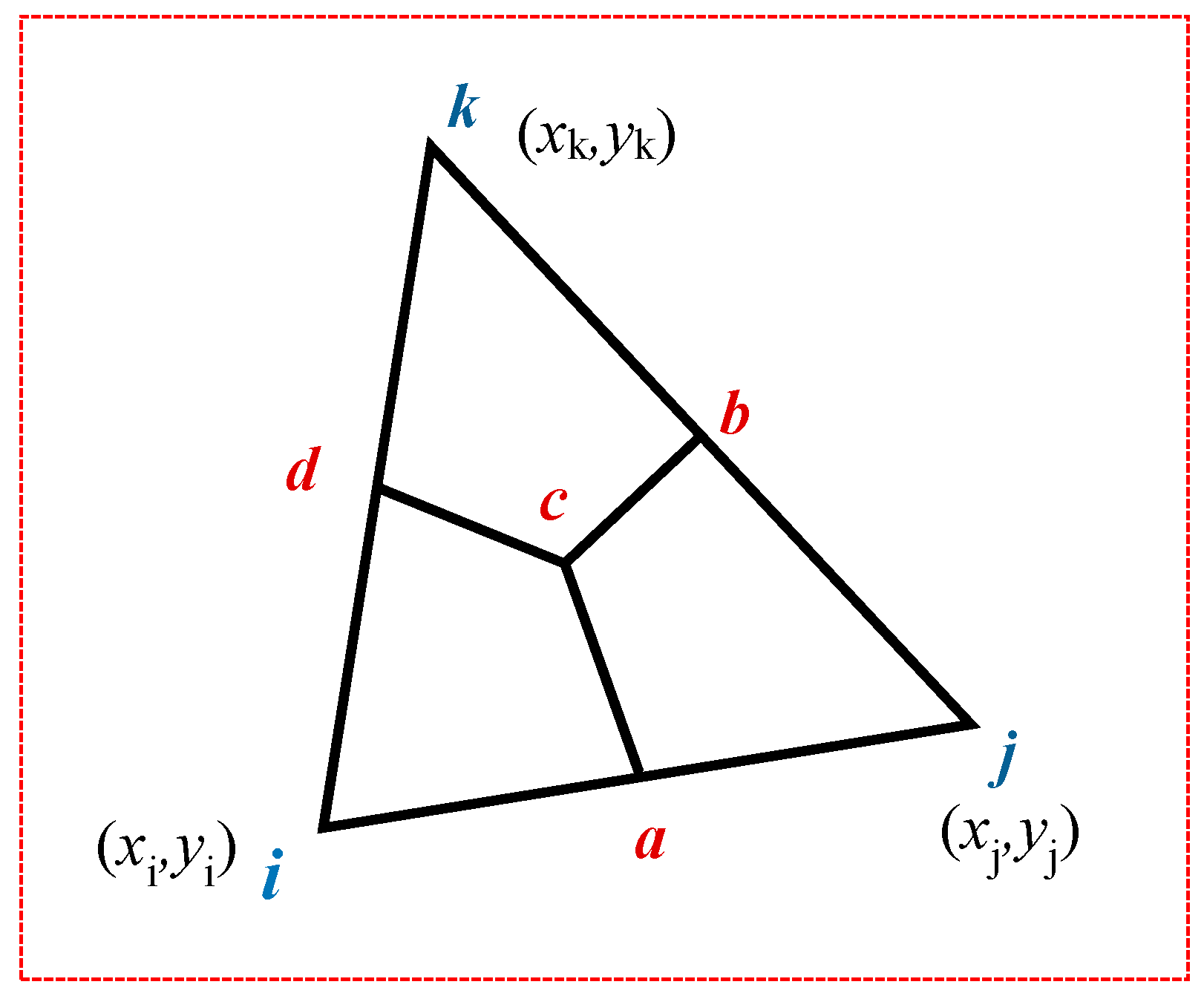
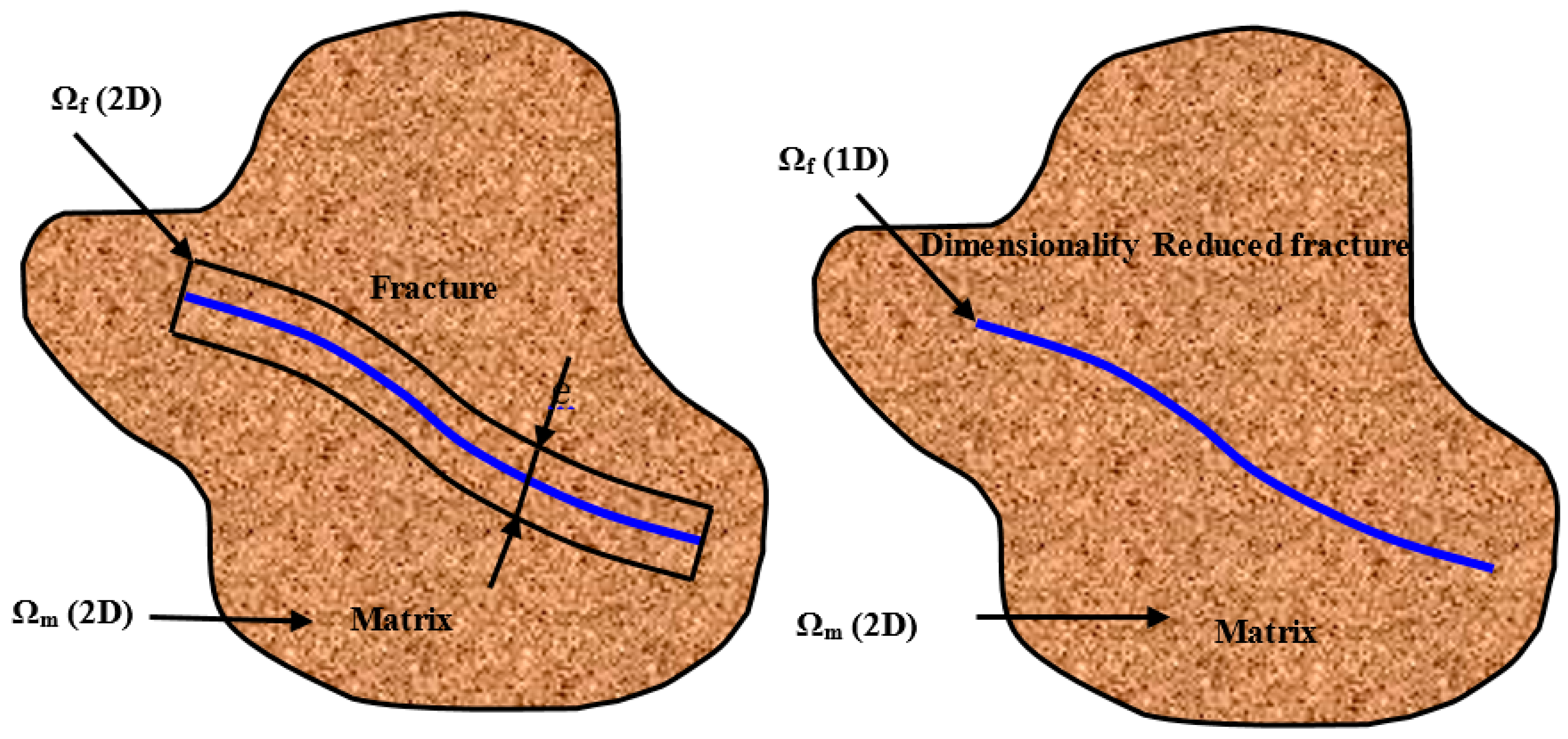


| Reservoir Parameters | Value | Reservoir Parameters | Value |
|---|---|---|---|
| Formation thickness, h, m | 50 | Reservoir boundary, X × Y, m | 800 × 500 |
| Initial reservoir pressure, pi, MPa | 20 | Reservoir temperature, T, °C | 100 |
| Gas specific gravity, rg, fraction | 0.6 | Bottom-hole pressure, pw, MPa | 4 |
| Horizontal wellbore length, L, m | 1000 | Hydraulic fractures numbers, N | 5 |
| Half fracture length, xf, m | 100 | Langmuir pressure, PL, MPa | 4 |
| Inner region fracture porosity, Φf1, % | 3 | Inner region fracture permeability, kf1, mD | 0.01 |
| Outer region fracture porosity, Φf2, % | 1 | Outer region fracture permeability, kf2, mD | 0.001 |
| Matrix porosity, Φm, % | 1 | Matrix permeability, km, mD | 0.0001 |
| Hydraulic fracture width, m | 0.001 | Stress sensitive coefficient, α, MPa−1 | 0.01 |
| Langmuir Volume, VL, m3/m3 | 10 | - | - |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, D.; Dai, Y.; Ma, X.; Zhang, L.; Zhong, B.; Wu, J.; Tao, Z. An Analysis for the Influences of Fracture Network System on Multi-Stage Fractured Horizontal Well Productivity in Shale Gas Reservoirs. Energies 2018, 11, 414. https://doi.org/10.3390/en11020414
Zhang D, Dai Y, Ma X, Zhang L, Zhong B, Wu J, Tao Z. An Analysis for the Influences of Fracture Network System on Multi-Stage Fractured Horizontal Well Productivity in Shale Gas Reservoirs. Energies. 2018; 11(2):414. https://doi.org/10.3390/en11020414
Chicago/Turabian StyleZhang, Deliang, Yu Dai, Xinhua Ma, Liehui Zhang, Bing Zhong, Jianfa Wu, and Zhengwu Tao. 2018. "An Analysis for the Influences of Fracture Network System on Multi-Stage Fractured Horizontal Well Productivity in Shale Gas Reservoirs" Energies 11, no. 2: 414. https://doi.org/10.3390/en11020414
APA StyleZhang, D., Dai, Y., Ma, X., Zhang, L., Zhong, B., Wu, J., & Tao, Z. (2018). An Analysis for the Influences of Fracture Network System on Multi-Stage Fractured Horizontal Well Productivity in Shale Gas Reservoirs. Energies, 11(2), 414. https://doi.org/10.3390/en11020414




