Investigation into Window Insulation Retrofitting of Existing Buildings Using Thin and Translucent Frame-Structure Vacuum Insulation Panels
Abstract
:1. Introduction
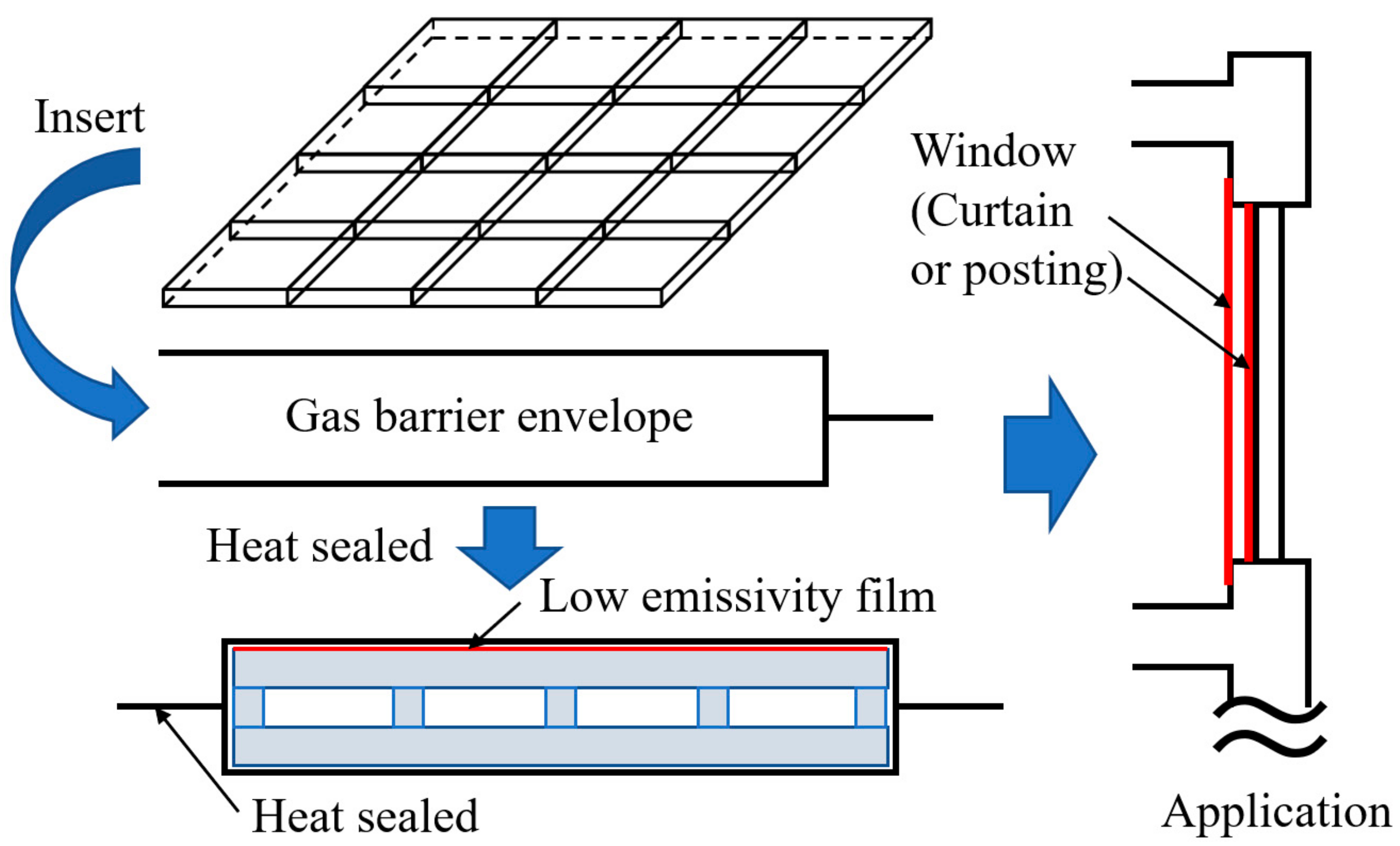
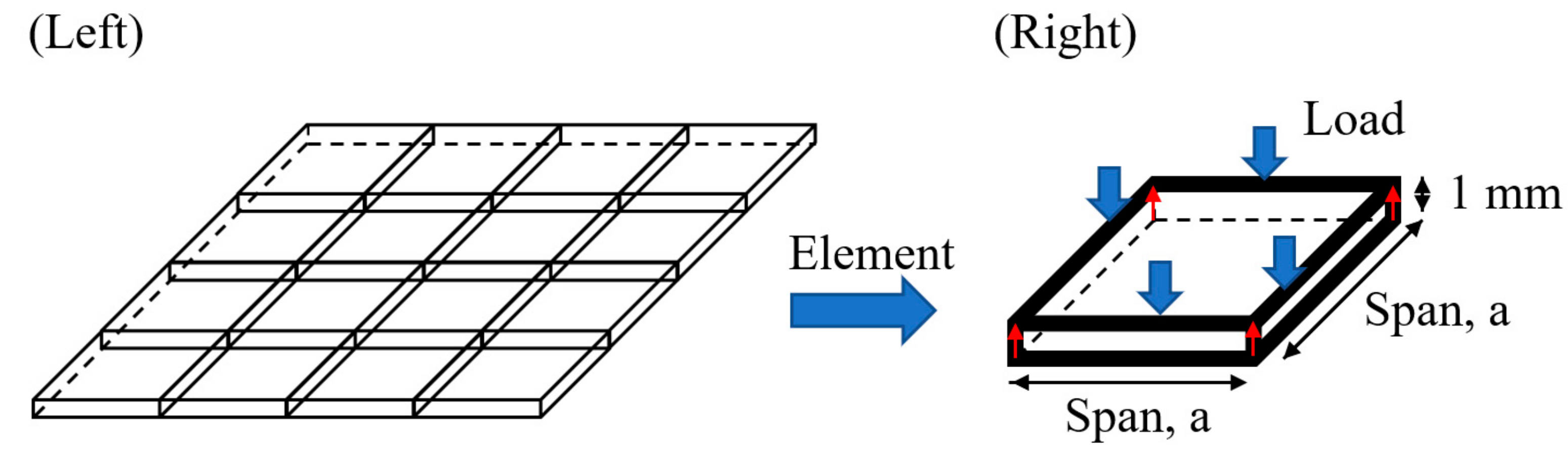
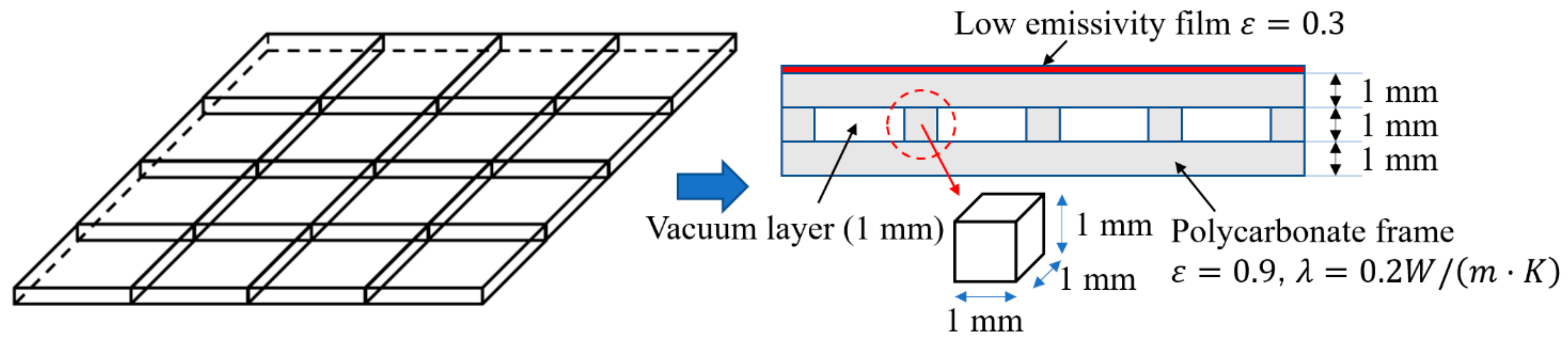
2. Core Structural Design for Frame-Structure VIP
3. Derivation of Heat Transmittance in a VIP Element
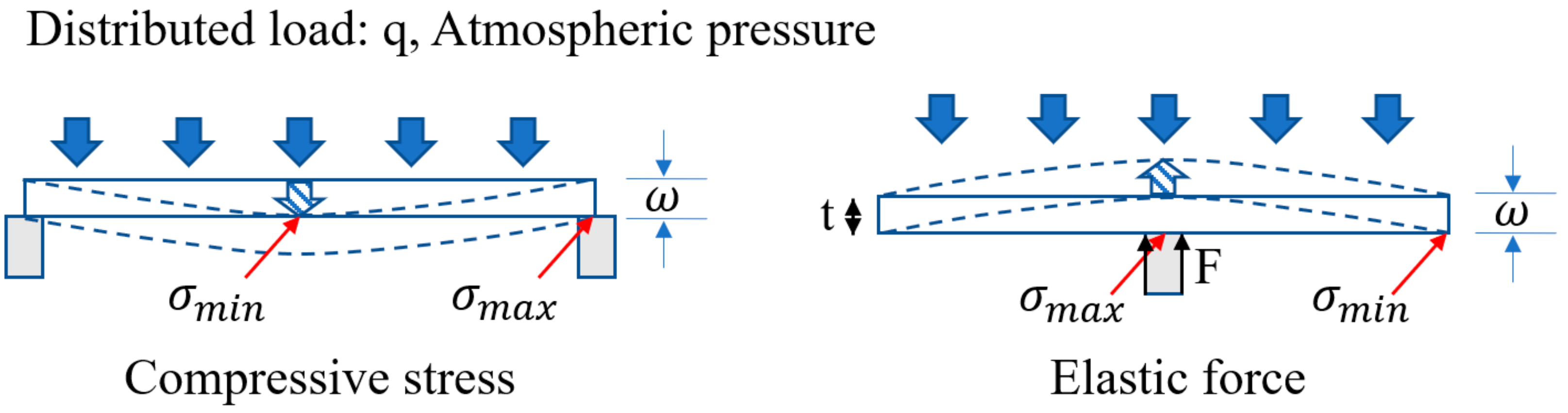
3.1. Calculation and Design of the Vacuum Layer
3.2. Establishment of the Numerical Model of Heat Transmittance
4. Validation of the Numerical Model with Experimental Results
4.1. Specimen Production and its Cost Performance Evaluation
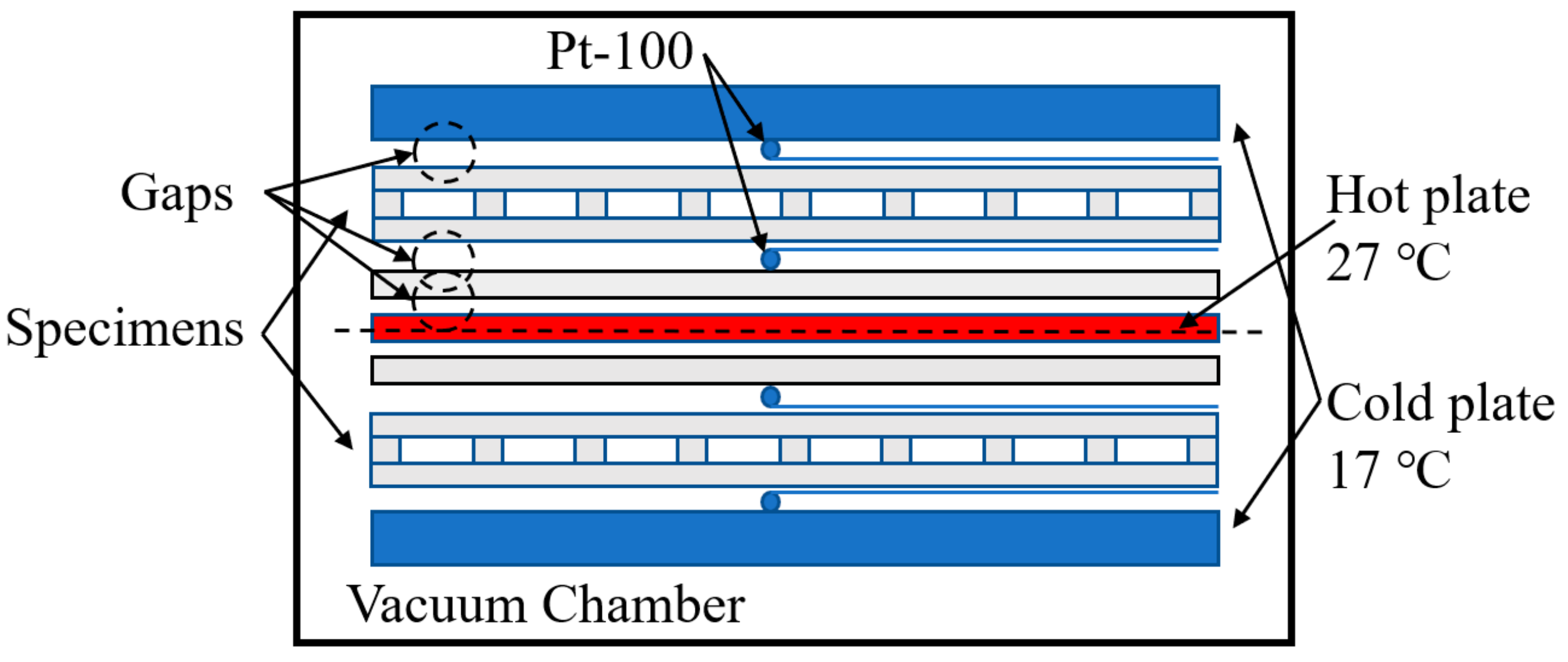
4.2. Guarded Hot Plate Apparatus Framework
4.3. VIP Specimen Using GHP Method, Calculation Conditions, and Results
5. Case Studies for Vacuum Layer Type Translucent VIP
5.1. Calculation Conditions
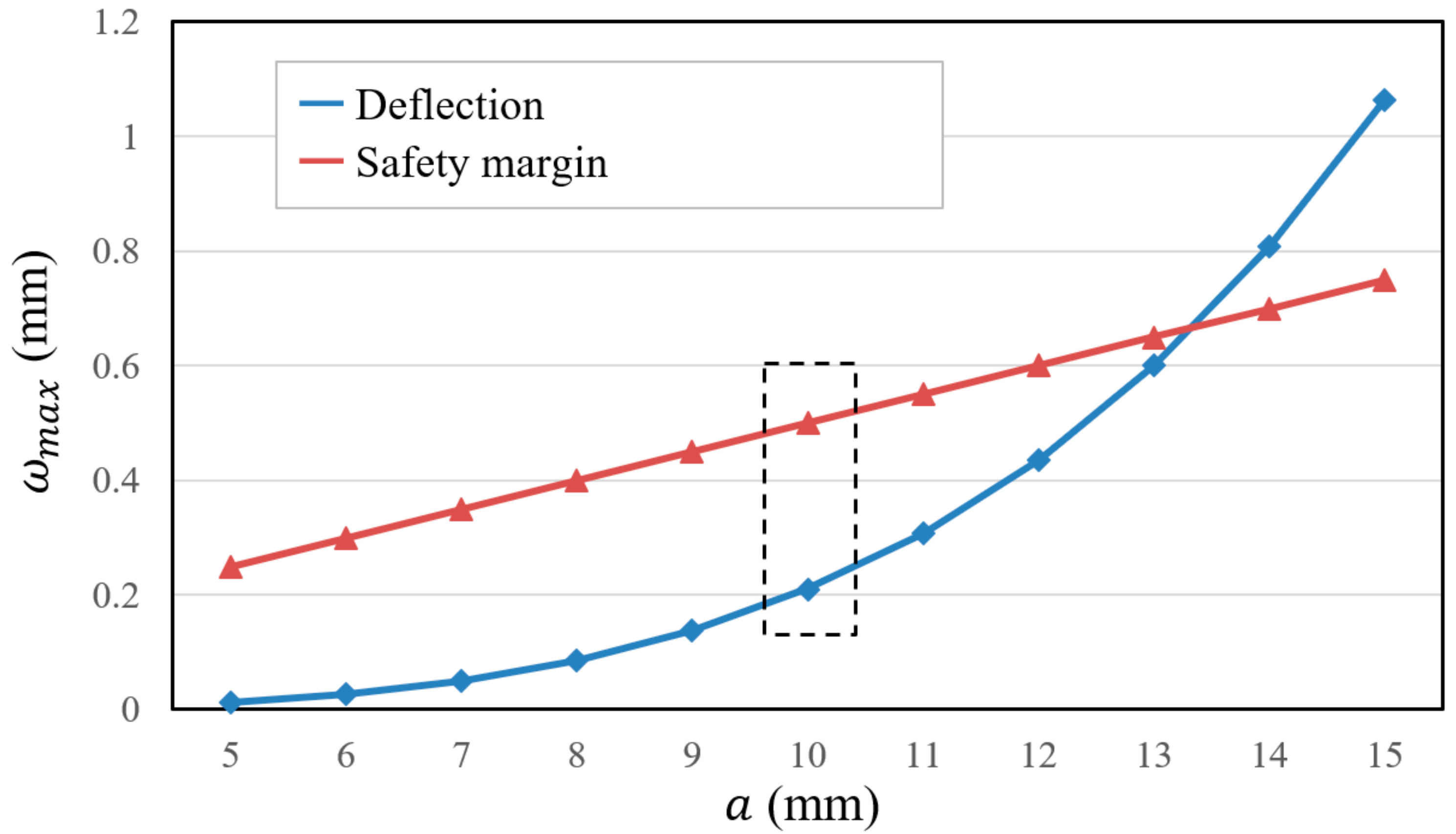
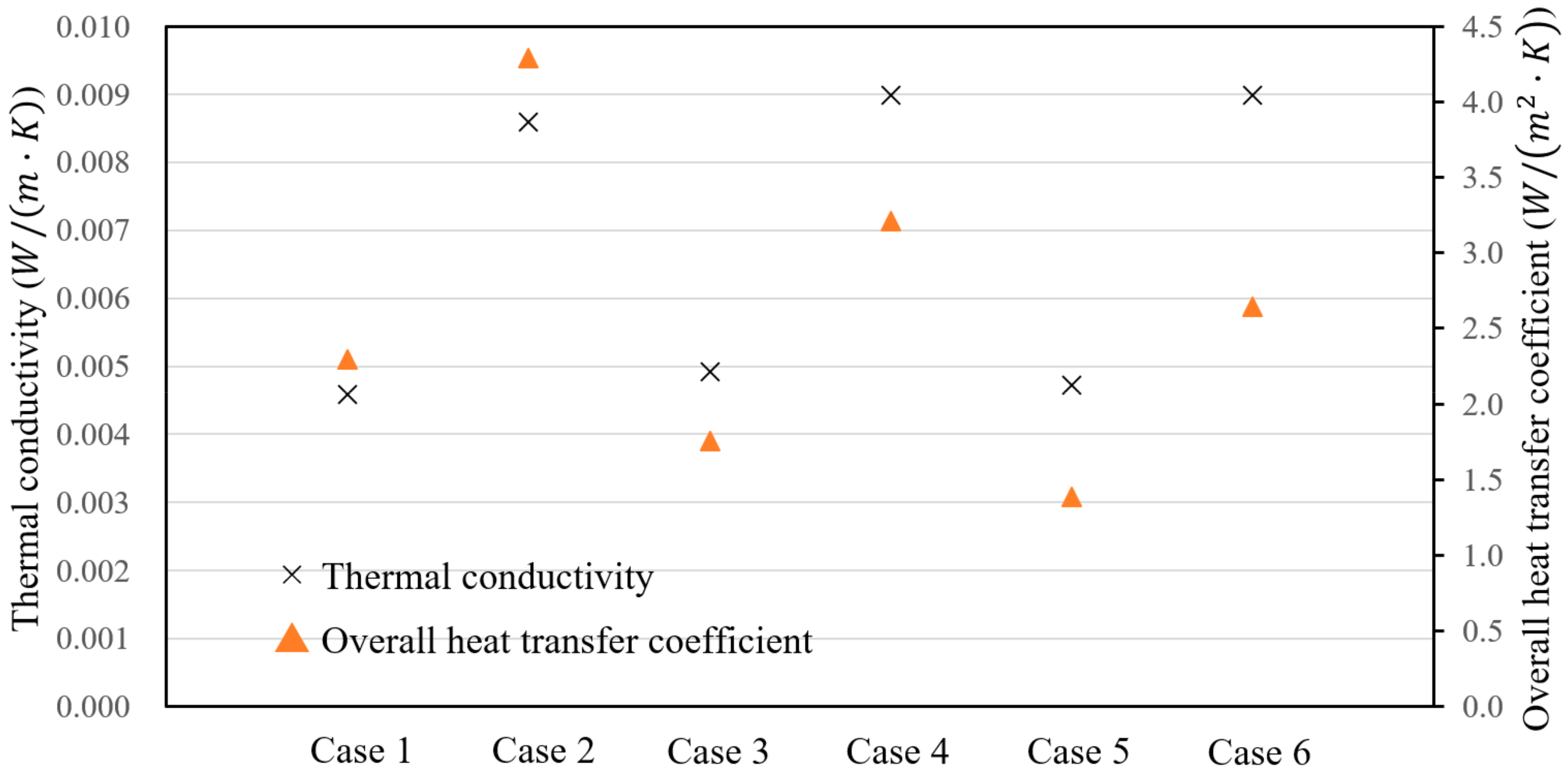
5.2. Results and Discussion
6. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Butler, D. Architecture: Architects of a low-energy future. Nature 2008, 452, 520–523. [Google Scholar] [CrossRef] [PubMed]
- Saari, A.; Kalamees, T.; Jokisalo, J.; Michelsson, R.; Alanne, K.; Kurnitski, J. Financial viability of energy-efficiency measures in a new detached house design in Finland. Appl. Energy 2012, 92, 76–83. [Google Scholar] [CrossRef]
- Lim, T.; Seok, J.; Kim, D.D. A Comparative Study of Energy Performance of Fumed Silica Vacuum Insulation Panels in an Apartment Building. Energies 2017, 10, 2000. [Google Scholar] [CrossRef]
- Capozzoli, A.; Fantucci, S.; Favoino, F.; Perino, M. Vacuum Insulation Panels: Analysis of the Thermal Performance of Both Single Panel and Multilayer Boards. Energies 2015, 8, 2528–2547. [Google Scholar] [CrossRef]
- Park, S.; Choi, B.-H.; Lim, J.-H.; Song, S.-Y. Evaluation of Mechanically and Adhesively Fixed External Insulation Systems Using Vacuum Insulation Panels for High-Rise Apartment Buildings. Energies 2014, 7, 5764–5786. [Google Scholar] [CrossRef]
- Collins, R.E.; Turner, G.M.; Fisher-Cripps, A.C. Vacuum Glazing—A New Component for Insulating Windows. Build. Environ. 1995, 30, 459–492. [Google Scholar] [CrossRef]
- Fisher-Cripps, A.C.; Collins, R.E.; Turner, G.M.; Bezzel, E. Stress and Fracture Probability in Evacuated Glazing. Build. Environ. 1995, 30, 41–59. [Google Scholar] [CrossRef]
- Garrison, J.D.; Collins, R.E. Manufacture and cost of Vacuum Glazing. Sol. Energy 1995, 55, 151–161. [Google Scholar] [CrossRef]
- Turner, G.M.; Collins, R.E. Measurement of Heat flow through Vacuum Glazing at elevated Temperature. Int. J. Heat Mass Transf. 1997, 40, 1437–1446. [Google Scholar] [CrossRef]
- Lenzen, M.; Collins, R.E. Long-term Field Tests of Vacuum Glazing. Sol. Energy 1997, 61, 11–15. [Google Scholar] [CrossRef]
- Collins, R.E.; Simko, T.M. Current Status of the Science and Technology of Vacuum Glazing. Sol. Energy 1998, 62, 189–213. [Google Scholar] [CrossRef]
- Simko, T.M.; Fisher-Cripps, A.C.; Collins, R.E. Temperature-induced in Vacuum Glazing Modelling and Experimental Validation. Sol. Energy 1998, 63, 1–21. [Google Scholar] [CrossRef]
- Nippon Sheet Glass Co. Available online: http://www.nsg-spacia.co.jp (accessed on 18 May 2005).
- Griffiths, P.W.; Di Leo, M.; Cartwright, P.; Eames, P.C.; Yianoulis, P.; Leftheriotis, G.; Norton, B. Fabrication of evacuated glazing at low temperature. Sol. Energy 1998, 63, 243–249. [Google Scholar] [CrossRef]
- Wittwer, V. Private Communication; Fraunhofer Institute for Solar Energy Systems: Freiburg, Germany, 2005. [Google Scholar]
- Baechli, E. Thermally Insulating Construction and/or Lighting Element. Eur. Pat. No. 0247098B1, 25 March 1992. [Google Scholar]
- Baechli, E. Gastight Edge Seal. Eur. Pat. No. 0434802B1, 23 March 1994. [Google Scholar]
- Sager-Hintermann, K.; Baechli, E. Method and Equipment for Making Heat-Insulating Construction and/or Lighting Elements. Eur. Pat. No. 1221526, 10 July 2002. [Google Scholar]
- Yang, Z.; Katsura, T.; Aihara, M.; Nakamura, M.; Nagano, K. Development of Numerical Heat Transfer and the Structural Model to Design Slim and Translucent Vacuum Layer Type Insulation Panels to Retrofitting Insulation in Existing Buildings. Energies 2017, 10, 2108. [Google Scholar] [CrossRef]
- Collins, R.E.; Davis, C.A.; Dey, C.J.; Robinson, S.J.; Tang, J.Z.; Turner, G.M. Measurement of local heat flow in flat evacuated glazing. Int. J. Heat Mass Transf. 1993, 36, 2553–2563. [Google Scholar] [CrossRef]
- Timoshenko, S.; Woinowsky-Krieger, S. Theory of Plate and Shell; McGraw-Hill Book Company: Columbus, OH, USA, 1959; pp. 106–108. [Google Scholar]
- Young, W.C.; Budynas, R.G. Roark’s Formulas for Stress and Strain, 7th ed.; McGraw-Hill Professional: New York, NY, USA, 2001; pp. 508–509. [Google Scholar]
- Gan, G. Thermal transmittance of multiple glazing: Computational fluid dynamics prediction. Appl. Therm. Eng. 2001, 21, 1583–1592. [Google Scholar] [CrossRef]
- Springer, G.S. Heat Transfer in Rare Field Gases. Adv. Heat Transf. 1971, 7, 163–218. [Google Scholar]
- Jousten, K.; Jitschin, W.; Sharipov, F.; Pumps, P.D.; Dirscherl, J.; Lachenmann, R.; Jünemann, A.; Friedrichsen, I.; Lippelt, E.; Kossek, B.; et al. Handbook of Vacuum Technology, 1st ed.; Jousten, K., Ed.; Wiley: Weinheim, Germany, 2008; pp. 51–81. [Google Scholar]
- Eckert, E.R.G.; Drake, R.M. Heat and Mass Transfer, 2nd Revised ed.; McGraw-Hill Inc.: Columbus, OH, USA, 1959. [Google Scholar]
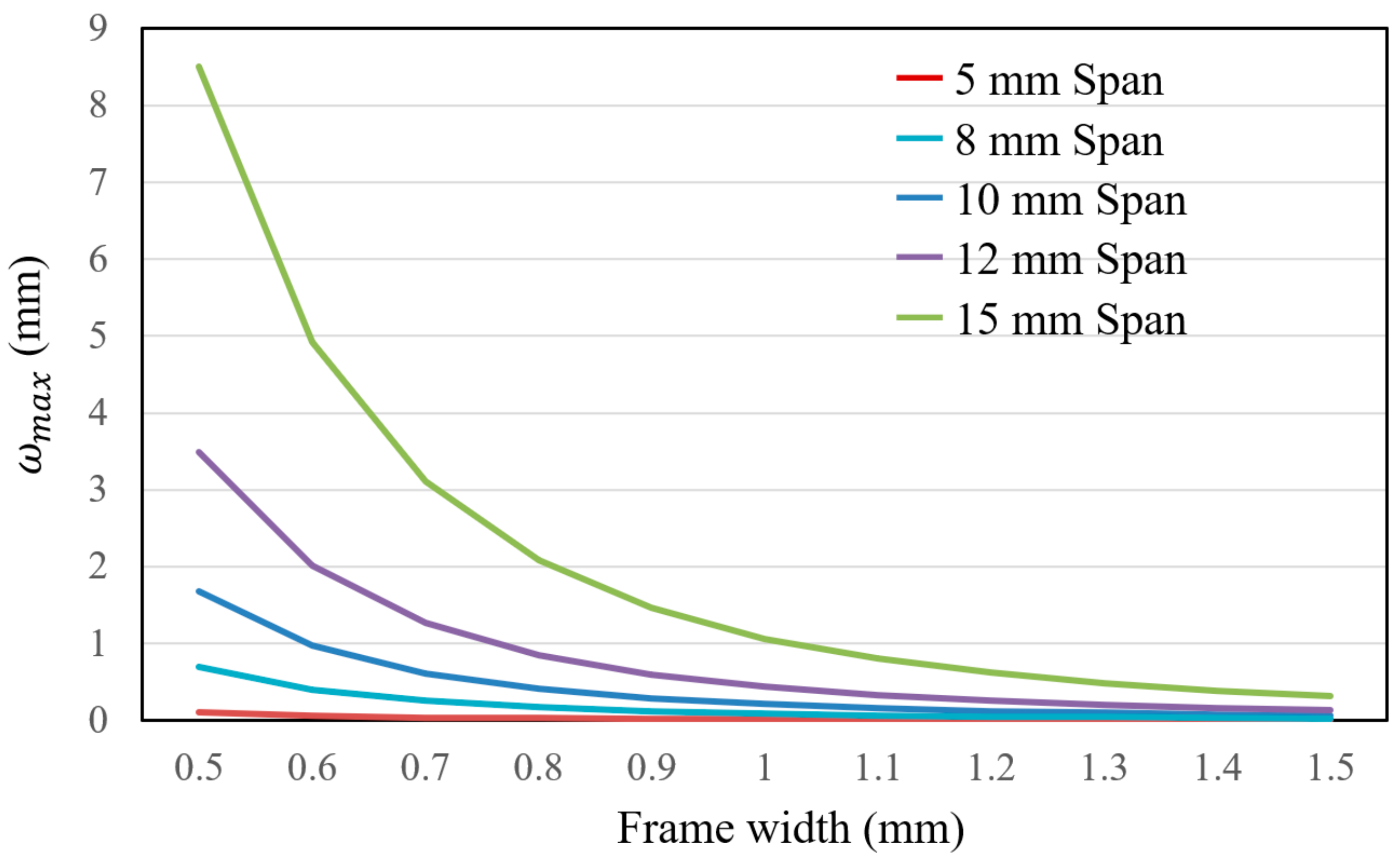
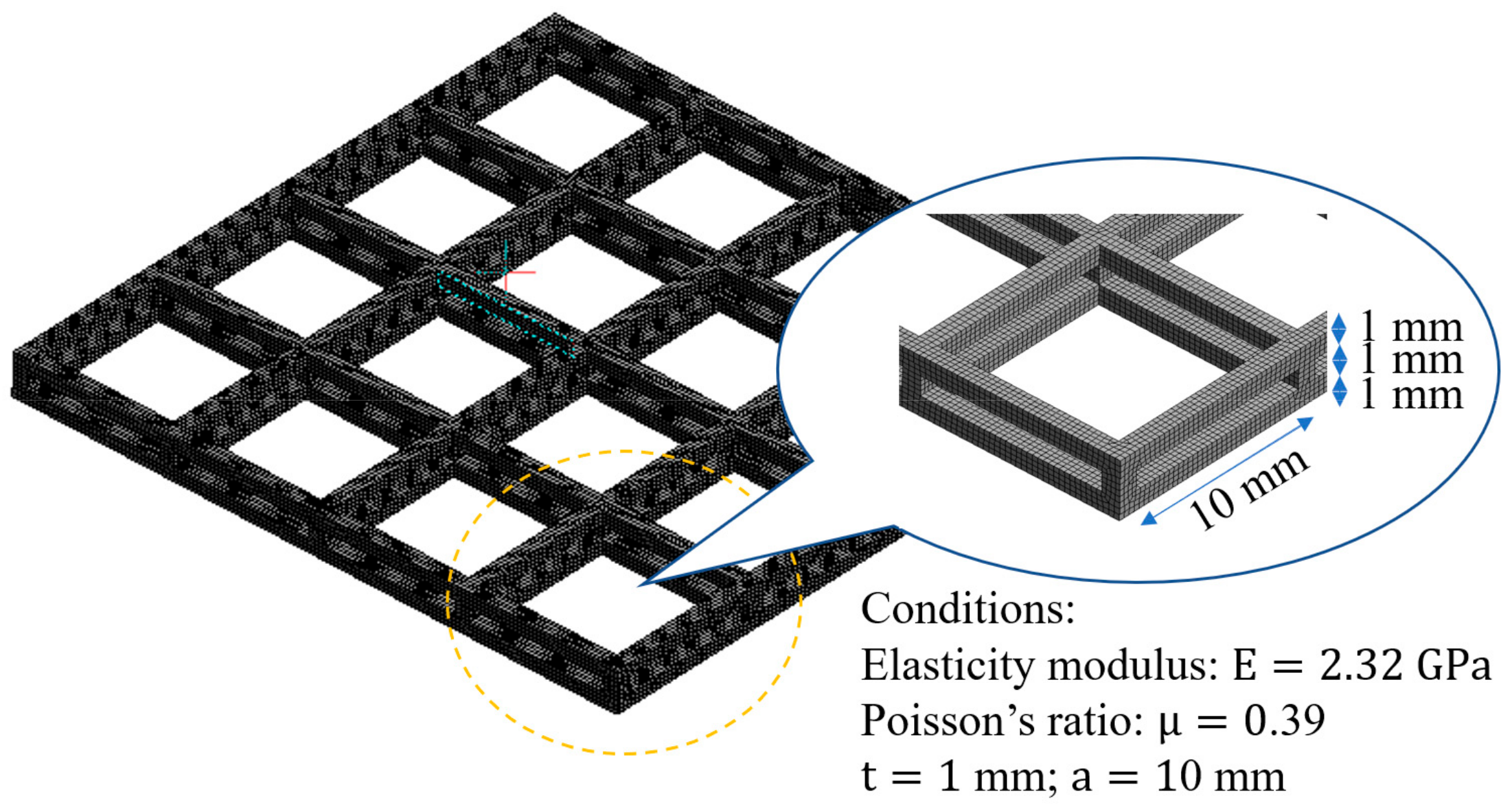
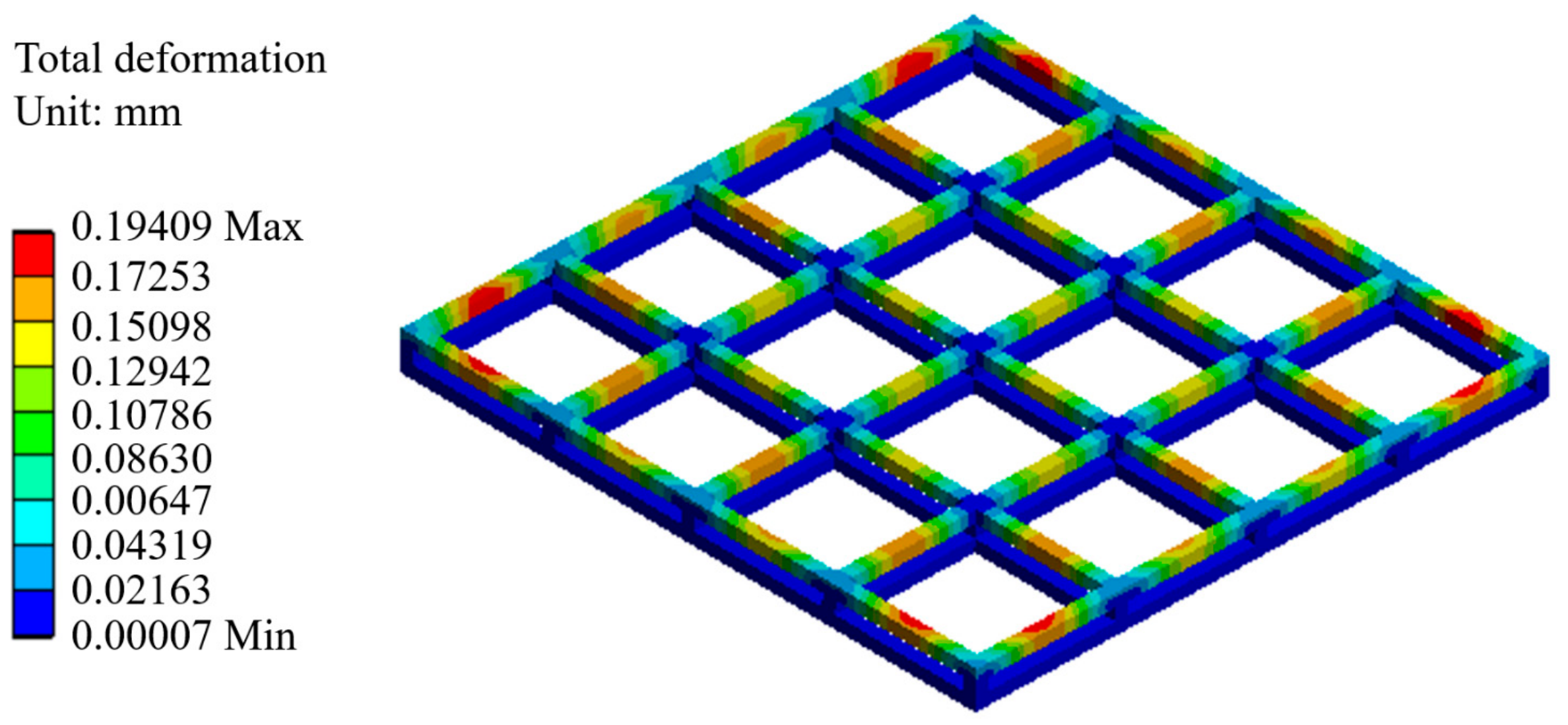
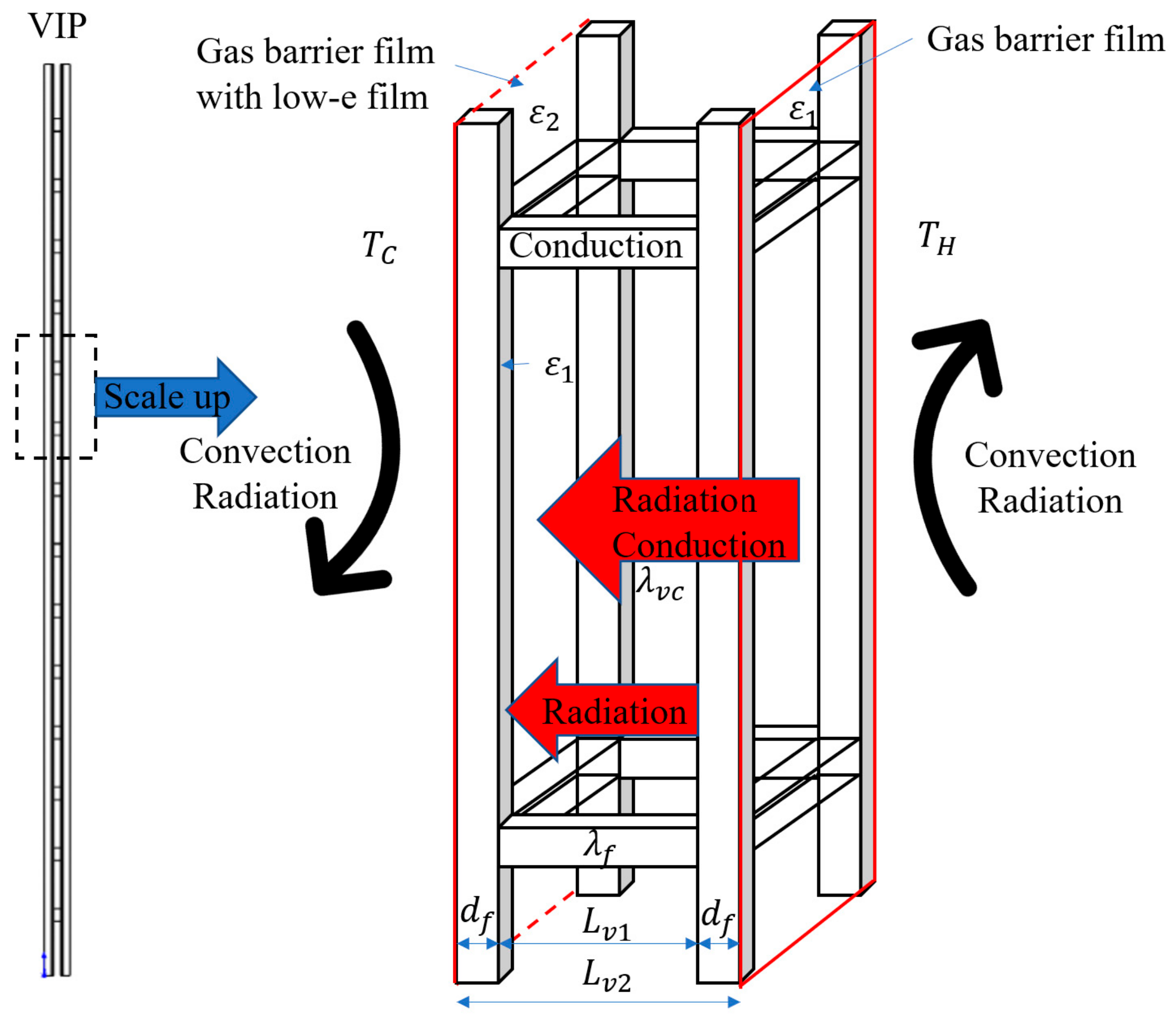
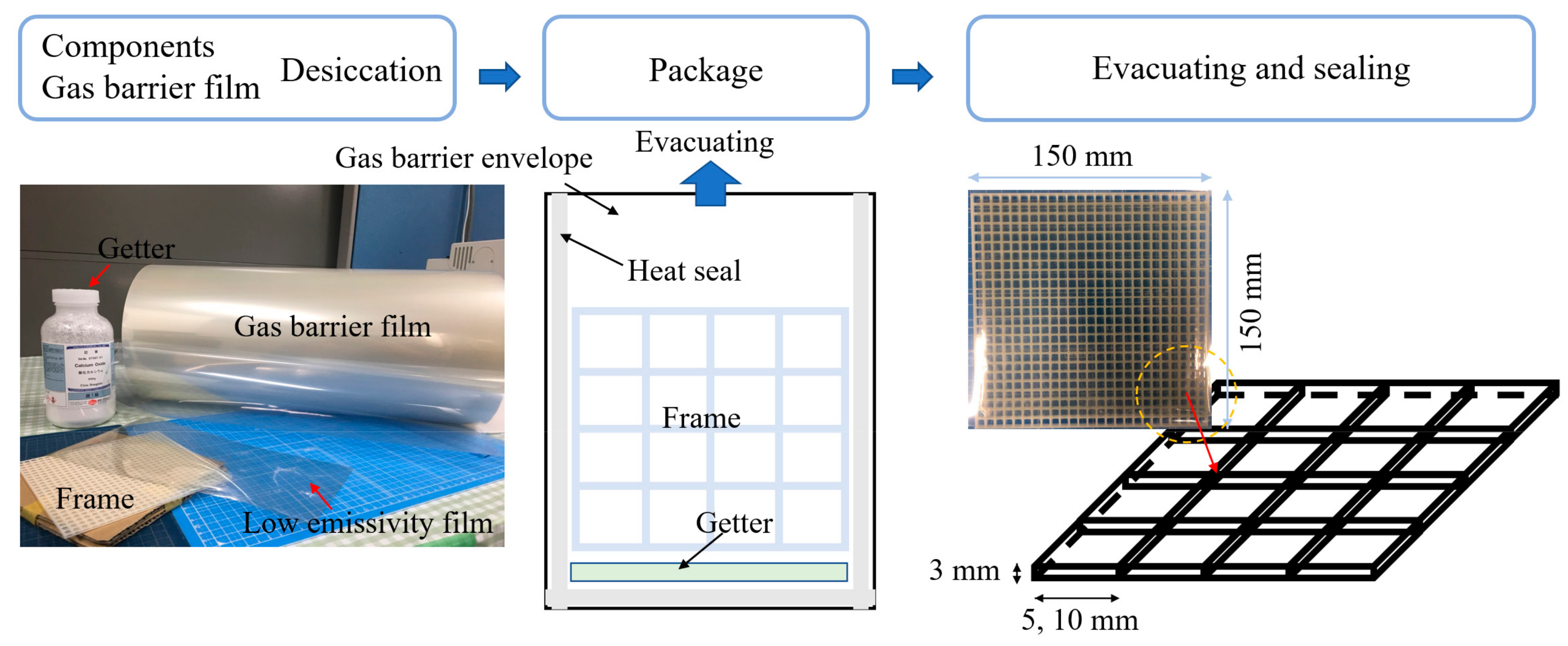
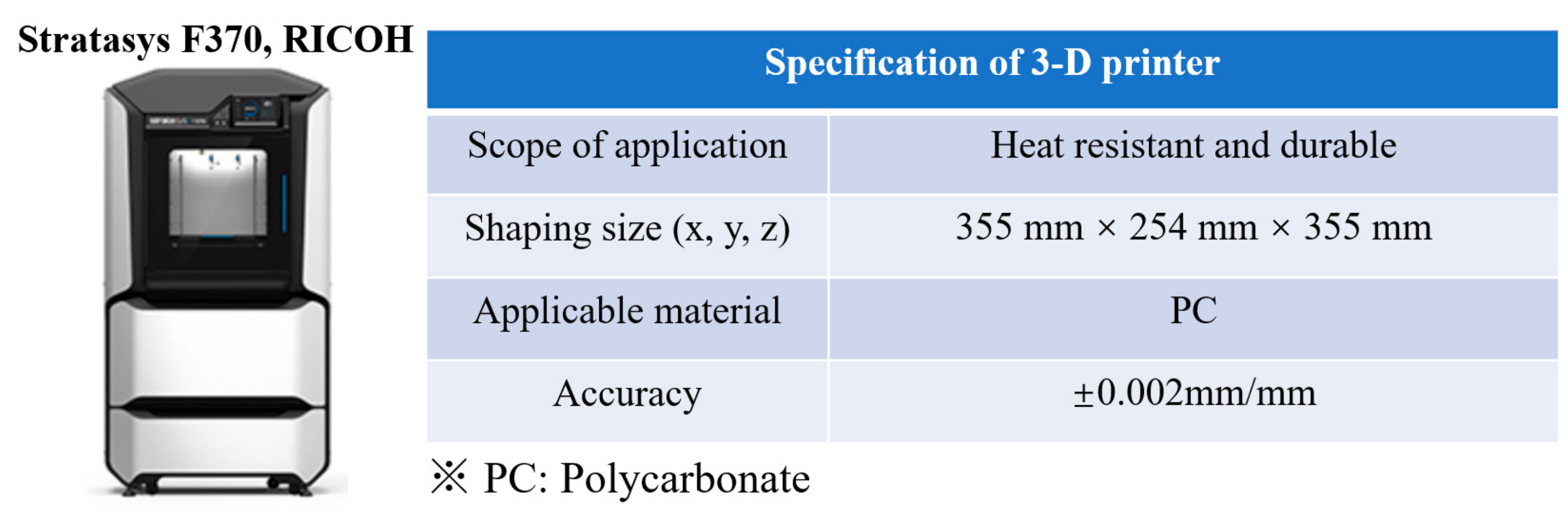
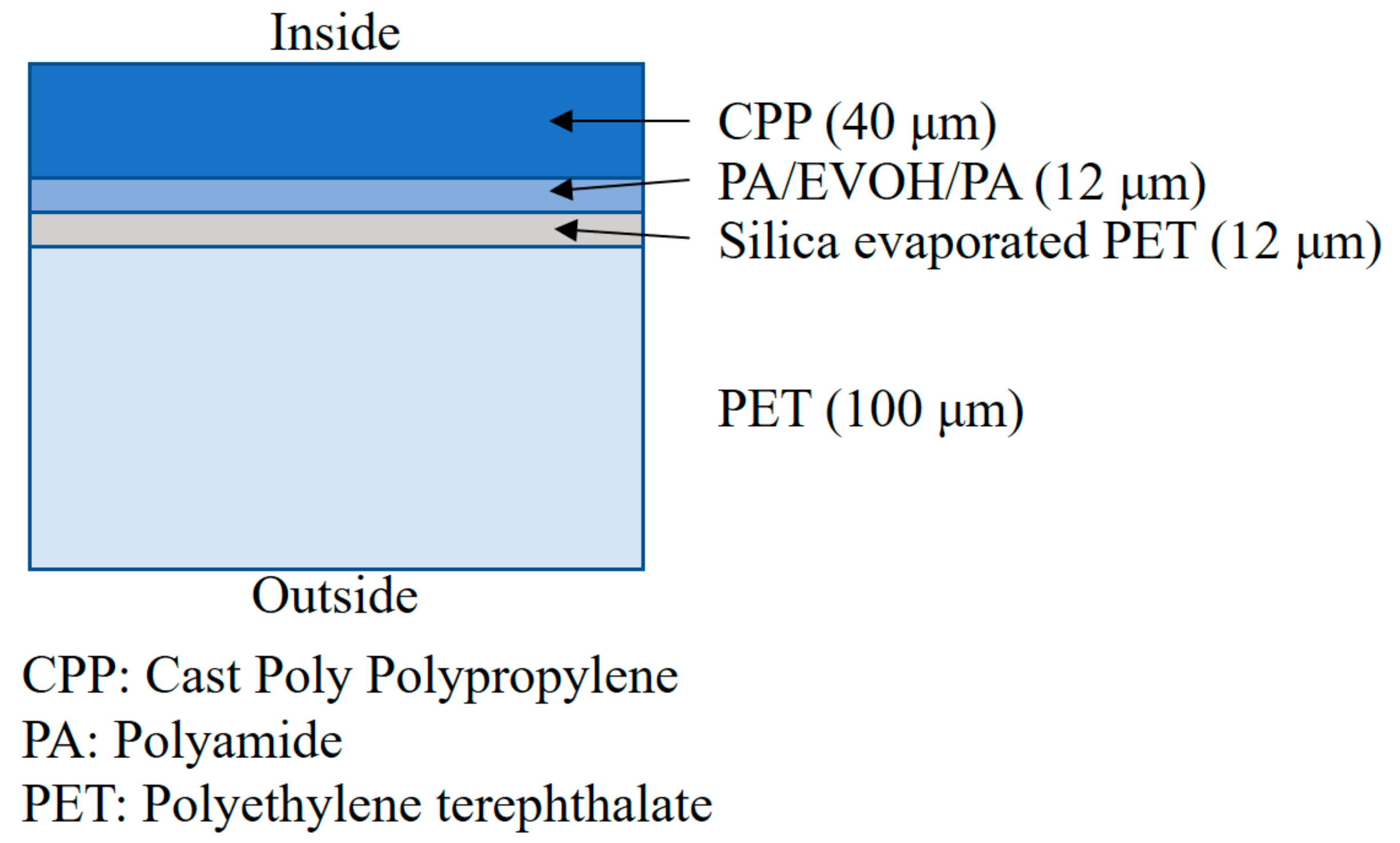
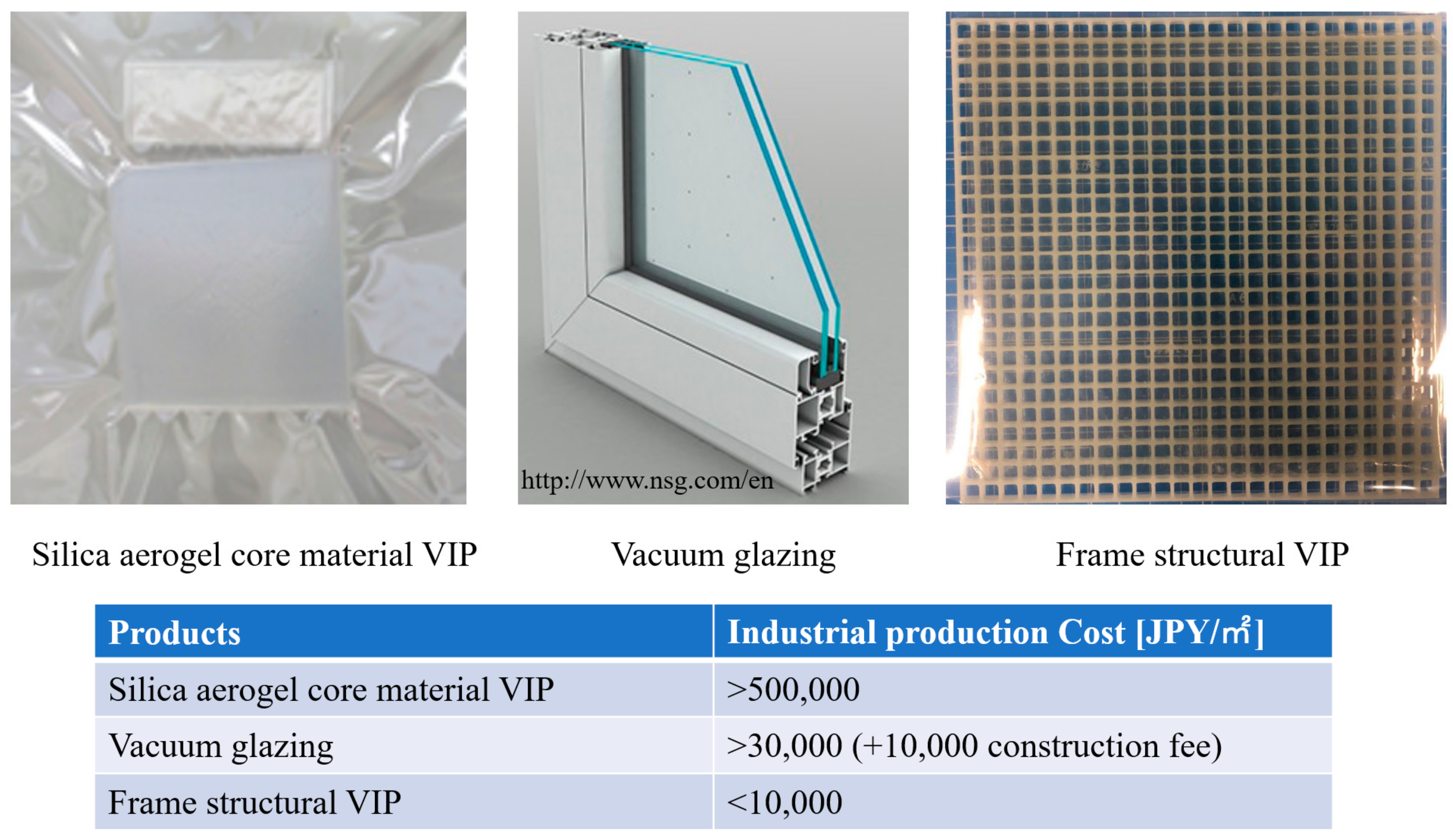
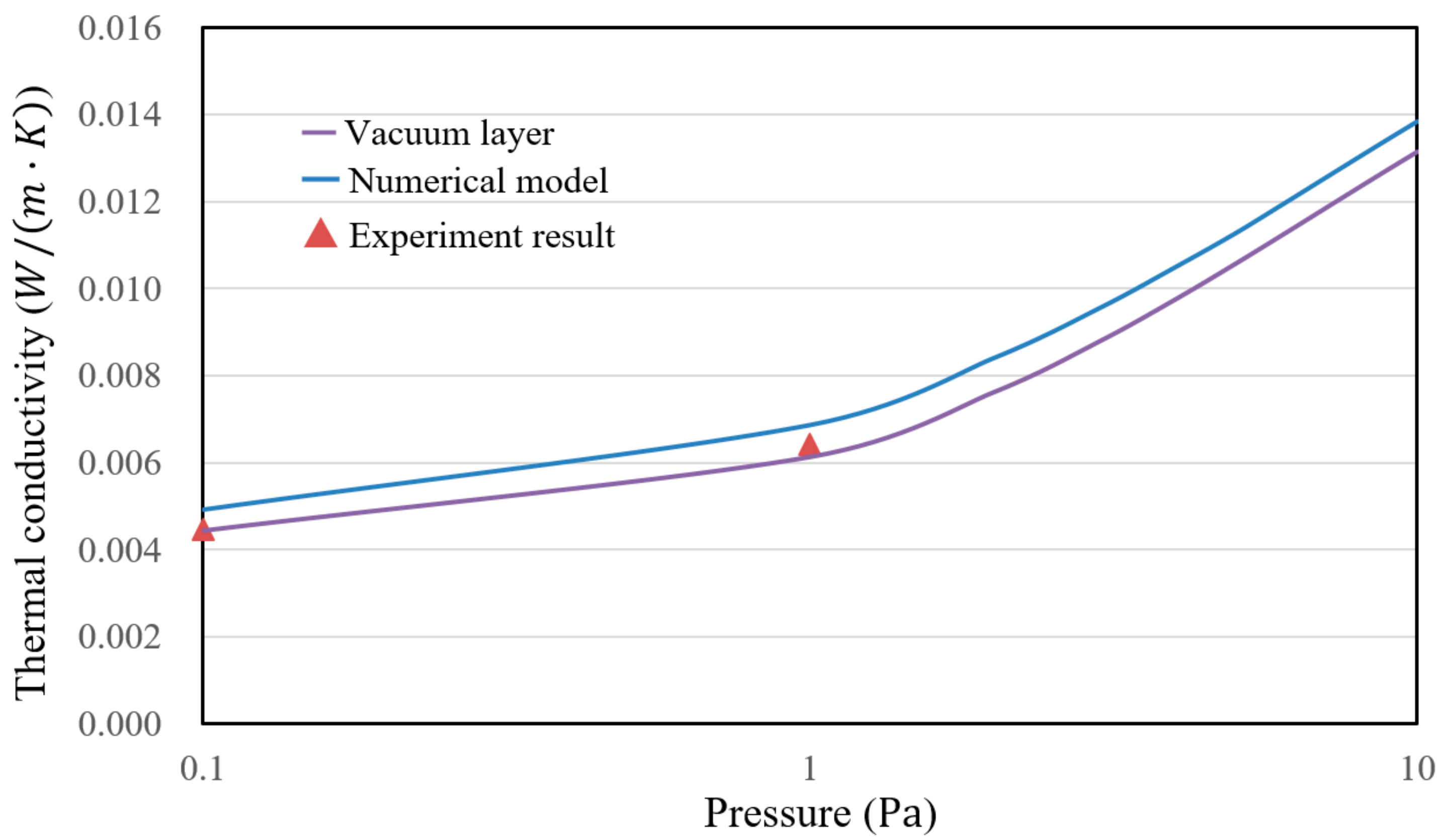
| Pressure (Pa) | Experimental Thermal Conductivity λm (mW/(m·K)) | Experimental Thermal Resistance Rm ((m2·K)/W) | Thermal Resistance of Gaps Rva ((m2·K)/W) | Thermal Resistance of VIP (Optimized) RVIP ((m2·K)/W) | Thermal Conductivity of VIP (Optimized) λVIP (mW/(m·K)) |
|---|---|---|---|---|---|
| 0.1 | 2.7 | 2.10 | 0.21 | 1.27 | 4.2 |
| 1 | 3.4 | 1.57 | 0.17 | 0.88 | 6.2 |
| Conditions | Lv (m) | P (Pa) | Frame Width (mm) | Span (mm) | ε1 | ε2 | Thickness (mm) |
|---|---|---|---|---|---|---|---|
| Case 1 | 1.0 × 10–3 | 0.1 | 0.5 | 5 | 0.3 | 0.9 | 2 |
| Case 2 | 1 | 0.5 | 5 | 0.9 | 0.9 | 2 | |
| Case 3 | 0.1 | 0.9 | 10 | 0.3 | 0.9 | 2.8 | |
| Case 4 | 1 | 0.9 | 10 | 0.3 | 0.9 | 2.8 | |
| Case 5 | 0.1 | 1.2 | 15 | 0.9 | 0.9 | 3.4 | |
| Case 6 | 1 | 1.2 | 15 | 0.3 | 0.9 | 3.4 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, Z.; Katsura, T.; Aihara, M.; Nakamura, M.; Nagano, K. Investigation into Window Insulation Retrofitting of Existing Buildings Using Thin and Translucent Frame-Structure Vacuum Insulation Panels. Energies 2018, 11, 298. https://doi.org/10.3390/en11020298
Yang Z, Katsura T, Aihara M, Nakamura M, Nagano K. Investigation into Window Insulation Retrofitting of Existing Buildings Using Thin and Translucent Frame-Structure Vacuum Insulation Panels. Energies. 2018; 11(2):298. https://doi.org/10.3390/en11020298
Chicago/Turabian StyleYang, Zhang, Takao Katsura, Masahiro Aihara, Makoto Nakamura, and Katsunori Nagano. 2018. "Investigation into Window Insulation Retrofitting of Existing Buildings Using Thin and Translucent Frame-Structure Vacuum Insulation Panels" Energies 11, no. 2: 298. https://doi.org/10.3390/en11020298
APA StyleYang, Z., Katsura, T., Aihara, M., Nakamura, M., & Nagano, K. (2018). Investigation into Window Insulation Retrofitting of Existing Buildings Using Thin and Translucent Frame-Structure Vacuum Insulation Panels. Energies, 11(2), 298. https://doi.org/10.3390/en11020298







