A Multi-Attribute Expansion Planning Model for Integrated Gas–Electricity System
Abstract
:1. Introduction
- With the proposed MADM method, a decision maker has freedom to choose a plan in which either the expansion cost of each of the energy parties or risk level of the system has priority in decision making.
- Using the proposed MADM method, privacy of gas and electricity network operators is preserved with a minimum data exchange.
2. Operation Model
2.1. Electricity Network Operation
2.2. Gas Network Operation
3. Expansion Planning Model
3.1. Cost Minimization Model of Expansion
3.2. Proposed Attributes
3.2.1. EEC
3.2.2. GEC
3.2.3. MMR
3.2.4. β_R
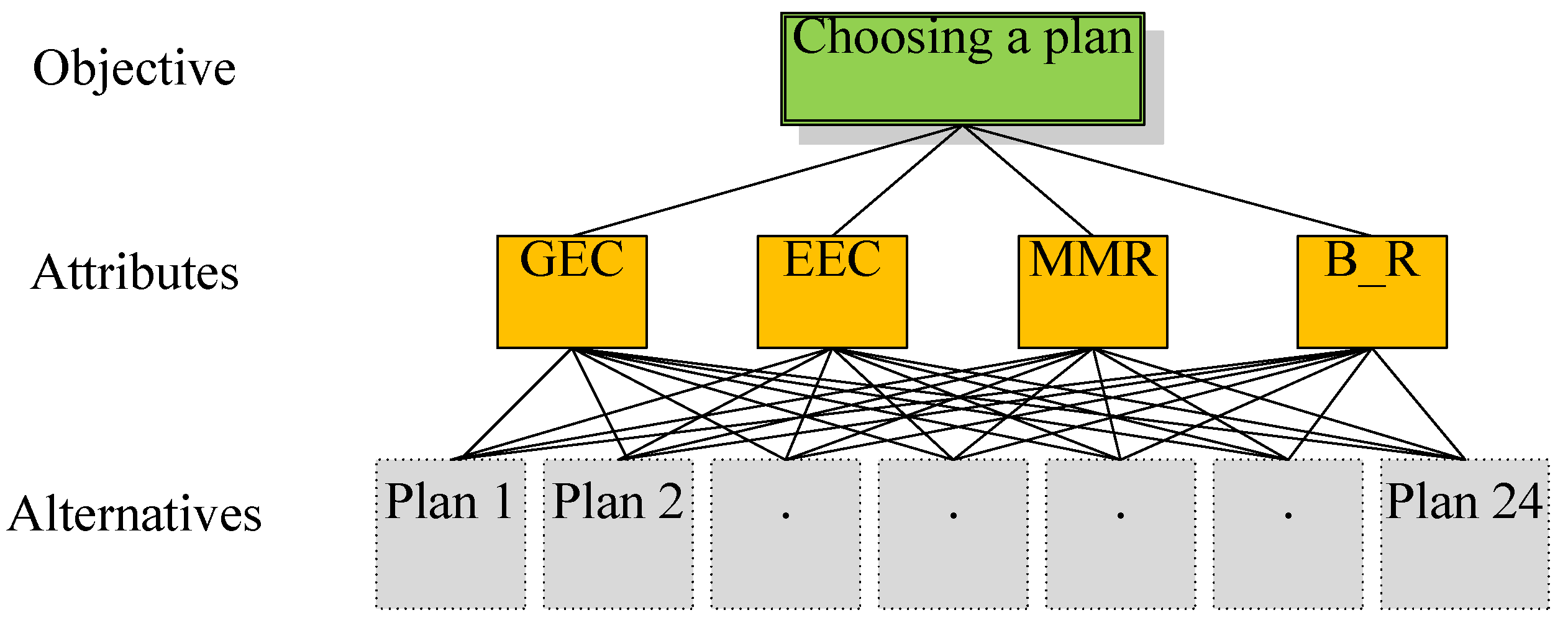
3.3. Decision Making with MADM
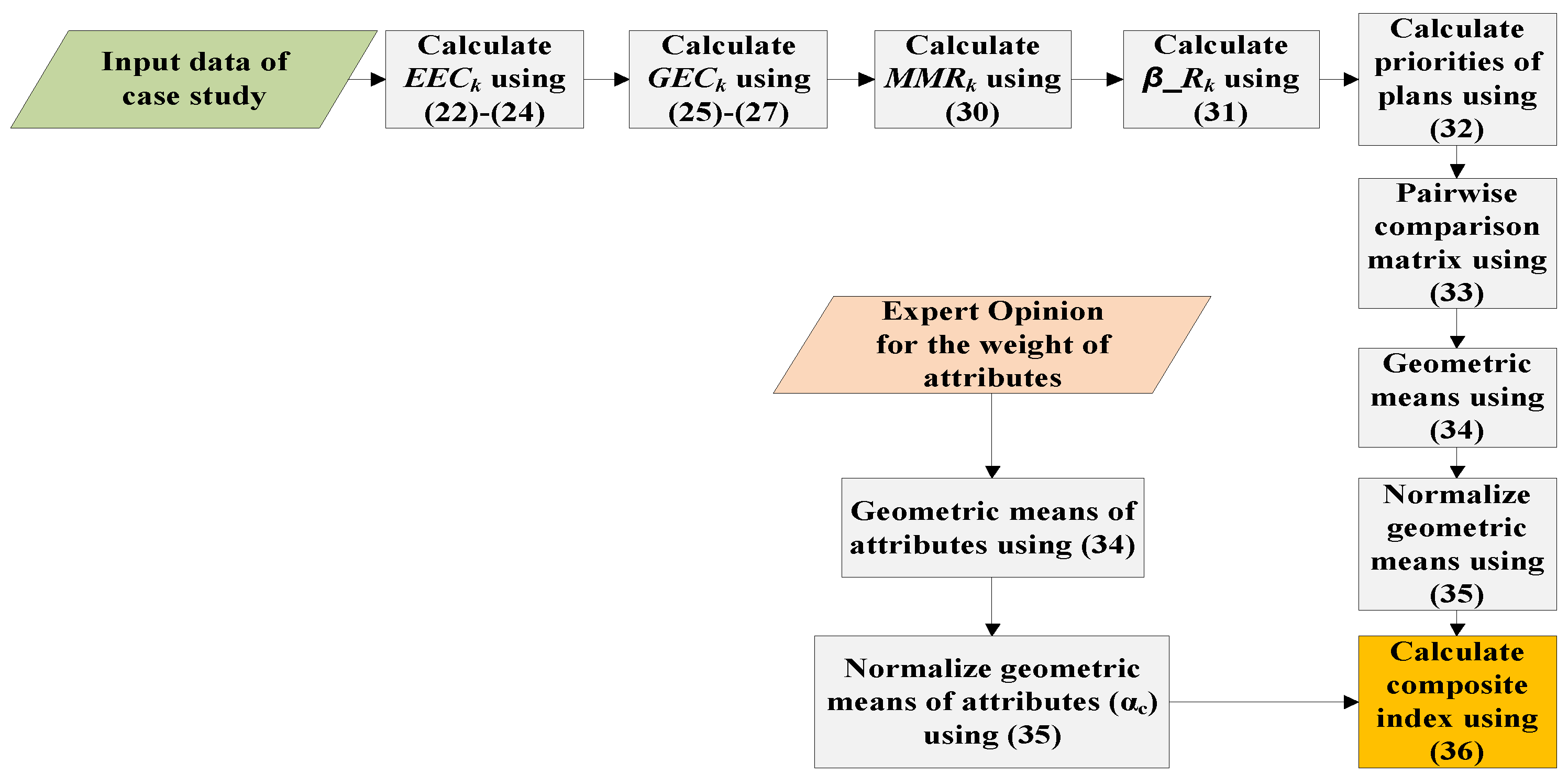
3.4. Solution Method
| Step 1 | Calculate EEC for each plan according to the optimization problem (22)–(24) |
| Step 2 | Calculate GEC for each plan according to the optimization problem (25)–(27) |
| Step 3 | Calculate MMR for each plan using (30) |
| Step 4 | Calculate β_R for each plan using (31) |
| Step 5 | Measure the priorities of plans at each attribute using (32) |
| Step 6 | Build the pairwise comparison matrix using (33) |
| Step 7 | Calculate the geometric mean for each row of the pairwise comparison matrix using (34) |
| Step 8 | Normalize the calculated geometric means using (35) |
| Step 9 | Compute the composite index of MADM method using (36) |
4. Discussion
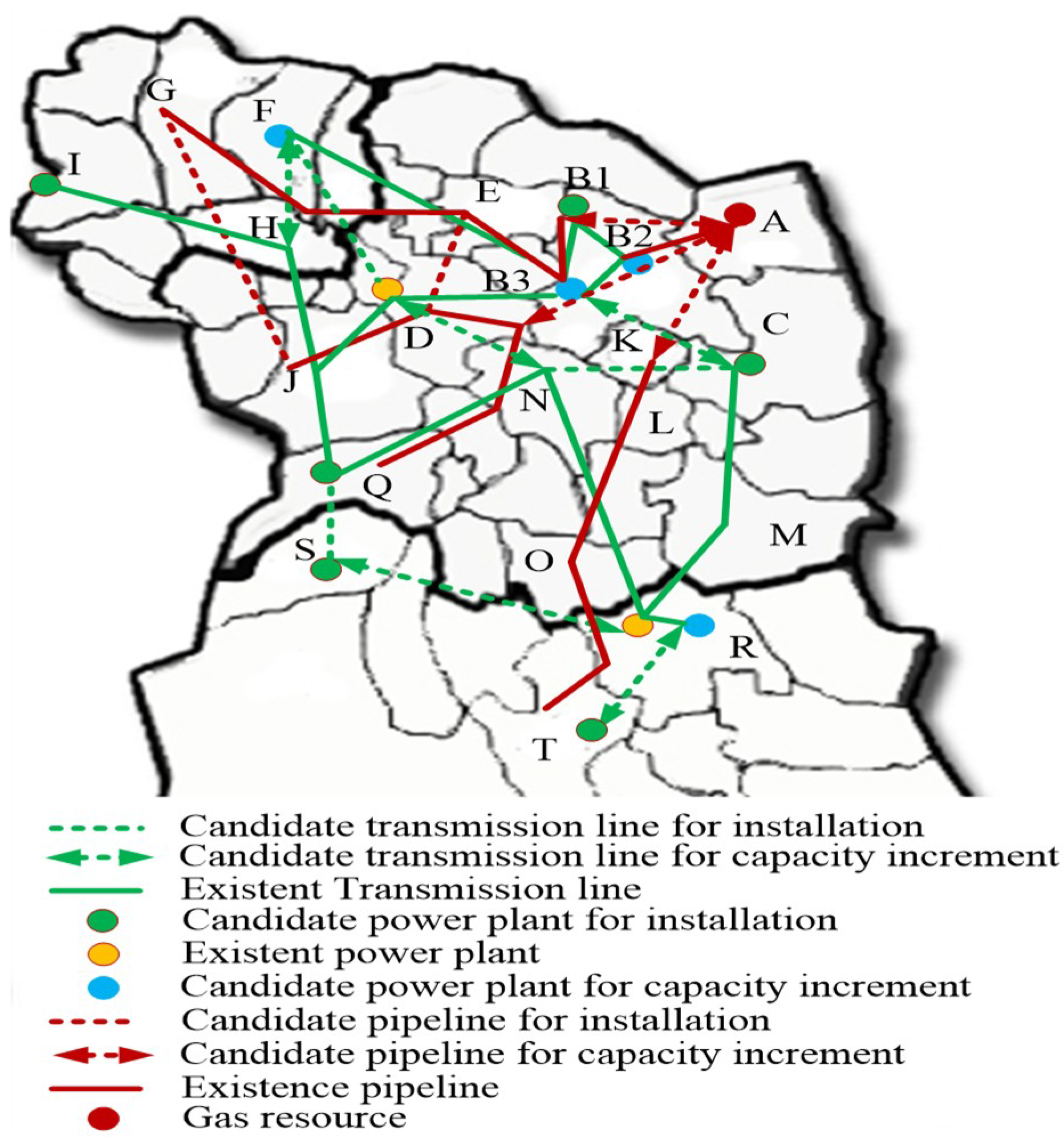
4.1. Data
4.2. Numerical Results
- Case 1: gas and electricity systems have the same priorities
- Case 2: electricity system has higher priority than gas system
- Case 3: gas system has higher priority than electricity system
- Case 4: MMR has higher priority than the other attributes
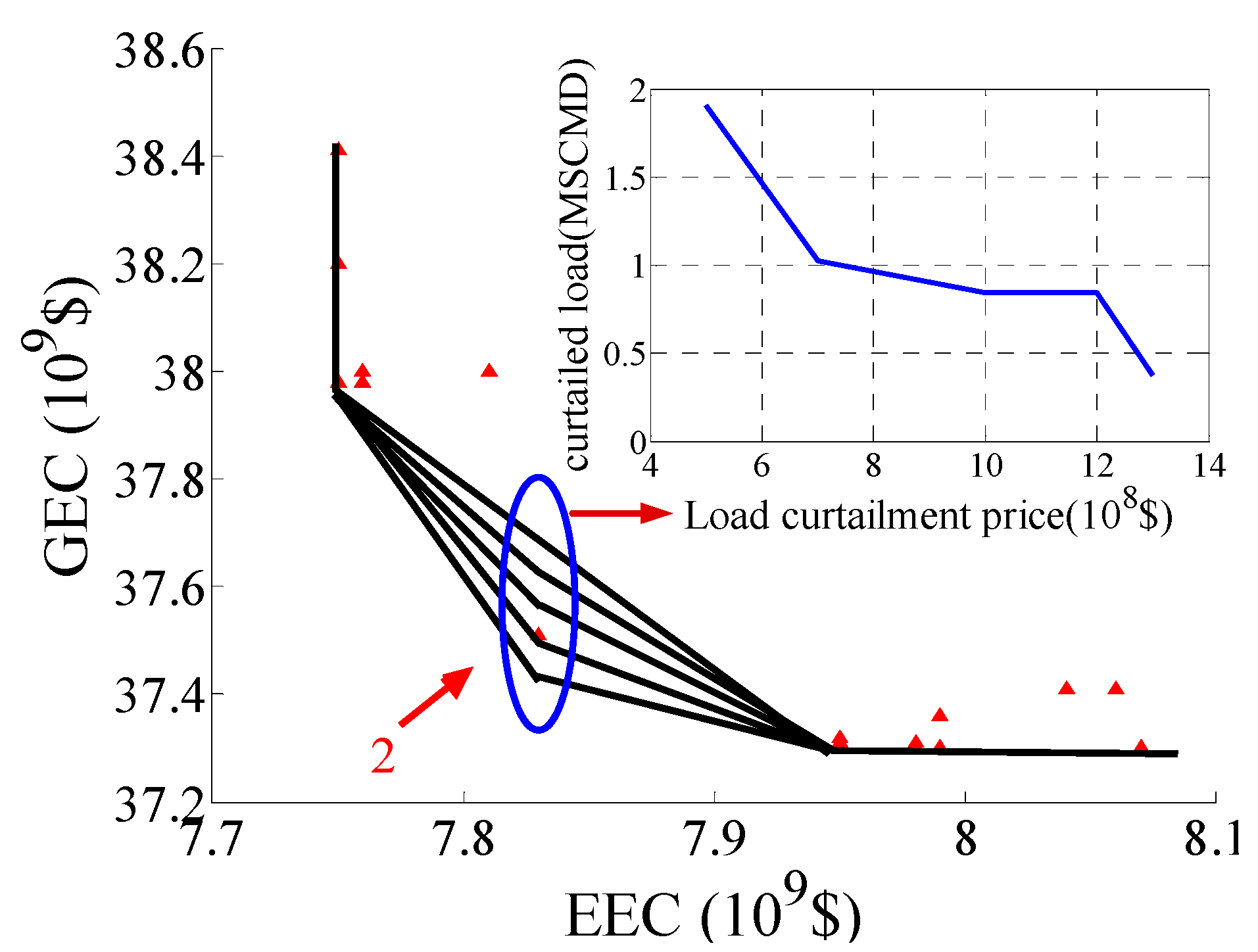
4.3. Risk Analysis
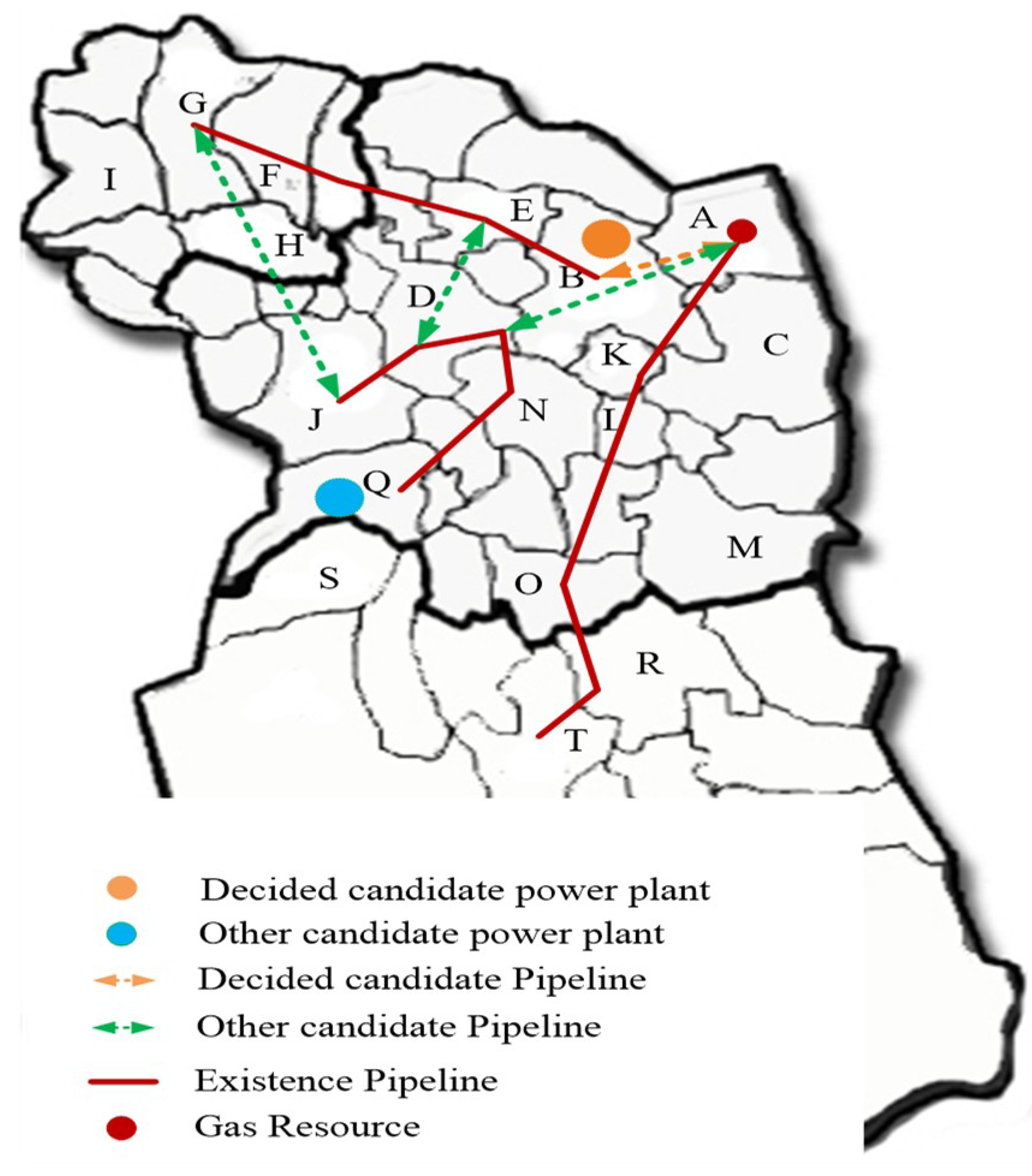
4.4. Comparing the Results with Pareto Optimal Method
4.5. Impact of Investment Budget Restriction
- Scenario 1: $0.8 billion investment budget for a gas network and no limit for an electricity network.
- Scenario 2: $19 million investment budget for a gas network and no limit for an electricity network.
- Scenario 3: $0.1 billion investment budget for an electricity network and no limit for a gas network.
- Scenario 4: $0.3 billion investment budget for an electricity network and no limit for a gas network.
- Scenario 5: $0.8 and $0.3 dollar investment budget for gas and electricity networks, respectively.
5. Conclusions
- ME as a decision maker has freedom to choose a plan in which the total expansion cost of both systems is minimized or one of the network has priority over the other one.
- Using the proposed MADM method privacy of gas and electricity networks was preserved, because the only shared information among gas and electricity system operators was gas consumption of GCGUs.
- Different attributes including expansion cost of gas and electricity network, β_Robustness and MMR of the proposed integrated energy system were considered in the decision-making process.
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| Indices and Sets | |
| Indices of gas nodes | |
| Indices of electricity buses | |
| Index of load period (off, mid and high peak) | |
| Index of days | |
| Index of years | |
| Index of all/gas consuming generation units (GCGUs) | |
| Index of expansion plans | |
| C | Index of attributes |
| Set of nodes/buses of gas/electricity network | |
| Set of transmission lines | |
| Sets of all/active/passive pipelines | |
| Set of daily load periods | |
| Set of all generation units | |
| Y | Set of years |
| D | Set of days |
| K | Set of expansion plans |
| C | Set of decision making attributes |
| Variables | |
| Gas flow of pipeline ij in Million Standard Cubic Meters per Day (MSCMD) | |
| Gas injection at node i in MSCMD | |
| Gas demand of GCGUs at node i | |
| Gas loss at compressor of node i | |
| Curtailed gas demand at load of node i | |
| Gas pressure | |
| Operation cost of electricity system in period t | |
| Fuel consumption of unit gu of bus m | |
| Power flow of line mn | |
| Generation power of unit gu of bus m at period t | |
| Voltage angle of bus m at period t | |
| Curtailed power at load of bus m at time t | |
| Binary variable indicating existence of pipeline ij/transmission line mn/generating unit h of bus m | |
| Priority of plan k in attribute c | |
| Obtained amount for plan k in the case of attribute c | |
| Composite index of plan k | |
| Parameters | |
| Weymouth constant | |
| Pressure ratio in active pipelines | |
| Constant defining compressor gas consumption in active pipeline ij | |
| Gas price at node i in $/MSCM | |
| Gas curtailment price at node i ($/MSCM) | |
| Length of pipeline ij (km) | |
| Diameter of pipeline ij (inch) | |
| Gas demand of non-generation unit loads at node i | |
| Length of transmission line mn | |
| Rated power of unit gu of bus m | |
| Power demand at bus m | |
| Load curtailment price at bus m in $/MW | |
| Fuel price in unit gu of bus m in $/MSCMD | |
| Series admittance of line mn | |
| Base of power | |
| Gross heating value of fuel in unit h | |
| Investment cost of pipeline ij (k$/inch-km)/transmission line mn (k$/km)/generation unit gu of bus m (k$/MW) | |
| Planning period | |
| Investment budget of electricity network | |
| Investment budget of gas network | |
| Duration of period | |
| Interest rate | |
| Preferred amount of attribute c | |
| Non-preferred amount of attribute c |
References
- Jiang, Y.; Xu, J.; Sun, Y.; Wei, C.; Wang, J.; Liao, S.; Ke, D.; Li, X.; Yang, J.; Peng, X. Coordinated operation of gas-electricity integrated distribution system with multi-CCHP and distributed renewable energy sources. Appl. Energy 2018, 211, 237–248. [Google Scholar] [CrossRef]
- Devlin, J.; Li, K.; Higgins, P.; Foley, A. The importance of gas infrastructure in power systems with high wind power penetrations. Appl. Energy 2016, 167, 294–304. [Google Scholar] [CrossRef] [Green Version]
- Annual Energy Outlook. 2008. Available online: https://www.eia.gov/outlooks/aeo/ (accessed on 6 February 2018).
- Nastasi, B.; Basso, G.L. Power-to-gas integration in the transition towards future urban energy systems. Int. J. Hydrog. Energy 2017, 42, 23933–23951. [Google Scholar] [CrossRef]
- Diagoupis, T.D.; Andrianesis, P.E.; Dialynas, E.N. A planning approach for reducing the impact of natural gas network on electricity markets. Appl. Energy 2016, 175, 189–198. [Google Scholar] [CrossRef]
- Khaligh, V.; Buygi, M.O.; Moghaddam, A.A.; Guerrero, J.M. Leader-Follower Approach to Gas-Electricity Expansion Planning Problem. In Proceedings of the IEEE 18th International Conference on Environment and Electrical Engineering and 2nd Industrial and Commercial Power Systems Europe (EEEIC 2018), Palermo, Italy, 12–15 June 2018. [Google Scholar]
- Greening, L.A.; Bernow, S. Design of coordinated energy and environmental policies: Use of multi-criteria decision-making. Energy Policy 2004, 32, 721–735. [Google Scholar] [CrossRef]
- Wen, Y.; Qu, X.; Li, W.; Liu, X.; Ye, X. Synergistic operation of electricity and natural gas networks via admm. IEEE Trans. Smart Grid 2017. [Google Scholar] [CrossRef]
- Zhou, X.; Guo, C.; Wang, Y.; Li, W. Optimal expansion co-planning of reconfigurable electricity and natural gas distribution systems incorporating energy hubs. Energies 2017, 10, 124. [Google Scholar] [CrossRef]
- Unsihuay-Vila, C.; Marangon-Lima, J.W.; de Souza, A.Z.; Perez-Arriaga, I.J.; Balestrassi, P.P. A model to long-term, multiarea, multistage, and integrated expansion planning of electricity and natural gas systems. IEEE Trans. Power Syst. 2010, 25, 1154–1168. [Google Scholar] [CrossRef]
- Saldarriaga, C.A.; Hincapié, R.A.; Salazar, H. A holistic approach for planning natural gas and electricity distribution networks. IEEE Trans. Power Syst. 2013, 28, 4052–4063. [Google Scholar] [CrossRef]
- Chaudry, M.; Jenkins, N.; Qadrdan, M.; Wu, J. Combined gas and electricity network expansion planning. Appl. Energy 2014, 113, 1171–1187. [Google Scholar] [CrossRef]
- Qiu, J.; Dong, Z.Y.; Zhao, J.H.; Xu, Y.; Zheng, Y.; Li, C.; Wong, K.P. Multi-stage flexible expansion co-planning under uncertainties in a combined electricity and gas market. IEEE Trans. Power Syst. 2015, 30, 2119–2129. [Google Scholar] [CrossRef]
- Barati, F.; Seifi, H.; Sepasian, M.S.; Nateghi, A.; Shafie-khah, M.; Catalao, J.P.S. Multi-period integrated framework of generation, transmission, and natural gas grid expansion planning for large-scale systems. IEEE Trans. Power Syst. 2015, 30, 2527–2537. [Google Scholar] [CrossRef]
- Qiu, J.; Dong, Z.Y.; Zhao, J.H.; Meng, K.; Zheng, Y.; Hill, D.J. Low carbon oriented expansion planning of integrated gas and power systems. IEEE Trans. Power Syst. 2015, 30, 1035–1046. [Google Scholar] [CrossRef]
- Shao, C.; Shahidehpour, M.; Wang, X.; Wang, X.; Wang, B. Integrated planning of electricity and natural gas transportation systems for enhancing the power grid resilience. IEEE Trans. Power Syst. 2017, 32, 4418–4429. [Google Scholar] [CrossRef]
- Qiu, J.; Yang, H.; Dong, Z.Y.; Zhao, J.H.; Meng, K.; Luo, F.J.; Wong, K.P. A linear programming approach to expansion co-planning in gas and electricity markets. IEEE Trans. Power Syst. 2016, 31, 3594–3606. [Google Scholar] [CrossRef]
- Zhang, X.; Shahidehpour, M.; Alabdulwahab, A.S.; Abusorrah, A. Security-constrained co-optimization planning of electricity and natural gas transportation infrastructures. IEEE Trans. Power Syst. 2015, 30, 2984–2993. [Google Scholar] [CrossRef]
- Zhao, B.; Conejo, A.J.; Sioshansi, R. Coordinated Expansion Planning of Natural Gas and Electric Power Systems. IEEE Trans. Power Syst. 2017, 33, 3064–3075. [Google Scholar] [CrossRef]
- Ding, T.; Hu, Y.; Bie, Z. Multi-Stage Stochastic Programming with Nonanticipativity Constraints for Expansion of Combined Power and Natural Gas Systems. IEEE Trans. Power Syst. 2018, 33, 317–328. [Google Scholar] [CrossRef]
- Odetayo, B.; MacCormack, J.; Rosehart, W.D.; Zareipour, H. A sequential planning approach for Distributed generation and natural gas networks. Energy 2017, 127, 428–437. [Google Scholar] [CrossRef]
- Zeng, Q.; Zhang, B.; Fang, J.; Chen, Z. A bi-level programming for multistage co-expansion planning of the integrated gas and electricity system. Appl. Energy 2017, 200, 192–203. [Google Scholar] [CrossRef]
- He, C.; Wu, L.; Liu, T.; Bie, Z. Robust co-optimization planning of interdependent electricity and natural gas systems with a joint N-1 and probabilistic reliability criterion. IEEE Trans. Power Syst. 2018, 33, 2140–2154. [Google Scholar] [CrossRef]
- Nunes, J.B.; Mahmoudi, N.; Saha, T.K.; Chattopadhyay, D. A stochastic integrated planning of electricity and natural gas networks for Queensland, Australia considering high renewable penetration. Energy 2018, 153, 539–553. [Google Scholar] [CrossRef]
- Zhang, Y.; Hu, Y.; Ma, J.; Bie, Z. A Mixed-integer Linear Programming Approach to Security-constrained Co-optimization Expansion Planning of Natural Gas and Electricity Transmission Systems. IEEE Trans. Power Syst. 2018. [Google Scholar] [CrossRef]
- Zhang, X.; Che, L.; Shahidehpour, M.; Alabdulwahab, A.S.; Abusorrah, A. Reliability-based optimal planning of electricity and natural gas interconnections for multiple energy hubs. IEEE Trans. Smart Grid 2015, 1–10. [Google Scholar] [CrossRef]
- Bent, R.; Blumsack, S.; Van Hentenryck, P.R.; Sanchez, C.B.; Shahriari, M. Joint Electricity and Natural Gas Transmission Planning with Endogenous Market Feedbacks. IEEE Trans. Power Syst. 2018. [Google Scholar] [CrossRef]
- Odetayo, B.; Kazemi, M.; MacCormack, J.; Rosehart, W.; Zareipour, H.; Seifi, A.R. A Chance Constrained Programming Approach to the Integrated Planning of Electric Power Generation, Natural Gas Network and Storage. IEEE Trans. Power Syst. 2018. [Google Scholar] [CrossRef]
- Shaaban, M.; Scheffran, J.; Böhner, J.; Elsobki, M.S. Sustainability assessment of electricity generation technologies in Egypt using multi-criteria decision analysis. Energies 2018, 11, 1117. [Google Scholar] [CrossRef]
- Pambour, K.A.; Sopgwi, R.T.; Hodge, B.M.; Brancucci, C. The value of day-ahead coordination of power and natural gas network operations. Energies 2018, 11, 1628. [Google Scholar] [CrossRef]
- Wang, D.X.; Qiu, J.; Meng, K.; Gao, X.D.; Dong, Z.Y. Coordinated expansion co-planning of integrated gas and power systems. J. Mod. Power Syst. Clean Energy 2017, 5, 314–325. [Google Scholar] [CrossRef]
- Li, G.; Zhang, R.; Jiang, T.; Chen, H.; Bai, L.; Li, X. Security-constrained bi-level economic dispatch model for integrated natural gas and electricity systems considering wind power and power-to-gas process. Appl. Energy 2017, 194, 696–704. [Google Scholar] [CrossRef]
- Menon, E.S. Gas Pipeline Hydraulics; CRC Press: Boca Raton, FL, USA, 2005. [Google Scholar]
- Tabkhi, F.; Pibouleau, L.; Azzaro-Pantel, C.; Domenech, S. Total cost minimization of a high-pressure natural gas network. J. Energy Resour. Technol. 2009, 131, 043002. [Google Scholar] [CrossRef]
- Ojeda-Esteybar, D.M.; Rubio-Barros, R.G.; Añó, O. Vargas, Integration of electricity and natural gas systems-identification of coordinating parameters. In Proceedings of the 2014 IEEE PES Transmission & Distribution Conference and Exposition-Latin America (PES T&D-LA), Medellin, Colombia, 10–13 September 2014. [Google Scholar]
- Osiadacz, A. Simulation and Analysis of Gas Networks; Department of Energy Office of Scientific and Technical Information: Washington, DC, USA, 1987.
- Aissi, H.; Bazgan, C.; Vanderpooten, D. Min–max and min–max regret versions of combinatorial optimization problems: A survey. Eur. J. Oper. Res. 2009, 197, 427–438. [Google Scholar] [CrossRef] [Green Version]
- Saaty, T.L. A scaling method for priorities in hierarchical structures. J. Math. Psychol. 1977, 15, 234–281. [Google Scholar] [CrossRef]
- Saaty, R.W. The analytic hierarchy process—What it is and how it is used. Math. Model. 1987, 9, 161–176. [Google Scholar] [CrossRef]
- Rosenthal, R.E. GAMS—A User’s Guide; CRC Press: Boca Raton, FL, USA, 2004. [Google Scholar]
- Bonami, P.; Lee, J. BONMIN user’s manual. Numer. Math. 2007, 4, 1–32. [Google Scholar]
- Seyedi, H.; Sanaye-Pasand, M. New centralised adaptive load-shedding algorithms to mitigate power system blackouts. IET Gener. Transm. Distrib. 2009, 3, 99–114. [Google Scholar] [CrossRef]




| Scale | Degree of Priority |
|---|---|
| 1 | Equal importance |
| 3 | Weak |
| 5 | Strong |
| 7 | Very strong |
| 9 | Extreme importance |
| 2, 4, 6, 8 | Intermediate values |
| Pipelines | Cost (k$/inch-km) | Trans. Lines | Cost (k$/km) | Gen. | Cost (k$/MW) |
|---|---|---|---|---|---|
| A-B1 | 40 | S-Q | 240 | C | 900 |
| A-K | 40 | N-C | 240 | S | 900 |
| A-D | 60 | B-C | 360 | Q | 900 |
| E-D | 60 | N-D | 480 | F | 900 |
| G-J | 60 | F-H | 480 | I | 900 |
| R-T | 480 | T | 1170 | ||
| R-S | 480 | B | 1440 | ||
| F-D | 480 | R | 1080 | ||
| Plan | Transmission Candidates | Generation Candidates | Pipeline Candidates |
|---|---|---|---|
| 1 | B-C, N-D | I, B2 | A-B1, A-D |
| 2 | F-H | I, S | A-B1 |
| 3 | F-H, B-C | Q, B2 | A-B1, A-D |
| 4 | F-H | C, S, B2 | A-B1 |
| 5 | F-H, B-C | C, R, B2 | A-B1 |
| 6 | F-H, B-C | C, S, B2 | A-B1 |
| 7 | - | I, B1, S | A-B1, A-D |
| 8 | - | I, B1, Q, S | A-B1, A-D |
| 9 | F-H | F, B1, C, S | A-B1 |
| 10 | F-H | F, B1, R, S | A-B1 |
| 11 | F-H | F, B1, C, S | A-B1 |
| 12 | F-H | B1, B3, C, S | A-B1 |
| 13 | F-H | F, B1, S, T | A-B1 |
| 14 | F-H | S, B2 | A-B1 |
| 15 | F-H, B-C, S-Q | B3, S, B2 | A-B1 |
| 16 | F-H | F, B1, S | A-B1 |
| 17 | F-H | F, S, B2 | A-B1 |
| 18 | F-H | F, T, B2 | A-B1 |
| 19 | F-H, B-C | F, S, B2 | A-B1 |
| 20 | F-H, N-D | F, S, B2 | A-B1 |
| 21 | F-H, N-C | F, S, B2 | A-B1 |
| 22 | F-H, S-Q | C, S, B2 | A-B1 |
| 23 | F-H, B-C | Q, B2 | E-D, A-B1 |
| 24 | F-H, B-C | Q, B2 | G-J, A-B1 |
| Plan | Transmission Candidates | Generation Candidates | Pipeline Candidates |
|---|---|---|---|
| 1 | F-H | I, C, S | A-B1 |
| 2 | - | I, C | A-B1 |
| 3 | F-H | F, C | A-B1 |
| 4 | F-H | C, B1 | A-B1 |
| 5 | F-H | C, B3 | A-B1 |
| 6 | F-H | C, S | A-B1 |
| 7 | F-H, B-C, S-Q | F, S, Q | A-B1 |
| 8 | - | C, R | A-B1 |
| 9 | F-H | C, B2 | A-B1 |
| 10 | F-H | R | A-B1 |
| 11 | F-H | S, R, B2 | A-B1 |
| Attribute | EEC | GEC | MMR | β_R | ||||
|---|---|---|---|---|---|---|---|---|
| EEC | 1 I | 1 II | 1 | 9 | 3 | 9 | 3 | 9 |
| 1 III | 1 IV | 0.11 | 1 | 3 | 0.11 | 3 | 3 | |
| GEC | 1 | 0.11 | 1 | 1 | 3 | 3 | 3 | 3 |
| 9 | 1 | 1 | 1 | 9 | 0.11 | 9 | 3 | |
| MMR | 0.33 | 0.11 | 0.33 | 0.33 | 1 | 1 | 1 | 1 |
| 0.33 | 9 | 0.11 | 9 | 1 | 1 | 1 | 9 | |
| β_R | 0.33 | 0.11 | 0.33 | 0.33 | 1 | 1 | 1 | 1 |
| 0.33 | 0.33 | 0.11 | 0.33 | 1 | 0.11 | 1 | 1 | |
| Plans | Case 1 | Case 2 | Case 3 | Case 4 |
|---|---|---|---|---|
| 1 | 0.054443 | 0.083611 | 0.027955 | 0.025225 |
| 2 | 0.064093 | 0.067421 | 0.047787 | 0.057411 |
| 3 | 0.058764 | 0.092075 | 0.029584 | 0.026467 |
| 4 | 0.039715 | 0.028995 | 0.048416 | 0.049109 |
| 5 | 0.038042 | 0.027150 | 0.048119 | 0.047833 |
| 6 | 0.039715 | 0.028995 | 0.048416 | 0.049109 |
| 7 | 0.054079 | 0.083444 | 0.027607 | 0.024561 |
| 8 | 0.045014 | 0.065687 | 0.024190 | 0.021956 |
| 9 | 0.035424 | 0.024940 | 0.045173 | 0.047016 |
| 10 | 0.031251 | 0.019238 | 0.043081 | 0.044623 |
| 11 | 0.035424 | 0.02494 | 0.045173 | 0.047016 |
| 12 | 0.030384 | 0.018038 | 0.042713 | 0.044343 |
| 13 | 0.031251 | 0.019238 | 0.043081 | 0.044623 |
| 14 | 0.039715 | 0.028995 | 0.048416 | 0.049109 |
| 15 | 0.031455 | 0.018063 | 0.045325 | 0.044642 |
| 16 | 0.037731 | 0.027033 | 0.047511 | 0.047744 |
| 17 | 0.040026 | 0.029112 | 0.049024 | 0.049198 |
| 18 | 0.036608 | 0.025387 | 0.047494 | 0.047356 |
| 19 | 0.040026 | 0.029112 | 0.049024 | 0.049198 |
| 20 | 0.040026 | 0.029112 | 0.049024 | 0.049198 |
| 21 | 0.038042 | 0.027150 | 0.048119 | 0.047833 |
| 22 | 0.036608 | 0.025387 | 0.047494 | 0.047356 |
| 23 | 0.052601 | 0.089148 | 0.024929 | 0.020871 |
| 24 | 0.049563 | 0.087728 | 0.022344 | 0.018201 |
| Point | Plan | Transmission Candidates | Generation Candidates | Pipeline Candidates |
|---|---|---|---|---|
| 1 | 3 | F-H, B-C | Q, B2 | A-B1, A-D |
| 23 | F-H, B-C | Q, B2 | E-D, A-B1 | |
| 24 | F-H, B-C | Q, B2 | G-J, A-B1 | |
| 2 | 2 | F-H | I, S | A-B1 |
| 3 | 4 | F-H | C, S, B2 | A-B1 |
| 5 | F-H, B-C | C, R, B2 | A-B1 | |
| 6 | F-H, B-C | C, S, B2 | A-B1 | |
| 14 | F-H | S, B2 | A-B1 | |
| 17 | F-H | F, S, B2 | A-B1 | |
| 19 | F-H, B-C | F, S, B2 | A-B1 | |
| 20 | F-H, N-D | F, S, B2 | A-B1 | |
| 21 | F-H, N-C | F, S, B2 | A-B1 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khaligh, V.; Oloomi Buygi, M.; Anvari-Moghaddam, A.; M. Guerrero, J. A Multi-Attribute Expansion Planning Model for Integrated Gas–Electricity System. Energies 2018, 11, 2573. https://doi.org/10.3390/en11102573
Khaligh V, Oloomi Buygi M, Anvari-Moghaddam A, M. Guerrero J. A Multi-Attribute Expansion Planning Model for Integrated Gas–Electricity System. Energies. 2018; 11(10):2573. https://doi.org/10.3390/en11102573
Chicago/Turabian StyleKhaligh, Vahid, Majid Oloomi Buygi, Amjad Anvari-Moghaddam, and Josep M. Guerrero. 2018. "A Multi-Attribute Expansion Planning Model for Integrated Gas–Electricity System" Energies 11, no. 10: 2573. https://doi.org/10.3390/en11102573
APA StyleKhaligh, V., Oloomi Buygi, M., Anvari-Moghaddam, A., & M. Guerrero, J. (2018). A Multi-Attribute Expansion Planning Model for Integrated Gas–Electricity System. Energies, 11(10), 2573. https://doi.org/10.3390/en11102573







