Analysis of Pressure Rise in a Closed Container Due to Internal Arcing
Abstract
:1. Introduction
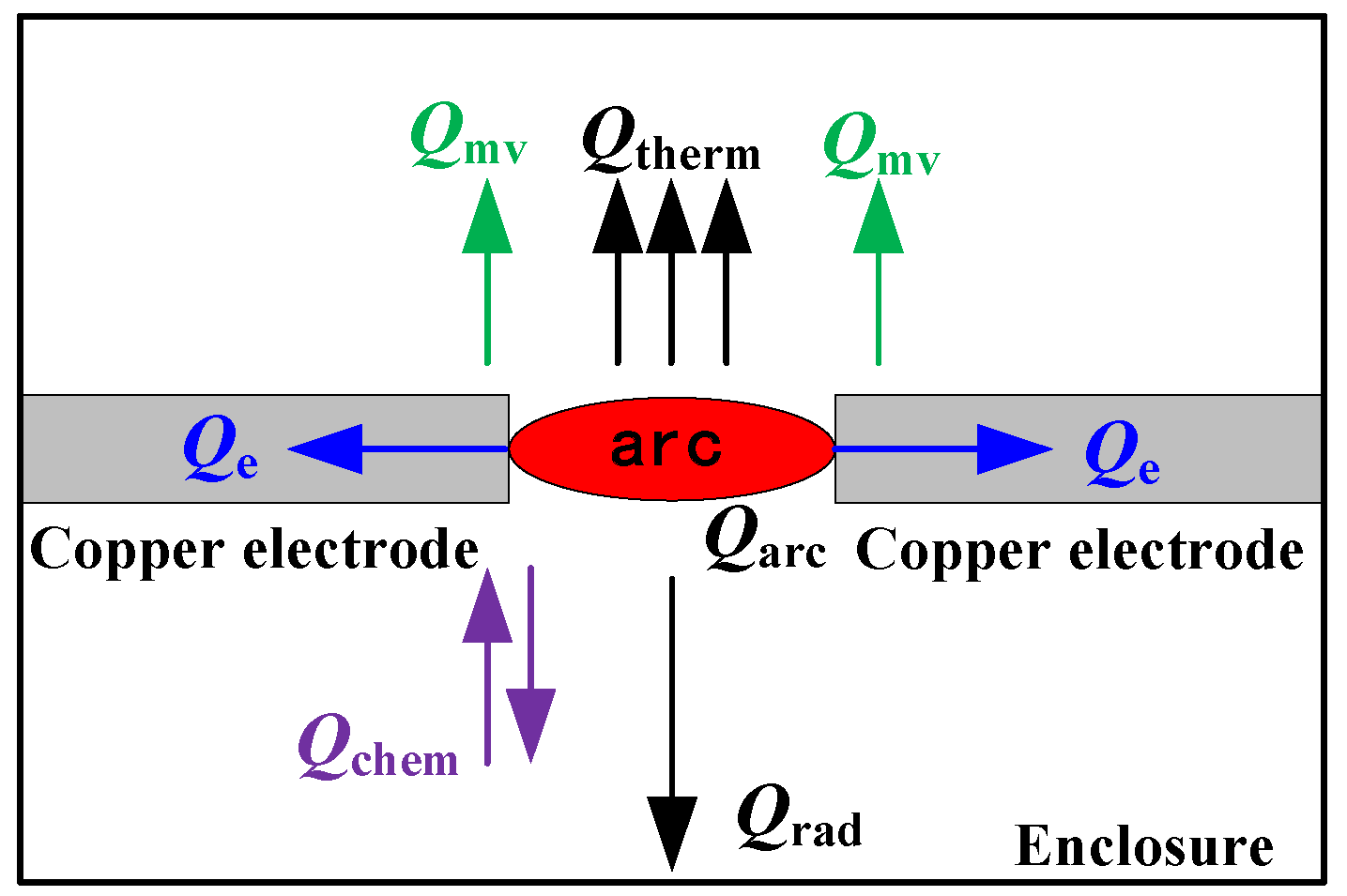
2. Energy Balance of Arc Fault in an MV Switchgear
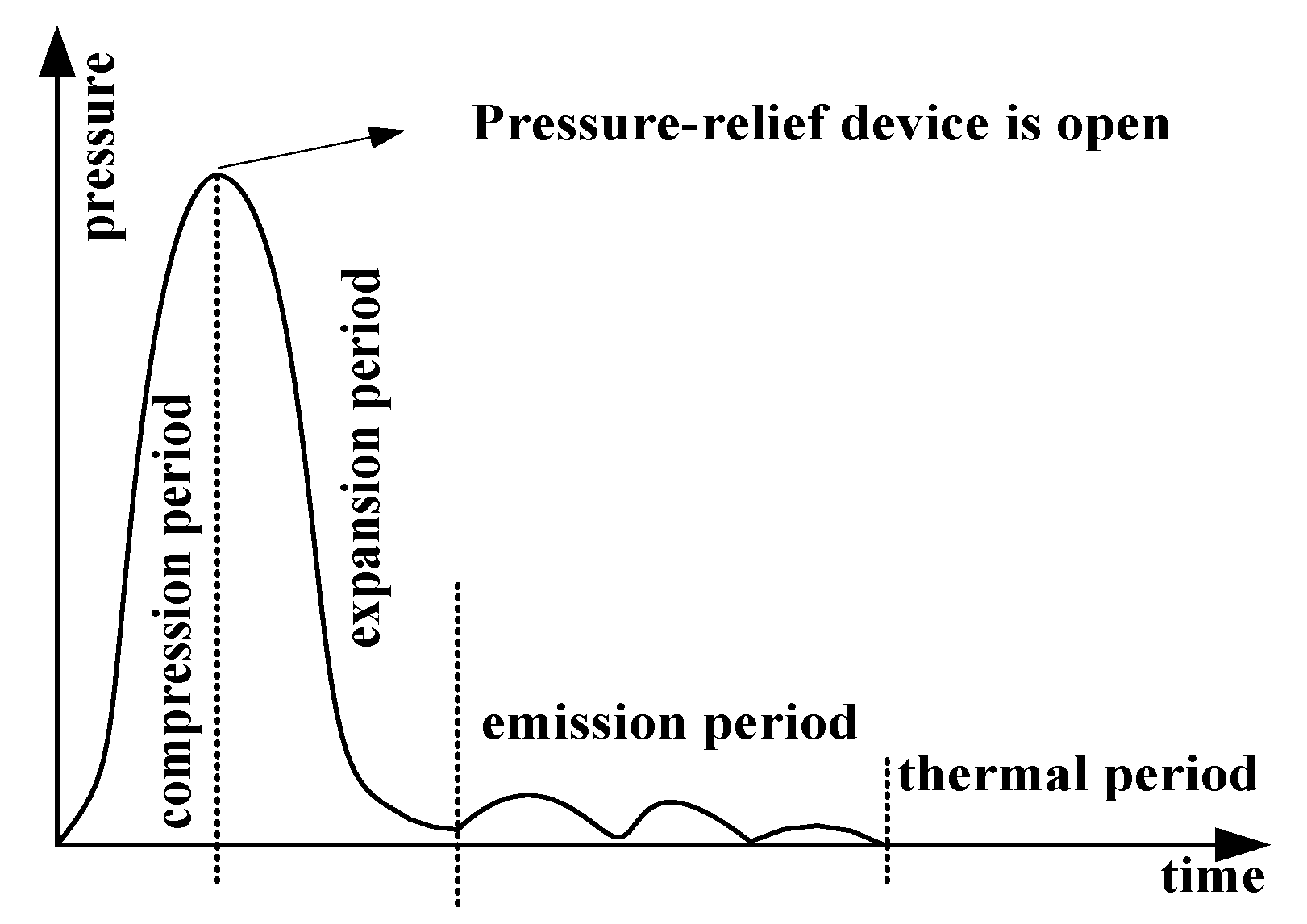
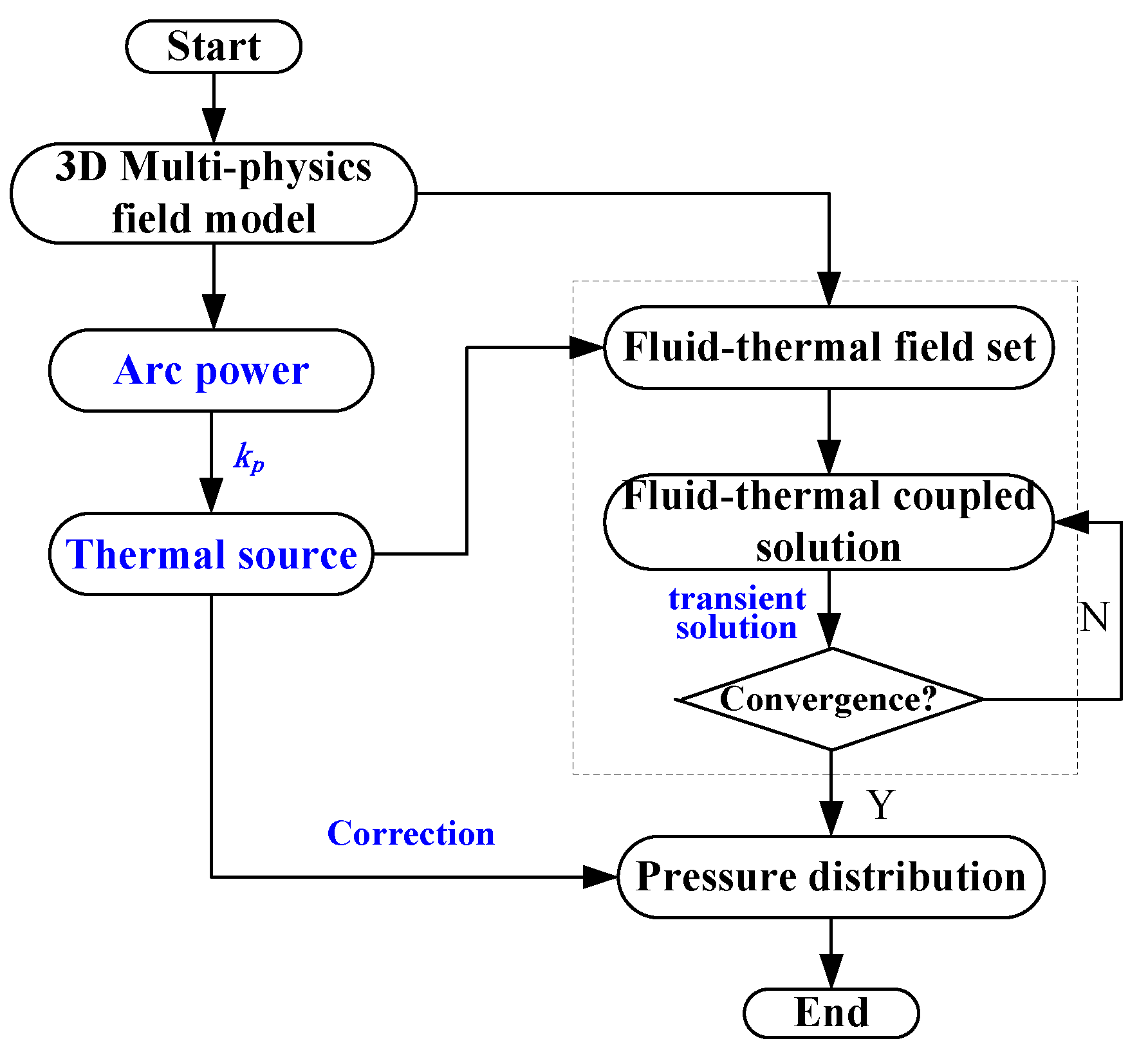
3. Calculation Method of the Pressure Rise Caused by Internal Arcing in the Switchgear
3.1. Calculation Process of Pressure Rise
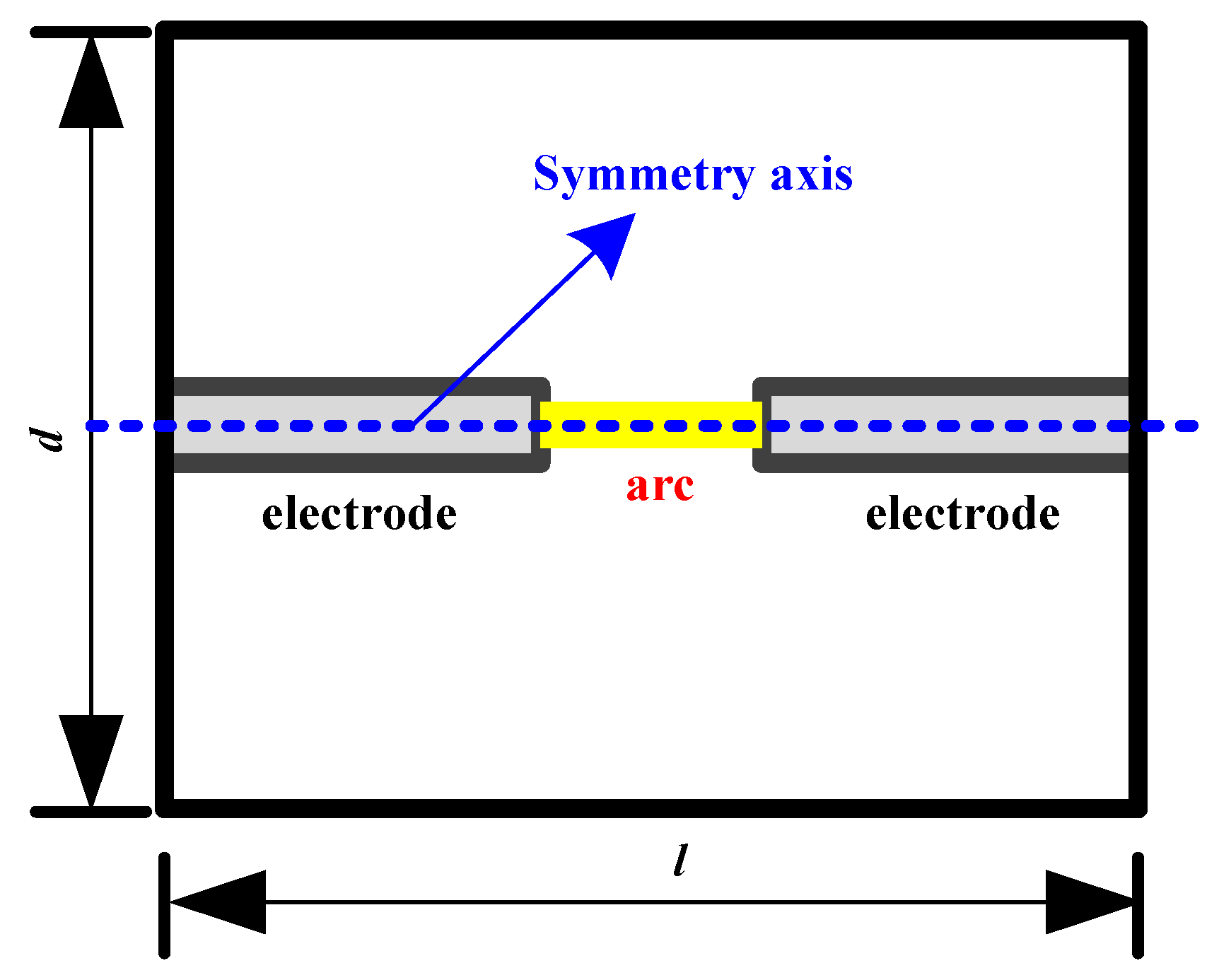
3.2. CFD Calculation Method
3.2.1. Governing Equations
3.2.2. Energy Input and Boundary Conditions
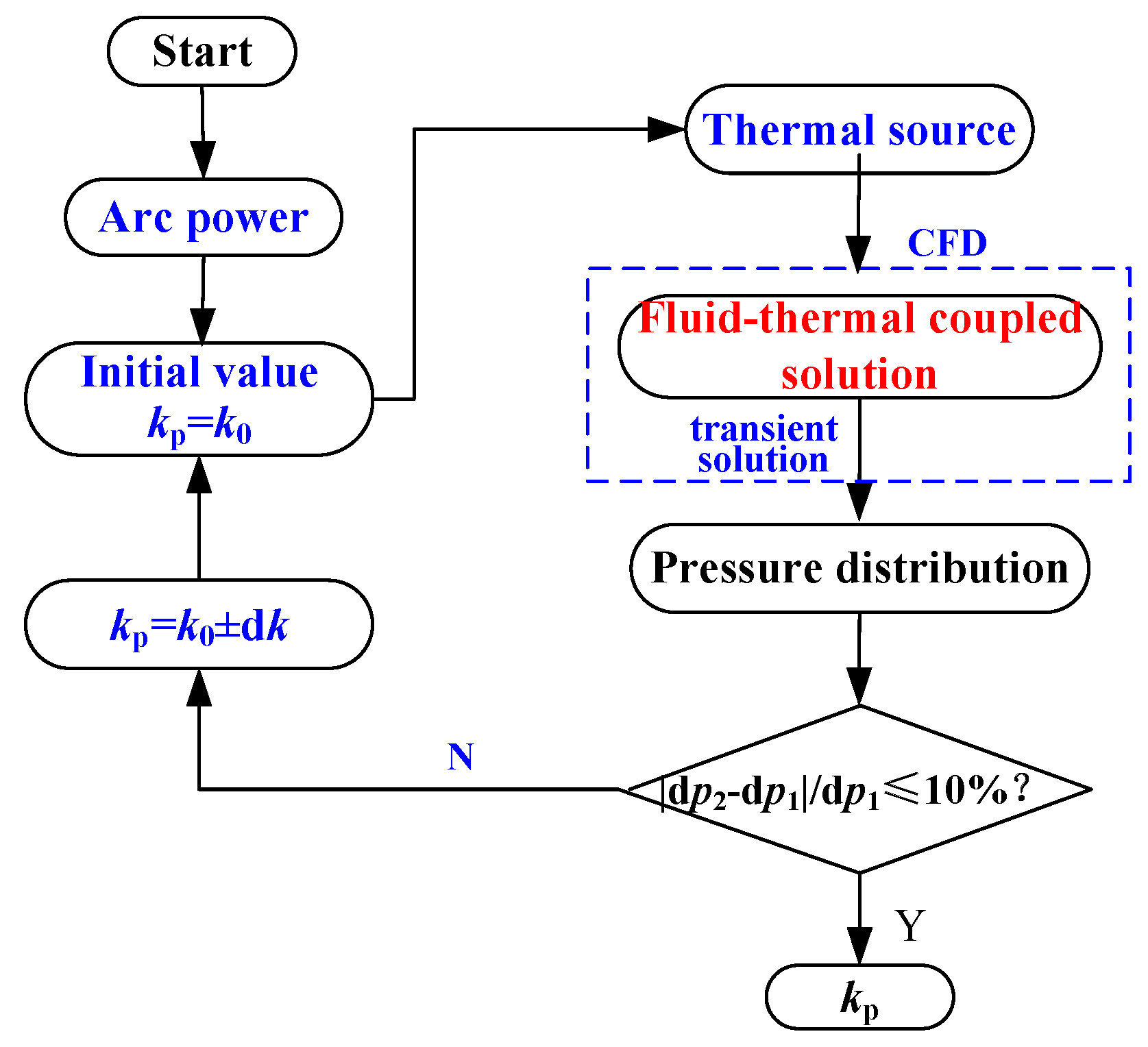
3.3. Determination of the “kp-factor”
3.4. Influence of the Arc Volume on the Calculation of Pressure Rise
4. Internal Arc Fault Experiment and Pressure Rise Calculation in a Closed Container
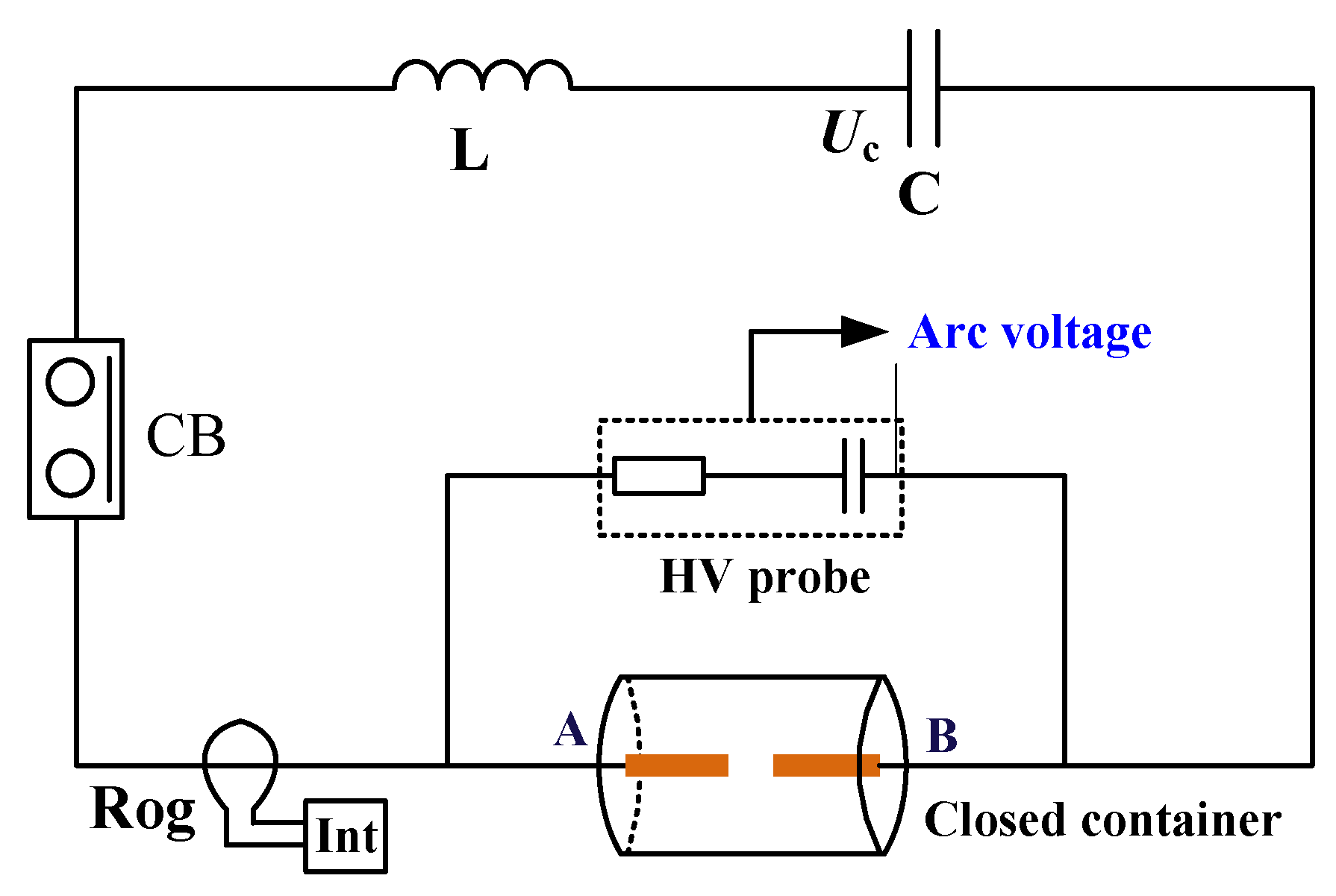
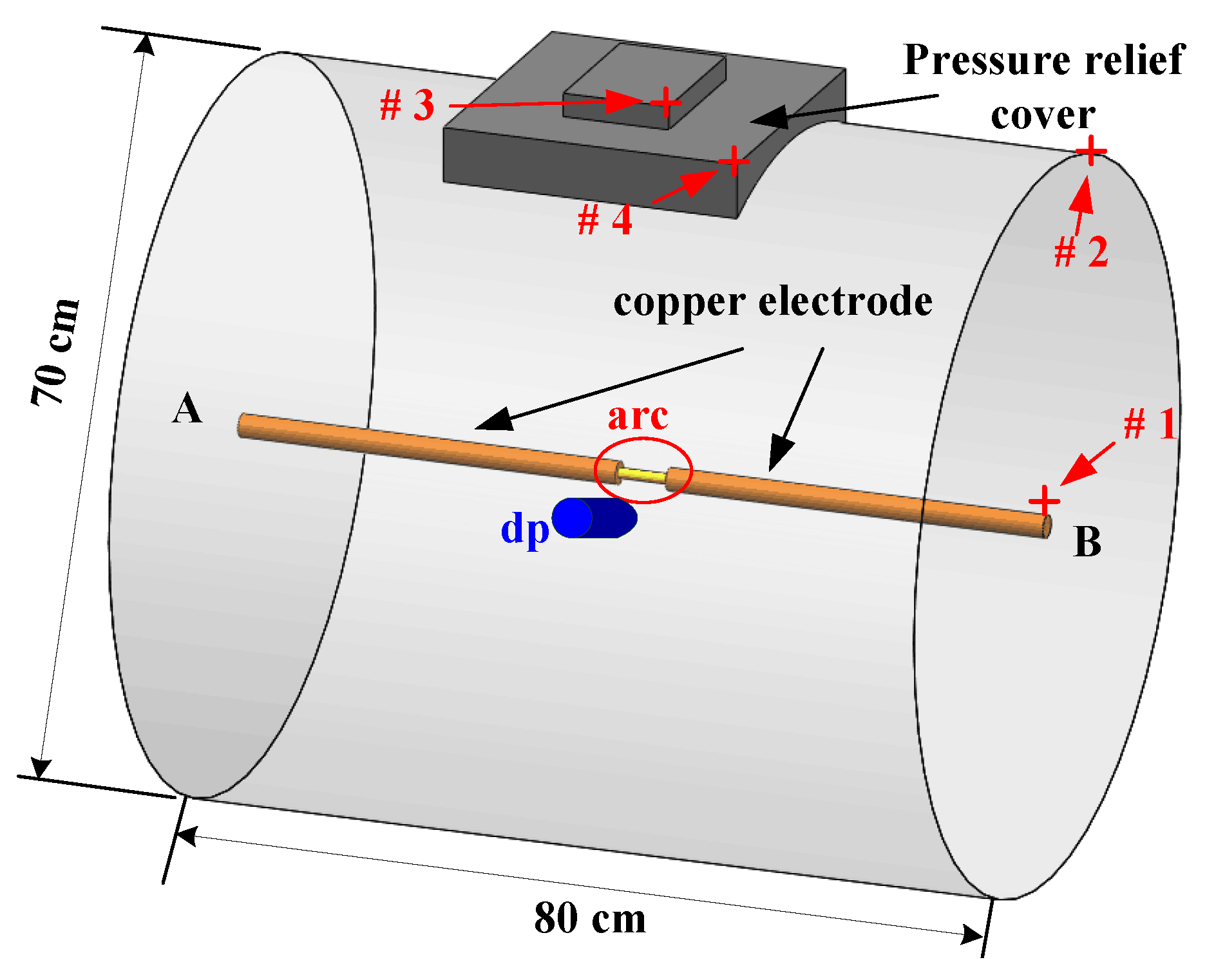
4.1. Expermental Method and Arrangement
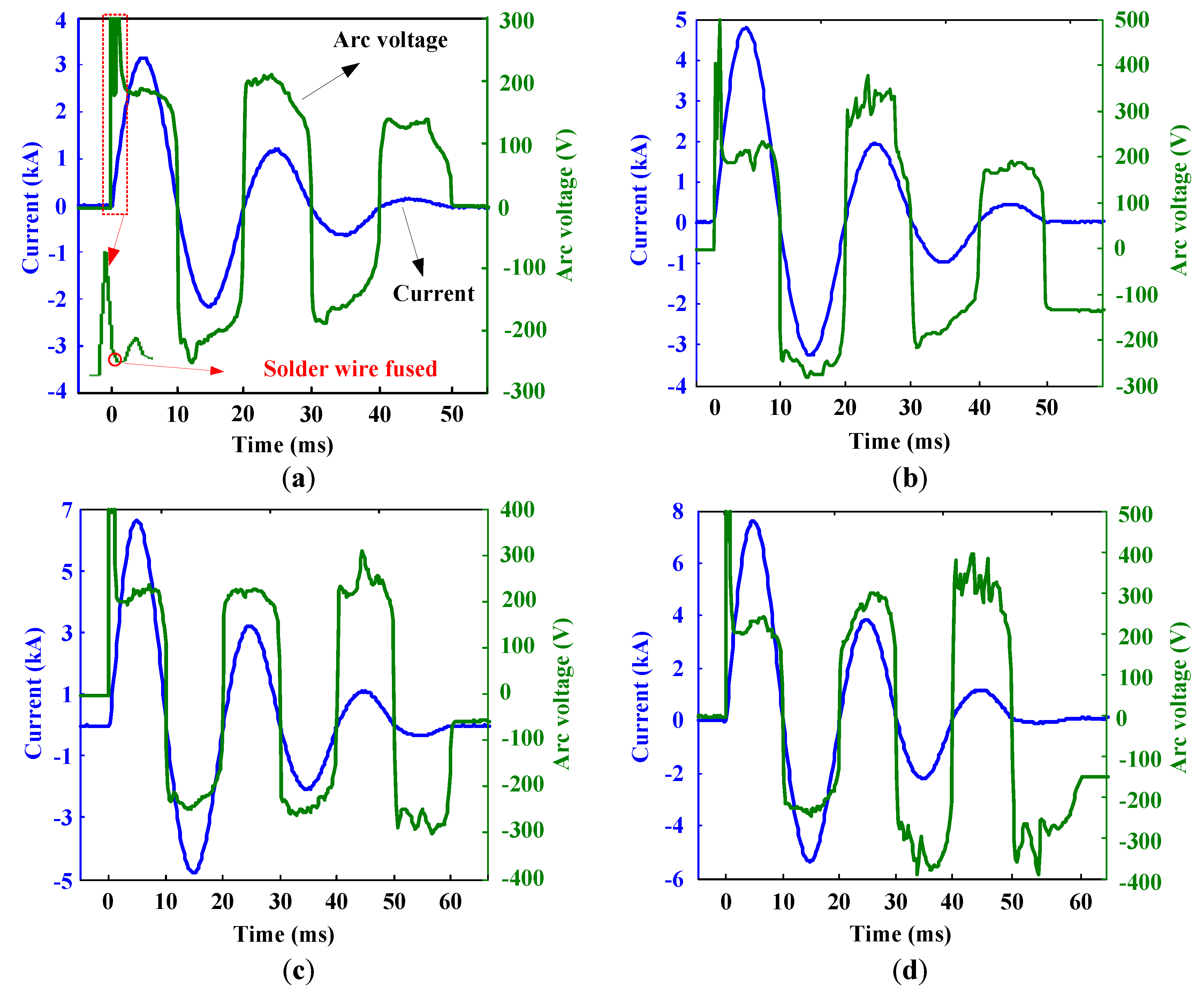
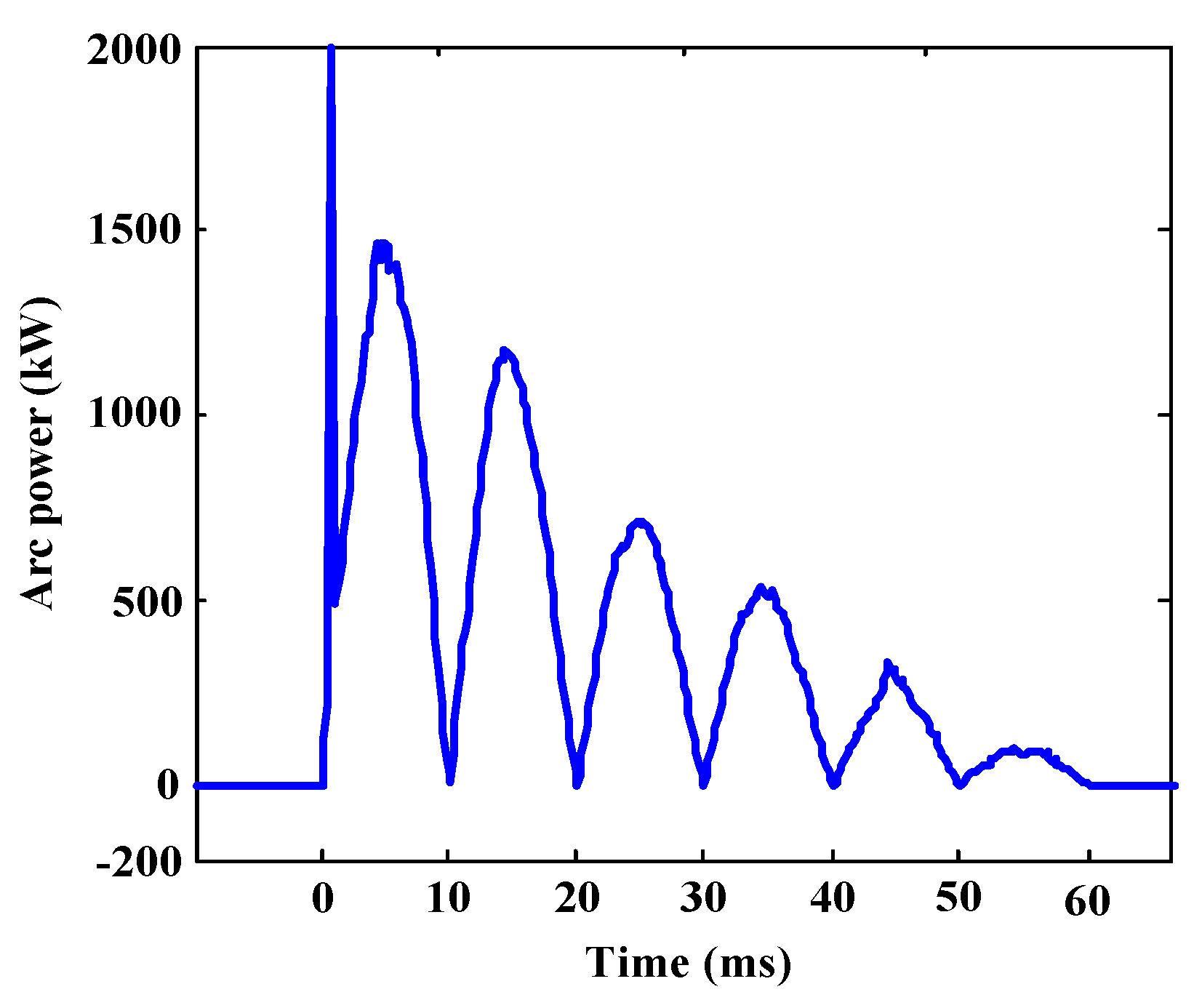
4.2. Change of the Arc Voltage with Current
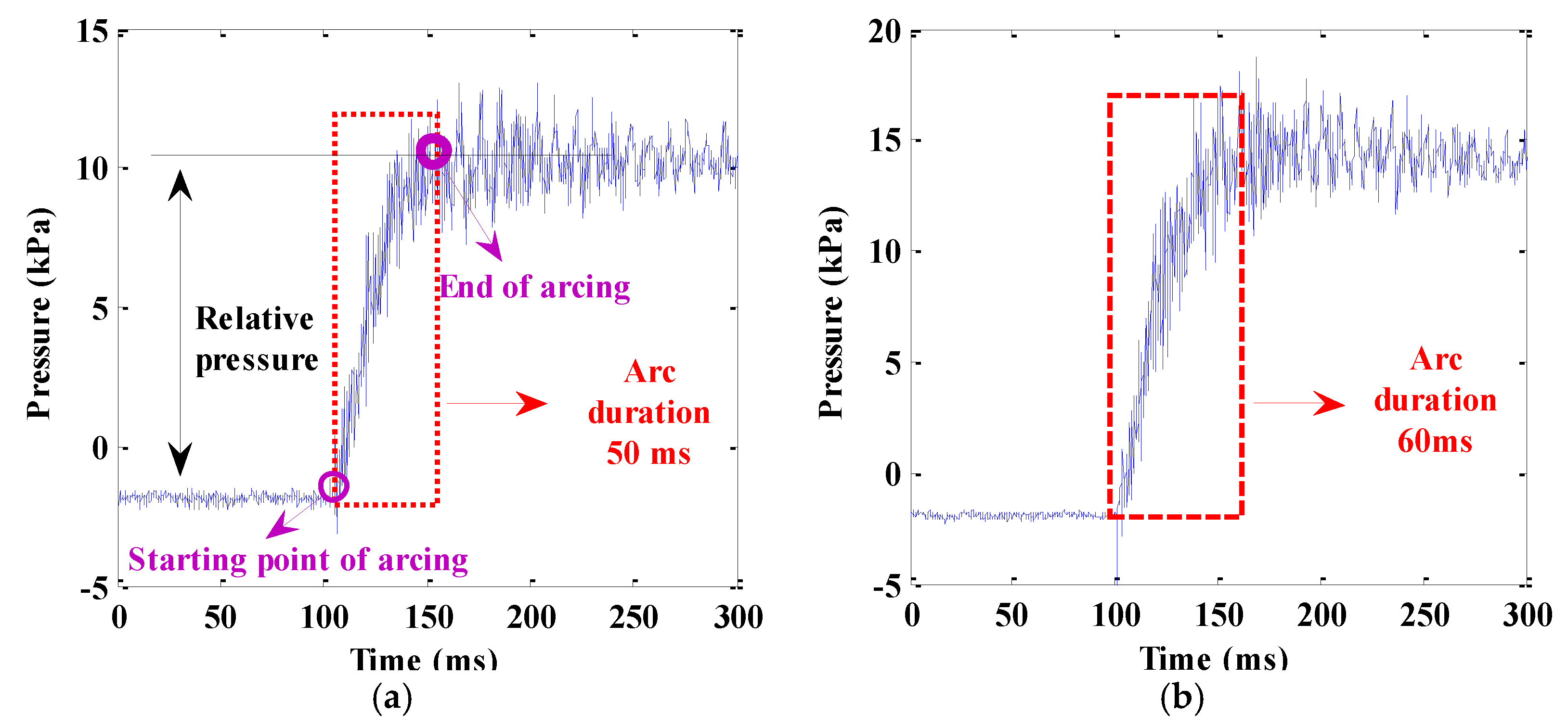
4.3. Pressure Rise in the Container
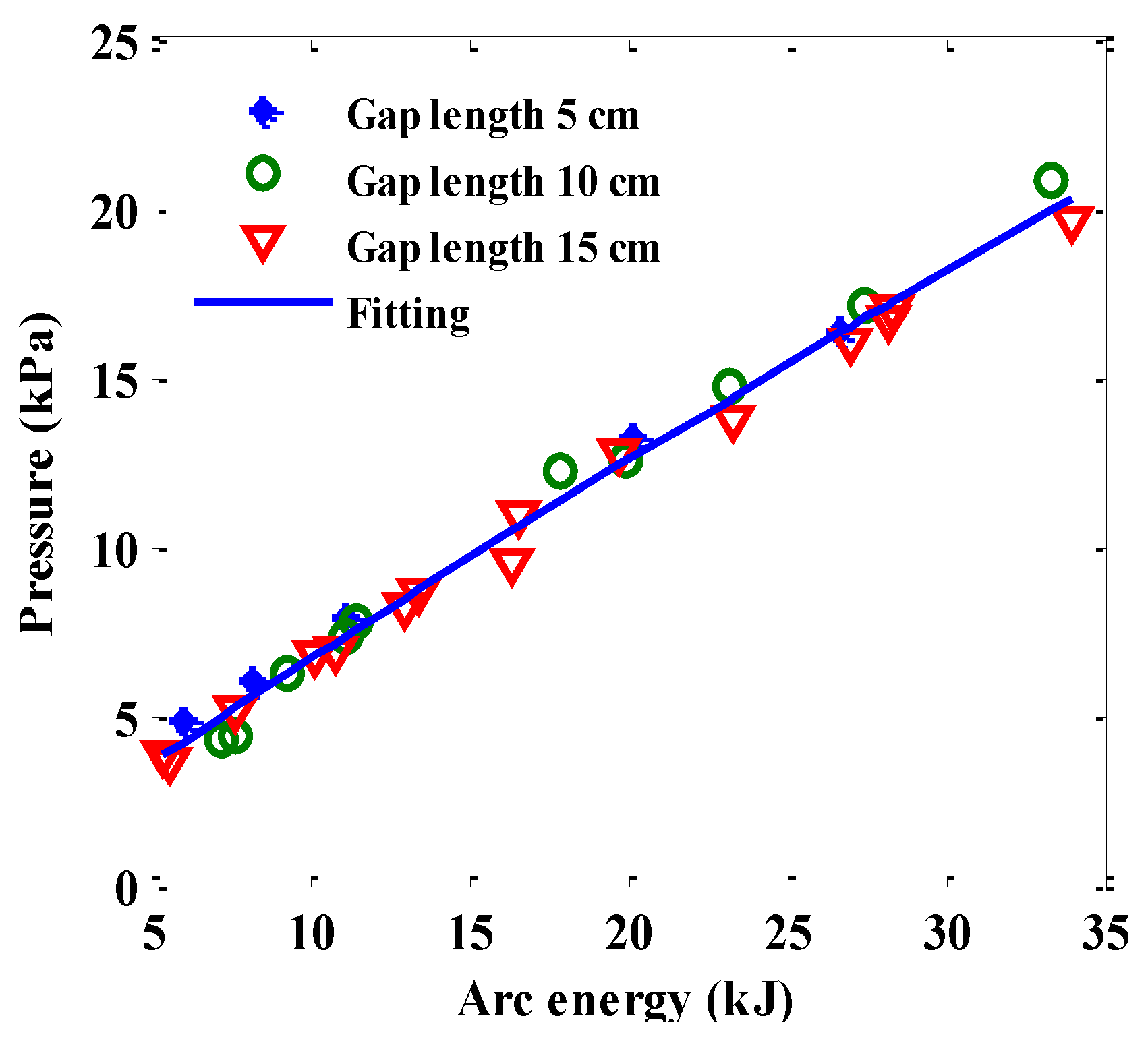
4.4. Relationship Between Pressure Rise and Arc Energy
4.5. Simulation Results Verification and Pressure Distribution in the Container
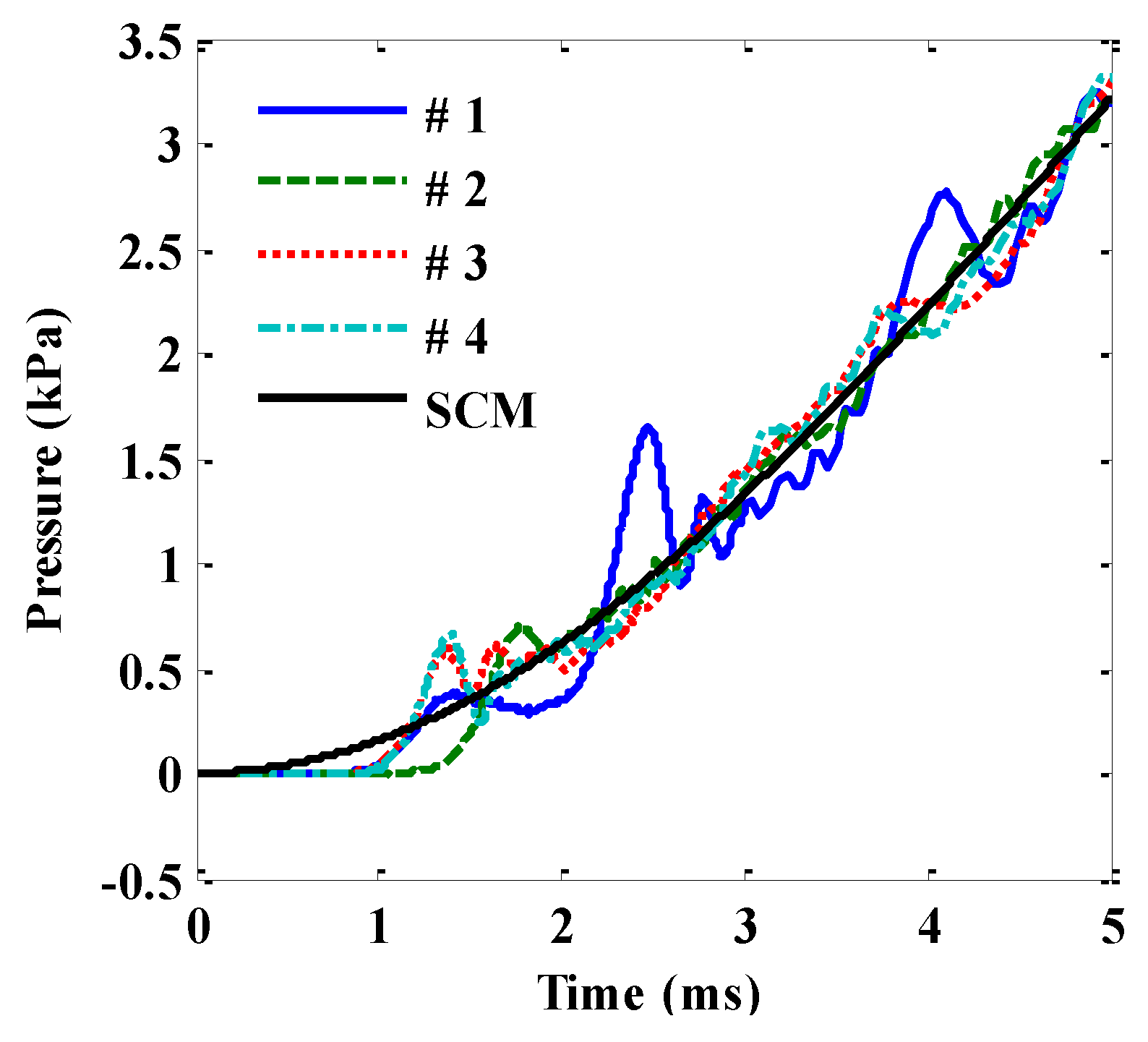
4.5.1. Verification of the Simulation Method
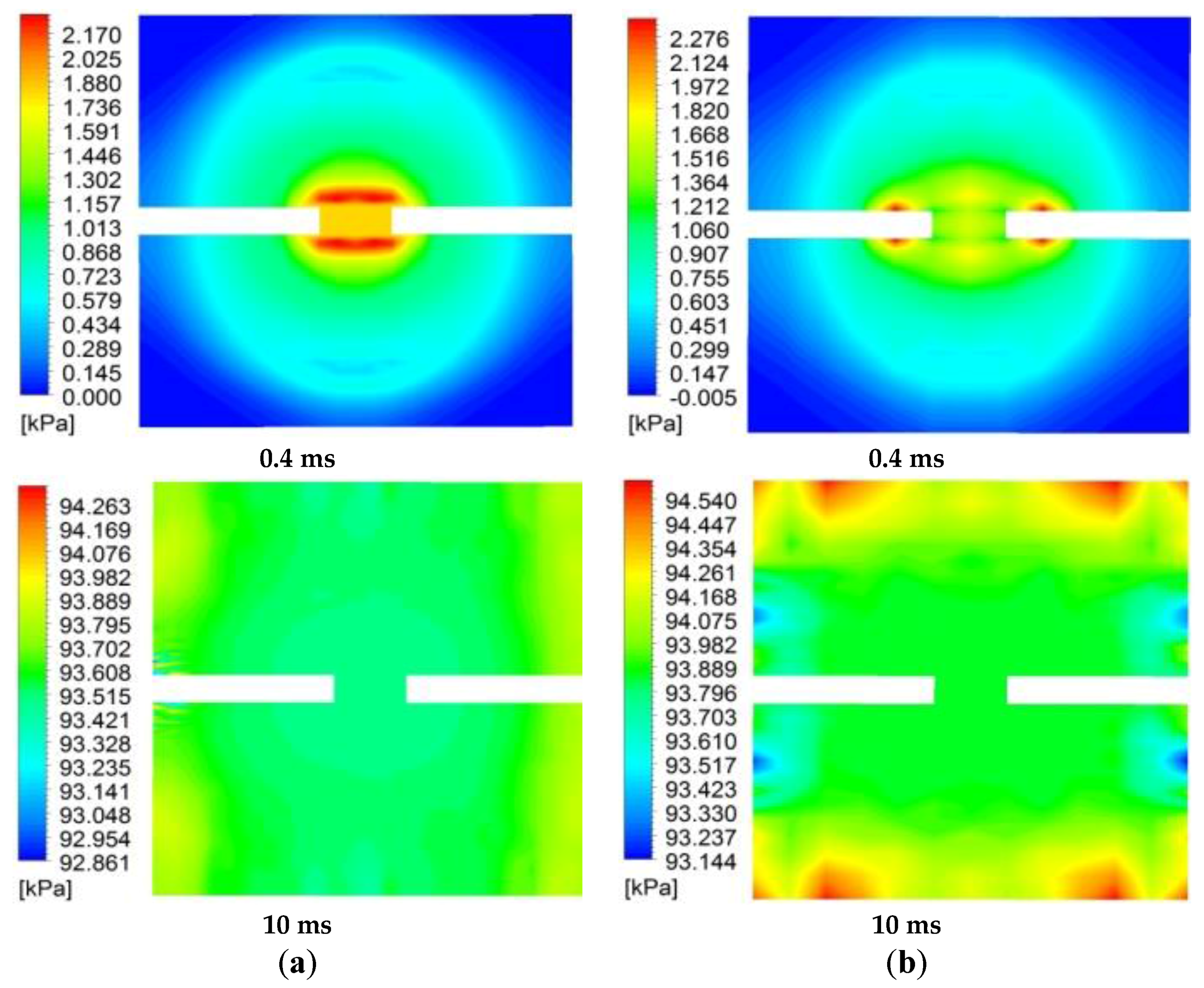
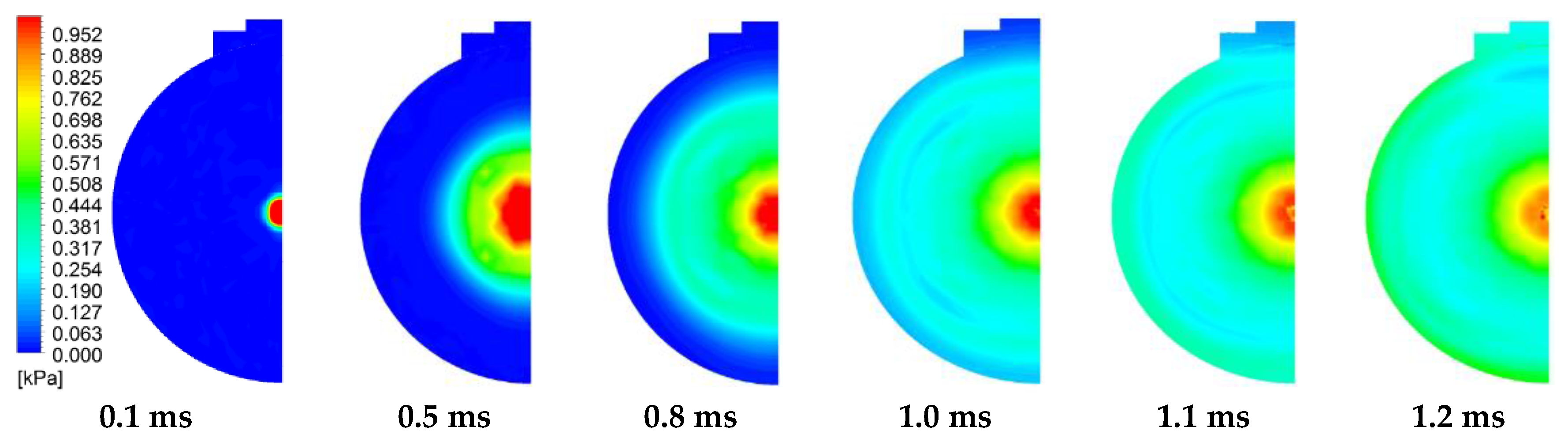
4.5.2. Pressure Distribution in the Container
5. Conclusions
- (1)
- Arc volume has less influence on the calculation of pressure distribution when the arc duration is short and the arc length is relatively fixed. The relative error of the average pressure rise between the measurement and calculation is about 2%. Hence, the CFD-method can produce a good spatial pressure distribution in a closed container. The application in a real switchgear will be carried out in the further research.
- (2)
- The arc voltage shows fluctuations with the increase of pressure and temperature in a closed container, especially at the end period of arcing (at low currents). Arc voltage RMS changing with arc current shows a large degree of randomness, and the fluctuations of which increase with the increase of gap length. When the gap lengths are 5, 10 and 15 cm, the average potential gradients of the arc are about 26, 20 and 16 V/cm, respectively.
- (3)
- The pressure rise in the container increases with the increase of arc energy, and both show a good relation with linear function. The proportion (kp) of the arc energy leading to pressure rise in the container is about 44.9%. There is less influence of gap length and arc energy on kp when the arc energy is low. For higher arc energy, the change of the pressure rise and kp under different electrode types (rod or plane electrodes) needs to be performed in further research.
- (4)
- When the pressure waves reach the container wall, their reflection and superimposition effects will appear on the wall, which causes the maximum overpressure near the wall to increase. The peak value of the overpressure in the positions with a large corner is greater than in other areas with a small corner at the beginning of arcing. After a few milliseconds, pressure waves are no longer of any importance in a closed container.
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Cai, B.; Chen, D.G.; Li, Z.P. Simulation and experiments on internal arcing faults in MV metal-clad switchgear. Trans. China Soc. Eletr. Tech. 2004, 19, 82–87. [Google Scholar]
- Zhang, X.; Zhang, J.; Gockenbach, E. Calculation of pressure and temperature in medium-voltage electrical installations due to fault arcs. J. Phys. D Appl. Phys. 2008, 41, 1–11. [Google Scholar] [CrossRef]
- AC Metal-Enclosed Switchgear and Controlgear for Rated Voltages above 1 kV and Up to and Including 52 kV; IEC 62271-200; International Electrotechnical Commission: Geneva, Switzerland, 2011.
- Dasbach, A.; Pietsch, G.J. Calculation of pressure waves in substation buildings due to arcing faults. IEEE Trans. Power Del. 1990, 5, 1760–1765. [Google Scholar] [CrossRef]
- Friberg, G.; Pietsch, G.J. Calculation of pressure rise due to arcing faults. IEEE Trans. Power Del. 1999, 14, 365–370. [Google Scholar] [CrossRef]
- Rong, M.; Li, M.; Wu, Y.; Yang, F.; Liu, W.; Li, Y.; Chen, Z. 3-D MHD modeling of internal fault arc in a closed container. IEEE Trans. Power Del. 2014. [Google Scholar] [CrossRef]
- Wu, Y.; Li, M.; Rong, M.; Yang, F.; Murphy, A.B.; Wu, Y.F.; Yuan, D. Experimental and theoretical study of internal fault arc in a closed container. J. Phys. D Appl. Phys. 2014, 47, 1–14. [Google Scholar] [CrossRef]
- Anantavanich, K.; Pietsch, G.J.; Gockenbach, E. Modelling of SF6-air mixtures in MV switchgear during internal arcing using a CFD tool. In Proceedings of the 15th International Symposium on High Voltage Engineering (ISH), Ljubljana, Slovenia, 27–31 August 2007.
- Anantavanich, K.; Pietsch, G.J. Calculation of overpressure in the surroundings of fault arcs in SF6-air mixtures. In Proceedings of the 17th International Conference on Gas Discharges and Their Applications, Cardiff, UK, 7–12 September 2008.
- Zhang, X.; Pietsch, G.; Gockenbach, E. Investigation of the thermal transfer coefficient by the energy balance of fault arcs in electrical installations. IEEE Trans. Power Del. 2006, 21, 425–431. [Google Scholar] [CrossRef]
- Iwata, M.; Anantavanich, K.; Pietsch, G. Influence of arc current on fraction kp of electric arc energy leading to pressure rise in a closed container. In Proceedings of the 17th International Conference on Gas Discharges and Their Applications, Cardiff, UK, 7–12 September 2008.
- Iwata, M.; Anantavanich, K.; Pietsch, G.J. Influence of current and electrode material on fraction kp of electric arc energy leading to pressure rise in a closed container during internal arcing. IEEE Trans. Power Del. 2010, 25, 2028–2029. [Google Scholar] [CrossRef]
- Iwata, M.; Tanaka, S.I.; Ohtaka, T.; Amakawa, T.; Anantavanich, K.; Pietsch, G.J. CFD calculation of pressure rise due to internal AC and DC arcing in a closed container. IEEE Trans. Power Del. 2011, 26, 1700–1709. [Google Scholar] [CrossRef]
- Wahle, A.; Pietsh, G.J. Effect of energy absorption systems on internal arc testing of medium voltage switchgear. In Proceedings of the 17th International Conference on Gas Discharges and Their Applications, Cardiff, UK, 7–12 September 2008.
- Rochette, D.; Clain, S.; Bussiére, W.; André, P.; Besnard, C. Porous filter optimization to improve the safety of the medium-voltage electrical installations during an internal arc fault. IEEE Trans. Power Del. 2010, 25, 2464–2471. [Google Scholar] [CrossRef]
- Rochette, D.; Clain, S.; André, P.; Bussiére, W.; Gentils, F. Two-dimensional modeling of internal arc effects in an enclosed MV cell provided with a protection porous filter. J. Phys. D Appl. Phys. 2007, 40, 3137–3144. [Google Scholar] [CrossRef]
- Anantavanich, K. Calculation of Pressure Rise in Electrical Installations Due to Internal Arcs Considering SF6-air Mixtures and Arc Energy Absorbers. Ph.D. Thesis, RWTH Aachen University, Aachen, Germany, March 2010. [Google Scholar]
- Finke, S.; Koenig, D.; Kaltenborn, U. Effects of fault arcs on insulating walls in electrical switchgear. In Proceedings of the International Symposium on Electrical Insulation, Anaheim, CA, USA, 2–5 April 2000.
- Wactor, M.; Olsen, T.W.; Ball, C.J.; Lemmerman, D.J.; Puckett, R.J.; Zawadzki, J. Strategies for mitigation the effects of internal arcing faults in medium-voltage metal-enclosed switchgear. In Proceedings of the IEEE/PES Transmission and Distribution Conference and Exposition, Developing New Perspectives, Atlanta, GA, USA, 28 October–2 November 2001.
- Sweeting, D. Arcing faults in electrical equipment. In Proceedings of the 56th Petroleum and Chemical Industry Conference, Anaheim, CA, USA, 14–16 September 2009.
- Sweeting, D. Arcing faults in electrical equipment. IEEE Trans. Ind. Appl. 2011, 47, 387–397. [Google Scholar] [CrossRef]
- Bjortuft, T.R.; Granhaug, O.; Hagen, S.T.; Kuhlefelt, J.H.; Salge, G.; Skryten, P.K.; Stangherlin, S. Internal arc fault testing of gas insulated metal enclosed MV switchgear. In Proceedings of the 18th International Conference and Exhibition on Electricity Distribution, Turin, Italy, 6–9 June 2005.
- Lowke, J.J. Simple theory of free-burning arcs. J. Phys. D Appl. Phys. 1979, 12, 1873–1886. [Google Scholar] [CrossRef]
- Sing, A.; Ahmmed, M.; Atharparvez, M. Numerical simulation of arc splitting process in a L V switching device considering thermo-field emission mechanism. In Proceedings of the 2015 IEEE 61st Holm Conference on Electrical Contacts, San Diego, CA, USA, 11–14 October 2015.
- Fjeld, E.; Hagen, S.T. Small scale arc fault testing in air. In Proceedings of the 20th International Conference and Exhibition on Electricity Distribution-Part 1, Prague, Czech Republic, 8–11 June 2009.
- Fjeld, E.; Hagen, S.T. Small-scale arc fault testing of medium-voltage metal-enclosed switchgear. IEEE Trans. Power Del. 2016, 31, 37–43. [Google Scholar] [CrossRef]
- Liang, D.W.; Li, B.; Rong, W. Thermodynamic characteristics of thermally perfect gas and solution of N-S equations. J. Nanjing Univ. Aeronaut. Astronaut. 2003, 35, 424–429. [Google Scholar]
- Chen, X.F.; Zhang, Y.; Zhang, Y. Effect of CH4-air ratios on gas explosion flame microstructure and propagation behaviors. Energies 2012, 5, 4132–4146. [Google Scholar] [CrossRef]


| Melting Point (°C) | Density (g/cm3) | Specific Heat Capacity (kJ·kg−1·K−1) | Electrical Resistivity (Ω·m) |
|---|---|---|---|
| 183 | 8.4 | 0.19 | 14.5 × 10−8 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license ( http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, P.; Ruan, J.; Huang, D.; OuYang, Z.; Zhang, L.; Long, M.; Wei, M. Analysis of Pressure Rise in a Closed Container Due to Internal Arcing. Energies 2017, 10, 294. https://doi.org/10.3390/en10030294
Li P, Ruan J, Huang D, OuYang Z, Zhang L, Long M, Wei M. Analysis of Pressure Rise in a Closed Container Due to Internal Arcing. Energies. 2017; 10(3):294. https://doi.org/10.3390/en10030294
Chicago/Turabian StyleLi, Peng, Jiangjun Ruan, Daochun Huang, Ziqing OuYang, Li Zhang, Mingyang Long, and Mengting Wei. 2017. "Analysis of Pressure Rise in a Closed Container Due to Internal Arcing" Energies 10, no. 3: 294. https://doi.org/10.3390/en10030294
APA StyleLi, P., Ruan, J., Huang, D., OuYang, Z., Zhang, L., Long, M., & Wei, M. (2017). Analysis of Pressure Rise in a Closed Container Due to Internal Arcing. Energies, 10(3), 294. https://doi.org/10.3390/en10030294





