Simulating Extreme Directional Wave Conditions
Abstract
1. Introduction
- Defining extreme combinations of significant wave height and period relating to specified return periods
- Calculating, and subsequently incorporating, observed spectral and directional complexity in the defined extreme sea states
- Scaling considerations: wavemaker limits, depth ratios
- Sea state correction and validation in the wave basin
1.1. The European Marine Energy Centre: Site and Data Overview
1.2. The FloWave Ocean Energy Research Facility
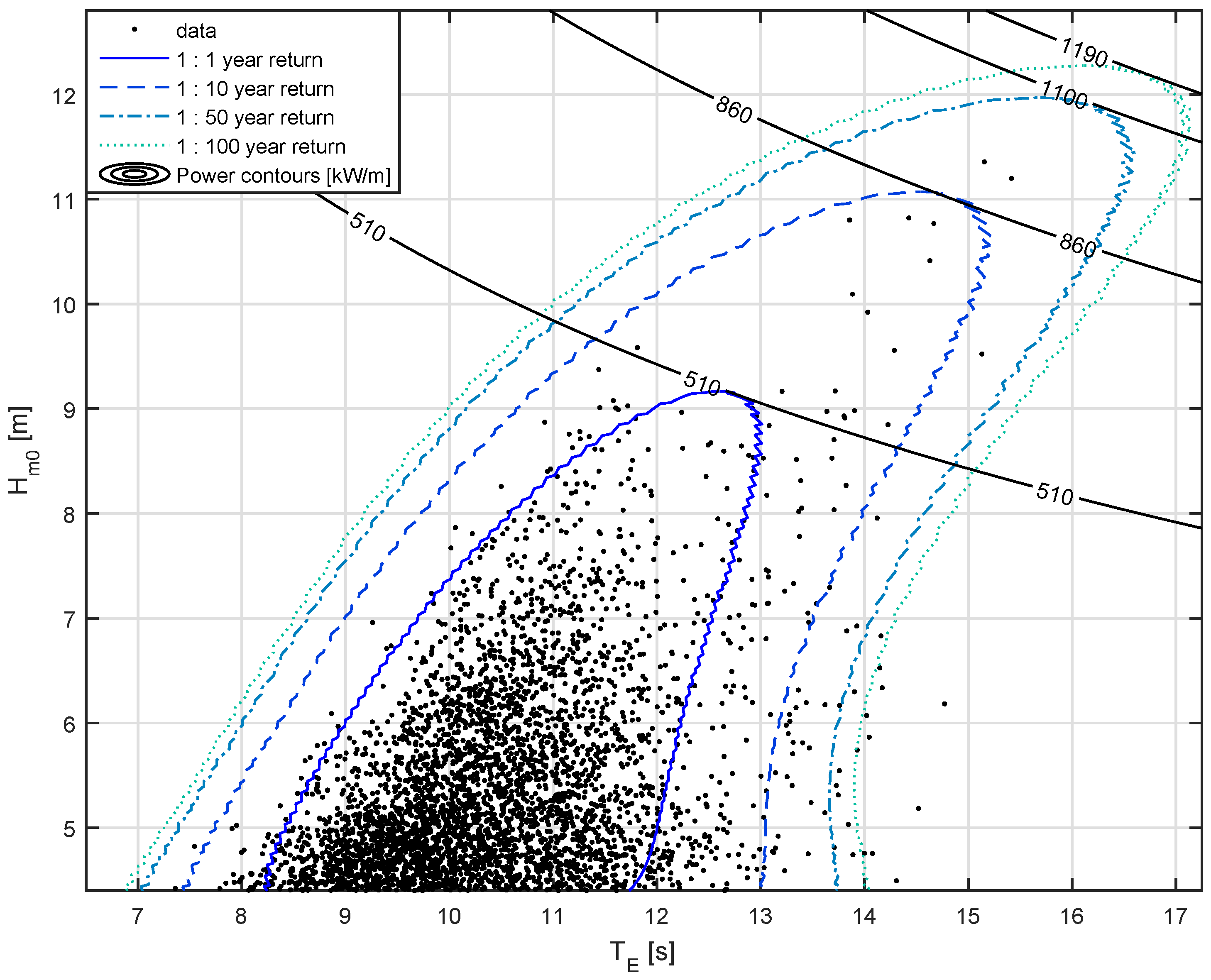
2. Defining Extreme Directional Wave Conditions
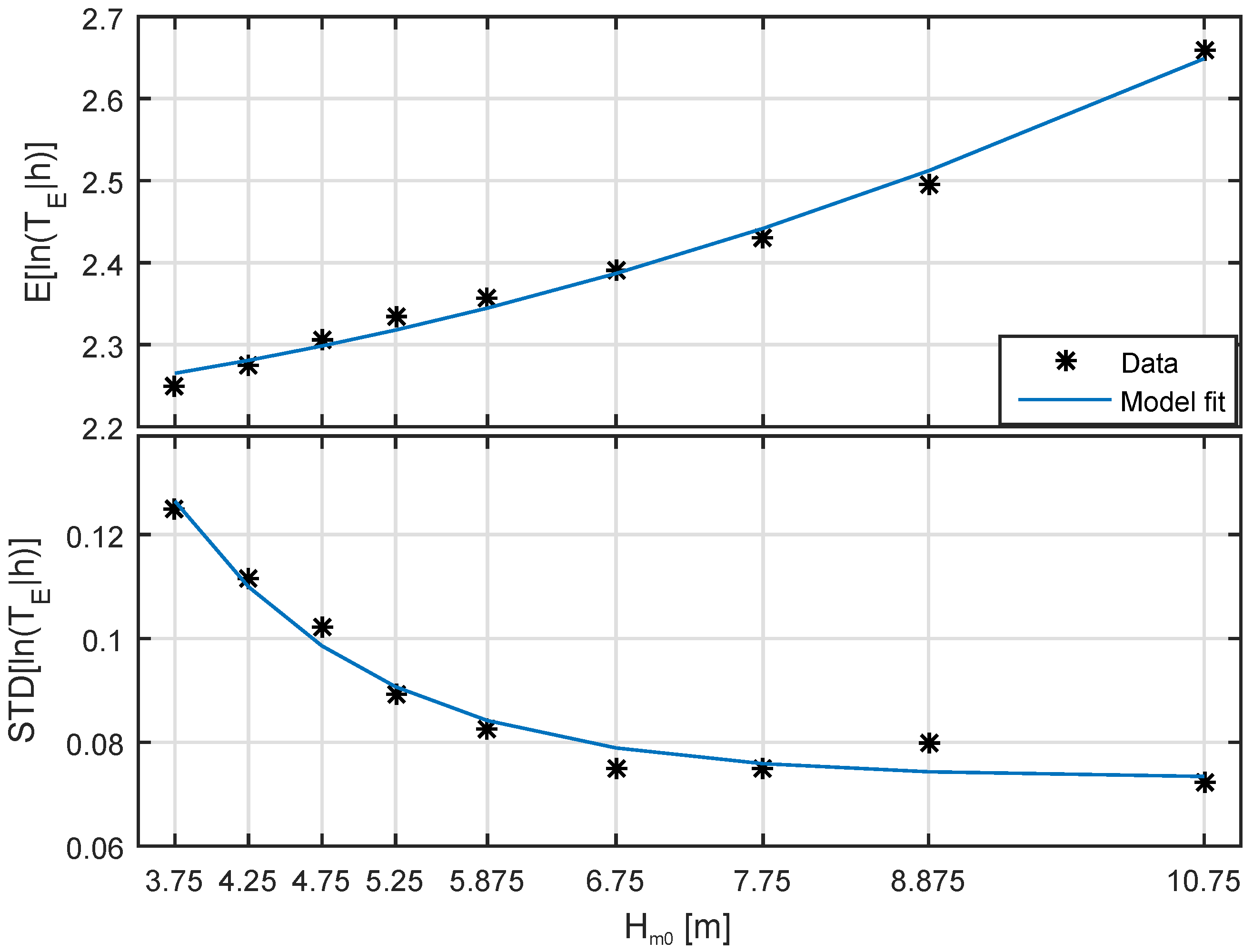
2.1. Defining Extreme Wave Height and Period
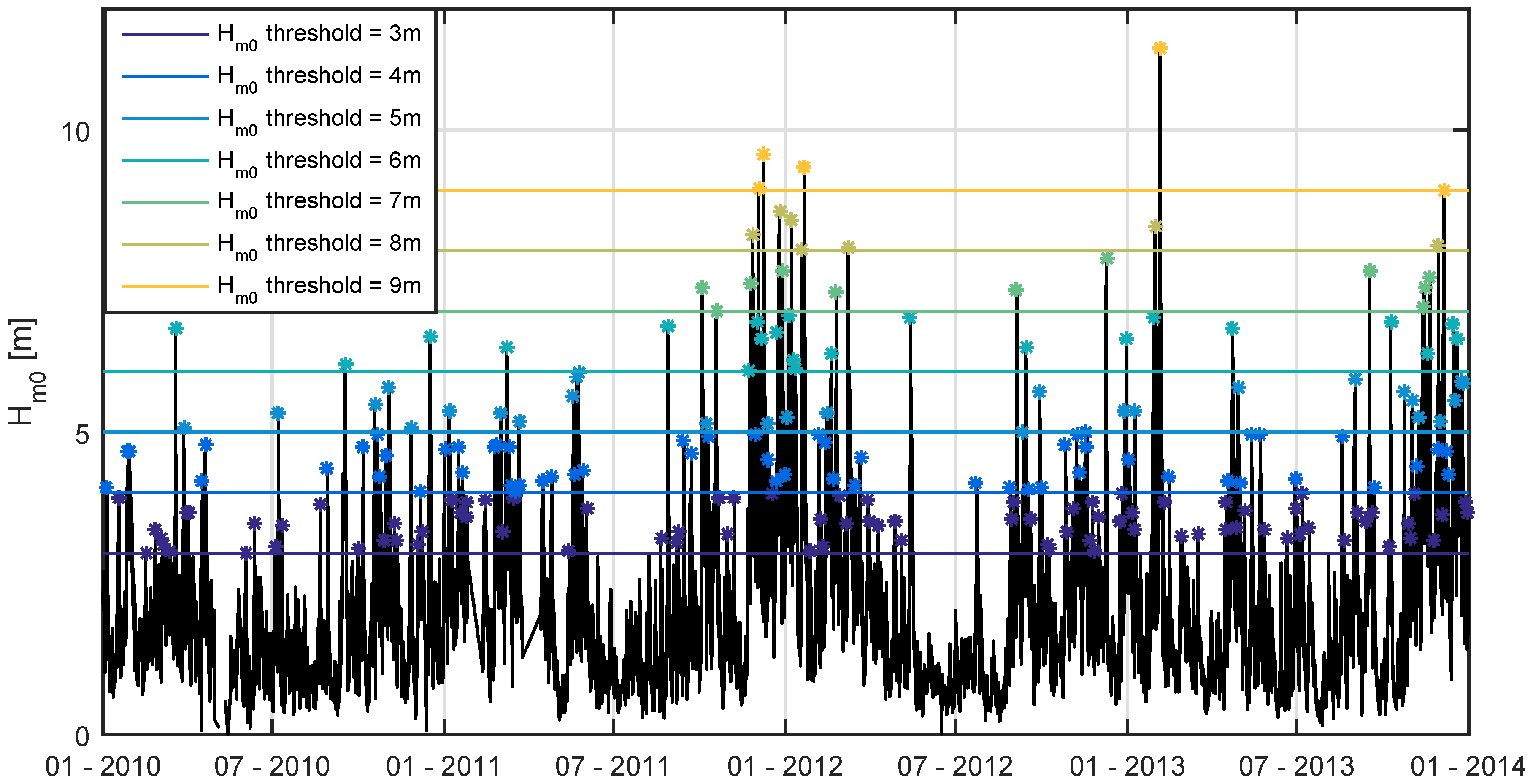
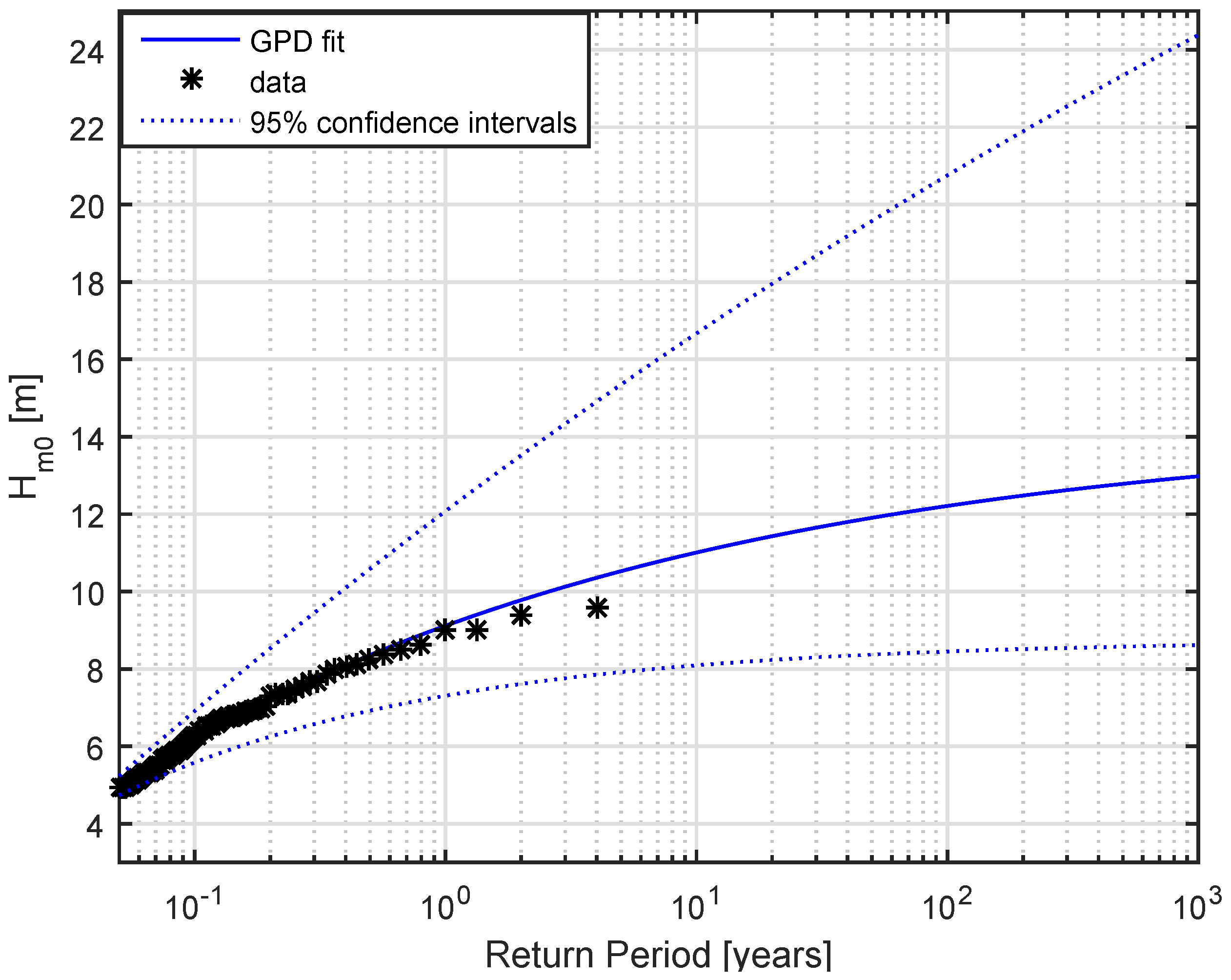
2.1.1. Significant Wave Height
- where: = shape parameter, = scale parameter, = rate, u = threshold.
- Type I: = 0, Type II: > 0, Type III: < 0
2.1.2. Energy Period
2.1.3. Combined Conditions
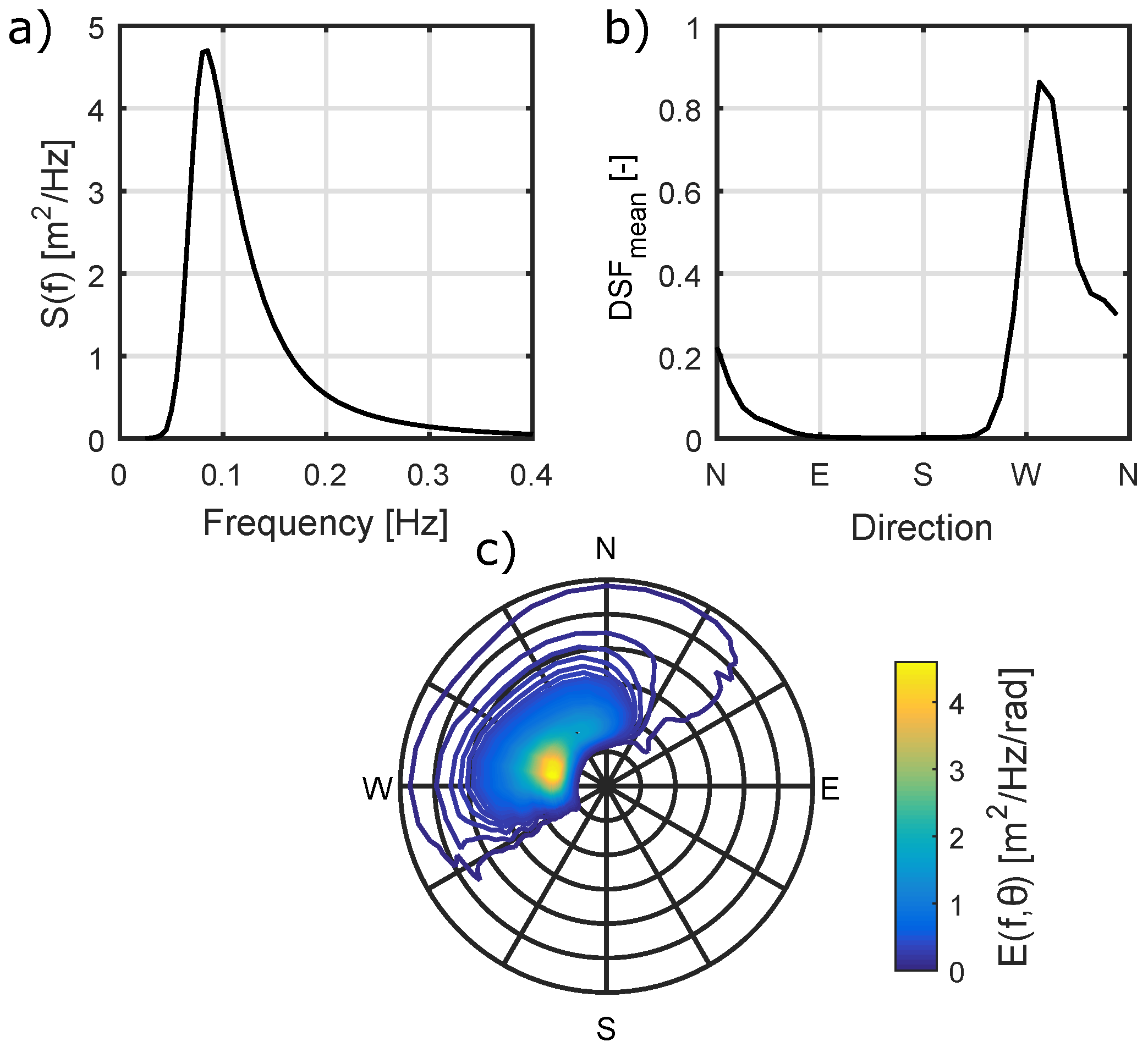
2.2. Defining Extreme Directional Spectra
2.2.1. Reconstructing Directional Spectra from Buoy Data
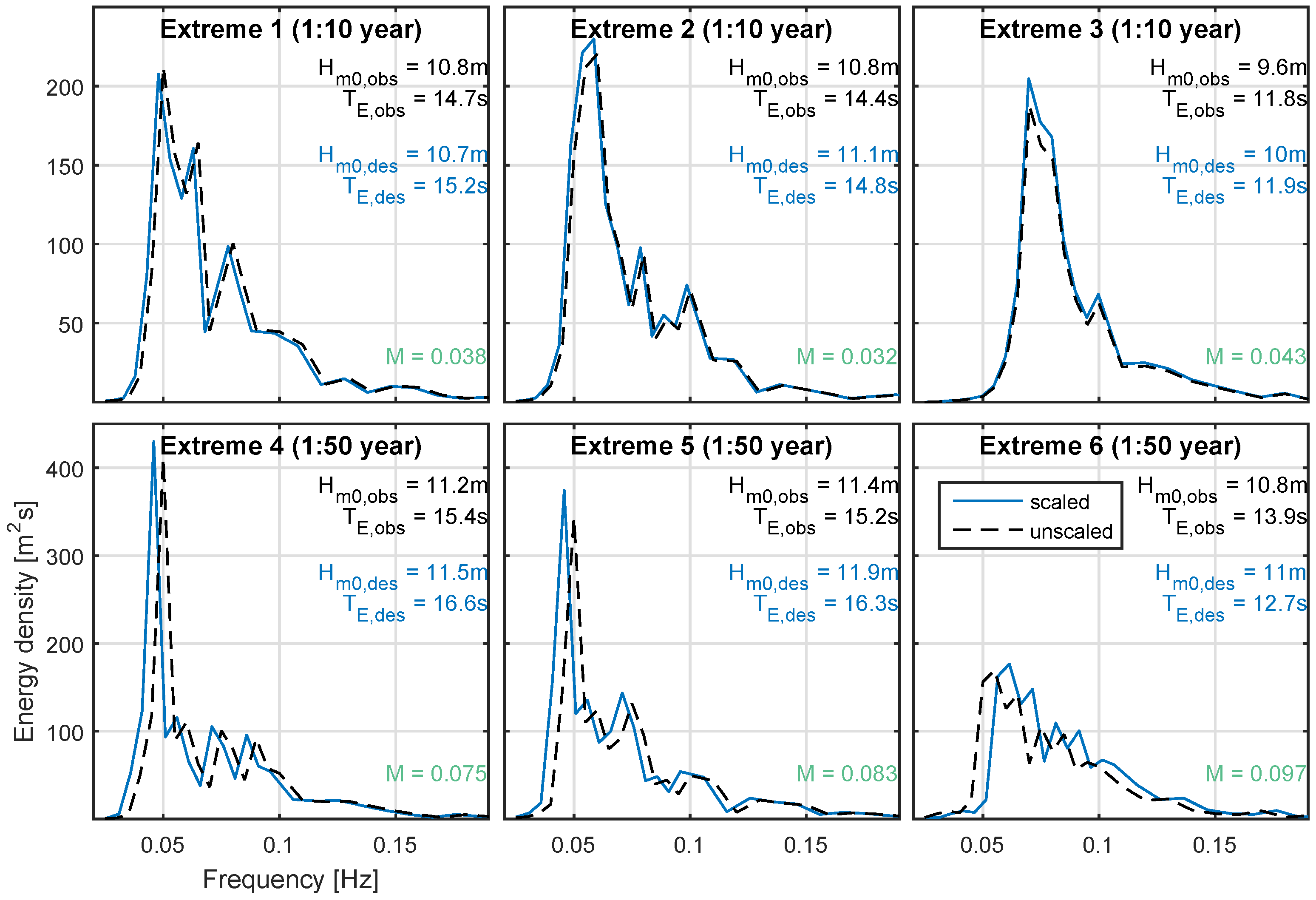
2.2.2. Applying Scaling-Shifting Procedure to Observed Extreme Spectra
3. Simulating Extreme Directional Wave Conditions
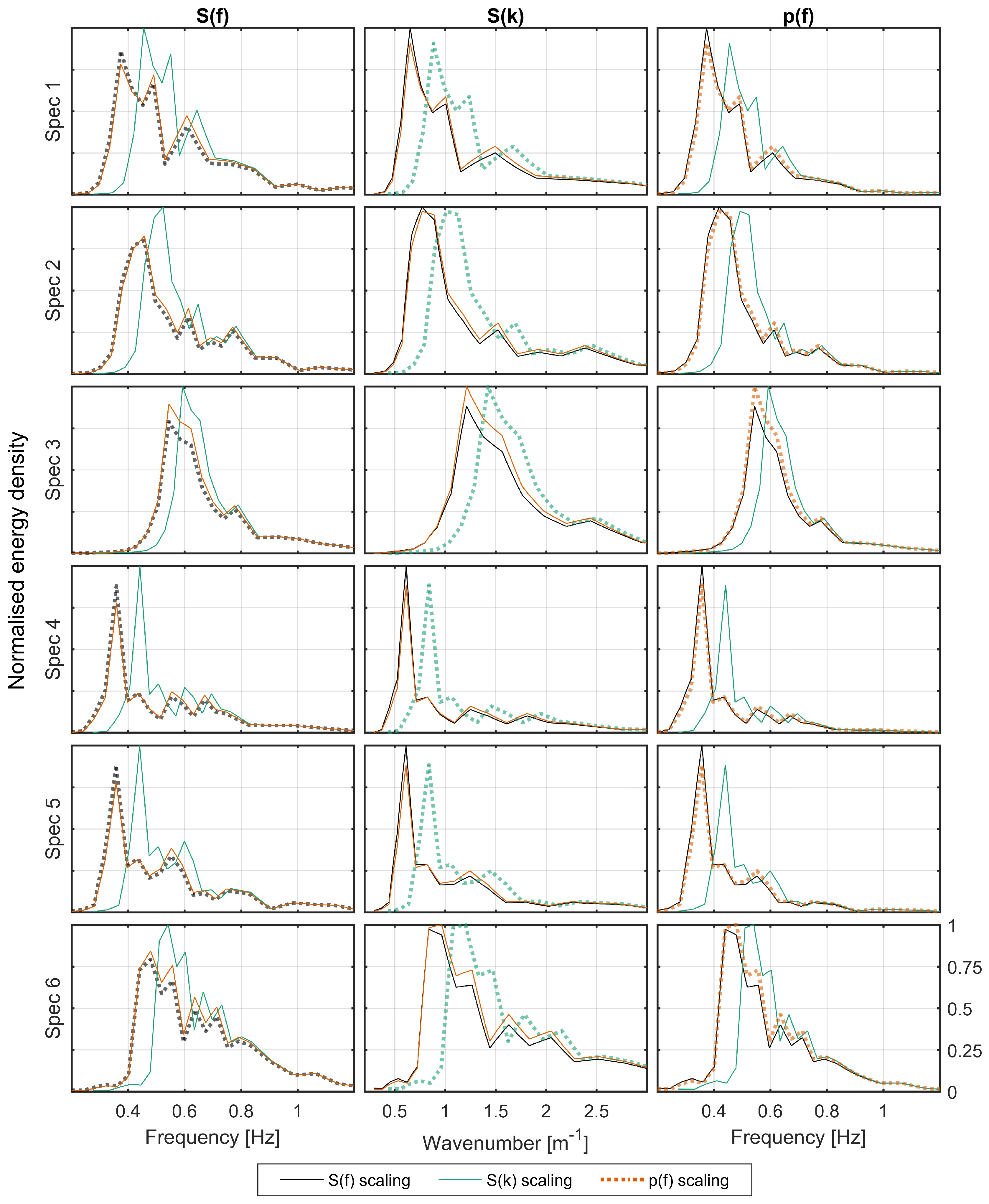
3.1. Scaling Sea States for Tank Tests
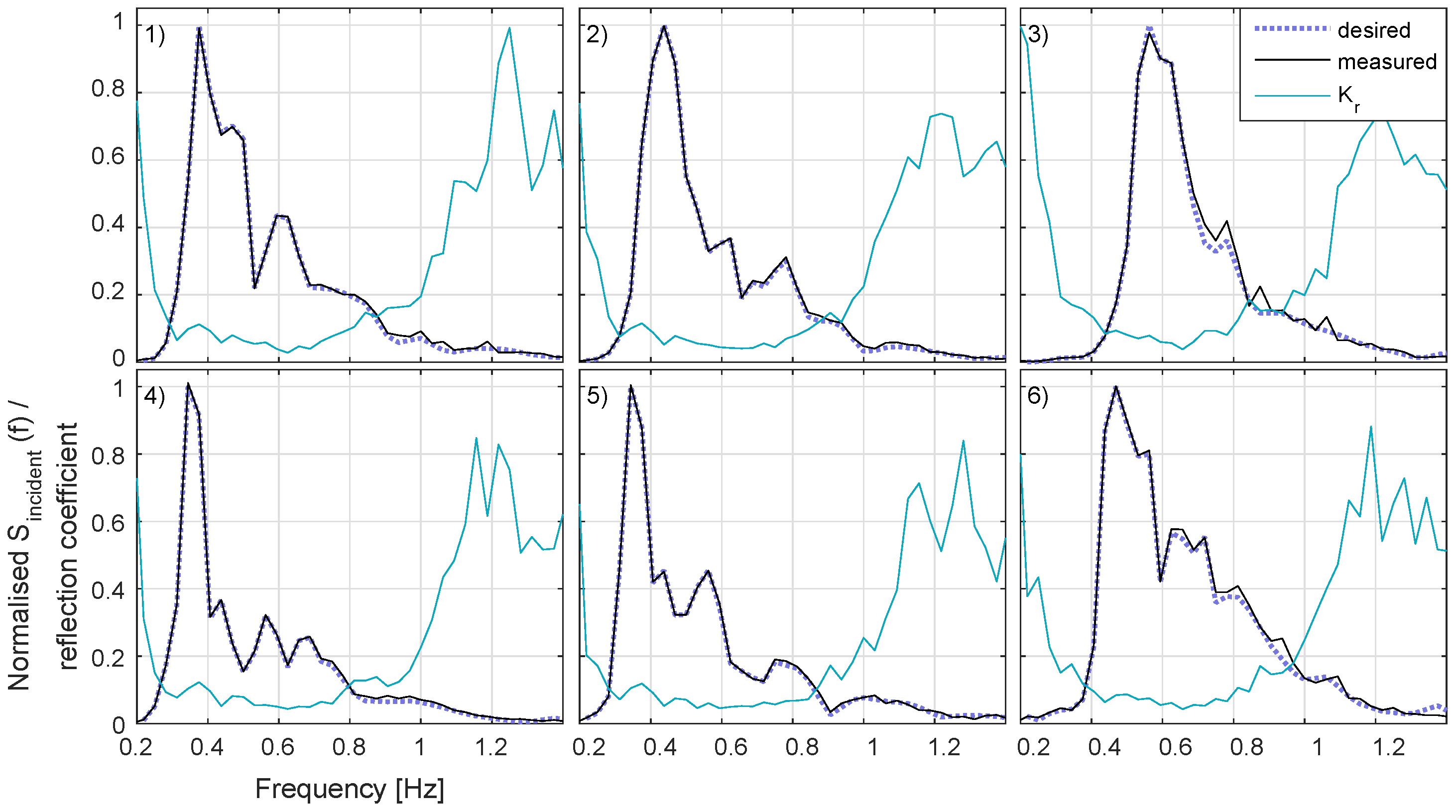
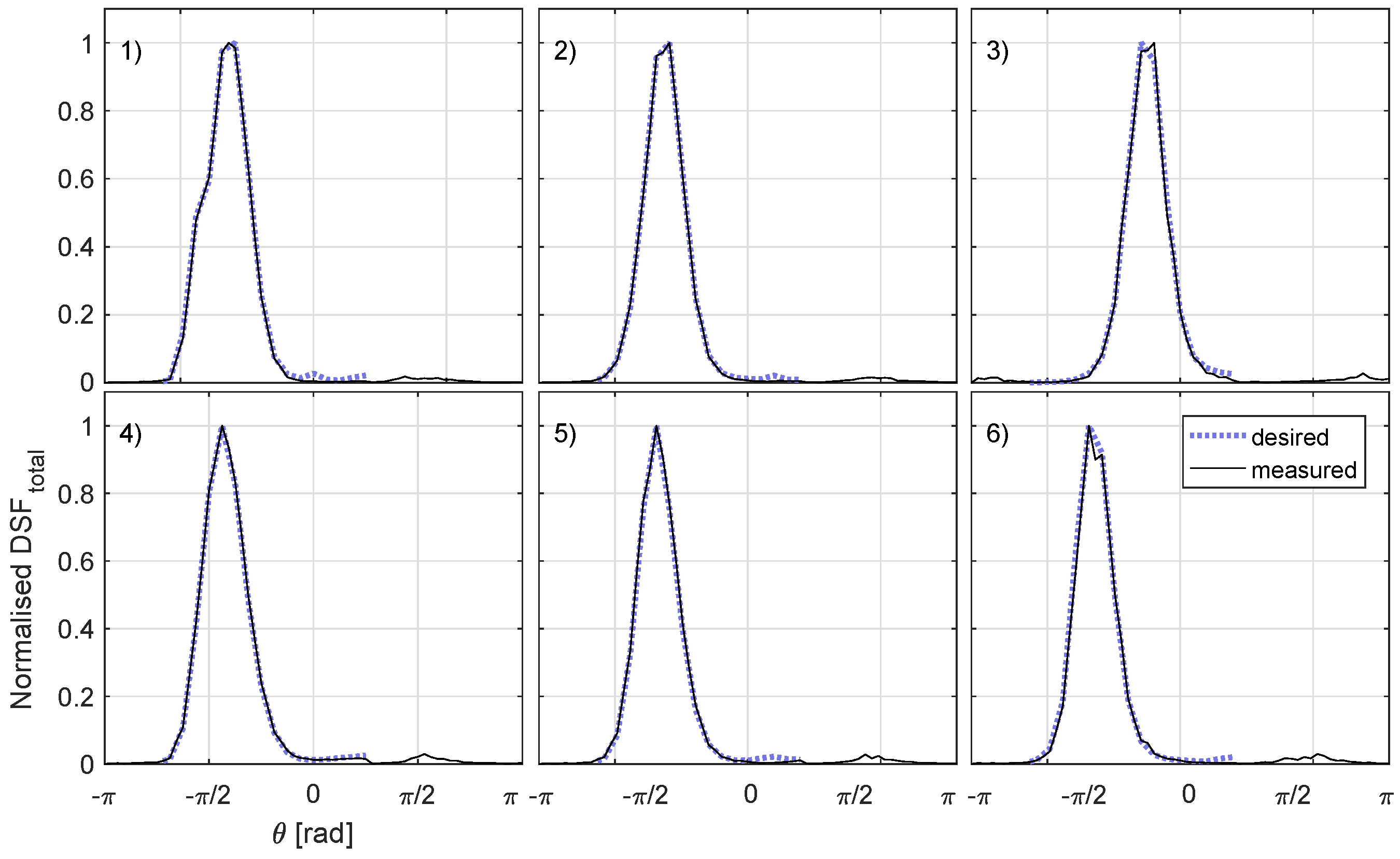
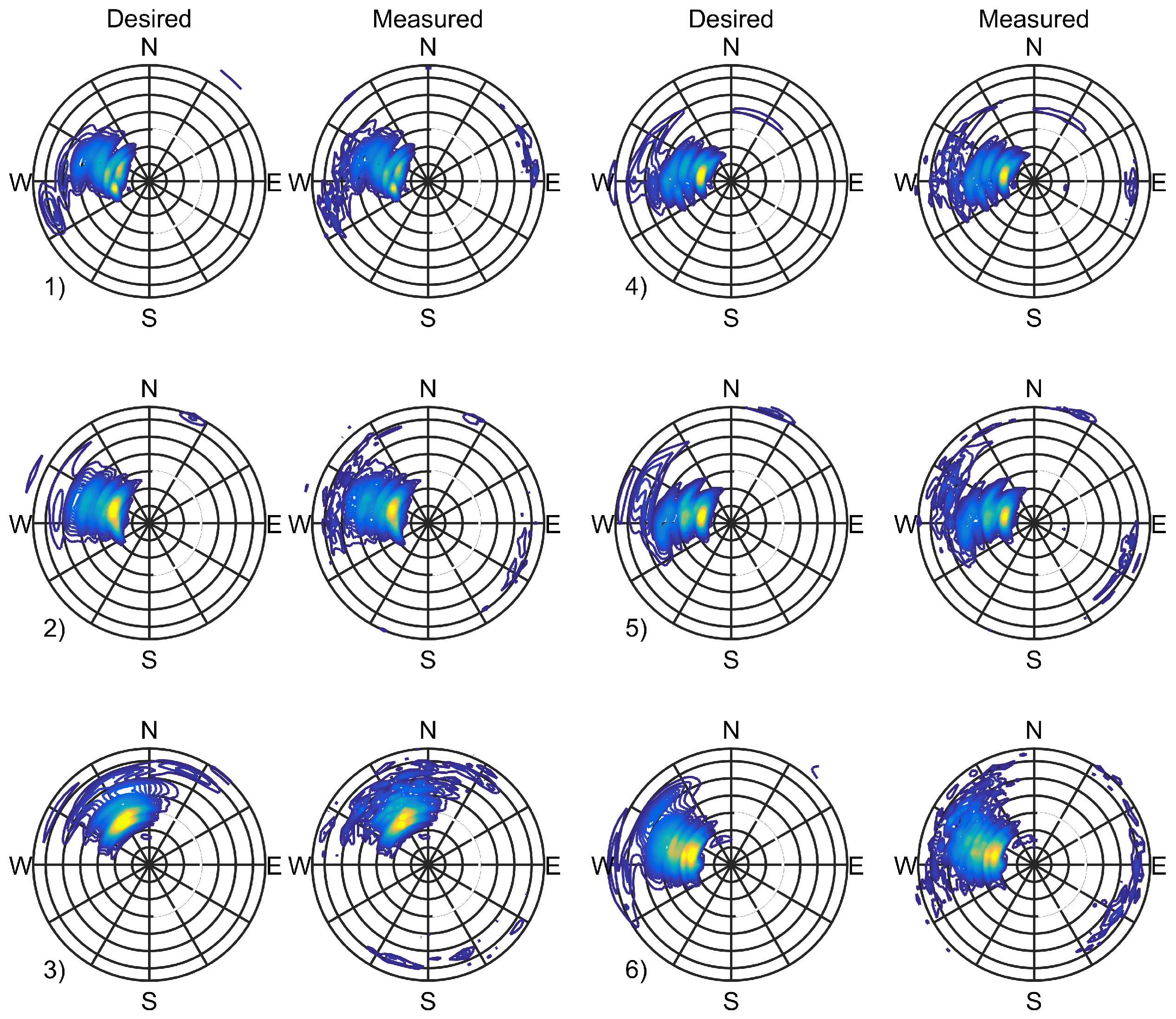
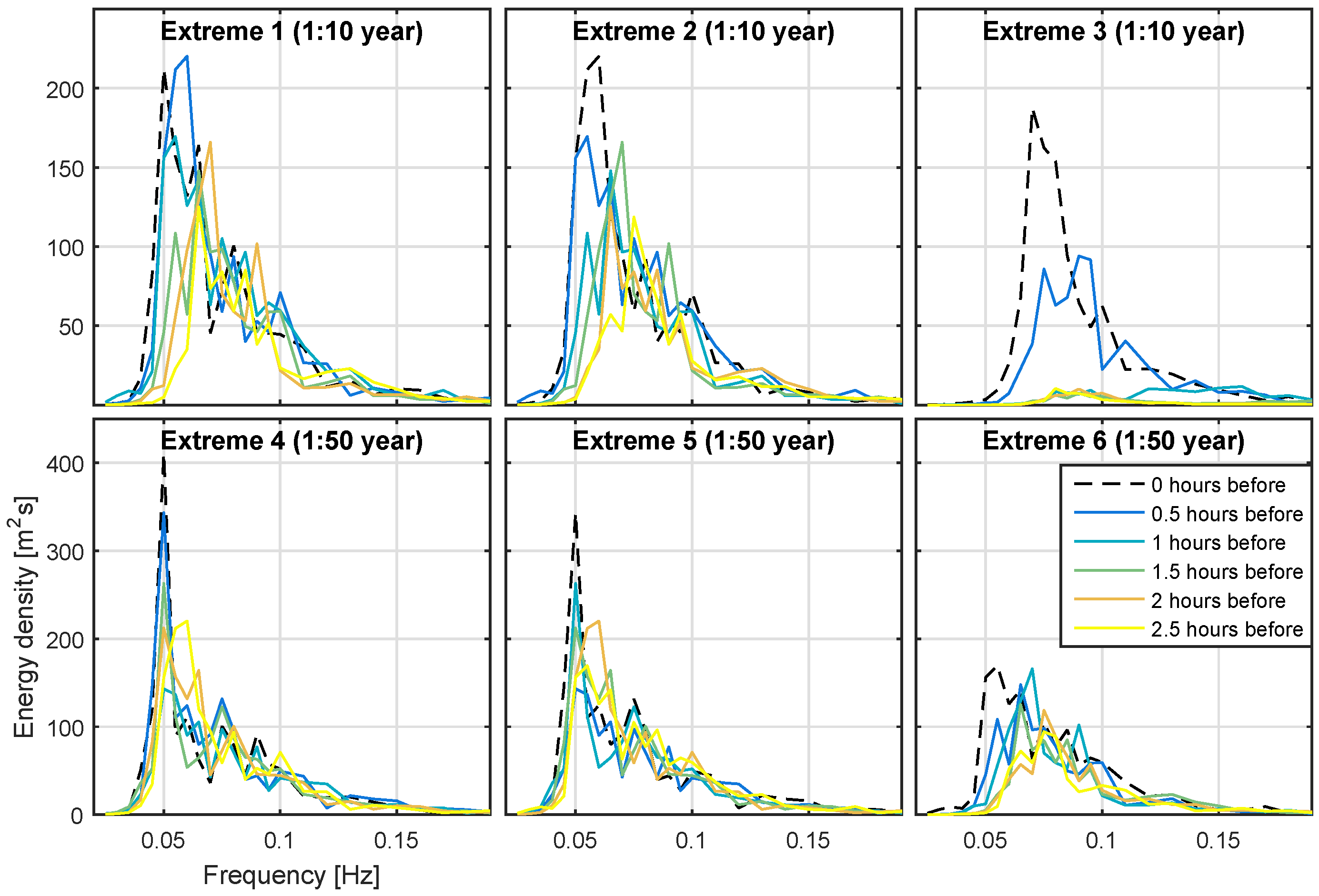
3.2. Generating, Measuring and Validating Extreme Directional Sea States
4. Discussion
4.1. – Contour Creation
4.2. Scaling-Shifting Procedure
4.2.1. Magnitude of Transformation
4.2.2. Spectral Variability
4.3. Froude Scaling Issues
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
Appendix A. Frequency, Wavenumber and Power Spectrum Scaled Spectra
References
- Goda, Y. Random Seas and Design of Maritime Structures; World Scientific: Singapore, 2010. [Google Scholar]
- Coles, S.G. An Introduction to Statistical Modeling of Extreme Values; Springer-Verlag: London, UK, 2001; p. 221. [Google Scholar]
- Caires, S. Extreme Value Analysis: Wave Data; Technical Report, No. 57; Joint Technical Commission for Oceanography and Marine Meteorology (JCOMM): Geneva, Switzerland, 2011. [Google Scholar]
- Teena, N.V.; Sanil Kumar, V.; Sudheesh, K.; Sajeev, R. Statistical analysis on extreme wave height. Nat. Hazards 2012, 64, 223–236. [Google Scholar] [CrossRef]
- Haver, S.; Nyhus, K. A wave climate description for long term response calculations. In Proceedings of the Fifth International Symposium on Offshore Mechanics and Arctic Engineering, Tokyo, Japan, 13–18 April 1986. [Google Scholar]
- Winterstein, S.R.; Ude, T.C.; Cornell, C.A.; Bjerager, P.; Haver, S. Environmental Parameters for Extreme Response: Inverse Form with Omission Factors. In Proceedings of the ICOSSAR ’93, the 6th International Conference on Structural Safety and Reliability, Innsbruck, Austria, 9–13 August 1993. [Google Scholar]
- Berg, J.C. Extreme Ocean Wave Conditions for Northern California Wave Energy Conversion Device; Sandia National Laboratories: Albuquerque, NM, USA; Livermore, CA, USA, 2011.
- Davey, T.; Venugopal, V.; Girard, F.; Smith, H.; Smith, G.; Lawrence, J.; Caveleri, L.; Bertotti, L.; Prevosto, M.; Holmes, B. D2.7 Protocols for Wave and Tidal Resource Assessment; Technical Report, No. 213380; European Commission: Brussels, Belgium, 2010. [Google Scholar]
- Doherty, K.; Folley, M. Extreme Value Analysis of Wave Energy Converters. In Proceedings of the 21st International Offshore and Polar Engineering (ISOPE) Conference, Maui, HI, USA, 19–24 June 2011; Volume 8, pp. 557–564. [Google Scholar]
- Ochi, M.K.; Hubble, E.N. Six-Parameter Wave Spectra. In Proceedings of the 15th International Conference on Coastal Engineering, Honolulu, HI, USA, 11–17 July 1976; Volume 1, pp. 301–328. [Google Scholar]
- Mansour, A.E.; Ertekin, R.C. Report of technical committee I.1 environment. In Proceedings of the 15th International Ship and Offshore Structures Congress, 1st ed.; Elsevier Science: Amsterdam, The Netherlands, 2003; Volume 1. [Google Scholar]
- Saulnier, J.B.; Maisondieu, C.; Ashton, I.; Smith, G.H. Refined sea state analysis from an array of four identical directional buoys deployed off the Northern Cornish coast (UK). Appl. Ocean Res. 2012, 37, 1–21. [Google Scholar] [CrossRef]
- Saulnier, J.B.; Clément, A.; Falcão, A.F.D.O.; Pontes, T.; Prevosto, M.; Ricci, P. Wave groupiness and spectral bandwidth as relevant parameters for the performance assessment of wave energy converters. Ocean Eng. 2011, 38, 130–147. [Google Scholar] [CrossRef]
- Gilloteaux, J.C.; Ringwood, J. Influences of wave directionality on a generic point absorber. In Proceedings of the 8th European Wave and Tidal Energy Conference, Uppsala, Sweden, 7–10 September 2009; pp. 979–988. [Google Scholar]
- Zhang, S.; Zhang, J. A new approach to estimate directional spreading parameters of a cosine-2s model. J. Atmos. Ocean.Technol. 2006, 23, 287–301. [Google Scholar] [CrossRef]
- Holmes, B. Tank Testing of Wave Energy Conversion Systems; The European Marine Energy Centre Ltd (EMEC): Orkney, UK, 2009. [Google Scholar]
- Draycott, S.; Davey, T.; Ingram, D.M.; Lawrence, J.; Day, A.; Johanning, L. Applying Site-Specific Resource Assessment: Emulation of Representative EMEC seas in the FloWave Facility. In Proceedings of the 25th (2015) International Ocean and Polar Engineering Conference, Kona, HI, USA, 21–26 June 2015; pp. 815–821. [Google Scholar]
- Draycott, S.; Davey, T.; Ingram, D.M.; Day, A.; Johanning, L. The SPAIR method: Isolating incident and reflected directional wave spectra in multidirectional wave basins. Coast. Eng. 2016, 114, 265–283. [Google Scholar] [CrossRef]
- Naess, A.; Karpa, O. Statistics of bivariate extreme wind speeds by the ACER method. J. Wind Eng. Ind. Aerodyn. 2015, 139, 82–88. [Google Scholar] [CrossRef]
- Troldborg, N.; Sørensen, J. A simple atmospheric boundary layer model applied to large eddy simulations of wind turbine wakes. Wind Energy 2014, 17, 657–669. [Google Scholar] [CrossRef]
- Markose, S.; Alentorn, A. Option Pricing and the Implied Tail Index with the Generalized Extreme Value (GEV) Distribution 1. J. Deriv. 2011, 1–29. [Google Scholar] [CrossRef]
- Leadbetter, M.R. Extremes and local dependence in stationary sequences. Zeitschrift fur Wahrscheinlichkeitstheorie und Verwandte Gebiete 1983, 65, 291–306. [Google Scholar] [CrossRef]
- Abild, J.; Andersen, E.Y.; Rosbjerg, D. The climate of extreme winds at the great belt, Denmark. J. Wind Eng. Ind. Aerodyn. 1992, 41, 521–532. [Google Scholar] [CrossRef]
- Burrows, R.; Salih, B.A. Statistical Modelling of Long-Term Wave Climates. In Proceedings of the 20th International Conference on Coastal Engineering, Taipei, Taiwan, 9–14 November 1986; pp. 42–56. [Google Scholar]
- Goggins, J.; Finnegan, W. Shape optimisation of floating wave energy converters for a specified wave energy spectrum. Renew. Energy 2014, 71, 208–220. [Google Scholar] [CrossRef]
- Sheng, W.; Lewis, A. Assessment of Wave Energy Extraction From Seas: Numerical Validation. J. Energy Resour. Technol. 2012, 134, 041701. [Google Scholar] [CrossRef]
- Palm, J.; Eskilsson, C.; Paredes, G.M.; Bergdahl, L. Coupled mooring analysis for floating wave energy converters using CFD: Formulation and validation. Int. J. Mar. Energy 2016, 16, 83–99. [Google Scholar] [CrossRef]
- Hann, M.; Greaves, D.; Raby, A. Snatch loading of a single taut moored floating wave energy converter due to focussed wave groups. Ocean Eng. 2015, 96, 258–271. [Google Scholar] [CrossRef]
- International Electrotechnical Commission. IEC 61400-3: Wind Turbines—Part 1: Design Requirements; International Electrotechnical Commission: Geneva, Switzerland, 2005. [Google Scholar]
- International Electrotechnical Commission. PD IEC/TS 62600-200: 2016 Marine Energy—Wave, Tidal and Other Water Current Converters Energy Converters; International Electrotechnical Commission: Geneva, Switzerland, 2016. [Google Scholar]
- Ruiz, M.T. Dynamics and Hydrodynamics for Floating Wave Energy Converters; Technical Report; Instituto Superior Técnico: Lisbon, Portugal, 2010. [Google Scholar]
- Valamanesh, V.; Myers, A.T.; Arwade, S.R. Multivariate analysis of extreme metocean conditions for offshore wind turbines. Struct. Saf. 2015, 55, 60–69. [Google Scholar] [CrossRef]
- Nwogu, O.; Mansard, E.; Miles, M.; Isaacson, M. Estimation of directional wave spectra by the maximum entropy method. In Proceedings of the IAHR Seminar on Wave Analysis and Generation in Laboratory Basins, 22nd IAHR Congress, Lausanne, Switzerland, 31 August–4 September 1987; pp. 363–376. [Google Scholar]
- Benoit, M. Practical comparative performance survey of methods used for estimating directional wave spectra from heave-pitch-roll data. In Proceedings of the 23rd International Conference on Coastal Engineering, Venice, Italy, 4–9 October 1992. [Google Scholar]
- Benoit, M.; Frigaard, P.; Schaffer, H.A. Analyzing multidirectional wave spectra: A tentative classification of available methods. In Proceedings of the 1997 IAHR Conference, San Francisco, CA, USA, 1–12 November 1997; pp. 131–158. [Google Scholar]
- Kim, T.; Lin, L.H.; Wang, H. Application of Maximum Entropy Method to the Real Sea Data. Coast. Eng. 1994, 24, 340–355. [Google Scholar]
- Hashimoto, N. Analysis of the directional wave spectra from field data. Adv. Coast. Ocean Eng. 1997, 3, 103–144. [Google Scholar]
- Noble, D.R.; Draycott, S.; Davey, T.A.; Bruce, T. Design diagrams for wavelength discrepancy in tank testing with inconsistently scaled intermediate water depth. Int. J. Mar. Energy 2017, 18, 109–113. [Google Scholar] [CrossRef]
- Miles, M.D.; Funke, E.R. A Comparison of Methods for Synthesis of Directional Seas. J. Offshore Mech. Arct. Eng. 1989, 111, 43–48. [Google Scholar]
- Grice, J.R.; Taylor, P.H.; Taylor, R.E. Second-order statistics and ‘designer’ waves for violent free-surface motion around multi-column structures. Philos. Trans. R. Soc. Lond. A Math. Phys. Eng. Sci. 2015, 373, 20140113. [Google Scholar] [CrossRef] [PubMed]
- Lawrence, J.; Kofoed-Hansen, H.; Chevalier, C. High-resolution metocean modelling at EMEC’s (UK) marine energy test sites. In Proceedings of the 8th European Wave and Tidal Energy Conference, Uppsala, Sweden, 7–10 September 2009; pp. 1–13. [Google Scholar]
- Euan, C.; Ortega, J.; Alvarez-esteban, P.C. Detecting Stationary intervals for random waves using time series. In Proceedings of the ASME 2014 33rd International Conference on Ocean, Offshore and Arctic Engineering OMAE 2014, San Francisco, CA, USA, 8–13 June 2014. [Google Scholar]
- Alvarez-Esteban, P.C.; Euán, C.; Ortega, J. Time Series Clustering using the Total Variation Distance with Applications in Oceanography. Environmetrics 2015, 27, 355–369. [Google Scholar] [CrossRef]

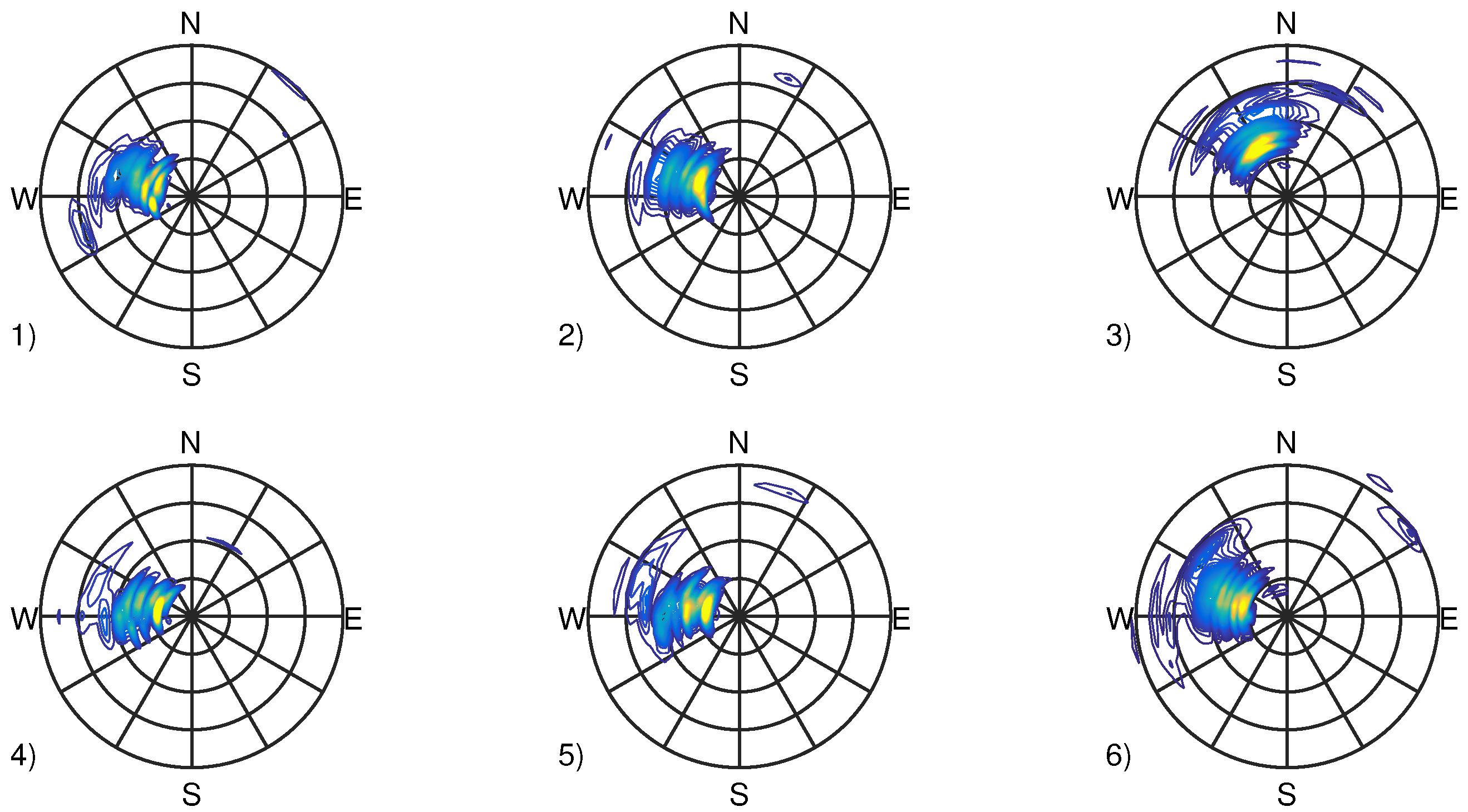


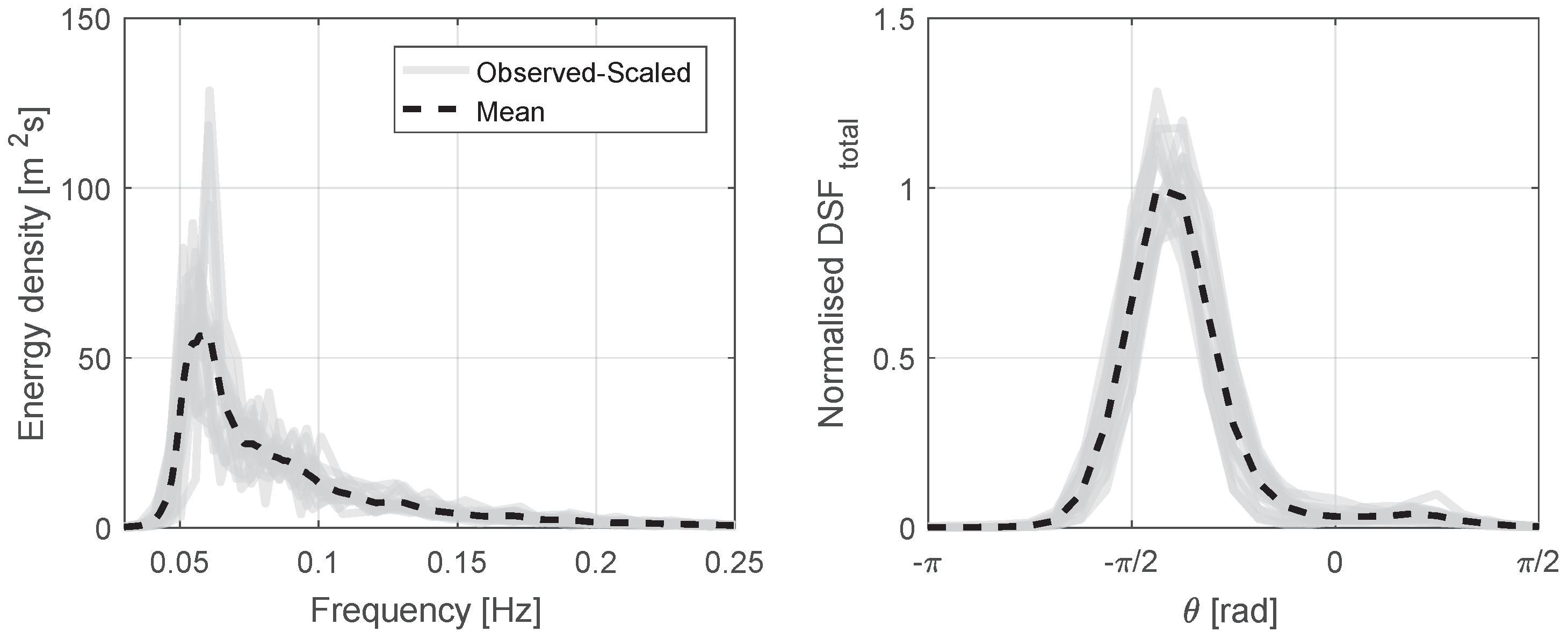
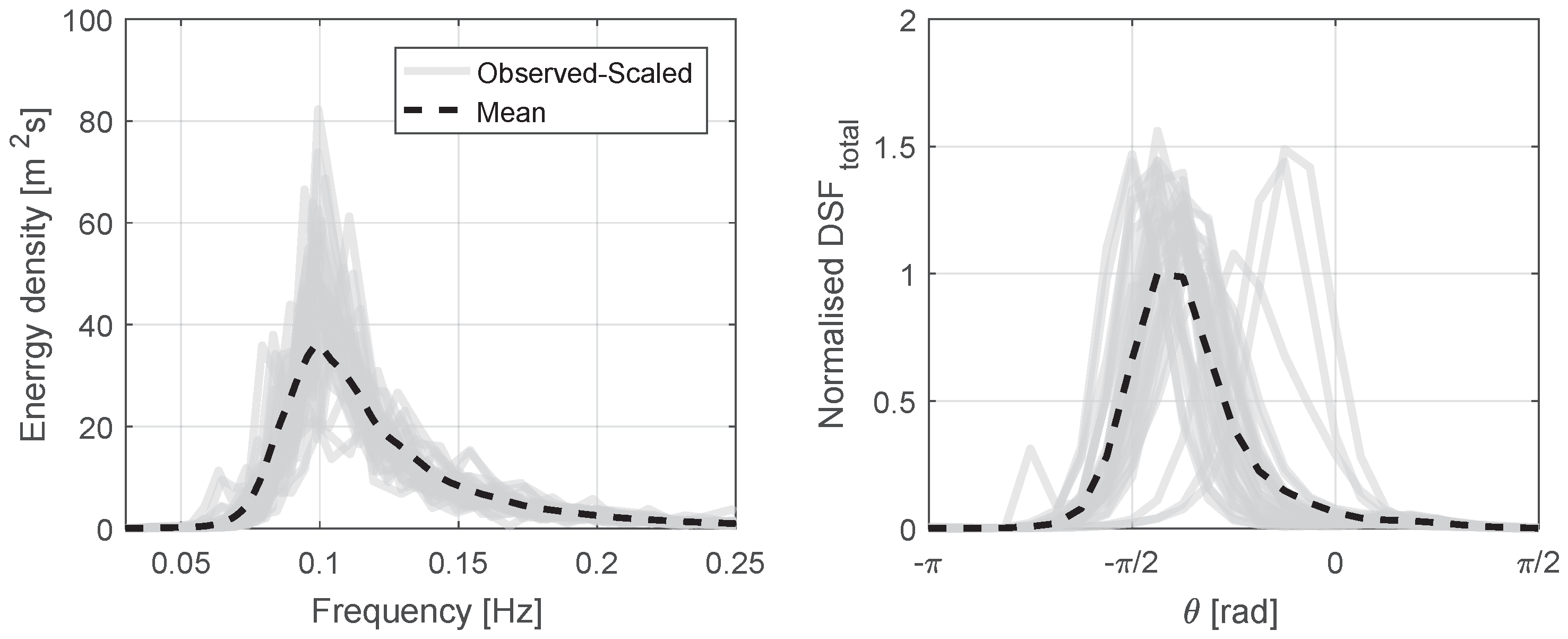
| Extreme Sea State | 1 (1:10) | 2 (1:10) | 3 (1:10) | 4 (1:50) | 5 (1:50) | 6 (1:50) |
|---|---|---|---|---|---|---|
| 10.65 | 11.05 | 10.01 | 11.45 | 11.86 | 11.02 | |
| 15.21 | 14.78 | 11.86 | 16.61 | 16.32 | 12.66 |
| Scaling | Scaling | Scaling | |
|---|---|---|---|
| f | |||
| k | |||
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Draycott, S.; Davey, T.; Ingram, D.M. Simulating Extreme Directional Wave Conditions. Energies 2017, 10, 1731. https://doi.org/10.3390/en10111731
Draycott S, Davey T, Ingram DM. Simulating Extreme Directional Wave Conditions. Energies. 2017; 10(11):1731. https://doi.org/10.3390/en10111731
Chicago/Turabian StyleDraycott, Samuel, Thomas Davey, and David M. Ingram. 2017. "Simulating Extreme Directional Wave Conditions" Energies 10, no. 11: 1731. https://doi.org/10.3390/en10111731
APA StyleDraycott, S., Davey, T., & Ingram, D. M. (2017). Simulating Extreme Directional Wave Conditions. Energies, 10(11), 1731. https://doi.org/10.3390/en10111731







