CO₂ Storage Capacity for Multi-Well Pads Scheme in Depleted Shale Gas Reservoirs

Abstract

As a promising technology to improve shale gas (SG) recovery and CO₂ storage capacity, the multi-well pads (MWPs) scheme has gained more and more attention. The semi-analytical pressure-buildup method has been used to estimate CO₂ storage capacity. It focuses on single multi-fractured horizontal wells (SMFHWs) and does not consider multi-well pressure interference (MWPI) induced by the MWPs scheme. This severely limits the application of this method as incidences of multi-well pressure interference have been widely reported. This paper proposed a new methodology to optimize the injection strategy of the MWPs scheme and maximize CO₂ storage capacity. The new method implements numerical discretization, the superposition theory, Gauss elimination, and the Stehfest numerical algorithm to obtain pressure-buildup solutions for the MWPs scheme. The solution by the new method was validated with numerical simulation and pressure-buildup curves were generated to identify MWPI. Using the new method, we observed that the fracture number and fracture half-length have a positive influence on CO₂ storage capacity. Both can be approximately related to the CO₂ storage capacity by a linear correlation. For a given injection pressure, there is an optimal fracture number; the bigger the limited injection pressure, the smaller the optimal fracture number. Stress sensitivity has positive influences on CO₂ storage capacity, thus extending the injection period would improve CO₂ storage capacity. This work gains some insights into the CO₂ storage capacity of the MWPs scheme in depleted SG reservoirs, and provides considerable guidance on injection strategies to maximize CO₂ storage capacity in depleted SG reservoirs.

1. Introduction

Greenhouse gas control has been a focus of research attention due to climate change induced by the emission of greenhouse gases such as carbon dioxide [1,2]. Geological sequestration is considered one of the most effective ways to mitigate CO₂ emissions. This knowledge is based on capturing CO₂ from emission sources and injecting it into deep geological structures. There are several sequential options for sequestrating CO₂ in geological sinks, including injecting CO₂ into deep saline aquifers, mature oil and gas reservoirs for the purpose of both of sequestration and production enhancement, injecting CO₂ into depleted oil and gas reservoirs, and injecting CO₂ into unconventional coal seams and shale layers [3,4] to enhance gas recovery and CO₂ storage capacity simultaneously, which has been a central topic for many years.

The estimation methods of CO₂ storage capacity in shale formations can be classified into four categories: (1) volume-based calculation methods; (2) production-based calculation methods; (3) numerical simulation methods; and (4) semi-analytical model methods. The volume-based calculation method is easy to apply. Busch et al.; Kang et al and Tian et al. [5,6,7,8,9] experimentally measured the storage volume of CO₂ in shale samples from Barnett Shale, TX, USA, and Sichuan, China. In return, the CO₂ storage capacity can be easily calculated by incorporating the whole volume of shale formation. The production-based method was introduced by Tao et al. [10] to evaluate the CO₂ sequestration capacity of the Marcellus Shale. They estimated the CO₂ storage capacity based on historical and projected shale gas production data. The production-based calculation method is attractive due to the rapid process. Unfortunately, its accuracy may decrease due to poor-quality production data. Numerical simulation methods were established to evaluate the storage capacity of CO₂ injection [11], which can also be used to simulate CO₂ flow behavior in shale. However, this method requires grid discretion, which is time-consuming in a field-scale application [12,13]. Edwards et al. [14] established a two-component (CO₂ and CH₄) model of gas flow in a shale gas formation including adsorption effects. They used the method to evaluate the CO₂ storage capacity of Barnet shale. Borrowing some thoughts from the literature [15,16,17,18,19,20], Chen et al. [21] and Xiao et al. [22] establish a semi-analytical model based on transient pressure buildup of an injection well for CO₂ storage in depleted shale gas reservoirs.

Developing unconventional shale resources has challenged conventional methodologies and engineers for the past few decades. Fortunately, advancements in technology and new analysis methods have allowed SMFHWs to become more economical. After the abandonment of a depleted shale gas reservoir, SMFHWs could be a feasible path to transporting CO₂ into a shale play. Recently, the MWPs scheme has been a center of attention as a promising improved shale gas (SG) recovery and economical CO₂ storage technology [23,24]. Micro-seismic fracturing mapping showed hydraulic fractures extending between wells (Figure 1), gaining the existence of MWPI [25,26]. On the one hand, compared with SMFHWs, the MWPs scheme can provide a more high-conductivity path to transport CO₂ into an underground shale play, and thereby seemingly could gain much more CO₂ storage capacity [27]. On the other hand, MWPI can also stimulate injection pressure, which in turn sharply decreases the CO₂ injection capacity of MWPs [23]. This outstanding contradiction urges us to determine whether multi-wells should be injected simultaneously or sequentially and how to inject them under different injection rates. As a result, this technology strongly challenges the previously effective methodology for estimating CO₂ storage capacity using the semi-analytical pressure-buildup method mentioned above, which focuses on SMFHWs without pressure MWPI [21,22]. Therefore, finding a methodology to optimize the injection strategy of the MWPs scheme to maximize CO₂ storage capacity is a necessity.

Our objectives were to determine the maximum CO₂ storage capacity of the MWPs scheme in depleted shale gas reservoir by addressing the following questions:

• What kinds of pressure buildup can we obtain for the MWPs scheme? What is the difference between the MWPs scheme and SMWHF?
• How can we determine the occurrence of MWPI in the MWPs scheme when we inject CO₂?
• How can we optimize the injection strategy of the MWPs scheme for maximizing CO₂ storage capacity with MWPI?

To answer the questions outlined above, we propose a methodology consisting of four issues that should be solved sequentially and systematically:

• Development of an efficient semi-analytical pressure-buildup model for the MWPs scheme;
• Based on the proposed semi-analytical pressure-transient model, the CO₂ storage capacity of the MWPs scheme is calculated;
• Based on the proposed semi-analytical pressure-transient model, we identify MWPI using pressure-buildup curves;
• Based on the proposed semi-analytical pressure-transient model and the identification of MWPI, we optimize the CO₂ multi-wells injection strategy (whether multi-wells have CO₂ injected simultaneously or sequentially).

In our Section 2, we mainly focus on the first issues; the remaining three issues and a case study will be explained in our Section 3. To facilitate methodology development, this paper is organized as follows. First, we describe the conceptual models of SG formation and MWPs scheme. Second, CO₂ seepage models of shale gas reservoir and HFs are sequentially established and then dynamically coupled. After that, by numerical discretization, superposition theory, Gauss elimination, and Stehfest numerical algorithm, pressure-buildup solutions of MWPs scheme are obtained. Then, to validate our model, numerical simulation is utilized with CMG-GEM module (2015.10.5641.22410, Computer Modelling Group Ltd., Calgary, AB, Canada). Finally, sensitivity analysis is also conducted.

Described below are the attributes of our methodology framework, including details of the development of semi-analytical pressure-buildup model, calculation of CO₂ storage capacity, sensitivity analysis, discussion of results, and related insights. More information on model derivation is provided in the Appendix.

2. Development of Pressure-Buildup Model for MWPs Scheme

Single-phase CO₂ gas (regardless of phase change, such as supercritical CO₂) was used for the development of a semi-analytical pressure-buildup model. The pressure-buildup response of the MWPs scheme was a result of CO₂ flow in a shale gas (SG) system and stimulated HFs system. CO₂ flows from a wellbore into the HFs system, then through the HFs system into a shale play. In this paper, we assumed no frictional pressure loss inside the wellbore, thus, we envisaged two distinct flow regimes governed by different physics: SG reservoir flow and HFs system flow. In the following sections, we first describe a conceptual model of the SG system and MWPs scheme used and then mathematically model these two flow processes. Finally, the SG flow model and fracture system flow model are coupled dynamically.

2.1. Description of Conceptual Model

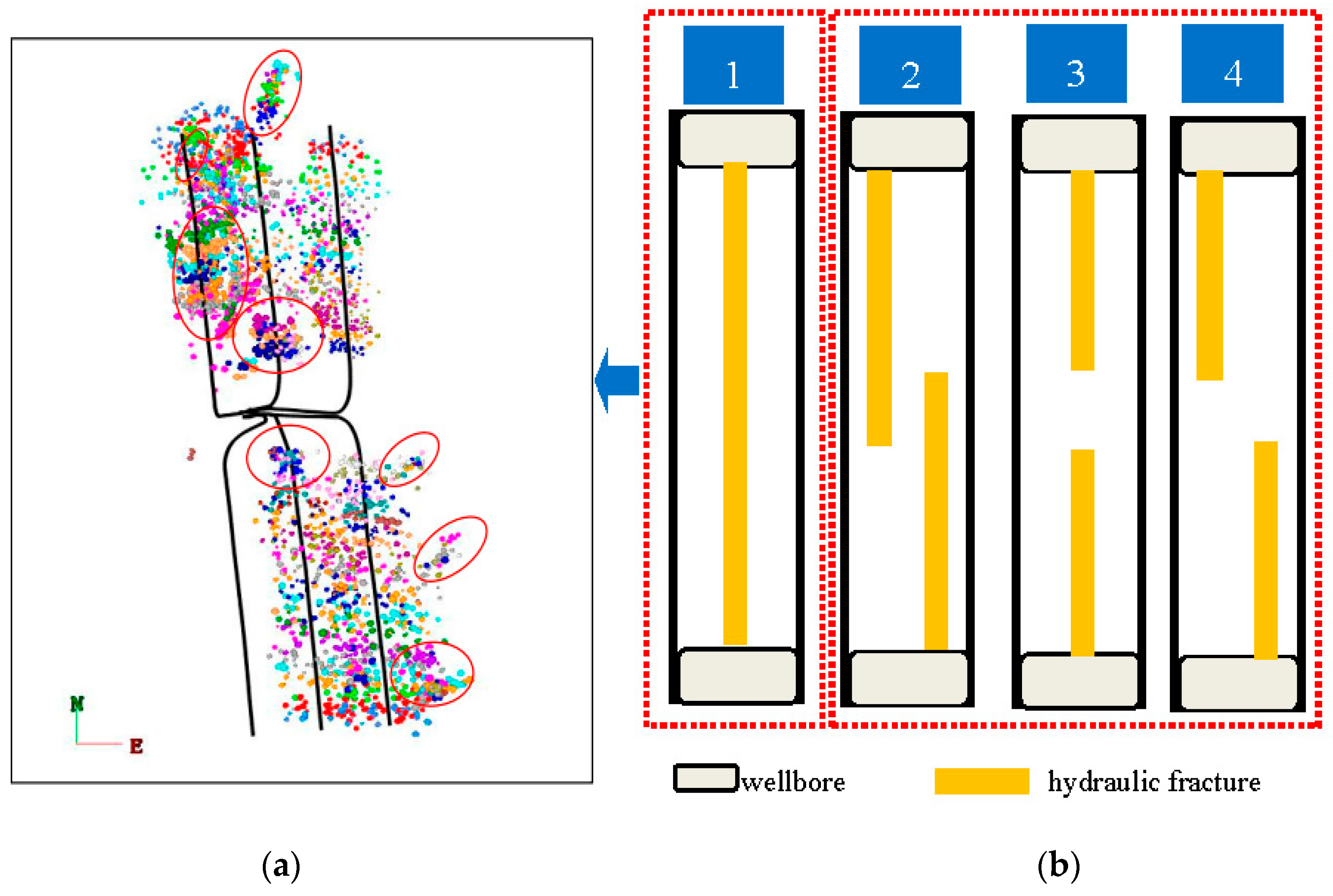
2.1.1. Multi-Well Pad Production (MWPs) Scheme

Figure 2 illustrates the placement of MWP schemes in a SG reservoir. Each MWP scheme has one shared production platform and can contain several SMFHWs. Figure 2a illustrates the micro-seismic surveillance within an MWP scheme. Based on the micro-seismic experiment, we can clearly observe that the distribution of HFs can be classified into four types (see Figure 2b) [23].

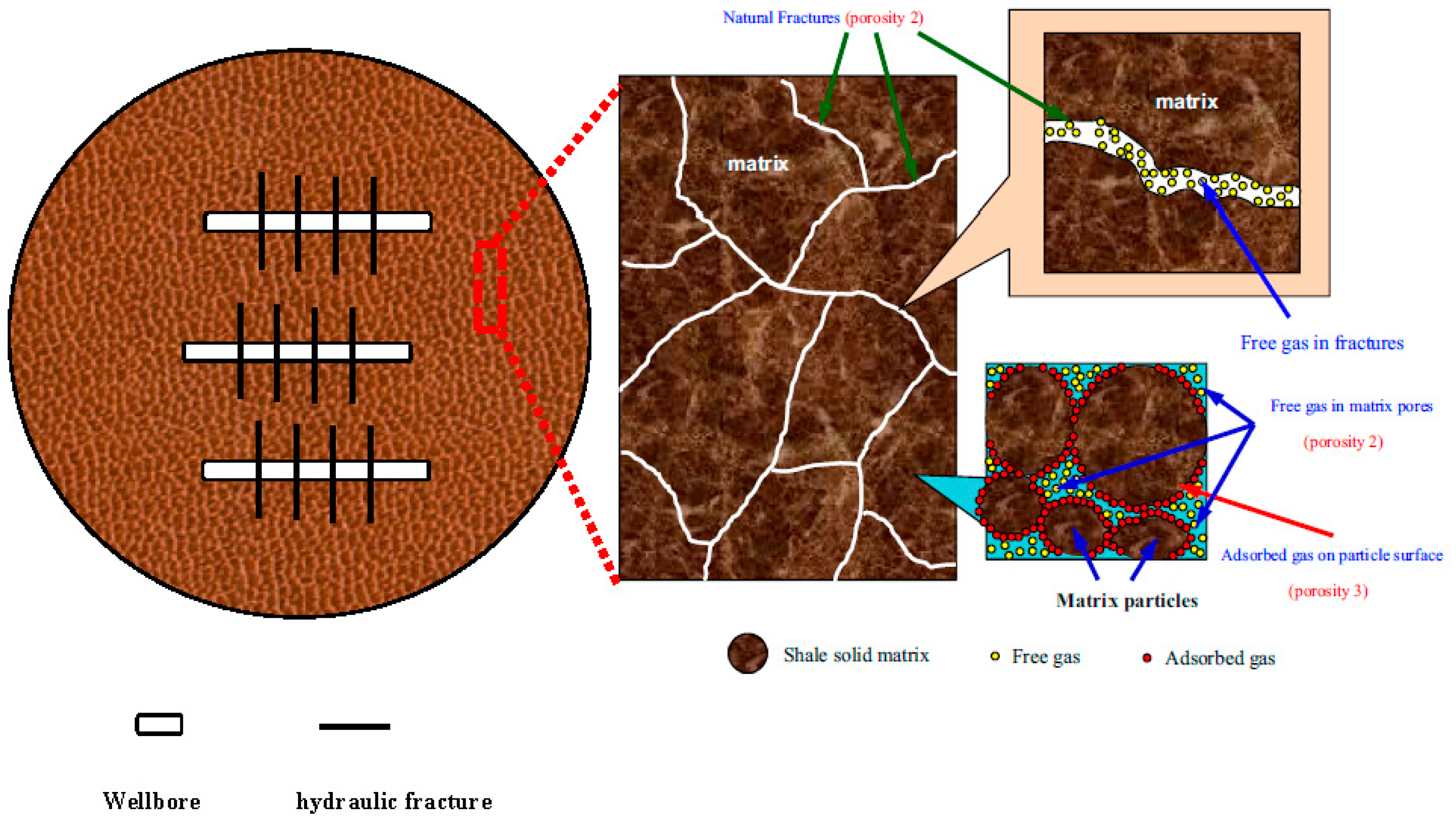
2.1.2. SG Formation Model

The conceptual model of a SG formation can be illustrated as in Figure 3. The reservoir, within infinite boundary, is treated as 2D due to the fact that the reservoir thickness is far smaller than its radius. A SG reservoir is assumed to be isotropic, including a natural fracture system and a matrix system, and bounded by upper and lower impermeable strata. Three media exist in the SG reservoir: (1) the lowest permeable shale matrix distributed in the reservoir; (2) the moderate permeable NFs in the reservoir; and (3) the highest permeable HFs, perpendicular to the wellbore.

To conveniently describe our methodology, we chose three SMFHWs to be our research objective. However, our methodology could be easily extended to a MWPs scheme with more than three wells. Three SMFHWs were produced at constant gas rates q₁, q₂, and q₃. CO₂ in HFs flows into a shale play at flow-rate strength q_f. To conveniently derive a pressure-buildup model, some other assumptions were as follows:

• Three horizontal wells were intercepted by several symmetric HFs. The fractures were assumed to fully penetrate the SG reservoir.
• A SG reservoir has uniform thickness h. Depleted pressure and temperature are P_d and T.
• CO₂ seepage within a natural fracture system obeyed Darcy’s law. The shape of the matrix was simplified as a sphere and CO₂ flow within the matrix was assumed to obey Fick’s first law. The natural fracture system was stress-dependent with initial permeability k_ri.
• The compressibility coefficient of the slightly compressible CO₂ was constant;
• The impacts of gravity and capillary pressure were neglected.
• CO₂ absorption and adsorption meet the Langmuir isotherm equation.
• Wellbore storage and skin factor can be considered.
• No frictional pressure loss inside the wellbore was considered.
• Because of the lack of supercritical adsorption data, CO₂ was assumed to be persistently in the gas phase during the injection, with a constant Z-factor and viscosity. CO₂ leakage is assumed when CO₂ is injected into a depleted SG reservoir.

2.2. Mathematical Model

2.2.1. Hydraulic Fracture Discrete

As one can see in Figure 4, the properties of hydraulic fracture for Well 1 include fracture permeability, k_f1; fracture width, w_f1; and fracture half-length, L_f1. The properties of hydraulic fracture for Well 2 include fracture permeability, k_f2; fracture width, w_f2; and fracture half-length, L_f2. The properties of hydraulic fracture for Well 3 include fracture permeability, k_f3; fracture width, w_f3; and fracture half-length, L_f3. The distances between two of these three wells were L_w12 and L_w32. The fracture distance between two of these three wells was L_f12 and L_f32. To establish a mathematical model, we discretized a hydraulic fracture system of the MWPs scheme. We assumed that one hydraulic fracture of Well 1, Well 2, and Well 3 was divided into N₁, N₂, and N₃ sub-fracture segments, respectively. The hydraulic fracture numbers of Well 1, Well 2, and Well 3 were M₁, M₂, and M₃. The length of each fracture segment for Well 1, Well 2, and Well 3 can be presented as ∆L_f1, ∆L_f2, and ∆L_f3, respectively. After that, each sub-fracture segment was considered as an independent fracture unit, and the pressure responses caused by these fracture segments are calculated.

2.2.2. Model of CO₂ Flow in SG Reservoir System

To develop mathematical models in a SG reservoir, the formulae of the NFs system and matrix system can be established separately and then dynamically coupled [19,20,22]. Therefore, in a Laplace domain, the dimensionless governing equations with consideration of stress sensitivity and pseudo-steady diffusion can be presented as follows (more information on dimensionless definition and model development are provided in Appendix A and Appendix B):

$$\frac{{\mathrm{d}}^2\overline{{\eta }_D}}{\mathrm{d}{r_D}^2}+\frac{1}{r_D}\frac{\mathrm{d}\overline{{\eta }_D}}{\mathrm{d}{r}_D}=\omega u\overline{{\eta }_D}+(1-\omega )u\overline{V_D}$$

(1)

$$u\overline{V_D}=\lambda (\overline{V_{ED}}-\overline{V_D})$$

(2)

Dimensionless boundary conditions:

$$\overline{{\eta }_D}(r_D\to \infty ,u)=0$$

(3)

$$r_D\frac{\mathrm{d}\overline{{\eta }_D}}{\mathrm{d}{r}_D}{|}_{r_D\to 0}=-\overline{q_D}$$

(4)

Substituting Equation (2) into Equation (1), one can get the following governing equation combining the natural fracture system and the matrix system:

$$\frac{{\mathrm{d}}^2\overline{{\eta }_D}}{\mathrm{d}{r_D}^2}+\frac{1}{r_D}\frac{\mathrm{d}\overline{{\eta }_D}}{\mathrm{d}{r}_D}=f(u)\overline{{\eta }_D}$$

(5)

Equations (3)–(5) comprise a comprehensive mathematical model in a SG reservoir coupling the NFs system and the matrix system. The typical solution of the models has been given in the literature [19,22] and is also in Appendix B. Based on the solution, by applying the principle of integration, the pressure distribution of a random position (x_D, y_D) caused by one fracture segment (x_WD, y_WD) is given by the following equation:

$$\overline{{\psi }_{uD}}(u;x_D,y_D;x_{wD},y_{wD})=\frac{\overline{q_D}}{\Delta L_{fiD}}{\displaystyle {\int }_{x_{wD}-\Delta L_{fiD}/2}^{x_{wD}+\Delta L_{fiD}/2}{K}_0[\sqrt{f(u)}\sqrt{{(x_D-v)}^2+{(y_D-y_{wD})}^2}]\mathrm{d}v},i=1,2$$

(6)

where:

$$\gamma =\frac{3.684\times {10}^{-3}{P}_{sc}{q}_{sc}T}{k_{r}h{T}_{sc}}\frac{{\psi }_L{V}_L}{{({\psi }_L+{\psi }_i)}^2},\lambda =\frac{{\mathsf{\pi }}^{2}D\mu \Lambda {L_{ref}}^2}{3.6k_r{R_m}^2}$$

2.2.3. Model of CO₂ Flow in a HF System

As described by some previous researchers [21,28,29,30], the CO₂ flow within a hydraulic fracture system was assumed to be compressible and can be described as a 1D coordinate system (Figure 5). CO₂ flow within hydraulic fracture also obeys Darcy’s law. As seen in Figure 2b and Figure 5, the interaction of hydraulic fractures between two wells for the MWPs scheme can be classified into two scenarios: with direct connection and without direct connection.

With the definition of dimensionless variables mentioned in Appendix A, the flow equations with the dimensionless formula can be presented as follows (the model derivation can be found in Appendix C):

$$\frac{{\partial }^2{\psi }_{fD}}{\partial {l_D}^2}-\frac{2\pi q_{fD}(l_D)}{C_{fD}(l_D)}=\frac{1}{C_{\eta D}}\frac{\partial {\psi }_{fD}}{\partial t_D}$$

(7)

At initial condition,

$${\psi }_{fD}{|}_{t_D=0}=0$$

(8)

When hydraulic fractures are not in direct connection between two wells, with the inner boundary in contact with the wellbore,

$$\frac{\partial {\psi }_{fD}}{\partial l_D}{|}_{l_D=0}=\frac{q_{cD}(0)}{C_{fD}}$$

(9)

The outer boundary condition can be presented as follows because of no flow transportation at the tip of the fracture:

$$\frac{\partial {\psi }_{fD}}{\partial l_D}{|}_{l_D=L_{fD}}=0$$

(10)

When hydraulic fractures are a direct connection between two wells, the two tips of hydraulic fracture separately make contact with Well 1 and Well 2, thus giving

$$\frac{\partial P_{fD}}{\partial l_D}{|}_{l_D=0}=\frac{q_{cD1}(0)}{C_{f1D}}$$

(11)

$$\frac{\partial P_{fD}}{\partial l_D}{|}_{l_D=L_{f1D}+L_{f2D}}=\frac{q_{cD2}(0)}{C_{f2D}}$$

(12)

2.3. Solution of Pressure-Buildup for MWPs Scheme

2.3.1. SG Reservoir System

Traditionally, we employed the superposition principle [31] to calculate the pressure response for SMFHWs. Similarly, we also can apply the superposition principle to the MWPs scheme. On the basis of Equation (6), we can obtain the transient pressure response at the center of each segment of the HFs system in the MWPs scheme with the superposition principle:

$$\begin{array}{l}\overline{{\eta }_D}(x_{Do},y_{Do})={\displaystyle \sum _{a=1}^{M_1}{\displaystyle \sum _{b=j}^{N_1}\frac{\overline{q_{fi,jD}}}{\Delta L_{f1D}}}\overline{{\eta }_{uD}}(x_{Do},y_{Do};x_{Da,b},y_{Da,b})}+{\displaystyle \sum _{i=1}^{M_2}{\displaystyle \sum _{j=1}^{N_2}\frac{\overline{q_{fi,jD}}}{\Delta L_{f2D}}}\overline{{\eta }_{uD}}(x_{Do},y_{Do};x_{Di,j},y_{Di,j})}\\ +{\displaystyle \sum _{c=1}^{M_3}{\displaystyle \sum _{d=1}^{N_3}\frac{\overline{q_{fc,dD}}}{\Delta L_{f3D}}}\overline{{\eta }_{uD}}(x_{Do},y_{Do};x_{Dc,d},y_{Dc,d})},o=1,.....,(M_1\cdot N_1+M_2\cdot N_2+M_3\cdot N_3)\end{array}$$

(13)

where

$$\overline{{\eta }_{uD}}(x_{Do},y_{Do};x_{Da,b},y_{Da,b})={\displaystyle {\int }_{x_{Da,b}-\Delta L_{f1D}/2}^{x_{Da,b}+\Delta L_{f1D}/2}{K}_0[\sqrt{s}\sqrt{{(x_{Do}-u)}^2+{(y_{Do}-y_{Da,b})}^2}]\mathrm{d}u}$$

(14)

$$\overline{{\eta }_{uD}}(x_{Do},y_{Do};x_{Di,j},y_{Di,j})={\displaystyle {\int }_{x_{Di,j}-\Delta L_{f2D}/2}^{x_{Di,j}+\Delta L_{f2D}/2}{K}_0[\sqrt{s}\sqrt{{(x_{Do}-u)}^2+{(y_{Do}-y_{Di,j})}^2}]\mathrm{d}u}$$

(15)

$$\overline{{\eta }_{uD}}(x_{Do},y_{Do};x_{Dc,d},y_{Dc,d})={\displaystyle {\int }_{x_{Dc,d}-\Delta L_{f3D}/2}^{x_{Dc,d}+\Delta L_{f3D}/2}{K}_0[\sqrt{s}\sqrt{{(x_{Do}-u)}^2+{(y_{Do}-y_{Dc,d})}^2}]\mathrm{d}u}.$$

(16)

$\overline{{\eta }_{uD}}(x_{Do},y_{Do};x_{Da,b},y_{Da,b})$ is the pressure response at the o-th fracture segment, caused by the flux of the b-th fracture segment in the a-th hydraulic fracture for Well 1; $\overline{{\eta }_{uD}}(x_{Do},y_{Do};x_{Di,j},y_{Di,j})$ is the pressure response at the o-th fracture segment, caused by the flux of the j-th fracture segment in the i-th hydraulic fracture for Well 2; $\overline{{\eta }_{uD}}(x_{Do},y_{Do};x_{Dc,d},y_{Dc,d})$ is the pressure response at the o-th fracture segment, caused by the flux of the d-th fracture segment in the c-th hydraulic fracture for Well 3.

2.3.2. Hydraulic Fracture System

In this section, we will describe the later issue about fluid transferring at the interacting point between the hydraulic fracture and the natural fracture. Due to the possible connection of the HFs system, it is a key point when dealing with the fluid transferring between those two fracture media. Zhou et al. [30] and Chen et al. [21] artificially determined the flow direction, while Jia et al. [29] employed complex star-transformation to automatically determine the flow direction. In our work, the connecting HFs system is simple (sparse hydraulic fractures), therefore we artificially specify the flow direction and solve the issue of fluid transferring between those two fracture media.

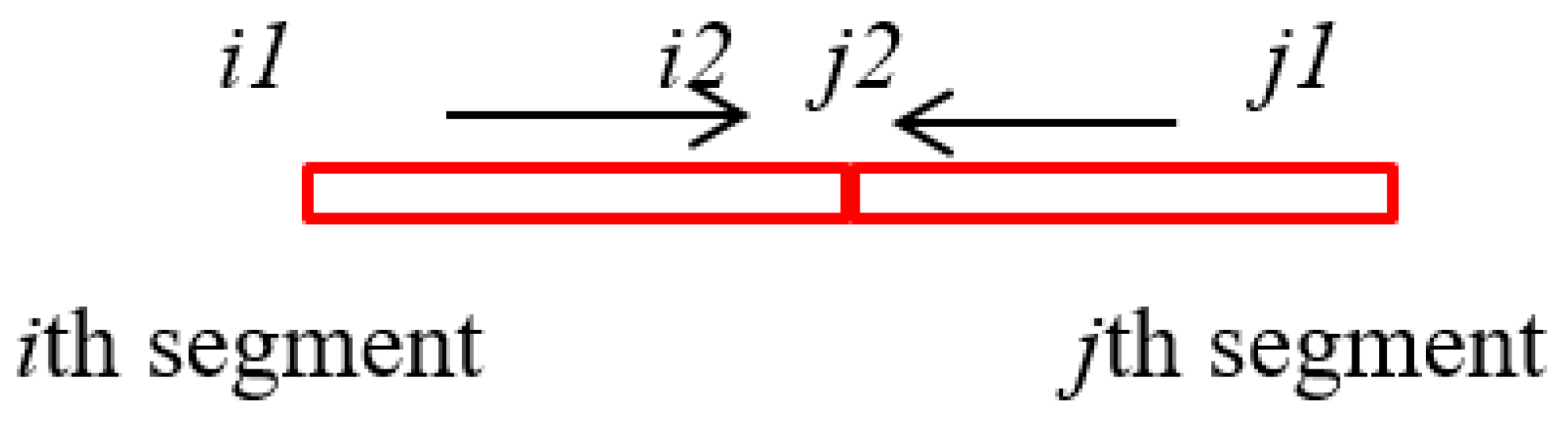
Independent Fracture Segment

Here, a semi-analytical method [21,28,30,32] is applied to solve models of fracture systems considering finite hydraulic fracture conductivity. Zeng et al. [28] proposed a semi-analytical method that divided the fracture system into several segments; each segment was solved analytically. As one can see in Figure 6, we chose the ith fracture segment to analyze the flow equation. The fluid flow rate from position $l_{D1}$ to $l_{D2}$, because of the fluid supplement of segment flux q_fi, increases from q_ci1 to q_ci2 along $l$.

We take hydraulic fracture as an example, and the solution for a natural fracture is similar. Therefore, based on Equation (7), the governing equation of the ith fracture segment can be established as:

$$\frac{{\mathrm{d}}^2\overline{{\psi }_{fD}}}{\mathrm{d}{l_D}^2}-\frac{2\mathsf{\pi }}{C_{fD}}\cdot \overline{q_{ciD}}=\frac{u}{C_{\eta D}}\overline{{\psi }_{fD}}$$

(17)

and boundary conditions at ${\epsilon }_{\mathrm{i}1}$ and ${\epsilon }_{\mathrm{i}1}$ given by

$$\frac{\mathrm{d}\overline{{\psi }_{fD}}}{\mathrm{d}{l}_D}{|}_{l_D=l_{i1D}}=\frac{2\mathsf{\pi }}{C_{fD}}\overline{q_{cD}(l_{i1D})}$$

(18)

$$\frac{\mathrm{d}\overline{{\psi }_{fD}}}{\mathrm{d}{l}_D}{|}_{l_D=l_{i2D}}=\frac{2\mathsf{\pi }}{C_{fD}}\overline{q_{cD}(l_{i2D})}$$

(19)

Combining with Equations (17)–(19), and integrating with the fracture segment, we can obtain the pressure distribution within the ith fracture segment. The method proposed by Zeng et al. [28] is applied to obtain an analytical solution in this fracture segment. The solution in Laplace space for the ith fracture segment is a function of the flow rate at both sides of the fracture segment and the fluid influx from the SG reservoir:

$$\overline{{\psi }_{fiD}}(l_D,u)=b_i(l_D)\overline{q_{cDi1}}+c_i(l_D)\overline{q_{cDi2}}+d_i\overline{q_{fDi}}$$

(20)

Therefore, the pressure at the center of the ith fracture segment is

$$\overline{{\psi }_{fiD}}(\frac{l_{Di1}+l_{Di2}}{2},u)=b_i(\frac{l_{Di1}+l_{Di2}}{2})\overline{q_{cDi1}}+c_i(\frac{l_{Di1}+l_{Di2}}{2})\overline{q_{cDi2}}+d_i\overline{q_{fDi}}$$

(21)

where

$$b_i(l_D)=\frac{2\mathsf{\pi }}{C_{fD}\sqrt{u/C_{\eta D}}}\left\{\frac{2\cosh [(l_D-l_{i1D})\sqrt{u/C_{\eta D}}]}{e^{2(l_{i2D}-l_{i1D})\sqrt{u/C_{\eta D}}}-1}+e^{-(l_D-l_{i1D})\sqrt{u/C_{\eta D}}}\right\}$$

(22)

$$c_i(l_D)=\frac{-2\mathsf{\pi }}{C_{fD}\sqrt{u/C_{\eta D}}}\left\{\frac{2\cosh [(l_{i2D}-l_D)\sqrt{u/C_{\eta D}}]}{e^{2(l_{i2D}-l_{i1D})\sqrt{u/C_{\eta D}}}-1}+e^{-(l_{i2D}-l_D)\sqrt{u/C_{\eta D}}}\right\}$$

(23)

$$d_i(l_D)=\frac{-2\mathsf{\pi }{C}_{\eta D}}{C_{fD}u}$$

(24)

Connecting Fracture Segment

As illustrated in Figure 5b, if the hydraulic fractures of two wells interact with each other, we need to pay special attention to the mass balance at the connecting point. As illustrated by Zhou et al. [30] in Figure 7, due to the relatively small size of the interacting point, the fluid accumulation can be ignored and therefore the inflow should be equal to the outflow. In this work, we consider a simple fracture system, so it was suitable to determine the flow direction artificially. For the vertex, in conditions of mass conservation and pressure continuity, we can obtain

$$\overline{q_{cDi2}}+\overline{q_{cDj2}}=0$$

(25)

$$\overline{{\psi }_{fi2D}}=\overline{{\psi }_{fj2D}}$$

(26)

2.3.3. Solution Methodology

From the proposed equations, we see that there are three unknowns, $\overline{P_{\mathrm{f}Di1}}$, $\overline{q_{cDi1}}$, and $\overline{q_{\mathrm{f}Di}}$, for each fracture segment. Therefore, the total number of unknowns is equal to [3(M₁ × N₁ + M₂ × N₂ + M₃ × N₃) + 3]. We can obtain a closed [3(M₁ × N₁ + M₂ × N₂) + 3]-order matrix with the following conditions:

Combining Equation (13) with Equation (21), the pressure at the center of fracture area is continuity:

$$\overline{{\psi }_D}(x_{Di},y_{Di})=\overline{{\psi }_{fiD}}$$

(27)

After rewriting Equation (27) for every fracture segment of the MWPs scheme, we can obtain a (M₁ × N₁+ M₂ × N₂ + M₃ × N₃)-order matrix.

For two connecting fracture segments, the ith segment and (i + 1)th segment, the pressure and fluid rate of connecting point are equal to each other, namely,

$$q_{cDi2}=q_{cD(i+1)1}$$

(28)

$$b_i(l_{i1D})\overline{q_{cDi1}}+c_i(l_{i2D})\overline{q_{cDi2}}+d_i\overline{q_{fiD}}=\overline{{\psi }_{f(i+1)D}}(l_{(i+1)1D},u)$$

(29)

For the connecting vertex, Equation (25) can describe the mass conservation of this point. At the same time, considering the boundary condition of HFs, after rewriting Equations (25) and (26) for every fracture segment, we obtain two more (M₁ × N₁ + M₂ × N₂ + M₃ × N₃)-equations.

Another three equations were required to form a closed matrix. Well 1, Well 2, and Well 3 produced at the constant rates of q₁, q₂, and q₃, respectively. Here, we define three new variables, ε₁, ε₂, and ε₃, representing the ratio between q₁, q₂, q₃ and (q₁ + q₂ + q₃). Namely, ${\epsilon }_1={{\displaystyle q}}_1/{\displaystyle \sum _{i=1}^3{{\displaystyle \mathrm{q}}}_{\mathrm{i}}}$, ${\epsilon }_2={{\displaystyle q}}_2/{\displaystyle \sum _{i=1}^3{{\displaystyle \mathrm{q}}}_{\mathrm{i}}}$, and ${\epsilon }_3={{\displaystyle q}}_3/{\displaystyle \sum _{i=1}^3{{\displaystyle \mathrm{q}}}_{\mathrm{i}}}$. Therefore, the remaining three equations referring to the rate conversion of the three wells are as follows:

$${\displaystyle \sum _{i=1}^{M_1}\overline{q_{cDi}(0)}}=\frac{{\epsilon }_1}{s}$$

(30)

$${\displaystyle \sum _{i=1}^{M_2}\overline{q_{cDi}(0)}}=\frac{{\epsilon }_2}{s}$$

(31)

$${\displaystyle \sum _{i=1}^{M_3}\overline{q_{cDi}(0)}}=\frac{{\epsilon }_3}{s}$$

(32)

Finally, we can form a closed [3(M₁ × N₁ + M₂ × N₂ + M₃ × N₃) + 3]-order matrix. By applying Gaussian elimination and a Stehfest numerical algorithm, the pressure transient solution, $\overline{{\eta }_{Dwf1}}$, and $\overline{{\eta }_{Dwf2}}$ of the mathematical model can be solved. Using the Stehfest numerical invention algorithm [33] we can obtain the solution in real space, namely by converting $\overline{{\eta }_{Wfin}}\to {\eta }_{Wfin}$. One can get the bottom-hole pressure for the MWPs scheme in a SG reservoir by taking the stress sensitivity into consideration:

$${\psi }_{WfD1}=-\frac{1}{{\zeta }_D}\ln (1-{\zeta }_D{\eta }_{Dwfin1})$$

(33)

$${\psi }_{wfD2}=-\frac{1}{{\zeta }_D}\ln (1-{\zeta }_D{\eta }_{Dwfin2})$$

(34)

$${\psi }_{wfD3}=-\frac{1}{{\zeta }_D}\ln (1-{\zeta }_D{\eta }_{Dwfin3})$$

(35)

3. Results and Discussion

In this part, four cases are used for (1) discretization of hydraulic fractures; (2) model verification; (3) calculation of CO₂ storage capacity for MWPs scheme; and (4) sensitivity analysis. The parameters of some cases are shown in

Table 1. The basic parameters for numerical simulation.

Type	Parameters	Value
Reservoir	Depleted SG reservoir pressure, Pd (MPa)	0.5
	Formation temperature , T (K)	320
	Formation thickness, h (m)	20
	Total compressibility of reservoir, Ct (MPa−1)	2.5 × 10−4
	Porosity of reservoir Φ (fraction)	0.06
	Reservoir area, (m × m)	1000 × 1000
	Initial SG reservoir permeability, kri (D)	0.001
	CO2 Langmuir pressure, PL (MPa)	5
	CO2 Langmuir volume, VL (sm3/m3)	6
	CO2 Gas diffusion coefficient, D (m2/s)	0.0001
	CO2 viscosity, μ (mPa·s)	0.01
	CO2 Z-factor, Z, fraction	0.8
Well 1	Hydraulic fracture permeability, kf1 (D)	10
	Hydraulic-fracture width, wf1 (m)	0.005
	Hydraulic-fracture half-length, Lf1 (m)	50
	Hydraulic-fracture number, M1	3
	Hydraulic-fracture porosity, Φf1 (fraction)	0.35
	Wellbore length, Lw1 (m)	1000
	Injection rate, q1 (m3/d)	50,000
Well 2	Hydraulic fracture permeability, kf2 (D)	10
	Hydraulic-fracture width, wf2 (m)	0.005
	Hydraulic-fracture half-length, Lf2 (m)	50
	Hydraulic-fracture number, M2	3
	Hydraulic-fracture porosity, Φf2 (fraction)	0.35
	Wellbore length, Lw2 (m)	1000
	Injection rate, q2 (m3/d)	100,000
Well 3	Hydraulic fracture permeability, kf3(D)	10
	Hydraulic-fracture width, wf3 (m)	0.005
	Hydraulic-fracture half-length, Lf3 (m)	50
	Hydraulic-fracture number, M3	3
	Hydraulic-fracture porosity, Φf3 (fraction)	0.35
	Wellbore length, Lw3 (m)	1000
	Injection rate, q3 (m3/d)	150,000

. The detailed procedure can be presented as follows.

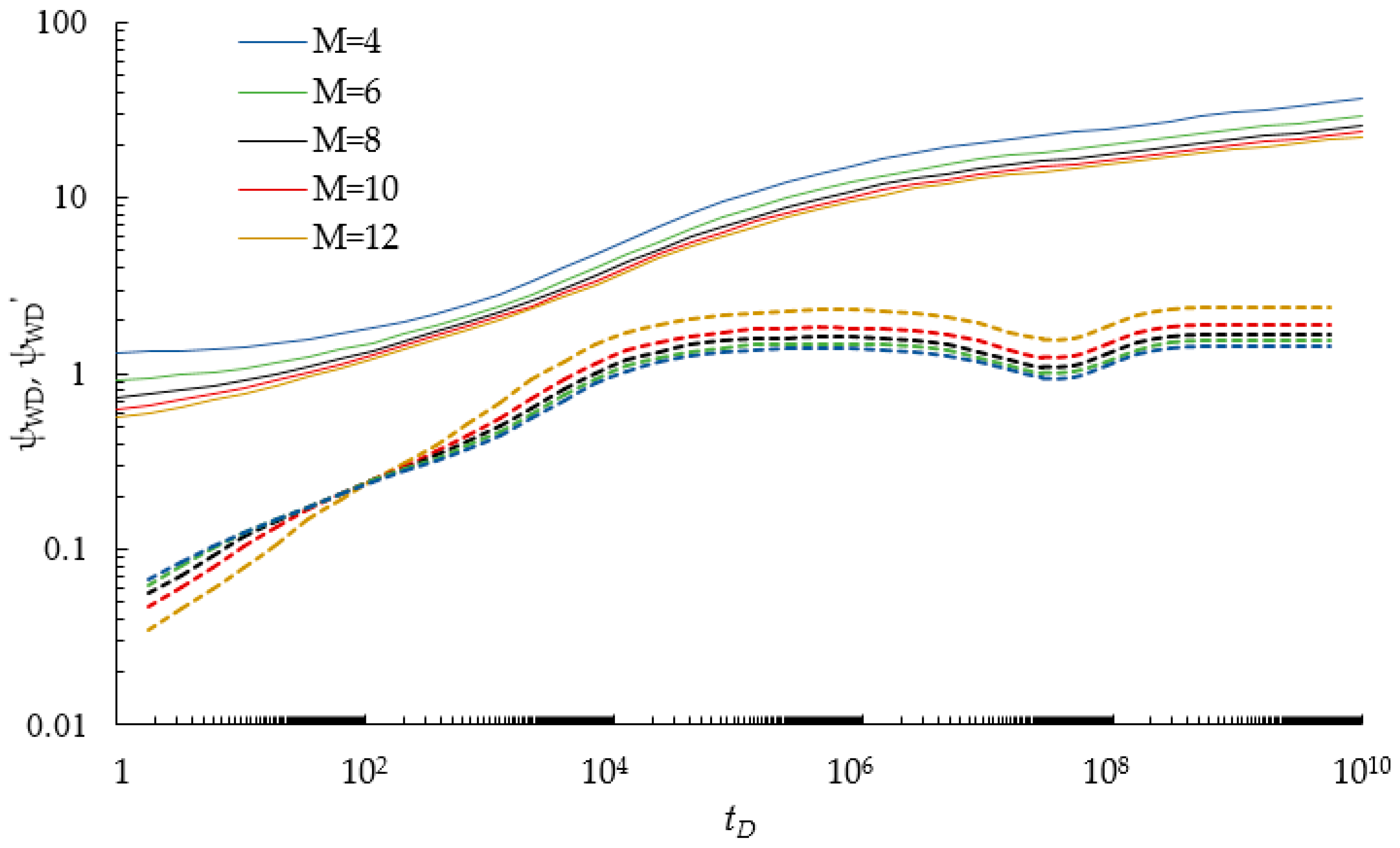
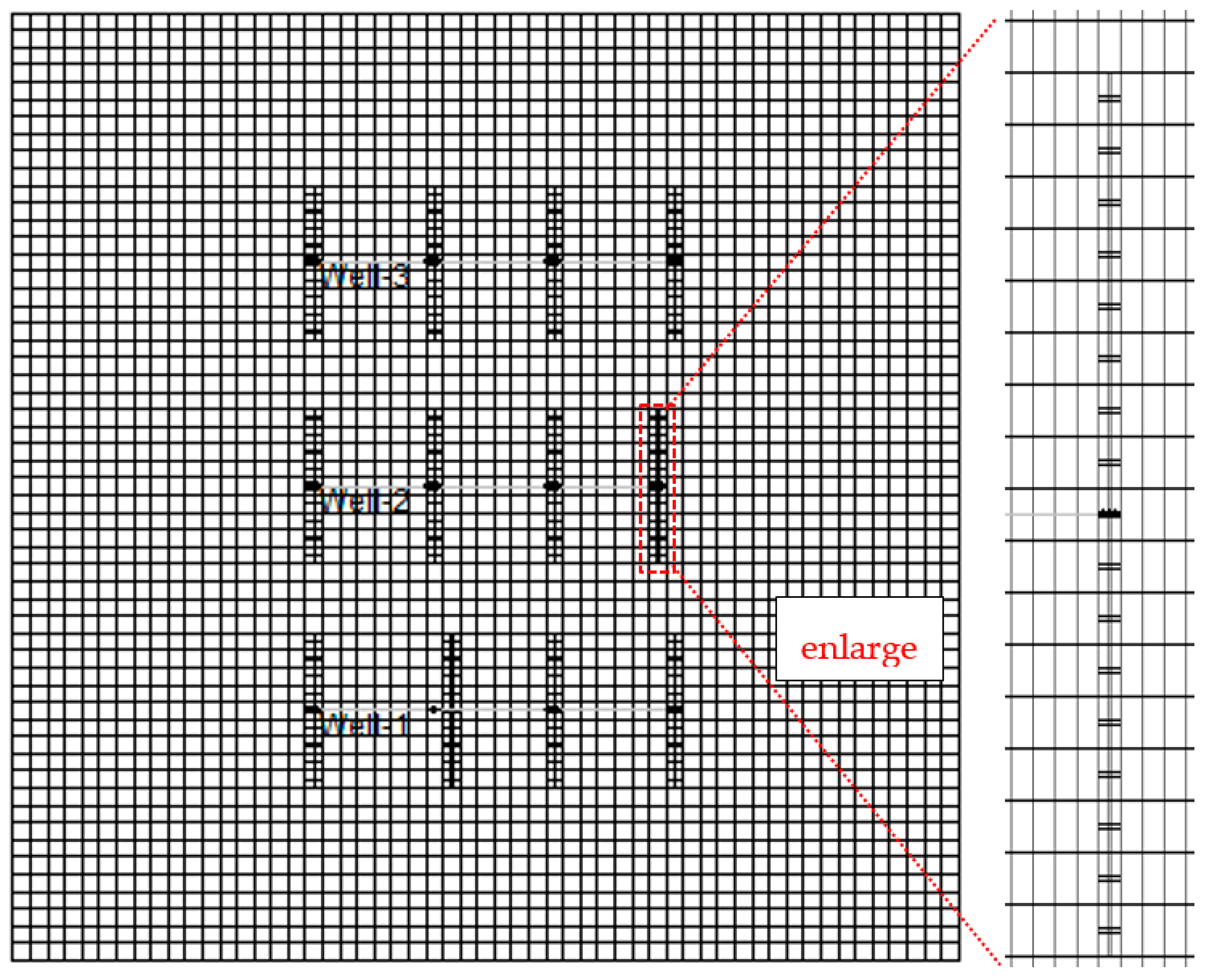
3.1. Discretization Level of Fracture System

Due to the inappropriate discretization of HFs, early transient pressure response can be distorted by artifacts of fracture subdivision [21]. Therefore, the accuracy of our approach partially depends on the discretization level of the fracture system. The basic dimensionless parameters are as follows: ζ_D = 0, λ = 0.002, γ = 0.15, ω = 0.0035, C_ηD = 10⁵, L_f1D = 200, L_f2D = 200, L_f3D = 200, L_w12D = 3000, L_w32D = 3000, L_f12D = 1000, L_f32D = 1000, C_f1D = 125, C_f2D = 125, and C_f3D = 125. Figure 8 shows the effects of a divided fracture number on the pressure-transient performance. In Figure 8, ψ_wD represents the dimensionless pseudo-pressure (DPP), dψ_wD/d(lnt_D) represents the derivative of dimensionless pseudo-pressure ψ_wD with respect to the natural logarithm of dimensionless time t_D. As we can see, when more than 10 fracture segments, M, are divided for each hydraulic fracture, the results do not change appreciably. Therefore, each fracture is divided into 10 segments in our work, including the model validation and sensitivity analysis.

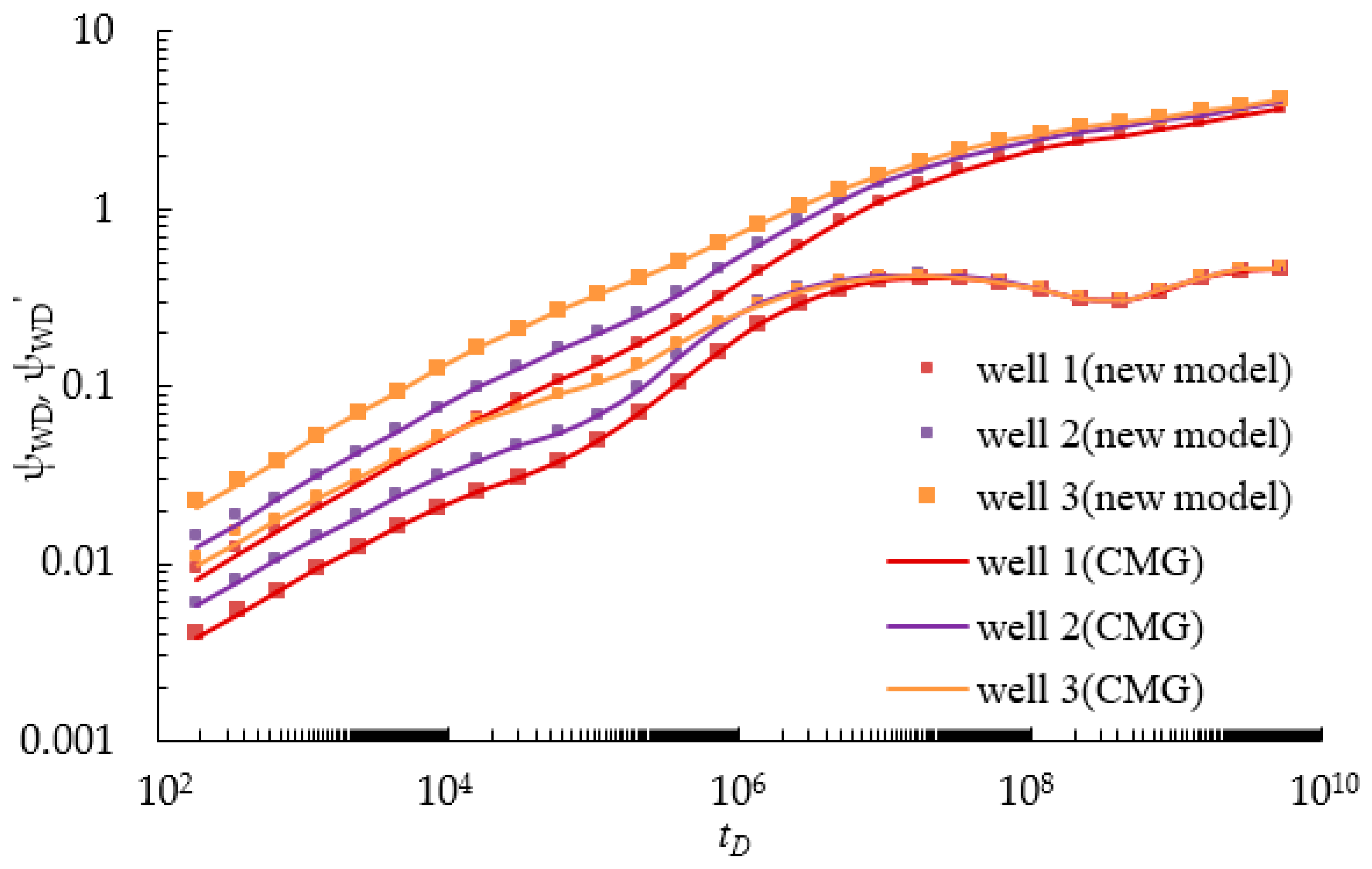
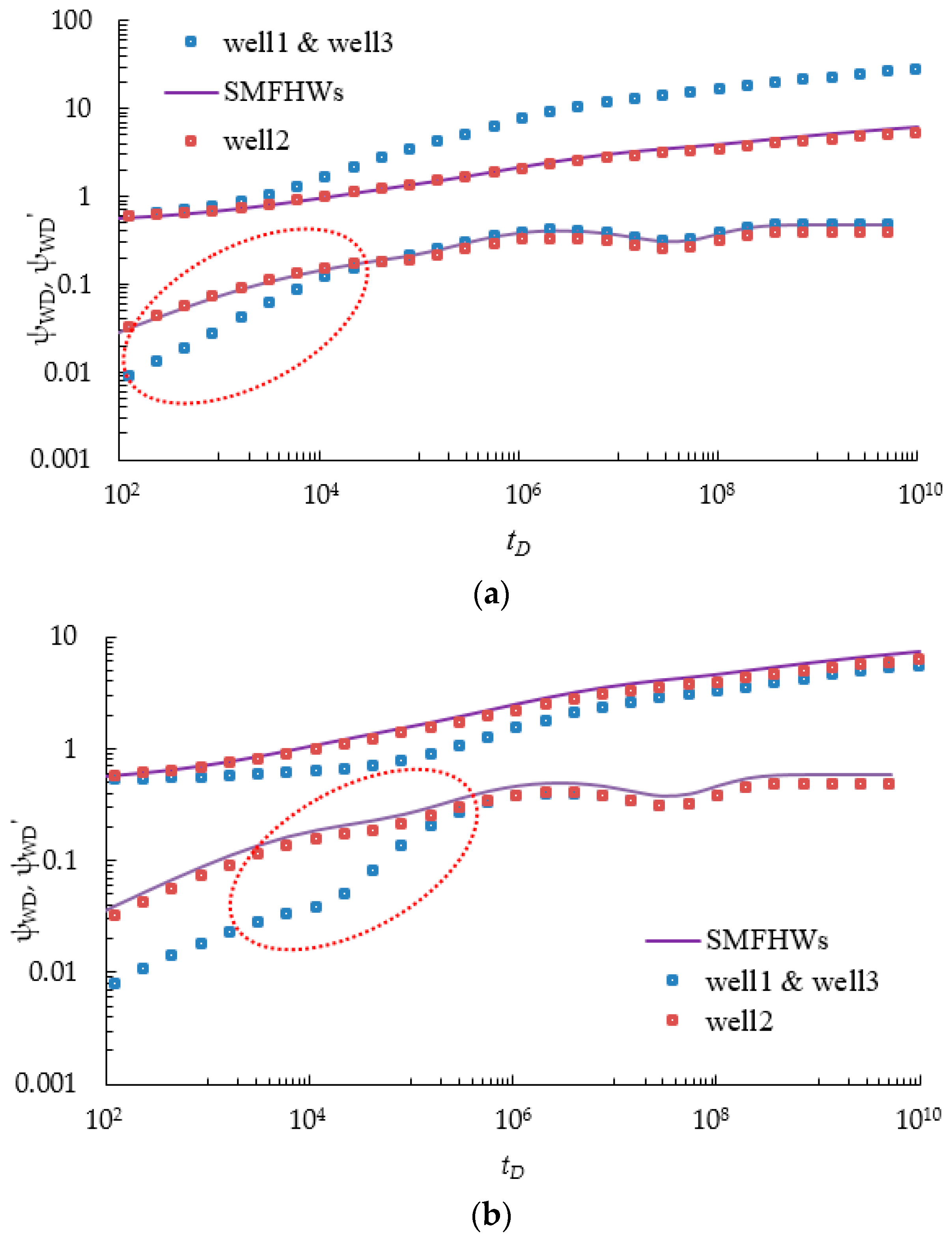
3.2. Model Validation

Currently, semi-analytical/analytical pressure-transient models for multi-wells have not existed. Therefore, Case II is also applied for numerical validation of the MWPs scheme. Case II is modeled by a commercial simulator, CMG-2015, and the top view is shown in Figure 9. The model considers SG reservoir to be a dual-porosity system and the CMG-GEM module is selected to simulate the proposed MWPs scheme. Seen in Figure 9, one representative segment is modeled that represents one part of the reservoir volume around a hydraulic fracture. This segment contains four hydraulic fractures orthogonal to the horizontal well at 250 m fracture spacing. This is a 2D model with 55 grid cells in the x-direction, 55 grid cells in y-direction, and only one grid cell in the z-direction. The multi-porosity model and multiple interacting continua (MINC) method are applied to subdivide the matrix so that the transient diffusion in the matrix can be simulated. It is characterized as an instant-desorption model. Double Klinkenberg permeability-Logarithmically Space-Local Grid Refinement (DK-LS-LGR) technology is employed to characterize a multi-stage hydraulic fractures system. Each hydraulic fracture is represented by a 3 × 3 locally refined grid 0.025 m wide. The ROCKTAB keyword is used to indicate the stress sensitivity. Furthermore, the keywords “DIFFCBM” and “LANGMEXT” are employed to describe the mechanisms of gas diffusion and adsorption. Then, the pressure solution of Case II is calculated under a constant production rate. We assume that q₁:q₂:q₃ = 2:3:5, and the fracture properties of the three wells are consistent. After that, the numerical solutions are compared with the semi-analytical results calculated in this paper. As we can see in Figure 10, there is a good agreement between our results and CMGs, which indicates that our model is reliable.

Besides the comparison of calculation accuracy, the speed of the computations for these two models was also investigated. With the same hardware platform, the CPU times are 285.2 s and 203.5 s for the semi-analytical and fully numerical model, respectively. Compared with the simulator, the computation performance presented is not significantly improved. We suggest that this comparison for the computation performance should be a rough evaluation due to the simple fracture configuration (perpendicular to wellbore), the relatively small total length of the fracture, and the implementation (programming with MATLAB codes without optimization for our model). The improvement may be encouraged by the fracture network with complex geometry or some programming techniques.

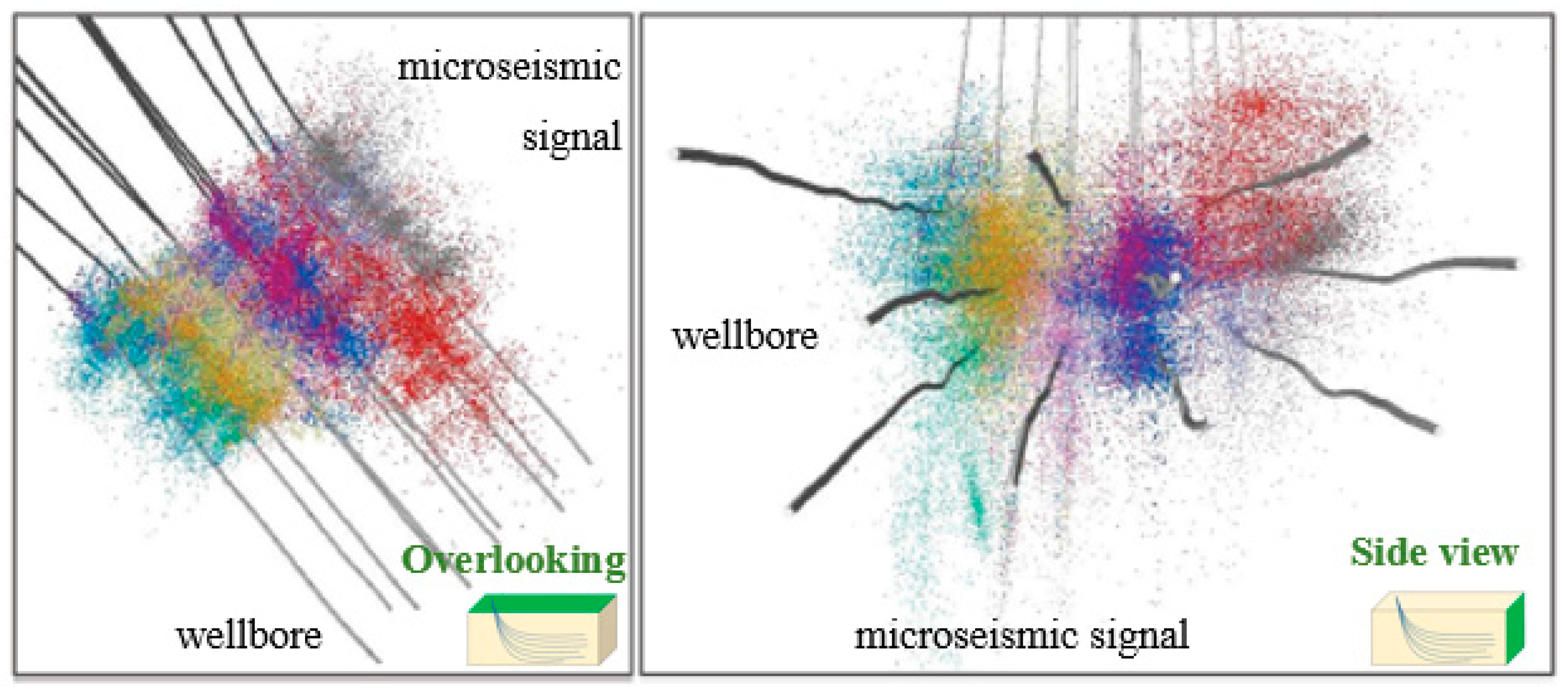
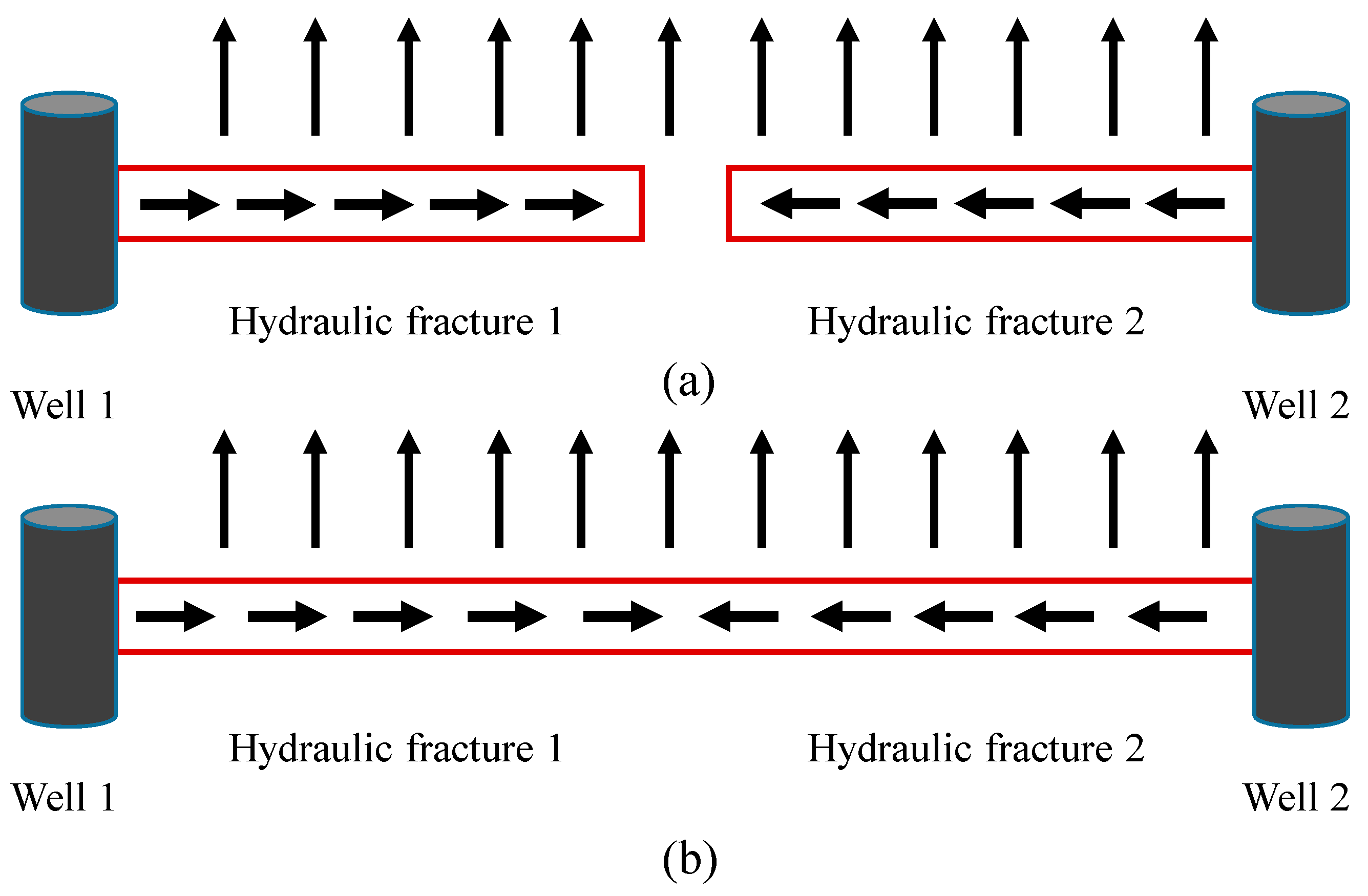
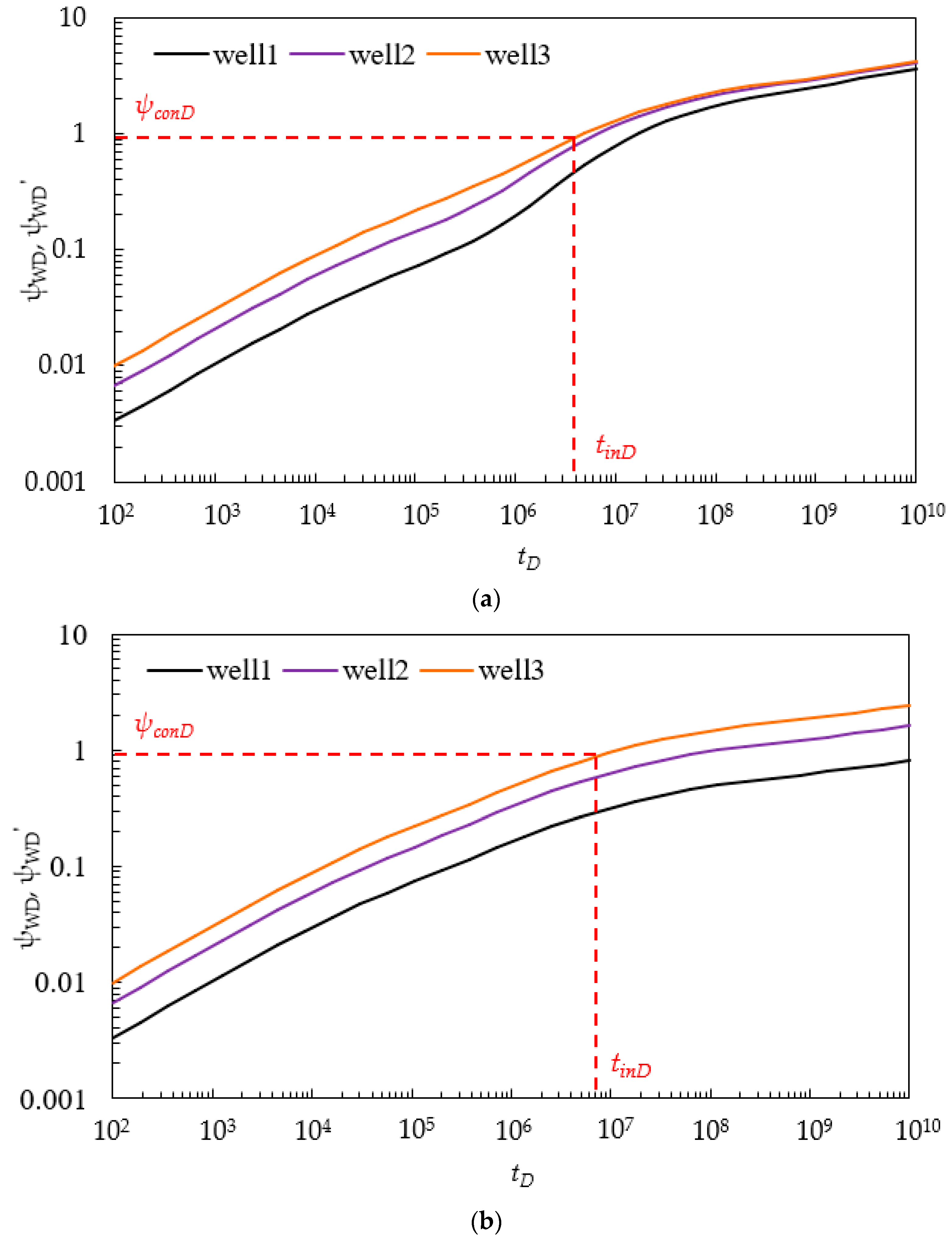
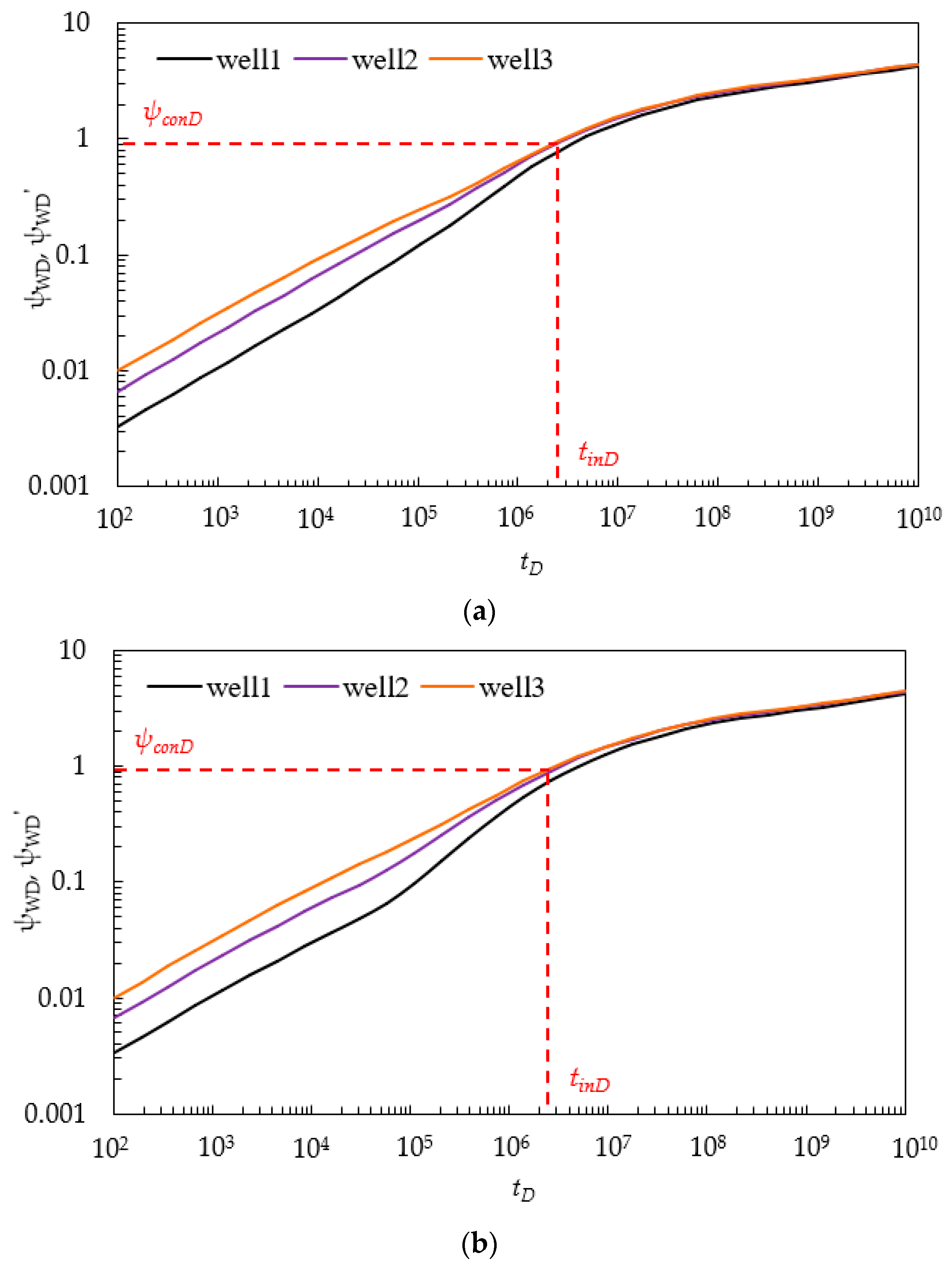
3.3. Multi-Well Pressure Interference

Due to the existence of MWPI, some expected flow regimes, such as fracture linear flow, fracture-formation bi-linear flow, and formation linear flow, are apparently distorted (Figure 11). The slope of type curves that characterize the linear or bi-linear flow regime is no longer equal to 0.5 or 0.25. To clearly describe the distortion of type curves, we will compare the characteristics of pressure curves without and with MWPI. We can realize this condition by shutting one well or setting the distance between two wells long enough, and therefore the MWPs scheme can be regarded as SMFHWs. In this part, we just give some proof that the MWPI is significant for the MWPs scheme, which cannot be ignored during CO₂ capture and storage. Some detailed information about how to quantitatively determine the MWPI time will be described in this part.

Enhancement of the possibility of MWPI induced by the MWPs scheme can stimulate the injection pressure to rapidly reach constraint pressure, which in turn sharply decreases the CO₂ injection capacity of MWPs. We also need to note that MWPI is different for different wells; as a result, different wells may reach the limited injection pressure at different times. Under this situation, part of wells will shut down, while the remaining wells will continue to inject CO₂. Thus, the virtual-well theory and pressure superposition method need to be employed to solve this problem. These issues are related to the optimization of a CO₂ multi-wells injection strategy about whether multi-wells inject CO₂ simultaneously or sequentially, which will also be considered in our Section 2. In that part, some additional mathematical derivations will be added.

Here, to conveniently implement the calculation of CO₂ storage capacity for the MWPs scheme, we make the assumption that all of the wells will shut down as long as one of them reaches the limited injection pressure.

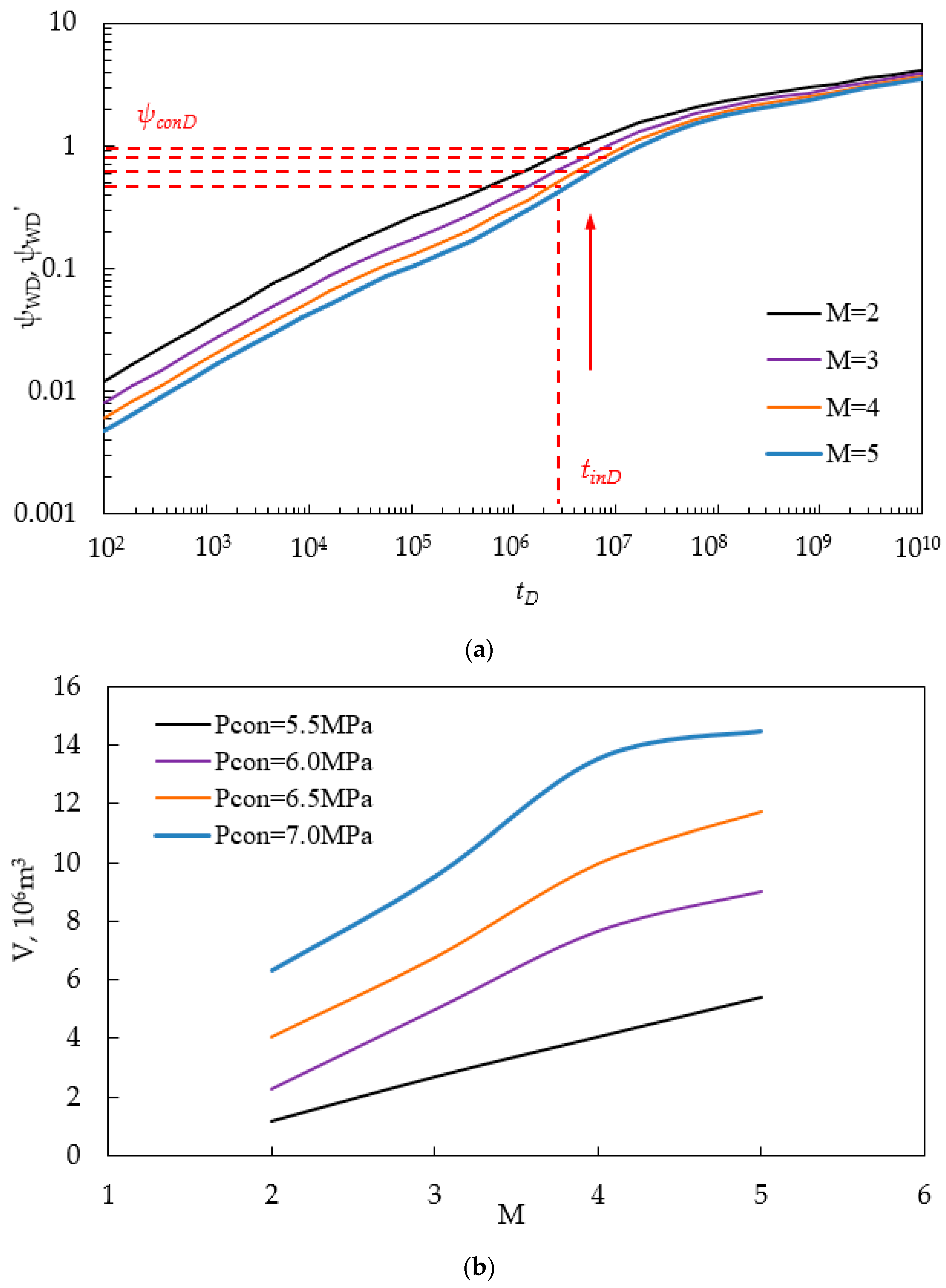
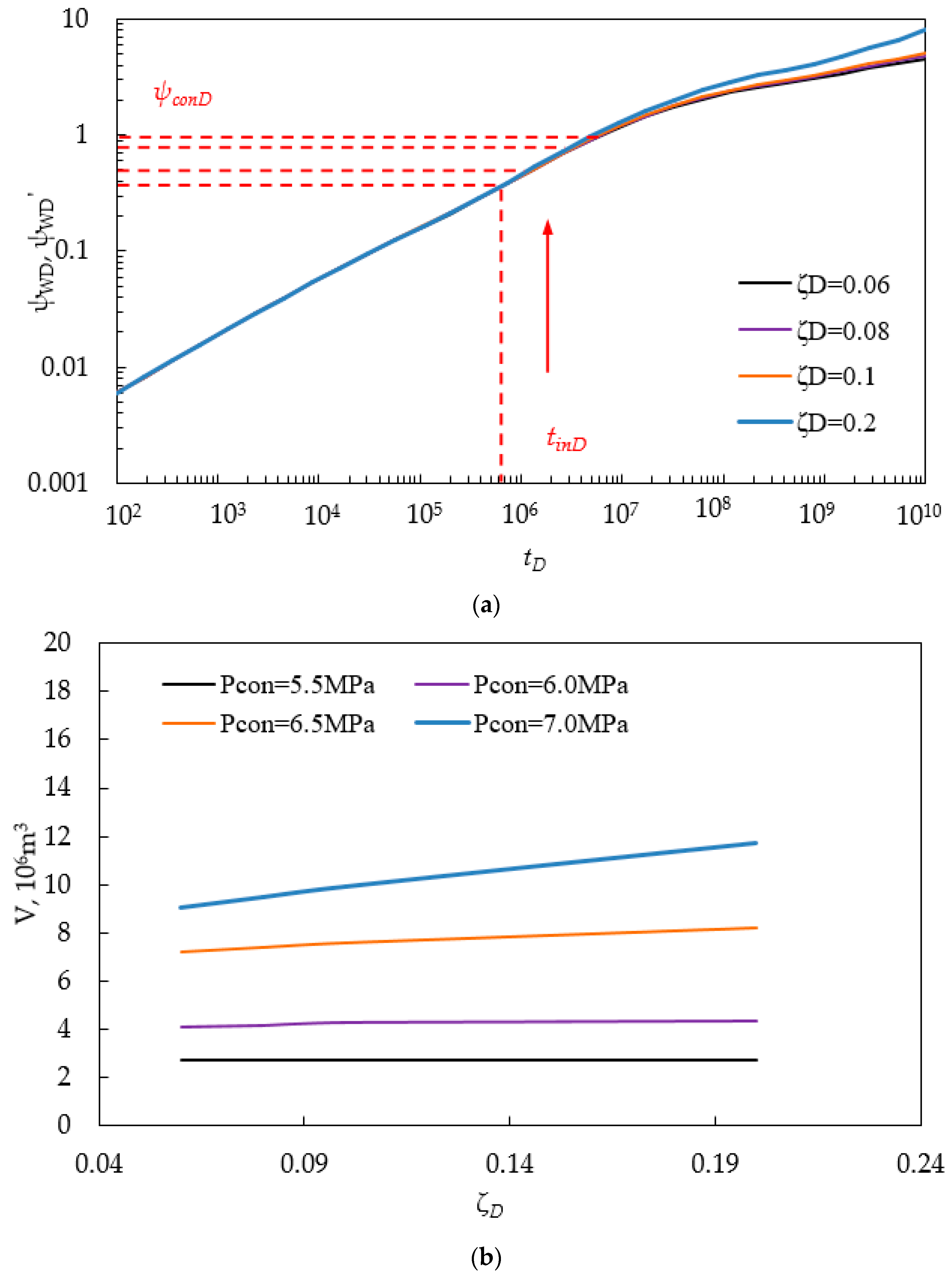
3.4. Calculation of CO₂ Storage Capacity

The main goal of our work is the calculation of the CO₂ storage capacity of the MWPs scheme in a depleted SG reservoir. Similar to the calculation method proposed by Chen et al. [21], which assumed that the limited injection pressure should be set to below the lithostatic pressure (8.55 MPa) and CO₂ supercritical pressure (which we set at 7.0 MPa, which is slightly lower than the supercritical pressure of 7.239 MPa).

Firstly, we specify the injection rate of each well, q_i, and calculate the ratio ε₁, ε₂, ε₃ between q₁, q₂, q₃ and (q₁ + q₂ + q₃). Based on the pressure transient solution in Equations (33)–(35), the pressure transient curves of the MWPs scheme can be obtained at a specific injection rate. We set a constrained injection pressure P_con, and then calculated the dimensionless constrained injection pressure ψ_conD. When downhole pressure of one well reaches the dimensionless constrained pressure ψ_conD, we can get the dimensionless injection time t_inD. Finally, we can calculate the injection time t_in; therefore, the total injected CO₂ volume will be:

$$V_{\mathrm{t}}={\displaystyle \sum _{i=1}^3{Q}_i}\times t_{in}$$

(36)

The parameters of the depleted SG reservoir, rock, wells, and CO₂ are shown in Table 1. Here, we take Figure 2b (3) as an example. L_w12 = L_w32 = 300, and L_f12 = L_f32 = 0. The calculation of the CO₂ storage capacity can be undertaken via the following steps:

(1)

Based on a specific injection rate, the ratio ε₁, ε₂, ε₃ between q₁, q₂, q₃ and (q₁ + q₂ + q₃) can be calculated as

$${\epsilon }_1=\frac{q_1}{{\displaystyle \sum _{i=1}^3{q}_i}}=\frac{1}{6},{\epsilon }_2=\frac{q_2}{{\displaystyle \sum _{i=1}^3{q}_i}}=\frac{1}{3},{\epsilon }_2=\frac{q_2}{{\displaystyle \sum _{i=1}^3{q}_i}}=\frac{1}{2}$$

(37)

(2)

Calculation of dimensionless limited injection pressure ${\psi }_{conD}$ with the dimensionless parameters and constrained injection pressure P_con:

$${\psi }_{conD}=\frac{k_{ri}{T}_{sc}h({P_{con}}^2-{P_d}^2)}{3.684\times {10}^{-3}\mu Z{p}_{sc}T{\displaystyle \sum _{i=1}^3{q}_i}}=\frac{0.001\times 293.15\times 20\times (7^2-{0.5}^2)}{3.684\times {10}^{-3}\times 0.01\times 0.8\times 0.101\times 3\times {10}^5\times 320}=0.9994$$

(38)

(3)

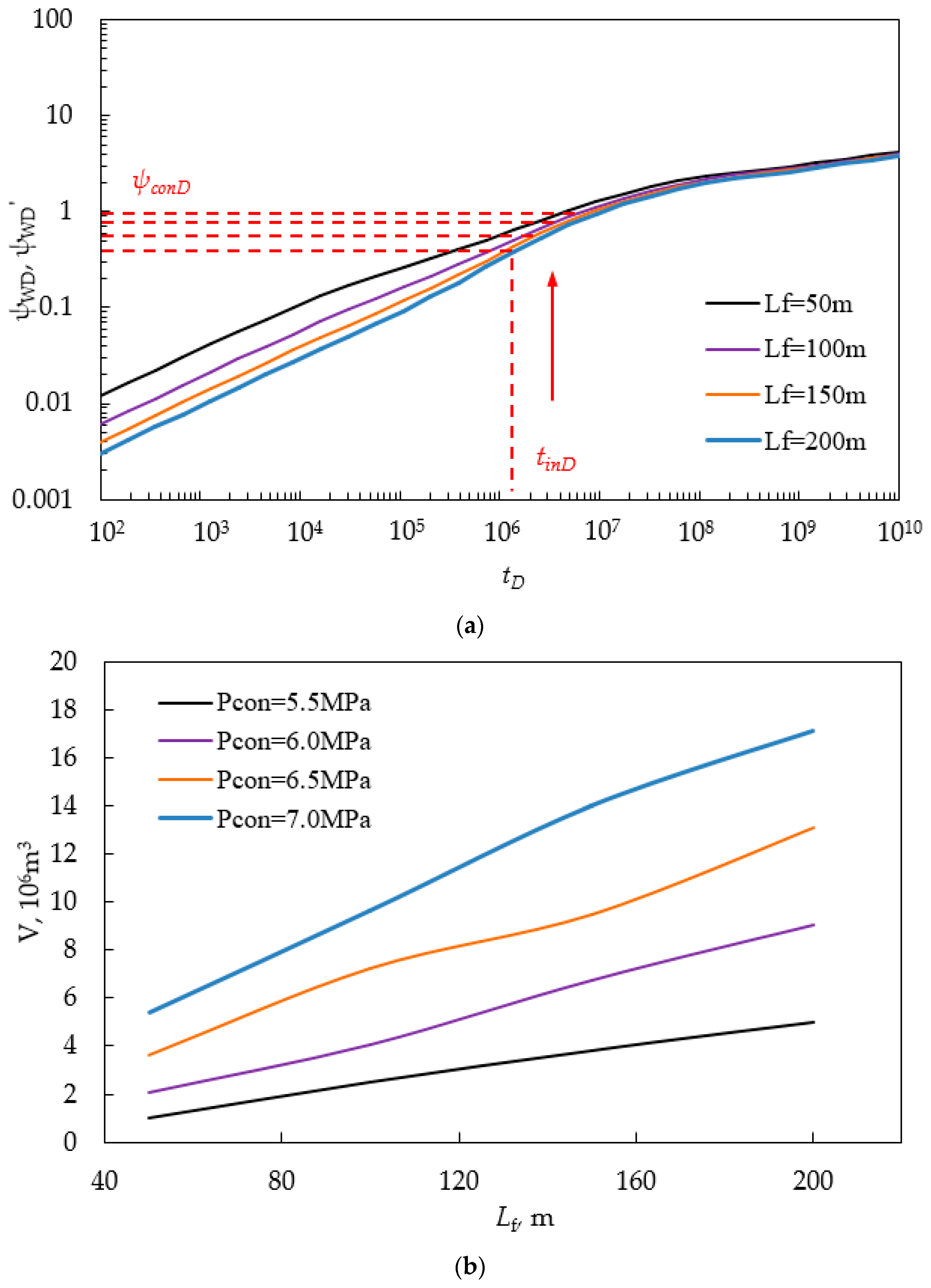
Based on the pressure curve in Figure 12, Well 3 reaches limited injection pressure ${\psi }_{conD}$. Thus, dimensionless injection time can be obtained as

$$t_{inD}=4.89\times {10}^6$$

(39)

(4)

Based on obtained injection time t_inD, total CO₂ injection volume can be calculated as follows:

$$V=\frac{\mu \Lambda {L_{ref}}^2}{3.6k_{ri}}{\displaystyle \sum _{i=1}^3\frac{t_{inD}}{24}{q}_i}=\frac{0.01\times 7.8\times {10}^{-3}\times {0.1}^2}{3.6\times 0.001\times 24}\times 4.89\times {10}^6\times 3\times {10}^5=13.24\times {10}^6{\mathrm{m}}^3$$

(40)

The MWPI induced by the MWPs scheme can stimulate injection pressure to rapidly reach constraint injection pressure. Here, we also compare the SMFHWs without considering the existing MWPI, still assuming that all wells will shut down as long as one of them reaches the limited injection pressure. Repeating the above steps, the final results can be summarized in

Table 2. Comparison between MWPs, SMFHWs, and numerical results.

Model	SMFHWs			MWPs			CMG Simulator
	Well 1	Well 2	Well 3	Well 1	Well 2	Well 3	Well 1	Well 2	Well 3
q, 104 m3/d	5	10	15	5	10	15	5	10	15
tinD	1.01 × 107			4.89 × 106			5.15 × 106
Q, 106 m3	4.55	9.12	13.68	2.21	4.42	6.61	2.32	4.64	6.98
V, 106 m3	27.35			13.24			13.94
Difference, %	96.2			3.93			0

. The SMFHWs overestimate the CO₂ storage capacity, which demonstrates that MWPI has a significant influence on estimations of CO₂ storage capacity.

3.5. Sensitivity Analysis

3.5.1. Effects of Different MWPs Schemes

The pressure build-up is partially determined by different distribution of HFs in MWPs scheme (Figure 2b). We can set some common parameters for those three wells: M₁ = 3, M₂ = 3, M₃ = 3, ζ_D = 0, λ = 0.002, γ = 0.15, ω = 0.0035, C_ηD = 10⁵, L_f1D = L_f2D = L_f3D = 500, C_f1D = C_f2D = C_f2D = 10. q₁:q₂:q₃ = 1:2:3. Another four parameters, L_w12D, L_w32D, L_f12D, and L_f32D, determine the different interference types illustrated in Figure 2b, which can be mathematically described as follows:

• Figure 2b (1): L_w12D = L_w32D = L_f1D + L_f2D = 1000, and L_f12D = L_f32D = 0;
• Figure 2b (2): L_w12D = L_w32D = L_f1D + L_f2D − 250 = 750, and L_f12D = L_f32D = 1000;
• Figure 2b (3): L_w12D = L_w32D = L_f1D + L_f2D + 2000 = 3000, and L_f12D = L_f32D = 0;
• Figure 2b (4): L_w12D = L_w32D = L_f1D + L_f2D + 2000 = 3000, and L_f12D = L_f32D = 1000;

Based on these pressure types in Figure 13 and the results in

Table 3. Comparison of different MWPs.

MWP Scheme	Figure 2b (1)	Figure 2b (2)	Figure 2b (3)	Figure 2b (4)
tinD	1.65 × 106	2.35 × 106	4.89 × 106	4.75 × 106
V, 106 m3	4.47	6.36	13.24	12.86

, when wells are directly connected with hydraulic fracture for MWPs scheme, the occurrence of MWPI is much earlier than in the other three kinds of MWPs schemes. In our case, when limited injection P_con = 7 MPa, CO₂ storage capacity approximately decreases by 200% from the interference type Figure 2b (3) and (4).

3.5.2. Effects of Ration of Well Rate ε₁, ε₂, ε₃

We define a new concept of injection capacity (IC), the total injection volume of MWPs per day. IC ranges from 20 to 150 × 10⁴ m³/d. Fracture number M₁ = M₂ = M₃ = 2. The value of ε₁:ε₂:ε₃ is 1:3:1, 1:1:1, and 2:1:2. We take Figure 2b (3) as an example. L_w12 = L_w32 = 200, and L_f12 = L_f32 = 0. L_f1 = L_f2 = L_f3 = 50 m. Figure 14a shows the pressure curves of wells that reach limited injection pressure. Figure 14b shows CO₂ storage capacity under different injection capacity IC and values of ε₁:ε₂:ε₃. It indicates that CO₂ storage capacity will decrease with the increasing of the injection capacity (IC); moreover, the ratio of CO₂ storage capacity decreases with the increasing of IC. In our case, when IC is bigger than 100 × 10⁴ m³/d, the CO₂ storage capacity remains approximately constant. This surprising result suggests that a small CO₂ injection rate can CO₂ storage capacity. In addition, because Well 2 is severely influenced by MWPI, CO₂ storage capacity is the lowest when Well 2 has a higher injection rate. In our case, we obtain much more CO₂ storage capacity when the injection rates of Well 1 and Well 3 are bigger than that of Well 2. More information about the effects of MWPI on the CO₂ injection strategy will be given in our Section 2.

3.5.3. Effects of Hydraulic Fracture Half-Length

We take Figure 2b (4) as an example. L_w12 = L_w32 = 300, and L_f12 = L_f32 = 100. IC = 50 × 10⁵ m³/d. Fracture number M₁ = M₂ = M₃ = 2. ε₁:ε₂:ε₃ = 2:1:2. Hydraulic fracture half-length L_f1 = L_f2 = L_f3 ranges from 50 to 200. Figure 15a shows the pressure curves of wells that reach limited injection pressure. Figure 15b shows the effects of hydraulic fracture half-length L_f1 = L_f2 = L_f3 on CO₂ storage capacity for MWPs scheme in a depleted SG reservoir under different constrained injection pressure P_con. It indicates that CO₂ storage capacity increases with the increasing of fracture half-length; moreover, CO₂ storage capacity has an approximately linear relationship with L_f. In addition, as limited injection pressure P_con increases, CO₂ storage capacity also increases; what is more, the longer the fracture half-length, the more the CO₂ storage capacity increases when we set a higher limited injection pressure. This means that when a SG reservoir has bigger P_con, longer L_f is beneficial to improve CO₂ storage capacity.

3.5.4. Effects of Hydraulic Fracture Number

We take Figure 2b (3) as an example. L_w12 = L_w32 = 300, and L_f12 = L_f32 = 0. IC = 50 × 10⁵ m³/d. ε₁:ε₂:ε₃ = 2:1:2. L_f1 = L_f2 = L_f3 = 50 m. Fracture number M₁ = M₂ = M₃ ranges from 2 to 4. Figure 16a shows the pressure curves of wells that reach limited injection pressure. Figure 16b shows the effects of hydraulic fracture number M₁ = M₂ = M₃ on CO₂ storage capacity for the MWPs scheme in a depleted SG reservoir under different constrained injection pressure P_con. It indicates that CO₂ storage capacity increases with the increase in the fracture number. There is an optimal fracture number beyond which the CO₂ storage capacity will not increase appreciably. The bigger the limited injection pressure P_con, the smaller the optimal fracture number. In our case, when limited injection pressure P_con = 7.0 MPa, the optimal fracture number is 5. Similarly, as limited injection pressure P_con increases, the CO₂ storage capacity also increases.

3.5.5. Effects of Stress Sensitivity

We take Figure 2b (3) as an example. L_w12 = L_w32 = 300, and L_f12 = L_f32 = 0. IC = 50 × 10⁵ m³/d. Fracture number M₁ = M₂ = M₃ = 2. ε₁:ε₂:ε₃ = 2:1:2. L_f1 = L_f2 = L_f3 = 50 m. ζ_D ranges from 0.01 to 0.05. Figure 17a shows the pressure curves of wells that reach limited injection pressure. Figure 17b shows the effects of stress sensitivity coefficient ζ_D on CO₂ storage capacity for the MWPs scheme in a depleted SG reservoir under different constrained injection pressure P_con. Our results demonstrate that stress sensitivity coefficient ζ_D has a positive influence on CO₂ storage capacity. Because stress sensitivity can increase the permeability of a SG reservoir, as ζ_D increases, CO₂ storage capacity increases. We also need to note that effects of stress sensitivity mainly occur at the intermediate–late production stage; therefore, ζ_D will not improve CO₂ storage capacity if wells reach limited injection pressure very early. As indicated in Figure 17, when P_con is below 6.5 MPa, stress sensitivity is not beneficial to CO₂ storage capacity. Therefore, we should take action to prolong the injection time, e.g., decreasing the injection rate or increasing the limited injection pressure.

4. Conclusions

To gain quick and reasonable estimations of CO₂ storage capacity for the MWPs scheme in a depleted SG reservoir, in this paper we develop a semi-analytical pressure-buildup model with consideration of MWPI. Model validation is implemented and calculation of CO₂ storage capacity and sensitivity analysis are also conducted. Some meaningful conclusions are summarized as following:

• There is good agreement between our model and the numerical simulation; moreover, our approach runs faster than the numerical simulation, which demonstrates the accuracy and efficiency of our method.
• Comparing to SMFHWs, transient-pressure analysis demonstrates that the MWPs scheme has a severe MWPI phenomenon. Direct connection between wells through hydraulic fracture can stimulate the occurrence of MWPI. Our results suggest that wells will rapidly reach limited injection pressure due to the MWPI. CO₂ storage capacity will be extremely overestimated by ignoring the MWPI.
• Increasing injection capacity (IC) will decrease the CO₂ storage capacity. To improve CO₂ storage capacity, it is beneficial to assign small CO₂ injection rate to wells that are severely impacted by MWPI.
• Both the fracture number and fracture half-length have a positive influence on the CO₂ storage capacity. Both of them have an approximate linear relationship with the CO₂ storage capacity. Longer L_f is much more beneficial to improve CO₂ storage capacity when the SG reservoir has bigger P_con. For a given injection pressure, there is an optimal fracture number; the bigger the limited injection pressure, the smaller the optimal fracture number.
• Stress sensitivity has a positive influence on CO₂ storage capacity; however, stress sensitivity generally occurs at the late production stage. Therefore, prolonging the injection period can improve CO₂ storage capacity due to the occurrence of stress sensitivity.

This work proposes a systematic methodology for the estimation of the CO₂ storage capacity for the MWPs scheme in depleted SG reservoirs. It provides new insights into CO₂ storage capacity due to the existence of MWPI. Although we consider simple fracture configuration and only two wells, our method can be extended to complex fracture network configurations. Especially for the non-orthogonal fracture topology, our free-simulator method will enhance the calculation speed, which will be the objective in the future.