The Effects of Securitization for Managing Banking Risk Using Alternative Tranching Schemes

Abstract

Diversification practices by banks affect their own risk of failing and the risk of the banking system as a whole (systemic risk). A seminal theoretical work has shown that linear diversification can reduce the risk of a bank failing, but at the cost of increasing systemic risk. Later, a follow-up study showed that a particular strategy of securitization with tranches can help avoid this trade-off. We extend the theoretical work on securitization by considering all possible strategies for securitization with two tranches, by finding their corresponding optimal diversification solutions, and by discussing their implications for individual and systemic risk. We show that securitization that involves exchanging a portion of both tranches brings back the dark side of diversification. In addition, we also show how different strategies of securitization can have risk amplification effects among banks when confidence shocks occur.

1. Introduction

The relationship between diversification and risk is a recurrent and relevant research topic within theoretical and empirical financial studies. This line of research has been intensified since the global 2007–2008 financial crisis. A common argument is that when banks diversify their portfolios to reduce their exposure to risk, the probability of joint failure (or systemic failure) increases because as diversification becomes larger, the portfolios of banking institutions become more similar (Allen et al. 2012; Allen and Carletti 2006; Ibragimov et al. 2011; Yang et al. 2020). This phenomenon has been called the dark side of diversification, and Wagner (2010) developed an important theoretical framework using linear diversification strategies to illustrate this point.

Later, van Oordt (2014) extended the model by adding securitization, which consists of tranching the loan’s portfolio into different levels of seniority. Securitization is built into the model by using two different tranches: one senior tranche and one junior tranche. The senior tranche is served first, and the junior tranche is served later if there is a residual payment available. van Oordt (2014) found that if a bank keeps the junior tranche and exchanges the senior with other banks (a strategy we call retaining the junior tranche: RJT), then the dark side of diversification can be avoided. He also showed that securitization comes at a cost for banks, as it introduces non-linear effects in the financial system.

Some empirical studies have suggested that, under certain conditions, there seems to be a positive and significant impact of securitization (or diversification) on systemic risk (Abdelsalam et al. 2022; Altunbas et al. 2022; Bégin et al. 2019; Ben Salah and Fedhila 2012; De Jonghe 2010; Ivanov and Jiang 2020; Slijkerman et al. 2013). According to a systemic review of the literature by Deku et al. (2019), securitization is not a destabilizing tool per se and, at least up to 2019, the empirical literature in the post 2007–2009 crisis on bank securitization behavior tends to be extremely limited due to important data gaps in many relevant markets of the world. Given the predominance of empirical studies around this topic and some of its data limitations, we think that theoretical work can potentially contribute to gain a better understanding with regards to how securitization instruments are designed and used; and to how they affect the stability of the banking system.

In Cadenas and Gzyl (2021), we extended the theoretical results of the model developed by van Oordt (2014) by allowing short positions. In this paper, we extend further the implications of the model by allowing banks to exchange any portion of both tranches in their long positions to further understand the implications in terms of incentives and the risk of failure of the banking system as a whole. We first show that linear diversification can be easily derived from securitization (we call it vertical distribution). We then develop full results for the optimal strategy of retaining the senior tranche (RST), which was not shown in van Oordt’s (2014) paper, and present results for the case when banks exchange some portion of both tranches (mixed strategy). We compare our results in the light of diversification and systemic risk, and we study the non-linear effects in the financial system for all possible cases (no diversification, linear diversification, RJT, RST and mixed strategy). Section 2 describes the basic setup of the model. In Section 3, we describe how each diversification strategy works and show their corresponding optimal solutions in terms of minimizing the risk of individual failure and its effects on systemic risk. Section 4 analyzes and presents our results in the light of the non-linear effects in the financial system that arise from sudden shocks in depositors’ confidence. Section 5 concludes and contrasts some of our results in the light of the most recent literature on securitization and the banking crisis. The analytical proofs and computational details are included in Appendix A and Appendix B.

2. Basic Setup of the Model

Our framework builds upon Wagner (2010)’s seminal model and van Oordt’s (2014) important extension. There are two banks, each obtaining one unit of funds from risk-neutral investors. A fraction d of the funds is in the form of deposits and the rest is equity capital. Two assets, X and $Y,$ which can be considered as loan portfolios, provide investment opportunities. The assets’ gross returns, x and $y,$ are identically and independently distributed with a probability density function $\varphi (·)$, which has full support on $[0,s],s>d.$ It is further assumed the expected gross return of each portfolio is not less than d; that is, we suppose that1:

$$\begin{array}{c}\hfill \mathbb{E}\left[v\right]={\int }_0^{s}v\varphi \left(v\right)dv\ge d,\mathrm{for}v=x,y.\end{array}$$

(1)

There are three periods in the investment horizon. At date 1, bank 1 invests its funds in asset X and bank 2 invests its funds in asset $Y.$ At date 2, the asset returns x and y are revealed and become public information. If the return is less than d and hence is insufficient to pay the creditors, the bank becomes insolvent, and it will experience a run2. Consequently, its assets have to be liquidated. The other bank, as long as it is not in distress, purchases the liquidated assets at a discount $c.$ However, if the other bank is also insolvent, the situation can be characterized as a systemic failure, in which case a nonbank firm, as an outside bidder, purchases the liquidated assets at a larger discount $qc$ with $q>1$ due to the nonbank firm’s lack of knowledge and skill in managing loan portfolio. At date 3, the asset returns are realized.

Denote the bank i’s gross return, if not liquidated, by $v_i$ and the probability of a run on bank i by ${\pi }_i^I,$ and the probability of a systemic crisis by ${\pi }^S.$ Then, the bank i’s total expected value is expressed as

$$\begin{array}{c}\hfill W_i=\mathbb{E}\left[v_i\right]-c({\pi }_i^I+q{\pi }^S)\end{array}$$

(2)

Bank i makes investment decisions that maximize $W_i,$ which effectively maximizes the expected return to its investors, who provided the funds, deposits and equity3. Notice that the probability of bank i’s failure (${\pi }_i$) is the sum of the probability of individual failure (${\pi }_i^I$) plus the probability of systemic failure (${\pi }^S$), i.e., ${\pi }_i={\pi }_i^I+{\pi }^S.$ More precisely, the individual and systemic failures are taken as mutually exclusive events. The economy’s total welfare is the sum of the two banks’ expected value:

$$\begin{array}{c}\hfill W_1+W_2=\mathbb{E}\left[v_1\right]+\mathbb{E}\left[v_2\right]-c({\pi }_1^I+{\pi }_2^I+2q{\pi }^S)\end{array}$$

(3)

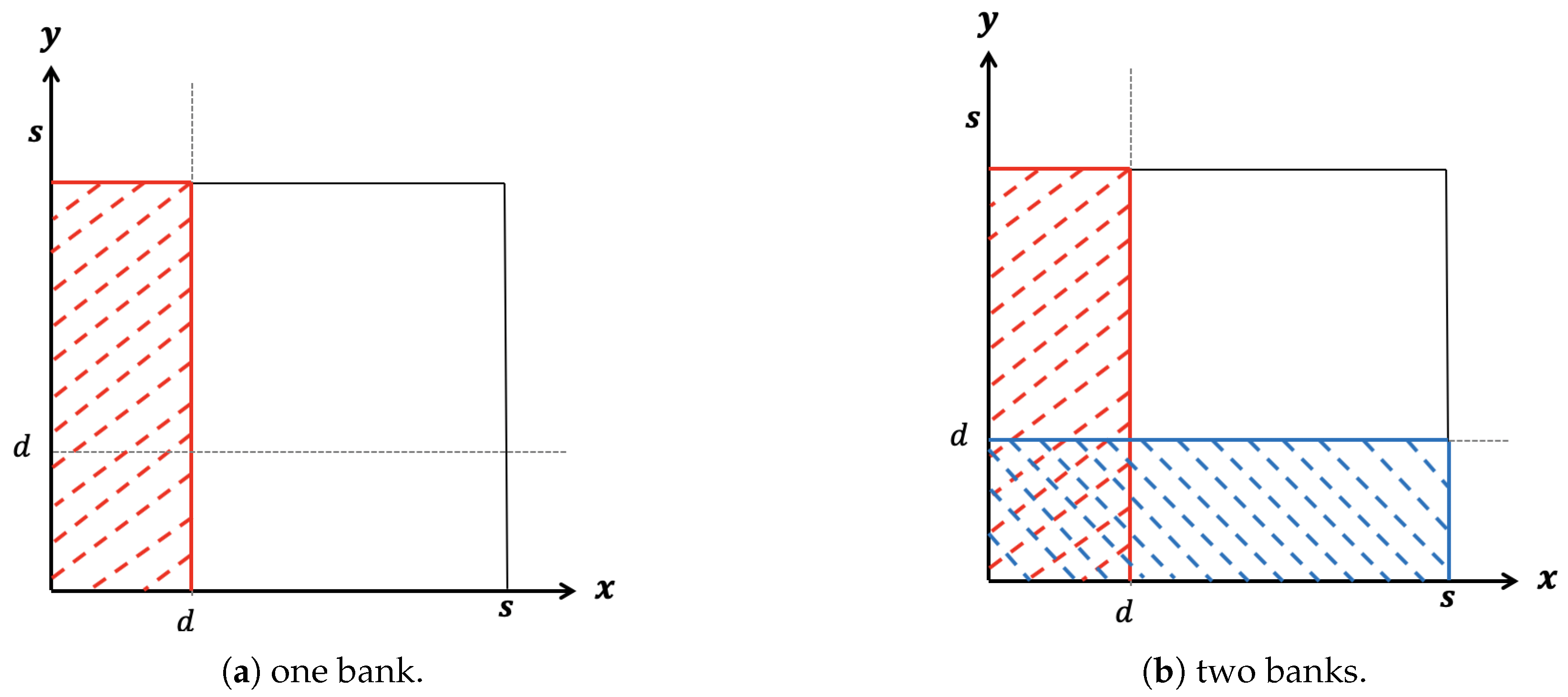
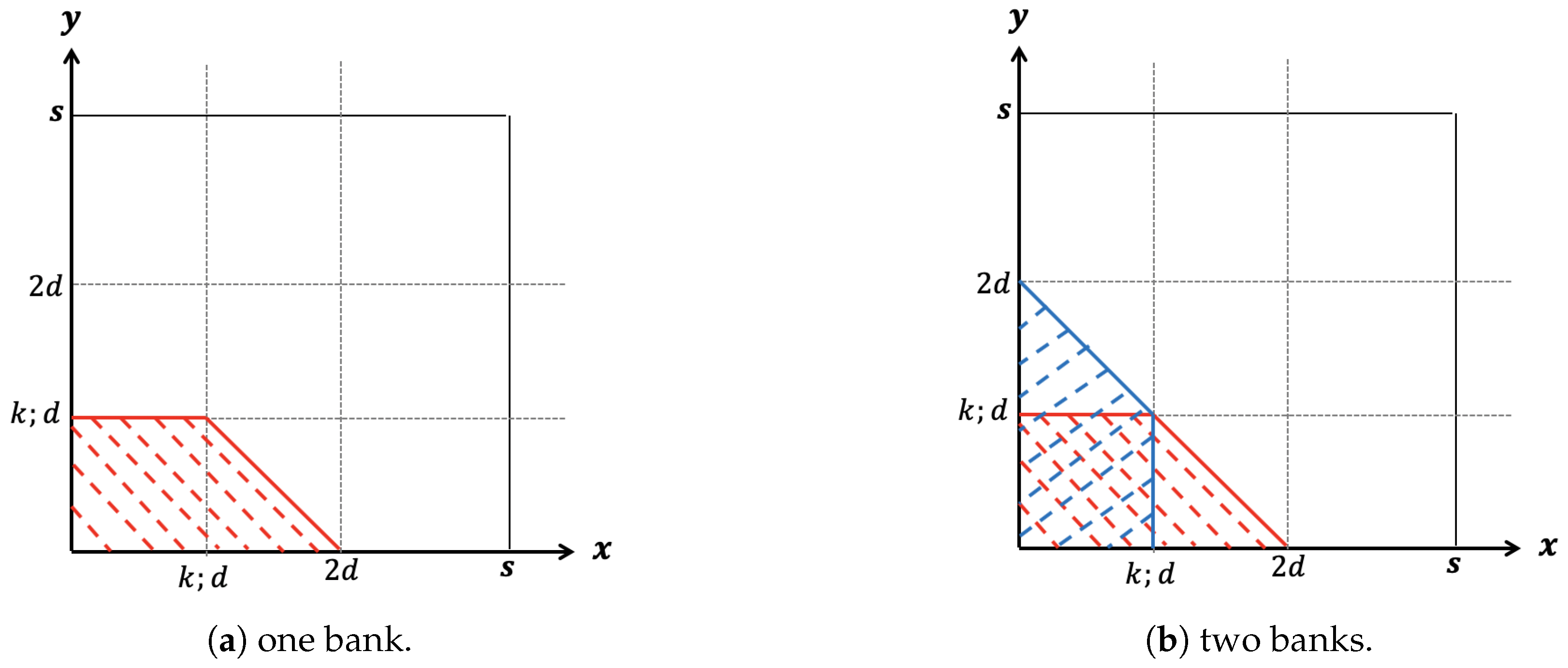
Now, without diversification, loan portfolio X would be the only asset of bank 1, and loan portfolio Y would be the only asset of bank 2. Hence, the gross return of each of the banks, if not liquidated, would simply be $v_1=x$ and $v_2=y,$ respectively. Since a bank becomes insolvent and experiences a run whenever the gross return on the bank’s asset is insufficient to cover $d,$ the bank run condition for bank 1 can be displayed as in Figure 1a; the dashed area represents the set of outcomes for bank 1’s failure. Bank 2’s case is added in Figure 1b, where the double dashed square represents the set of outcomes for a joint bank failure.

Figure 1. Bank run outcomes in case of no diversification. (a) one bank (b) two banks.

However, once banks diversify by investing in both loan portfolios in various ways, the gross returns would change and, more importantly, the probabilities of individual and systemic bank failures would also change. This can be graphically verified by noting that a change in $v_i$ would change the border line, which divides bank run outcomes and no-bank run outcomes, which eventually would modify the probabilities of individual and join bank failures. The point is that a bank’s asset diversification affects not only the risk at the level of the individual bank but also the systemic risks of the entire banking sector. Let us now discuss each of the possible diversification strategies.

3. Diversification Strategies

To extend van Oordt (2014)’s model, we first present a general case of securitization, where the diversification strategies suggested in Wagner (2010) and van Oordt (2014) can be considered special cases. Suppose banks tranche the loan portfolio into securities with two different seniority levels and can sell these trenches to the other bank. On date 3, when the return on the bank asset is realized, the senior tranche is served first, up to the maximum $k\in [0,s]$ set by the bank, whereas the junior tranche obtains the residual. Accordingly, the senior tranche on asset X has the payoff equal to $x^S\left(k\right)=\min \{x,k\}$, and the junior tranche has the payoff equal to $x^J\left(k\right)=\mathrm{Max}\{x-k,0\}$. Similarly, each of the senior and junior tranches on asset Y has the payoff equal to $y^S\left(k\right)=\min \{y,k\}$ and $y^J\left(k\right)=\mathrm{Max}\{y-k,0\}$, respectively.

Bank i can choose to exchange fraction $r_i^S$ of the senior tranche and fraction $r_i^J$ of the junior tranche. The remaining portions $(1-r_i^S)$ and $(1-r_i^J)$ of the senior and junior tranches are kept on bank i’s balance sheet. Then, bank 1’s and bank 2’s respective returns on investment, if not liquidated, would be obtained as,

$$\begin{array}{c}\hfill v_1(x,y)=(1-r_1^S)\min \{x,k\}+r_1^S\min \{y,k\}+(1-r_1^J)\mathrm{Max}\{x-k,0\}+r_1^J\mathrm{Max}\{y-k,0\}\\ \hfill v_2(x,y)=(1-r_2^S)\min \{y,k\}+r_2^S\min \{x,k\}+(1-r_2^J)\mathrm{Max}\{y-k,0\}+r_2^J\mathrm{Max}\{x-k,0\}\end{array}$$

(4)

In this setup, different diversification strategies could emerge from the various combinations of $r_i^S$ and $r_i^J.$ The simplest case would be no diversification, i.e., $r_i^S=r_i^J=0,$ which would generate bank run outcomes described in Figure 1a,b. As mentioned before, in the rest of this section, we consider four diversification strategies: linear diversification without securitization, securitization with exchanging senior tranches only, securitization with exchanging junior tranches only, securitization with vertical distribution (which derives in linear diversification) and exchanging a portion of both tranches (mixed strategy).

3.1. Linear Diversification without Securitization

A bank can simply exchange a portion of its asset with the other bank without tranching. This type of diversification, which can be called linear diversification, is considered in Wagner (2010), and we recall it here for completeness. Since there is no tranching, we can have $r_i,$ without the superscript S and $J,$ denoting the fraction of bank i’s portfolio that is exchanged. Bank i’s return on investment, if not liquidated, is then

$$\begin{array}{c}\hfill v_1(x,y)=(1-r_1)x+r_{1}y\\ \hfill v_2(x,y)=(1-r_2)y+r_{2}x\end{array}$$

(5)

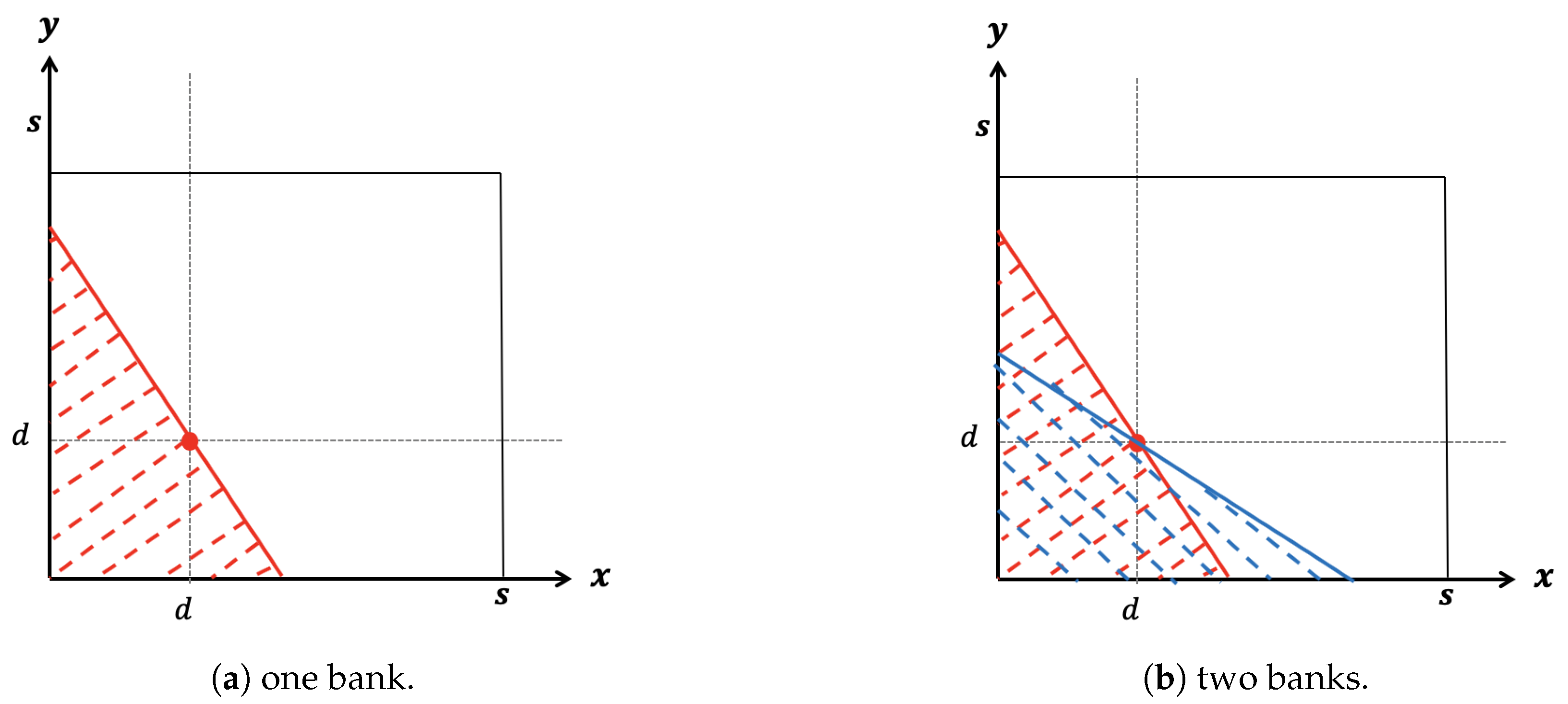
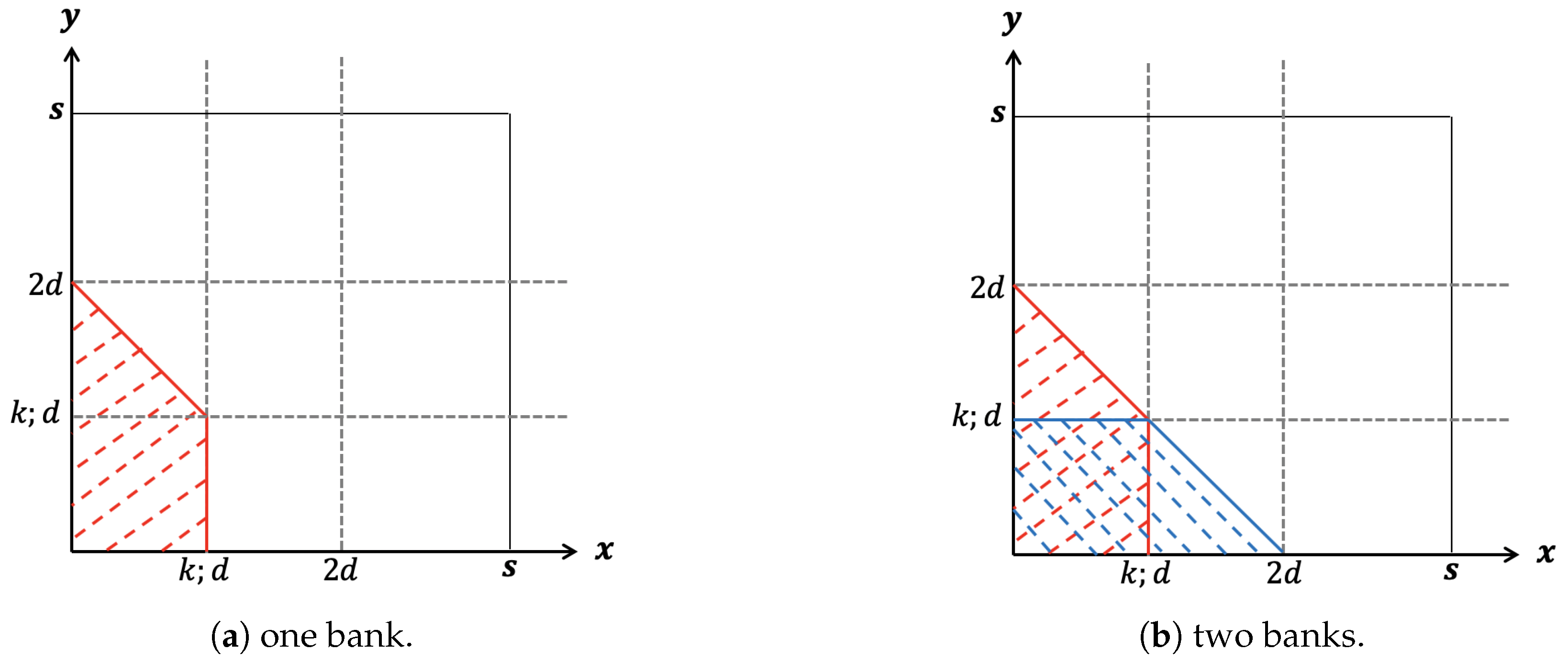
In this case, the bank run outcomes for bank 1 are represented by the dashed line in Figure 2a. The bank 2 failure outcomes are added in Figure 2b where the double dashed area represents the outcomes for a systemic crisis.

Figure 2. Bank run outcomes in case of linear diversification. (a) one bank (b) two banks.

Wagner shows that linear diversification may reduce the probability of bank run on an individual bank when compared with no diversification, but it increases the probability of a systemic crisis. One can see this by observing Figure 2. For a formal proof, see Wagner (2010). This point illustrates the dark side of diversification4.

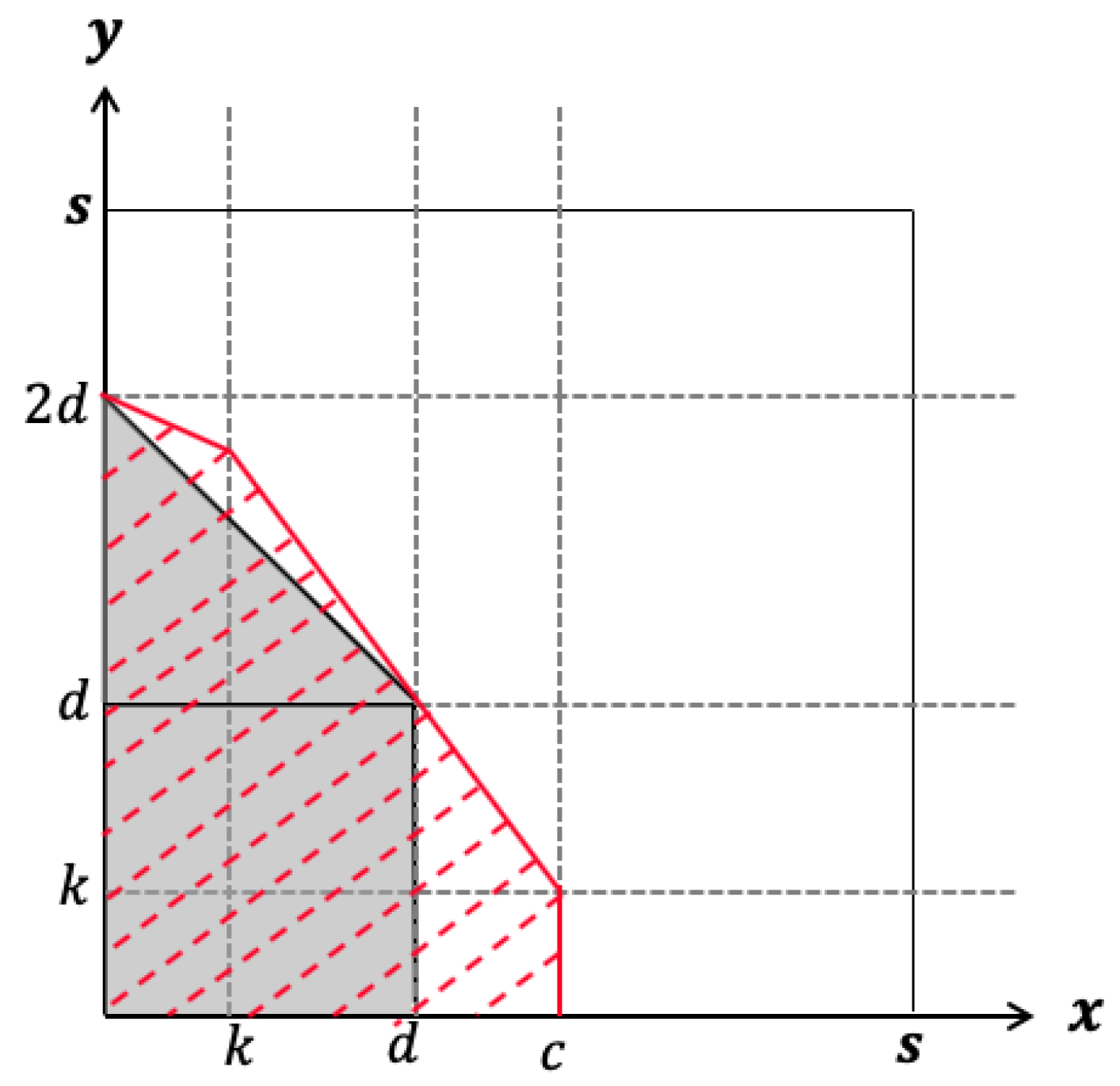
3.2. Securitization Retaining Junior Tranche (RJT)

In comparison to Wagner (2010)’s linear diversification, van Oordt (2014) introduces a non-linear diversification where banks securitize the loan portfolio into tranches and sell the senior tranche only. We call this strategy Retaining the Junior Tranche (RJT), and it corresponds to setting $r_i^J=0.$ In this case, bank i’s return on investment in Equation (4), if not liquidated, becomes

$$\begin{array}{c}\hfill v_1^S(x,y)=(1-r_1^S)x+r_1^S\mathrm{Max}\{x-k,0\}+r_1^S\min \{y,k\}\\ \hfill v_2^S(x,y)=(1-r_2^S)y+r_2^S\mathrm{Max}\{y-k,0\}+r_2^S\min \{x,k\}\end{array}$$

(6)

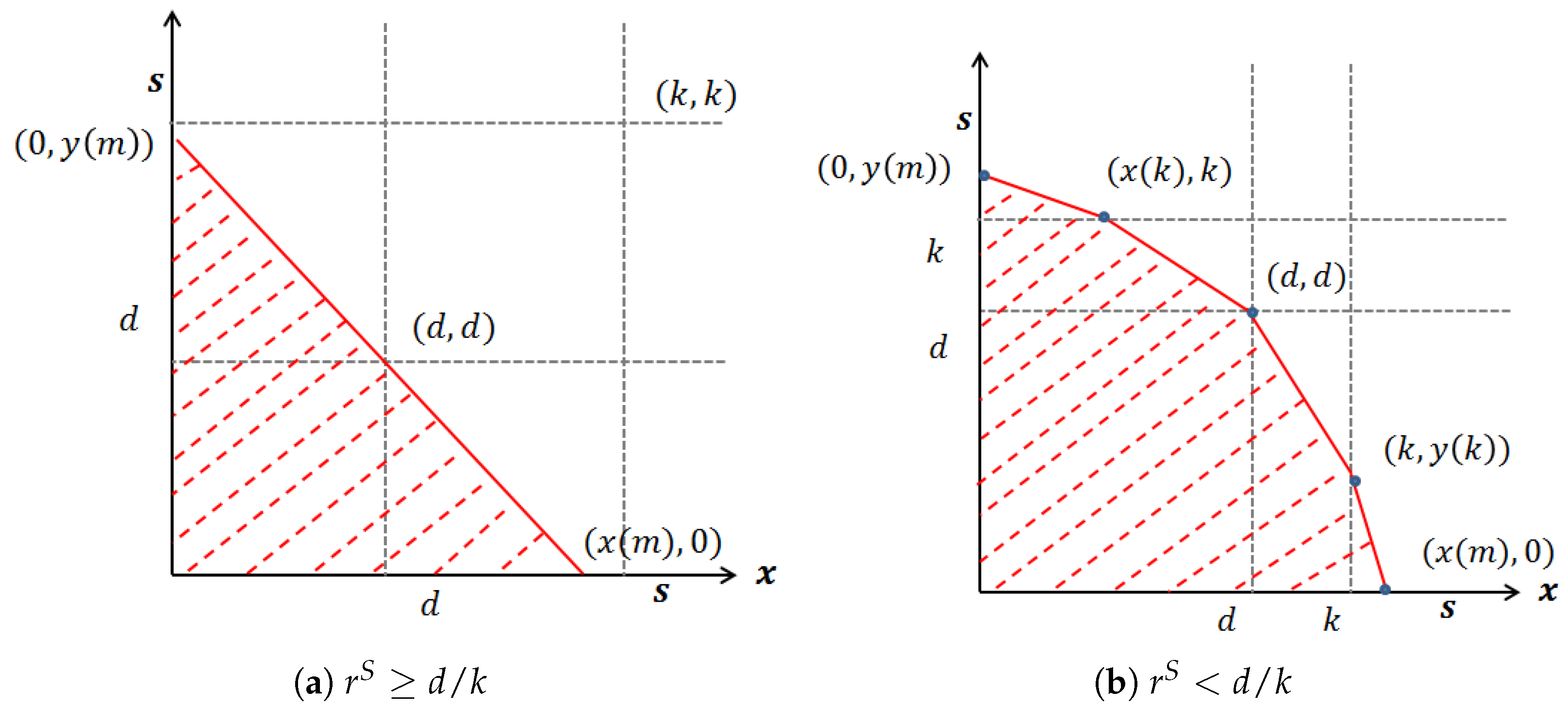
In this case, obtaining the bank run outcomes becomes complicated as it depends on the level of k vis-à-vis the level of $d.$ van Oordt (2014) finds the different classes for the bank run outcomes and proves that for securitization that entails exchanging only the senior tranches, it holds that: (i) the probability of a systemic crisis cannot be decreased even with the RJT securitization; (ii) bank i’s optimal diversification strategy is the RJT securitization with $k=d$ and $r_i^S=1$ (individual optimality); and (iii) both banks adopting the strategy described in part (ii) is socially optimal (social optimality)5. If banks follow the optimal strategy suggested by van Oordt (2014), then the probabilities of failure would look as in Figure 3:

Figure 3. Bank run outcomes for the optimal diversification strategy in case of securitization with keeping a senior tranche. (a) one bank (b) two banks.

3.3. Securitization with Vertical Distribution

If bank i sells the same portion of each tranche, i.e., $r_i^S=r_i^J$, what we called vertical distribution, its investment return will be the same as that of the linear diversification strategy examined in Section 3.1. Hence, we obtain the following result,

Proposition 1.

Setting $r_i^S=r_i^J$ makes the securitization with vertical distribution effectively identical to the linear diversification strategy.

Proof. 

$r_i^S=r_i^J$ makes Equation (4) effectively identical to Equation (5). □

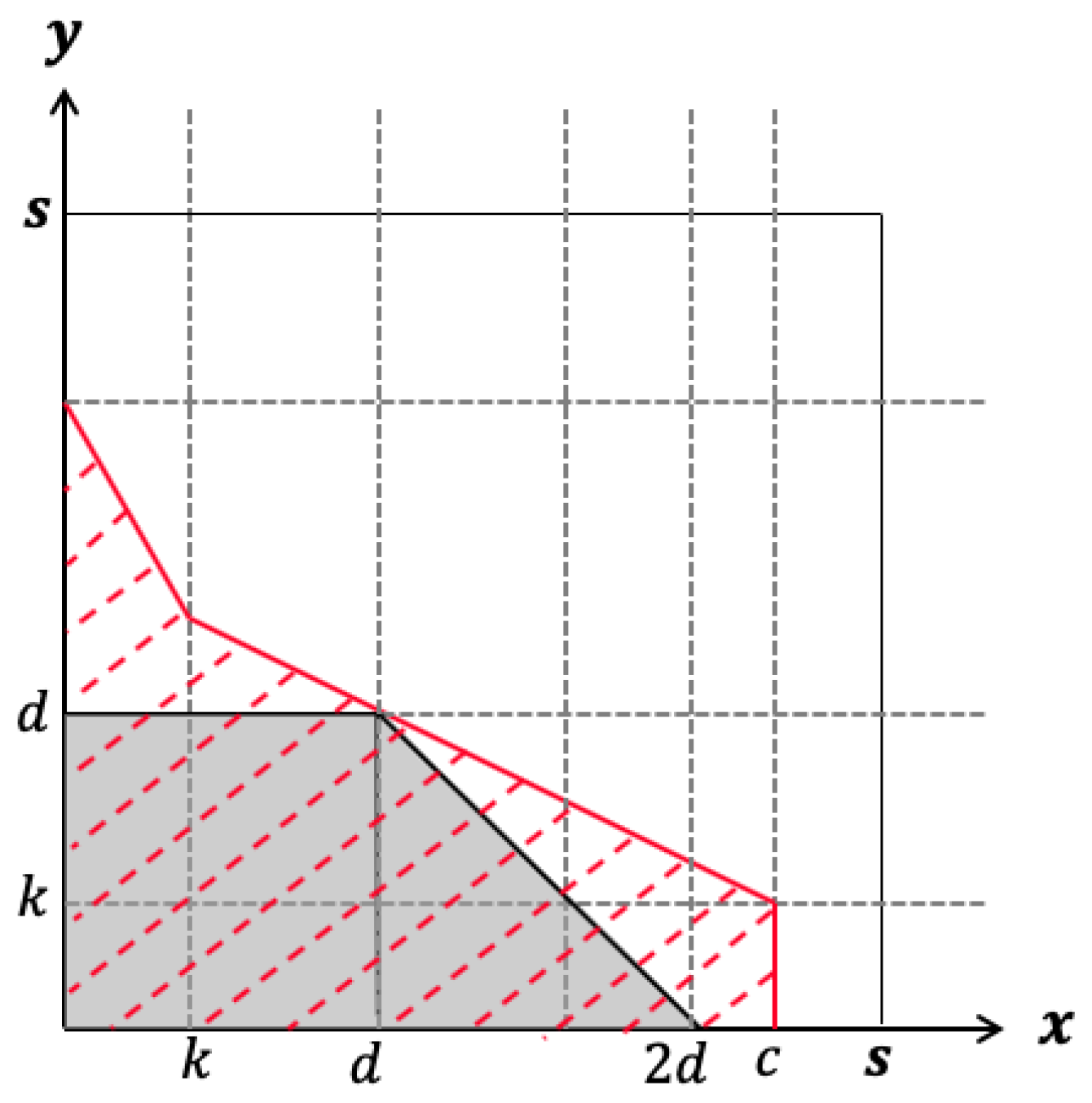
3.4. Securitization with Keeping Senior Tranche (RST)

When introducing the securitization with tranching into Wagner (2010)’s original model, van Oordt (2014) assumed that banks keep the junior tranche on their own balance sheet and exchange a fraction of the senior tranches. Although he states on a footnote that “It is irrelevant to the results whether we assume that banks exchange senior tranches and keep the junior tranche or vice versa”, we explore the validity of this assertion here. Our results are in agreement with van Oordt (2014), but we are interested in briefly exploring the implications of this result for the case of the non-linear effects in the financial system. As a further justification, van Oordt (2014) briefly mentioned asymmetric information between originators and buyers of securities as a potential mechanism that makes the strategy of exchanging senior tranches only an optimal structure. The information asymmetry may possibly arise when the buyers are outsiders such as institutional investors or nonbank firms without an understanding of the nature and composition of the securities they are purchasing. However, in the current model, the buyers of the securities are banks who themselves are securities originators and therefore may be better positioned than the outsiders when bidding for the tranches.

In this section, as mentioned before, we explicitly explore the possibility of the alternative strategy of keeping the senior tranche and selling the junior tranche only. We do this within the confines of the given model. The alternative securitization strategy we suggest corresponds to setting $r_i^S=0.$ In this case, bank i’s return on investment is rewritten, if not liquidated, as

$$\begin{array}{c}\hfill v_1^J(x,y)=(1-r_1^J)x+r_1^J\min \{x,k\}+r_1^J\mathrm{Max}\{y-k,0\}\\ \hfill v_2^J(x,y)=(1-r_2^J)y+r_2^J\min \{y,k\}+r_2^J\mathrm{Max}\{x-k,0\}\end{array}$$

(7)

Or, alternatively,

$$\begin{array}{c}{v}_1^J(x,y)=x+r_1^J[\mathrm{Max}\{y-k,0\}-\mathrm{Max}\{x-k,0\}]\\ v_2^J(x,y)=y+r_2^J[\mathrm{Max}\{x-k,0\}-\mathrm{Max}\{y-k,0\}]\end{array}$$

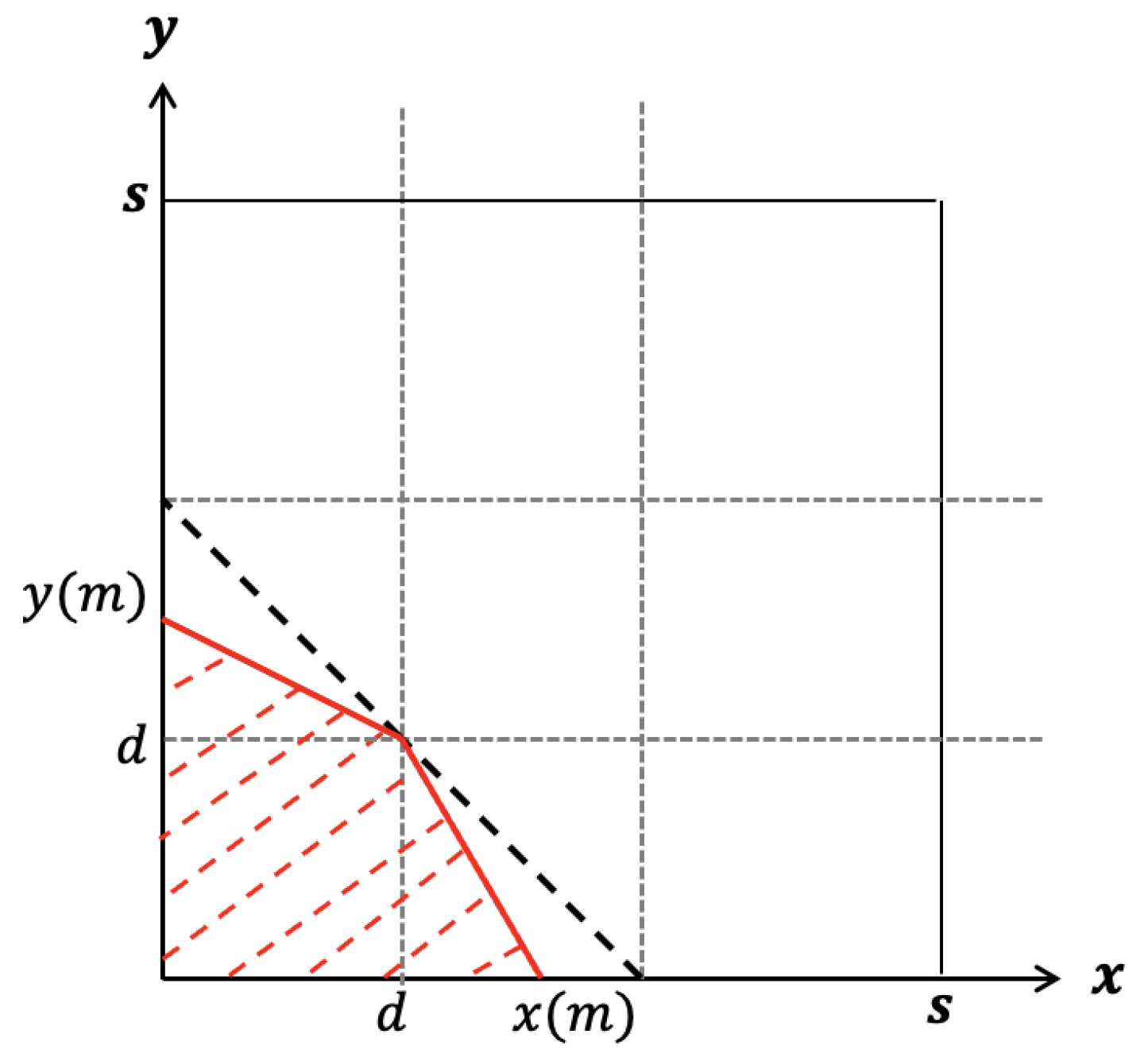
Taking $r_1^J=r,$ we find the no-bank run border for bank 1 to be:

Class 1: conditional on $k⩾d,$ we have

$$\begin{array}{c}\hfill {\overline{y}}_1\left(x\right)=\left\{\begin{array}{c}(d-x+rk)/r,\mathrm{if}x\in [0,d),\hfill \\ 0,\mathrm{if}x\in [d,s],\hfill \end{array}\right.\end{array}$$

(8)

Class 2: conditional on $k<d,$ we have

$$\begin{array}{c}\hfill {\overline{y}}_1\left(x\right)=\left\{\begin{array}{c}(d-x+rk)/r,\mathrm{if}x\in [0,k),\hfill \\ (d-x+rx)/r,\mathrm{if}x\in [k,\frac{d-rk}{1-r}),\hfill \\ 0,\mathrm{if}x\in [\frac{d-rk}{1-r},s],\hfill \end{array}\right.\end{array}$$

(9)

The set of bank run outcomes for a single bank within each of the two classes are displayed in the following Figure 46.

Figure 4. No-bank run border for one bank under the strategy of exchanging only the junior tranche.

Results

Proposition 2.

If banks maintain the senior tranches only, as in (7), or if they keep both tranches in the same proportion (linear diversification), the probability of a joint failure cannot be decreased when compared to the no diversification case.

Although tranching does not decrease the probability of a joint failure, it does decrease the probability of a run on each individual bank. van Oordt (2014) shows this to be true for retaining the junior tranche only, and it will be shown in Proposition 3 to be true also for retaining the senior tranche in full.

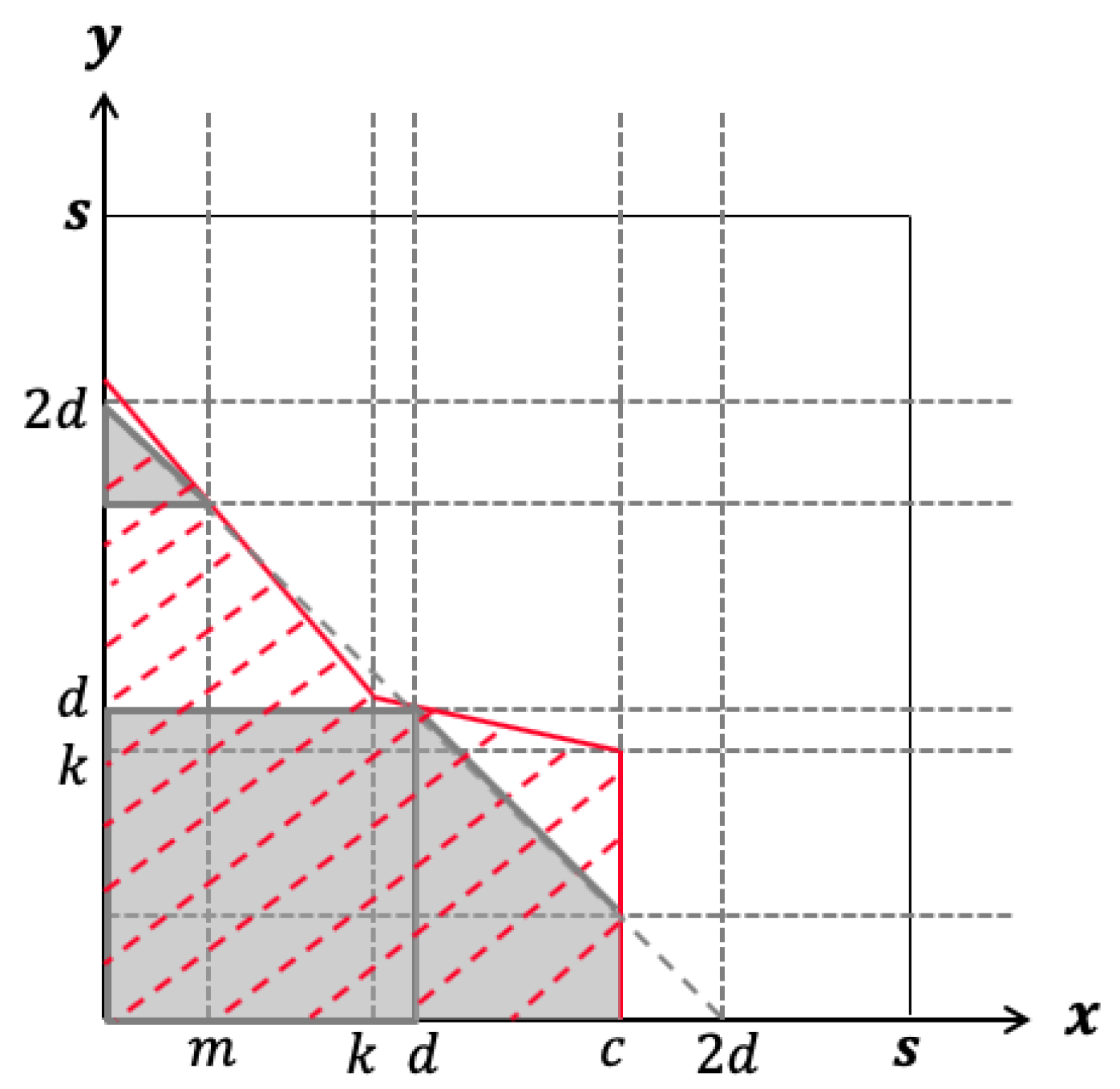
Proposition 3.

The probability of a run on bank i is minimized if and only if bank i sets the maximum payoff of the senior tranche equal to its level of deposits, i.e., $d=k,$ and exchanges its junior tranche entirely ($r_i^J=1$).

We illustrate the effectiveness of Proposition 3 in Figure 5a,b. Following (9), the no bank-run border for bank 1 under Proposition 3 is given by,

$$\begin{array}{c}\hfill {\overline{y}}_1^{*}\left(x\right)=\left\{\begin{array}{c}2d-x,\mathrm{if}x\in [0,d),\hfill \\ 0,\mathrm{if}x\in [d,s],\hfill \end{array}\right.\end{array}$$

(10)

Figure 5. Bank run outcomes for the optimal diversification strategy in case of securitization with keeping junior tranche.

The probability of bank 1 becoming insolvent under the strategy proposed is,

$$\begin{array}{cc}\hfill {\pi }_1^{*}={\int }_0^d{\int }_0^d\varphi \left(x\right)\varphi \left(y\right)dydx+{\int }_0^d{\int }_d^{2d-x}\varphi \left(x\right)\varphi \left(y\right)dydx& \hfill \end{array}$$

(11)

The expression in (11) is the outcome for the dashed area in Figure 5a. As it can be observed, the probability of a simultaneous bank run (Figure 5b) is not increased by trenching as defined in Proposition 3. As a consequence, minimizing the bank run probability also minimizes the expected costs of liquidation.

Proposition 4.

The optimal investment strategy that maximizes the total welfare in the economy, when exchanging only the junior tranche, is to set the maximum payoff of the senior tranche equal to its level of deposits, i.e., $d=k,$ while exchanging its junior tranche entirely ($r_1^J=r_2^J=1$). Since this strategy is the same when exchanging only the senior tranche, whether banks follow the optimal strategy from exchanging only the junior tranche or follow the optimal strategy from exchanging only the senior tranche, the total welfare of the economy is the same.

In other words, the total welfare of the economy does not change in situations where only the senior tranche, or only the junior tranche, is exchanged.

3.5. Securitization without Keeping Senior or Junior Tranche (Mixed)

In this section, we study the case in which none of the banks keeps the senior or junior tranche in full. As mentioned in Section 3, the returns for bank 1 and bank 2 if not liquidated are given by,

$$\begin{array}{c}\hfill v_1(x,y)=(1-r_1^S)\min \{x,k\}+r_1^S\min \{y,k\}+(1-r_1^J)\mathrm{Max}\{x-k,0\}+r_1^J\mathrm{Max}\{y-k,0\}\\ \hfill v_2(x,y)=(1-r_2^S)\min \{y,k\}+r_2^S\min \{x,k\}+(1-r_2^J)\mathrm{Max}\{y-k,0\}+r_2^J\mathrm{Max}\{x-k,0\}\end{array}$$

(12)

where in this particular case, $r_1^S,$$r_2^S,$$r_1^J$ and $r_2^J\in (0,1)$. Notice that zero and one are excluded from the set of options. These extreme cases are dealt with at the end. This case was not explored in van Oordt (2014). In Appendix B, we study the default region in the case ($k\ne d$). From this geometric analysis, we gain intuition about how to move the parameters to minimize the size of the default probability. That analysis leads us to concentrate on case $k=d$ where the best hedging portfolios come up.

As the $v_1(x,y)$ is a piecewise linear function, the default boundary is determined by the obvious break points The analysis in Appendix B is split into the cases $k\le d$ and $d<k,$ and this last case is further split into two cases according to whether $r^S\ge d/k$ or $r^S<d/k.$ In addition to finding the breakpoints at the boundary, we determined how the points move when $r^S$ and $r^J$ vary. When the distribution of assets is uniform, the probability of default is the (normalized) area of the default region and it is not difficult to compute. Certainly, when $k\to d,$ the top and lateral breakpoints move to $(d,d).$ In this case, the position of the bank is given by:

$$v_1(x,y)=(1-r_1^S)\min \{x,d\}+r_1^S\min \{y,d\}+(1-r_1^J)\mathrm{Max}\{x-d,0\}+r_1^J\mathrm{Max}\{y-d,0\}.$$

Note that when $r^S=r^J=r$ or when $1-r^S=r^J,$ that is along the diagonal and along the anti-diagonal in the parameter space, the hedges reduce to the linear diversification case. However, in the general case, there are two free parameters, and this allows us to move the default boundary to further decrease the default probability. It is in Appendix B.2.4 of Appendix B where we collect the computational details. Here, we mention that when $k=d,$ and $d<s/2,$ and the assets are uniformly distributed, the probability of default is given by (A26), that is, by:

$$P={\left(\frac{d}{s}\right)}^2\left(1+\frac{1}{2}\left(\frac{1-r^S}{r^J}+\frac{r^S}{1-r^J}\right)\right).$$

(13)

Note that at $(r^S,r^J)=(1/2,1/2)$, we obtain that the probability of individual failure is given by $P=2d^2/s^2.$ It is shown in Appendix B.2.5 of Appendix B that the point $(1/2,1/2)$ is a saddle point for $P(r^S,r^J)$ given by (13). This drops out of the fact that the $2\times 2$—Hessian matrix of $P(r^S,r^J)$ has a positive and a negative eigenvalue. We denote by ${\lambda }_{-}$ the negative eigenvalue and by ${\mathit{e}}_{-}$ its eigenvector. When a small enough $a\in \mathbb{R}$ is chosen, so that $(r^S,r^J)=(1/2,1/2)+a{\mathit{e}}_{-}\in (0,1)\times (0,1),$ then

$$P(r^S,r^J)=2{\left(\frac{d}{s}\right)}^2+\frac{a^2}{2}{\lambda }_{-}<2{\left(\frac{d}{s}\right)}^2.$$

(14)

This explains why the bi-parametric hedge offers more possibilities for controlling default probability than the mono-parametric hedge. To sum up, we have the following result.

Theorem 1.

With the notations and under the assumptions introduced above, when each bank keeps the junior and the senior tranches, each bank may choose parameters $r_1^S,r_1^J$ (respectively, $r_2^S,r_2^J$) in $(0,1)$ in such a way that their individual default probabilities become (13) and satisfy (14); i.e., they are smaller than the individual default probability of the optimal linear hedge.

It is important to observe that the intersection of the individual default regions (i.e., the systemic risk) may have a larger probability of default than if no hedging is implemented. Hence, the dark side of diversification cannot be avoided when $r_1^S,r_1^J$) in $(0,1)$. The dark side of diversification can only be avoided in the extreme cases: that is, when $r_1^S=0$ and $r_1^J=1$ or when $r_1^S=1$ and $r_1^J=0$. The two-parametric dependence allows us two extreme cases, such that each bank can adopt one of them and lower the individual default probability without increasing the probability of joint default. This is discussed in Appendix B.2.6. The result is summed up in the following theorem. The result is stated without referring to any particular bank.

Theorem 2.

Suppose that the hedges of each bank are such that their returns are given by (12). For each bank, there are two extreme cases corresponding to $r^S=1$ or $r^S=0.$ In the two cases, the default regions are as depicted in Figure A7a,b. In the first case, for $0\le r^J<1/2$, and in the second, for $1/2<r^J\le 1,$ the probabilities of individual default satisfy

$$\frac{3}{2}{\left(\frac{d}{s}\right)}^2\le P<2{\left(\frac{d}{s}\right)}^2.$$

When the two banks adopt complementary hedges, their common region of default coincides with the no hedge region of default. Therefore, the dark side of diversification is avoided.

Controlling the Probability of Default

The results obtained above bring up the themes developed in Cadenas et al. (2021) or in Cadenas and Gzyl (2021). In the latter, we showed that one can control the individual and the systemic probability of default by considering short positions, whereas in the former, we discussed how and why the probability of default and loss level are complementary. If one assigns a probability of default, this determined what the default level is. The underlying reason is that risk involves two complementary aspects: frequency and severity.

The next result follows the approach in Cadenas et al. (2021). The idea is to fix the default rate and determine the default level so that the probability of default is at most the chosen probability. Let us denote that by p and note that if we equate the right hand side of (14) to p, we obtain

$$p=2{\left(\frac{d}{s}\right)}^2+\frac{a^2}{2}{\lambda }_{-}\Rightarrow d=s\frac{1}{\sqrt{2}}{\left(p-\frac{a^2{\lambda }_{-}}{2}\right)}^{1/2}$$

which allows us to choose an appropriate default level d if p is preassigned. When d is preassigned, a similar calculation allows us to choose the value of the parameter a as to lower the individual default probability to a value less than $2d^2/s^2.$

4. Securitization and Confidence Shocks

Following van Oordt (2014), we analyze the results of retaining the senior tranche in the context of confidence shocks. van Oordt (2014) found that the benefits of securitization, when compared with linear diversification strategies, which consist of decreasing the probability of individual failures without increasing the probability of systemic risk, come at a cost. In other words, although structuring claims on loan portfolios using different seniority levels does not necessarily increase the probability of joint failures, it introduces non-linear effects in the financial system. Once banking institutions adopt the optimal strategy, small unanticipated shocks in confidence can increase considerably the risk of systemic failures. Given that banks do not have the knowledge to perfectly calibrate the payoffs of the tranches by setting $k=d,$ the effects of confidence shocks become relevant to the analysis of trenching.

In this section, we compare the non-linear effects of different strategies: no diversification, linear diversification, retaining the junior tranche (RJT), retaining the senior tranche (RST), and exchanging a portion of both tranches (Mixed). We are particularly interested in comparing the RST, RJT and Mixed strategies, since this analysis has not been completed within the model. The shock in depositor’s confidence is modeled in the same way as in van Oordt (2014). The shock in depositors confidence occurs after the banks define their investment strategy at date 1. Depositors participate in a bank run if the return on the bank’s investments at date 2 is smaller than $\delta .$ Therefore, if $\delta >d$, a bank run may take place in a solvent bank.

We show here the expected liquidation costs for the four strategies mentioned and for specific levels of $\delta .$ The expected default costs in the case of no tranching and no diversification are given by,

$$\begin{array}{cc}\hfill c({\pi }_1^I+{\pi }_2^I+2q{\pi }^s)=2c\frac{s{\delta }^2-{\delta }^2}{s^2}+2cq\frac{{\delta }^2}{s^2}& \hfill \end{array}$$

(15)

If banks follow the social optimal strategy with linear diversification as defined by Wagner (2010) where $r^{*}=1/(1+\sqrt{2q-1})$, the expected default costs are equal to,

$$\begin{array}{cc}\hfill c({\pi }_1^I+{\pi }_2^I+2q{\pi }^s)=2c(q+\sqrt{2q-1})\frac{{\delta }^2}{s^2}& \hfill \end{array}$$

(16)

If banks adopt the tranching strategy by retaining the junior tranche, the expected default costs are,

$$\begin{array}{c}\hfill c({\pi }_1^I+{\pi }_2^I+2q{\pi }^s)=\left\{\begin{array}{c}2c\frac{k\delta -(1/2){\delta }^2}{s^2}+2cq\frac{{\delta }^2}{s^2},\mathrm{if}\delta \in [0,k],\hfill \\ \\ 2c\frac{s\delta -{\delta }^2}{s^2}+2cq\frac{{\delta }^2}{s^2}+c(2q-1)\frac{k^2}{s^2},\mathrm{if}\delta \in (k,s].\hfill \end{array}\right.\end{array}$$

(17)

If banks adopt the tranching strategy by retaining the senior tranche, we find the expected default costs to be,

$$\begin{array}{c}\hfill c({\pi }_1^I+{\pi }_2^I+2q{\pi }^s)=\left\{\begin{array}{c}2c\frac{k\delta -(1/2){\delta }^2}{s^2}+2cq\frac{{\delta }^2}{s^2},\mathrm{if}\delta \in [0,k],\hfill \\ \\ c\frac{w}{s^2}+cq\frac{z}{s^2},\mathrm{if}\delta \in (k,s].\hfill \end{array}\right.\end{array}$$

(18)

where $w=k^2+2(s-\delta -k)k+(s-\delta )(\delta -k)$, and $z=k\delta +(\delta -k)k+{(\delta -k)}^2+(s-\delta )k.$

Finally, we explore what would happen if banks adopt the Mixed trenching strategy with $r_i^J=r_i^S=1/2$, and we find the expected default costs to be,

$$\begin{array}{c}\hfill c({\pi }_1^I+{\pi }_2^I+2q{\pi }^s)=\left\{\begin{array}{c}2cq\frac{2{\delta }^2}{s^2},\mathrm{if}\delta \in [0,k/2],\hfill \\ \\ 2cq\frac{{\delta }^2+{(2\delta -k)}^2+{(k-\delta )}^2}{s^2},\mathrm{if}\delta \in (k/2,k),\hfill \\ \\ 2cq\frac{{\delta }^2+k^2+2(\delta -k)k+{(\delta -k)}^2}{s^2},\mathrm{if}\delta \in [k,s].\hfill \end{array}\right.\end{array}$$

(19)

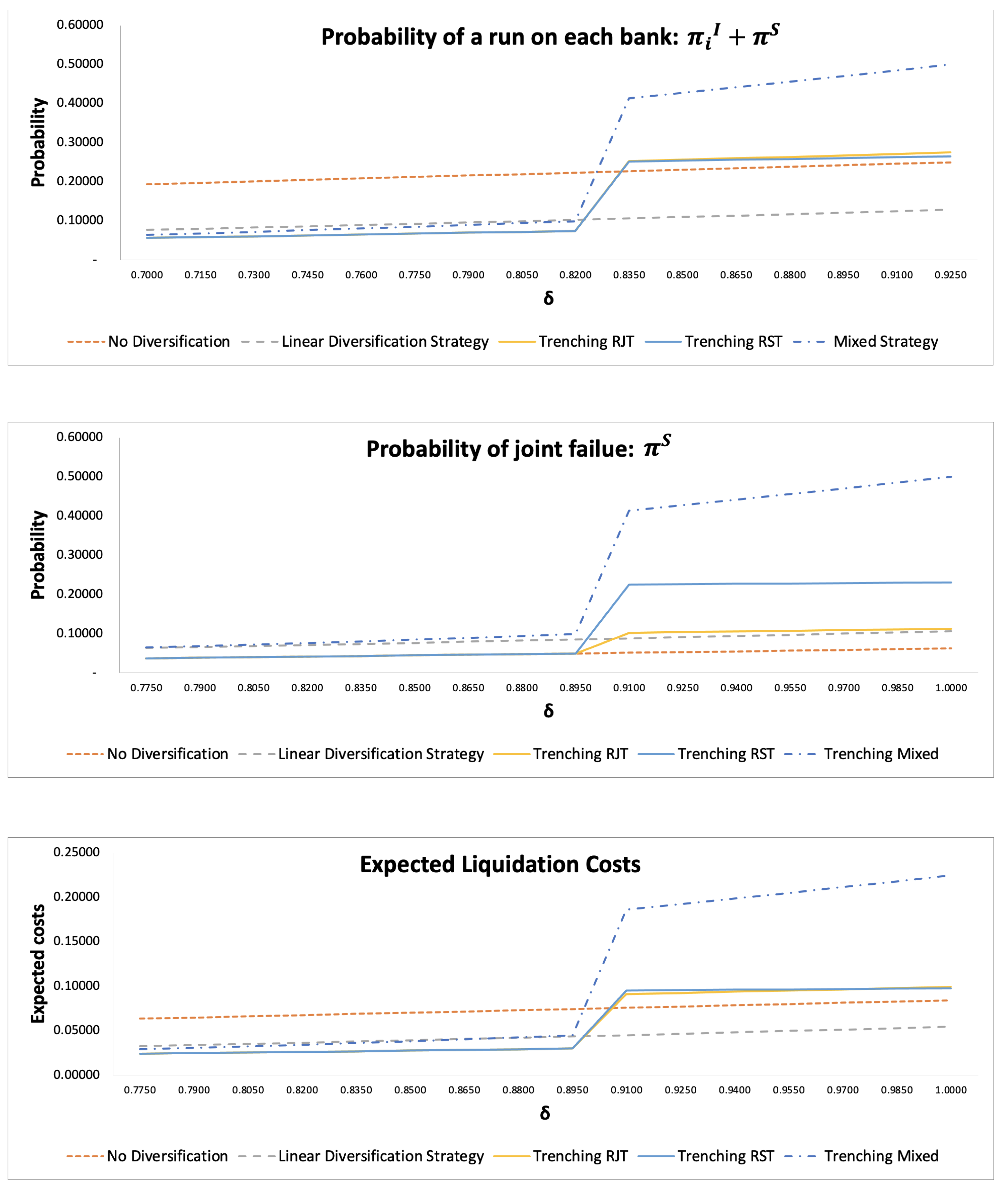
Figure 6 shows the results of expected liquidation costs, joint probability failure and individual bank failure for different levels of $\delta$7. We found that when there is a sudden loss of confidence from depositors, the strategy based on retaining the senior tranche creates a considerable increase in the probability of both bank failings when compared with RJT. However, when retaining the senior tranche, the probability of individual failures becomes slightly better than the RJT strategy. Notice how the expected default costs are lower for both RST and RJT when compared with the no tranche and linear diversification strategy whenever $d>\delta .$ As soon as this changes, that is after a confidence shock takes place, the expected default costs for both tranching strategies become considerably higher. One contribution of our simulation to the analysis of expected liquidation costs lies in showing that evaluating which strategy is considered “superior” depends on the magnitude of the shock. By observing the bottom graph in Figure 6, we see that RJT generates lower expected liquidation costs right after the shock takes place (i.e., for values of $\delta$ relatively close to 0.90) and that RST generates lower expected liquidation whenever $\delta >0.975.$ It then follows, in the case of relatively “big” surprises in depositor’s confidence (we can measure the size of the surprise as the difference between $\delta$ and d), that the total welfare of the economy would be better managed by banks if they use the RST strategy over the RJT strategy.

Figure 6. Diversification outcomes after a confidence shock.

Although, as it can be observed in Figure 6, the RST strategy leads to a considerably higher probability of systemic failure than the RJT strategy after the shock, RST does make the probability of individual failure smaller. In the case of securitization, as argued by Ordoñez (2018), the growth and collapse of securitization depend critically on the growth and collapse conditions that determine confidence in the system. Confidence, in turn, depends on reputation. Ordoñez (2018) defines reputation as the probability assigned by the market that a given firm is financially healthy. This generates incentives for banks to avoid bankruptcy whenever possible. It follows that a reasonable strategy for banks to avoid confidence shocks would be to avoid bankruptcy which, in this model, can be achieved by minimizing the probability of a banking institution failing. Under this assumption, banks will try to minimize the probability of a run by choosing the RST strategy. Such a decision would be even more clear if governments provide liquidity and support in the case of systemic crisis as the consequences of increasing the probability of a systemic failure would be mitigated; and the reputation problem of a bank failure will be carried by the entire banking system and not by one single institution.

The intuition of why the RST strategy seems to reduce the probability of individual failure comes from the fact that whenever the returns of the bank’s asset (say asset X) are very low, the return on the senior tranche that was exchanged in the case of the RJT strategy is bounded by $k.$ Hence, even if the return of asset Y is quite high, the bank can only achieve in this case a return of $k.$ In contrast, the return on the junior tranche that was exchanged with the other bank in the case of the RST strategy is not bounded by a minimum value, and it may leave open the possibility of no failure whenever y is sufficiently high.

Securitization strategies that exchange both tranches illustrate, along with vertical distribution, the dark side of diversification. It may be worth emphasizing the point that the mixed strategy can reduce the probability of individual failure when compared with the linear diversification strategy, but it does so at the cost of considerably increasing the probability of joint failure and the expected liquidation costs of banks in the case of confidence shocks. The analysis of confidence shocks reinforces the point that, from the perspective of managing risk, banks are better off by only exchanging one of the tranches rather than exchanging two.

5. Discussion

Banks can construct portfolios that are identical to linear diversification by using securitization strategies. That is to say, linear diversification is actually a subcase of securitization strategies designed around senior and junior tranches. As it has been argued by Wagner (2010) and van Oordt (2014), with linear strategies comes the problem of the dark side of diversification. In other words, linear diversification strategies have the problem that as banks reduce the probability of individual failure, then the failure of the entire banking system becomes more likely. Similar points have been illustrated in other theoretical and empirical studies (Acharya 2009; Allen et al. 2012; Allen and Carletti 2006; Ibragimov et al. 2011; Wagner 2010; Yang et al. 2020).

One way to avoid the dark side of diversification, as discussed by van Oordt (2014), is to keep the junior tranche and exchange the senior tranche (RJT). However, what about all other theoretical possibilities that are available within the securitization scheme? The present study provides an answer to this question by generalizing van Oordt’s (2014) model for long positions and studying the problem of diversification and systemic risk. In particular, it shows that the dark side of diversification can be also avoided by exchanging the entire junior tranche and keeping in full the senior (RST). We also showed that, in this case, there is no difference from the point of view of the welfare of the entire system. However, the strategy of keeping the senior tranche and exchanging the junior leads to worst outcomes, from the point of view of systemic risk, when confidence shocks are being considered. From the point of view of expected liquidation costs, we did not find major differences between RST and RJT. Regulators could potentially contribute to reduce confidence shocks by providing liquidity support in the case of a systemic failure.

When it comes to the use of a mixed strategy (i.e., exchanging a portion of both tranches), it is possible to reduce the probability of individual failure when compared to linear diversification. Nevertheless, such a strategy does not avoid the dark side of diversification. From the point of view of confidence shocks, mixed strategies that are close or equal to exchanging portions of both tranches in the same proportion result in worst outcomes than all other possible strategies. Hence, mixed strategies are problematic and should be avoided by banks. Accordingly, the benefits of securitization can only be felt at the extremes. By exchanging one of the tranches in its entirety, banks can reduce the risk of individual failure without increasing systemic risk, reduce social costs, and minimize idiosyncratic and systemic risks in the case of confidence shocks when compared with other securitization strategies.

The theoretical suggestion that banks are better off by adopting securitization strategies that exchange only one tranche seems to be in accordance with the idea that transparency is central in avoiding systemic crisis. After all, exchanging only one portion makes the banks portfolios less complicated, easier to track and, consequently, more transparent. As it has been argued by Klein et al. (2021), poor transparency of asset-backed securities exacerbated the latest subprime lending crisis. Our theoretical results do not contradict the importance of transparency in the banking system. In fact, it reinforces the relevance of this idea for banking institutions and regulators. In addition, our theoretical results are in line with the empirical work of Deku et al. (2019) in the sense that securitization is not a destabilizing tool per se. The destabilizing forces that are introduced by securitization depend on how the instruments are designed and used. Regulation, in turn, can eliminate undesired outcomes by, for example, enforcing the design of securitization instruments that don’t allow the exchange of both tranches.

We believe that both theoretical and empirical work are necessary for a better understanding of securitization, diversification and the fragility of the banking system. Theory can help illuminate the extent and detail of empirical studies, and empirical studies can guide the questions posed in theoretical work. To the best of our knowledge, most of the recent research in this area (during the past four years) has been predominantly empirical in its approach. Part of our contribution is based on the idea that theory may provide further sources for understanding the use of securitization tools and to better inform policy makers and regulators.

One direction that remains to be explored is to investigate if the conclusions of the theoretical framework presented in the present study hold when more banks and assets are considered.

Appendix A.1. Derivation of the No Bank Run Border ${\overline{y}}_1$(x)

The set of possible returns $(x,y)$ for bank i to be solvent is derived from solving $v_i^J(x,y)\ge d.$ Following (7), bank 1’s returns are given by,

$$v_1^J(x,y)=x+r_1^J[\mathrm{Max}\{y-k,0\}-\mathrm{Max}\{x-k,0\}]$$

The solution to the no bank run border can be found by the union of the following subsets:

$$A=\{x\in [0,k)\wedge y\in [0,k],\mathrm{where}x\ge d\}$$

$$B=\{x\in [0,k)\wedge y\in (k,s],\mathrm{where}y\ge (d-x+rk)/r\}$$

$$C=\{x\in [k,s]\wedge y\in [0,k],\mathrm{where}x\ge (d-rk)/(1-r\left)\right\}$$

$$D=\{x\in [k,s]\wedge y\in (k,s],\mathrm{where}y\ge (d-x+rx)/r\}$$

For keeping the senior tranche entirely, we separate the solution into two cases as shown in (8) and (9): case 1 where $k\ge d,$ and case 2 where $k<d.$

Case 1: Subset A is empty (i.e., the bank always fails) for $x\in [0,d)$. If $x\in [d,k)$ and $y\in [0,k]$; then, the condition is always fulfilled and the bank never fails. Subset B needs to satisfy $y\ge (d-x+rk)/r$ for $x\in [0,d)$ and $y\in [k,s]$. Within subset B if $x\in [d,k)$, then the condition is always satisfied since $(d-x)/r<0$ and $y\ge k.$ Subset C is always true because $x\ge k$ implies that $x\ge (d-rk)/(1-r)$ (i.e., $(x-rx)\ge (d-rk)$ since $x\ge d$ and $k\ge d$). Finally, the condition in subset D is always satisfied because $x-d\ge r(x-y)$, which is an expression that comes from rewriting $y\ge (d-x+rx)/r.$ The union of all subsets can be written as Equation (8), which provides the minimum return y that is necessary for bank 1 to be solvent given the returns of $x,$ whenever $k\ge d.$

Case 2: Subset A is empty given that $x\le d$ ($x\le k<d$). Subset B needs to satisfy the condition $y\ge (d-x+rk)/r$ for $x\in [0,k)$ as $(d-x)/r>0.$ Subset C is empty when $x\in [k,(d-rk)/(1-r\left)\right)$ since the condition would fail in this case. Notice that $\frac{d-rk}{1-r}>d$ (because $d-rk>d-rd$) and $\frac{d-rk}{1-r}>k$ (since $(d-rk)>(k-rk)$), given $k<d.$ If $x\in \left[\frac{d-rk}{1-r},s\right]$, then the condition of subset C is true and there is no bank run. The condition in subset D for $x\in [k,s]$ also has two parts. For $x\in \left[k,\frac{d-rk}{1-r}\right)$, the condition of D needs to be satisfied. If, on the other hand, $x\in \left[\frac{d-rk}{1-r},s\right]$, then the condition is true for the entire interval. To see this, notice that $y\ge \frac{d-x+rx}{r}\iff ry+x(1-r)\ge d.$ If we substitute the lowest possible value that x can take, namely $\frac{d-rk}{1-r}$, we would obtain $ry\ge d-\frac{d-rk}{1-r}(1-r)\iff ry\ge rk$ which is true since $y>k.$ If $ry+x(1-r)\ge d$ holds for $x=(d-rk)/(1-r)$, then it must hold also for $x>\frac{d-rk}{1-r}$; since $(1-r)>0.$ Hence, for this last interval, the condition in D is always satisfied, and there is no bank failure. The unions of these subsets result in Equation (9).

Appendix A.2. Proof of Proposition 1

The no tranching or no diversification case is given by setting $r_i^J=r_i^S=0.$ For bank 1, we know that its returns are given by,

$$v_1(x,y)=(1-r_1^J)\mathrm{Max}\{x-k,0\}+r_1^J\mathrm{Max}\{y-k,0\}+(1-r_1^S)\min \{x,k\}+r_1^S\min \{y,k\}$$

For $r_i^J=r_i^S=0$; $v_1(x,y)=x.$ Therefore, in this case, the return of each bank will depend solely on the asset owned. The no bank run border is given by Equations (8) and (9). Making $r=0,$ and if $x\in [0,d)$, then the banks fail in both case 1 and case 2. For case 1, we would end up with the condition that $d-x\le 0$ (after substituting r by zero), which cannot be true for $x<d.$ For case 2, first observe that the expression $\frac{d-rk}{1-r}=d$ when $r=0.$ If $x<k$, then we need to consider the first of the three expressions. In the first case, when substituting r by zero, we would have the same expression as in case 1, which is false for $x<d.$ If $x\in [k,d]$, we would then also have that $d-x\le 0,$ which fails for $x\le d.$ It follows that for the case of no trenching–no diversification, a systemic failure occurs when $x<d$ and $y<d.$

When allowing for trenching (RST) and linear diversification (via vertical distribution: $r_i^S=r_i^J$), we have the following results: For RST, if $x<d$ and $k\ge d,$ then Equation (8) cannot be satisfied since $ry\ge d-x+rk\iff x+ry\ge d+rk$, which is false for $x<d$ and $y<d.$ If $k<d,$ then we find $x+ry\ge d+rk$ is also false for $x<k<d$ and $y<d.$ When $x\in [k,d]$, then $ry\ge d-x+rx\iff r(y-x)\ge d-x$ fails for $y<d.$ Hence, for RST, both banks fail at the same time—due to symmetry—whenever $x<d\wedge y<d$ for any $k\in [0,s]$ and any $r\in [0,1]$.

For the case of linear diversification or vertical distribution, as shown by Wagner (2010) and van Oordt (2014), both banks fail whenever $x<d$ and $y<d,$ which holds in our case for any $r_i^S\in [0,1]$ and $r_i^J\in [0,1]$ as long as $r_i^S=r_i^J.$ Therefore, irrespective of tranching or linear diversification, a systemic failure takes place when both $x<d$ and $y<d$.

Appendix A.3. Proof of Proposition 2

We follow the same general strategy, and notation, used by van Oordt (2014). That is to say, we prove this proposition by showing that ${\pi }_1\ge {\pi }_1^{*}$ for any $k\in [0,s]$ and any $r\in [0,1]$, except $k=d$ and $r=1.$ The probability of a bank run for each strategy can be obtained by taking the surface integral of $\varphi (x,y)$ over the area below the no bank run border.

Figure A1. $0<r<1/2$.

Figure A2. $r>1/2,k\le \frac{(2r-1)d}{r}$.

Figure A3. $r>1/2,k>\frac{(2r-1)d}{r}$.

$$\begin{array}{cc}\hfill {\pi }_1={\int }_0^{\infty }{\int }_0^{{\overline{y}}_1\left(x\right)}\varphi (x,y)dydx& \hfill \end{array}$$

(A1)

$$\begin{array}{cc}\hfill {\pi }_1={\int }_0^d{\int }_0^d\varphi (x,y)dydx+{\int }_d^{\infty }{\int }_0^{{\overline{y}}_1\left(x\right)}\varphi (x,y)dydx+{\int }_0^d{\int }_d^{{\overline{y}}_1\left(x\right)}\varphi (x,y)dydx& \hfill \end{array}$$

(A2)

$$\begin{array}{cc}\hfill \equiv I_A^{*}+I_B+I_C& \hfill \end{array}$$

(A3)

$$\begin{array}{cc}\hfill {\pi }_1^{*}={\int }_0^d{\int }_0^d\varphi (x,y)dydx+{\int }_d^{2d}{\int }_0^{2d-x}\varphi (x,y)dydx& \hfill \end{array}$$

(A4)

$$\begin{array}{cc}\hfill \equiv I_A^{*}+I_B^{*}& \hfill \end{array}$$

(A5)

$$\begin{array}{cc}\hfill {\pi }_1^{*}={\int }_0^d{\int }_0^d\varphi (x,y)dydx+{\int }_0^d{\int }_d^{2d-x}\varphi (x,y)dydx& \hfill \end{array}$$

(A6)

$$\begin{array}{cc}\hfill \equiv I_A^{*}+I_C^{*}& \hfill \end{array}$$

(A7)

where $I_B^{*}=I_C^{*}.$ The strategy for proving ${\pi }_1\ge {\pi }_1^{*}$ is to show that either $I_B>I_B^{*}$ or $I_C>I_C^{*}.$ Comparing (A2), (A4) and (A6), it follows that it is sufficient to demonstrate either

$$\begin{array}{cc}\hfill {\overline{y}}_1\left(x\right)\ge 2d-x\mathrm{for}0<x<d,& \hfill \end{array}$$

(A8)

or

$$\begin{array}{cc}\hfill {\overline{y}}_1\left(x\right)\ge 2d-x\mathrm{for}d<x<2d& \hfill \end{array}$$

(A9)

We need to show this for any $k\in [0,s]$ and $r\in [0,1]$, except $k=d$ and $r=1.$ Starting with case 1 ($k\ge d$), the no bank run border is given by Equation (8). For $x\in [0,d)$, we must show that $(d-x+rk)/r\ge 2d-x.$ We can rewrite this expression as,

$$\begin{array}{cc}\hfill d-x+rk\ge (2d-x)r& \hfill \end{array}$$

(A10)

$$\begin{array}{cc}\hfill d-x\ge r(2d-x-k)& \hfill \end{array}$$

(A11)

Since $d\ge 2d-k,$ then the condition ${\overline{y}}_1\left(x\right)\ge 2d-x$ will always be true for any $r\in [0,1]$. For $x\in [d,s]$, there is no probability of failure, so it follows that ${\pi }_1={\pi }_1^{*}=0.$

We now consider Case 2 ($k<d$) where the no bank run border is given by Equation (9). First, we examine the case for $r\in [0,1/2)$ (Figure A1). For $x\in [0,d)$, there are two no bank run borders: one for $x\in [0,k)$ and another for $x\in [k,d)$. We start with $x\in [0,k)$, where we need to show that Equation (A11) is true for $r\in [0,1/2)$ but when $d>k.$ We begin by noticing that $(2d-x-k)>0$ and $(d-x)>0$ and that both are linear functions. Hence, the left-hand expression of Equation (A11) is bounded above by $\frac{1}{2}(2d-k)=d-\frac{k}{2}$, in which case the right-hand expression is equal to d satisfying the condition. Checking the other extreme, we notice that the right-hand expression of (A11) is bounded below by zero, in which case the right-hand side is $d-x$, satisfying the condition as well. It follows that ${\overline{y}}_1\left(x\right)\ge 2d-x$ for $r\in [0,1/2]$ and $k<d.$

For the case when $r\in (1/2,1]$ and $k\le \frac{(2r-1)d}{r}$, where Figure A2 provides an illustration, because of symmetry, we obtain,

$$\begin{array}{cc}\hfill {\int }_0^d{\int }_d^{2d}\varphi (x,y)dydx={\int }_d^{2d}{\int }_0^{2d-x}\varphi (x,y)dydx& \hfill \end{array}$$

(A12)

For $d<x<2d,$ we need to consider two parts. First, let us consider what happens when $d<x<\frac{d-rx}{1-r}$. From Equation (9), we need to show that $d-x+rx\ge 2rd-rx.$ This is equivalent to $2rx-x\ge 2rd-d\iff x(2r-1)\ge d(2r-2)$, which is true for $r\in (1/2,1]$. Second, we consider the case when $\frac{d-rk}{1-r}\le x<2d,$ in which case the bank cannot fail and, thus, there is no probability of failure (i.e., ${\pi }_1={\pi }_1^{*}=0$).

The last case, when $r\in (1/2,1]$ and $k<\frac{(2r-1)d}{r}$, is illustrated in Figure A3. For the first part of this case, we start by observing, in similar fashion as van Oordt (2014), that:

$$\begin{array}{cc}\hfill {\int }_0^m{\int }_d^{2d-x}\varphi (x,y)dydx>{\int }_0^m{\int }_c^{2d-x}\varphi (x,y)dydx={\int }_c^{2d}{\int }_0^{2d-x}\varphi (x,y)dydx& \hfill \end{array}$$

(A13)

where m is the value of x for which ${\overline{y}}_1\left(x\right)=2d-x$ in the interval $0<x<k.$ That results in $m=\frac{d(1-2r)+rk}{(1-r)}$. The inequality in (A13) holds because $c=\frac{d-rk}{1-r}>d,$ and equality holds because of the symmetry of $\varphi (x,y)$. We also know that,

$$\begin{array}{cc}\hfill {\int }_d^c{\int }_0^{2d-x}\varphi (x,y)dydx+{\int }_0^m{\int }_c^{2d-x}\varphi (x,y)dydx={\int }_d^{2d}{\int }_0^{2d-x}\varphi (x,y)dydx=I_B^{*}& \hfill \end{array}$$

(A14)

where,

$$\begin{array}{cc}\hfill {\int }_0^m{\int }_d^{2d-x}\varphi (x,y)dydx+{\int }_d^c{\int }_0^{2d-x}\varphi (x,y)dydx>I_B^{*}& \hfill \end{array}$$

(A15)

As a consequence, we need to demonstrate that ${\overline{y}}_1\left(x\right)\ge 2d-x$ for both $0<x<m$ and $d<x<c.$ In this manner, we will prove that ${\pi }_1>{\pi }_1^{*}.$ For $x\in (0,m)$, we need to demonstrate that $d-x+rk>2rd-rx.$ This is the same as $d(1-2r)+rk>x(1-r)\iff x<\frac{d(1-2r)+rk}{(1-r)}$. This is precisely the value of m, which is the upper bound of this interval. It follows that this condition is true for any $r\in (1/2,1]$. For $d<x<c,$ we need to show that $d-x+rx>2rd-rx.$ This expression can be also be written as $x(2r-1)>d(2r-1)$. This is true for any value of x in the interval and for any $r\in (1/2,1]$. Hence, ${\pi }_1>{\pi }_1^{*}$.

Appendix A.4. Proof of Proposition 3

The returns for bank 1 and 2 that are obtained from retaining the senior tranche (RST) strategy ($r_i^S=0\wedge r_i^J\in [0,1]$) are defined as,

$$\begin{array}{cc}\hfill v_1^J(x,y)=(1-r_1^J)x+r_1^J\min \{x,k\}+r_1^J\mathrm{Max}\{y-k,0\}& \hfill \end{array}$$

(A16)

$$\begin{array}{cc}\hfill v_2^J(x,y)=(1-r_2^J)y+r_2^J\min \{y,k\}+r_2^J\mathrm{Max}\{x-k,0\}& \hfill \end{array}$$

(A17)

The returns for bank 1 and 2 that are obtained from retaining the junior tranche (RJT) strategy ($r_i^J=0\wedge r_i^S\in [0,1]$) are defined as,

$$\begin{array}{cc}\hfill v_1^S(x,y)=(1-r_1^S)x+r_1^S\min \{y,k\}+r_1^S\mathrm{Max}\{x-k,0\}& \hfill \end{array}$$

(A18)

$$\begin{array}{cc}\hfill v_2^S(x,y)=(1-r_2^S)y+r_2^S\min \{x,k\}+r_2^S\mathrm{Max}\{y-k,0\}& \hfill \end{array}$$

(A19)

Since the returns on x and y are independent and identically distributed, and by comparing (A16) with (A19), and (A17) with (A18), it follows that:

$$\begin{array}{cc}\hfill \mathbb{E}\left[v_1^J(x,y)\right]=\mathbb{E}\left[v_2^S(x,y)\right]& \hfill \end{array}$$

(A20)

and

$$\begin{array}{cc}\hfill \mathbb{E}\left[v_2^J(x,y)\right]=\mathbb{E}\left[v_1^S(x,y)\right]& \hfill \end{array}$$

(A21)

The expected value for bank i is written as,

$$\begin{array}{cc}\hfill W_i=\mathbb{E}\left[v_i^J\right]-c({\pi }_i^I+q{\pi }^S)& \hfill \end{array}$$

Since the expected values are the same in both strategies (RST and RJT), it follows that maximizing the welfare is equivalent to minimizing the probability of individual failure. Since, as it was shown in the previous proposition, minimizing the risk entails setting $k=d$ and $r=1$ for both strategies, it then follows that the expected value of each bank is the same regardless of which of the two strategies is adopted. In addition, given that the total welfare of the economy is given by,

$$\begin{array}{cc}\hfill W_1+W_2=\mathbb{E}\left[v_1^J\right]+\mathbb{E}\left[v_2^J\right]-c({\pi }_1^I+{\pi }_2^I+2q{\pi }^S)& \hfill \end{array}$$

We see that the total welfare of the economy is the same if banks follow the optimal strategy for both cases (i.e., RST and RJT).

Appendix B.1. Case k ≤ d

The intercept of the $v_1=d$ with the vertical $\{x=0\}$ axis is $(0,y(m\left)\right)$ with $y\left(m\right)=\left(d+k(r^J-r^S)\right)/r^J.$ Notice that $y\left(m\right)>d\ge k.$ Of course, the point $(0,d)$ is in the default region.

The point $(k,y(k\left)\right)$ where the vertical line $\{x=k\}$ intersects $v_1=d$ is $y\left(k\right)=\left(d-k(1-r^J)\right)/r^J.$ It is easily verified that $d>y\left(k\right)>k.$ The boundary line determined by these points and $(d,d)$ consists of the lines

$$\begin{array}{c}\hfill y=y\left(m\right)-\frac{(1-r^S)}{r^J}x,\mathrm{between}(0,y\left(m\right))\mathrm{and}(k,y\left(k\right)),\\ \hfill y=y\left(k\right)-\frac{(1-r^J)}{r^J}(x-k),\mathrm{between}(k,y\left(k\right))\mathrm{and}(d,d).\end{array}$$

To determine the intercept of the line $v_1=d$ with the x-axis, solve $v_1(x\left(m\right),0)=d$ to obtain $x\left(m\right)=\left(d-k(r^J-r^S)\right)/(1-r^J).$ It follows that $x\left(m\right)>d\ge k.$ Let us now consider the intercept of the line $\{y=k\}$ with $v_1=d.$ Solving $v_1(x\left(k\right),k)=d$, we obtain $x\left(k\right)=\left(d-k{r}^J\right)/(1-r^J)>k.$ The rest of the boundary is given by the lines joining $(d,d)$ to $\left(x\right(k),k)$ and $\left(x\right(k),k)$ to $\left(x\right(m),0).$ These lines are given by

$$\begin{array}{c}\hfill y=d-\frac{1-r^J}{r^J}(x-d),\mathrm{between}(d,d)\mathrm{and}(x\left(k\right),k),\\ \hfill y=k-\frac{(1-r^J)}{r^S}(x-x\left(k\right)),\mathrm{between}(x\left(k\right),k)\mathrm{and}(x\left(m\right),0).\end{array}$$

Observe that the two central segments combine into one single line. The default region in this case is displayed in Figure A4.

Figure A4. Case $k\le d$.

Motion of the Breakpoints for the Case k < d

Let us examine how the breakpoints in Figure A4 move when the parameters $r^J$ and $r^S$ change. Let us examine the signs of the derivatives of $y\left(m\right),y\left(k\right),x\left(m\right),x\left(k\right)$ with respect to these parameters.

$$\begin{array}{c}\hfill \frac{dy\left(m\right)}{d{r}^J}=-\frac{y\left(m\right)-k}{r^J}<0;\frac{dy\left(m\right)}{d{r}^S}=-\frac{k}{r^J}<0;\frac{dy\left(k\right)}{d{r}^J}=-\frac{y\left(k\right)-k}{r^J}<0.\\ \\ \hfill \frac{dx\left(k\right)}{d{r}^J}=\frac{x\left(k\right)-k}{1-r^J}>0;\frac{dx\left(m\right)}{d{r}^J}=\frac{x\left(m\right)-k}{1-r^J}>0;\frac{dx\left(m\right)}{d{r}^S}=\frac{k}{1-r^J}>0.\end{array}$$

That is, the breakpoints on the “upper lid” of the default region move down along the line $y=k$ as the parameters increase, but the “right lid” moves out. This suggests that $r^J=r^S=1/2$ could be an optimal point.

Appendix B.2. Case d < k

This time, we have to consider two cases depending on whether $r^S\ge d/k$ or $r^S<d/k.$

Appendix B.2.1. Case r^S ≥ d/k

We have to examine two cases for the intercept $(0,y(m\left)\right)$ with $v_1=d$ . Either $d<y\left(m\right)\le k$ or $y\left(m\right)>k.$ In the first case, we have $y\left(m\right)=d/r^S$, which is compatible with the condition $r^S\ge d/k.$ In the second case, we obtain $y\left(m\right)=\left(d+k(r^J-r^S)\right)/r^J.$ The condition $y\left(m\right)>k$ implies that we must have $d/k>r^S$, which contradicts our assumption.

In the first case, the line

$$y=\frac{d}{r^S}-\frac{1-r^S}{r^S}x$$

is the boundary line connecting $(0,y(m\left)\right)$ to $(d,d).$ To find what happens to the right of $(d,d)$, we must consider two cases for the intercept $\left(x\right(m),0)$ and the line $v_1=d.$ Either $d\le x\left(m\right)\le k$ or $k<x\left(m\right).$ In the first case, we obtain $x\left(m\right)=d/(1-r^S)$ and the condition $x\left(m\right)\le k$ implies $k\ge 2d.$ In this case, the line from $(d,d)$ to $\left(x\right(m),0)$ is the default boundary. It is given by

$$y=d-\frac{1-r^S}{r^S}(x-d)$$

The default boundary in this case is displayed in Figure A5a below. Note that since the slope of the two segments is the same, the line is similar to that of Figure 1a.

Appendix B.2.2. Case r^S < d/k

In this case, it is easy to rule out the possibility of $d<y\left(m\right)\le k$ as incompatible with $r^S<d/k.$ The alternative $y\left(m\right)>k$ is $y\left(m\right)=\left(d+k(r^J-r^S)\right)/r^J.$ Then, in this case, there is a break point at $\left(x\right(k),k)$ with $x\left(k\right)=\left(d-r^{S}k\right)/(1-r^S).$ The boundary between $(0,y(m\left)\right)$ and $(d,d)$ consists of two lines given by

$$\begin{array}{c}\hfill y=\frac{d+k(r^J-r^S)}{r^J}-\frac{1-r^S}{r^J}x,\mathrm{between}(0,y\left(m\right))\mathrm{and}(x\left(k\right),k),\\ \hfill y=k-\frac{(1-r^S)}{r^S}(x-x\left(k\right)),\mathrm{between}(x\left(k\right),k)\mathrm{and}(d,d).\end{array}$$

To determine the default boundary beyond $(d,d)$, we also consider two possibilities for the intercept of $v_1=d$ and the $x$-axis. Either $d<x\left(m\right)\le k$ or $k<x\left(m\right).$ The first case leads to $x\left(m\right)=d/(1-r^S)$ with the condition $d\le 2k.$ The other case yields $x\left(m\right)=\left(d-k(r^J-r^S)\right)/(1-r^J).$ In this case, there is a break point at $(k,y(k\left)\right)$ with $y\left(k\right)=\left(d-k(1-r^S)\right)/r^S.$

$$\begin{array}{c}\hfill y=d-\frac{1-r^S}{r^S}(x-d),\mathrm{between}(d,d)\mathrm{and}(k,y\left(k\right)),\\ \hfill y=\frac{d-k(1-r^S)}{r^S}-\frac{(1-r^J)}{r^S}(x-k),\mathrm{between}(k,y\left(k\right))\mathrm{and}(x\left(m\right),0).\end{array}$$

Notice that again, the slopes of the lines $\left(x\right(k),k)\to (d,d)$ and $(d,d)\to (k,(y\left(k\right))$ are equal and the common line passes through $(d,d).$ This case is similar to the first case except that the roles of d and k are interchanged. The default region is displayed in Figure A5b.

Figure A5. The default region when $d<k$.

Appendix B.2.3. Motion of the Break Points for the Case k > d and r^S ≤ d/k

This time, we examine how the break points in Figure A5b move when the parameters $r^J$ and $r^S$ change. Again, we compute the derivatives of $y\left(m\right),x\left(k\right),y\left(k\right),x\left(m\right)$ with respect to these parameters.

$$\begin{array}{c}\hfill \frac{dy\left(m\right)}{d{r}^J}=-\frac{y\left(m\right)-k}{r^J}<0;\frac{dy\left(m\right)}{d{r}^S}=-\frac{k}{r^J}<0;\frac{dx\left(k\right)}{d{r}^S}=-\frac{k-x\left(k\right)}{1-r^S}<0.\\ \\ \hfill \frac{dy\left(k\right)}{d{r}^S}=\frac{x\left(k\right)+k}{1-r^S}>0;\frac{dx\left(m\right)}{d{r}^J}=\frac{x\left(m\right)-k}{1-r^J}>0;\frac{dx\left(m\right)}{d{r}^S}=\frac{k}{1-r^J}>0.\end{array}$$

Note that as $r^J$ increases, the point $x\left(k\right)$ moves to the right along the line that joins $(0,k)$ to $(k,k).$ Similarly, $y\left(k\right)$ slides up along the line from $(k,0)$ to $(k,k)$ when $r^S$ increases.

Appendix B.2.4. Analysis of the Effect of the Tranching When k = d

From the above calculations, it is clear that to decrease the individual probabilities of default, we must consider the case $k=d.$ We restrict our analysis to the case $d\le s/2.$ When $k=d$, the middle break point disappears, and the default boundary consists of two straight lines joining the points $(0,y(m\left)\right)$ and $\left(x\right(m),0)$ to $(d,d).$ Since this time (dropping the unnecessary subscript):

$$v_1(x,y)=(1-r^S)\min \{x,d\}+r^S\min \{y,d\}+(1-r^J)\mathrm{Max}\{x-d,0\}+r^J\mathrm{Max}\{y-d,0\}$$

As the cases $r^S=r^J$ or $1-r^S=r^J$ reduce to the case studied by Wagner, we shall keep them different and examine which combination of values is such that the default region is below the line $(x+y)/2=d.$ For that, we are going to examine the values $(r^S,r^J)\in {(0,1)}^2$ such that $d<y\left(m\right)<2d$ or $d<x\left(m\right)<2d.$ The computations are not difficult, and the result is contained in the following result.

Theorem A1.

With the notations introduced above, the intercepts $y\left(m\right)$ and $x\left(m\right)$ of the broken line $\{v_1(x,y)=d\}$ with the coordinate axes are given by

$$y\left(m\right)=\frac{1-r^S+r^J}{r^J}d,andx\left(m\right)=\frac{1+r^S-r^J}{1-r^J}d.$$

(A23)

The constraints upon $r^S$ and $r^J$ for $d<y\left(m\right)<2d$ and $d<x\left(m\right)<2d$ are given by:

$$r^S+r^J>1\Rightarrow d<y\left(m\right)<2dand{r}^S+r^J<1\Rightarrow d<x\left(m\right)<2d.$$

(A24)

The extreme cases will be discussed separately below. Note also that when $k=d$, the points $y\left(m\right)$ and $x\left(m\right)$ move in opposite directions along their axes when $r^S$ or $r^J$ change:

$$\begin{array}{c}\hfill \frac{\partial y\left(m\right)}{\partial r^J}<0;\frac{\partial y\left(m\right)}{\partial r^S}<0.\\ \\ \hfill \frac{\partial x\left(m\right)}{d{r}^J}>0;\frac{\partial x\left(m\right)}{\partial r^S}>0.\end{array}$$

(A25)

A little computation shows that the probability of default in this case is

$$P={\left(\frac{d}{s}\right)}^2\left(1+\frac{1}{2}\left(\frac{1-r^S}{r^J}+\frac{r^S}{1-r^J}\right)\right).$$

(A26)

Observe that for values of $r^J$ close to 0 or to $1,$ the expression for P becomes larger than $1.$ Therefore, we suppose that $r^J$ is close to $1/2$ in a range that depends on $r^S$ and $d/s.$

Appendix B.2.5. Analysis of the Probability of Default (A26)

We shall now verify that $(1/2,1/2)$ is a saddle point for the probability given by (A26). For short, let us write $K=d^2/2s^2$ for a while.

$$\frac{\partial P}{\partial r^S}=K\left(\frac{1-2r^J}{r^J(1-r^J)}\right),\frac{\partial P}{\partial r^J}=K\left(-\frac{1-r^S}{{\left(r^J\right)}^2}+\frac{r^S}{{(1-r^J)}^2}\right)$$

$r^J=1/2,r^S=1/2$ is a root of the system. The Hessian matrix at $(1/2,1/2)$ can be computed by differentiating the equations displayed above with respect to $r^S$ and/or $r^J$ and evaluating at $(1/2,1/2).$ It is given by

$$H\left(P\right)=\left(\begin{array}{cc}0& 8K\\ 8K& 16K\end{array}\right)$$

Its determinant is negative, which suggests a saddle point. The eigenvalues of $H\left(P\right)$ are

$${\lambda }_{+}=8K(1+\sqrt{2})>0>{\lambda }_{-}=8K(1-\sqrt{2}).$$

The two un-normalized eigenvectors are:

$${\mathit{v}}_{+}=\left(\genfrac{}{}{0pt}{}{\sqrt{2}-1}{1}\right),{\mathit{v}}_{+}=\left(\genfrac{}{}{0pt}{}{\sqrt{2}+1}{-1}\right)$$

They are clearly orthogonal. Let us denote their normalized versions by ${\mathit{e}}_{+}$ and ${\mathit{e}}_{-}.$ Notice now that if we set

$$\left(\genfrac{}{}{0pt}{}{r^S}{r^J}\right)=\left(\genfrac{}{}{0pt}{}{1/2}{1/2}\right)+a{\mathit{e}}_{-}$$

(A27)

with a small enough, then expanding $P(r^S,r^J)$ up to second order in a, we obtain

$$P(r^S,r^J)\approx 2{\left(\frac{d}{s}\right)}^2+\frac{a^2}{2}{\lambda }_{-}=2{\left(\frac{d}{s}\right)}^2\left(1-a^2(\sqrt{2}-1)\right).$$

(A28)

Similarly, substituting $r^S$ and $r^J$ obtained from (A27) into Equation (A23) yields the intersection points of the default domain with the $x,y$-axes. We round up the above remarks into:

Theorem A2.

With the notations introduced above, let $a\in \mathbb{R}$ be small enough so that $(r^S,r^J)=(1/2,1/2)+a{\mathit{e}}_{\in }{(0,1)}^2.$ Then, the probability of default of the hedge

$$v_1(x,y)=(1-r^S)\min \{x,d\}+r^S\min \{y,d\}+(1-r^J)\mathrm{Max}\{x-d,0\}+r_1^J\mathrm{Max}\{y-d,0\}$$

is given by (A28); that is, a can be chosen so that $P<2{(d/s)}^2.$

In Figure A6, we show the default region for this case relative to Wagner’s optimal case.

Figure A6. Default region for $k=d$.

Appendix B.2.6. Extreme Cases

Case 1: $r^S=r^J=r.$

This case reduces to Wagner’s (2010) case

$$v_1(x,y)=(1-r)x+ry.$$

In this case, we have two subcases for the intersects with the axes. If $2r>1$, we have $y\left(m\right)<2d$ and $x\left(m\right)>2d$ and the opposite happening when $2r<1.$ This was analyzed from a different point of view in Cadenas and Gzyl (2021).

Case 2: $r^S+r^J=1.$

Here, we obtain an extension of Wagner’s and Oordt’s hedges, which is given by

$$v_1(x,y)=(1-r)\min \{x,d\}+r\max \{x-d,0\}+r\min \{y,d\}+(1-r)\max \{y-d,0\}.$$

(A29)

We put $r^J=r$ for brevity. This is a rather curious case, as the probability of default of the bank is independent of the hedge, and it is easy to see from (A26) that it equals

$$P=2{\left(\frac{d}{s}\right)}^2$$

independently of the value of $r.$ It can be verified that in this case, the default boundary coincides with that of the previous case when $r=1/2.$

Case 3: $y\left(m\right)=d$

To set $y\left(m\right)=d$ requires that $r^S=1$, which in turn implies that $x\left(m\right)=d\left(1+1/(1-r^J)\right)\ge 2d.$ With an eye on the second equation in (A25), we see that if we make $r^J$ as small as possible, $x\left(m\right)$ will be as close as possible to $2d$ without violating the requirement $r^S+r^J>1$ for $d=y\left(m\right).$ Not only that, this is nice, because for $r^J$ being small, we insure that $2d\ge x\left(m\right)<s$ as well.

In this case, the probability of default (A26) reduces to

$$P={\left(\frac{d}{s}\right)}^2\left(1+\frac{1}{2}\frac{1}{1-r^J}\right).$$

(A30)

In this case, this is smaller than $2{(d/s)}^2$ for $r<1/2$ and tends to $3d^2/2s^2.$

Case 4: $x\left(m\right)=d.$

This time, it is required that $r^S=0$ and since $0\le r^J\le 1,$ necessarily $y\left(m\right)\ge 2d$ for the condition $r^S+r^J>1$ can not be satisfied. In addition, when $x\left(m\right)=d$, then $y\left(m\right)=d(1+r^J)/r^J\ge 2d$ for $0<r^J<1,$ and $y\left(m\right)=2d$ for $r^J=1.$ As above, when $r^J$ is close to $1,$ then $r^J>d/s$, and this is nice because $s>y\left(m\right)\ge 2d.$

Now, the probability of default is

$$P={\left(\frac{d}{s}\right)}^2\left(1+\frac{1}{2}\frac{1}{r^J}\right).$$

(A31)

We have $3d^2/2s^2\le P<2d^2/2s^2$ for $1/2<r^J\le 1.$

We close this section by pointing out that in Figure A7a,b, we display the case when the two banks adopt complementary hedges. This time, the systemic probability of default is not increased, while the individual default probabilities can be lowered. Apart from the possibility of using short positions analyzed in Cadenas and Gzyl (2021), this seems to be the only possibility of lowering the individual default probabilities without increasing the probability of systemic default.

Figure A7. Complementary hedges for the two banks.