Mean Heart Dose Prediction Using Parameters of Single-Slice Computed Tomography and Body Mass Index: Machine Learning Approach for Radiotherapy of Left-Sided Breast Cancer of Asian Patients
Abstract
1. Introduction
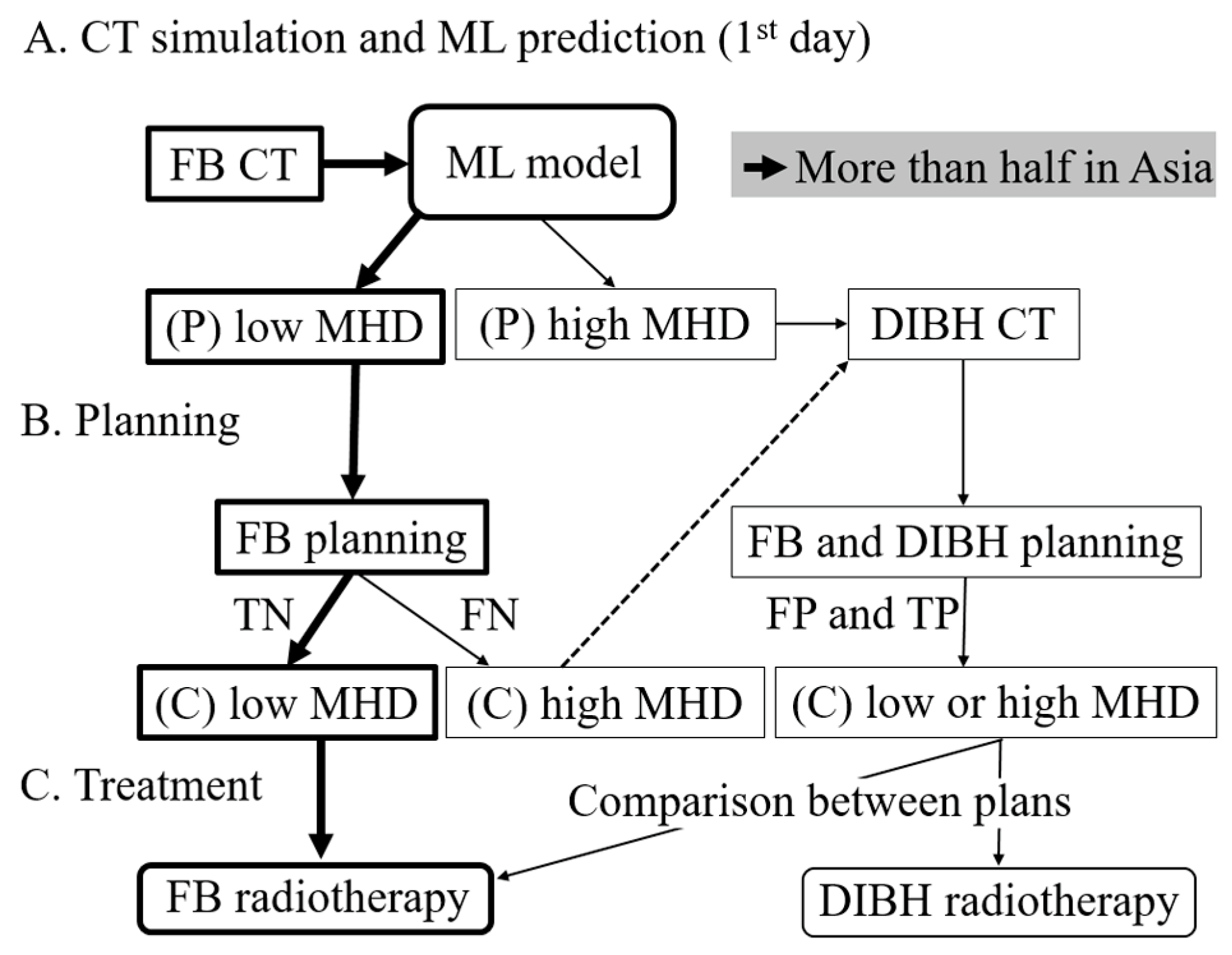
2. Materials and Methods
2.1. Dataset and Study Setting
2.2. ML Algorithms
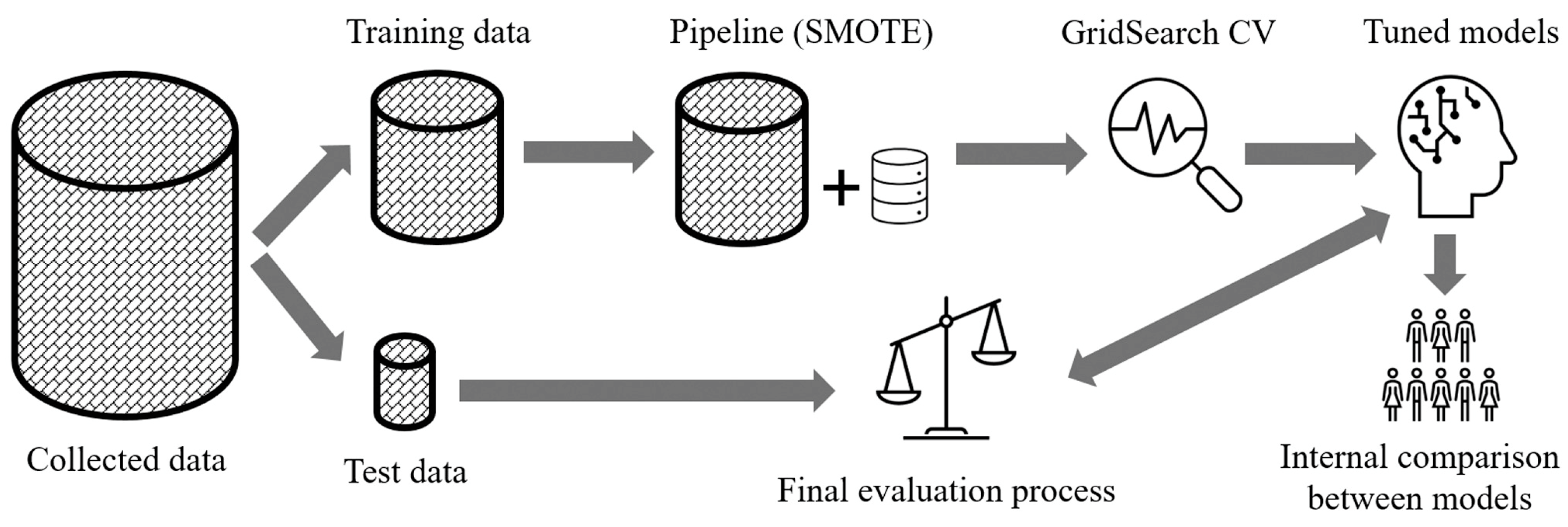
2.3. Models Building
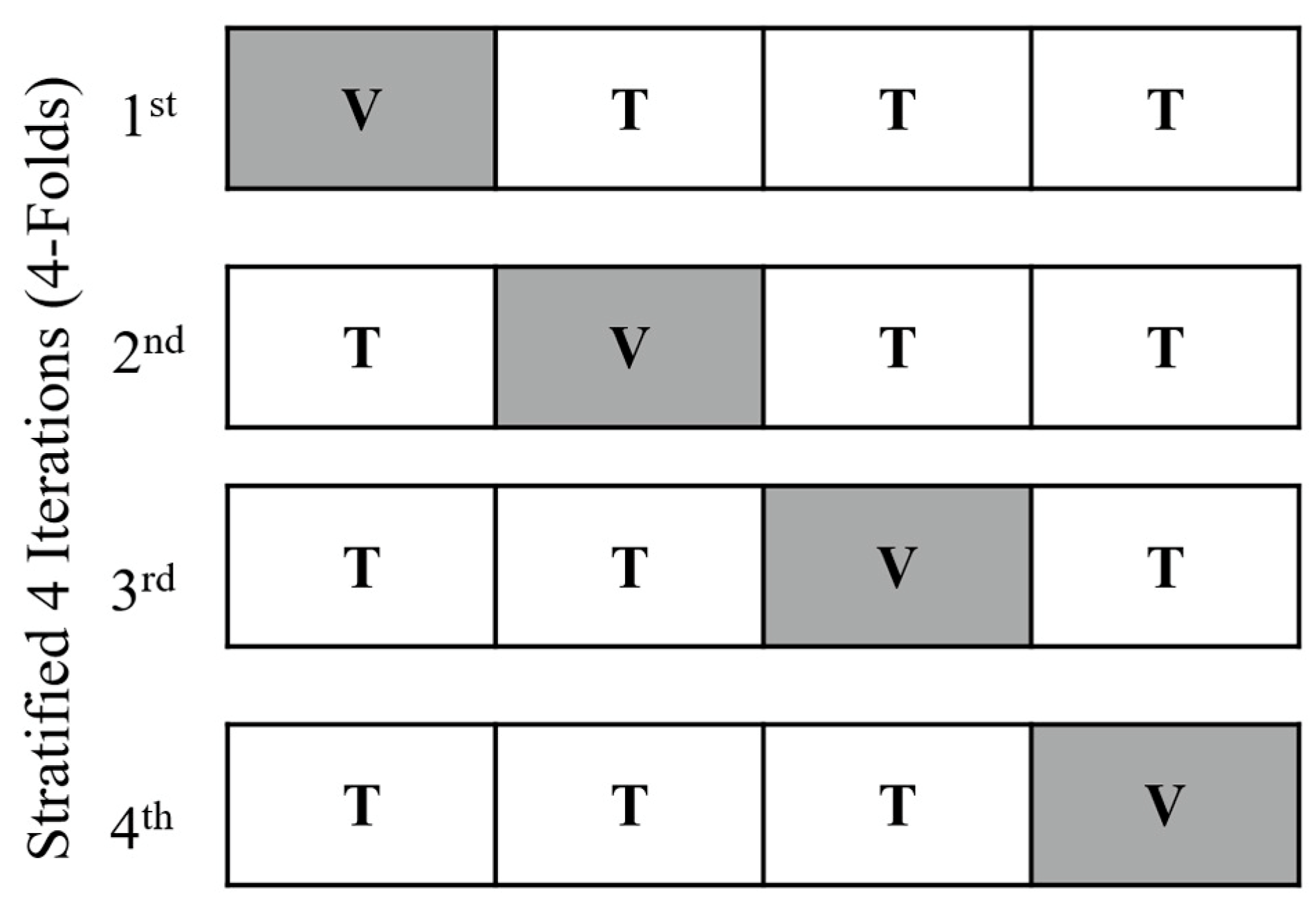
2.4. Models Performance Testing
3. Results
3.1. Hyperparameter Tuning
3.2. Internal Comparison between Models
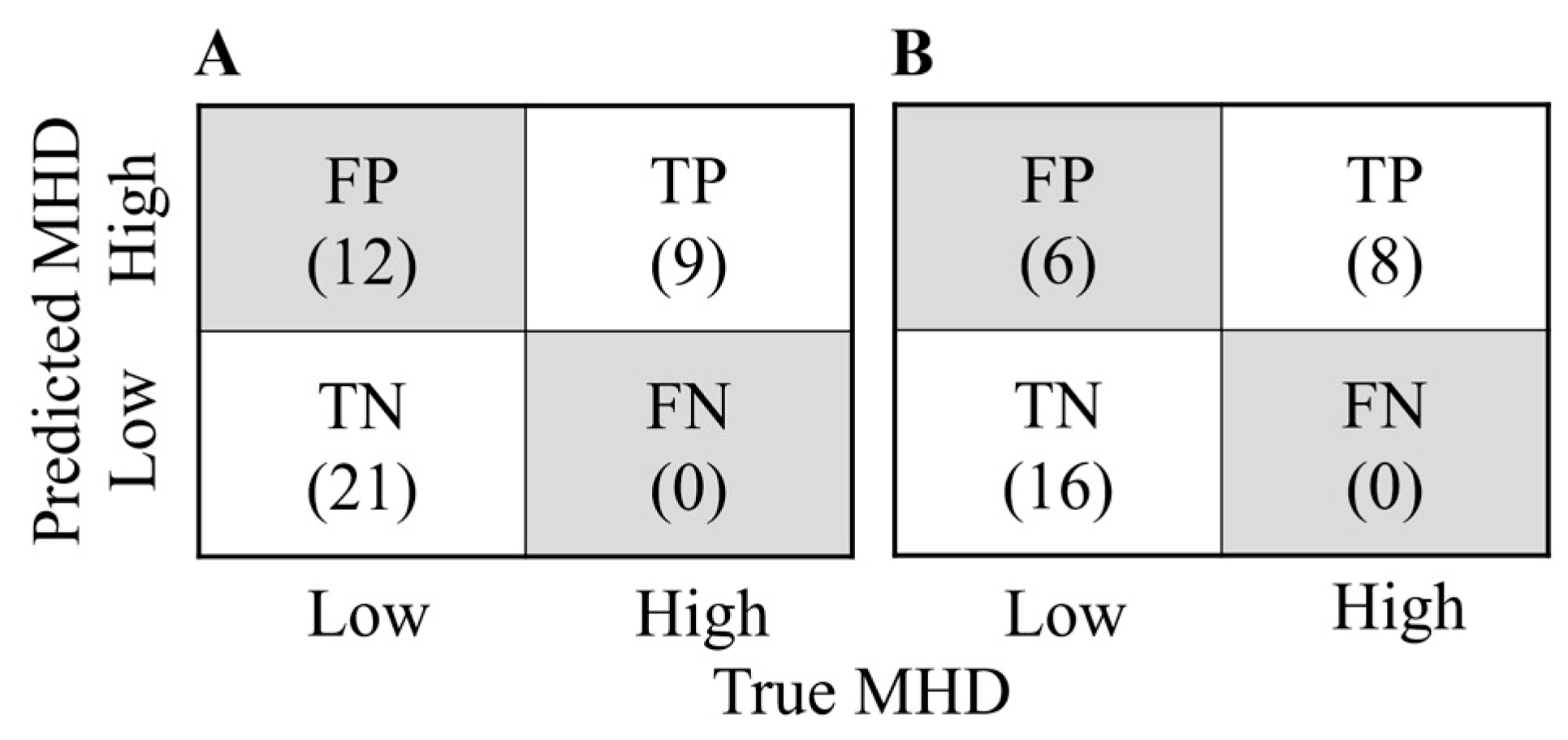
3.3. Final Evaluation of Model Performances
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Darby, S.; McGale, P.; Correa, C.; Taylor, C.; Arriagada, R.; Clarke, M.; Cutter, D.; Davies, C.; Ewertz, M.; Godwin, J.; et al. Effect of radiotherapy after breast-conserving surgery on 10-year recurrence and 15-year breast cancer death: Meta-analysis of individual patient data for 10,801 women in 17 randomised trials. Lancet 2011, 378, 1707–1716. [Google Scholar] [CrossRef]
- Clarke, M.; Collins, R.; Darby, S.; Davies, C.; Elphinstone, P.; Evans, V.; Godwin, J.; Gray, R.; Hicks, C.; James, S.; et al. Effects of radiotherapy and of differences in the extent of surgery for early breast cancer on local recurrence and 15-year survival: An overview of the randomised trials. Lancet 2005, 366, 2087–2106. [Google Scholar] [CrossRef]
- Meattini, I.; Guenzi, M.; Fozza, A.; Vidali, C.; Rovea, P.; Meacci, F.; Livi, L. Overview on cardiac, pulmonary and cutaneous toxicity in patients treated with adjuvant radiotherapy for breast cancer. Breast Cancer 2016, 24, 52–62. [Google Scholar] [CrossRef]
- Taylor, C.; Correa, C.; Duane, F.K.; Aznar, M.C.; Anderson, S.J.; Bergh, J.; Dodwell, D.; Ewertz, M.; Gray, R.; Jagsi, R.; et al. Estimating the risks of breast cancer radiotherapy: Evidence from modern radiation doses to the lungs and heart and from previous randomized trials. J. Clin. Oncol. 2017, 35, 1641–1649. [Google Scholar] [CrossRef]
- Drost, L.; Yee, C.; Lam, H.; Zhang, L.; Wronski, M.; McCann, C.; Lee, J.; Vesprini, D.; Leung, E.; Chow, E. A systematic review of heart dose in breast radiotherapy. Clin. Breast Cancer 2018, 18, e819–e824. [Google Scholar] [CrossRef] [PubMed]
- Beaton, L.; Bergman, A.; Nichol, A.; Aparicio, M.; Wong, G.; Gondara, L.; Speers, C.; Weir, L.; Davis, M.; Tyldesley, S. Cardiac death after breast radiotherapy and the QUANTEC cardiac guidelines. Clin. Transl. Radiat. Oncol. 2019, 19, 39–45. [Google Scholar] [CrossRef] [PubMed]
- Lu, Y.; Yang, D.; Zhang, X.; Teng, Y.; Yuan, W.; Zhang, Y.; He, R.; Tang, F.; Pang, J.; Han, B.; et al. Comparison of deep inspiration breath hold versus free breathing in radiotherapy for left sided breast cancer. Front. Oncol. 2022, 12, 845037. [Google Scholar] [CrossRef]
- Falco, M.; Masojć, B.; Macała, A.; Łukowiak, M.; Woźniak, P.; Malicki, J. Deep inspiration breath hold reduces the mean heart dose in left breast cancer radiotherapy. Radiol. Oncol. 2021, 55, 212–220. [Google Scholar] [CrossRef] [PubMed]
- Yamauchi, R.; Mizuno, N.; Itazawa, T.; Saitoh, H.; Kawamori, J. Dosimetric evaluation of deep inspiration breath hold for left-sided breast cancer: Analysis of patient-specific parameters related to heart dose reduction. J. Radiat. Res. 2020, 61, 447–456. [Google Scholar] [CrossRef]
- Darapu, A.; Balakrishnan, R.; Sebastian, P.; Kather Hussain, M.R.; Ravindran, P.; John, S. Is the deep inspiration breath-hold technique superior to the free breathing technique in cardiac and lung sparing while treating both left-sided post-mastectomy chest wall and supraclavicular regions. Case Rep. Oncol. 2017, 10, 37–51. [Google Scholar] [CrossRef]
- Teshima, T.; Owen, J.B.; Hanks, G.E.; Sato, S.; Tsunemoto, H.; Inoue, T. A comparison of the structure of radiation oncology in the United States and Japan. Int. J. Radiat. Oncol. Biol. Phys. 1996, 34, 235–242. [Google Scholar] [CrossRef]
- Nakamura, K.; Konishi, K.; Komatsu, T.; Sasaki, T.; Shikama, N. Patterns of radiotherapy infrastructure in Japan and in other countries with well-developed radiotherapy infrastructures. Jpn. J. Clin. Oncol. 2018, 48, 476–479. [Google Scholar] [CrossRef] [PubMed]
- Ishizaka, H.; Kuroda, M.; Tekiki, N.; Khasawneh, A.; Barham, M.; Hamada, K.; Konishi, K.; Sugimoto, K.; Katsui, K.; Sugiyama, S.; et al. Investigation into the effect of breast volume on irradiation dose distribution in Asian women with breast cancer. Acta Med. Okayama 2021, 75, 307–314. [Google Scholar] [CrossRef] [PubMed]
- Siddique, S.; Chow, J.C.L. Artificial intelligence in radiotherapy. Rep. Pract. Oncol. Radiother. 2020, 25, 656–666. [Google Scholar] [CrossRef]
- Kang, J.; Schwartz, R.; Flickinger, J.; Beriwal, S. Machine learning approaches for predicting radiation therapy outcomes: A clinician’s perspective. Int. J. Radiat. Oncol. Biol. Phys. 2015, 93, 1127–1135. [Google Scholar] [CrossRef]
- Luo, Y.; Chen, S.; Valdes, G. Machine learning for radiation outcome modeling and prediction. Med. Phys. 2020, 47, e178–e184. [Google Scholar] [CrossRef]
- Raman, R.; Gupta, N.; Jeppu, Y. Framework for formal verification of machine learning based complex system-of-systems. INSIGHT 2023, 26, 91–102. [Google Scholar] [CrossRef]
- Tekiki, N.; Kuroda, M.; Ishizaka, H.; Khasawneh, A.; Barham, M.; Hamada, K.; Konishi, K.; Sugimoto, K.; Katsui, K.; Sugiyama, S.; et al. New field-in-field with two reference points method for whole breast radiotherapy: Dosimetric analysis and radiation-induced skin toxicities assessment. Mol. Clin. Oncol. 2021, 15, 193. [Google Scholar] [CrossRef]
- Alghamdi, M.; Al-Mallah, M.; Keteyian, S.; Brawner, C.; Ehrman, J.; Sakr, S. Predicting diabetes mellitus using SMOTE and ensemble machine learning approach: The Henry Ford ExercIse Testing (FIT) project. Liu B, editor. PLoS ONE 2017, 12, e0179805. [Google Scholar] [CrossRef]
- Khushi, M.; Shaukat, K.; Alam, T.M.; Hameed, I.A.; Uddin, S.; Luo, S.; Yang, X.; Reyes, M.C. A comparative performance analysis of data resampling methods on imbalance medical data. IEEE Access 2021, 9, 109960–109975. [Google Scholar] [CrossRef]
- Koide, Y.; Shimizu, H.; Wakabayashi, K.; Kitagawa, T.; Aoyama, T.; Miyauchi, R.; Tachibana, H.; Kodaira, T. Synthetic breath-hold CT generation from free-breathing CT: A novel deep learning approach to predict cardiac dose reduction in deep-inspiration breath-hold radiotherapy. J. Radiat. Res. 2021, 62, 1065–1075. [Google Scholar] [CrossRef] [PubMed]
- Brodin, N.P.; Schulte, L.; Velten, C.; Martin, W.; Shen, S.; Shen, J.; Basavatia, A.; Ohri, N.; Garg, M.K.; Carpenter, C.; et al. Organ-at-risk dose prediction using a machine learning algorithm: Clinical validation and treatment planning benefit for lung SBRT. J. Appl. Clin. Med. Phys. 2022, 23, e13609. [Google Scholar] [CrossRef] [PubMed]
- Ahn, S.H.; Kim, E.; Kim, C.; Cheon, W.; Kim, M.; Lee, S.B.; Lim, Y.K.; Kim, H.; Shin, D.; Kim, D.Y.; et al. Deep learning method for prediction of patient-specific dose distribution in breast cancer. Radiat. Oncol. 2021, 16, 154. [Google Scholar] [CrossRef] [PubMed]
- Koide, Y.; Aoyama, T.; Shimizu, H.; Kitagawa, T.; Miyauchi, R.; Tachibana, H.; Kodaira, T. Development of deep learning chest X-ray model for cardiac dose prediction in left-sided breast cancer radiotherapy. Sci. Rep. 2022, 12, 13706. [Google Scholar] [CrossRef]
- Mkanna, A.; Mohamad, O.; Ramia, P.; Thebian, R.; Makki, M.; Tamim, H.; Jalbout, W.; Youssef, B.; Eid, T.; Geara, F.; et al. Predictors of cardiac sparing in deep inspiration breath-hold for patients with left sided breast cancer. Front. Oncol. 2018, 8, 564. [Google Scholar] [CrossRef]
- Koide, Y.; Shimizu, H.; Aoyama, T.; Kitagawa, T.; Miyauchi, R.; Watanabe, Y.; Tachibana, H.; Kodaira, T. Preoperative spirometry and BMI in deep inspiration breath-hold radiotherapy: The early detection of cardiac and lung dose predictors without radiation exposure. Radiat. Oncol. 2022, 17, 35. [Google Scholar] [CrossRef] [PubMed]
- Sarker, I.H. Machine learning: Algorithms, real-world applications and research directions. SN Comput. Sci. 2021, 2, 160. [Google Scholar] [CrossRef] [PubMed]
- Uddin, S.; Khan, A.; Hossain, M.E.; Moni, M.A. Comparing different supervised machine learning algorithms for disease prediction. BMC Med. Inform. Decis. Mak. 2019, 19, 281. [Google Scholar] [CrossRef]
- Hou, C.; Zhong, X.; He, P.; Xu, B.; Diao, S.; Yi, F.; Zheng, H.; Li, J. Predicting breast cancer in Chinese women using machine learning techniques: Algorithm development. JMIR Med. Inform. 2020, 8, e17364. [Google Scholar] [CrossRef]
- Deist, T.M.; Dankers, F.J.W.M.; Valdes, G.; Wijsman, R.; Hsu, I.; Oberije, C.; Lustberg, T.; van Soest, J.; Hoebers, F.; Jochems, A.; et al. Machine learning algorithms for outcome prediction in (chemo)radiotherapy: An empirical comparison of classifiers. Med. Phys. 2018, 45, 3449–3459. [Google Scholar] [CrossRef]
- Li, B. Multi-Chaos, Fractal and Multi-Fractional Artificial Intelligence of Different Complex Systems; Elsevier Science: Amsterdam, The Netherlands, 2022; pp. 263–276. Available online: https://www.google.co.jp/books/edition/Multi_Chaos_Fractal_and_Multi_Fractional/D4RTEAAAQBAJ?hl=en&gbpv=1&dq=cross+validation+different+from+final+test&pg=PA269&printsec=frontcover (accessed on 29 December 2022).
- Bleeker, S.E.; Moll, H.A.; Steyerberg, E.W.; Donders, A.R.T.; Derksen-Lubsen, G.; Grobbee, D.E.; Moons, K.G. External validation is necessary in prediction research: A clinical example. J. Clin. Epidemiol. 2003, 56, 826–832. [Google Scholar] [CrossRef] [PubMed]
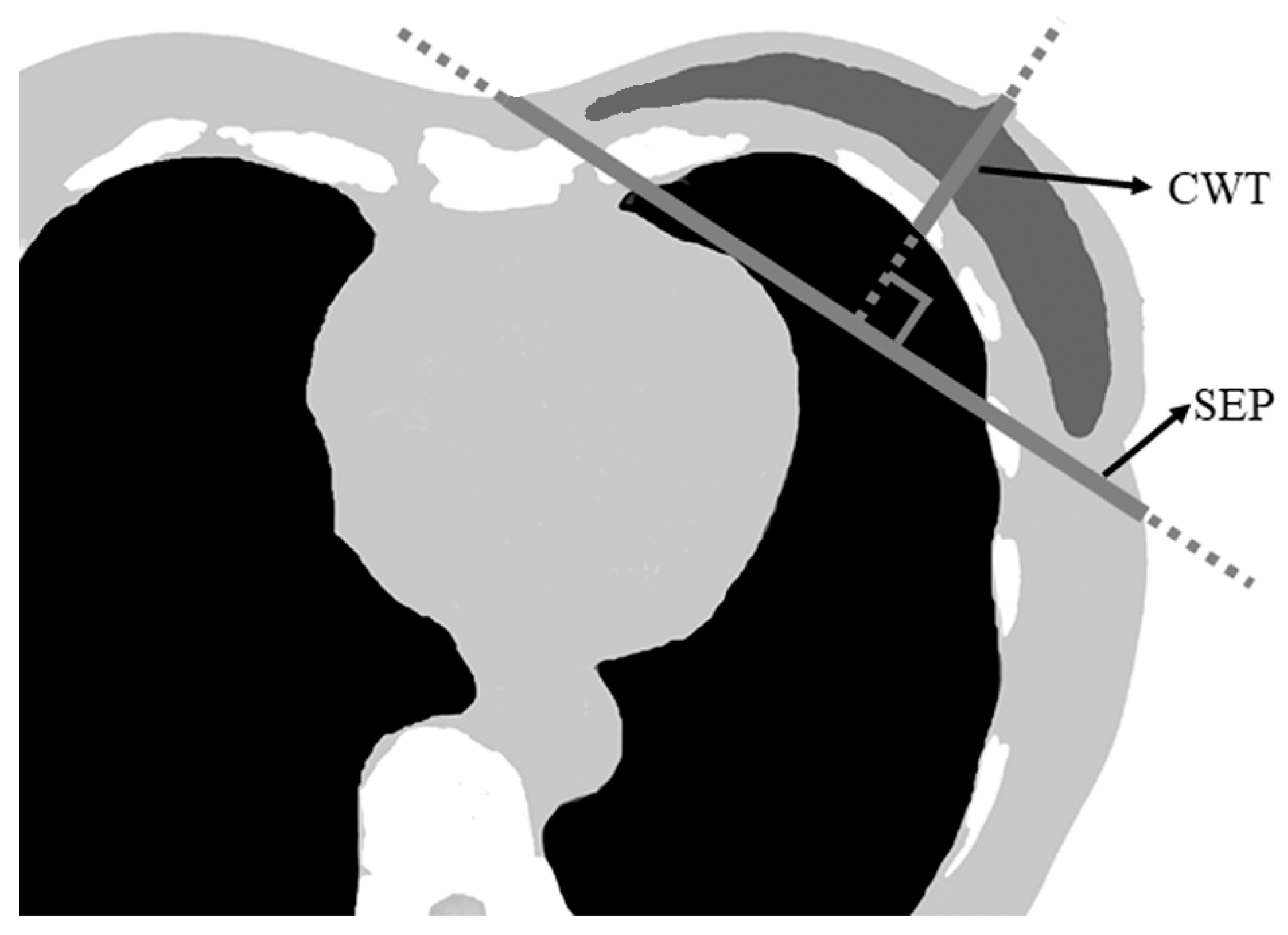

| Variable | Characteristics | Correlation Coefficient | p-Value |
|---|---|---|---|
| Body mass index (median (IQR)) | 22.2 (20.2–25.2) | 0.408 a | <0.001 |
| Chest wall thickness (median (IQR), cm) | 6 (5.1–6.8) | 0.335 a | <0.001 |
| Separation (median (IQR), cm) | 19 (17.1–20.2) | 0.290 a | <0.001 |
| Tumor site (%) | 0.134 b | 0.456 | |
| Upper-inner quadrant | 27.1% | ||
| Lower-inner quadrant | 9.2% | ||
| Upper-outer quadrant | 51.7% | ||
| Lower-outer quadrant | 4.8% | ||
| Central portion | 7.2% | ||
| Radiation method; n | 0.054 b | 0.452 | |
| FIF-1RP | 70 | ||
| FIF-2RP | 137 | ||
| Age (median (IQR), years) | 56 (46–64) | 0.006 a | 0.926 |
| Machine Learning Algorithm | Hyperparameter Name | Best Value |
|---|---|---|
| Deep Neural Network | batch_size | 32 |
| dropout | 0.1 | |
| epoch | 10 | |
| optimizer | “adam” | |
| activation | “relu”, “sigmoid” | |
| init | “uniform” | |
| dense_nparams | 256 | |
| Random Forest | max_depth | 2 |
| max_features | “sqrt” | |
| min_samples_split | 2 | |
| n_estimators | 5 | |
| K-Nearest Neighbors | metric | “euclidean” |
| n_neighbors | 37 | |
| weights | “uniform” | |
| Bagging | max_samples | 0.1 |
| n_estimators | 37 | |
| Gradient Boosting | learning_rate | 0.001 |
| max_depth | 2 | |
| n_estimators | 15 | |
| subsample | 0.1 | |
| Support Vector Machine | C | 0.11 |
| gamma | “scale” | |
| kernel | “rbf” | |
| Decision Tree | max_depth | 1 |
| min_samples_split | 2 | |
| Naïve Bayes | alpha | 0.081 |
| Ridge Classifier | var_smoothing | 0.001 |
| Logistic Regression | C | 0.01 |
| Penalty | “l2” | |
| solver | “liblinear” |
| Classifier | F2 Score | AUC | Recall | Accuracy | Cohen’s Kappa | MCC |
|---|---|---|---|---|---|---|
| Deep Neural Network | 0.600 | 0.607 | 0.677 | 0.617 | 0.199 | 0.251 |
| Random Forest | 0.606 | 0.681 | 0.760 | 0.636 | 0.250 | 0.297 |
| K-Nearest Neighbors | 0.665 | 0.722 | 0.850 | 0.648 | 0.298 | 0.364 |
| Bagging | 0.619 | 0.709 | 0.732 | 0.696 | 0.320 | 0.352 |
| Gradient Boosting | 0.654 | 0.724 | 0.791 | 0.685 | 0.342 | 0.382 |
| Support Vector Machine | 0.621 | 0.687 | 0.795 | 0.624 | 0.235 | 0.282 |
| Decision Tree | 0.584 | 0.648 | 0.763 | 0.581 | 0.193 | 0.241 |
| Naïve Bayes | 0.580 | 0.671 | 0.701 | 0.654 | 0.255 | 0.285 |
| Ridge Classifier | 0.504 | 0.634 | 0.590 | 0.660 | 0.208 | 0.227 |
| Logistic Regression | 0.587 | 0.679 | 0.701 | 0.666 | 0.274 | 0.301 |
| Classifier | F2 Score | AUC | Recall | Accuracy | Cohen’s Kappa | MCC |
|---|---|---|---|---|---|---|
| Deep Neural Network | 0.789 | 0.818 | 1 | 0.714 | 0.428 | 0.522 |
| Random Forest | 0.775 | 0.803 | 1 | 0.690 | 0.397 | 0.497 |
| K-Nearest Neighbors | 0.775 | 0.803 | 1 | 0.690 | 0.397 | 0.497 |
| Bagging | 0.775 | 0.803 | 1 | 0.690 | 0.397 | 0.497 |
| Gradient Boosting | 0.762 | 0.787 | 1 | 0.666 | 0.367 | 0.474 |
| Support Vector Machine | 0.762 | 0.787 | 1 | 0.666 | 0.367 | 0.474 |
| Decision Tree | 0.725 | 0.742 | 1 | 0.714 | 0.287 | 0.409 |
| Naïve Bayes | 0.714 | 0.762 | 0.888 | 0.690 | 0.363 | 0.431 |
| Ridge Classifier | 0.714 | 0.762 | 0.888 | 0.690 | 0.363 | 0.431 |
| Logistic Regression | 0.701 | 0.747 | 0.888 | 0.666 | 0.333 | 0.406 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Al-Hammad, W.E.; Kuroda, M.; Kamizaki, R.; Tekiki, N.; Ishizaka, H.; Kuroda, K.; Sugimoto, K.; Oita, M.; Tanabe, Y.; Barham, M.; et al. Mean Heart Dose Prediction Using Parameters of Single-Slice Computed Tomography and Body Mass Index: Machine Learning Approach for Radiotherapy of Left-Sided Breast Cancer of Asian Patients. Curr. Oncol. 2023, 30, 7412-7424. https://doi.org/10.3390/curroncol30080537
Al-Hammad WE, Kuroda M, Kamizaki R, Tekiki N, Ishizaka H, Kuroda K, Sugimoto K, Oita M, Tanabe Y, Barham M, et al. Mean Heart Dose Prediction Using Parameters of Single-Slice Computed Tomography and Body Mass Index: Machine Learning Approach for Radiotherapy of Left-Sided Breast Cancer of Asian Patients. Current Oncology. 2023; 30(8):7412-7424. https://doi.org/10.3390/curroncol30080537
Chicago/Turabian StyleAl-Hammad, Wlla E., Masahiro Kuroda, Ryo Kamizaki, Nouha Tekiki, Hinata Ishizaka, Kazuhiro Kuroda, Kohei Sugimoto, Masataka Oita, Yoshinori Tanabe, Majd Barham, and et al. 2023. "Mean Heart Dose Prediction Using Parameters of Single-Slice Computed Tomography and Body Mass Index: Machine Learning Approach for Radiotherapy of Left-Sided Breast Cancer of Asian Patients" Current Oncology 30, no. 8: 7412-7424. https://doi.org/10.3390/curroncol30080537
APA StyleAl-Hammad, W. E., Kuroda, M., Kamizaki, R., Tekiki, N., Ishizaka, H., Kuroda, K., Sugimoto, K., Oita, M., Tanabe, Y., Barham, M., Sugianto, I., Shimizu, Y., Nakamitsu, Y., & Asaumi, J. (2023). Mean Heart Dose Prediction Using Parameters of Single-Slice Computed Tomography and Body Mass Index: Machine Learning Approach for Radiotherapy of Left-Sided Breast Cancer of Asian Patients. Current Oncology, 30(8), 7412-7424. https://doi.org/10.3390/curroncol30080537





