Effect of Parameters of Pool Geometry on Flow Structure in Nature-like Fishway
Abstract
:1. Introduction
2. Materials and Methods
2.1. Study Sites
2.2. Generalization Strategy and Numerical Model of Nature-Like Fishways
2.3. Model Validation
2.4. Cases
2.5. Indicators and Analysis Methods
3. Results and Discussion
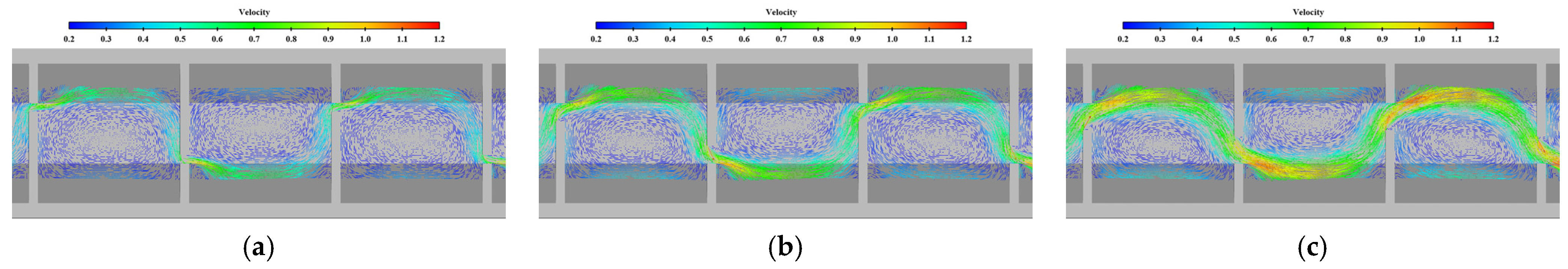
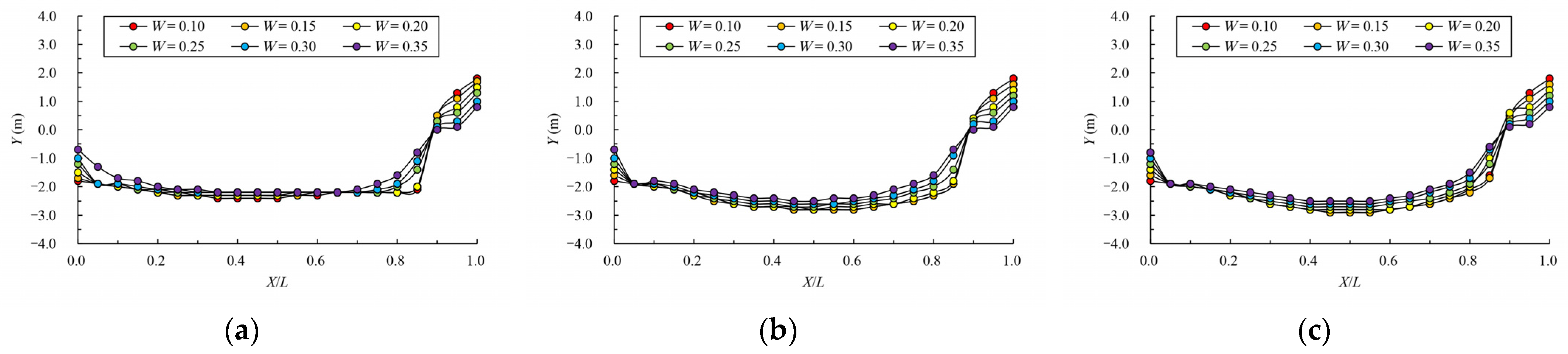
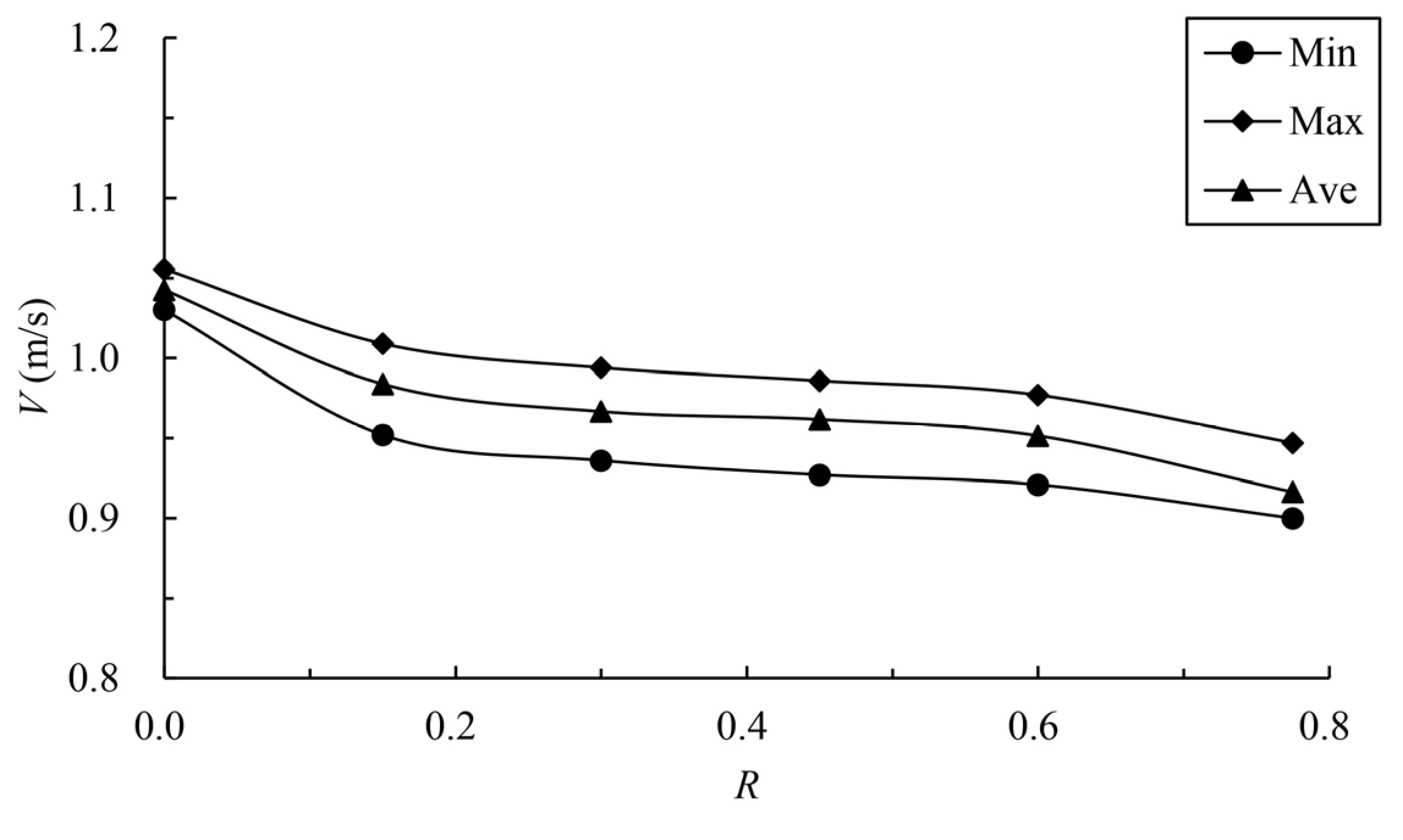
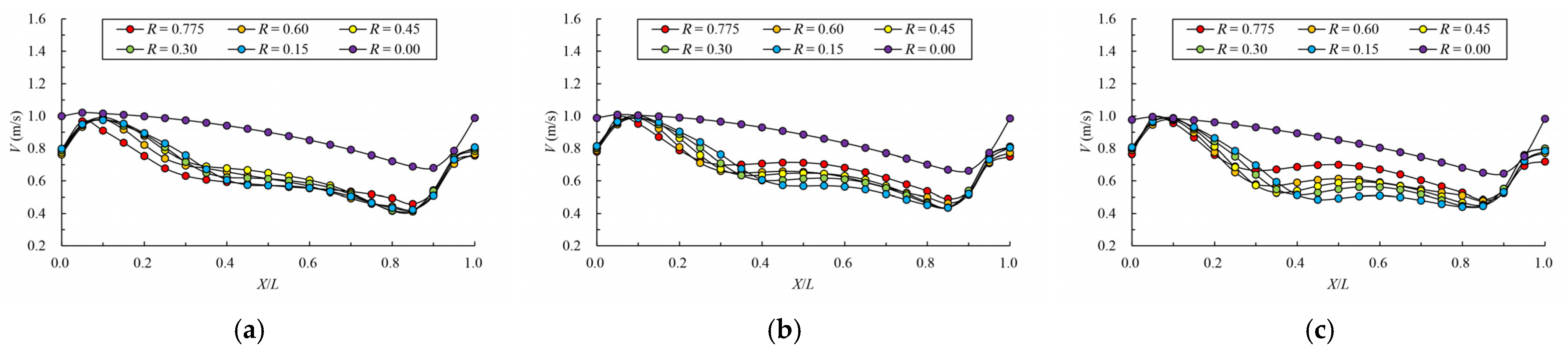
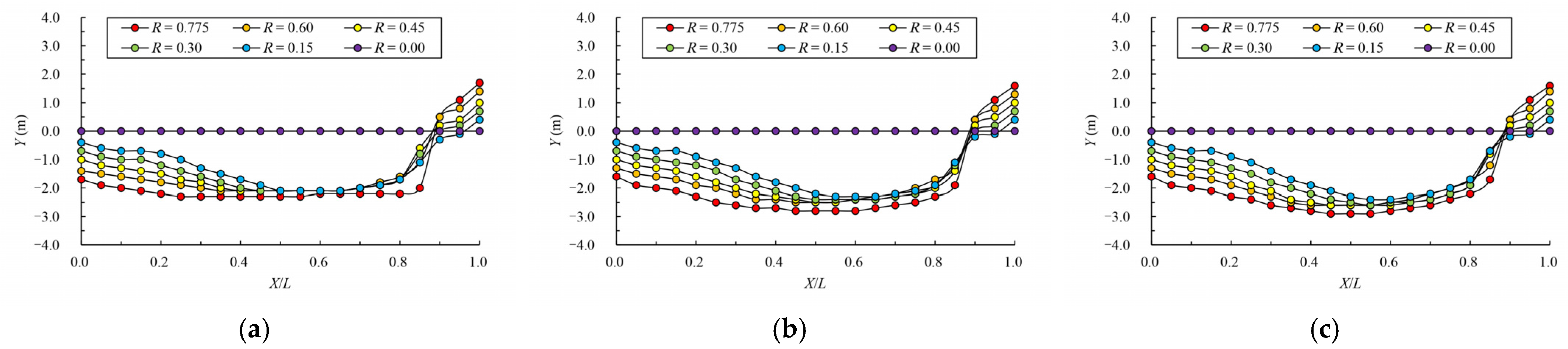
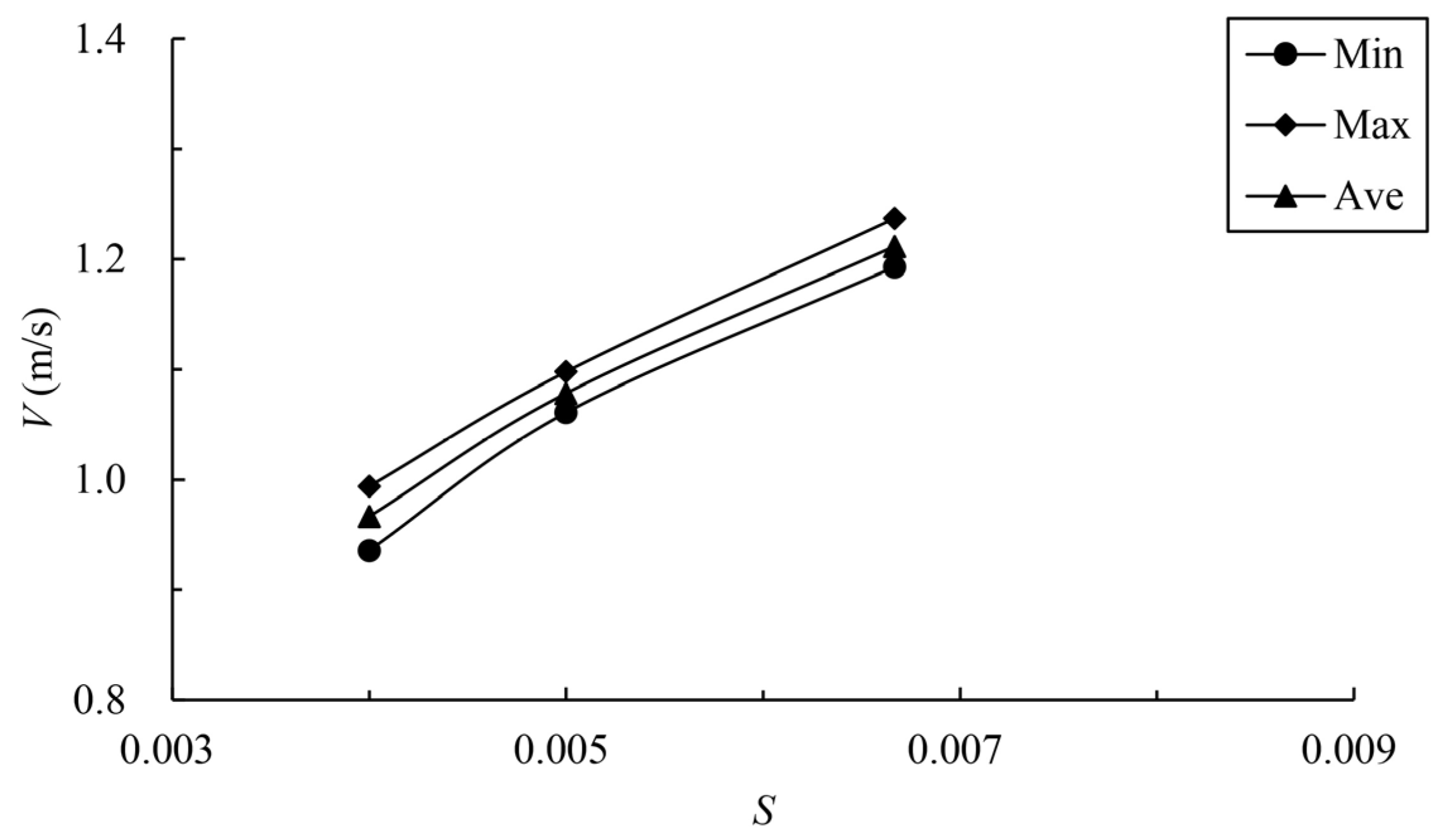
3.1. Influence of Permeability Ratio on Velocity
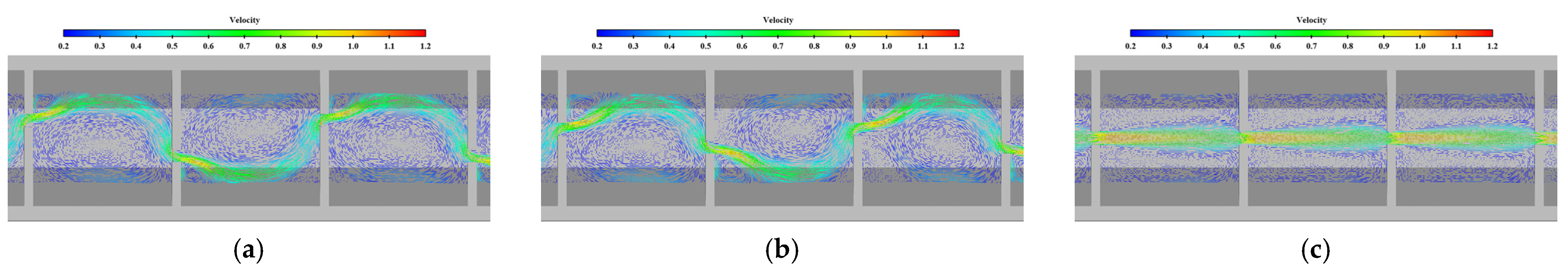
3.2. Influence of Offset Ratio on Velocity
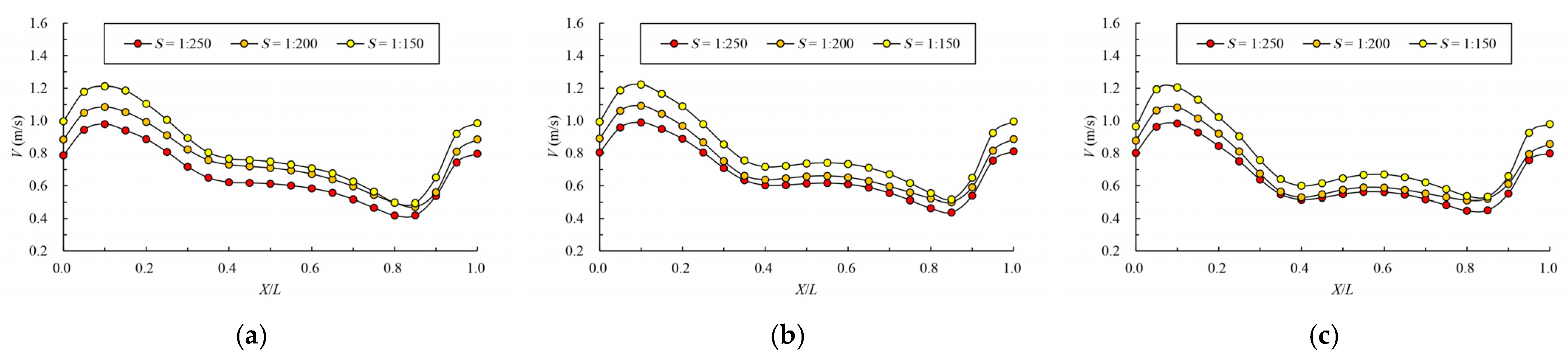
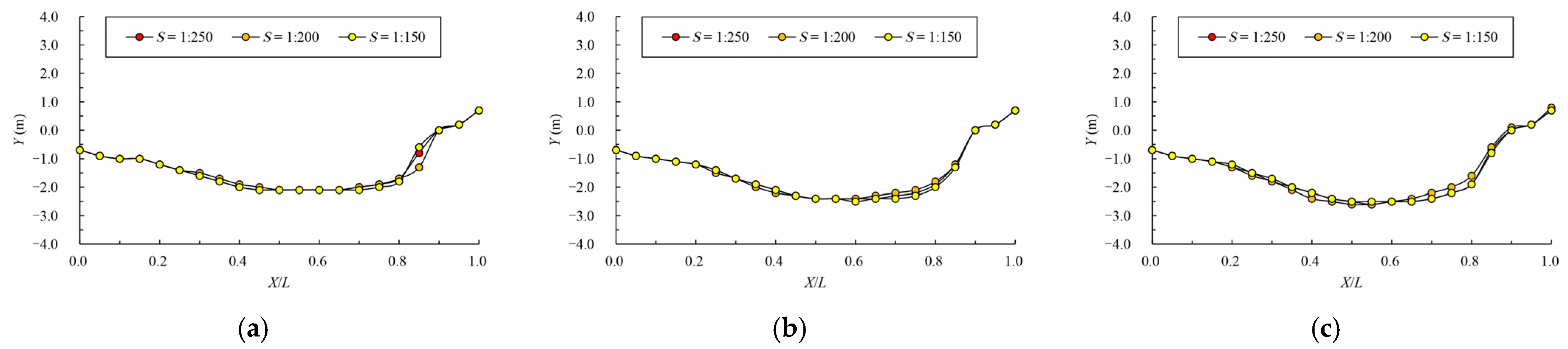
3.3. Influence of Bottom Slope on Velocity
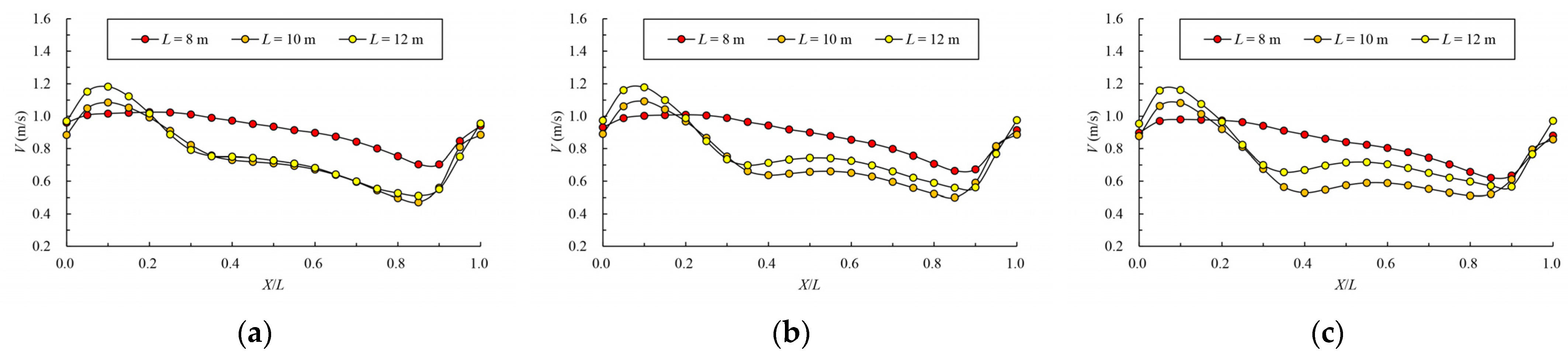
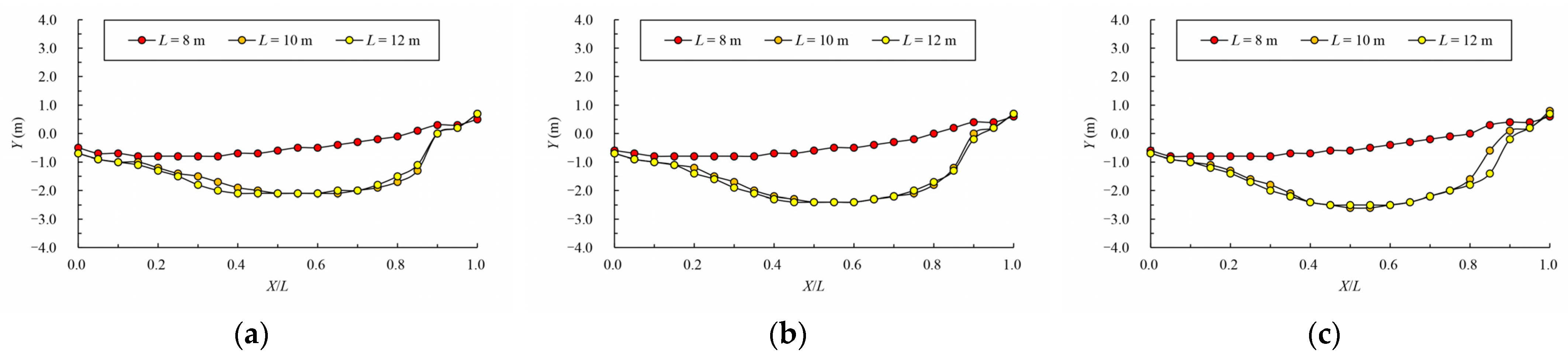
3.4. Influence of Pool Length on Velocity
3.5. The Field Applications
4. Conclusions
- (1)
- The study simplifies and generalizes the irregular nature-like fishway based on two important causes of flow energy consumption in a nature-like fishway: water blockage by the partition wall and mainstream bypass deflection of the pool. The water-permeable area of the partition wall is expressed by the permeability ratio of the control section, and the mainstream deflection length of the pool is expressed by the offset ratio of the control section of the adjacent pool. Combining the bottom slope and pool length, the effect of the above parameters on the flow structure is investigated through a 3D turbulent numerical simulation, and the design parameter thresholds satisfying the requirements are summarized to provide guidance for fishway design. The relevant design parameters and optimization strategies can provide a reference for similar projects.
- (2)
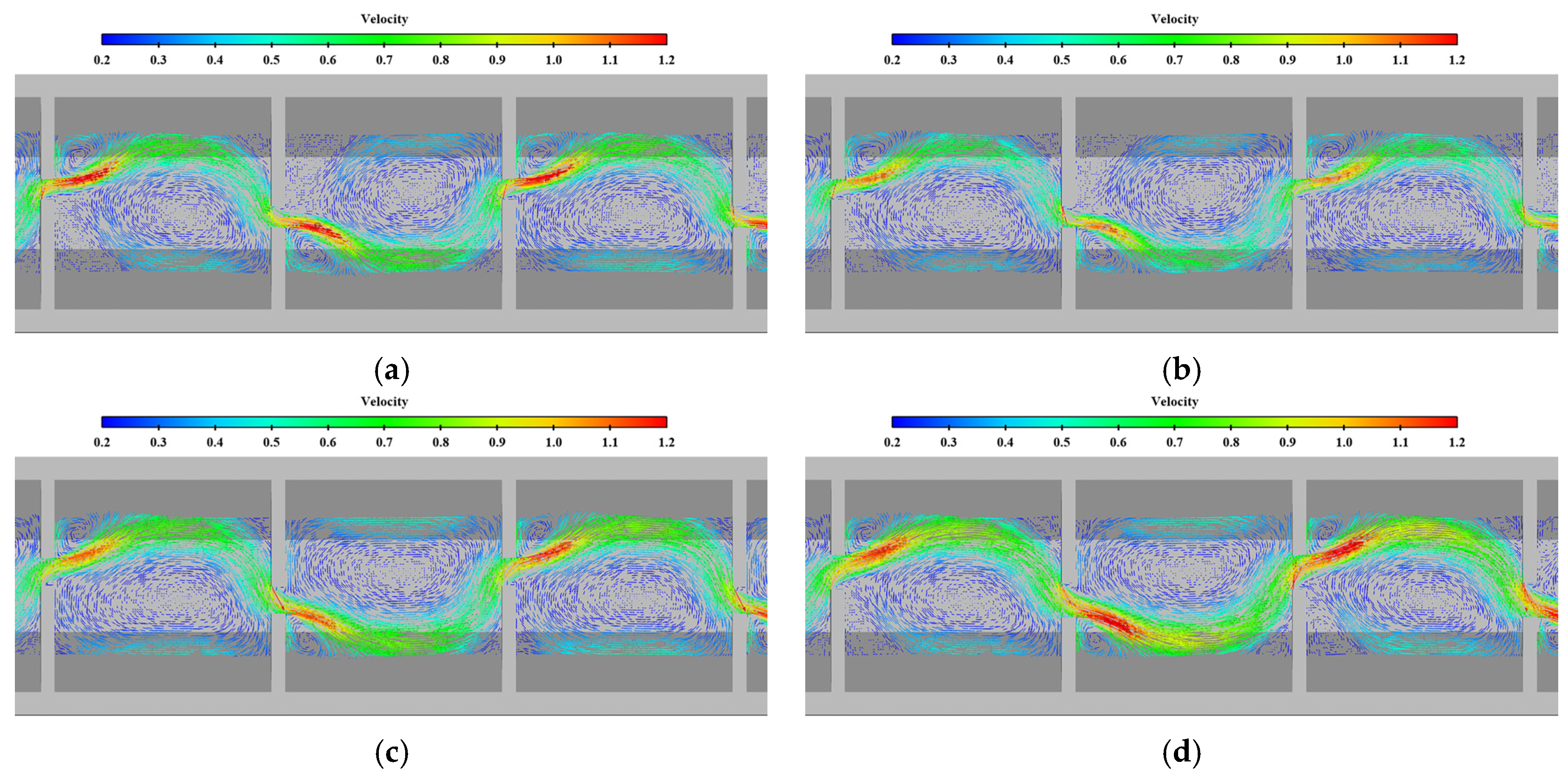
- The study showed that there are two basic flow patterns in the fishway pool: the mainstream is biased to one side and the mainstream tends towards the center. Under the same conditions, when the offset ratio is smaller and the pool length is shorter, the mainstream tends towards the center of the pool, and vice versa, the mainstream is biased to one side. Different flow patterns have a great influence on the velocity of the control section, the attenuation law of the main flow velocity and the main flow trajectory. The attenuation law of the main flow velocity and the main flow trajectory are basically the same under the same flow pattern.
- (3)
- Permeability ratio, bottom slope and pool length are the main factors affecting the maximum velocity of the control section. Under the same conditions, the maximum velocity of the control section increases with the increase in permeability ratio, bottom slope and pool length. The offset ratio has limited effect on the maximum velocity of the control section. Under the same conditions, the offset ratio decreases, the velocity of the control section only slightly increases, and the mainstream becomes smoother.
- (4)
- Within the recommended design parameter thresholds for the Mopiling nature-like fishway, the maximum velocity at the vertical slot can be basically controlled at about 1.0 m/s. The velocity distribution at the vertical slot is uneven, so that even if the maximum velocity exceeds 1.0 m/s, there is still a sufficient area available for upstream. The mainstream in the pool is clear and the flow pattern with the “high velocity zone” and “low velocity zone” is good, which can basically satisfy the requirements of fish passing.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Chen, K.; Chang, Z.; Cao, X.; Ge, H. Status and prospection of fish pass construction in China. J. Hydraul. Eng. 2012, 43, 182–188+197. (In Chinese) [Google Scholar]
- Wu, X.; Shi, J. Construction and management of fish passage on Shaliu River adjacent to Qinghai Lake based on ecological restoration. Trans. Chin. Soc. Agric. Eng. 2014, 30, 130–136. (In Chinese) [Google Scholar]
- Peng, W.; Liu, X.; Wang, Y.; Zou, X. Review and prospect of progress in water environment and water ecology research. J. Hydraul. Eng. 2018, 49, 1055–1067. (In Chinese) [Google Scholar]
- Zhang, Y.; He, Z.; He, Y.; Zhang, J.; Zhang, K. Analysis on the efficiency of fishway for the low-head gate dam. J. Hydraul. Eng. 2017, 48, 748–756. (In Chinese) [Google Scholar]
- Daneshvar, F.; Nejadhashemi, A.P.; Woznicki, S.A.; Herman, M.R. Applications of computational fluid dynamics in fish and habitat studies. Ecohydrol. Hydrobiol. 2017, 17, 53–62. [Google Scholar] [CrossRef] [Green Version]
- Mao, X. Review of fishway research in China. Ecol. Eng. 2018, 115, 91–95. [Google Scholar] [CrossRef]
- Marriner, B.A.; Baki, A.B.M.; Zhu, D.Z.; Cooke, S.J.; Katopodis, C. The hydraulics of a vertical slot fishway: A case study on the multi-species Vianney-Legendre fishway in Quebec, Canada. Ecol. Eng. 2016, 90, 190–202. [Google Scholar] [CrossRef]
- Marriner, B.A.; Baki, A.B.M.; Zhu, D.Z.; Thiem, J.D.; Cooke, S.J.; Katopodis, C. Field and numerical assessment of turning pool hydraulics in a vertical slot fishway. Ecol. Eng. 2014, 63, 88–101. [Google Scholar] [CrossRef]
- Córdoba, F.; Fuentes, J.F.; Valbuena-Castro, J.; Azagra, A.; Sanz-Ronda, F.J. Turning Pools in Stepped Fishways: Biological Assessment via Fish Response and CFD Models. Water 2021, 13, 1186. [Google Scholar] [CrossRef]
- Xu, T.; Sun, S. Numerical simulation of the flow structure in vertical slot fishway. J. Hydraul. Eng. 2009, 40, 1386–1391. (In Chinese) [Google Scholar]
- An, R.; Li, J.; Liang, R.; Tuo, Y. Three-dimensional simulation and experimental study for optimising a vertical slot fishway. J. Hydro-Environ. Res. 2016, 12, 119–129. [Google Scholar] [CrossRef]
- Ballu, A.; Pineau, G.; Calluaud, D.; David, L. Influence of Macro-Roughnesses on Vertical Slot Fishways. In Proceedings of the 7th International Symposium on Hydraulic Structures, Aachen, Germany, 15–18 May 2018. [Google Scholar]
- Bombač, M.; Četina, M.; Novak, G. Study on flow characteristics in vertical slot fishways regarding slot layout optimization. Ecol. Eng. 2017, 107, 126–136. [Google Scholar] [CrossRef]
- Li, Y.; Wang, X.; Xuan, G.; Liang, D. Effect of parameters of pool geometry on flow characteristics in low slope vertical slot fishways. J. Hydraul. Res. 2019, 58, 395–407. [Google Scholar] [CrossRef]
- Xia, W.; Gao, Z.; Shi, X.; Tan, J.; Luo, K.; Chen, X. Three-demensional numerical simulation of hydraulic characteristics of vertical slot fishway. Water Resour. Power 2017, 35, 86–89+65. (In Chinese) [Google Scholar]
- Fuentes-Perez, J.F.; Silva, A.T.; Tuhtan, J.A.; Garcia-Vega, A.; Carbonell-Baeza, R. 3D modelling of non-uniform and turbulent flow in vertical slot fishways. Environ. Model. Softw. 2018, 99, 156–169. [Google Scholar] [CrossRef]
- Wei, Y.; Luo, K.; Tan, J.; Tang, L.; Wang, J. Study on hydraulic characteristics of vertical slot fishway based on three turbulence models. Water Resour. Power 2020, 38, 71–74+78. (In Chinese) [Google Scholar]
- Roth, M.S.; Jhnel, C.; Stamm, J.; Schneider, L.K. Turbulent eddy identification of a meander and vertical-slot fishways in numerical models applying the IPOS-framework. J. Ecohydraulics 2021, 6, 1–20. [Google Scholar] [CrossRef]
- Kirk, M.; Caudill, C.; Syms, J.; Tonina, D. Context-dependent responses to turbulence for an anguilliform swimming fish, Pacific lamprey, during passage of an experimental vertical-slot weir. Ecol. Eng. 2017, 106, 296–307. [Google Scholar] [CrossRef]
- Tan, J.; Gao, Z.; Dai, H.; Shi, X. The correlation analysis between hydraulic characteristics of vertical slot fishway and fish movement characteristics. J. Hydraul. Eng. 2017, 48, 924–932+944. (In Chinese) [Google Scholar]
- Yang, P.; Tan, J.; Gao, Z.; Dai, H.; Shi, X.; Huang, T. The analysis of fish movement behavior in vertical slot fishway based on video tracking. Acta Hydrobiol. Sin. 2018, 42, 250–254. (In Chinese) [Google Scholar]
- Zhang, H.; Li, G.; Han, Y.; Sun, S.; Liu, H.; Zheng, T.; Zhao, H. Study on migration behaviors of juvenile Schizothorax prenanti in a vertical slot fish-way model. Water Resour. Hydropower Eng. 2020, 51, 180–187. (In Chinese) [Google Scholar]
- Syms, J.; Kirk, M.; Caudill, C.; Tonina, D. A biologically based measure of turbulence intensity for predicting fish passage behaviours. J. Ecohydraulics 2021, 1–13. [Google Scholar] [CrossRef]
- Gao, Z.; Andersson, H.I.; Dai, H.; Jiang, F.; Zhao, L. A new Eulerian–Lagrangian agent method to model fish paths in a vertical slot fishway. Ecol. Eng. 2016, 88, 217–225. [Google Scholar] [CrossRef] [Green Version]
- Jiang, Y.; Yang, Z.; Shi, X.; Wu, L.; Nie, L.; Wei, Y. The simulation of fish migratory trajectory in a vertical slot fishway based on multi-hydraulic indices. Chin. J. Ecol. 2018, 37, 1282–1290. (In Chinese) [Google Scholar]
- Jin, Z.; Ma, W.; Zhang, Y.; Chen, M.; Tan, J.; Shi, X. Assessing the swimming ability and performance of Schizothorax oconnori to cross velocity barriers in fishway. J. Hydraul. Eng. 2018, 49, 512–522. (In Chinese) [Google Scholar]
- Cai, L.; Hou, Y.; Jin, Y.; Yang, Z.; Hu, W.; Chen, X.; Chen, J.; Huang, Y.; Han, D. Response of fish swimming ability to body length and its application in fishway design. Trans. Chin. Soc. Agric. Eng. 2021, 37, 209–215. (In Chinese) [Google Scholar]
- Shi, X.; Chen, Q.; Huang, Y.; Liu, D.; Zhuang, P. Review on the methods to quantify fish’s ability to cross velocity barriers in fish passage. Acta Ecol. Sin. 2011, 31, 6967–6972. (In Chinese) [Google Scholar]
- Gustafsson, S.; Österling, M.; Skurdal, J.; Schneider, L.D.; Calles, O. Macroinvertebrate colonization of a nature-like fishway: The effects of adding habitat heterogeneity. Ecol. Eng. 2013, 61, 345–353. [Google Scholar] [CrossRef]
- Dodd, J.R.; Cowx, I.G.; Bolland, J.D. Efficiency of a nature-like bypass channel for restoring longitudinal connectivity for a river-resident population of brown trout. J. Environ. Manag. 2017, 204, 318–326. [Google Scholar] [CrossRef] [PubMed]
- Song, W.; Xu, Q.; Fu, X.; Wang, C.; Pang, Y.; Song, D. EFDC simulation of fishway in the Diversion Dahaerteng River to Danghe Reservoir, China. Ecol. Indic. 2019, 102, 704–715. [Google Scholar] [CrossRef]
- FAO; DVWK. Fish passes—Design, dimensions and monitoring; Food and Agriculture Organization of the United Nations: Rome, Italy, 2002. [Google Scholar]
- Li, S.; Ding, X.; Liu, D. Overview of nature-simulating fishway. Yangtze River 2014, 45, 70–73+96. (In Chinese) [Google Scholar]
- Acharya, M.; Kells, J.; Katopodis, C. Some Hydraulic Design Aspects of Nature-Like Fishways. In Proceedings of the Joint Conference on Water Resource Engineering and Water Resources Planning and Management, Minneapolis, MN, USA, 30 July 30–2 August 2000; American Society of Civil Engineers: Minneapolis, MN, USA, 2000. [Google Scholar]
- He, Y.; An, R.; Li, J.; Yi, W.; Zeng, Z. Hydraulic characteristics of nature-like fishways of rock-ramp type. J. Hydroelectr. Eng. 2016, 35, 40–47. (In Chinese) [Google Scholar]
- Baki, A.B.M. Numerical Modelling of Rock-Weir Type Nature-Like Fishpasses; In Proceedings of the Leadership in Sustainable Infrastructure, Vancouver, Canada, 31 May–3 June 2017.
- Zhang, Y.; Hu, X.; Yang, F.; Lv, W.; Zhang, J.; Mo, W. Experimental study on structure improvement and hydraulic characteristics of artificial natural-like fishway. Yangtze River 2021, 52, 225–229. (In Chinese) [Google Scholar]
- Hu, Q.; Zhu, L. Numerical research on the flows in nature-like fishway combined with slot and orifice. China Rural. Water Hydropower 2020, 3, 119–123+128. (In Chinese) [Google Scholar]
- Ma, W.; Liang, Y.; Wang, M.; Feng, M. Study on hydraulic characteristics of vertical slot-surface outlet combined nature-imitated fishway. Water Resour. Hydropower Eng. 2020, 51, 122–129. (In Chinese) [Google Scholar]
- Li, G.; Sun, S.; Liu, H.; Zhang, C.; Zhao, G.; Zheng, T. Improving effect of hydraulic characteristics of nature-like fishway with pools and cobblestone weirs. Trans. Chin. Soc. Agric. Eng. 2017, 33, 184–189. (In Chinese) [Google Scholar]
- Xu, J.; Wang, X.; Xuan, G.; Zheng, F.; Huang, Y. Physical model test study on nature-like fishways. Adv. Water Sci. 2017, 28, 879–887. (In Chinese) [Google Scholar]
- Zhu, L.; Hu, Q.; Wang, C.; Wang, X. Numerical Simulation and Optimization of Flow Conditions in Zongyang Nature-like Fishway. China Rural. Water Hydropower 2022, 6, 1–7+15. (In Chinese) [Google Scholar]
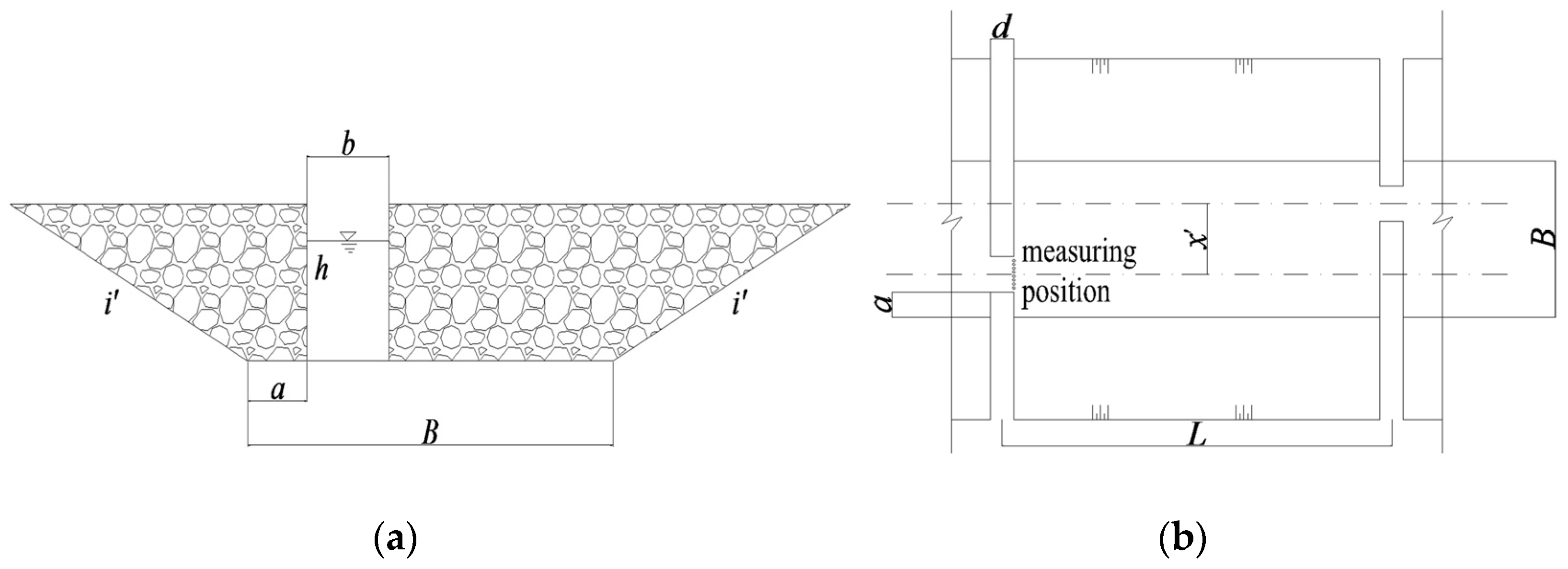

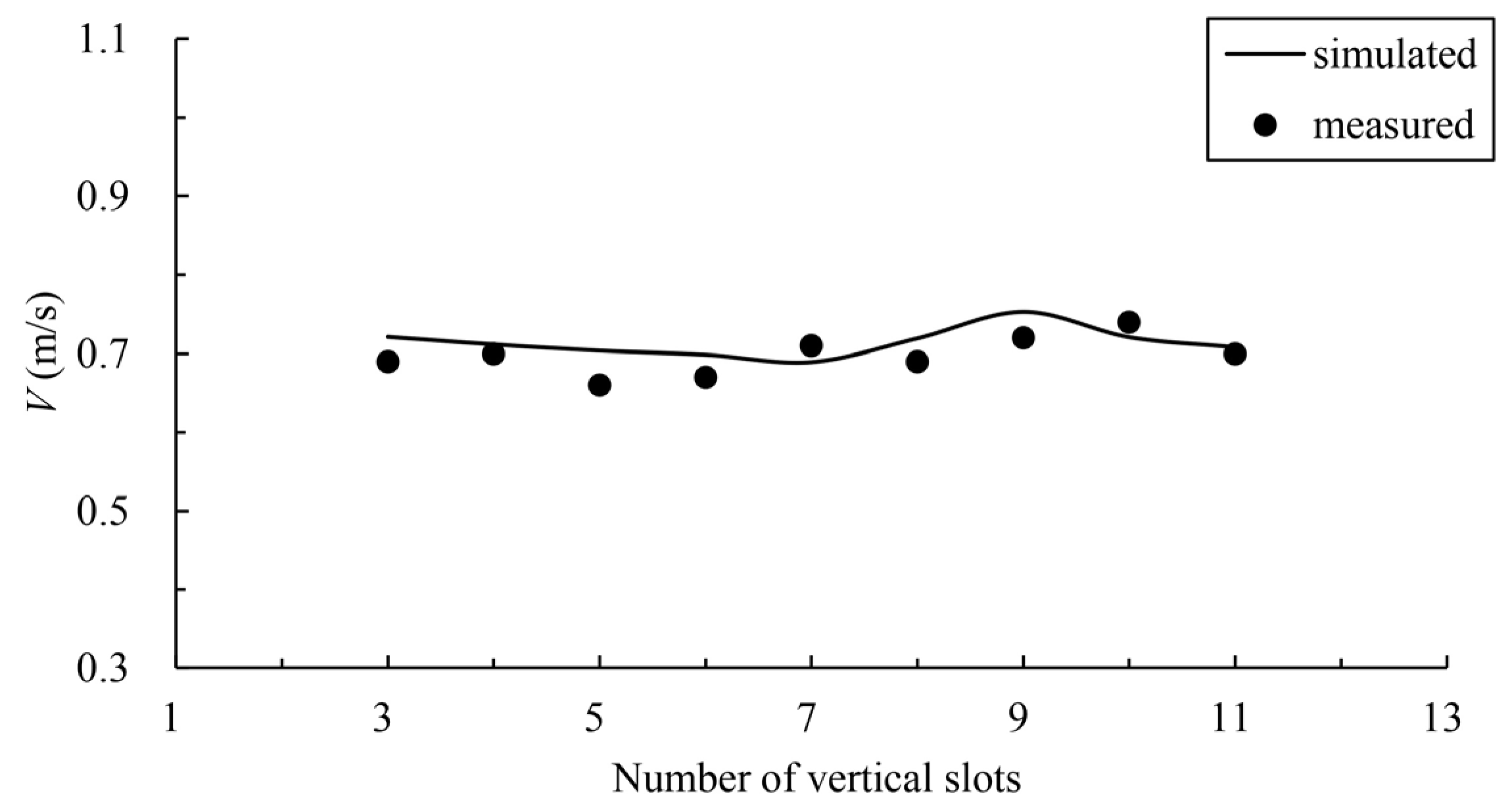
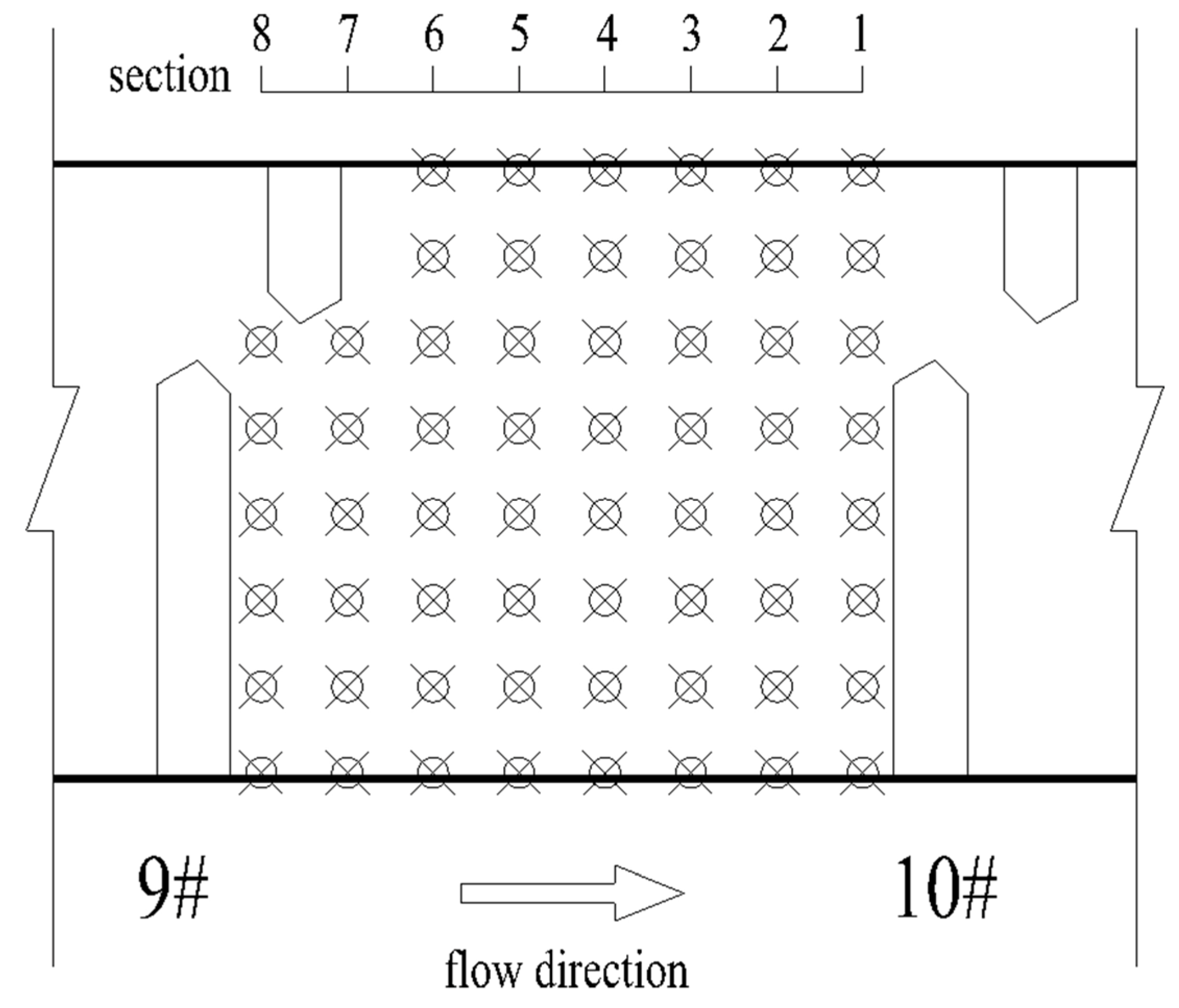

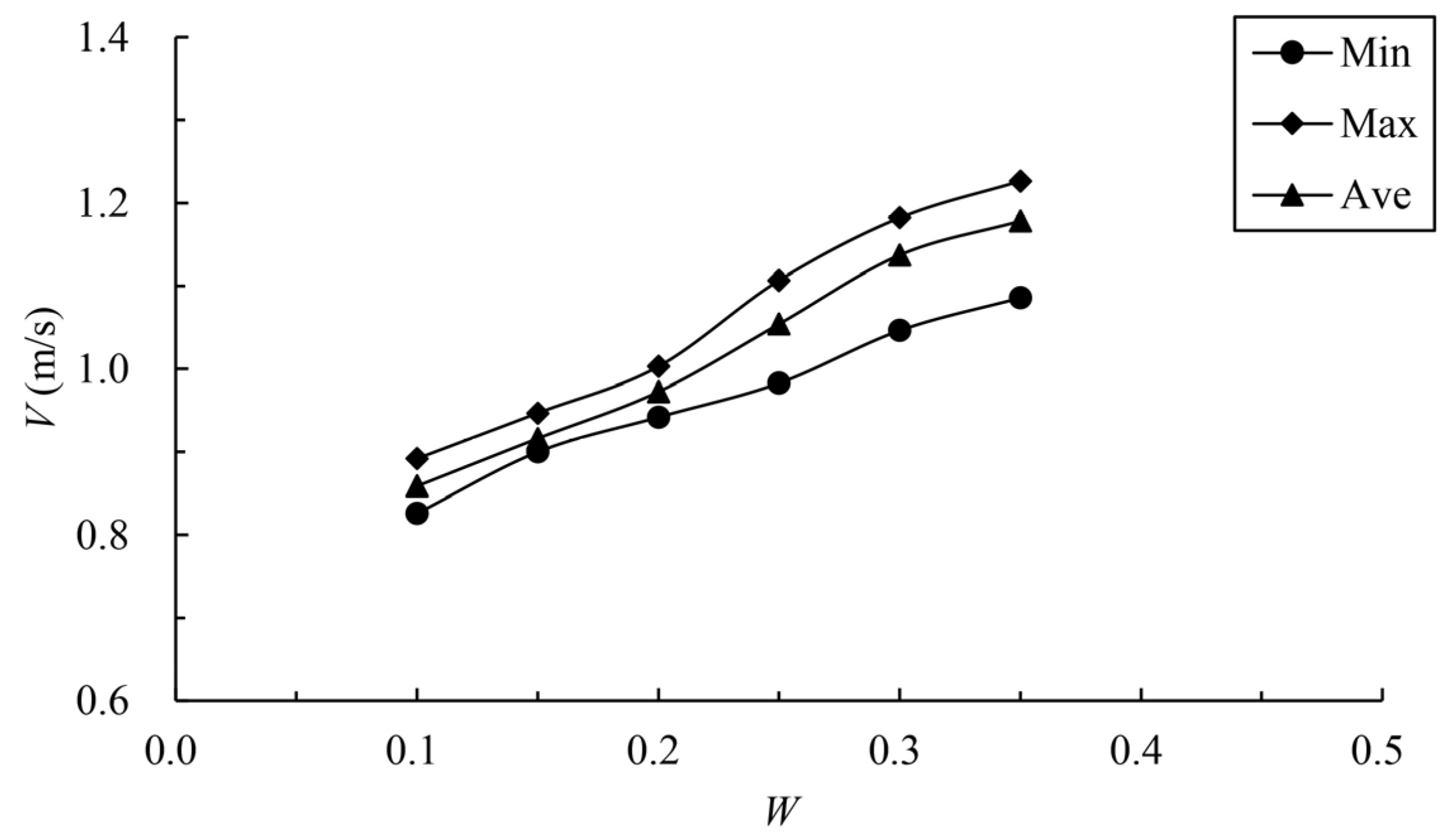



| Case | b (m) | W | a (m) | R | S | L (m) |
|---|---|---|---|---|---|---|
| 1 | 0.6 | 0.1 | 0 | 0.85 | 1/250 | 10 |
| 2 | 0.9 | 0.15 | 0 | 0.775 | 1/250 | 10 |
| 3 | 1.2 | 0.2 | 0 | 0.7 | 1/250 | 10 |
| 4 | 1.5 | 0.25 | 0 | 0.625 | 1/250 | 10 |
| 5 | 1.8 | 0.3 | 0 | 0.55 | 1/250 | 10 |
| 6 | 2.1 | 0.35 | 0 | 0.475 | 1/250 | 10 |
| 7 | 0.9 | 0.15 | 0.35 | 0.6 | 1/250 | 10 |
| 8 | 0.9 | 0.15 | 0.65 | 0.45 | 1/250 | 10 |
| 9 | 0.9 | 0.15 | 0.95 | 0.3 | 1/250 | 10 |
| 10 | 0.9 | 0.15 | 1.25 | 0.15 | 1/250 | 10 |
| 11 | 0.9 | 0.15 | 1.55 | 0 | 1/250 | 10 |
| 12 | 0.9 | 0.15 | 0.95 | 0.3 | 1/200 | 10 |
| 13 | 0.9 | 0.15 | 0.95 | 0.3 | 1/150 | 10 |
| 14 | 1.2 | 0.2 | 0.8 | 0.3 | 1/200 | 10 |
| 15 | 1.5 | 0.25 | 0.65 | 0.3 | 1/200 | 10 |
| 16 | 0.9 | 0.15 | 0.95 | 0.3 | 1/200 | 8 |
| 17 | 0.9 | 0.15 | 0.95 | 0.3 | 1/200 | 12 |
| 18 | 1.2 | 0.2 | 0.8 | 0.3 | 1/200 | 8 |
| W | b (m) | a (m) | Maximum Velocity of Vertical Slots V (m/s) | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| 1# | 2# | 3# | 4# | 5# | 6# | Min | Max | Ave | |||
| 0.10 | 0.60 | 0 | 0.868 | 0.858 | 0.892 | 0.842 | 0.867 | 0.826 | 0.826 | 0.892 | 0.859 |
| 0.15 | 0.90 | 0 | 0.901 | 0.947 | 0.909 | 0.937 | 0.904 | 0.900 | 0.900 | 0.947 | 0.916 |
| 0.20 | 1.20 | 0 | 0.942 | 0.986 | 0.959 | 1.003 | 0.984 | 0.962 | 0.942 | 1.003 | 0.973 |
| 0.25 | 1.50 | 0 | 0.983 | 1.022 | 1.036 | 1.081 | 1.107 | 1.097 | 0.983 | 1.107 | 1.054 |
| 0.30 | 1.80 | 0 | 1.046 | 1.125 | 1.182 | 1.145 | 1.158 | 1.168 | 1.046 | 1.182 | 1.137 |
| 0.35 | 2.10 | 0 | 1.086 | 1.170 | 1.185 | 1.215 | 1.188 | 1.227 | 1.086 | 1.227 | 1.179 |
| W | a (m) | R | Maximum Velocity of Vertical Slots V (m/s) | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| 1# | 2# | 3# | 4# | 5# | 6# | Min | Max | Ave | |||
| 0.15 | 0.00 | 0.775 | 0.901 | 0.947 | 0.909 | 0.937 | 0.904 | 0.900 | 0.900 | 0.947 | 0.916 |
| 0.15 | 0.35 | 0.600 | 0.954 | 0.977 | 0.958 | 0.957 | 0.921 | 0.942 | 0.921 | 0.977 | 0.952 |
| 0.15 | 0.65 | 0.450 | 0.934 | 0.927 | 0.983 | 0.986 | 0.959 | 0.981 | 0.927 | 0.986 | 0.962 |
| 0.15 | 0.95 | 0.300 | 0.936 | 0.967 | 0.968 | 0.994 | 0.966 | 0.969 | 0.936 | 0.994 | 0.967 |
| 0.15 | 1.25 | 0.150 | 0.952 | 1.002 | 0.983 | 0.994 | 1.009 | 0.963 | 0.952 | 1.009 | 0.984 |
| 0.15 | 1.55 | 0.000 | 1.056 | 1.053 | 1.043 | 1.030 | 1.036 | 1.038 | 1.030 | 1.056 | 1.043 |
| W | R | S | Maximum Velocity of Vertical Slots V (m/s) | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| 1# | 2# | 3# | 4# | 5# | 6# | Min | Max | Ave | |||
| 0.15 | 0.30 | 1:250 | 0.936 | 0.967 | 0.968 | 0.994 | 0.966 | 0.969 | 0.936 | 0.994 | 0.967 |
| 0.15 | 0.30 | 1:200 | 1.061 | 1.072 | 1.079 | 1.073 | 1.098 | 1.088 | 1.061 | 1.098 | 1.079 |
| 0.15 | 0.30 | 1:150 | 1.227 | 1.218 | 1.197 | 1.237 | 1.193 | 1.198 | 1.193 | 1.237 | 1.212 |
| 0.20 | 0.30 | 1:200 | 1.074 | 1.102 | 1.141 | 1.131 | 1.160 | 1.132 | 1.074 | 1.160 | 1.123 |
| 0.25 | 0.30 | 1:200 | 1.128 | 1.203 | 1.249 | 1.238 | 1.274 | 1.257 | 1.128 | 1.274 | 1.225 |
| W | R | S | L (m) | Maximum Velocity of Vertical Slots V (m/s) | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1# | 2# | 3# | 4# | 5# | 6# | Min | Max | Ave | ||||
| 0.15 | 0.30 | 1:200 | 8.0 | 0.986 | 1.012 | 1.037 | 0.987 | 1.002 | 0.990 | 0.986 | 1.037 | 1.002 |
| 0.15 | 0.30 | 1:200 | 10.0 | 1.061 | 1.072 | 1.079 | 1.073 | 1.098 | 1.088 | 1.061 | 1.098 | 1.079 |
| 0.15 | 0.30 | 1:200 | 12.0 | 1.139 | 1.152 | 1.126 | 1.137 | 1.115 | 1.111 | 1.111 | 1.152 | 1.130 |
| 0.20 | 0.30 | 1:200 | 8.0 | 1.106 | 1.140 | 1.071 | 1.077 | 1.049 | 1.086 | 1.049 | 1.140 | 1.088 |
| 0.20 | 0.30 | 1:200 | 10.0 | 1.074 | 1.102 | 1.141 | 1.131 | 1.160 | 1.132 | 1.074 | 1.160 | 1.123 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hu, Q.; Wang, X.; Zhu, L.; Du, S.; He, F. Effect of Parameters of Pool Geometry on Flow Structure in Nature-like Fishway. Int. J. Environ. Res. Public Health 2022, 19, 9389. https://doi.org/10.3390/ijerph19159389
Hu Q, Wang X, Zhu L, Du S, He F. Effect of Parameters of Pool Geometry on Flow Structure in Nature-like Fishway. International Journal of Environmental Research and Public Health. 2022; 19(15):9389. https://doi.org/10.3390/ijerph19159389
Chicago/Turabian StyleHu, Qiaoyi, Xiaogang Wang, Long Zhu, Shuai Du, and Feifei He. 2022. "Effect of Parameters of Pool Geometry on Flow Structure in Nature-like Fishway" International Journal of Environmental Research and Public Health 19, no. 15: 9389. https://doi.org/10.3390/ijerph19159389
APA StyleHu, Q., Wang, X., Zhu, L., Du, S., & He, F. (2022). Effect of Parameters of Pool Geometry on Flow Structure in Nature-like Fishway. International Journal of Environmental Research and Public Health, 19(15), 9389. https://doi.org/10.3390/ijerph19159389






