A Simulation Analysis of Maternal Pelvic Floor Muscle
Abstract
:1. Introduction
2. Methods
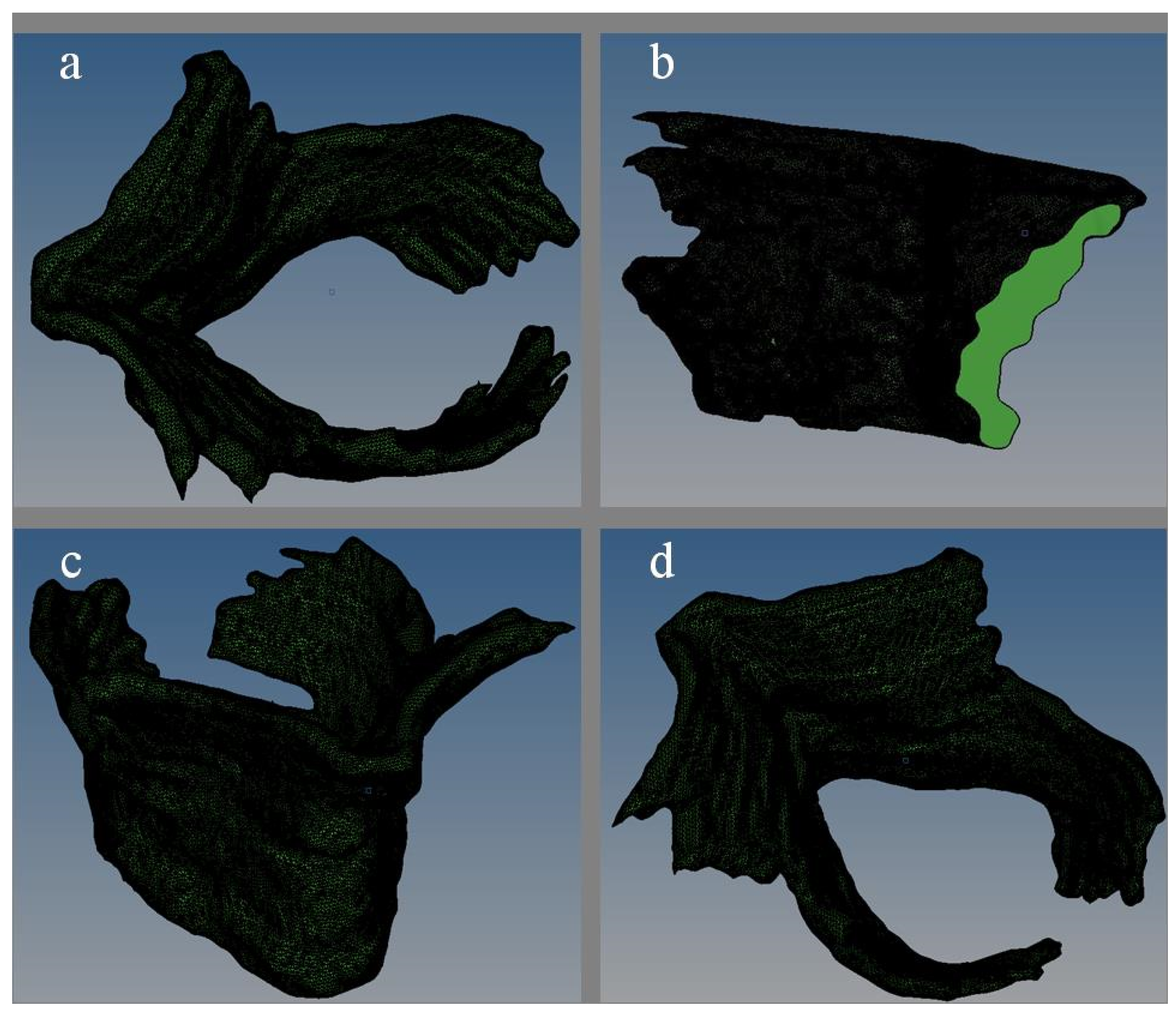
2.1. Model Construction
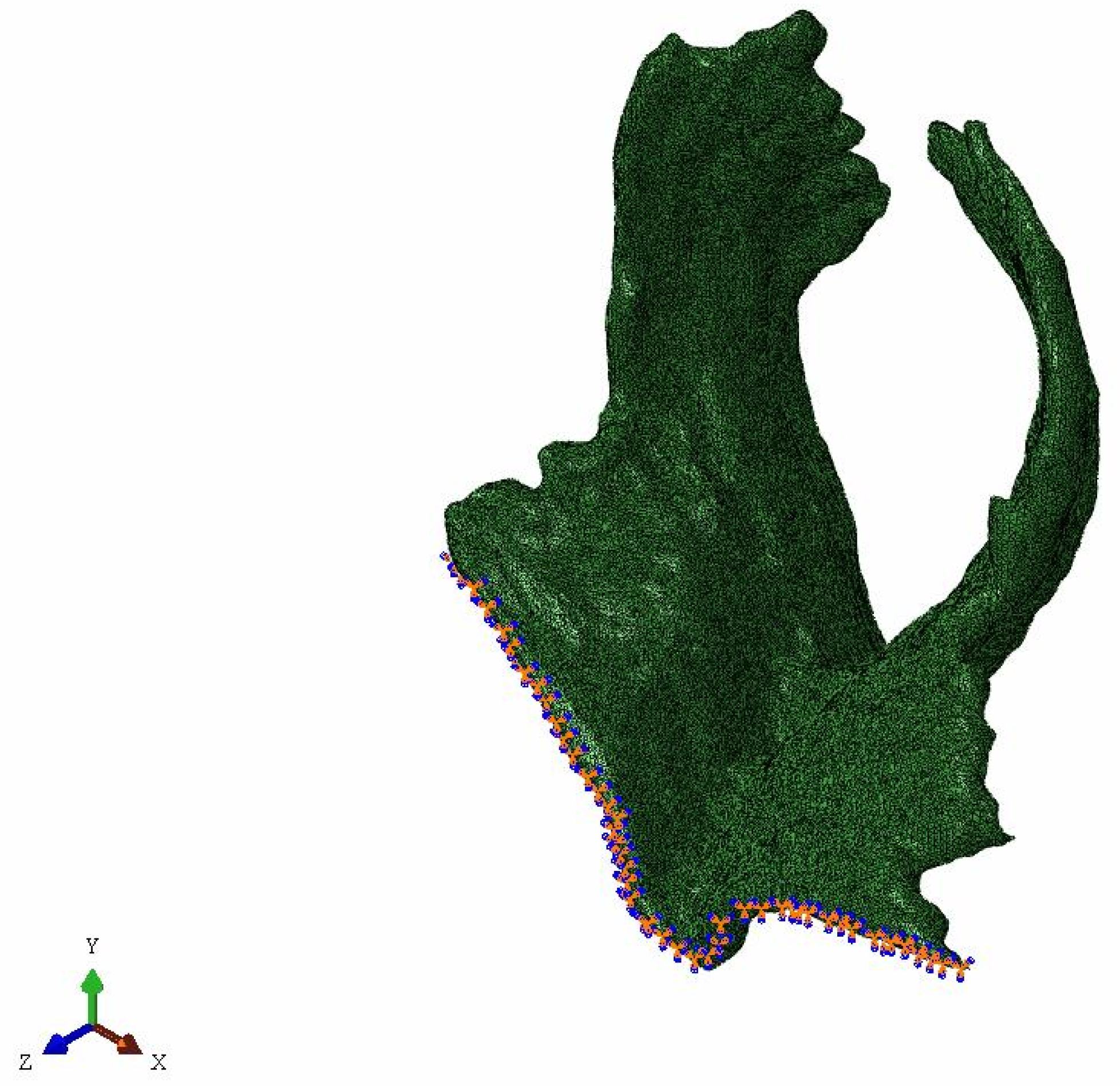
2.2. Load and Boundary Conditions
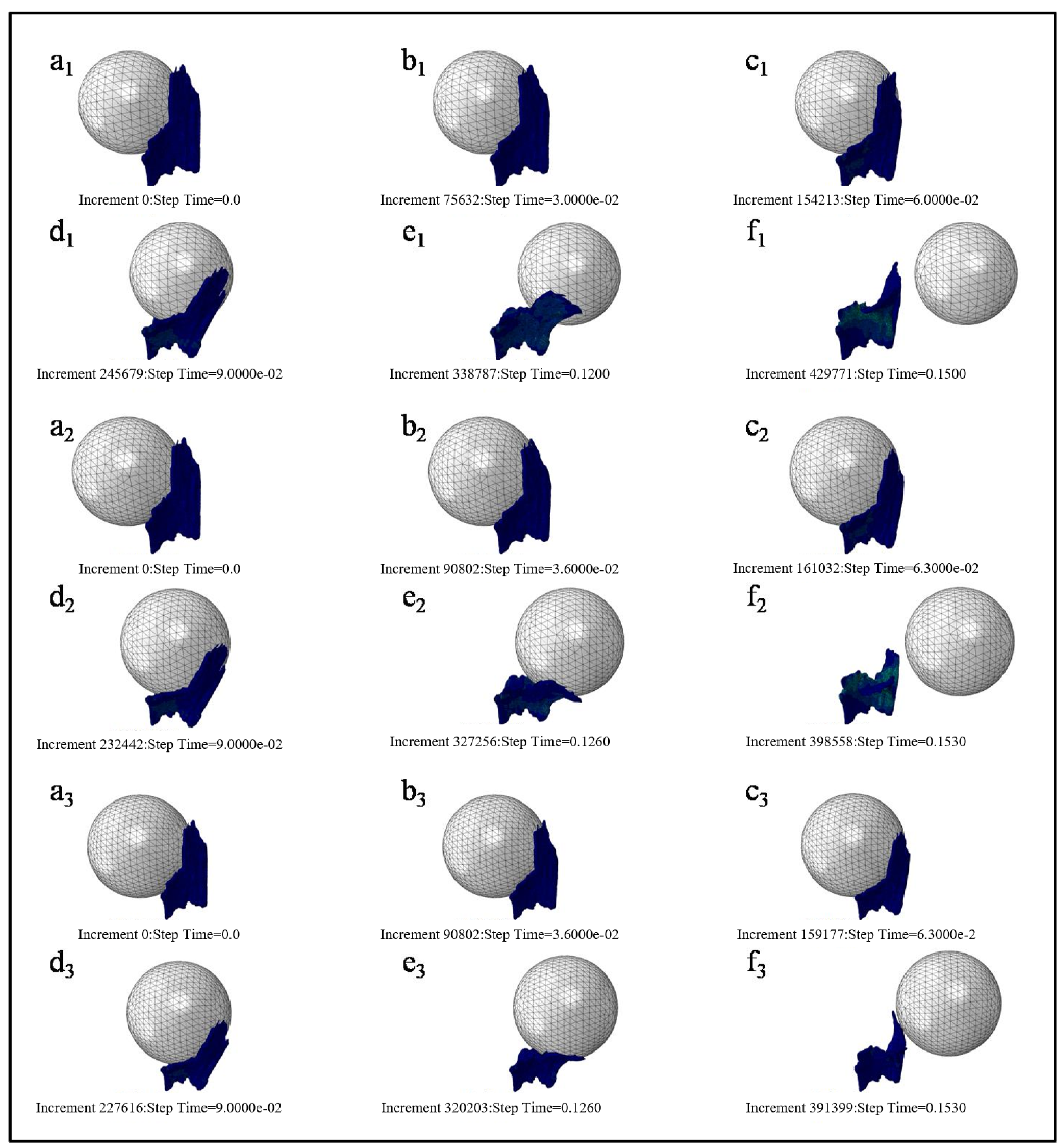
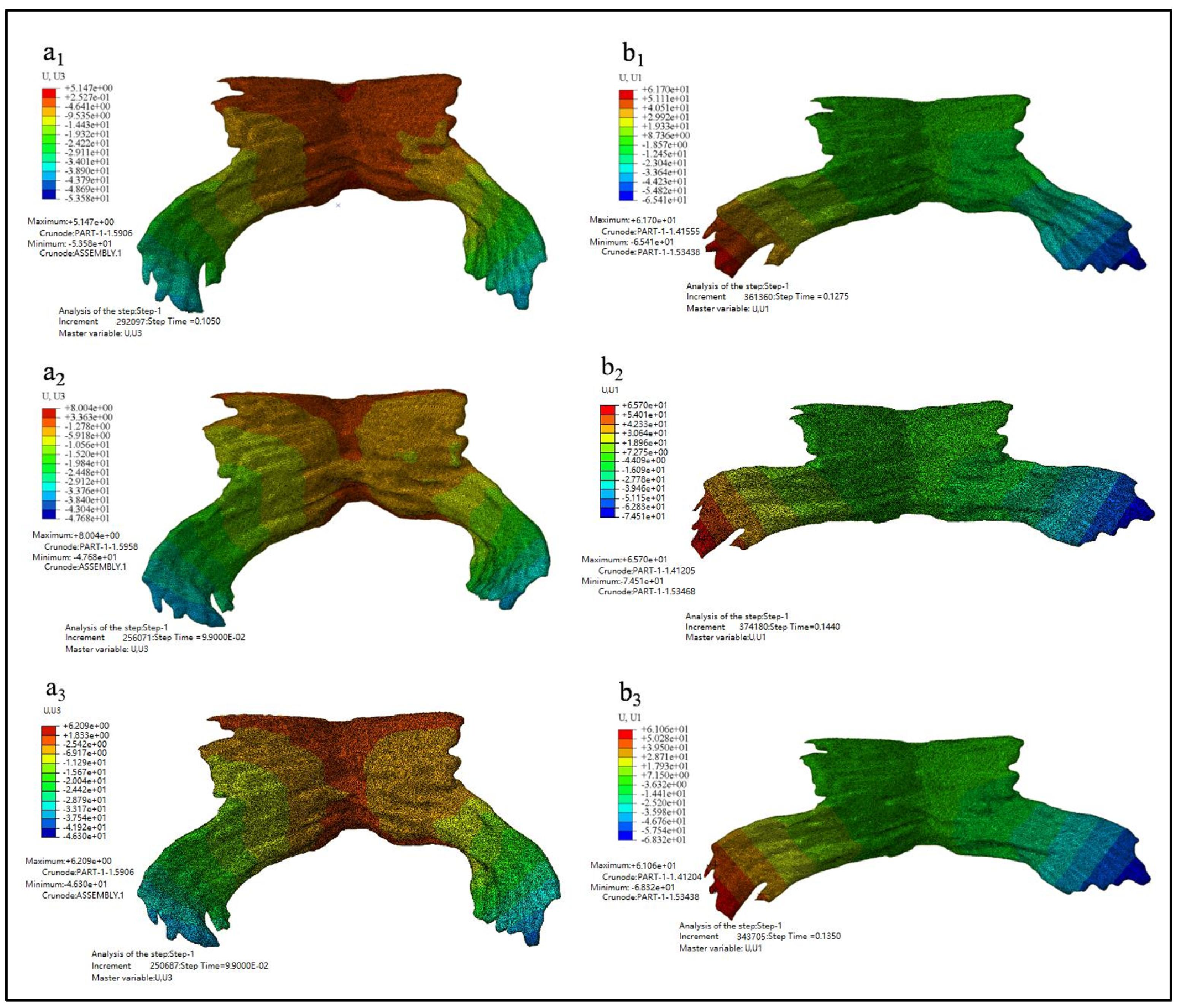
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- DeLancey, J.O.L.; Kearney, R.; Chou, Q.; Speights, S.; Binno, S. The appearance of levator ani muscle abnormalities in magnetic resonance images after vaginal delivery. Obstet. Gynecol. 2003, 101, 46–53. [Google Scholar] [CrossRef] [PubMed]
- Patel, P.D.; Amrute, K.V.; Badlani, G.H. Pelvic organ prolapse and stress urinary incontinence: A review of etiological factors. Indian J. Urol. 2007, 23, 135–141. [Google Scholar] [CrossRef] [PubMed]
- Chen, Y.; DeSautel, M.; Anderson, A.; Badlani, G.; Kushner, L. Collagen synthesis is not altered in women with stress urinary incontinence. Neurourol. Urodyn. 2004, 23, 367–373. [Google Scholar] [CrossRef]
- Jack, G.S.; Nikolova, G.; Vilain, E.; Raz, S.; Rodríguez, L.V. Familial tranmission of genitovaginal prolapse. Int. Urogynecol. J. 2006, 17, 498–501. [Google Scholar] [CrossRef]
- Graham, C.A.; Mallett, V.T. Race as a predictor of urinary incontinence and pelvic organ prolapse. Am. J. Obstet. Gynecol. 2001, 185, 116–120. [Google Scholar] [CrossRef]
- Sze, E.H.M.; Sherard, G.B.; Dolezal, J.M. Pregnancy, labor, delivery, and pelvic organ prolapse. Obstet. Gynecol. 2002, 100, 981–986. [Google Scholar] [CrossRef] [PubMed]
- Woodman, P.J.; Swift, S.E.; O’Boyle, A.L.; Valley, M.T.; Bland, D.R.; Kahn, M.A.; Schaffer, J.I. Prevalence of severe pelvic organ prolapse in relation to job description and socioeconomic status: A multicenter cross-sectional study. Int. Urogynecol. J. 2006, 17, 340–345. [Google Scholar] [CrossRef]
- Beckmann, M.M.; Stock, O.M. Antenatal perineal massage for reducing perineal trauma. Cochrane Database Syst. Rev. 2013, 2013. [Google Scholar] [CrossRef] [Green Version]
- Kwon, H.; Park, H.S.; Shim, J.Y.; Lee, K.W.; Choi, S.J.; Choi, G.Y. Randomized, double-blind, placebo-controlled trial on the efficacy of hyaluronidase in preventing perineal trauma in nulliparous women. Yonsei Med. J. 2020, 61, 79–84. [Google Scholar] [CrossRef]
- Janda, Š.; Van Der Helm, F.C.T.; De Blok, S.B. Measuring morphological parameters of the pelvic floor for finite element modelling purposes. J. Biomech. 2003, 36, 749–757. [Google Scholar] [CrossRef]
- Dobben, A.C.; Terra, M.P.; Slors, J.F.M.; Deutekom, M.; Gerhards, M.F.; Beets-Tan, R.G.H.; Bossuyt, P.M.M.; Stoker, J. External anal sphincter defects in patients with fecal incontinence: Comparison of endoanal MR imaging and endoanal US. Radiology 2007, 242, 463–471. [Google Scholar] [CrossRef]
- Budzik, J.F.; Le Thuc, V.; Demondion, X.; Morel, M.; Chechin, D.; Cotten, A. In vivo MR tractography of thigh muscles using diffusion imaging: Initial results. Eur. Radiol. 2007, 17, 3079–3085. [Google Scholar] [CrossRef] [PubMed]
- Froeling, M.; Oudeman, J.; Van Den Berg, S.; Nicolay, K.; Maas, M.; Strijkers, G.J.; Drost, M.R.; Nederveen, A.J. Reproducibility of diffusion tensor imaging in human forearm muscles at 3.0 T in a clinical setting. Magn. Reson. Med. 2010, 64, 1182–1190. [Google Scholar] [CrossRef] [PubMed]
- Zijta, F.M.; Froeling, M.; Van Der Paardt, M.P.; Lakeman, M.M.E.; Bipat, S.; Montauban Van Swijndregt, A.D.; Strijkers, G.J.; Nederveen, A.J.; Stoker, J. Feasibility of diffusion tensor imaging (DTI) with fibre tractography of the normal female pelvic floor. Eur. Radiol. 2011, 21, 1243–1249. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Brandão, S.; Parente, M.; Mascarenhas, T.; da Silva, A.R.G.; Ramos, I.; Jorge, R.N. Biomechanical study on the bladder neck and urethral positions: Simulation of impairment of the pelvic ligaments. J. Biomech. 2015, 48, 217–223. [Google Scholar] [CrossRef] [PubMed]
- Berardi, M.; Martinez-Romero, O.; Elías-Zúñiga, A.; Rodríguez, M.; Ceretti, E.; Fiorentino, A.; Donzella, G.; Avanzini, A. Levator ani deformation during the second stage of labour. Proc. Inst. Mech. Eng. Part H J. Eng. Med. 2014, 228, 501–508. [Google Scholar] [CrossRef]
- Jing, D.; Ashton-Miller, J.A.; DeLancey, J.O.L. A subject-specific anisotropic visco-hyperelastic finite element model of female pelvic floor stress and strain during the second stage of labor. J. Biomech. 2012, 45, 455–460. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Martins, J.A.C.; Pato, M.P.M.; Pires, E.B.; Natal Jorge, R.M.; Parente, M.; Mascarenhas, T. Finite element studies of the deformation of the pelvic floor. Ann. N. Y. Acad. Sci. 2007, 1101, 316–334. [Google Scholar] [CrossRef] [PubMed]
- Ashton-Miller, J.A.; DeLancey, J.O.L. Mechanisms of pelvic floor trauma during vaginal delivery. In Pelvic Floor Disorders: A Multidisciplinary Textbook; Santoro, G.A., Wieczorek, A.P., Sultan, A.H., Eds.; Springer: Cham, Switzerland, 2021; pp. 189–209. [Google Scholar]
- Kruger, J.A.; Yan, X.; Li, X.; Nielsen, P.M.F.; Nash, M.P. Applications of pelvic floor modeling and simulation. In Biomechanics of the Female Pelvic Floor; Hoyte, L., Damaser, M., Eds.; Academic Press: Cambridge, MA, USA, 2016; pp. 367–382. [Google Scholar]
- Hoyte, L.; Schierlitz, L.; Zou, K.; Flesh, G.; Fielding, J.R. Two- and 3-dimensional MRI comparison of levator ani structure, volume, and integrity in women with stress incontinence and prolapse. Am. J. Obstet. Gynecol. 2001, 185, 11–19. [Google Scholar] [CrossRef] [Green Version]
- Lien, K.-C.; Mooney, B.; DeLancey, J.O.L.; Ashton-Miller, J.A. Levator ani muscle stretch induced by simulated vaginal birth. Obstet. Gynecol. 2004, 103, 31–40. [Google Scholar] [CrossRef]
- Oliveira, D.A.; Parente, M.P.L.; Calvo, B.; Mascarenhas, T.; Natal Jorge, R.M. Numerical simulation of the damage evolution in the pelvic floor muscles during childbirth. J. Biomech. 2016, 49, 594–601. [Google Scholar] [CrossRef]
- Hoyte, L.; Damaser, M.S.; Warfield, S.K.; Chukkapalli, G.; Majumdar, A.; Choi, D.J.; Trivedi, A.; Krysl, P. Quantity and distribution of levator ani stretch during simulated vaginal childbirth. Am. J. Obstet. Gynecol. 2008, 199, 198.e1–198.e5. [Google Scholar] [CrossRef] [PubMed]
- Fung, Y. Biomechanics: Mechanical Properties of Living Tissues; Springer: Cham, Switzerland, 2013; pp. 1–559. [Google Scholar]
- D’Aulignac, D.; Martins, J.A.C.; Pires, E.B.; Mascarenhas, T.; Jorge, R.M.N. A shell finite element model of the pelvic floor muscles. Comput. Methods Biomech. Biomed. Eng. 2005, 8, 339–347. [Google Scholar] [CrossRef] [Green Version]
- Parente, M.P.L.; Jorge, R.M.N.; Mascarenhas, T.; Fernandes, A.A.; Martins, J.A.C. Deformation of the pelvic floor muscles during a vaginal delivery. Int. Urogynecol. J. 2008, 19, 65–71. [Google Scholar] [CrossRef] [PubMed]
- Gao, Y.; Ren, S.; Zhou, H.; Xuan, R. Impact of physical activity during pregnancy on gestational hypertension. Phys. Act. Health 2020, 4, 32–39. [Google Scholar] [CrossRef] [Green Version]
- Ren, S.; Gao, Y.; Yang, Z.; Li, J.; Xuan, R.; Liu, J.; Chen, X.; Thirupathi, A. The effect of pelvic floor muscle training on pelvic floor dysfunction in pregnant and postpartum women. Phys. Act. Health 2020, 4, 130–141. [Google Scholar] [CrossRef]
- Zhang, Q.; Chon, T.; Zhang, Y.; Baker, J.S.; Gu, Y. Finite element analysis of the lumbar spine in adolescent idiopathic scoliosis subjected to different loads. Comput. Biol. Med. 2021, 136, 104745. [Google Scholar] [CrossRef]
- Yu, L.; Mei, Q.; Mohamad, N.I.; Gu, Y.; Fernandez, J. An exploratory investigation of patellofemoral joint loadings during directional lunges in badminton. Comput. Biol. Med. 2021, 132, 104302. [Google Scholar] [CrossRef]





| Pelvic Floor Muscle | Fetal Biparietal Diameter | ||||
|---|---|---|---|---|---|
| Length (mm) | Width (mm) | Height (mm) | Diameter D1 (mm) | Diameter D2 (mm) | Diameter D3 (mm) |
| 70 | 60 | 40 | 80 | 90 | 100 |
| Part Name | Young Modulus (MPa) | Poisson Ratio | Density (ton/mm3) |
|---|---|---|---|
| Pelvic floor muscle | 0.2 | 0.4 | 1.12 × 10−9 |
| Fetal head | 140,000 | 0.3 | 7.86 × 10−9 |
| Fetal Biparietal Diameter D (mm) | Maximum Equivalent Stress (MPa) | Occurrence Time of the Maximum Equivalent Stress (s) | Equivalent Stress Value (t = 0.15 s) (MPa) |
|---|---|---|---|
| 80 | 0.1494 | 0.0750 | 0.0782 |
| 90 | 0.1982 | 0.0900 | 0.0804 |
| 100 | 0.2218 | 0.1080 | 0.2509 |
| Fetal Biparietal Diameter D (mm) | Maximum Principal Stress (MPa) | Occurrence Time of the Maximum Principal Stress (s) | Principal Stress Value (t = 0.15 s) (MPa) |
|---|---|---|---|
| 80 | 0.1172 | 0.0825 | 0.0375 |
| 90 | 0.1941 | 0.0810 | 0.0575 |
| 100 | 0.2136 | 0.0540 | 0.1243 |
| Fetal Biparietal Diameter D (mm) | Maximum Principal Strain | Occurrence Time of the Maximum Principal Strain (s) | Principal Strain Value (t = 0.15 s) |
|---|---|---|---|
| 80 | 0.4507 | 0.0825 | 0.3215 |
| 90 | 0.7834 | 0.0720 | 0.3336 |
| 100 | 0.8270 | 0.0630 | 0.2710 |
| Fetal Biparietal Diameter D (mm) | Maximum uZ (mm) | Extension Ratio in Z Direction | Occurrence Time of the Maximum uZ (s) | Maximum uX (mm) | Occurrence Time of the Maximum uX (s) | Extension Ratio in X Direction |
|---|---|---|---|---|---|---|
| 80 | 50.3580 | 128.90% | 0.1050 | 127.1100 | 0.1275 | 181.59% |
| 90 | 50.6840 | 126.71% | 0.0990 | 140.2100 | 0.1440 | 200.30% |
| 100 | 52.5090 | 131.27% | 0.0990 | 129.3800 | 0.1350 | 184.83% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xuan, R.; Yang, M.; Gao, Y.; Ren, S.; Li, J.; Yang, Z.; Song, Y.; Huang, X.-H.; Teo, E.-C.; Zhu, J.; et al. A Simulation Analysis of Maternal Pelvic Floor Muscle. Int. J. Environ. Res. Public Health 2021, 18, 10821. https://doi.org/10.3390/ijerph182010821
Xuan R, Yang M, Gao Y, Ren S, Li J, Yang Z, Song Y, Huang X-H, Teo E-C, Zhu J, et al. A Simulation Analysis of Maternal Pelvic Floor Muscle. International Journal of Environmental Research and Public Health. 2021; 18(20):10821. https://doi.org/10.3390/ijerph182010821
Chicago/Turabian StyleXuan, Rongrong, Mingshuwen Yang, Yajie Gao, Shuaijun Ren, Jialin Li, Zhenglun Yang, Yang Song, Xu-Hao Huang, Ee-Chon Teo, Jue Zhu, and et al. 2021. "A Simulation Analysis of Maternal Pelvic Floor Muscle" International Journal of Environmental Research and Public Health 18, no. 20: 10821. https://doi.org/10.3390/ijerph182010821
APA StyleXuan, R., Yang, M., Gao, Y., Ren, S., Li, J., Yang, Z., Song, Y., Huang, X.-H., Teo, E.-C., Zhu, J., & Gu, Y. (2021). A Simulation Analysis of Maternal Pelvic Floor Muscle. International Journal of Environmental Research and Public Health, 18(20), 10821. https://doi.org/10.3390/ijerph182010821








