Experimental Study of the Dynamic Compressive and Tensile Anisotropic Mechanical Properties and Failure Modes of Shale
Abstract
1. Introduction
2. Experimental Methods
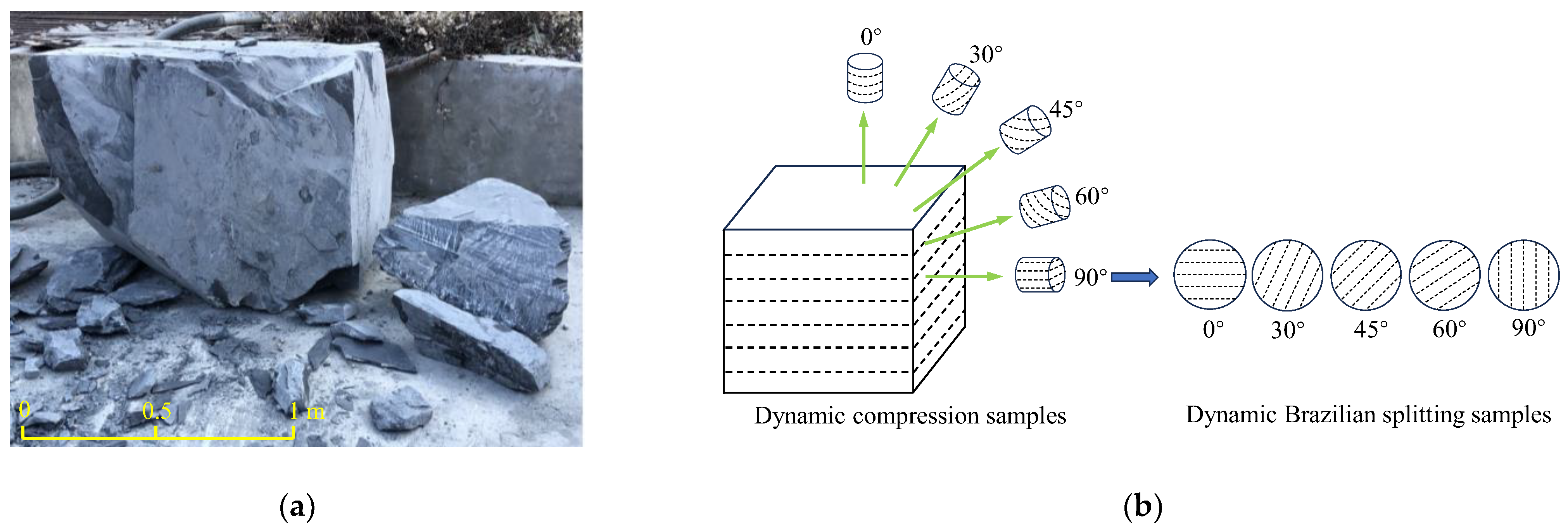
2.1. Shale Sample Selection and Preparation
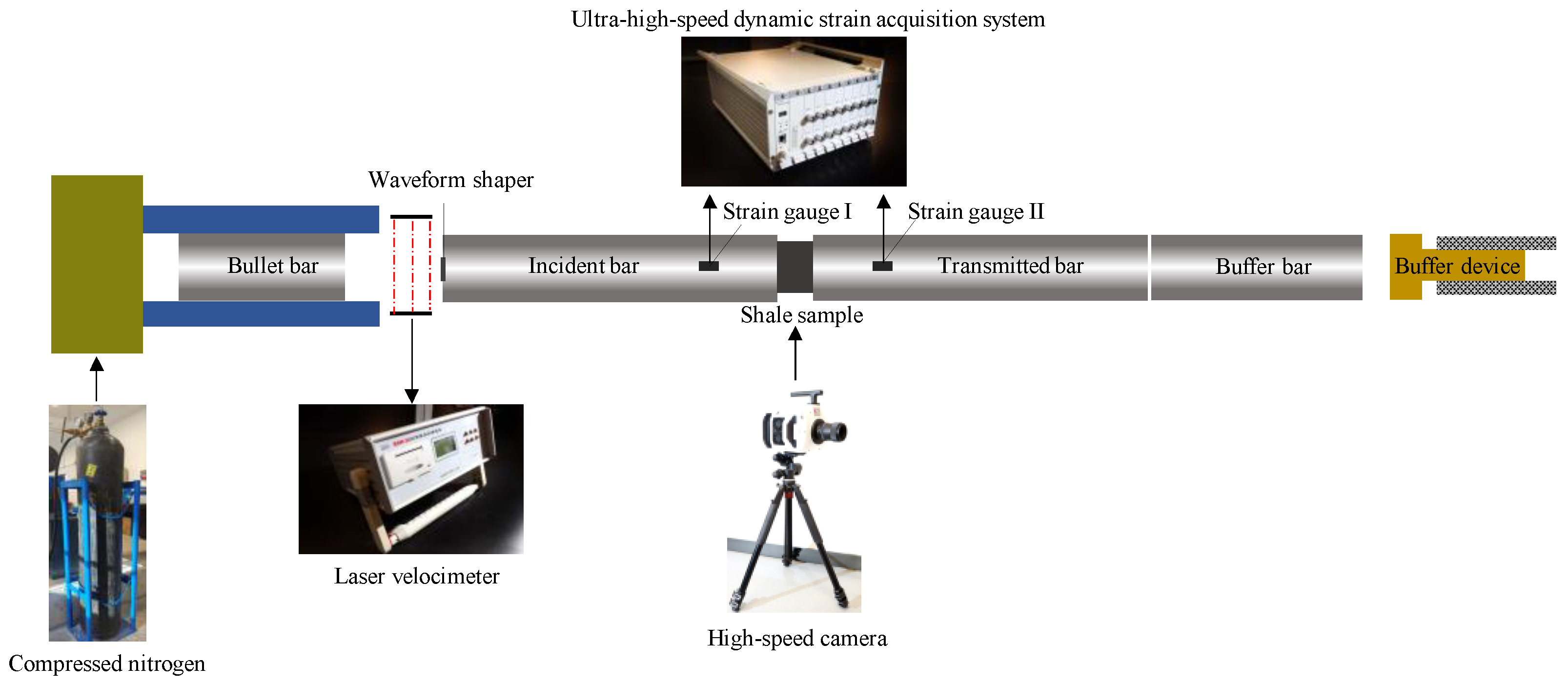
2.2. Experimental Equipment
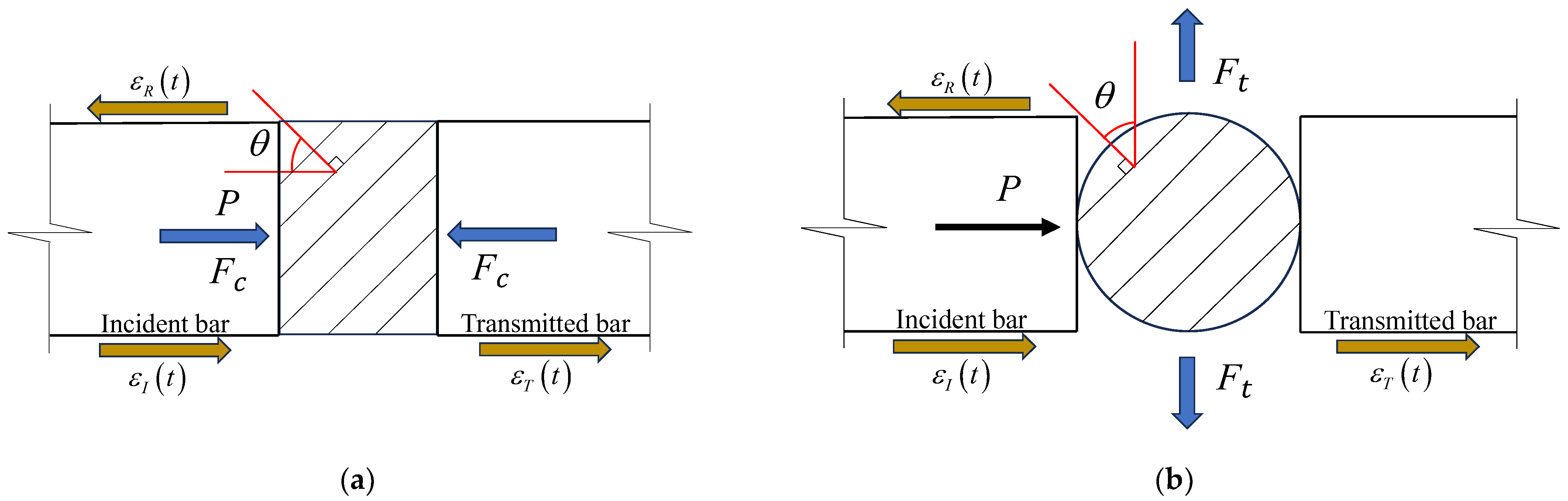
2.3. Scheme and Principle of the Shale Dynamic Compressive Test
2.4. Scheme and Principle of the Dynamic Brazilian Splitting Test for the Shale
3. Dynamic Compressive Mechanical Properties of Shale
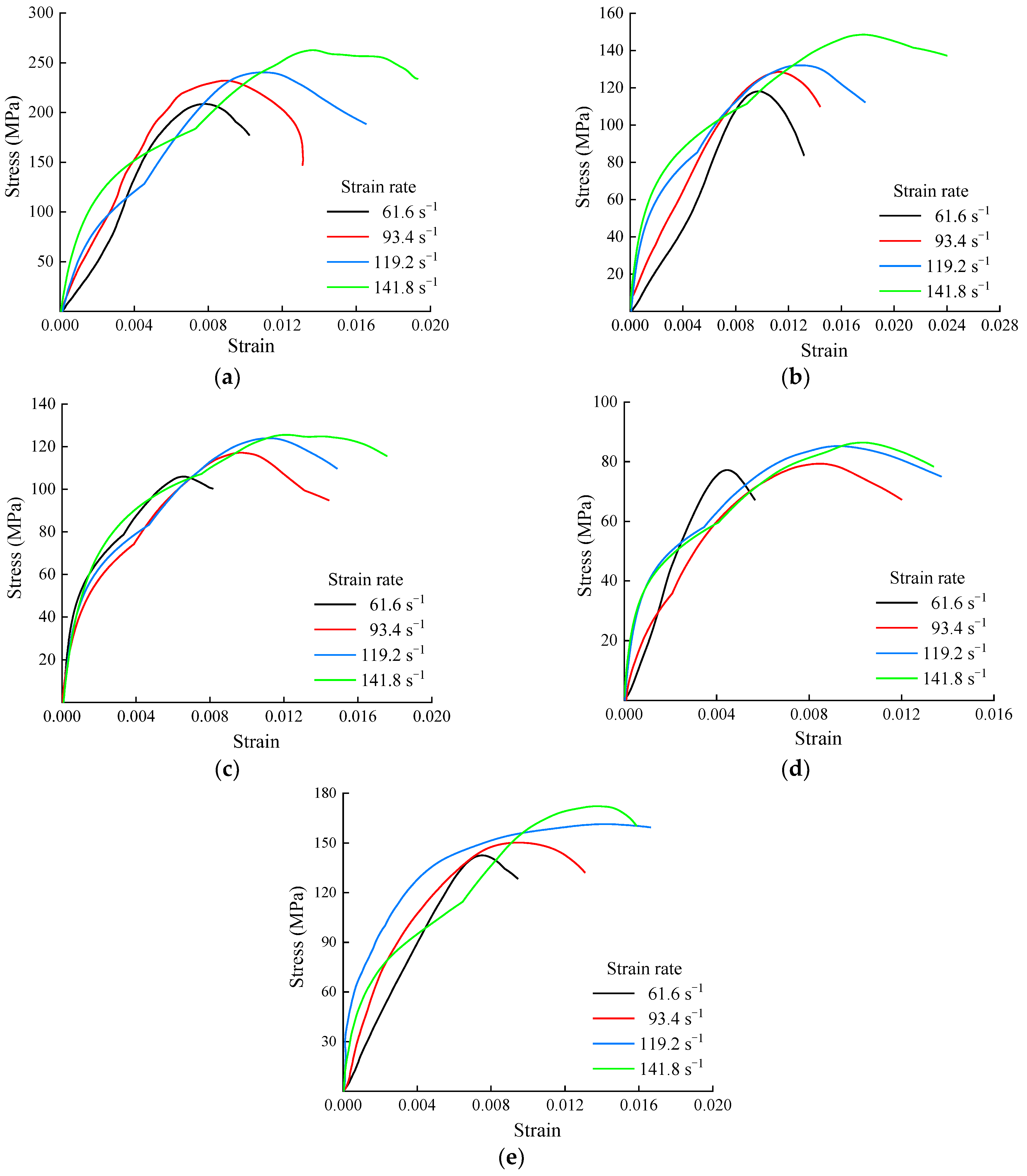
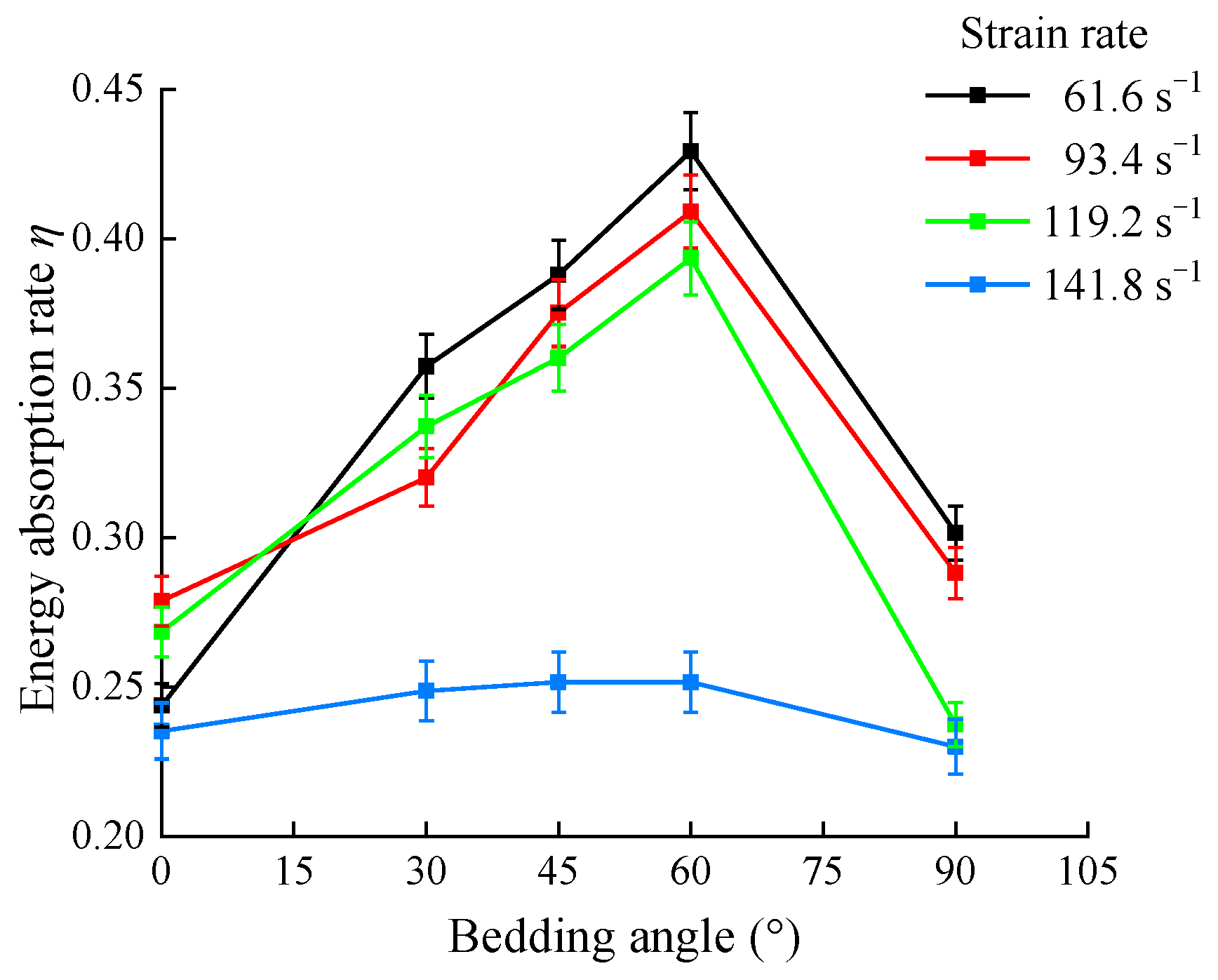
3.1. Dynamic Stress–Strain Curves and Mechanical Parameters
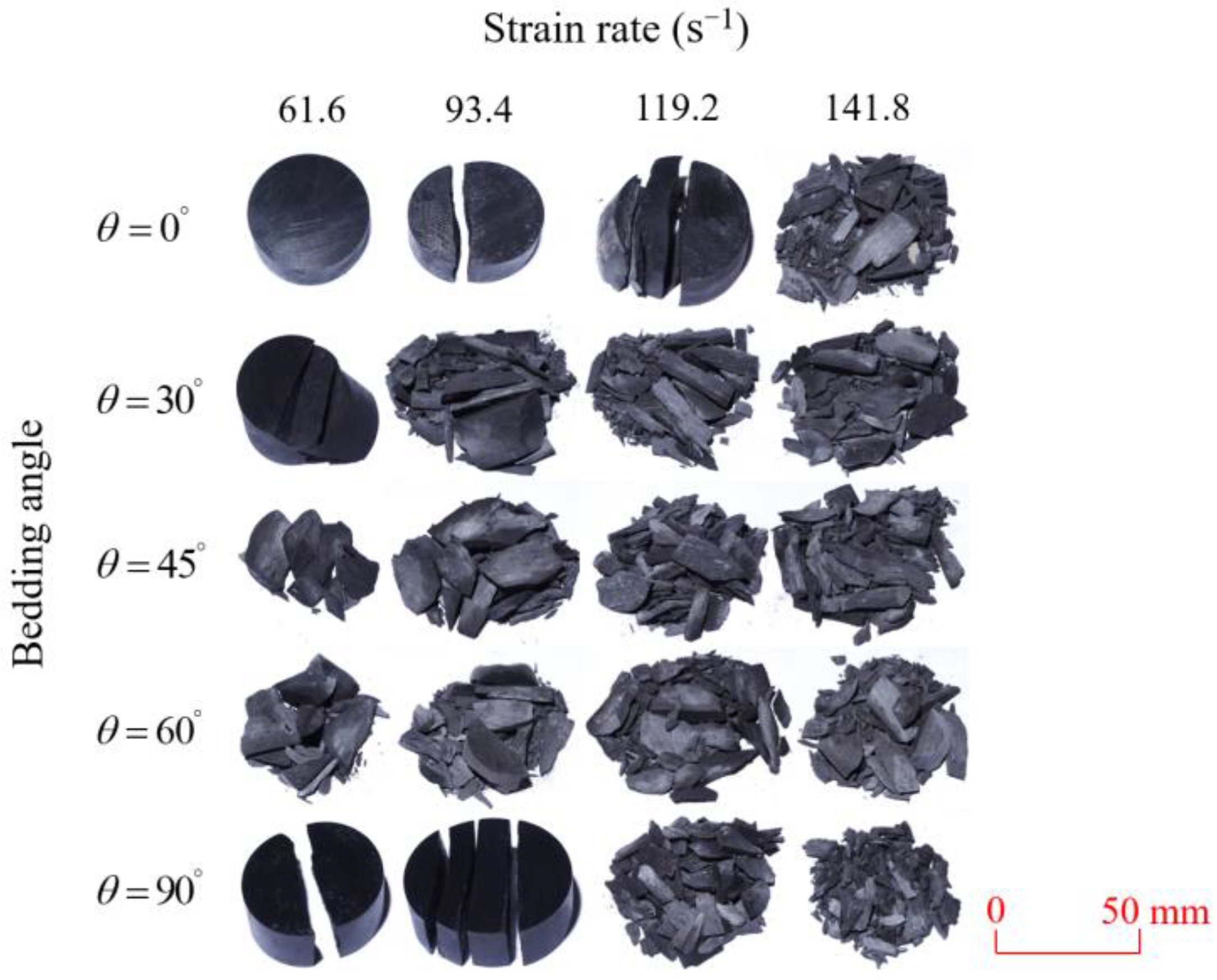
3.2. Dynamic Compressive Failure Mode
4. Dynamic Tensile Mechanical Properties of Shale
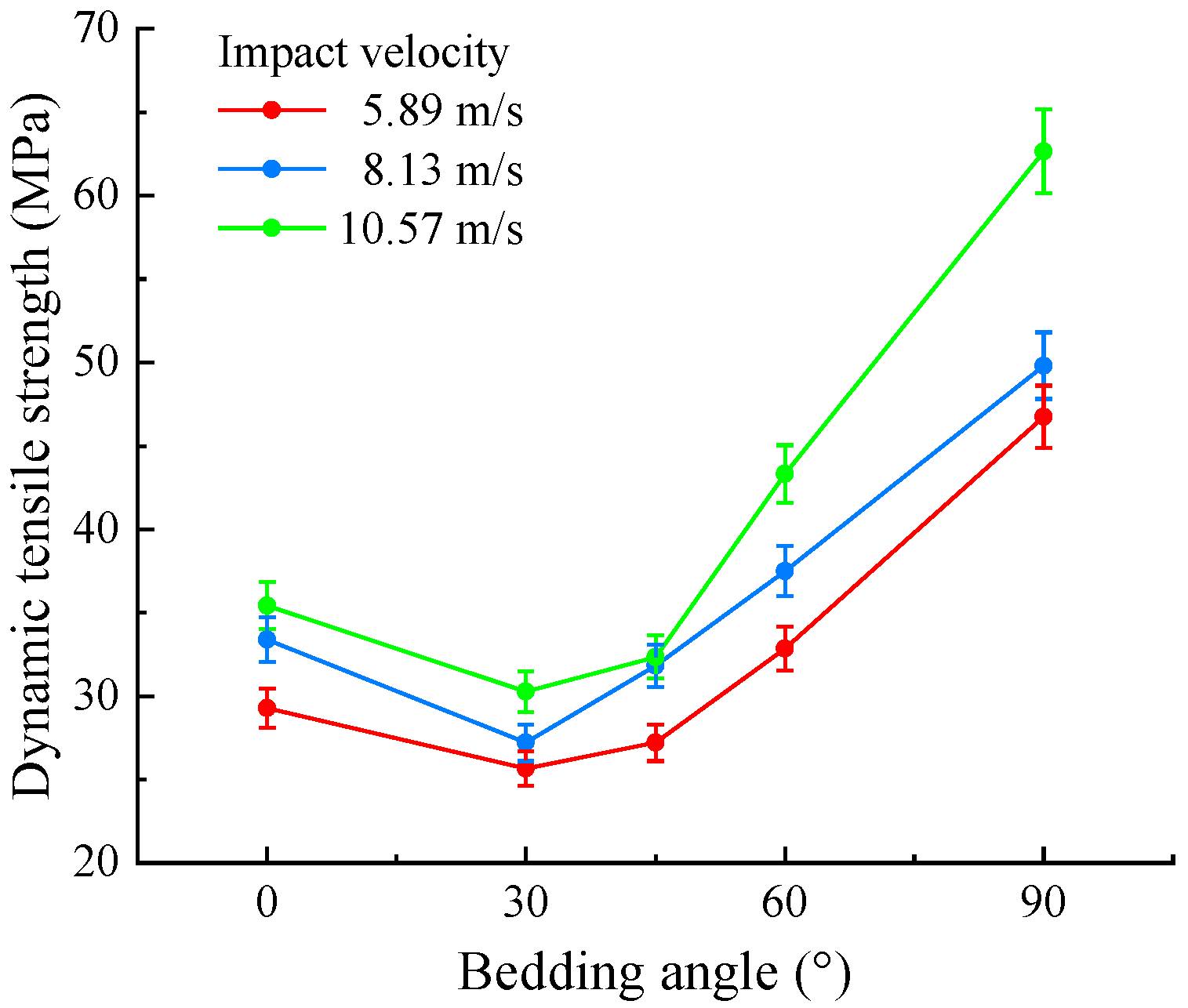
4.1. Dynamic Tensile Strength Analysis
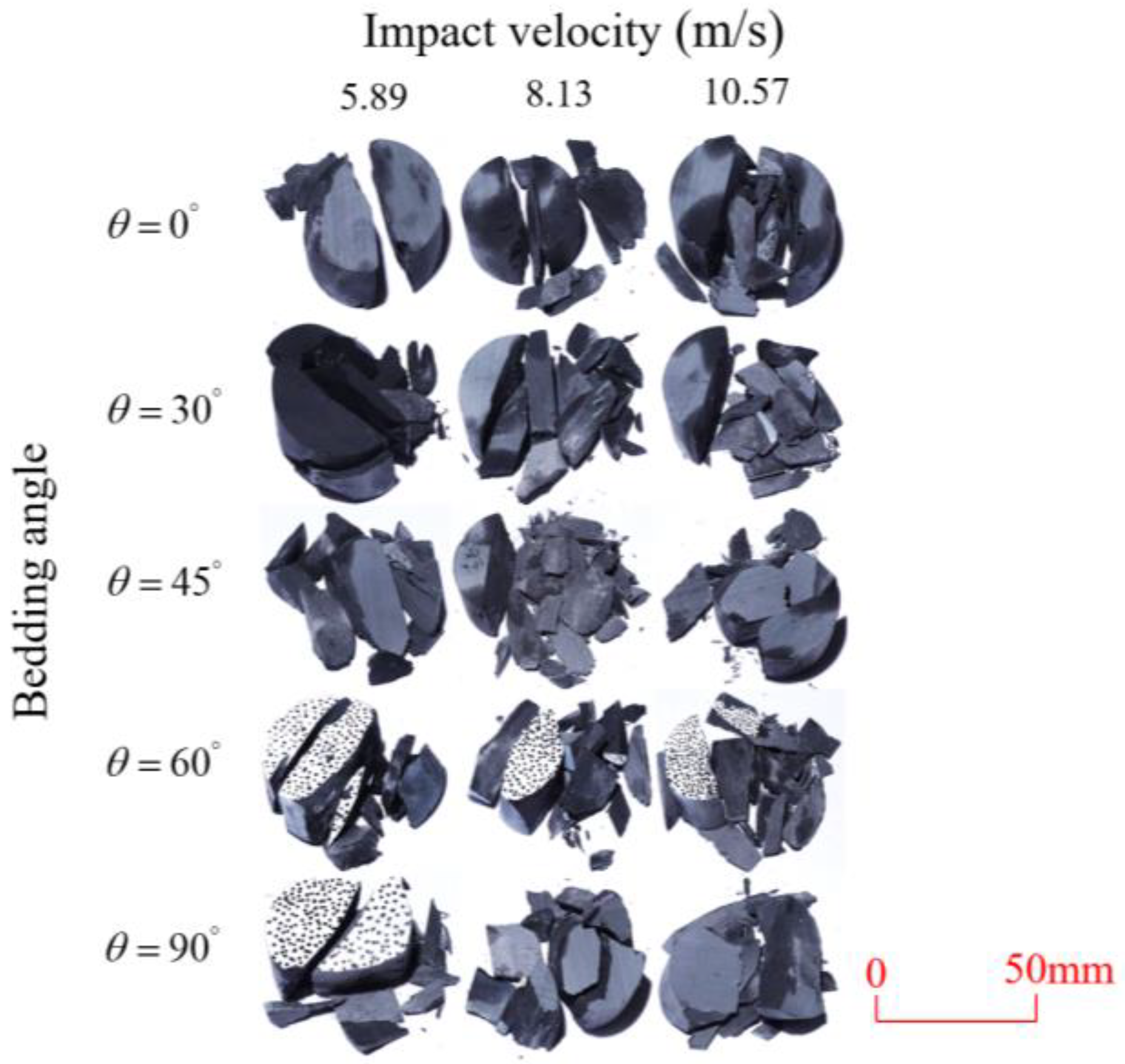
4.2. Dynamic Tensile Failure Mode
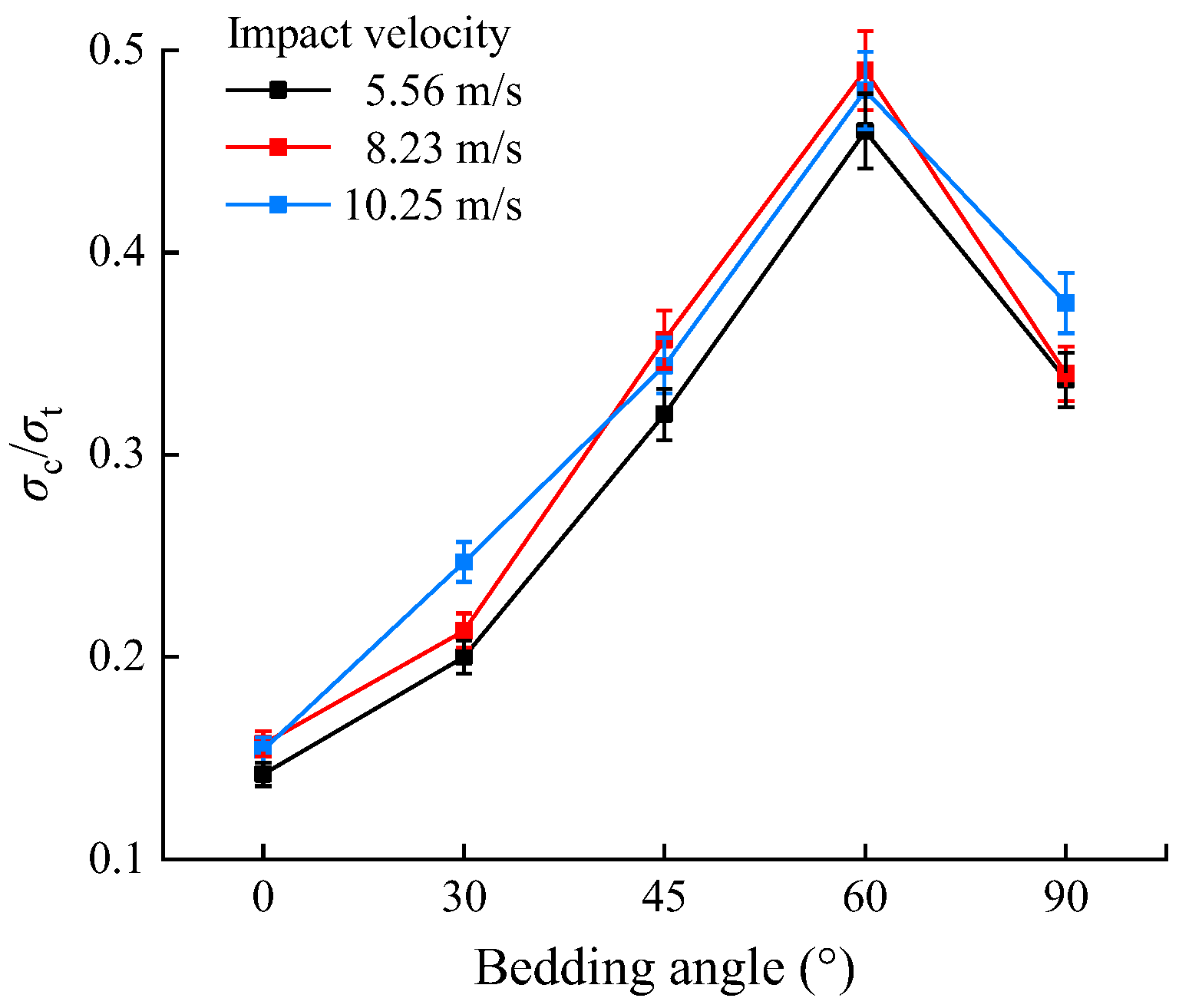
5. Comparison of the Dynamic Compressive and Tensile Mechanical Properties and Failure Modes of Shale
6. Conclusions
- (1)
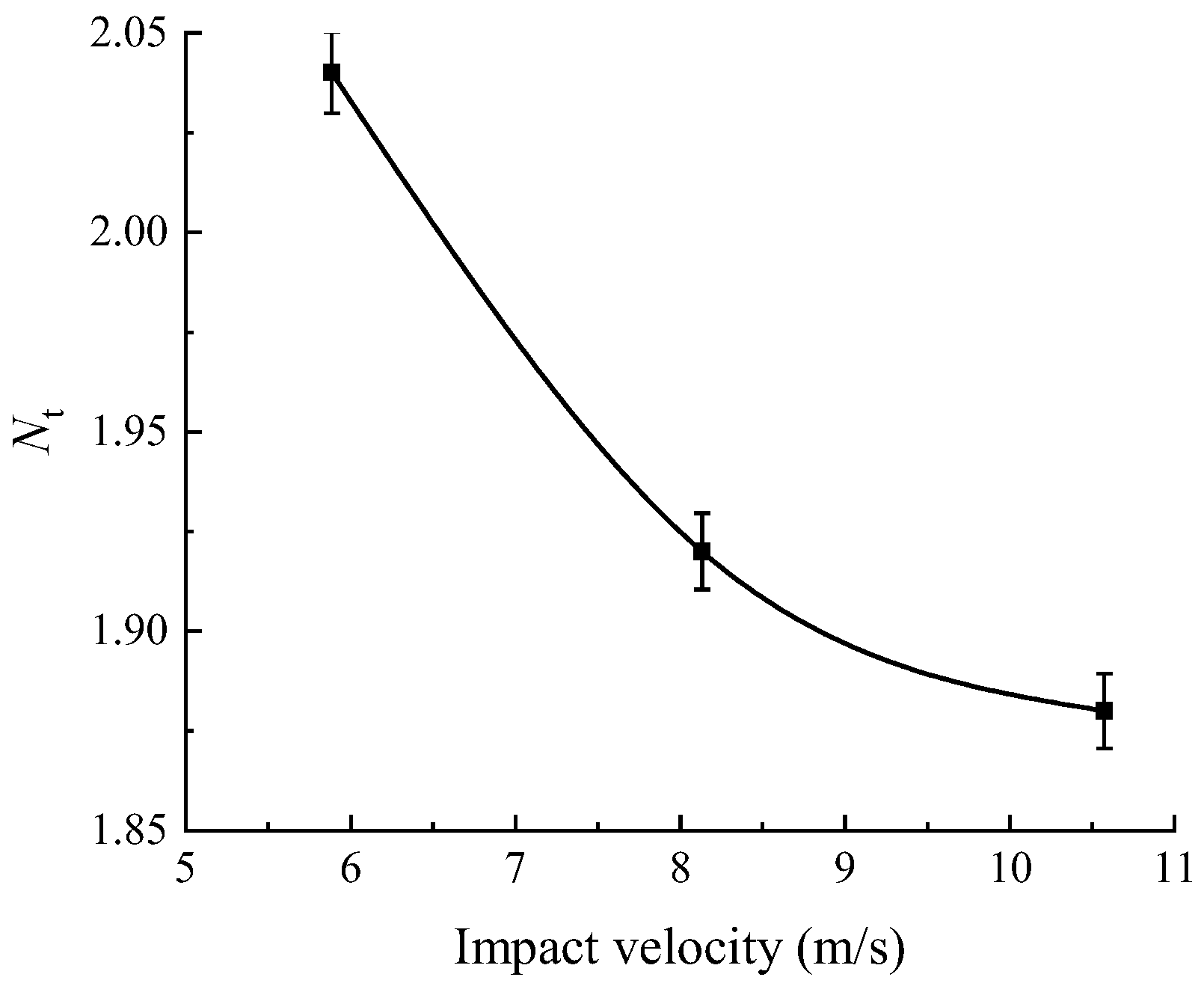
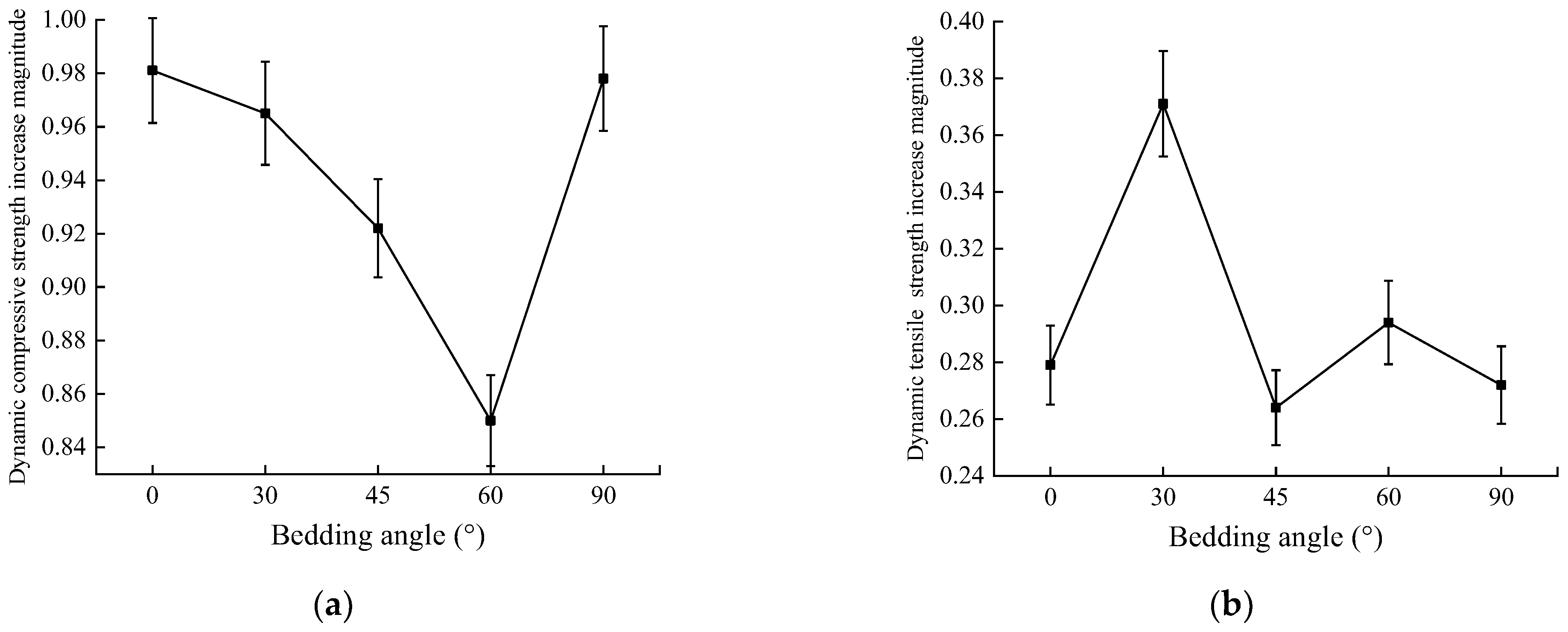
- The dynamic compressive strength and tensile strength of the shale increases with the increases in the strain rate and impact velocity, and the dynamic compressive strength demonstrated a significantly higher sensitivity to both the strain rate and impact velocity compared to the dynamic tensile strength. With the increase in bedding angle, the dynamic strength of the shale exhibited a pattern of an initial decrease followed by increase because the minimum value of dynamic compressive strength is achieved at a bedding angle of 60°, while the minimum value of the dynamic tensile strength was achieved at a bedding angle of 30°. This indicates that the dynamic strength of the shale exhibited significant anisotropy. Furthermore, as the strain rate and impact velocity increased, the degree of anisotropy in the dynamic mechanical strength of the shale decreased.
- (2)
- The dynamic compressive failure mode of the shale also exhibited anisotropic characteristics and was closely related to the strain rate. For the shale samples with bedding angles of 0° and 90°, the dynamic compressive failure modes under different strain rates primarily consist of splitting tensile failure within the shale matrix and along the bedding planes, respectively. For samples with bedding angles of 30°, 45°, and 60°, the failure mode was primarily shear failure along the bedding planes at low strain rates and evolved into a composite shear failure along both the bedding planes and the shale matrix as the strain rate increased.
- (3)
- The dynamic tensile failure mode of the shale was influenced by the bedding angle and impact velocity. For the shale samples with a bedding angle of 0°, the dynamic tensile failure mode is characterized by tensile failure along the bedding planes. For samples with bedding angles of 30° and 45°, the primary failure mode involved a combination of tensile and shear failure along the bedding planes. For samples with bedding angles of 60° and 90°, the tensile and shear failure occurred along the bedding planes and within the shale matrix at lower impact velocities. However, at higher impact velocities, tensile failure along the loading direction within the shale matrix predominates.
- (4)
- The dynamic strength characteristics of the shale samples were significantly correlated with their failure modes. When the failure mode was dominated by shear failure along the bedding planes (e.g., at a bedding angle of 60° in the dynamic compression tests and 30° in the dynamic Brazilian splitting tests), the dynamic strength of the shale was the lowest. Conversely, when the failure mode was dominated by failure within the shale matrix (e.g., at a bedding angle of 0° in the dynamic compression tests and 90° in the dynamic Brazilian splitting tests), the dynamic strength of the shale was the greatest.
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Josh, M.; Esteban, L.; Piane, C.D.; Sarout, J.; Dewhurst, D.N.; Clennell, M.B. Laboratory characterisation of shale properties. J. Pet. Sci. Eng. 2012, 88/89, 107–124. [Google Scholar] [CrossRef]
- Wu, D.; Yu, L.; Su, H.; Geng, S.; Zhang, T.; Wei, C. The effects of three-dimensional bedding on shale fracture behavior: Insights from experimental and numerical investigations. Eng. Fract. Mech. 2024, 307, 110295. [Google Scholar] [CrossRef]
- Liu, J.; Zhang, Y.; Yang, X.; Su, C. Experimental study on the uniaxial compression failure and ultrasonic transmission of brittle shale considering the bedding effect. IEEE Access 2020, 8, 217883–217896. [Google Scholar] [CrossRef]
- Jin, J.; Wang, L.; Yan, Z.; Li, G.; Wang, J. Effects of strain rate and bedding on shale fracture mechanisms. Int. J. Mech. Sci. 2024, 277, 109398. [Google Scholar] [CrossRef]
- Chen, T.; Feng, X.; Zhang, X.; Cao, W.; Fu, C. Experimental study on mechanical and anisotropic properties of black shale. Chin. J. Rock Mech. Eng. 2014, 33, 1772–1779. [Google Scholar]
- Zheng, Z.; Xiao, M.; Zhuo, L.; Liu, H. Transversely isotropic mechanical properties of water-bearing shale: An experimental investigation. IOP Conf. Ser. Earth Environ. Sci. 2021, 861, 022008. [Google Scholar] [CrossRef]
- He, R.; Ren, L.; Zhang, R.; Zhu, Z.; Sun, X. Anisotropy characterization of the elasticity and energy flow of Longmaxi shale under uniaxial compression. Energy Rep. 2022, 8, 1410–1424. [Google Scholar] [CrossRef]
- Dong, Q.; Kang, J.; Sun, J.; Li, J.; Zhang, Z. Experimental study on anisotropic mechanical characteristics of shale under triaxial loading. Appl. Sci. 2024, 14, 3849. [Google Scholar] [CrossRef]
- Rybacki, E.; Meier, T.; Dresen, G. What controls the mechanical properties of shale rocks?–Part II: Brittleness. J. Pet. Sci. Eng. 2016, 144, 39–58. [Google Scholar] [CrossRef]
- Ren, L.; Xie, H.; Sun, X.; Zhang, R.; Li, C.; Xie, J.; Zhang, Z. Characterization of anisotropic fracture properties of Silurian Longmaxi shale. Rock Mech. Rock Eng. 2021, 54, 665–678. [Google Scholar] [CrossRef]
- Sayers, C.M. The effect of anisotropy on the young’s moduli and poisson’s ratios of shales. Geophys. Prospect. 2013, 61, 416–426. [Google Scholar] [CrossRef]
- Wan, Y.; Pan, Z.; Tang, S.; Connell, L.D.; Down, D.D.; Camilleri, M. An experimental investigation of diffusivity and porosity anisotropy of a Chinese gas shale. J. Nat. Gas Sci. Eng. 2015, 23, 70–79. [Google Scholar] [CrossRef]
- Niandou, H.; Shao, J.F.; Henry, J.P.; Fourmaintraux, D. Laboratory investigation of the mechanical behaviour of tournemire shale. Int. J. Rock Mech. Min. Sci. 1997, 34, 3–16. [Google Scholar] [CrossRef]
- Geng, Z.; Chen, M.; Jin, Y.; Yang, S.; Du, X. Experimental study of brittleness anisotropy of shale in triaxial compression. J. Nat. Gas Sci. Eng. 2016, 36, 510–518. [Google Scholar] [CrossRef]
- Heng, S.; Li, X.; Liu, X.; Chen, Y. Experimental study on the mechanical properties of bedding planes in shale. J. Nat. Gas Sci. Eng. 2020, 76, 103161. [Google Scholar] [CrossRef]
- Jin, Z.; Li, W.; Jin, C.; Hambleton, J.; Cusatis, G. Anisotropic elastic, strength, and fracture properties of Marcellus shale. Int. J. Rock Mech. Min. Sci. 2018, 109, 124–137. [Google Scholar] [CrossRef]
- Tavallali, A.; Vervoort, A. Effect of layer orientation on the failure of layered sandstone under brazilian test conditions. Int. J. Rock Mech. Min. Sci. 2010, 47, 313–322. [Google Scholar] [CrossRef]
- Tien, Y.M.; Kuo, M.C.; Juang, C.H. An experimental investigation of the failure mechanism of simulated transversely isotropic rocks. Int. J. Rock Mech. Min. Sci. 2006, 43, 1163–1181. [Google Scholar] [CrossRef]
- Wang, J.; Xie, L.; Xie, H.; Ren, L.; He, B.; Li, C.; Yang, Z.; Gao, C. Effect of layer orientation on acoustic emission characteristics of anisotropic shale in Brazilian tests. J. Nat. Gas Sci. Eng. 2016, 36, 1120–1129. [Google Scholar] [CrossRef]
- Qiu, J.; Li, D.; Li, X. Dynamic failure of a phyllite with a low degree of metamorphism under impact brazilian test. Int. J. Rock Mech. Min. Sci. 2017, 94, 10–17. [Google Scholar] [CrossRef]
- Yang, G.; Liu, J.; Li, X.; Bi, J. Effect of temperature on shale strength under dynamic impact loading. Arab. J. Geosci. 2020, 13, 432. [Google Scholar] [CrossRef]
- Shi, X.; Zhao, Y.; Yao, W.; Gong, S.; Danesh, N.N. Dynamic tensile failure of layered sorptive rocks: Shale and coal. Eng. Fail. Anal. 2022, 138, 106346. [Google Scholar] [CrossRef]
- Fan, X.; Luo, N.; Yuan, Y.; Liang, H.; Zhai, C.; Qu, Z.; Li, M. Dynamic mechanical behavior and damage constitutive model of shales with different bedding under compressive impact loading. Arab. J. Geosci. 2021, 14, 1752. [Google Scholar] [CrossRef]
- Zhang, H.; Wang, L.; Zheng, K.; Bakura, T.J.; Totakhil, P.G. Research on compressive impact dynamic behavior and constitutive model of polypropylene fiber reinforced concrete. Constr. Build. Mater. 2018, 187, 584–595. [Google Scholar] [CrossRef]
- Ai, D.; Zhao, Y.; Wang, Q.; Li, C. Crack propagation and dynamic properties of coal under SHPB impact loading: Experimental investigation and numerical simulation. Theor. Appl. Fract. Mech. 2020, 105, 102393. [Google Scholar] [CrossRef]
- Sun, Q.; Zhang, Z.; Li, P.; Sun, Z.; Lu, D.; Gao, F. Study on the bedding effect and damage constitutive model of black shale under dynamic loading. Chin. J. Rock Mech. Eng. 2019, 38, 1319–1331. [Google Scholar]
- Guo, Y.; Li, X.; Huang, L. Insight into spontaneous water-based working fluid imbibition on the dynamic tensile behavior of anisotropic shale. Eng. Geol. 2022, 308, 106830. [Google Scholar] [CrossRef]
- Zhang, Q.; Zhao, J. A review of dynamic experimental techniques and mechanical behaviour of rock materials. Rock Mech. Rock Eng. 2014, 47, 1411–1478. [Google Scholar] [CrossRef]
- Li, W.; Xu, J. Impact characterization of basalt fiber reinforced geopolymeric concrete using a 100-mm-diameter split Hopkinson pressure bar. Mater. Sci. Eng. A 2009, 513, 145–153. [Google Scholar] [CrossRef]
- Wang, Z.; Lu, Z. Application of SHPB in Experimental Teaching of Rock Dynamics. Res. Explor. Lab. 2014, 33, 214–258. [Google Scholar]
- Kolsky, H. An investigation of the mechanical properties of materials at very high rates of loading. Proc. Phys. Soc. B 1949, 62, 676–700. [Google Scholar] [CrossRef]
- Follansbee, P.S.; Frantz, C. Wave propagation in the split hopkinson pressure bar. J. Eng. Mater. Technol. 1983, 105, 61–66. [Google Scholar] [CrossRef]
- Chen, W.; Song, B. Split Hopkinson (Kolsky) Bar: Design, Testing and Applications; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2010. [Google Scholar]
- Xia, K.; Yao, W. Dynamic rock tests using split Hopkinson (Kolsky) bar system–A review. J. Rock Mech. Geotech. Eng. 2015, 7, 27–59. [Google Scholar] [CrossRef]
- Ping, Q.; Ma, Q.; Zhang, J.; Yuan, P.; Chen, B. SHPB test and analysis of dynamic tensile performance of sandstone under high strain rate. Chin. J. Rock Mech. Eng. 2012, 31 (Suppl. S1), 3363–3369. [Google Scholar]
- Li, X.; Feng, F.; Li, D.; Du, K.; Ranjith, P.G.; Rostami, J. Failure characteristics of granite influenced by sample height-to-width ratios and intermediate principal stress under true-triaxial unloading conditions. Rock Mech. Rock Eng. 2018, 51, 1321–1345. [Google Scholar] [CrossRef]
- Liu, H.; Feng, X.; Liu, L.; Li, T.; Tang, C. Mechanical properties and failure characteristics of anisotropic shale with circular hole under combined dynamic and static loading. Int. J. Rock Mech. Min. Sci. 2023, 170, 105524. [Google Scholar] [CrossRef]
- Singh, J.; Ramamurthy, T.; Rao, G.V. Strength anisotropies in rocks. Indian Geotech. J. 1989, 19, 147–166. [Google Scholar]
- Li, X.; Li, H.; Zhang, Q.; Jiang, J.; Zhao, J. Dynamic fragmentation of rock material: Characteristic size, fragment distribution and pulverization law. Eng. Fract. Mech. 2018, 199, 739–759. [Google Scholar] [CrossRef]
- Zhang, Z.; Kou, S.; Jiang, L.; Lindqvist, P.A. Effects of loading rate on rock fracture: Fracture characteristics and energy partitioning. Int. J. Rock Mech. Min. Sci. 2000, 37, 745–762. [Google Scholar] [CrossRef]
- Ping, Q.; Zhang, C.; Sun, H.; Han, X. Dynamic mechanical properties and energy dissipation analysis of sandstone after high temperature cycling. Shock Vib. 2020, 2020, 8848595. [Google Scholar] [CrossRef]



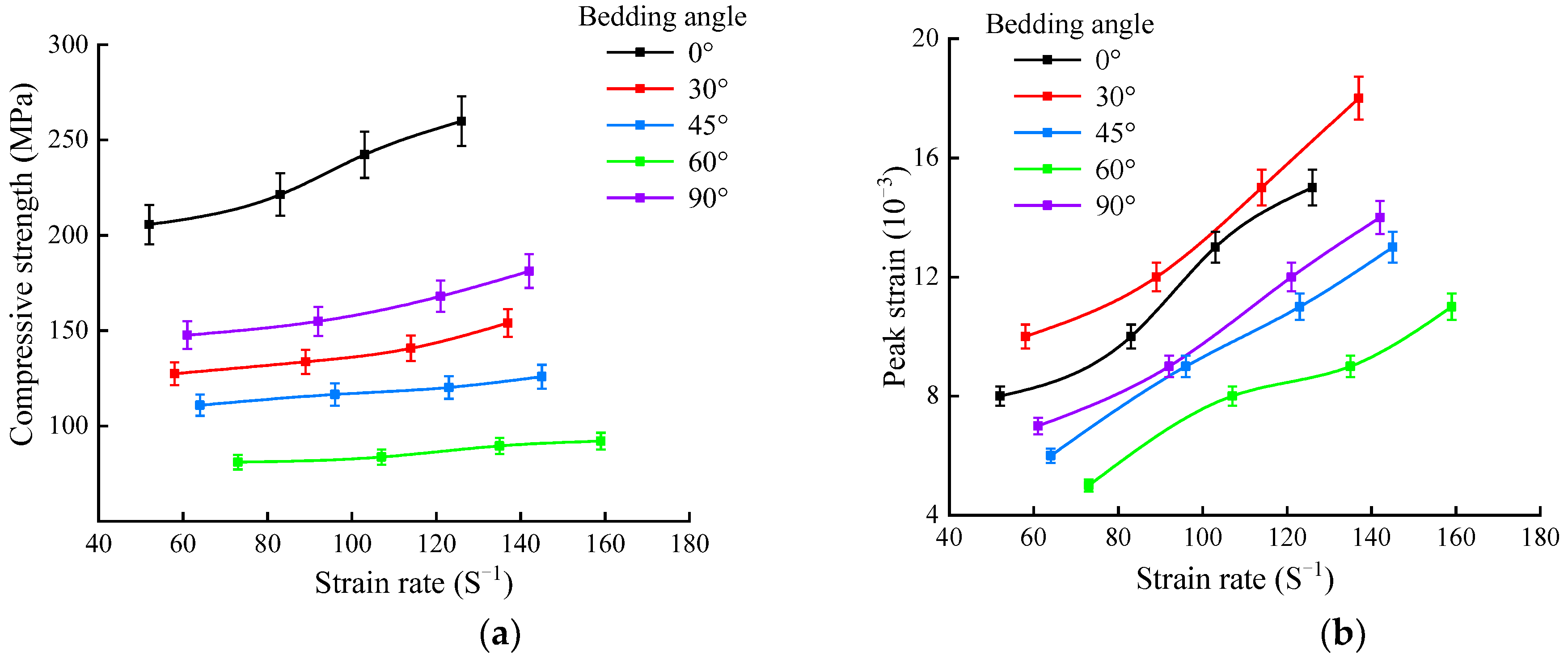
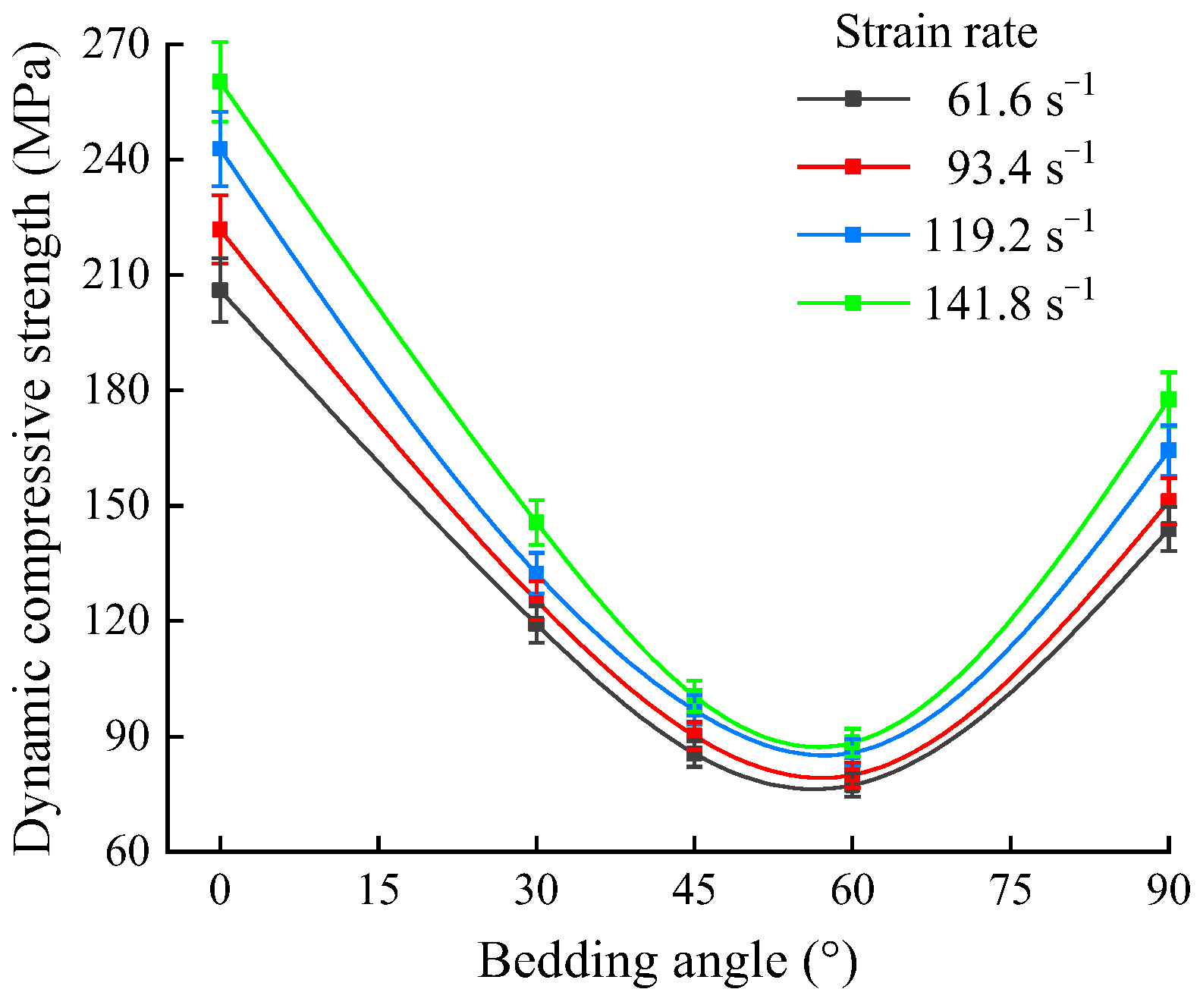


| Bedding Angle (°) | Impact Pressure (MPa) | Impact Velocity (m/s) | Compressive Strength (MPa) | Peak Strain (10−3) | Strain Rate (s−1) |
|---|---|---|---|---|---|
| 0 | 0.1 | 5.16 | 206.08 | 8 | 52 |
| 0.2 | 8.16 | 221.79 | 10 | 83 | |
| 0.3 | 10.13 | 242.75 | 13 | 103 | |
| 0.4 | 12.36 | 260.21 | 15 | 126 | |
| 30 | 0.1 | 5.42 | 119.20 | 10 | 58 |
| 0.2 | 8.39 | 125.31 | 12 | 89 | |
| 0.3 | 10.23 | 132.44 | 15 | 114 | |
| 0.4 | 12.39 | 145.69 | 18 | 137 | |
| 45 | 0.1 | 5.23 | 85.53 | 6 | 64 |
| 0.2 | 8.42 | 90.15 | 9 | 96 | |
| 0.3 | 10.34 | 96.90 | 11 | 123 | |
| 0.4 | 11.98 | 100.52 | 13 | 145 | |
| 60 | 0.1 | 5.13 | 72.36 | 5 | 73 |
| 0.2 | 8.35 | 79.56 | 8 | 107 | |
| 0.3 | 10.24 | 89.81 | 9 | 135 | |
| 0.4 | 12.44 | 97.41 | 11 | 159 | |
| 90 | 0.1 | 5.14 | 143.96 | 7 | 61 |
| 0.2 | 8.35 | 151.16 | 9 | 92 | |
| 0.3 | 10.67 | 164.36 | 12 | 121 | |
| 0.4 | 12.07 | 177.56 | 14 | 142 |
| Bedding Angle (°) | Impact Pressure (MPa) | Impact Velocity (m/s) | Dynamic Tensile Strength (MPa) |
|---|---|---|---|
| 0° | 0.1 | 5.81 | 29.27 |
| 0.2 | 8.01 | 34.88 | |
| 0.3 | 10.61 | 37.43 | |
| 30° | 0.1 | 6.08 | 23.81 |
| 0.2 | 8.29 | 26.70 | |
| 0.3 | 10.24 | 32.65 | |
| 45° | 0.1 | 5.85 | 27.33 |
| 0.2 | 8.37 | 32.19 | |
| 0.3 | 10.31 | 34.54 | |
| 60° | 0.1 | 5.92 | 33.32 |
| 0.2 | 7.86 | 38.99 | |
| 0.3 | 10.70 | 43.32 | |
| 90° | 0.1 | 5.77 | 48.46 |
| 0.2 | 8.14 | 51.35 | |
| 0.3 | 11.01 | 61.62 |
| Bedding Angle (°) | Impact Velocity (m/s) | |||
|---|---|---|---|---|
| 5.89 | 8.13 | 10.57 | ||
| 0 | Original image |  |  |  |
| DIC image | 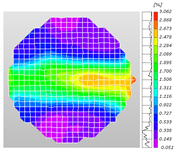 | 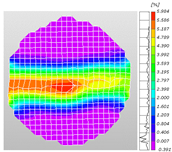 |  | |
| 30 | Original image |  |  |  |
| DIC image | 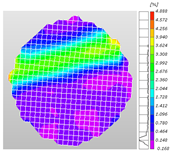 |  |  | |
| 45 | Original image |  | 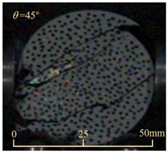 |  |
| DIC image | 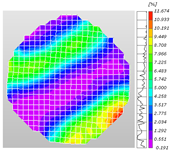 |  | 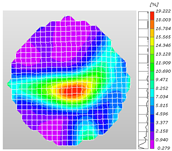 | |
| 60 | Original image |  |  | 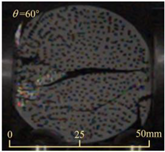 |
| DIC image |  |  |  | |
| 90 | Original image |  | 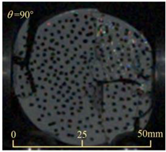 |  |
| DIC image | 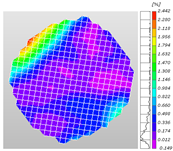 |  |  | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dong, Q.; Tong, H.; Sun, J.; Peng, S.; Jia, J. Experimental Study of the Dynamic Compressive and Tensile Anisotropic Mechanical Properties and Failure Modes of Shale. Sensors 2025, 25, 2905. https://doi.org/10.3390/s25092905
Dong Q, Tong H, Sun J, Peng S, Jia J. Experimental Study of the Dynamic Compressive and Tensile Anisotropic Mechanical Properties and Failure Modes of Shale. Sensors. 2025; 25(9):2905. https://doi.org/10.3390/s25092905
Chicago/Turabian StyleDong, Qian, Hao Tong, Jinshan Sun, Songlin Peng, and Jijie Jia. 2025. "Experimental Study of the Dynamic Compressive and Tensile Anisotropic Mechanical Properties and Failure Modes of Shale" Sensors 25, no. 9: 2905. https://doi.org/10.3390/s25092905
APA StyleDong, Q., Tong, H., Sun, J., Peng, S., & Jia, J. (2025). Experimental Study of the Dynamic Compressive and Tensile Anisotropic Mechanical Properties and Failure Modes of Shale. Sensors, 25(9), 2905. https://doi.org/10.3390/s25092905






