Non-Invasive Real-Time Monitoring of Bacterial Activity by Non-Contact Impedance Spectroscopy for Off-the-Shelf Labware
Abstract
1. Introduction
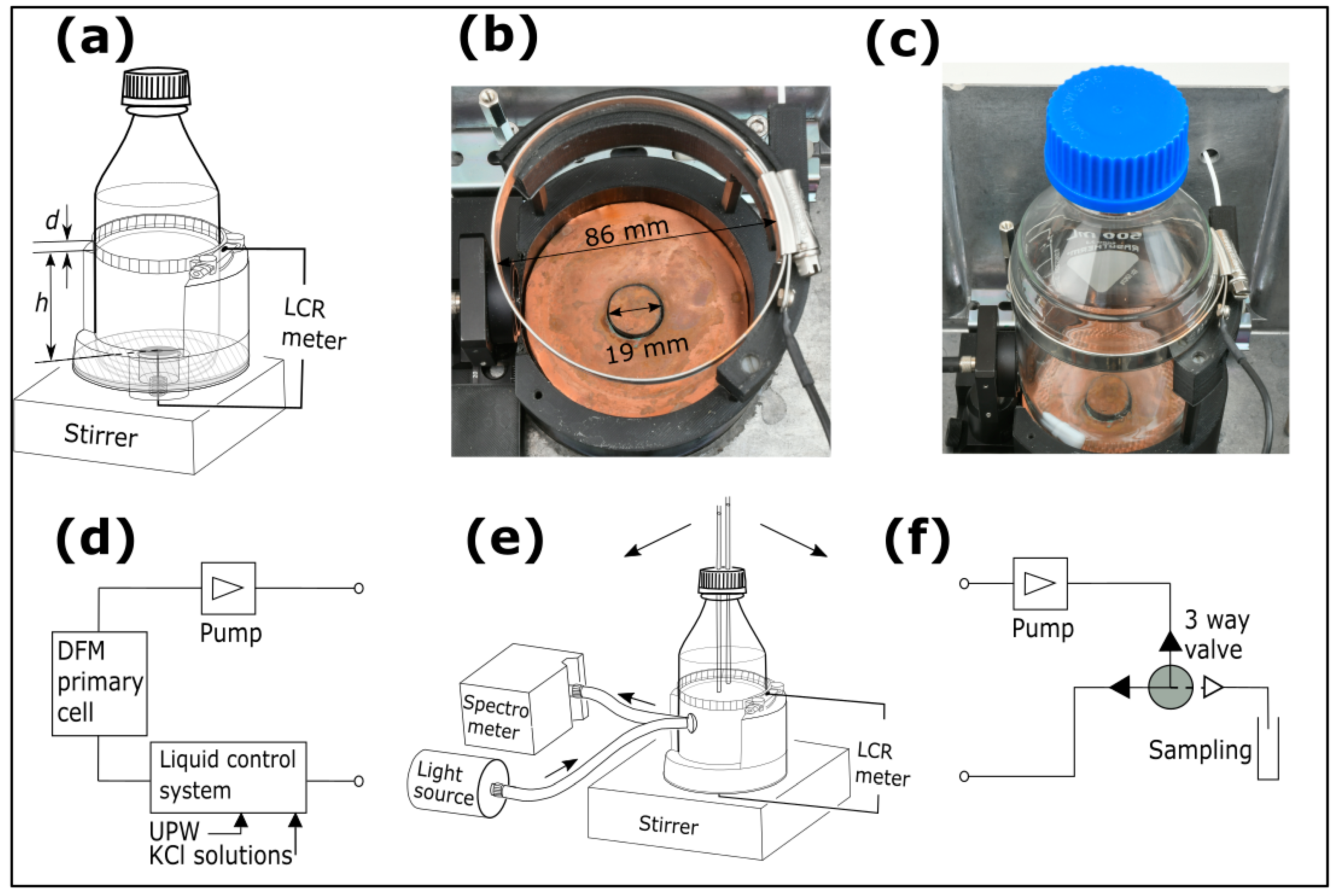
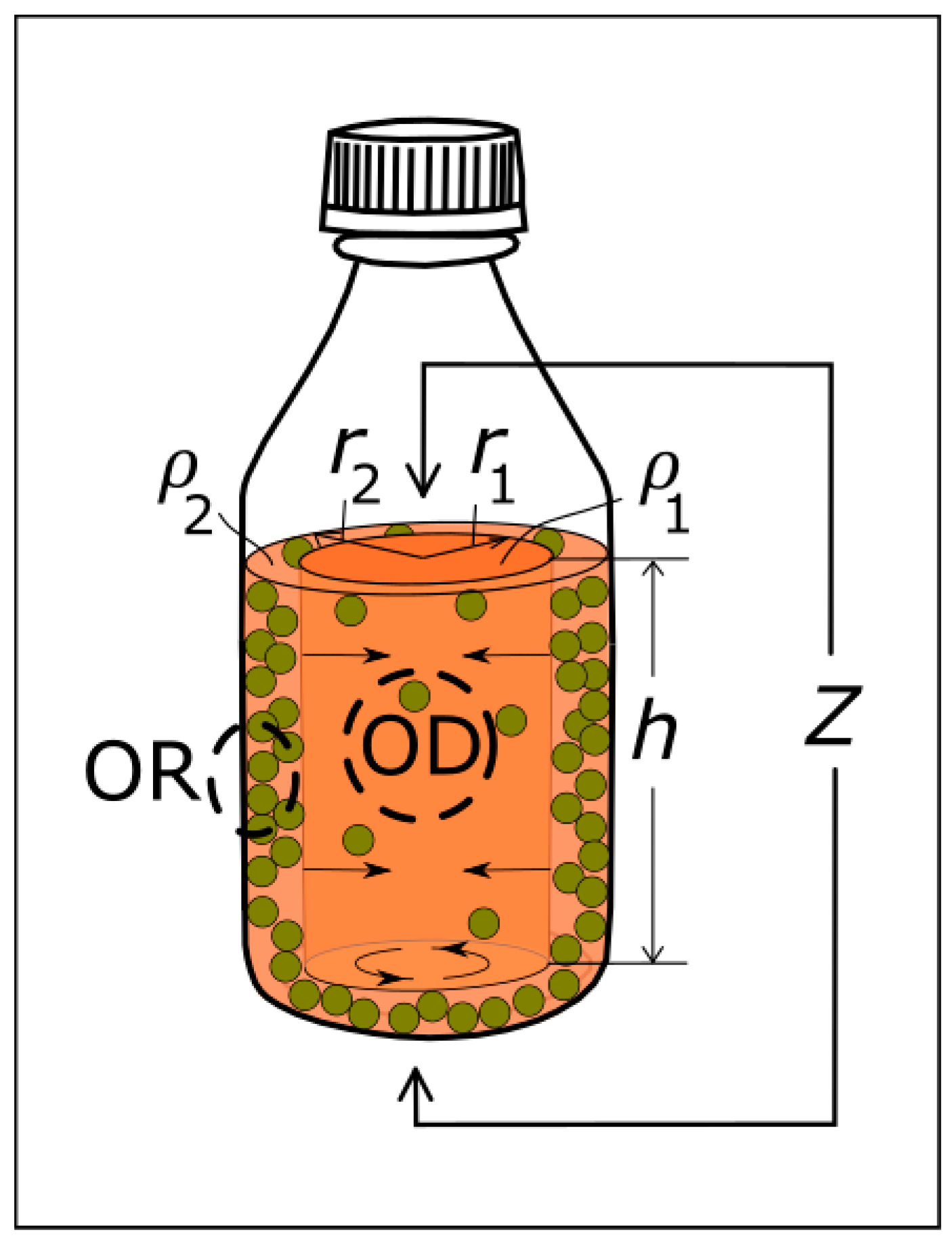
2. Materials and Methods
3. Results and Discussion
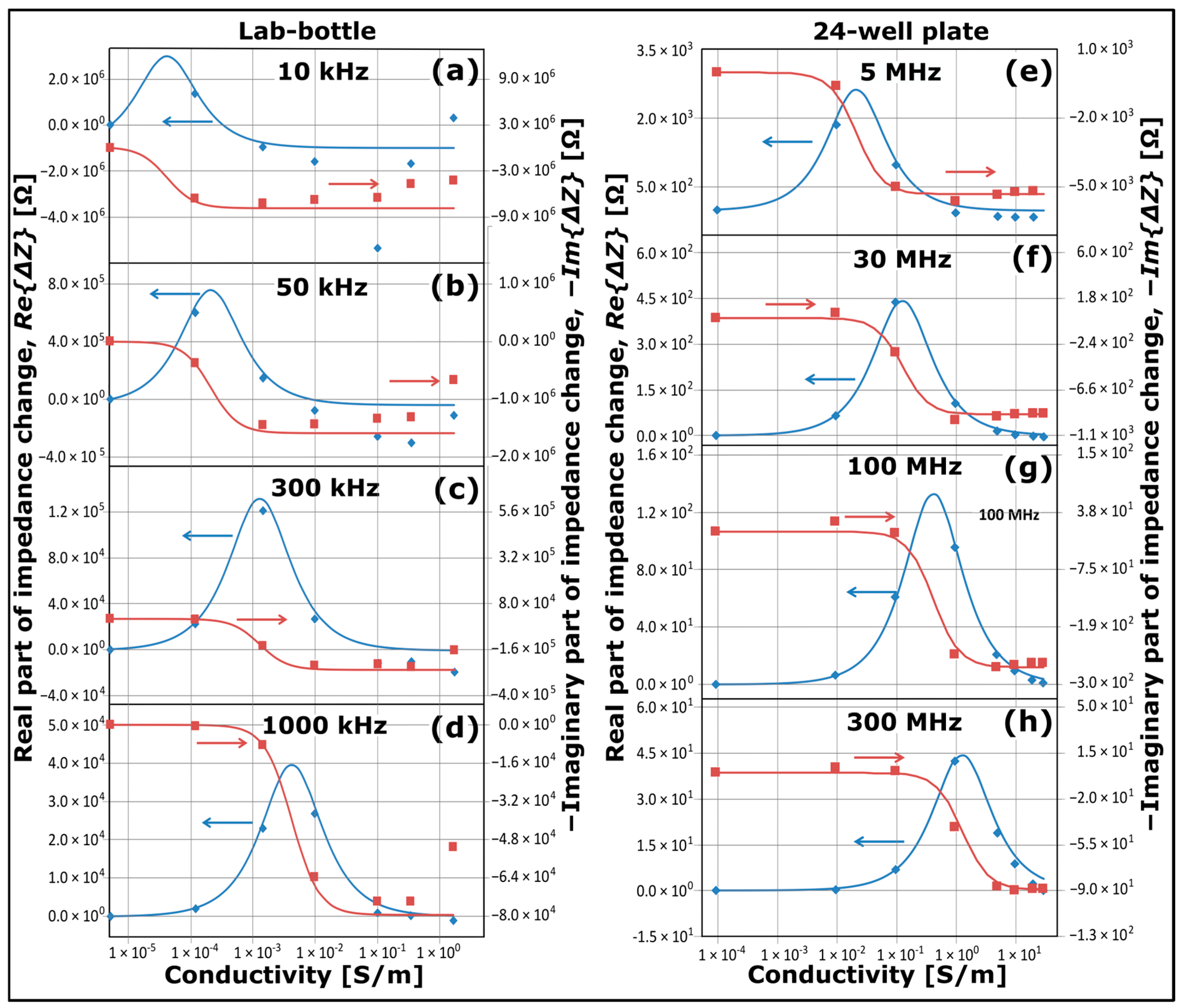
3.1. Measurements on Pure Water and KCl Solutions
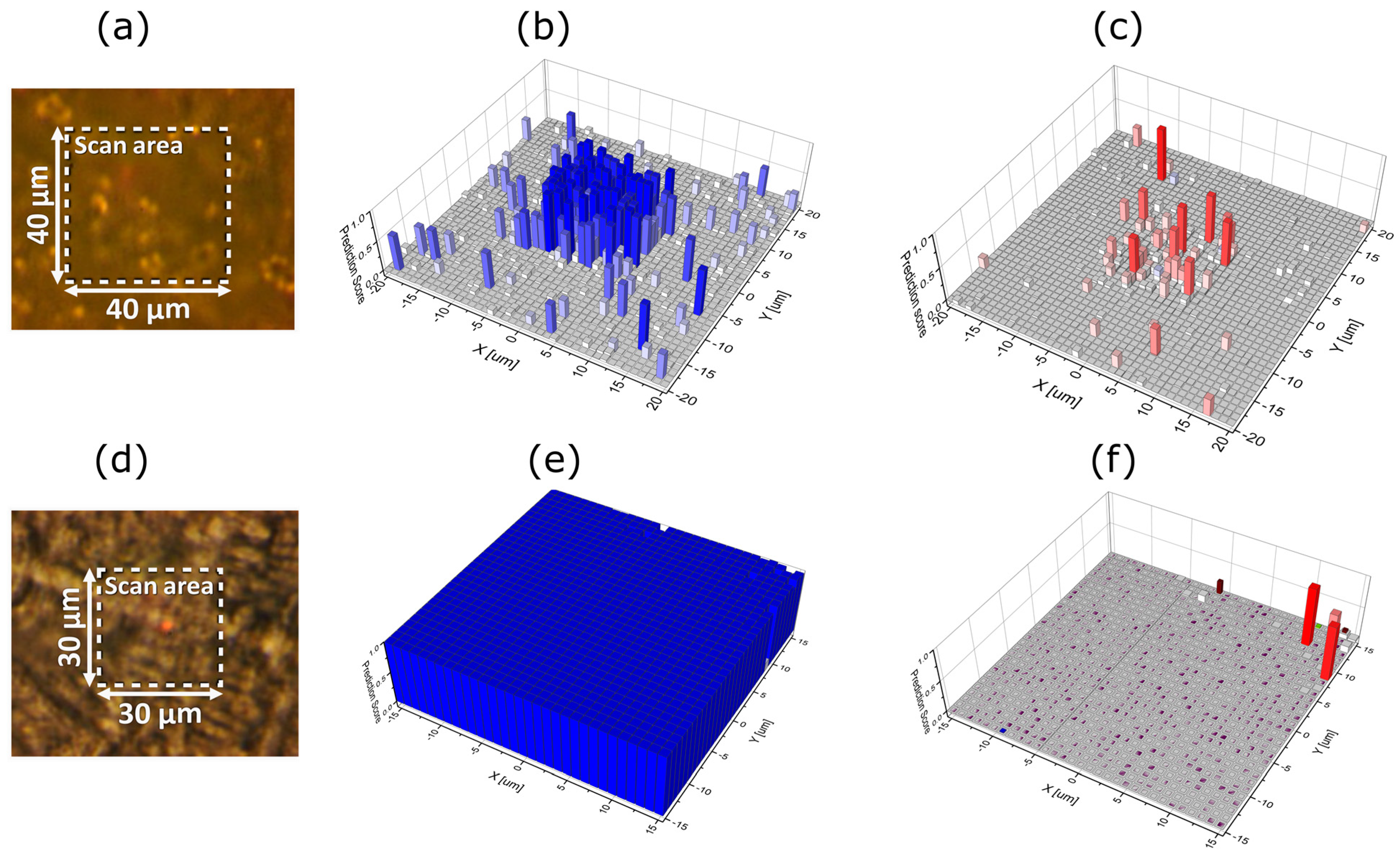
3.2. Measurements of Growth of Bacteria
3.3. Future Directions
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Barsoukov, E.; Macdonald, J. Impedance Spectroscopy: Theory, Experiment, and Applications, 2nd ed.; John Wiley & Sons: Hoboken, NJ, USA, 2005; pp. 1–537. [Google Scholar]
- Kanoun, O.; Kallel, A.Y.; Nouri, H.; Atitallah, B.B.; Haddad, D.; Hu, Z.; Talbi, M.; Al-Hamry, A.; Munjal, R.; Wendler, R.; et al. Impedance Spectroscopy: Applications, Advances and Future Trends. IEEE Instrum. Meas. Mag. 2023, 25, 11–21. [Google Scholar] [CrossRef]
- Weber, C.; Tahedl, M.; Kanoun, O. Modeling for improved performance of non-contacting capacitive sensors for detecting aqueous solutions. In Impedance Spectroscopy: Advanced Applications: Battery Research, Bioimpedance, System Design; Kanoun, O., Ed.; De Gruyter: Berlin, Gemany; Boston, MA, USA, 2019; pp. 107–124. [Google Scholar]
- Zhang, X.; Jiang, X.; Yang, Q.; Wang, X.; Zhao, J.; Qu, K.; Zhao, C. Online Monitoring of Bacterial Growth with Electrical Sensor. Anal. Chem. 2018, 90, 6006–6011. [Google Scholar] [CrossRef] [PubMed]
- Flores-Cosío, G.; Herrera-López, E.; Arellano-Plaza, M.; Gschaedler-Mathis, A.; Kirchmayr, M.; Amaya-Delgado, L. Application of Dielectric Spectroscopy to Unravel the Physiological State of Microorganisms: Current State, Prospects and Limits. Appl. Microbiol. Biotechnol. 2020, 104, 6101–6113. [Google Scholar] [CrossRef]
- Buscaglia, L.A.; Oliveira, O.N.; Carmo, J.P. Roadmap for Electrical Impedance Spectroscopy for Sensing: A Tutorial. IEEE Sens. J. 2021, 21, 22246–22257. [Google Scholar] [CrossRef]
- Castanet, S.; Pasquier, A.; Dinh, T.-H.N.; Boukharouba, H.; Serfaty, S.; Joubert, P.-Y. Non-Contact Fruit Ripening Monitoring Using a Radiofrequency Passive Resonator. Sens. Actuator A Phys. 2022, 347, 113902. [Google Scholar] [CrossRef]
- Kumar, S.; Nguyen, A.T.; Goswami, S.; Ferracane, J.; Koley, D. Real-Time Monitoring of Biofilm Formation Using a Noninvasive Impedance-Based Method. Sens. Actuator Chem. B 2023, 376, 133034. [Google Scholar] [CrossRef]
- Ameer, S.; Ibrahim, H.; Yaseen, M.U.; Kulsoom, F.; Cinti, S.; Sher, M. Electrochemical Impedance Spectroscopy-Based Sensing of Biofilms: A Comprehensive Review. Biosensors 2023, 13, 777. [Google Scholar] [CrossRef]
- Stupin, D.D.; Kuzina, E.A.; Abelit, A.A.; Emelyanov, A.K.; Nikolaev, D.M.; Ryazantsev, M.N.; Koniakhin, S.V.; Dubina, M.V. Bioimpedance Spectroscopy: Basics and Applications. ACS Biomater. Sci. Eng. 2021, 7, 1962–1986. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Z.; Huang, X.; Liu, K.; Lan, T.; Wang, Z.; Zhen, Z. Recent Advances in Electrical Impedance Sensing Technology for Single-Cell Analysis. Biosensors 2021, 11, 470. [Google Scholar] [CrossRef]
- Swami, P.; Anand, S.; Holani, A.; Gupta, S. Impedance Spectroscopy for Bacterial Cell Monitoring, Analysis, and Antibiotic Susceptibility Testing. Langmuir 2024, 40, 21907–21930. [Google Scholar] [CrossRef]
- Simić, M.; Kojić, T.; Radovanović, M.; Stojanović, G.M.; Al-Salami, H. Impedance Spectroscopy Analysis of the Interdigitated Flexible Sensor for Bacteria Detection. IEEE Sens. J. 2020, 20, 12791–12798. [Google Scholar] [CrossRef]
- Magar, H.S.; Hassan, R.Y.A.; Mulchandani, A. Electrochemical Impedance Spectroscopy (EIS): Principles, Construction, and Biosensing Applications. Sensors 2021, 21, 6578. [Google Scholar] [CrossRef] [PubMed]
- Song, J.; Li, Y.; Yin, F.; Zhang, Z.; Ke, D.; Wang, D.; Yuan, Q.; Zhang, X.-E. Enhanced Electrochemical Impedance Spectroscopy Analysis of Microbial Biofilms on an Electrochemically In Situ Generated Graphene Interface. ACS Sens. 2020, 5, 1795–1803. [Google Scholar] [CrossRef]
- Couniot, N.; Afzalian, A.; van Overstraeten-Schlögel, N.; Francis, L.A.; Flandre, D. Capacitive Biosensing of Bacterial Cells: Sensitivity Optimization. IEEE Sens. J. 2016, 16, 586–595. [Google Scholar] [CrossRef]
- de Araujo, A.L.A.; Claudel, J.; Kourtiche, D.; Nadi, M. Use of an Insulation Layer on the Connection Tracks of a Biosensor with Coplanar Electrodes to Increase the Normalized Impedance Variation. Biosensors 2019, 9, 108. [Google Scholar] [CrossRef]
- Yuskina, E.A.; Panchuk, V.V.; Kirsanov, D.O. Evolution of Contactless Conductometry Methods. J. Anal. Chem. 2024, 79, 654–662. [Google Scholar] [CrossRef]
- Hinkelmann, H. Ein Verfahren Zur Elektrodenlosen Messung Der Elektrischen Leitfähigkeit Von Elektrolyten. Z. Angew. Phys. 1958, 10, 500–503. [Google Scholar]
- Niksan, O.; Fowler, J.D.; Balasubramanian, V.; Shah, A.; Pakpour, S.; Zarifi, M.H. A Waveguide Resonator Sensor for Bacterial Growth Monitoring: Towards Antibiotic Susceptibility Testing. In Proceedings of the 2023 IEEE/MTT-S International Microwave Symposium—IMS 2023, San Diego, CA, USA, 11–16 June 2023; pp. 847–850. [Google Scholar]
- Narang, R.; Mohammadi, S.; Ashani, M.M.; Sadabadi, H.; Hejazi, H.; Zarifi, M.H.; Sanati-Nezhad, A. Sensitive, Real-Time and Non-Intrusive Detection of Concentration and Growth of Pathogenic Bacteria Using Microfluidic-Microwave Ring Resonator Biosensor. Sci. Rep. 2018, 8, 15807. [Google Scholar] [CrossRef]
- Turick, C.E.; Colon-Mercado, H.; Bagwell, C.E.; Greenway, S.D.; Amoroso, J.W. Non-Contact Electrochemical Evaluation of Biofilms. SN Appl. Sci. 2020, 2, 389. [Google Scholar] [CrossRef]
- Gasser, A.; Eveness, J.; Kiely, J.; Attwood, D.; Luxton, R. A Non-Contact Impedimetric Biosensing System for Classification of Toxins Associated with Cytotoxicity Testing. Bioelectrochemistry 2020, 133, 107448. [Google Scholar] [CrossRef]
- Kubáň, P.; Hauser, P.C. 20th Anniversary of Axial Capacitively Coupled Contactless Conductivity Detection in Capillary Electrophoresis. Trends Anal. Chem. 2018, 102, 311–321. [Google Scholar] [CrossRef]
- Funari, R.; Shen, A.Q. Detection and Characterization of Bacterial Biofilms and Biofilm-Based Sensors. ACS Sens. 2022, 7, 347–357. [Google Scholar] [CrossRef] [PubMed]
- Thirstrup, C.; Snedden, A.; Jensen, H.D. Bridging the Gap Between Ultrapure Water and Reference Materials in Electrolytic Conductivity Measurements. IEEE Trans. Instrum. Meas. 2017, 66, 2745–2754. [Google Scholar] [CrossRef]
- Jensen, H.D.; Thirstrup, C. Direct Traceability for Ultra-Pure Water Conductivity. NCLI Meas. J. Meas. Sci. 2014, 9, 68–72. [Google Scholar] [CrossRef]
- Seitz, S.; Manzin, A.; Jensen, H.D.; Jakobsen, P.; Spitzer, P. Traceability of Electrolytic Conductivity Measurements to the International System of Units in the Sub mSm−1 Region and Review of Models of Electrolytic Conductivity Cells. Electrochim. Acta 2010, 55, 6323–6331. [Google Scholar] [CrossRef]
- Ho, C.-S.; Jean, N.; Hogan, C.A.; Blackmon, L.; Jeffrey, S.S.; Holodniy, M.; Banaei, N.; Saleh, A.A.; Ermon, S.; Dionne, J. Rapid Identification of Pathogenic Bacteria Using Raman Spectroscopy and Deep Learning. Nat. Commun. 2019, 10, 4927. [Google Scholar] [CrossRef]
- Lundquist-Thomsen, B.; Christensen, J.B.; Rodenko, O.; Usenov, I.; Grønnemose, R.B.; Andersen, T.E.; Lassen, M. Accurate and Fast Identification of Minimally Prepared Bacteria Phenotypes Using Raman Spectroscopy Assisted by Machine Learning. Sci. Rep. 2022, 12, 16436. [Google Scholar]
- Ilchenko, O.; Pilhun, Y.; Kutsyk, A.; Slobodianiuk, D.; Goksel, Y.; Dumont, E.; Vaut, L.; Mazzoni, C.; Morelli, L.; Boisen, S.; et al. Optics Miniaturization Strategy for Demanding Raman Spectroscopy Applications. Nat. Commun. 2014, 15, 3049. [Google Scholar] [CrossRef]
- Thirstrup, C.; Deleebeeck, L. Review on Electrolytic Conductivity Sensors. IEEE Trans. Instrum. Meas. 2021, 70, 1008222. [Google Scholar] [CrossRef]
- Mertens, M.; Chavoshi, M.; Reytral-Rieu, O.; Grenier, K.; Schreurs, D. Dielectric Spectroscopy. IEEE Microw. Mag. 2023, 24, 49–62. [Google Scholar] [CrossRef]
- Furst, A.L.; Francis, M.B. Impedance-Based Detection of Bacteria. Chem. Rev. 2019, 119, 700–726. [Google Scholar] [CrossRef] [PubMed]
- Drude, P. Zur Elektronentheorie der Metalle. Ann. Phys. 1900, 306, 566–613. [Google Scholar] [CrossRef]
- Kiwi, M.; Rössler, J. The Drude Model. J. Rev. Bras. Ensino Fis. 2024, 46, e20240199. [Google Scholar] [CrossRef]
- Peleg, M.; Corradini, M.G. Microbial Growth Curves: What the Models Tell Us and What They Cannot. Crit. Rev. Food Sci. Nutr. 2011, 51, 917–945. [Google Scholar] [CrossRef]
- Yamaguchi, T.; Ueno, A. Capacitive-Coupling Impedance Spectroscopy Using a Non-Sinusoidal Oscillator and Discrete-Time Fourier Transform: An Introductory Study. Sensors 2020, 20, 6392. [Google Scholar] [CrossRef] [PubMed]
- Saulnier, J.; Jose, C.; Lagarde, F. Electrochemical Techniques for Label-Free and Early Detection of Growing Microbial Cells. Bioelectrochemistry 2024, 155, 108587. [Google Scholar] [CrossRef]
- Kiani, M.; Tannert, A.; Du, N.; Hübner, U.; Skorupa, I.; Danilo, B.; Zhao, X.; Blaschke, D.; Rebohle, L.; Cherkouk, C.; et al. Towards Bacteria Counting in DI Water of Several Microliters or Growing Suspension Using Impedance Biochips. Biosensors 2020, 10, 82. [Google Scholar] [CrossRef]
- Muñoz-Berbel, X.; Vigues, N.; Cortina-Puig, M.; Escude, R.; Garcia-Aljaro, C.; Mas, J.; Muñoz, F.X. Impedimetric Approach for Monitoring Bacterial Cultures Based on the Changes in the Magnitude of the Interface Capacitance. Anal. Methods 2020, 2, 1036–1042. [Google Scholar] [CrossRef]
- Park, J.; Lee, Y.; Hwang, Y.; Cho, S. Interdigitated and Wave-Shaped Electrode-Based Capacitance Sensor for Monitoring Antibiotic Effects. Sensors 2020, 20, 5237. [Google Scholar] [CrossRef]
- Li, J.; Ran, X.; Zhou, M.; Wang, K.; Wan, H.; Wang, Y. Oxidative Stress and Antioxidant Mechanisms of Obligate Anaerobes Involved in Biological Waste Treatment Processes: A Review. Sci. Total Environ. 2022, 838, 156454. [Google Scholar] [CrossRef]
- Spitzer, P.; Fisicaro, P.; Seitz, S. pH and Electrolytic Conductivity as Parameters to Characterize Bioethanol. Accred. Qual. Assur. 2009, 14, 671–676. [Google Scholar] [CrossRef]
- Muñoz-Berbel, X.; Vigués, N.; Jenkins, A.T.A.; Mas, J.; Muñoz, F.J. Impedimetric approach for quantifying low bacteria concentrations based on the changes produced in the electrode–solution interface during the pre-attachment stage. Biosens. Bioelectron. 2008, 23, 1540–1546. [Google Scholar] [CrossRef] [PubMed]
- Shukla, A.K.; Boruah, J.S.; Park, S.; Kim, B. Rapid Detection and Counting of Bacteria Using Impact Electrochemical Impedance Spectroscopy. J. Phys. Chem. C 2024, 128, 13458–13463. [Google Scholar] [CrossRef]
- Stilman, W.; Lenzi, M.C.; Wackers, G.; Deschaume, O.; Yongabi, D.; Mathijssen, G.; Bartic, C.; Gruber, J.; Wübbenhorst, M.; Heyndrickx, M.; et al. Low Cost, Sensitive Impedance Detection of E. coli Bacteria in Food-Matrix Samples Using Surface-Imprinted Polymers as Whole-Cell Receptors. Phys. Status Solidi (A) 2022, 219, 2100405. [Google Scholar] [CrossRef]
- Hammond, R.J.H.; Falconer, K.; Powell, T.; Bowness, R.; Gillespie, S.H. A simple label-free method reveals bacterial growth dynamics and antibiotic action in real-time. Sci. Rep. 2022, 12, 19393. [Google Scholar] [CrossRef] [PubMed]




| Time Parameters in Hours | Symbol | OD | Re{ΔZ} 15 kHz | Re{ΔZ} 300 kHz | Change in OR |
|---|---|---|---|---|---|
| S. epidermidis | |||||
| Model based on individual data fitting | |||||
| Growth time constant | 0.5 | 0.5 | 1.0 | 0.5 | |
| Time lag | 7.2 | 5.6 | 7.2 | 5.1 | |
| Unified model as depicted in Figure 7 | |||||
| Growth time constant | - | 1.0 | - | ||
| Time lag for growth from the walls | - | 5.6 | - | ||
| Time lag for growth in the bulk | - | 7.2 | - | ||
| E. coli | |||||
| Model based on individual data fitting | |||||
| Growth time constant | 0.5 | 0.5 | 1.0 | 0.5 | |
| Time lag | 6.4 | 3.7 | 5.4 | 4.0 | |
| Unified model as depicted in Figure 7 | |||||
| Growth time constant | - | 1.0 | - | ||
| Time lag for growth from the walls | - | 3.7 | - | ||
| Time lag for growth in the bulk | - | 5.4 | - | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Thirstrup, C.; Nielsen, O.S.; Lassen, M.; Andersen, T.E.; Aslan, H. Non-Invasive Real-Time Monitoring of Bacterial Activity by Non-Contact Impedance Spectroscopy for Off-the-Shelf Labware. Sensors 2025, 25, 2427. https://doi.org/10.3390/s25082427
Thirstrup C, Nielsen OS, Lassen M, Andersen TE, Aslan H. Non-Invasive Real-Time Monitoring of Bacterial Activity by Non-Contact Impedance Spectroscopy for Off-the-Shelf Labware. Sensors. 2025; 25(8):2427. https://doi.org/10.3390/s25082427
Chicago/Turabian StyleThirstrup, Carsten, Ole Stender Nielsen, Mikael Lassen, Thomas Emil Andersen, and Hüsnü Aslan. 2025. "Non-Invasive Real-Time Monitoring of Bacterial Activity by Non-Contact Impedance Spectroscopy for Off-the-Shelf Labware" Sensors 25, no. 8: 2427. https://doi.org/10.3390/s25082427
APA StyleThirstrup, C., Nielsen, O. S., Lassen, M., Andersen, T. E., & Aslan, H. (2025). Non-Invasive Real-Time Monitoring of Bacterial Activity by Non-Contact Impedance Spectroscopy for Off-the-Shelf Labware. Sensors, 25(8), 2427. https://doi.org/10.3390/s25082427








