Double-Cavity Fabry–Perot Interferometer Sensor Based on Polymer-Filled Hollow Core Fiber for Simultaneous Measurement of Temperature and Gas Pressure
Abstract
1. Introduction
2. Fabrication and Principle
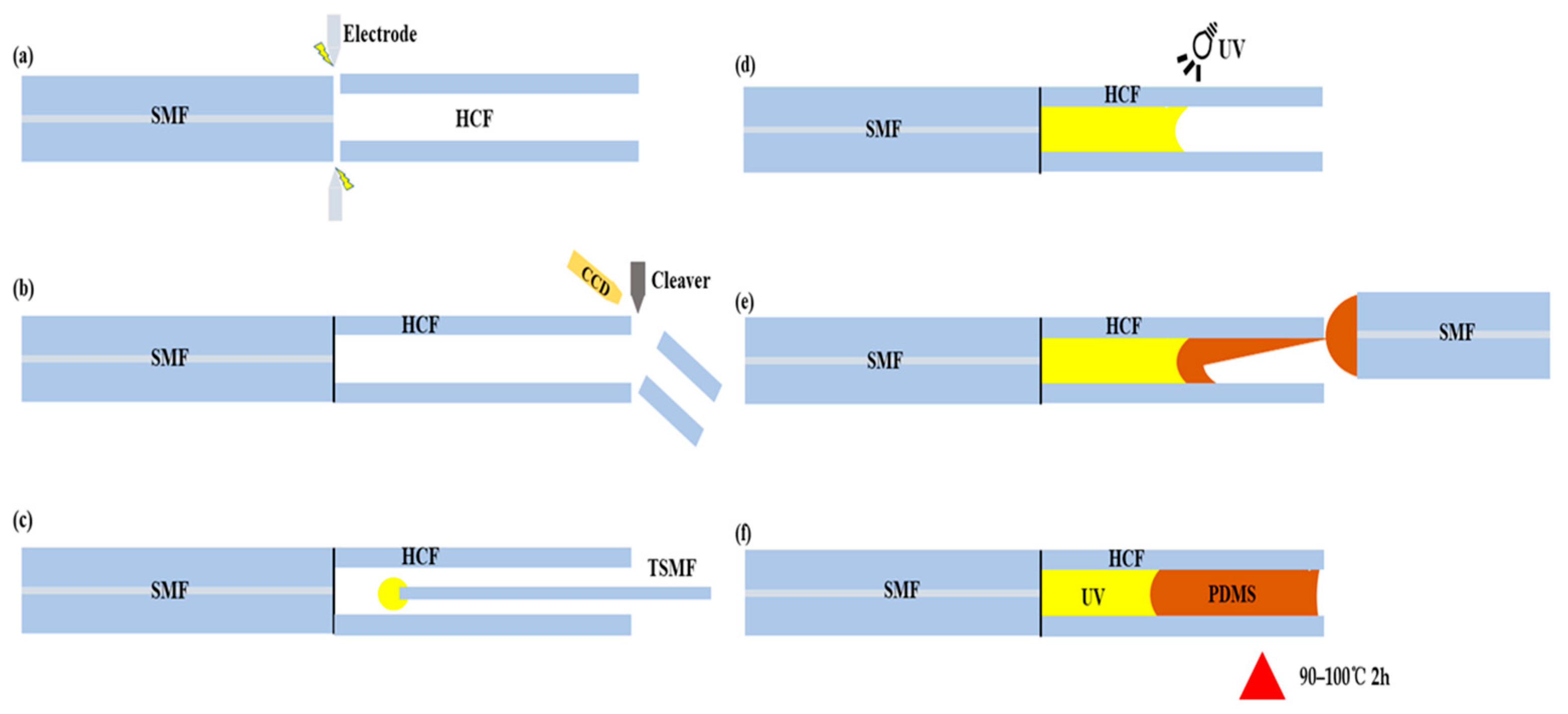
2.1. Sensor Fabrication
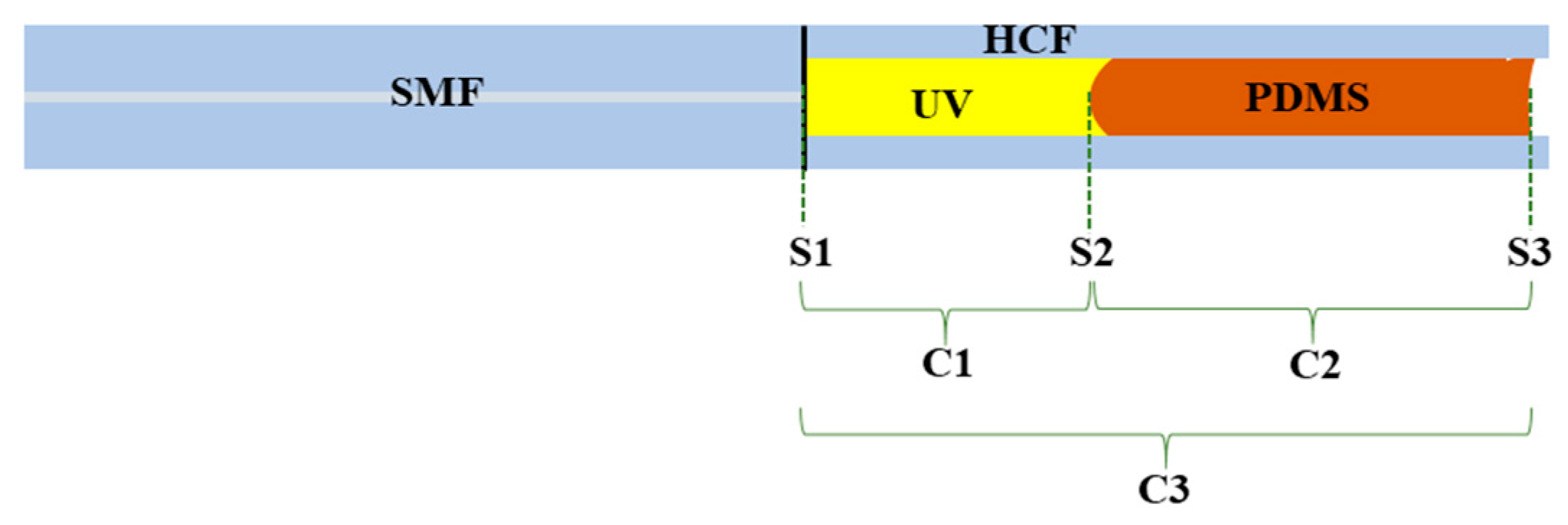
2.2. Sensor Principle
3. Experimental Setup
4. Results and Discussion
4.1. Relationship Between Sensor Cavity Length and Sensitivity
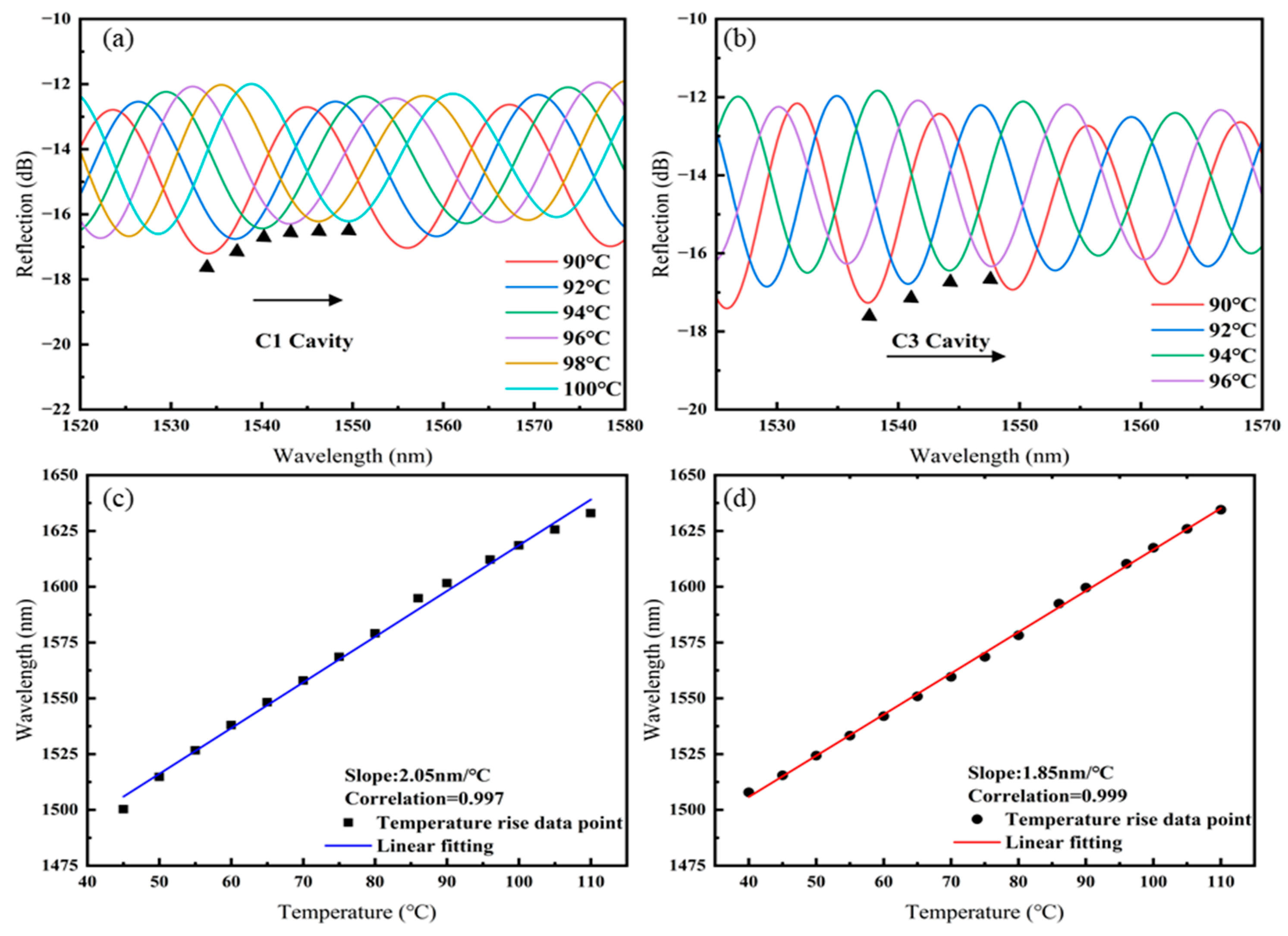
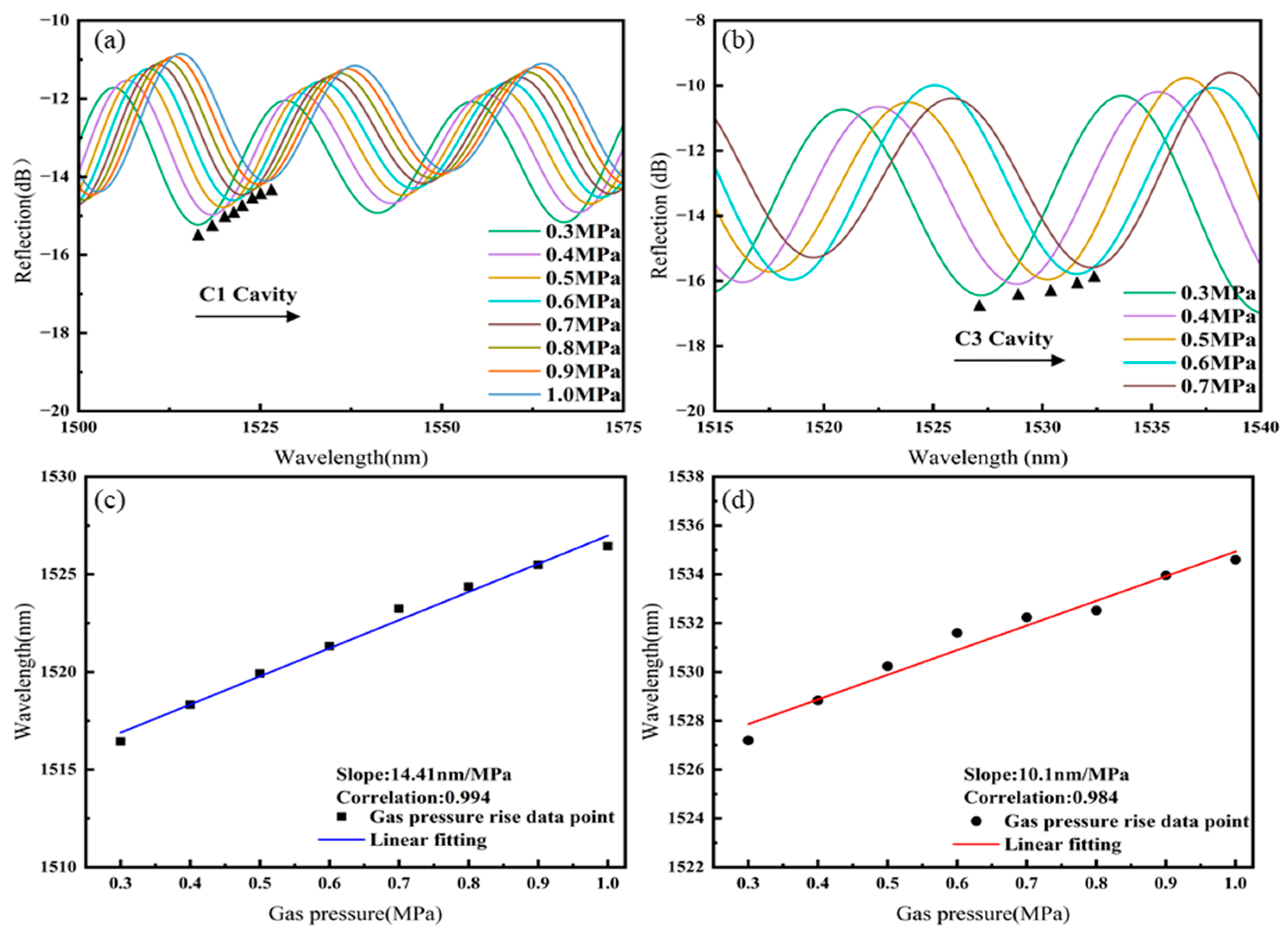
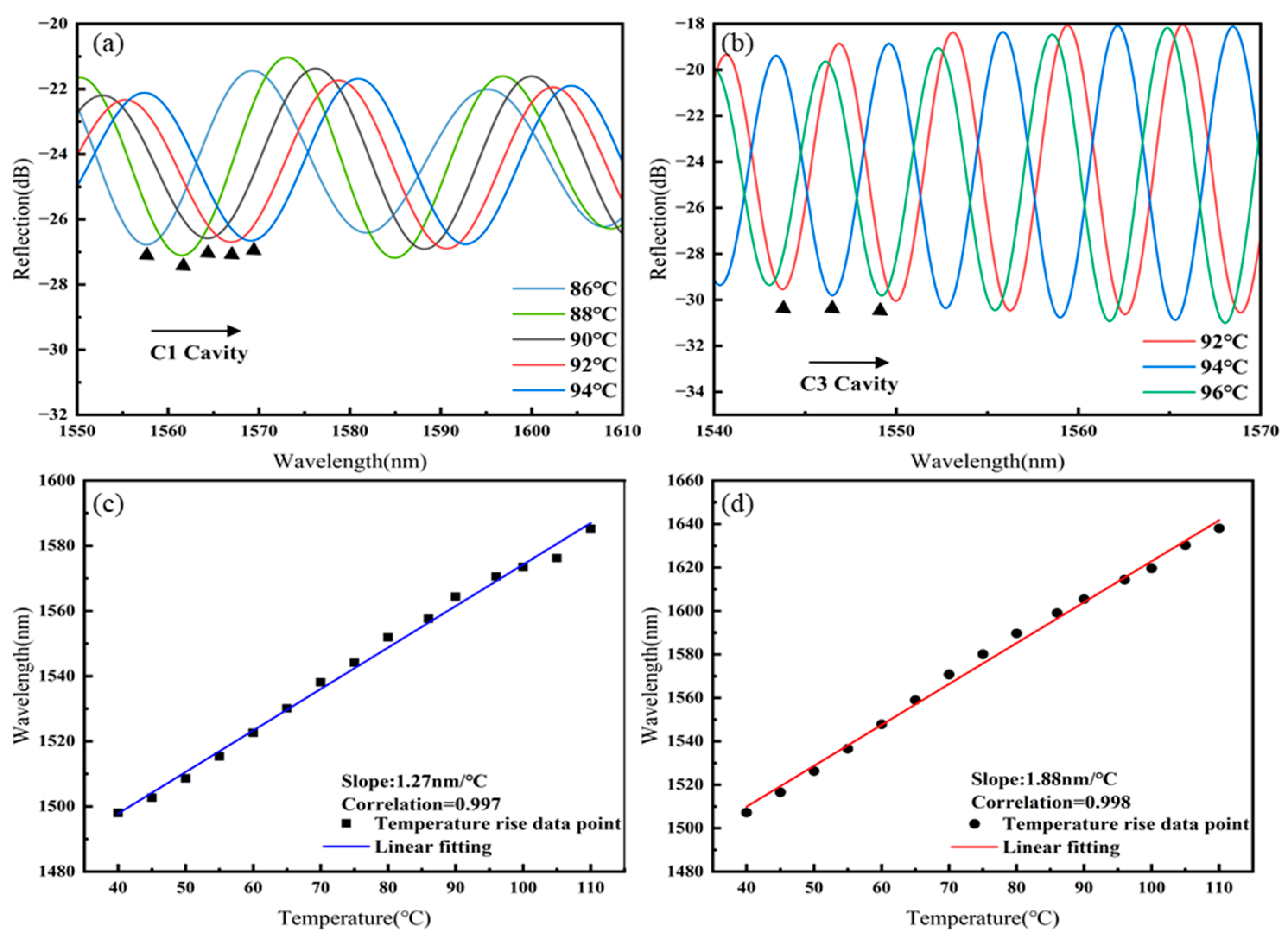
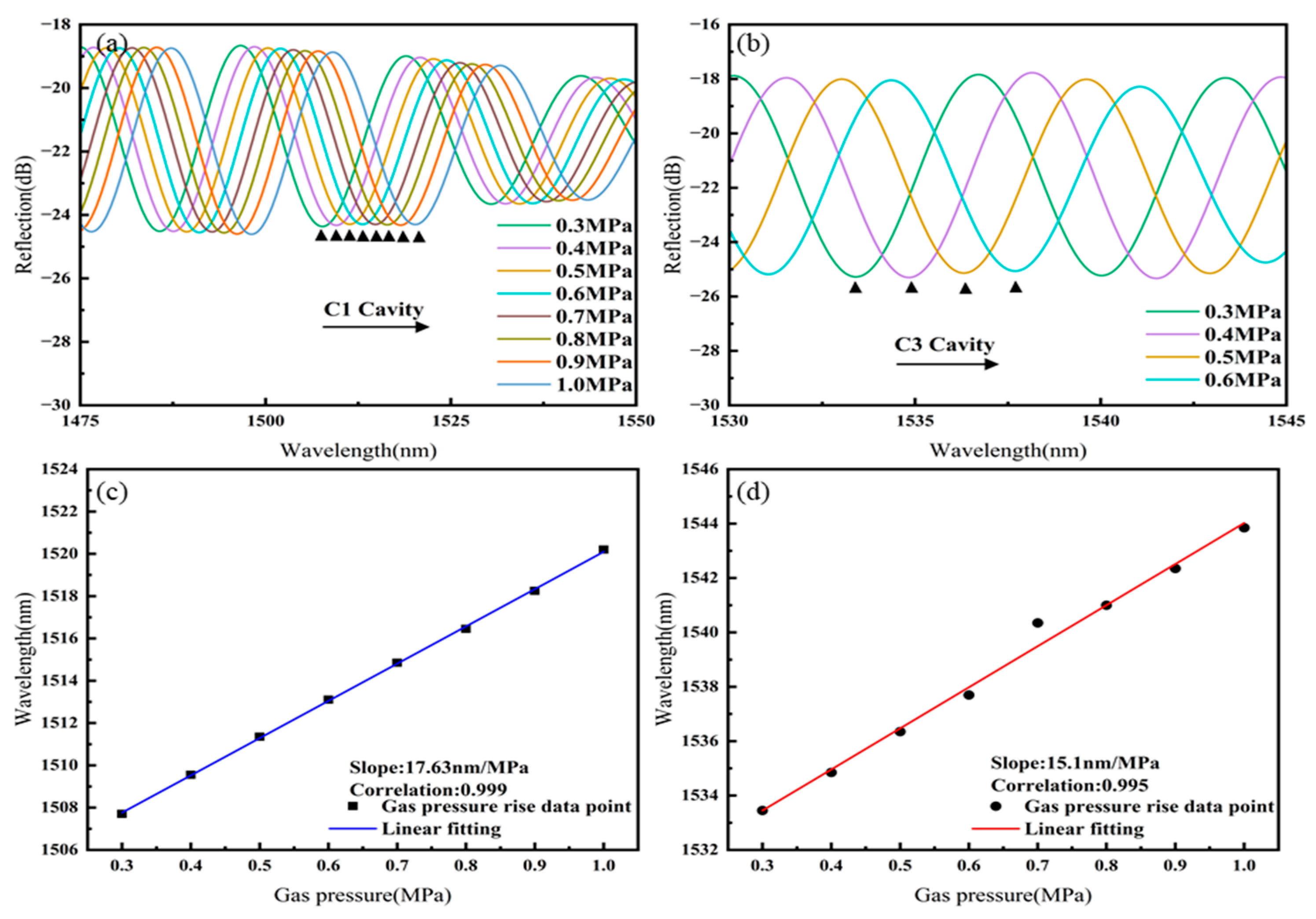
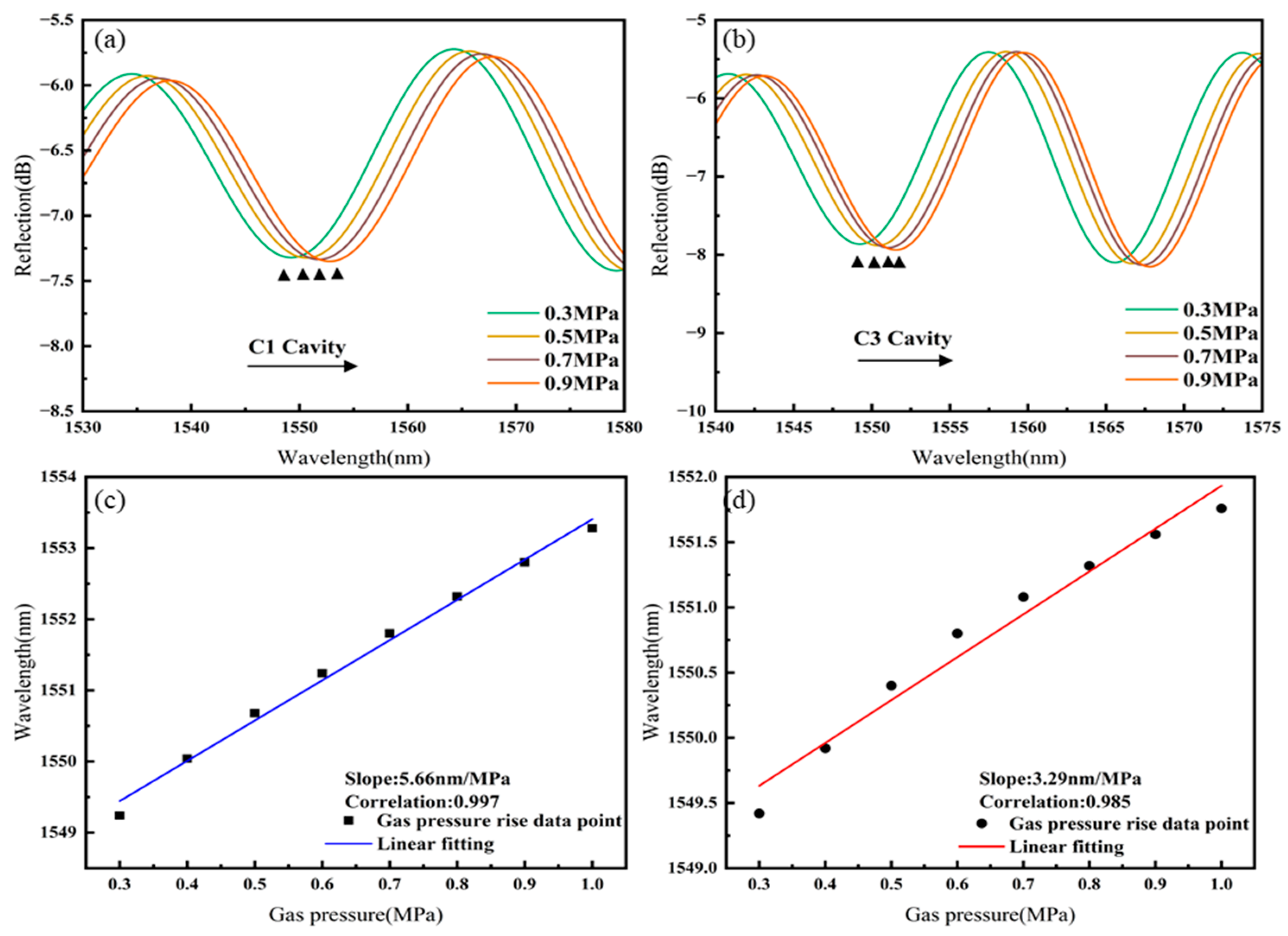
4.1.1. Sensor 1 Temperature and Gas Pressure Responses
4.1.2. Sensor 2 Temperature and Gas Pressure Responses
4.1.3. Sensor 3 Temperature and Gas Pressure Responses
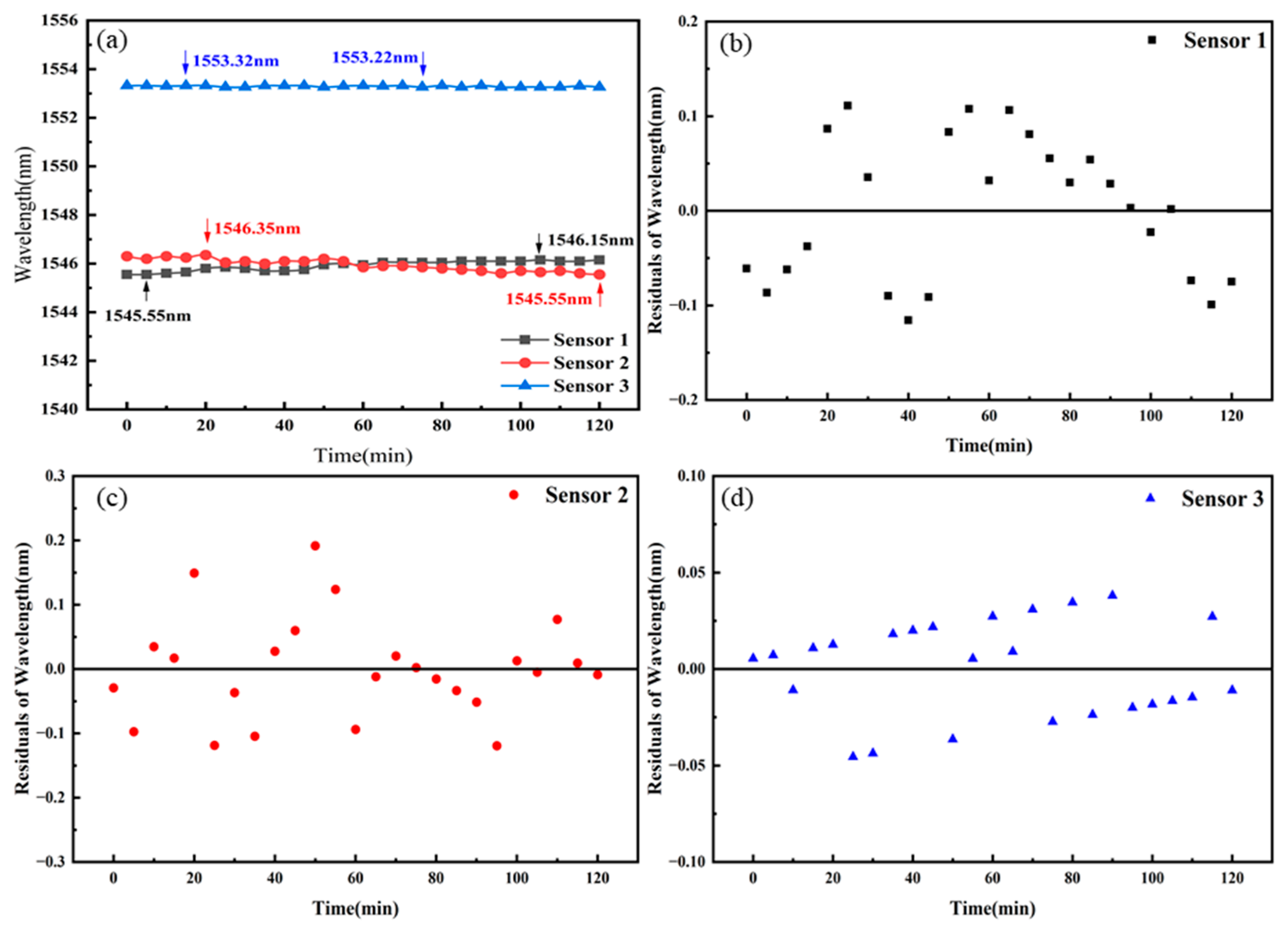
4.2. Stability Testing of Sensors
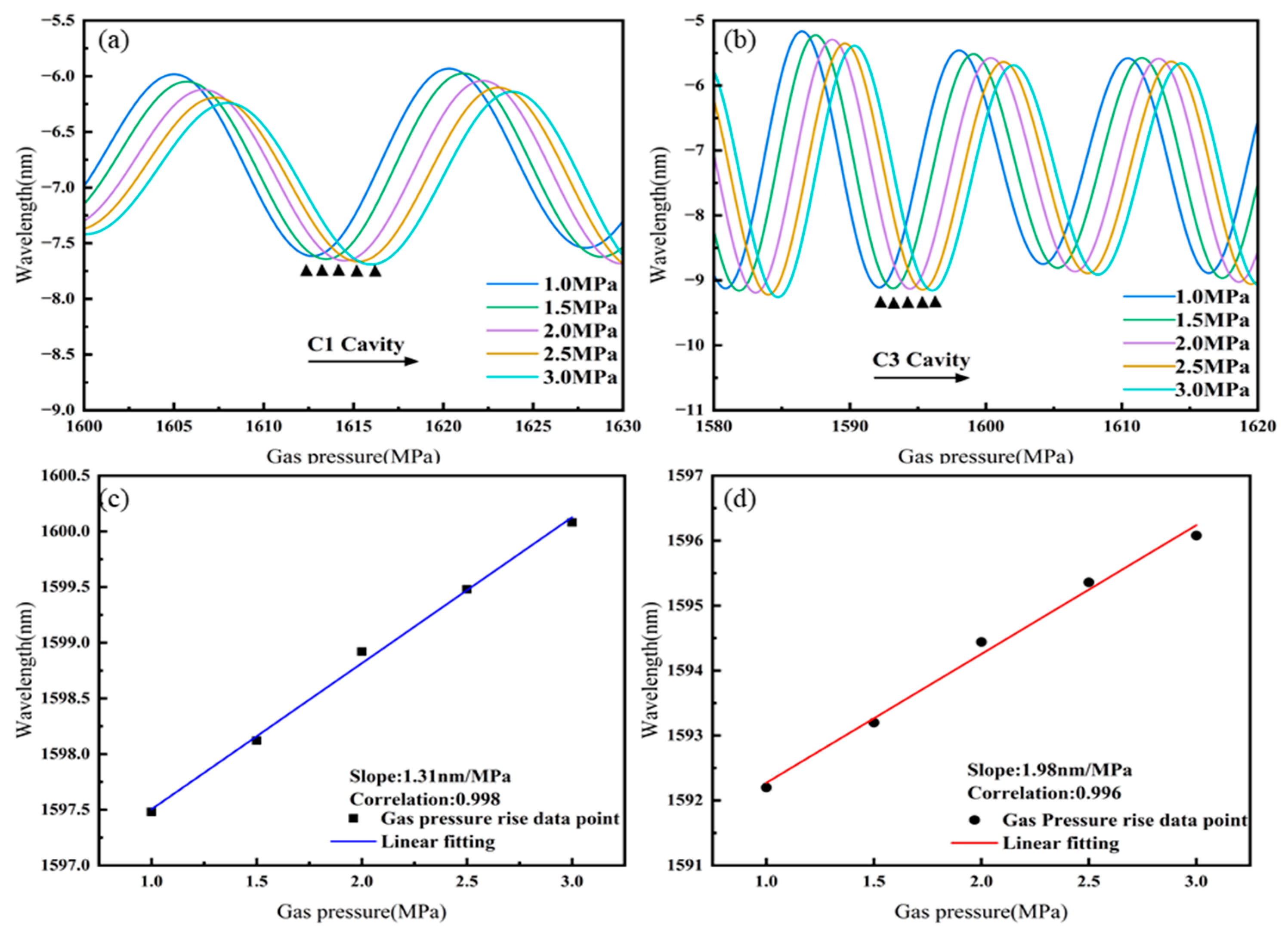
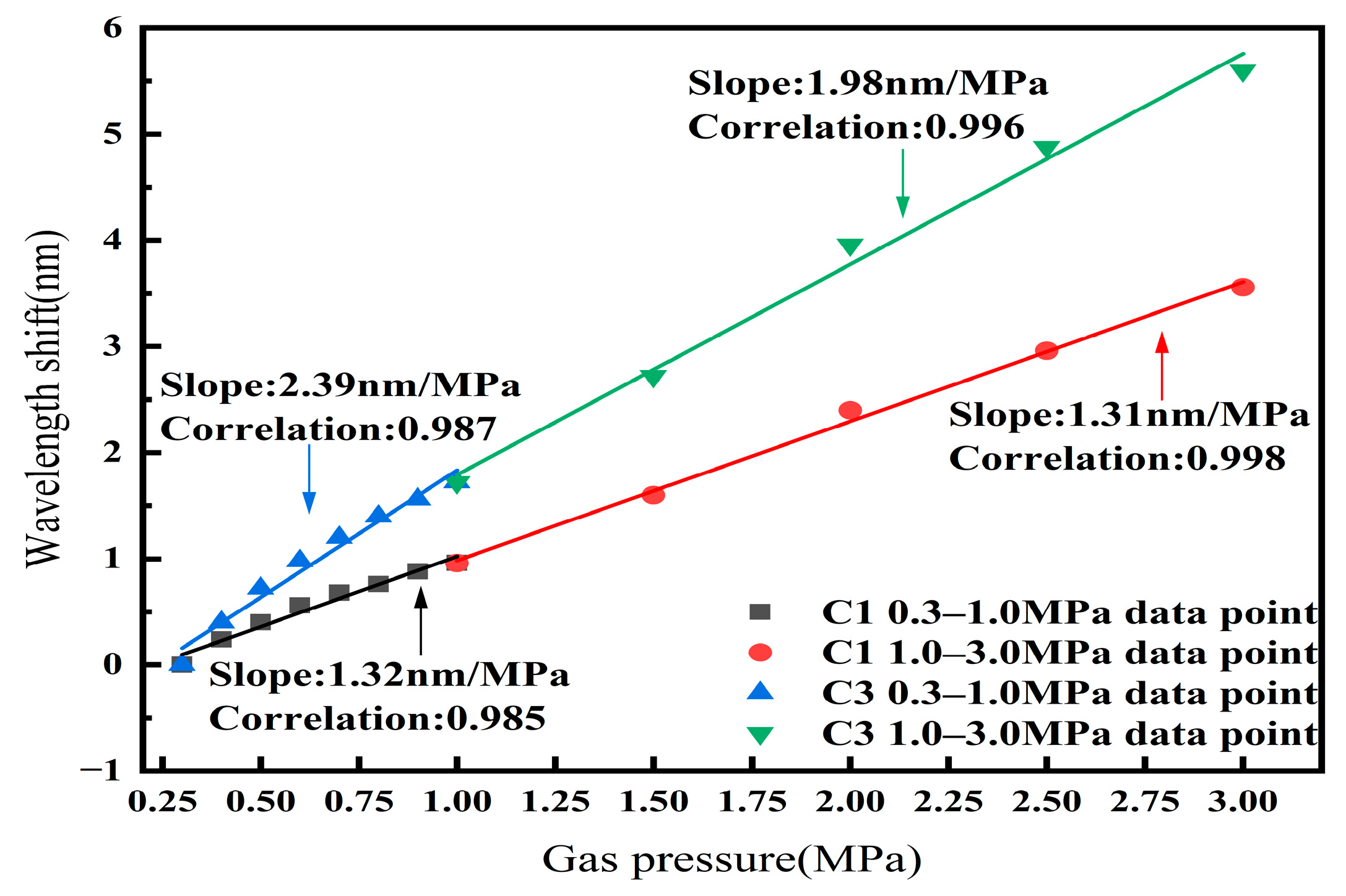
4.3. High-Gas-Pressure Response Test of Sensors
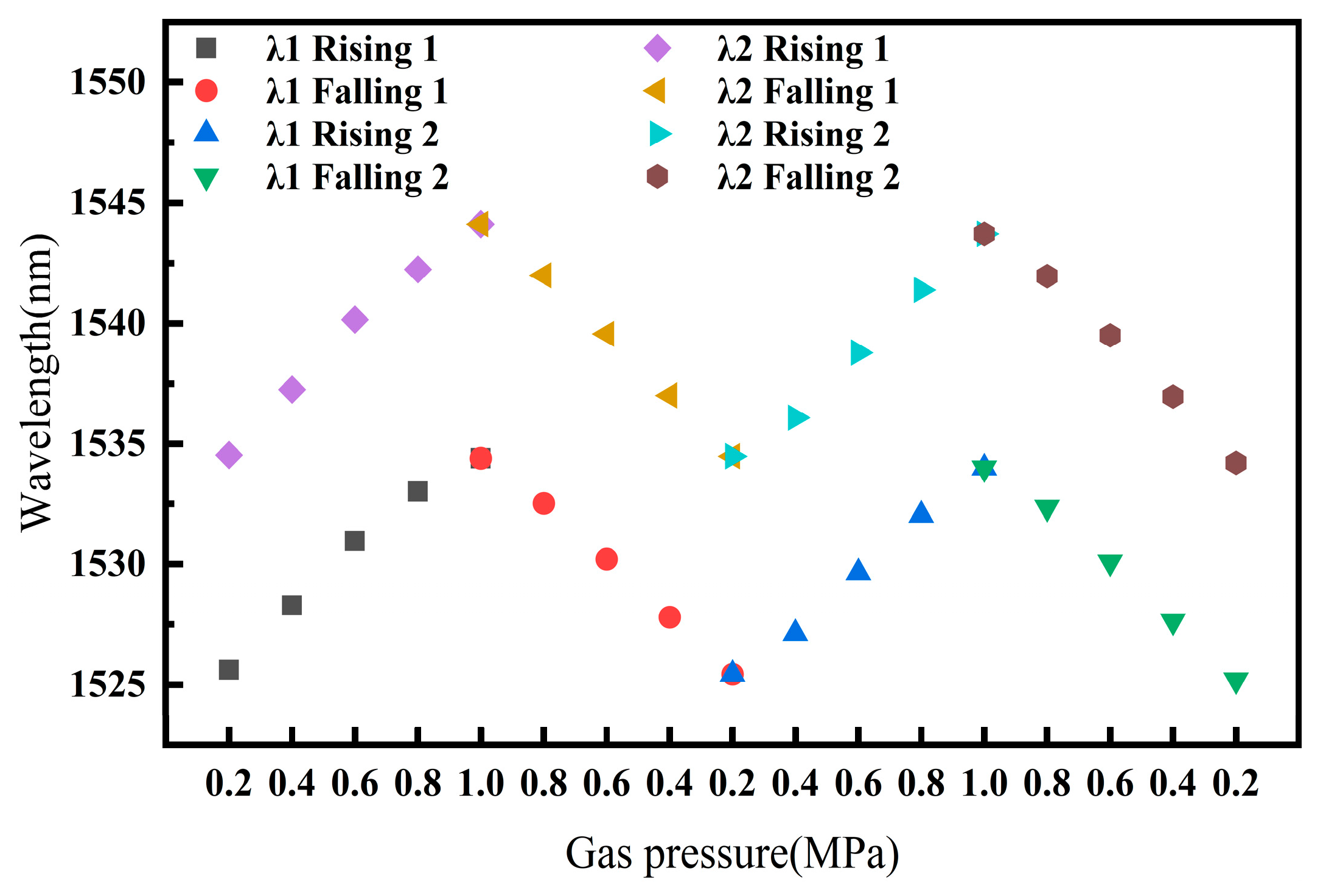
4.4. Repeatability Testing of Sensors
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Xu, B.J.; Chen, G.F.; Xu, X.Z.; Liu, S.; Liao, C.; Weng, X.; Liu, L.; Qu, J.; Wang, Y.; He, J. Highly birefringent side-hole fiber Bragg grating for high-temperature pressure sensing. Opt. Lett. 2024, 49, 1233–1236. [Google Scholar] [CrossRef] [PubMed]
- Fu, X.H.; Zhang, Y.P.; Wang, Y.F.; Fu, G.; Jin, W.; Bi, W. A temperature sensor based on tapered few mode fiber long-period grating induced by CO2 laser and fusion tapering. Opt. Laser Technol. 2020, 121, 105825. [Google Scholar] [CrossRef]
- Zhao, F.; Xiao, D.R.; Lin, W.H.; Chen, Y.; Wang, G.; Hu, J.; Liu, S.; Yu, F.; Xu, W.; Yang, X.; et al. Sensitivity Enhanced Rsefractive Index Sensor with In-Line Fiber Mach-Zehnder Interferometer Based on Double-Peanut and Er-Doped Fiber Taper Structure. J. Light. Technol. 2020, 40, 245–251. [Google Scholar] [CrossRef]
- Zhao, Y.J.; Lin, H.F.; Zhou, C.M.; Deng, H.; Zhou, A.; Yuan, L. Cascaded Mach-Zehnder Interferometers with Vernier Effect for Gas Pressure Sensing. IEEE Photon. Technol. Lett. 2019, 31, 591–594. [Google Scholar] [CrossRef]
- Gao, H.T.; Xu, D.P.; Ye, Y.Y.; Zhang, Y.; Shen, J.; Li, C. Fiber-tip polymer filled probe for high-sensitivity temperature sensing and polymer refractometers. Opt. Express 2022, 30, 8104–8114. [Google Scholar] [CrossRef]
- Tian, P.X.; Guan, C.Y.; Xiong, Z.Y.; Gao, S.; Ye, P.; Yang, J.; He, X.; Shi, J.; Yuan, L. High-sensitivity gas pressure sensor with low temperature cross-talk based on Vernier effect of cascaded Fabry-Perot interferometers. Opt. Fiber Technol. 2023, 75, 103160. [Google Scholar] [CrossRef]
- Zhang, Y.N.; Wang, M.Y.; Zhu, N.S.; Han, B.; Liu, Y. Optical fiber hydrogen sensor based on self-assembled PDMS/Pd-WO3 microbottle resonator. Sens. Actuators B-Chem. 2023, 375, 132866. [Google Scholar] [CrossRef]
- Yang, K.M.; He, J.; Wang, Y.; Liu, S.; Liao, C.; Li, Z.; Yin, G.; Sun, B.; Wang, Y. Ultrasensitive Temperature Sensor Based on a Fiber Fabry-Perot Interferometer Created in a Mercury-Filled Silica Tube. IEEE Photon. J. 2015, 7, 1–9. [Google Scholar] [CrossRef]
- Feng, C.C.; Niu, H.; Wang, H.Y.; Wang, D.; Wei, L.; Ju, T.; Yuan, L. Probe-Type Multi-Core Fiber Optic Sensor for Simultaneous Measurement of Seawater Salinity, Pressure, and Temperature. Sensors 2024, 24, 1766. [Google Scholar] [CrossRef]
- Fu, D.Y.; Liu, X.J.; Shang, J.Y.; Sun, W.M.; Liu, Y.J. A Simple, Highly Sensitive Fiber Sensor for Simultaneous Measurement of Pressure and Temperature. IEEE Photon. Technol. Lett. 2020, 32, 747–750. [Google Scholar] [CrossRef]
- Liu, Y.G.; Huang, L.; Dong, J.F.; Li, B.; Song, X. High sensitivity fiber-optic temperature sensor based on PDMS glue-filled capillary. Opt. Fiber Technol. 2021, 67, 102699. [Google Scholar] [CrossRef]
- Deng, L.F.; Jiang, C.; Hu, C.J.; Li, L.; Gao, J.; Li, H.; Sun, S.; Liu, C.; Shu, Y. Highly Sensitive Temperature and Gas Pressure Sensor Based on Long-Period Fiber Grating Inscribed in Tapered Two-Mode Fiber and PDMS. IEEE Sens. J. 2023, 23, 15578–15585. [Google Scholar] [CrossRef]
- Zhou, D.; Sun, J.; Wang, T.; Zhang, S.; Zhang, W.; Zhou, N.; Liu, Y. Highly Sensitive Miniature Optical Fiber Liquid Crystal Sensor for Tumor Temperature Detection. IEEE Sens. J. 2024, 24, 32119–32126. [Google Scholar] [CrossRef]
- Zheng, Y.W.; Chen, Y.Z.; Zhang, Q.F.; Tang, Q.; Zhu, Y.; Yu, Y.; Du, C.; Ruan, S. High-Sensitivity Temperature Sensor Based on Fiber Fabry-Pérot Interferometer with UV Glue-Filled Hollow Capillary Fiber. Sensors 2023, 23, 7687. [Google Scholar] [CrossRef]
- Zhang, T.; Liu, Y.G.; Yang, D.Q.; Wang, Y.; Fu, H.; Jia, Z.; Gao, H. Constructed fiber-optic FPI-based multi-parameters sensor for simultaneous measurement of pressure and temperature, refractive index and temperature. Opt. Fiber Technol. 2019, 49, 64–70. [Google Scholar] [CrossRef]
- Zhang, Y.X.; Liu, P.L.; Yu, J.J.; Ye, L.; Tang, X.; Zhang, Y.; Liu, Z.; Yuan, L. Highly humidity sensitive Fabry-Perot interferometer sensor based on a liquid-solid microcavity for breath monitoring. Opt. Fiber Technol. 2024, 84, 103761. [Google Scholar] [CrossRef]
- Wu, S.N.; Yan, G.F.; Wang, C.L.; Lian, Z.; Chen, X.; He, S. FBG Incorporated Side-Open Fabry–Perot Cavity for Simultaneous Gas Pressure and Temperature Measurements. J. Light. Technol. 2016, 34, 3761–3767. [Google Scholar] [CrossRef]
- Han, Z.; Xin, G.G.; Nan, P.Y.; Liu, J.; Zhu, J.; Yang, H. Hypersensitive high-temperature gas pressure sensor with Vernier effect by two parallel Fabry-Perot interferometers. Optik 2021, 241, 166956. [Google Scholar] [CrossRef]
- Lang, C.P.; Liu, Y.; Liao, Y.Y.; Li, J.; Qu, S. Ultra-Sensitive Fiber-Optic Temperature Sensor Consisting of Cascaded Liquid-Air Cavities Based on Vernier Effect. IEEE Sens. J. 2020, 20, 5286–5291. [Google Scholar] [CrossRef]
- Zhang, H.Z.; Jiang, Q. Highly Sensitive Air Pressure Sensor Based on Fabry-Perot Interference. IEEE Sens. J. 2022, 22, 6637–6643. [Google Scholar] [CrossRef]
- Shu, Y.K.; Jiang, C.; Hu, C.J.; Deng, L.; Li, L.; Gao, J.; Huang, H. Ultra-sensitive air pressure sensor based on gelatin diaphragm-based Fabry-Peroy interferometers and Vernier effect. Opt. Commun. 2024, 557, 130337. [Google Scholar] [CrossRef]
- Zhang, X.F. Research on Optical Fiber Fabry-Perot Temperature Sensor Based on Capillary Filled PDMS. Master’s Thesis, Tianjin University, Tianjin, China, 2021. [Google Scholar]
- Wei, X.Y.; Song, X.K.; Li, C.; Hou, L.; Li, Z.; Li, Y.; Ran, L. Optical Fiber Gas Pressure Sensor Based on Polydimethylsiloxane Microcavity. J. Light. Technol. 2021, 39, 2988–2993. [Google Scholar] [CrossRef]
- Liu, Z.H.; Zhao, B.C.; Zhang, Y.; Zhang, Y.; Sha, C.; Yang, J.; Yuan, L. Optical fiber temperature sensor based on Fabry-Perot interferometer with photopolymer material. Sens. Actuators A-Phys. 2022, 347, 113894. [Google Scholar] [CrossRef]
- Li, Z.Y.; Liao, C.R.; Wang, Y.P.; Xu, L.; Wang, D.; Dong, X.; Liu, S.; Wang, Q.; Yang, K.; Zhou, J. Highly-sensitive gas pressure sensor using twin-core fiber based in-line Mach-Zehnder interferometer. Opt. Express 2015, 23, 6673–6678. [Google Scholar] [CrossRef] [PubMed]
- Liu, Y.G.; Zhang, T.; Wang, Y.X.; Yang, D.; Liu, X.; Fu, H.; Jia, Z. Simultaneous measurement of gas pressure and temperature with integrated optical fiber FPI sensor based on in-fiber micro-cavity and fiber-tip. Opt. Fiber Technol. 2018, 46, 77–82. [Google Scholar] [CrossRef]
- Sun, B.; Wang, Y.P.; Qu, J.L.; Liao, C.; Yin, G.; He, J.; Zhou, J.; Tang, J.; Liu, S.; Li, Z.; et al. Simultaneous measurement of pressure and temperature by employing Fabry-Perot interferometer based on pendant polymer droplet. Opt. Express 2015, 23, 1906–1911. [Google Scholar] [CrossRef]
- Ge, M.; Li, Y.; Han, Y.H.; Xia, Z.; Guo, Z.; Gao, J.; Qu, S. High-sensitivity double-parameter sensor based on the fibre-tip Fabry-Perot interferometer. J. Mod. Opt. 2017, 64, 596–600. [Google Scholar] [CrossRef]





| Sample Number | C1 Length (μm) | C1 Frequency (nm−1) | C2 Length (μm) | C2 Frequency (nm−1) | C3 Length (μm) | C3 Frequency (nm−1) |
|---|---|---|---|---|---|---|
| 1 | 36.0 | 0.044 | 30.7 | 0.036 | 66.7 | 0.080 |
| 2 | 35.3 | 0.043 | 85.2 | 0.099 | 120.5 | 0.143 |
| 3 | 24.6 | 0.030 | 29.4 | 0.034 | 54.0 | 0.065 |
| Sample Number | C1 Length (μm) | C1 Temperature Sensitivity (nm/°C) | C1 Gas Pressure Sensitivity (nm/MPa) | C3 Length (μm) | C3 Temperature Sensitivity (nm/°C) | C3 Gas Pressure Sensitivity (nm/MPa) |
|---|---|---|---|---|---|---|
| 1 | 36.0 | 2.05 | 14.41 | 66.7 | 1.85 | 10.10 |
| 2 | 35.3 | 1.27 | 17.63 | 120.5 | 1.88 | 15.10 |
| 3 | 24.6 | 0.785 | 5.66 | 54.0 | 0.996 | 3.29 |
| Type | Max. Temperature Sensitivity (nm/°C) | Temperature Sensing Range (°C) | Max. Gas Pressure Sensitivity (nm/MPa) | Gas Pressure Sensing Range (MPa) | Reference |
|---|---|---|---|---|---|
| FBG + F-P | 0.748 | 30–70 | 8.45 | 0.1–0.7 | [15] |
| F-P with photopolymer material | −1.18 | 20–110 | _ | _ | [24] |
| LPFG coated with PDMS | −0.484 | 30–60 | −18.26 | 0–0.4 | [12] |
| Twin core fiber with M-Z | 0.043 | 10–100 | 8.45 | 0–2.0 | [25] |
| All-fiber cavity F-P | 0.01083 | 25–300 | 4.1587 | 0–0.8 | [26] |
| Fiber-tip with UV | 0.249 | 40–90 | 1.13 | 0.1–2.5 | [27] |
| Fiber-tip with two materials | 0.68968 | 20–75 | _ | _ | [28] |
| HCF with PDMS film | 0.131 | 20–50 | 52.143 | 0.1–0.7 | [23] |
| Double-cavity with two materials | 2.05 | 40–110 | 17.63 | 0.3–3.0 | This work |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, Y.; Zhang, Y.; Tang, Q.; Li, S.; Zheng, H.; Liang, D.; Xiao, H.; Du, C.; Yu, Y.; Ruan, S. Double-Cavity Fabry–Perot Interferometer Sensor Based on Polymer-Filled Hollow Core Fiber for Simultaneous Measurement of Temperature and Gas Pressure. Sensors 2025, 25, 2396. https://doi.org/10.3390/s25082396
Zhu Y, Zhang Y, Tang Q, Li S, Zheng H, Liang D, Xiao H, Du C, Yu Y, Ruan S. Double-Cavity Fabry–Perot Interferometer Sensor Based on Polymer-Filled Hollow Core Fiber for Simultaneous Measurement of Temperature and Gas Pressure. Sensors. 2025; 25(8):2396. https://doi.org/10.3390/s25082396
Chicago/Turabian StyleZhu, Yixin, Yufeng Zhang, Qianhao Tang, Shengjie Li, Huaijin Zheng, Dezhi Liang, Haibing Xiao, Chenlin Du, Yongqin Yu, and Shuangchen Ruan. 2025. "Double-Cavity Fabry–Perot Interferometer Sensor Based on Polymer-Filled Hollow Core Fiber for Simultaneous Measurement of Temperature and Gas Pressure" Sensors 25, no. 8: 2396. https://doi.org/10.3390/s25082396
APA StyleZhu, Y., Zhang, Y., Tang, Q., Li, S., Zheng, H., Liang, D., Xiao, H., Du, C., Yu, Y., & Ruan, S. (2025). Double-Cavity Fabry–Perot Interferometer Sensor Based on Polymer-Filled Hollow Core Fiber for Simultaneous Measurement of Temperature and Gas Pressure. Sensors, 25(8), 2396. https://doi.org/10.3390/s25082396






