Analysis and Experiments of Resonant Coupling Wireless Power Transfer System for Nonuniform Powering of Multiple Sensors
Abstract
1. Introduction
- (1)
- Nonuniform powering of multiple receivers: considering the different power levels that the IoT sensors demand, we present a simple method for achieving the specified power ratio of the multiple receivers using the equivalent circuit model and reflected impedance technique.
- (2)
- Generalized multi-receiver approach: unlike prior studies focusing on single receivers or requiring complex impedance matching, our approach provides a simple approach of extending the analysis to multiple receivers and achieves relatively high efficiencies.
- (3)
- Experimental validation of a non-invasive tuning method: using a large transmitter and multiple smaller receivers in an asymmetric WPT configuration, our experiments confirm that the desired power ratio can be achieved by adjusting receiver positions, eliminating the need for circuit modifications.
2. Analysis
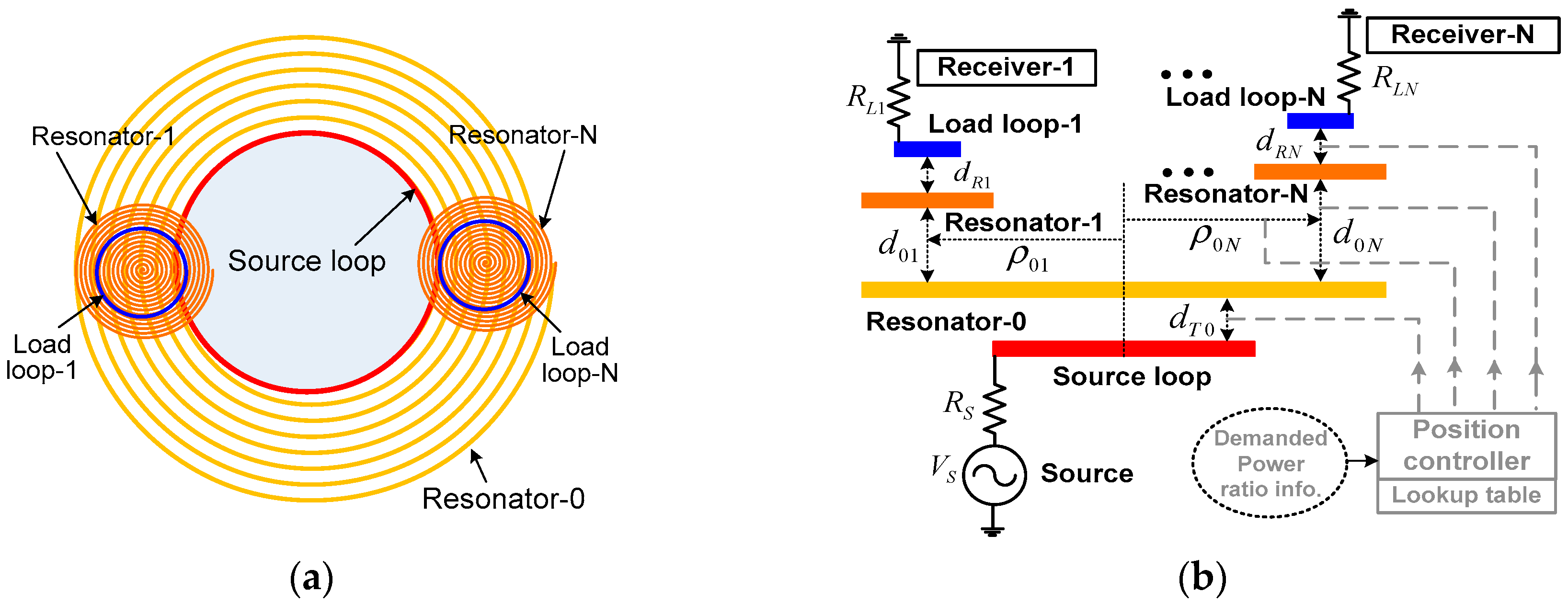
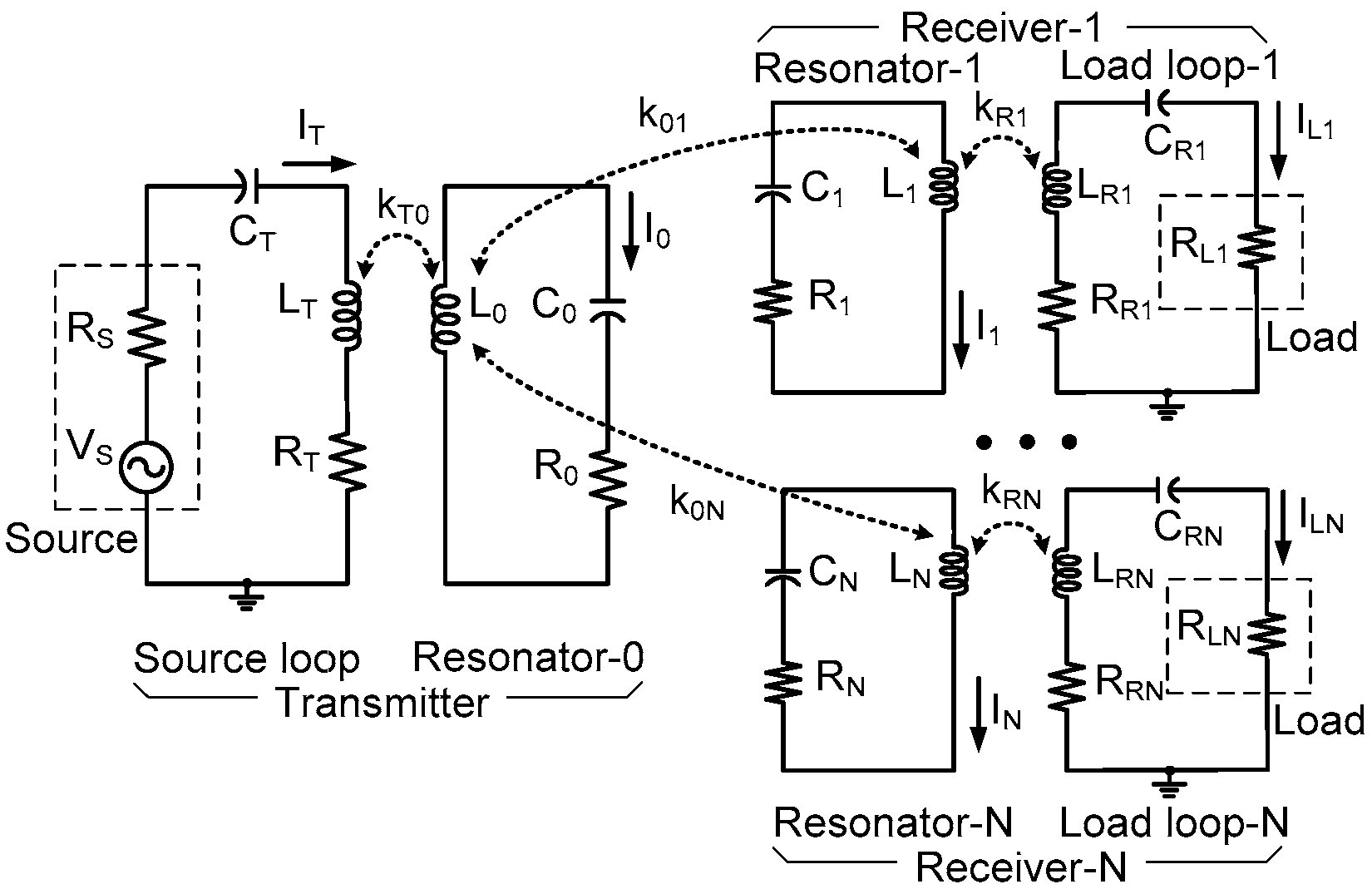
2.1. Proposed WPT System for Multiple Receivers
2.2. Analysis of Power Transfer and Division
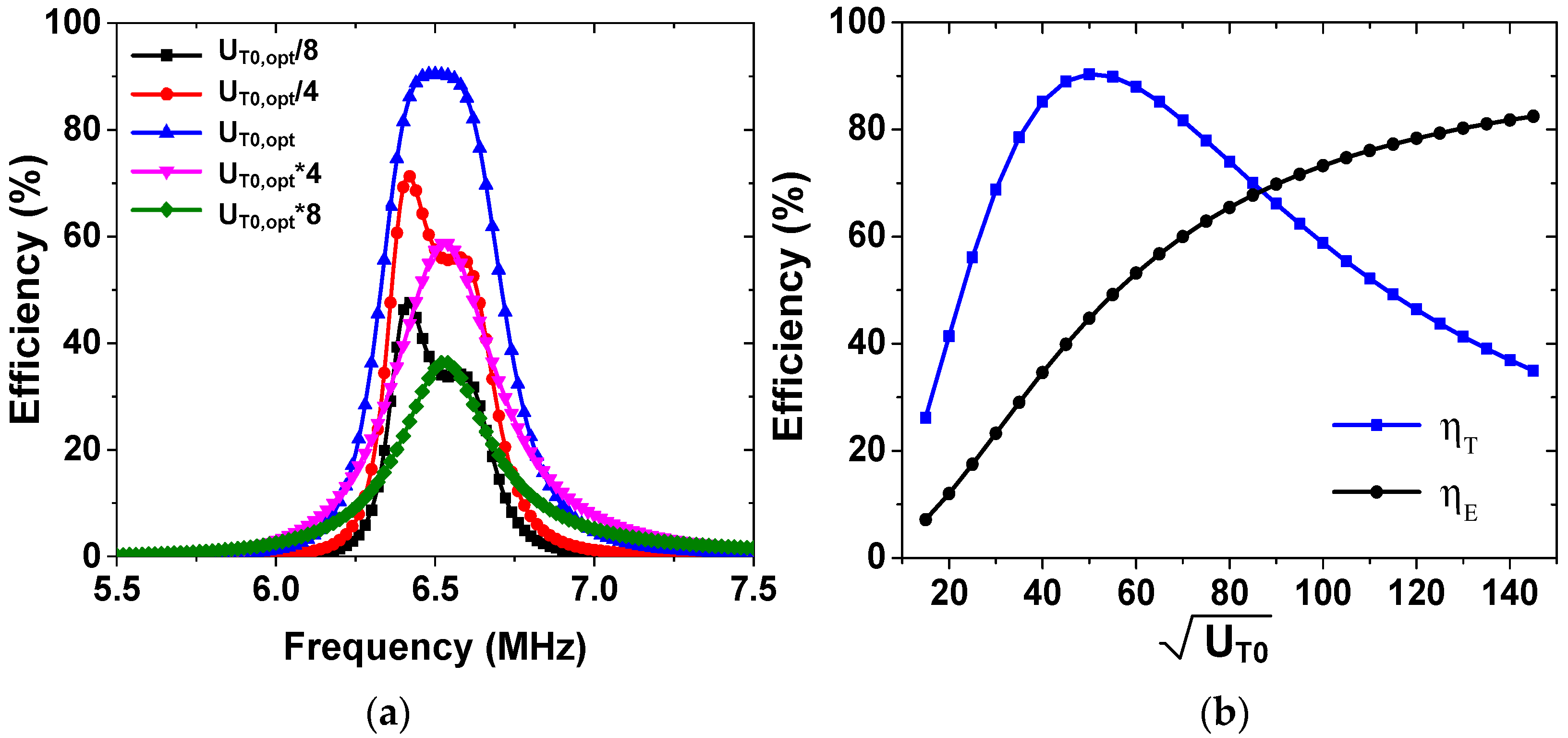
2.3. Analysis of Efficiency
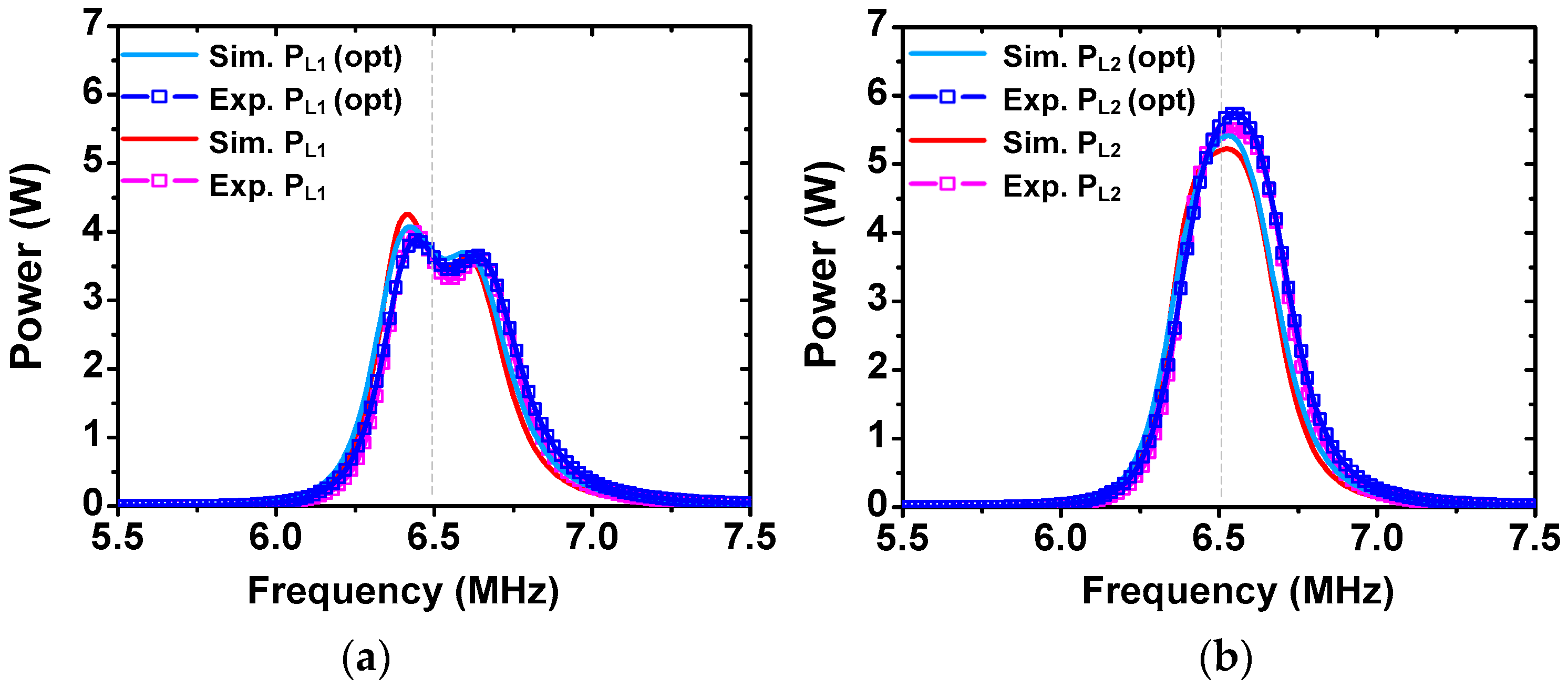
3. Experimental Results
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Symbol | Definition |
| , , , and | Inductance of the source loop, resonator-0, resonator-i, and load loop-i |
| , , , and | Capacitance of the source loop, resonator-0, resonator-i, and load loop-i |
| , , , and | Resistance of the source loop, resonator-0, resonator-i, and load loop-i |
| and | Source resistance and load resistance |
| , , and | Vertical distance between the source loop and resonator-0, resonator-0 and resonator-i, and resonator-i and load loop-i |
| Lateral displacement from the center of resonator-0 | |
| , and | Coupling coefficient of the transmitter, load, and receiver |
| , and | Normalized coupling coefficient of the transmitter, load, and receiver |
| , , and | Coupling factor of the transmitter, load, and receiver |
| , , , and | Loss rate of the source loop, resonator-0, resonator-i, and load loop-i |
| Quality factor of the source loop, resonator-0, resonator-i, and load loop-i | |
| , , and | Reflected impedance seen from the load loop-i to resonator-i, from resonator-i to resonator-0, and from resonator-0 to the source loop |
| , , and | Mutual inductance between the source loop and resonator-0, resonator-0 and resonator-i, and resonator-i and load loop-i |
| , , , and | Current in the source loop, resonator-0, resonator-i, and load loop-i |
| , , and | Power transferred to the resonator-0, resonator-i, and load loop-i |
| , , and | Input power, loss power, and output load power |
| Overall loading of N receivers | |
| Power ratio between two receivers | |
| and | System efficiency and overall power transfer efficiency |
| Optimal coupling value for maximum power transfer efficiency | |
References
- Xiao, H.; Qi, N.; Yin, Y.; Yu, S.; Sun, X.; Xuan, G.; Liu, J.; Xiao, S.; Li, Y.; Li, Y. Investigation of self-powered IoT sensor nodes for harvesting hybrid indoor ambient light and heat energy. Sensors 2023, 23, 3796. [Google Scholar] [CrossRef]
- Condon, F.; Martínez, J.M.; Eltamaly, A.M.; Kim, Y.-C.; Ahmed, M.A. Design and implementation of a cloud-IoT-based home energy management system. Sensors 2023, 23, 176. [Google Scholar] [CrossRef] [PubMed]
- Kurs, A.; Karalis, A.; Moffatt, R.; Joannopoulos, J.D.; Fisher, P.; Soljacic, M. Wireless power transfer via strongly coupled magnetic resonance. Science 2007, 317, 83–86. [Google Scholar] [CrossRef] [PubMed]
- Duong, T.P.; Lee, J.W. Experimental results of high-efficiency resonant coupling wireless power transfer using a variable coupling method. IEEE Microw. Wireless Compon. Lett. 2011, 21, 442–444. [Google Scholar] [CrossRef]
- Kim, N.Y.; Kim, K.Y.; Kim, C.W. Automated frequency tracking system for efficient mid-range magnetic resonance wireless power transfer. Microw. Opt. Technol. Lett. 2012, 54, 1423–1426. [Google Scholar] [CrossRef]
- Beh, T.C.; Kato, M.; Imura, T.; Oh, S.; Hori, Y. Automated impedance matching system for robust wireless power transfer via magnetic resonance coupling. IEEE Trans. Ind. Electron. 2013, 60, 3689–3698. [Google Scholar] [CrossRef]
- Kim, J.; Son, H.C.; Kim, K.H.; Park, Y.J. Efficiency analysis of magnetic resonance wireless power transfer with intermediate resonant coil. IEEE Antennas Wireless Propag. Lett. 2011, 10, 389–392. [Google Scholar] [CrossRef]
- Zhong, W.; Lee, C.K.; Hui, R. General analysis on the use of Tesla’s resonators in domino forms for wireless power transfer. IEEE Trans. Ind. Electron. 2013, 60, 261–270. [Google Scholar] [CrossRef]
- Huu, B.; Thanh, P.; Hiep, L.T.H.; Anh, N.H.; Tung, D.K.; Khuyen, B.X.; Tung, B.S.; Lam, V.D.; Zheng, H.; Chen, L.; et al. Enhancing wireless power transfer performance based on a digital honeycomb metamaterial structure for multiple charging locations. Crystals 2024, 14, 999. [Google Scholar] [CrossRef]
- Chen, H.; Qiu, D.; Rong, C.; Zhang, B. A double-transmitting coil wireless power transfer system based on parity time symmetry principle. IEEE Trans. Power Electron. 2023, 38, 13396–13404. [Google Scholar] [CrossRef]
- Zhang, Y.; Pan, W.; Wang, H.; Shen, Z.; Wu, Y.; Dong, J.; Mao, X. Misalignment-tolerant dual-transmitter electric vehicle wireless charging system with reconfigurable topologies. IEEE Trans. Power Electron. 2022, 37, 8816–8819. [Google Scholar] [CrossRef]
- Jun, Y.; Kim, J.; Lee, S.; Rhee, J.; Woo, S.; Huh, S.; Lee, C.; Ryu, S.; Lee, H.; Ahn, S. Multiple-split transmitting coils for stable output power in wireless power transfer system with variable airgaps. Energies 2024, 17, 4025. [Google Scholar] [CrossRef]
- Hao, X.; Yin, K.; Zou, J.; Wang, R.; Huang, Y.; Ma, X.; Dong, T. Frequency-stable robust wireless power transfer based on high-order pseudo-Hermitian physics. Phys. Rev. Lett. 2023, 130, 77202. [Google Scholar]
- Wu, L.; Zhang, B.; Jiang, Y.; Zhou, J. A robust parity-time-symmetric WPT system with extended constant-power range for cordless kitchen appliances. IEEE Trans. Ind. Electron. 2022, 58, 1179–1189. [Google Scholar]
- Shu, X.; Zhang, B.; Wei, Z.; Rong, C.; Sun, S. Extended-distance wireless power transfer system with constant output power and transfer efficiency based on parity-time-symmetric principle. IEEE Trans. Power Electron. 2021, 36, 8861–8871. [Google Scholar]
- Zhou, J.; Zhang, B.; Xiao, W.; Qiu, D.; Chen, Y. Nonlinear parity-time-symmetric model for constant efficiency wireless power transfer: Application to a drone-in-flight wireless charging platform. IEEE Trans. Ind. Electron. 2019, 66, 4097–4107. [Google Scholar]
- Wu, L.; Zhang, B.; Jiang, Y. Position-independent constant current or constant voltage wireless electric vehicles charging system without dual-side communication and DC-DC converter. IEEE Trans. Ind. Electron. 2022, 69, 7930–7939. [Google Scholar] [CrossRef]
- He, L.; Huang, X.; Cheng, B. Robust class E2 wireless power transfer system based on parity-time symmetry. IEEE Trans. Power Electron. 2023, 38, 4279–4288. [Google Scholar]
- Wei, Z.; Zhang, B. Transmission range extension of PT-symmetry-based wireless power transfer system. IEEE Trans. Power Electron. 2021, 36, 11135–11147. [Google Scholar]
- Qu, Y.; Zhang, B.; Gu, W.; Shu, X. Wireless power transfer system with high-order compensation network based on parity-time-symmetric principle and relay coil. IEEE Trans. Power Electron. 2023, 38, 1314–1323. [Google Scholar] [CrossRef]
- Casanova, J.J.; Low, Z.N.; Lin, J. A loosely coupled planar wireless power system for multiple receivers. IEEE Trans. Ind. Electron. 2009, 56, 3060–3068. [Google Scholar]
- Ahn, D.; Hong, S. Effect of coupling between multiple transmitters or multiple receivers on wireless power transfer. IEEE Trans. Ind. Electron. 2013, 60, 2602–2613. [Google Scholar] [CrossRef]
- Lee, S.; Kim, S.; Seo, C. Design of multiple receivers for wireless transfer using metamaterial. In Proceedings of the 2013 Asia Pacific Microwave Conference (APMC), Seoul, Republic of Korea, 5–8 November 2013; pp. 1036–1038. [Google Scholar]
- Koh, K.E.; Beh, T.C.; Imura, T.; Hori, Y. Impedance matching and power division using impedance inverter for wireless power transfer via magnetic resonant coupling. IEEE Trans. Ind. Appl. 2014, 50, 2061–2070. [Google Scholar] [CrossRef]
- Kurs, A.; Moffatt, R.; Soljačić, M. Simultaneous mid-range power transfer to multiple devices. Appl. Phys. Lett. 2020, 96, 044102. [Google Scholar]
- Kim, J.; Son, H.-C.; Kim, D.-H.; Park, Y.-J. Impedance matching considering cross coupling for wireless power transfer to multiple receivers. In Proceedings of the IEEE Wireless Power Transfer Conference (WPTC), Perugia, Italy, 15–16 May 2013; pp. 226–229. [Google Scholar]
- Luo, C.; Qiu, D.; Lin, M.; Zhang, B. Circuit model and analysis of multi-load wireless power transfer system based on parity-time symmetry. Energies 2020, 13, 3260. [Google Scholar] [CrossRef]
- Cui, H.; Dong, Z.; Kim, H.J.; Li, C.; Chen, W.; Xu, G.; Qiu, C.W.; Ho, J.S. High-efficiency selective wireless power transfer with a bistable parity-time-symmetric circuit. Phys. Rev. Appl. 2022, 18, 044076. [Google Scholar]
- Zhu, Z.; Yuan, H.; Zhang, R.; Yang, A.; Wang, X.; Rong, M. Parity–time symmetric model and analysis for stable multi-load wireless power transfer. World Electr. Veh. J. 2021, 12, 226. [Google Scholar] [CrossRef]
- Fu, M.; Yin, H.; Ma, C. Megahertz multiple-receiver wireless power transfer systems with power flow management and maximum efficiency point tracking. IEEE Trans. Microw. Theory Tech. 2017, 65, 4285–4293. [Google Scholar] [CrossRef]
- Ahn, D.; Kim, S.M.; Kim, S.W.; Moon, J.I.; Cho, I.K. Wireless power transfer receiver with adjustable coil output voltage for multiple receivers application. IEEE Trans. Ind. Electron. 2019, 66, 4003–4012. [Google Scholar]
- Cannon, B.L.; Hoburg, J.F.; Stancil, D.D.; Goldstein, S.C. Magnetic resonant coupling as a potential means for wireless power transfer to multiple small receivers. IEEE Trans. Power Electron. 2009, 24, 1819–1825. [Google Scholar]
- Hui, S.Y.; Zhong, W.X.; Lee, C.K. A critical review of recent progress in mid-range wireless power transfer. IEEE Trans. Power Electron. 2014, 29, 4500–4511. [Google Scholar] [CrossRef]


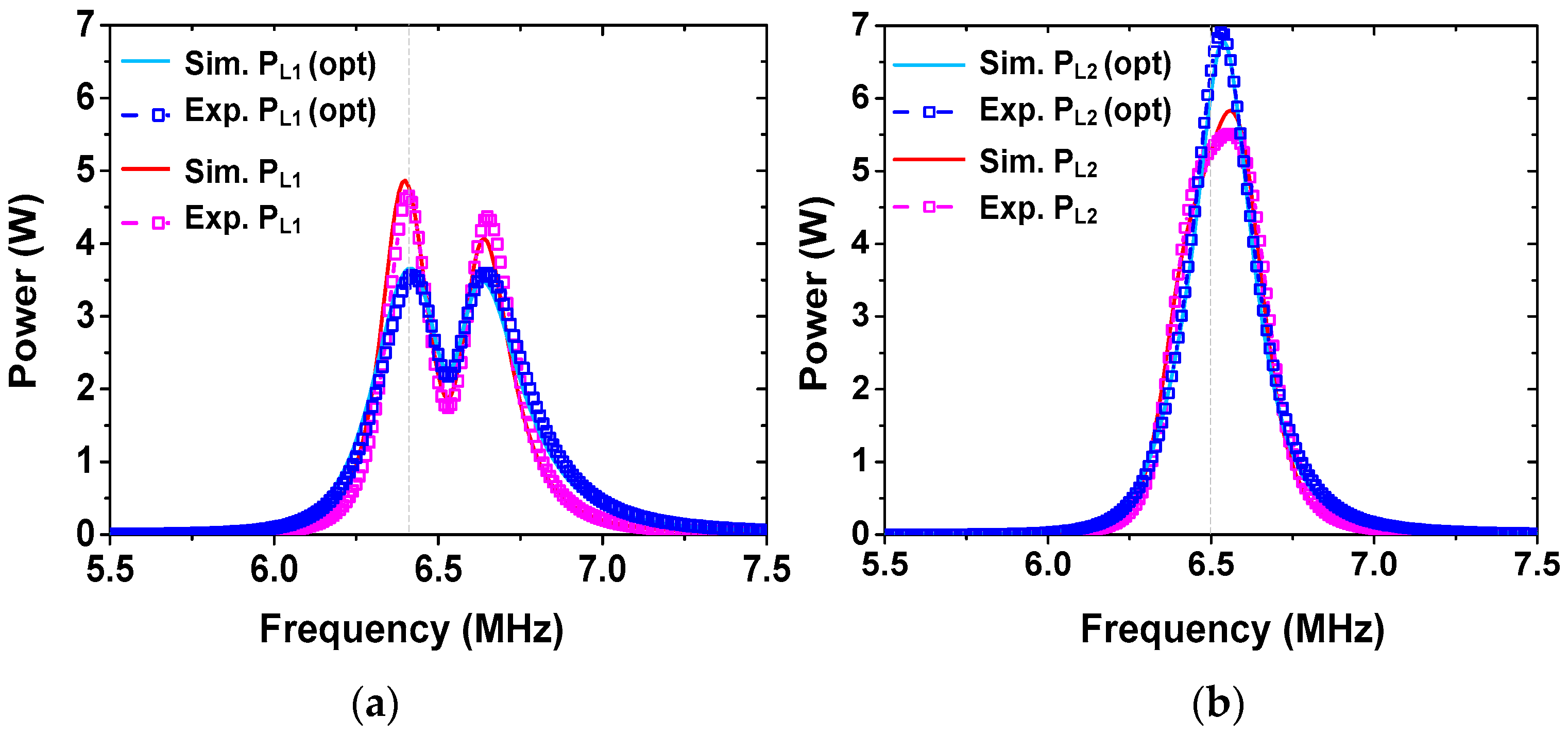
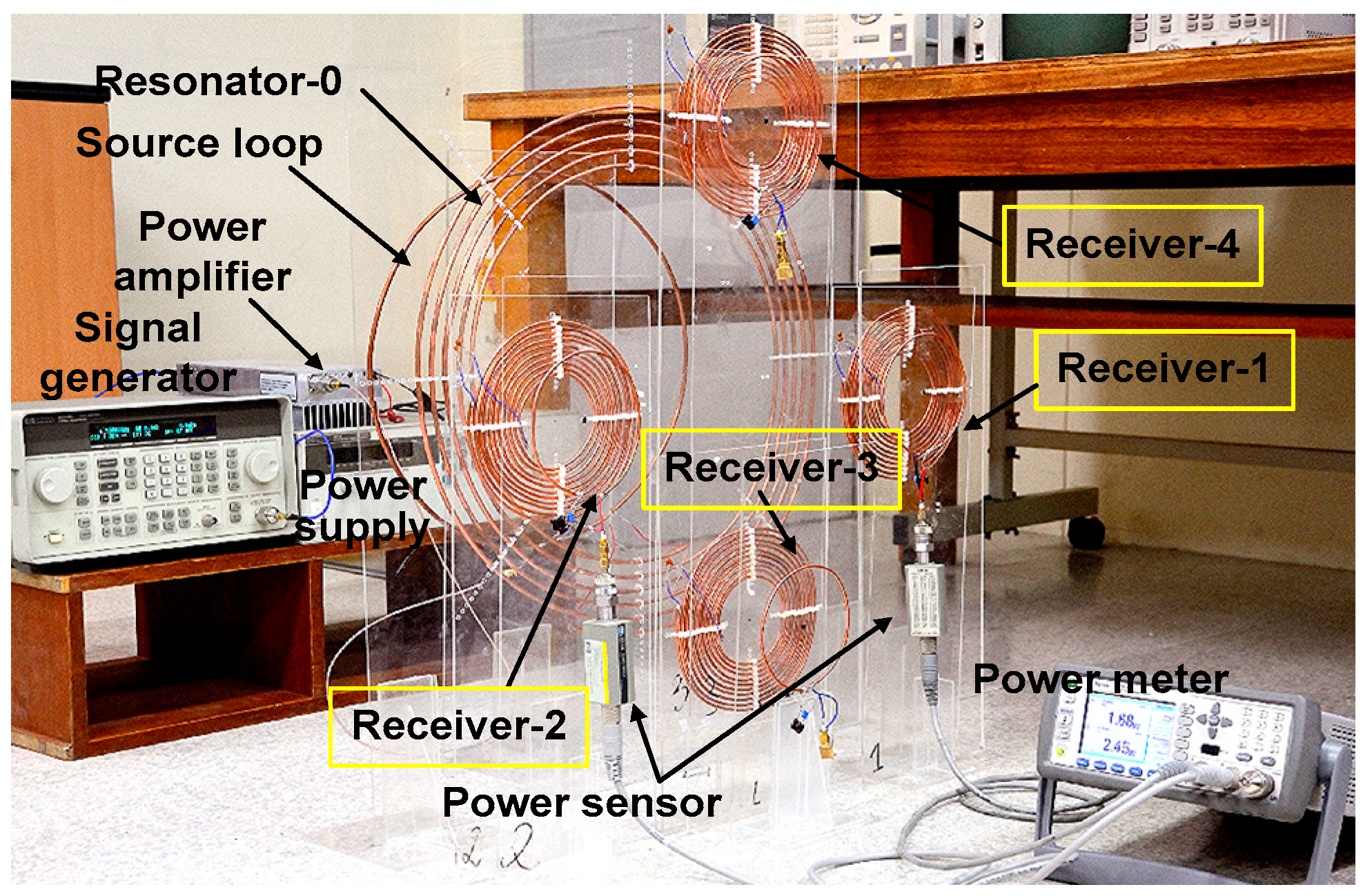
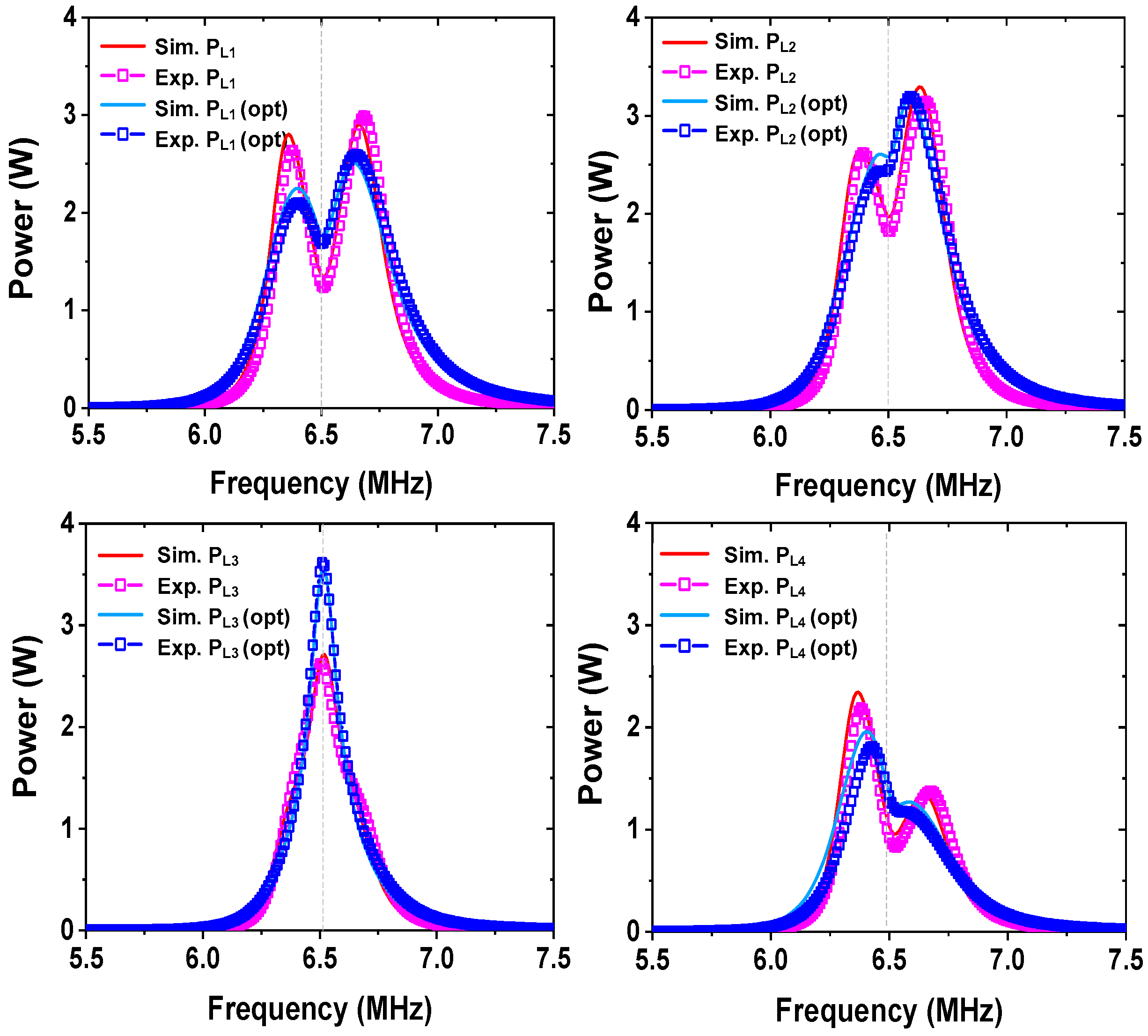
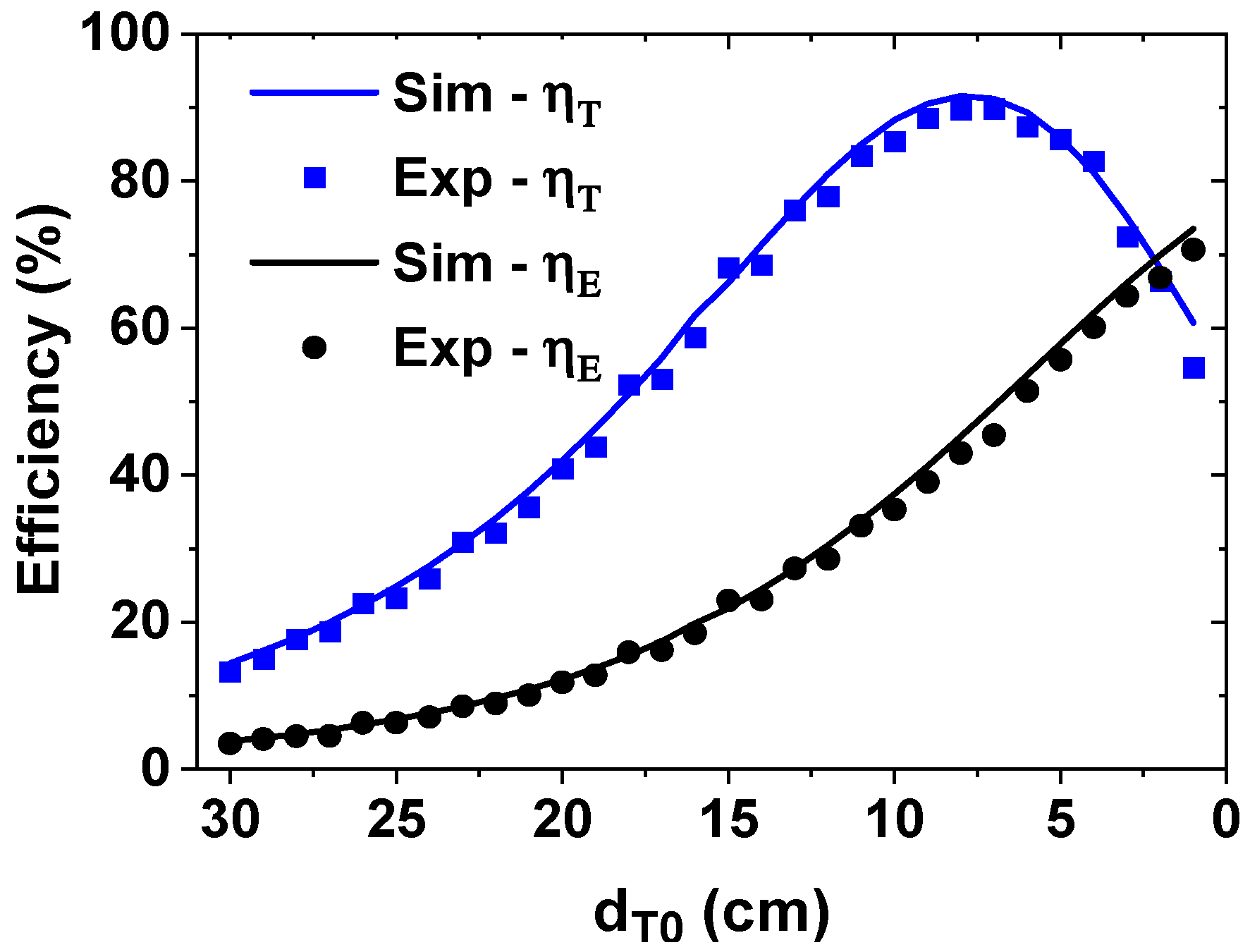
| Transmitter | Receiver-1 | Receiver-2 |
|---|---|---|
| = 50 Ω | RL1 = 50 Ω | RL2 = 50 Ω |
| = 2.12 × | = 2.26 × | = 2.58 × |
| = 3.15 × = 0.06–0.6 = 225–22,468 | = 3.32 × = 0.0408 = 0.475 = 976 = 12,555 = 238.1 | = 2.89 × = 0.0517 = 0.386 = 1372 = 8313 = 277.8 |
| Transmitter | Inductance (nH) | Resistance (Ω) | Freq. (MHz) | Q-Factor |
|---|---|---|---|---|
| Source loop | 1180 | 0.5 | 6.5 | 96.3 |
| Resonator-0 | 20,800 | 1.3 | 6.45 | 648.1 |
| Load loop-1 | 316 | 0.21 | 6.51 | 61.5 |
| Resonator-1 | 15,540 | 0.7 | 6.49 | 904.8 |
| Load loop-2 | 310 | 0.18 | 6.51 | 70.4 |
| Resonator-2 | 15,580 | 0.8 | 6.48 | 792.5 |
| Load loop-3 | 321 | 0.26 | 6.51 | 50.5 |
| Resonator-3 | 15,500 | 0.76 | 6.52 | 835.1 |
| Load loop-4 | 319 | 0.23 | 6.48 | 56.4 |
| Resonator-4 | 15,550 | 0.72 | 6.52 | 884.3 |
| Transmitter | 50 Ω | 2.12 × | 3.13 × | 1–30 cm |
| Receiver-1 | 50 Ω | 2.25 × | 3.32 × | 0.03 | 0.475 | 528 | 12,555 | 238 |
| Receiver-2 | 50 Ω | 2.57 × | 2.91 × | 0.03 | 0.387 | 462 | 8313 | 278 |
| Receiver-1 | 50 Ω | 2.25 × | 3.32 × | 0.03 | 0.475 | 528 | 12,572 | 238 |
| Receiver-2 | 50 Ω | 2.57 × | 2.91 × | 0.03 | 0.386 | 462 | 8294 | 278 |
| Receiver-3 | 50 Ω | 2.45 × | 4.05 × | 0.024 | 0.274 | 312 | 3164 | 192 |
| Receiver-4 | 50 Ω | 2.32 × | 3.63 × | 0.023 | 0.447 | 303 | 9965 | 217 |
| [9] | [10] | [16] | [23] | [26] | [28] | This Work | |
|---|---|---|---|---|---|---|---|
| WPT type | Resonant | Resonant | Resonant | Resonant | Resonant | Resonant | Resonant |
| No. of coils * | 4 | 3 | 2 | 2 | 3 | 2 | 4 |
| No. of receivers | 1 | 1 | 1 | 4 | 3 | 3 | 4 |
| No. of transmitters | 1 | 2 | 1 | 1 | 1 | 1 | 1 |
| Area ratio of Tx and Rx | 28:1 | 1:1 | 1.86:1 | 29:1 | 8.4:1 | 3:1 | 9:1 |
| Power division to multiple Rx | N | N | N | Y | Y | Y | Y |
| Unequal power division | N | N | N | N | N | Y | Y |
| Frequency () | 13.56 | 0.15 | 1 | 13.56 | 6.7 | 1.28 | 6.5 |
| Peak efficiency (%) | 64 | 94.2 | 93.6 | 40.4 | 88.3 | 65 | 91.7 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Duong, T.P.; Phi, N.H.; Ahmad, B.; Jayasekara, S.; Lee, J.-W. Analysis and Experiments of Resonant Coupling Wireless Power Transfer System for Nonuniform Powering of Multiple Sensors. Sensors 2025, 25, 2342. https://doi.org/10.3390/s25072342
Duong TP, Phi NH, Ahmad B, Jayasekara S, Lee J-W. Analysis and Experiments of Resonant Coupling Wireless Power Transfer System for Nonuniform Powering of Multiple Sensors. Sensors. 2025; 25(7):2342. https://doi.org/10.3390/s25072342
Chicago/Turabian StyleDuong, Thuc Phi, Ngoc Hung Phi, Bilal Ahmad, Sasani Jayasekara, and Jong-Wook Lee. 2025. "Analysis and Experiments of Resonant Coupling Wireless Power Transfer System for Nonuniform Powering of Multiple Sensors" Sensors 25, no. 7: 2342. https://doi.org/10.3390/s25072342
APA StyleDuong, T. P., Phi, N. H., Ahmad, B., Jayasekara, S., & Lee, J.-W. (2025). Analysis and Experiments of Resonant Coupling Wireless Power Transfer System for Nonuniform Powering of Multiple Sensors. Sensors, 25(7), 2342. https://doi.org/10.3390/s25072342





