Investigating the Influence of Laser Polarization on Filamentation Thresholds in Transparent Media via Supercontinuum Coherence
Abstract
1. Introduction
2. Experiment Setup
3. Results and Discussion
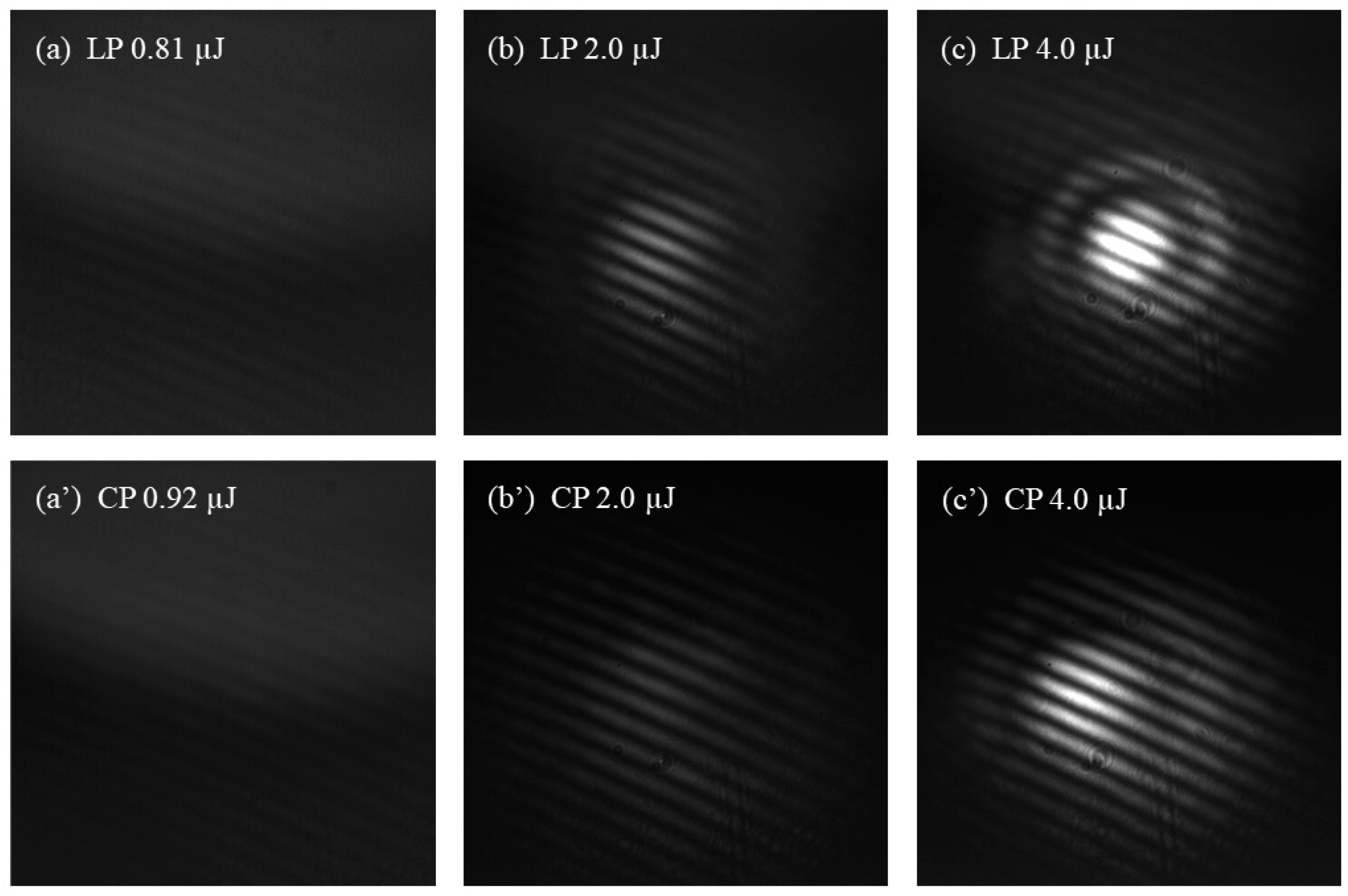
3.1. Filament Characteristics
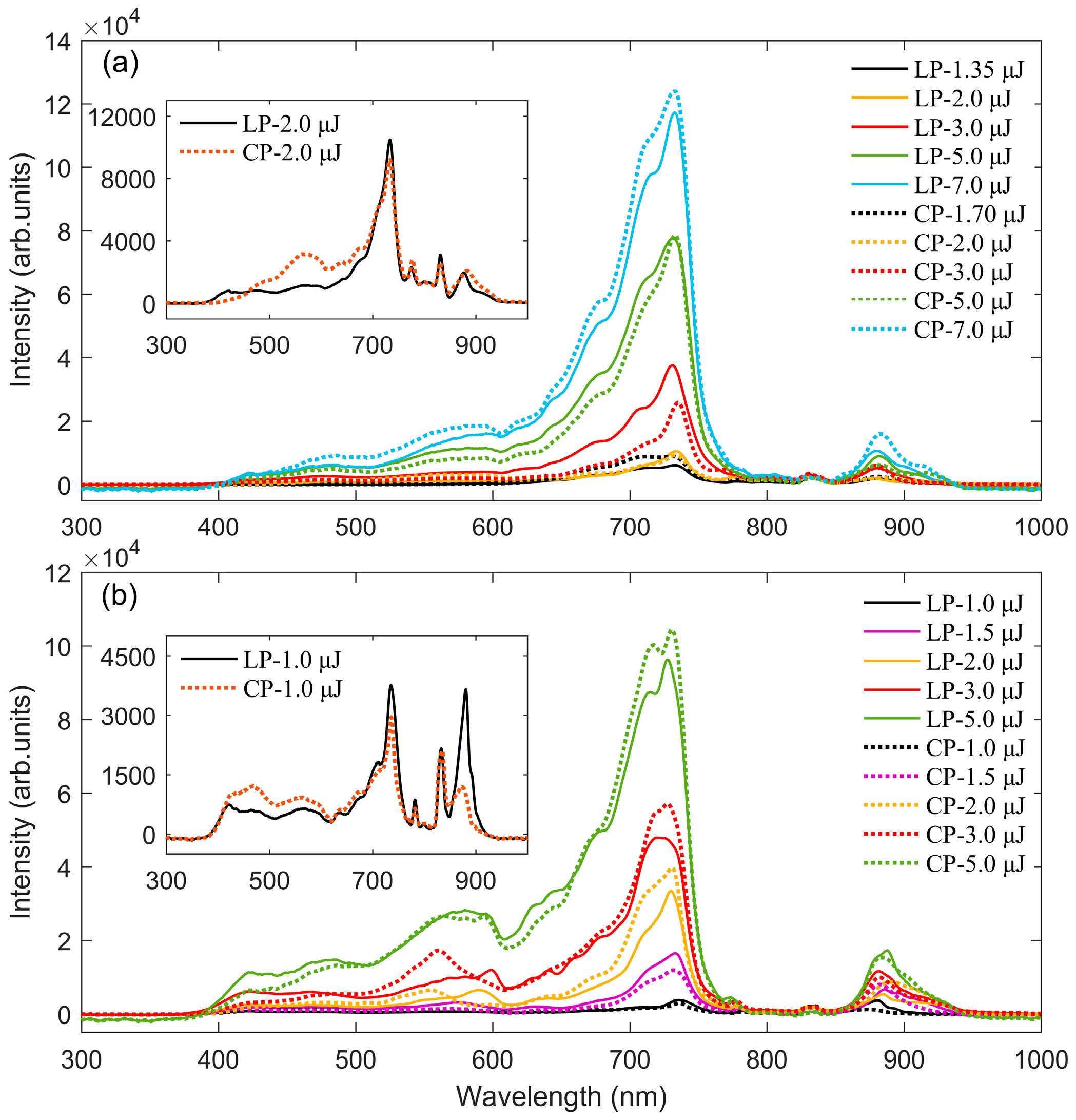
3.2. Supercontinuum Generation
3.3. Filamentation Threshold
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Xu, H.L.; Chin, S.L. Femtosecond laser filamentation for atmospheric sensing. Sensors 2011, 11, 32–53. [Google Scholar] [CrossRef] [PubMed]
- Yang, L.; Zhang, B.; Wu, T.; Zhao, Y.; Hou, J. Watt-level mid-infrared supercontinuum generation from 2.7 to 4.25 μm in an erbium-doped ZBLAN fiber with high slope efficiency. Opt. Lett. 2018, 43, 3061–3064. [Google Scholar] [PubMed]
- Swartling, J.; Bassi, A.; D’Andrea, C.; Pifferi, A.; Torricelli, A.; Cubeddu, R. Dynamic time-resolved diffuse spectroscopy based on supercontinuum light pulses. Appl. Opt. 2005, 44, 4684–4692. [Google Scholar]
- Rairoux, P.; Schillinger, H.; Niedermeier, S.; Rodriguez, M.; Ronneberger, F.; Sauerbrey, R.; Stein, B.; Waite, D.; Wedekind, C.; Wille, H.; et al. Remote sensing of the atmosphere using ultrashort laser pulses. Appl. Phys. B 2000, 71, 573–580. [Google Scholar]
- Kasparian, J.; Sauerbrey, R.; Mondelain, D.; Niedermeier, S.; Yu, J.; Wolf, J.P.; André, Y.B.; Franco, M.; Prade, B.; Tzortzakis, S.; et al. Infrared extension of the super continuum generated by femtosecond terawatt laser pulses propagating in the atmosphere. Opt. Lett. 2000, 25, 1397–1399. [Google Scholar]
- Cheng, C.; Zeng, Y.; Ou, Y.; Lv, H.; Lv, Q.; Zhang, J. Few-cycle pulse generation from compression of supercontinuum by optimizing initial chirp in ANDi-PCF. Optik 2017, 133, 66–72. [Google Scholar] [CrossRef]
- De Boni, L.; Andrade, A.; Misoguti, L.; Mendonça, C.; Zilio, S. Z-scan measurements using femtosecond continuum generation. Opt. Express 2004, 12, 3921–3927. [Google Scholar] [CrossRef]
- Balu, M.; Hales, J.; Hagan, D.J.; Stryland, E.V. White-light continuum Z-scan technique for nonlinear materials characterization. Opt. Express 2004, 12, 3820–3826. [Google Scholar] [CrossRef]
- Kasparian, J.; Rodriguez, M.; Méjean, G.; Yu, J.; Salmon, E.; Wille, H.; Bourayou, R.; Frey, S.; André, Y.B.; Mysyrowicz, A.; et al. White-light filaments for atmospheric analysis. Science 2003, 301, 61–64. [Google Scholar]
- Luo, Q.; Hosseini, S.A.; Liu, W.; Gravel, J.F.; Kosareva, O.G.; Panov, N.A.; Aközbek, N.; Kandidov, V.P.; Roy, G.; Chin, S.L. Effect of beam diameter on the propagation of intense femtosecond laser pulses. Appl. Phys. B 2005, 80, 35–38. [Google Scholar]
- Liu, W.; Chin, S.L. Direct measurement of the critical power of femtosecond Ti: Sapphire laser pulse in air. Opt. Express 2005, 13, 5750–5755. [Google Scholar] [CrossRef] [PubMed]
- Petit, S.; Talebpour, A.; Proulx, A.; Chin, S.L. Polarization dependence of the propagation of intense laser pulses in air. Opt. Commun. 2000, 175, 323–327. [Google Scholar] [CrossRef]
- Panov, N.A.; Kosyreva, O.G.; Savel’ev, A.B.; Uryupina, D.S.; Perezhogin, I.A.; Makarov, V.A. Filamentation of femtosecond Gaussian pulses with close-to-linear or-circular elliptical polarization. Quantum Electron. 2011, 41, 160. [Google Scholar] [CrossRef]
- Théberge, F.; Liu, W.; Simard, P.T.; Becker, A.; Chin, S.L. Plasma density inside a femtosecond laser filament in air: Strong dependence on external focusing. Phys. Rev. E 2006, 74, 036406. [Google Scholar] [CrossRef] [PubMed]
- Vuong, L.T.; Grow, T.D.; Ishaaya, A.; Gaeta, A.L.; ’tHooft, G.W.; Eliel, E.R.; Fibich, G. Collapse of optical vortices. Phys. Rev. Lett. 2006, 96, 133901. [Google Scholar] [CrossRef]
- Varela, O.; Zaïr, A.; Román, J.S.; Alonso, B.; Sola, I.J.; Prieto, C.; Roso, L. Above-millijoule super-continuum generation using polarisation dependent filamentation in atoms and molecules. Opt. Express 2009, 17, 3630–3639. [Google Scholar] [CrossRef]
- Kolesik, M.; Moloney, J.V.; Wright, E.M. Polarization dynamics of femtosecond pulses propagating in air. Phys. Rev. E 2001, 64, 046607. [Google Scholar] [CrossRef] [PubMed]
- Yang, H.; Zhang, J.; Zhang, Q.; Hao, Z.; Li, Y.; Zheng, Z.; Wang, Z.; Dong, Q.; Lu, X.; Wei, Z.; et al. Polarization-dependent supercontinuum generation from light filaments in air. Opt. Lett. 2005, 30, 534–536. [Google Scholar] [CrossRef]
- Sandhu, A.S.; Banerjee, S.; Goswami, D. Suppression of supercontinuum generation with circularly polarized light. Opt. Commun. 2000, 181, 101–107. [Google Scholar] [CrossRef]
- Srivastava, A.; Goswami, D. Control of supercontinuum generation with polarization of incident laser pulses. Appl. Phys. B 2003, 77, 325–328. [Google Scholar]
- Zhang, H.; Jing, C.; Li, G.; Xie, H.; Yao, J.; Zeng, B.; Chu, W.; Ni, J.; Xu, H.; Cheng, Y. Abnormal dependence of strong-field-ionization-induced nitrogen lasing on polarization ellipticity of the driving field. Phys. Rev. A 2013, 88, 063417. [Google Scholar]
- Mitryukovskiy, S.; Liu, Y.; Ding, P.; Houard, A.; Couairon, A.; Mysyrowicz, A. Plasma luminescence from femtosecond filaments in air: Evidence for impact excitation with circularly polarized light pulses. Phys. Rev. Lett. 2015, 114, 063003. [Google Scholar]
- Rostami, S.; Chini, M.; Lim, K.; Palastro, J.P.; Durand, M.; Diels, J.C.; Arissian, L.; Baudelet, M.; Richardson, M. Dramatic enhancement of supercontinuum generation in elliptically-polarized laser filaments. Sci. Rep. 2016, 6, 20363. [Google Scholar]
- Chen, N.; Wang, T.J.; Zhu, Z.; Guo, H.; Liu, Y.; Yin, F.; Sun, H.; Leng, Y.; Li, R. Laser ellipticity-dependent supercontinuum generation by femtosecond laser filamentation in air. Opt. Lett. 2020, 45, 4444–4447. [Google Scholar] [CrossRef] [PubMed]
- Bergé, L.; Gouédard, C.; Schjødt-Eriksen, J.; Ward, H. Filamentation patterns in Kerr media vs. beam shape robustness, nonlinear saturation and polarization states. Phys. D 2003, 176, 181–211. [Google Scholar]
- Schimpf, D.N.; Eidam, T.; Seise, E.; Hädrich, S.; Limpert, J.; Tünnermann, A. Circular versus linear polarization in laser-amplifiers with kerr-nonlinearity. Opt. Express 2009, 17, 18774–18781. [Google Scholar]
- Liu, C.; Zang, H.; Li, H.; Yu, Y.; Xu, H. Polarization effect on critical power and luminescence in an air filament. Chin. Opt. Lett. 2017, 15, 120201. [Google Scholar]
- Bellini, M.; Hänsch, T.W. Phase-locked white-light continuum pulses: Toward a universal optical frequency-comb synthesizer. Opt. Lett. 2000, 25, 1049–1051. [Google Scholar]
- Dudley, J.M.; Coen, S. Coherence properties of supercontinuum spectra generated in photonic crystal and tapered optical fibers. Opt. Lett. 2002, 27, 1180–1182. [Google Scholar]
- Cook, K.; Kar, A.K.; Lamb, R.A. White-light supercontinuum interference of self-focused filaments in water. Appl. Phys. Lett. 2003, 83, 3861–3863. [Google Scholar]
- Semenova, V.A.; Tsypkin, A.V.; Putilin, S.E.; Bespalov, V.G. A method for the coherence measurement of the supercontinuum source using Michelson interferometer. J. Phys. Conf. Ser. 2014, 536, 012027. [Google Scholar]
- Zhang, Y.; Xia, Y.; Liang, C.; Chen, A.; Li, S.; Jin, M. Exploring the femtosecond filamentation threshold in liquid media using a Mach–Zehnder interferometer. Sensors 2023, 23, 9163. [Google Scholar] [CrossRef]
- Zhang, H.; Zhang, Y.; Lin, S.; Chang, M.; Yu, M.; Wang, Y.; Chen, A.; Jiang, Y.; Li, S.; Jin, M. Testing the coherence of supercontinuum generated by optical vortex beam in water. J. Phys. B At. Mol. Opt. Phys. 2021, 54, 165401. [Google Scholar]
- Fibich, G.; Ilan, B. Self-focusing of elliptic beams: An example of the failure of the aberrationless approximation. J. Opt. Soc. Am. B 2000, 17, 1749–1758. [Google Scholar]
- Kandidov, V.P.; Fedorov, V.Y. Properties of self-focusing of elliptic beams. Quantum Electron. 2004, 34, 1163. [Google Scholar]
- Nuter, R.; Skupin, S.; Bergé, L. Chirp-induced dynamics of femtosecond filaments in air. Opt. Lett. 2005, 30, 917–919. [Google Scholar]
- Sutherland, R.L. Handbook of Nonlinear Optics; Marcel Dekker: New York, NY, USA, 1996; p. 299. [Google Scholar]
- Alfano, R.R.; Shapiro, S.L. Observation of self-phase modulation and small-scale filaments in crystals and glasses. Phys. Rev. Lett. 1970, 24, 592–594. [Google Scholar]
- Gaeta, A.L. Catastrophic collapse of ultrashort pulses. Phys. Rev. Lett. 2000, 84, 3582–3585. [Google Scholar]
- Liu, W.; Petit, S.; Becker, A.; Aközbek, N.; Bowden, C.M.; Chin, S.L. Intensity clamping of a femtosecond laser pulse in condensed matter. Opt. Commun. 2002, 202, 189–197. [Google Scholar]
- Couairon, A.; Chakraborty, H.S.; Gaarde, M.B. From single-cycle self-compressed filaments to isolated attosecond pulses in noble gases. Phys. Rev. A 2008, 77, 053814. [Google Scholar]
- Kiran, P.P.; Bagchi, S.; Arnold, C.L.; Krishnan, S.R.; Kumar, G.R.; Couairon, A. Filamentation without intensity clamping. Opt. Express 2010, 18, 21504–21510. [Google Scholar] [PubMed]
- Panov, N.A.; Makarov, V.A.; Fedorov, V.Y.; Kosareva, O.G. Filamentation of arbitrary polarized femtosecond laser pulses in case of high-order Kerr effect. Opt. Lett. 2013, 38, 537–539. [Google Scholar]
- Zhang, X.; Wang, T.J.; Guo, H.; Chen, N.; Lin, L.; Zhang, L.; Sun, H.; Liu, J.; Liu, J.; Shen, B.; et al. Polarization sensitive laser intensity inside femtosecond filament in air. arXiv 2019, arXiv:1902.03432. [Google Scholar]
- Li, S.; Wang, X.; Zhang, Y.; Yu, M.; Wang, Y.; Liu, F.; Jin, M. Femtosecond filamentation in water studied by the interference of supercontinuum. Phys. Scr. 2023, 98, 015501. [Google Scholar]
- Couairon, A.; Mysyrowicz, A. Femtosecond filamentation in transparent media. Phys. Rep. 2007, 441, 47–189. [Google Scholar] [CrossRef]
- Marburger, J.H. Self-focusing: Theory. Prog. Quantum Electron. 1975, 4, 35–110. [Google Scholar] [CrossRef]
- Talanov, V.I. Focusing of Light in Cubic Media. JETP Lett. 1970, 11, 199–201. [Google Scholar]
- Gu, X.; Akturk, S.; Trebino, R. Spatial chirp in ultrafast optics. Opt. Commun. 2004, 242, 599–604. [Google Scholar]
- Guo, H.; Dong, X.; Wang, T.J.; Zhang, X.; Chen, N.; Yin, F.; Wang, Y.; Zhang, L.; Sun, H.; Liu, J.; et al. Polarization dependent clamping intensity inside a femtosecond filament in air. Chin. Opt. Lett. 2021, 19, 103201. [Google Scholar]





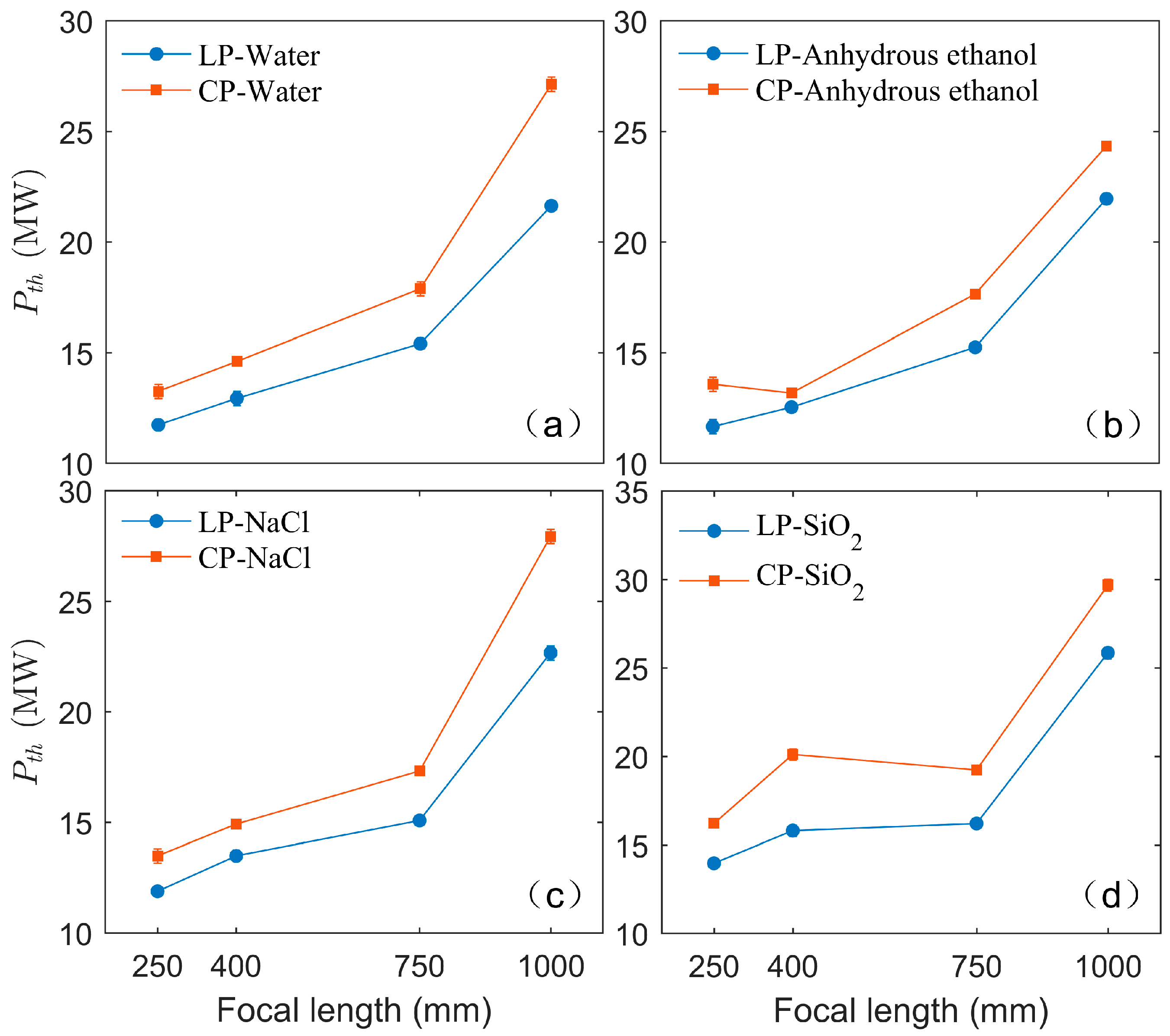
| Material | Polarization | Pth (MW) | |||
|---|---|---|---|---|---|
| f = 250 mm | f = 400 mm | f = 750 mm | f = 1000 mm | ||
| Water | LP | 11.73 | 12.93 | 15.40 | 21.63 |
| CP | 13.25 | 14.60 | 17.88 | 27.13 | |
| Anhydrous ethanol | LP | 11.65 | 12.53 | 15.24 | 21.95 |
| CP | 13.57 | 13.17 | 17.64 | 24.34 | |
| NaCl (100 mg/mL) | LP | 11.89 | 13.49 | 15.08 | 22.67 |
| CP | 13.49 | 14.92 | 17.32 | 27.93 | |
| SiO2 | LP | 13.97 | 15.80 | 16.20 | 25.86 |
| CP | 16.20 | 20.11 | 19.23 | 29.69 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, Y.; Xia, Y.; Liang, C.; Xiong, Y.; Zhang, J.; Lin, S.; Li, S.; Jin, M. Investigating the Influence of Laser Polarization on Filamentation Thresholds in Transparent Media via Supercontinuum Coherence. Sensors 2025, 25, 2285. https://doi.org/10.3390/s25072285
Zhang Y, Xia Y, Liang C, Xiong Y, Zhang J, Lin S, Li S, Jin M. Investigating the Influence of Laser Polarization on Filamentation Thresholds in Transparent Media via Supercontinuum Coherence. Sensors. 2025; 25(7):2285. https://doi.org/10.3390/s25072285
Chicago/Turabian StyleZhang, Yun, Yu Xia, Canneng Liang, Yuyao Xiong, Jingyuan Zhang, Shuang Lin, Suyu Li, and Mingxing Jin. 2025. "Investigating the Influence of Laser Polarization on Filamentation Thresholds in Transparent Media via Supercontinuum Coherence" Sensors 25, no. 7: 2285. https://doi.org/10.3390/s25072285
APA StyleZhang, Y., Xia, Y., Liang, C., Xiong, Y., Zhang, J., Lin, S., Li, S., & Jin, M. (2025). Investigating the Influence of Laser Polarization on Filamentation Thresholds in Transparent Media via Supercontinuum Coherence. Sensors, 25(7), 2285. https://doi.org/10.3390/s25072285






