Accuracy Evaluation Method for Blade Vibration Measurement in Blade Tip Timing Based on Direct Calibration Using Time of Arrival
Abstract
1. Introduction
2. Accuracy Evaluation Method for Blade Tip Timing
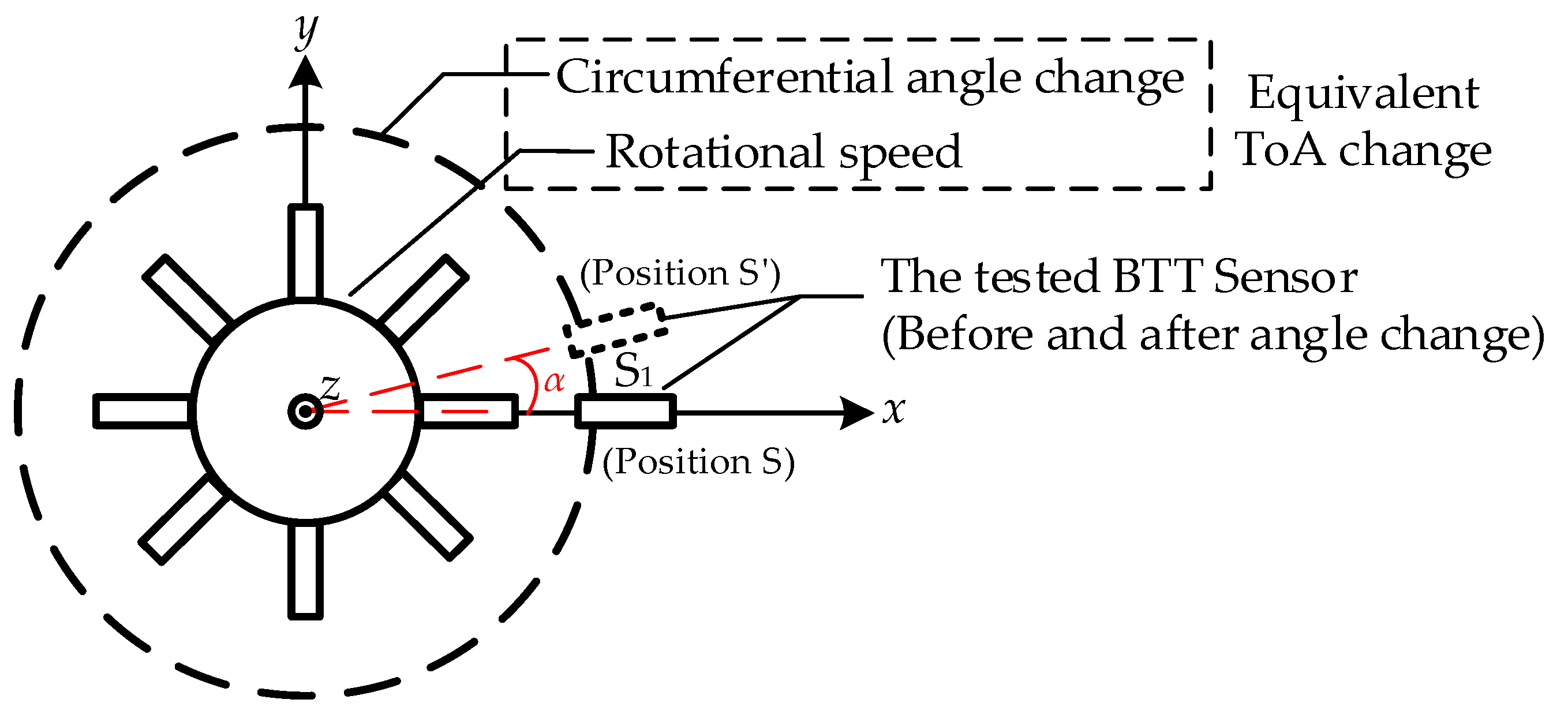
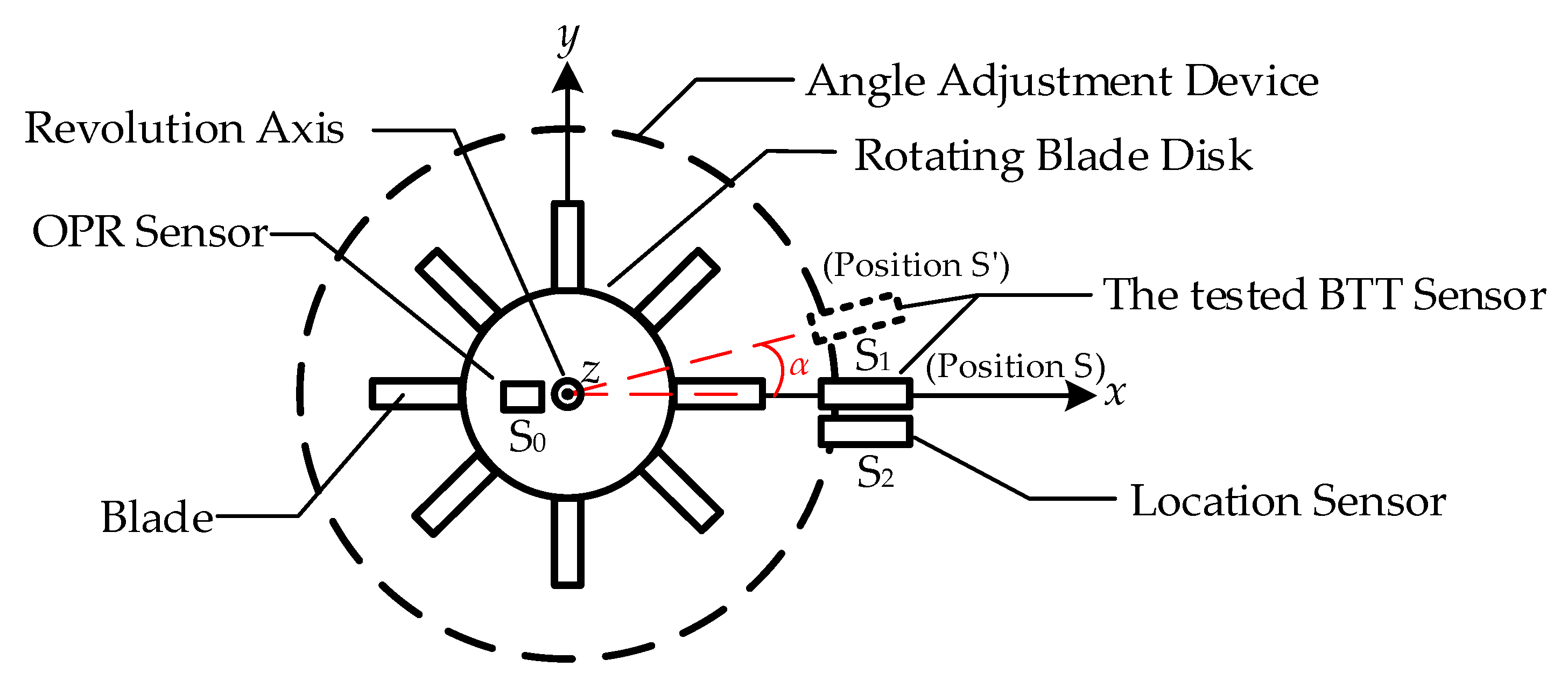
2.1. Direct Calibration Method of Time of Arrival Based on Circumferential Angle Difference
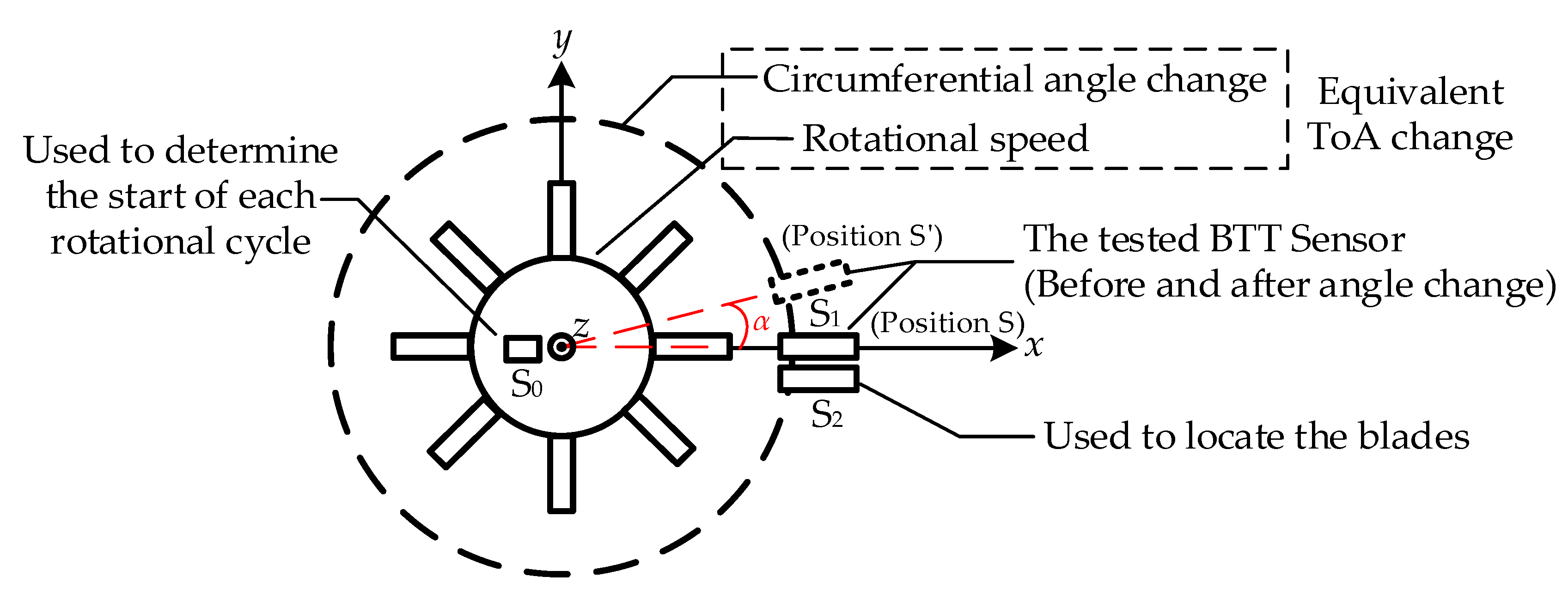
2.2. Blade Tip Timing Accuracy Evaluation Method Based on Time of Arrival
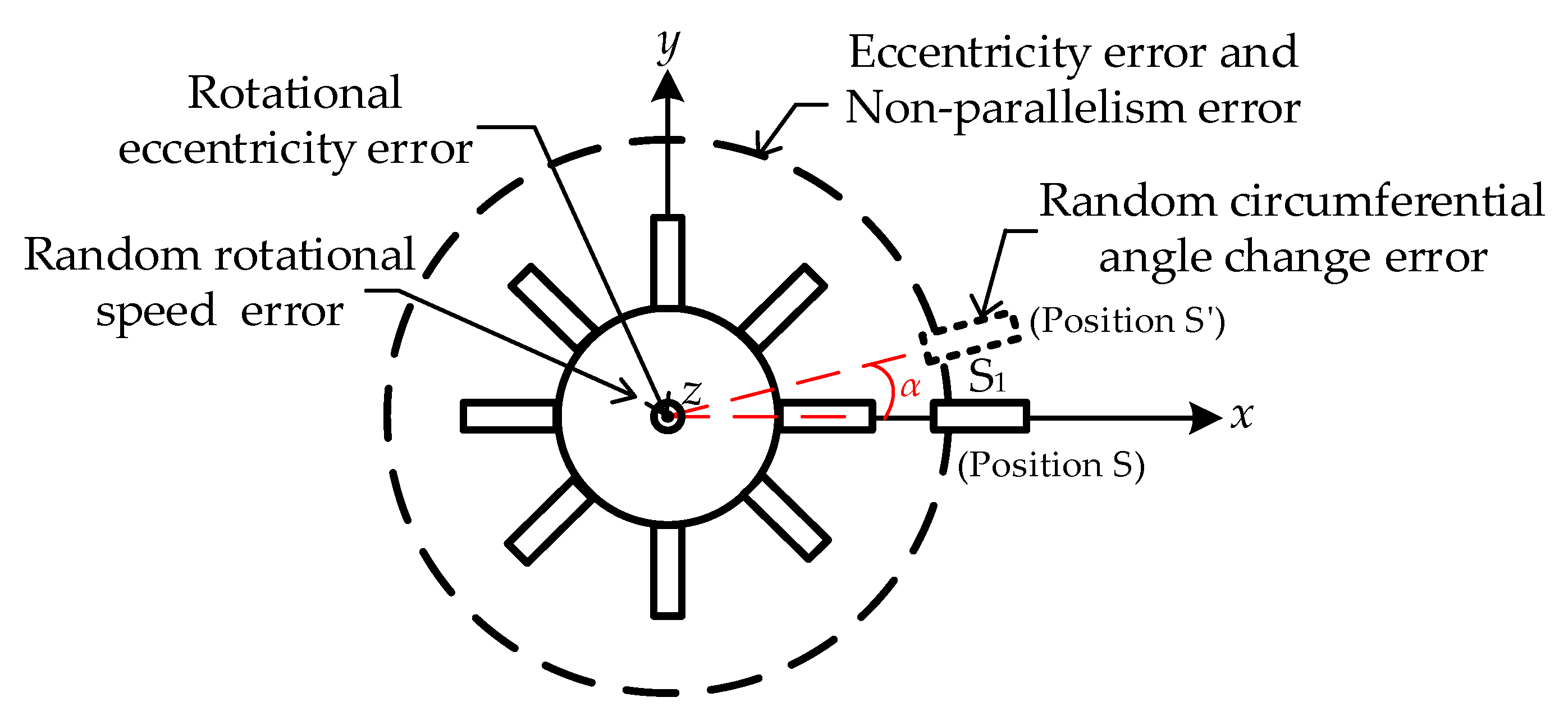
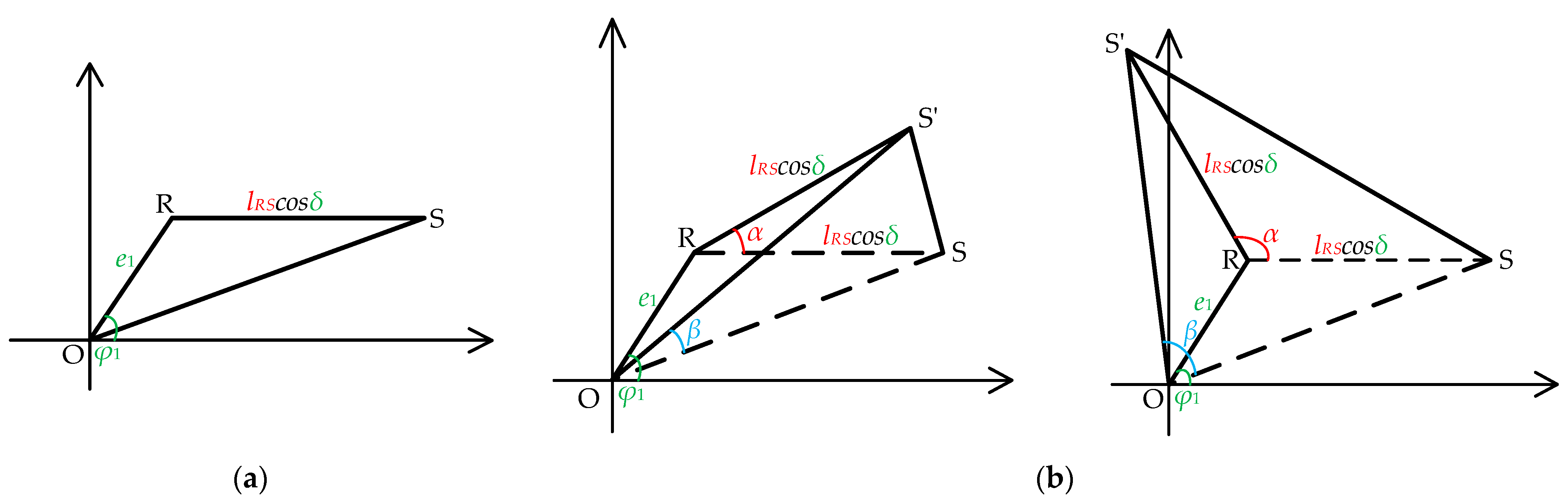
2.3. Error Source Analysis
3. Blade-Tip-Timing Accuracy Evaluation Device
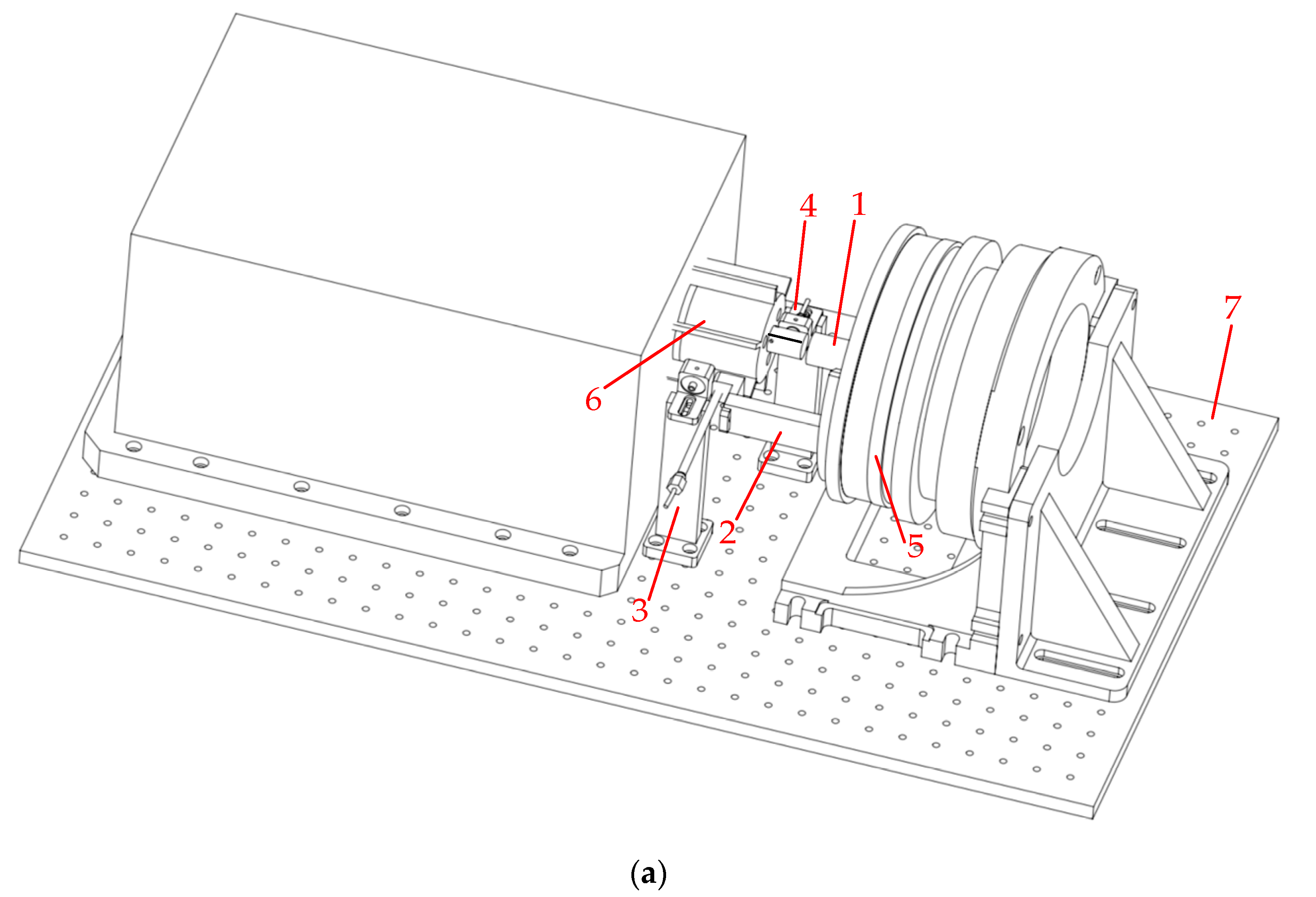
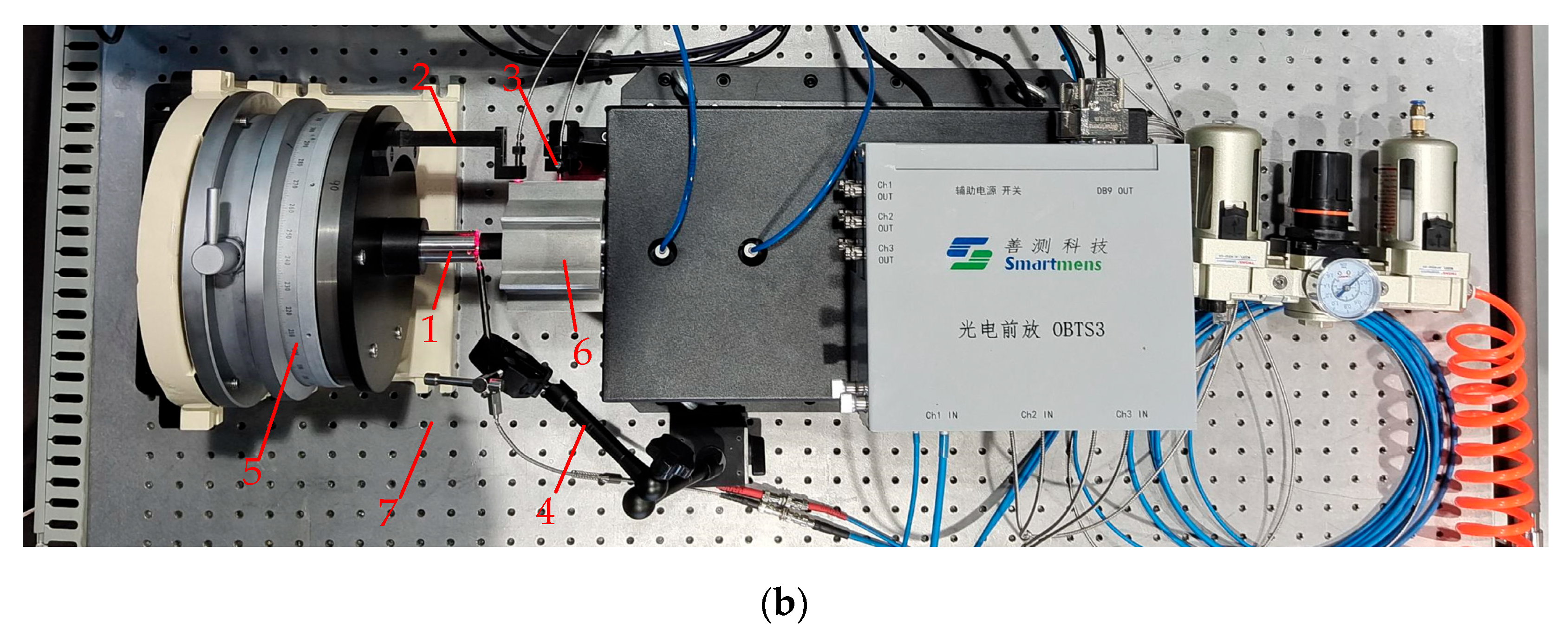
3.1. Device Design
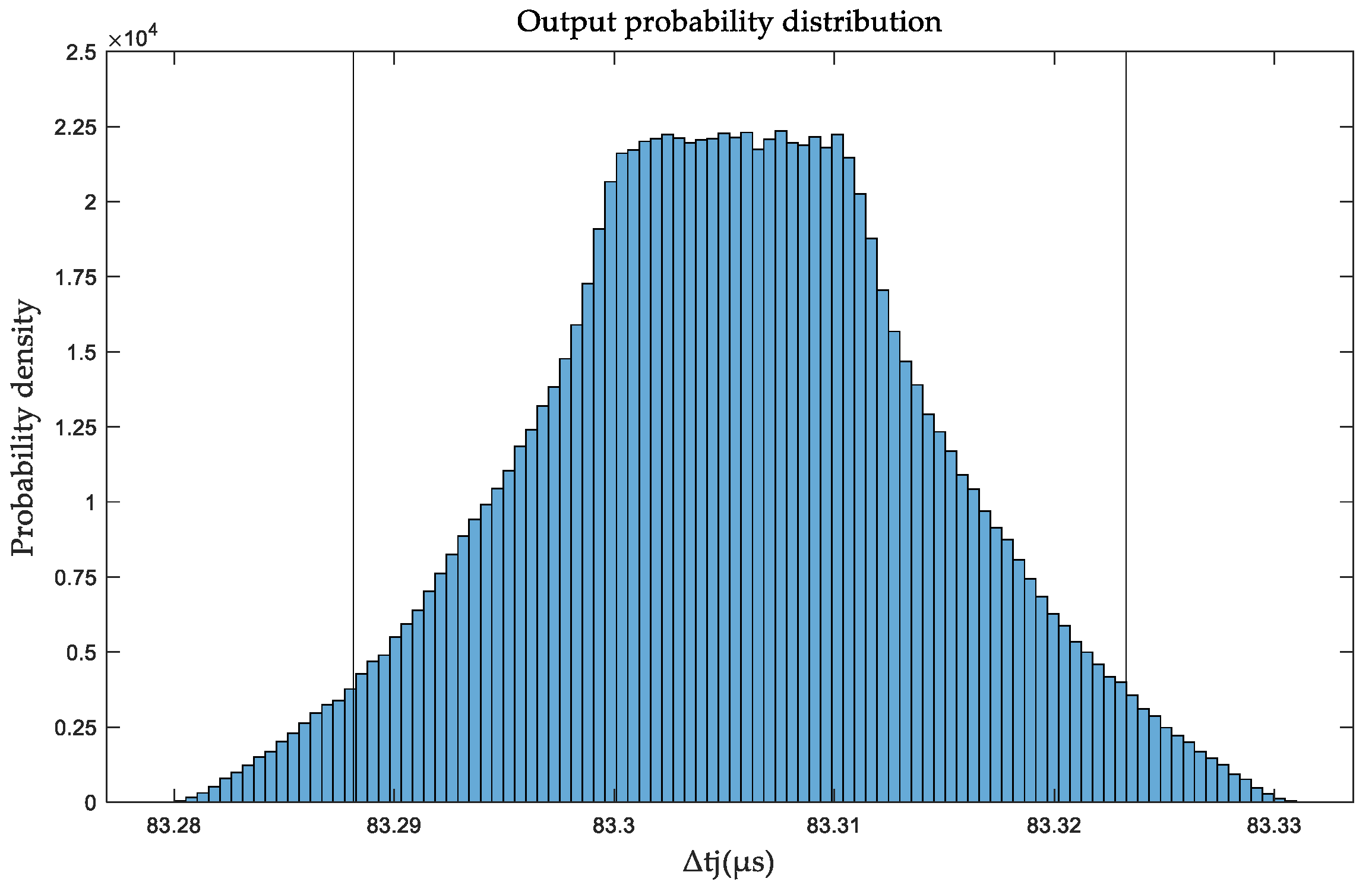
3.2. Uncertainty Evaluation of Device Based on Monte Carlo Method
4. Blade-Tip-Timing Accuracy Evaluation Test
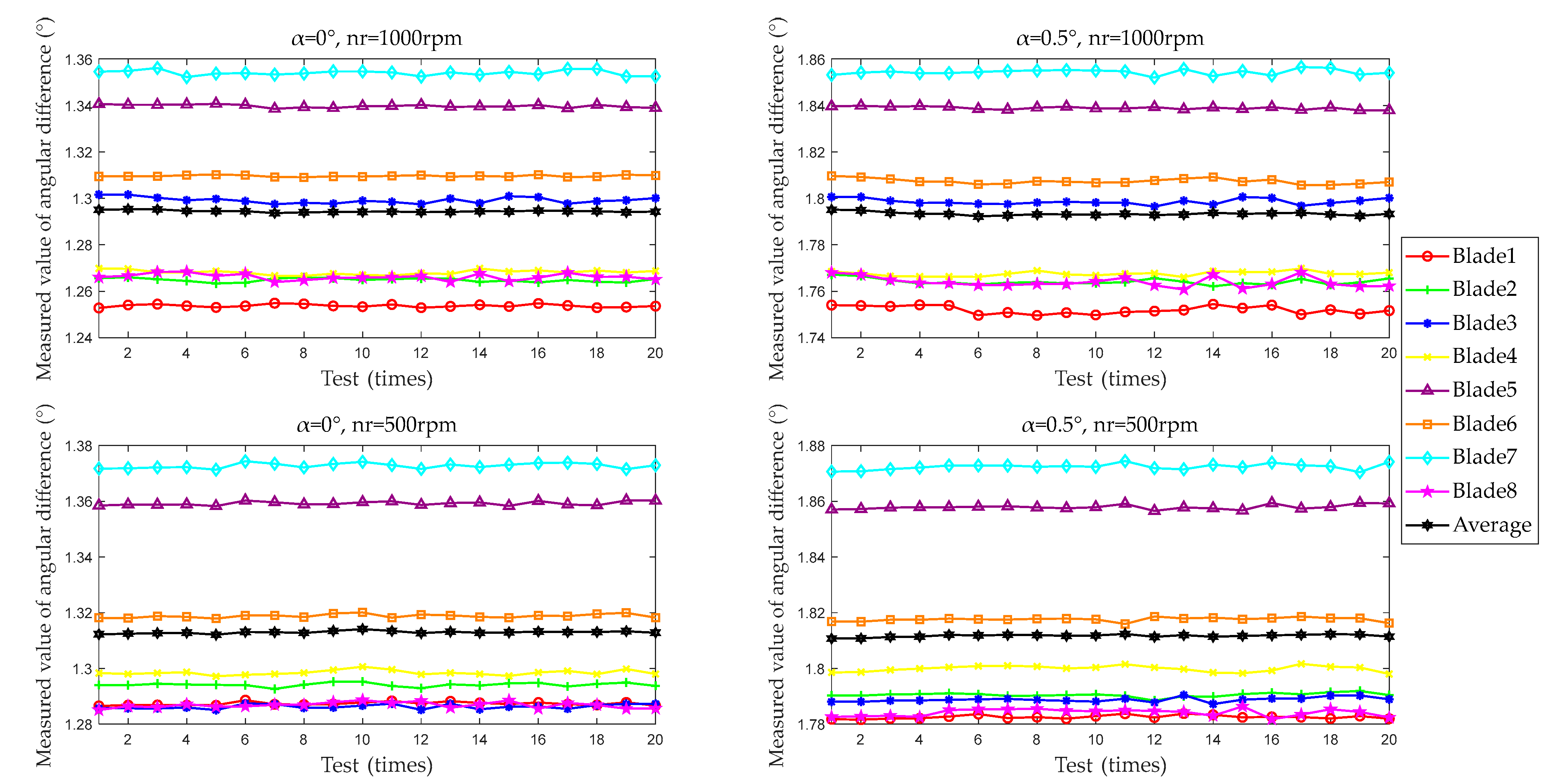
4.1. Test Scheme and Measurement Data
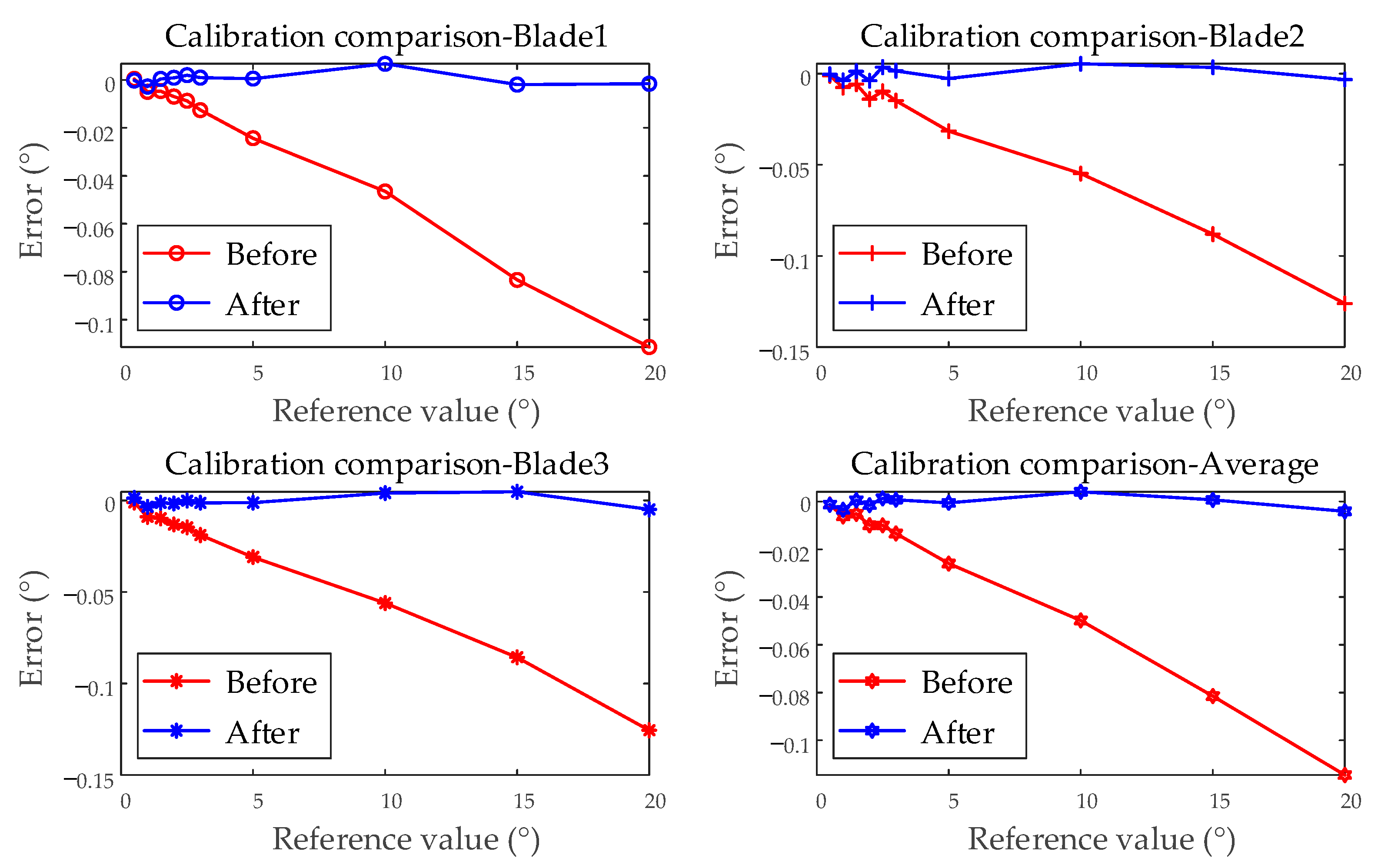
4.2. System Error Compensation of BTT Measurement System
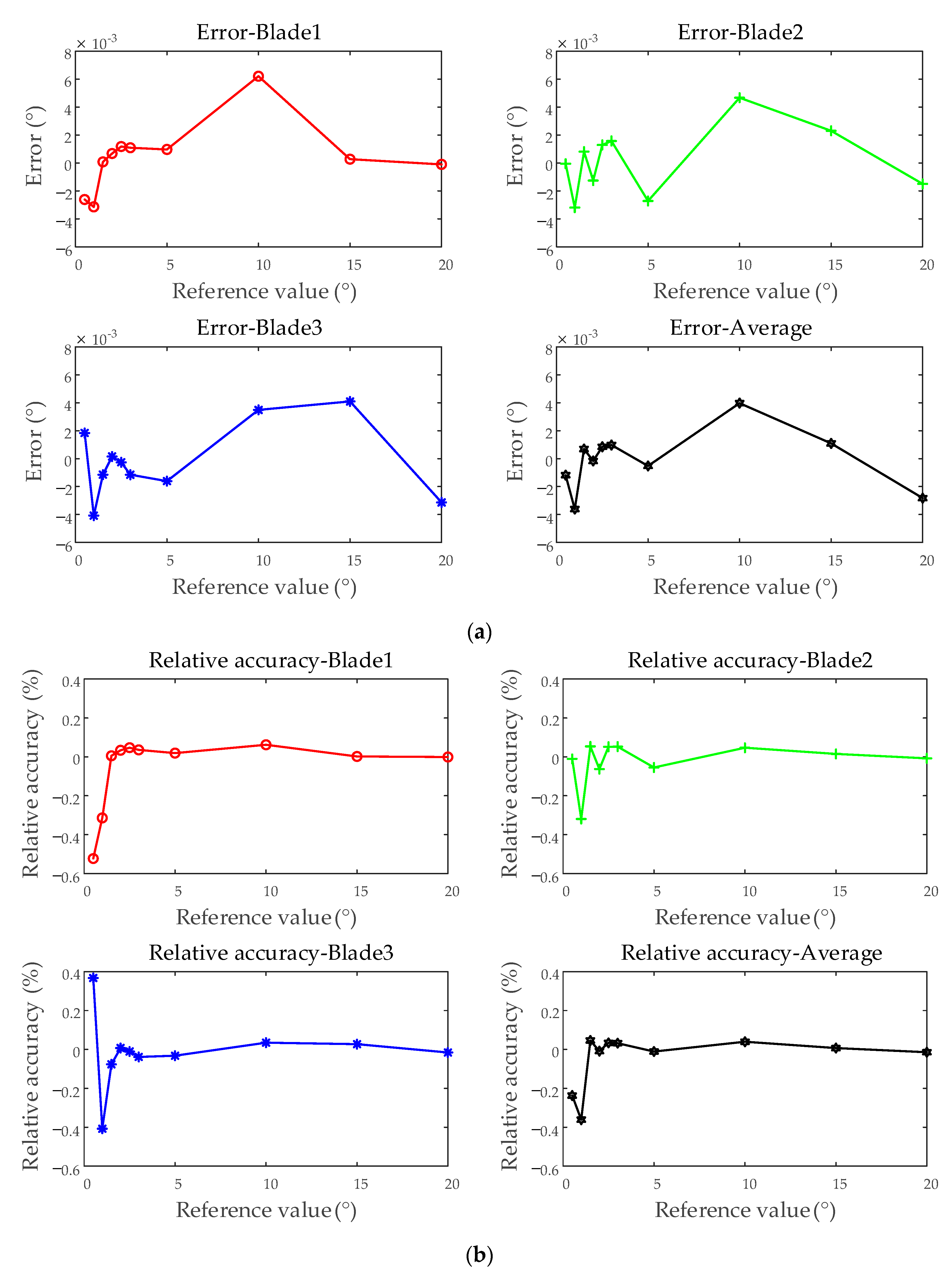
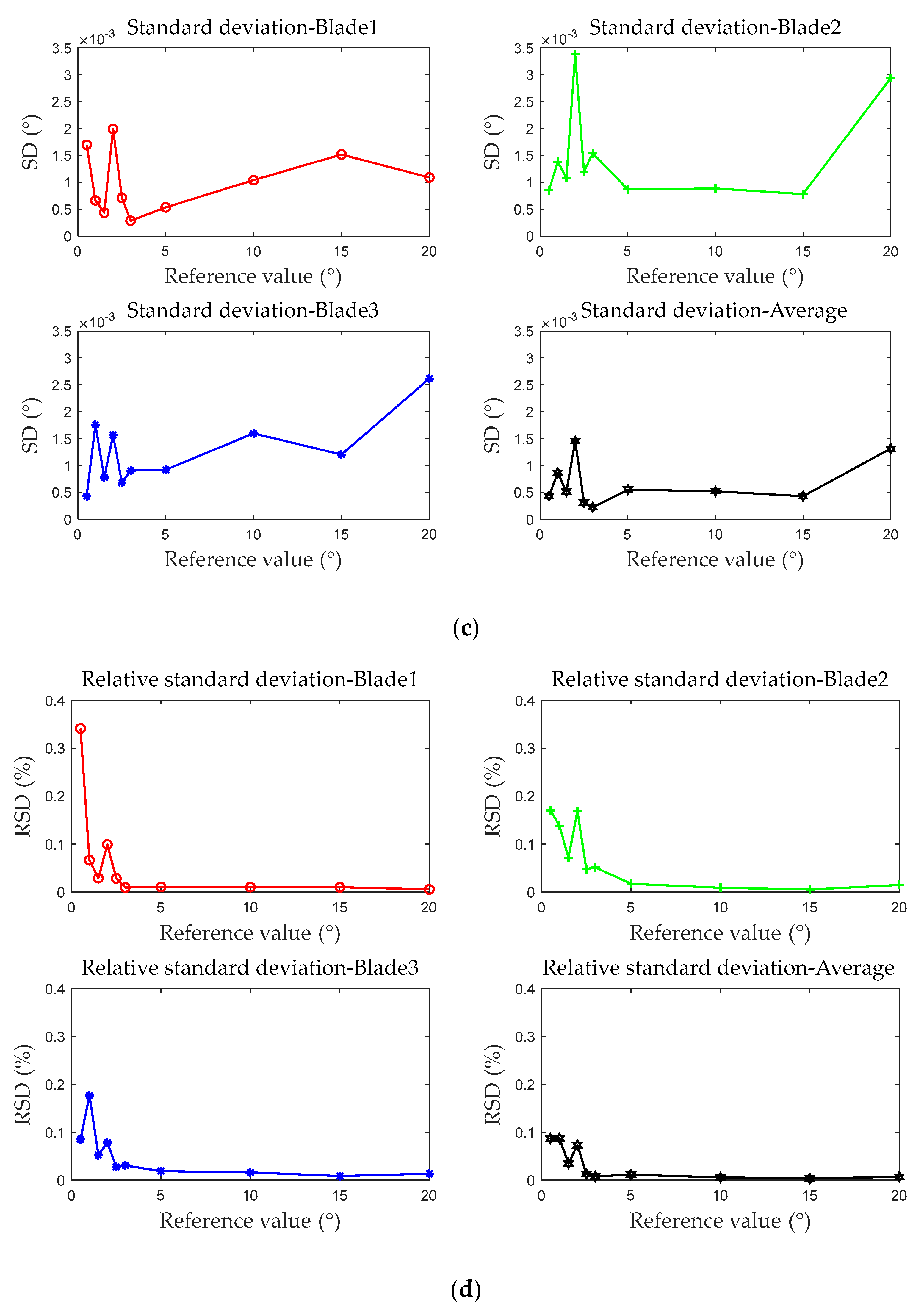
4.3. Accuracy Evaluation of BTT Measurement System
5. Conclusions
- (1)
- A ToA direct calibration method is proposed, which equivalently transforms the ToA variation caused by blade vibration into the circumferential angle difference between the BTT sensor and the rotating blade disk, while accounting for the effects of eccentricity, non-parallelism, and other errors. As ToA serves as the fundamental data for the BTT method, the accuracy of the BTT measurement system is evaluated using the ToA obtained through this direct calibration approach.
- (2)
- A BTT accuracy evaluation device is designed. The device incorporates a multi-tooth indexing table, a high-precision turntable, and the necessary fixtures to realize the required functions. Considering both system and random factors, the device’s uncertainty is evaluated using the Monte Carlo method. Under the conditions of 0.5° and 1000 rpm, the estimated uncertainty is 83.3055 μs, with the standard uncertainty of 8.824 × 10−3 μs, and the 95% confidence interval of [83.2881, 83.3233] μs.
- (3)
- Accuracy evaluation tests of the BTT measurement system were performed using the developed device. The device simulates varying vibration displacement and rotational speed conditions. The optical fiber BTT system’s measurement accuracy is evaluated across four metrics: error, relative accuracy, SD, and RSD. The relative accuracy of the tested optical fiber BTT system is better than 0.8%, and the RSD is below 0.5%. The results demonstrate that the proposed method and device successfully evaluate the accuracy and stability of the BTT measurement system while directly assessing its ToA.
6. Patents
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| BTT | Blade tip timing |
| ToA | Time of arrival |
| OPR | Once per revolution |
| i (i = 1, 2, …, I) | Number of BTT sensors |
| j (j = 1, 2, …, J) | Number of blades on the blade disk |
| yij | Vibration displacement for different BTT sensors and blades |
| tij | Time for vibrating blade j to reach BTT sensor i |
| t’ij | Time for blade j to reach BTT sensor i when not vibrating |
| Δtij | Difference between the time when blade j reaches BTT sensor i while vibrating and the theoretical ToA |
| S1 | The tested BTT sensor |
| r | Rotor radius |
| ω | Rotor angular velocity |
| α | Theoretical angular displacement of S1 |
| Δtj | Theoretical ToA difference before and after S1 rotation |
| an, an-1, an-2, …, a1, a0 | Calibration coefficient |
| S0 | OPR sensor |
| S2 | Location sensor |
| f(ΔS1) | ToA difference between before and after S1 rotation |
| f(ΔS12) | ToA difference between S1 and S2 before S1 rotation |
| f(ΔS12’) | ToA difference between S1 and S2 after S1 rotation |
| T | Initial test duration |
| nr | Initial rotational speed |
| K | Initial number of effective rotations |
| q(t) | Continuous blade-tip sensing signal generated from blade passing S1 |
| tk | Sampling time interval |
| q(ntk) | Discrete signal from sampling and quantizing q(t) at time intervals tk |
| ToA(ntk) | BTT signal obtained through ToA extraction algorithm |
| ToA1(ntk) | Initial BTT signal of S1 |
| ToA2(ntk) | Initial BTT signal of S2 |
| ToA1(j,k) | Initial BTT signal of S1 expressed in terms of each blade and per rotation |
| ToA2(j,k) | Initial BTT signal of S2 expressed in terms of each blade and per rotation |
| q0(ntk) | Initial OPR signal of S0 |
| T0(k) | Initial OPR signal of S0 expressed in terms of per rotation |
| ΔToA12(j,k) | BTT signal difference between S1 and S2 |
| ΔToA12(j) | Average of rotation part of △ToA12(j,k) |
| T’ | Test duration after angular change |
| nr’ | Rotational speed after angular change |
| K’ | Effective number of rotations after angular change |
| ΔToA12’(j,k) | BTT signal difference between S1 and S2 afte angular change |
| ΔToA12’(j) | Average of rotation part of △ToA12’(j,k) after angular change |
| T0’(k) | OPR signal of S0 expressed in terms of per rotation after angular change |
| ΔToAj | Measured ToA difference before and after S1 rotation |
| Δ | BTT measurement error |
| O | Theoretical center of rotation |
| R | Center of angular change |
| S | Initial position of S1 |
| S’ | Position of S1 after angular change |
| lRS | Distance between S and R |
| φ1 | Eccentricity angle formed by eccentricity error between rotation and angular change |
| e1 | Eccentricity magnitude formed by eccentricity error between rotation and angular change |
| δ | Non-parallel angle formed by non-parallelism error between rotation and angular change |
| lOS | Distance between S and O |
| lSS’ | Distance between S and S’ |
| lOS’ | Distance between S’ and O |
| β | Actual rotation angle of S1 |
| β-α | Angular change-induced eccentricity and non-parallelism error |
| O’ | Actual center of rotation |
| φ2 | Rotation eccentricity angle |
| φ02 | Initial rotation eccentricity angle |
| e2 | Rotation eccentricity magnitude |
| Δφ | Real-time rotation eccentricity angle |
| γS | Measured angle of S1 at position S |
| γ0S | Initial measured angle of S1 at position S |
| γS’ | Measured angle of S1 at position S’ |
| γ0S’ | Initial measured angle of S1 at position S’ |
| γ | Measured angle of S1 |
| ΔO’SS’ | Virtual triangle formed by angular change and rotation |
| lO’S | Distance between S and O’ |
| lO’S’ | Distance between S’ and O’ |
| γ-β | Rotation eccentricity error considering angular change-induced eccentricity and non-parallelism |
| γ-α | Eccentricity and non-parallelism errors from rotation and angular change |
| α1 | Initial angle of the multi-tooth indexing table |
| α2 | Angle of the multi-tooth indexing table after angular change |
| θE | Uncertainty component due to indication error of the multi-tooth indexing table |
| θie | Uncertainty component due to eccentricity from inhomogeneity of the coaxial positioning device and BTT sensor fixture |
| θinp | Uncertainty due to non-parallelism from inhomogeneity of the coaxial positioning device and BTT sensor fixture |
| θre | Uncertainty component due to rotor rotational eccentricity |
| θmr | Uncertainty component from repeatability of angle measurement of the multi-tooth indexing table |
| ωrsf | Uncertainty component from rotor rotational speed fluctuations |
| ωmr | Uncertainty component from rotational speed measurement repeatability during rotor rotation |
| εm | Uncertainty component from environmental fluctuations (e.g., temperature, random noise) during testing |
| ΔToA12 | ToA difference measurement value between S1 and S2 |
| ωm | Rotational speed measurement value |
| αm12 | Angle difference measurement value between S1 and S2 |
| αm | Angular change measurement value |
| ValΔToA | Measured value of the BTT measurement system |
| ValΔt | Reference value of the BTT accuracy evaluation device |
| R2 | Coefficient of determination |
| SD | Standard deviation |
| RSD | Relative standard deviation |
References
- Mohamed, E.M.; Bonello, P.; Russhard, P.; Procházka, P.; Mekhalfia, M.L.; Tchuisseu, E.B.T. Experimental Validation of FEM-Computed Stress-to-Tip Deflection Ratios of Aero-Engine Compressor Blade Vibration Modes and Quantification of Associated Uncertainties. Mech. Syst. Signal Process. 2022, 178, 109257. [Google Scholar] [CrossRef]
- Zhao, F.; Jing, X.; Yang, M.; Wang, D.; Sha, Y.; Luan, X. Experimental Study of Rotor Blades Vibration and Noise in Multistage High-Pressure Compressor and Their Relevance. Chin. J. Aeronaut. 2020, 33, 870–878. [Google Scholar] [CrossRef]
- Song, C.; Cheng, W.; Chen, X.; Wang, J.; Qiao, B.; Ge, X.; Gao, L.; Huang, H. Identification and Separation of Coupled Vibration Sources in Multi-Rotor Gas Turbines under Time-Varying Speed Conditions. Mech. Syst. Signal Process. 2023, 188, 110037. [Google Scholar] [CrossRef]
- Liu, S.; Wang, H.; Tang, J.; Zhang, X. Research on Fault Diagnosis of Gas Turbine Rotor Based on Adversarial Discriminative Domain Adaptation Transfer Learning. Measurement 2022, 196, 111174. [Google Scholar] [CrossRef]
- Liska, J.; Vasicek, V.; Jakl, J. A Novel Method of Impeller Blade Monitoring Using Shaft Vibration Signal Processing. Sensors 2022, 22, 4932. [Google Scholar] [CrossRef]
- Sablosky, I. Non-Contact Measurement of Turbine Blade Vibration; National Defense Industry Press: Beijing, China, 1986; pp. 33–60. [Google Scholar]
- Russhard, P. Development of a Blade Tip Timing-Based Engine Health Monitoring System. Ph.D. Thesis, The University of Manchester, Manchester, UK, 2010. [Google Scholar]
- Chen, Z.; Sheng, H.; Xia, Y.; Wang, W.; He, J. A comprehensive review on blade tip timing-based health monitoring: Status and future. Mech. Syst. Signal Process. 2021, 149, 107330. [Google Scholar] [CrossRef]
- Fan, C.; Wu, Y.; Russhard, P.; Wang, A. An improved blade tip-timing method for vibration measurement of rotating blades during transient operating conditions. J. Vib. Eng. Technol. 2020, 8, 859–868. [Google Scholar] [CrossRef]
- Bornassi, S.; Berruti, T.; Firrone, C.; Brighenti, A. Vibration Parameters Identification of Turbomachinery Rotor Blades under Transient Condition Using Blade Tip-Timing Measurements. Measurement 2021, 173, 109861. [Google Scholar] [CrossRef]
- Gil-García, J.; Solís, A.; Aranguren, G.; Zubia, J. An architecture for on-line measurement of the tip clearance and time of arrival of a bladed disk of an aircraft engine. Sensors 2017, 17, 2162. [Google Scholar] [CrossRef]
- Wu, S.; Tian, S.; Chen, X.; Yang, Z. Digital Twin-Driven Blade Rub-Impact Diagnosis Using Blade Tip Timing. Measurement 2024, 231, 114539. [Google Scholar] [CrossRef]
- Zhao, X.; Zhou, Q.; Yang, S. Rotating Stall Induced Non-Synchronous Blade Vibration Analysis for an Unshrouded Industrial Centrifugal Compressor. Sensors 2019, 19, 4995. [Google Scholar] [CrossRef] [PubMed]
- Du Toit, R.; Diamond, D.; Heyns, P. A Stochastic Hybrid Blade Tip Timing Approach for the Identification and Classification of Turbomachine Blade Damage. Mech. Syst. Signal Process. 2018, 121, 389–411. [Google Scholar]
- Fan, Z.; Li, H.; Huang, J.; Liu, S. Blade Vibration Difference-Based Circumferential Fourier Fitting Algorithm for Synchronous Vibration Parameter Identification of Rotation Blades. Sensors 2024, 24, 8083. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Q.; Liu, D.; Duan, J.; Qin, J.; Zhou, Y. Research on the Identification of Asynchronous Vibration Parameters of Rotating Blades Based on Blade Tip Timing Vibration Measurement Theory. Measurement 2023, 206, 112280. [Google Scholar] [CrossRef]
- Rigosi, G.; Battiato, G.; Berruti, T. Synchronous vibration parameters identification by tip timing measurements. Mech. Res. Commun. 2017, 79, 7–14. [Google Scholar]
- Bouchain, A.; Picheral, J.; Lahalle, E.; Chardon, G.; Vercoutte, A.; Talon, A. Blade Vibration Study by Spectral Analysis of Tip-Timing Signals with OMP Algorithm. Mech. Syst. Signal Process. 2019, 130, 108–121. [Google Scholar] [CrossRef]
- Zielinski, M.; Ziller, G. Noncontact Vibration Measurements on Compressor Rotor Blades. Sensors 2000, 11, 847–856. [Google Scholar]
- Ouyang, T.; Duan, F.; Zhang, Y.; Li, M. Magnetic-Electric Pulse Sensor Principle and Blade Tip Timing Error Analysis. Sensors 2008, 8, 4. [Google Scholar]
- García, I.; Beloki, J.; Zubia, J.; Aldabaldetreku, G.; Illarramendi, M.; Jiménez, F. An Optical Fiber Bundle Sensor for Tip Clearance and Tip Timing Measurements in a Turbine Rig. Sensors 2013, 13, 7385–7398. [Google Scholar] [CrossRef]
- Guo, H.; Duan, F.; Cheng, Z. Numerical Analysis of the Blade Tip-Timing Signal of a Fiber Bundle Sensor Probe. Opt. Eng. 2015, 54, 034103. [Google Scholar]
- Chen, R.; Jiang, Y.; Pei, Z. High Frequency and High Accuracy Laser Ranging System Based on Double Thresholds Leading-Edge Timing Discrimination. Acta Opt. Sin. 2013, 33, 147–154. [Google Scholar]
- Wang, M.; Duan, F.; Guo, H.; Zhang, J. Signal Processing Method of Increasing Precision of Optical Fiber Sensor Based Tip-Timing System. Nanotechnol. Precis. Eng. 2016, 14, 6. [Google Scholar]
- Zhou, C.; Duan, F.; Liu, Z.; Li, J. Research on High-precision Extraction Method of Blade Tip Arrival Time Based on Centroid Algorithm. J. Sens. Technol. 2022, 35, 952–959. [Google Scholar]
- Knappett, D.; Garcia, J. Blade tip timing and strain gauge correlation on compressor blades. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2008, 222, 497–506. [Google Scholar] [CrossRef]
- Grant, K. Experimental Testing of Tip-Timing Methods Used for Blade Vibration Measurement in the Aero-Engine. Ph.D. Thesis, Cranfield University, Bedford, UK, 2004. [Google Scholar]
- Ao, C.; Qiao, B.; Chen, L.; Xu, J.; Liu, M.; Chen, X. Blade dynamic strain non-Intrusive measurement using L1/2-norm regularization and transmissibility. Measurement 2022, 190, 110677. [Google Scholar] [CrossRef]
- Gallego-Garrido, J.; Dimitriadis, G.; Carrington, I.B.; Wright, J.R. A Class of Methods for the Analysis of Blade Tip Timing Data from Bladed Assemblies Undergoing Simultaneous Resonances—Part II: Experimental Validation. Int. J. Rotating Mach. 2007, 2007, ID-73624. [Google Scholar] [CrossRef]
- Di Maio, D.; Ewins, D. Measurement of out-of-plane vibrations of a rotating bladed disk using blade tip timing measurement method. In Proceedings of the Eighth International Conference on Vibration Measurements by Laser Techniques: Advances and Applications, Ancona, Italy, 18–20 June 2008; Volume 7098, pp. 88–102. [Google Scholar]
- Meng, Y.; Xiao, Z.; Ouyang, H. High-accuracy Experimental Analysis and Correction of Blade Tip Timing Measurement Error. J. Aerosp. Power 2024, 39, 243–254. [Google Scholar]
- Chai, P.; Xiao, Z.; Wang, S.; Tian, J.; Ouyang, H. Improvement of BTT precision considering transient rotating speed fluctuations. Mech. Syst. Signal Process. 2023, 193, 110247. [Google Scholar] [CrossRef]


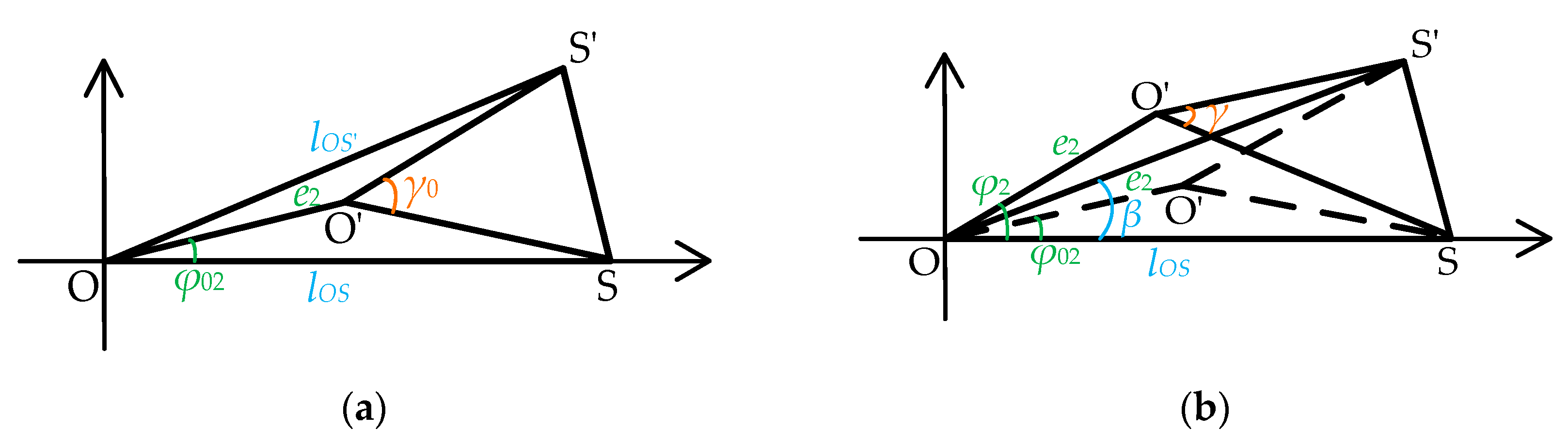




| Input Variables | Distribution Parameters | Probability Distribution | |||
|---|---|---|---|---|---|
| μ | σ | a | b | ||
| α/° | 0.5 | 0 | / | / | N(μ, σ2) |
| lRS/mm | / | / | 43.48 | 43.52 | R(a, b) |
| e1/mm | / | / | −0.01 | 0.01 | R(a, b) |
| φ1/° | / | / | −180 | 180 | R(a, b) |
| δ/° | / | / | −0.1 | 0.1 | R(a, b) |
| e2/mm | / | / | −0.003 | 0.003 | R(a, b) |
| φ2/° | / | / | −180 | 180 | R(a, b) |
| nr/rpm | 1000.3345 | 2.249 × 10−4 | / | / | N(μ, σ2) |
| nrsf/rpm | / | / | −0.01 | 0.01 | R(a, b) |
| Test Type | Condition Number | Multi-Tooth Indexing Table Rotation Angle α/° | Rotational Blade Disk Set Speed nr/rpm | ToA Difference Measurement ΔToA12/μs | Rotational Speed Measurement nrm/rpm | Angle Difference Measurement αm12/° |
|---|---|---|---|---|---|---|
| Simulating vibration displacement changes | 1 | 0 | 1000 | 215.6666 | 1000.3351 | 1.2944 |
| 2 | 0.5 | 1000 | 298.7925 | 1000.3346 | 1.7934 | |
| 3 | 1 | 1000 | 381.2203 | 1000.3342 | 2.2881 | |
| 4 | 1.5 | 1000 | 464.7491 | 1000.3344 | 2.7894 | |
| 5 | 2 | 1000 | 547.4308 | 1000.3346 | 3.2857 | |
| 6 | 2.5 | 1000 | 630.4690 | 1000.3344 | 3.7841 | |
| 7 | 3 | 1000 | 713.3132 | 1000.3345 | 4.2813 | |
| 8 | 5 | 1000 | 1044.3971 | 1000.3343 | 6.2685 | |
| 9 | 10 | 1000 | 1873.4700 | 1000.3344 | 11.2446 | |
| 10 | 15 | 1000 | 2701.3150 | 1000.3344 | 16.2133 | |
| 11 | 20 | 1000 | 3528.9697 | 1000.3344 | 21.1809 | |
| Simulating rotational speed changes | 12 | 0 | 500 | 437.5131 | 500.1668 | 1.3130 |
| 13 | 0.5 | 500 | 603.6859 | 500.1672 | 1.8117 | |
| 14 | 0 | 1500 | 146.5108 | 1500.4989 | 1.3190 | |
| 15 | 0.5 | 1500 | 201.7924 | 1500.5009 | 1.8167 | |
| 16 | 0 | 2000 | 109.5501 | 2000.6677 | 1.3150 | |
| 17 | 0.5 | 2000 | 151.0234 | 2000.6701 | 1.8129 | |
| 18 | 0 | 2500 | 87.6196 | 2500.8351 | 1.3147 | |
| 19 | 0.5 | 2500 | 120.8415 | 2500.8340 | 1.8132 | |
| 20 | 0 | 3000 | 72.6806 | 3001.0036 | 1.3087 | |
| 21 | 0.5 | 3000 | 100.3465 | 3001.0159 | 1.8068 | |
| 22 | 0 | 4000 | 54.9741 | 4001.3608 | 1.3198 | |
| 23 | 0.5 | 4000 | 75.7061 | 4001.3923 | 1.8176 | |
| 24 | 0 | 5000 | 44.2745 | 5002.6730 | 1.3289 | |
| 25 | 0.5 | 5000 | 60.8521 | 5002.9860 | 1.8267 |
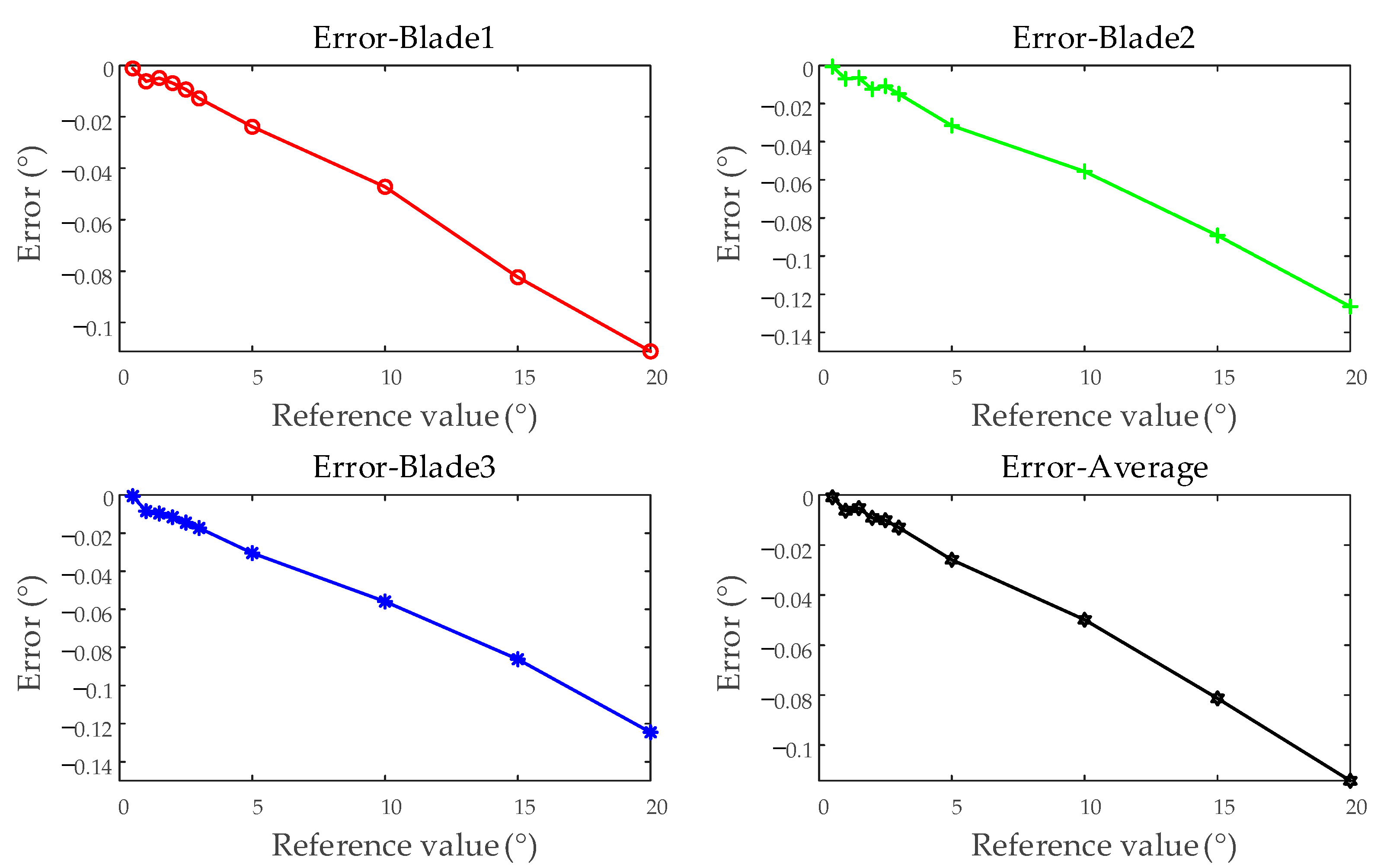
| Multi-Tooth Indexing Table Rotation Angle α/° | Circumferential Angle Measurement αm/° | Error/° | Relative Accuracy/% |
|---|---|---|---|
| 0.5 | 0.4989 | −1.079 × 10−3 | −0.2158 |
| 1 | 0.9937 | −6.347 × 10−3 | −0.6347 |
| 1.5 | 1.4950 | −5.006 × 10−3 | −0.3337 |
| 2 | 1.9913 | −8.750 × 10−3 | −0.4375 |
| 2.5 | 2.4896 | −1.035 × 10−2 | −0.4142 |
| 3 | 2.9869 | −1.312 × 10−2 | −0.4374 |
| 5 | 4.9740 | −2.596 × 10−2 | −0.5191 |
| 10 | 9.9501 | −4.985 × 10−2 | −0.4985 |
| 15 | 14.9189 | −8.112 × 10−2 | −0.5408 |
| 20 | 19.8865 | −1.135 × 10−1 | −0.5677 |
| Rotational Blade Disk Set Speed nr/rpm | Circumferential Angle Measurement αm/° | Error/° | Relative Accuracy/% |
|---|---|---|---|
| 500 | 0.4987 | −1.314 × 10−3 | −0.2628 |
| 1000 | 0.4989 | −1.079 × 10−3 | −0.2158 |
| 1500 | 0.4977 | −2.298 × 10−3 | −0.4596 |
| 2000 | 0.4978 | −2.152 × 10−3 | −0.4305 |
| 2500 | 0.4985 | −1.507 × 10−3 | −0.3014 |
| 3000 | 0.4982 | −1.8385 × 10−3 | −0.3677 |
| 4000 | 0.4978 | −2.2467 × 10−3 | −0.4493 |
| 5000 | 0.4977 | −2.2922 × 10−3 | −0.4584 |
| Blade Number | a1 | a0 | R2 | Blade Number | a1 | a0 | R2 |
|---|---|---|---|---|---|---|---|
| 1 | 1.0057 | −0.0035 | 0.99999985 | 6 | 1.0058 | −0.0016 | 0.99999991 |
| 2 | 1.0063 | −0.0024 | 0.99999982 | 7 | 1.0057 | −0.0049 | 0.99999993 |
| 3 | 1.0061 | −0.0006 | 0.99999984 | 8 | 1.0054 | −0.0045 | 0.99999972 |
| 4 | 1.0052 | −0.0039 | 0.99999986 | Average | 1.0057 | −0.0029 | 0.99999989 |
| 5 | 1.0056 | −0.0017 | 0.99999986 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, Q.; Niu, G.; Liu, M.; Teng, G.; Duan, F.; Li, F.; Liu, H.; Li, F. Accuracy Evaluation Method for Blade Vibration Measurement in Blade Tip Timing Based on Direct Calibration Using Time of Arrival. Sensors 2025, 25, 1956. https://doi.org/10.3390/s25071956
Zhou Q, Niu G, Liu M, Teng G, Duan F, Li F, Liu H, Li F. Accuracy Evaluation Method for Blade Vibration Measurement in Blade Tip Timing Based on Direct Calibration Using Time of Arrival. Sensors. 2025; 25(7):1956. https://doi.org/10.3390/s25071956
Chicago/Turabian StyleZhou, Qi, Guangyue Niu, Meiru Liu, Guangrong Teng, Fajie Duan, Fangyi Li, Hao Liu, and Fafu Li. 2025. "Accuracy Evaluation Method for Blade Vibration Measurement in Blade Tip Timing Based on Direct Calibration Using Time of Arrival" Sensors 25, no. 7: 1956. https://doi.org/10.3390/s25071956
APA StyleZhou, Q., Niu, G., Liu, M., Teng, G., Duan, F., Li, F., Liu, H., & Li, F. (2025). Accuracy Evaluation Method for Blade Vibration Measurement in Blade Tip Timing Based on Direct Calibration Using Time of Arrival. Sensors, 25(7), 1956. https://doi.org/10.3390/s25071956






